Simulation on Deformation Damage and Strain Rate Effect of Nb3Sn Composite Superconductors under Cycling Load at Extreme Low Temperature
-
摘要: Nb3Sn超导体在循环载荷下的变形损伤行为研究对揭示超导体临界性能不可逆退化背后的力学机制具有重要意义。采用分子动力学模拟方法研究了极低温条件下单晶和多晶Nb3Sn/Nb复合材料在循环载荷下的变形损伤行为,同时分析了应变率对Nb3Sn/Nb复合材料变形损伤和断裂行为的影响。结果表明:单晶Nb3Sn/Nb复合材料在循环载荷作用后,Nb3Sn层出现滑移,当滑移带交错处的局部应力大于材料强度时,在滑移带交错处微裂纹萌生,致使复合材料中Nb3Sn层断裂失效;而多晶Nb3Sn/Nb复合材料则由于晶界处应力在循环载荷下得不到松弛,当应力峰值超过晶界强度时,在晶界处萌生微裂纹,导致复合材料中Nb3Sn层发生沿晶断裂。Nb3Sn/Nb复合材料在不同应变率下表现出不同的断裂方式。随着应变率的增加,单晶Nb3Sn层中的滑移带数量增加,导致单晶Nb3Sn/Nb复合材料的韧性增强。而多晶Nb3Sn/Nb复合材料中,晶界对材料强度的影响随着应变率的增加而降低,高应变率下,复合材料在Nb3Sn层局部断裂后具有较大的剩余强度。研究结果将有助于理解Nb3Sn/Nb复合材料在循环载荷下的损伤演化过程,为材料的性能优化设计提供一定的理论指导。
-
关键词:
- Nb3Sn/Nb复合材料 /
- 分子动力学模拟 /
- 循环载荷 /
- 变形损伤 /
- 应变率效应
Abstract: The study on damage and fracture of superconducting Nb3Sn under cyclic loading is an indispensable part of understanding the origin of the irreversible strain limit in Nb3Sn. This paper uses molecular dynamics simulation to investigate the fracture and deformation damage behavior of polycrystalline and single crystal Nb3Sn/Nb composite materials under cyclic loading at extremely low temperatures. The effects of strain rate on crack initiation and growth were carefully analyzed in both polycrystalline and single crystal Nb3Sn/Nb composite materials. The results indicate that slip occurs in single crystal Nb3Sn/Nb composite materials after cyclic loading. When the local stress at the slip band intersection exceeds the material strength, microcracks initiate at the slip band intersection, leading to fracture failure of the composite material. In contrast, the failure of polycrystalline Nb3Sn/Nb composite materials is due to the inability of stress at grain boundaries to relax under cyclic loading, which leads to the initiation of microcracks at the grain boundaries and intergranular fracture of the composite material. The analysis of the different damage, fracture, and failure mechanisms of polycrystalline and single crystal Nb3Sn/Nb composite materials at different strain rates shows that the fracture is brittle at low strain rates. As the strain rate rises, the number of slip bands in the single crystal Nb3Sn layer increases, enhancing the toughness of the single crystal Nb3Sn/Nb composite material. Conversely, the influence of grain boundaries on material strength decreases in polycrystalline materials as the strain rate increases. Moreover, polycrystalline Nb3Sn/Nb composite materials exhibit significant residual strength after local fracture of Nb3Sn at high strain rates. The research results will contribute to a better understanding of the damage evolution process of Nb3Sn/Nb composite materials under cyclic loading and offer theoretical guidance for optimizing material performance. -
Nb3Sn材料因其出色的超导性能被广泛应用于大型磁体领域[1–4],并成为未来大型强子对撞机和超导谐振腔体的备选材料[5]。通常,Nb3Sn以管内电缆导体(cable-in-conduit conductors,CICC)的形式应用于低温、强磁场、高电流环境中,承受电磁与热的周期性载荷。研究表明,这种冷却过程与运行过程中造成的应力循环会导致Nb3Sn的临界性能退化。因此,循环载荷对Nb3Sn材料力学性能的影响是超导磁体安全性、稳定性设计中必须考虑的关键因素。
在过去的几十年中,学者们针对Nb3Sn超导线在循环载荷作用下的超导性能、变形损伤以及应变率效应进行了广泛的实验研究。早在1979年,Eisenstatt等[6]、Clark等[7]通过压缩和疲劳实验研究了高温下多晶Nb3Sn的塑性变形,给出了多晶Nb3Sn的屈服应力与温度及应变率的关系:当实验变量只有温度和应变率时,多晶Nb3Sn的屈服应力与温度负相关,与应变率正相关。他们还通过金相法发现多晶Nb3Sn在压缩时的主要失效模式为韧性断裂过程,包括孔洞产生、扩大、连接和宏观断裂。1991年,Ochiai等[8]对Nb3Sn进行了室温下的循环加载实验,证明了循环载荷会导致4.2 K下Nb3Sn的临界性能下降,但受实验器材的限制,无法对循环下临界电流的退化程度进行精准检测。自2004年起,Wessel等[9]、Nijhuis等[10–12]启用了新的应变响应测试装置(test arrangement for strain influence on strands,TARSIS)来测量Nb3Sn的应变对临界性能的影响,通过不同的探头组合,先后对几种Nb3Sn超导链进行了轴向和横向周期性载荷应力-应变测量,并依据加载和卸载时曲线的线性部分确定不同制备方式得到的Nb3Sn超导线材在循环过程中弹性模量的变化,通过卸载至零应力状态并逐步递增循环应力的方法,观察到Nb3Sn超导线材在循环载荷下的应变对临界电流和硬化指数n产生了影响,并确定了不同类型超导线材的不可逆应变极限。实验结果表明,Nb3Sn的临界电流Ic极度依赖于应变,在相对宽的应变范围内,施加循环载荷时,临界电流Ic会发生由可逆变化向不可逆变化的转变。根据Nijhuis等[10–12]的报道,对于由内锡法加工的Nb3Sn超导线材,在横向载荷作用下,当弯曲应变小于0.3%时,其临界电流是可逆的。同时,临界性能的不可逆退化伴随着循环载荷作用下超导体的损伤累积和演化过程。根据文献[12]的报道,青铜法制备的Nb3Sn超导丝的不可逆应变极限为0.55%。在金相显微镜下观察到:当本征应变为0.43%时,Nb3Sn超导丝中没有观测到裂纹,然而,当Nb3Sn超导丝达到不可逆应变极限,即Nb3Sn的本征应变为0.55%时,超导丝内部裂纹快速扩展。Mitchell[13]同样指出,在实验循环载荷下,不可逆变形会造成Nb3Sn临界性能下降,并指出拉伸过程中Nb3Sn内裂纹扩展与此相关。Sheth等[14]通过扫描电子显微镜(scanning electron microscope,SEM)观测到Nb3Sn/Cu复合超导丝在疲劳载荷下的裂纹演化和分布情况,通过对比不同应变下Nb3Sn超导丝的裂纹密度,在Nijhuis等[10–12]的研究基础上,提出Nb3Sn在高应变下比低应变下对循环载荷更敏感,在高应变下,Nb3Sn内裂纹密度随循环次数的增加而显著增加。为了避免机械抛光对样品产生二次影响,Shen等[15]采用X射线断层扫描观察了拉伸载荷下多丝Nb3Sn的断裂行为,发现在Nb3Sn超导丝中绝大多数裂纹为横向裂纹和孔洞缺陷。Jiang等[16]开展了Nb3Sn/Cu超导线材在不同应变下的疲劳实验,提出了一种损伤演化模型,该模型能够准确描述Nb3Sn超导线材由于刚度退化和塑性应变累积而导致的力学性能退化,并预测不同应变下超导线材的疲劳寿命。大量实验结果表明,循环载荷下Nb3Sn超导线材内部芯线的脆性断裂是导致其临界性能退化的主要原因。同时,Nb3Sn超导线材内的损伤扩展大致分为3个阶段:首先,Nb3Sn材料中芯丝产生损伤和断裂;然后,损伤趋于稳定;最后,基体中产生大量裂纹,使超导体内部裂纹快速增长。
由于Nb3Sn超导体是由嵌入韧性基体中的脆性超导丝组成的,在循环载荷作用下表现出强非线性变形特征[17–18],因此,学者们对其循环载荷下的非线性力学行为进行了理论和数值模拟研究。Mitchell[19]通过建立一维弹塑性模型,计算了超导复合丝在室温与4 K环境温度下循环加载时的力学行为,但没有解释卸载过程中滞后回线产生的原因。Wang等[20]以内锡法制备的超导复合丝为例,建立了三维有限元仿真模型,分析了循环过程中Nb3Sn中微裂纹的演化和断裂对超导复合丝超导性能的影响,并认为初始热应力和裂纹扩展是卸载过程中滞后回线产生的主要原因。Jiang等[21]研究了不对称循环应力作用下Nb3Sn/Cu超导线材的损伤演化过程,分析了卸载应力与材料能量损耗之间的非线性关系,认为材料的损伤退化是造成能量耗散非线性的主要原因。基于有限元方法的分析模型从宏观角度分析了循环载荷作用下Nb3Sn复合超导体中的机械损伤,进一步的研究需要从原子尺度揭示极端低温环境中循环载荷作用下Nb3Sn复合材料结构中变形损伤演化过程,以补充有限元方法对原子尺度变形信息获取的不足,对于优化设计Nb3Sn复合超导材料结构具有重要的理论和工程意义。
本研究通过建立单晶和多晶Nb3Sn/Nb复合材料模型,采用分子动力学(molecular dynamics,MD)方法探究Nb3Sn/Nb复合材料在循环载荷作用下的变形损伤和断裂机制,同时考虑应变率效应对Nb3Sn/Nb复合材料变形损伤和断裂机制的影响,以期深入理解Nb3Sn/Nb复合材料在循环载荷作用下的损伤演化机制和应变率效应,提升材料在实际工程应用中的可靠性。
1. 分子动力学模型与方法
1.1 模型建立与模拟过程
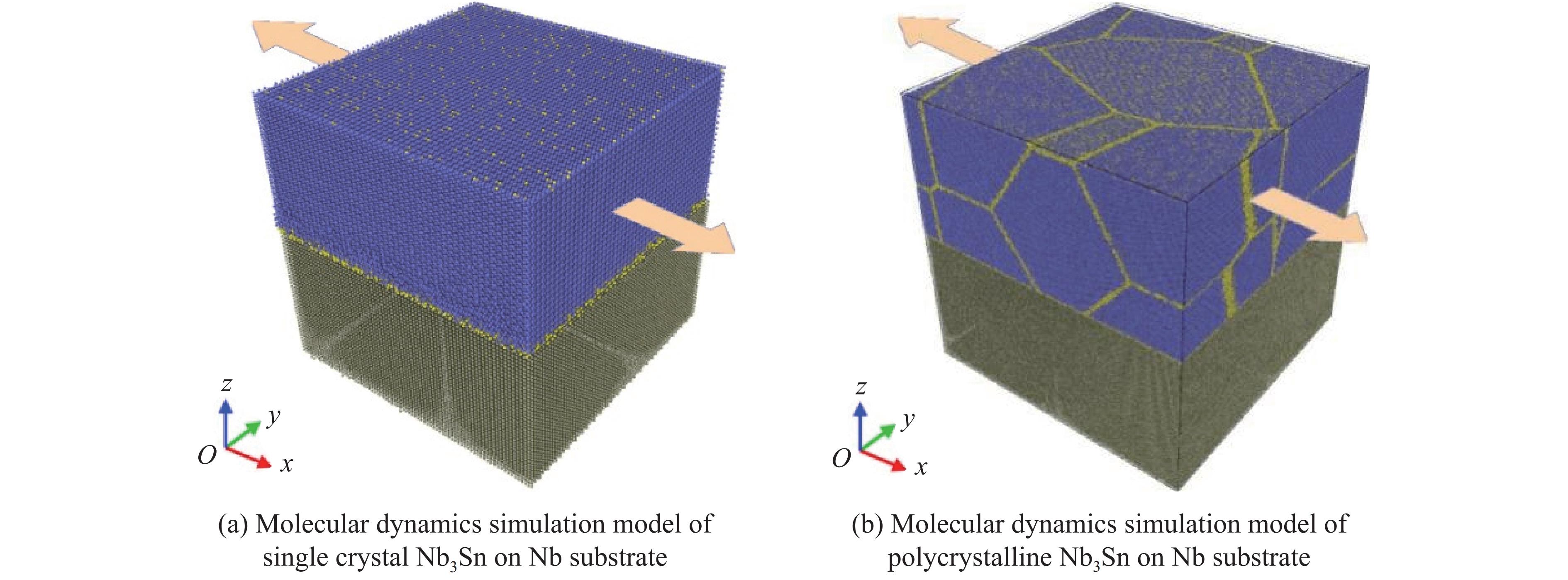
根据工程实践中Nb3Sn/Nb的材料结构特征,建立三维分子动力学模型,如图1所示,在该模型中,Nb3Sn和Nb的晶格常数分别为3.324和5.332 Å[22]。其中,单晶模型的尺寸为25.0 nm×25.0 nm×25.0 nm,Nb3Sn层与Nb基体层厚度均为12.5 nm,x、y、z轴分别对应Nb3Sn晶体的[100]、[010]、[001]晶向,模型共包含911 596个原子;多晶模型的尺寸为81.7 nm×81.7 nm×78.0 nm,Nb3Sn层与Nb基体层厚度均为39.0 nm,模型中共包含29 187 566个原子。为了减小尺寸效应的影响,在x、y、z 3个方向上均采用周期性边界条件。
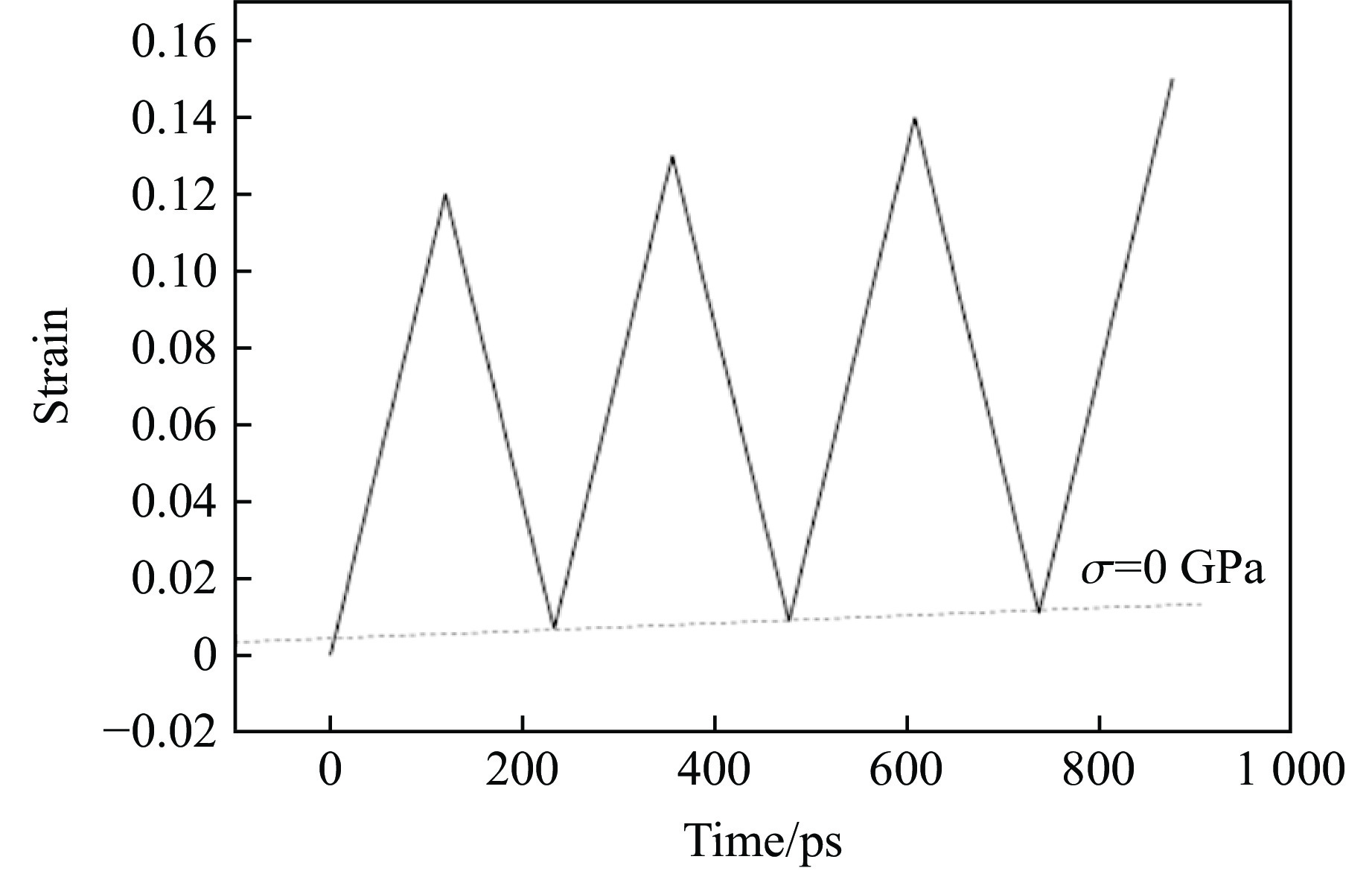
完成Nb3Sn/Nb复合材料的建模后,采用分子动力学模拟方法对循环载荷下的力学变形行为进行研究。采用共轭梯度算法对原子系统进行松弛,以达到系统能量最小化,在NPT系综下使用Nose-Hoover恒温器将模型温度控制在4.2 K极低温下充分弛豫,以释放材料内部应力,使模型达到平衡状态,此时模拟单元各方向上应力(σ)为零。平衡后,在NVT系综中选用恒定应变率实现对Nb3Sn/Nb复合超导体沿x方向循环加载的模拟,具体循环过程如图2所示。在循环过程中选用相同应变率进行加载与卸载,卸载过程中,当模拟单元应力为零时,停止卸载,继续加载,每次循环中应变增幅为1%。循环过程中使用Nose-Hoover控温器保持Nb3Sn/Nb复合材料在循环过程中处于4.2 K的极低温环境,并设定模拟步长为1 fs。选用1×109 s−1作为循环载荷模拟时的应变率来分析循环过程中晶体的变形损伤行为;同时,分别选用1×109、1×1010和1×1011 s−1 3组应变率来研究Nb3Sn/Nb复合材料在循环过程中的应变率效应。
在分子动力学模拟过程中,原子间的相互作用势是进行原子水平计算的基础。为了准确描述原子间的相互作用,分别采用Zhang等[23]开发的嵌入原子势(embedded atom method,EAM)、修正的嵌入原子势(modified embedded atom method,MEAN)[24]和L-J势[25]对Nb、Sn和Nb-Sn原子间的相互作用进行描述。模拟完成后,借助后处理软件OVITO对循环过程中晶体内部变形和损伤演化进行分析。
1.2 分子动力学中应力的表达
在分子动力学研究中,应力计算对研究循环加载条件下材料的力学行为十分重要,而原子尺度的应力计算对分析材料内应力的分布、了解循环过程中裂纹生成与扩展的机理起到至关重要的作用。与宏观应力的定义有所区别,在微观应力计算中,通过定义原子间相互作用的强度来定义应力大小。在上述循环过程中,模拟单元内应力σ的表达式为
σ=NkBTV+1VdN′∑i=1ri⋅fi (1) 式中:
N 为模型中的原子总数;kB 为玻尔兹曼常数;T 为系统温度;d 为模型维度;V 为模型体积;等式右边第2项为维里表达式,用于计算原子之间的相互作用,其中ri 和fi 分别为原子i的位置矢量和力矢量。由于在周期性边界条件下进行模拟,必须考虑相邻域即空原子的位置和力矢量,因此,将空原子包含在原子总数中,共有N′ 个原子。当采用非周期性边界条件时,模型中原子总数N′=N 。原子i的应力张量表达式为
σαβi=−mivαivβi−Wαβi (2) 式中:α和β的取值为x、y、z,以生成张量的各个分量。式(2)右边第1项为原子i的动能,第2项为维里表达式,用于计算原子间的相互作用势。其中原子i的维里表达式[26]为
Wαβ=12Np∑n=1(rα1Fβ1+rα2Fβ2)+12Nb∑n=1(rα1Fβ1+rα2Fβ2)+⋯ (3) 式中:等式右边第1项为原子i在邻域内原子间的成对能贡献,
Np 为邻域内原子总数,r1和r2为成对相互作用中的两个原子的位置,F1 和F2 为成对作用产生在两个原子上的力;第2项为原子i参与的键能贡献,Nb 为原子i参与的金属键的总数;省略号表示其他可能出现的角度贡献、不当贡献和K-空间贡献[27]等。2. 结果与讨论
2.1 模拟结果验证
表1给出了分子动力学模拟得到的Nb3Sn单晶的弹性常数和晶格常数,与实验结果和第一性原理模拟结果[28–31]基本吻合。对于立方相Nb3Sn晶体,弹性稳定性准则为:
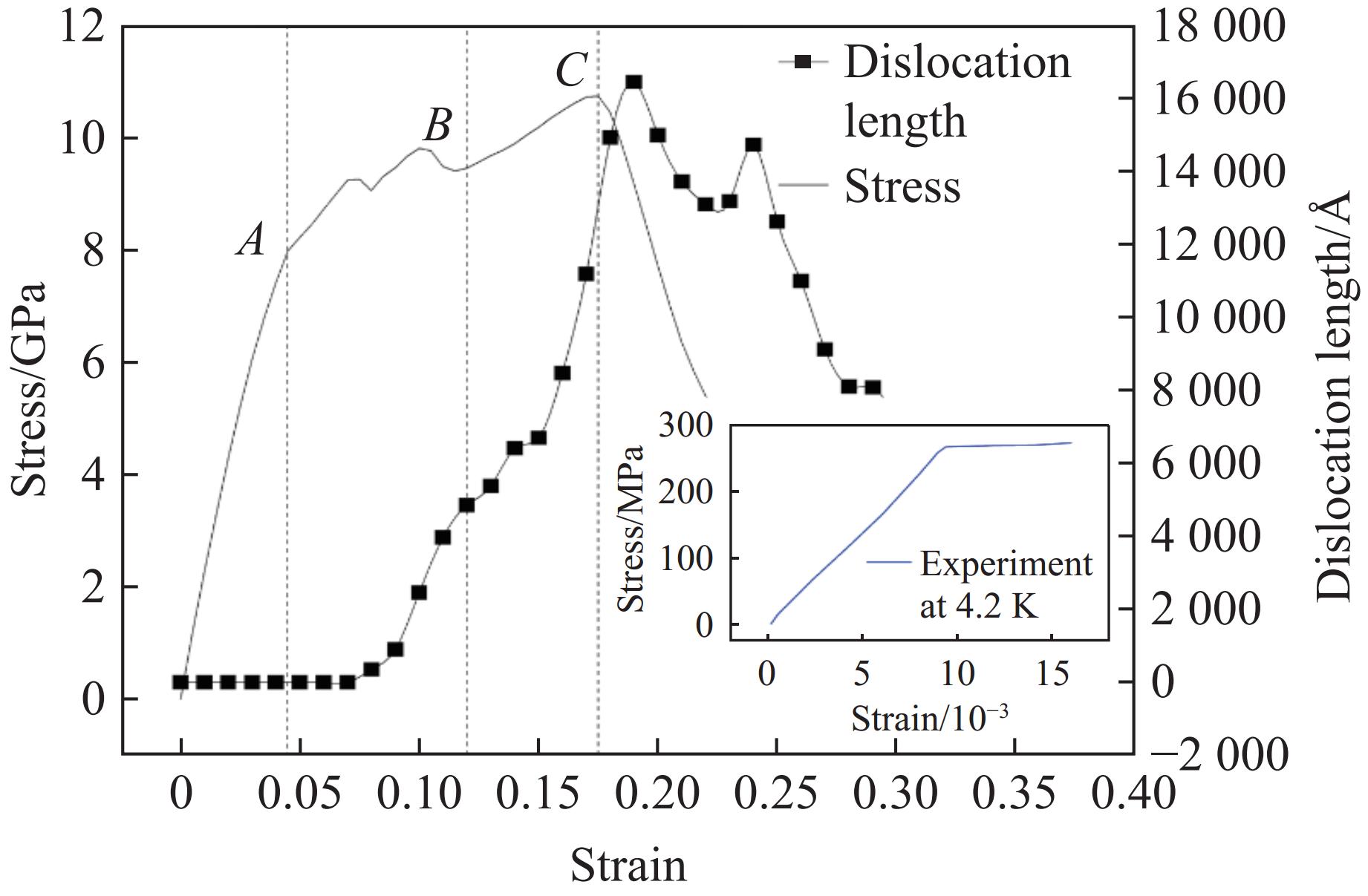
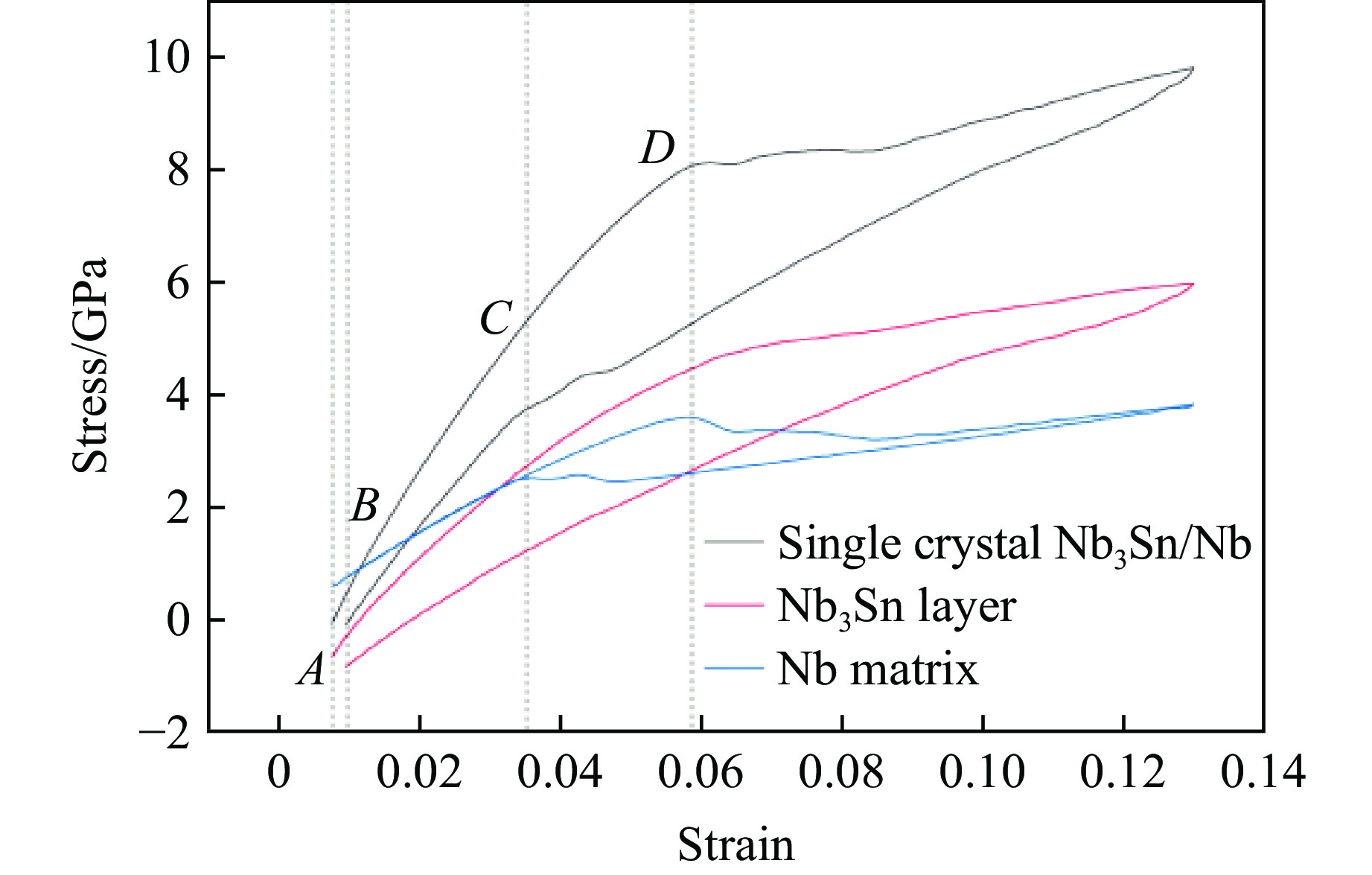
C11+2C12>0 ,C11–C12 > 0 和C44 > 0 [32](Cij 为弹性常数分量)。本研究得到的Nb3Sn单晶的弹性常数符合普遍接受的弹性稳定性准则。表 1 Nb3Sn单晶的弹性常数和晶格常数Table 1. Elastic constant and lattice constant of Nb3Sn single crystalMethod C11/GPa C12/GPa C44/GPa Lattice constants/Å MD simulations (300 K) 284.11 95.84 53.76 5.21 Experimental results (300 K) 253.85 112.44 39.62 5.29 MD simulations (4.2 K) 333.18 127.09 53.71 5.17 Ab initio simulations (0 K) 284.83 107.73 67.07 5.32 在模拟循环载荷作用前,首先对Nb3Sn/Nb复合结构进行了拉伸模拟,以确定合适的初始卸载应变峰值。图3给出了4.2 K的低温环境下,单晶Nb3Sn/Nb复合材料结构沿[100]方向拉伸模拟得到的应力-应变曲线(恒定应变率为1×109 s−1),同时给出了Nb基体中位错长度随施加应变的变化曲线。从图3可以看出:在A点之前,复合材料处于弹性变形阶段,Nb3Sn层与Nb基体中均没有产生位错;在AC段,复合材料发生塑性变形,基体内的位错长度随着施加应变的增加而增大;在C点之后,复合材料内的应力快速下降,此时Nb3Sn层中产生孔洞并开始扩展。模拟结果与实验观测结果[33]定性吻合。
为了更好地观察循环载荷作用过程中的微观结构演变和单晶Nb3Sn/Nb复合材料的力学性能变化,选择图3中AC段曲线中的B点作为初始应变峰值,并开始模拟卸载与循环加载过程。在该初始应变峰值下,复合材料中已经产生了塑性变形,Nb3Sn层中存在滑移,Nb基体中存在大量位错。
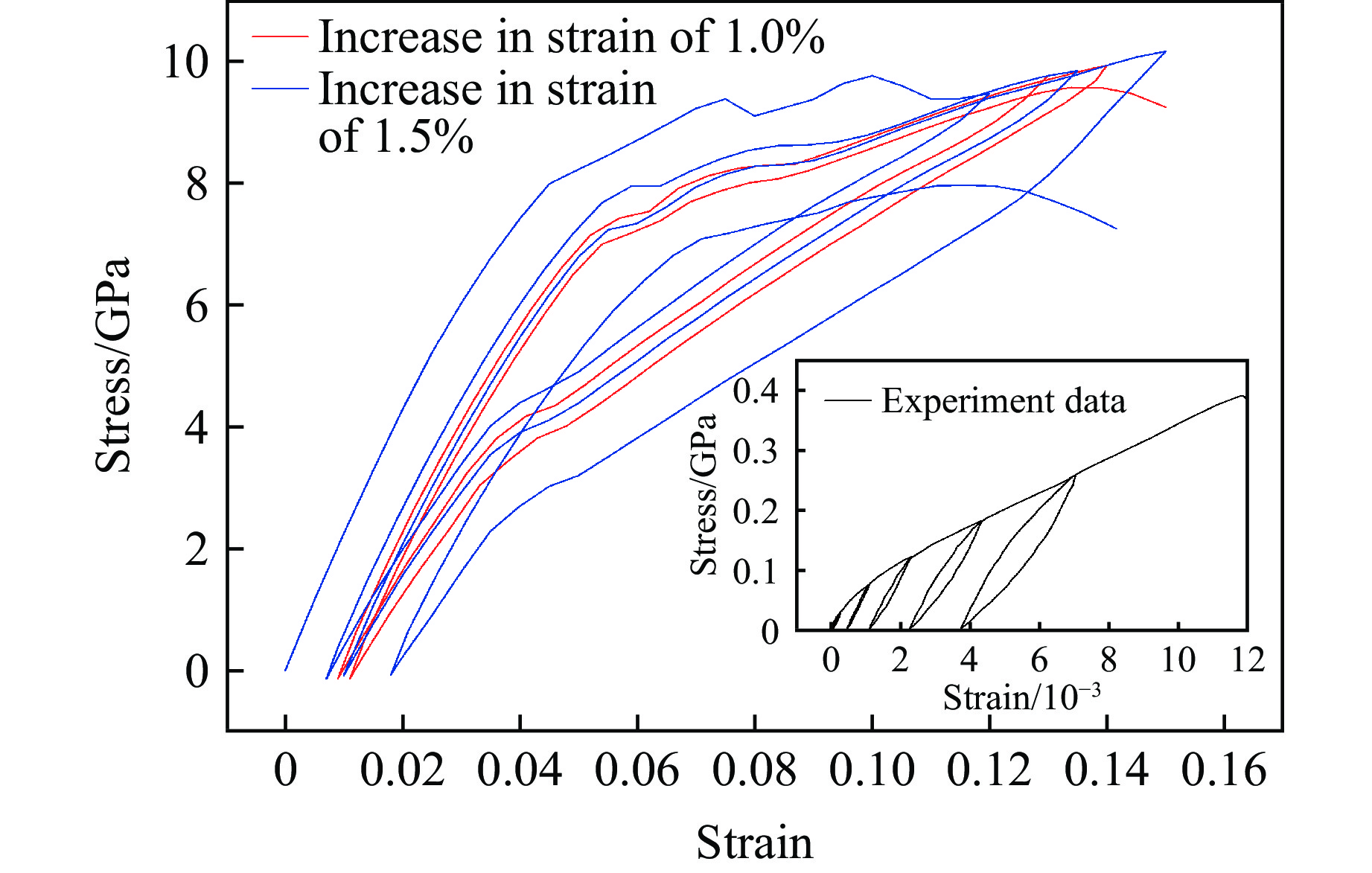
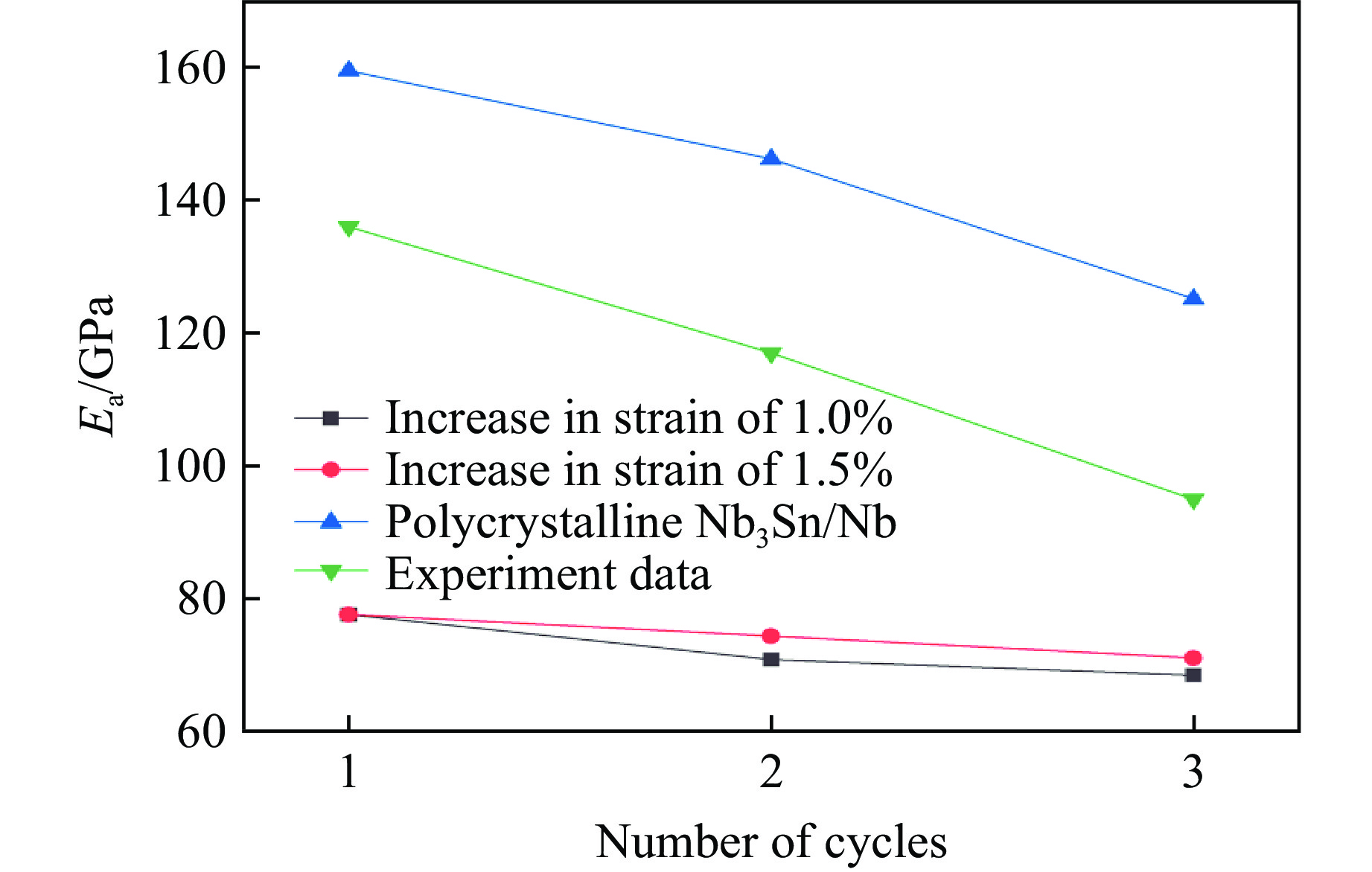
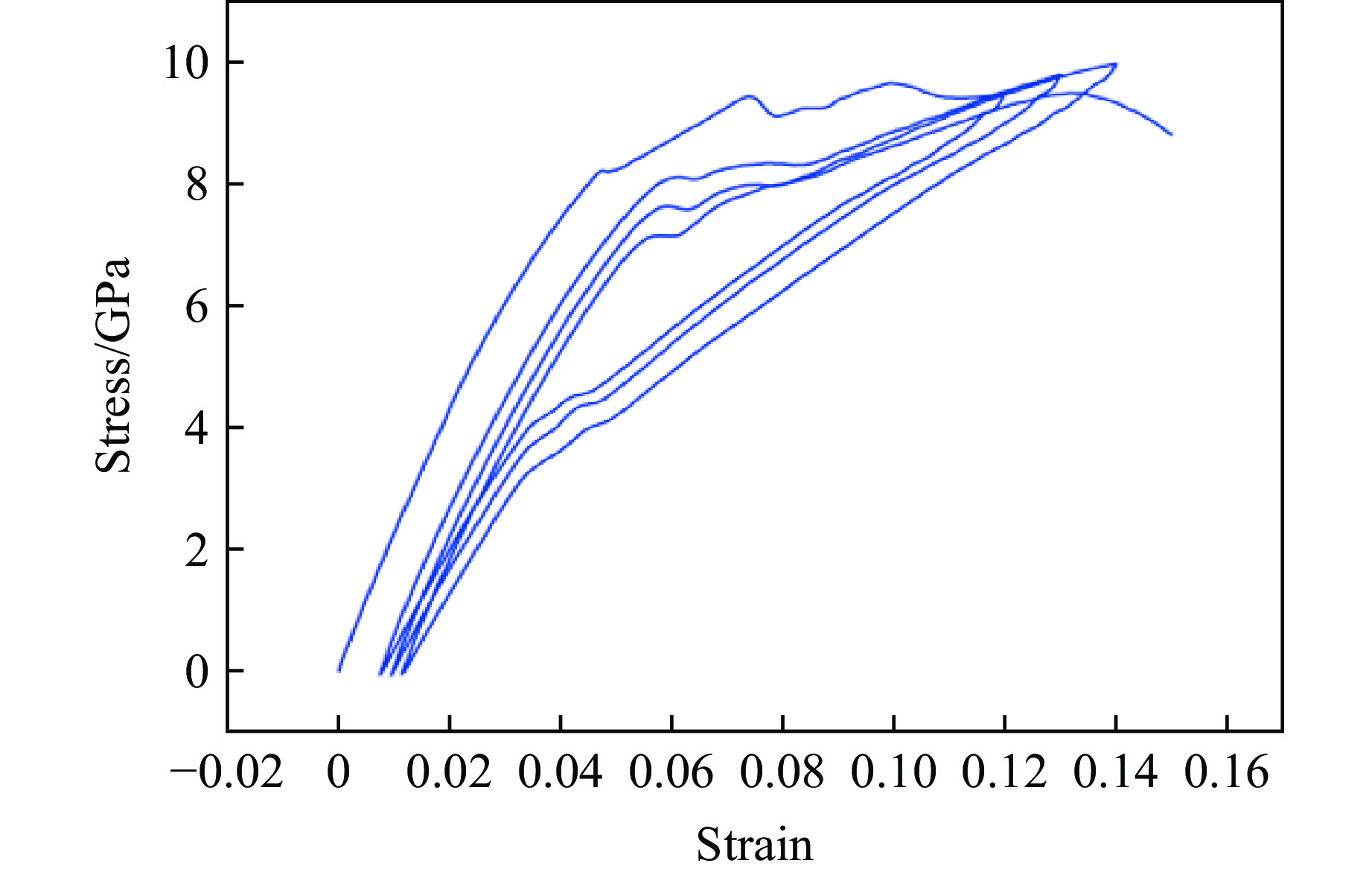
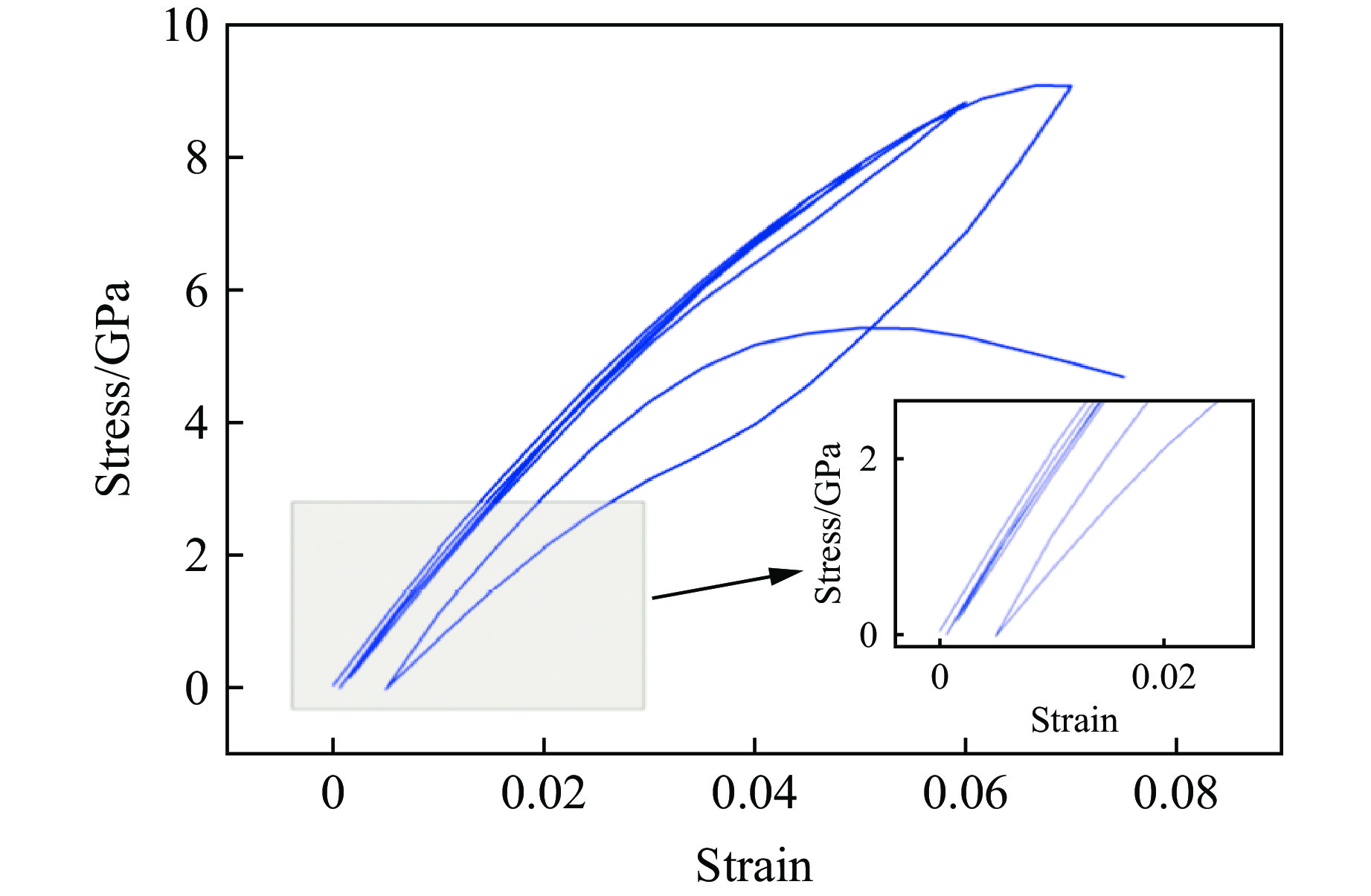
根据确定的预设峰值应变,得到循环载荷下应变增幅分别为1.0%和1.5%的单晶Nb3Sn/Nb复合材料的应力-应变曲线,如图4所示。从图4可以看出,在前几次循环过程中,模拟单元中没有微裂纹萌生(或未达到临界尺寸),应力随着应变的增加而增加。在最后一次循环过程中,裂纹扩展并且发生失稳断裂,造成复合材料内应力的下降。同时,从卸载和再加载曲线中可以观察到明显的非线性响应,并且响应曲线的非线性随着卸载时应变的增加而增强。通过计算循环过程中卸载阶段的模量Ea,得到卸载模量Ea随循环次数的变化趋势,如图5所示。从图5可以看出,Nb3Sn/Nb复合材料在循环载荷作用下的卸载模量Ea的变化趋势与实验结果[11]一致,均呈下降趋势,并且与单晶结构相比,多晶结构中模量的下降趋势更加明显。模拟结果与低温下Nb3Sn复合超导体在非对称应力下的循环实验结果[10]定性吻合。需要说明的是,模拟过程中采用了理想的材料模型,并没有考虑微裂纹、孔洞和杂质等缺陷,而实验中的样本则含有这些缺陷,因此,实验得到的拉伸强度和断裂应变较模拟值偏小。
2.2 极低温环境中Nb3Sn/Nb复合材料在循环载荷作用下的力学行为
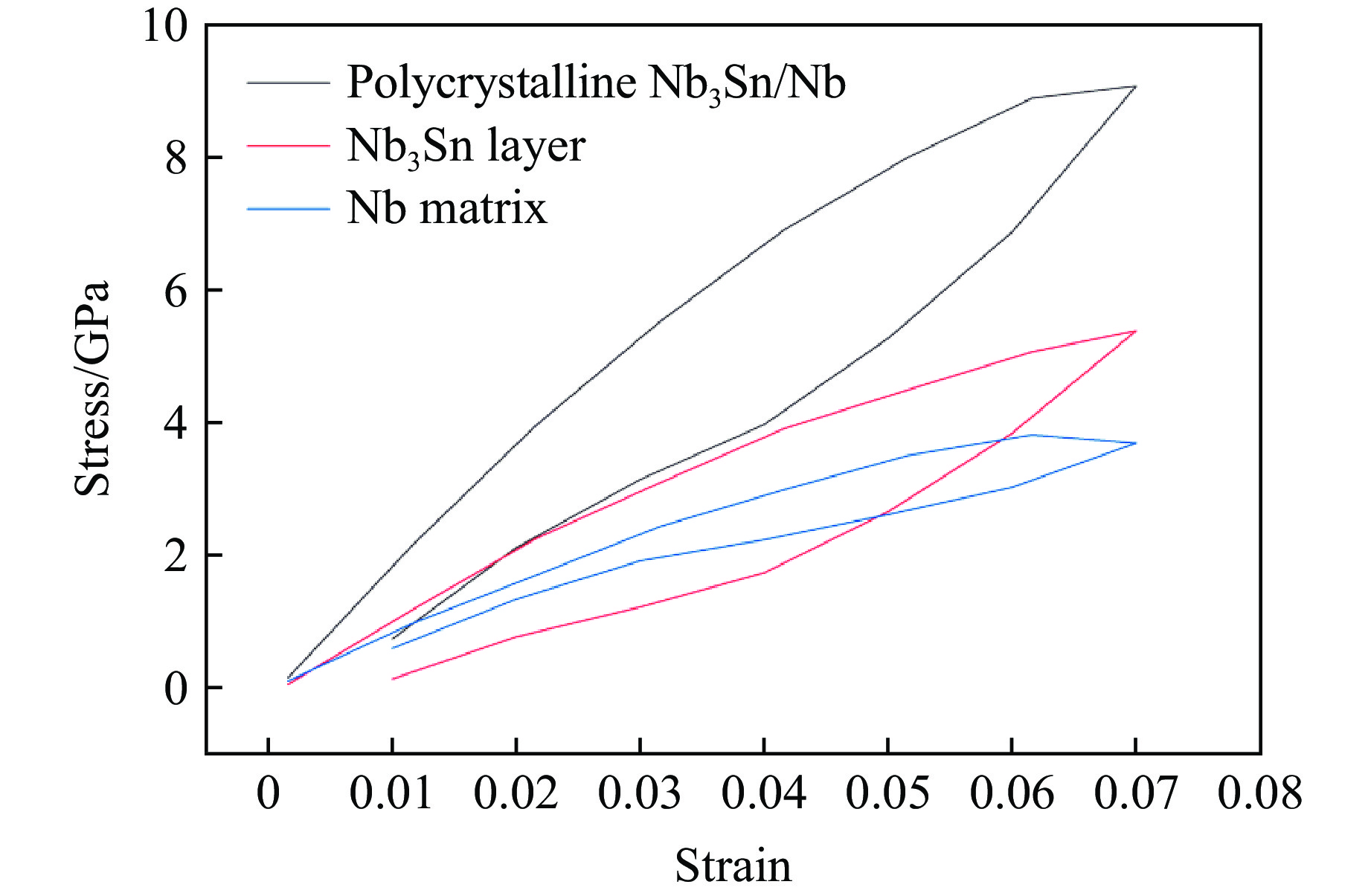
根据图6所示的循环载荷作用下应变增幅为1.0%的单晶Nb3Sn/Nb复合材料的模拟结果,可以看出,单晶Nb3Sn/Nb复合材料在循环载荷作用下的断裂应变远小于拉伸载荷作用下的断裂应变。在第3次再加载过程中,复合材料内的裂纹开始萌生;第4次再加载过程中,裂纹扩展并超过裂纹的临界尺寸,导致材料内应力下降。通过对比不同应变增幅下的循环曲线(图4),可以得到单晶Nb3Sn/Nb复合材料在循环载荷下的断裂应变0.135≤ε≤0.140。由于复合材料在循环载荷下表现出非线性响应,为了更好地研究这种滞回现象,通过计算给出循环过程中Nb3Sn层和Nb基体内的应力分布曲线,如图7所示。在加载过程中,Nb基体的模量在D点之后减小,应力增长缓慢,这是由于Nb基体中产生了位错。在卸载过程中,Nb基体的应力在C点迅速下降,这是因为Nb基体中的位错消失,使得Nb基体恢复了弹性状态,模量增加。同时,从Nb3Sn层的应力-应变曲线中可以看出,当复合材料应力为零时(即曲线中B点),由于Nb3Sn-Nb界面错配应变的作用,Nb3Sn层处于压缩状态(Nb3Sn层内应力小于零)。
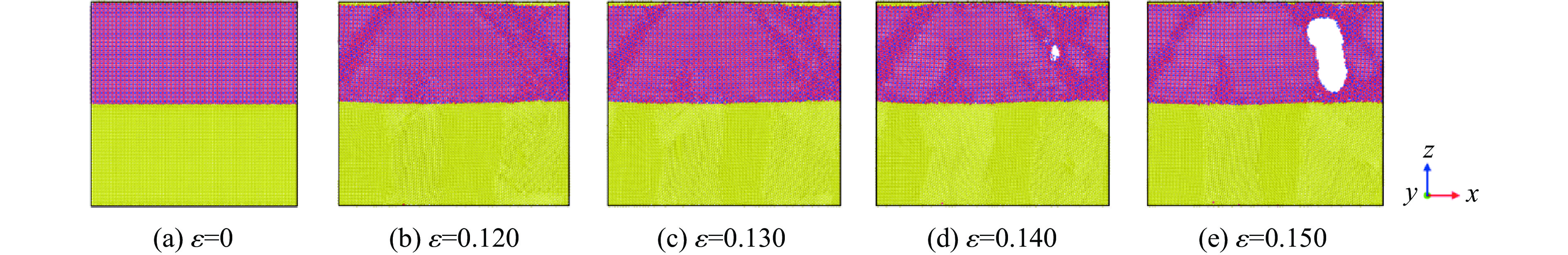
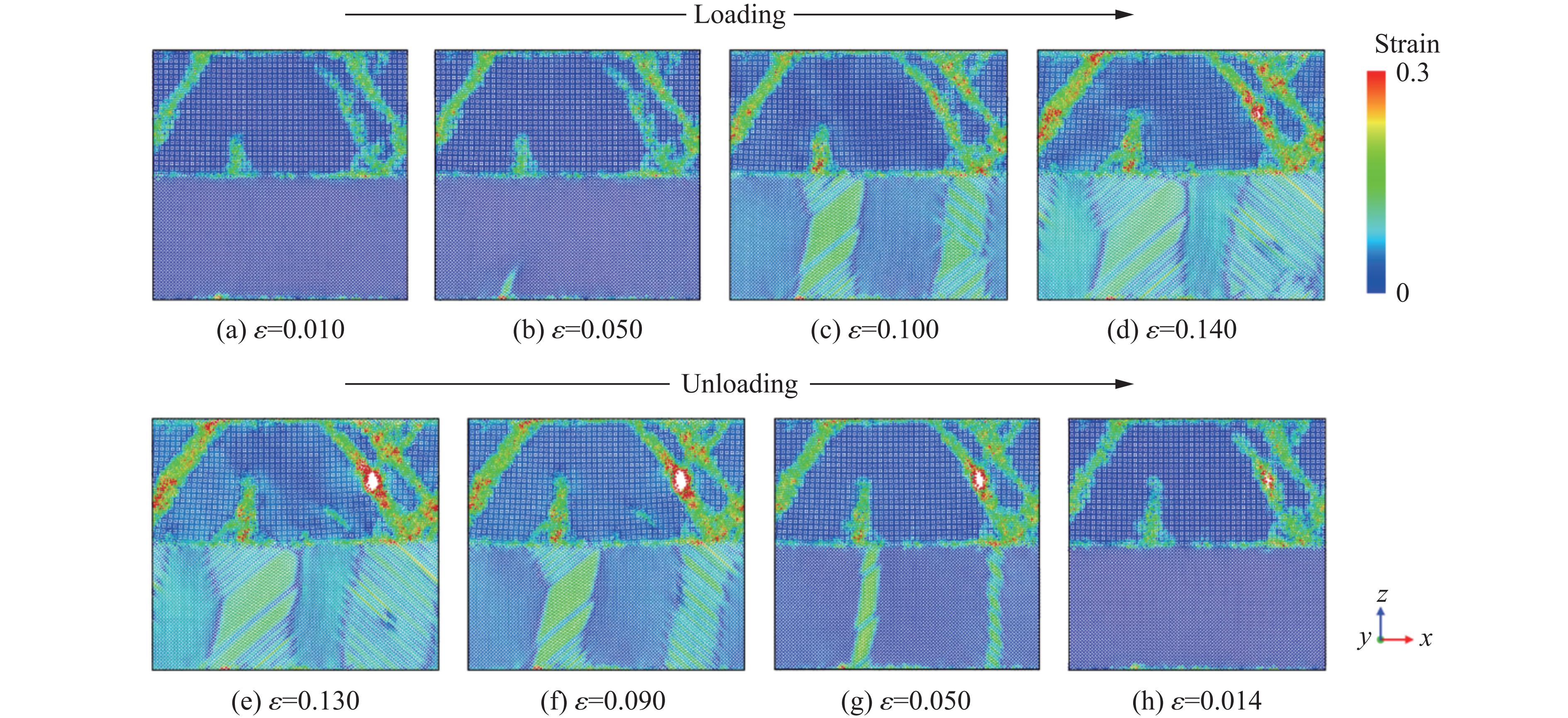
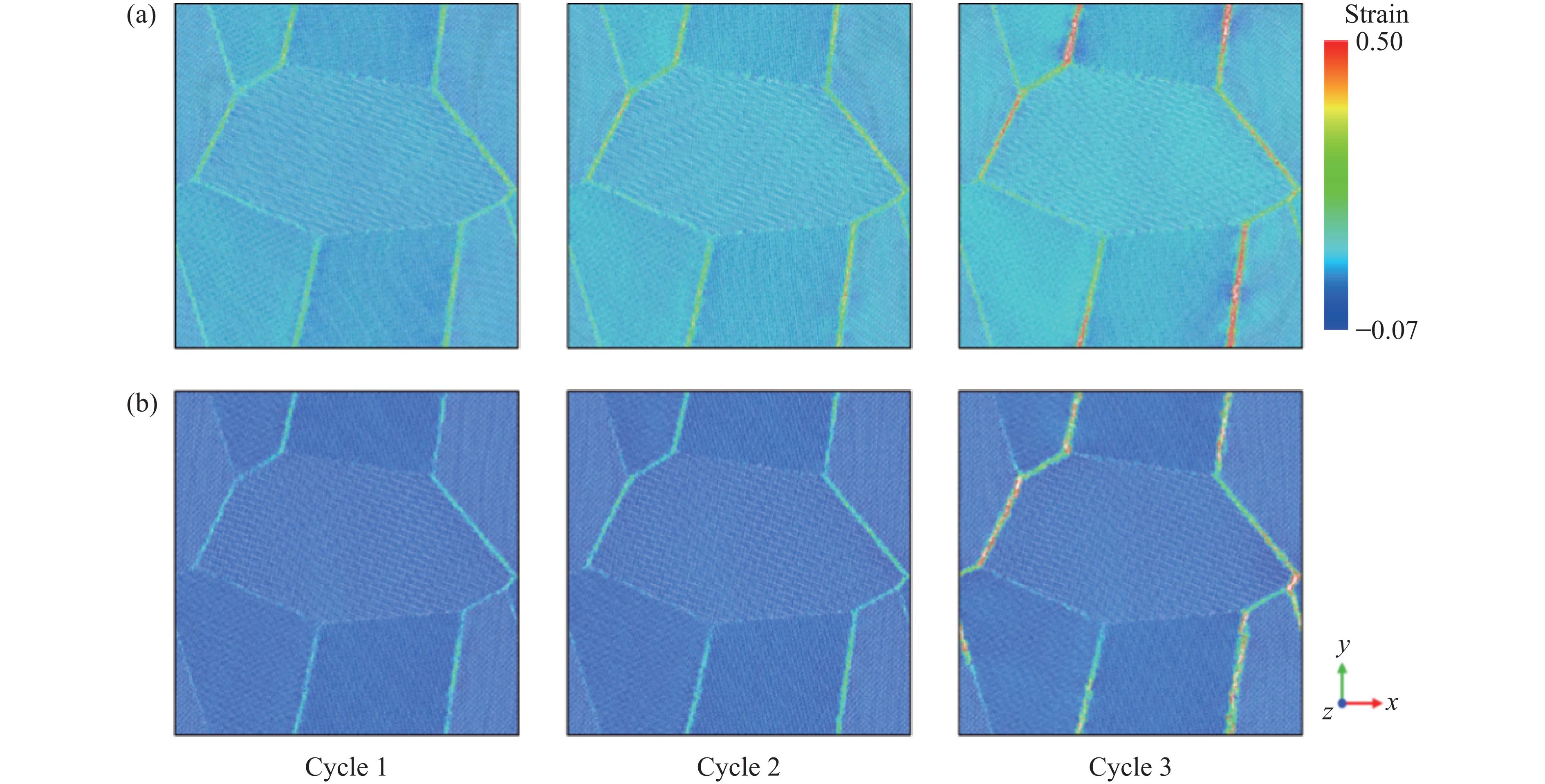
根据循环载荷作用下的应力-应变曲线,图8给出了单晶Nb3Sn/Nb复合材料中Nb3Sn层在应变ε=0时的微观结构和各个应变峰值时的单晶Nb3Sn损伤演化。从图8可以看出,在循环载荷作用下,Nb3Sn层的变形伴随着滑移带的产生和扩展以及孔洞、微裂纹等缺陷的演化过程。当应力超过Nb3Sn层的弹性极限时,与拉伸轴成45º方向上产生滑移带并扩展、交错,如图8(b)所示。这些滑移带不会随着外载荷的卸载而消失,再加载过程中,位于Nb3Sn层中的疲劳源被重新激活[34],使得Nb3Sn层中的滑移变形增加。当ε=0.140时,单晶Nb3Sn层内在滑移带交错的地方形成孔洞,当ε=0.150时,孔洞进一步扩大,发生断裂并导致复合材料内的应力下降。
为了更好地说明循环过程中复合材料内发生的弹塑性损伤变形,图9给出了加载与卸载阶段材料中的原子应变分布。从图9可以看出,当ε<0.050时(见图9(b)),Nb3Sn层中的应变集中区域没有发生明显改变,且非滑移区的原子排列整齐,表明Nb3Sn层中滑移带并没有发生扩展行为。随着应变增加,Nb基体中开始发生相变,从图9(c)和图9(d)可以看出,Nb基体内应变不均匀,在已发生相变的区域集中分布。在Nb基体作用下,位于Nb3Sn层中与Nb基体界面处的疲劳源被激活,开始向Nb3Sn层内发射,并形成位错滑移带,此时应力增长缓慢,材料处于塑性变形阶段。当ε=0.160时,在Nb3Sn层内滑移带交汇处已经产生微孔洞缺陷,但在应力-应变曲线(图6)中没有表现出应力下降趋势,表明微小的裂纹、孔洞等缺陷不会导致单晶Nb3Sn/Nb复合材料的承载能力下降。在卸载过程中,Nb3Sn层中的非滑移区会首先发生收缩,位错滑移带在其他非滑移区原子的作用下变窄。因为位于滑移带上的孔洞周围原子之间的相互作用势较小,所以当应力卸载时,非滑移区首先发生收缩,导致孔洞扩大。继续卸载时,微孔洞在周围原子的作用下发生收缩,但不会完全消失。当应力卸载至零时,如图9(h)所示,孔洞处于闭合状态。
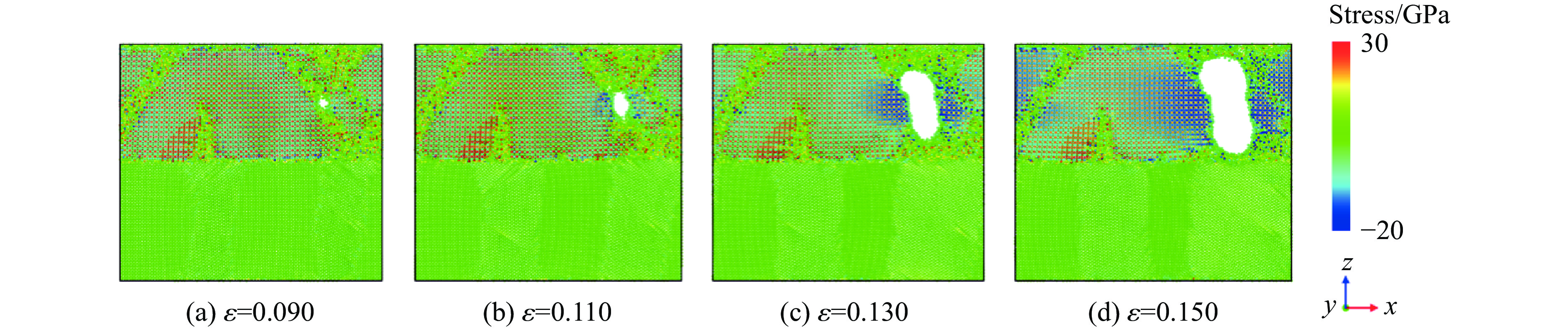
在第4次再加载过程中,在材料的强度极限下复合超导体材料失效,表明材料内部裂纹扩展并超过临界尺寸。为了研究Nb3Sn层在变形过程中伴随的裂纹扩展和损伤演化,绘制了Nb3Sn层中孔洞附近的应力云图,如图10所示。从图10可以看出,当ε=0.110时,孔洞开始扩展。在孔洞扩展过程中,孔洞垂直于x轴方向(孔洞前端)存在局部应力集中区,平行于x轴方向的应力迅速下降。随着应变加载,孔洞前端应力大于材料强度,导致孔洞垂直于x轴发生解理断裂,同时,位于滑移带且在孔洞扩展路径上的其他孔洞相互连接,进一步造成Nb3Sn层内损伤变形,导致Nb3Sn层内裂纹向滑移带方向倾斜,近似与x轴垂直。裂纹在扩展至Nb基体界面后,向Nb基体中延伸,并导致Nb基体界面原子变得无序,存在位错堆积,进而导致材料的应力下降。
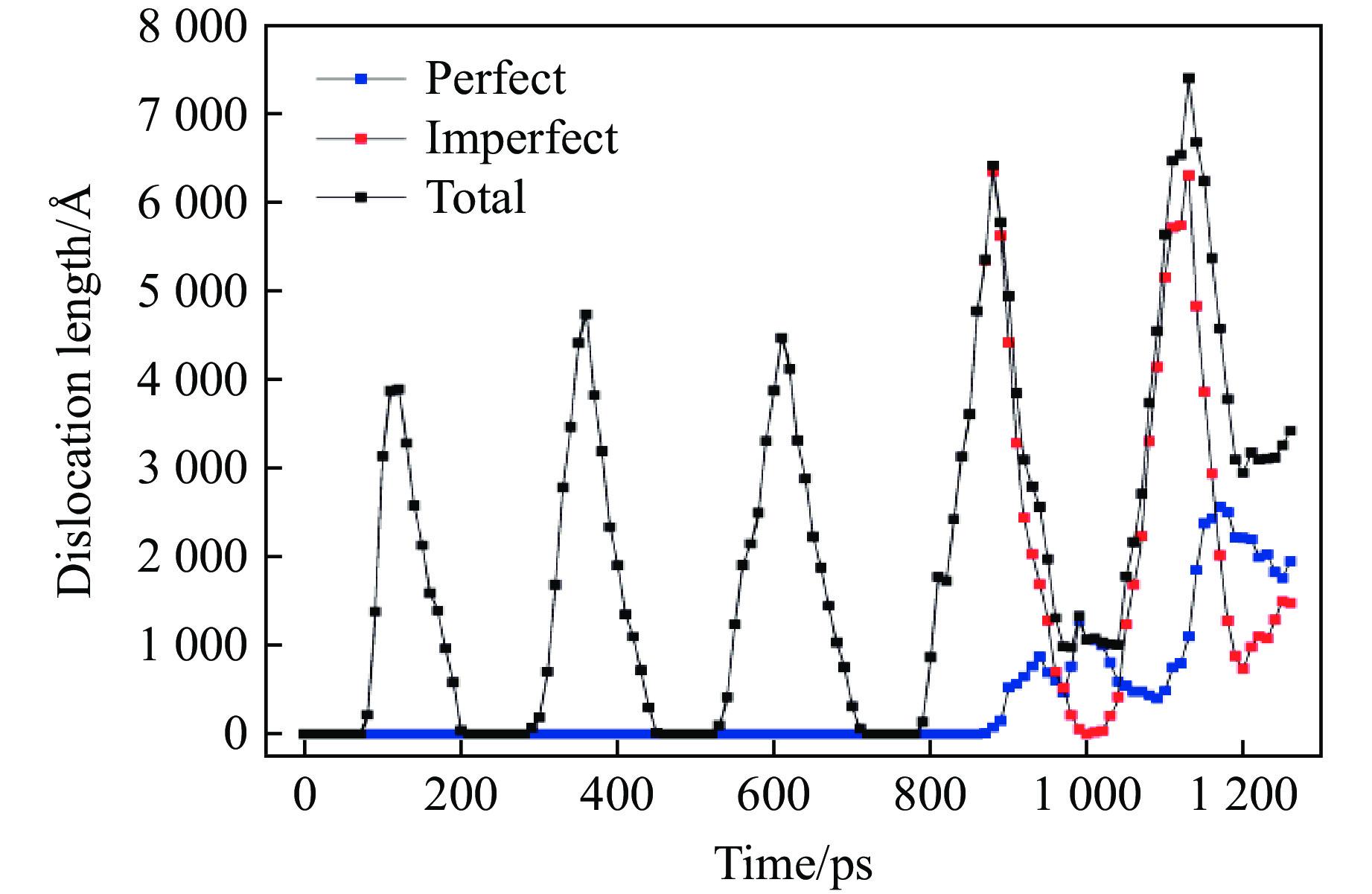
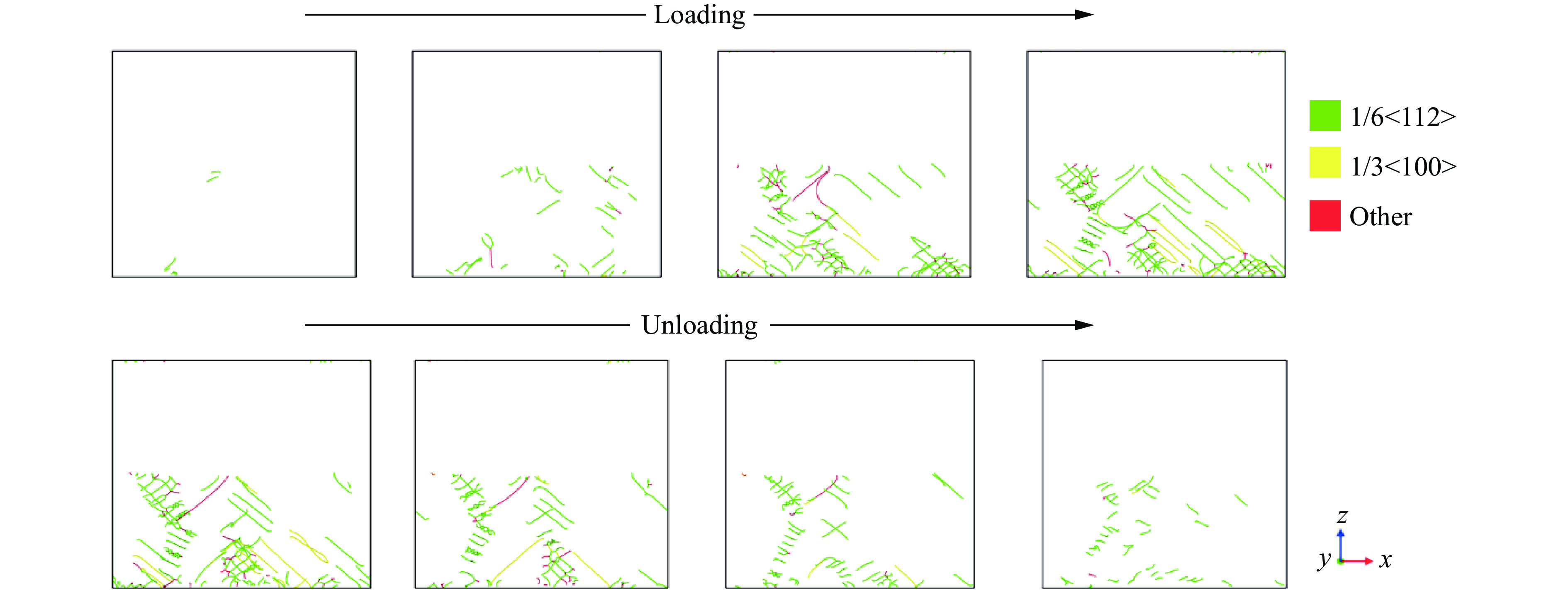
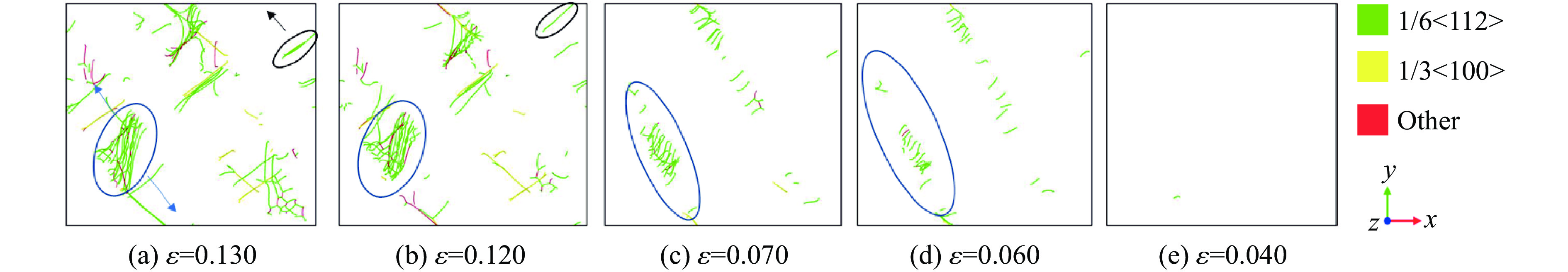
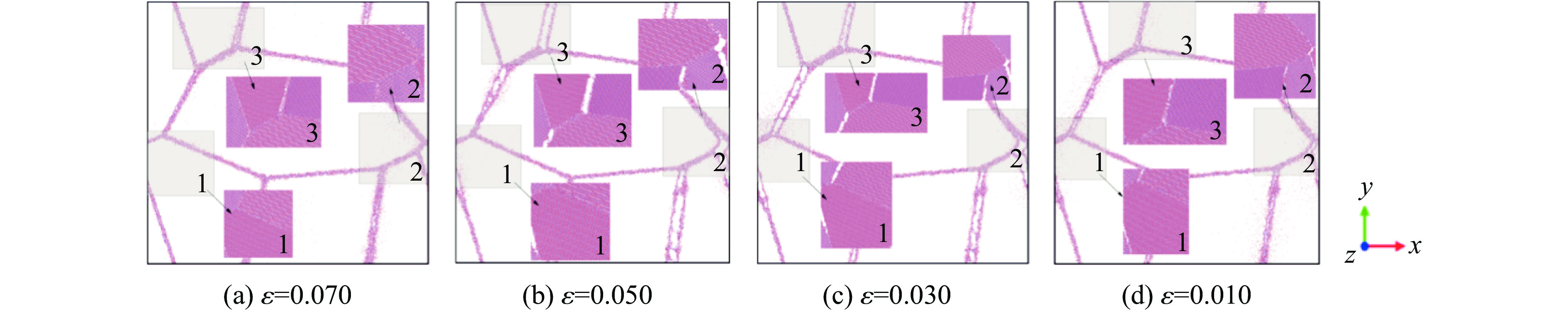
在Nb3Sn/Nb复合材料中,基体中的变形对Nb3Sn超导层的损伤演化存在影响。当施加应变大于微裂纹萌生临界应变后,继续施加循环载荷,得到了4.2 K低温环境中Nb基体部分位错密度随循环次数的变化,如图11所示。从图11可以看出,前3次卸载后,Nb基体中的位错密度恢复为零,而在第4次卸载后,Nb基体中的位错并未完全消失,与Liang等[35]对珠光体钢疲劳模拟得到的结果一致,说明在循环载荷下Nb基体中存在位错湮灭机制。Essmann等[36]报道的体心立方(BCC)金属在低温环境循环实验中位错湮灭的微观过程,为位错湮灭机制提供了实验支持。在此基础上,Liang等[35]根据模拟结果进一步指出,在复合材料界面处存在两种不同的位错湮灭机制:一种是位错滑移到相反的界面而自然消失,另一种是位错在同一界面上形成位错核然后消失。这两种相互竞争的湮灭机制使材料的位错密度在前几次循环卸载后大幅度下降直至为零,在之后的循环卸载过程中,位错密度下降但不为零。结合Liang等[35]的模拟结果,可以推测在循环载荷下Nb基体中同样存在两种相互竞争的位错湮灭机制。图12展示了循环过程中Nb基体内主要的位错类型与位错分布,其中,绿色、黄色和红色分别对应Nb基体内1/6<112>、1/3<100 >和其他类型的位错。从图12中可以看出,Nb基体内主要的位错是肖克利不全位错。在应力加载过程中,首先从Nb3Sn-Nb界面处产生1/6<112>肖克利不全位错,然后随着应变的增加,位错扩展并向Nb基体内迁移,此时有少量1/3<100>位错产生。卸载阶段中,观察到位于Nb3Sn-Nb界面的位错先消失,然后Nb基体内部位错向界面处移动,最终消失。
为了更直观地观察Nb基体内位错的迁移与湮灭过程,图13展示了第3次卸载过程中Nb基体内的位错运动。从图13(a)可以看出,黑色圆圈内位错沿最大切应力方向,即黑色箭头方向发生迁移,最终移动到Nb基体界面并排出模拟单元,从而自然消失。蓝色圆圈内存在的大量位错经过一段时间卸载后,位错密度下降。这是由于在卸载过程中,部分伯氏矢量等大且方向反向的位错相遇并相互抵消,发生湮灭。剩余的位错经过一段时间后,通过位错迁移的方式沿最大切应力方向离开模拟单元。通过对比黑色圆圈与蓝色圆圈内的位错运动,可以发现位错的滑移并不是连续的[37–38],而是先经过一个短暂的蓄力期,然后开始发生移动,并且不同位错的蓄力期长短不一。
在前3次卸载过程中,大部分位错通过位错迁移的方式排出Nb基体自然消失,而在第4次卸载后Nb基体内有位错存在,表明位错成核机制成为不全位错消失的主要原因。图14展示了第4次卸载过程中Nb基体内的全位错类型与分布。由图14可知,在Nb基体界面处产生了1/2<111>全位错以及少量<100>和其他类型的位错。这是因为不全位错沿最大切应力方向运动到Nb3Sn-Nb界面时,Nb3Sn-Nb界面在Nb3Sn层滑移带以及微裂纹、孔洞缺陷的作用下变得无序,位错在Nb基体界面处不能全部湮灭。部分位错发生位错反应生成新的位错核,并且新的位错向Nb基体内部扩展。从图14(d)中可以得到验证,Nb基体的孔洞缺陷使得Nb3Sn-Nb界面变得无序,Nb基体内位错在孔洞附近集中分布。同时,Nb基体中的全位错促进了单晶Nb3Sn/Nb复合材料中裂纹的扩展,进一步加剧了材料内部的损伤演化。
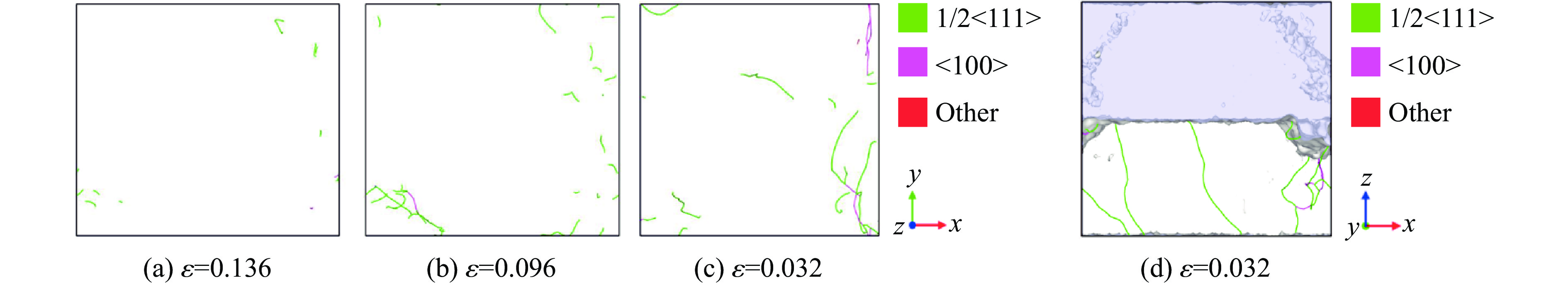
工程实用的Nb3Sn材料多为多晶体结构,图15给出了多晶Nb3Sn/Nb复合材料在循环载荷作用下的应力-应变曲线。从图15中可以看出,相较于单晶结构,多晶结构在循环载荷作用下的极限强度更小。在材料失效断裂之前,多晶结构卸载后的残余应变较小,当应变达到0.070时,裂纹开始萌生并扩展,此时多晶Nb3Sn/Nb复合材料在卸载时表现出明显的非线性响应,并且卸载后的残余应变增加。从图16可以看出,在第3次卸载过程中,Nb3Sn层晶界处产生微裂纹,而在Nb3Sn层内微裂纹的作用下,Nb基体在卸载后也产生了少量的塑性累积。与单晶结构类似,由于卸载后Nb3Sn-Nb界面错配应变作用,导致Nb3Sn层处于被压缩状态。在第4次再加载过程中,复合材料中的微裂纹扩展并最终导致断裂,从而使应力下降。根据递增塑性法,可以推断循环载荷下多晶Nb3Sn/Nb复合材料的断裂应变为0.060≤ε≤0.070。与实验结果[10]相比,多晶体复合结构的塑性变形不明显,可能有以下3点原因:(1) 模拟中采用的多晶Nb3Sn/Nb为理想结构,而实验中采用的Nb3Sn股线中还含有Cu基体,Cu基体中的塑性变形是整体塑性变形的重要组成部分;(2) 模拟中多晶Nb3Sn层的平均晶粒尺寸较小,在没有初始微裂纹、孔洞等缺陷干扰的情况下,裂纹在晶界处产生,而晶粒内部没有产生明显的滑移带与微裂纹;(3) 模拟中晶界呈现规则的几何形状,晶界交汇点较少,对裂纹的扩展不能起到很好的抑制作用,使晶界处的微裂纹通过三叉晶界处后沿晶界迅速扩展形成解理裂纹并导致断裂。
循环载荷作用下多晶Nb3Sn/Nb复合材料的原子应变云图如图17所示,其中图17(a)和图17(b)分别表示循环中峰值应变与卸载后多晶Nb3Sn的应变分布。从图17(a)可以看出,多晶Nb3Sn内的应变集中主要发生在晶界处。由于晶界处的应力集中得不到松弛,应力峰会越来越高,当超过晶界强度时就会在晶界处产生微裂纹。从图17(a)还可以看出,在第3次循环应变分布图中,多晶Nb3Sn在晶界处有微裂纹萌生。从图17(b)可以看出,在第3次卸载后的应变分布中,Nb3Sn晶界处的微裂纹收缩。
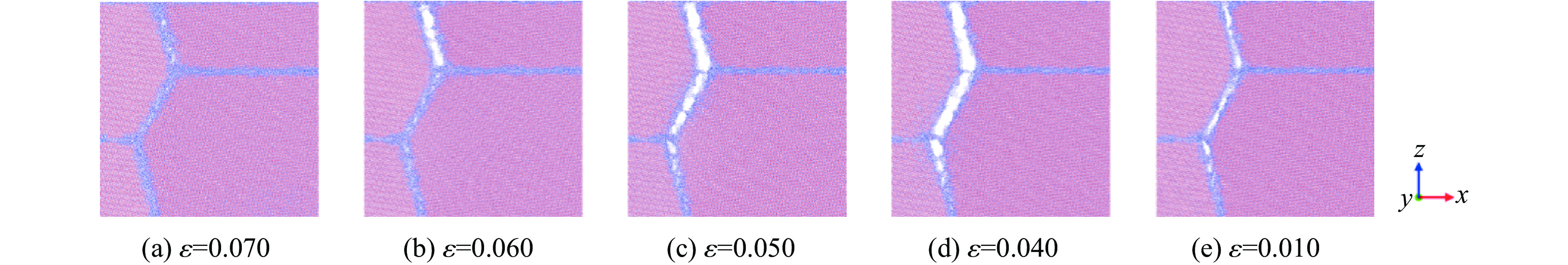
卸载过程中多晶Nb3Sn晶界处的损伤演化过程如图18所示。从图18可以看出,开始卸载时,部分晶界(如图18中的区域2和区域3)处的微裂纹先扩展,然后逐渐收缩,而其他部分晶界(区域1)在卸载过程中产生微裂纹。研究表明,纳米材料晶界处的微裂纹萌生和扩展与晶粒旋转和晶界滑动[39]有关。Kumar等[40]报道了位错活动和晶界空位对晶粒尺寸为30 nm的NC(纳米)Ni金属材料断裂过程中裂纹扩展的影响。Ovid’ko等[41]为实验观察提供了理论支持,认为纳米材料在疲劳过程中由于晶界滑动和三叉点储存的弹性势能释放,其裂纹在三叉点附近成核,即产生空位。图19给出了循环载荷下多晶Nb3Sn层晶界处的裂纹扩展与收缩过程。在循环载荷作用下,晶界滑动使多晶Nb3Sn在三叉晶界处产生孔洞缺陷,如图19(a)所示。卸载时,Nb3Sn晶粒收缩,晶粒内原子间相互作用力大于晶界处原子间相互作用力,此时晶界处受到相邻晶粒间的拉伸作用,加剧晶界处的裂纹萌生。当微裂纹扩展至三叉晶界处时,如图19(b)所示,裂纹的进一步扩展受到附近晶界的阻碍。根据Ovid’ko等[42]提出的理论模型,裂纹钝化程度和抗增殖能力与晶粒大小成反比。在本研究中,Nb3Sn晶粒尺寸均大于40 nm,晶界处的微裂纹受到阻碍后,通过晶界脱聚的方式使裂纹通过三叉晶界处,此时晶界处二面角钝化,随后裂纹尖端通过连接点、孔洞等缺陷的方式沿着与拉伸轴较大夹角的晶界扩展,形成弯曲裂纹,如图19(c)所示。Nb3Sn层晶界处裂纹的扩展方式符合Ovid’ko等[42]的理论模型。经过一段时间的卸载后,即当ε=0.040时,晶粒内弹性变形恢复,在Nb3Sn-Nb界面错配应变的作用下,Nb3Sn晶界处受力发生改变,位于晶界处的微裂纹重新收缩,直至复合材料的应力完全卸载。
在实际工程应用中,Nb3Sn超导体内通常存在晶界偏析现象,并且从Nb3Sn裂纹断口处可以检测到高浓度的Cu和Sn元素[43]。为了减少晶界处Sn原子的偏析现象,通常在Nb3Sn制备过程中运用退火工艺,这是因为退火过程中晶界处偏析的Sn原子与Nb原子充分反应,使Nb3Sn晶粒继续生长,然而这将会导致材料强度下降[44]。同时,在制备过程中还需要添加Ti等元素,利用晶界处偏析的Ti原子或形成富Ti的析出相促进Sn原子在Nb3Sn层中的有效扩散,使Nb3Sn晶粒细化,提高Nb3Sn的产率和超导性能[45]。然而,在力学性能方面,添加Ti会导致Nb3Sn超导丝的抗拉强度和断后伸长率下降[46]。在后续的研究中,晶界偏析现象对于低温循环载荷作用下力学性能衰退的影响仍需要进一步讨论。
2.3 极低温环境中单晶和多晶Nb3Sn/Nb复合材料在循环载荷作用下的应变率效应
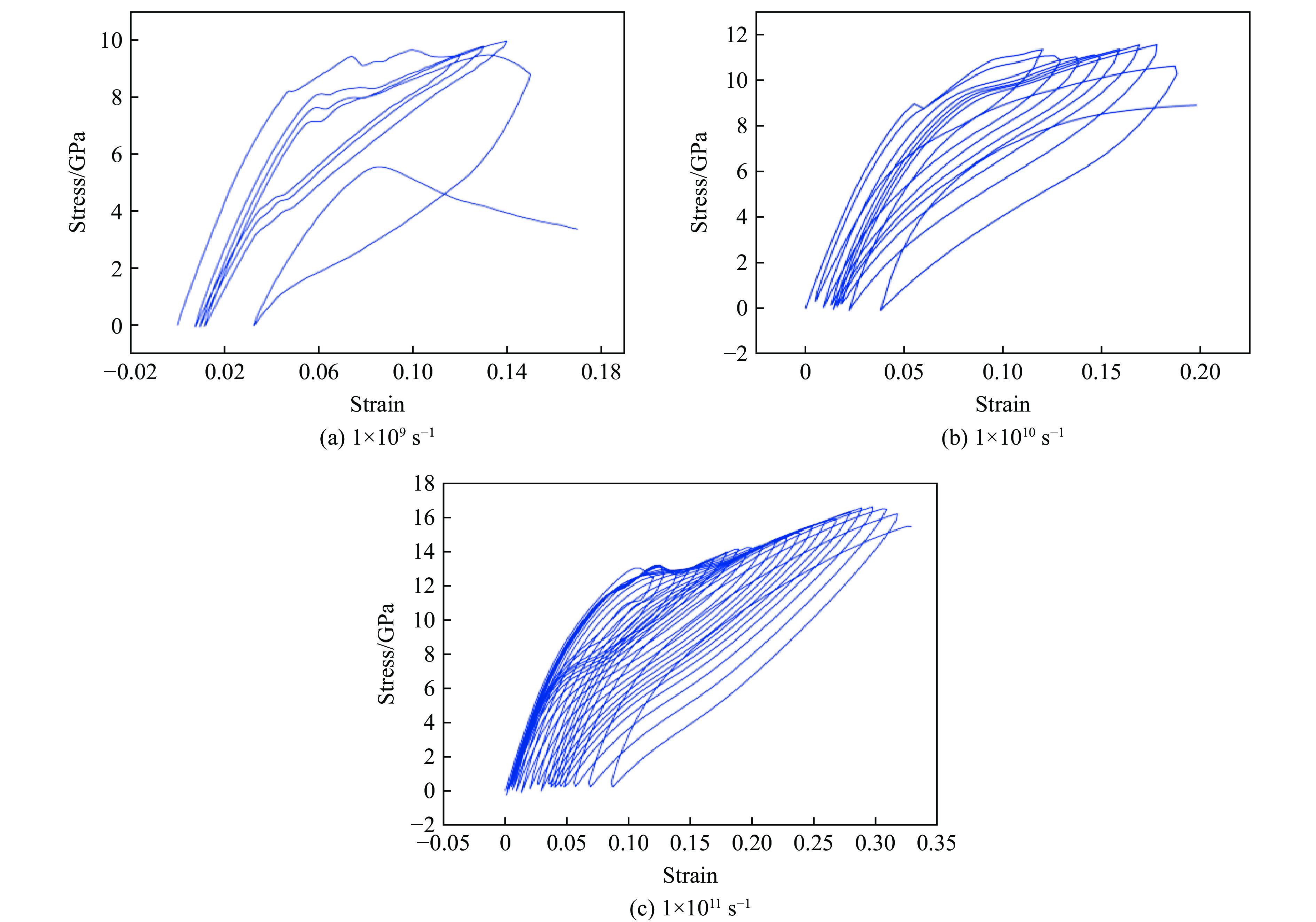
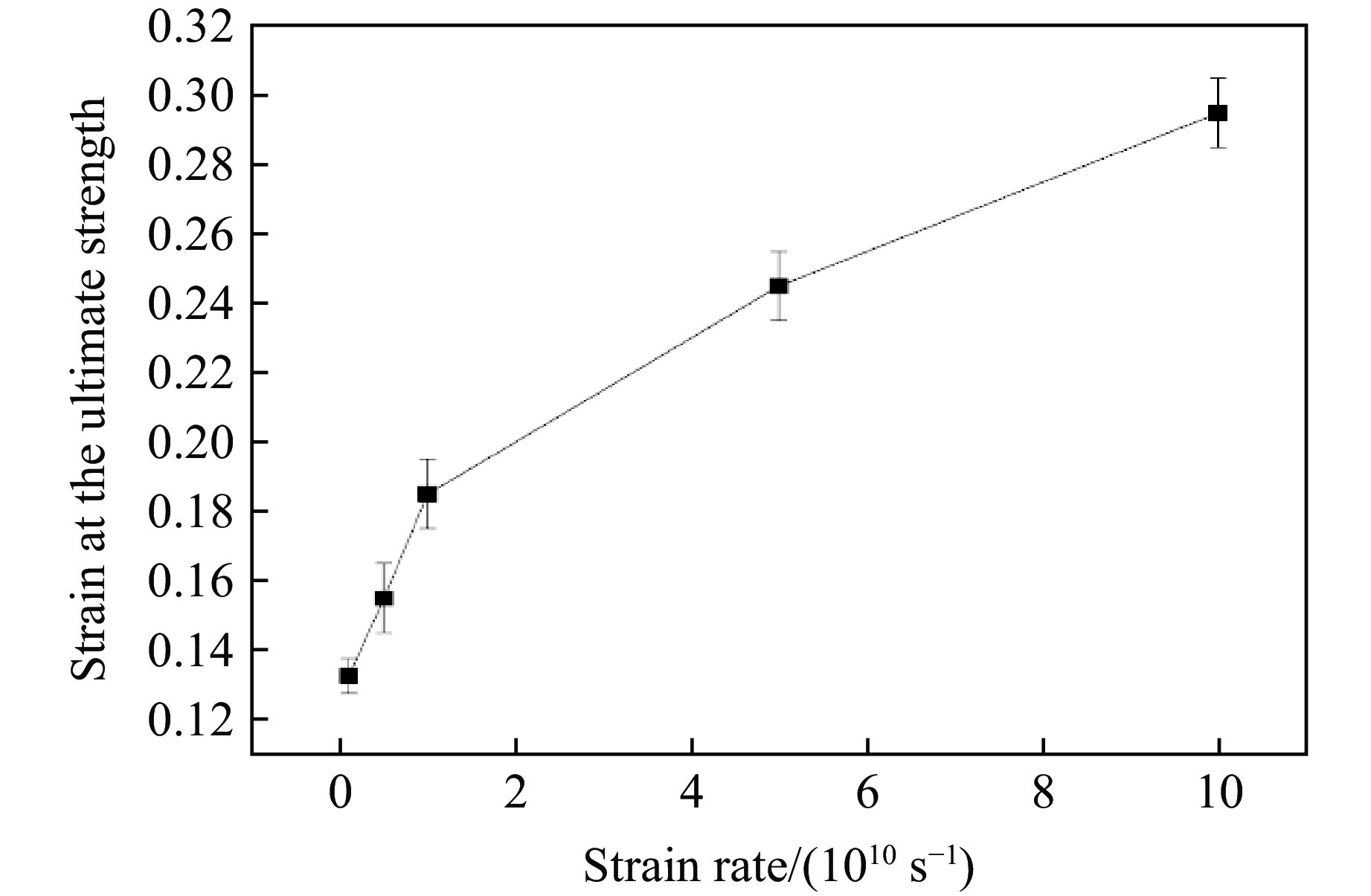
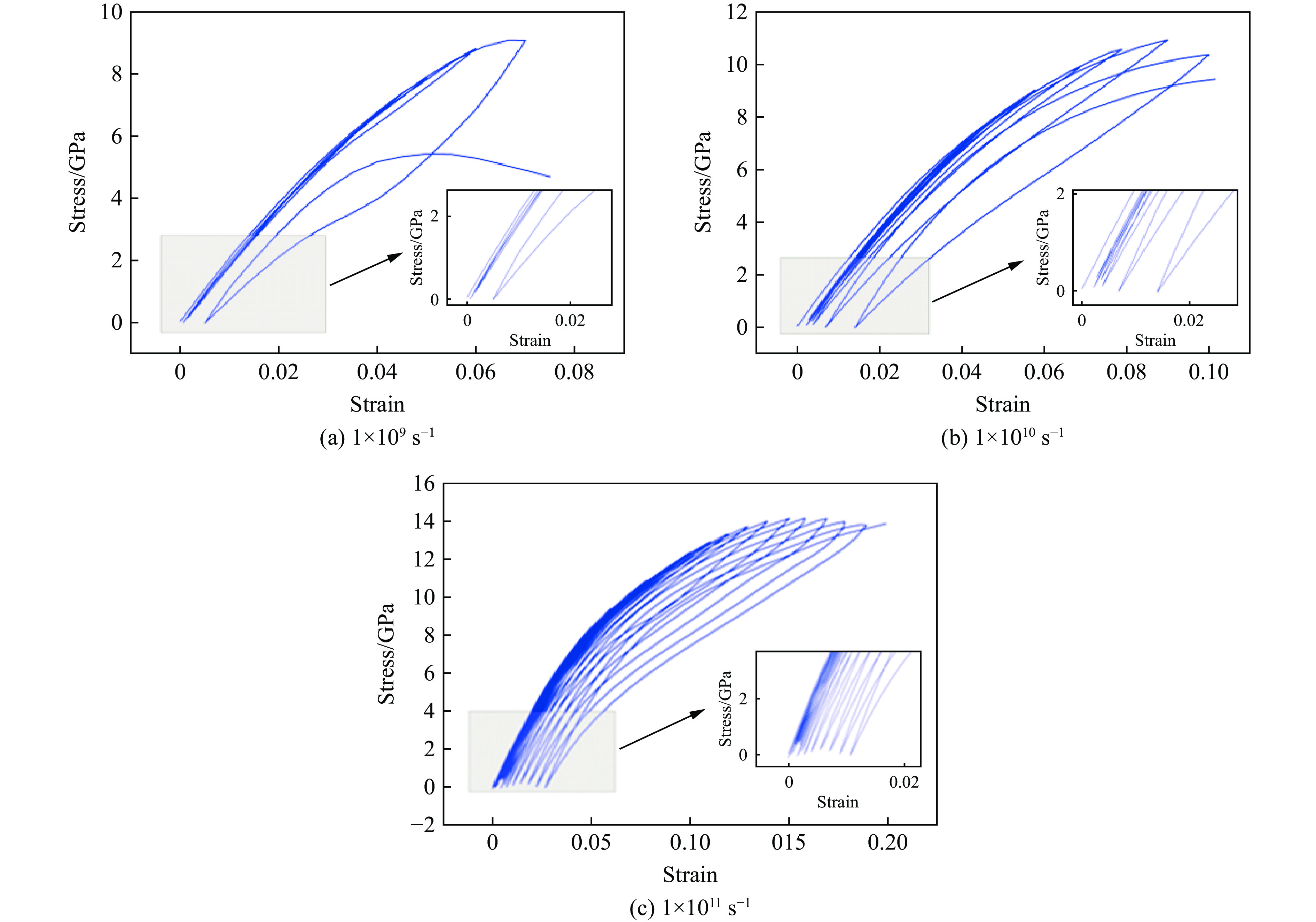
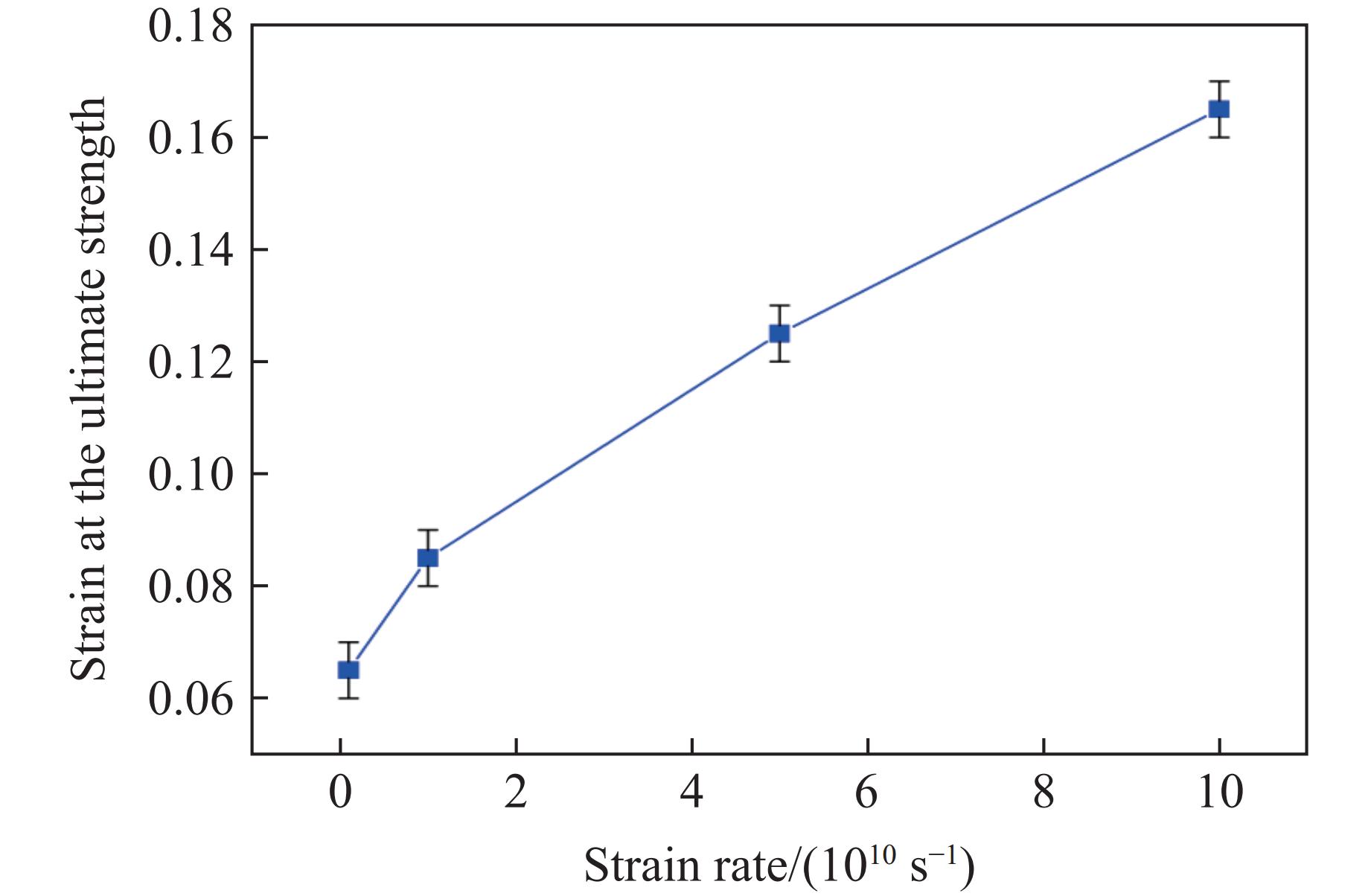
为了分析极低温环境中不同应变率对单晶与多晶Nb3Sn/Nb复合材料在循环载荷下晶体损伤变形的影响,进行了3组模拟实验,应变率分别为1×109、1×1010、1×1011 s−1。图20给出了4.2 K低温环境下不同应变率下单晶Nb3Sn/Nb复合材料的应力-应变曲线。从图20中可以看出,不同应变率下,单晶Nb3Sn/Nb复合材料的屈服应力不同,并且随着应变率的增加而增加。在低应变率作用下,材料中的裂纹会快速扩展并失稳断裂,导致材料的力学性能退化;而在高应变率下,裂纹会在循环载荷作用下缓慢扩展,导致材料产生应力松弛和力学性能退化。根据单晶Nb3Sn/Nb复合材料在循环载荷下的极限强度,得到极限强度下应变与应变率之间的关系,如图21所示。从图21可以看出,在循环载荷作用下,材料在极限强度下的应变与应变率呈正相关,即随着应变率的增加而增加。同时,随着应变率的增加,应变率对应变极限的影响逐渐减小。
在4.2 K的相同环境温度下,当应变峰值为0.150时,不同应变率下的单晶Nb3Sn/Nb复合材料的卸载结果如图22所示。从图22可以看出,不同应变率作用下,单晶Nb3Sn/Nb复合材料先发生弹性变形,应力-应变曲线基本重合,随着应变增加,低应变率作用下的材料进入塑性变形阶段,而高应变率作用下材料仍处于弹性变形阶段,进一步验证了应变率会影响材料的屈服强度,高应变率下材料的屈服强度提高。当ε=0.150,即到达设定的应变峰值时,应变率越高,材料内的应力越大。此时,对材料采用不同应变率进行卸载过程模拟,可以观察到不同应变率下卸载时应力-应变曲线各不相同,在高应变率下材料的卸载过程呈现非线性增强现象。
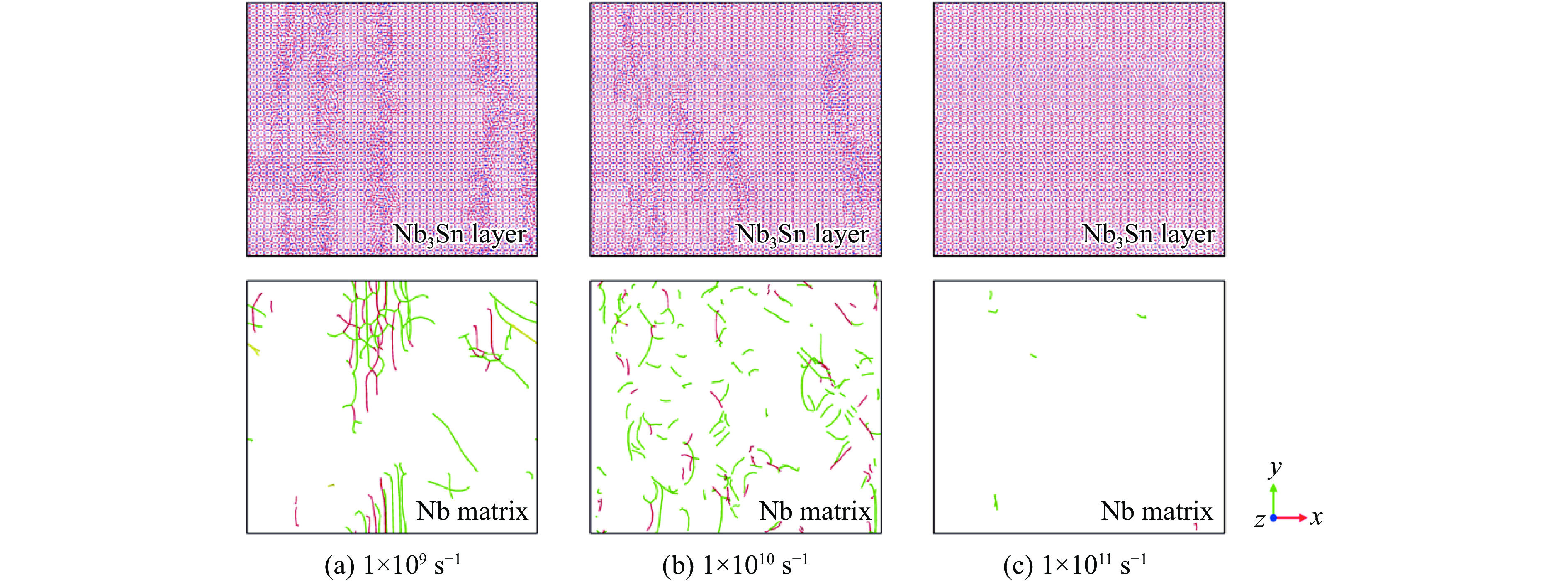
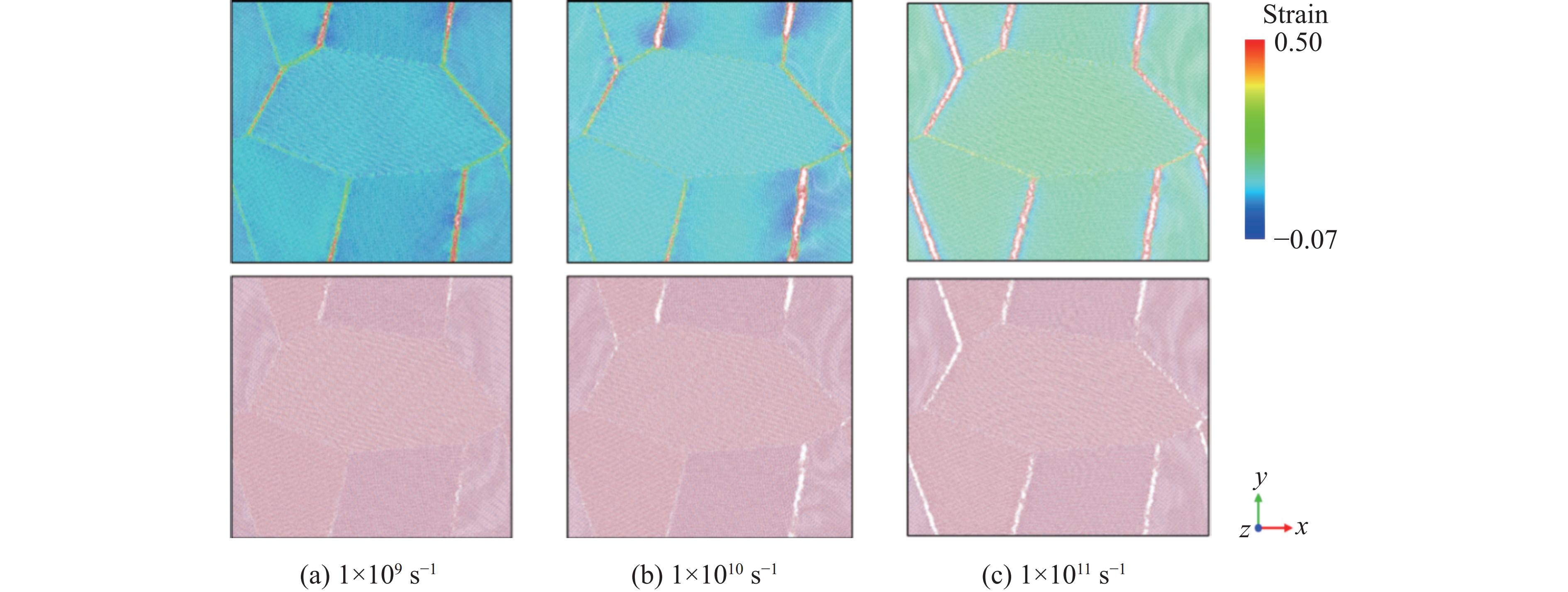
图23给出了应变峰值为0.150时,不同应变率下单晶Nb3Sn层中滑移带分布以及Nb基体中的位错分布。当应变率为1×109 s−1时, Nb3Sn层内有多条平行的位错滑移带。在Nb基体内,位错主要分布在Nb3Sn层内位错滑移带的正下方。当应变率为1×1010 s−1时,Nb3Sn层内存在少量位错滑移带,而Nb基体内位错分布相对均匀。当应变率为1×1011 s−1时,Nb3Sn层内没有位错滑移带产生,并且Nb基体内只能观察到少量不全位错。通过对比不同应变率下Nb3Sn层与Nb基体中位错和滑移带的分布情况,可以验证单晶Nb3Sn/Nb复合材料的拉伸强度随应变率的增加而增加。具体来说,随着应变率的增加,Nb3Sn层内滑移带的数量减少,同时Nb基体中的位错密度也减少,且位错分布趋向均匀,这些因素共同导致了材料拉伸强度的增大。
不同应变率载荷作用下,当应力达到极限强度时,Nb3Sn层中的微观原子结构如图24所示。当应变率为1×109 s−1时(图24(a)),Nb3Sn层内存在相互平行的位错滑移带,在滑移带上可观察到极微小的孔洞缺陷,在再加载过程中,这些微小的孔洞缺陷快速扩展形成微裂纹,使Nb3Sn层断裂,应力值下降。当应变率为1×1010 s−1时(图24(b)),Nb3Sn层内位错滑移带数量比应变率为1×109 s−1时多,并在位错滑移带上存在较大的孔洞缺陷。Nb3Sn层中孔洞的扩展是导致复合材料力学性能下降的主要原因。当应变率为1×1011 s−1时(图24(c)),Nb3Sn层内存在大量位错,但没有观察到明显的位错滑移带,在位错堆积处存在多个孔洞缺陷。
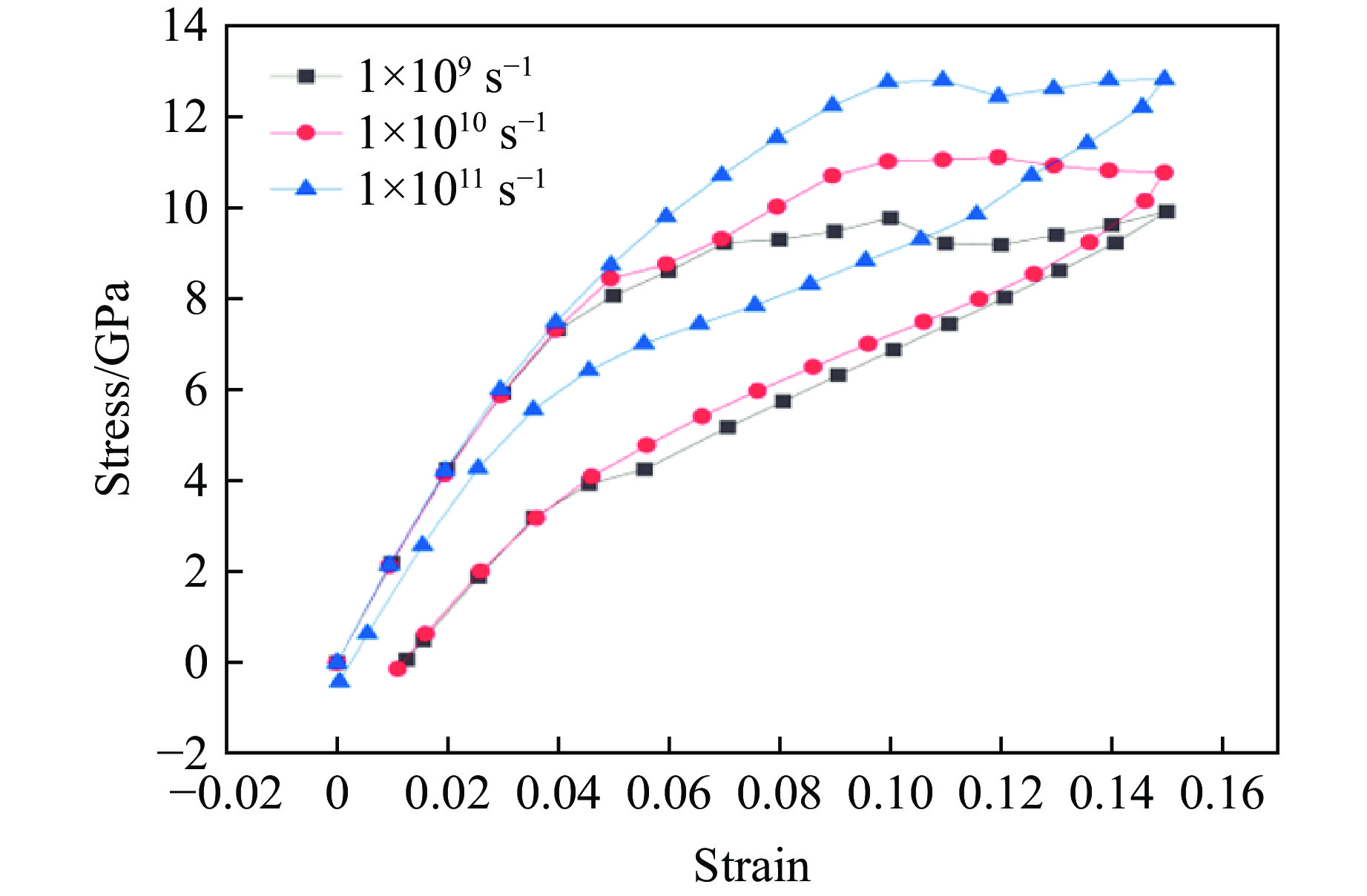
图25给出了不同应变率下多晶Nb3Sn/Nb复合材料在循环载荷下的应力-应变曲线。从图25可以看出,随着应变率的增加,多晶Nb3Sn/Nb复合材料的拉伸强度增大。在循环载荷下,多晶Nb3Sn/Nb复合材料的极限强度受应变率影响,循环载荷作用下材料极限强度应变随应变率的变化趋势如图26所示。从图26可以看出,随着应变率的增加,多晶材料在极限强度下的应变也逐渐增加,与单晶结构的结果相似。同时,随着应变率的增加,应变率对材料极限强度应变的影响逐渐减小。这是因为在高应变率下,材料的损伤过程更加复杂,导致应变率对极限强度的影响减弱。与单晶结构相比,多晶结构的极限强度比单晶结构要小得多。这是由于单晶结构中发生破坏并造成力学性能退化的主要原因是Nb3Sn晶体内部产生微裂纹、孔洞等缺陷,而多晶材料中力学性能退化的主要原因是晶界附近产生微裂纹。相比于晶体内部微裂纹的产生,晶界处微裂纹具有更低的临界应力值,更容易产生微裂纹。
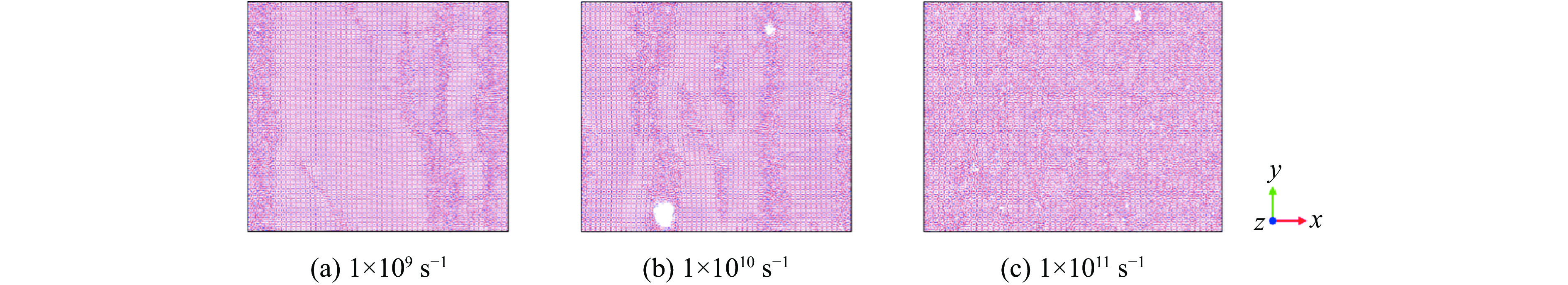
在不同应变率下,当应力达到极限强度时,多晶Nb3Sn层中的微结构与应变分布如图27所示。从应变云图中可以看出,随着应变率的增加,Nb3Sn的屈服应力增大,多晶Nb3Sn晶粒中应变增大。从微结构图中可以看出,当应变率为1×109 s−1时,多晶结构中晶界处的微裂纹扩展是导致材料力学性能退化的主要原因。随着应变率的增加,晶界对材料力学性能的影响逐渐降低,当应变率为1×1011 s−1时,多晶Nb3Sn/Nb复合材料的韧性增加,当应力达到极限强度时,Nb3Sn晶界处微裂纹数量增加,晶界处微裂纹扩展造成复合材料应力下降。同时,由于高应变率(1×1011 s−1)下,Nb3Sn层与Nb基体的相互作用减小,使得复合材料在循环载荷下的应力-应变曲线过峰后,即Nb3Sn层出现大量微裂纹时,Nb3Sn/Nb复合材料仍具有较高的剩余强度。
综上所述,在循环载荷下,单晶和多晶Nb3Sn/Nb复合材料发生失效断裂的原因不同。在单晶结构中,Nb3Sn层内的滑移带随着循环次数的增加而不断加宽,当滑移带交错处的局部应力大于材料强度时,会出现孔洞等缺陷,从而导致复合材料断裂失效。而在多晶结构中,Nb3Sn层晶界处的应力集中得不到松弛,当应力峰值超过晶界强度时,晶界处会产生裂纹,导致Nb3Sn层发生沿晶断裂,进而使得复合材料断裂失效。此外,单晶和多晶Nb3Sn/Nb复合材料在循环载荷下的变形损伤都表现出应变率效应,并且材料的屈服强度随着应变率的增加而增加。在单晶Nb3Sn/Nb复合材料中,随着应变率的增加,Nb3Sn层中的滑移带数量增加,导致复合材料的韧性增强。在多晶Nb3Sn/Nb复合材料中,随着应变率的增加,晶界对材料强度的影响降低,同时Nb3Sn层对Nb基体的作用减小。在低应变率下,多晶Nb3Sn/Nb复合材料同样发生脆性断裂;而在高应变率下,复合材料在Nb3Sn层局部断裂后具有较大的剩余强度。
3. 结 论
采用分子动力学模拟方法,对单晶和多晶Nb3Sn/Nb复合材料在循环载荷下的材料变形与裂纹扩展行为以及应变率对循环模拟的影响进行了深入分析,得到以下结论。
(1) 4.2 K极低温环境和高应变率条件下,循环载荷作用时,单晶Nb3Sn/Nb复合材料中的Nb3Sn层在受力后出现滑移,当应变增大时,Nb3Sn层内的解理裂纹垂直于拉伸轴扩展并穿过Nb-Nb3Sn晶界向Nb基体内扩展。而多晶Nb3Sn/Nb复合材料则由于晶界处应力在循环载荷下得不到松弛,当应力峰值超过晶界强度时,在晶界处萌生微裂纹导致复合材料发生沿晶断裂。
(2) 在循环载荷作用下,Nb基体内的位错存在两种位错湮灭机制。Nb-Nb3Sn界面在变形中起着多种作用,可以吸收位错,促进位错湮灭,也可以作为位错的成核点。在循环塑性变形过程中,位错成核与位错湮灭之间存在竞争关系。在循环载荷作用的初期阶段,位错通过位错运动排出Nb基体,自然消失。然而,当Nb3Sn层中出现孔洞并导致Nb基体界面处的无序结构增加时,位错成核机制占主导地位,产生全位错,进一步加剧Nb基体的塑性变形。
(3) 应变率的变化对循环载荷作用下单晶和多晶Nb3Sn/Nb复合材料的变形行为存在显著影响。随着应变率的增加,复合材料的屈服应力和断裂应变增加。在此过程中,单晶结构中的Nb3Sn层表现出不同的断裂方式。随着应变率的增加,多晶结构中Nb3Sn晶界对材料强度的影响降低。
本研究分析了Nb3Sn/Nb复合材料在循环载荷下的力学性能和微观结构变化之间的关系,研究结果对于理解超导体在循环载荷下的力学行为及其应变率效应具有重要的参考意义。
-
表 1 Nb3Sn单晶的弹性常数和晶格常数
Table 1. Elastic constant and lattice constant of Nb3Sn single crystal
Method C11/GPa C12/GPa C44/GPa Lattice constants/Å MD simulations (300 K) 284.11 95.84 53.76 5.21 Experimental results (300 K) 253.85 112.44 39.62 5.29 MD simulations (4.2 K) 333.18 127.09 53.71 5.17 Ab initio simulations (0 K) 284.83 107.73 67.07 5.32 -
[1] ZHANG R, GAO P F, WANG X Z. Strain dependence of critical superconducting properties of Nb3Sn with different intrinsic strains based on a semi-phenomenological approach [J]. Cryogenics, 2017, 86: 30–37. doi: 10.1016/j.cryogenics.2017.07.007 [2] DEVRED A, BACKBIER I, BESSETTE D, et al. Status of ITER conductor development and production [J]. IEEE Transactions on Applied Superconductivity, 2012, 22(3): 4804909. doi: 10.1109/TASC.2012.2182980 [3] KIYOSHI T, MATSUMOTO S, KOSUGE M, et al. Superconducting inserts in high-field solenoids [J]. IEEE Transactions on Applied Superconductivity, 2002, 12(1): 470–475. doi: 10.1109/TASC.2002.1018445 [4] DEVRED A, BACKBIER I, BESSETTE D, et al. Challenges and status of ITER conductor production [J]. Superconductor Science and Technology, 2014, 27(4): 044001. doi: 10.1088/0953-2048/27/4/044001 [5] BOTTURA L, DE RIJK G, ROSSI L, et al. Advanced accelerator magnets for upgrading the LHC [J]. IEEE Transactions on Applied Superconductivity, 2012, 22(3): 4002008. doi: 10.1109/TASC.2012.2186109 [6] EISENSTATT L R, WRIGHT R N. Plastic deformation in polycrystalline Nb3Sn [J]. Metallurgical Transactions A, 1980, 11(7): 1131–1138. doi: 10.1007/BF02668137 [7] CLARK J B, WRIGHT R N. Laboratory extrusion of Nb3Sn [J]. Metallurgical Transactions A, 1983, 14(11): 2295–2299. doi: 10.1007/BF02663304 [8] OCHIAI S, OSAMURA K. Influence of cyclic loading at room temperature on the critical current at 4.2 K of Nb3Sn superconducting composite wire [J]. Cryogenics, 1992, 32(6): 584–590. doi: 10.1016/0011-2275(92)90045-C [9] WESSEL W A J, NIJHUIS A, ILYIN Y, et al. A novel “test arrangement for strain influence on strands” (TARSIS): mechanical and electrical testing of ITER Nb3Sn strands [J]. AIP Conference Proceedings, 2004, 711(1): 466–473. [10] NIJHUIS A, VAN DEN EIJNDEN N C, ILYIN Y, et al. Impact of spatial periodic bending and load cycling on the critical current of a Nb3Sn strand [J]. Superconductor Science and Technology, 2005, 18(12): S273–S283. doi: 10.1088/0953-2048/18/12/009 [11] VAN DEN EIJNDEN N C, NIJHUIS A, ILYIN Y, et al. Axial tensile stress-strain characterization of ITER model coil type Nb3Sn strands in TARSIS [J]. Superconductor Science and Technology, 2005, 18(11): 1523–1532. doi: 10.1088/0953-2048/18/11/020 [12] NIJHUIS A, MIYOSHI Y, JEWELL M C, et al. Systematic study on filament fracture distribution in ITER Nb3Sn strands [J]. IEEE Transactions on Applied Superconductivity, 2009, 19(3): 2628–2632. doi: 10.1109/TASC.2009.2018082 [13] MITCHELL N. Analysis of the effect of Nb3Sn strand bending on CICC superconductor performance [J]. Cryogenics, 2002, 42(5): 311–325. doi: 10.1016/S0011-2275(02)00041-3 [14] SHETH M K, LEE P, MCRAE D M, et al. Procedures for evaluating filament cracking during fatigue testing of Nb3Sn strand [J]. AIP Conference Proceedings, 2012, 1435(1): 201–208. [15] SHEN F Z, ZHANG H C, HUANG C J, et al. Experimental study on strain sensitivity of internal-tin Nb3Sn superconducting strand based on non-destructive technology [J]. Physica C: Superconductivity and Its Applications, 2021, 584: 1353784. doi: 10.1016/j.physc.2020.1353784 [16] JIANG L, SU X Y, SHEN L Y, et al. Damage behavior of Nb3Sn/Cu superconducting strand at room temperature under asymmetric strain cycling [J]. Fusion Engineering and Design, 2021, 172: 112869. doi: 10.1016/j.fusengdes.2021.112869 [17] TABIN J, SKOCZEŃ B, BIELSKI J. Discontinuous plastic flow in superconducting multifilament composites [J]. International Journal of Solids and Structures, 2020, 202: 12–27. doi: 10.1016/j.ijsolstr.2020.05.033 [18] QIULI SUN E. Multi-scale nonlinear stress analysis of Nb3Sn superconducting accelerator magnets [J]. Superconductor Science and Technology, 2022, 35(4): 045019. doi: 10.1088/1361-6668/ac5a11 [19] MITCHELL N. Finite element simulations of elasto-plastic processes in Nb3Sn strands [J]. Cryogenics, 2005, 45(7): 501–515. doi: 10.1016/j.cryogenics.2005.06.003 [20] WANG X, LI Y X, GAO Y W. Mechanical behaviors of multi-filament twist superconducting strand under tensile and cyclic loading [J]. Cryogenics, 2016, 73: 14–24. doi: 10.1016/j.cryogenics.2015.11.002 [21] JIANG L, ZHANG X Y, ZHOU Y H. Nonlinear static and dynamic mechanical behaviors of Nb3Sn superconducting composite wire: experiment and analysis [J]. Acta Mechanica Sinica, 2023, 39(3): 122322. doi: 10.1007/s10409-022-22322-x [22] LEE J, POSEN S, MAO Z G, et al. Atomic-scale analyses of Nb3Sn on Nb prepared by vapor diffusion for superconducting radiofrequency cavity applications: a correlative study [J]. Superconductor Science and Technology, 2019, 32(2): 024001. doi: 10.1088/1361-6668/aaf268 [23] ZHANG Y, ASHCRAFT R, MENDELEV M I, et al. Experimental and molecular dynamics simulation study of structure of liquid and amorphous Ni62Nb38 alloy [J]. The Journal of Chemical Physics, 2016, 145(20): 204505. doi: 10.1063/1.4968212 [24] KO W S, KIM D H, KWON Y J, et al. Atomistic simulations of pure tin based on a new modified embedded-atom method interatomic potential [J]. Metals, 2018, 8(11): 900. doi: 10.3390/met8110900 [25] CHUDINOV V G, GOGOLIN V P, GOSHCHITSKII B N, et al. Simulation of collision cascades in intermetallic Nb3Sn compounds [J]. Physica Status Solidi (A), 1981, 67(1): 61–67. doi: 10.1002/pssa.2210670103 [26] LUTSKO J F. Stress and elastic constants in anisotropic solids: molecular dynamics techniques [J]. Journal of Applied Physics, 1988, 64(3): 1152–1154. doi: 10.1063/1.341877 [27] PENG Y X, KNIGHT C, BLOOD P, et al. Extending parallel scalability of LAMMPS and multiscale reactive molecular simulations [C]//Proceedings of the 1st Conference of the Extreme Science and Engineering Discovery Environment: Bridging from the eXtreme to the Campus and Beyond. Chicago: ACM, 2012: 37. [28] PAPADIMITRIOU I, UTTON C, TSAKIROPOULOS P. Ab initio investigation of the intermetallics in the Nb-Sn binary system [J]. Acta Materialia, 2015, 86: 23–33. doi: 10.1016/j.actamat.2014.12.017 [29] SUNDARESWARI M, RAMASUBRAMANIAN S, RAJAGOPALAN M. Elastic and thermodynamical properties of A15 Nb3X (X = Al, Ga, In, Sn and Sb) compounds-first principles DFT study [J]. Solid State Communications, 2010, 150(41/42): 2057–2060. [30] ZHANG R, GAO P F, WANG X Z, et al. First-principles study on elastic and superconducting properties of Nb3Sn and Nb3Al under hydrostatic pressure [J]. AIP Advances, 2015, 5(10): 107233. doi: 10.1063/1.4935099 [31] DE MARZI G, MORICI L, MUZZI L, et al. Strain sensitivity and superconducting properties of Nb3Sn from first principles calculations [J]. Journal of Physics: Condensed Matter, 2013, 25(13): 135702. doi: 10.1088/0953-8984/25/13/135702 [32] ESHELBY J D. Dynamical theory of crystal lattices: Max Born and Kun Huang: Oxford University Press, 1954, pp. xii+420. 50s. net. [J]. Journal of the Mechanics and Physics of Solids, 1955, 3(3): 231. [33] SCHEUERLEIN C, DI MICHIEL M, BUTA F, et al. Stress distribution and lattice distortions in Nb3Sn multifilament wires under uniaxial tensile loading at 4.2 K [J]. Superconductor Science and Technology, 2014, 27(4): 044021. doi: 10.1088/0953-2048/27/4/044021 [34] 时海芳, 任鑫. 材料力学性能 [M]. 2版. 北京: 北京大学出版社, 2015: 181–183.SHI H F, REN X. Mechanical properties of materials [M]. 2nd ed. Beijing: Peking University Press, 2015: 181–183. [35] LIANG L W, WANG Y J, CHEN Y, et al. Dislocation nucleation and evolution at the ferrite-cementite interface under cyclic loadings [J]. Acta Materialia, 2020, 186: 267–277. doi: 10.1016/j.actamat.2019.12.052 [36] ESSMANN U, MUGHRABI H. Annihilation of dislocations during tensile and cyclic deformation and limits of dislocation densities [J]. Philosophical Magazine A, 1979, 40(6): 731–756. doi: 10.1080/01418617908234871 [37] ZOTOV N, GRABOWSKI B. Molecular dynamics simulations of screw dislocation mobility in bcc Nb [J]. Modelling and Simulation in Materials Science and Engineering, 2021, 29(8): 085007. doi: 10.1088/1361-651X/ac2b02 [38] SPARKS G, MAAß R. Shapes and velocity relaxation of dislocation avalanches in Au and Nb microcrystals [J]. Acta Materialia, 2018, 152: 86–95. doi: 10.1016/j.actamat.2018.04.007 [39] PADILLA II H A, BOYCE B L. A review of fatigue behavior in nanocrystalline metals [J]. Experimental Mechanics, 2010, 50(1): 5–23. doi: 10.1007/s11340-009-9301-2 [40] KUMAR K S, SURESH S, CHISHOLM M F, et al. Deformation of electrodeposited nanocrystalline nickel [J]. Acta Materialia, 2003, 51(2): 387–405. doi: 10.1016/S1359-6454(02)00421-4 [41] OVID’KO I A, SHEINERMAN A G. Triple junction nanocracks in fatigued nanocrystalline materials [J]. Reviews on Advanced Materials Science, 2004, 7(1): 61–66. [42] OVID’KO I A, SHEINERMAN A G. Grain size effect on crack blunting in nanocrystalline materials [J]. Scripta Materialia, 2009, 60(8): 627–630. doi: 10.1016/j.scriptamat.2008.12.028 [43] SUENAGA M, JANSEN W. Chemical compositions at and near the grain boundaries in bronze-processed superconducting Nb3Sn [J]. Applied Physics Letters, 1983, 43(8): 791–793. doi: 10.1063/1.94457 [44] OCHIAI S, OSAMURA K, UEHARA T. Grain size and its relation to tensile strength of Nb3Sn compound in bronze-processed multi-filamentary superconducting materials [J]. Journal of Materials Science, 1987, 22(6): 2163–2168. doi: 10.1007/BF01132954 [45] OH S H, JEONG Y J, NA S H, et al. Atomic behavior of Ti in A15 Nb3Sn and its effects on diffusional growth of Nb3Sn layer [J]. Journal of Alloys and Compounds, 2023, 957: 170438. doi: 10.1016/j.jallcom.2023.170438 [46] OCHIAI S, OSAMURA K, MAEKAWA M. Comparison of mechanical and superconducting properties of titanium-added Nb3Sn composite wire with those of non-added ones [J]. Superconductor Science and Technology, 1991, 4(6): 262–269. doi: 10.1088/0953-2048/4/6/009 -








 下载:
下载:

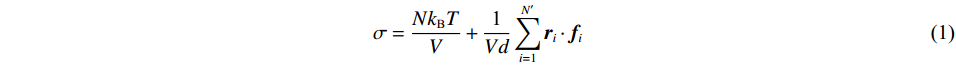
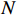
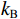
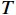
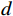
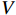
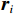
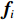
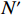

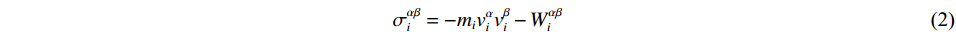
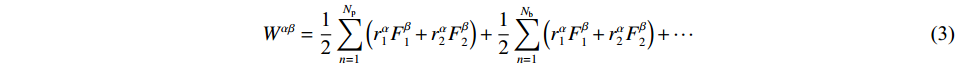
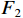
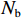
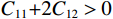
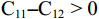
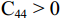
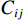


























 下载:
下载: