Understanding the atomic-scale behaviors of materials under dynamic loading conditions is crucial. This understanding helps us comprehend their complex responses at high strain rates and has implications across various research fields, such as material science, geophysics, and defense industries. One prevalent method for this investigation is dynamic X-ray diffraction (XRD). Dynamic XRD utilize pulsed X-ray sources to analyze the crystal structures of materials undergoing dynamic compression or decompression^[1]. A laser with a single pulse energy greater than 100 J can produce X-rays strong enough for diagnostic dynamic XRD experiments. Typical laser facilities used for this purpose include Omega^[2], NIF (National Ignition Facility)^[3], SG-Ⅱ^[4–5] , et al. Compared with third-generation light sources, the laser-generated X-ray light sources can serve as wide-angle light sources and are more suitable for diagnosing single crystal samples. Several researchers have utilized dynamic XRD in their studies. Kalantar et al.^[6–7] employed XRD to investigate shocked crystals and observe the α-ε transition in shock-compressed iron at Omega facility^[2]. Li et al.^[8] used XRD to diagnose the dynamic loading of an iron sample at the SG-Ⅱ facility. Rygg et al.^[9] conducted XRD on a powder sample at the TARDIS experimental platform of the NIF facility.

For powder sample XRD, the process based on laser-induced X-rays is the same as that used with third-generation X-ray facilities. There are several programs available for data-processing in XRD experiments, such as SHELX^[10]. Rygg et al.^[9] considered the thickness of the sample for Laue diffraction to improve the accuracy of the lattice parameters to a level of 0.4% at the TARDIS experimental platform of NIF. However, for single crystal XRD using divergent X-rays, the direction of the incident X-ray is not known. This makes the data processing more difficult than it is for powder XRD, where the direction of X-ray is known.

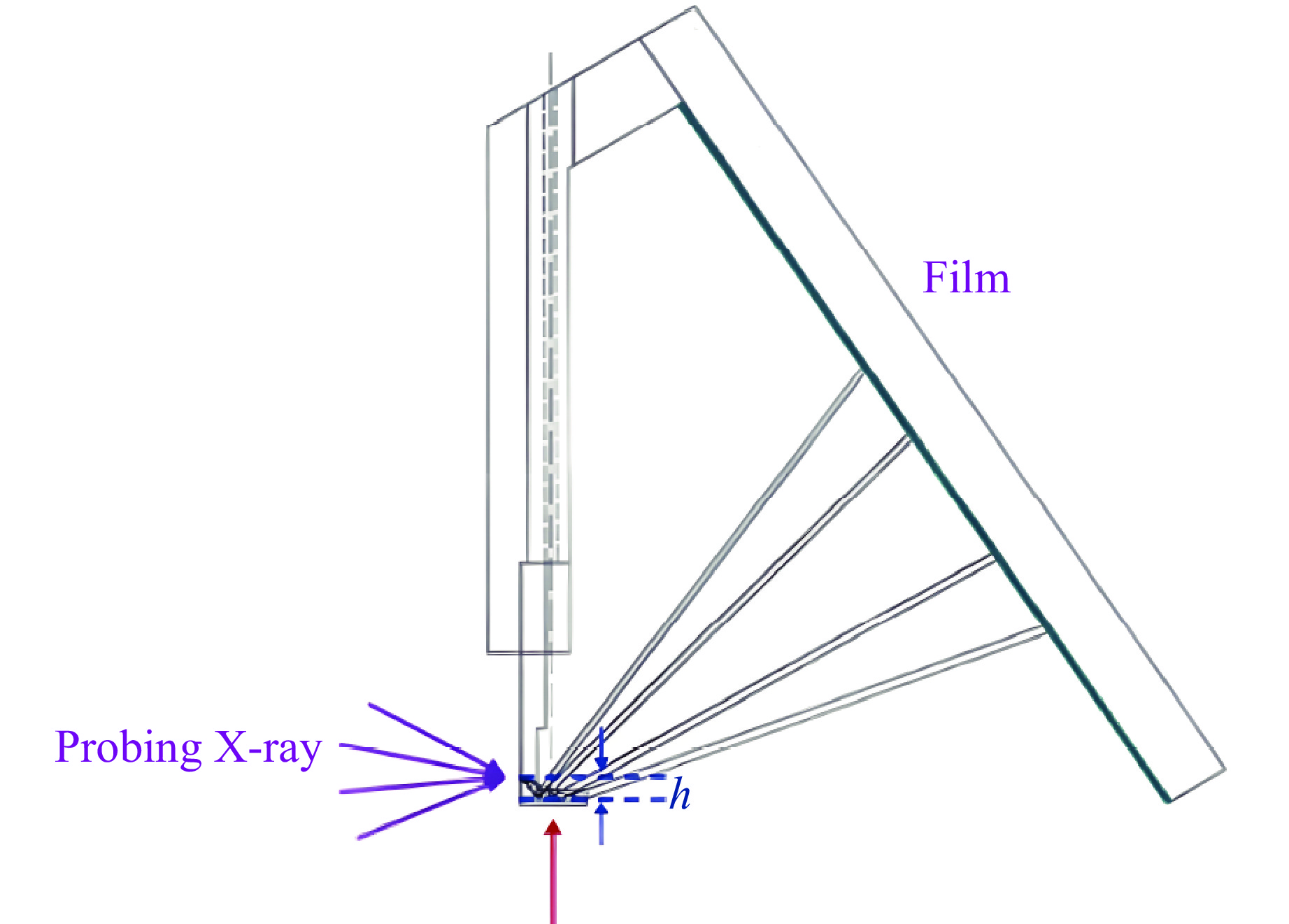
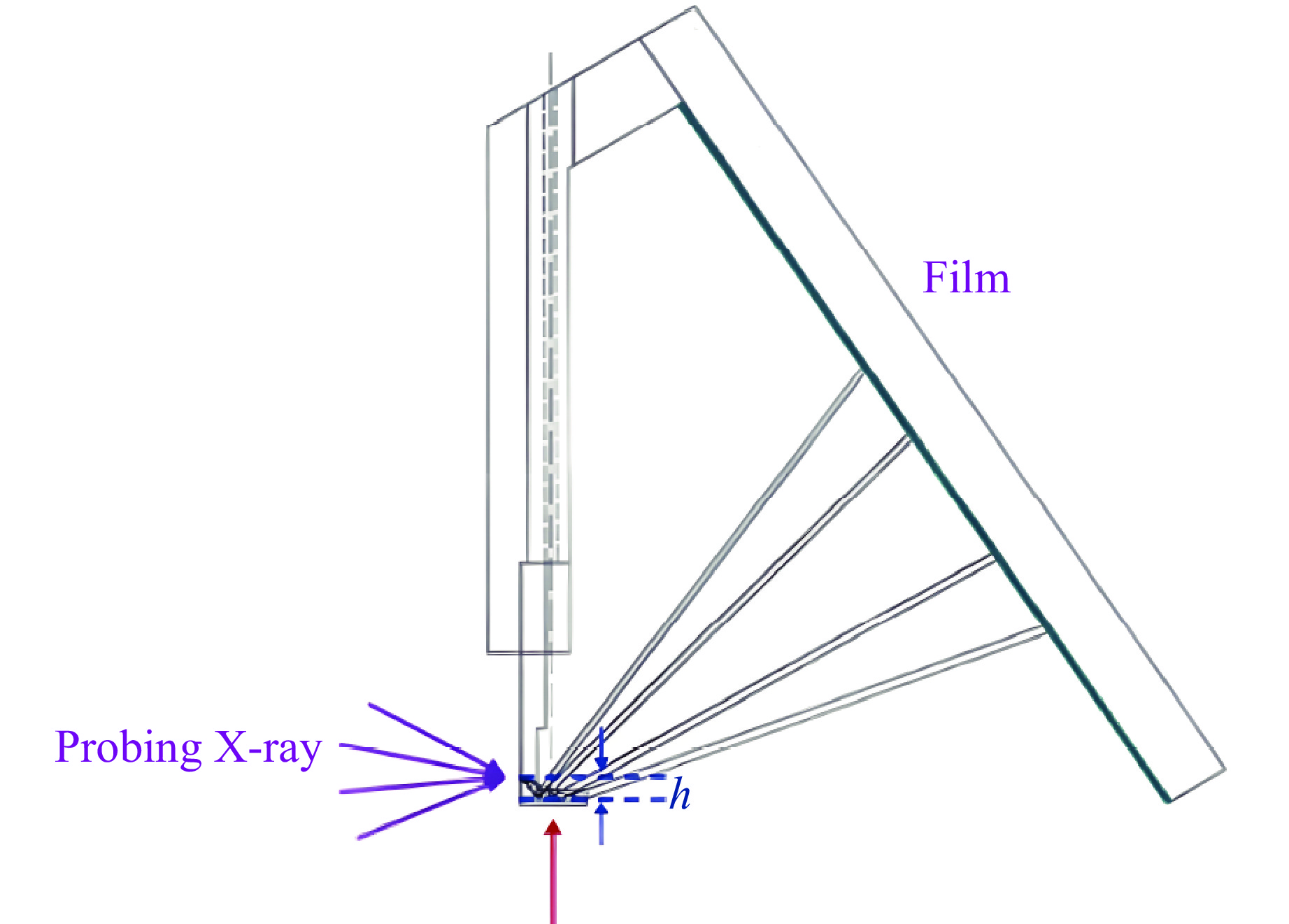
For single crystal XRD based on a laser-induced X-ray source, the X-ray source is divergent. The XRD projections obtained are called pseudo-Kossel projections, where the X-ray source is located at a distance from the crystal surface. As shown in Fig.1, the X-ray source is positioned at height of h above the surface of the sample. The divergent X-rays diagnose the sample and produce XRD peak lines on the film or image plate.

Figure  1.  Layout of single crystal XRD based on a laser-induced X-ray source

DownLoad: Full-Size Img PowerPoint

Kalantar et al.^[6] analyzed the XRD pattern of shock-compressed iron. They reported that the best-fit cone indicated a lattice spacing of 1.66 Å comparable to the actual spacing of 1.72 Å. The difference was higher than 4.0%. Hawreliak et al.^[11] analyzed single crystal experimental data through calculations using ray tracing. Lider et al.^[12] improved the X-ray divergent beam (Kossel) technique for analyzing XRD patterns, while Chen et al.^[13] employed the graphical method for the same purpose. These methods can handle numerous XRD peaks for one run. Lider et al.^[14] also described a method for precisely determining inter-plane distance in multiple surveys of X-ray diffraction. This method used the successive displacement of a photographic film by a strictly fixed inter-plane distance to improve the accuracy of crystal lattice parameters. However, this condition was not met in a single survey.

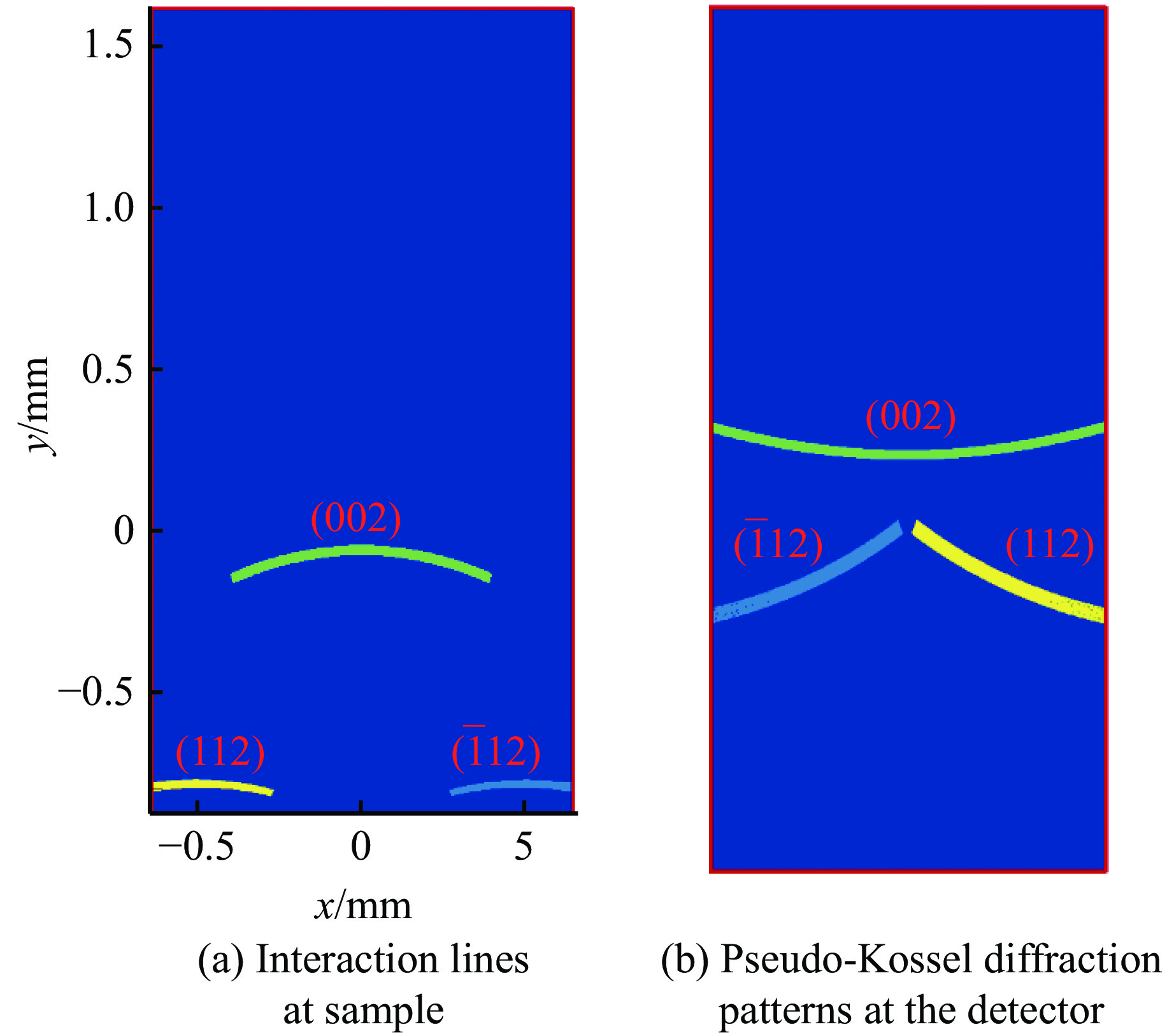
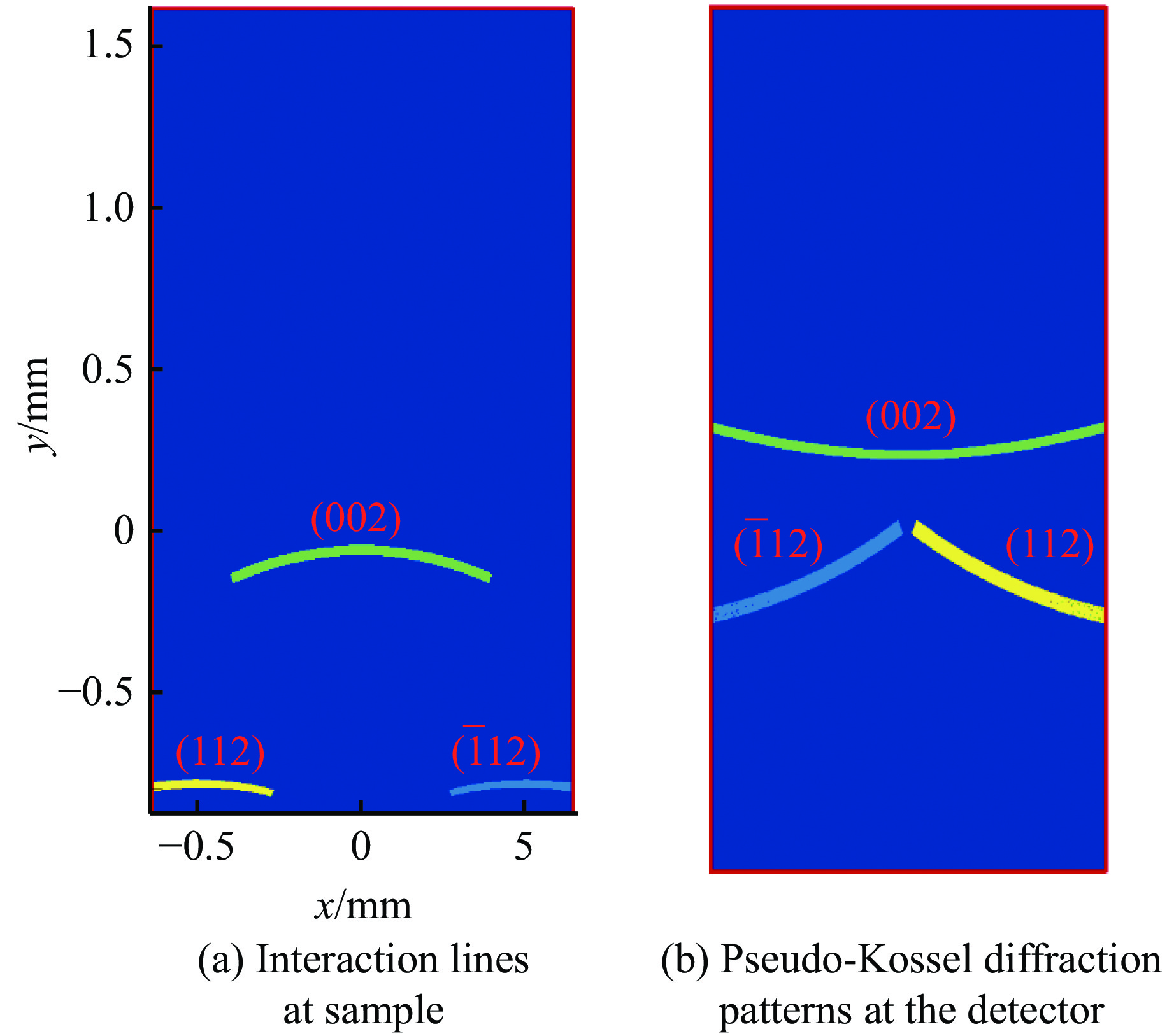
To date, the best method is the graphic method. However, it relies on an approximate hypothesis, assuming that the interaction of the sample occurs at the center of the sample with the coordinate (0, 0, 0). In other words, the Kossel pattern is used to approximate the pseudo-Kossel pattern. In contrast, interaction zones are not fixed points but several separately distributed lines on the sample (see Fig.2(a)). The X-ray beam diffracts at these different interaction lines, forming diffracted peak lines on the film plane with different magnifications (see Fig. 2(b)). In this paper, we propose a method to enhance the accuracy of lattice parameters by utilizing interaction lines on the crystal surface for the pseudo-Kossel pattern.

Figure  2.  Simulated results for the XRD of Fe single crystal (001)

DownLoad: Full-Size Img PowerPoint

1. Methodology

A diffracted peak line at the detected plane presents a Bragg angle and corresponds to a set of reflection planes. The coordinate of the diffracted peak line is satisfied with the Bragg-law

where (n_x, n_y, n_z) is the unit vector of the normal direction to the reflection plane, and (s_x, s_y, s_z) is the unit vector of the diffracted beam.

For the Kossel projection

The origin of the coordinate system is (0, 0, 0) at the crystal surface, which is assumed to be the center of the divergent X-ray at the sample.

For the pseudo-Kossel projection

where (x_i, y_i, z_i) are the coordinates of the interaction lines at the crystal surface. Additionally, the interaction line for the diffracted peak is also a curve.

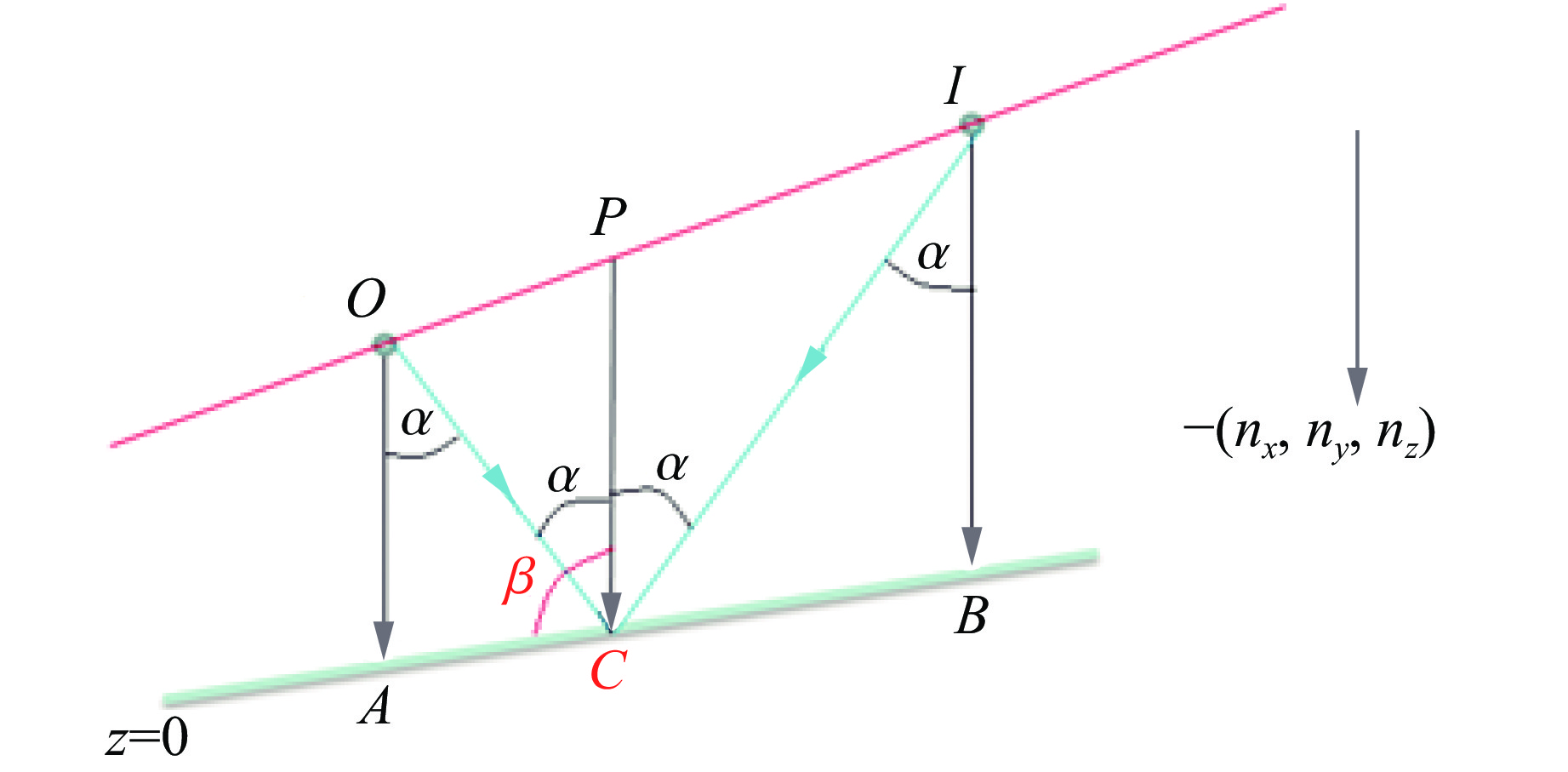
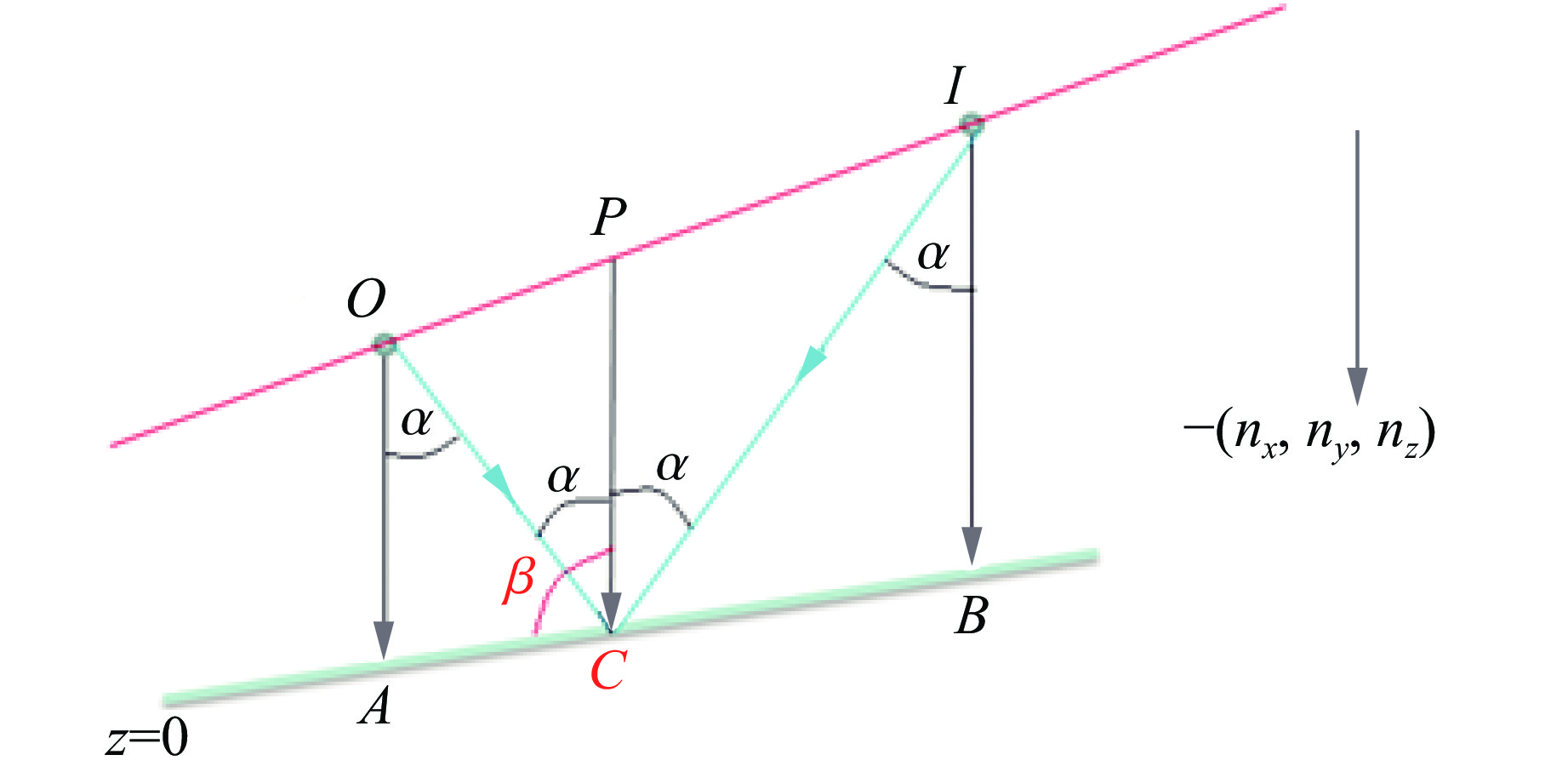
From the X-ray source points, O, and the image points, I, we can find a way to the interaction point, C, in Fig.3.

Figure  3.  Schematic showing the geometry for the relation between the source point, the interaction point, and the diffracted image point (The diffracted X-ray trace is depicted as a blue solid line from OC to CI.)

DownLoad: Full-Size Img PowerPoint

Firstly, a ray emanating from the source point O, with a direction vector (–n_x, –n_y, –n_z), intersects the plane z=0 at point A. Secondly, a ray emanating from the image point I, also with a direction vector (–n_x, –n_y, –n_z), intersects the plane z=0 at point B. Thirdly, the diffracted point at the crystal surface plane z=0 is marked as point C, which lies on the line AB and can be calculated using the following expression

where $\alpha = \text{π}/2 - \theta$, $\beta$ is the angle between the line CA and line CP, the direction cosine of CP is parallel to the normal to the crystallographic plane (reflection plane).

In practice, the direction of the normal to the crystallographic plane (reflection plane), denoted as (n_x, n_y, n_z), is unknown before the analysis. Therefore, we used the following iterative method to determine it step by step and simultaneously obtain the accurate lattice parameters of the pseudo-Kossel projection.

(1) Initial vector approximation: employ the Kossel projection condition (Eq. (3)) to calculate the preliminary direction vector (s_x, s_y, s_z); subsequently, determine the crystallographic normal vector (n_x, n_y, n_z) and the Bragg angle θ through nonlinear least-squares fitting of the Bragg law (Eq. (2)) to multiple diffraction positions in a single diffraction line recorded on the imaging plate.

(2) Interaction position refinement: reconstruct the interaction point set {C} based on the derived normal vector (n_x, n_y, n_z) and θ values for multiple points of the diffraction line on the imaging plate.

(3) Pseudo-Kossel projection optimization: implement the pseudo-Kossel formalism (Eq. (4)) to refine the source vector (s_x, s_y, s_z); recalculate (n_x, n_y, n_z) and θ by fitting the Bragg law (Eq. (2)) with multiple points of the diffraction line on the imaging plate.

(4) Iterative convergence: execute cyclical refinement by recursively returning to step (2) until the relative difference between consecutive θ values satisfies the convergence criterion.

2. Verification

2.1 Simulation

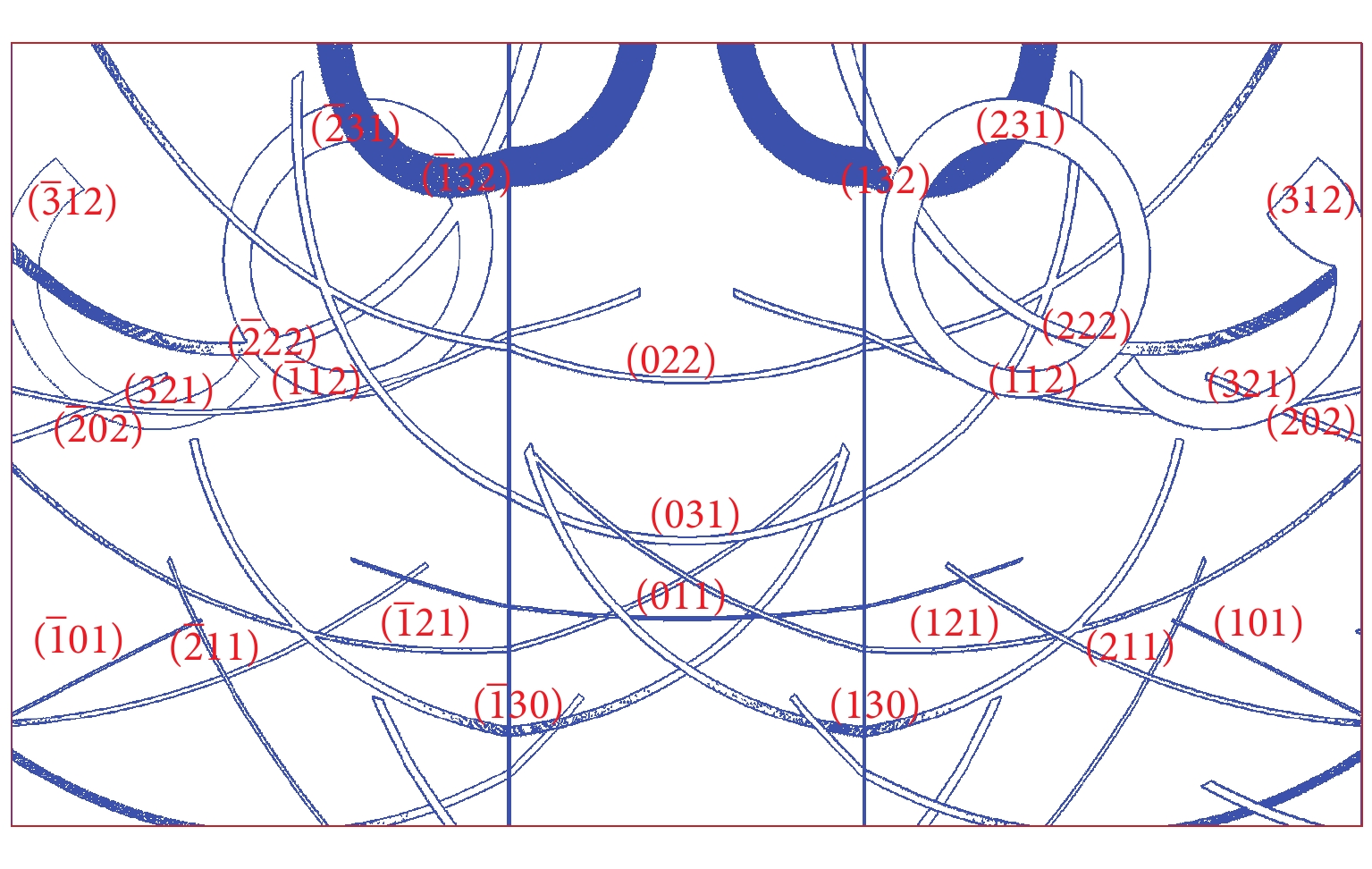
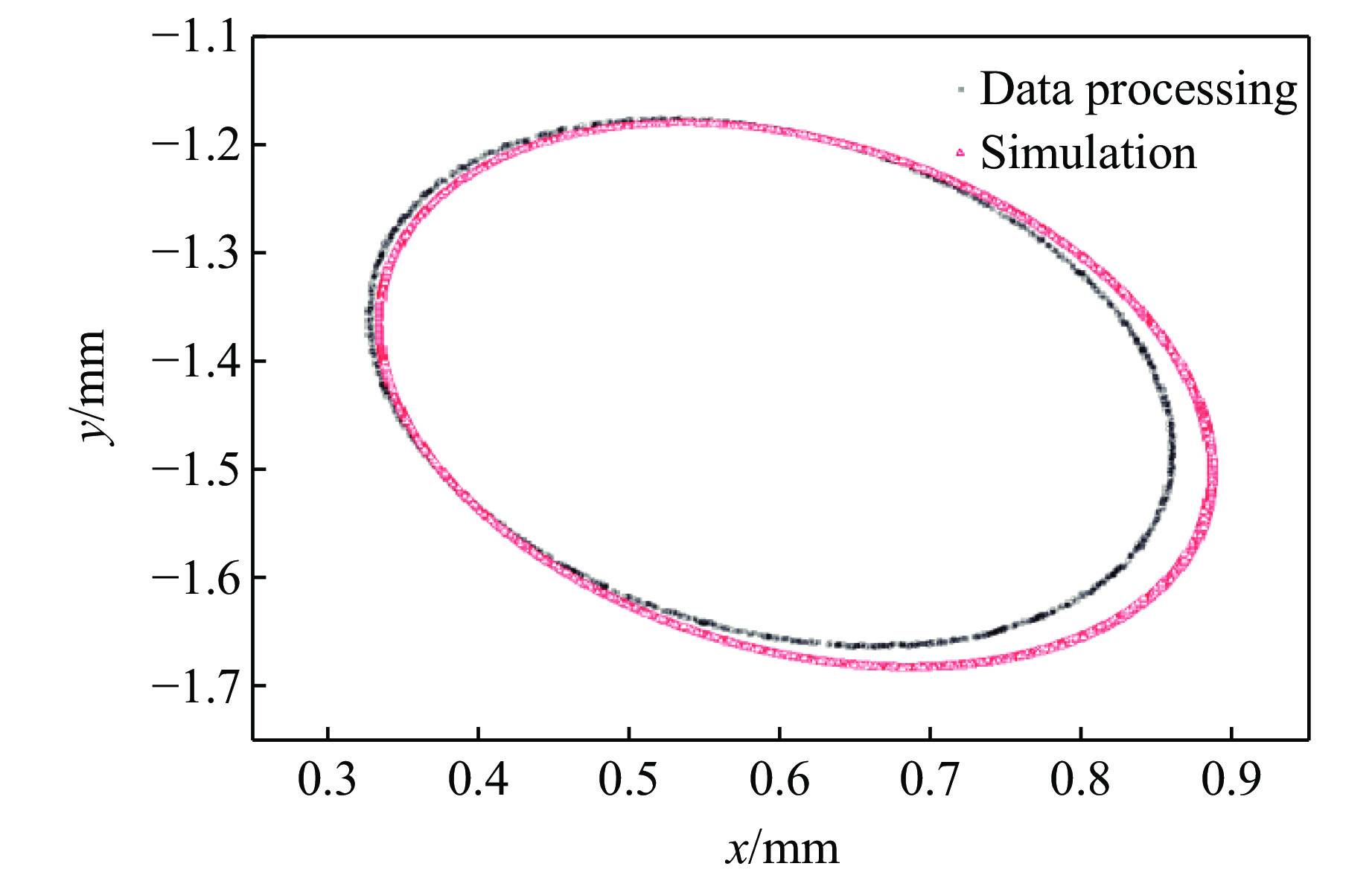
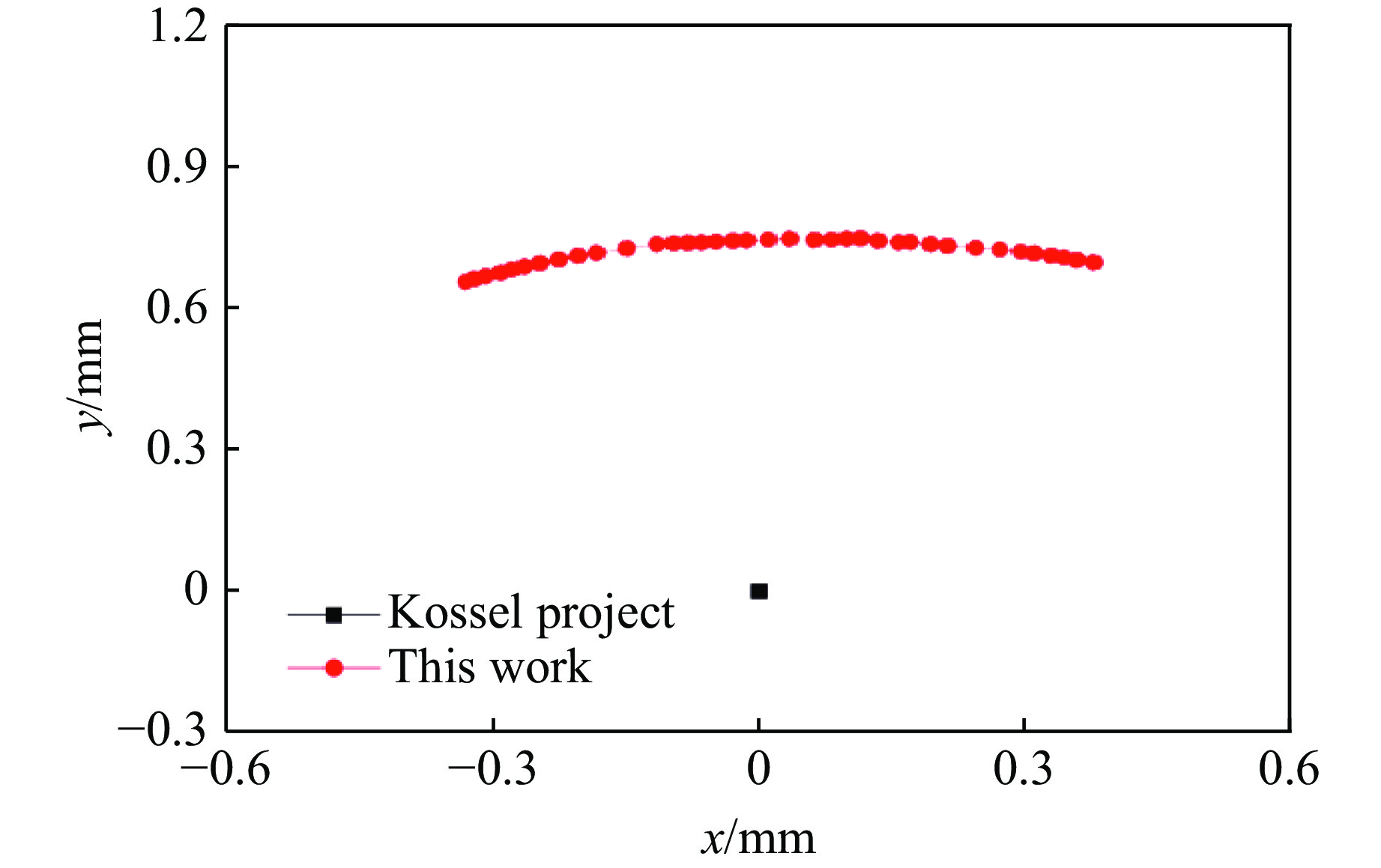
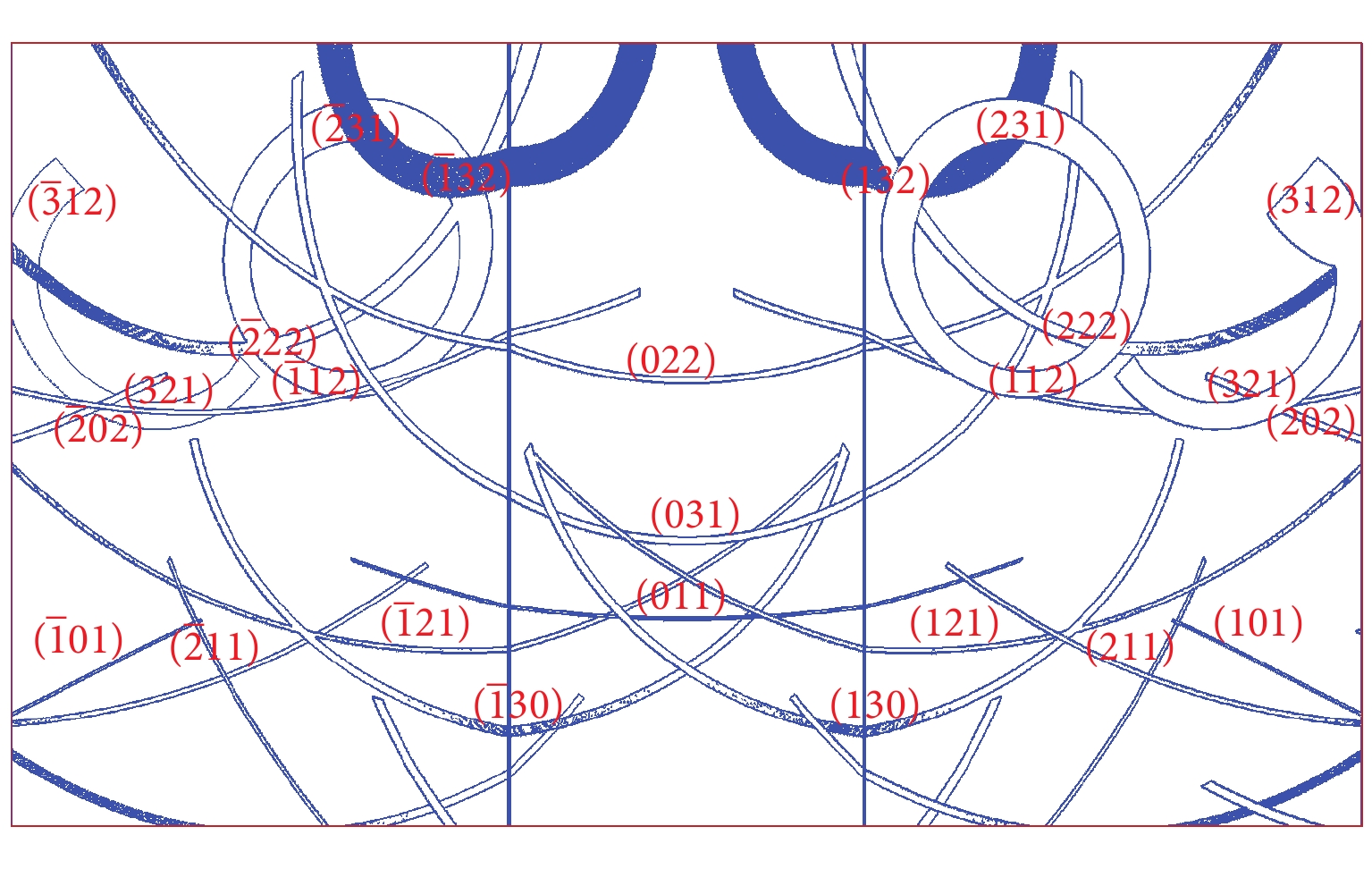
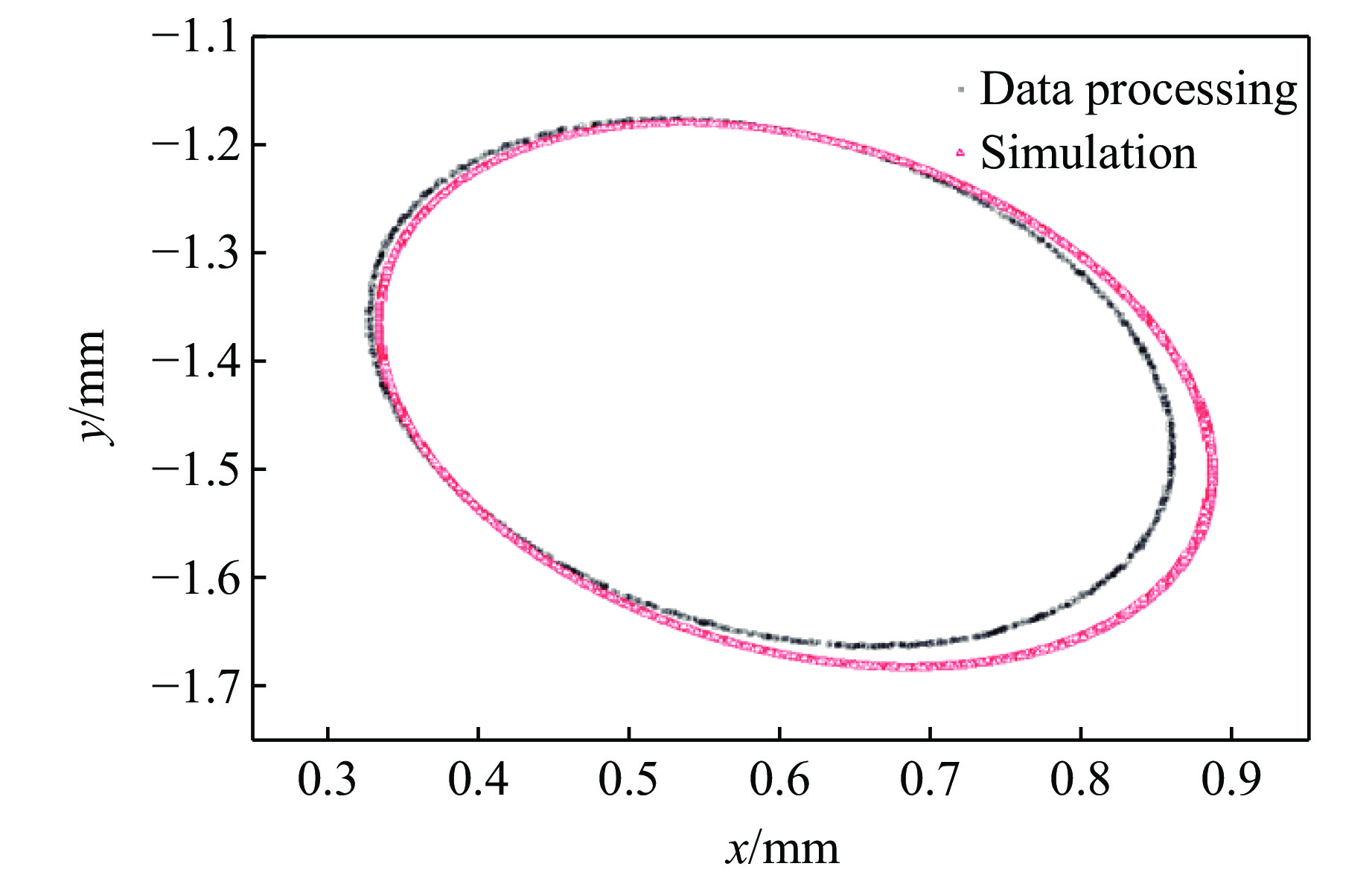
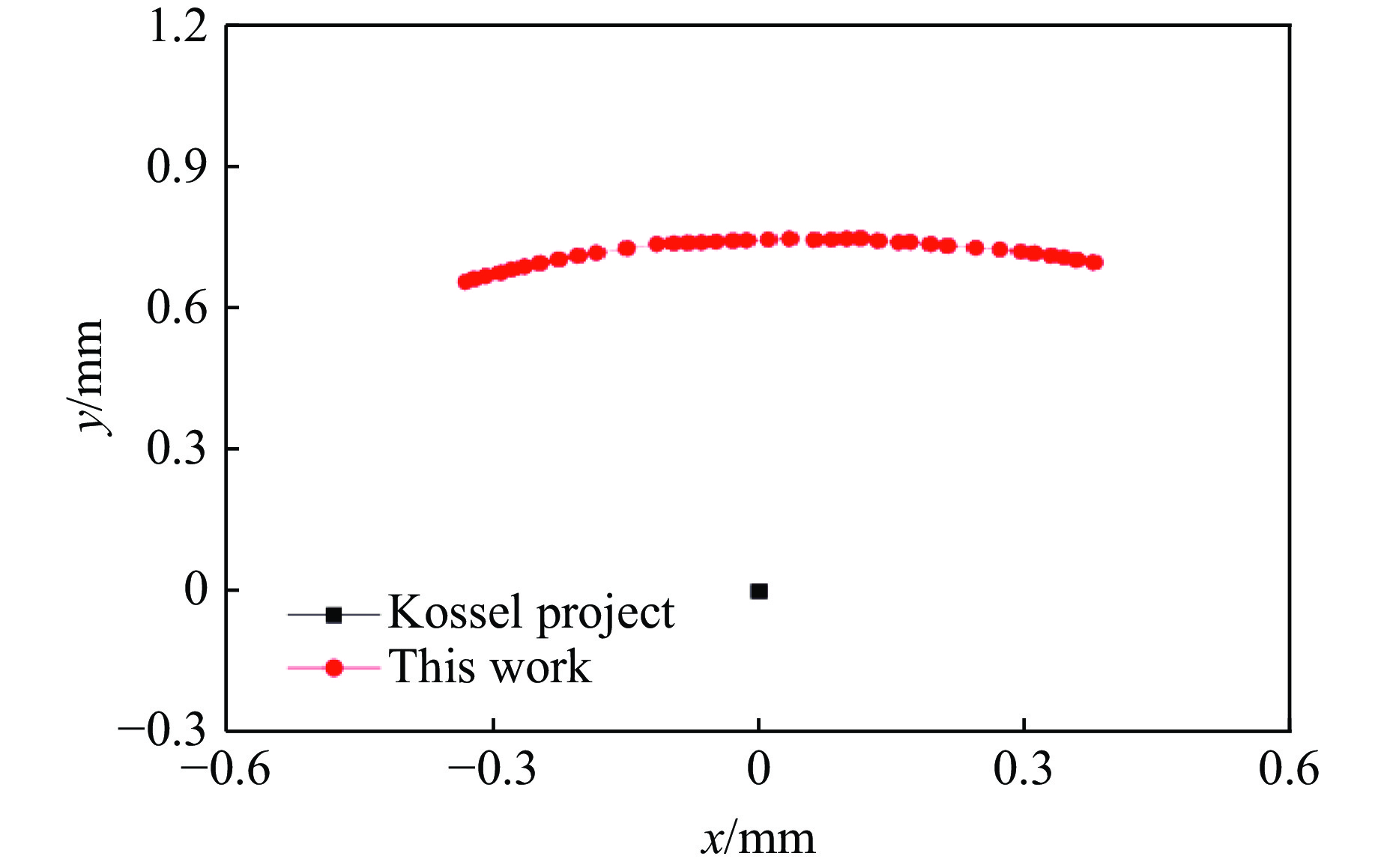
A simulation for the XRD experiment of the Fe (011) sample using copper target-based laser was worked out. The film detector was set for the central diffracted angle of 32.5° (Fig.4). The diffraction pattern was complex, even the diffraction line is similar to an oval, as shown in Fig.5. Using our method, we processed the diffraction pattern with the index (231). The interaction points obtained were plotted in Fig.6. Upon comparison, we found that the data-processed interaction points were closer to the true ones, as provided by forward simulation (red triangle points in Fig.6).

Figure  4.  Geometry of the large-angle film detector

DownLoad: Full-Size Img PowerPoint

Figure  5.  Diffraction pattern of Fe (011) sample using the copper target-based laser

DownLoad: Full-Size Img PowerPoint

Figure  6.  Interaction points for diffraction line (231) shown in Fig. 5

DownLoad: Full-Size Img PowerPoint

Additionally, we determined the plane direction through data processing. As shown in the Table 1, the direction of the plane converged towards the true value via iterations. The cosine of the angle between the solved direction and the true one exceeded 0.99993, and its angle is reduced to 0.68°. Therefore, we achieved the excellent results as expected.

Table  1.  Results of an iterative process for simulation pattern

Times	nx	ny	nz	sin θ	Error	Cosine	Angle of the cosine/(°)
True	−0.53452	0.37796	0.75593	0.97920
1	−0.52335	0.34581	0.77879	0.97826	0.000352	0.99915	2.36
2	−0.52711	0.37146	0.76432	0.97978	0.000154	0.99992	0.72
3	−0.52729	0.37272	0.76357	0.97985	0.000149	0.99993	0.68
Note: Cosine is the cosine value of the true unit vector (nx, ny, nz) of the normal direction to the reflection plane and the analyzed one.

 | Show Table

DownLoad: CSV

2.2 Static Experiment

The coordinates of the diffraction line constitute raw data for XRD data analysis. To minimize the error in these coordinates, we built an axis system of origin at the sample center for the XRD experiment according to the layout depicted in Fig.1. Then we expanded the image plates shown in Fig.4 in a plane and developed a standardized template (Fig.7). The standardized template features mapped three-dimensional coordinate values which is consistent with the real space coordinates of the image plate in Fig.4.

Figure  7.  Standardized template for quantifying diffraction line coordinates

DownLoad: Full-Size Img PowerPoint

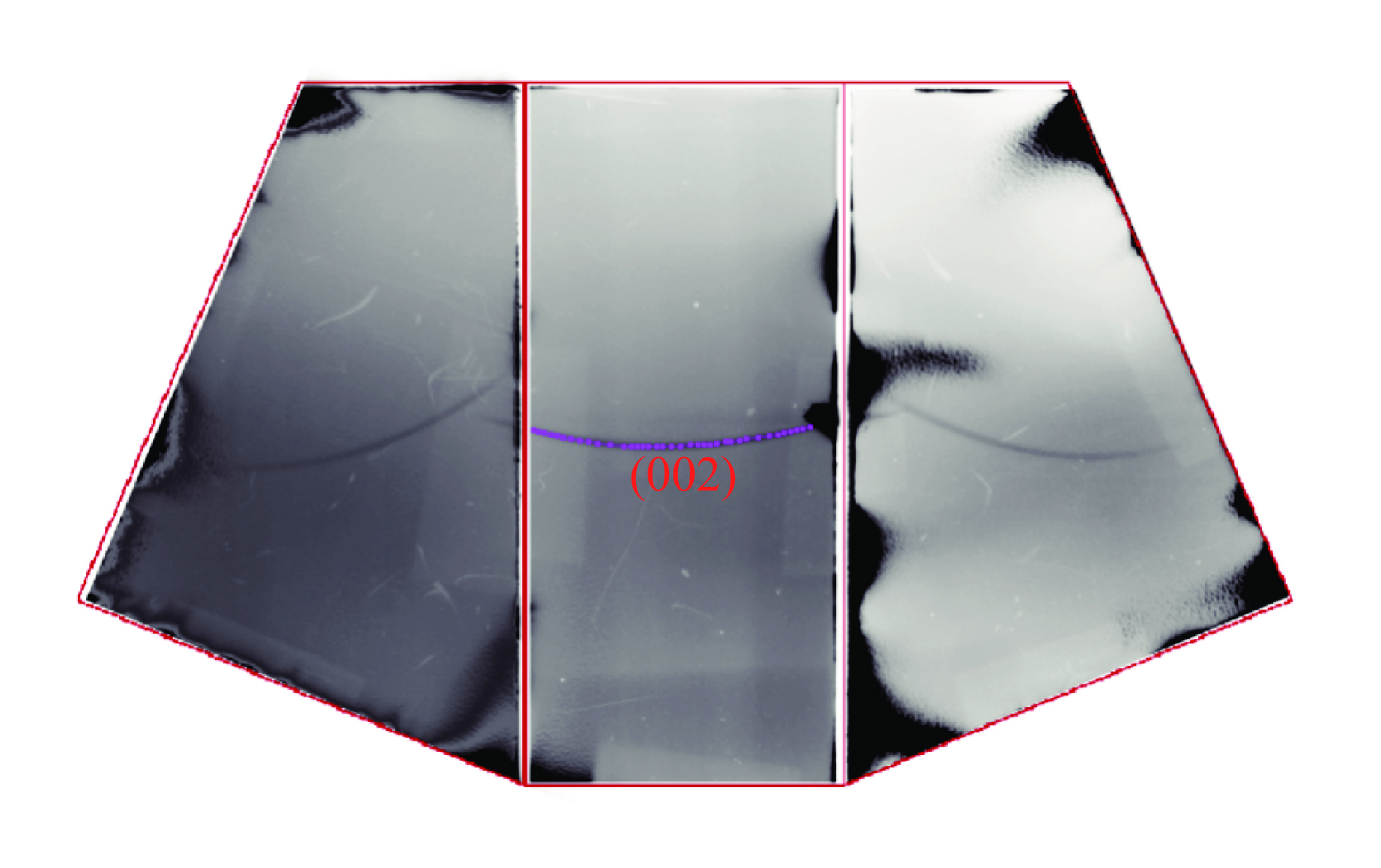
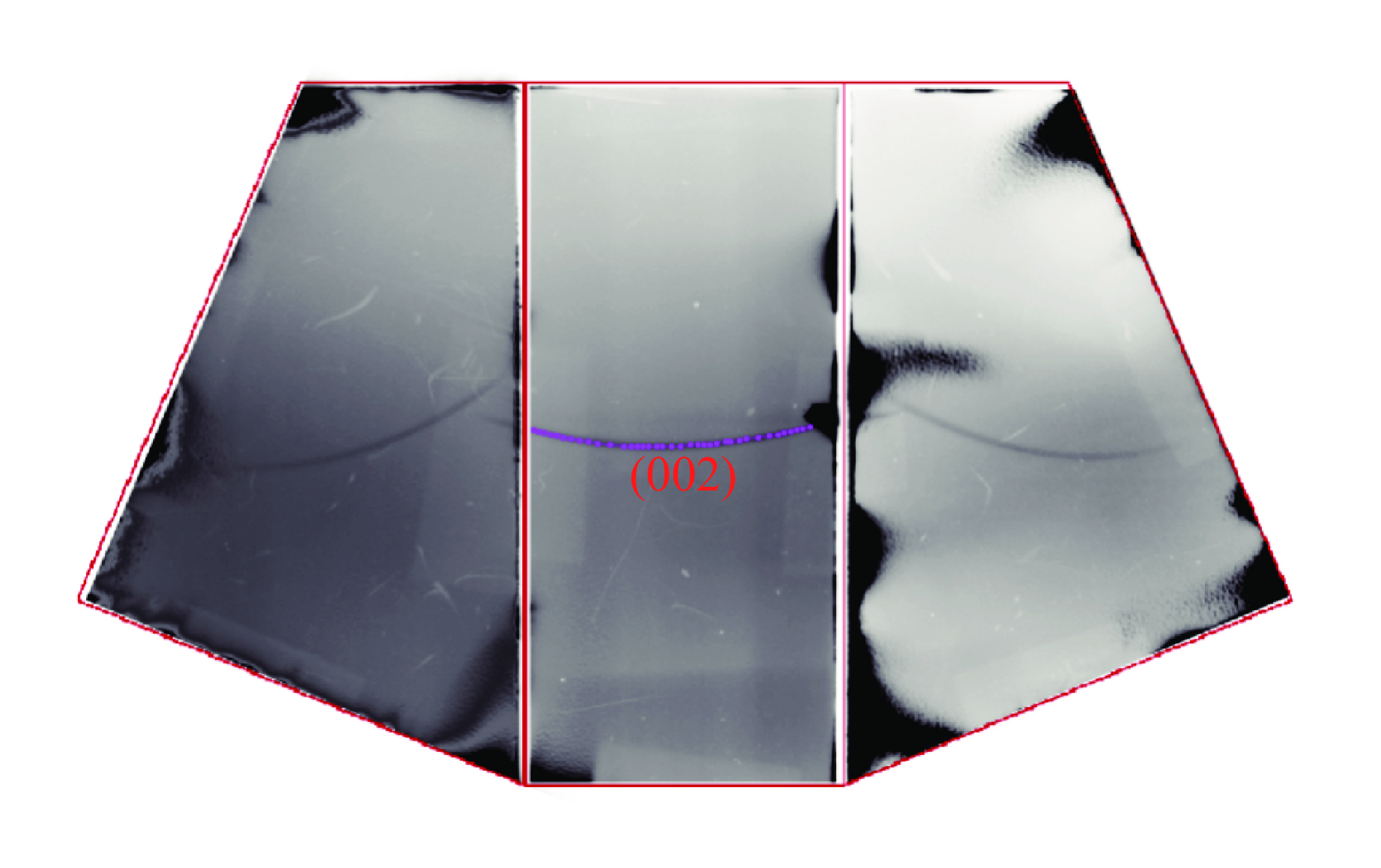
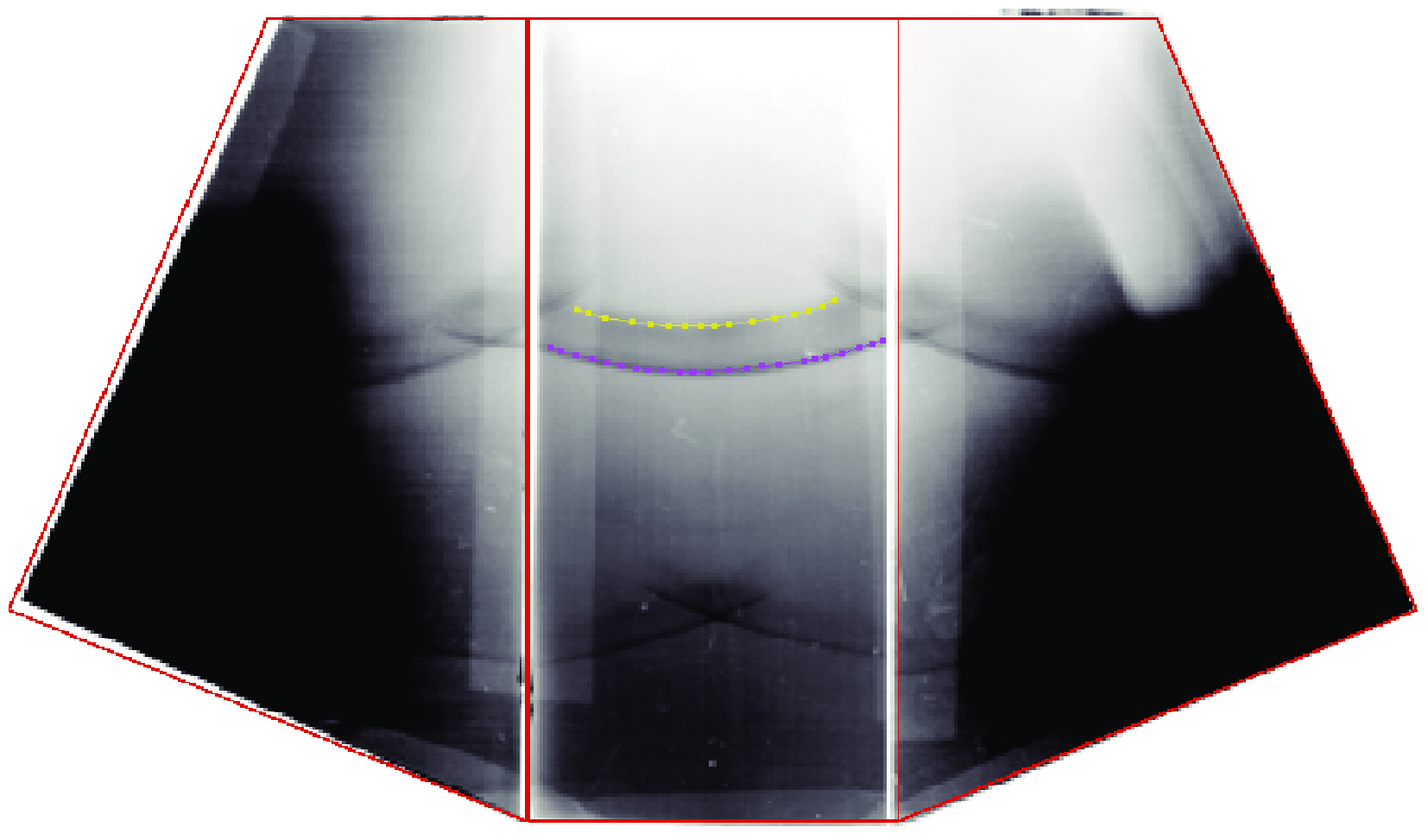
The experiment on iron single crystals was conducted using a film detector (Fig.4) at the SG-Ⅱ laser facility^[4]. The sample, Fe (001), was rotated 45° around the z-axis. The diffraction pattern, matched to the standardized template, is shown in Fig.8. The index of the diffraction line on the middle plane is (002). By applying the proposed method to analyze the diffraction line on the middle plane, we obtained the lattice parameters (Table 2). The first iteration results corresponded to the Kossel projection condition, with a deviation of approximately 1.76% from true value of 0.655. After tens of iterations, the results significantly improved, reducing the difference to about 0.35%. Meanwhile, we determined the interaction line between the X-ray beams and sample. As shown in Fig.9, the interaction points are not an individual point but a curve. By refining the interaction points, we achieved more accurate lattice parameters. Consequently, the proposed method enhances the accuracy of the lattice parameters, which is crucial for reducing uncertainty and enhancing data utility.

Figure  8.  Standardized static diffracted pattern on film detector

DownLoad: Full-Size Img PowerPoint

Table  2.  Results of the iterative method

Times	sin θ	Difference/%
1	0.6435	1.76
50	0.6523	0.35

 | Show Table

DownLoad: CSV

Figure  9.  Interaction points identified using different methods for the diffraction line on the middle film detector in Fig.8

DownLoad: Full-Size Img PowerPoint

3. Application

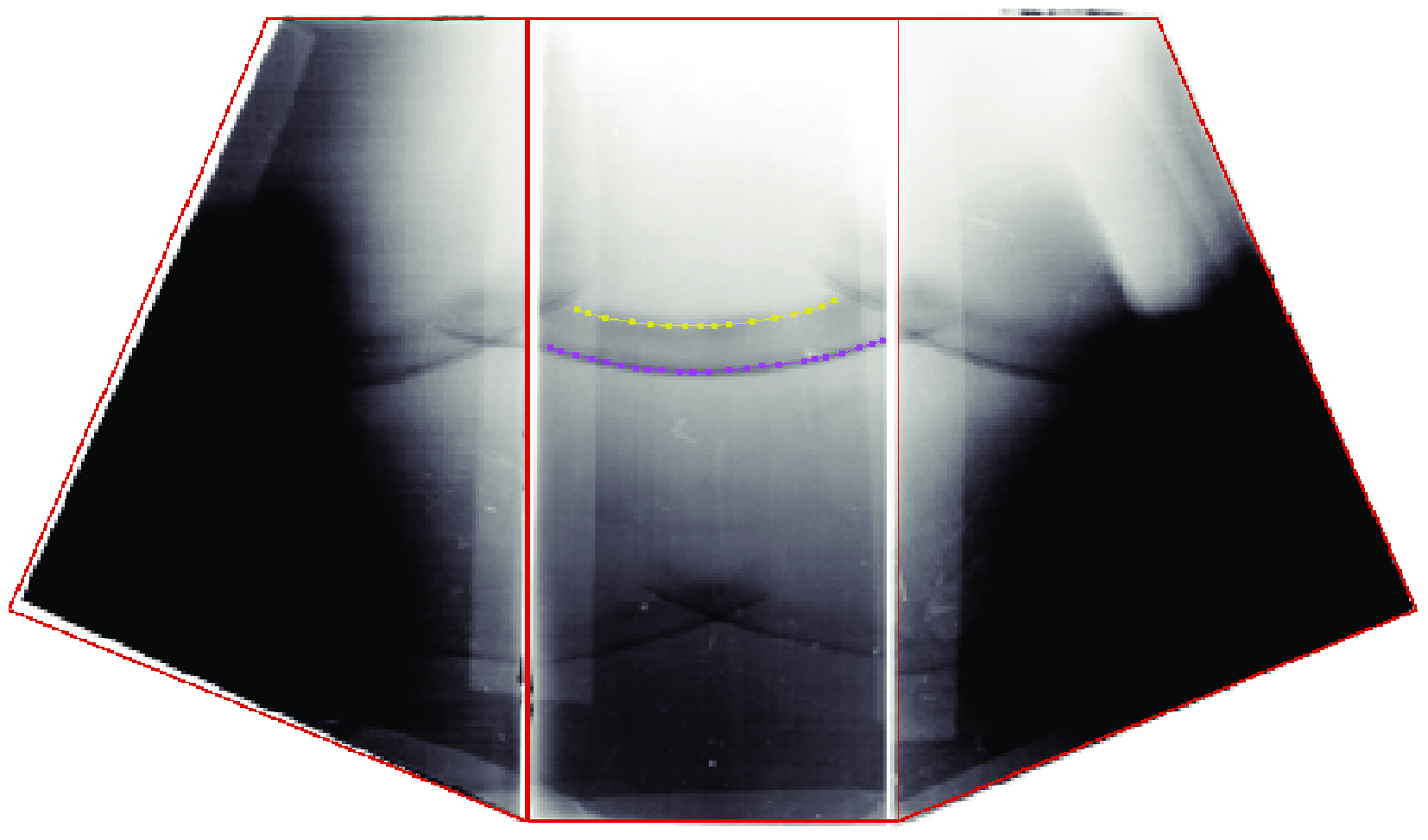
The diffraction pattern obtained from the LiF sample, oriented close to the [001] axis as the laser loading direction, is depicted in Fig.10. At a pressure of about 20 GPa, the dynamic diffraction line is far away from the static line. Using the iterative method proposed above, we calculated the sine values of the diffraction for the dynamic yellow dotted line, which is 0.8062, and for the static red dotted line, which is 0.7262. The compression ratio is 9.92%. Additionally, the two lines are not parallel, indicating that there is a rotation of the sample. From the solved normal directions of the diffraction planes for both the dynamic and static diffraction lines, we found that the angle of rotation is about 2.84°.

Figure  10.  Diffraction pattern of the (100) LiF sample at about 20 GPa (Static state: red dotted line; compressed state: yellow dotted line)

DownLoad: Full-Size Img PowerPoint

4. Conclusion and Discussion

We used an iterative method to locate the interaction points on the crystal surface, thereby enhancing the accuracy of the lattice parameters for the pseudo-Kossel pattern, a classic layout for the single crystal XRD utilizing laser technology. The accuracy was improved from 1.76% to 0.35%. Furthermore, we applied the iterative method to analyze the dynamic diffraction of (001) LiF sample. At a pressure of approximately 20 GPa, we obtained a compression ratio of about 9.92% and found the angle of rotation is about 2.84°.

Currently, we have extracted information about the crystal from the data processing, especially the lattice parameters. Next, we plane to employ this method to analyze more complex experiments and try to elucidate the mechanism of the dynamic process with a forward simulation program.