碳酸钾改性干水-六氟丙烷抑制甲烷爆炸特性

王涛; 孟帆; 弋伟斋; 田晓月; 李睿康; 苏彬; 刘利涛; 罗振敏

doi:10.11858/gywlxb.20240927

碳酸钾改性干水-六氟丙烷抑制甲烷爆炸特性

doi: 10.11858/gywlxb.20240927

西安科技大学安全科学与工程学院, 陕西西安　710054

基金项目: 国家自然科学基金（52004208，52304252）

详细信息

作者简介:
王　涛（1988－），男，博士，教授，主要从事工业燃爆灾害防控研究. E-mail：christfer@xust.edu.cn

中图分类号: O389; O521.9; X932
计量
- 文章访问数: 28
- HTML全文浏览量: 13
- PDF下载量: 8
出版历程
- 收稿日期: 2024-11-03
- 修回日期: 2024-11-29
- 录用日期: 2025-01-14
- 网络出版日期: 2025-03-18
- 刊出日期: 2025-04-05

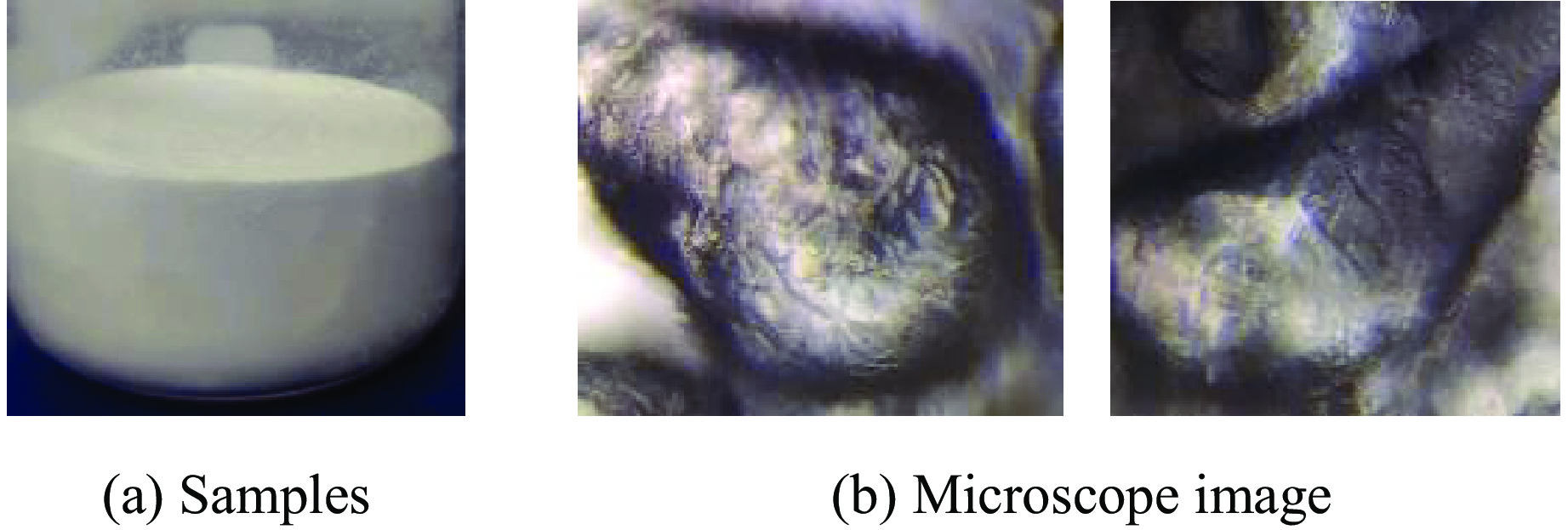
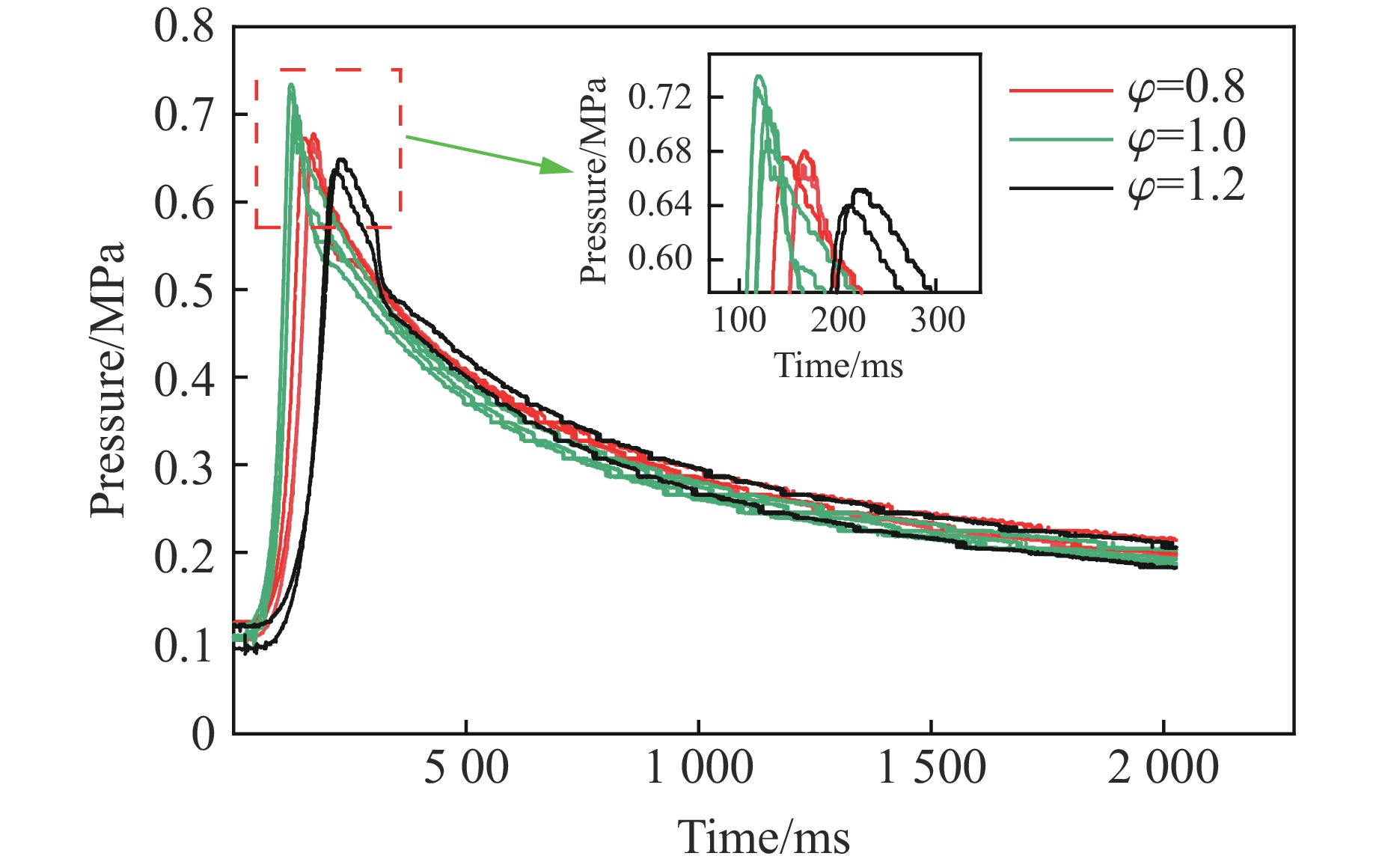
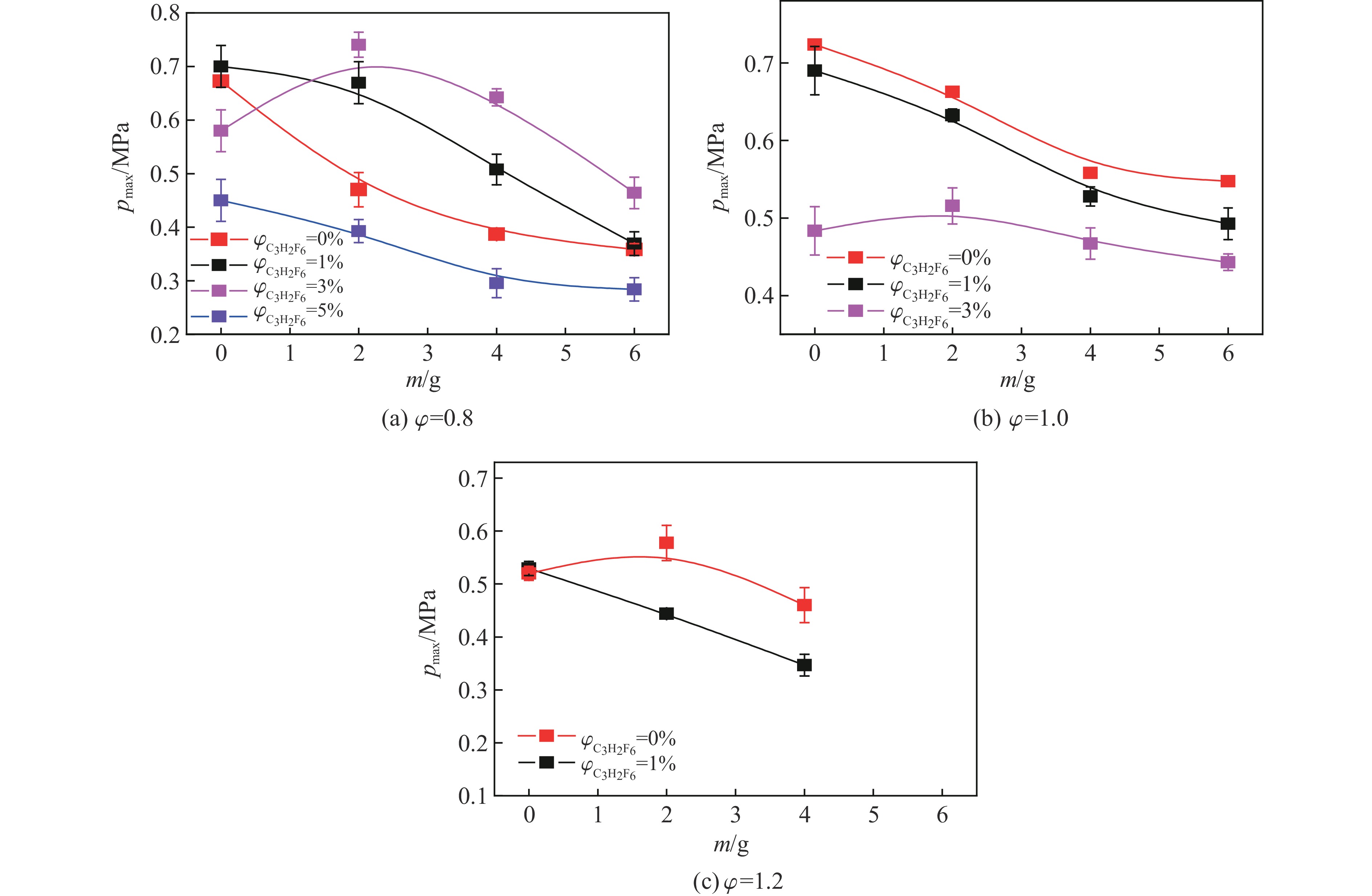
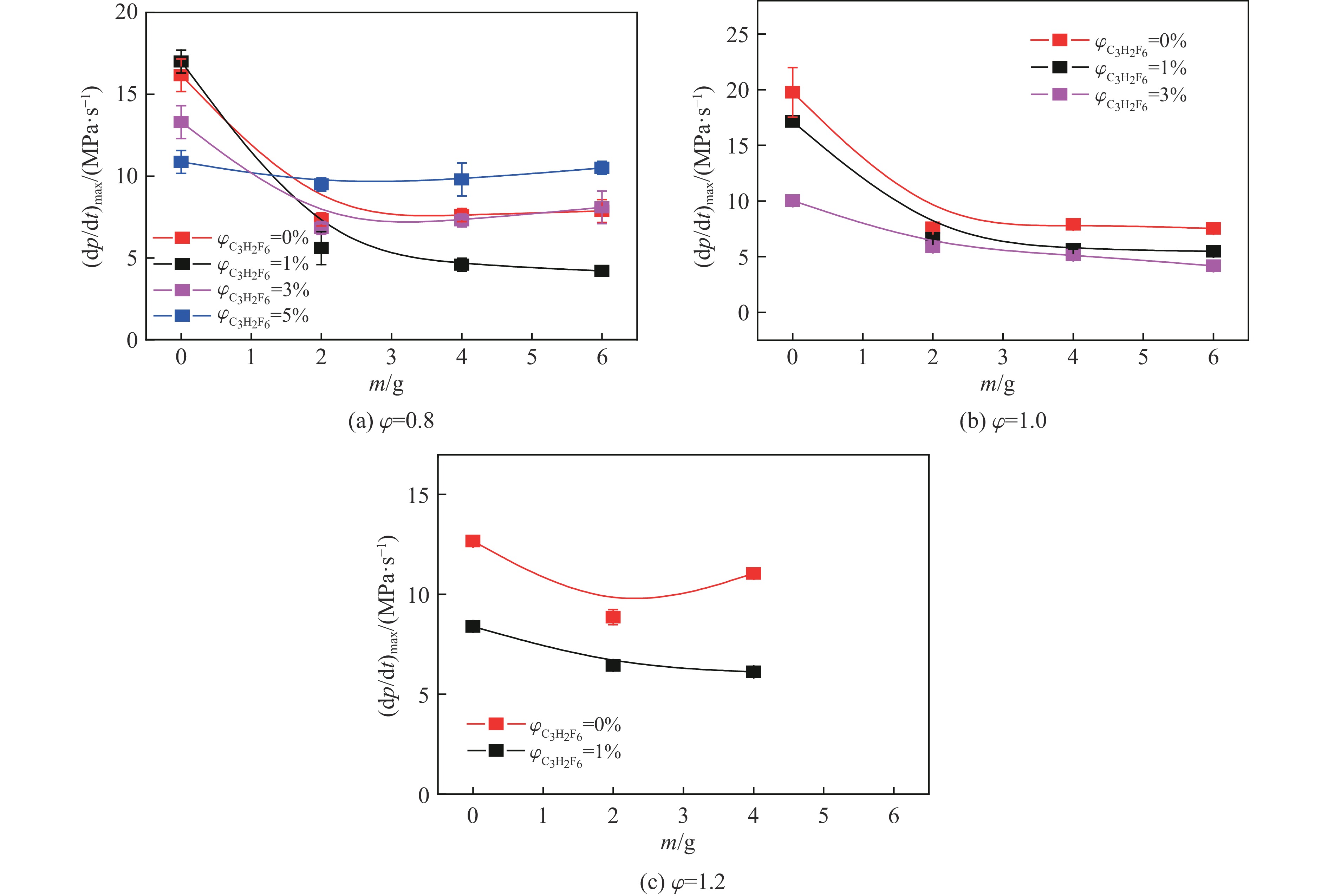
Coupling Inhibition Effects of Dry Water Modified by Potassium Carbonate and Hexafluoropropane on Methane Explosion

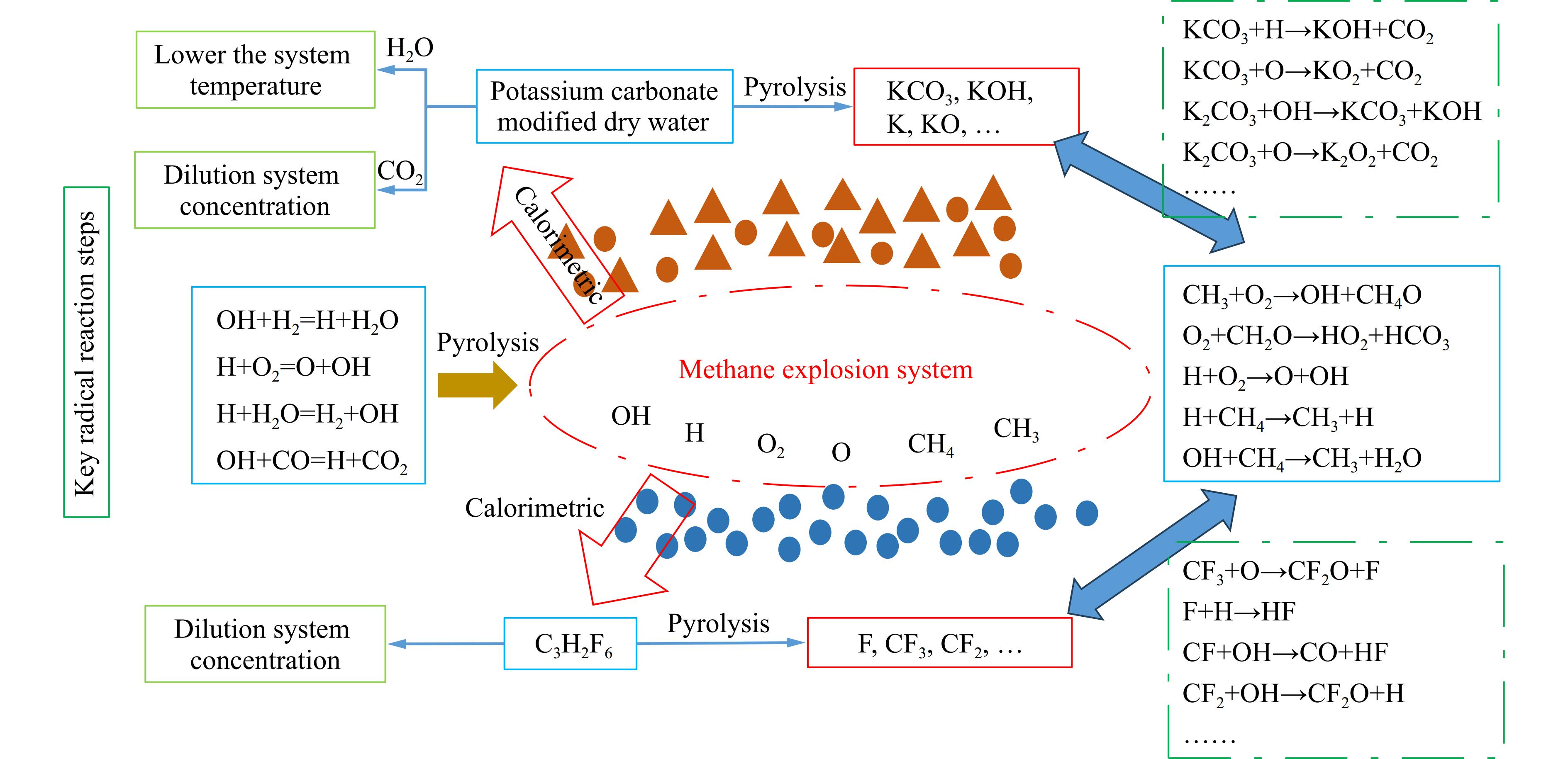
School of Safety Science and Engineering, Xi’an University of Science and Technology, Xi’an 710054, Shaanxi, China

摘要

摘要: 爆炸抑制技术是减轻瓦斯爆炸事故灾后影响的重要手段。为探究两相复合抑爆剂的抑制效果，选取碳酸钾改性干水粉体和六氟丙烷（C₃H₂F₆）气体为抑爆介质，通过试验研究了二者复配影响下甲烷爆炸压力和时间参数的变化规律，并对其协同抑爆机理开展了理论分析。试验结果表明，在富燃工况下，甲烷爆炸的快速燃爆时间和持续燃烧时间随着碳酸钾改性干水和C₃H₂F₆配比的增加而增加，碳酸钾改性干水大幅提升了C₃H₂F₆的抑制效果。贫燃、化学当量比和富燃工况下，气-固两相抑制剂的临界抑爆配比分别为5%-6 g、3%-6 g、1%-4 g。理论分析结果显示：复配抑爆剂对甲烷爆炸的物理抑制作用表现为稀释可燃物浓度、降低反应体系温度和稀释氧浓度；化学抑制作用方面，碳酸钾和C₃H₂F₆热解产生的KCO₃、KOH、OH和含氟基团降低了甲烷爆炸链式反应产生的关键自由基浓度。研究结果可为清洁抑爆材料及相应抑爆技术的研发提供理论依据。
- 甲烷爆炸 /
- 改性干水 /
- 六氟丙烷 /
- 爆炸特性 /
- 抑制机理
Abstract: Explosion suppression technology plays a vital role in reducing the hazardous effect of gas explosion incidents. This study aimed to investigate the explosion suppression effect of two-phase composite inhibitor mixtures of hexafluoropropane and dry water modified by potassium carbonate. The explosion pressure and time parameters of methane-air mixtures were obtained experimentally. Then the synergistic mechanisms on methane explosion suppression was analyzed theoretically. Results of the experiments shows that the combustion time of methane-air mixtures increase with the rising ratio of dry water modified by potassium carbonate in the coupled inhibitors. Dry water modified by potassium carbonate greatly enhanced the explosion suppression effect of C₃H₂F₆. The critical inhibition ratios of gas-solid inhibitors are 5%-6 g, 3%-6 g, and 1%-4 g for fuel-lean, stoichiometric, and fuel-rich methane-air mixtures, respectively. Moreover, the physical inhibition effects of the dilution in the premixed mixtures and the reduction in the flame temperature, as well as the chemical suppression effect, synergistically inhibit the deflagration of methane-air mixtures. In terms of the chemical inhibition, it is KCO₃, KOH, OH and fluorine-containing groups that produced by the pyrolysis of potassium carbonate and C₃H₂F₆ reduce the concentration of key radicals of methane explosion. The results of the work will help to providing the theoretical basis for the development of more effective explosion-suppressant and promoting the related explosion-suppressing technology.
- methane explosion /
- modified dry water /
- hexafluoropropane /
- explosion characteristics /
- inhibition mechanism

HTML全文

Understanding the atomic-scale behaviors of materials under dynamic loading conditions is crucial. This understanding helps us comprehend their complex responses at high strain rates and has implications across various research fields, such as material science, geophysics, and defense industries. One prevalent method for this investigation is dynamic X-ray diffraction (XRD). Dynamic XRD utilize pulsed X-ray sources to analyze the crystal structures of materials undergoing dynamic compression or decompression^[1]. A laser with a single pulse energy greater than 100 J can produce X-rays strong enough for diagnostic dynamic XRD experiments. Typical laser facilities used for this purpose include Omega^[2], NIF (National Ignition Facility)^[3], SG-Ⅱ^[4–5] , et al. Compared with third-generation light sources, the laser-generated X-ray light sources can serve as wide-angle light sources and are more suitable for diagnosing single crystal samples. Several researchers have utilized dynamic XRD in their studies. Kalantar et al.^[6–7] employed XRD to investigate shocked crystals and observe the α-ε transition in shock-compressed iron at Omega facility^[2]. Li et al.^[8] used XRD to diagnose the dynamic loading of an iron sample at the SG-Ⅱ facility. Rygg et al.^[9] conducted XRD on a powder sample at the TARDIS experimental platform of the NIF facility.

For powder sample XRD, the process based on laser-induced X-rays is the same as that used with third-generation X-ray facilities. There are several programs available for data-processing in XRD experiments, such as SHELX^[10]. Rygg et al.^[9] considered the thickness of the sample for Laue diffraction to improve the accuracy of the lattice parameters to a level of 0.4% at the TARDIS experimental platform of NIF. However, for single crystal XRD using divergent X-rays, the direction of the incident X-ray is not known. This makes the data processing more difficult than it is for powder XRD, where the direction of X-ray is known.

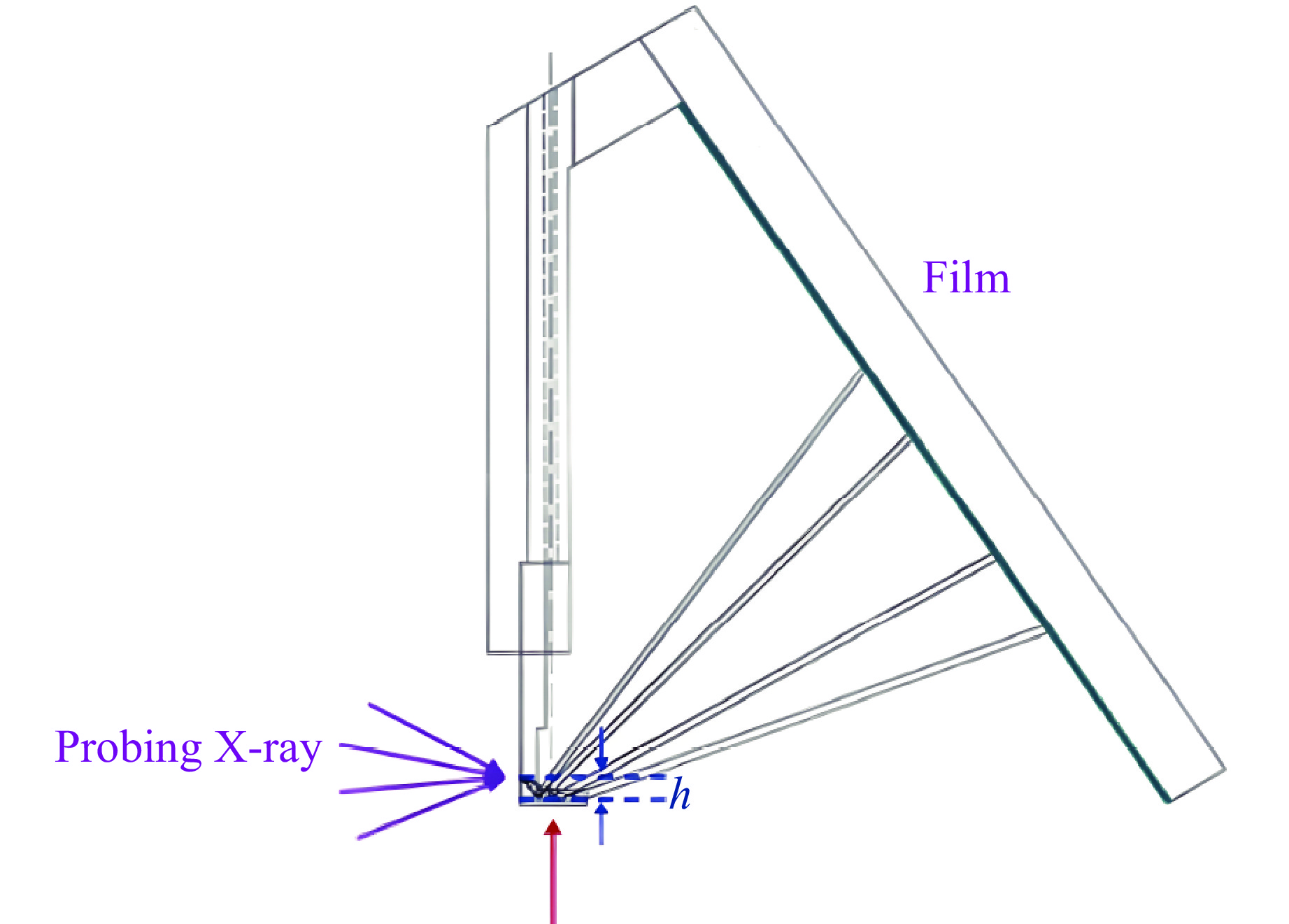
For single crystal XRD based on a laser-induced X-ray source, the X-ray source is divergent. The XRD projections obtained are called pseudo-Kossel projections, where the X-ray source is located at a distance from the crystal surface. As shown in Fig.1, the X-ray source is positioned at height of h above the surface of the sample. The divergent X-rays diagnose the sample and produce XRD peak lines on the film or image plate.

Figure 1. Layout of single crystal XRD based on a laser-induced X-ray source

DownLoad: Full-Size Img PowerPoint

Kalantar et al.^[6] analyzed the XRD pattern of shock-compressed iron. They reported that the best-fit cone indicated a lattice spacing of 1.66 Å comparable to the actual spacing of 1.72 Å. The difference was higher than 4.0%. Hawreliak et al.^[11] analyzed single crystal experimental data through calculations using ray tracing. Lider et al.^[12] improved the X-ray divergent beam (Kossel) technique for analyzing XRD patterns, while Chen et al.^[13] employed the graphical method for the same purpose. These methods can handle numerous XRD peaks for one run. Lider et al.^[14] also described a method for precisely determining inter-plane distance in multiple surveys of X-ray diffraction. This method used the successive displacement of a photographic film by a strictly fixed inter-plane distance to improve the accuracy of crystal lattice parameters. However, this condition was not met in a single survey.

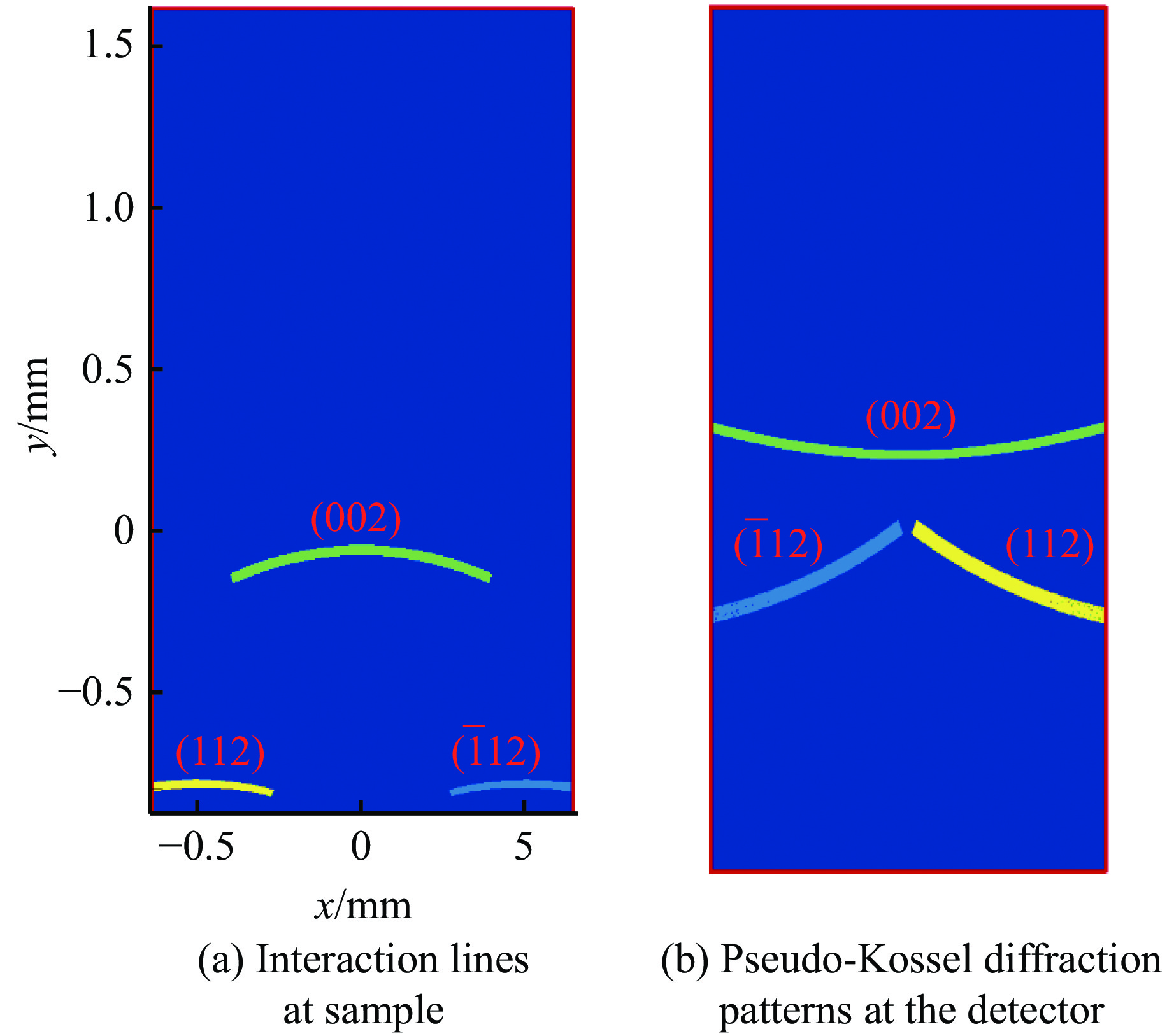
To date, the best method is the graphic method. However, it relies on an approximate hypothesis, assuming that the interaction of the sample occurs at the center of the sample with the coordinate (0, 0, 0). In other words, the Kossel pattern is used to approximate the pseudo-Kossel pattern. In contrast, interaction zones are not fixed points but several separately distributed lines on the sample (see Fig.2(a)). The X-ray beam diffracts at these different interaction lines, forming diffracted peak lines on the film plane with different magnifications (see Fig. 2(b)). In this paper, we propose a method to enhance the accuracy of lattice parameters by utilizing interaction lines on the crystal surface for the pseudo-Kossel pattern.

Figure 2. Simulated results for the XRD of Fe single crystal (001)

DownLoad: Full-Size Img PowerPoint

1. Methodology

A diffracted peak line at the detected plane presents a Bragg angle and corresponds to a set of reflection planes. The coordinate of the diffracted peak line is satisfied with the Bragg-law

$n\lambda = 2d \sin \theta$

(1)

$\sin \theta = {n_x}{s_x}+{n_y}{s_y}+{n_{\textit{z}}}{s_{\textit{z}}}$

(2)

where (n_x, n_y, n_z) is the unit vector of the normal direction to the reflection plane, and (s_x, s_y, s_z) is the unit vector of the diffracted beam.

For the Kossel projection

${s_x} = x/r,\;\;\;\;\;\;{\text{ }}{s_y} = y/r,\;\;\;\;\;\;{\text{ }}{s_{\textit{z}}} = \textit{z}/r,\;\;\;\;\;\;r = \sqrt {{x^2}+{y^2}+{{\textit{z}}^2}}$

(3)

The origin of the coordinate system is (0, 0, 0) at the crystal surface, which is assumed to be the center of the divergent X-ray at the sample.

For the pseudo-Kossel projection

${s_x} = x/r,\;\;\;\;\;\;{\text{ }}{s_y} = y/r,\;\;\;\;\;\;{\text{ }}{s_{\textit{z}}} = \textit{z}/r,\;\;\;\;\;\; \\ r = \sqrt {{{\left( {x - {x_{i} }} \right)}^2}+{{\left( {y - {y_i}} \right)}^2}+{{\left( {{\textit{z}} - {{\textit{z}}_i}} \right)}^2}}$

(4)

where (x_i, y_i, z_i) are the coordinates of the interaction lines at the crystal surface. Additionally, the interaction line for the diffracted peak is also a curve.

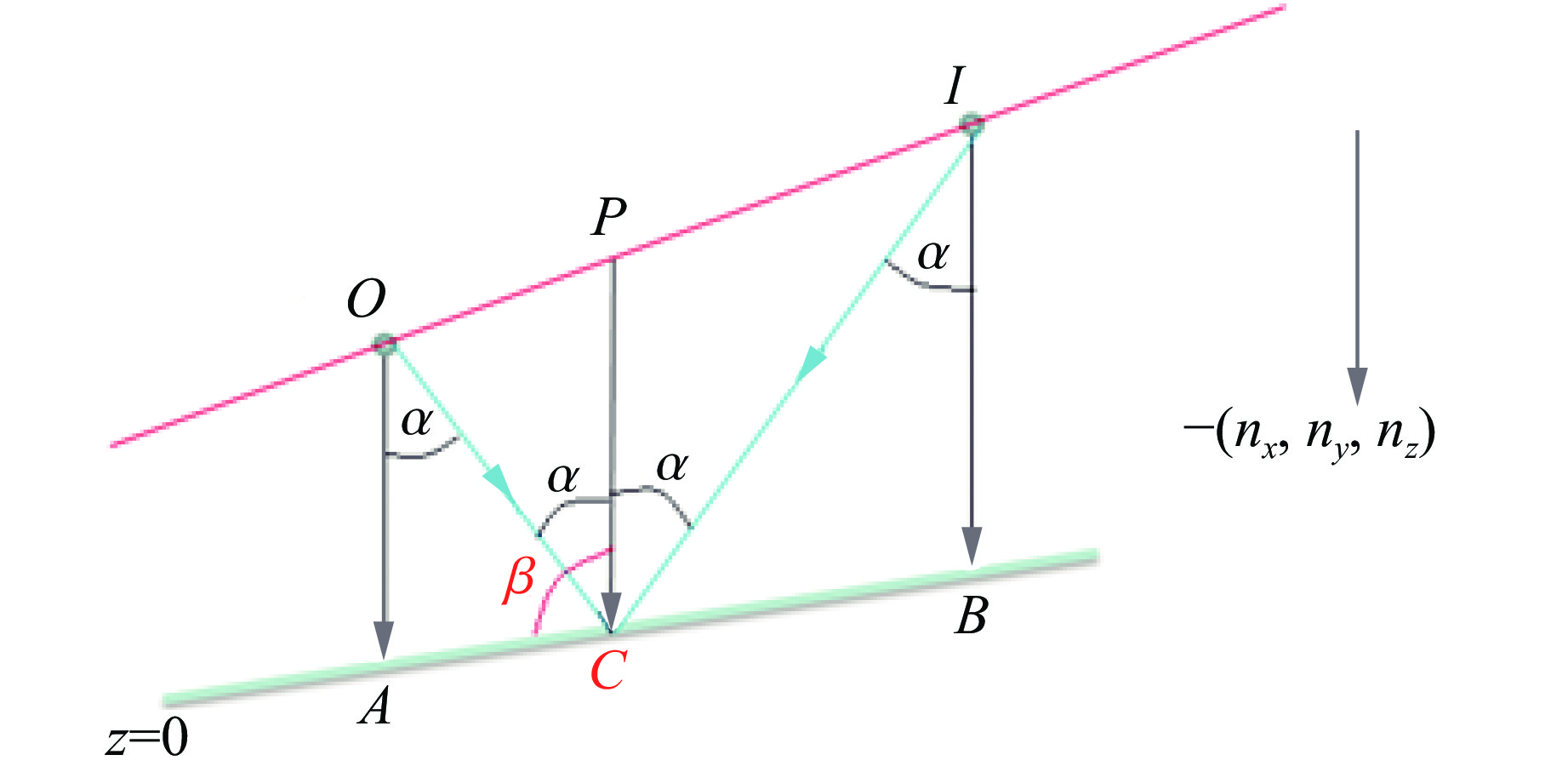
From the X-ray source points, O, and the image points, I, we can find a way to the interaction point, C, in Fig.3.

Figure 3. Schematic showing the geometry for the relation between the source point, the interaction point, and the diffracted image point (The diffracted X-ray trace is depicted as a blue solid line from OC to CI.)

DownLoad: Full-Size Img PowerPoint

Firstly, a ray emanating from the source point O, with a direction vector (–n_x, –n_y, –n_z), intersects the plane z=0 at point A. Secondly, a ray emanating from the image point I, also with a direction vector (–n_x, –n_y, –n_z), intersects the plane z=0 at point B. Thirdly, the diffracted point at the crystal surface plane z=0 is marked as point C, which lies on the line AB and can be calculated using the following expression

$\left\{ \begin{array}{l} \dfrac{{{l_{AC}}}}{{\sin\, \alpha }} = \dfrac{{{l_{OA}}}}{{\sin (\beta - \alpha )}} \\ \dfrac{{{l_{BC}}}}{{\sin\, \alpha }} = \dfrac{{{l_{IB}}}}{{\sin (\text{π} - \beta - \alpha )}} \\ \cos\, \beta = \overrightarrow {BA} \cdot \overrightarrow {AO} \\ \end{array}\right.$

(5)

where $\alpha = \text{π}/2 - \theta$ , $\beta$ is the angle between the line CA and line CP, the direction cosine of CP is parallel to the normal to the crystallographic plane (reflection plane).

In practice, the direction of the normal to the crystallographic plane (reflection plane), denoted as (n_x, n_y, n_z), is unknown before the analysis. Therefore, we used the following iterative method to determine it step by step and simultaneously obtain the accurate lattice parameters of the pseudo-Kossel projection.

(1) Initial vector approximation: employ the Kossel projection condition (Eq. (3)) to calculate the preliminary direction vector (s_x, s_y, s_z); subsequently, determine the crystallographic normal vector (n_x, n_y, n_z) and the Bragg angle θ through nonlinear least-squares fitting of the Bragg law (Eq. (2)) to multiple diffraction positions in a single diffraction line recorded on the imaging plate.

(2) Interaction position refinement: reconstruct the interaction point set {C} based on the derived normal vector (n_x, n_y, n_z) and θ values for multiple points of the diffraction line on the imaging plate.

(3) Pseudo-Kossel projection optimization: implement the pseudo-Kossel formalism (Eq. (4)) to refine the source vector (s_x, s_y, s_z); recalculate (n_x, n_y, n_z) and θ by fitting the Bragg law (Eq. (2)) with multiple points of the diffraction line on the imaging plate.

(4) Iterative convergence: execute cyclical refinement by recursively returning to step (2) until the relative difference between consecutive θ values satisfies the convergence criterion.

2. Verification

2.1 Simulation

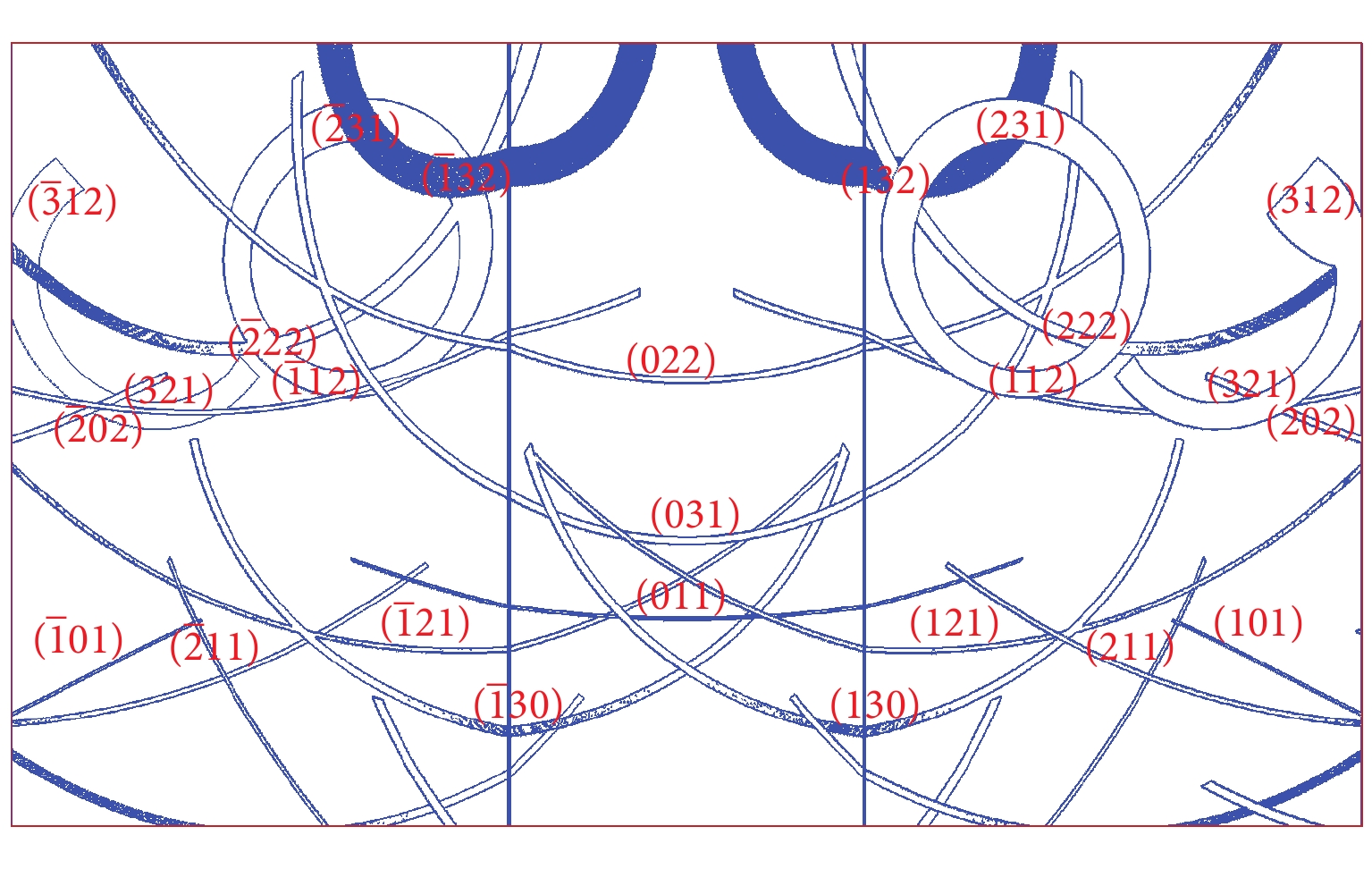
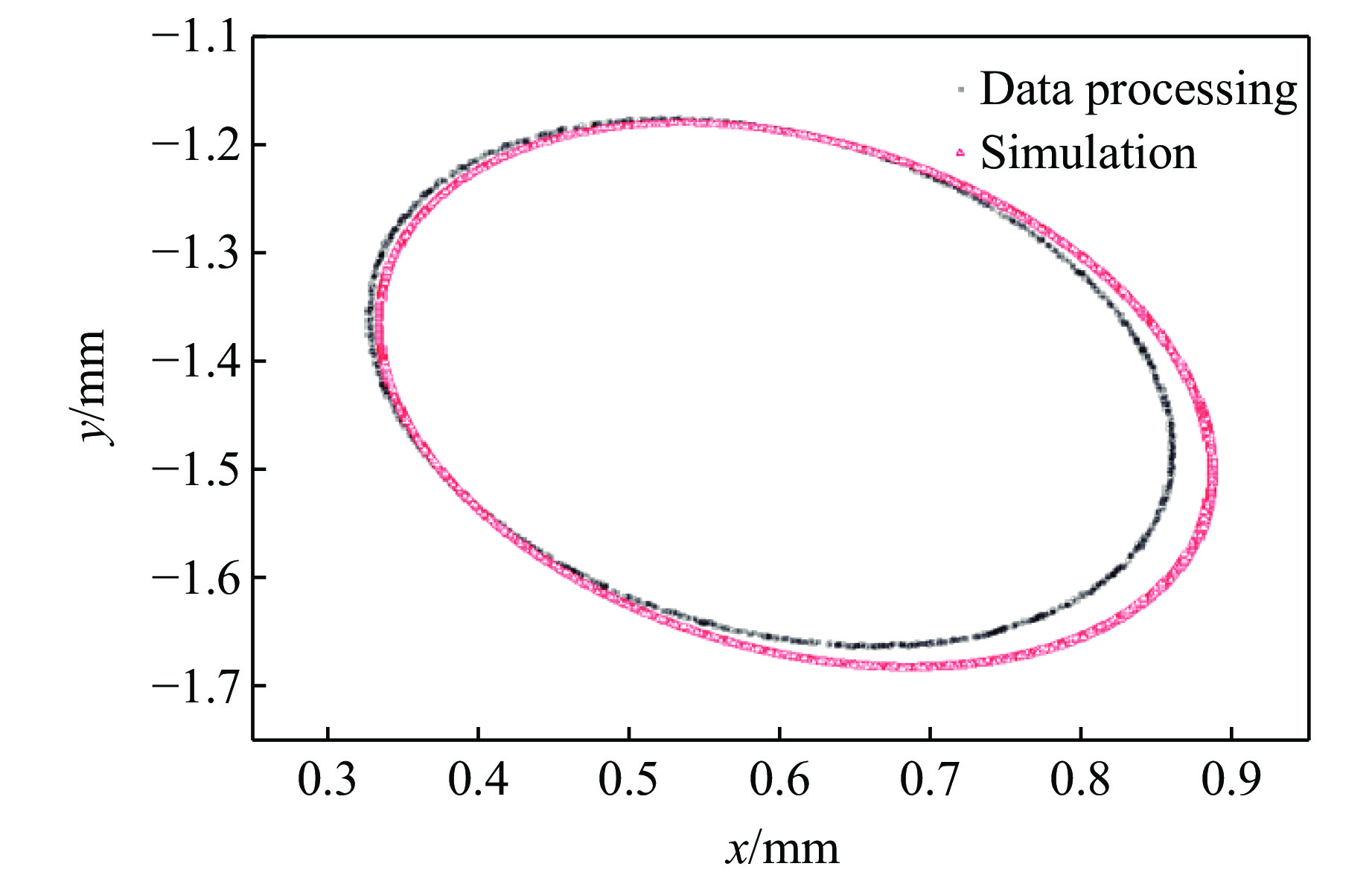
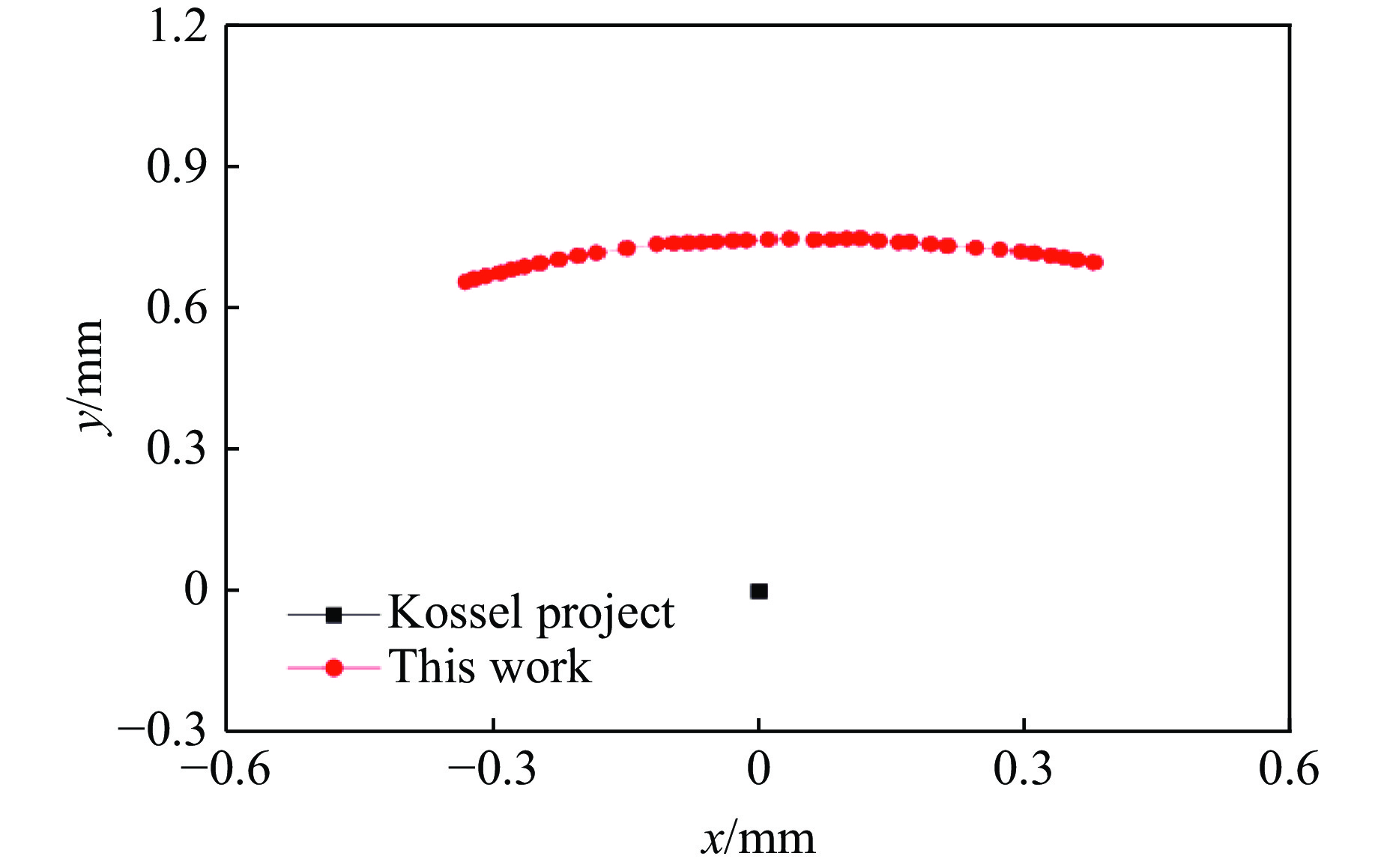
A simulation for the XRD experiment of the Fe (011) sample using copper target-based laser was worked out. The film detector was set for the central diffracted angle of 32.5° (Fig.4). The diffraction pattern was complex, even the diffraction line is similar to an oval, as shown in Fig.5. Using our method, we processed the diffraction pattern with the index (231). The interaction points obtained were plotted in Fig.6. Upon comparison, we found that the data-processed interaction points were closer to the true ones, as provided by forward simulation (red triangle points in Fig.6).

Figure 4. Geometry of the large-angle film detector

DownLoad: Full-Size Img PowerPoint

Figure 5. Diffraction pattern of Fe (011) sample using the copper target-based laser

DownLoad: Full-Size Img PowerPoint

Figure 6. Interaction points for diffraction line (231) shown in Fig. 5

DownLoad: Full-Size Img PowerPoint

Additionally, we determined the plane direction through data processing. As shown in the Table 1, the direction of the plane converged towards the true value via iterations. The cosine of the angle between the solved direction and the true one exceeded 0.99993, and its angle is reduced to 0.68°. Therefore, we achieved the excellent results as expected.

Table 1. Results of an iterative process for simulation pattern

Times	n_x	n_y	n_z	sin θ	Error	Cosine	Angle of the cosine/(°)
True	−0.53452	0.37796	0.75593	0.97920
1	−0.52335	0.34581	0.77879	0.97826	0.000352	0.99915	2.36
2	−0.52711	0.37146	0.76432	0.97978	0.000154	0.99992	0.72
3	−0.52729	0.37272	0.76357	0.97985	0.000149	0.99993	0.68
Note: Cosine is the cosine value of the true unit vector (n_x, n_y, n_z) of the normal direction to the reflection plane and the analyzed one.

| Show Table

DownLoad: CSV

2.2 Static Experiment

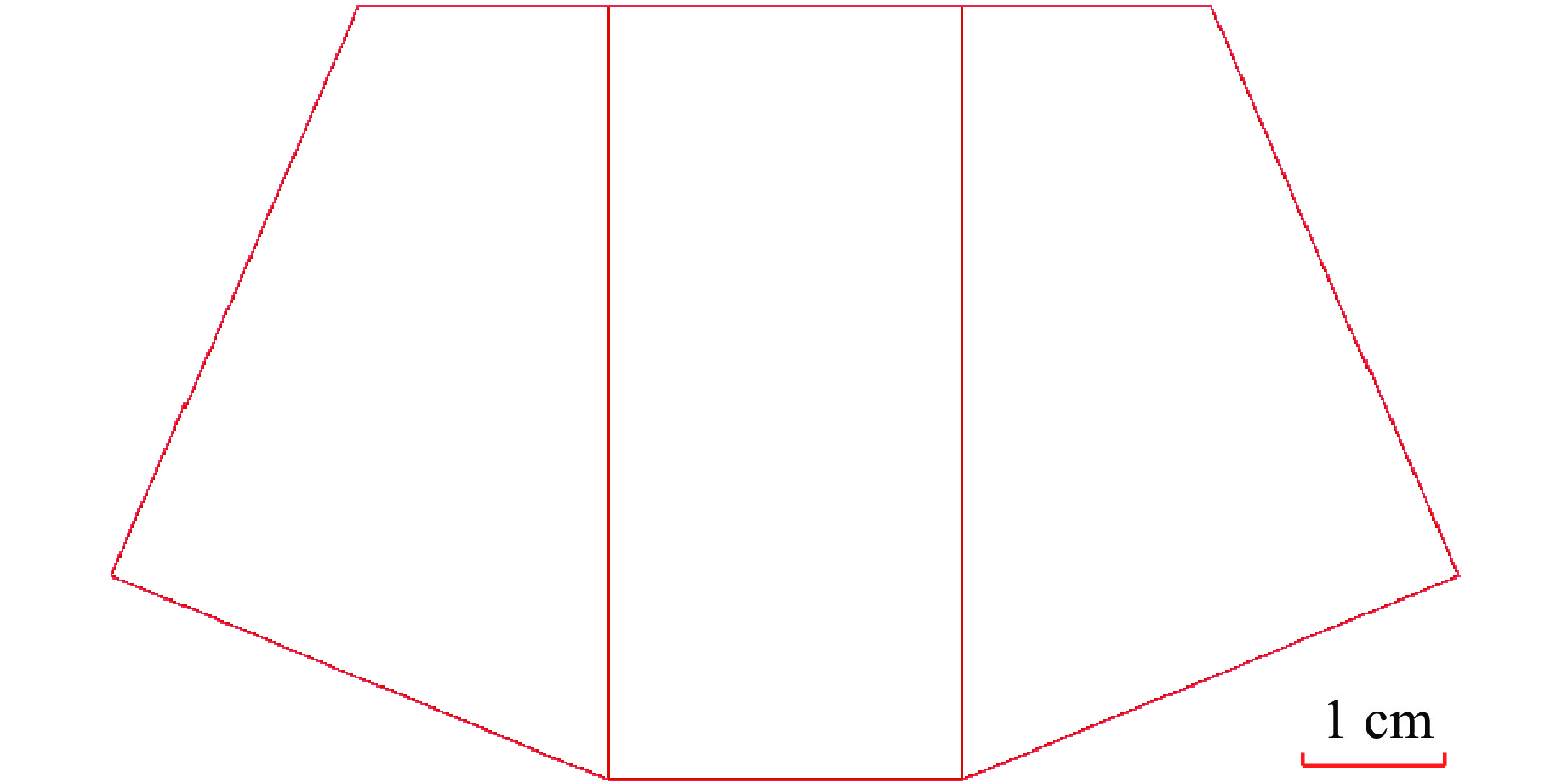
The coordinates of the diffraction line constitute raw data for XRD data analysis. To minimize the error in these coordinates, we built an axis system of origin at the sample center for the XRD experiment according to the layout depicted in Fig.1. Then we expanded the image plates shown in Fig.4 in a plane and developed a standardized template (Fig.7). The standardized template features mapped three-dimensional coordinate values which is consistent with the real space coordinates of the image plate in Fig.4.

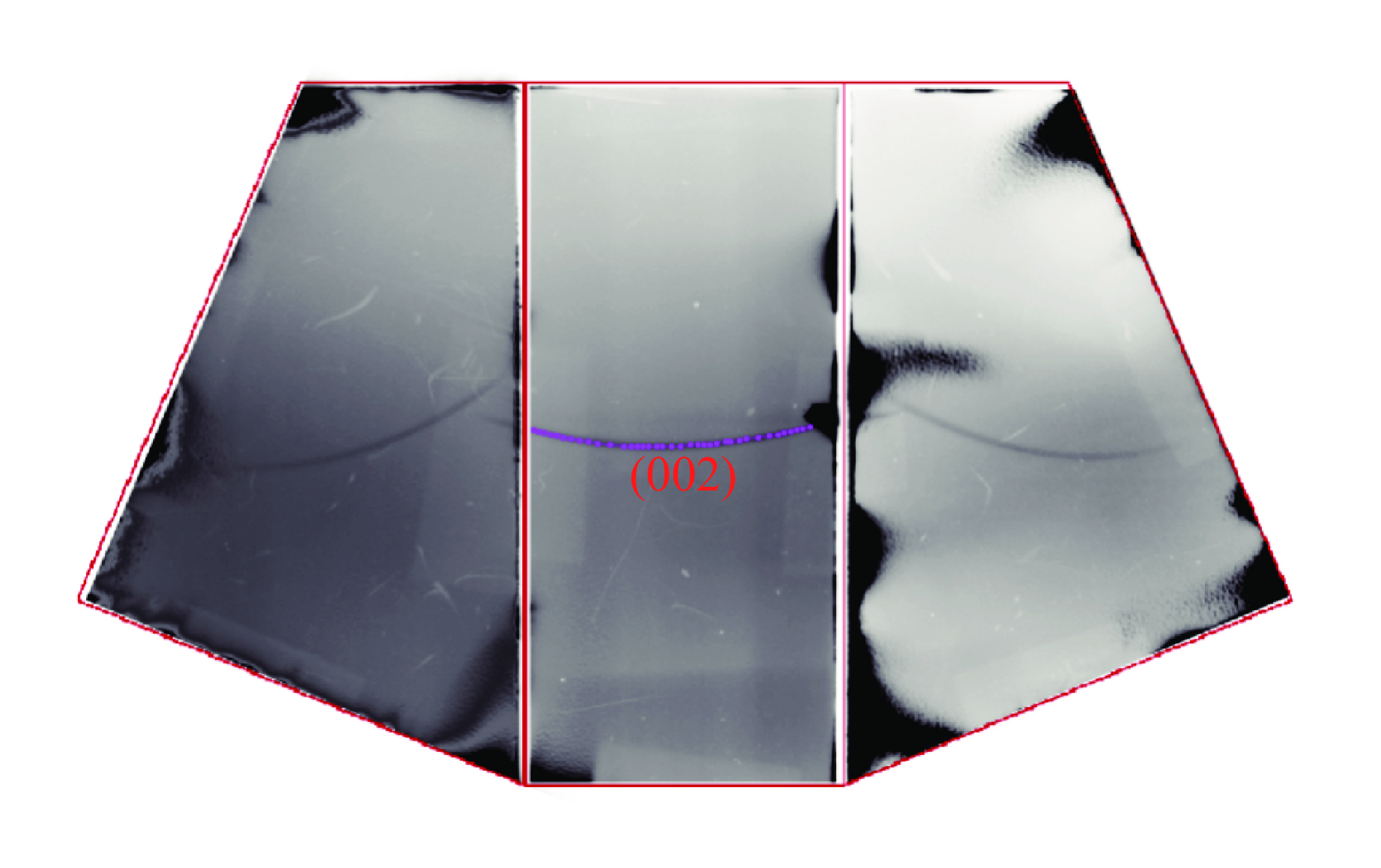
Figure 7. Standardized template for quantifying diffraction line coordinates

DownLoad: Full-Size Img PowerPoint

The experiment on iron single crystals was conducted using a film detector (Fig.4) at the SG-Ⅱ laser facility^[4]. The sample, Fe (001), was rotated 45° around the z-axis. The diffraction pattern, matched to the standardized template, is shown in Fig.8. The index of the diffraction line on the middle plane is (002). By applying the proposed method to analyze the diffraction line on the middle plane, we obtained the lattice parameters (Table 2). The first iteration results corresponded to the Kossel projection condition, with a deviation of approximately 1.76% from true value of 0.655. After tens of iterations, the results significantly improved, reducing the difference to about 0.35%. Meanwhile, we determined the interaction line between the X-ray beams and sample. As shown in Fig.9, the interaction points are not an individual point but a curve. By refining the interaction points, we achieved more accurate lattice parameters. Consequently, the proposed method enhances the accuracy of the lattice parameters, which is crucial for reducing uncertainty and enhancing data utility.

Figure 8. Standardized static diffracted pattern on film detector

DownLoad: Full-Size Img PowerPoint

Table 2. Results of the iterative method

Times	sin θ	Difference/%
1	0.6435	1.76
50	0.6523	0.35

| Show Table

DownLoad: CSV

Figure 9. Interaction points identified using different methods for the diffraction line on the middle film detector in Fig.8

DownLoad: Full-Size Img PowerPoint

3. Application

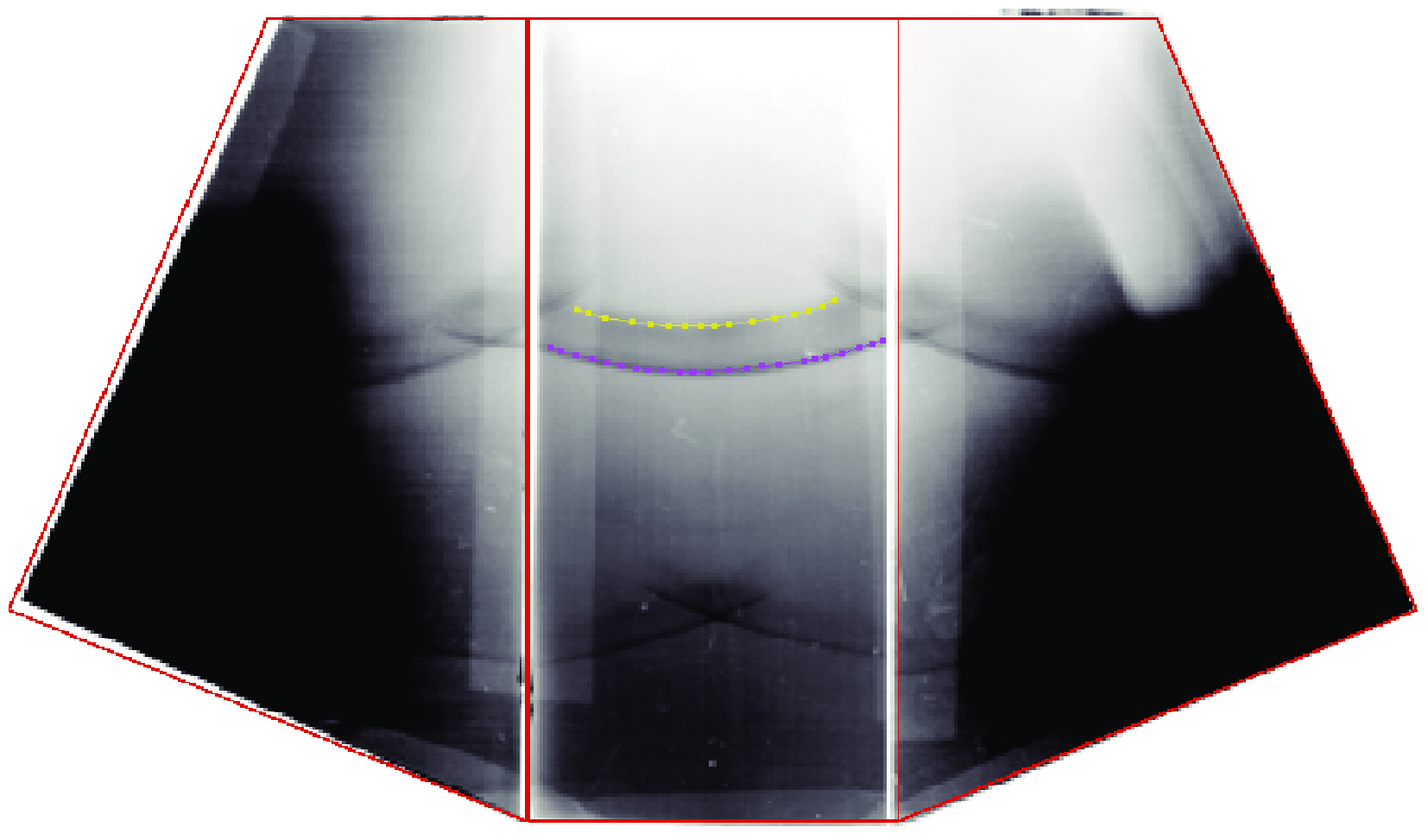
The diffraction pattern obtained from the LiF sample, oriented close to the [001] axis as the laser loading direction, is depicted in Fig.10. At a pressure of about 20 GPa, the dynamic diffraction line is far away from the static line. Using the iterative method proposed above, we calculated the sine values of the diffraction for the dynamic yellow dotted line, which is 0.8062, and for the static red dotted line, which is 0.7262. The compression ratio is 9.92%. Additionally, the two lines are not parallel, indicating that there is a rotation of the sample. From the solved normal directions of the diffraction planes for both the dynamic and static diffraction lines, we found that the angle of rotation is about 2.84°.

Figure 10. Diffraction pattern of the (100) LiF sample at about 20 GPa (Static state: red dotted line; compressed state: yellow dotted line)

DownLoad: Full-Size Img PowerPoint

4. Conclusion and Discussion

We used an iterative method to locate the interaction points on the crystal surface, thereby enhancing the accuracy of the lattice parameters for the pseudo-Kossel pattern, a classic layout for the single crystal XRD utilizing laser technology. The accuracy was improved from 1.76% to 0.35%. Furthermore, we applied the iterative method to analyze the dynamic diffraction of (001) LiF sample. At a pressure of approximately 20 GPa, we obtained a compression ratio of about 9.92% and found the angle of rotation is about 2.84°.

Currently, we have extracted information about the crystal from the data processing, especially the lattice parameters. Next, we plane to employ this method to analyze more complex experiments and try to elucidate the mechanism of the dynamic process with a forward simulation program.

图 1 20 L球形爆炸试验系统示意图

Figure 1. Schematic diagram of the 20 L spherical explosion test system

下载: 全尺寸图片幻灯片

图 2 碳酸钾改性干水材料

Figure 2. Dry water modified by potassium carbonate

下载: 全尺寸图片幻灯片

图 3 甲烷/空气预混气体在3种化学计量比下爆燃的压力-时间演化曲线

Figure 3. Pressure-time evolution curves of methane/air premixed gas deflagration at three equivalence ratios

下载: 全尺寸图片幻灯片

图 4 复配抑爆剂抑制甲烷的最大爆炸压力

Figure 4. Maximum pressure of methane inhibited by composite suppressants

下载: 全尺寸图片幻灯片

图 5 复配抑爆剂抑制甲烷的最大爆炸压力上升速率

Figure 5. Maximum pressure rising rate of methane inhibited by composite suppressants

下载: 全尺寸图片幻灯片

图 6 复配抑爆剂对甲烷爆炸压力峰值时间的抑制规律

Figure 6. Peak pressure time of methane inhibited by composite suppressants

下载: 全尺寸图片幻灯片

图 7 复配抑爆剂对甲烷爆炸升压速率峰值时间的抑制规律

Figure 7. Peak pressure rising rate time of methane inhibited by composite suppressants

下载: 全尺寸图片幻灯片

图 8 碳酸钾改性干水-C₃H₂F₆对甲烷爆炸的作用示意图

Figure 8. Explosion inhibition mechanism of the dry water modified by potassium carbonate-C₃H₂F₆ mixtures

下载: 全尺寸图片幻灯片

表 1 试验工况

Table 1. Test conditions

Compounding ratios	$\varphi_{{\rm{C}_3} {{\rm{H}_2}}{{\rm{F}_6}}}$ /%	m/g	Compounding ratios	$\varphi_{{\rm{C}_3} {{\rm{H}_2}}{{\rm{F}_6}}}$ /%	m/g
1%-2 g	1	2	5%-2 g	5	2
1%-4 g	1	4	5%-4 g	5	4
1%-6 g	1	6	5%-6 g	5	6
3%-2 g	3	2	7%-2 g	7	2
3%-4 g	3	4	7%-4 g	7	4
3%-6 g	3	6	7%-6 g	7	6

下载: 导出CSV

表 2 复配抑爆工况及抑爆结果

Table 2. Coupling inhibition conditions and the corresponding test results

Compounding ratio	Test results
Compounding ratio	ϕ=0.8	ϕ=1.0	ϕ=1.2
1%-2 g	Exploded	Exploded	Exploded
1%-4 g	Exploded	Exploded	Exploded
1%-6 g	Exploded	Exploded	Unexploded
3%-2 g	Exploded	Exploded	Unexploded
3%-4 g	Exploded	Exploded	Unexploded
3%-6 g	Exploded	Exploded	Unexploded
5%-2 g	Exploded	Unexploded	Unexploded
5%-4 g	Exploded	Unexploded	Unexploded
5%-6 g	Exploded	Unexploded	Unexploded
7%-2 g	Unexploded	Unexploded	Unexploded

下载: 导出CSV

表 3 抑制甲烷爆炸的临界配比

Table 3. Critical compounding ratios for methane explosion suppression

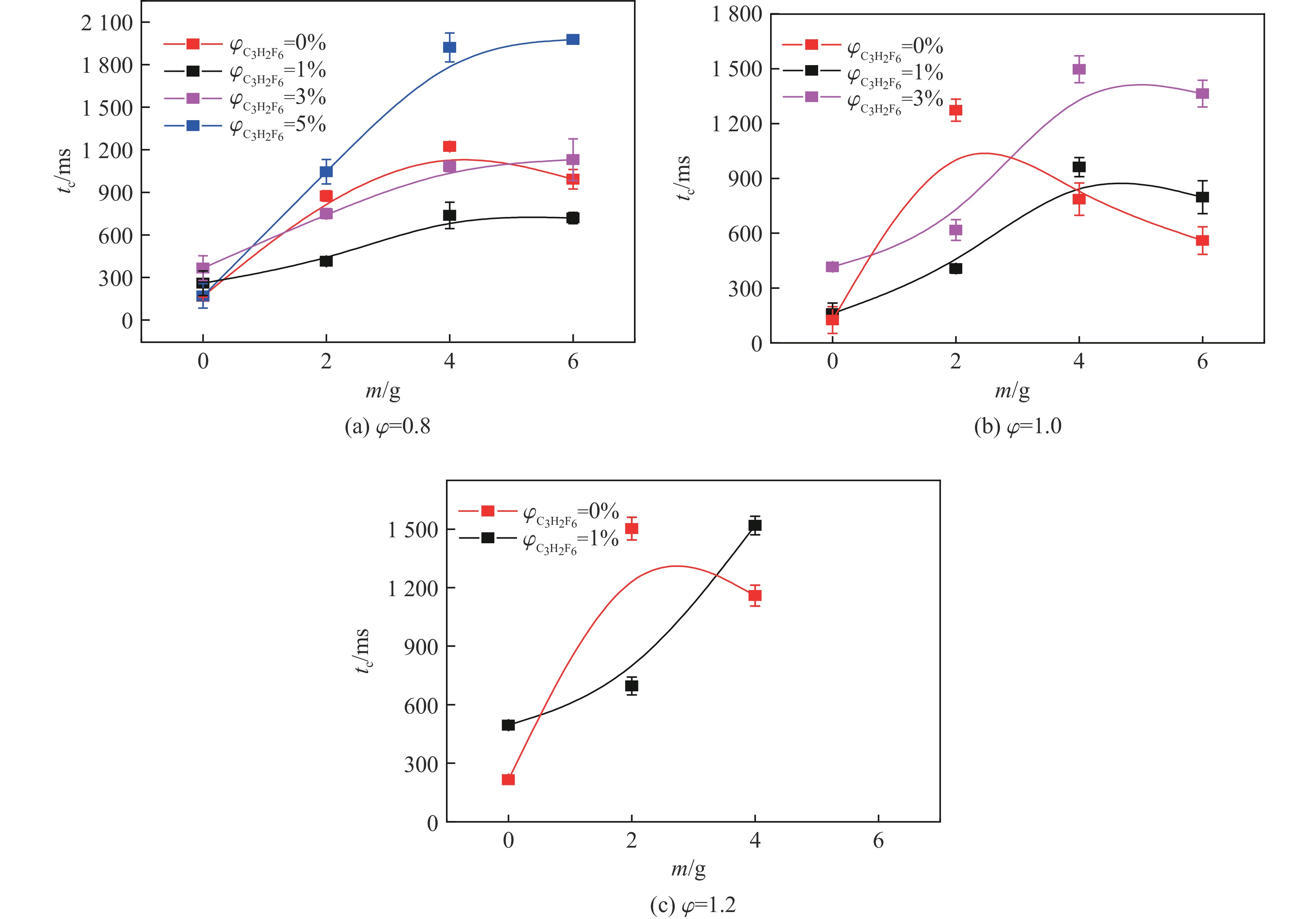
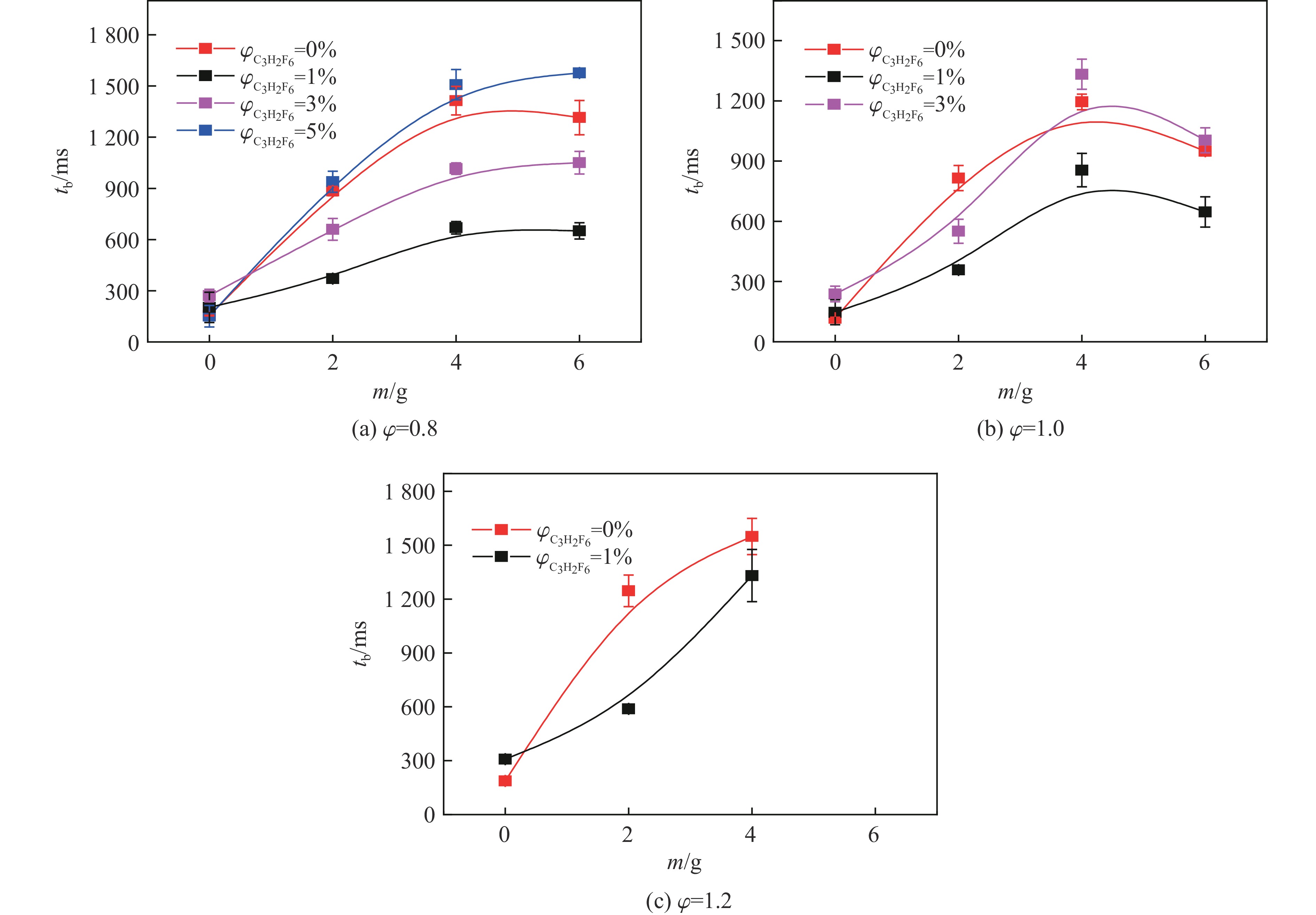
ϕ	$\varphi_{{\rm{C}_3} {{\rm{H}_2}}{{\rm{F}_6}}}$ /%	Critical compounding ratio
0.8	10	5%-6 g
1.0	7	3%-6 g
1.2	4	1%-4 g

下载: 导出CSV

表 4 碳酸钾热解的关键基元反应^[37]

Table 4. Key elementary reactions for the pyrolysis of potassium carbonate^[37]

Reactions	Indexing factor	E_a/(J·mol⁻¹)
KCO₃+H=KOH+CO₂	3×10¹²	70 040.2
KCO₃+O=KO₂+CO₂	5×10¹²	52 509.2
KHCO₃+KO=K₂CO₃+OH	6×10¹²	122 549.4
KHCO₃+KOH=K₂CO₃+H₂O	3×10¹²	157 569.4
KCO₃+KO=K₂CO₃+O	7×10¹²	87 529.3
KCO₃+KO₂=K₂CO₃+O₂	1×10¹³	52 509.2
K₂CO₃+M=K₂O+CO₂+M	5×10¹⁶	1 417 957.6
K₂CO₃+OH=KCO₃+KOH	3×10¹⁴	192 547.7
K₂CO₃+O=K₂O₂+CO₂	3×10¹⁴	192 547.7

下载: 导出CSV

参考文献(40)

[1]	邓军, 周佳敏, 白祖锦, 等. 瓦斯对煤低温氧化过程微观结构及热反应性的影响研究 [J]. 煤炭科学技术, 2023, 51(1): 304–312. DENG J, ZHOU J M, BAI Z J, et al. Effect of gas on microstructure and thermal reactivity of coal during low temperature oxidation [J]. Coal Science and Technology, 2023, 51(1): 304–312.
[2]	FAN W P, GAO Y, ZHANG Y M, et al. Experimental studies and modeling on flame velocity in turbulent deflagration in an open tube [J]. Process Safety and Environmental Protection, 2019, 129: 291–307. doi: 10.1016/j.psep.2019.07.013
[3]	HUANG L J, WANG Y, PEI S F, et al. Effect of elevated pressure on the explosion and flammability limits of methane-air mixtures [J]. Energy, 2019, 186: 115840. doi: 10.1016/j.energy.2019.07.170
[4]	SUN X X, LU S X. On the mechanisms of flame propagation in methane-air mixtures with concentration gradient [J]. Energy, 2020, 202: 117782. doi: 10.1016/j.energy.2020.117782
[5]	YI W, WEN X P, GUO Z D, et al. Experimental study on the propagation characteristics of hydrogen/methane/air premixed flames in a narrow channel [J]. International Journal of Hydrogen Energy, 2022, 47(9): 6377–6387. doi: 10.1016/j.ijhydene.2021.12.019
[6]	SKŘÍNSKÝ J, OCHODEK T, VEREŠ J, et al. The effect of low temperature on the explosion characteristics of a methane/air mixtures [J]. Energy Reports, 2022, 8(Suppl 15): 303–308.
[7]	程方明, 南凡, 罗振敏, 等. 瓦斯抑爆材料及机理研究进展与发展趋势 [J]. 煤炭科学技术, 2021, 49(8): 114–124. CHENG F M, NAN F, LUO Z M, et al. Research progress and development trend of gas explosion suppression materials and mechanism [J]. Coal Science and Technology, 2021, 49(8): 114–124.
[8]	JI H, LU R J, YANG K, et al. Experimental study on methane explosion suppression by heptafluoropropane drived modified ABC powder [J]. Process Safety and Environmental Protection, 2023, 170: 623–635. doi: 10.1016/j.psep.2022.12.031
[9]	WANG T, YANG P, YI W Z, et al. Effect of obstacle shape on the deflagration characteristics of premixed LPG-air mixtures in a closed tube [J]. Process Safety and Environmental Protection, 2022, 168: 248–256. doi: 10.1016/j.psep.2022.09.079
[10]	裴蓓, 李世梁, 韦双明, 等. N₂/超细水雾抑制甲烷爆炸点火和火焰传播特性 [J]. 中国矿业大学学报, 2023, 52(2): 329–341. PEI B, LI S L, WEI S M, et al. Inhibition effect on the ignition and flame propagation characteristic of methane explosion by N₂/ultrafine water mist [J]. Journal of China University of Mining & Technology, 2023, 52(2): 329–341.
[11]	杨克, 张平, 邢志祥, 等. 含NaCl超细水雾抑制甲烷爆炸实验研究 [J]. 中国安全生产科学技术, 2019, 15(3): 86–91. doi: 10.11731/j.issn.1673-193x.2019.03.014 YANG K, ZHANG P, XING Z X, et al. Experimental study on methane explosion suppression by ultrafine water mist containing NaCl additive [J]. Journal of Safety Science and Technology, 2019, 15(3): 86–91. doi: 10.11731/j.issn.1673-193x.2019.03.014
[12]	LIU R Z, ZHANG M C, JIA B S. Application of gas explosion nanometer powder suppression material in coal mine safety [J]. Integrated Ferroelectrics, 2021, 217(1): 240–254. doi: 10.1080/10584587.2021.1911317
[13]	覃欣欣, 司荣军, 李润之. 抑爆剂面密度对瓦斯爆炸传播抑制效果的影响 [J]. 煤矿安全, 2016, 47(12): 168–171. QIN X X, SI R J, LI R Z. Influence of surface density of explosion suppression agent on inhibiting effect of gas explosion propagation [J]. Safety in Coal Mines, 2016, 47(12): 168–171.
[14]	LUO Z M, SUN Y L, WANG T, et al. Synergistic inhibition of H₂/CH₄ explosions by CO₂/modified KHCO₃ powder [J]. Journal of Loss Prevention in the Process Industries, 2023, 86: 105197. doi: 10.1016/j.jlp.2023.105197
[15]	ZHENG L G, WANG Y L, YU S J, et al. The premixed methane/air explosion inhibited by sodium bicarbonate with different particle size distributions [J]. Powder Technology, 2019, 354: 630–640. doi: 10.1016/j.powtec.2019.06.034
[16]	纪文涛, 张国涛, 杨帅帅, 等. 惰性粉体抑制瓦斯/煤尘复合爆炸特性及机理研究 [J]. 煤炭科学技术, 2024, 52(11): 337–345. doi: 10.12438/cst.2023-1411 JI W T, ZHANG G T, YANG S S, et al. Study on the characteristics and mechanism of inert powder inhibition of gas/coal dust compound explosion [J]. Coal Science and Technology, 2024, 52(11): 337–345. doi: 10.12438/cst.2023-1411
[17]	YANG K, LI L K, JI H, et al. Experimental study of NaHCO₃/modified vermiculite composite powder for methane explosion suppression [J]. Fuel, 2024, 378: 132808. doi: 10.1016/j.fuel.2024.132808
[18]	CHEN J S, CHEN K, SHI W X, et al. The preparation of novel core-shell suppressor and its suppression mechanism on coal dust explosion flame [J]. Fuel, 2022, 313: 122997. doi: 10.1016/j.fuel.2021.122997
[19]	CHEN X F, FAN A, YUAN B H, et al. Renewable biomass gel reinforced core-shell dry water material as novel fire extinguishing agent [J]. Journal of Loss Prevention in the Process Industries, 2019, 59: 14–22. doi: 10.1016/j.jlp.2019.02.008
[20]	TIAN S Y, QIN B T, MA D, et al. Suppressive effects of alkali metal salt modified dry water material on methane-air explosion [J]. Energy, 2023, 285: 129547. doi: 10.1016/j.energy.2023.129547
[21]	LEE E, SON H, CHOI Y. Elucidating the effects of particle sizes on the fire extinguishing performance of core-shell dry water [J]. Korean Journal of Chemical Engineering, 2020, 37(10): 1642–1648. doi: 10.1007/s11814-020-0632-0
[22]	吴佩利. 改性干水粉体制备及灭火性能研究 [D]. 西安: 西安科技大学, 2022. WU P L. Study on preparation and fire extinguishing performance of modified dry water powder [D]. Xi’an: Xi’an University of Science and Technology, 2022.
[23]	WANG Q H, MA C, DENG J, et al. Gas explosion suppression by ammonium dihydrogen phosphate-modified dry water powder [J]. Powder Technology, 2023, 416: 118228. doi: 10.1016/j.powtec.2023.118228
[24]	ZHENG X Z, KOU Z Z, LIU S K, et al. Preparation and properties of a new core-shell-modified gel dry-water powder [J]. Powder Technology, 2023, 422: 118493. doi: 10.1016/j.powtec.2023.118493
[25]	WANG T, YANG Z, YANG P, et al. The deflagration suppression effect of ammonium salt-modified dry water on methane-air mixtures: an experimental investigation [J]. Powder Technology, 2024, 434: 119313. doi: 10.1016/j.powtec.2023.119313
[26]	程方明, 南凡, 肖旸, 等. CF₃I和CO₂抑制甲烷-空气爆炸实验研究 [J]. 爆炸与冲击, 2022, 42(6): 065402. doi: 10.11883/bzycj-2021-0386 CHENG F M, NAN F, XIAO Y, et al. Experimental study on the suppression of methane-air explosion by CF₃I and CO₂ [J]. Explosion and Shock Waves, 2022, 42(6): 065402. doi: 10.11883/bzycj-2021-0386
[27]	LIANG H, YAN X Q, SHI E H, et al. Flame evolution and pressure dynamics of premixed stoichiometric ammonia/hydrogen/air in a closed duct [J]. Fuel, 2024, 363: 130983. doi: 10.1016/j.fuel.2024.130983
[28]	王保. 20 L球内惰气抑制甲烷-乙烯与空气预混爆炸特性研究 [D]. 淮南: 安徽理工大学, 2022. WANG B. Study on the performance of 20 L inert gas in a sphere to suppress the premixed explosion of methane-ethylene with air [D]. Huainan: Anhui University of Science and Technology, 2022.
[29]	王燕, 林森, 李忠, 等. 惰性气体对KHCO₃冷气溶胶甲烷抑爆性能的影响研究 [J]. 煤炭科学技术, 2021, 49(2): 145–152. WANG Y, LIN S, LI Z, et al. Research on synergistic effect of inert gas on methane explosion suppression performance of KHCO₃ cold aerosol [J]. Coal Science and Technology, 2021, 49(2): 145–152.
[30]	DONG Z Q, LIU L J, CHU Y Y, et al. Explosion suppression range and the minimum amount for complete suppression on methane-air explosion by heptafluoropropane [J]. Fuel, 2022, 328: 125331. doi: 10.1016/j.fuel.2022.125331
[31]	杨克, 陈舒佳, 邢志祥, 等. 七氟丙烷与障碍物对甲烷爆炸耦合影响的试验研究 [J]. 中国矿业大学学报, 2023, 52(4): 750–760. YANG K, CHEN S J, XING Z X, et al. Experimental study of the coupling effect of heptafluoropropane and obstacle on the methane explosion [J]. Journal of China University of Mining and Technology, 2023, 52(4): 750–760.
[32]	王涛, 董哲, 盛禹淮, 等. 卤代烷气体灭火剂促进-抑制瓦斯燃爆特性试验 [J]. 煤炭科学技术, 2024, 52(4): 265–274. doi: 10.12438/cst.2023-1793 WANG T, DONG Z, SHENG Y H, et al. Experiment on the promoting-inhibiting effects on methane explosion by using haloalkanes [J]. Coal Science and Technology, 2024, 52(4): 265–274. doi: 10.12438/cst.2023-1793
[33]	孟祥卿. 气/固两相抑制剂的甲烷抑爆特性研究 [D]. 焦作: 河南理工大学, 2019. MENG X Q. Suppression characteristics of gas/solid two-phase inhibitors on methane explosion [D]. Jiaozuo: Henan Polytechnic University, 2019.
[34]	ZHAO T L, CHEN X K, LUO Z M, et al. Effect of N₂ inerting on the inhibition of methane explosions by a multicomponent powder [J]. Fuel, 2023, 337: 127203. doi: 10.1016/j.fuel.2022.127203
[35]	田志辉. 气-固混合抑制剂对矿井瓦斯的抑爆实验研究 [D]. 西安: 西安科技大学, 2013. TIAN Z H. Suppressing experimental study on mine methane explosion by the gas-solid mixied inhibitors [D]. Xi’an: Xi’an University of Science and Technology, 2013.
[36]	LUO Z M, WANG T, TIAN Z H, et al. Experimental study on the suppression of gas explosion using the gas-solid suppressant of CO₂/ABC powder [J]. Journal of Loss Prevention in the Process Industries, 2014, 30: 17–23. doi: 10.1016/j.jlp.2014.04.006
[37]	BABUSHOK V I, LINTERIS G T, HOORELBEKE P, et al. Flame inhibition by potassium-containing compounds [J]. Combustion Science and Technology, 2017, 189(12): 2039–2055. doi: 10.1080/00102202.2017.1347162
[38]	FERGUSON J D, JOHNSON N L, KEKENES-HUSKEY P M, et al. Unimolecular rate constants for HX or DX elimination (X=F, Cl) from chemically activated CF₃CH₂CH₂Cl, C₂H₅CH₂Cl, and C₂D₅CH₂Cl: threshold energies for HF and HCl elimination [J]. The Journal of Physical Chemistry A, 2005, 109(20): 4540–4551. doi: 10.1021/jp040735g
[39]	谈玲华, 李勤华, 冒爱琴, 等. 六氟丙烷热分解性能及机理研究 [J]. 南京理工大学学报(自然科学版), 2010, 34(5): 691–695. TAN L H, LI Q H, MAO A Q, et al. Thermal decomposition properties and mechanism of hexafluoropropane [J]. Journal of Nanjing University of Science and Technology (Natural Science), 2010, 34(5): 691–695.
[40]	BARRY J, LOCKE G, SCOLLARD D, et al. 1,1,1,3,3,-pentafluorobutane (HFC-365mfc): atmospheric degradation and contribution to radiative forcing [J]. International Journal of Chemical Kinetics, 1997, 29(8): 607–617. doi: 10.1002/(SICI)1097-4601(1997)29:8<607::AID-KIN6>3.0.CO;2-Y