First-Principles Investigation of the High-Pressure Phase Transition in Representative Alkali Metal Halides
-
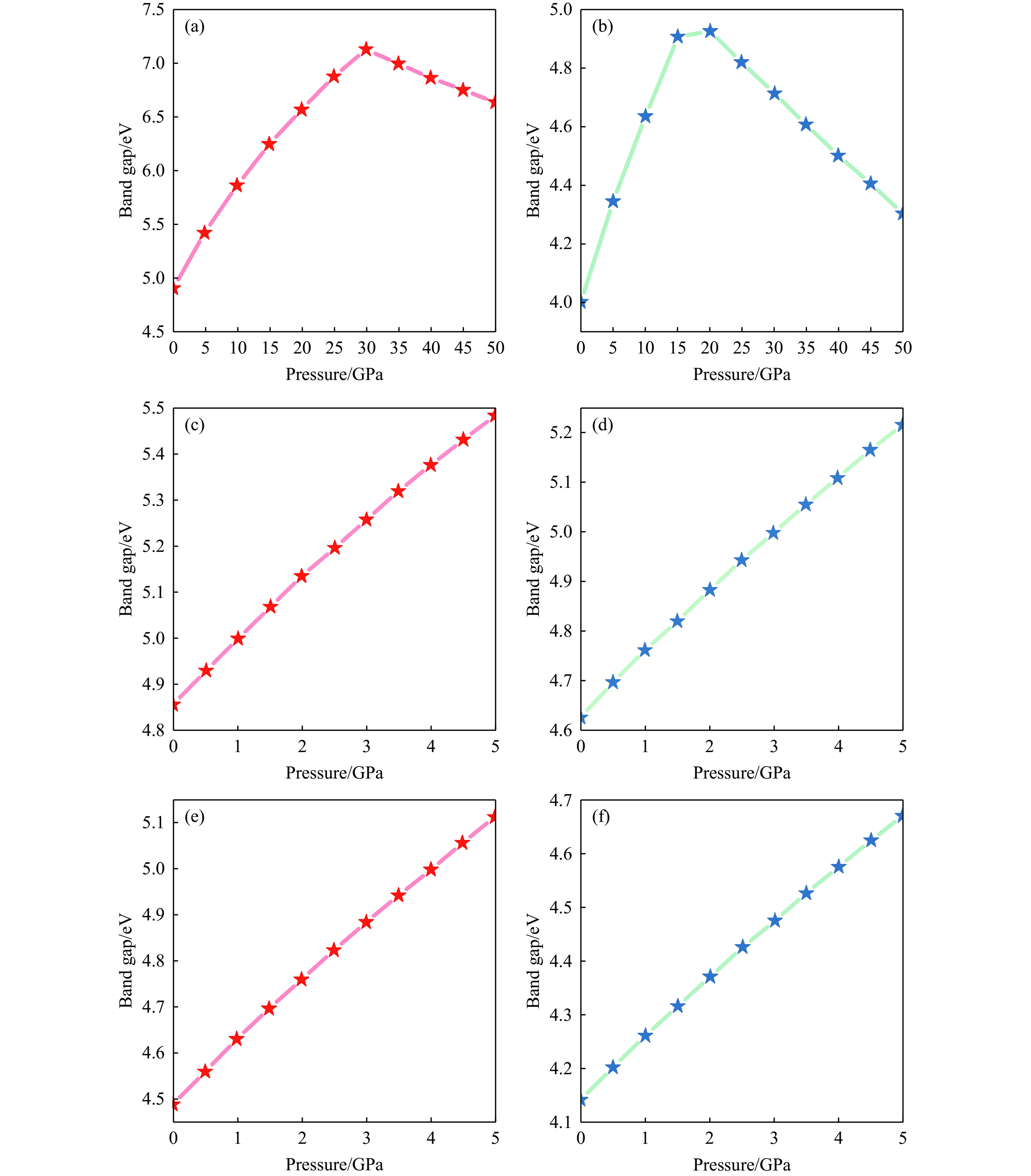
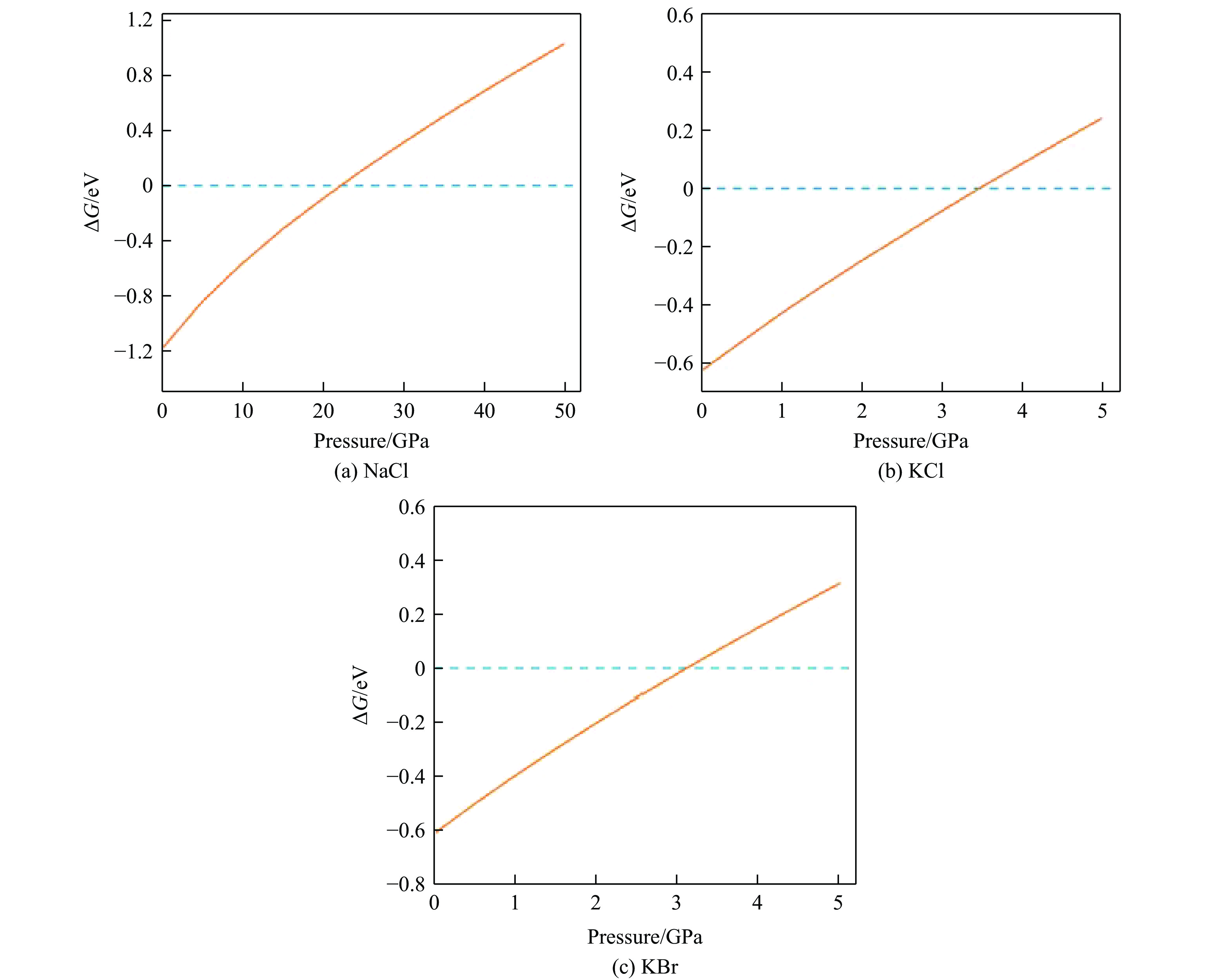
摘要: 基于密度泛函理论的第一性原理计算方法,研究了NaCl、KCl和KBr晶体在不同压力下相Ⅰ和相Ⅱ构型的几何、电子和力学性质,探讨了这些性质与相变点之间的关系,利用吉布斯自由能法对NaCl、KCl与KBr晶体的相变点进行判断。结果显示,对于NaCl的相Ⅰ结构,在0~30 GPa压力范围内,随着压力的升高,带隙不断增大;在30~50 GPa压力范围内,带隙随着压力的升高而下降,30 GPa正位于NaCl相Ⅰ结构的相变点。这表明根据电子结构判断金属卤化物在压力作用下的相变点具有一定的可行性。由高压下的晶体结构、声子谱以及力学稳定性无法对碱金属卤化物的相变点进行判断。通过吉布斯自由能法计算出了NaCl、KCl和KBr的相变点,分别为22.26、3.47和3.11 GPa。Abstract: Utilizing first-principles calculations based on density functional theory, this study investigates the geometric, electronic, and mechanical properties of NaCl, KCl, and KBr crystals in phase Ⅰ and phase Ⅱ structures under varying pressures. The relationships between these properties and the phase transition points are explored. Additionally, the Gibbs free energy method was employed to judge the phase transition points of NaCl, KCl, and KBr crystals. The results show that in the phase Ⅰ structure of NaCl, the band gap value increases with pressure from 0 to 30 GPa. However, in the range of 30−50 GPa, the band gap value decreases, indicating that 30 GPa is the phase transition point for NaCl phase Ⅰ. This suggests that pressure-induced changes in electronic structure can be indicative of metal halide phase transition points to some extent. However, pressure-induced alterations in crystal structure, phonon spectrum, and mechanical stability cannot reliably indicate alkali metal halide phase transition points. Furthermore, the phase transition points for NaCl, KCl and KBr calculated by Gibbs free energy method are 22.26, 3.47 and 3.11 GPa, respectively.
-
Key words:
- alkali halide /
- phase transition /
- first-principles /
- high pressure /
- band gap /
- Gibbs free energy
-
火炮发射时,后效期高温高压的火药气体从膛口瞬时流出,对火炮主体形成很强的后坐力,对炮架产生强冲击作用,影响火炮射击精度和机动性能,制约高性能火炮的发展。炮口制退器作为一种安装在炮口部位的排气装置,通过控制后效期火药气体的流量分配和气流速度,减小射击时火药气体作用于后坐部分的冲量,并为炮身提供制退力,减小火炮后坐动能和炮架的射击载荷,因而成为一种广泛应用的反后坐技术[1]。然而,炮口制退器的使用具有一定的负面效应。一方面,炮口制退器造成炮口区域强激波,加剧后效期火药气体流场的复杂性及膛口焰现象,加大火炮发射初速扰动及膛口冲击波超压,对火炮身管周围设备及操作人员造成不良影响;另一方面,加装制退器后火炮身管本身质量及挠度的增大也对射击精度造成不利影响。炮口制退器自身的质量和结构决定了其制退性能及负面危害表现,通过选材及结构的优化设计实现高性能、低危害的综合性能成为当前炮口制退器研究与发展的方向[2–6]。
按照结构形式的不同,传统的炮口制退器分为冲击式、反作用式及冲击反作用式。由于工艺、重量的限制,高效率与低负面效应往往难以兼顾。近年来,增材制造技术的发展为复杂异型结构的加工制造以及钛合金等难加工轻金属材料的成型制备提供了有力支撑[7],也为传统装置结构的优化与创新设计提供了更大的自由空间。随着计算机技术和计算流体力学的发展,数值模拟已成为低成本研究膛口流场及膛口装置的重要手段,例如:张焕好等[8–9]、代淑兰等[10]利用数值模拟方法,对不同类型炮口制退器的膛口流场波系、膛口激波及二次焰特征进行了详细研究,得到了制退器装置效率;Lei等[11]、Chaturvedi等[12]通过将流场仿真与流固耦合相结合,实现了炮口制退器结构应力与变形响应研究及性能评价。本研究利用增材制造技术优势,针对机载火炮小口径炮口制退器综合性能的设计需求,提出一种叠加冲击式与反作用式传统制退器结构特征与优点的钛合金新型炮口制退器结构方案,对膛口流场发展过程及特征进行数值模拟分析,并以传统的高效率冲击式炮口制退器为参照,对新型结构制退器的综合性能予以评价。
1. 制退器结构
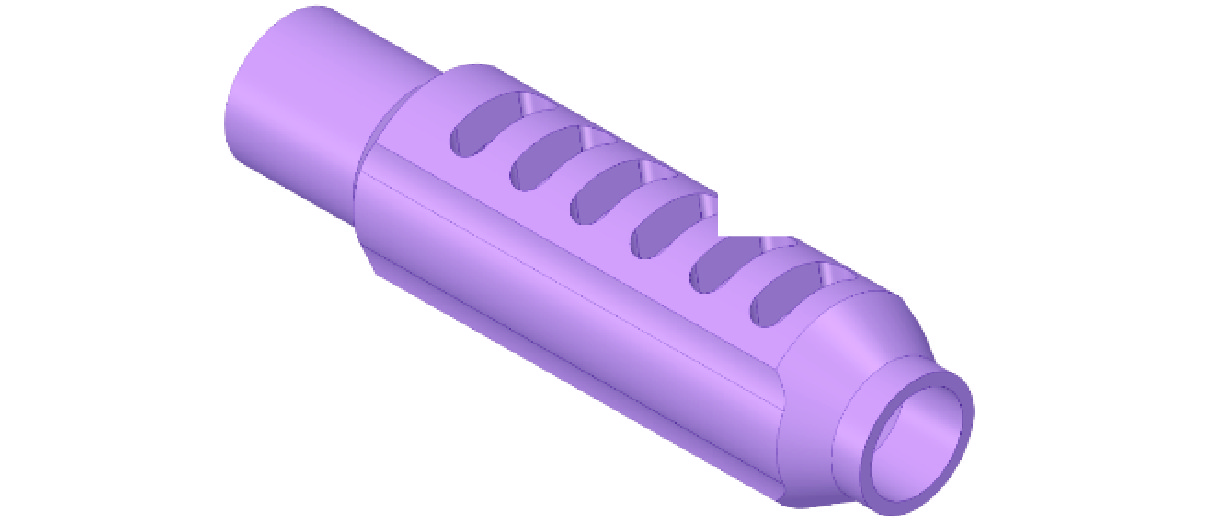
炮口制退器的基本构型是后端与炮管封闭相接,前端收缩,中间拥有膨胀腔室、内部挡板和侧孔的圆筒形结构。冲击式炮口制退器有较大的腔室直径和侧孔面积,火药气体膨胀较为充分,大部分经侧向流出并冲击反射挡板,制退效率高,但炮口冲击波危害也较大。反作用式炮口制退器的直径一般较小,拥有多排小面积侧孔,没有或有很小的反射挡板,制退效率较低,但膛口火药气体流场相对简单,对弹丸初速的扰动小,不会影响大威力脱壳穿甲弹发射。基于上述两种制退器的结构特征和性能的经验规律,本研究以低冲击波危害和高制退效率为导向,在三维建模平台上设计一种面向机载航炮的30 mm小口径新型结构炮口制退器,如图1所示。
新型制退器内层为冲击式内壁结构,见图1,其上设置编号为1~3的3组阵列式大面积后倾侧孔,旨在引导火药气体出膛后的侧向分流,以实现类似于冲击式炮口制退器的高制退效率。此外,3组内层侧孔的后倾角度及面积采用非均等设计,参照一般冲击式炮口制退器的结构参数,对三维设计模型直接设定或导出得到内层侧孔的非均等结构参数,见表1。其中:为了限制火药气体在临近膛口位置的向后偏转角度及炮口后方冲击波的强度,第1组侧孔的后倾角度及面积均小于其他两组;根据火药气体出膛以后沿轴向流量逐渐衰减的特点,第2组侧孔的面积设置为大于其他两组。新型结构制退器外层为胡椒瓶式套筒结构,其上设置成组分布的阵列式小面积圆孔,各组圆孔与内层侧孔周向重叠,旨在借鉴反作用式炮口制退器的特点,对火药气体进行分散和疏导,从而抑制侧向强激波的产生,实现较低的冲击波危害效益。新型制退器弹孔处为收缩式前端面,并设有分流孔,以在出口处进一步减小中央弹孔流量及火药气体在制退器出口部位的膨胀扰动。内层结构与外层结构通过布置在孔间的阵列式筋板相连,实现整体结构的一体化,便于增材制造成型。同时,结构中不存在过于复杂或与轴向夹角过大的成型曲面,满足增材制造高质量成型工艺要求。设计方案选材为3D打印钛合金粉末,材料参数[13]见表2。为评价新型炮口制退器的综合性能,以图2所示的传统冲击式炮口制退器为对比分析对象。
表 1 新型炮口制退器结构参数Table 1. Structural parameters of new-structure muzzle brakeStructure Backward angle/(°) Area/mm2 Inner side hole 1 5 392.96 Inner side hole 2 10 470.34 Inner side hole 3 10 392.96 Outer side hole 0 78.50 Front hole 146.54 表 2 3D打印钛合金材料参数Table 2. Material parameters of titanium alloyMaterial Density/(g·cm−3) Young’s modulus/GPa Yield strength/MPa Tensile strength/MPa Poisson’s ratio TC4 4.43 118 944 1058 0.3 2. 数值方法与模型参数
采用无黏三维Euler方程描述带制退器的膛口流场气体流动。考虑到计算目的及效率,对炮口气流进行适当简化,忽略气固两相性、多组分、化学反应以及运动弹丸的影响,将火药气体与外界大气视为理想气体,且满足气体状态方程。有限元仿真的封闭方程组为
∂U∂t+∂F∂x+∂G∂y+∂H∂z=0 (1) e=pγ−1+12ρ(u2+v2+w2) (2) pV=nRT (3) 式中:e为单位质量气体的总能量,
p 为火药气体压强,V为气体体积,T为体系温度,U=[ρ,ρu,ρv,ρw,e]T ,F=[ρu,ρu2+p,ρuv,ρuw,(e+p)u]T ,G=[ρv,ρuv,ρv2+p,ρvw,(e+p)v]T ,H=[ρw,ρuw,ρvw,ρw2+p,(e+p)w]T ,ρ 为火药气体密度,u、v、w分别为x、y、z方向的速度分量,γ 为理想气体绝热指数,R为通用气体常数,n为气体的物质的量。方程的离散采用有限体积法,时间推进采用二阶精度Runge-Kutta法,对流项采用二阶精度Roe格式,湍流模型采用单方程Spalart-Allmaras模型,数值计算在ANSYS FLUENT 19.0中进行。后效期火药气体排空过程数值模拟的计算域设置为以炮口端面中心为原点、直径为3 m的球形区域,以使火药气体充分发展。计算初始时刻为弹丸飞离炮口时刻,以膛底位置为x=0,此时膛内气体沿炮轴方向任意一点的压力px、速度vx和温度Tx的初始分布状态表示为
px=pd[1+mw2ψ1mq(1−x2L2)] (4) vx=vdLx (5) Tx=pxMgρgR (6) ρg=mwVc+LS (7) 式中:
ρ g为膛内火药气体的平均密度,mw、mq、Mg分别为装药量、弹丸质量、火药气体摩尔质量,Vc、L、S分别为药室容积、身管行程长度、身管横截面积,ψ1 为内弹道次要功系数,vd为弹底火药气体速度,pd为膛口处弹底压力。本算例中,未加装炮口制退器时火炮身管质量M0=7.09 kg,初始时刻弹底火药气体速度vd=780 m/s,pd=27.9 MPa,膛口外为常温常压条件。计算时间步长取0.01 ms,当膛内气压降低至约0.2 MPa时视为后效期结束,终止计算。3. 结果分析
3.1 膛口冲击波
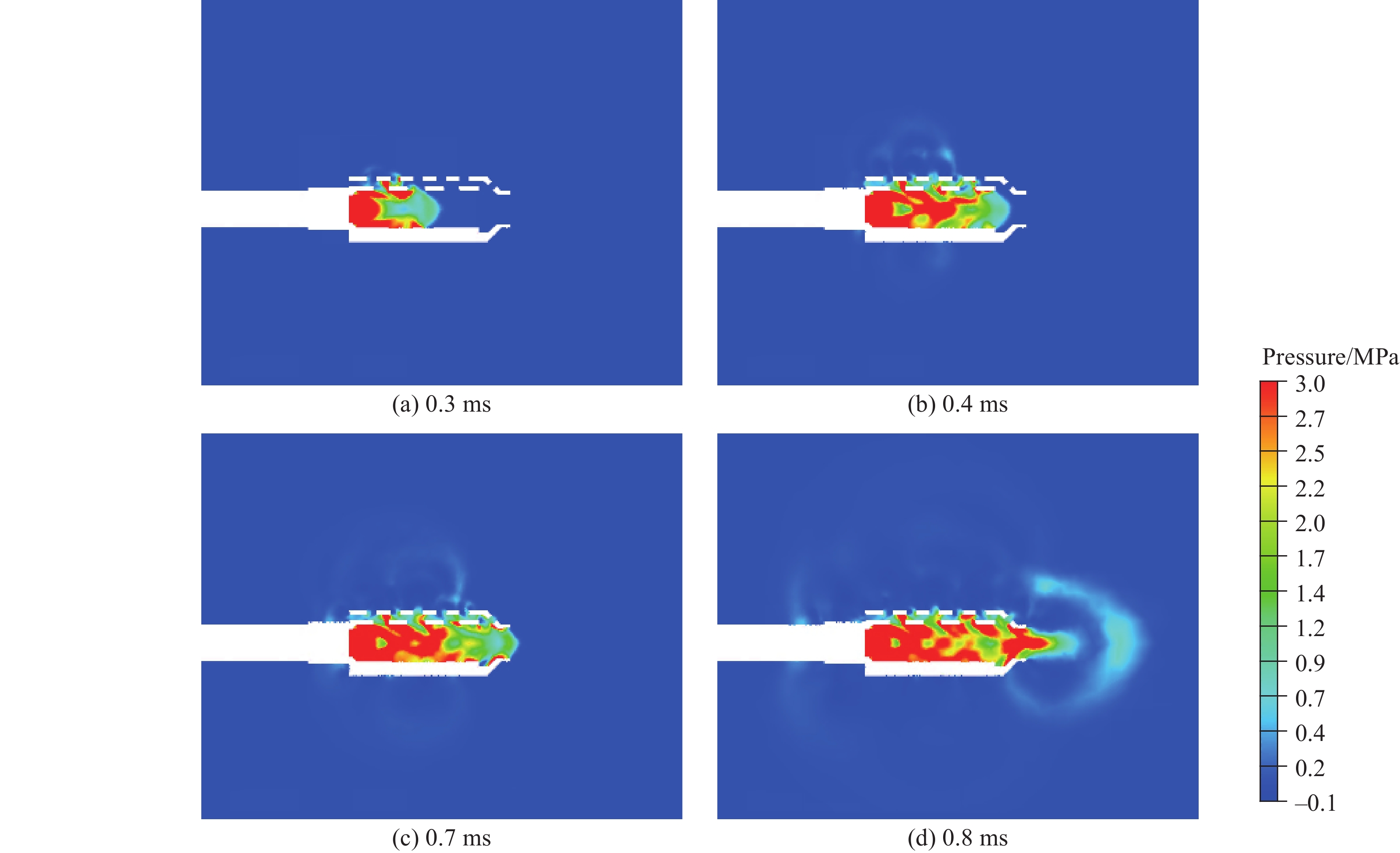
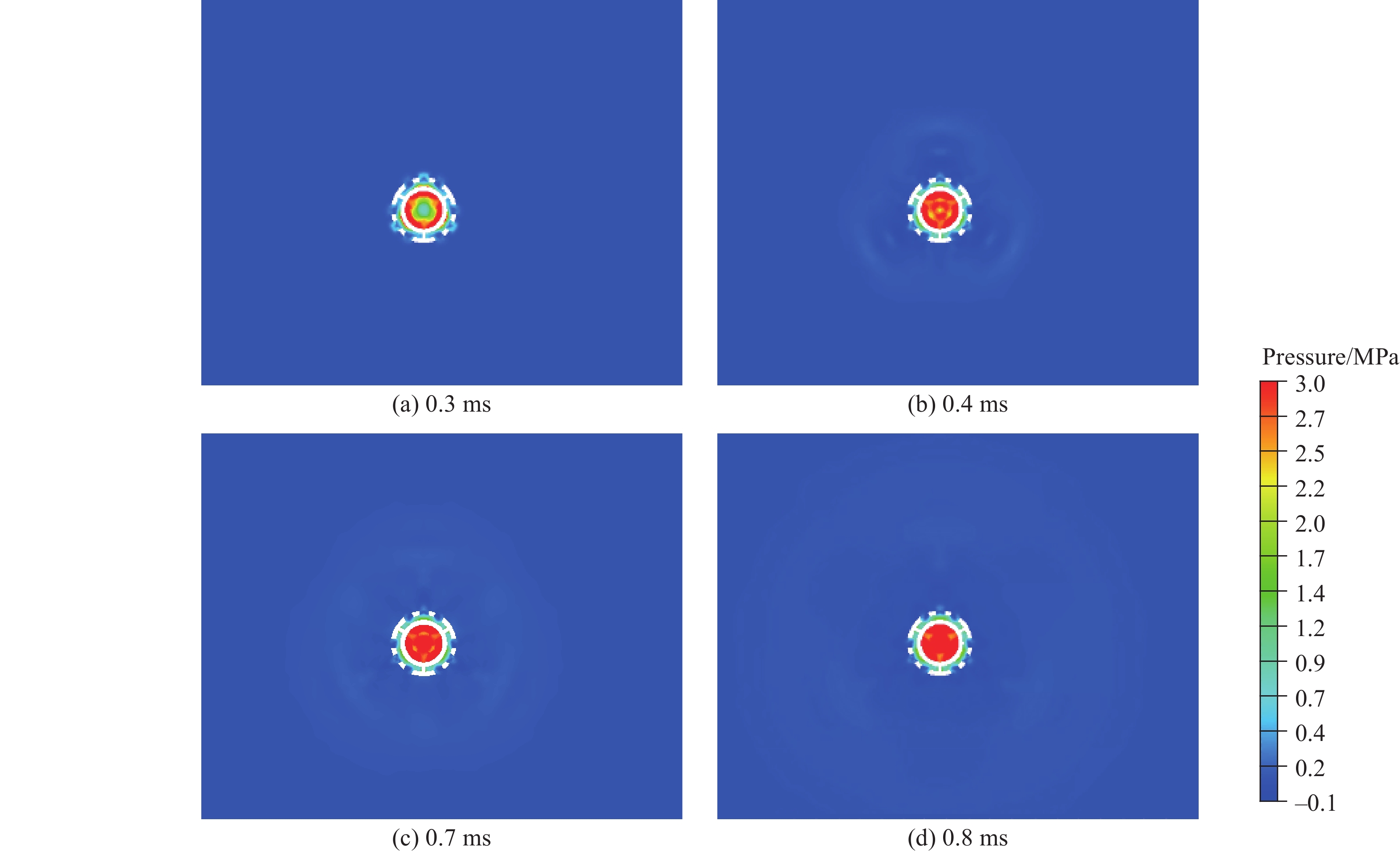
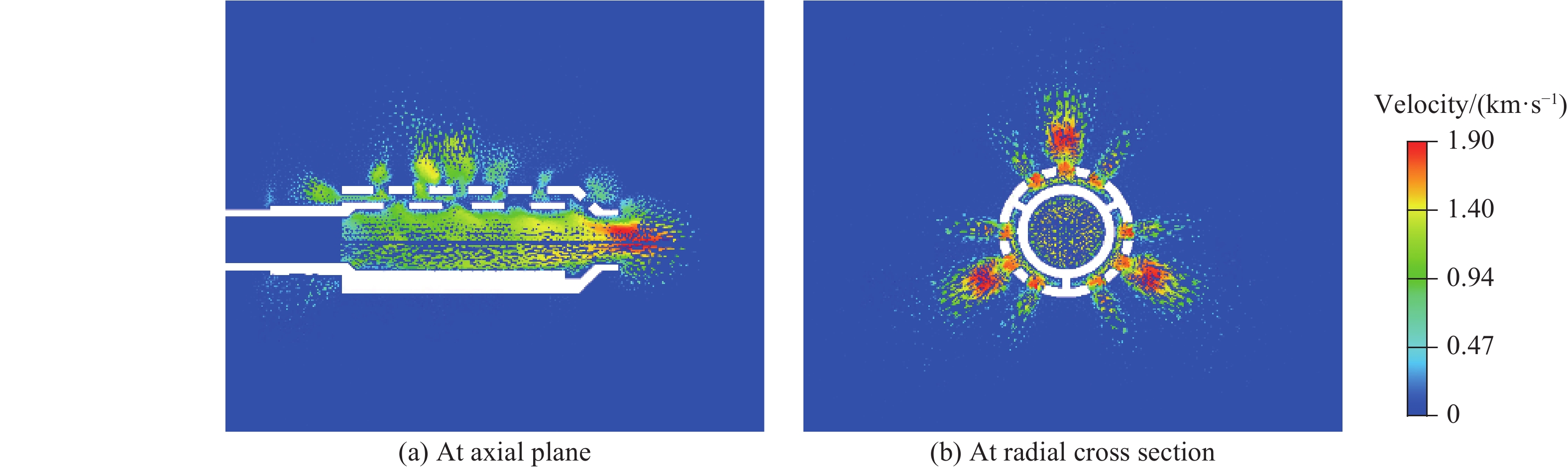
图3、图4所示为新型制退器轴向和径向截面处膛口冲击波在不同时刻的压力云图,为突出显示流场发展过程,图中隐去流场超高压及部分负压区域。图5为火药气体流场充分发展时轴向和径向截面处速度矢量图。可以看出,后效期内火药气体在流入和穿出新型制退器的过程中,与内外双层结构依次产生交互作用,形成独特的流场结构与激波形貌,并构成制退效益的基础。后效期膛口冲击波的形成过程可以分为3个阶段。(1)火药气体膨胀加速进入制退器腔室后,依次流经内层侧孔和外层小孔喷出,形成侧孔冲击波,见图3(a)和图4(a)。(2)随着火药气体的向前推进和持续补充,沿炮轴方向各外层圆孔依次形成各自的侧孔冲击波,并且伴有追赶、相交和叠加过程,最终在炮口一侧合并成为一个大的冲击波。由于膨胀速率不同,在此过程中出现了后排侧孔冲击波追赶并超过前排侧孔冲击波的现象,见图3(b)和图3(c);与此同时,周向上不同角度的三维侧孔冲击波也逐渐相交合并,见图4(b)和图4(c)。(3)火药气体流出弹孔以后,在出口处形成迅速膨胀的弹孔冲击波,并与侧孔冲击波相交,形成具有各向叠加特征的典型膛口波系结构,见图3(d)、图4(d)和图5。
3.2 超压分布
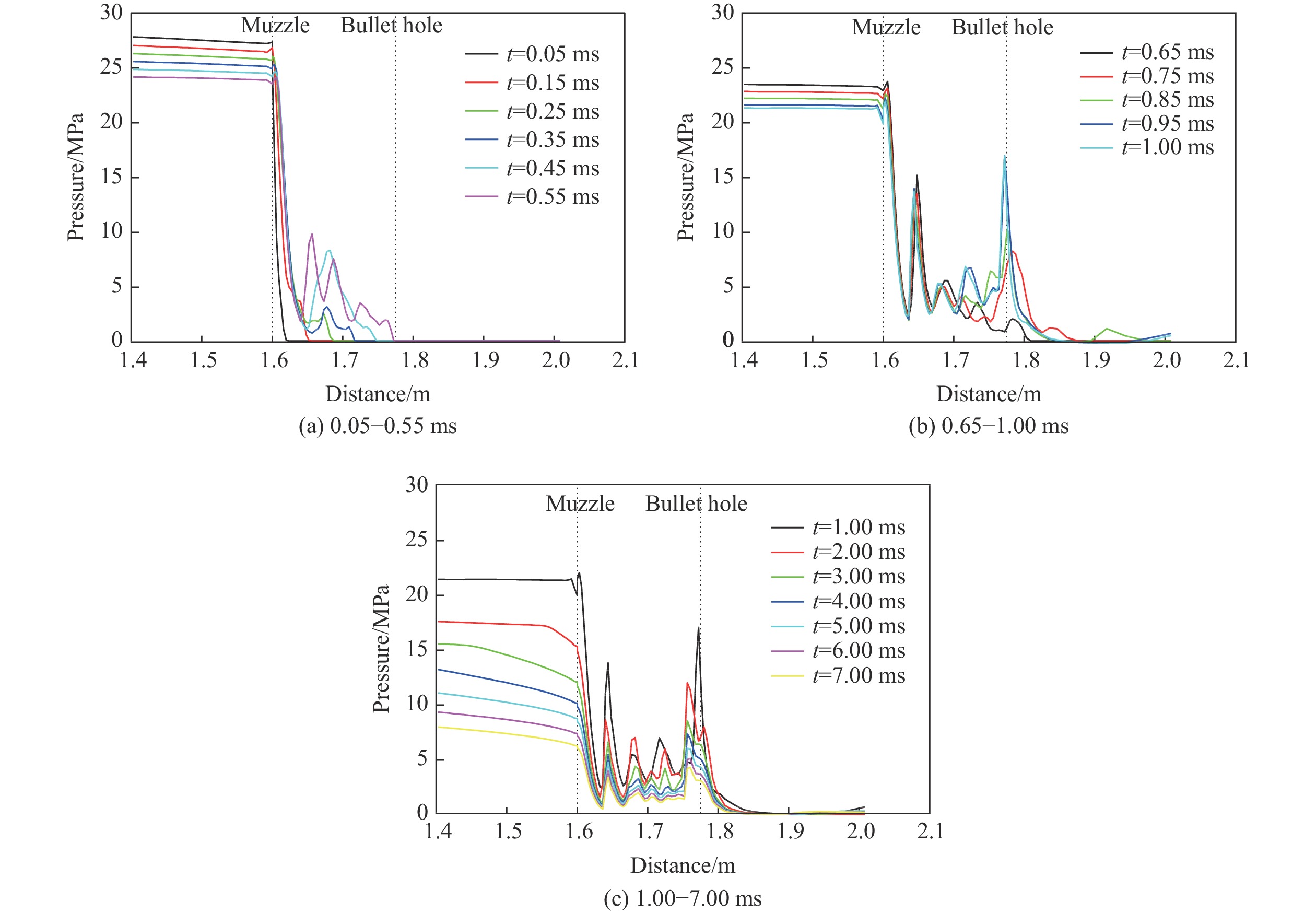
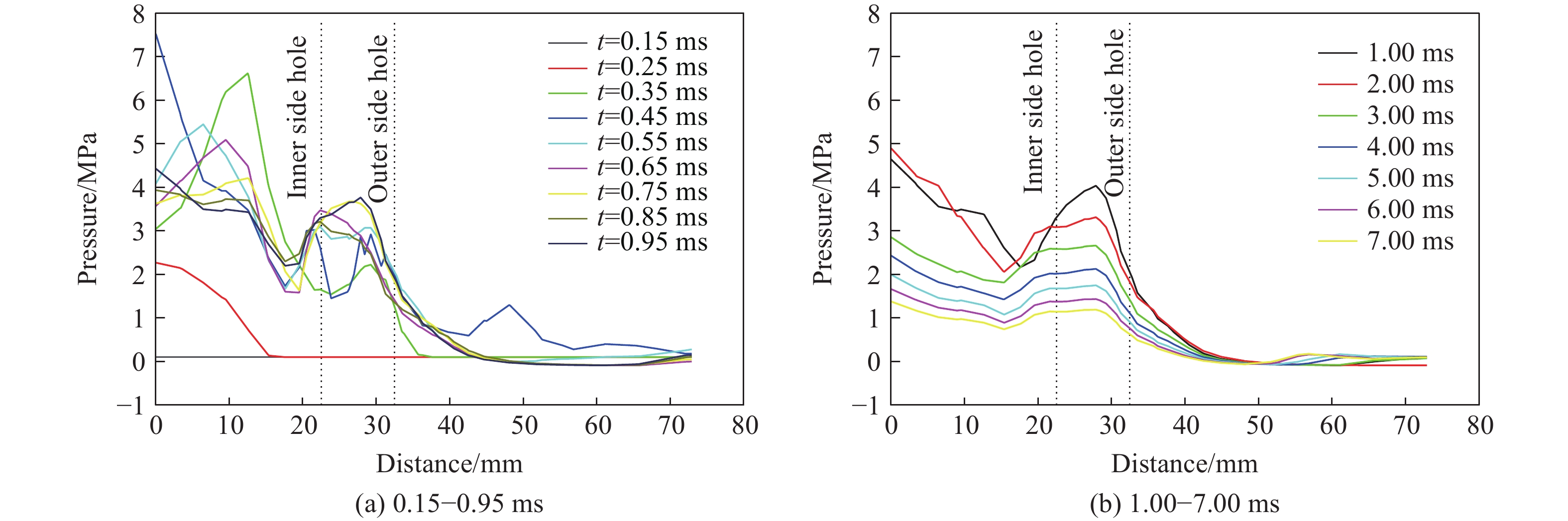
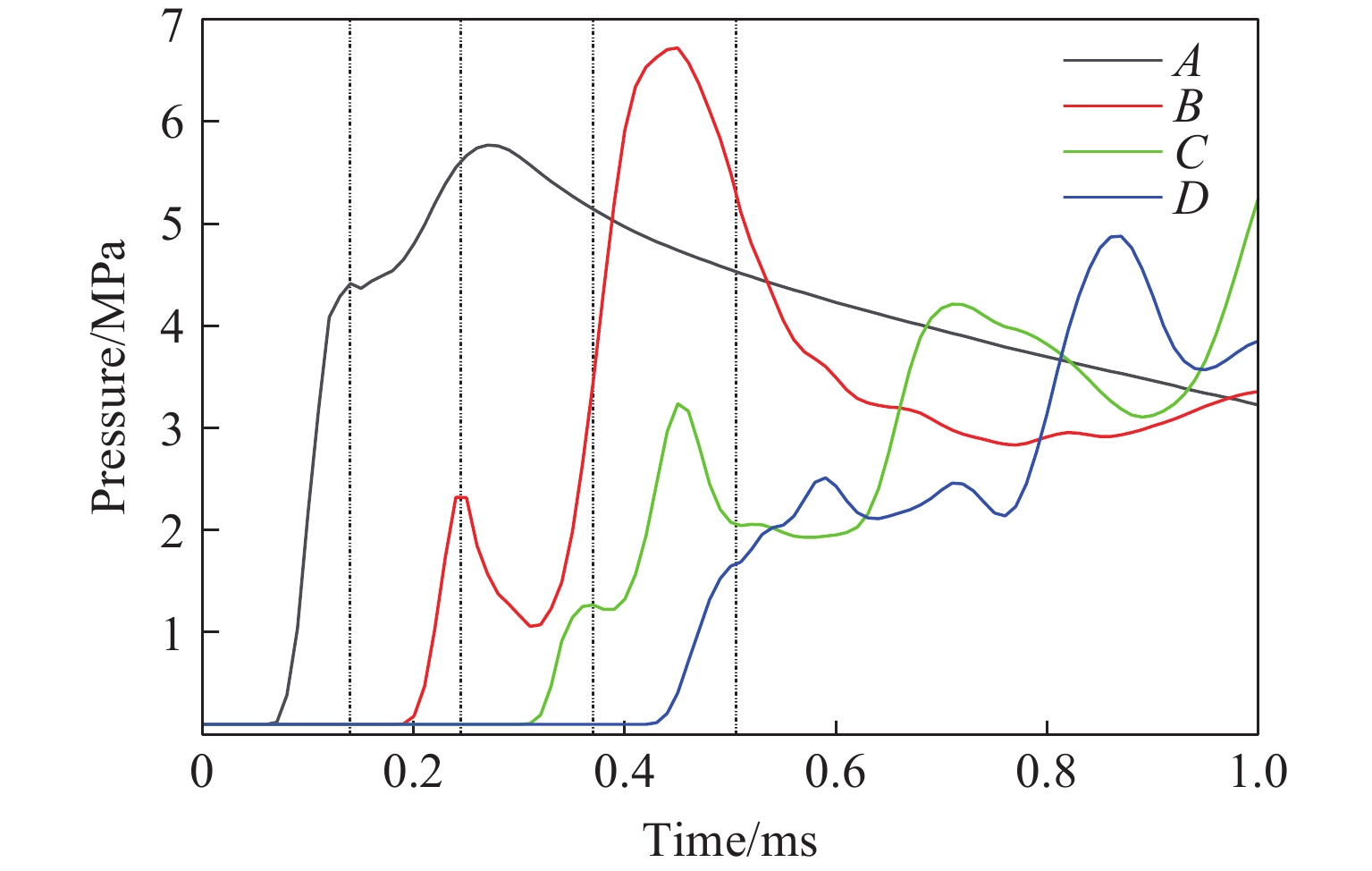
图6和图7为炮轴中心线、侧孔位置径向轴线处不同时刻压力分布曲线。可以看出,火药气体到达弹孔之前,不断提升新型制退器腔室内的压力水平。t=0.65 ms时,火药气体从中央弹孔流出并迅速提升当地压力,直至t=1.00 ms时弹孔处压力峰值超过制退器腔室内压力最大值,见图6(a)和图6(b)。与此同时,径向轴线方向的压力分布呈现随时间剧烈波动的高瞬态特征,见图7(a)。t=1.00 ms之后,火药气体流场逐渐衰减,腔室内外压力水平均逐渐下降(见图6(c)和图7(b)),同时径向轴线方向压力水平也呈现出规则的逐级下降趋势。
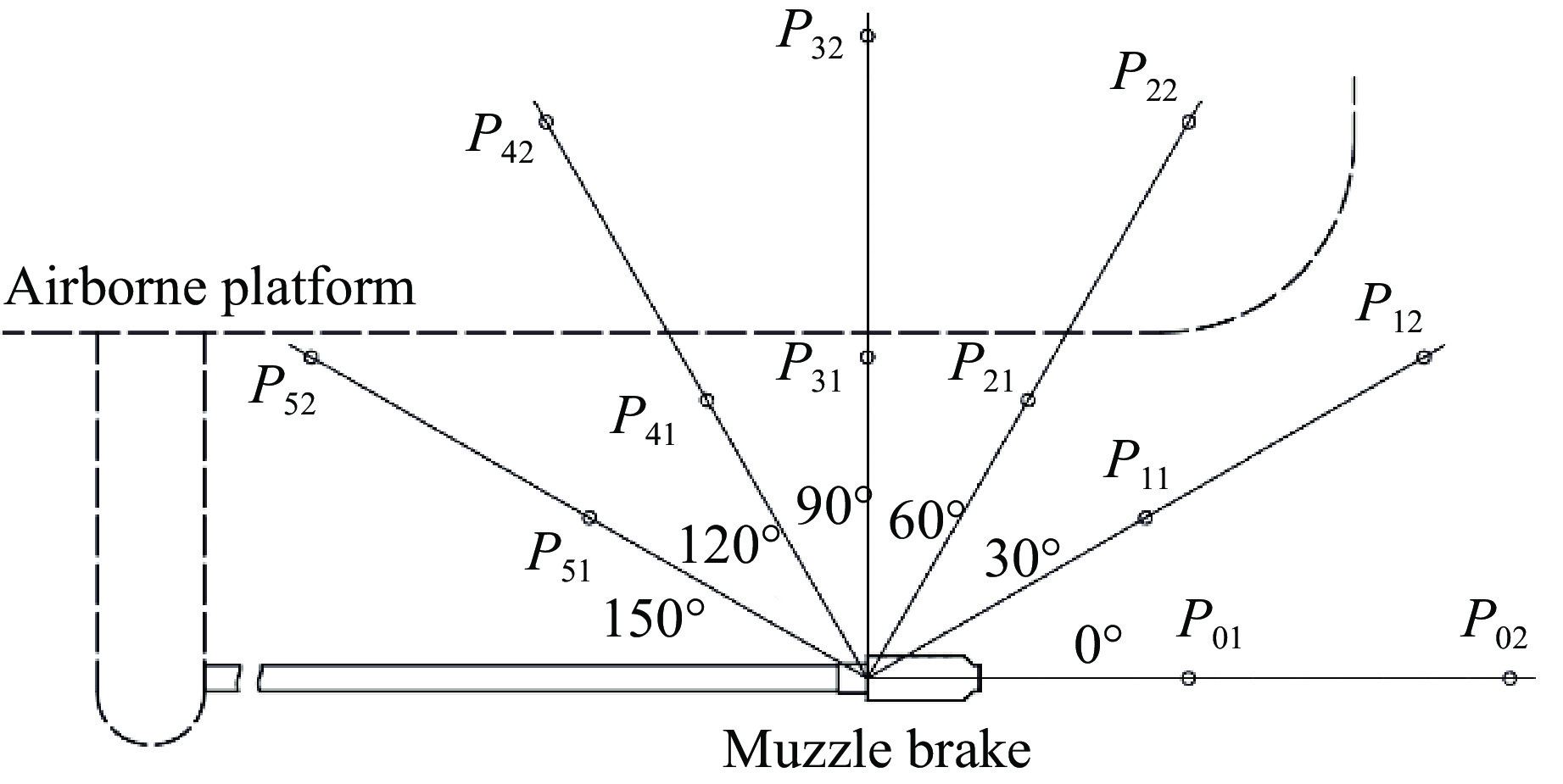
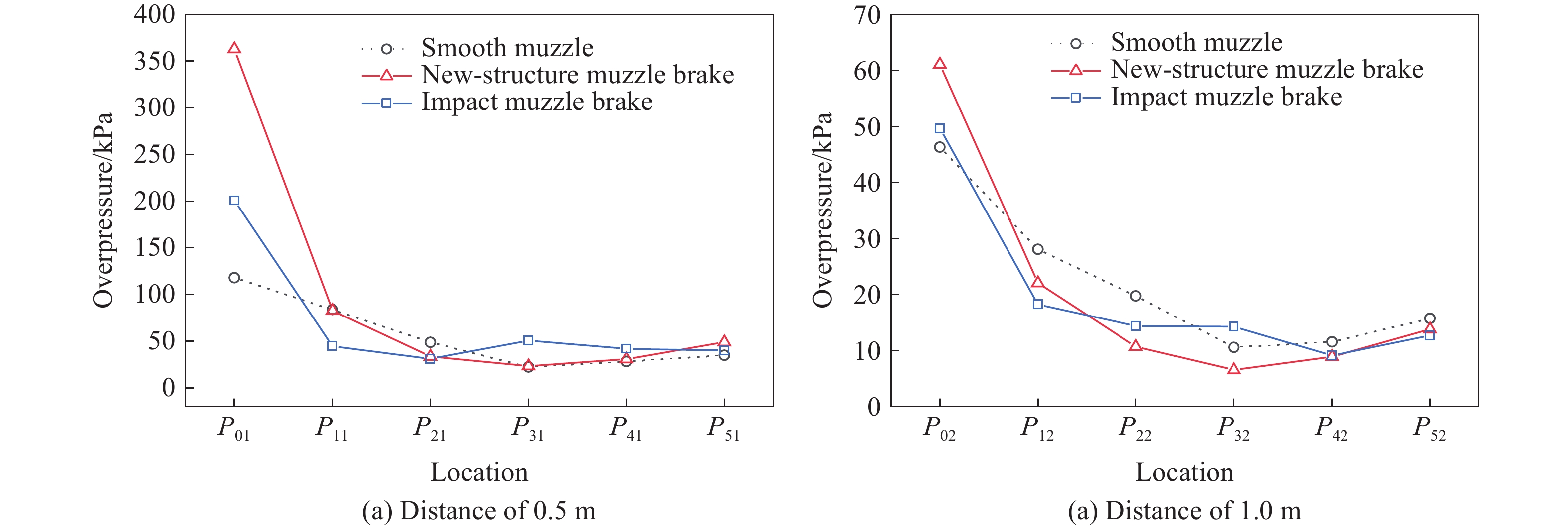
根据机载小口径航炮的实际工况,以炮口端面为中心、炮膛轴线为正方向,在0.5和1.0 m 的周向距离上,炮口区域侧向0°~150°范围内设置若干膛口流场超压监测点,如图8所示,以精确分析加装制退器后炮口区域的超压特征,评估炮口冲击波对机载平台的危害效应。得到的后效期内各点超压峰值监测结果与差值情况如表3所示。其中,超压值代表压力监测值高于环境压力的部分,加装新型制退器与传统冲击式炮口制退器情况下的超压峰值与光膛口情况下的超压峰值差值分别记为Δnew、Δimpact,负值代表产生减小超压效果,正值代表超压增大。同时,根据超压峰值,绘制了不同情况下炮口超压分布,如图9所示。
表 3 不同制退器的超压峰值监测结果Table 3. Overpressure peak values of different muzzle brakesMonitor point Overpressure peak/kPa Δnew/kPa Δimpact/kPa Smooth muzzle New-structure muzzle brake Impact muzzle brake P01 117.84 362.54 200.83 244.70 82.99 P02 46.31 65.46 53.20 19.15 6.89 P11 83.80 79.03 44.58 −4.76 −39.21 P12 28.07 23.61 19.56 −4.46 −8.51 P21 48.84 29.28 30.90 −19.56 −17.93 P22 19.76 11.45 15.40 −8.31 −4.36 P31 22.29 18.85 50.87 −3.45 28.57 P32 10.54 6.99 15.30 −3.55 4.76 P41 28.27 26.45 41.75 −1.82 13.48 P42 11.55 9.54 9.73 −2.01 −1.82 P51 34.75 44.68 40.09 9.93 5.34 P52 15.71 14.79 13.58 −0.91 −2.13 由表3和图9可知,相比于光膛口情况,两种类型制退器的膛口冲击波超压特点可总结如下:(1)炮口正前0°方向,0.5和1.0 m两个距离上两种制退器均造成超压峰值的明显增大,并且新型制退器的增压幅度更大;(2)自炮口侧向30°方向向后,新型制退器膛口冲击波超压峰值呈U形分布,冲击型制退器呈W形分布;(3)30°~120°侧向范围内,新型制退器均产生减小超压效果,各点超压峰值降低幅度为1.82~19.56 kPa,正侧方90°处超压峰值最小,该方位0.5和1.0 m距离处分别为18.85和6.99 kPa;(4)30°~120°侧向范围内,冲击型制退器对应的超压峰值高低不等,在正侧方90°方向0.5和1.0 m距离上均形成增大超压效果,超压峰值分别为50.87和15.30 kPa;(5)150°方向0.5 m处,新型制退器对应的超压峰值略高于冲击型制退器及光膛口情况,1.0 m处同样略高于冲击型制退器但低于光膛口情况。
综上可知,新型制退器的膛口冲击波表现出高超压集中于炮口正前方、低超压集中于正侧方(炮口与机载平台直线距离最近位置处)的分布特征,同时其后侧方的整体超压水平也较好地控制在低于或近似光膛口情况,相比传统冲击型炮口制退器,对于缓解因制退器安装导致的机身及炮口装置的冲击侵害更具优势。同时应该注意到,为了追求高制退效率,新型制退器设置了较大的侧孔后倾角度及向后偏转面积,因此在炮口后侧150°方向0.5 m距离处超压出现一定的增大,说明新型制退器的结构参数仍存在改进空间,以实现高制退效率和更低冲击波危害的进一步平衡。
3.3 制退效率及结构强度
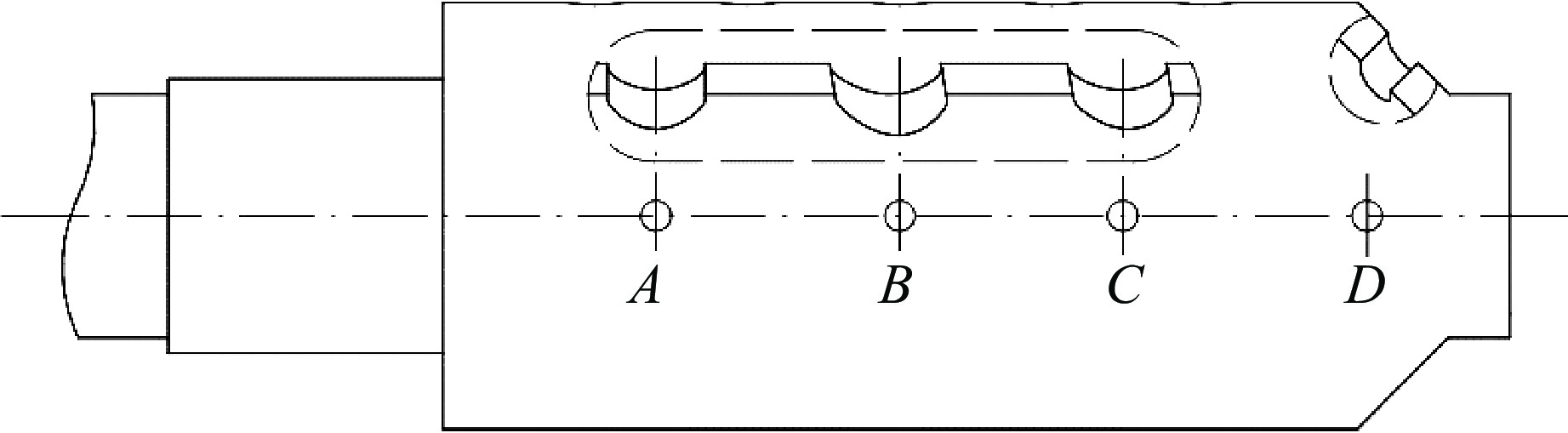
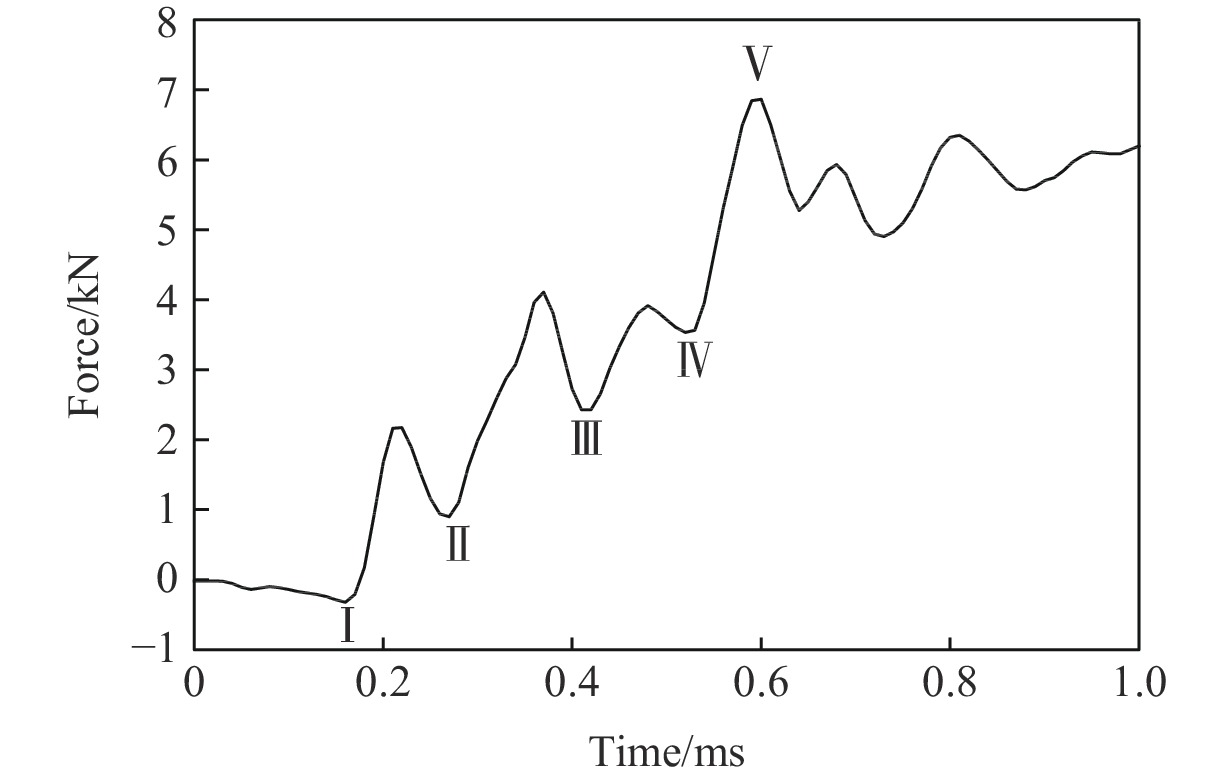
为分析后效期内新型制退器受载变化及制退力产生机制,沿炮膛轴线在新型炮口制退器腔室内层侧孔1~3位置及前端面侧孔处分别设置压力监测点,如图10所示。图11为后效期初期阶段各监测点压力随时间的变化曲线。图12和图13分别为后效期初期制退器受力、后效期全程火炮身管各部分受力随时间的变化曲线。
结合图11和图12可知,火药气体的流出和偏转作用不断产生对火炮身管的后坐力和向前的制退力,后效期初期制退器受力曲线呈现阶梯式上升趋势。图12中点Ⅰ~点Ⅳ处的抬升显示了当火药气体依次流经制退器内层侧孔时逐级产生的冲撞和制退效果。火药气体流出中央弹孔之前,对制退器前端面的冲击作用使制退器受力曲线继续抬升,直至达到峰值点Ⅴ,此后由于火药气体对制退器外壁面产生反向冲击作用,又使制退器的正向受力水平略降至峰值以下。
依据仿真监测结果,首先由总受力F随时间的变化曲线计算得到后效期火药气体对身管的总冲量I,再结合动量守恒定律及冲量定律,计算得到后效期起始时刻身管自由后坐速度v、后效期结束时刻身管最大后坐速度vmax以及火炮后坐动能E,进而依据定义得到制退器的效率
η ,具体计算公式为v=mq+0.5mwM+mw+mqvd (8) I=∫τ0Fdt=M(vmax−v) (9) E=Mv2max2 (10) η=E0−EE0×100% (11) 式中:
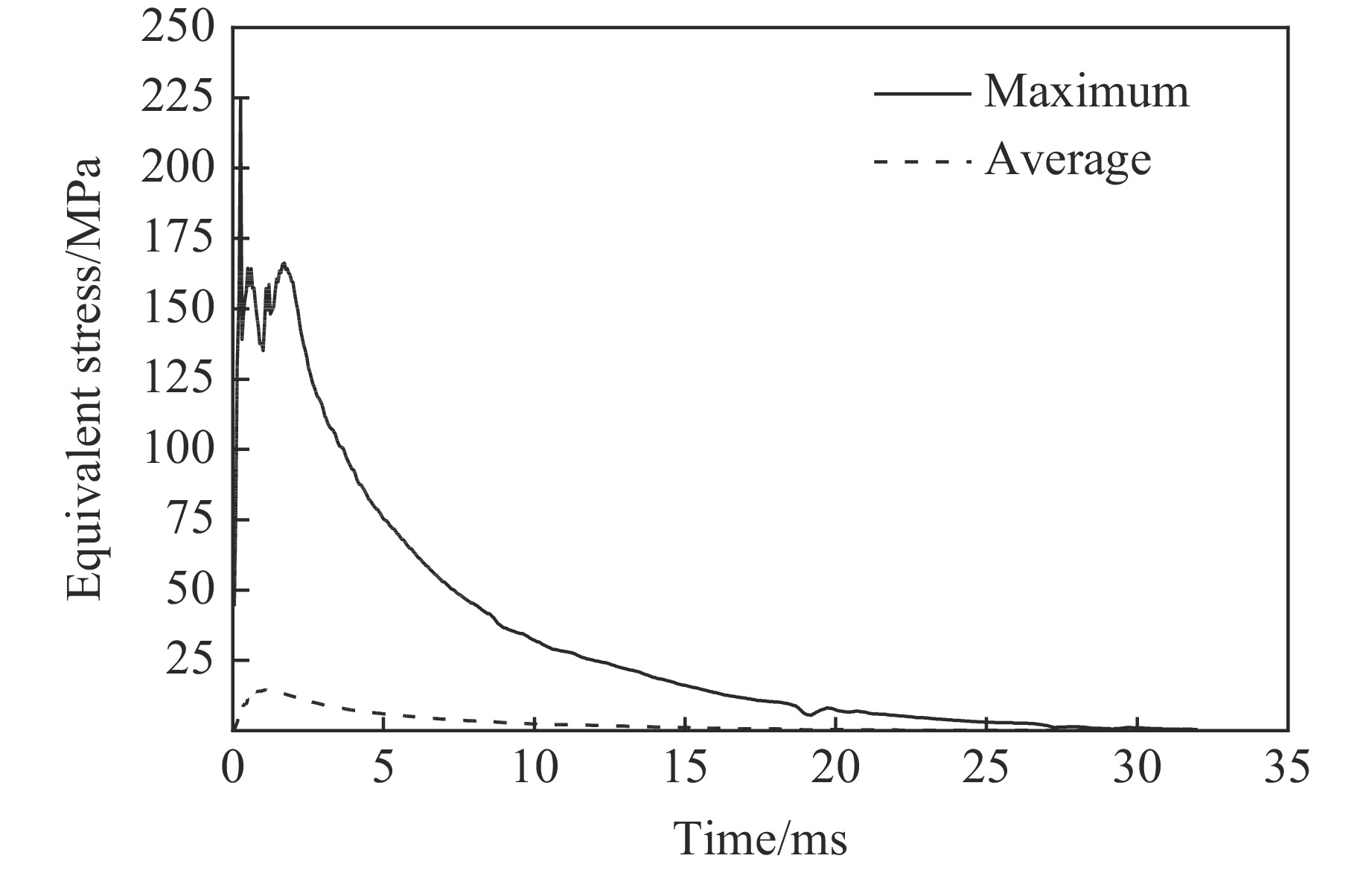
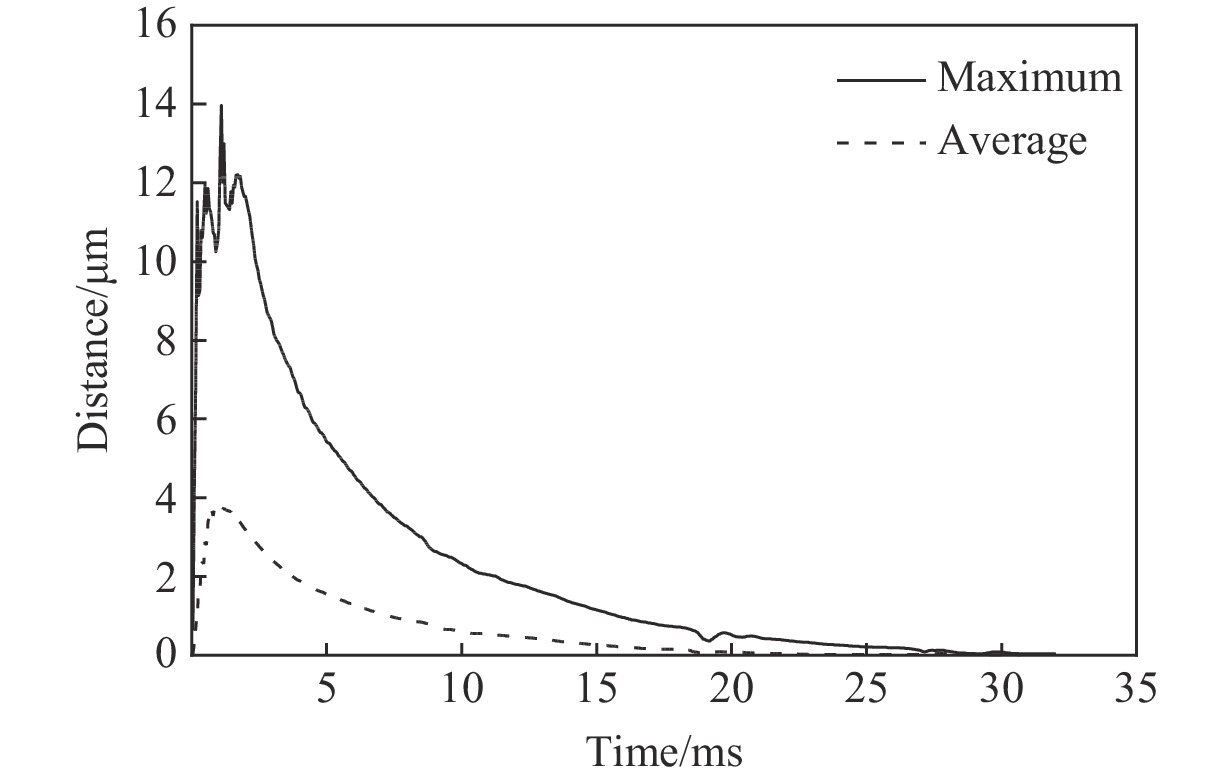
τ 为后效期持续时间,E0为光膛口时火炮后坐动能,M为加装不同制退器后身管总质量。本算例中,设光膛口时火炮身管质量为7.09 kg,各种情况下的计算结果如表4所示,其中负号代表火炮发射相反方向。可见,新型制退器的制退效率达到45.62%,满足小口径炮口制退器的使用要求,并略超过以高效率为特点的传统冲击式炮口制退器性能水平。不过,新型炮口制退器情况下火药气体对膛口装置的反作用冲量相比传统冲击式炮口制退器的提升不明显,说明新型炮口制退器在内部结构参数上还有优化空间,可以进一步提升新结构形式的制退性能。表 4 制退器效率对比Table 4. Comparison of efficiency for different muzzle brakesStructure M/kg I/(N·s) v/(m·s−1) vmax/(m·s−1) E/J η/% Smooth muzzle 7.09 −123.68 −47.00 −64.45 14719.0 New-structure muzzle brake 8.37 −29.40 −40.23 −43.74 8003.2 45.62 Impact muzzle brake 8.10 −26.15 −41.47 −44.70 8091.3 45.03 制退器与后效期火药气体相互作用过程中,其本身所承受的动态载荷作用通过流固耦合技术由流体计算结果传递至制退器各壁面,并通过瞬态动力学有限元计算方法求得变形与等效应力随时间的变化曲线,如图14和图15所示。后效期初期,高温高压的火药燃气出膛后迅速膨胀,在剧烈变化的流体压力动态载荷作用下,制退器所承受的等效应力及其变形也在短时间内迅速抬升达到峰值;约2 ms以后,随着流场的充分发展并衰减,制退器结构的应力及变形转为逐渐下降趋势。
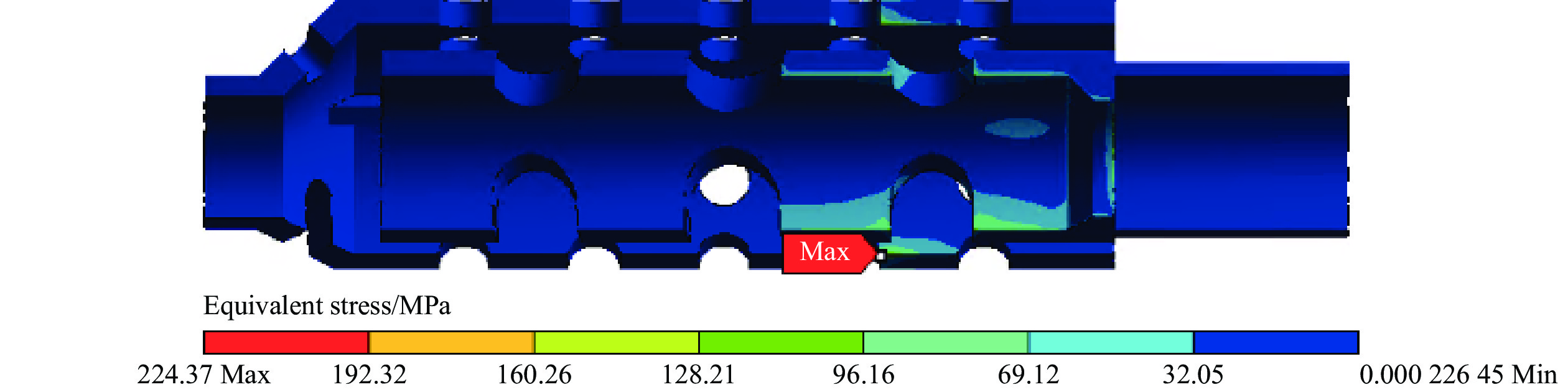
图16和图17分别为新型制退器的等效应力和变形在其峰值时刻的分布云图。t=0.25 ms时,火药气体首先对炮口端面处的侧孔产生冲击,并在该时刻造成距离炮口端面第2排外层圆孔处出现全局最大范式等效应力,为224.37 MPa。t=1.10 ms时,火药气体已从中央弹孔流出,火药气体对制退器前端面的冲击作用使得最大变形出现在前端面与中央弹孔的结合处,约为14 μm,其他部位的变形呈现从炮口端面沿炮口正向递减趋势。由以上结果可知,后效期内制退器结构的最大应力小于钛合金材料许用应力,满足结构强度的使用要求。
4. 结 论
提出了一种叠加冲击式内壁与反作用式外孔特征的新型小口径钛合金炮口制退器结构方案,基于膛口流场数值仿真、流固耦合技术等对其性能进行分析,并与传统冲击型炮口制退器进行对比。结果表明:新型结构方案满足小口径炮口制退器性能要求,制退效率相比传统冲击型制退器略有提高;在机载航炮应用背景下,其膛口冲击波超压分布特征及峰值水平相比传统冲击型制退器具有更好的机身与炮口装置防护优势和低冲击波危害效益,同时结构强度满足使用要求。新型结构方案对于新材料和新制造技术背景下炮口制退器的创新设计和发展具有参考意义。本研究所展示的新型制退器结构参数在高效率和更低负面危害的平衡上具有进一步改进与优化空间。研究过程中膛口流场数值模拟存在一定简化,仿真结果与实际情况存在一定偏差。另外,由于后效期膛口火药气体具有高温属性,一般借助防烧蚀涂层等技术强化钛合金装置的高温使用性能,因此未在结构强度分析中考虑热耦合效应。
-
图 2 6种结构在稳定压力区间的声子谱:(a) 零压下NaCl相Ⅰ,(b) 30.6 GPa下NaCl相Ⅱ,(c) 零压下KCl相Ⅰ,(d) 2.12 GPa下KCl相Ⅱ,(e) 零压下KBr相Ⅰ,(f) 2.20 GPa下KBr相Ⅱ
Figure 2. Phonon spectra for six structures in the stable pressure range: (a) NaCl phase Ⅰ at zero pressure; (b) NaCl phase Ⅱ at 30.6 GPa; (c) KCl phase Ⅰ at zero pressure; (d) KCl phase Ⅱ at 2.12 GPa; (e) KBr phase Ⅰ at zero pressure; (f) KBr phase Ⅱ at 2.20 GPa
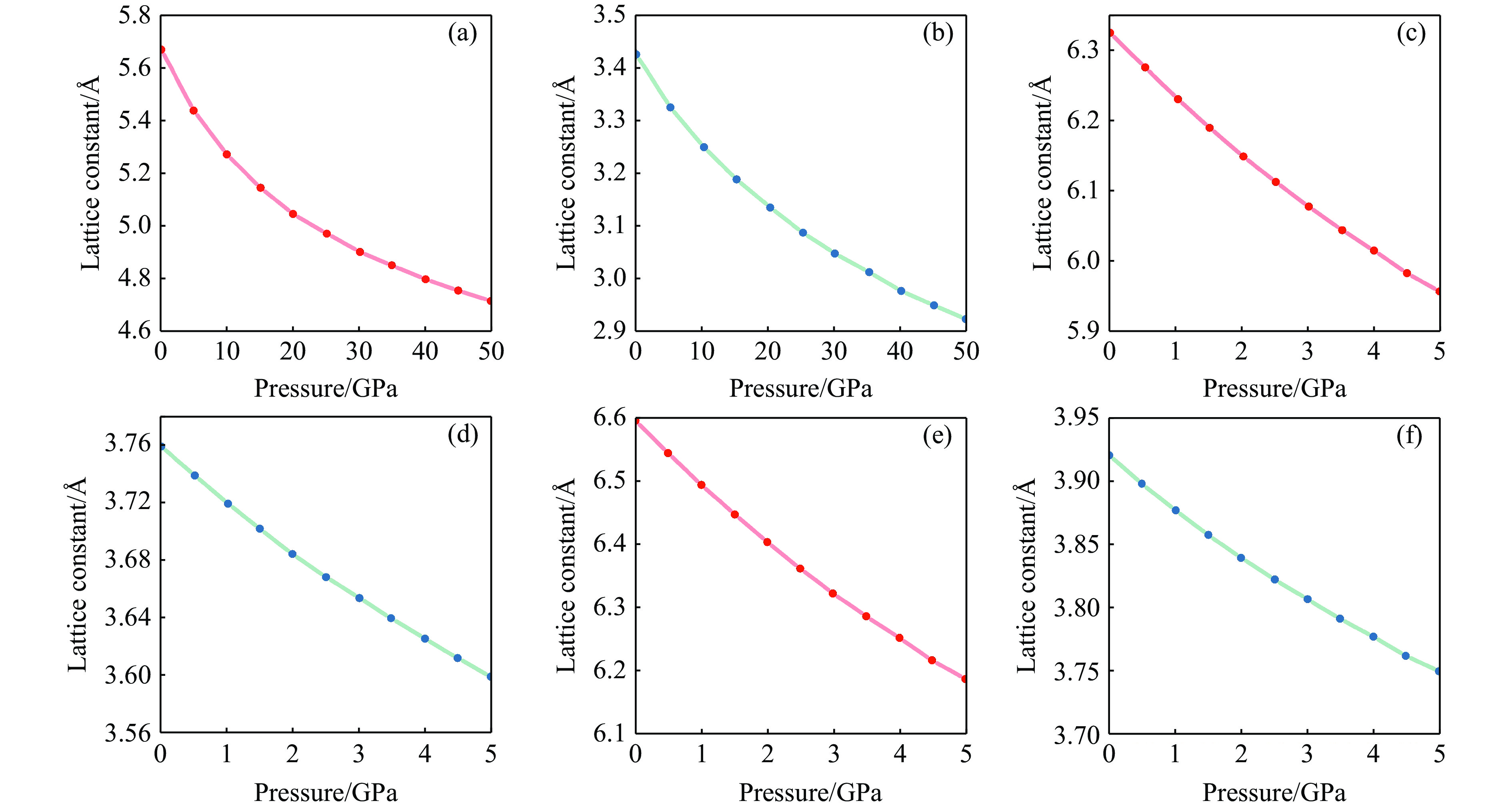
图 3 压力下6种结构的晶格常数a的变化:(a) 0~50 GPa下NaCl的相Ⅰ,(b) 0~50 GPa下NaCl的相Ⅱ,(c) 0~5 GPa下KCl的相Ⅰ,(d) 0~5 GPa下KCl的相Ⅱ,(e) 0~5 GPa下KBr的相Ⅰ,(f) 0~5 GPa下KBr的相Ⅱ
Figure 3. Lattice parameter a changes for six structures under pressure: (a) NaCl phase Ⅰ at 0−50 GPa; (b) NaCl phase Ⅱ at 0−50 GPa; (c) KCl phase Ⅰ at 0−5 GPa; (d) KCl phase Ⅱ at 0−5 GPa; (e) KBr phase Ⅰ at 0−5 GPa; (f) KBr phase Ⅱ at 0−5 GPa
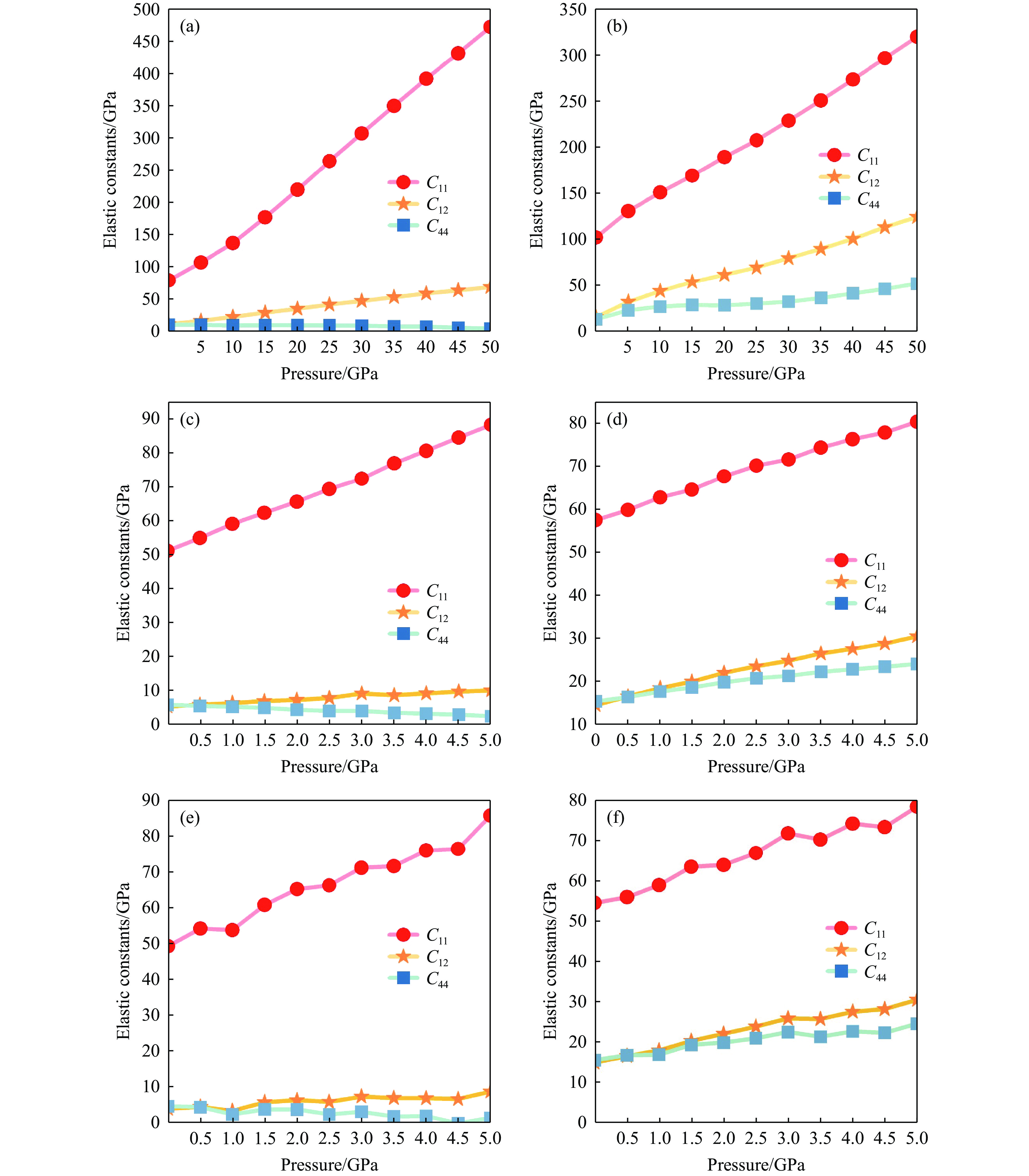
图 4 压力下6种结构的独立弹性常数:(a) 0~50 GPa下NaCl的相Ⅰ,(b) 0~50 GPa下NaCl的相Ⅱ,(c) 0~5 GPa下KCl的相Ⅰ,(d) 0~5 GPa下KCl的相Ⅱ,(e) 0~5 GPa下KBr的相Ⅰ,(f) 0~5 GPa下KBr的相Ⅱ
Figure 4. Independent elastic constants for six structures under pressure: (a) NaCl phase Ⅰ at 0−50 GPa; (b) NaCl phase Ⅱ at 0−50 GPa; (c) KCl phase Ⅰ at 0−5 GPa; (d) KCl phase Ⅱ at 0−5 GPa; (e) KBr phase Ⅰ at 0−5 GPa; (f) KBr phase Ⅱ at 0−5 GPa
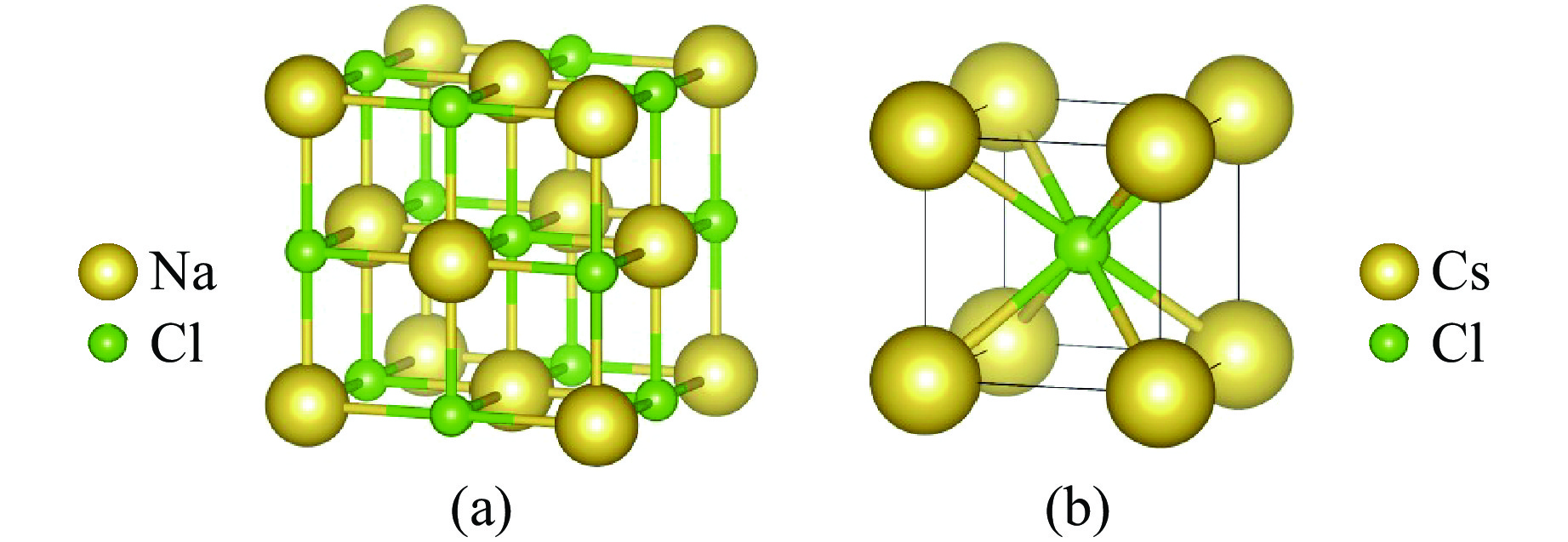
表 1 NaCl、KCl、KBr的相Ⅰ和相Ⅱ结构的晶格常数和原胞体积
Table 1. Lattice constant and cell volumes for phase Ⅰ and phase Ⅱ structures of NaCl, KCl and KBr
Material Phase Space group p/GPa a/Å V0/Å3 Method NaCl Ⅰ Fm¯3m 0 5.672 45.637 This work 0 5.640 44.830 Experiment[18] NaCl Ⅱ Pm¯3m 30.6 3.043 28.186 This work 30.6 3.010 27.271 Experiment[15] KCl Ⅰ Fm¯3m 0 6.325 63.274 This work 0 6.294 62.333 Experiment[19] KCl Ⅱ Pm¯3m 2.12 3.681 49.864 This work 2.12 3.670 49.431 Experiment[20] KBr Ⅰ Fm¯3m 0 6.598 71.826 This work 0 6.586 71.418 Experiment[21] KBr Ⅱ Pm¯3m 2.20 3.833 56.293 This work 2.20 3.740 52.314 Experiment[22] 表 2 NaCl、KCl、KBr的相Ⅰ(零压)和相Ⅱ(稳定压力)结构的独立弹性常数
Table 2. Independent elastic constants for phase Ⅰ (at zero pressure) and phase Ⅱ (at steady pressure) of NaCl, KCl and KBr
Material Phase C11/GPa C12/GPa C44/GPa NaCl Ⅰ 78.642 9.548 10.509 Ⅱ 230.431 79.373 31.849 KCl Ⅰ 51.087 5.107 5.587 Ⅱ 67.763 21.993 19.573 KBr Ⅰ 49.338 3.959 4.636 Ⅱ 65.451 22.265 19.708 表 3 NaCl、KCl、KBr的相Ⅰ和相Ⅱ结构的声子谱虚频统计
Table 3. Statistics of imaginary frequencies in the phase Ⅰ and phase Ⅱ phonon spectra for NaCl, KCl and KBr
Pressrue/GPa Imaginary frequency NaCl
(Phase Ⅰ)NaCl
(Phase Ⅱ)KCl
(Phase Ⅰ)KCl
(Phase Ⅱ)KBr
(Phase Ⅰ)KBr
(Phase Ⅱ)0 F F F F F F 0.5 F F F F F F 1.0 T F F F F F 1.5 T F F F F F 2.0 T F F F F F 2.5 T F F F F F 3.0 T F F F F F 3.5 T F F F F F 4.0 T F F F F F 4.5 T F F F F F 5.0 T F F F F F Note: “T” is imaginary frequency, while “F” is not imaginary frequency. -
[1] NATTLAND D, HEYER H, FREYLAND W. Metal-nonmetal transition in liquid alkali metal-alkalihalide melts: electrical conductivity and optical reflectivity study [J]. Zeitschrift für Physikalische Chemie, 1986, 149(1): 1–15. doi: 10.1524/zpch.1986.149.1.001 [2] 张程祥, 吴绍曾, 吴姝妍, 等. NaCl、KCl和NaF晶体高压结构相变点的计算 [J]. 高压物理学报, 1993, 7(3): 233–237. doi: 10.11858/gywlxb.1993.03.011ZHANG C X, WU S Z, WU S Y, et al. Calculations of the high pressure phase transition point of NaCl, KCl and NaF crystals [J]. Chinese Journal of High Pressure Physics, 1993, 7(3): 233–237. doi: 10.11858/gywlxb.1993.03.011 [3] 孙力, 王永生, 何志谊, 等. 电子俘获材料KCl: Eu2+的光存储特性研究 [J]. 激光与红外, 2000, 30(2): 117–118, 120. doi: 10.3969/j.issn.1001-5078.2000.02.016SUN L, WANG Y S, HE Z Y, et al. Optical storage properties of electron trapping material KCl: Eu2+ [J]. Laser & Infrared, 2000, 30(2): 117–118, 120. doi: 10.3969/j.issn.1001-5078.2000.02.016 [4] 崔守鑫, 蔡灵仓, 胡海泉, 等. 氯化钠晶体在高温高压下热物理参数的分子动力学计算 [J]. 物理学报, 2005, 54(6): 2826–2831. doi: 10.7498/aps.54.2826CUI S X, CAI L C, HU H Q, et al. Molecular dynamics simulation for thermophysical parameters of sodium chloride solids at high temperature and high pressure [J]. Acta Physica Sinica, 2005, 54(6): 2826–2831. doi: 10.7498/aps.54.2826 [5] 郑东阳, 刘贺, 李春, 等. 大截面溴化钾晶体生长工艺研究 [J]. 硅酸盐学报, 2015, 43(1): 60–64. doi: 10.14062/j.issn.0454-5648.2015.01.09ZHENG D Y, LIU H, LI C, et al. Growth process of large section crystal of potassium bromide [J]. Journal of the Chinese Ceramic Society, 2015, 43(1): 60–64. doi: 10.14062/j.issn.0454-5648.2015.01.09 [6] JIANG W, YIN L, CHEN H M, et al. NaCl nanoparticles as a cancer therapeutic [J]. Advanced Materials, 2019, 31(46): 1904058. doi: 10.1002/adma.201904058 [7] BANGARU S, MURALIDHARAN G, BRAHMANANDHAN G M. Thermoluminescence and optical studies on X-irradiated terbium-doped potassium bromide crystals [J]. Journal of Luminescence, 2010, 130(4): 618–622. doi: 10.1016/j.jlumin.2009.11.005 [8] BANGARU S, RAVI D, SARADHA K. Comparison of luminescence property of gamma-ray irradiated Tb3+-doped and Ce3+ Co-doped potassium halide single crystals [J]. Luminescence, 2017, 32(3): 358–363. doi: 10.1002/bio.3187 [9] BANGARU S, SARADHA K, MURALIDHARAN G. Thermoluminescence and photoluminescence studies on γ-ray-irradiated Ce3+, Tb3+-doped potassium chloride single crystals [J]. Luminescence, 2016, 31(3): 649–653. doi: 10.1002/bio.3005 [10] OKADA G, FUJIMOTO Y, TANAKA H, et al. Dynamics of radio-photoluminescence and thermally-stimulated luminescence in KBr: Sm [J]. Journal of Materials Science: Materials in Electronics, 2017, 28(21): 15980–15986. doi: 10.1007/s10854-017-7496-z [11] PERDEW J P, BURKE K, ERNZERHOF M. Generalized gradient approximation made simple [J]. Physical Review Letters, 1996, 77(18): 3865–3868. doi: 10.1103/PhysRevLett.77.3865 [12] PERDEW J P, CHEVARY J A, VOSKO S H, et al. Erratum: atoms, molecules, solids, and surfaces: applications of the generalized gradient approximation for exchange and correlation [J]. Physical Review B, 1993, 48(7): 4978. doi: 10.1103/PhysRevB.48.4978.2 [13] PERDEW J P, BURKE K, ERNZERHOF M. Generalized gradient approximation made simple [Phys. Rev. Lett. 77, 3865 (1996)] [J]. Physical Review Letters, 1997, 78(7): 1396. doi: 10.1103/PhysRevLett.78.1396 [14] GRIMME S. Semiempirical GGA-type density functional constructed with a long-range dispersion correction [J]. Journal of Computational Chemistry, 2006, 27(15): 1787–1799. doi: 10.1002/jcc.20495 [15] LIU L G, BASSETT W A. Compression of Ag and phase transformation of NaCl [J]. Journal of Applied Physics, 1973, 44(4): 1475–1479. doi: 10.1063/1.1662396 [16] PRABHAKAR N V K, SINGH R K, GAUR N K, et al. High-pressure phase transitions in some alkali halides [J]. Journal of Physics: Condensed Matter, 1990, 2(15): 3445–3449. doi: 10.1088/0953-8984/2/15/002 [17] RAMOLA Y, LOUIS C N, AMALRAJ A. Electronic band structure, density of states, phase transitions, metallization and superconducting transition of KBr under high pressure [J]. Orbital: the Electronic Journal of Chemistry, 2020, 12(2): 69–75. doi: 10.17807/orbital.v12i2.1401 [18] BASSETT W A, TAKAHASHI T, MAO H K, et al. Pressure-induced phase transformation in NaCl [J]. Journal of Applied Physics, 1968, 39(1): 319–325. doi: 10.1063/1.1655752 [19] COHEN A J, GORDON R G. Theory of the lattice energy, equilibrium structure, elastic constants, and pressure-induced phase transitions in alkali-halide crystals [J]. Physical Review B, 1975, 12(8): 3228–3241. doi: 10.1103/PhysRevB.12.3228 [20] LIVSHITS L D, RYABININ Y N, LARIONOV L V. Phase transitions of potassium chloride and its alloys with rubidium chloride at high pressures [J]. Soviet Physics Technical Physics, 1969, 14: 837. [21] LEIBSSLE H. Die piezooptischen eigenschaften einiger alkalihalogenide des steinsalz-typus [J]. Zeitschrift für Kristallographie: Crystalline Materials, 1960, 114(1): 457–467. doi: 10.1524/zkri.1960.114.16.457 [22] WEIR C E, PIERMARINI G J. Lattice parameters and lattice energies of high-pressure polymorphs of some alkali halides [J]. Journal of Research of the National Bureau of Standards A, 1964, 68A(1): 105–111. doi: 10.6028/jres.068A.009 [23] 高娟, 刘其军, 蒋城露, 等. 7大晶系的力学稳定性判据及其应用: 以SiO2为例 [J]. 高压物理学报, 2022, 36(5): 051101. doi: 10.11858/gywlxb.20220575GAO J, LIU Q J, JIANG C L, et al. Criteria of mechanical stability of seven crystal systems and its application: taking silica as an example [J]. Chinese Journal of High Pressure Physics, 2022, 36(5): 051101. doi: 10.11858/gywlxb.20220575 [24] GAO J, LIU Q J, TANG B. Elastic stability criteria of seven crystal systems and their application under pressure: taking carbon as an example [J]. Journal of Applied Physics, 2023, 133(13): 135901. doi: 10.1063/5.0139232 -








 下载:
下载:

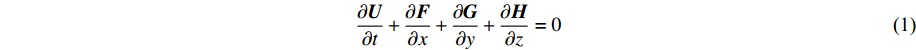
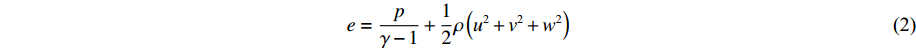
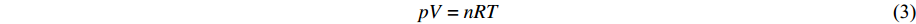
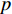
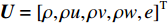
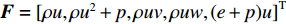

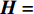
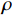
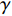
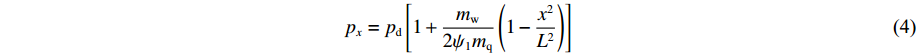
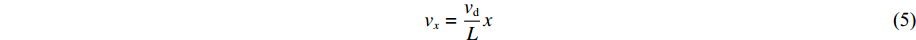
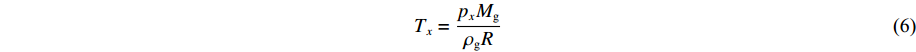
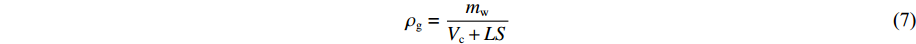
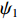











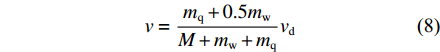
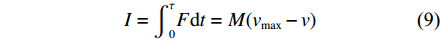
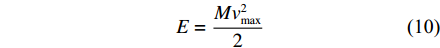
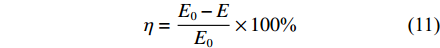




 下载:
下载: