磁驱动固体套筒实验模拟中的电流系数

阚明先; 陈涵; 吴凤超; 贾月松; 张南川; 傅贞; 段书超

doi:10.11858/gywlxb.20240844

磁驱动固体套筒实验模拟中的电流系数

doi: 10.11858/gywlxb.20240844

中国工程物理研究院流体物理研究所, 四川绵阳　621999

基金项目: 国家自然科学基金（12075226）

详细信息

作者简介:
阚明先（1971－），男，硕士，副研究员，主要从事磁流体力学数值方法、程序开发、磁驱动实验模拟及理论研究. E-mail：kanmx@caep.cn

中图分类号: O521.3; O361.3
计量
- 文章访问数: 152
- HTML全文浏览量: 62
- PDF下载量: 29
出版历程
- 收稿日期: 2024-07-04
- 修回日期: 2024-09-20
- 网络出版日期: 2024-10-23
- 刊出日期: 2024-01-05

Current Coefficient Law in Simulation of Magnetically Driven Solid Liner Experiment

Institute of Fluid Physics, China Academy of Engineering Physics, Mianyang 621999, Sichuan, China

摘要

摘要: 采用不可压缩理论模型，对FP-2装置上开展的磁驱动固体套筒实验进行了模拟分析。模拟结果表明，无论是二维磁流体力学理论模型，还是其他不可压缩理论模型，回流罩结构磁驱动固体套筒的边界磁感应强度公式中都包含一个小于1的套筒电流系数。对不同套筒厚度、不同套筒半径条件下磁驱动固体套筒实验的电流系数进行了模拟，发现电流系数不仅与套筒内半径有关，还与套筒厚度有关；套筒内半径越大，套筒电流系数越小；套筒厚度越大，套筒电流系数越小。准确掌握磁驱动固体套筒电流系数的变化规律，可使磁流体程序从磁驱动固体套筒实验的后验模拟发展为精确预测，使磁流体力学模型真正具备正确设计和指导磁驱动固体套筒相关实验的理论能力。
- 磁驱动固体套筒实验 /
- 二维磁驱动数值模拟程序 /
- 磁流体力学 /
- 电流系数 /
- 回流罩结构
Abstract: The magnetically driven solid liner experiments on the FP-2 device were simulated and analyzed based on the incompressible theoretical model. The simulation results show that the boundary magnetic induction strength formula for the magnetically driven solid liner of the reflux hood structure contains a liner current coefficient of less than 1, regardless of whether it is a two-dimensional magnetohydrodynamic (MHD) theoretical model or other incompressibility theoretical models. The current coefficient law of the magnetically driven solid liner experiment on the FP-2 was studied by simulating the magnetically driven solid liner experiment with different thickness and radii. The current coefficient of magnetically driven solid liner experiment is not only related to the liner’s inner radius, but also to the liner’s thickness. The larger the inner radius of the liner, the smaller the current coefficient, and the larger the thickness of the liner, the smaller the current coefficient. Exploring the current coefficient law in magnetically driven solid liner experiment with reflux hood structure can make the MHD code develop from post-simulation to accurate prediction. And the MHD theoretical model can be employed to design correctly and guide the related experiments of magnetically driven solid liner with a reflux hood structure.
- magnetically driven solid liner experiment /
- two-dimensional magnetically driven simulation code /
- magnetohydrodynamics /
- current coefficient /
- reflux hood structure

HTML全文

磁驱动固体套筒内爆是指电流通过金属套筒表面时，在洛仑兹力的作用下金属套筒径向向内箍缩内爆的物理过程。1973年，Turchi等^[1]首次提出磁驱动固体套筒内爆的概念。自20世纪90年代以来，磁驱动固体套筒实验被广泛应用于高压状态方程^[2]、材料本构^[3]、层裂损伤^[4]、磁瑞利-泰勒（Magneto-Rayleigh-Taylor，MRT）不稳定性发展^[5–6]、Richtmyer-Meshkov（RM）不稳定性发展^[7]等研究。

磁驱动固体套筒实验涉及热扩散、磁扩散、焦耳加热、弹塑性、断裂、层裂等物理过程，并伴有大变形、界面不稳定性等现象。磁驱动固体套筒理论有薄壳模型^[8–10]、不可压缩模型^[11–13]、电作用量-速度模型^[14–15]、全电路模型^[15]和磁流体力学模型^[16–17]等。这些理论模型已被用于脉冲功率装置、磁驱动固体套筒实验的模拟、设计和研究^[7–17]。阚明先等^[17]采用二维磁流体力学程序MDSC2模拟回流罩结构磁驱动固体套筒实验时发现，根据回流罩结构磁驱动固体套筒实验测量的电流或回路电流不能直接模拟磁驱动固体套筒，模拟的套筒速度总是比测量速度大，即回路电流并不完全从固体套筒表面流过。回路电流与固体套筒上通过的电流之间存在一个电流系数。由于MDSC2程序^[17]以外的理论计算或数值模拟都未提到电流系数，因此，本研究采用其他理论模型对磁驱动固体套筒实验进行模拟，分析回路电流与通过固体套筒的电流之间的关系，通过模拟分析不同回流罩结构固体套筒实验，进一步探讨磁驱动固体套筒实验中电流系数的影响因素和变化规律。

1. 负载结构

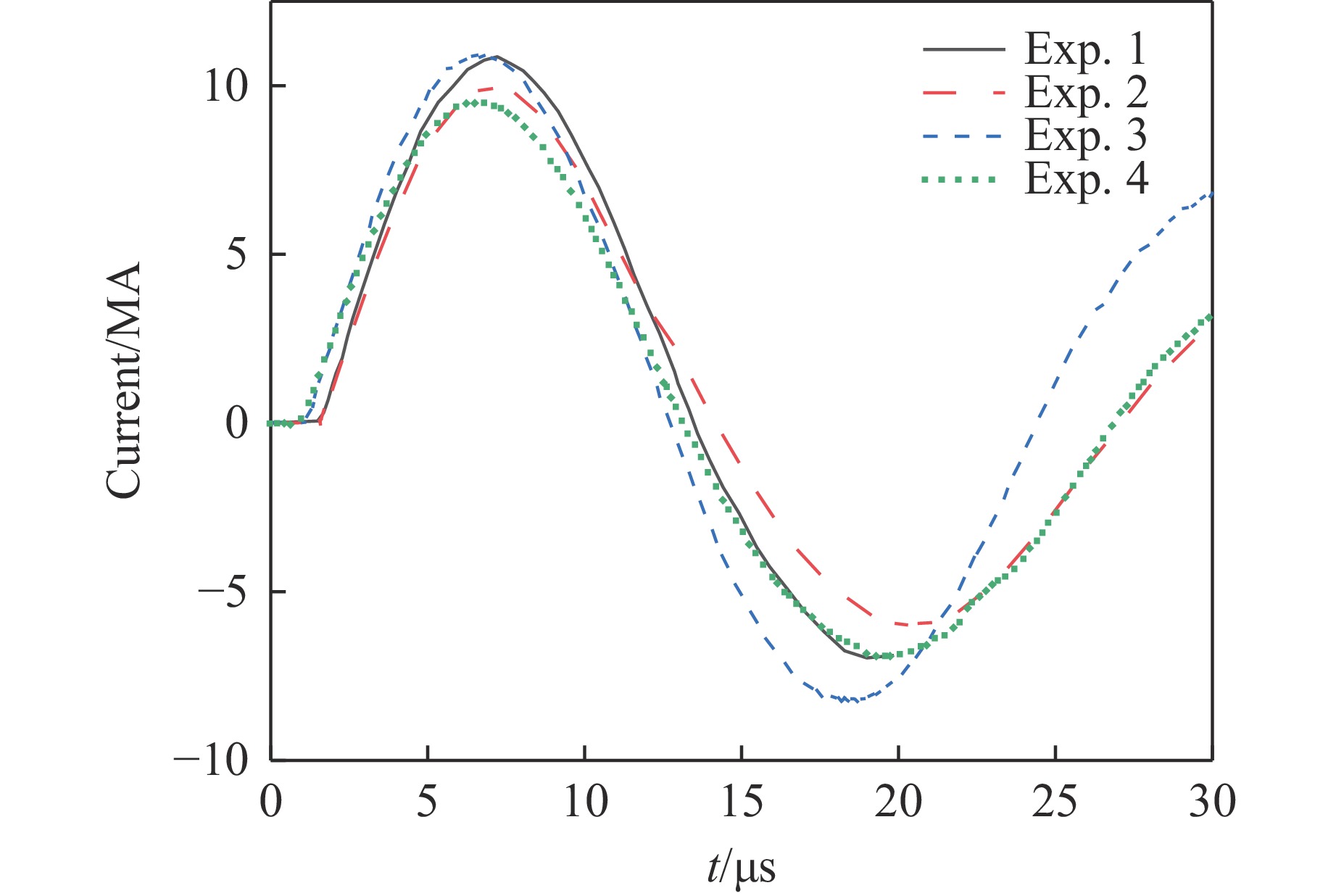
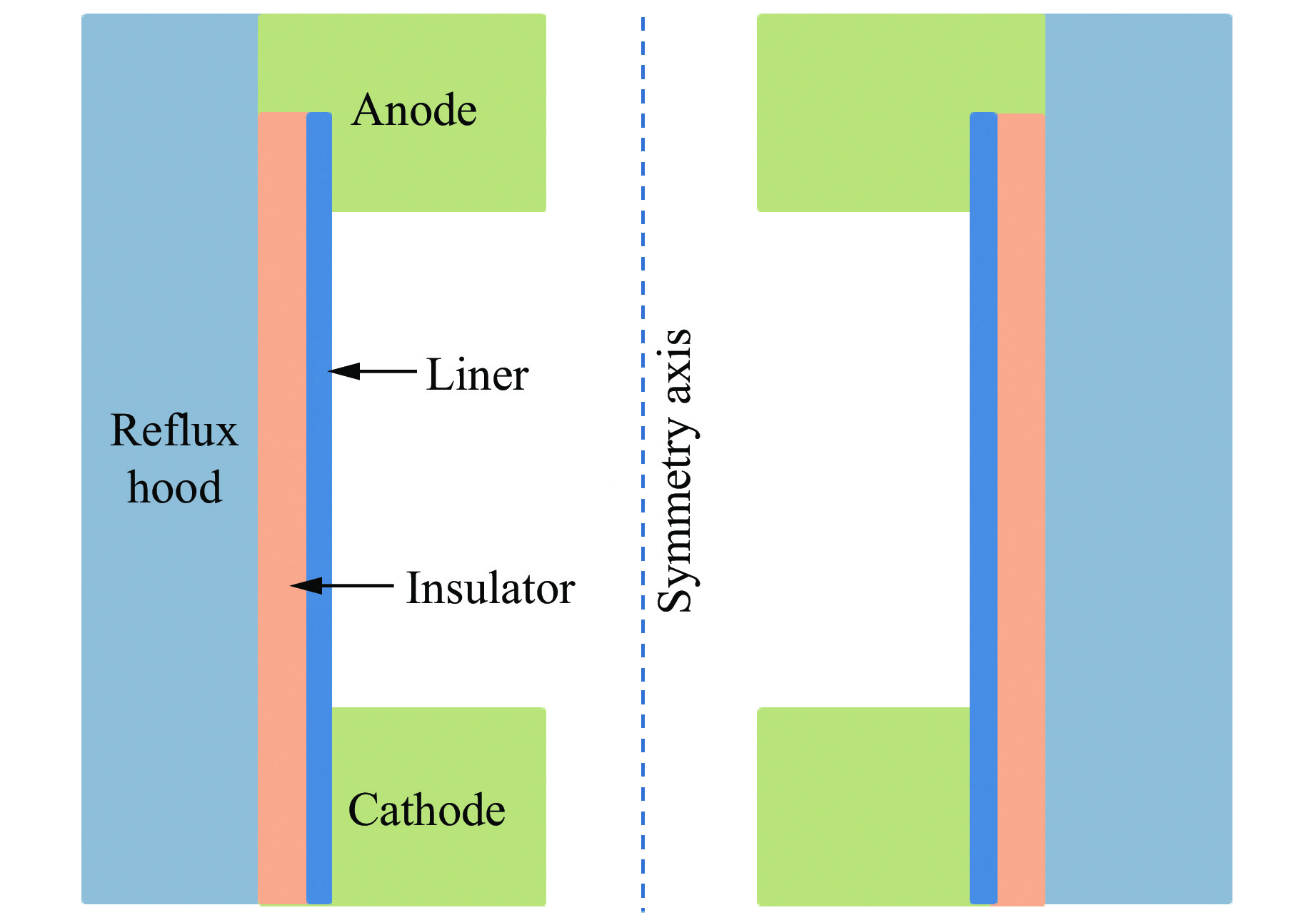
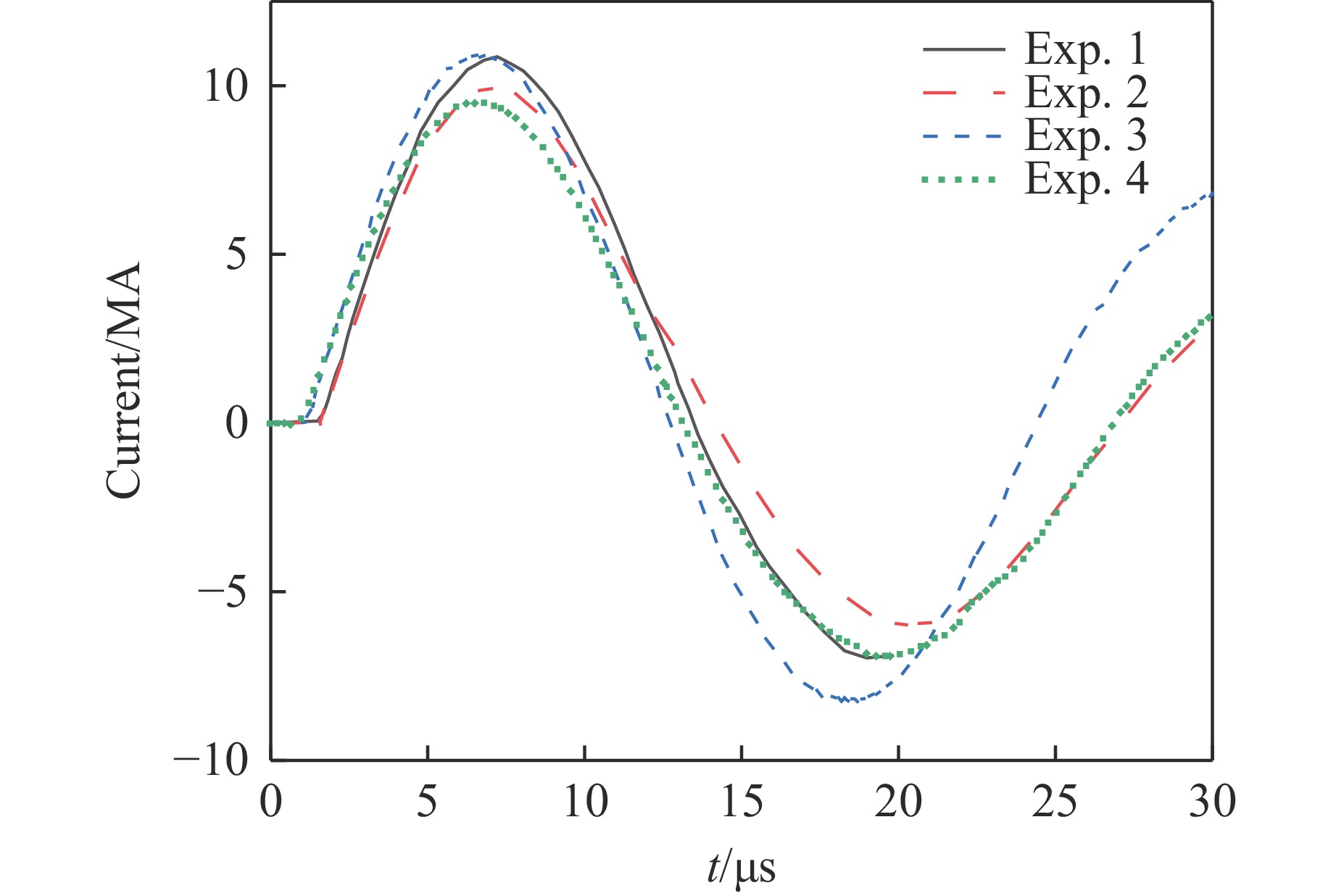
大电流脉冲装置上的固体套筒实验通常采用回流罩结构^{[15, 17–18]}。回流罩结构固体套筒实验的初始结构的rz剖面如图1所示，其中，虚线为对称轴。回流罩结构固体套筒实验装置从外到内依次为金属回流罩、绝缘材料和金属套筒，套筒两端为金属电极，上端为阳极，下端为阴极。回路电流从回流罩金属流入，绕过绝缘材料，经过套筒的外表面从阴极流出。电流加载后，电极外面的固体套筒被切割成与阴阳极之间的间隙等高的套筒，在洛仑兹力作用下沿径向向内箍缩。表1为FP-2装置^[19]中回流罩结构磁驱动固体套筒实验的套筒参数。图2显示了FP-2装置上不同实验测得的电流变化曲线，电流的上升时间约为5500 ns，电流峰值为9～11 MA。

图 1 回流罩固体套筒实验装置的初始结构剖面

Figure 1. Cross section of magnetically driven solid liner experiment setup with a reflux hood

下载: 全尺寸图片幻灯片

表 1 磁驱动固体套筒实验的套筒参数

Table 1. Liner parameters of the magnetically driven solid liner experiments

Exp. No.	Liner material	Liner’s inner radius/mm	Liner’s thickness/mm
1	Al	45	0.6
2	Al	30	0.6
3	Al	45	1.6
4	Al	30	1.9

下载: 导出CSV

| 显示表格

图 2 磁驱动固体套筒实验测得的电流

Figure 2. Measured currents for magnetically driven solid liner experiments

下载: 全尺寸图片幻灯片

2. 电流系数的不可压缩模型验证

在薄壳模型、不可压缩模型、电作用量-速度模型、全电路模型、磁流体力学模型等^[8–16]适用于磁驱动固体套筒的理论模型中，固体套筒边界的磁感应强度（B）为

$B(t) = \frac{{{\mu _0}{I_{\exp }}(t)}}{{2\text{π} {r_{\mathrm{o}}}}}$

(1)

式中： ${\mu _0}$ 为真空磁导率，I_exp(t)为磁驱动实验测量电流，r_o为固体套筒的外半径。

二维磁驱动数值模拟程序MDSC2是由中国工程物理研究院流体物理研究所开发的二维磁流体力学程序^[20–21]。该程序已被广泛应用于磁驱动飞片发射、超薄飞片、磁驱动准等熵压缩、磁驱动样品等实验的模拟研究^[22–25]。最近，研究人员发现，采用MDSC2程序模拟FP-2装置上的磁驱动固体套筒实验时，基于实验测量的电流或回路电流并不能正确模拟套筒的动力学过程，模拟的套筒速度总是比实验测量值大。为正确模拟FP-2装置上的磁驱动固体套筒实验，需将边界磁感应强度公式^[17]修正为

$B(t)=\frac{{\mu }_{0}{f}_{{\mathrm{c}}}{I}_{\mathrm{exp}}(t)}{2\text{π} {r}_{{\mathrm{o}}}}$

(2)

式中：f_c为回流罩结构rz柱面套筒的电流系数，f_c<1。由于文献[17]之外的理论计算或数值模拟中均未提到电流系数f_c，因此，需要确定f_c是回流罩固体套筒实验固有的，还是MDSC2程序造成的。下面采用固体套筒的不可压缩模型理论确认电流系数是否存在。

在磁驱动固体套筒的不可压缩模型^[11–13]中，不考虑套筒的磁扩散，假设磁压只作用于套筒的外表面，且磁压做功全部转化为套筒动能，套筒不可压缩，只作径向运动。设 $\rho$ 为套筒密度，h为套筒高度，v_o为套筒外界面速度，r_i、v_i分别为套筒内半径和内界面速度，r、v为套筒内某点的径向位置（r_i≤r≤r_o）和速度，由不可压缩假设，有

${r}_{{\mathrm{i}}}{v}_{{\mathrm{i}}}={r}_{{\mathrm{o}}}{v}_{{\mathrm{o}}}$

(3)

$rv = {r_{\mathrm{o}}}{v_{\mathrm{o}}}$

(4)

则套筒总动能E_k为

${E}_{{\mathrm{k}}}={\displaystyle {\int }_{{r}_{{\mathrm{i}}}}^{{r}_{{\mathrm{o}}}}}\rho \text{π} rh{v}^{2}{\rm d}r=\text{π}\rho h{r}_{{\mathrm{o}}}^{2}{v}_{{\mathrm{o}}}^{2}\mathrm{ln}\frac{{r}_{{\mathrm{o}}}}{{r}_{{\mathrm{i}}}}$

(5)

由于磁压只作用于套筒的外表面，且磁压做功全部转化为套筒动能，则

$\frac{{\mathrm{d}}{E}_{{\mathrm{k}}}}{{\mathrm{d}}t}=\frac{2\text{π} }{{\mu }_{0}}{r}_{{\mathrm{o}}}h{v}_{{\mathrm{o}}}{B}^{2}$

(6)

将式(5)代入式(6)并积分，可得

$\frac{{\mathrm{d}}{v}_{{\mathrm{o}}}}{{\mathrm{d}}t}=-\frac{{v}_{{\mathrm{o}}}^{2}}{{r}_{{\mathrm{o}}}}-\frac{1}{\mathrm{ln}({r}_{{\mathrm{o}}}/{r}_{{\mathrm{i}}})}\left[\frac{{B}^{2}}{2{\mu }_{0}\rho {r}_{{\mathrm{o}}}}+\frac{{v}_{{\mathrm{o}}}^{2}}{2{r}_{{\mathrm{o}}}}\left(1-\frac{{r}_{{\mathrm{o}}}^{2}}{{r}_{{\mathrm{i}}}^{2}}\right)\right]$

(7)

$\frac{{\mathrm{d}}{v}_{{\mathrm{i}}}}{{\mathrm{d}}t}=-\frac{{v}_{{\mathrm{i}}}^{2}}{{r}_{{\mathrm{i}}}}-\frac{1}{\mathrm{ln}({r}_{{\mathrm{o}}}/{r}_{{\mathrm{i}}})}\left[\frac{{B}^{2}}{2{\mu }_{0}\rho {r}_{{\mathrm{i}}}}+\frac{{v}_{{\mathrm{o}}}^{2}}{2{r}_{{\mathrm{i}}}}\left(1-\frac{{r}_{{\mathrm{i}}}^{2}}{{r}_{{\mathrm{o}}}^{2}}\right)\right]$

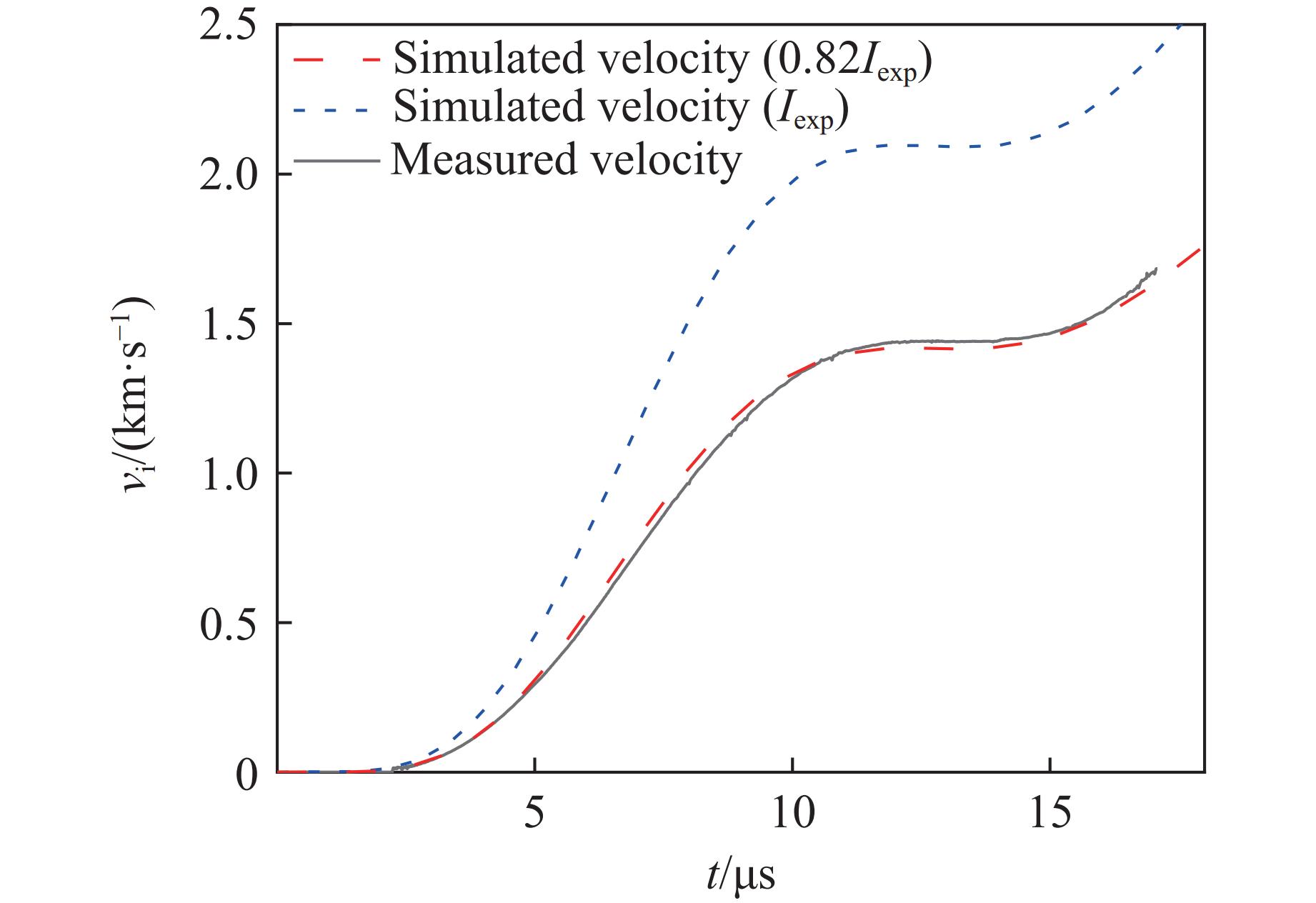
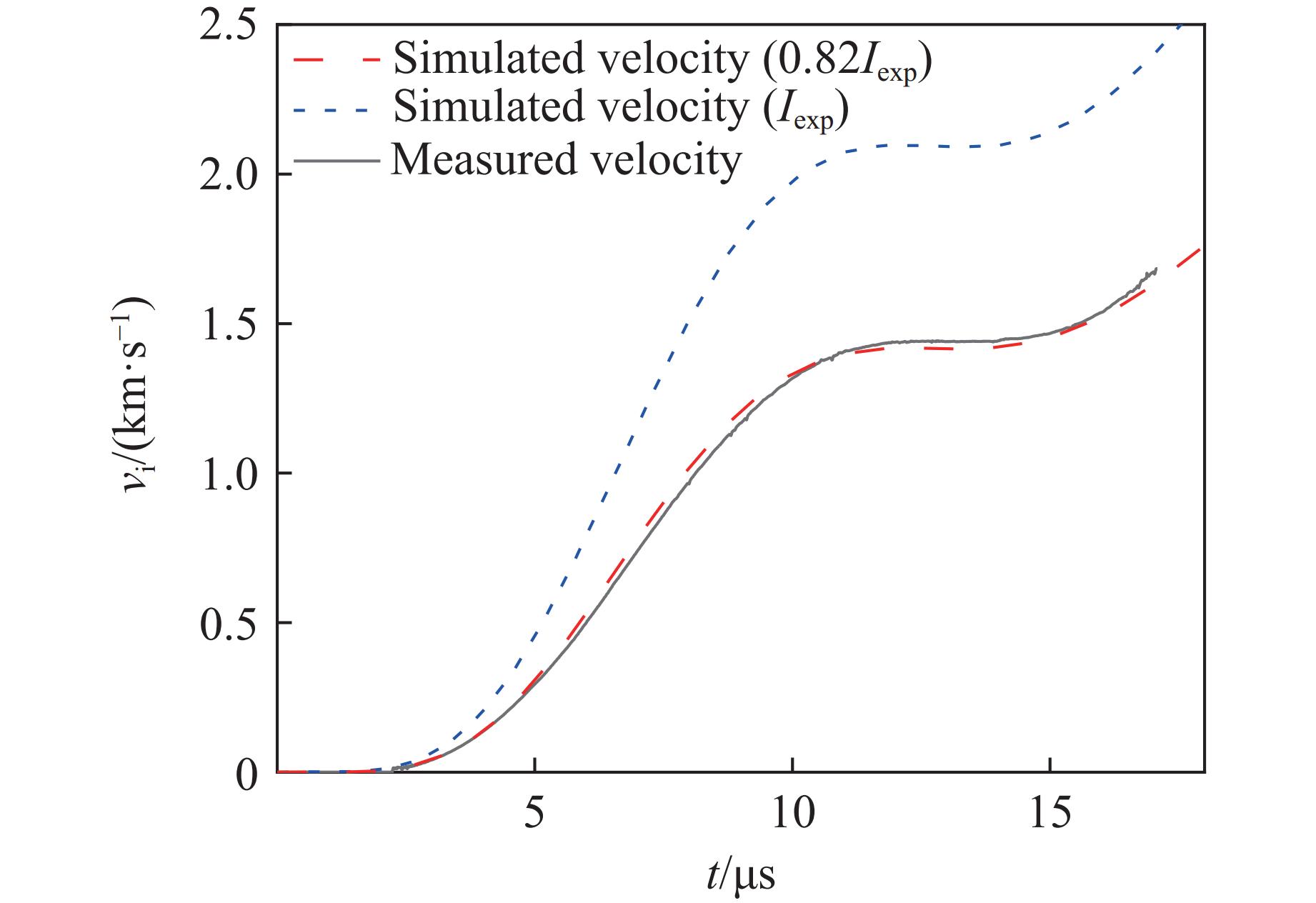
(8)

采用上述不可压缩模型，对固体套筒实验4进行不可压缩模型模拟验证。图3给出了采用不可压缩模型模拟得到的套筒内界面速度。显然，采用回路电流或测量电流直接模拟的套筒速度明显比实验测量速度大，后者是前者的0.82倍，即计算不可压缩模型的边界磁感应强度时不能用式(1)，而是用式(2)。不可压缩模型的模拟结果表明，对于回流罩固体套筒实验，回路电流或测量电流与固体套筒上通过的电流之间的电流系数不是MDSC2程序造成的，而是回流罩固体套筒实验固有的。

图 3 不可压缩模型模拟得到的套筒内界面速度

Figure 3. Liner interface velocity simulated by incompressible model

下载: 全尺寸图片幻灯片

3. 电流系数规律

从第2节的模拟可知，磁驱动固体套筒理论的边界磁感应强度公式中包含电流系数，它反映了有多少回路电流从套筒实际流过。在磁驱动实验中，实验测量的电流是流入回流罩之前的电流，即回路电流，而不是从套筒直接流过的电流。从套筒流过的电流很难被直接测量，因此，电流系数难以预知。回流罩的结构比较复杂，阴阳电极之间连有金属套筒、绝缘材料，金属套筒与绝缘材料之间是真空，回流罩结构的分流机制包括阴阳极间的并联电路分流、漏磁、真空击穿等。事实上，电流系数是通过数值模拟发现的，由磁流体力学程序模拟速度与磁驱动套筒实验测量速度的对比确定。当前的固体套筒实验的模拟都是后验的，无法直接正确预测，因此，研究电流系数的变化规律非常重要，是正确设计和预测固体套筒实验的基础。

由于磁流体力学模型^{[21, 26]}是包含固体弹塑性、热扩散、磁扩散等物理过程的可压缩模型，能够比不可压缩模型更加准确地描述磁驱动固体套筒实验，因此，下面将采用MDSC2程序对FP-2装置上开展的磁驱动固体套筒实验的电流系数变化规律进行研究。

图4给出了实验1～实验4的套筒内界面模拟速度。可以看出，应用式(2)的磁流体力学模型能正确描述磁驱动固体套筒实验。然而，不同的磁驱动固体套筒实验对应的电流系数是不同的。回流罩结构磁驱动固体套筒实验的电流系数和套筒的初始尺寸列于表2。

图 4 实验 1～实验4的套筒内界面速度

Figure 4. Interface velocities of the experimental liners for Exp. 1−Exp. 4

下载: 全尺寸图片幻灯片

表 2 磁驱动固体套筒实验的电流系数

Table 2. Current coefficients of the magnetically driven solid liner experiments

Exp. No.	Liner’s inner radius/mm	Liner’s thickness/mm	f_c
1	45	0.6	0.87
2	30	0.6	0.90
3	45	1.6	0.85
4	30	1.9	0.88

下载: 导出CSV

| 显示表格

由表2可知：电流系数是常数，不随时间的发展而变化，即电流系数与实验过程无关；对于不同的套筒，电流系数有所不同，说明电流系数与套筒的初始结构有关。由实验1和实验2可知，当套筒厚度相同时，若套筒内半径不同，则电流系数不同，且内半径越大，电流系数越小。对比实验1和实验3，或者实验2和实验4可知，当套筒内半径相同时，若套筒厚度不同，则电流系数不同，且套筒厚度越大，电流系数越小。

4. 结　论

采用不可压缩模型验证了回流罩结构磁驱动固体套筒实验中电流系数的存在，即回流罩结构磁驱动固体套筒实验的实验电流/回路电流并不完全从负载套筒的表面通过，实验电流/回路电流与套筒表面流过的电流之间存在一个电流系数。采用包含固体弹塑性、热扩散、磁扩散的磁流体力学模型，对回流罩结构磁驱动固体套筒实验的电流系数进行了确定和分析，结果显示，磁流体力学模型和有电流系数的边界磁感应强度公式能正确模拟回流罩结构磁驱动固体套筒实验。电流系数与套筒结构的关系为：

(1) 不同套筒对应的电流系数不同；

(2) 电流系数与实验过程无关，由套筒初始结构决定；

(3) 套筒厚度相同时，电流系数由套筒内半径决定，套筒内半径越大，电流系数越小；

(4) 套筒内半径相同时，电流系数由套筒厚度决定，套筒厚度越大，电流系数越小。

正确认识磁驱动固体套筒实验的电流系数变化规律，使磁驱动固体套筒实验的磁流体模拟从后验模拟发展成先验的准确设计和预测，有助于降低实验成本，加快柱面相关的实验研究。

图 1 回流罩固体套筒实验装置的初始结构剖面

Figure 1. Cross section of magnetically driven solid liner experiment setup with a reflux hood

下载: 全尺寸图片幻灯片

图 2 磁驱动固体套筒实验测得的电流

Figure 2. Measured currents for magnetically driven solid liner experiments

下载: 全尺寸图片幻灯片

图 3 不可压缩模型模拟得到的套筒内界面速度

Figure 3. Liner interface velocity simulated by incompressible model

下载: 全尺寸图片幻灯片

图 4 实验 1～实验4的套筒内界面速度

Figure 4. Interface velocities of the experimental liners for Exp. 1−Exp. 4

下载: 全尺寸图片幻灯片

表 1 磁驱动固体套筒实验的套筒参数

Table 1. Liner parameters of the magnetically driven solid liner experiments

Exp. No. Liner material Liner’s inner radius/mm Liner’s thickness/mm

1 Al 45 0.6
2 Al 30 0.6
3 Al 45 1.6
4 Al 30 1.9

下载: 导出CSV

表 2 磁驱动固体套筒实验的电流系数

Table 2. Current coefficients of the magnetically driven solid liner experiments

Exp. No. Liner’s inner radius/mm Liner’s thickness/mm f_c

1 45 0.6 0.87
2 30 0.6 0.90
3 45 1.6 0.85
4 30 1.9 0.88

下载: 导出CSV

参考文献(26)

[1]	TURCHI P J, BAKER W L. Generation of high-energy plasmas by electromagnetic implosion [J]. Journal of Applied Physics, 1973, 44(11): 4936–4945. doi: 10.1063/1.1662066
[2]	MARTIN M R, LEMKE R W, MCBRIDE R D, et al. Solid liner implosions on Z for producing multi-megabar, shockless compressions [J]. Physics of Plasmas, 2012, 19(5): 056310. doi: 10.1063/1.3694519
[3]	BUYKO A M, ZMUSHKO V V, ATCHISION W L, et al. Results and prospects of material strength studies on electrophysical facilities based on perturbation growth in liner systems [J]. IEEE Transactions on Plasma Science, 2008, 36(1): 104–111. doi: 10.1109/TPS.2007.914072
[4]	KAUL A M, IVANOVSKY A V, ATCHISON W L, et al. Damage growth and recollection in aluminum under axisymmetric convergence using a helical flux compression generator [J]. Journal of Applied Physics, 2014, 115(2): 023516. doi: 10.1063/1.4861411
[5]	REINOVSKY R E, ANDERSON W E, ATCHISO W L, et al. Instability growth in magnetically imploded high-conductivity cylindrical liners with material strength [J]. IEEE Transactions on Plasma Science, 2002, 30(5): 1764–1776. doi: 10.1109/TPS.2002.805418
[6]	REINOVSKY R E, ATCHISO W L, DIMONTE G, et al. Pulsed-power hydrodynamics: an application of pulsed-power and high magnetic fields to the exploration of material properties and problems in experimental hydrodynamics [J]. IEEE Transactions on Plasma Science, 2008, 36(1): 112–124. doi: 10.1109/TPS.2007.914708
[7]	ZHANG S L, LIU W, WANG G L, et al. Investigation of convergent Richtmyer-Meshkov instability at tin/xenon interface with pulsed magnetic driven imploding [J]. Chinese Physics B, 2019, 28(4): 044702. doi: 10.1088/1674-1056/28/4/044702
[8]	RYUTOV D D, DERZON M S, MATZEN M K. The physics of fast Z pinches [J]. Reviews of Modern Physics, 2000, 72(1): 167–223. doi: 10.1103/RevModPhys.72.167
[9]	丁宁, 杨震华, 宁成. Z箍缩等离子体内爆实验金属丝阵负载优化设计分析 [J]. 物理学报, 2004, 53(3): 808–817. doi: 10.7498/aps.53.808 DING N, YANG Z H, NING C. Optimization design of a wire array load for Z-pinch plasma implosion experiments [J]. Acta Physica Sinica, 2004, 53(3): 808–817. doi: 10.7498/aps.53.808
[10]	丁宁, 张扬, 宁成, 等. PTS装置Z箍缩负载设计分析 [J]. 物理学报, 2008, 57(5): 3027–3037. doi: 10.7498/aps.57.3027 DING N, ZHANG Y, NING C, et al. Design and analysis of the Z-pinch loads on the PTS facility [J]. Acta Physica Sinica, 2008, 57(5): 3027–3037. doi: 10.7498/aps.57.3027
[11]	章征伟, 魏懿, 孙奇志, 等. 材料强度对电磁驱动固体套筒内爆过程的影响 [J]. 强激光与粒子束, 2016, 28(4): 045017. doi: 10.11884/HPLPB201628.125017 ZHANG Z W, WEI Y, SUN Q Z, et al. Effect of material strength on electromagnetic driven solid liner implosion [J]. High Power Laser and Particle Beams, 2016, 28(4): 045017. doi: 10.11884/HPLPB201628.125017
[12]	张绍龙, 章征伟, 孙奇志, 等. 电流脉冲前沿对电磁驱动固体套筒内爆的影响 [J]. 强激光与粒子束, 2017, 29(10): 105002. doi: 10.11884/HPLPB201729.170161 ZHANG S L, ZHANG Z W, SUN Q Z, et al. Effect of current rise time on electromagnetic driven solid liner implosion [J]. High Power Laser and Particle Beams, 2017, 29(10): 105002. doi: 10.11884/HPLPB201729.170161
[13]	章征伟, 王贵林, 张绍龙, 等. 电作用量在磁驱动固体套筒内爆设计分析中的应用 [J]. 物理学报, 2020, 69(5): 050701. doi: 10.7498/aps.69.20191690 ZHANG Z W, WANG G L, ZHANG S L, et al. Application of electrical action to design and analysis of magnetically driven solid liner implosion [J]. Acta Physica Sinica, 2020, 69(5): 050701. doi: 10.7498/aps.69.20191690
[14]	TUCKER T J, TOTH R P. EBW1: a computer code for the prediction of the behavior of electrical circuits containing exploding wire elements: SAND-75-0041 [R]. Albuquerque: Sandia National Laboratory, 1975.
[15]	章征伟. 磁驱动固体套筒内爆理论与实验研究 [D]. 绵阳: 中国工程物理研究院, 2020: 49−59. ZHANG Z W. Theoretic and experimental study on magnetically driven solid liner implosion [D]. Mianyang: China Academy of Engineering Physics, 2020: 49−59.
[16]	张扬, 戴自换, 孙奇志, 等. FP-1装置铝套筒内爆动力学过程的一维磁流体力学模拟 [J]. 物理学报, 2018, 67(8): 080701. doi: 10.7498/aps.67.20172300 ZHANG Y, DAI Z H, SUN Q Z, et al. One-dimensional magneto-hydrodynamics simulation of magnetically driven solid liner implosions on FP-1 facility [J]. Acta Physica Sinica, 2018, 67(8): 080701. doi: 10.7498/aps.67.20172300
[17]	阚明先, 贾月松, 张南川, 等. 回流罩结构Z-箍缩实验的数值模拟 [J]. 强激光与粒子束, 2023, 35(2): 025003. doi: 10.11884/HPLPB202335.220271 KAN M X, JIA Y S, ZHANG N C, et al. Simulation of Z-pinch experiments with a reflux hood structure [J]. High Power Laser and Particle Beams, 2023, 35(2): 025003. doi: 10.11884/HPLPB202335.220271
[18]	ROUSCULP C L, ORO D M, GRIEGO J R, et al. Investigation of surface phenomena in shocked tin in converging geometry: LA-UR-16-21901 [R]. Los Alamos: Los Alamos National Laboratory, 2016.
[19]	SUN Q Z, JIA Y S, ZHANG Z W, et al. Cylindrical metal liner implosion at extremes of pressure and material velocity on an intense pulsed power facility-FP-2 [J]. Review of Scientific Instruments, 2022, 93(1): 013904. doi: 10.1063/5.0064238
[20]	阚明先, 王刚华, 赵海龙, 等. 磁驱动飞片二维磁流体力学数值模拟 [J]. 强激光与离子束, 2013, 25(8): 2137–2141. doi: 10.3788/HPLPB20132508.2137 KAN M X, WANG G H, ZHAO H L, et al. Two-dimensional magneto-hydrodynamic simulations of magnetically accelerated flyer plates [J]. High Power Laser and Particle Beams, 2013, 25(8): 2137–2141. doi: 10.3788/HPLPB20132508.2137
[21]	阚明先, 蒋吉昊, 王刚华, 等. 衬套内爆ALE方法二维MHD数值模拟 [J]. 四川大学学报(自然科学版), 2007, 44(1): 91–96. doi: 10.3969/j.issn.0490-6756.2007.01.020 KAN M X, JIANG J H, WANG G H, et al. ALE simulation of 2D MHD for liner [J]. Journal of Sichuan University (Natural Science Edition), 2007, 44(1): 91–96. doi: 10.3969/j.issn.0490-6756.2007.01.020
[22]	KAN M X, ZHANG Z H, XIAO B, et al. Simulation of magnetically driven flyer plate experiments with an improved magnetic field boundary formula [J]. High Energy Density Physics, 2018, 26: 38–43. doi: 10.1016/j.hedp.2017.12.002
[23]	阚明先, 段书超, 王刚华, 等. 自由面被烧蚀磁驱动飞片的数值模拟 [J]. 强激光与粒子束, 2017, 29(4): 045003. doi: 10.11884/HPLPB201729.160482 KAN M X, DUAN S C, WANG G H, et al. Numerical simulation of magnetically driven flyer plate of ablated free surface [J]. High Power Laser and Particle Beams, 2017, 29(4): 045003. doi: 10.11884/HPLPB201729.160482
[24]	阚明先, 段书超, 王刚华, 等. 磁驱动飞片发射实验结构系数初步研究 [J]. 强激光与粒子束, 2020, 32(8): 085002. doi: 10.11884/HPLPB202032.200072 KAN M X, DUAN S C, WANG G H, et al. Structure coefficient in magnetically driven flyer plate experiment [J]. High Power Laser and Particle Beams, 2020, 32(8): 085002. doi: 10.11884/HPLPB202032.200072
[25]	阚明先, 刘利新, 南小龙, 等. 磁驱动样品实验数值模拟研究 [J]. 高压物理学报, 2023, 37(6): 062301. doi: 10.11858/gywlxb.20230683 KAN M X, LIU L X, NAN X L, et al. Numerical simulation of magnetically driven sample experiment [J]. Chinese Journal of High Pressure Physics, 2023, 37(6): 062301. doi: 10.11858/gywlxb.20230683
[26]	阚明先, 段书超, 张朝辉, 等. 二维磁驱动数值模拟程序MDSC2的验证与确认 [J]. 强激光与粒子束, 2019, 31(6): 065001. doi: 10.11884/HPLPB201931.180300 KAN M X, DUAN S C, ZHANG Z H, et al. Verification and validation of two dimensional magnetically driven simulation code MDSC2 [J]. High Power Laser and Particle Beams, 2019, 31(6): 065001. doi: 10.11884/HPLPB201931.180300