Molecular Dynamics Simulation Study on Spallation Failure of [100] Single Crystal Aluminum under Different Waveform Loadings
-
摘要: 采用分子动力学方法模拟了[100]单晶铝在等冲量斜波和方波作用下的形变和层裂行为，分析了加载波形与层裂行为之间的相关性。研究表明，脉冲形状与热力学路径的协同作用影响了材料层裂。不同加载波形下单晶铝层裂强度的差异并非受缺陷主导的非均匀孔洞形核影响，而是由不同热力学路径下温升的差异决定。例如：当最大加载速度为3.00 km/s时，单晶铝均经历均匀层裂，但斜波加载下铝的层裂强度较方波加载时提升56.6%。斜波加载会产生逐渐增强的压缩波，使单晶铝产生相比于冲击加载更轻度的损伤。这一现象随着加载速度的提高而变得更加显著。Abstract: In this study, molecular dynamics method was used to simulate the deformation and spallation behavior of [100] single crystal aluminum under the action of equivalent ramp waves and square waves. Accordingly, the correlation between loading waveform and spallation behavior was analyzed. The results showed that the synergistic effect of the pulse shape transition and the thermodynamic path affected the material spallation. The nucleation of non-uniform holes dominated by defects was not the decisive factor affecting the spallation strength of materials. The difference of spallation characteristics of materials under different loading waveforms was mainly determined by the difference of temperature rise under different thermodynamic paths, which led to uniform spallation at maximum velocity of 3.00 km/s, but the spall strength of ramp wave group was 56.6% higher than that of square wave group. Due to the gradual compression and slight temperature softening effect, the ramp wave loading made the material presented milder damage than the impact loading, which became more significant with the increase of loading speed.
-
Key words:
- spallation /
- single crystal aluminum /
- molecular dynamics simulation /
- ramp wave loading
-
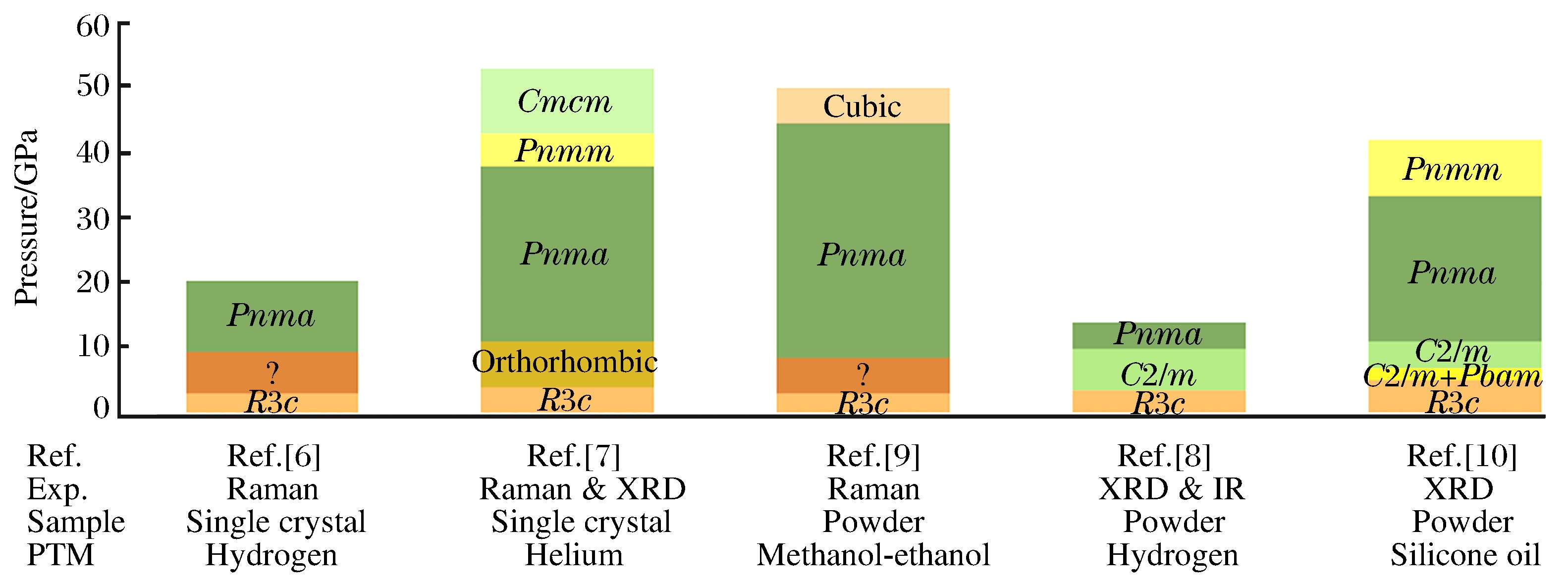
多铁材料是指在一定温度范围内同时具有两种或两种以上铁性体特征的材料，铁性体特征包括铁电性(反铁电性)、铁磁性(反铁磁性)、铁弹性(反铁弹性)等[1-4]。BiFeO3是少数在室温下同时具有铁电性和铁磁性的单相多铁材料之一。BiFeO3的铁电居里转变温度为1 104 K，反铁磁尼尔转变温度为643 K。BiFeO3在室温下为扭曲的三方钙钛矿结构，空间群为R3c。自从20世纪60年代BiFeO3被发现以来，其结构和性质已有广泛的研究。高压下BiFeO3晶体结构变化一直是研究的热点。2006年，Ravindran等[5]通过第一性原理计算预测，当压强升高到13 GPa时，BiFeO3将从R3c结构转变为正交相Pnma结构。Haumont等[6]和Guennou等[7]的研究也证实了这一点。但是Haumont等[6]认为在BiFeO3从R3c结构转变为正交相Pnma的过程中，还存在一个不确定的过渡相；随后他们采用X射线衍射(X-Ray Diffraction，XRD)测试确定了过渡相为单斜晶系C2/m[8]。而Guennou等[7]则认为在R3c和Pnma之间还存在3种过渡的正交相。至今在0~20 GPa压强范围内，BiFeO3晶体结构的变化一直存在争议。
图 1列出了2006年以来关于BiFeO3在高压下结构变化的部分研究结果。由图 1可以看出，BiFeO3从R3c结构完全转变为正交相Pnma发生在10 GPa左右。在40 GPa以上的高压区，BiFeO3的结构变化依旧存在一定争议。随着压强的升高，正交相Pnma可能转变为正交相Pnmm，也可能转变为更高对称性的立方晶系。
图 1 BiFeO3的高压拉曼光谱测试实验结果总结(其中PTM代表传压介质)Figure 1. Schematic summary of Raman of BiFeO3 (Where PTM denotes pressure transmitting medium)原位XRD测试和拉曼光谱测试是获得高压下结构转变行为最主要的两种测试方法。本研究利用原位拉曼光谱和金刚石压腔(Diamond Anvil Cell，DAC)技术，测量0~44 GPa下BiFeO3的拉曼光谱。
1. 实验
BiFeO3粉晶采用六面顶大压机在高温高压下合成[11]。将高纯金属氧化物Bi2O3(纯度≥99.9%)和Fe2O3(纯度≥99.9%)粉末按化学计量比混合，研磨均匀后利用模具压制成片。样品置于钼样品腔中，采用叶蜡石为传压介质，石墨管加热。将立方体组装块放入六面顶大压机中，在3 GPa压强下加温至800 ℃，保温15 min后降低加热功率使样品温度降至室温，然后缓慢卸压。合成的样品通过充分研磨后进行铜靶X射线衍射测试，对样品进行结构分析。
高压拉曼光谱实验中使用对称型金刚石压腔对BiFeO3粉晶进行加压，金刚石对顶砧台面直径为300 μm。采用厚度为250 μm的金属铼作为垫片，垫片预压厚度约为40 μm，样品孔直径约为120 μm。BiFeO3样品经充分研磨后被压成薄片放入样品腔内，充入氖气作为传压介质，在靠近样品的位置放一颗红宝石，通过红宝石的荧光峰计算压强[12]。原位拉曼光谱测试在型号为Omni-λ500的国产三光栅拉曼光谱仪上完成，激发光源为532 nm激光，测量波数范围为0~500 cm-1。
2. 结果与分析
2.1 XRD分析
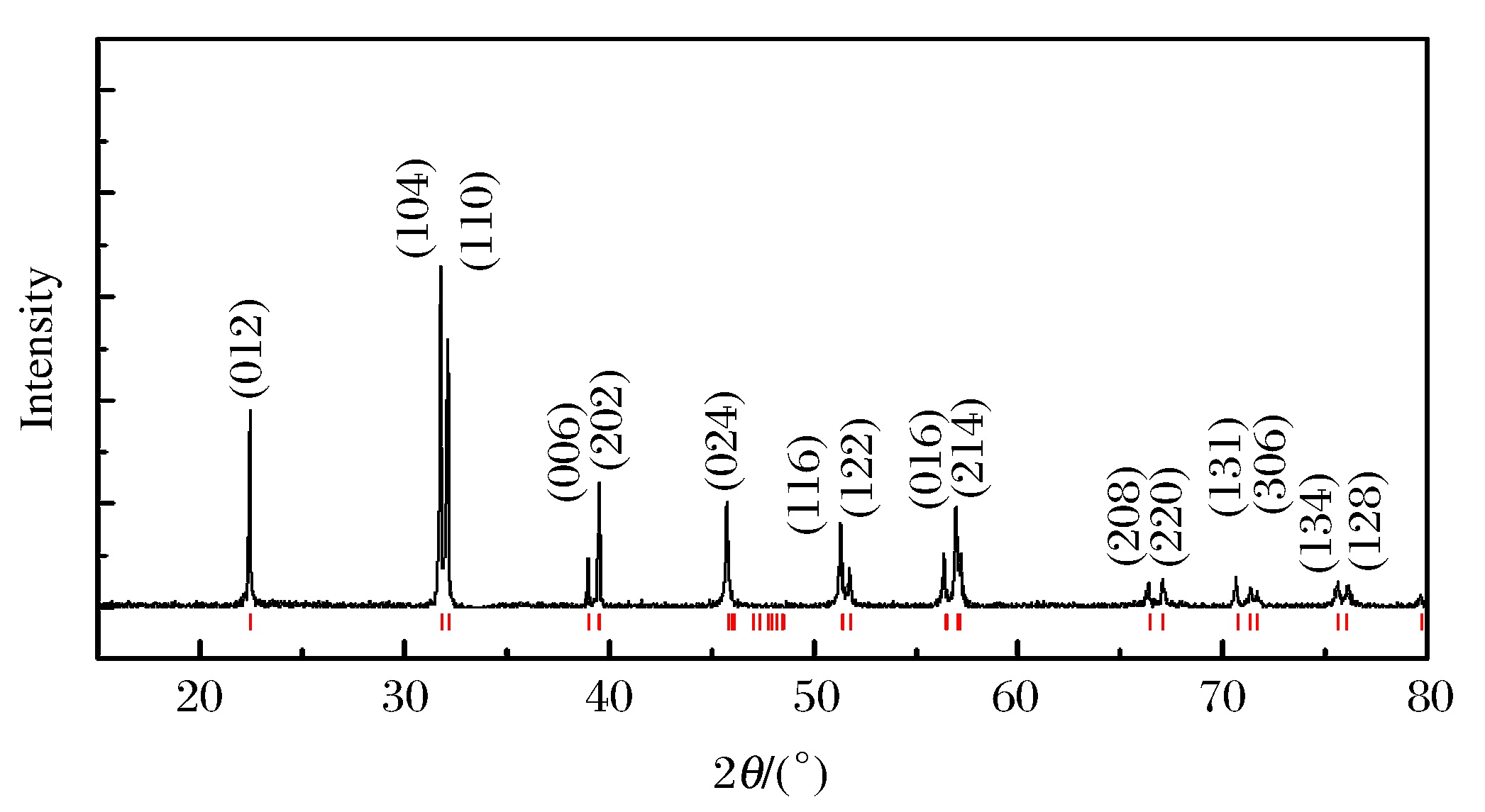
常压下BiFeO3粉晶样品的X射线衍射谱如图 2所示。仪器型号为德国布鲁克AXS公司生产的D8 Advance X射线衍射仪，扫描步长为0.02°，扫描范围为10°~80°。
图 2 常温常压下BiFeO3粉晶样品的XRD谱图Figure 2. X-ray diffraction pattern of BiFeO3 powder at ambient conditions从图 2中可以看出，BiFeO3样品XRD图谱的每个衍射峰均能指标化为R3c结构，无多余的衍射峰。这说明所合成的BiFeO3粉晶样品为纯相结构，没有其他杂相。相比于传统的固相烧结法，高压下合成BiFeO3纯相具有加热时间短、化学反应彻底的优势。
2.2 微结构表征
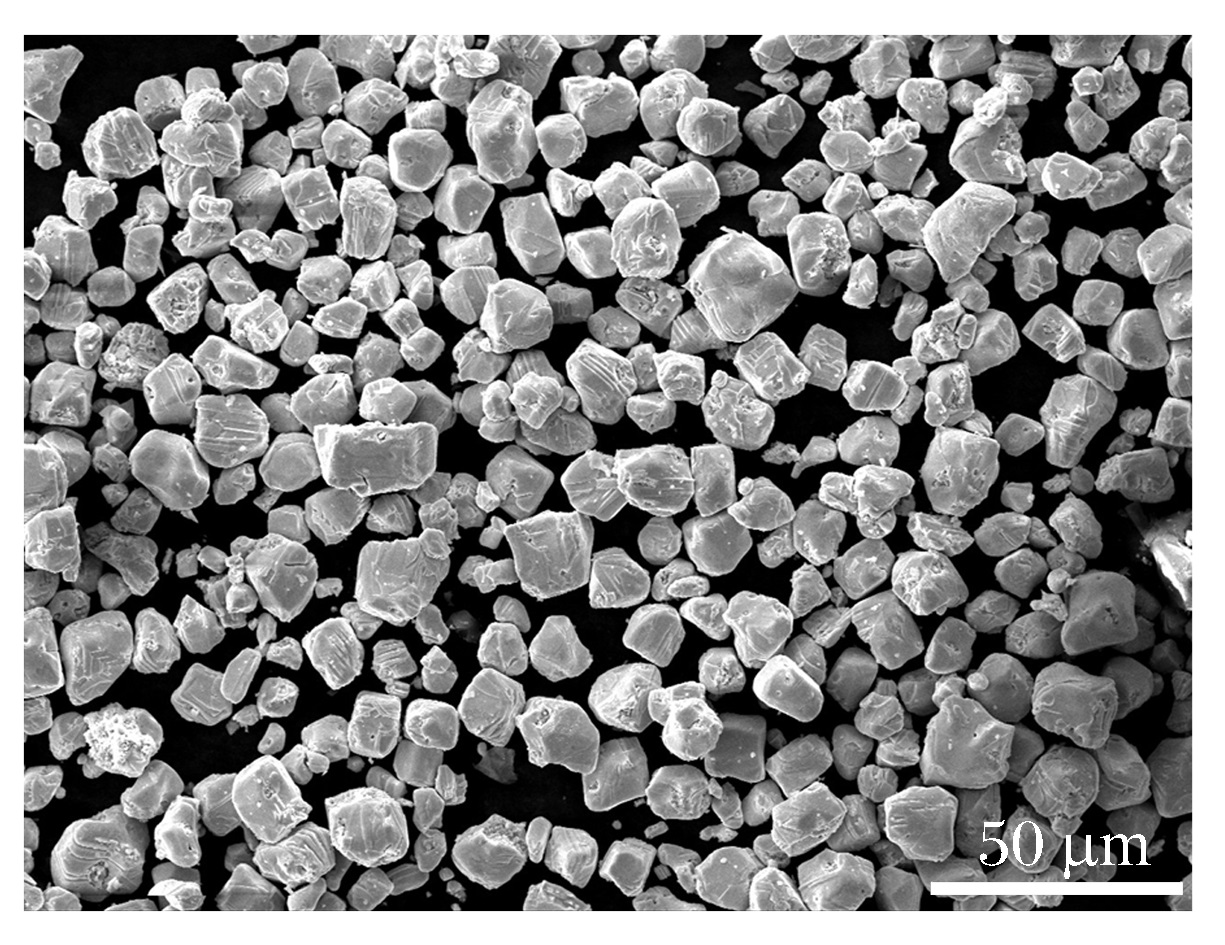
图 3为BiFeO3粉晶粉末样品的扫描电镜(Scanning Electron Microscope，SEM)二次电子像。仪器型号为JSM-IT300，放大倍数为500倍，加速电压为20 kV。
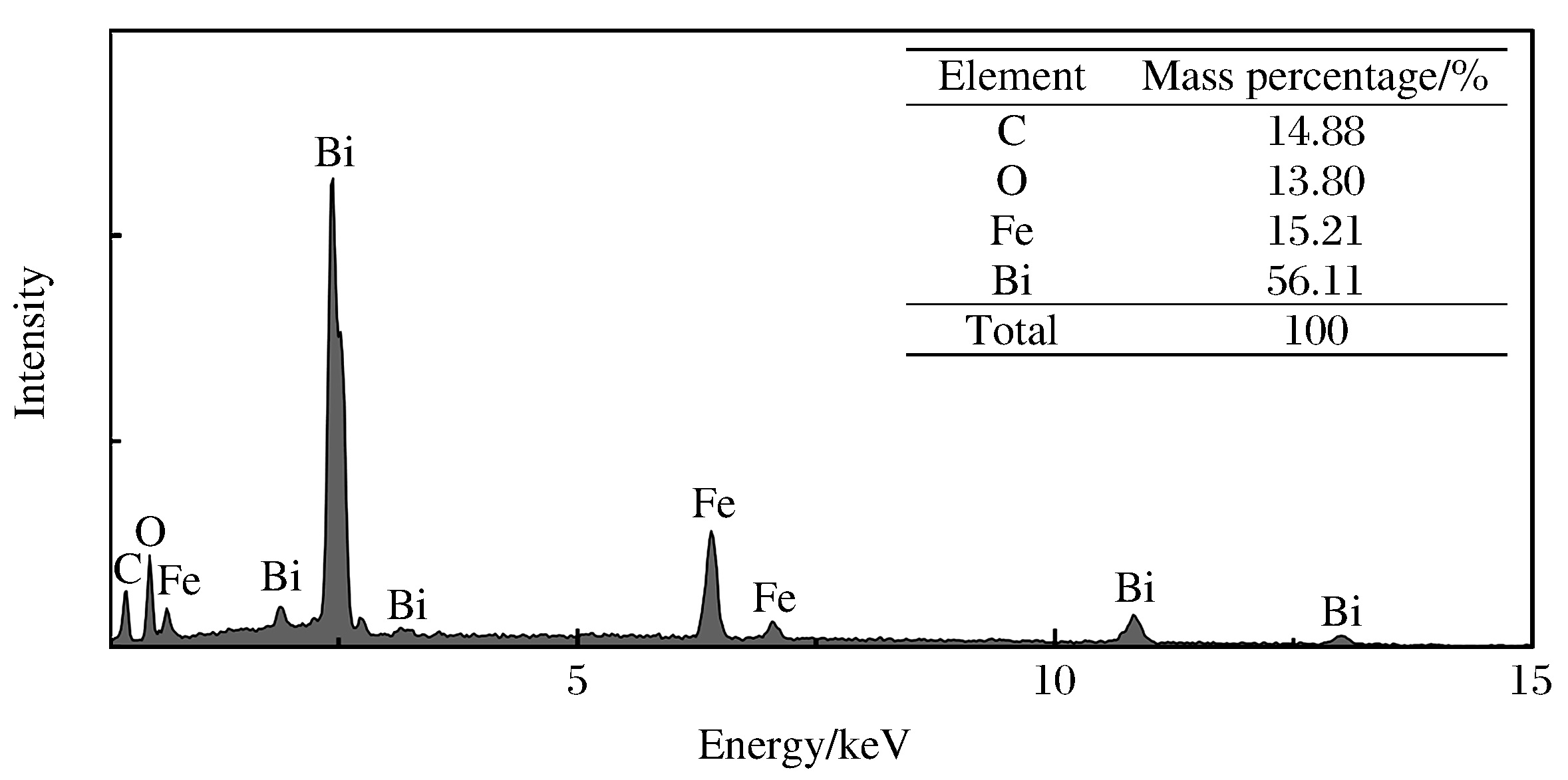
由图 3中可以看出，BiFeO3晶粒比较均匀，晶粒边缘清晰，粒径(直径)约为10 μm。图 4为BiFeO3粉末样品经过镀碳处理后测得的元素能谱图(Energy Dispersive Spectrum，EDS)。元素Bi和Fe的摩尔比约为1:1。
2.3 高压拉曼光谱分析
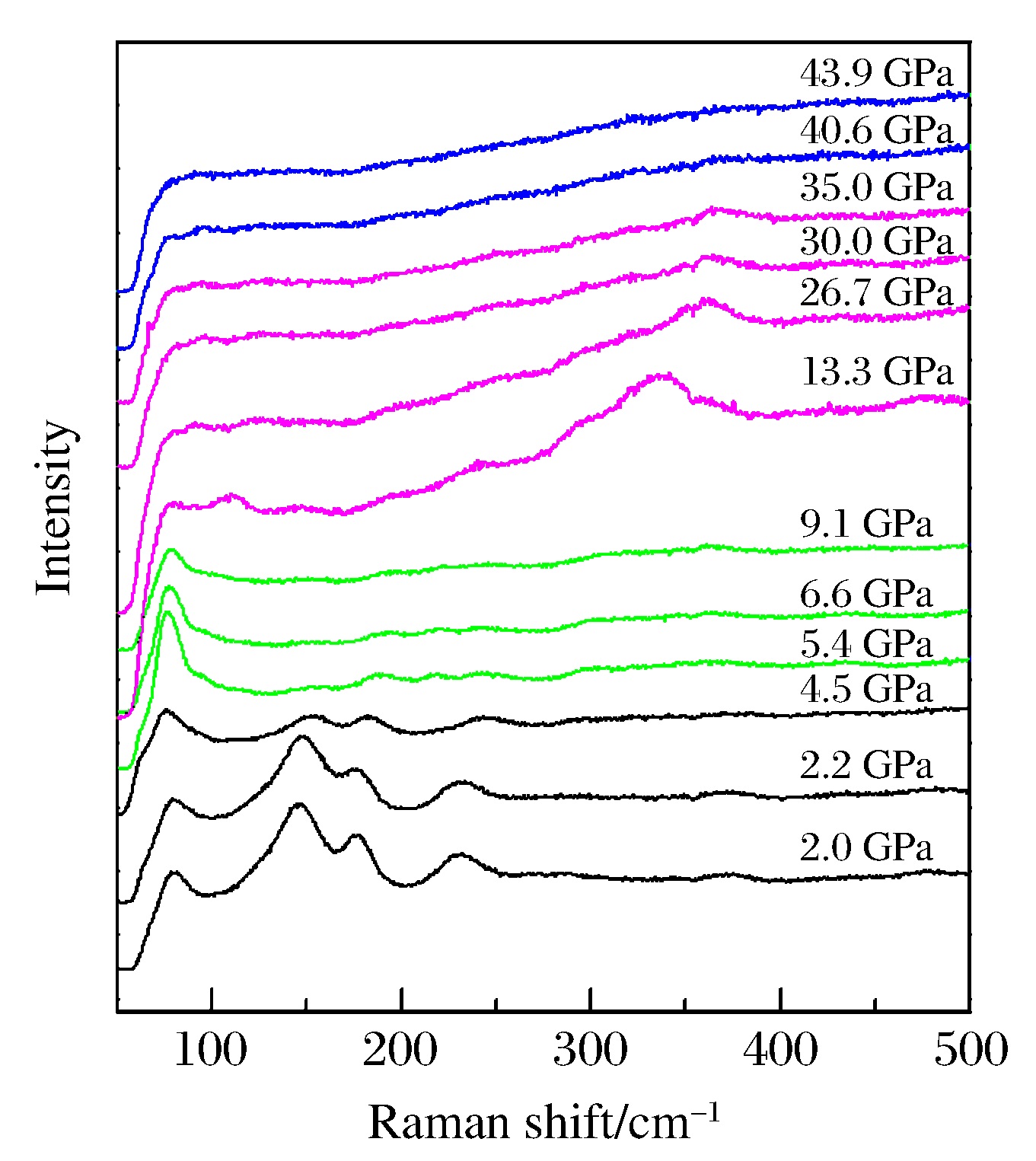
图 5为BiFeO3粉晶在不同压强下的拉曼光谱。在室温下，BiFeO3样品为扭曲的三方钙钛矿结构，空间群为R3c。根据群论分析，ГRaman=4A1+9E，其中只有A1模式位于100 cm-1以下。从图 5可以看出，随着压强的升高，低波数的拉曼峰开始向右迁移并变宽。位于145、177和231 cm-1的拉曼峰逐渐减弱，表明BiFeO3晶体结构开始发生变化。直到压强升至5 GPa左右，位于145 cm-1的拉曼峰消失，与此同时在217 cm-1处出现新的拉曼峰，标志着BiFeO3发生第1次相变。由BiFeO3拉曼振动模式随压强的变化(见图 6)可以看出，大约5 GPa时，177 cm-1处的拉曼峰突然向高波数偏移，与Yang等[9]的实验结果一致。第2次相变发生在11 GPa左右，位于340 cm-1处的拉曼峰显现，与此同时低波数(＜200 cm-1)拉曼峰消失，谱线上仅剩下两个明显的拉曼振动峰。Buhot等[13]的实验显示：在BiFeO3转变为Pnma结构时，整条谱线上仅有两个明显的拉曼峰；并且随着压强的增大，高波数的拉曼峰向右偏移，而低波数的拉曼峰向左偏移。这说明在13.3 GPa时，BiFeO3已经完全转变为正交晶系Pnma结构。Guennou等[7]和Haumont等[8]的实验显示，BiFeO3从R3c结构转变为Pnma结构的过程中存在一个过渡相，可能为正交晶系或者单斜晶系C2/m。由此可以暂时推论，BiFeO3在5~11 GPa的压强区间内，可能处于从R3c结构向正交晶系Pnma结构转变的过渡结构，确定其空间群还需要进一步的XRD实验。随着压强的继续增加，位于340 cm-1处、属于正交晶系Pnma结构的拉曼峰强度逐渐减弱，并在压强达到40.6 GPa之后消失。此时谱线上并无任何明显的拉曼振动峰，说明BiFeO3在38 GPa左右处发生了第3次结构相变。根据前人研究结果，BiFeO3在40 GPa附近可能会由Pnma结构转变为Pnmm或更高对称性的立方晶系结构，拉曼光谱无法给出高压相的晶体结构，还需通过高压下的原位XRD实验确定。
图 6 不同压强下BiFeO3的振动模式Fig. 6 Pressure dependence of all the vibrational modes of BiFeO3 sample3. 结论
通过六面顶大压机在高温高压下合成了纯的BiFeO3粉晶样品，并结合金刚石压腔和拉曼光谱测试，得到了BiFeO3粉晶在高压下拉曼光谱的变化。结果表明，BiFeO3粉晶在0~44 GPa的压强变化过程中发生了3次结构相变:(1)在5 GPa左右，BiFeO3由常压下扭曲的R3c结构可能转变为单斜晶系C2/m或者特征峰不明显的正交晶系；(2)在11 GPa左右，BiFeO3进一步转变为正交晶系Pnma结构；(3)在38 GPa附近，BiFeO3发生第3次结构转变，可能由正交晶系Pnma结构转变为Pnmm结构或更高对称的立方晶系。整体相变过程与Yang等[9]的实验结果类似，在5~11 GPa的过渡区间和38 GPa以上的压强区间不能确定晶体结构。BiFeO3高压相的具体晶体结构将在之后的研究中结合原位高压XRD实验确定。
-
图 1 (a)活塞法生成层裂示意图，(b) 载荷时程曲线
Figure 1. (a) Schematic diagram of spallation generation by piston method; (b) loading history curves
图 2 层裂强度（整体法）对最大速度和脉冲形状的依赖性
Figure 2. Dependence of spall strength (from bulk) on the maximum velocity and pulse shape
图 3 层裂强度（自由面法）对最大速度和脉冲形状的依赖性
Figure 3. Dependence of spall strength (from surface) on the maximum velocity and pulse shape
图 5 外加拉伸应变率与层裂温度及脉冲形状的关系
Figure 5. Dependency of applied tensile strain rate on spall temperature and pulse shape
图 7 层裂强度与层裂面温度的关系
Figure 7. Relationship between spall strength and temperature on the spall plane
图 8 应力波抵达自由表面时系统的原子构型
Figure 8. Atomic configuration of the system when the stress wave reaches the free surface
图 9 vp 为1.00和2.00 km/s时方波和斜波组中σxx的位置-时间（x-t）图
Figure 9. Position of σxx versus time (x-t) diagrams when vp is 1.00 and 2.00 km/s under square wave and ramp wave loading
图 11 vp 为1.25和1.50 km/s时斜波组层裂面上的缺陷结构随时间的演化
Figure 11. Time evolution of corresponding defect structure on the spall plane when vp is 1.25 and 1.50 km/s under ramp wave loading
图 12 斜波和方波加载下空洞数量和损伤比的演变历程
Figure 12. Evolution history of void number and damage ratio under square wave and ramp wave loading
表 1 不同工况下的层裂强度、应变速率和层裂温度
Table 1. Spall strength, strain rate, and spall temperature of different cases
Group vp/(km·s−1) σsp/GPa ˙ε/(109 s−1) Tsp/K From bulk From surface Ramp wave 1.00 9.748 8.536 1.864 223.82 1.25 8.814 7.528 2.350 272.24 1.50 9.847 7.851 2.983 224.95 1.75 9.941 8.678 3.282 227.39 2.00 10.084 8.233 3.664 230.75 2.25 10.358 7.546 4.123 233.08 2.50 10.321 7.077 4.556 236.06 2.75 10.308 6.764 4.855 236.80 3.00 10.261 6.325 5.284 239.73 Square wave 1.00 8.180 7.725 2.287 333.47 1.25 8.228 7.151 2.477 313.84 1.50 9.511 8.646 2.670 291.85 1.75 9.227 8.282 3.263 350.29 2.00 8.689 7.578 3.611 404.72 2.25 7.977 7.039 4.055 494.94 2.50 7.293 6.809 4.461 561.94 2.75 6.900 6.518 4.901 579.97 3.00 6.551 6.135 5.312 594.51 
下载: 导出CSV
-
[1] LI C, LI B, HUANG J Y, et al. Spall damage of a mild carbon steel: effects of peak stress, strain rate and pulse duration [J]. Materials Science and Engineering: A, 2016, 660: 139–147. doi: 10.1016/j.msea.2016.02.080 [2] LI C, YANG K, TANG X C, et al. Spall strength of a mild carbon steel: effects of tensile stress history and shock-induced microstructure [J]. Materials Science and Engineering: A, 2019, 754: 461–469. doi: 10.1016/j.msea.2019.03.019 [3] NGUYEN T, LUSCHER D J, WILKERSON J W. A physics-based model and simple scaling law to predict the pressure dependence of single crystal spall strength [J]. Journal of the Mechanics and Physics of Solids, 2020, 137: 103875. doi: 10.1016/j.jmps.2020.103875 [4] LI C, YANG K, GAO Y H, et al. Dislocation-dominated void nucleation in shock-spalled single crystal copper: mechanism and anisotropy [J]. International Journal of Plasticity, 2022, 155: 103331. doi: 10.1016/j.ijplas.2022.103331 [5] REMINGTON T P, HAHN E N, ZHAO S, et al. Spall strength dependence on grain size and strain rate in tantalum [J]. Acta Materialia, 2018, 158: 313–329. doi: 10.1016/j.actamat.2018.07.048 [6] PANG B, CASE S, JONES I P, et al. The defect evolution in shock loaded tantalum single crystals [J]. Acta Materialia, 2018, 148: 482–491. doi: 10.1016/j.actamat.2017.11.052 [7] HAHN E N, FENSIN S J, GERMANN T C, et al. Orientation dependent spall strength of tantalum single crystals [J]. Acta Materialia, 2018, 159: 241–248. doi: 10.1016/j.actamat.2018.07.073 [8] DONGARE A M, LAMATTINA B, RAJENDRAN A M. Atomic scale studies of spall behavior in single crystal Cu [J]. Procedia Engineering, 2011, 10: 3636–3641. doi: 10.1016/j.proeng.2011.04.598 [9] MACKENCHERY K, VALISETTY R R, NAMBURU R R, et al. Dislocation evolution and peak spall strengths in single crystal and nanocrystalline Cu [J]. Journal of Applied Physics, 2016, 119(4): 044301. doi: 10.1063/1.4939867 [10] MA K, CHEN J, DONGARE A M. Role of pre-existing dislocations on the shock compression and spall behavior in single-crystal copper at atomic scales [J]. Journal of Applied Physics, 2021, 129(17): 175901. doi: 10.1063/5.0040802 [11] WANG J, WANG F, ZENG X G, et al. Unraveling the plasticity performance and melting in single crystal tantalum damaged by shock compression [J]. Engineering Fracture Mechanics, 2022, 276: 108921. doi: 10.1016/J.ENGFRACMECH.2022.108921 [12] KRASNIKOV V S, MAYER A E. Plasticity driven growth of nanovoids and strength of aluminum at high rate tension: molecular dynamics simulations and continuum modeling [J]. International Journal of Plasticity, 2015, 74: 75–91. doi: 10.1016/j.ijplas.2015.06.007 [13] ZHOU T T, HE A M, WANG P, et al. Spall damage in single crystal Al with helium bubbles under decaying shock loading via molecular dynamics study [J]. Computational Materials Science, 2019, 162: 255–267. doi: 10.1016/j.commatsci.2019.02.019 [14] TANG F, JIAN Z Y, XIAO S F, et al. Molecular dynamics simulation of cylindrically converging shock response in single crystal Cu [J]. Computational Materials Science, 2020, 183: 109845. doi: 10.1016/j.commatsci.2020.109845 [15] XIONG Q L, KITAMURA T, LI Z H. Cylindrical voids induced deformation response of single crystal coppers during low-speed shock compressions: a molecular dynamics study [J]. Mechanics of Materials, 2019, 138: 103167. doi: 10.1016/j.mechmat.2019.103167 [16] TIAN X, CUI J Z, MA K P, et al. Shock-induced plasticity and damage in single-crystalline Cu at elevated temperatures by molecular dynamics simulations [J]. International Journal of Heat and Mass Transfer, 2020, 158: 120013. doi: 10.1016/j.ijheatmasstransfer.2020.120013 [17] LIAO Y, XIANG M Z, ZENG X G, et al. Molecular dynamics studies of the roles of microstructure and thermal effects in spallation of aluminum [J]. Mechanics of Materials, 2015, 84: 12–27. doi: 10.1016/j.mechmat.2015.01.007 [18] HAWKINS M C, THOMAS S A, FENSIN S J, et al. Spall and subsequent recompaction of copper under shock loading [J]. Journal of Applied Physics, 2020, 128(4): 045901. doi: 10.1063/5.0011645 [19] ZHU Y L, HU J N, WEI Q Q, et al. Enhanced spall strength of single crystal aluminum by temperature rise mitigation and structural phase transition under shock pulse [J]. Mechanics of Materials, 2023, 186: 104809. doi: 10.1016/j.mechmat.2023.104809 [20] 黄海军, 沈强, 罗国强, 等. 利用多层阻抗梯度飞片产生准等熵压缩的理论解析 [J]. 物理学报, 2007, 56(3): 1538–1542. doi: 10.3321/j.issn:1000-3290.2007.03.050HUANG H J, SHEN Q, LUO G Q, et al. Theoritical analysis of quasi-isentropic compression via flier-plate with grade wave impadence [J]. Acta Physica Sinica, 2007, 56(3): 1538–1542. doi: 10.3321/j.issn:1000-3290.2007.03.050 [21] ZHU Y L, HU J N, HUANG S L, et al. Molecular dynamics simulation on spallation of [111] Cu/Ni nano-multilayers: voids evolution under different shock pulse duration [J]. Computational Materials Science, 2022, 202: 110923. doi: 10.1016/J.COMMATSCI.2021.110923 [22] ZHAKHOVSKII V V, INOGAMOV N A, PETROV Y V, et al. Molecular dynamics simulation of femtosecond ablation and spallation with different interatomic potentials [J]. Applied Surface Science, 2009, 255(24): 9592–9596. doi: 10.1016/j.apsusc.2009.04.082 [23] JIANG D D, SHAO J L, WU B, et al. Sudden change of spall strength induced by shock defects based on atomistic simulation of single crystal aluminum [J]. Scripta Materialia, 2022, 210: 114474. doi: 10.1016/j.scriptamat.2021.114474 [24] KADAU K, GERMANN T C, LOMDAHL P S, et al. Atomistic simulations of shock-induced transformations and their orientation dependence in bcc Fe single crystals [J]. Physical Review B, 2005, 72(6): 064120. doi: 10.1103/PhysRevB.72.064120 [25] SRINIVASAN S G, BASKES M I, WAGNER G J. Atomistic simulations of shock induced microstructural evolution and spallation in single crystal nickel [J]. Journal of Applied Physics, 2007, 101(4): 043504. doi: 10.1063/1.2423084 [26] ASHITKOV S I, AGRANAT M B, KANEL’ G I, et al. Behavior of aluminum near an ultimate theoretical strength in experiments with femtosecond laser pulses [J]. JETP Letters, 2010, 92(8): 516–520. doi: 10.1134/S0021364010200051 [27] ELIEZER S, MOSHE E, ELIEZER D, et al. Laser-induced tension to measure the ultimate strength of metals related to the equation of state [J]. Laser and Particle Beams, 2002, 20(1): 87–92. doi: 10.1017/S0263034602201123 [28] GARKUSHIN G V, KANEL G I, RAZORENOV S V, et al. Resistance to deformation and fracture of aluminum AD1 under shock-wave loading at temperatures of 20 and 600 ℃ [J]. Physics of The Solid State, 2010, 52(11): 2369–2375. doi: 10.1134/S1063783410110247 [29] KANEL G I, RAZORENOV S V, GRADY D E, et al. Spall fracture properties of aluminum and magnesium at high temperatures [J]. Journal of Applied Physics, 1996, 79(11): 8310–8317. doi: 10.1063/1.362542 [30] RAZORENOV S V, KANEL G I, FORTOV V E, et al. Submicrosecond strength of aluminum and alloy AMg6M at normal and elevated temperatures [J]. Fizika Metallov Ⅰ Metallovedenie, 2003, 95(1): 91–96. [31] KANEL G I, RAZORENOV S V, SINGER J, et al. Dynamic yield and tensile strength of aluminum single crystals at temperatures up to the melting point [J]. Journal of Applied Physics, 2001, 90(1): 136–143. doi: 10.1063/1.1374478 [32] STRACHAN A, ÇAĞIN T, GODDARD Ⅲ W A. Critical behavior in spallation failure of metals [J]. Physical Review B, 2001, 63(6): 060103. doi: 10.1103/PhysRevB.63.060103 -
下载:
下载:
点击查看大图
计量
- 文章访问数: 206
- HTML全文浏览量: 80
- PDF下载量: 53
