Molecular Dynamics Simulation Study on Spallation Failure of [100] Single Crystal Aluminum under Different Waveform Loadings
-
摘要: 采用分子动力学方法模拟了[100]单晶铝在等冲量斜波和方波作用下的形变和层裂行为，分析了加载波形与层裂行为之间的相关性。研究表明，脉冲形状与热力学路径的协同作用影响了材料层裂。不同加载波形下单晶铝层裂强度的差异并非受缺陷主导的非均匀孔洞形核影响，而是由不同热力学路径下温升的差异决定。例如：当最大加载速度为3.00 km/s时，单晶铝均经历均匀层裂，但斜波加载下铝的层裂强度较方波加载时提升56.6%。斜波加载会产生逐渐增强的压缩波，使单晶铝产生相比于冲击加载更轻度的损伤。这一现象随着加载速度的提高而变得更加显著。Abstract: In this study, molecular dynamics method was used to simulate the deformation and spallation behavior of [100] single crystal aluminum under the action of equivalent ramp waves and square waves. Accordingly, the correlation between loading waveform and spallation behavior was analyzed. The results showed that the synergistic effect of the pulse shape transition and the thermodynamic path affected the material spallation. The nucleation of non-uniform holes dominated by defects was not the decisive factor affecting the spallation strength of materials. The difference of spallation characteristics of materials under different loading waveforms was mainly determined by the difference of temperature rise under different thermodynamic paths, which led to uniform spallation at maximum velocity of 3.00 km/s, but the spall strength of ramp wave group was 56.6% higher than that of square wave group. Due to the gradual compression and slight temperature softening effect, the ramp wave loading made the material presented milder damage than the impact loading, which became more significant with the increase of loading speed.
-
Key words:
- spallation /
- single crystal aluminum /
- molecular dynamics simulation /
- ramp wave loading
-
自13世纪黑火药应用到军事领域以来，制造更低敏感度及更高做功能力的炸药一直是高能材料研究领域最大和最重要的挑战之一。1, 3, 5-三氨基-2, 4, 6-三硝基苯(TATB，晶体及分子结构见图 1)是目前唯一被美国能源部批准可单独应用于核武器的单质炸药，对撞击和摩擦极其钝感。鉴于其极低的敏感度以及相对优秀的爆炸性能，国内外相继开展并积累了大量针对TATB敏感度和反应特性的研究工作，其中既有电子结构[1-4]、力学性能[5-9]等静力学性质的相关研究，也有热分解/冲击分解机理[10-11]、爆轰行为[12]等动力学性质研究以及以TATB为基的复合炸药性质研究[9]。在这些研究中，对工程预测TATB爆轰性能[13]十分重要的反应动力学性质研究仍处于初级阶段。
现有的TATB反应动力学研究主要包括热刺激或高速冲击下的初始分解机理研究[10, 14-16]、反应中碳团簇的形成及演化机理研究[11, 17-18]。在初始热分解机理问题上，通过热分解实验得到的初期反应过程与通过模拟计算反应能垒所预测的结果基本一致。然而在分解反应后期或爆轰条件下，反应路径数目和反应速率明显增加，无论实验还是理论计算都很难有效地检测其每一步的反应机理及演化过程，因此到目前为止仍没有一个明确的认识。为了更深入地了解TATB在爆轰条件下的反应过程，本研究从外部反应条件入手，采用计算模拟方式研究TATB在高温下的固相分解，综合分析温度、压力以及黏结剂等因素对反应机理和反应速率的影响。
1. 计算方法及模型
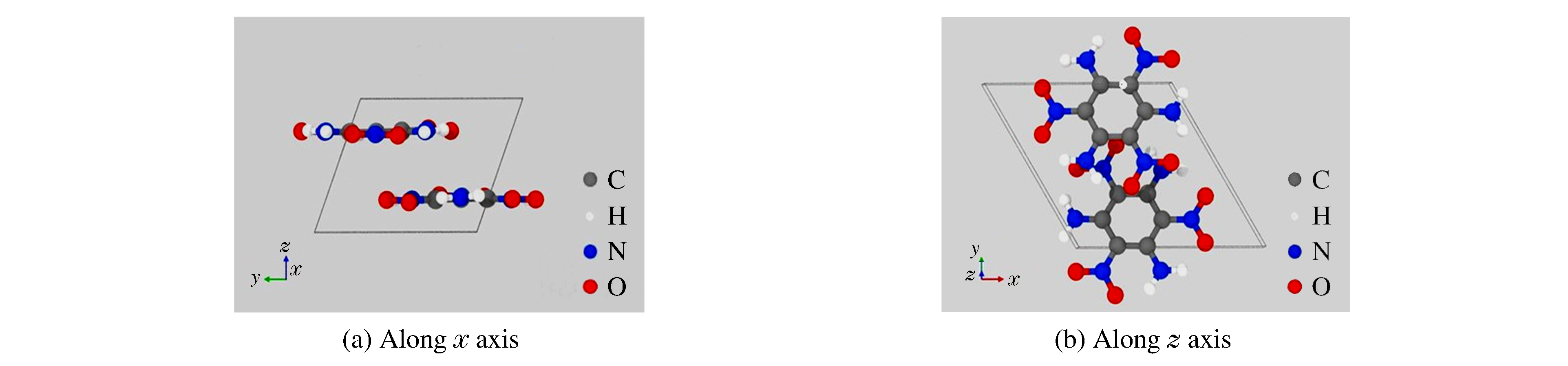
为保证计算精度，所有动力学模拟均采用从头算分子动力学方法，计算程序为北京应用物理与计算数学研究所含能材料团队自主研发的第一性原理软件包HASEM[19]。该计算程序采用原子轨道组合(LCAO)，利用密度泛函理论方法计算能量。在0 K下经优化得到TATB晶格参数，如表 1所示，其中a、b、c分别为晶胞3个轴的长度，α、β、γ为晶轴之间的夹角。除了c有5%的相对误差之外，其他晶格参数与实验值的相对误差均在1%以内。
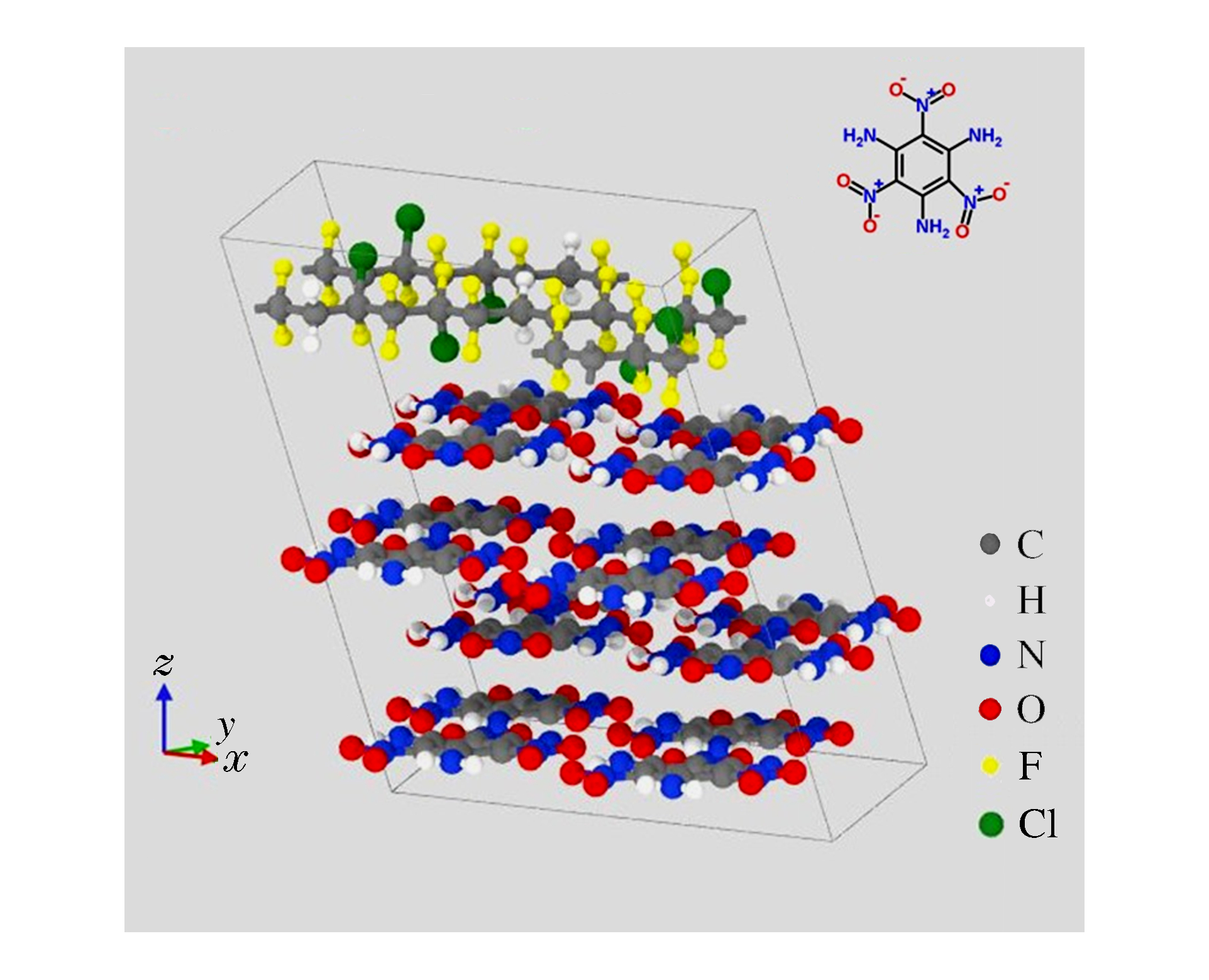
表 1 TATB晶格参数Table 1. Lattice parameters of TATBMethod a/nm b/nm c/nm α/(°) β/(°) γ/(°) HASEM 0.909 0.901 0.648 107.79 91.80 119.88 Experiment[20] 0.901 0.903 0.681 108.59 91.82 119.97 整个模拟工作主要分为3部分：(1)相同初始压力(0 GPa)、不同温度下的反应动力学过程模拟，模拟温度分别为2 500、3 000、3 500和4 000 K；(2)相同温度(2 500 K)、不同密度(不同初始压力)下的反应过程模拟，通过改变晶体密度实现对不同压力下反应进程的模拟，所采用的体系密度为0.8d0、1.2d0和1.3d0(d0为晶体在常压、0 K下的密度)；(3)相同压力(0 GPa)、不同温度条件下带有黏结剂的反应过程模拟，模拟温度为2 500、3 000和3 500 K。在实际应用中，黏结剂是炸药的重要组成成分，本研究主要考虑以TATB为基、氟聚物为黏结剂的复合炸药体系。目前投入使用的PBX-9502聚合物炸药的组成成分为：TATB(质量分数为95%)、F23xx系列氟聚物黏结剂(质量分数为5%)。据此构建了TATB质量分数为86.7%的TATB-F2312复合体系并进行模拟，如图 2所示，其中氟聚物均匀分布于晶体c面，分布方式满足晶体的周期性边界条件，以使F2312分子相互连接。所有模拟均采用NVT正则系综(等温等体积)，模拟晶胞大小为2×2×2，共包含16个TATB分子。为了考察晶格尺寸效应对反应过程的影响，特别构建一个晶胞大小为2×3×4的纯TATB模型(共48个TATB分子)进行验证计算，模拟时也采用NVT(等温等体积)系综，模拟温度为3 500 K，初始压力为0 GPa。
2. 计算结果与讨论
2.1 TATB分解机理
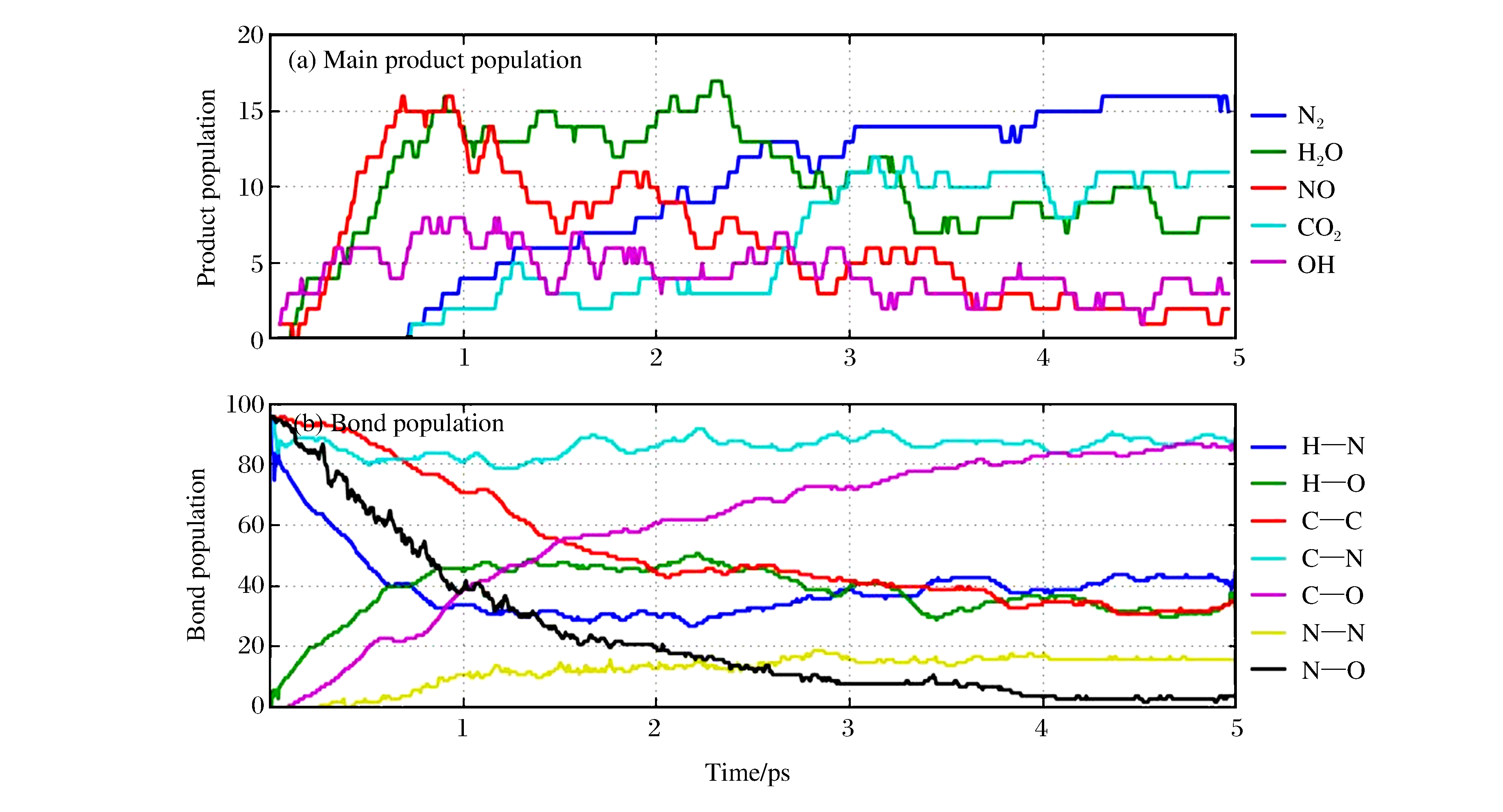
Wu等[20]指出TATB分解过程的第一步是质子转移和C─NO2键断裂。本研究采用团簇分析方法，统计了几种主要中间产物及成键的数量随时间的变化，如图 3所示。从最早生成的产物NO2、NO和H2O以及N─H键、N─O键的变化可以确认，在TATB反应初期质子转移和C─NO2键断裂确实是最先发生的。在后续的变化中可以看到，主要的气态产物为N2、CO2以及H2O，其中N2和CO2的形成均伴随着NO2和H2O的消耗。下面就H2O、N2和CO2这3种主要产物的生成机制进行详细的说明。
图 3 3 500 K下TATB分解的主要产物和原子间成键数演化曲线((a)主要产物数目; (b)原子间成键数)Figure 3. Main products and bond population curves of TATB decomposition at 3 500 K((a) Main product population; (b) bond population)2.1.1 H2O的形成
H2O是TATB分解过程中最早生成的最终产物，其中的H来源于整个反应过程中最先发生的质子(H)转移。氨基(-NH2)中的H首先脱落，转移到邻近的亚硝基(-NO2)上与O结合，形成OH-基团。H转移可以细分为分子内转移和分子间转移，其中最先观察到分子内转移，其次为分子间转移。由于TATB特殊的层状堆积结构，分子间H转移还可以细分为层内分子间转移和层间分子间转移。虽然层间转移所需移动的距离更远，但在实际模拟中发现层间H转移与层内H转移的概率相差不大。这主要受氢键的影响：-NH2与-NO2基团之间存在较强的相互作用，在热运动下层间分子会相对运动，加之C─NO2和C─NH2键的旋转，层间分子的相对距离明显缩短，为层间分子间的H转移创造了条件。H转移会产生OH-，而OH-+H+→H2O很容易进行，因此H2O自然成为所有产物中最先生成的物质。
2.1.2 N2的形成
与H转移过程同时发生的还有C─NO2键的均裂过程。静态计算[21]表明，气体状态下C─NO2键的断裂能垒比H转移能垒低，但在晶体中两个过程的能垒趋于一致。该过程会在炸药内部产生-NO2自由基，并与H相互作用生成NO+和OH-，其中NO+是形成N2的关键要素。通过反向追踪N2分子中两个N原子的运动轨迹，确定大部分N2分子的生成依赖于-C─NH+NO+→-C+N2+OH-这一过程，其中由于C─NH键的强度远大于C─NO键，因此-NH基团通常留在苯环上，而NO+则作为自由基在分子间运动。由于N≡N键非常牢固，N2一旦生成便基本不再参与其他反应过程，是所有产物中最稳定的物质。
2.1.3 CO2的形成
CO2的形成机理比较复杂，不过由于所有的C都集中在TATB分子中心的苯环中，在形成CO2之前苯环必然经历裂解过程，因此苯环裂解可以作为CO2形成的主要参考标准。在模拟结果中观察到苯环裂解存在多种方式，大多数情况下在某一位置断开，少数在苯环的对位同时断开，然后与体系中近邻的自由基反应。同时还注意到发生裂解的苯环所带的基团数量明显少于其他分子，且断键位置为自由基脱落位置的邻位。为了分析自由基脱落对苯环稳定性的影响，计算了脱落一个硝基的TATB分子苯环上碳键的COHP(Crystal Orbital Hamilton Population)和原子电荷布局。COHP可以呈现两原子间结合力的强弱，且COHP的绝对值越大，两原子间结合力越强。若将苯环上的C原子按顺时针分别标记为C1、C2、C3、C4、C5和C6，其中C1所连接的-NO2基团脱落。结果显示：硝基脱落位置C1与邻位C2、C6所成键的COHP为-6.1 eV，邻位C2与间位C3之间的键以及C6与C5之间键的COHP约为-4.0 eV，而苯环上其他C原子间成键的COHP约为-5.0 eV。说明硝基脱落位的C与邻位C的成键增强，而邻位与间位的成键变弱，由此认为在自由基脱落之后，苯环的稳定性受到影响，更容易在其自由基脱落位置(C1)的邻位(C2或C6)与间位(C3或C5)处发生环裂。
2.1.4 C─N团簇的形成
在反应前期，H2O的生成过程消耗了一部分O原子；而在反应后期，由于TATB为负氧平衡的分子，苯环裂解生成的短链基团中只有少部分C能与O结合生成CO或CO2，剩下的C、N倾向于保持杂链/杂环状态，这些杂链可能会纠缠在一起形成大型团簇。大型团簇相对来说比较稳定，但是由于温度较高，本研究也观察到这些团簇与H2O以及CO2的相互作用，使得H2O和CO2的数量在反应本应趋于平缓的情况下出现较大波动。
从能量变化角度来看，虽然体系中残留着大型C-N-O团簇，但体系的能量和物种仅有小波动，基本保持不变，可以认为此时体系已经处于化学平衡状态。考虑到采用NVT系综模拟时体积是原本形成固态晶体时的体积，反应后包含大量气态产物的体系将处于过压状态(约15 GPa)，最终产物在该体积及温度下无法以气态存在，由此认为：虽然模拟达到了平衡，但是实际上只反映了爆轰反应区前段的分解过程，在反应区后段压力逐渐降低，反应区物质密度有较大的变化，从而影响反应机理，而该过程应由压力主导，其相关机理不在本研究所讨论的范围之内。
2.2 外部环境对分解过程的影响
仅从能量角度上看，整个反应进程可以分为吸热(势能上升)和放热(势能下降)两个阶段，且吸热阶段时间小于放热阶段。通过对比势能和中间产物随时间的变化，可以确定在吸热阶段主要进行TATB初始分解的质子转移以及C─NO2均裂过程。
2.2.1 温度的影响
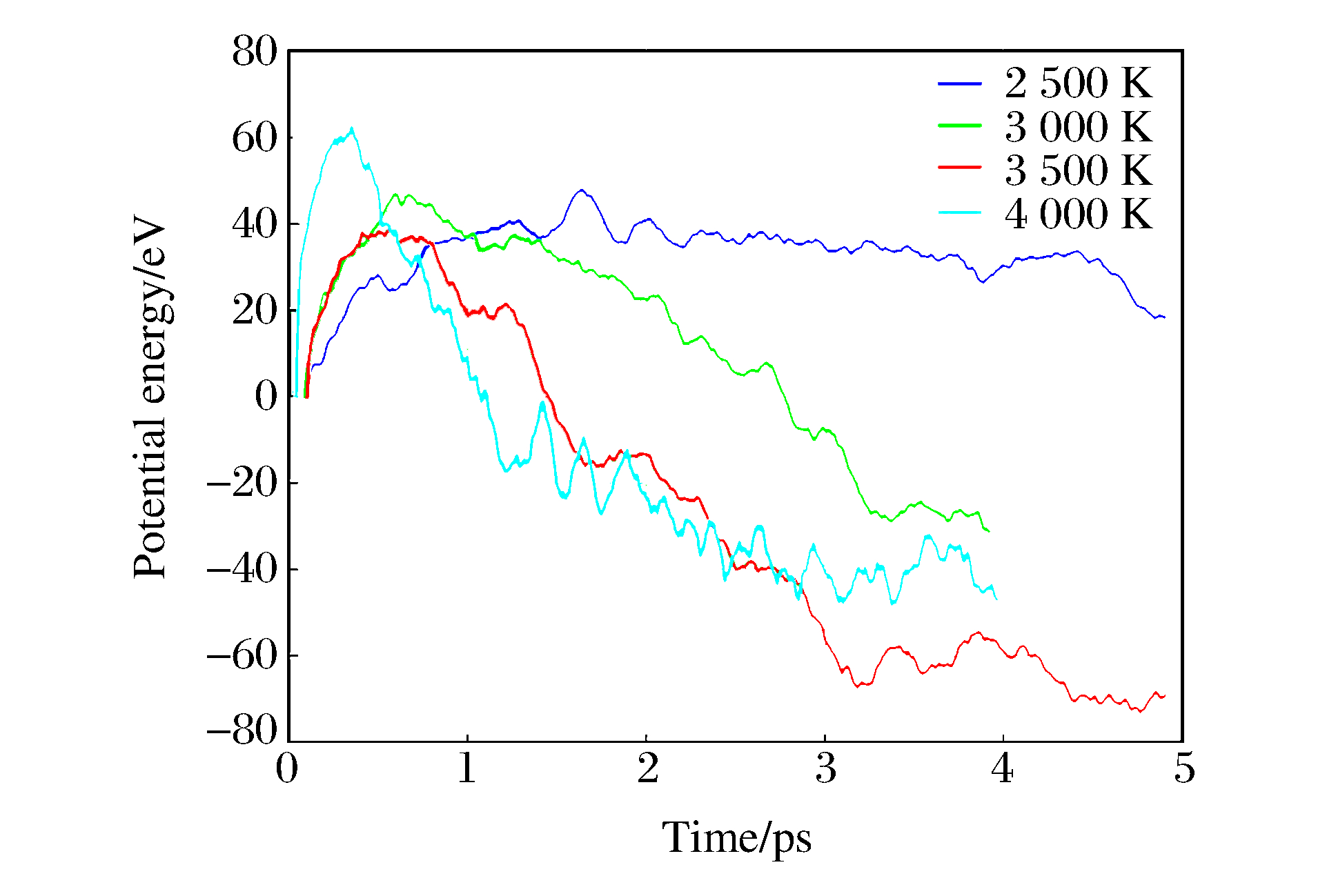
通过考察不同温度下体系的势能曲线(见图 4)，可以估算各温度下TATB的反应速率。从图 4中可以看出，温度越高，达到平衡所需的时间越短，意味着反应速度越高。设τ为放热阶段达到平衡所需时间，通过(1)式对势能曲线进行拟合，可以得到反应特征时间τ。
U(t)=U0+Δq exp(−t−tindτ) (1) 
图 4 不同温度下TATB体系势能的演化曲线Figure 4. Potential energy curves of TATB decomposition at different temperatures式中：U为体系内能；t为时间；U0为终产物内能；Δq为反应放热；tind为放热开始时间，即图 3中能量开始释放的时间。假设反应速率k与反应特征时间τ成反比，即k ∝1/τ，则得到不同温度下的反应速率k(T)。利用阿伦尼乌斯公式
k(T)=A exp(−Ea/kBT) (2) 对k(T)进行拟合，得到表观反应激活能Ea和指前因子A((2)式中T为温度，kB为玻尔兹曼常数)。针对吸热阶段，如果将放热开始时间tind定义为吸热的特征时间，则同样也可以得到吸热阶段的表观反应激活能和指前因子，最终得到反应速率模型参数，如表 2所示。
表 2 TATB反应动力学参数Table 2. Chemical kinetic parameters of TATBReaction step A/(lg s-1) Ea/(kJ·mol-1) Δq/(J·g-1) 1 31.8 127.30 925 2 30.0 209.76 -2 797 需要注意的是，在用阿伦尼乌斯公式拟合计算反应速率时假定反应机理不随温度变化，否则激活能将不再是定值，而随温度的变化而改变。在实际模拟过程中，注意到随着温度的上升，N2的生成方式逐渐发生变化。在较低温度下，N2的生成主要依赖于-NO基团与-NH基团的作用。而在高温情况下，苯环裂解发生得很快，在没有基团脱落的情况下，苯环也会发生均裂。在这种情况下，体系内只有少量-NO生成，大量的C-N-O杂链相互碰撞产生N2、CO2、H2O等气体分子。由于NO2在脱离苯环之前苯环就裂解了，所以部分N被束缚在杂链中无法有效地参与N2的生成，最终导致高温下N2的产率比低温下低。同时，分析显示，温度的变化对于体系中C-N-O团簇的大小和各元素配比几乎没有影响，说明后续C-N-O团簇的演化很可能受环境压力的控制。
2.2.2 压力的影响
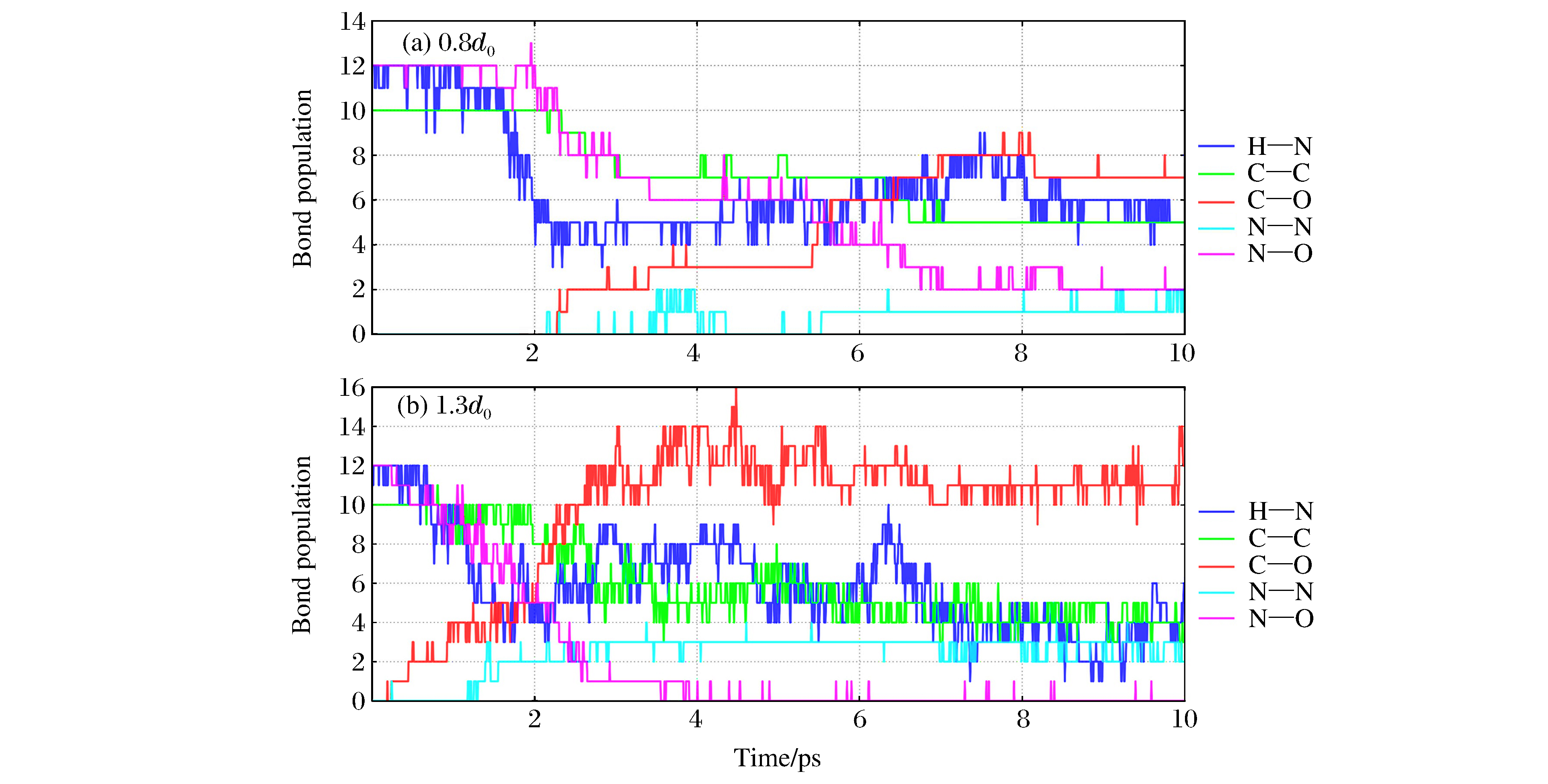
模拟了0.8d0、0.9d0、1.2d0和1.3d0 4种密度的TATB晶体在2 500 K下的反应过程。4种密度所对应的初始压力分别为0、0、16和25 GPa。模拟结果显示，密度越大，反应速率越高。从成断键变化曲线(见图 5)上看，1.3d0体系中各种键的断裂速度均高于0.8d0体系。如果排除不同体系中各种键断裂前的平台阶段，注意到H─N键和N─O键的减少速率在不同压力下几乎相同，甚至在0.8d0体系下H─N键的减少速率更快些。而其他键，包括C─O键、N─N键这些与N2和CO2相联系的键的变化速率则在高压下更快。通过观察分子的运动轨迹发现：在低密度情况下，不同层间的TATB分子在反应初期出现相对滑移；而在高密度情况下，分子靠得较近，几乎没有滑移情况出现。
图 5 不同密度和压力下TATB分解产物随时间的变化曲线Figure 5. Products population curves of TATB at different densities and pressures综上所述，可以认为：第1阶段中NO2的分解依赖于压力，且压力越大，物质越紧密，NO2越难从基体上脱落；但是随后的放热过程依靠苯环的分解和邻近自由基的反应，材料内部剩下的都是较小的自由基，自由基靠得越近，反应概率越大。该现象与实验上密度越高则爆压和爆速越高的特性一致。
2.2.3 黏结剂的影响
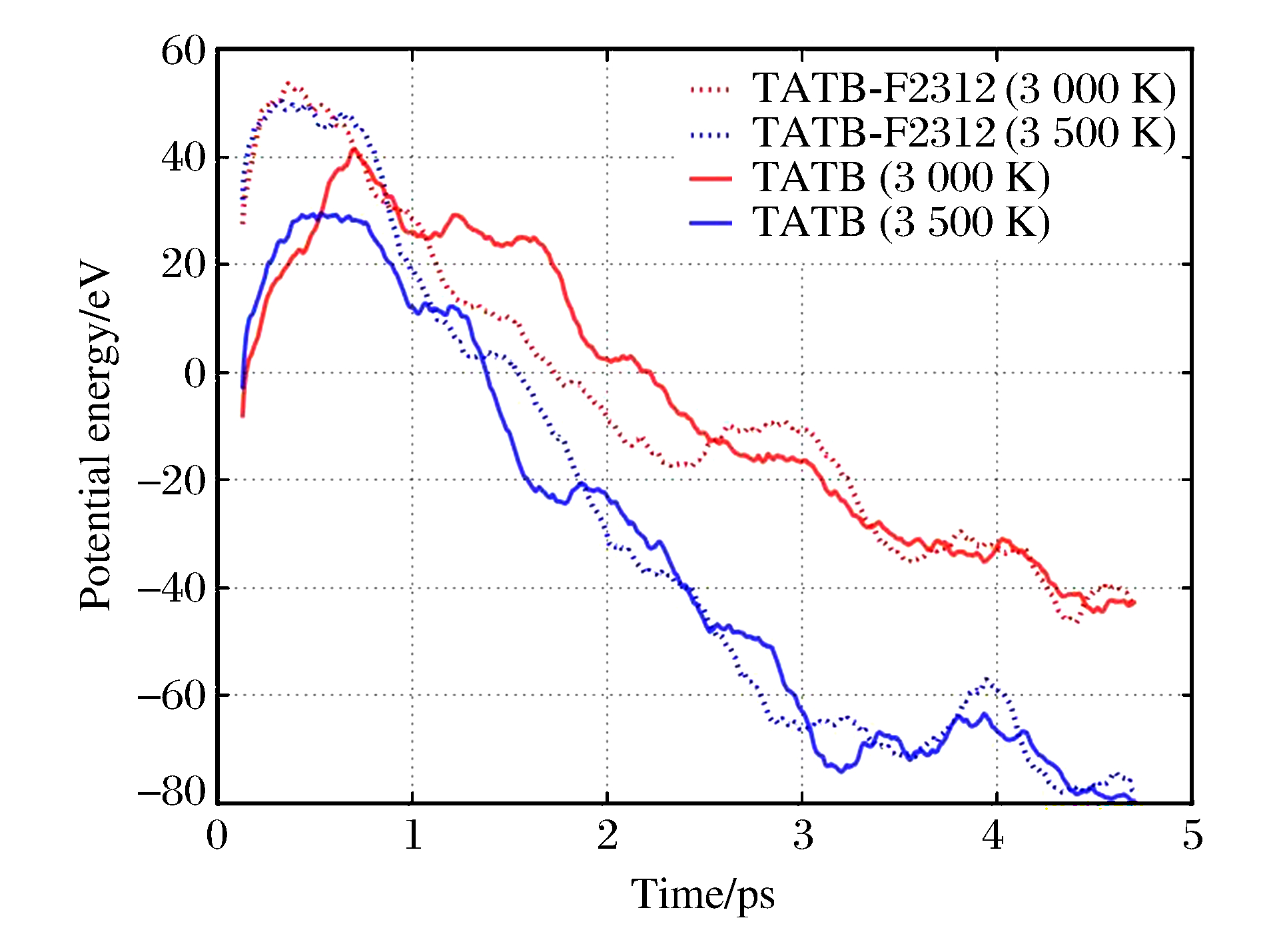
图 6显示了3 000及3 500 K下带有F2312氟聚物黏结剂及纯TATB体系的势能随时间的变化。为便于比较，将相同温度下不同体系的势能曲线在横轴末端对齐。从图 6中可以明显地看出，带有黏结剂的TATB体系放能更多。然而经过换算得出，带有黏结剂的TATB体系的单位质量放能仅为纯TATB体系的83%，说明黏结剂虽然参与反应且释放能量，但是仍然无法与等密度TATB对体系能量的贡献相比，最终导致整个体系的能量水平降低。另一方面，单独观察3 000或3 500 K下体系的能量释放情况可知，含有黏结剂的TATB体系在吸热阶段的势能变化速率略高于纯TATB体系。在经历一段快速放热之后，含有黏结剂的TATB体系与纯TATB体系的势能曲线变化趋势几乎一致。
图 6 不同温度下含有氟聚物黏结剂和纯TATB体系的势能演化曲线Figure 6. Potential energy curves of TATB with or without fluoropolymer binders at different temperatures为了理解氟聚物黏结剂如何参与反应进程，研究了中间物质和断键情况。分析结果显示，黏结剂的首步分解为F原子的转移，其次为碳链的扭转及断裂，而Cl原子几乎不参与反应。在TATB与黏结剂界面处，TATB首步分解过程中的H转移和C─NO2断裂速度高于TATB内部分解速度。对于黏结剂而言，F原子的转移速度低于TATB中H原子的转移速度，同时TATB初始分解生成的游离H与F原子结合生成HF；该过程会消耗H，使体系中的O原子有更多机会参与CO2的生成过程，而非与H结合生成OH-和H2O，最终导致产物中CO2的比例提升，而H2O的比例下降。氟聚物的主体为长碳链，在反应中碳链互相缠绕形成较大的团簇，在形成团簇过程中部分游离的NO+和OH-被团簇捕获，体系中游离的NO+和OH-减少，限制了N2和CO2的生成速率。由此认为，正是该过程导致含有黏结剂体系的前期反应速率高于纯TATB体系而后期反应速率却降至与纯TATB体系一致。此外，还发现由TATB演化形成的C团簇具有较高比例的N、O原子，其中N原子数与C原子数的比值可达0.8，而由黏结剂形成的C团簇中N的比例则低很多，C原子与N原子的最终演化过程可能与纯TATB体系中C团簇的演化机理不同。
综合以上分析，证实了氟聚物黏结剂的引入会降低TATB热分解反应的单位质量放能，黏结剂与TATB反应生成HF，导致产物中H2O的含量降低，CO2的含量增加。氟聚物对反应速率的影响是多种因素综合作用的结果，无法得出明确结论。同时，由于现有系综无法对碳聚物的演化过程进行模拟，黏结剂分解生成C团簇的影响仍需要进一步的研究和确认。
3. 结论
采用从头算分子动力学方法，详细地研究了TATB在高温环境下的分解过程。通过追踪原子的移动以及产物随时间的变化，确认TATB在高温下分解的首步为质子转移以及C─NO2键断裂，中间产物为H2O和NO，最终产物有N2、CO2和H2O。这些反应过程大部分依赖于不同基团之间的碰撞和扩散，因此温度越高，自由基的运动能力越强，反应速率越快。压力的影响比较复杂：在高密度情况下，一方面依赖于碰撞的反应几率增大，使反应速率增大，而另一方面由于高密度带来笼型效应，产生自由基的反应速率变低；总体上，压力越高，反应速率越高。黏结剂在较高温度下会参与反应，且对反应路径及最终产物的配比存在一定的影响。
-
图 1 (a)活塞法生成层裂示意图，(b) 载荷时程曲线
Figure 1. (a) Schematic diagram of spallation generation by piston method; (b) loading history curves
图 2 层裂强度（整体法）对最大速度和脉冲形状的依赖性
Figure 2. Dependence of spall strength (from bulk) on the maximum velocity and pulse shape
图 3 层裂强度（自由面法）对最大速度和脉冲形状的依赖性
Figure 3. Dependence of spall strength (from surface) on the maximum velocity and pulse shape
图 5 外加拉伸应变率与层裂温度及脉冲形状的关系
Figure 5. Dependency of applied tensile strain rate on spall temperature and pulse shape
图 7 层裂强度与层裂面温度的关系
Figure 7. Relationship between spall strength and temperature on the spall plane
图 8 应力波抵达自由表面时系统的原子构型
Figure 8. Atomic configuration of the system when the stress wave reaches the free surface
图 9 vp 为1.00和2.00 km/s时方波和斜波组中σxx的位置-时间（x-t）图
Figure 9. Position of σxx versus time (x-t) diagrams when vp is 1.00 and 2.00 km/s under square wave and ramp wave loading
图 11 vp 为1.25和1.50 km/s时斜波组层裂面上的缺陷结构随时间的演化
Figure 11. Time evolution of corresponding defect structure on the spall plane when vp is 1.25 and 1.50 km/s under ramp wave loading
图 12 斜波和方波加载下空洞数量和损伤比的演变历程
Figure 12. Evolution history of void number and damage ratio under square wave and ramp wave loading
表 1 不同工况下的层裂强度、应变速率和层裂温度
Table 1. Spall strength, strain rate, and spall temperature of different cases
Group vp/(km·s−1) σsp/GPa ˙ε/(109 s−1) Tsp/K From bulk From surface Ramp wave 1.00 9.748 8.536 1.864 223.82 1.25 8.814 7.528 2.350 272.24 1.50 9.847 7.851 2.983 224.95 1.75 9.941 8.678 3.282 227.39 2.00 10.084 8.233 3.664 230.75 2.25 10.358 7.546 4.123 233.08 2.50 10.321 7.077 4.556 236.06 2.75 10.308 6.764 4.855 236.80 3.00 10.261 6.325 5.284 239.73 Square wave 1.00 8.180 7.725 2.287 333.47 1.25 8.228 7.151 2.477 313.84 1.50 9.511 8.646 2.670 291.85 1.75 9.227 8.282 3.263 350.29 2.00 8.689 7.578 3.611 404.72 2.25 7.977 7.039 4.055 494.94 2.50 7.293 6.809 4.461 561.94 2.75 6.900 6.518 4.901 579.97 3.00 6.551 6.135 5.312 594.51 
下载: 导出CSV
-
[1] LI C, LI B, HUANG J Y, et al. Spall damage of a mild carbon steel: effects of peak stress, strain rate and pulse duration [J]. Materials Science and Engineering: A, 2016, 660: 139–147. doi: 10.1016/j.msea.2016.02.080 [2] LI C, YANG K, TANG X C, et al. Spall strength of a mild carbon steel: effects of tensile stress history and shock-induced microstructure [J]. Materials Science and Engineering: A, 2019, 754: 461–469. doi: 10.1016/j.msea.2019.03.019 [3] NGUYEN T, LUSCHER D J, WILKERSON J W. A physics-based model and simple scaling law to predict the pressure dependence of single crystal spall strength [J]. Journal of the Mechanics and Physics of Solids, 2020, 137: 103875. doi: 10.1016/j.jmps.2020.103875 [4] LI C, YANG K, GAO Y H, et al. Dislocation-dominated void nucleation in shock-spalled single crystal copper: mechanism and anisotropy [J]. International Journal of Plasticity, 2022, 155: 103331. doi: 10.1016/j.ijplas.2022.103331 [5] REMINGTON T P, HAHN E N, ZHAO S, et al. Spall strength dependence on grain size and strain rate in tantalum [J]. Acta Materialia, 2018, 158: 313–329. doi: 10.1016/j.actamat.2018.07.048 [6] PANG B, CASE S, JONES I P, et al. The defect evolution in shock loaded tantalum single crystals [J]. Acta Materialia, 2018, 148: 482–491. doi: 10.1016/j.actamat.2017.11.052 [7] HAHN E N, FENSIN S J, GERMANN T C, et al. Orientation dependent spall strength of tantalum single crystals [J]. Acta Materialia, 2018, 159: 241–248. doi: 10.1016/j.actamat.2018.07.073 [8] DONGARE A M, LAMATTINA B, RAJENDRAN A M. Atomic scale studies of spall behavior in single crystal Cu [J]. Procedia Engineering, 2011, 10: 3636–3641. doi: 10.1016/j.proeng.2011.04.598 [9] MACKENCHERY K, VALISETTY R R, NAMBURU R R, et al. Dislocation evolution and peak spall strengths in single crystal and nanocrystalline Cu [J]. Journal of Applied Physics, 2016, 119(4): 044301. doi: 10.1063/1.4939867 [10] MA K, CHEN J, DONGARE A M. Role of pre-existing dislocations on the shock compression and spall behavior in single-crystal copper at atomic scales [J]. Journal of Applied Physics, 2021, 129(17): 175901. doi: 10.1063/5.0040802 [11] WANG J, WANG F, ZENG X G, et al. Unraveling the plasticity performance and melting in single crystal tantalum damaged by shock compression [J]. Engineering Fracture Mechanics, 2022, 276: 108921. doi: 10.1016/J.ENGFRACMECH.2022.108921 [12] KRASNIKOV V S, MAYER A E. Plasticity driven growth of nanovoids and strength of aluminum at high rate tension: molecular dynamics simulations and continuum modeling [J]. International Journal of Plasticity, 2015, 74: 75–91. doi: 10.1016/j.ijplas.2015.06.007 [13] ZHOU T T, HE A M, WANG P, et al. Spall damage in single crystal Al with helium bubbles under decaying shock loading via molecular dynamics study [J]. Computational Materials Science, 2019, 162: 255–267. doi: 10.1016/j.commatsci.2019.02.019 [14] TANG F, JIAN Z Y, XIAO S F, et al. Molecular dynamics simulation of cylindrically converging shock response in single crystal Cu [J]. Computational Materials Science, 2020, 183: 109845. doi: 10.1016/j.commatsci.2020.109845 [15] XIONG Q L, KITAMURA T, LI Z H. Cylindrical voids induced deformation response of single crystal coppers during low-speed shock compressions: a molecular dynamics study [J]. Mechanics of Materials, 2019, 138: 103167. doi: 10.1016/j.mechmat.2019.103167 [16] TIAN X, CUI J Z, MA K P, et al. Shock-induced plasticity and damage in single-crystalline Cu at elevated temperatures by molecular dynamics simulations [J]. International Journal of Heat and Mass Transfer, 2020, 158: 120013. doi: 10.1016/j.ijheatmasstransfer.2020.120013 [17] LIAO Y, XIANG M Z, ZENG X G, et al. Molecular dynamics studies of the roles of microstructure and thermal effects in spallation of aluminum [J]. Mechanics of Materials, 2015, 84: 12–27. doi: 10.1016/j.mechmat.2015.01.007 [18] HAWKINS M C, THOMAS S A, FENSIN S J, et al. Spall and subsequent recompaction of copper under shock loading [J]. Journal of Applied Physics, 2020, 128(4): 045901. doi: 10.1063/5.0011645 [19] ZHU Y L, HU J N, WEI Q Q, et al. Enhanced spall strength of single crystal aluminum by temperature rise mitigation and structural phase transition under shock pulse [J]. Mechanics of Materials, 2023, 186: 104809. doi: 10.1016/j.mechmat.2023.104809 [20] 黄海军, 沈强, 罗国强, 等. 利用多层阻抗梯度飞片产生准等熵压缩的理论解析 [J]. 物理学报, 2007, 56(3): 1538–1542. doi: 10.3321/j.issn:1000-3290.2007.03.050HUANG H J, SHEN Q, LUO G Q, et al. Theoritical analysis of quasi-isentropic compression via flier-plate with grade wave impadence [J]. Acta Physica Sinica, 2007, 56(3): 1538–1542. doi: 10.3321/j.issn:1000-3290.2007.03.050 [21] ZHU Y L, HU J N, HUANG S L, et al. Molecular dynamics simulation on spallation of [111] Cu/Ni nano-multilayers: voids evolution under different shock pulse duration [J]. Computational Materials Science, 2022, 202: 110923. doi: 10.1016/J.COMMATSCI.2021.110923 [22] ZHAKHOVSKII V V, INOGAMOV N A, PETROV Y V, et al. Molecular dynamics simulation of femtosecond ablation and spallation with different interatomic potentials [J]. Applied Surface Science, 2009, 255(24): 9592–9596. doi: 10.1016/j.apsusc.2009.04.082 [23] JIANG D D, SHAO J L, WU B, et al. Sudden change of spall strength induced by shock defects based on atomistic simulation of single crystal aluminum [J]. Scripta Materialia, 2022, 210: 114474. doi: 10.1016/j.scriptamat.2021.114474 [24] KADAU K, GERMANN T C, LOMDAHL P S, et al. Atomistic simulations of shock-induced transformations and their orientation dependence in bcc Fe single crystals [J]. Physical Review B, 2005, 72(6): 064120. doi: 10.1103/PhysRevB.72.064120 [25] SRINIVASAN S G, BASKES M I, WAGNER G J. Atomistic simulations of shock induced microstructural evolution and spallation in single crystal nickel [J]. Journal of Applied Physics, 2007, 101(4): 043504. doi: 10.1063/1.2423084 [26] ASHITKOV S I, AGRANAT M B, KANEL’ G I, et al. Behavior of aluminum near an ultimate theoretical strength in experiments with femtosecond laser pulses [J]. JETP Letters, 2010, 92(8): 516–520. doi: 10.1134/S0021364010200051 [27] ELIEZER S, MOSHE E, ELIEZER D, et al. Laser-induced tension to measure the ultimate strength of metals related to the equation of state [J]. Laser and Particle Beams, 2002, 20(1): 87–92. doi: 10.1017/S0263034602201123 [28] GARKUSHIN G V, KANEL G I, RAZORENOV S V, et al. Resistance to deformation and fracture of aluminum AD1 under shock-wave loading at temperatures of 20 and 600 ℃ [J]. Physics of The Solid State, 2010, 52(11): 2369–2375. doi: 10.1134/S1063783410110247 [29] KANEL G I, RAZORENOV S V, GRADY D E, et al. Spall fracture properties of aluminum and magnesium at high temperatures [J]. Journal of Applied Physics, 1996, 79(11): 8310–8317. doi: 10.1063/1.362542 [30] RAZORENOV S V, KANEL G I, FORTOV V E, et al. Submicrosecond strength of aluminum and alloy AMg6M at normal and elevated temperatures [J]. Fizika Metallov Ⅰ Metallovedenie, 2003, 95(1): 91–96. [31] KANEL G I, RAZORENOV S V, SINGER J, et al. Dynamic yield and tensile strength of aluminum single crystals at temperatures up to the melting point [J]. Journal of Applied Physics, 2001, 90(1): 136–143. doi: 10.1063/1.1374478 [32] STRACHAN A, ÇAĞIN T, GODDARD Ⅲ W A. Critical behavior in spallation failure of metals [J]. Physical Review B, 2001, 63(6): 060103. doi: 10.1103/PhysRevB.63.060103 -
下载:
下载:
点击查看大图
计量
- 文章访问数: 206
- HTML全文浏览量: 80
- PDF下载量: 53
