三周期极小曲面结构混合设计及其在冲击载荷下的力学行为

刘嘉婧; 李子豪; 王志华; 刘志芳; 李世强

doi:10.11858/gywlxb.20240783

三周期极小曲面结构混合设计及其在冲击载荷下的力学行为

doi: 10.11858/gywlxb.20240783

太原理工大学机械与运载工程学院应用力学研究所, 山西太原　030024

基金项目: 国家自然科学基金（12072219，12272254）；山西省自然科学研究面上项目（202203021211170）；海安太原理工大学先进制造与智能装备产业研究院开放研发项目（2023HA-TYUTKFYF016）；山西省科技创新人才团队（领军）专项（202204051002006）

详细信息

作者简介:
刘嘉婧（1997－），女，硕士研究生，主要从事轻质材料冲击动力学行为研究.E-mail：liujiajing199709@163.com

通讯作者:
李世强（1986－），男，博士，副教授，主要从事结构冲击动力学行为研究. E-mail：lishiqiang@tyut.edu.cn

中图分类号: O521.9; O347.1
计量
- 文章访问数: 363
- HTML全文浏览量: 98
- PDF下载量: 29
出版历程
- 收稿日期: 2024-04-03
- 修回日期: 2024-04-23
- 录用日期: 2024-06-19
- 网络出版日期: 2024-08-13
- 刊出日期: 2024-09-29

Hybrid Design of Triply Periodic Minimal Surface Structure and Its Mechanical Behavior under Impact Loading

Institute of Applied Mechanics, College of Mechanical and Vehicle Engineering, Taiyuan University of Technology, Taiyuan 030024, Shanxi, China

摘要

摘要: 三周期极小曲面（triply periodic minimal surface，TPMS）结构材料作为一种高孔隙率和高能量吸收效率的多孔介质，在许多领域得到广泛应用。以Gyroid和IWP结构作为设计基元，利用Sigmoid函数构建圆柱形过渡层，将外层IWP结构与内层Gyroid结构连接，设计了内外嵌套的GIP混合胞元结构。通过选择性激光熔融技术打印了Gyroid结构、IWP结构和GIP混合结构试样，并利用直撞式霍普金森杆对其进行了实验研究。结合LS-DYNA软件进行了更大冲击速度范围的数值模拟，分析了试件的变形演化过程和动态应力-应变关系。结果表明：结构的初始峰值应力和比吸能表现出不同程度的应变率敏感性。与Gyroid和IWP结构相比，GIP混合结构材料的应力-应变曲线表现出更明显的应变硬化趋势和更强的能量吸收能力。相较于GIP-1结构（冲击方向与圆柱形过渡层轴线方向相同），随着冲击速度的提高，GIP-2结构（冲击方向与圆柱形过渡层轴线方向垂直）具有更低的初始峰值应力和更大的比吸能，因而具有更优异的抗冲击性能。
- TPMS混合结构 /
- 3D打印 /
- 动态加载 /
- 能量吸收
Abstract: Triply periodic minimal surface (TPMS) structural material is widely used in many fields as a porous medium with high porosity and high energy absorption efficiency. In this paper, the Gyroid and IWP structures were used as the design elements, and the Sigmoid function was used to construct the cylindrical transition layer. The outer IWP structure was connected with the inner Gyroid structure, hence the inner and outer nested GIP hybrid cellular structure was designed. Gyroid structure, IWP structure and GIP hybrid structure samples were printed by selective laser melting technology, and the experimental study was performed by direct impact Hopkinson bar. Combined with LS-DYNA software, the numerical simulation of larger impact velocity range was carried out, and the deformation evolution process as well as dynamic stress-strain relationship of the specimen were analyzed. The results show that the initial peak stress and specific energy absorption of the structure present different strain rate sensitivity. Compared with Gyroid and IWP structures, the stress-strain curves of GIP hybrid structural materials exhibit more obvious strain hardening trend and stronger energy absorption capacity. With the increase in impact velocity, the GIP-2 structure (the impact direction is perpendicular to the axis direction of the cylindrical transition layer) presents lower initial peak stress and larger specific energy absorption than the GIP-1 structure (the impact direction is the same as the axis direction of the cylindrical transition layer), which demonstrates its better impact resistance.
- TPMS hybrid structure /
- 3D printing /
- dynamic loading /
- energy absorption

HTML全文

泡沫金属质轻，具有较高的比刚度和比强度，以及隔热、电磁屏蔽等物理性能^[1]，在汽车交通、铁路、航空航天等领域广泛应用，如用于飞机外壳夹层、汽车防冲档。除此之外，泡沫金属在受到压缩时，由于其应变滞后于应力，压缩应力-应变曲线中有一个很长的低应力平台，可承受较大的塑性变形，因此泡沫金属具有良好的吸能特性，可用于缓和冲击的工程构件、能量吸收和防振构件^[2]。

目前，常温下泡沫金属的静/动态本构关系已经得到广泛的研究。Chen和Lu^[3]提出了一个依赖于特征应力和总应变的应力势，在此基础上建立了一个唯象的可压缩弹塑性本构模型的框架。该模型避免了人为区分应力-应变的弹塑性区带来的影响。王二恒等^[4]利用Chen和Lu^[3]提出的唯象本构模型框架，建立了一个泡沫金属准静态本构模型，得到了泡沫金属在三维等比例压缩和侧向受约束轴向压缩时的宏观应力-应变曲线。王志华等^[5]提出了一个多参数的非线性弹塑性唯象本构模型，可以全面地描述泡沫金属材料线弹性段、应力平台段和密实段的典型三阶段变形特征。

泡沫金属是一种典型的低成本轻质材料，其复合结构有望作为近空间飞行器中的重要结构部件。但是，近空间飞行器苛刻的应用环境不但要求其结构轻质化，而且要求泡沫金属在高温下有较好的承载、隔热和冲击吸能能力。目前，考虑温度效应的泡沫金属力学行为的研究还比较少。Hakamada等^[6]开展了ALPORAS闭孔泡沫铝及其基体材料在温度范围573~ 773 K内的准静态压缩试验，发现闭孔泡沫铝在高温下的变形机制与其基体材料的变形机制本质上是相同的。Aly^[7]开展了ALPORAS闭孔泡沫铝在常温和高温下的压缩实验，研究了相对密度和实验温度的影响，研究发现，胞壁屈曲是闭孔泡沫铝的主要变形机制，增大密度与升高实验温度对泡沫金属力学性能的影响刚好相反。Cady等^[8]研究了ALPORAS泡沫铝在不同应变率(0.001~1 800 s^-1)和不同温度条件(77~295 K)下的力学性能，结果表明，闭孔泡沫铝的力学性能对温度具有很强的依赖性。

考虑泡沫金属温度效应的本构关系相关研究也比较匮乏。习会峰等^[9]开展了-50~300 ℃范围内不同温度下泡沫铝的静态压缩实验，建立了考虑温度效应的泡沫铝静态压缩本构模型。王鹏飞等^[10]基于Sherwood和Frost提出的本构关系框架，分析了泡沫铝本构方程中温度效应与应变率效应的耦合关系，对本构方程中的应变率敏感系数进行了适当修正，修正后的本构关系与实验结果的重合性较好，在此基础上得到了泡沫铝在一定密度范围内包含温度、应变率影响的较为完备的本构方程。

本研究通过开展不同密度(0.322~0.726 g/cm³)的泡沫铝在不同温度(25~500 ℃)下的准静态压缩实验，分析泡沫铝在不同温度下的力学性能，测得不同温度下泡沫铝的单轴压缩应力-应变曲线。基于Liu-Subhash模型^[11]，对不同温度下的实验数据进行拟合，建立考虑温度效应和密度效应的泡沫铝准静态压缩本构模型。

1. 实验方法及方案

实验材料为闭孔泡沫铝，由上海奥深特金属复合材料科技有限公司提供。本研究采用的闭孔泡沫铝材料原尺寸为400 mm×400 mm×600 mm，孔径介于2~5 mm之间。准静态压缩实验采用线切割技术加工圆柱形试件。为了保证均匀性，排除胞孔尺寸影响，试件高度和直径不小于5个胞孔，试件尺寸为Ø32 mm×15 mm。实验前，对每个试样进行称量，得到其真实的相对密度，测得试样密度范围为0.322~0.796 g/cm³。

准静态实验在MTS810材料试验机上进行，加载速率为0.001 s^-1。实验温度分别取25、200、275、350、425和500 ℃，高温实验在MTS810自带的高温箱中进行，温度误差为±5 ℃。

2. Liu-Subhash本构模型

为了准确描述泡沫材料应力-应变曲线的三阶段特征，Liu与Subhash^[11]提出了一维六参数本构模型

$\sigma \text{=}{{p}_{1}}\frac{{{\text{e}}^{{{p}_{2}}\varepsilon }}-1}{{{p}_{6}}+{{\text{e}}^{{{p}_{\text{3}}}\varepsilon }}}+{{\text{e}}^{{{p}_{4}}}}\left( {{\text{e}}^{{{p}_{5}}\varepsilon }}-1 \right)$

(1)

式中：σ为压缩应力，ε为压缩应变，p₁、p₂、p₃、p₄、p₅和p₆为用于描述泡沫材料性质的参数。p₁主要描述压缩时屈服应力的变化；p₂和p₃为无量纲参数，主要描述应力平台阶段的硬化性能；p₄和p₅主要描述密实阶段的起点和密实阶段斜率的大小；p₆主要描述弹性段斜率的大小。从模型中可以看出，参数p₆的作用可以用p₁和p₃代替。习会峰等^[9]提出简化模型，用常数1取代参数p₆，将其简化为五参数模型

$\sigma \text{=}{{p}_{1}}\frac{{{\text{e}}^{{{p}_{2}}\varepsilon }}-1}{1+{{\text{e}}^{{{p}_{\text{3}}}\varepsilon }}}+{{\text{e}}^{{{p}_{4}}}}\left( {{\text{e}}^{{{p}_{5}}\varepsilon }}-1 \right)$

(2)

3. 实验结果与分析

3.1 包含密度效应的泡沫铝本构模型

3.1.1 密度对应力-应变曲线的影响

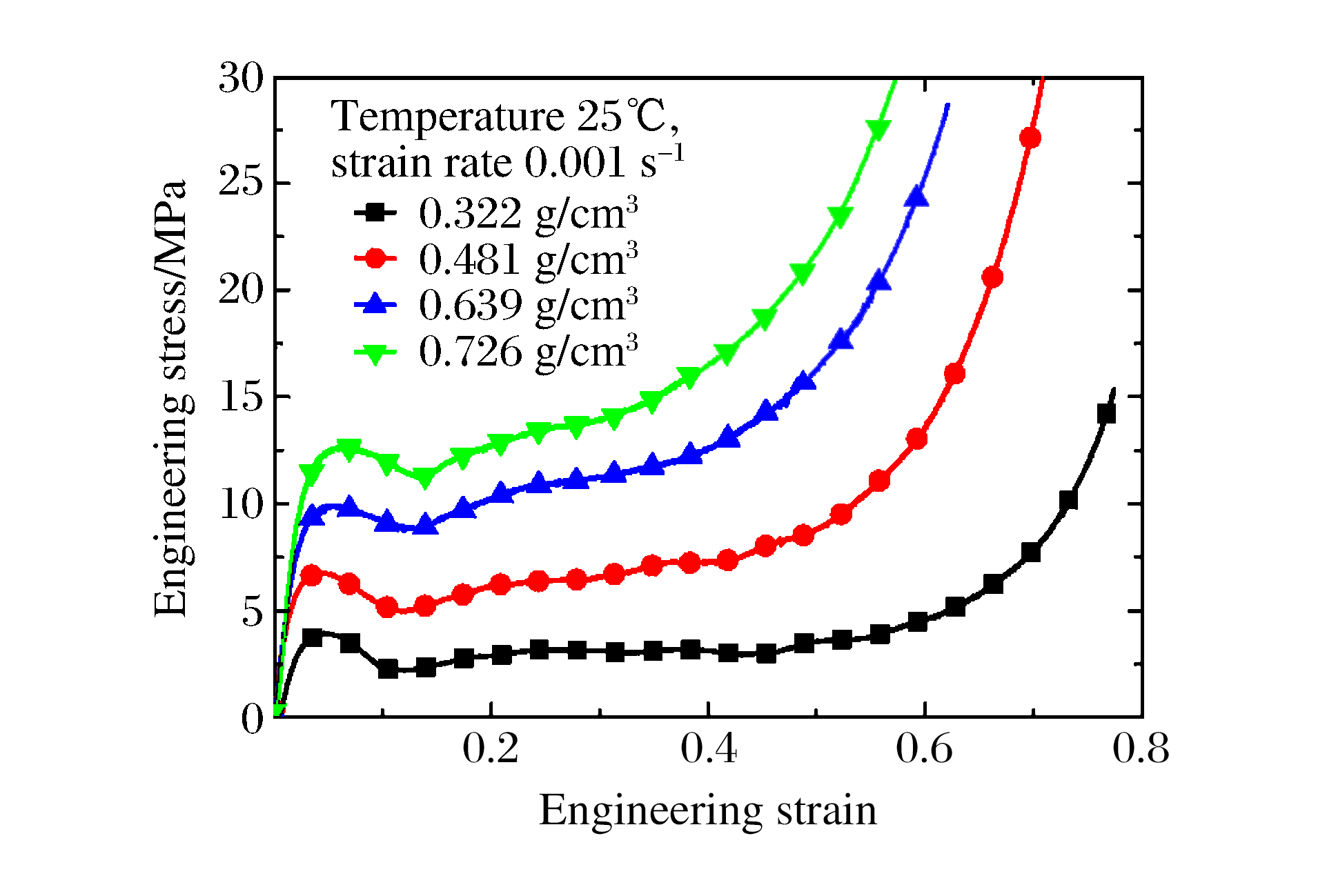
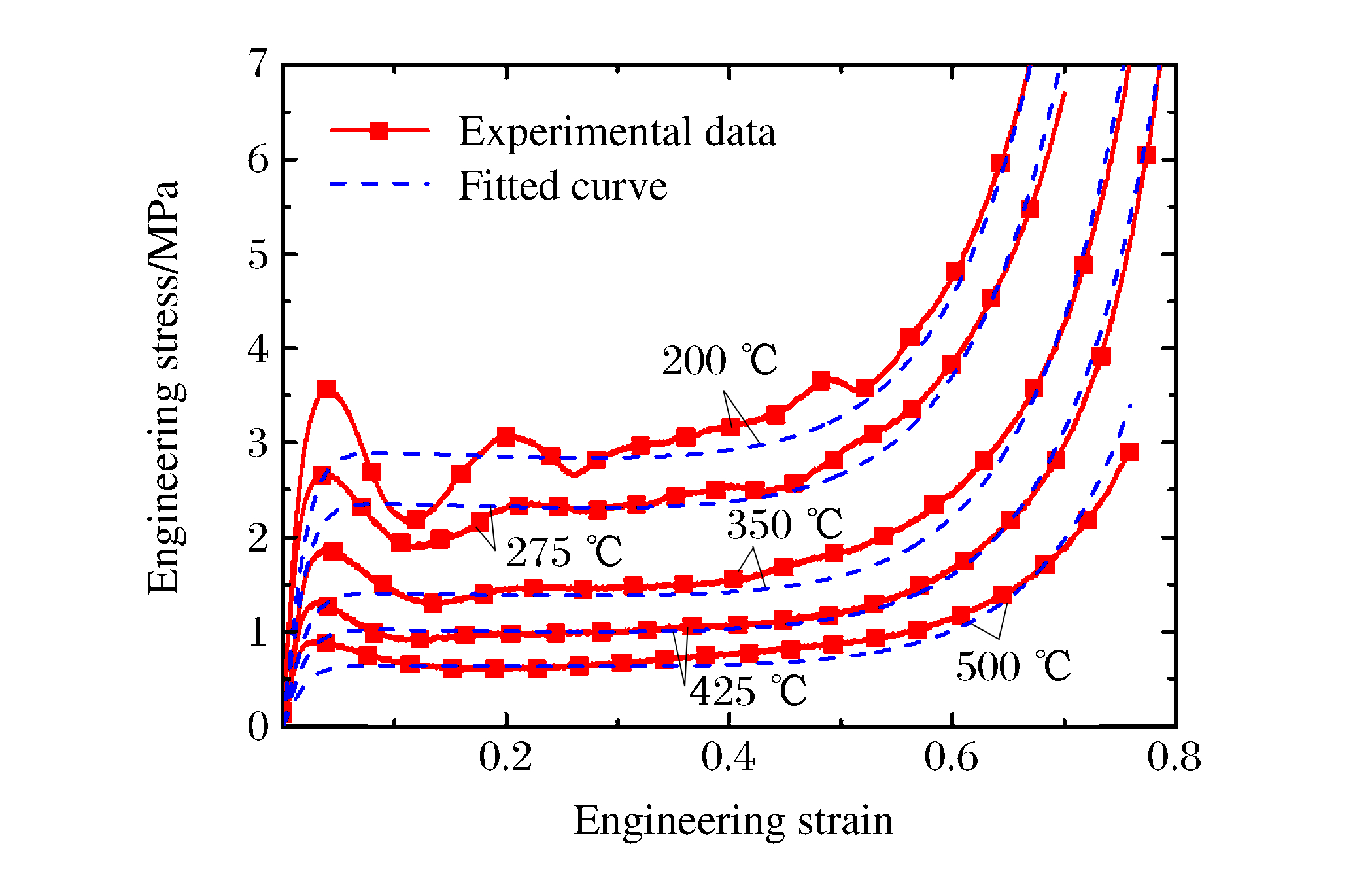
图 1为不同密度ρ下泡沫铝的准静态压缩应力-应变实验曲线。从实验结果来看，泡沫铝的应力-应变曲线的形状及发展趋势相似，呈典型的三阶段特性，即：较小应变下的线弹性阶段、对应于胞壁塑性坍塌而缓缓上升的应力平台阶段和密实后的应力迅速上升阶段。从图 1中可以看出，泡沫铝材料的初始密度对其应力-应变曲线影响巨大，随着密度的增大，曲线依次抬高，屈服强度也相应增大，但压缩至致密段的最大应变量减小。因此，在基体材料相同的情况下，在一定的密度范围内，泡沫铝材料的力学性能主要由材料的初始密度决定，初始密度越大，材料承载能力越强。

图 1 常温下不同密度泡沫铝的准静态应力-应变曲线

Figure 1. Quasi-static stress-strain curves of aluminum foam with different densities at room temperature

下载: 全尺寸图片幻灯片

3.1.2 基于Liu-Subhash模型的实验数据拟合

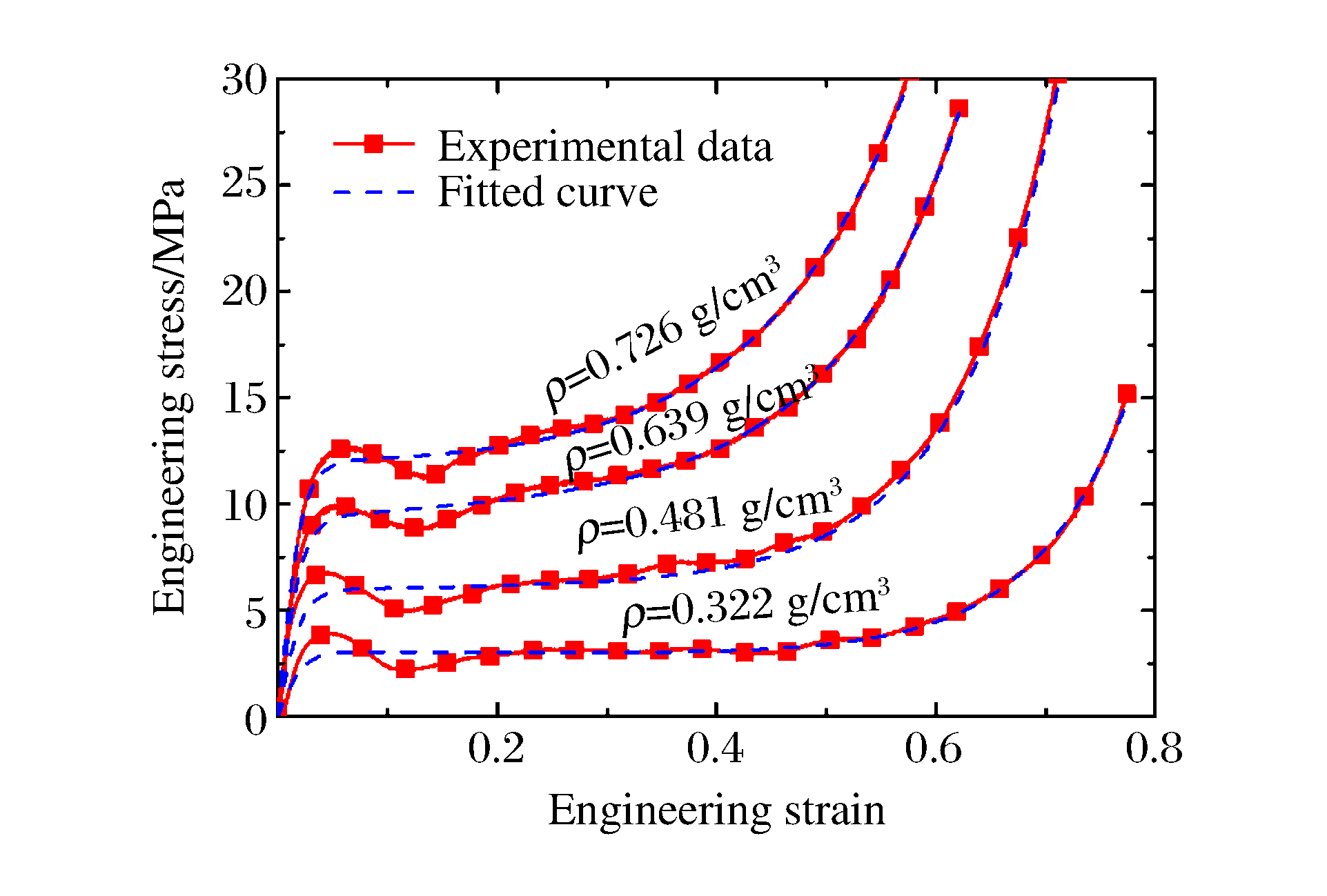
基于简化的Liu-Subhash模型，对实验得到的常温下不同密度泡沫铝的准静态应力-应变曲线进行拟合，得到不同密度泡沫铝材料的模型参数值，如表 1所示。实验曲线和拟合曲线如图 2所示，可见拟合效果较好，可采用该模型描述泡沫铝的单轴准静态压缩应力-应变关系。

表 1 不同密度下的模型参数值

Table 1. Parameter values for different densities

Density/(g·cm^-3)	Parameter
Density/(g·cm^-3)	p₁	p₂	p₃	p₄	p₅
0.322	3.08	86.09	86.22	-6.49	11.60
0.481	5.98	90.83	90.74	-4.66	10.98
0.639	9.31	91.20	90.89	-3.00	9.41
0.726	12.01	92.04	92.14	-1.33	7.41

下载: 导出CSV

| 显示表格

图 2 常温下不同密度泡沫铝的应力-应变曲线拟合情况

Figure 2. Fitting of stress-strain curves of aluminum foam with different densities at room temperature

下载: 全尺寸图片幻灯片

3.1.3 考虑密度效应的修正模型

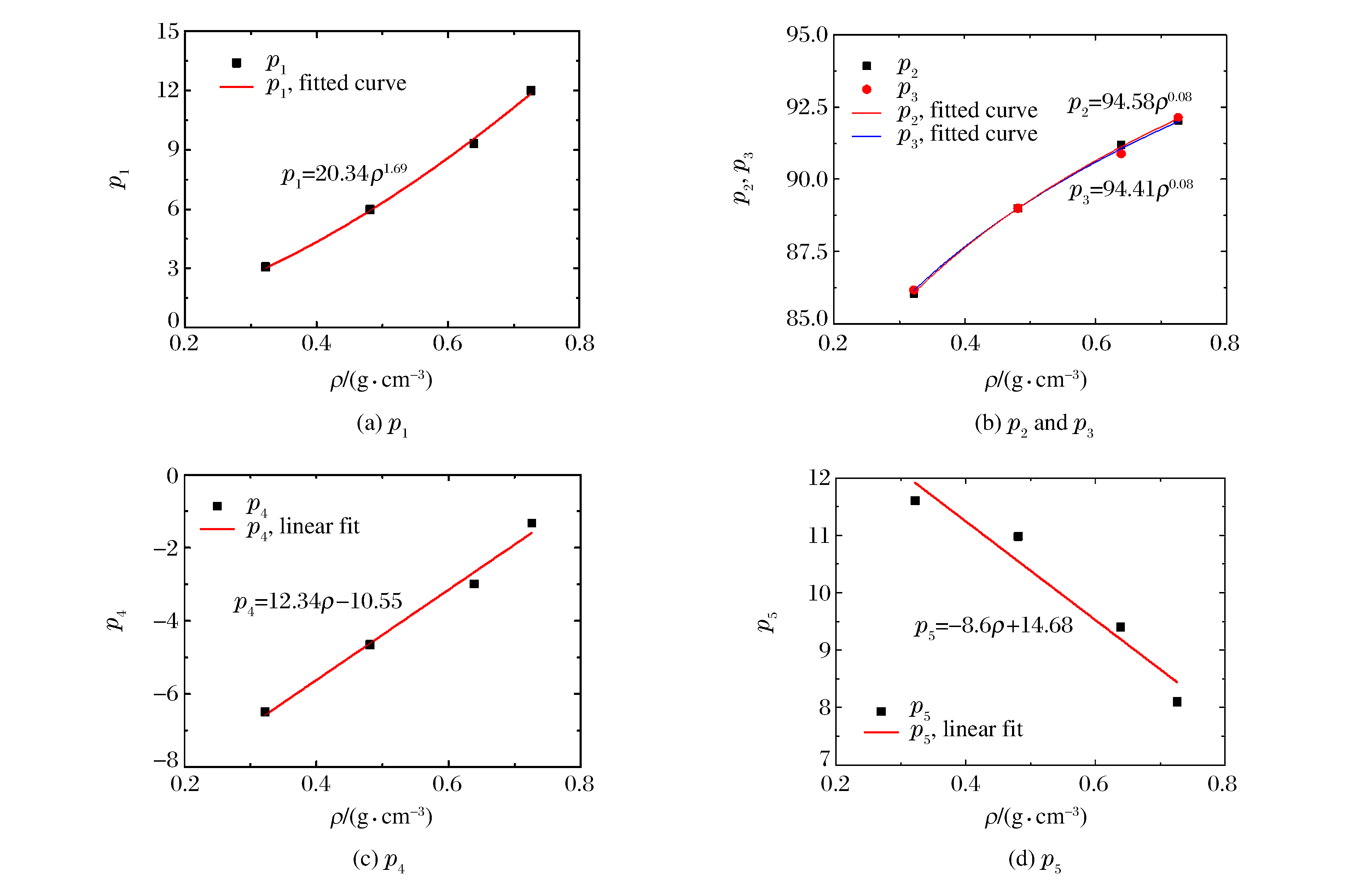
由表 1可知，参数p₁、p₂、p₃、p₄、p₅随密度的变化而变化，显然这5个参数都是密度ρ的函数。因此，(2)式可以写成

$\sigma \text{=}{{p}_{1}}\left( \rho \right)\frac{{{\text{e}}^{{{p}_{2}}\left( \rho \right)\varepsilon }}-1}{1+{{\text{e}}^{{{p}_{\text{3}}}\left( \rho \right)\varepsilon }}}+{{\text{e}}^{{{p}_{4}}\left( \rho \right)}}\left[ {{\text{e}}^{{{p}_{5}}\left( \rho \right)\varepsilon }}-1 \right]$

(3)

(3) 式即为考虑了密度影响的泡沫铝的常温准静态压缩本构模型。图 3为模型中5个参数随密度的变化情况，对实验数据进行拟合，得到5个参数与密度的关系为

$\left\{ \begin{align} & {{p}_{1}}\left( \rho \right)=20.34{{\rho }^{1.69}} \\ & {{p}_{2}}\left( \rho \right)=94.58{{\rho }^{0.08}} \\ & {{p}_{3}}\left( \rho \right)=94.41{{\rho }^{0.08}} \\ & {{p}_{4}}\left( \rho \right)=12.34\rho -10.55 \\ & {{p}_{5}}\left( \rho \right)=-8.6\rho +14.68 \\ \end{align} \right.$

(4)

图 3 常温下参数随密度的变化规律

Figure 3. Variation of parameters with density at room temperature

下载: 全尺寸图片幻灯片

由(3)式和(4)式得到考虑密度效应的闭孔泡沫铝静态压缩本构模型，该模型可用于描述不同密度下泡沫铝的应力-应变曲线，具体形式如下

$\sigma =20.34{{\rho }^{1.69}}\frac{{{\text{e}}^{94.58{{\rho }^{0.08}}\varepsilon }}-1}{1+{{\text{e}}^{94.41{{\rho }^{0.08}}\varepsilon }}}\text{+}{{\text{e}}^{12.34\rho -10.55}}\left[ {{\text{e}}^{\left( -8.6\rho +14.68 \right)\varepsilon }}-1 \right]$

(5)

3.2 包含温度效应的泡沫铝本构模型

3.2.1 温度对应力-应变曲线的影响

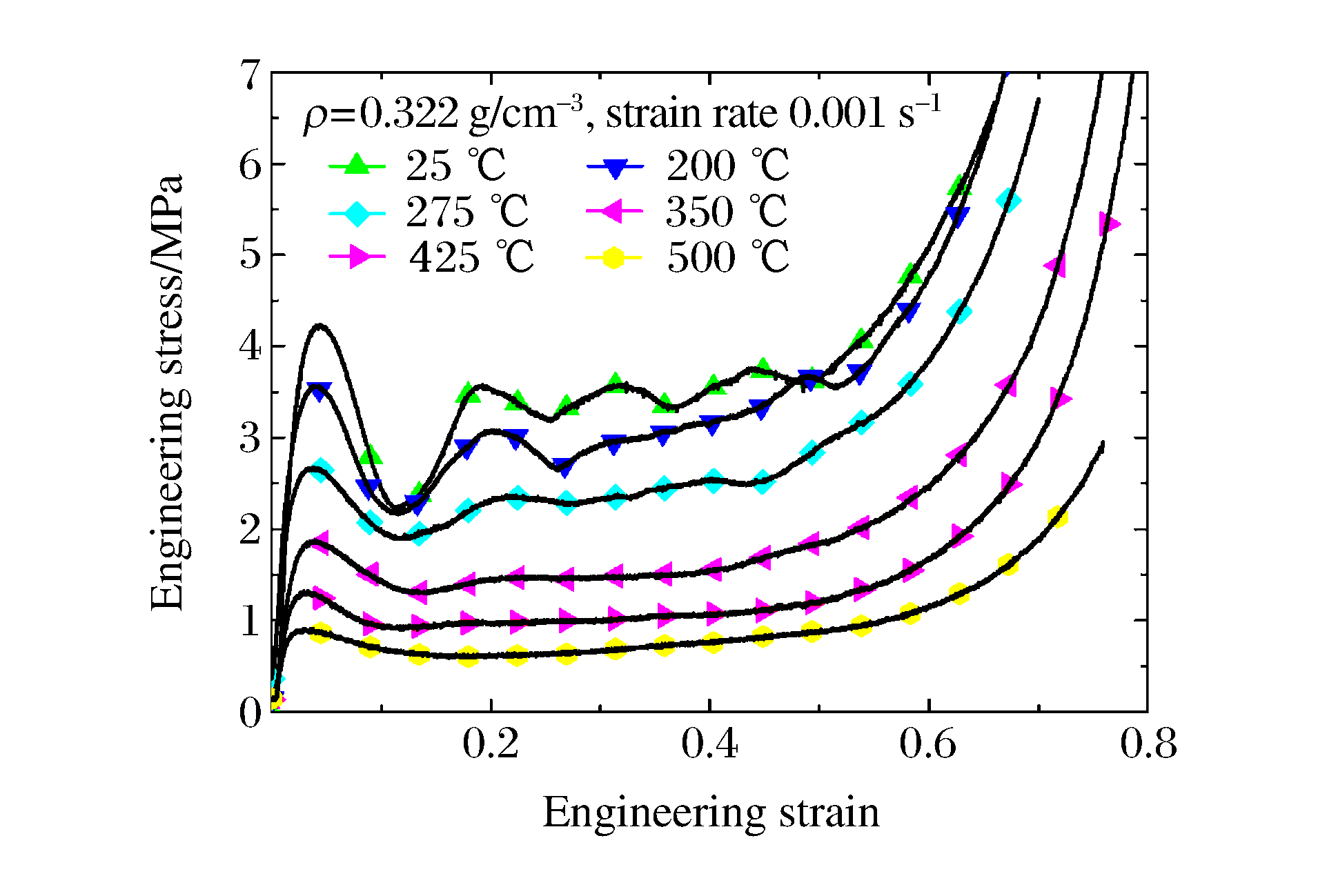
图 4是密度为0.322 g/cm³的泡沫铝在不同温度下的准静态应力-应变曲线。由图 4可知，随着温度的升高，材料由硬变软，由脆变韧。材料呈现明显的温度软化效应，当温度从25 ℃上升到500 ℃时，泡沫铝屈服强度从4.70 MPa下降到0.88 MPa。

图 4 不同温度下泡沫铝的准静态应力-应变曲线

Figure 4. Quasi-static stress-strain curves of aluminum foam at different temperatures

下载: 全尺寸图片幻灯片

3.2.2 考虑温度效应的修正模型

为了得到包含温度效应的泡沫铝本构模型，引入温度软化项

$H\left( T \right)=1-{{T}^{*m}}$

(6)

式中：T^*为无量纲温度项，m为指数。

${{T}^{*}}=\frac{T-{{T}_{\text{room}}}}{{{T}_{\text{melt}}}-{{T}_{\text{room}}}}$

(7)

式中：T_room为室温，T_room=298 K；T_melt为铝合金的熔点，T_melt=933 K。

因此，(3)式可进一步写成

$\sigma =\left\{ {{p}_{1}}\left( \rho \right)\frac{{{\text{e}}^{{{p}_{2}}\left( \rho \right)\varepsilon }}-1}{1+{{\text{e}}^{{{p}_{3}}\left( \rho \right)\varepsilon }}}\text{+}{{\text{e}}^{{{p}_{4}}\left( \rho \right)}}\left[ {{\text{e}}^{{{p}_{5}}\left( \rho \right)\varepsilon }}-1 \right] \right\}\cdot \left[ 1-{{\left( \frac{T-{{T}_{\text{room}}}}{{{T}_{\text{melt}}}-{{T}_{\text{room}}}} \right)}^{m}} \right]$

(8)

(8) 式即为考虑温度效应和密度效应的泡沫铝准静态本构模型。基于该模型，对不同温度下得到的准静态压缩应力-应变曲线进行拟合，得到参数m=1.31。不同温度下泡沫铝的应力-应变实验及拟合曲线如图 5所示。从图 5中可以看到，应力平台阶段和压实段拟合曲线与实验曲线吻合较好，说明该模型可用于描述不同温度下泡沫铝的应力-应变曲线。但在弹性段，拟合曲线与实验结果吻合得不太好，主要原因是Liu-Subhash模型不能很好地描述应力-应变曲线中的应力降现象，另一方面是由于实验时温度控制不精确产生的误差。总体上拟合曲线可以反映实验曲线的特征，拟合得到的参数值是可信的。

图 5 不同温度下泡沫铝应力-应变曲线的拟合情况

Figure 5. Fitting of stress-strain curves of aluminum foam at different temperatures

下载: 全尺寸图片幻灯片

4. 结论

利用MTS万能材料试验机研究了不同密度(0.322~0.726 g/cm³)的闭孔泡沫铝在不同温度(25~500 ℃)下的静态压缩力学性能，实验结果表明：泡沫铝材料的初始密度对其应力-应变曲线影响巨大，随着密度的增大，屈服强度也相应增大，材料承载能力增强；随着温度的升高，泡沫铝材料力学特性由硬变软，呈现明显的温度软化效应。利用Liu-Subhash模型对不同密度下的实验数据进行拟合，拟合效果很好，分析并确定了模型中5个参数随密度变化的函数，并代入Liu-Subhash模型，得到了考虑密度效应的本构模型；又引入温度软化项对本构模型进行修正，建立了综合考虑温度效应和密度效应的泡沫铝准静态压缩本构模型。

图 1 2种TPMS单胞构型

Figure 1. Single-cell configurations of two TPMSs

下载: 全尺寸图片幻灯片

图 2 水平集常数与相对密度的关系

Figure 2. Level set constants versus relative densities

下载: 全尺寸图片幻灯片

图 3 试件的几何模型

Figure 3. Geometric modeling of the specimens

下载: 全尺寸图片幻灯片

图 4 SLM打印试样

Figure 4. SLM printed specimens

下载: 全尺寸图片幻灯片

图 5 SLM打印标准件在单轴拉伸下的应力-应变曲线

Figure 5. Stress-strain curves of SLM-printed standard parts in uniaxial tension

下载: 全尺寸图片幻灯片

图 6 直撞式霍普金森杆实验布局

Figure 6. Direct impact Hopkinson bar experiment setup

下载: 全尺寸图片幻灯片

图 7 实验与数值模拟验证

Figure 7. Experimental and numerical simulation validation

下载: 全尺寸图片幻灯片

图 8 50 m/s冲击加载下单一结构和混合结构的实验与模拟变形模态

Figure 8. Deformation modes of experiment and simulation for single and hybrid structures under 50 m/s impact loading

下载: 全尺寸图片幻灯片

图 9 50 m/s冲击加载下GIP-1结构的1/2有限元模型剖面

Figure 9. 1/2 finite element model section of GIP-1 structure at 50 m/s impact loading

下载: 全尺寸图片幻灯片

图 10 不同加载速度下结构的应力-应变曲线

Figure 10. Stress-strain curves of structures with different loading velocities

下载: 全尺寸图片幻灯片

图 11 单一结构和混合结构的能量吸收效率曲线和密实化应变

Figure 11. Energy absorption efficiency curves and densification strains for single structures and hybrid structures

下载: 全尺寸图片幻灯片

图 12 加载速度对初始峰值应力和比吸能的影响

Figure 12. Effect of loading velocity on initial peak stress and specific energy absorption

下载: 全尺寸图片幻灯片

图 13 加载速度对归一化参数的影响

Figure 13. Effect of loading velocity on normalized parameters

下载: 全尺寸图片幻灯片

表 1 测试试样的质量

Table 1. Masses of test specimens

Specimen	Designed mass/g	Specimen mass/g	Mass deviation/%	Designed relative density/%	Relative density of specimen/%	Relative density deviation/%
Gyroid-1	38.96	40.52	4.00	36	37.43	3.97
Gyroid-2	38.96	40.35	3.56	36	37.27	3.52
IWP-1	38.96	40.21	3.20	36	37.14	3.16
IWP-2	38.96	40.12	2.97	36	37.06	2.94
GIP-1	38.96	40.65	4.33	36	37.55	4.30
GIP-2	38.96	40.37	3.61	36	37.29	3.58

下载: 导出CSV

参考文献(25)

[1]	ZHANG J W, ZHAO J X, RONG Q G, et al. Machine learning guided prediction of mechanical properties of TPMS structures based on finite element simulation for biomedical titanium [J]. Materials Technology, 2022, 37(1): 1–8.
[2]	CHATZIGEORGIOU C, PIOTROWSKI B, CHEMISKY Y, et al. Numerical investigation of the effective mechanical properties and local stress distributions of TPMS-based and strut-based lattices for biomedical applications [J]. Journal of the Mechanical Behavior of Biomedical Materials, 2022, 126: 105025. doi: 10.1016/j.jmbbm.2021.105025
[3]	ZHANG S N, DA D, WANG Y J. TPMS-infill MMC-based topology optimization considering overlapped component property [J]. International Journal of Mechanical Sciences, 2022, 235: 107713. doi: 10.1016/j.ijmecsci.2022.107713
[4]	SANTIAGO R, RAMOS H, ALMAHRI S, et al. Modelling and optimisation of TPMS-based lattices subjected to high strain-rate impact loadings [J]. International Journal of Impact Engineering, 2023, 177: 104592. doi: 10.1016/j.ijimpeng.2023.104592
[5]	冯根柱, 于博丽, 李世强, 等. 多层级夹芯结构的变形与能量吸收 [J]. 高压物理学报, 2019, 33(5): 055902. FENG G Z, YU B L, LI S Q, et al. Deformation and energy absorption of multi-hierarchical sandwich structures [J]. Chinese Journal of High Pressure Physics, 2019, 33(5): 055902.
[6]	FENG J W, FU J Z, SHANG C, et al. Porous scaffold design by solid T-splines and triply periodic minimal surfaces [J]. Computer Methods in Applied Mechanics and Engineering, 2018, 336: 333–352. doi: 10.1016/j.cma.2018.03.007
[7]	LIU B, LIU M Y, CHENG H Q, et al. A new stress-driven composite porous structure design method based on triply periodic minimal surfaces [J]. Thin-Walled Structures, 2022, 181: 109974. doi: 10.1016/j.tws.2022.109974
[8]	FENG J W, LIU B, LIN Z W, et al. Isotropic porous structure design methods based on triply periodic minimal surfaces [J]. Materials & Design, 2021, 210: 110050.
[9]	WANG H, TAN D W, LIU Z P, et al. On crashworthiness of novel porous structure based on composite TPMS structures [J]. Engineering Structures, 2022, 252: 113640. doi: 10.1016/j.engstruct.2021.113640
[10]	ZHANG L, FEIH S, DAYNES S, et al. Energy absorption characteristics of metallic triply periodic minimal surface sheet structures under compressive loading [J]. Additive Manufacturing, 2018, 23: 505–515. doi: 10.1016/j.addma.2018.08.007
[11]	AL-KETAN O, ROWSHAN R, ABU AL-RUB R K. Topology-mechanical property relationship of 3D printed strut, skeletal, and sheet based periodic metallic cellular materials [J]. Additive Manufacturing, 2018, 19: 167–183. doi: 10.1016/j.addma.2017.12.006
[12]	LI X, XIAO L J, SONG W D. Compressive behavior of selective laser melting printed gyroid structures under dynamic loading [J]. Additive Manufacturing, 2021, 46: 102054. doi: 10.1016/j.addma.2021.102054
[13]	NAZIR A, HUSSAIN S, ALI H M, et al. Design and mechanical performance of nature-inspired novel hybrid triply periodic minimal surface lattice structures fabricated using material extrusion [J]. Materials Today Communications, 2024, 38: 108349. doi: 10.1016/j.mtcomm.2024.108349
[14]	LI S, ZHU H, FENG G, et al. Influence mechanism of cell-arrangement strategy on energy absorption of dual-phase hybrid lattice structure [J]. International Journal of Impact Engineering, 2023, 175: 104528. doi: 10.1016/j.ijimpeng.2023.104528
[15]	YU G J, XIAO L J, SONG W D. Deep learning-based heterogeneous strategy for customizing responses of lattice structures [J]. International Journal of Mechanical Sciences, 2022, 229: 107531. doi: 10.1016/j.ijmecsci.2022.107531
[16]	ZHANG J, XIE S, LI T, et al. A study of multi-stage energy absorption characteristics of hybrid sheet TPMS lattices [J]. Thin-Walled Structures, 2023, 190: 110989. doi: 10.1016/j.tws.2023.110989
[17]	SREEDHAR N, THOMAS N, AL-KETAN O, et al. Mass transfer analysis of ultrafiltration using spacers based on triply periodic minimal surfaces: effects of spacer design, directionality and voidage [J]. Journal of Membrane Science, 2018, 561: 89–98. doi: 10.1016/j.memsci.2018.05.028
[18]	AL-KETAN O, ABU AL-RUB R K. MSLattice: a free software for generating uniform and graded lattices based on triply periodic minimal surfaces [J]. Material Design & Processing Communications, 2021, 3(6): e205.
[19]	MASKERY I, STURM L, AREMU A O, et al. Insights into the mechanical properties of several triply periodic minimal surface lattice structures made by polymer additive manufacturing [J]. Polymer, 2018, 152: 62–71. doi: 10.1016/j.polymer.2017.11.049
[20]	YIN H F, ZHENG X J, WEN G L, et al. Design optimization of a novel bio-inspired 3D porous structure for crashworthiness [J]. Composite Structures, 2021, 255: 112897. doi: 10.1016/j.compstruct.2020.112897
[21]	NOVAK N, TANAKA S, HOKAMOTO K, et al. High strain rate mechanical behaviour of uniform and hybrid metallic TPMS cellular structures [J]. Thin-Walled Structures, 2023, 191: 111109. doi: 10.1016/j.tws.2023.111109
[22]	厉雪, 肖李军, 宋卫东. 3D打印梯度Gyroid结构的动态冲击响应 [J]. 高压物理学报, 2021, 35(3): 034201. LI X, XIAO L J, SONG W D. Dynamic behavior of 3D printed graded gyroid structures under impact loading [J]. Chinese Journal of High Pressure Physics, 2021, 35(3): 034201.
[23]	李吉祥. 基于三周期极小曲面的三维点阵结构的防护性能研究 [D]. 泉州: 华侨大学, 2023. LI J X. Study on protective performance of three-dimensional lattice structures based on triply periodic minimal surfaces [D]. Quanzhou: Huaqiao University, 2023.
[24]	DUAN Y, DU B, SHI X P, et al. Quasi-static and dynamic compressive properties and deformation mechanisms of 3D printed polymeric cellular structures with Kelvin cells [J]. International Journal of Impact Engineering, 2019, 132: 103303. doi: 10.1016/j.ijimpeng.2019.05.017
[25]	XI H, ZHOU Z, ZHANG H, et al. Multi-morphology TPMS structures with multi-stage yield stress platform and multi-level energy absorption: design, manufacturing, and mechanical properties [J]. Engineering Structures, 2023, 294: 116733. doi: 10.1016/j.engstruct.2023.116733

施引文献

资源附件(0)

访问统计

点击查看大图

图(13) / 表(1)

计量

文章访问数: 363
HTML全文浏览量: 98
PDF下载量: 29

1. 实验方法及方案
2. Liu-Subhash本构模型
3. 实验结果与分析
3.1 包含密度效应的泡沫铝本构模型
3.2 包含温度效应的泡沫铝本构模型
4. 结论

三周期极小曲面结构混合设计及其在冲击载荷下的力学行为

doi: 10.11858/gywlxb.20240783

作者简介: 刘嘉婧（1997－），女，硕士研究生，主要从事轻质材料冲击动力学行为研究.E-mail：liujiajing199709@163.com

通讯作者: 李世强（1986－），男，博士，副教授，主要从事结构冲击动力学行为研究. E-mail：lishiqiang@tyut.edu.cn

计量

出版历程