内爆炸载荷下双向波纹夹芯管的动力响应及抗爆性能预测

唐波; 李子豪; 刘志芳; 李世强

doi:10.11858/gywlxb.20240782

内爆炸载荷下双向波纹夹芯管的动力响应及抗爆性能预测

doi: 10.11858/gywlxb.20240782

唐波^1,,
李子豪¹,
刘志芳^{1, 2},
李世强^{1, 2, 3,*, ,}

1.
太原理工大学机械与运载工程学院应用力学研究所, 山西太原　030024)
2.
材料强度与结构冲击山西省重点实验室, 山西太原　030024
3.
北京理工大学爆炸科学与安全防护全国重点实验室，北京 100081

基金项目: 国家自然科学基金（12072219，12272254）；山西省自然科学研究面上项目（202203021211170）；海安太原理工大学先进制造与智能装备产业研究院开放研发项目（2023HA-TYUTKFYF016）；山西省科技创新人才团队（领军）专项（202204051002006）；爆炸科学与安全防护全国重点实验室（北京理工大学）开放课题（KFJJ24-03M）

详细信息

作者简介:
唐　波（1999－），男，硕士研究生，主要从事轻质结构冲击动力学行为研究. E-mail：tb15881862576@163.com

通讯作者:
李世强（1986－），男，博士，副教授，主要从事结构冲击动力学研究. E-mail：lishiqiang@tyut.edu.cn

中图分类号: O347.1; O521.9
计量
- 文章访问数: 127
- HTML全文浏览量: 50
- PDF下载量: 24
出版历程
- 收稿日期: 2024-04-03
- 修回日期: 2024-05-08
- 录用日期: 2024-06-04
- 网络出版日期: 2024-11-25
- 刊出日期: 2024-12-05

Blast Resistance and Prediction of Bi-Directional Corrugated Sandwich Tubes under Internal Blast Loading

TANG Bo^1
,,
LI Zihao¹,
LIU Zhifang^{1, 2},
LI Shiqiang^{1, 2, 3,*
, ,}

1.
Institute of Applied Mechanics, College of Mechanical and Vehicle Engineering, Taiyuan University of Technology, Taiyuan 030024, Shanxi, China
2.
Shanxi Key Laboratory of Material Strength and Structural Impact, Taiyuan University of Technology, Taiyuan 030024, Shanxi, China
3.
State Key Laboratory of Explosion Science and Safety Protection, Beijing Institute of Technology, Beijing 100081, China

摘要

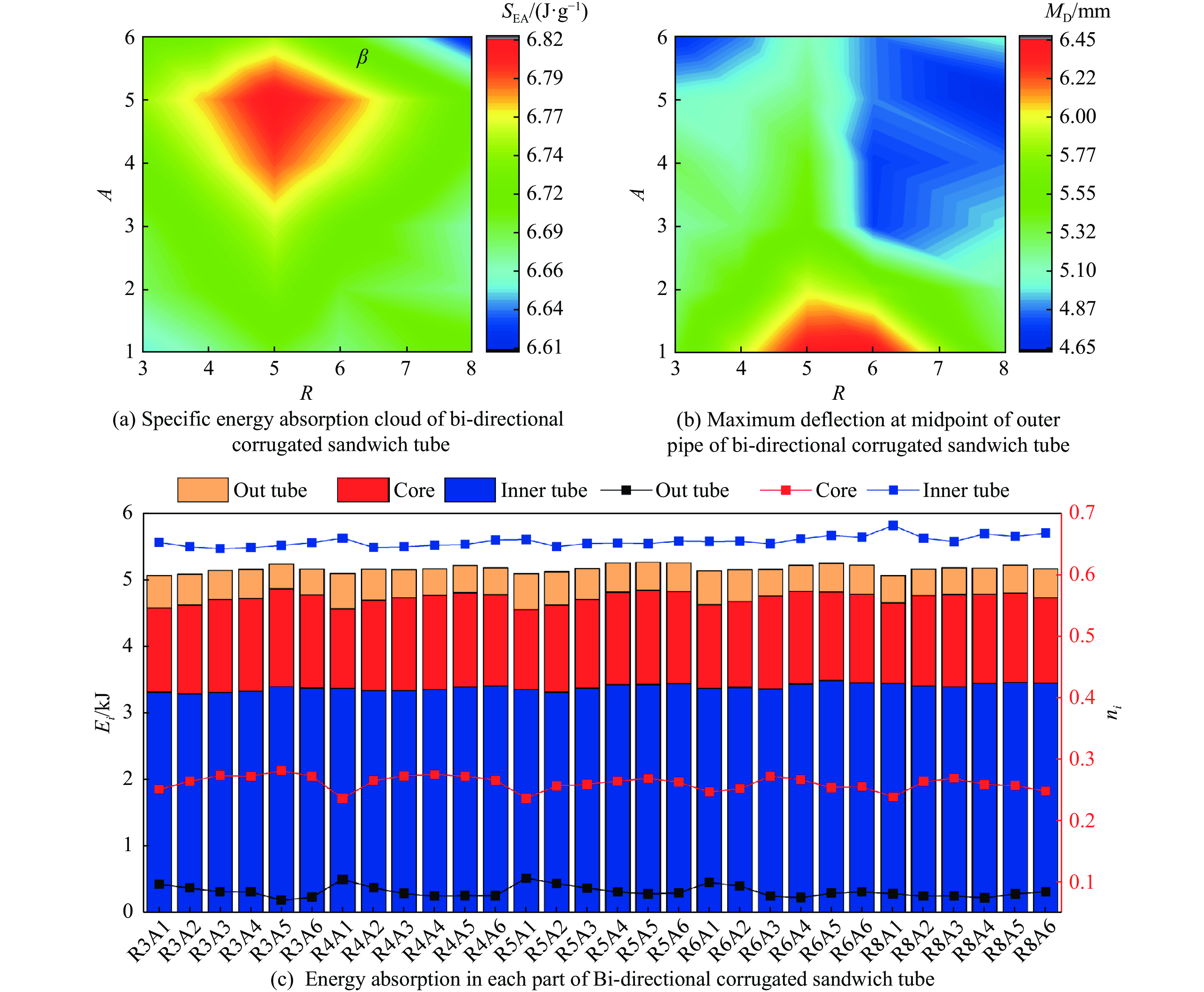
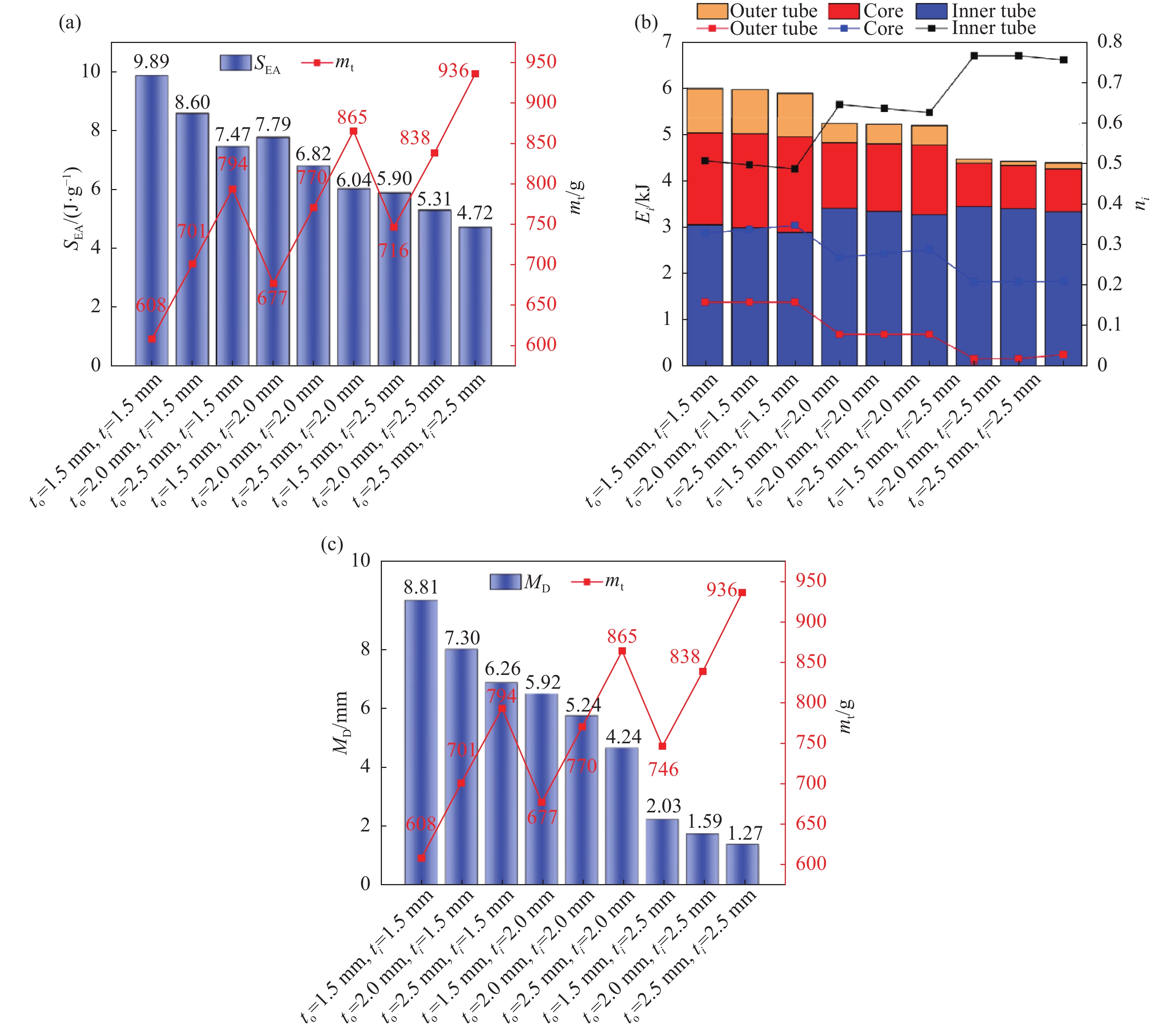
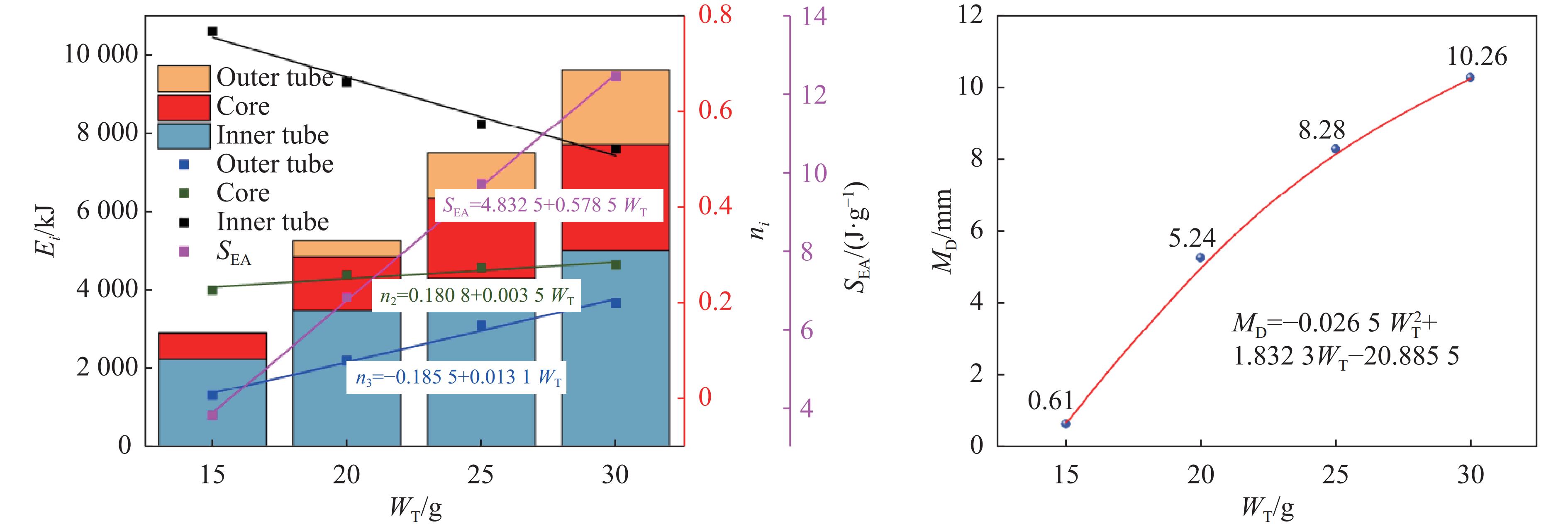
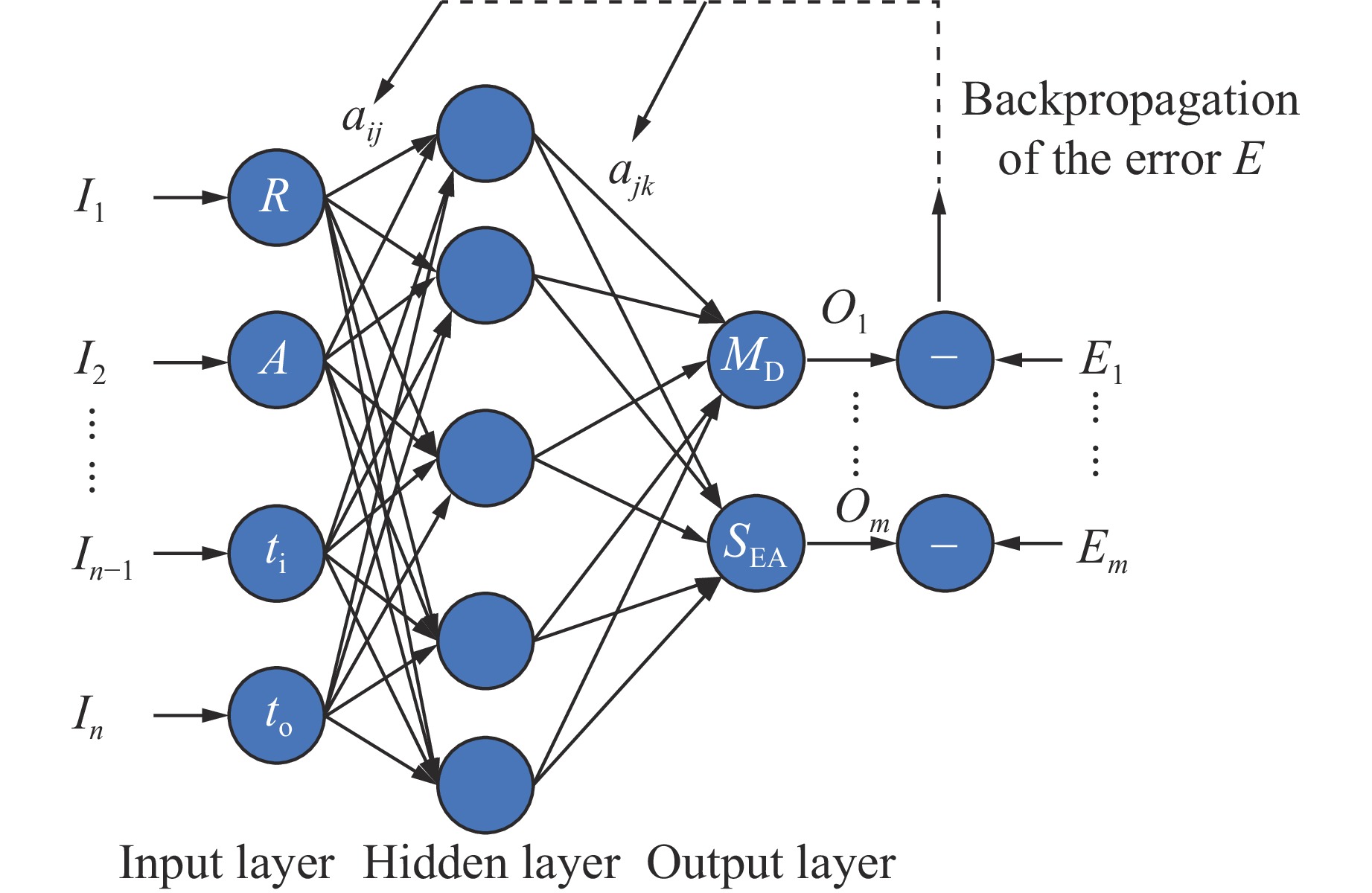
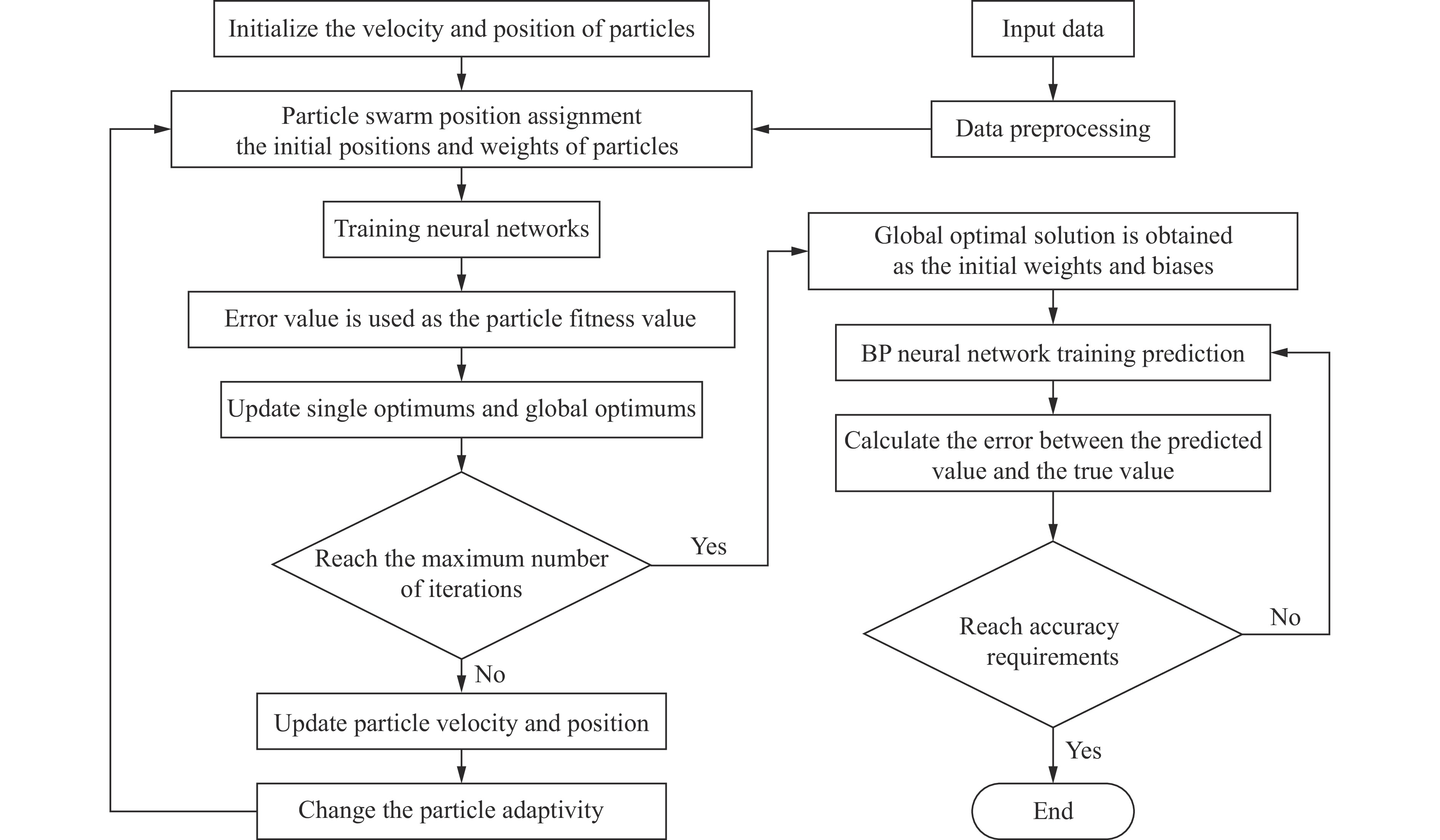
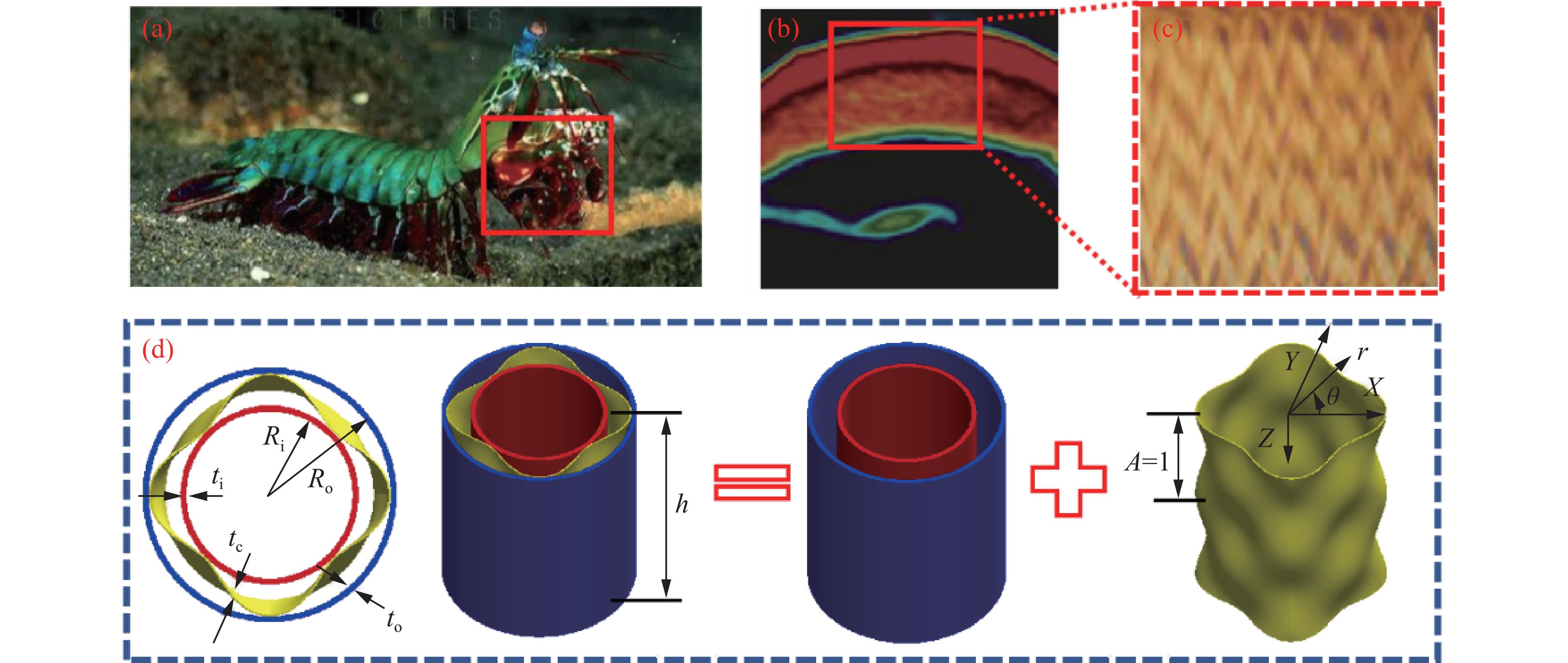
摘要: 受孔雀螳螂虾前鄂抗冲击区结构启发，设计并制备了双向波纹夹芯管结构，采用实验和数值模拟相结合的方法研究了其在内爆炸载荷下的动态响应及能量吸收机制。实验获得了结构的外管中点最大挠度和3种典型变形模式：局部塑性变形、塑性大变形以及撕裂破坏。内外管中点最大挠度和结构最终变形模态的数值模拟结果与实验结果吻合较好。通过数值模拟研究了芯层波纹数、内外管壁厚以及炸药质量对外管中点最大挠度和能量吸收特性的影响，结果表明：随着波纹数增大，结构比吸能先增大后减小；增大内管壁厚和减小外管壁厚能有效地提高结构的抗爆性能，当结构内管壁厚为2.5 mm、外管壁厚为1.5 mm时，相比于内管壁厚为1.5 mm、外管壁厚为2.5 mm时，外管中点最大挠度降低了67.6%，质量降低了6.0%；随着TNT当量的增加，内管吸收的能量占比逐渐下降，而芯层和外管吸收的能量占比增加。建立了BP（back propagation）神经网络模型、PSO-BP（particle swarm optimization-back propagation）神经网络模型以及响应面分析模型，分别对结构的比吸能与外管中点最大挠度进行预测，优化了所提出的结构。
- 夹芯管 /
- 双向波纹芯层 /
- 内爆炸载荷 /
- 抗爆性能
Abstract: A bi-directional corrugated sandwich tube structure was proposed, inspired by the front jaw of peacock mantis shrimp. The dynamic responses and energy absorption characteristics of bi-directional corrugated sandwich tubes under inner blast loading were investigated numerically and experimentally. It was found that three typical deformation modes including localized plastic deformation, elliptical plastic large deformation and laceration. The numerical results of the mid-point deflection of the outer tube and the final deformation mode of the structure agree well with the experimental results. Subsequently, the effects of the number of corrugation of the bi-directional corrugated core tube, the inner and outer tube wall thicknesses and TNT dose on its dynamic response and energy absorption characteristics were investigated thoroughly. The results show that the energy absorption ratio of the structure increases first, and then decreases with the increase of the number of corrugation. Increasing the inner tube wall thickness and decreasing the outer tube wall thickness can improve the shock resistance performance. Compared with the inner tube wall thickness of 1.5 mm and an outer tube wall thickness of 2.5 mm, the structure with an inner tube wall thickness of 2.5 mm and an outer tube wall thickness of 1.5 mm can reduce the maximum mid-point deflection (MD) of the outer tube by 67.6% and reduce the mass by 6.0%. As the TNT dose increases, the percentage of energy absorbed by the inner tube decreases gradually, while the percentage of energy absorbed by the core and outer tube increases. Finally, the specific energy absorption (SEA) of the structure and MD of the outer tube were predicted using BP (back propagation) neural network model, PSO-BP (particle swarm optimization-back propagation) neural network model, and RSM (response surface methodology) model to optimize the proposed structure.
- sandwich tube /
- bi-directional corrugate core /
- internal blast loading /
- blast resistance performance

HTML全文

光滑粒子流体动力学(Smoothed Particle Hydrodynamics, SPH)方法中的搜索算法较耗时，即每个时间步都要对领域粒子进行搜索，粒子越多，耗时情况越突出，与有限元法相比，SPH方法的计算效率要低得多。为了解决SPH方法计算效率低的问题，Johnson等^[1-2]和Attaway等^[3]将有限元与SPH方法相结合，提出了SPH-FEM耦合的算法，即:在小变形区域使用有限元法，大变形区域仍使用SPH方法。该方法不仅提高了计算效率，而且适应性较强。

目前，采用SPH方法对爆炸焊接进行数值模拟的相关报道较少，而且多采用二维SPH方法。Tanaka^[4]采用SPH方法对爆炸焊接的斜碰撞过程进行了数值模拟，成功地模拟出射流、波形和涡旋，波长的模拟结果相对实验结果偏大。李晓杰等^[5]采用SPH方法及热塑性流体力学模型对爆炸复合板的斜碰撞过程中出现的界面波进行了数值模拟，模拟结果与张登霞等^[6-7]实验结果的一致性较好。刘江等^[8]利用AUTODYN软件中的SPH方法模拟了爆炸复合的斜碰撞，结合模拟中有效塑性变形、温度及剪切应力呈现的变化规律发现，爆炸复合的结合机理集塑性变形、熔化和扩散为一体。本研究将采用三维SPH方法对双面爆炸焊接过程进行模拟，将其结果与实验及理论结果进行对比，分析SPH-FEM耦合方法对爆炸焊接模拟的有效性。

1. 计算模型及参数选取

1.1 计算模型

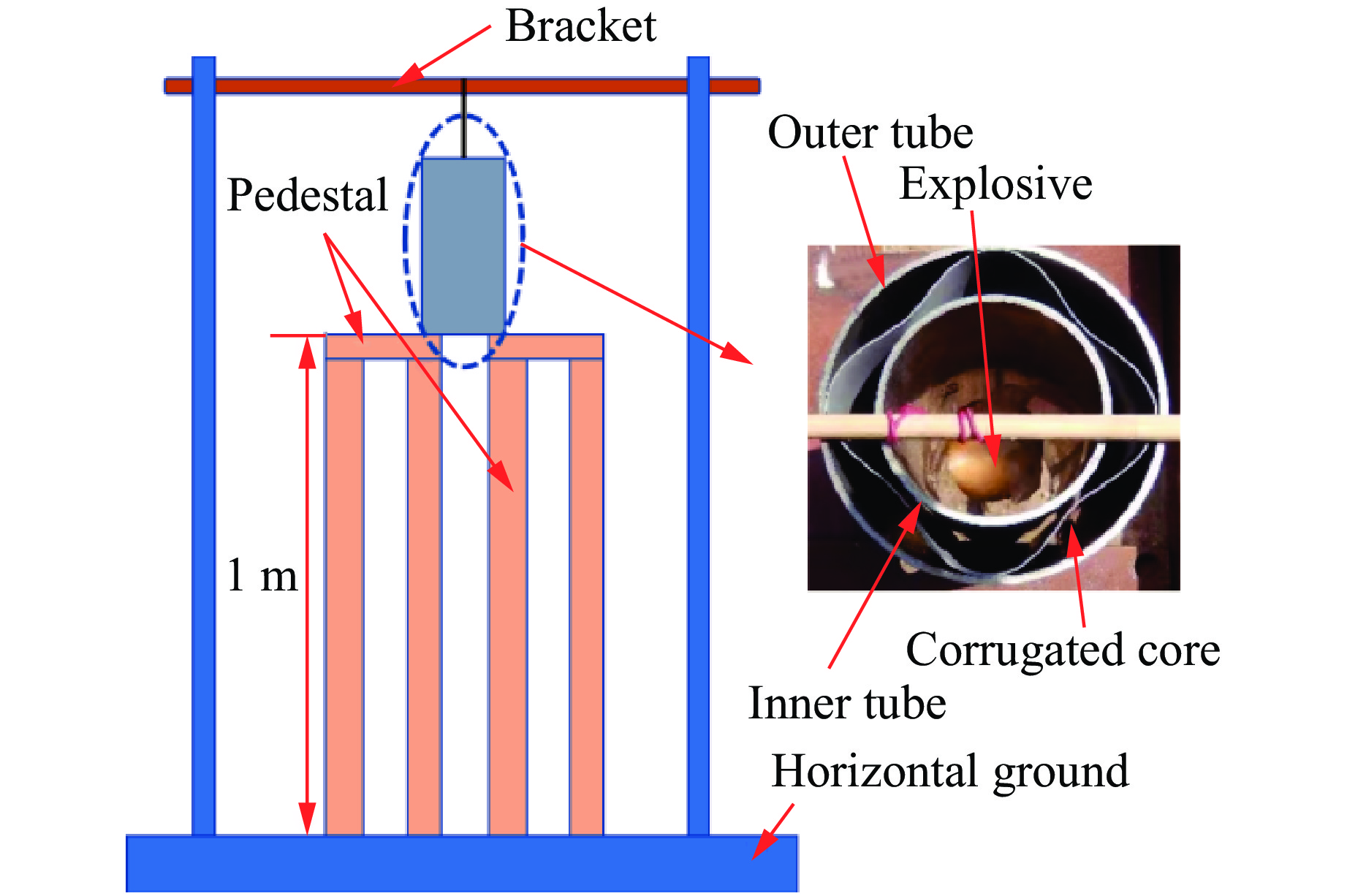
以前期45钢/Q235钢双面爆炸焊接实验^[9]为基础，考虑到计算效率，利用LS-DYNA建立如图 1及图 2所示的两组双面爆炸焊接SPH-FEM耦合的三维真实计算模型，选用的炸药为乳化炸药(玻璃微球的质量分数为5%)，计算模型中基板和复板的材料、尺寸、间隙(δ)及药厚如表 1所示。起爆方式为点起爆。

图 1 计算模型Ⅰ(10 mm药厚)

Figure 1. Calculation model Ⅰ with explosive thickness of 10 mm

下载: 全尺寸图片幻灯片

图 2 计算模型Ⅱ(5 mm药厚)

Figure 2. Calculation model Ⅱ with explosive thickness of 5 mm

下载: 全尺寸图片幻灯片

表 1 计算模型中材料的相关参数

Table 1. Related parameters of materials in calculation models

Calculationmodel	Flyer plate		Base plate		Gap δ/mm	Size of explosive/(mm×mm×mm)
Calculationmodel	Material	Size/(mm×mm×mm)	Material	Size/(mm×mm×mm)	Gap δ/mm	Size of explosive/(mm×mm×mm)
Ⅰ	45 steel	300×150×2	Q235	300×150×16	6	300×150×10
Ⅱ	45 steel	300×150×2	Q235	300×150×16	6	300×150×5

下载: 导出CSV

| 显示表格

基、复板采用3D Solid 164实体单元，单元边长为0.1 cm；炸药划分为光滑粒子，粒子的大小Δr取为0.1 cm。考虑到模型的对称性，为了提高计算效率，采用1/2模型进行计算。单位制为cm-g-μs。

1.2 材料模型及参数设定

数值计算中乳化炸药采用高能燃烧模型^[10-11]及JWL状态方程^[12]。JWL状态方程表达式为

$p={{A}_{\text{JWL}}}\left( 1-\frac{\omega }{{{R}_{1}}v} \right){{\text{e}}^{-{{R}_{1}}v}}+{{B}_{\text{JWL}}}\left( 1-\frac{\omega }{{{R}_{2}}v} \right){{\text{e}}^{-{{R}_{2}}v}}+\frac{\omega {{E}_{0}}}{v}$

(1)

式中：A_JWL、B_JWL、R₁、R₂和ω为材料参数；p为爆轰产物压力，GPa；E₀为初始比内能，kJ/cm³；v为爆轰气体产物的相对比容，为无量纲量。炸药的相关参数见表 2，其中：ρ为密度，D为炸药爆速。

表 2 乳化炸药的JWL状态参数^[13]

Table 2. JWL equation-of-state parameters of emulsion explosives^[13]

ρ/(g·cm^-3)	D/(m·s^-1)	A_JWL/GPa	B_JWL/GPa	R₁	R₂	ω	E₀/(kJ·cm^-3)
1.12	4 510	326.42	5.808 9	5.80	1.56	0.57	3.323

下载: 导出CSV

| 显示表格

数值计算中，基、复板均采用Mie-Grüneisen状态方程^[14]和Johnson-Cook材料模型^[15]。Johnson-Cook材料模型的形式如下

$\sigma =\left( A+B\varepsilon _{\text{p}}^{n} \right)\left( 1+C\ln \dot{\varepsilon }_{\text{p}}^{*} \right)\left( 1-{{T}^{*m}} \right)$

(2)

式中：ε_p为有效塑性应变； $\dot{\varepsilon }_{\text{p}}^{*}={{\dot{\varepsilon }}_{\text{p}}}/\dot{\varepsilon }_{\text{p}}^{0}$ 为有效塑性应变率，其中 $\dot{\varepsilon }_{\text{p}}^{0}$ 为参考应变率；A、B、C、m及n为与材料相关的常数；无量纲温度T^*表示为T^*=(T-T_r)/(T_m-T_r)，其中T_r为室温, T_m为熔点。45钢选用与Q235钢相同的Johnson-Cook材料模型参数，具体参数如表 3所示。

表 3 Q235钢的Johnson-Cook模型参数^[16]

Table 3. Johnson-Cook parameters of Q235 steel^[16]

ρ/(g·cm^-3)	G/GPa	A/GPa	B/GPa	C	n	m	T_m/K	T_r/K
7.83	77	0.792	0.51	0.014	0.26	1.03	1 793	294

下载: 导出CSV

| 显示表格

2. 模拟结果与分析

2.1 10 mm药厚的模拟结果

2.1.1 碰撞点位移

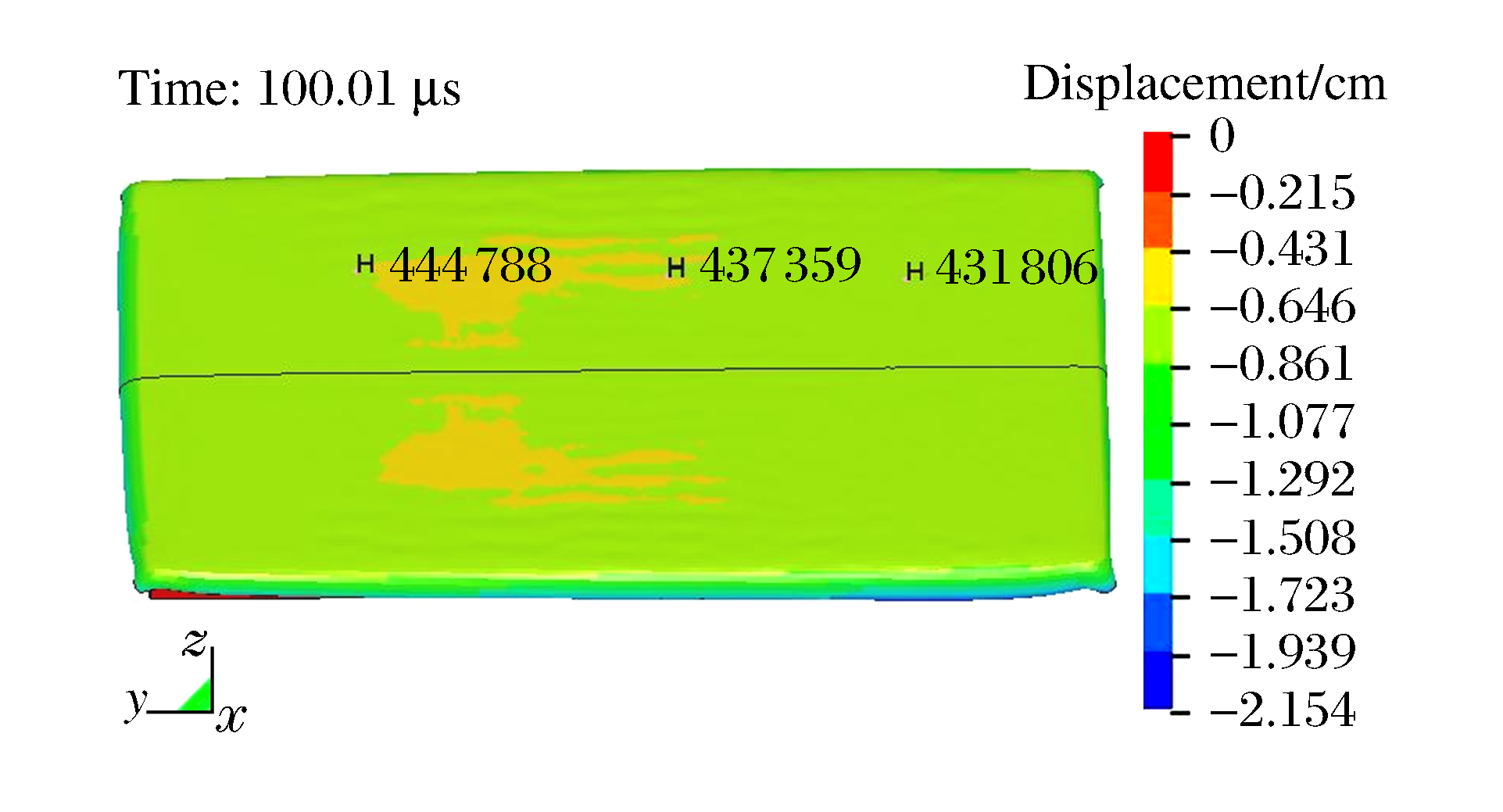
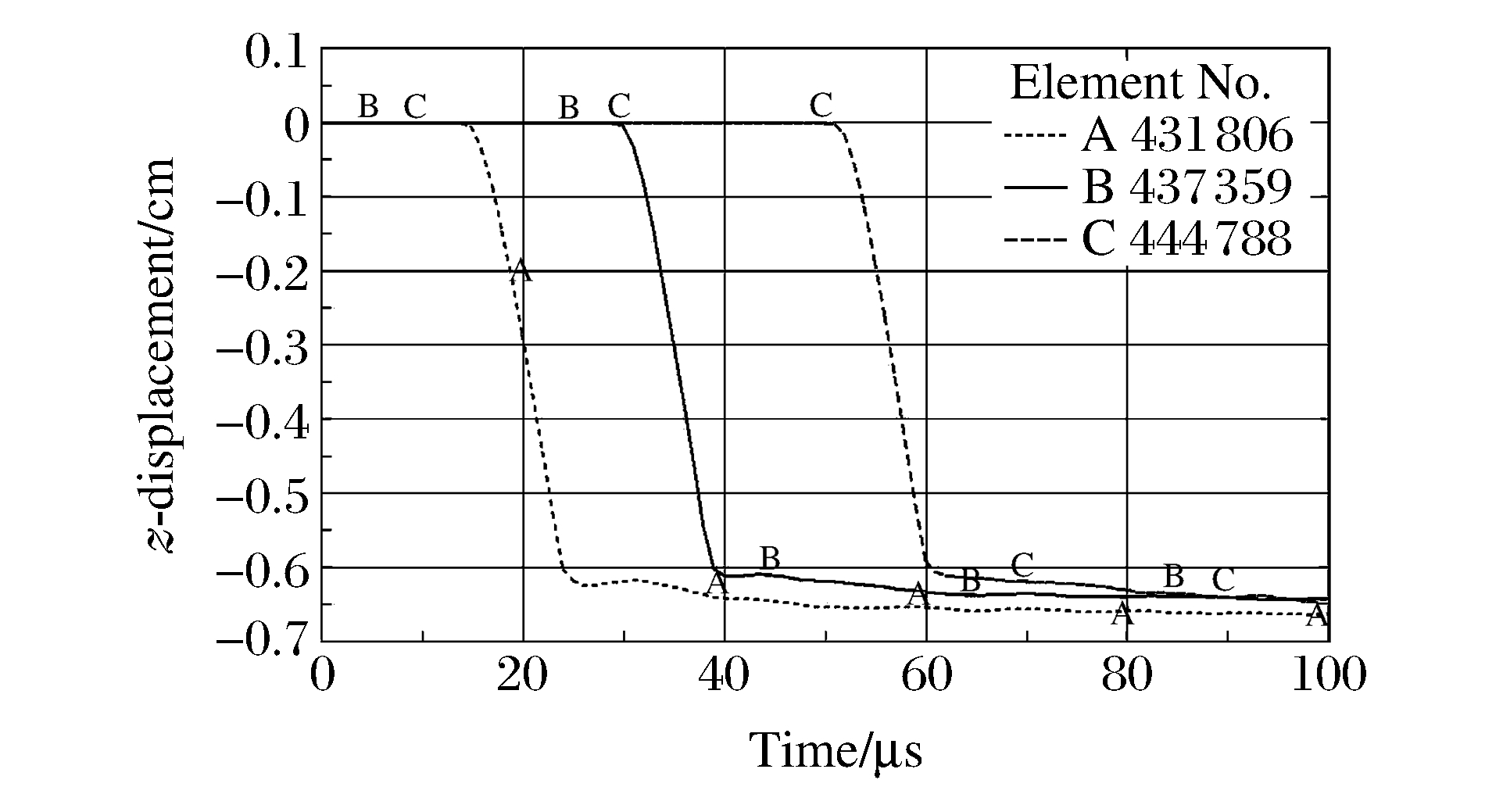
图 3所示是爆炸焊接结束时复板的竖向位移云图。由图 3可看出，复板的位移大致均为6 mm，表明基、复板已完全复合。为了更直观地观察复板单元位移的变化情况，在复板上选择3个特征单元(431 806、437 359、444 788)，输出其位移-时间曲线，如图 4所示。由图 4可看出，特征单元的竖向位移均略大于间隙(6 mm)，这是由于在爆炸载荷作用下复板有一定程度的减薄率所致。

图 3 10 mm药厚下爆炸焊接结束时复板的z向位移云图

Figure 3. z-direction displacement contour of flyer plate with explosive thickness of 10 mm at the end of explosive welding

下载: 全尺寸图片幻灯片

图 4 10 mm药厚下复板上3个特征单元的z向位移-时间历程

Figure 4. z-direction displacement histories of 3 characteristic elements with explosive thickness of 10 mm

下载: 全尺寸图片幻灯片

2.1.2 复板碰撞速度

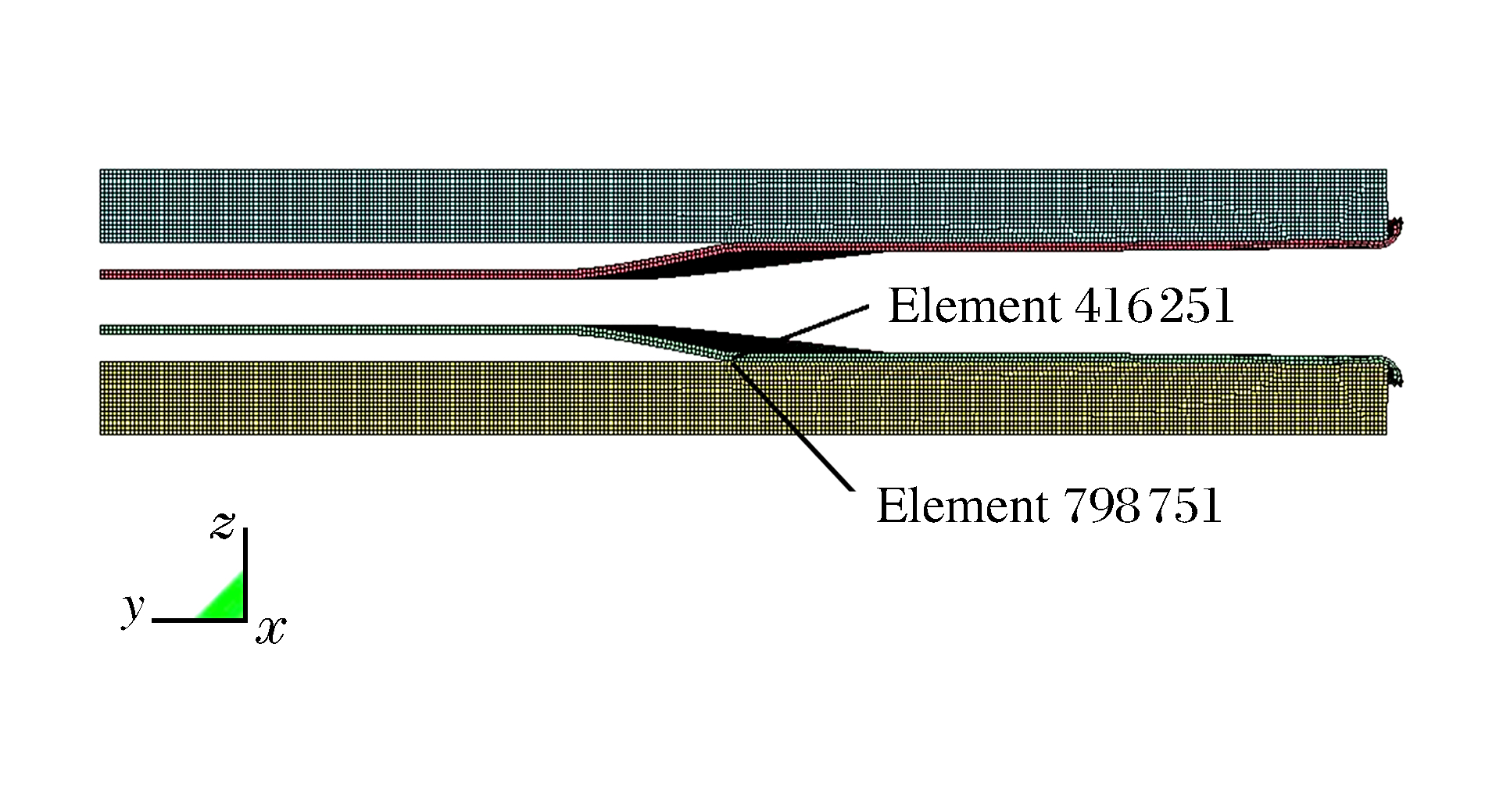
图 5所示是一对分别取自基板与复板结合界面处的特征单元(基板单元：798 751；复板单元：416 251)，特征单元的选取与前期实验^[9]中金相试样的取样位置一致。

图 5 10 mm药厚下的一对特征单元

Figure 5. A pair of characteristic elements with explosive thickness of 10 mm

下载: 全尺寸图片幻灯片

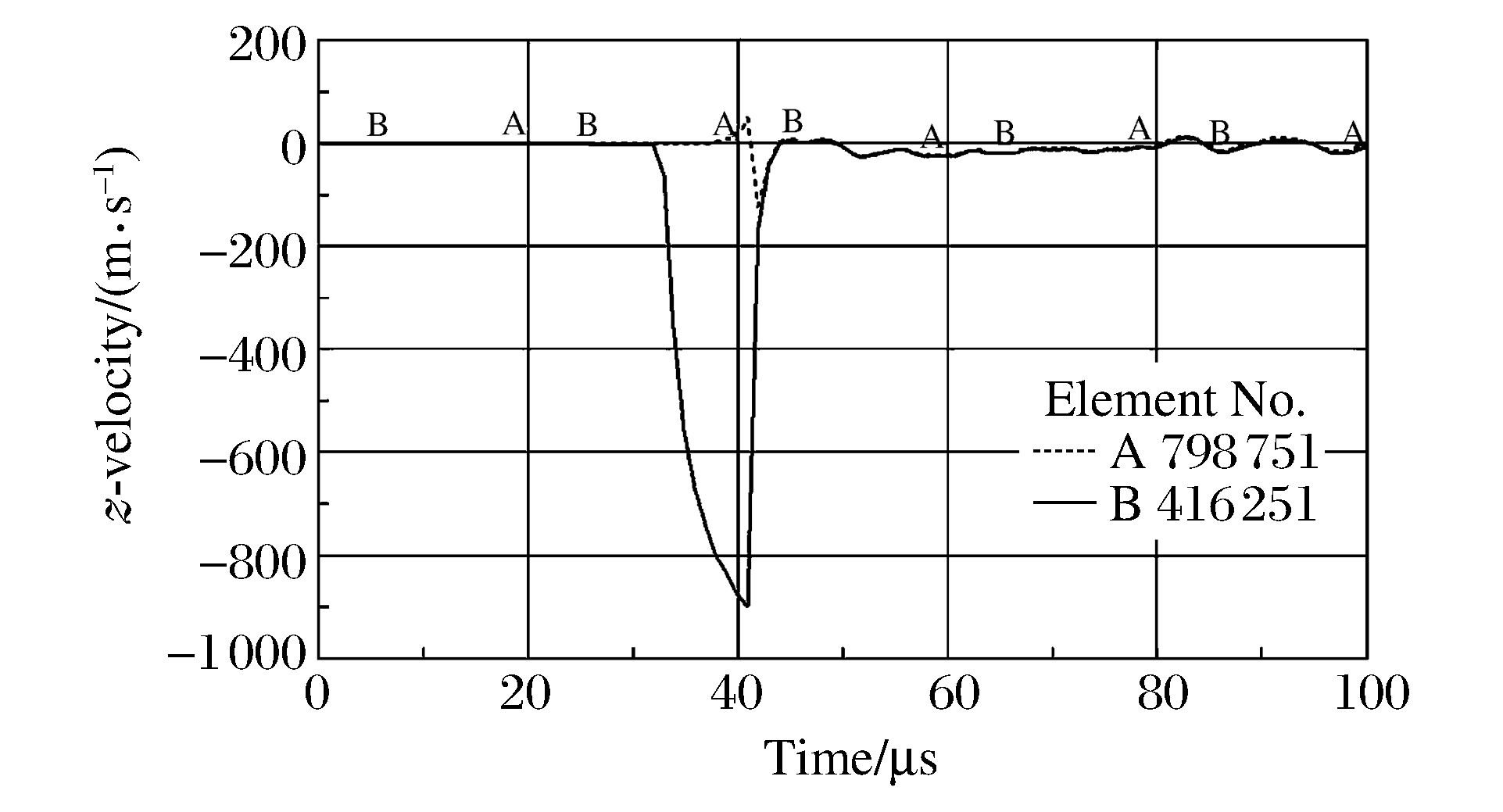
图 6所示是这对特征单元的速度-时间曲线。可以看出，基板在碰撞前有一个正的速度峰；该现象的产生如文献[17]所述，是由于爆轰产物不断堆积以及前碰撞点在待复合区产生的振动能所致。复板上所取单元的最大碰撞速度为897 m/s。

图 6 一对特征单元(见图 5)的速度-时间曲线

Figure 6. Velocity-time curves of the pair of characteristic elements (see Fig. 5)

下载: 全尺寸图片幻灯片

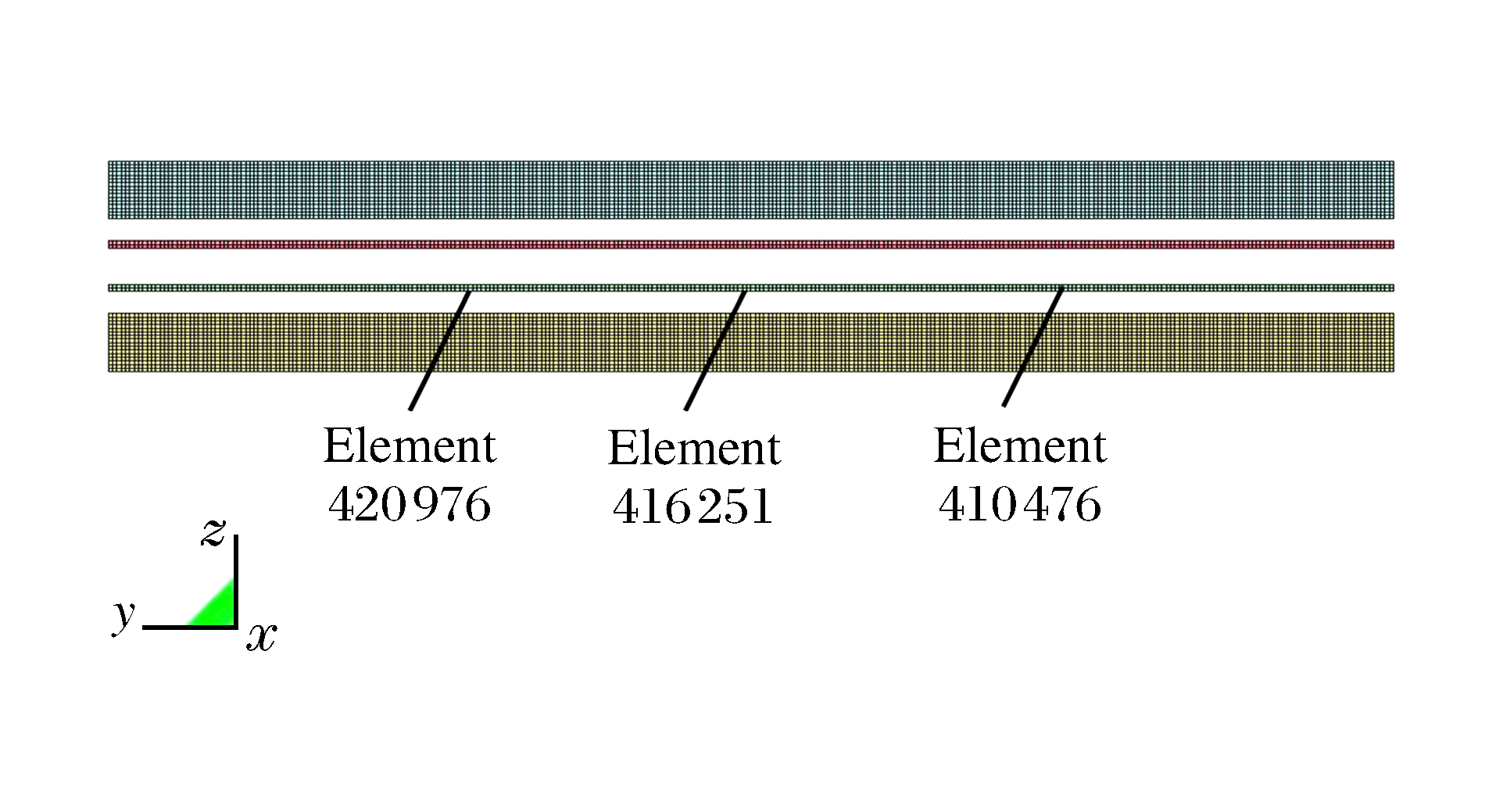
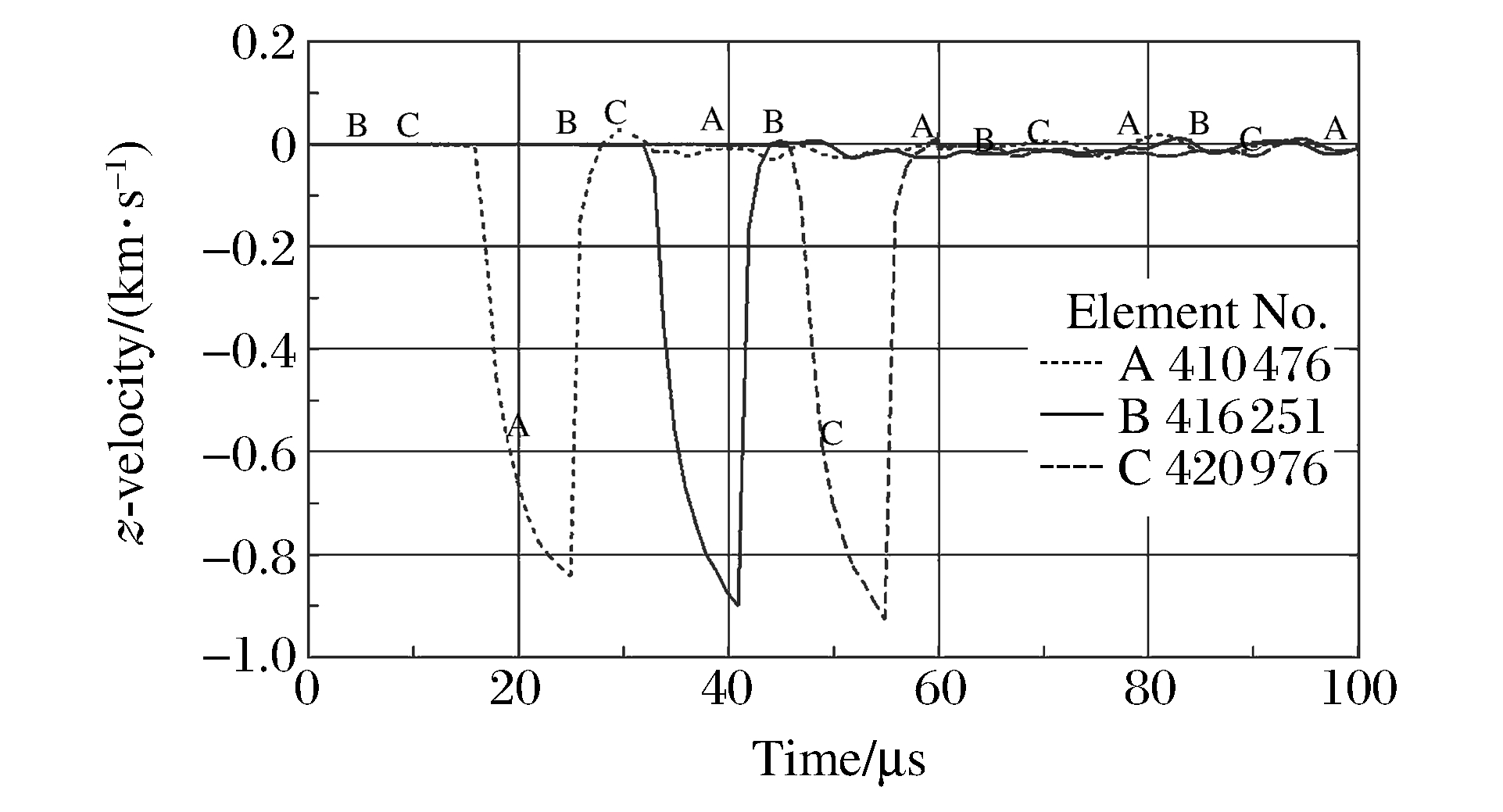
图 7所示是在复板结合界面处所选取的3个特征单元(410 476、416 251、420 976)。图 8所示是这3个特征单元的速度-时间曲线。

图 7 10 mm药厚下复板结合界面处的3个特征单元

Figure 7. 3 characteristic elements at the bonding interface of flyer plate with explosive thickness of 10 mm

下载: 全尺寸图片幻灯片

图 8 10 mm药厚下3个特征单元的速度-时间曲线

Figure 8. Velocity-time curves of 3 characteristic elements with explosive thickness of 10 mm

下载: 全尺寸图片幻灯片

由图 8可以看出，随着距起爆端距离的增加，复板的碰撞速度增大。由文献[17]的结论可知，该现象是由于基板与复板的碰撞在金属板的待复合区产生了强烈振动引起的。

2.1.3 碰撞点压力分布

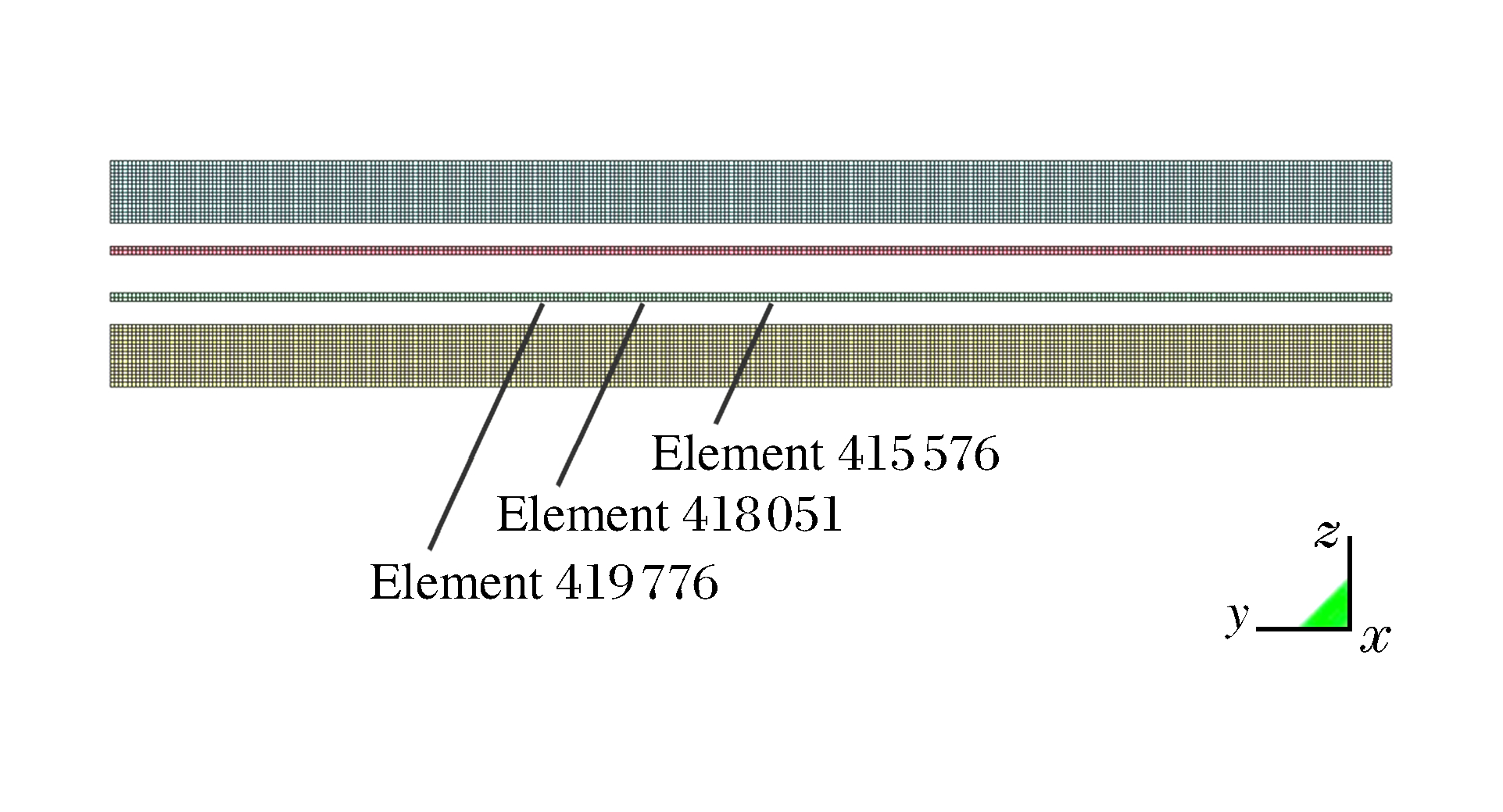
图 9所示是在结合界面处选取的3个特征单元(415 576、418 051、419 776)，单元415 576取在复板中心处，与前期实验^[7]中取样做金相观察的位置一致。图 10所示是3个特征单元的压力历程。

图 9 10 mm药厚下复板结合界面处的3个特征单元

Figure 9. 3 characteristic elements at the bonding interface of flyer plate with explosive thickness of 10 mm

下载: 全尺寸图片幻灯片

图 10 10 mm药厚下3个特征单元的压力-时间曲线

Figure 10. Pressure-time curves of 3 characteristic elements with explosive thickness of 10 mm

下载: 全尺寸图片幻灯片

由图 10可以看出，随着距起爆端距离的增加，复板的碰撞压力增大。由文献[17]的结论可知，该现象是爆轰产物不断堆积以及前碰撞点在金属板待复合区振动能不断增加的共同作用结果。

2.2 5 mm药厚的模拟结果

2.2.1 碰撞点位移

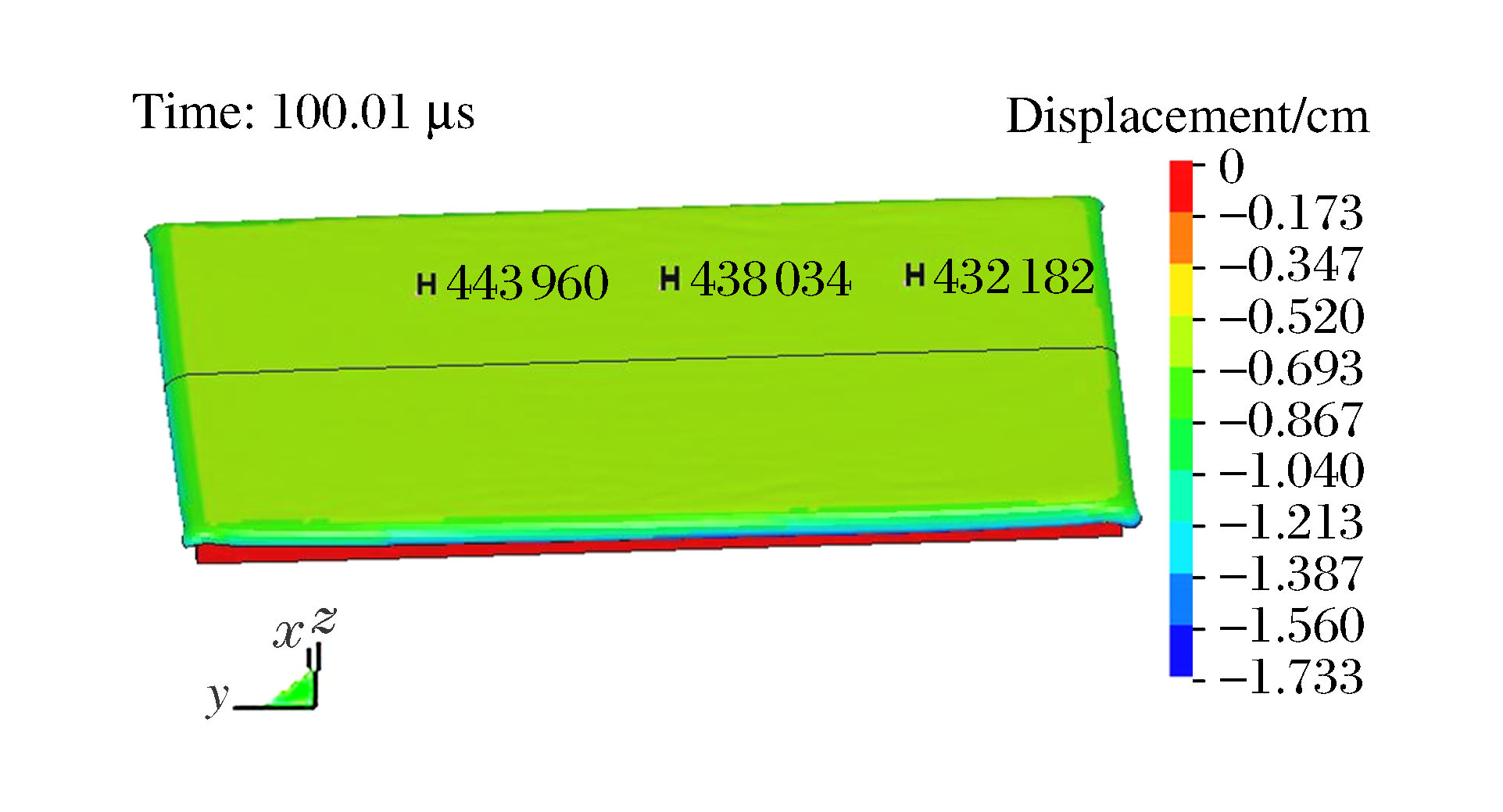
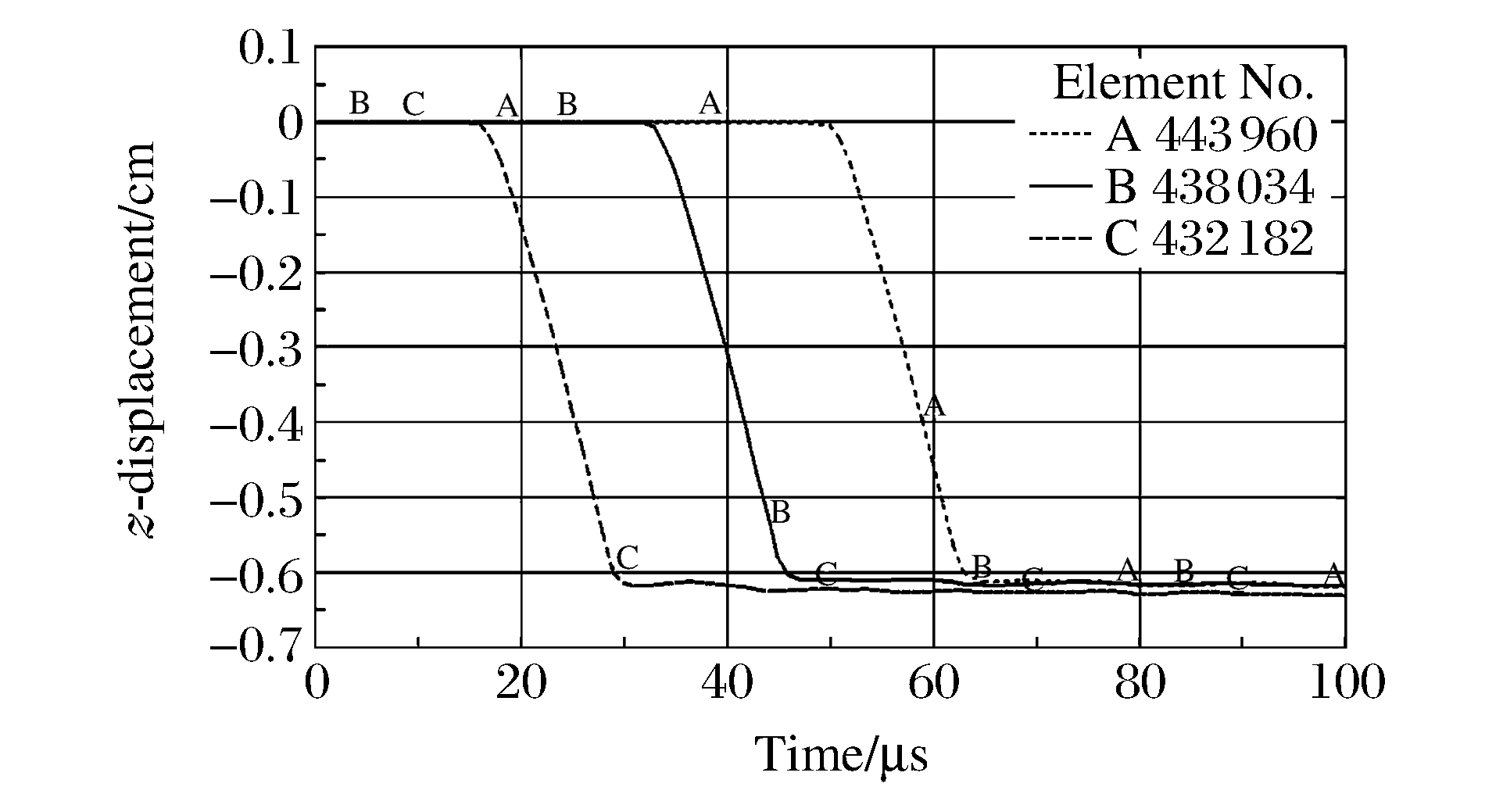
图 11所示是爆炸焊接结束时复板的竖向位移云图。由图 11可看出，复板的位移大致均为6 mm，表明基、复板已完全复合。为了更加直观地观察复板单元位移的变化情况，在复板上选择3个特征单元(432 182、438 034、443 960)，输出其位移-时间曲线，如图 12所示。由图 12可看出，特征单元的竖向位移均略大于6 mm，但较10 mm药厚下的竖向位移小。这是由于5 mm药厚下的爆炸载荷作用比10 mm药厚下小，导致5 mm药厚下的复板减薄率比10 mm药厚下低。

图 11 5 mm药厚下爆炸复合结束时复板的z向位移云图

Figure 11. z-displacement contour of flyer plate with explosive thickness of 5 mm at the end of explosive welding

下载: 全尺寸图片幻灯片

图 12 5 mm药厚下复板上特征单元的z向位移-时间历程

Figure 12. z-displacement histories of 3 characteristic elements with explosive thickness of 5 mm

下载: 全尺寸图片幻灯片

2.2.2 复板碰撞速度

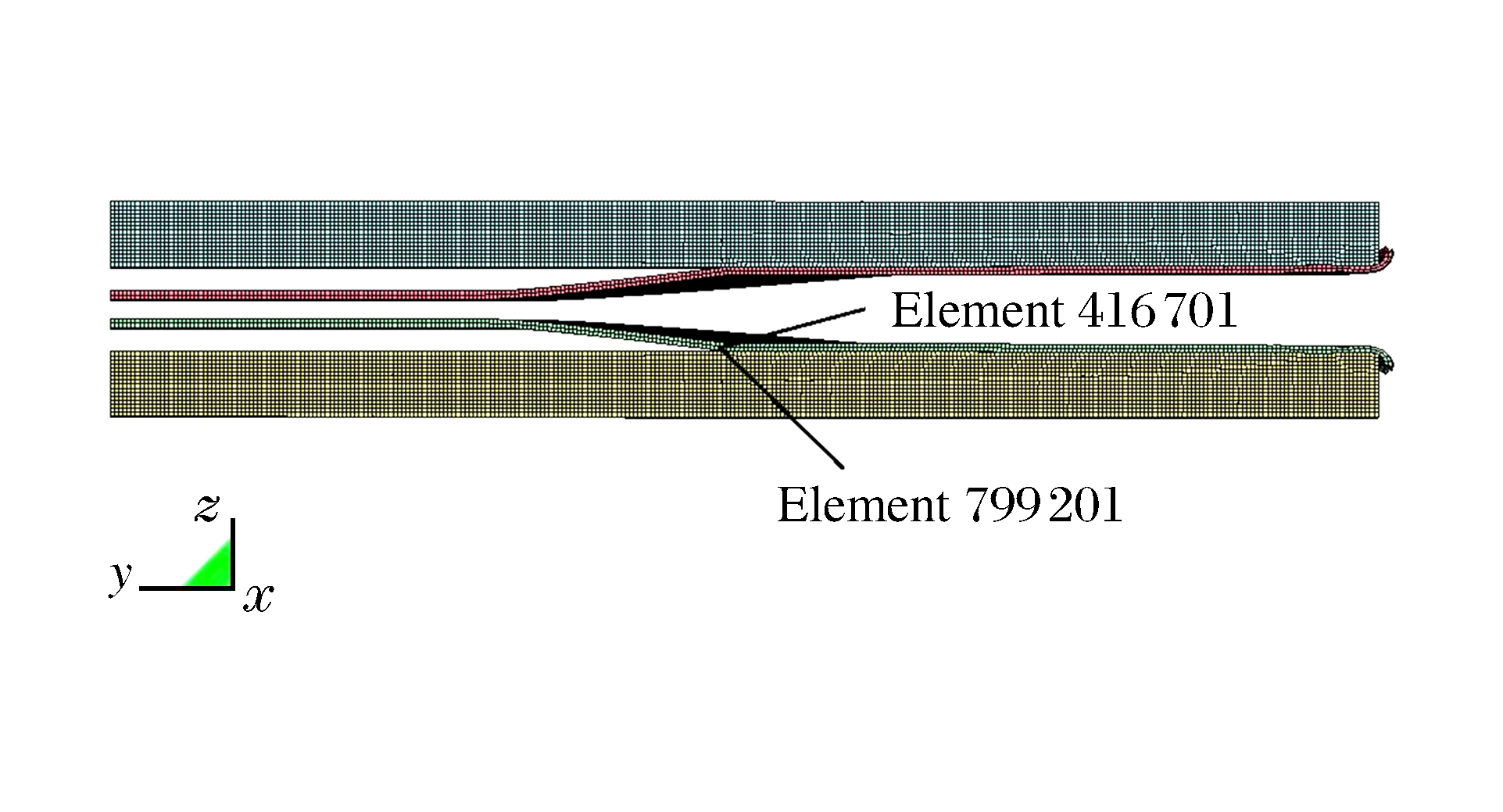
图 13所示是一对分别取自基板与复板结合界面处的特征单元(基板单元：799 201；复板单元：416 701)，特征单元的选取与前期实验^[9]中金相试样的取样位置一致。

图 13 5 mm药厚下的一对特征单元

Figure 13. A pair of characteristic elements with explosive thickness of 5 mm

下载: 全尺寸图片幻灯片

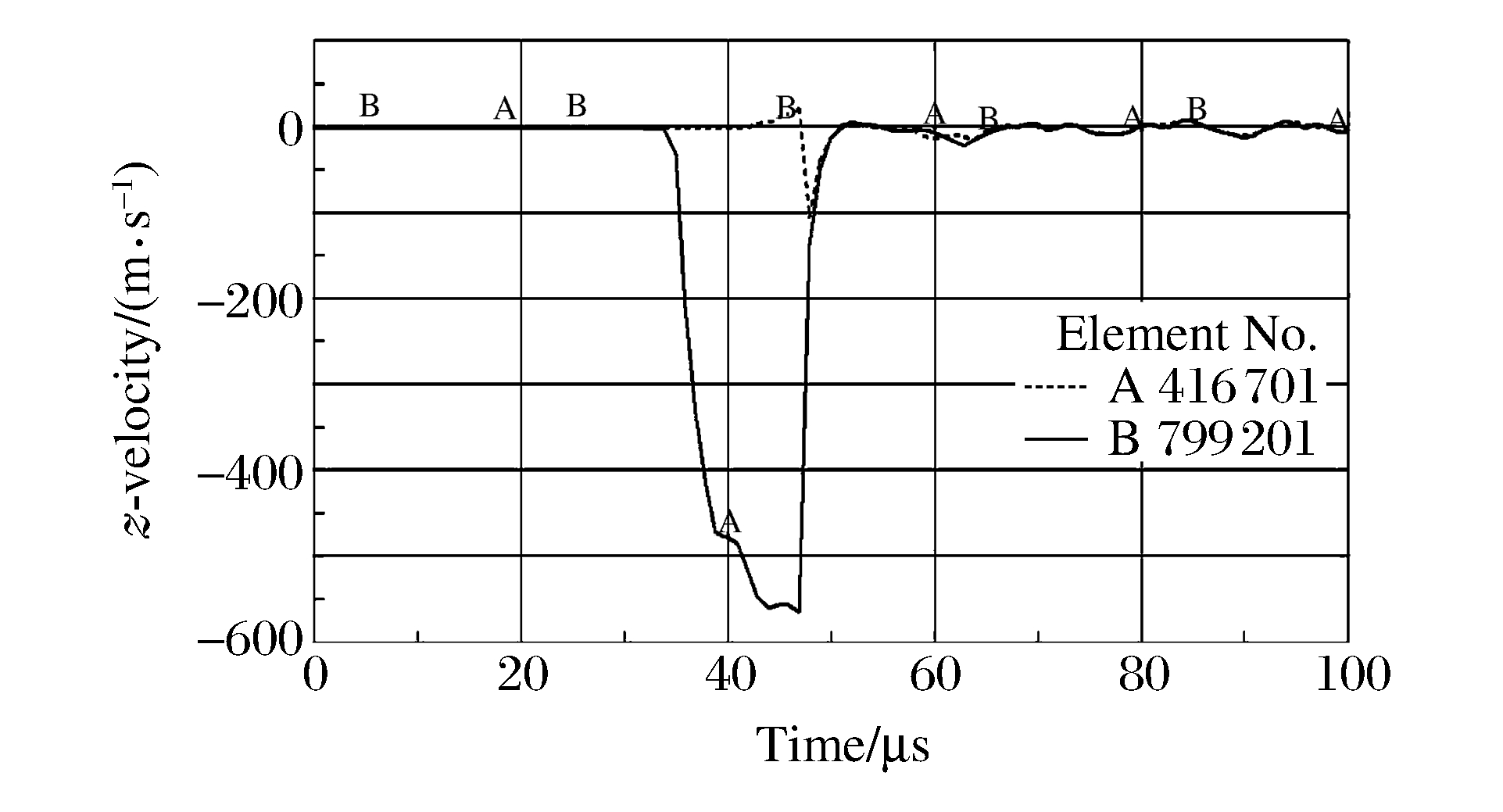
图 14所示是这对特征单元的速度-时间曲线, 可以看出，基板在碰撞前也有一个正的速度峰。复板上所取单元的最大碰撞速度为565 m/s。

图 14 一对特征单元(见图 13)的速度-时间曲线

Figure 14. Velocity-time curves of the pair of characteristic elements (see Fig. 13)

下载: 全尺寸图片幻灯片

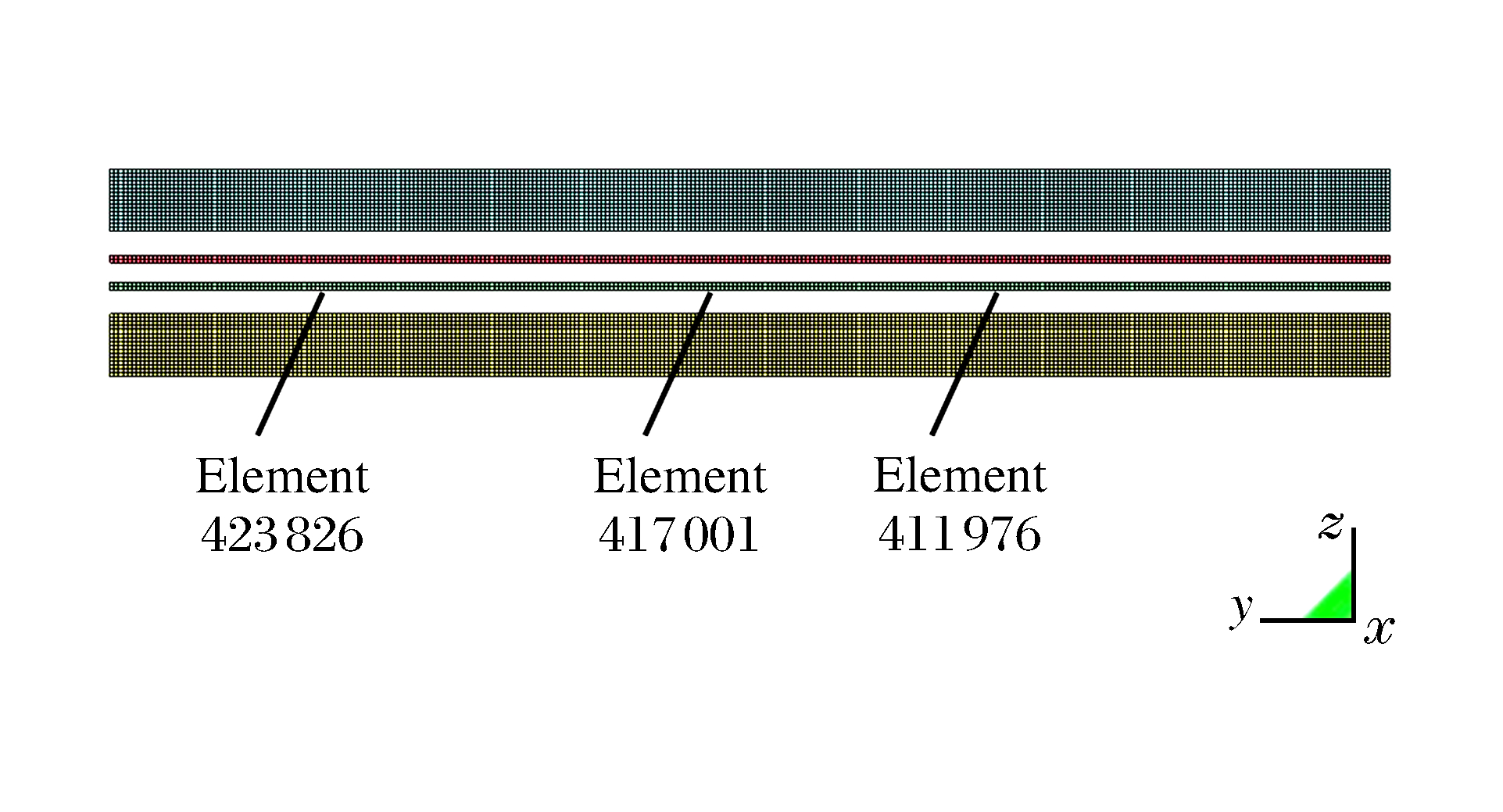
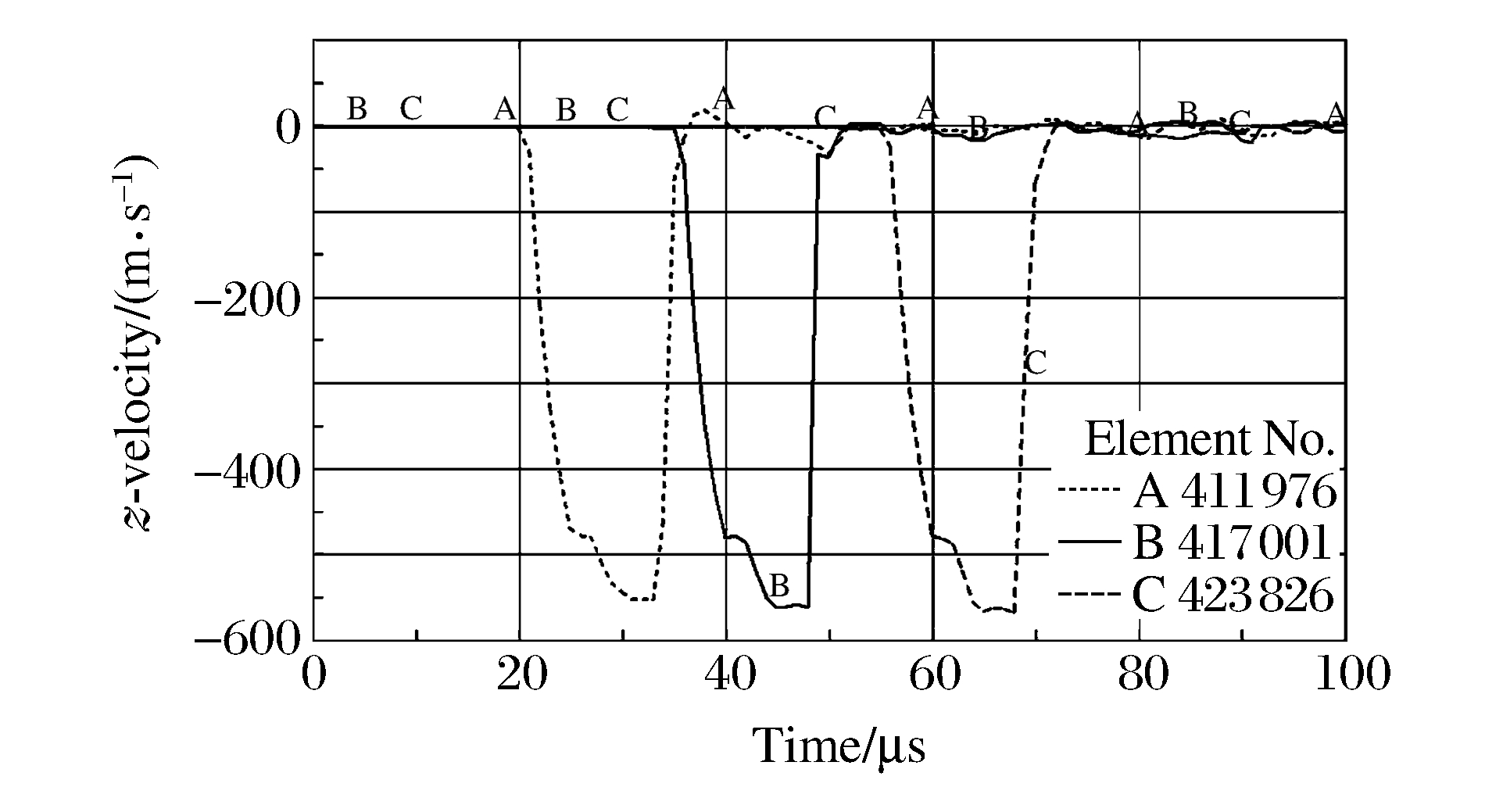
图 15所示是在复板结合界面处所选取的3个特征单元(411 976、417 001、423 826)。图 16所示是这3个特征单元的速度-时间曲线。由图 16可以看出，随着距起爆端距离的增加，复板的碰撞速度增大。

图 15 5 mm药厚下复板结合界面处的3个特征单元

Figure 15. 3 characteristic elements at the bonding interface of flyer plate with explosive thickness of 5 mm

下载: 全尺寸图片幻灯片

图 16 5 mm药厚下3个特征单元的速度-时间曲线

Figure 16. Velocity-time curves of 3 characteristic elements with explosive thickness of 5 mm

下载: 全尺寸图片幻灯片

2.2.3 碰撞点压力分布

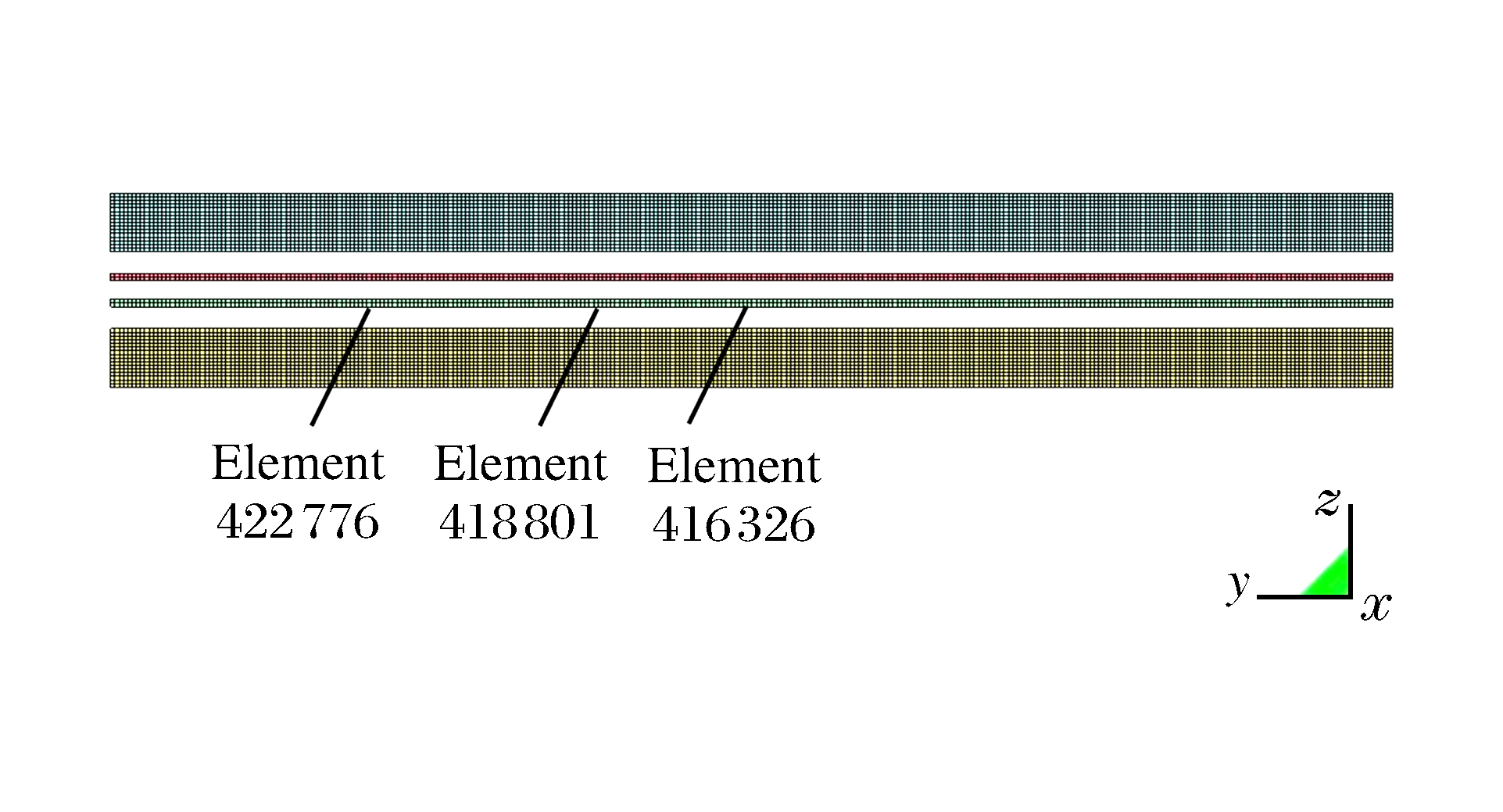
图 17所示是在结合界面处选取的3个特征单元(416 326、418 801、422 776)，单元416 326取在复板中心处，与前期实验^[7]中取样做金相观察的位置一致。图 18所示是这3个特征单元的压力历程。由图 18可以看出，随着距起爆端距离的增加，复板的碰撞压力增大。

图 17 5 mm药厚下复板结合界面处的3个特征单元

Figure 17. 3 characteristic elements at the bonding interface of flyer plate with explosive thickness of 5 mm

下载: 全尺寸图片幻灯片

图 18 5 mm药厚下3个特征单元的压力历程

Figure 18. Pressure histories of 3 characteristic elements with explosive thickness of 5 mm

下载: 全尺寸图片幻灯片

2.3 分析与讨论

由图 6可以看出，10 mm药厚下复板的最大碰撞速度为897 m/s。由图 14可以看出，5 mm药厚下复板的最大碰撞速度为565 m/s。利用前期工作^[18]中提到的3种理论公式(Gurney公式、Aziz公式、Deribas公式)计算了复板的碰撞速度，如表 4、表 5所示，并与数值模拟结果进行了比较。由表 4和表 5可以看出：Gurney公式和Aziz公式的计算结果均存在较大的偏差；而由Deribas公式计算的两组结果与数值模拟结果较接近，误差均未超过5%，且与前期实验结果较吻合，证明了SPH-FEM耦合算法的可靠性。

表 4 10 mm药厚下碰撞速度理论计算结果与数值模拟结果的比较

Table 4. Comparison of collision velocity between theoretical calculation and numerical simulation with explosive thickness of 10 mm

Theoreticalformula	Massfraction	Collision velocity/(m·s^-1)		Error/%
Theoreticalformula	Massfraction	Theoretical calculation^[18]	Simulation	Error/%
Gurney	0.75	1 089	897	-21.0
Aziz	0.75	711	897	20.0
Deribas	0.75	853	897	4.9

下载: 导出CSV

| 显示表格

表 5 5 mm药厚下碰撞速度理论计算结果与数值模拟结果的比较

Table 5. Comparison of collision velocity between theoretical calculation and numerical simulation with explosive thickness of 5 mm

Theoreticalformula	Massfraction	Collision velocity/(m·s^-1)		Error/%
Theoreticalformula	Massfraction	Theoretical calculation^[18]	Simulation	Error/%
Gurney	0.45	863	565	-52.7
Aziz	0.45	480	565	15.0
Deribas	0.45	576	565	-1.9

下载: 导出CSV

| 显示表格

由图 10可以看出，10 mm药厚下复板单元415 576处的碰撞压力为17.08 GPa。由图 18可以看出，5 mm药厚下复板单元416 326处的碰撞压力为11.25 GPa。

Ezra等提出的碰撞压力的计算公式为^[16]

$p=\frac{{{\rho }_{1}}{{v}_{\text{s}, 1}}{{v}_{\text{p}}}}{1+\frac{{{\rho }_{1}}{{v}_{\text{s}, 1}}}{{{\rho }_{2}}{{v}_{\text{s, }2}}}}$

(3)

式中：v_{s, 1}、v_{s, 2}分别表示复板、基板的声速，m·s^-1；ρ₁、ρ₂分别表示复板、基板的密度，g·cm^-3；v_p表示复板的碰撞速度，m·s^-1。

结合表 4和表 5中3种理论公式计算得到的碰撞速度，通过(3)式可得到复板的碰撞压力，表 6及表 7为其理论计算值与数值模拟结果的比较。可见：Gurney公式和Aziz公式的计算结果均存在较大的偏差；而由Deribas公式计算的两组结果与数值模拟结果较接近，误差均未超过5%，说明Deribas公式和SPH-FEM耦合方法对双面爆炸焊接具有较好的指导意义。

表 6 10 mm药厚下碰撞压力理论计算结果与数值模拟结果的比较

Table 6. Comparison of collision pressure betweentheoretical calculation and numerical simulationwith explosive thickness of 10 mm

Theoreticalformula	Collision pressure/GPa		Error/%
Theoreticalformula	Calculation	Simulation	Error/%
Gurney	22.08	17.08	-29.3
Aziz	14.42	17.08	15.6
Deribas	17.30	17.08	-1.3

下载: 导出CSV

| 显示表格

表 7 5 mm药厚下碰撞压力理论计算结果与数值模拟结果的比较

Table 7. Comparison of collision pressure betweentheoretical calculation and numerical simulationwith explosive thickness of 5 mm

Theoreticalformula	Collision pressure/GPa		Error/%
Theoreticalformula	Calculation	Simulation	Error/%
Gurney	17.50	11.25	-55.6
Aziz	9.73	11.25	13.5
Deribas	11.68	11.25	-3.8

下载: 导出CSV

| 显示表格

3. 结论

利用LS-DYNA软件和SPH-FEM耦合方法对前期双面爆炸焊接实验进行了三维数值模拟，并将模拟结果与实验及理论计算结果进行了对比，得到如下结论。

(1) 10 mm药厚和5 mm药厚下复板位移均略大于间隙值6 mm，这是由于爆轰载荷作用下复板有一定的减薄率所致。

(2) 10 mm药厚下，复板中部的最大碰撞速度为897 m/s，碰撞压力为17.08 GPa；5 mm药厚下，复板中部的最大碰撞速度为565 m/s，碰撞压力为11.25 GPa。通过与3种理论公式(Gurney公式、Aziz公式、Deribas公式)计算得到的碰撞速度进行比较发现，数值模拟结果与Deribas公式的计算结果较接近，误差较小，且与实验结果较吻合，证明了SPH-FEM耦合方法用于双面爆炸复合模拟的有效性，同时Deribas公式和SPH-FEM耦合方法对双面爆炸复合具有较好的指导意义。

(3) 10 mm药厚和5 mm药厚下复板的碰撞速度及碰撞压力均随着距起爆端距离的增加而增大，该现象是由于爆轰产物的不断堆积和前碰撞点在金属板待复合区振动能的不断增加共同作用的结果。

图 1 (a) 螳螂虾的CT扫描图像^[18]，(b) 冲击区^[18]，(c) 冲击区的宏观微观结构^[18]，(d) 夹芯管示意图

Figure 1. (a) CT image of mantis shrimp^[18]; (b) impact zone^[18]; (c) macroscopic and microscopic structure of shrimp claws^[18]; (d) schematic diagram of sandwich tube

下载: 全尺寸图片幻灯片

图 2 3种波纹芯层示意图

Figure 2. Schematic diagram of three types of corrugated core

下载: 全尺寸图片幻灯片

图 3 实验装置示意图

Figure 3. Schematic diagram of experimental setup

下载: 全尺寸图片幻灯片

图 4 双向波纹管内外管变形图

Figure 4. Deformation diagram of inner and outer tubes of bidirectional bellows

下载: 全尺寸图片幻灯片

图 5 内部爆炸载荷下双向波纹夹芯管的实验结果

Figure 5. Experimental results of bi-directionally corrugated sandwich tubes under inner blast loading

下载: 全尺寸图片幻灯片

图 6 应力-应变曲线

Figure 6. Stress-strain curves

下载: 全尺寸图片幻灯片

图 7 R4A2试件的数值模拟与实验变形模态对比

Figure 7. Comparison of the deformation modes between the simulation and experimental results of R4A2

下载: 全尺寸图片幻灯片

图 8 不同波纹夹芯管的抗爆性能对比

Figure 8. Comparison of the blast resistance for different corrugated sandwich tubes

下载: 全尺寸图片幻灯片

图 9 不同波纹数的双向波纹夹芯管的抗爆性能

Figure 9. Blast resistance of bi-directional corrugated sandwich tubes with different corrugation numbers

下载: 全尺寸图片幻灯片

图 10 不同内外管厚度下R5A5结构的抗爆性能

Figure 10. Blast resistance of R5A5 structure with different thicknesses of inner and outer tubes

下载: 全尺寸图片幻灯片

图 11 不同TNT当量下R5A5的抗爆性能

Figure 11. Blast resistance of R5A5 under different equivalent TNT dosage

下载: 全尺寸图片幻灯片

图 12 BP神经网络模型

Figure 12. BP neural network structure diagram

下载: 全尺寸图片幻灯片

图 13 粒子群优化神经网络算法流程

Figure 13. Flow chart of particle swarm optimization neural network algorithm

下载: 全尺寸图片幻灯片

图 14 预测结果对比：(a) S_EA的BP预测与FE结果比较，(b) M_D的BP预测与FE结果比较，(c) S_EA的PSO-BP预测与FE结果比较，(d) M_D的PSO-BP预测与FE结果比较，(e) S_EA的RSM预测与FE结果比较，(f) M_D的RSM预测与FE结果比较

Figure 14. Comparison of predicted results: (a) comparison of BP prediction with FE results of S_EA; (b) comparison of BP prediction with FE results of M_D; (c) comparison of PSO-BP prediction with FE results of S_EA; (d) comparison of PSO-BP prediction with FE results of M_D; (e) comparison of RSM prediction with FE results of S_EA; (f) comparison of RSM prediction with FE results of M_D

下载: 全尺寸图片幻灯片

图 15 RSM生成的样本点与对照组的动态响应

Figure 15. Dynamic responses of the sample nodes by RSM and the control group

下载: 全尺寸图片幻灯片

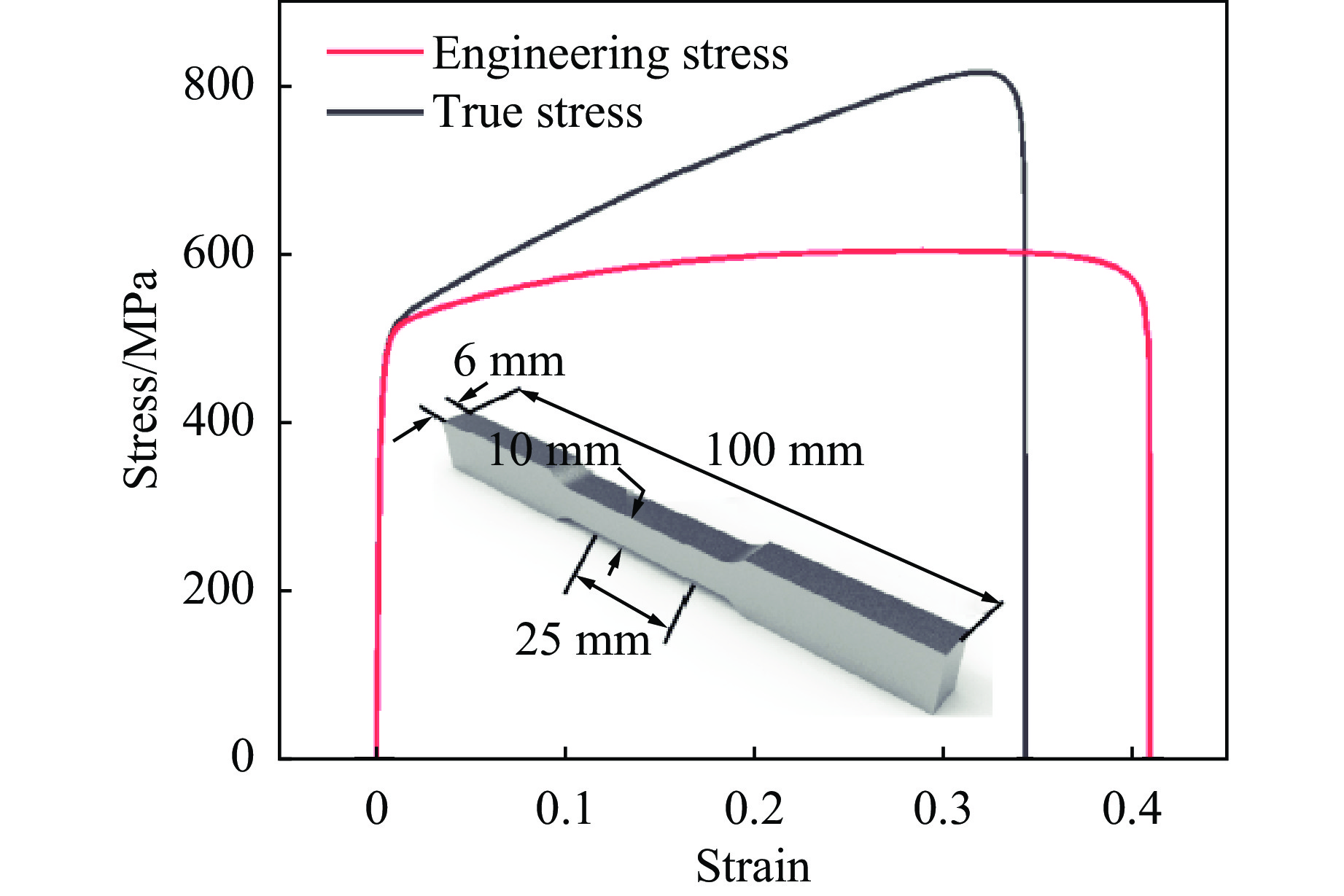
表 1 材料参数

Table 1. Material parameters

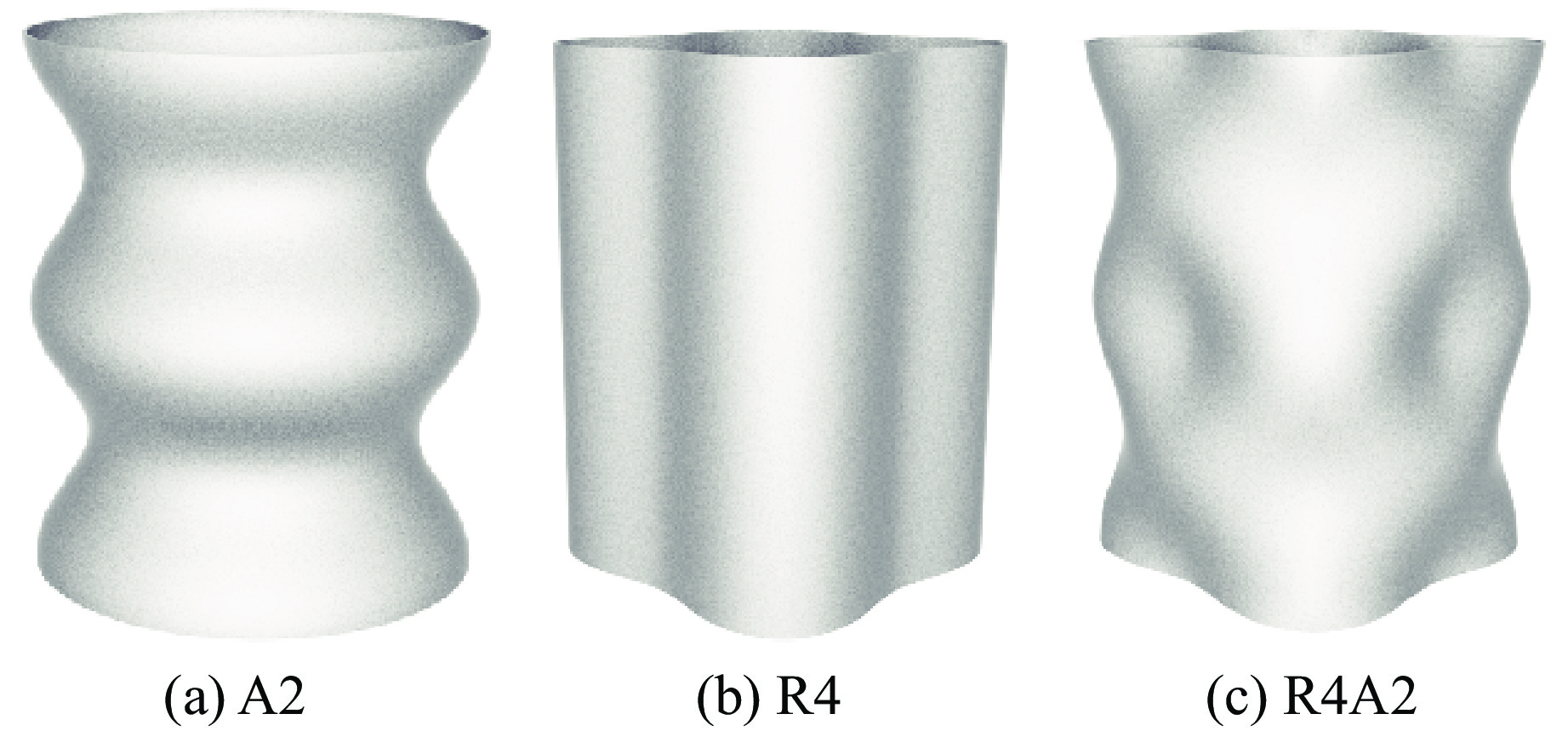
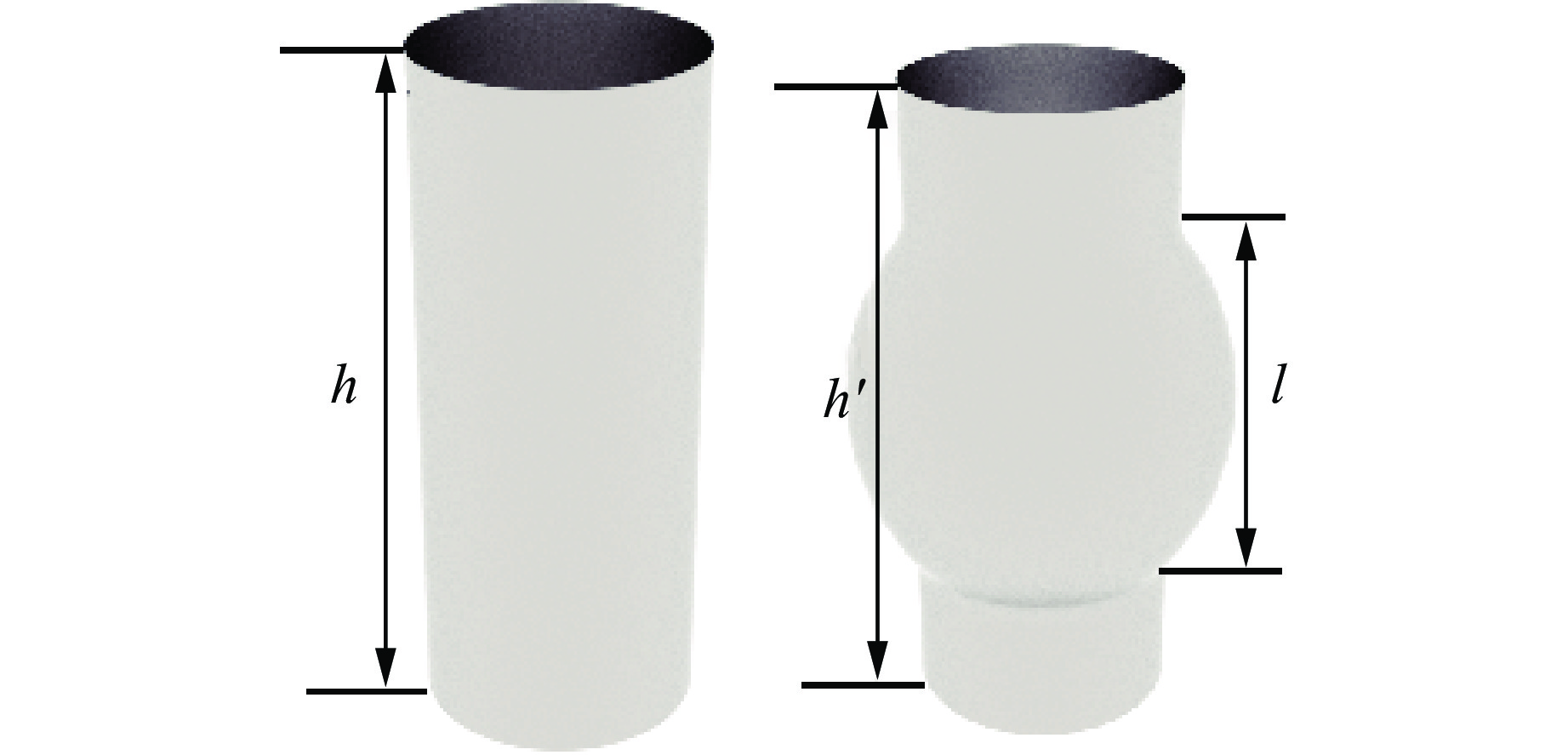
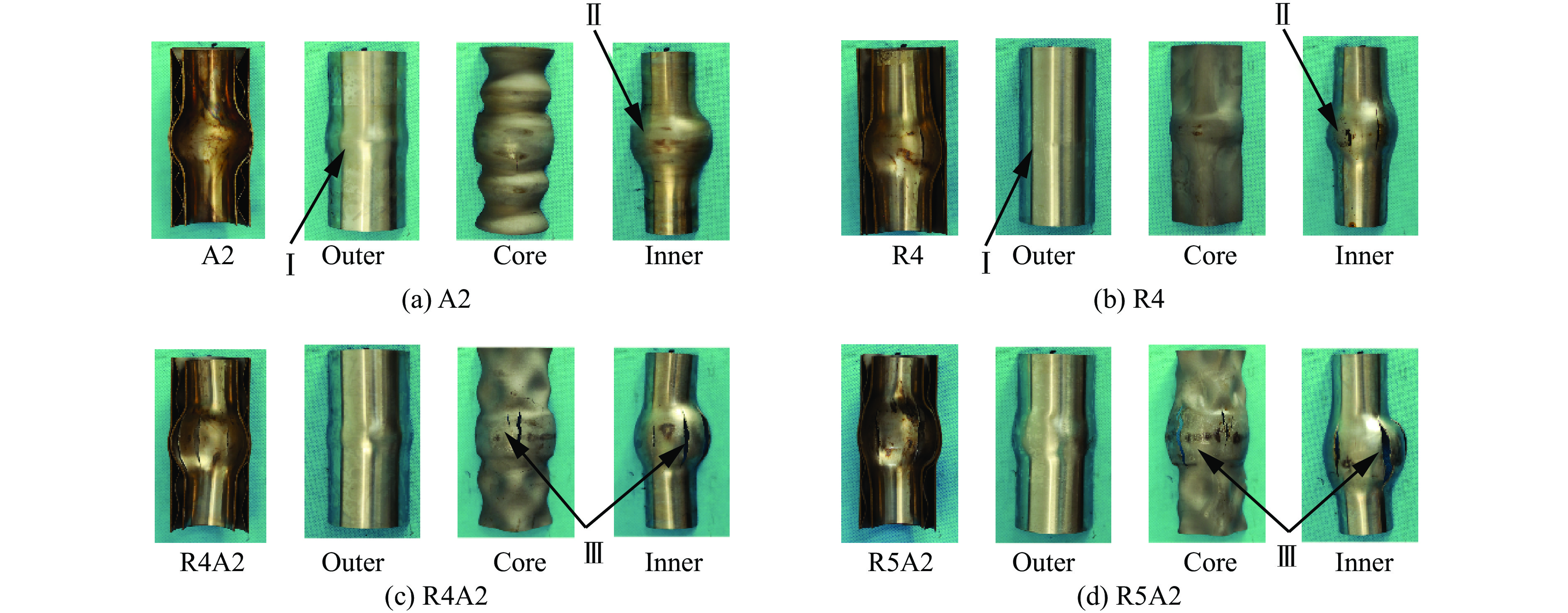
Material	Density/(kg∙m⁻³)	Young’s modulus/GPa	Poisson’s ratio	Yield stress/MPa
316L steel	7830	185	0.30	470
304 steel	7830	193	0.25	250

下载: 导出CSV

表 2 双向波纹夹芯管的变形/失效实验测定值

Table 2. Deformation/failure test values of bidirectional bellows sandwich tube

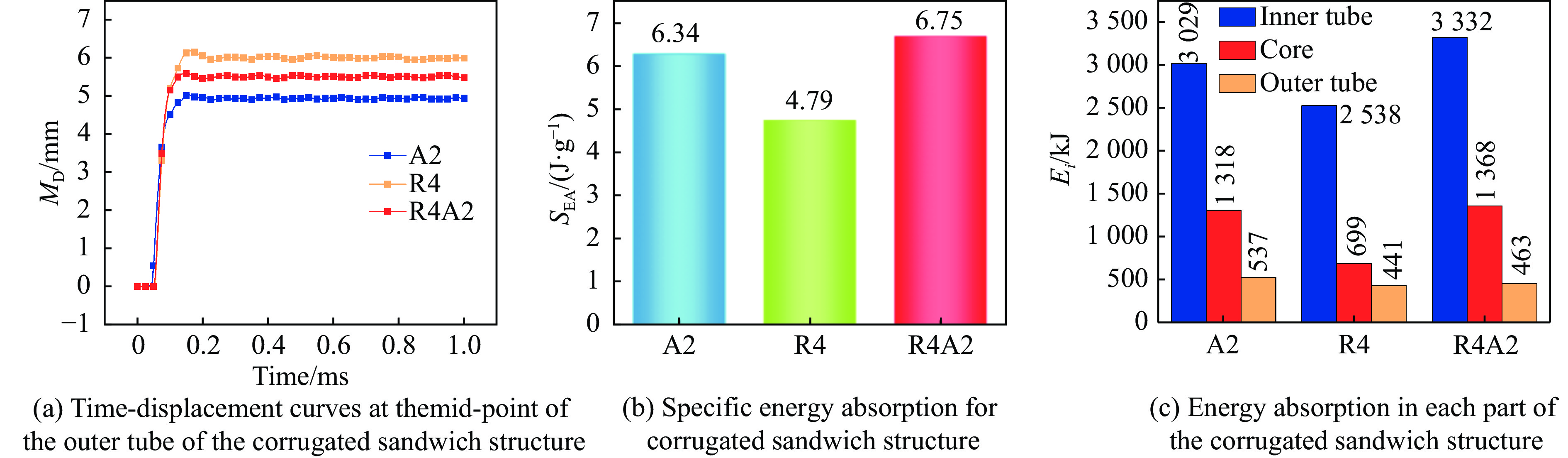
Sample	${\varepsilon _h}$ /%		$l$ /mm		c_l/mm			c_w/mm
Sample	Inner tube	Outer tube	Inner tube	Outer tube	Inner tube crack	Core crack	Outer tube crack	Inner tube crack	Core crack	Outer tube crack
A2	4.1	2.6	85	48
R4	5.1	2.1	112	30	46			0.3
R4A2	4.6	2.6	127	40	76	57		14	3
R5A2	4.6	3.1	145	64	80	72		11	5

下载: 导出CSV

表 3 内外管中点挠度的数值模拟与实验结果的对比

Table 3. Comparison between numerical simulation and experimental results for mid-point deflection of inner and outer tubes

Sample	R	A	t_i/mm	t_o/mm	M_D for inner tube			M_D for outer tube
Sample	R	A	t_i/mm	t_o/mm	Sim./mm	Exp./mm	Error/%	Sim./mm	Exp./mm	Error/%
A2	0	2	2	2	10.8	11.2	3.7	4.9	4.6	−6.1
R4	4	0	2	2	11.8	12.0	1.7	6.0	5.6	−6.7
R4A2	4	2	2	2	15.2	14.0	−7.9	5.5	5.0	−8.2
R5A2	5	2	2	2	18.8	18.0	−4.2	9.5	8.5	−10.5

下载: 导出CSV

表 4 计算模型数与CPU计算时间

Table 4. Calculated model number and CPU calculation time

Model	Number of CPU^*	Number of meshes	Calculation time/min
Model	Number of CPU^*	Number of meshes	k=50	k=100	k=200	k=500	k=1000
FE	8	55850	475	950	1 900	4750	9500
BP	2		950	951	952	954	958
PSO-BP	2		954	959	968	996	1042
RSM	2		950	950	951	954	957
Note: * Intel (R) Core (TM) i7-13700 2.10 GHz

下载: 导出CSV

表 5 预测模型误差分析

Table 5. Error analysis of prediction models

Model	${\theta _{{\text{relative}}}}$ /%		${\theta _{{\text{average}}}}$ /%		${R^2}$
Model	S_EA	M_D	S_EA	M_D	S_EA	M_D
BP	2.05	33.2	0.48	6.65	0.999	0.975
PSO-BP	2.15	28.3	0.35	3.52	0.999	0.994
RSM	0.97	22.1	0.25	0.24	0.999	0.996

下载: 导出CSV

最大 $M_{\mathrm{D}}$ 约束下最大化 $S_{\mathrm{EA}}$ 的优化设计

Optimal design of maximized $S_{\mathrm{EA}}$ with maximum $M_{\mathrm{D}}$ constraints

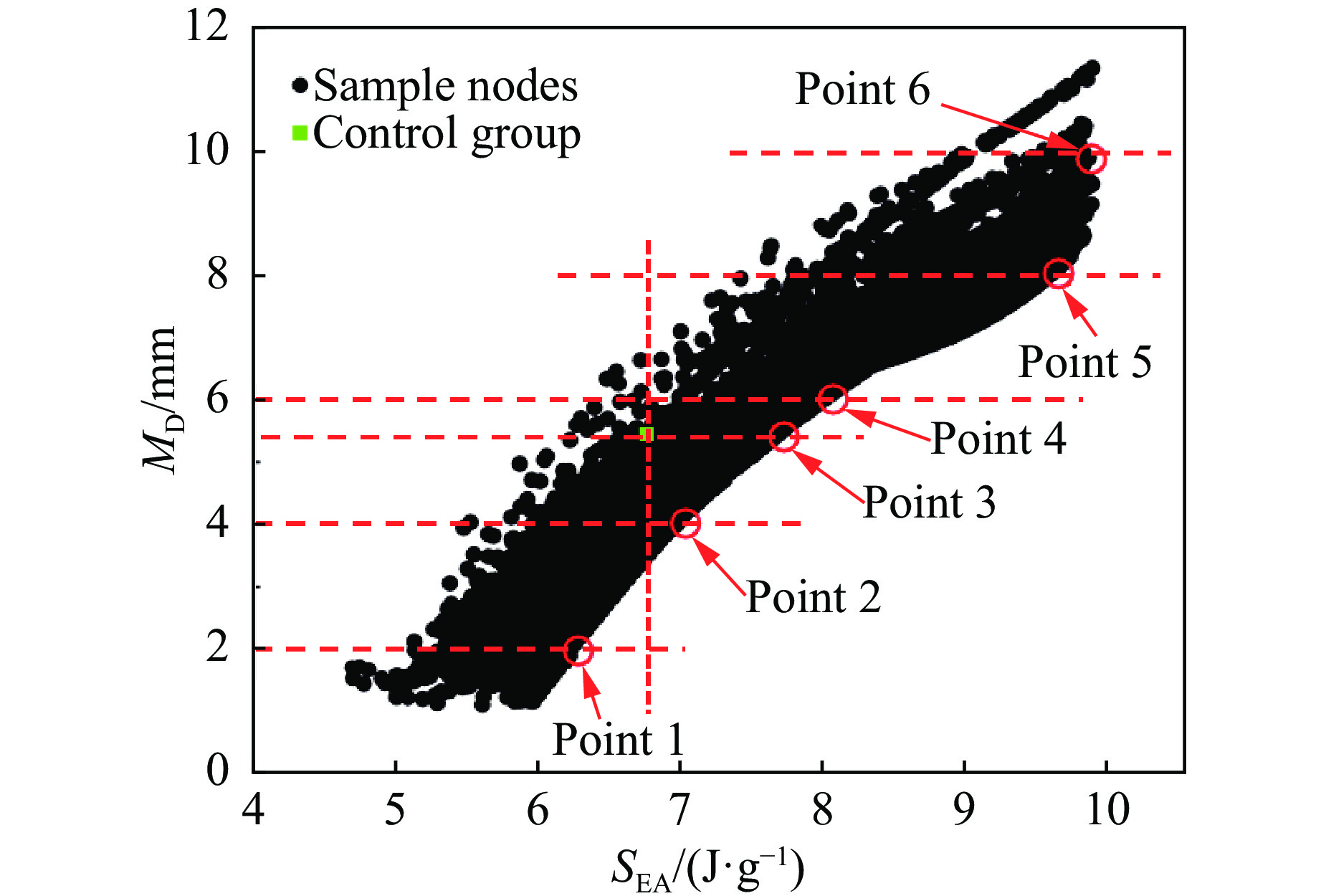
No.	R	A	t_i/mm	t_o/mm	M_D/mm		S_EA/(J∙g⁻¹)
No.	R	A	t_i/mm	t_o/mm	RSM	FE	RSM	FE
1	3	6	2.40	1.50	2.00	2.12	6.27	6.28
2	6	4	2.20	1.50	4.00	4.00	6.96	6.97
3	6	5	2.05	1.50	5.24	5.22	7.57	7.56
4	6	5	1.94	1.50	6.00	5.97	8.00	8.02
5	6	6	1.58	1.50	8.00	8.05	9.40	9.44
6	5	5	1.50	1.50	10.00	8.80	9.89	9.93

下载: 导出CSV

参考文献(24)

[1]	PANDARKAR A, GOEL M D, HORA M S. Axial crushing of hollow and foam filled tubes: an overview [J]. Sādhanā, 2016, 41(8): 909–921.
[2]	YAO R Y, PANG T, ZHANG B, et al. On the crashworthiness of thin-walled multi-cell structures and materials: state of the art and prospects [J]. Thin-Walled Structures, 2023, 189: 110734. doi: 10.1016/j.tws.2023.110734
[3]	XUE Z Y, HUTCHINSON J W. A comparative study of impulse-resistant metal sandwich plates [J]. International Journal of Impact Engineering, 2004, 30(10): 1283–1305. doi: 10.1016/j.ijimpeng.2003.08.007
[4]	ZHANG P, CHENG Y S, LIU J, et al. Experimental and numerical investigations on laser-welded corrugated-core sandwich panels subjected to air blast loading [J]. Marine Structures, 2015, 40: 225–246. doi: 10.1016/j.marstruc.2014.11.007
[5]	WEI Y C, ZHANG C J, YUAN Y, et al. Blast response of additive manufactured Ti-6Al-4V sandwich panels [J]. International Journal of Impact Engineering, 2023, 176: 104553. doi: 10.1016/j.ijimpeng.2023.104553
[6]	SHEN J H, LU G X, ZHAO L M, et al. Short sandwich tubes subjected to internal explosive loading [J]. Engineering Structures, 2013, 55: 56–65. doi: 10.1016/j.engstruct.2011.12.005
[7]	LIU X R, TIAN X G, LU T J, et al. Blast resistance of sandwich-walled hollow cylinders with graded metallic foam cores [J]. Composite Structures, 2012, 94(8): 2485–2493. doi: 10.1016/j.compstruct.2012.02.029
[8]	LIANG M Z, LI X Y, LIN Y L, et al. Multiobjective blast-resistance optimization of gradient foam sandwiched cylindrical container [J]. Thin-Walled Structures, 2020, 157: 107114. doi: 10.1016/j.tws.2020.107114
[9]	LI S Q, LU G X, WANG Z H, et al. Finite element simulation of metallic cylindrical sandwich shells with graded aluminum tubular cores subjected to internal blast loading [J]. International Journal of Mechanical Sciences, 2015, 96/97: 1–12. doi: 10.1016/j.ijmecsci.2015.03.011
[10]	ZHANG T H, LIU Z F, LI S Q, et al. Dynamic response and energy absorption performance of aluminum foam-filled sandwich circular tubes under internal blast loading [J]. International Journal of Impact Engineering, 2023, 173: 104458. doi: 10.1016/j.ijimpeng.2022.104458
[11]	YANG F, XIE W H, MENG S H. Impact and blast performance enhancement in bio-inspired helicoidal structures: a numerical study [J]. Journal of the Mechanics and Physics of Solids, 2020, 142: 104025. doi: 10.1016/j.jmps.2020.104025
[12]	王海任, 李世强, 刘志芳, 等. 爆炸载荷下双向梯度仿生夹芯圆板的力学行为 [J]. 爆炸与冲击, 2021, 41(4): 043201. doi: 10.11883/bzycj-2020-0132 WANG H R, LI S Q, LIU Z F, et al. Mechanical behaviors of bi-directional gradient bio-inspired circular sandwich plates under blast loading [J]. Explosion and Shock Waves, 2021, 41(4): 043201. doi: 10.11883/bzycj-2020-0132
[13]	GHAZLAN A, NGO T, VAN LE T, et al. Blast performance of a bio-mimetic panel based on the structure of nacre: a numerical study [J]. Composite Structures, 2020, 234: 111691. doi: 10.1016/j.compstruct.2019.111691
[14]	YANG X F, MA J X, SHI Y L, et al. Crashworthiness investigation of the bio-inspired bi-directionally corrugated core sandwich panel under quasi-static crushing load [J]. Materials & Design, 2017, 135: 275–290.
[15]	YANG J K, GU D D, LIN K J, et al. Optimization of bio-inspired bi-directionally corrugated panel impact-resistance structures: numerical simulation and selective laser melting process [J]. Journal of the Mechanical Behavior of Biomedical Materials, 2019, 91: 59–67. doi: 10.1016/j.jmbbm.2018.11.026
[16]	DALIRI V, ZEINEDINI A. Flexural behavoiur of the composite sandwich panels with novel and regular corrugated cores [J]. Applied Composite Materials, 2019, 26(3): 963–982. doi: 10.1007/s10443-019-09761-x
[17]	SHAHBAZI A, ZEINEDINI A. Impact response of E-glass/epoxy composite bi-directional corrugated core sandwich panels [J]. Polymers and Polymer Composites, 2021, 29(9): 1563–1574. doi: 10.1177/0967391120982751
[18]	黄晗, 许述财, 陈姮. 仿生波纹夹层结构耐撞性分析及优化 [J] 爆炸与冲击, 2021, 41(8): 083102. HUANG H, XU S C, CHEN H. Crashworthiness analysis and optimization of bionic corrugated sandwich structures [J]. Explosion and Shock Waves, 2021, 41(8): 083102.
[19]	LI X, XU R, ZHANG X, et al. Inner blast response of fiber reinforced aluminum tubes [J]. International Journal of Impact Engineering, 2023, 172: 104416. doi: 10.1016/j.ijimpeng.2022.104416
[20]	LI S Q, YU B L, KARAGIOZOVA D, et al. Experimental, numerical, and theoretical studies of the response of short cylindrical stainless steel tubes under lateral air blast loading [J]. International Journal of Impact Engineering, 2019, 124: 48–60. doi: 10.1016/j.ijimpeng.2018.10.004
[21]	CHENG J H, GUSSEV M, ALLEN J, et al. Deformation and failure of PrintCast A356/316L composites: digital image correlation and finite element modeling [J]. Materials & Design, 2020, 195: 109061.
[22]	XIAO D B, DONG Z C, LI Y, et al. Compression behavior of the graded metallic auxetic reentrant honeycomb: experiment and finite element analysis [J]. Materials Science and Engineering: A, 2019, 758: 163–171. doi: 10.1016/j.msea.2019.04.116
[23]	朱玉富, 赵春风, 周志航. 基于机器学习的钢筋混凝土板在爆炸作用下的最大位移预测模型 [J] 高压物理学报, 2023, 37(2): 024205. ZHU Y F, ZHAO C F, ZHOU Z H. Prediction model of maximum displacement for RC slabs under blast load based on machine learning [J]. Chinese Journal of High Pressure Physics, 2023, 37(2): 024205.
[24]	CHEN D, JING L, YANG F. Optimal design of sandwich panels with layered-gradient aluminum foam cores under air-blast loading [J]. Composites Part B: Engineering, 2019, 166: 169–186. doi: 10.1016/j.compositesb.2018.11.125

施引文献

资源附件(0)

访问统计

点击查看大图

图(15) / 表(6)

计量

文章访问数: 127
HTML全文浏览量: 50
PDF下载量: 24

1. 计算模型及参数选取
1.1 计算模型
1.2 材料模型及参数设定
2. 模拟结果与分析
2.1 10 mm药厚的模拟结果
2.2 5 mm药厚的模拟结果
2.3 分析与讨论
3. 结论

内爆炸载荷下双向波纹夹芯管的动力响应及抗爆性能预测

doi: 10.11858/gywlxb.20240782

作者简介: 唐 波（1999－），男，硕士研究生，主要从事轻质结构冲击动力学行为研究. E-mail：tb15881862576@163.com

通讯作者: 李世强（1986－），男，博士，副教授，主要从事结构冲击动力学研究. E-mail：lishiqiang@tyut.edu.cn

计量

出版历程

Blast Resistance and Prediction of Bi-Directional Corrugated Sandwich Tubes under Internal Blast Loading

1. 计算模型及参数选取

1.1 计算模型

1.2 材料模型及参数设定

2. 模拟结果与分析

2.1 10 mm药厚的模拟结果

2.1.1 碰撞点位移

2.1.2 复板碰撞速度

2.1.3 碰撞点压力分布

2.2 5 mm药厚的模拟结果

2.2.1 碰撞点位移

2.2.2 复板碰撞速度

2.2.3 碰撞点压力分布

2.3 分析与讨论

3. 结论

计量

出版历程

目录

1. 计算模型及参数选取

1.1 计算模型

1.2 材料模型及参数设定

2. 模拟结果与分析

2.1 10 mm药厚的模拟结果

2.2 5 mm药厚的模拟结果

2.3 分析与讨论

3. 结论

作者简介:
唐　波（1999－），男，硕士研究生，主要从事轻质结构冲击动力学行为研究. E-mail：tb15881862576@163.com

通讯作者:
李世强（1986－），男，博士，副教授，主要从事结构冲击动力学研究. E-mail：lishiqiang@tyut.edu.cn