硝酸铷高压相变和物理性质的第一性原理研究

王晓雪; 丁雨晴; 王晖

doi:10.11858/gywlxb.20240776

硝酸铷高压相变和物理性质的第一性原理研究

doi: 10.11858/gywlxb.20240776

哈尔滨师范大学物理与电子工程学院, 黑龙江哈尔滨　150025

基金项目: 国家自然科学基金（11974135）

详细信息

作者简介:
王晓雪（1998－），女，硕士研究生，主要从事高压下凝聚态物质结构与物性的理论研究. E-mail：wx@fysik.cn

通讯作者:
王　晖（1981－），男，博士，教授，主要从事高压下凝聚态物质结构与物性的理论研究. E-mail：wh@fysik.cn

中图分类号: O521.2; O469
计量
- 文章访问数: 294
- HTML全文浏览量: 92
- PDF下载量: 49
出版历程
- 收稿日期: 2024-04-01
- 修回日期: 2024-04-09
- 录用日期: 2024-04-15
- 刊出日期: 2024-07-25

First-Principles Study of the High-Pressure Phase Transition and Physical Properties of Rubidium Nitrate

School of Physics and Electronic Engineering, Harbin Normal University, Harbin 150025, Heilongjiang, China

摘要

摘要: 采用基于密度泛函理论的第一性原理计算，并结合CALYPSO晶体结构预测软件，系统地探索了零温下RbNO₃的高压结构与物理性质。基于RbNO₃-Ⅳ相的实验数据，比较了4种不同泛函的准确性，发现PBEsol泛函的准确性最高。基于该泛函预测到RbNO₃在零温下的相变序列为R3m → Pnma → Pmmn （实验Ⅴ相），相变压强依次为1.7 和8.2 GPa，2次相变均为一级相变，体积坍塌率分别为3.73%和2.54%。研究结果表明，低压下RbNO₃可能存在有别于实验（常温常压下）给出的P3₁结构的低温新相。3个相在各自能量稳定的压强范围内均满足“伯恩-黄昆”弹性稳定性判据，且在整个布里渊区中均不存在声子虚频现象，表明它们均具有动力学稳定性。电子性质分析表明，3个相均为半导体，相变引起的带隙变化普遍较小，加压普遍地抑制了铷离子的电荷向硝酸根离子转移。所预测的高压相变序列及各个相的弹性、晶格动力学、电子结构性质可为后续实验与理论研究提供参考。
- 高压 /
- 相变 /
- 第一性原理 /
- RbNO₃
Abstract: The high-pressure structure and physical properties of RbNO₃ at zero temperature was systematically explored using first-principles calculations based on density generalized theory combined with CALYPSO crystal structure predictions. The accuracy of four different functionals was compared based on experimental data of the RbNO₃-Ⅳ phase, and the revised PBE for solids (PBEsol) functional was found to be the most reliable. The zero-temperature phase transition sequence of RbNO₃ predicted is R3m→Pnma→Pmmn (experimental phaseⅤ) based on the PBEsol functional, and the two phase transition pressures are 1.7 and 8.2 GPa. The two phase transitions are first-order phase transitions, and the volume collapse rates reach 3.73% and 2.54%, respectively. This suggests that RbNO₃ at low pressure may have new low-temperature phases those are different from P3₁ structure given by experiment in the room temperature and ambient pressure. In the energy-stabilized pressure interval, all three phases satisfy the “Born-Huangkun” elastic stability criteria, and there is no phonon virtual frequency phenomenon in the whole Brillouin zone, which indicates that they are dynamically stable structures. The electronic property analysis showed that the three phases are semiconductors, and the band gap changes caused by the phase transition are generally small, but the pressure generally inhibits the charge transfer from alkali metal ions to nitrate ions. The high-pressure phase transition sequence predicted in this paper and the elasticity, lattice dynamics, and electronic structure properties of the individual phases can provide a reference for subsequent experimental and theoretical studies.
- high pressure /
- phase transition /
- first principles /
- RbNO₃

HTML全文

功能梯度材料(Functionally Graded Materials, FGM)的概念是1984年在航空飞机计划中首次提出的^[1]，FGM的特性在于它的组成和结构随着体积的变化而变化，从而导致材料相应性质发生改变。因其材料特性呈幂律分布^[2-3]，FGM被广泛应用于工程领域，如航空航天、机械工程、生物医学等。圆柱壳在联合荷载作用下的屈曲分析备受学术界关注^[4-5]。目前，对FGM板壳的研究较为深入^[6-8]。Beni等^[9]利用改进的偶应力理论，对FGM圆柱壳在不同边界条件下的动力屈曲进行了分析；Kargarnovin等^[10]研究了轴向荷载作用下FGM圆柱壳的动力屈曲；Sofiyev等^[11]研究了横向压力下功能梯度正交各向异性圆柱壳的动力屈曲，推导出基于一阶剪切变形理论的功能梯度正交各向异性圆柱壳的稳定性和相容性方程；Khazaeinejad等^[12]研究了弹性模量在厚度方向上连续变化的FGM圆柱壳在复合外压和轴向压缩载荷作用下的动力屈曲；Khalili等^[13]研究了横向冲击载荷作用下FGM圆柱壳的动力屈曲；Alashti等^[14]对变厚度FGM圆柱壳外压和轴向压缩的动力屈曲问题进行了分析。

基于以上研究，本研究讨论了FGM圆柱壳在轴向荷载作用下的动力屈曲。根据Donnell壳体理论和经典板壳理论，利用Hamilton变分原理得到FGM圆柱壳的动力屈曲控制方程；采用分离变量法求得动力屈曲临界荷载表达式；通过MATLAB软件计算动力屈曲临界荷载，讨论由不同材料(陶瓷和钛、陶瓷和铁、陶瓷和铜)组成的FGM圆柱壳的径厚比(R/h)、梯度指数(k)、环向模态数(m)、轴向模态数(n)等对临界荷载的影响。

1. FGM圆柱壳的屈曲控制方程

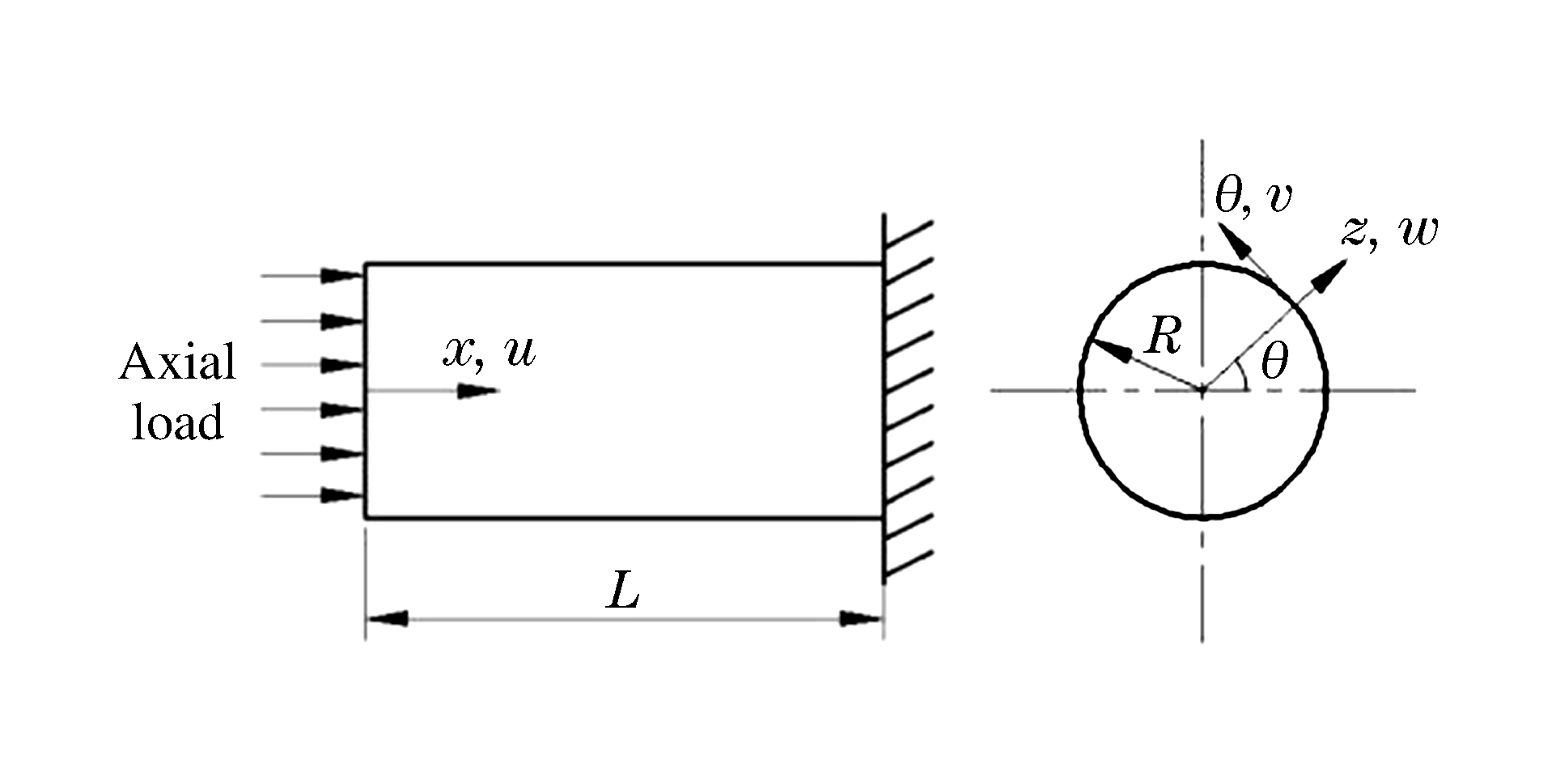
如图 1所示，FGM圆柱壳长度为l，半径为R，总厚度为h，选取柱坐标系(x, θ, z)，其相应位移为(u, v, w)。FGM的材料属性(弹性模量E、密度ρ、泊松比μ等)呈幂律分布^[2-3]，表示为

$P\left( z \right) = \left( {{P_1} - {P_2}} \right){\left( {\frac{{2z + h}}{{2h}}} \right)^k} + {P_2}$

(1)

图 1 圆柱壳坐标系统

Figure 1. Cylindrical shell coordinates

下载: 全尺寸图片幻灯片

式中：P为物性参数，下标“1”和“2”分别代表组分1和组分2；k为梯度指数，k∈(0, ∞)。圆柱壳内任意点的物性参数为

$\left\{ \begin{array}{l} E\left( z \right) = \left( {{E_1} - {E_2}} \right){\left( {\frac{{2z + h}}{{2h}}} \right)^k} + {E_2}\\ \rho \left( z \right) = \left( {{\rho _1} - {\rho _2}} \right){\left( {\frac{{2z + h}}{{2h}}} \right)^k} + {\rho _2}\\ \mu \left( z \right) = \left( {{\mu _1} - {\mu _2}} \right){\left( {\frac{{2z + h}}{{2h}}} \right)^k} + {\mu _2} \end{array} \right.$

(2)

根据Donnell壳体理论，圆柱壳的小挠度几何方程为

$\begin{array}{*{20}{c}} {\left\{ \begin{array}{l} {\varepsilon _x} = \varepsilon _x^0 + z{K_x}\\ {\varepsilon _\theta } = \varepsilon _\theta ^0 + z{K_\theta }\\ {\gamma _{x\theta }} = \gamma _{x\theta }^0 + z{K_{x\theta }} \end{array} \right.,}&{\left\{ \begin{array}{l} u = {u_0} - z\frac{{\partial w}}{{\partial x}}\\ v = {v_0} - z\frac{{\partial w}}{{R\partial \theta }}\\ w = {w_0} \end{array} \right.,}&{\left\{ \begin{array}{l} \varepsilon _x^0 = \frac{{\partial {u_0}}}{{\partial x}}\\ \varepsilon _\theta ^0 = \frac{{\partial {v_0}}}{{R\partial \theta }} - \frac{{{w_0}}}{R}\\ \gamma _{x\theta }^0 = \frac{{\partial {u_0}}}{{R\partial \theta }} - \frac{{\partial {v_0}}}{{\partial x}} \end{array} \right.,}&{\left\{ \begin{array}{l} {K_x} = - \frac{{{\partial ^2}{w_0}}}{{\partial {x^2}}}\\ {K_\theta }{\rm{ = }} - \frac{{{\partial ^2}{w_0}}}{{{R^2}\partial {\theta ^2}}}\\ {K_{x\theta }}{\rm{ = }} - \frac{{2{\partial ^2}{w_0}}}{{R\partial x\partial \theta }} \end{array} \right.} \end{array}$

(3)

式中：ε为正应变，γ为切应变，上、下标“0”表示壳体中面，K为壳体曲率。

根据经典板壳理论，FGM圆柱壳的内力N与内力矩M可表示为

$\left( {\begin{array}{*{20}{c}} {{N_x}}\\ {{N_\theta }}\\ {{N_{x\theta }}}\\ {{M_x}}\\ {{M_\theta }}\\ {{M_{x\theta }}} \end{array}} \right) = \left( {\begin{array}{*{20}{c}} {{A_{11}}}&{{A_{12}}}&{{A_{16}}}&{{B_{11}}}&{{B_{12}}}&{{B_{16}}}\\ {{A_{21}}}&{{A_{22}}}&{{A_{26}}}&{{B_{21}}}&{{B_{22}}}&{{B_{26}}}\\ {{A_{16}}}&{{A_{26}}}&{{A_{66}}}&{{B_{16}}}&{{B_{26}}}&{{B_{66}}}\\ {{B_{11}}}&{{B_{12}}}&{{B_{16}}}&{{D_{11}}}&{{D_{12}}}&{{D_{16}}}\\ {{B_{21}}}&{{B_{22}}}&{{B_{26}}}&{{D_{21}}}&{{D_{22}}}&{{D_{26}}}\\ {{B_{16}}}&{{B_{26}}}&{{B_{66}}}&{{D_{16}}}&{{D_{26}}}&{{D_{66}}} \end{array}} \right)\left( {\begin{array}{*{20}{c}} {\varepsilon _x^0}\\ {\varepsilon _\theta ^0}\\ {\gamma _{x\theta }^0}\\ {{K_x}}\\ {{K_\theta }}\\ {{K_{x\theta }}} \end{array}} \right)$

(4)

式中：A_ij、B_ij、D_ij(i, j=1, 2, 6)分别为FGM圆柱壳的拉伸刚度、耦合刚度和弯曲刚度系数矩阵分量。 ${A_{11}} = {A_{22}} = \int_{ - h/2}^{h/2} {\frac{{E\left( z \right)}}{{1 - {\mu ^2}\left( z \right)}}} {\rm{d}}z$ , ${A_{12}} = {A_{21}} = \int_{ - h/2}^{h/2} {\frac{{\mu \left( z \right)E\left( z \right)}}{{1 - {\mu ^2}\left( z \right)}}} {\rm{d}}z$ , ${A_{66}} = \int_{ - h/2}^{h/2} {\frac{{E\left( z \right)}}{{2\left[ {1 + \mu \left( z \right)} \right]}}} {\rm{d}}z$ , ${B_{11}} = {B_{22}} = \int_{ - h/2}^{h/2} {\frac{{E\left( z \right)}}{{1 - {\mu ^2}\left( z \right)}}z\;}{\rm{d}}z$ , ${B_{12}} = {B_{21}} = \int_{ - h/2}^{h/2} {\frac{{\mu \left( z \right)E\left( z \right)}}{{1 - {\mu ^2}\left( z \right)}}z\;} {\rm{d}}z$ , ${B_{66}} = \int_{ - h/2}^{h/2} {\frac{{E\left( z \right)}}{{2\left[ {1 + \mu \left( z \right)} \right]}}z\;} {\rm{d}}z$ , ${D_{11}} = {D_{22}} = \int_{ - h/2}^{h/2} {\frac{{E\left( z \right)}}{{1 - {\mu ^2}\left( z \right)}}{z^2}\;} {\rm{d}}z$ , ${D_{12}} = {D_{21}} = \int_{ - h/2}^{h/2} {\frac{{\mu \left( z \right)E\left( z \right)}}{{1 - {\mu ^2}\left( z \right)}}{z^2}\;} {\rm{d}}z$ , ${D_{66}} = \int_{ - h/2}^{h/2} {\frac{{E\left( z \right)}}{{2\left[ {1 + \mu \left( z \right)} \right]}}{z^2}\;} {\rm{d}}z$ 。FGM圆柱壳的力学性能为各向同性^[15]，那么：A₁₆=A₂₆=B₁₆=B₂₆=D₁₆=D₂₆=0。

对于圆柱壳，系统的应变能(不考虑剪力)为

$U = \frac{1}{2}\int_0^{2{\rm{ \mathsf{ π} }}} {\int_0^l {\left( {{N_x}\varepsilon _x^0 + {N_\theta }\varepsilon _\theta ^0 + {N_{x\theta }}\gamma _{x\theta }^0 + {M_x}{K_x} + {M_\theta }{K_\theta } + {M_{x\theta }}{K_{x\theta }}} \right)} R{\rm{d}}x{\rm{d}}\theta }$

(5)

动能为

$T = \frac{1}{2}\int_{ - h/2}^{h/2} {\int_0^{2{\rm{ \mathsf{ π} }}} {\int_0^l {\rho \left( z \right)\left[ {{{\left( {\frac{{\partial u}}{{\partial t}}} \right)}^2} + {{\left( {\frac{{\partial v}}{{\partial t}}} \right)}^2} + {{\left( {\frac{{\partial w}}{{\partial t}}} \right)}^2}} \right]R{\rm{d}}x{\rm{d}}\theta {\rm{d}}z} } }$

(6)

外力功为

$W = \frac{1}{2}\int_0^{2{\rm{ \mathsf{ π} }}} {\int_0^l {N\left( t \right){{\left( {\frac{{\partial {w_0}}}{{\partial x}}} \right)}^2}} R{\rm{d}}x{\rm{d}}\theta }$

(7)

Hamilton变分原理为

${\rm{ \mathsf{ δ} }}\int_{{t_2}}^{{t_1}} {\left( {T - U + W} \right){\rm{d}}t} = 0$

(8)

将(3)式~(7)式代入(8)式中，由Donnell壳体理论可知，圆柱壳内力沿环向均匀分布，忽略中面位移^[16]，由u₀、v₀、w₀的变分系数为零，整理得到FGM圆柱壳的动力屈曲控制方程为

$\begin{array}{l} 4{I_0}\frac{{{\partial ^2}{w_0}}}{{\partial t}} - {I_2}\left( {\frac{{{\partial ^4}{w_0}}}{{{R^2}\partial {\theta ^2}\partial {t^2}}} + \frac{{{\partial ^4}{w_0}}}{{\partial {x^2}\partial {t^2}}}} \right) = - 4{A_{22}}\frac{{{w_0}}}{{{R^2}}} - 4\frac{{{B_{12}}}}{{{R^2}}}\frac{{{\partial ^2}{w_0}}}{{\partial {x^2}}} - 4\frac{{{B_{22}}}}{R}\frac{{{\partial ^2}{w_0}}}{{{R^2}\partial {\theta ^2}}} - {D_{11}}\frac{{{\partial ^4}{w_0}}}{{\partial {x^4}}} - \\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;2\left( {{D_{12}} + 2{D_{66}}} \right)\frac{{{\partial ^4}{w_0}}}{{{R^2}\partial {x^2}\partial {\theta ^2}}} - {D_{22}}\frac{{{\partial ^4}{w_0}}}{{{R^4}\partial {\theta ^4}}} + N\left( t \right)\frac{{{\partial ^2}{w_0}}}{{\partial {x^2}}} \end{array}$

(9)

2. 控制方程的求解

设径向位移表示为^[17]

$w = Y\left( x \right)T\left( t \right){{\rm{e}}^{{\rm{i}}m\theta }}$

(10)

将(10)式代入(9)式中，分离变量得

$\left\{ \begin{array}{l} {Y^{\left( 4 \right)}} = {\alpha ^2}Y'' + {\beta ^2}Y = 0\\ \ddot T - \lambda T = 0 \end{array} \right.$

(11)

其中

${\alpha ^2} = \frac{1}{{{D_{11}}}}\left[ {4\frac{{{B_{12}}}}{{{R^2}}} + \frac{2}{{{R^2}}}\left( {{D_{12}} + 2{D_{66}}} \right){m^2} - N\left( t \right) - {I_2}\lambda } \right]$

(12)

${\beta ^2} = \frac{1}{{{D_{11}}}}\left[ {4\frac{{{A_{22}}}}{{{R^2}}} + 4\frac{{{B_{22}}}}{{{R^3}}} + \frac{{{D_{22}}}}{{{R^4}}}{m^4} + 4{I_0}\lambda - \frac{{{I_2}}}{{{R^2}}}{m^2}\lambda } \right]$

(13)

当α⁴＞4β²＞0且λ＞0时，圆柱壳屈曲^[18-20]，其动力屈曲解为

$Y\left( x \right) = {C_1}\sin \left( {{k_1}x} \right) + {C_2}\cos \left( {{k_1}x} \right) + {C_3}\sin \left( {{k_2}x} \right) + {C_4}\cos \left( {{k_2}x} \right)$

(14)

式中：C₁~C₄为系数， ${k_1} = \sqrt {\frac{{{\alpha ^2} - \sqrt {{\alpha ^4} - 4{\beta ^2}} }}{2}} , {k_2} = \sqrt {\frac{{{\alpha ^2} + \sqrt {{\alpha ^4} - 4{\beta ^2}} }}{2}}$ 。

(14)式满足下列两种边界条件：

(1) 对于一端夹支另一端固支的圆柱壳，其边界条件为

$\left\{ \begin{array}{l} Y\left( 0 \right) = Y'\left( 0 \right) = 0\\ Y\left( l \right) = Y'\left( l \right) = 0 \end{array} \right.$

(15)

(2) 对于一端简支另一端固支的圆柱壳，其边界条件为

$\left\{ \begin{array}{l} Y\left( 0 \right) = Y''\left( 0 \right) = 0\\ Y\left( l \right) = Y'\left( l \right) = 0 \end{array} \right.$

(16)

将(14)式代入(15)式中，整理得到如下齐次线性方程组

$\left( {\begin{array}{*{20}{c}} 0&1&0&1\\ {{k_1}}&0&{{k_2}}&0\\ {\sin \left( {{k_1}l} \right)}&{\cos \left( {{k_1}l} \right)}&{\sin \left( {{k_2}l} \right)}&{\cos \left( {{k_2}l} \right)}\\ {{k_1}\cos \left( {{k_1}l} \right)}&{ - {k_1}\sin \left( {{k_1}l} \right)}&{{k_2}\cos \left( {{k_2}l} \right)}&{ - {k_2}\sin \left( {{k_2}l} \right)} \end{array}} \right)\left( {\begin{array}{*{20}{c}} {{C_1}}\\ {{C_2}}\\ {{C_3}}\\ {{C_4}} \end{array}} \right) = 0$

(17)

若要(17)式有非平凡解，其系数行列式必为零，于是

$2{k_1}{k_2} - 2{k_1}{k_2}\cos \left( {{k_1}l} \right)\cos \left( {{k_2}l} \right) - \left( {k_1^2 + k_2^2} \right)\sin \left( {{k_1}l} \right)\sin \left( {{k_2}l} \right) = 0$

(18)

由k₁和k₂可得

$\left\{ \begin{array}{l} k_1^2 + k_2^2 = \left( {n_1^2 + n_2^2} \right){{\rm{ \mathsf{ π} }}^2}/{l^2} = {\alpha ^2}\\ k_1^2k_2^2 = n_1^2n_2^2{{\rm{ \mathsf{ π} }}^4}/{l^4} = {\beta ^2} \end{array} \right.$

(19)

将(19)式代入(12)式和(13)式中，得一端夹支另一端固支时FGM圆柱壳动力屈曲临界荷载N_cr，即

${N_{{\rm{cr}}}} = \frac{{{D_{11}}{{\rm{ \mathsf{ π} }}^2}\left( {n_1^2 + n_2^2} \right)}}{{{l^2}}} - \frac{{{B_{12}}}}{{{R^2}}} + \frac{{2\left( {{D_{12}} + 2{D_{66}}} \right){m^2}}}{{{R^2}}} + \frac{{{h^2}\left( {{D_{11}}n_1^2n_2^2{{\rm{ \mathsf{ π} }}^4}{R^4} - {A_{22}}{l^4}{R^2} + 4{B_{22}}{m^2}{R^2}{l^4} - {D_{22}}{l^4}{m^4}} \right)}}{{{l^4}{R^2}\left( {{h^2}{m^2} - 12{R^2}} \right)}}$

(20)

式中：n₁=n=1, 2, 3, …；m=1, 2, 3, …；n₂=n+2。

同理可得一端简支另一端固支时FGM圆柱壳动力屈曲临界荷载

(21)

此时，n₁=n=1, 2, 3, …；m=1, 2, 3, …；n₂=n+1。

将FGM退化成金属材料，得到金属材料圆柱壳动力屈曲临界荷载

${N_{{\rm{cr}}}} = \frac{{{D_{11}}{{\rm{ \mathsf{ π} }}^2}\left( {n_1^2 + n_2^2} \right)}}{{{l^2}}} + \frac{{2{D_{12}}{m^2}}}{{{R^2}}} + \frac{{{h^2}\left( {{D_{11}}n_1^2n_2^2{{\rm{ \mathsf{ π} }}^4}{R^4} - {A_{22}}{l^4}{R^2} - {D_{22}}{l^4}{m^4}} \right)}}{{{l^4}{R^2}\left( {{h^2}{m^2} - 12{R^2}} \right)}}$

(22)

(22)式与文献[16]中的表达式相同。

根据(10)式，取一端夹支另一端固支时圆柱壳动力屈曲解的表达式^[16]

$w = T\left( t \right)\left[ {\sin \left( {\frac{{{n_1}{\rm{ \mathsf{ π} }}x}}{l}} \right) - \frac{{{n_1}}}{{{n_2}}}\sin \left( {\frac{{{n_2}{\rm{ \mathsf{ π} }}x}}{l}} \right)} \right]\sin \left( {m\theta } \right)$

(23)

将(23)式代入控制方程(9)式中，计算并化简整理得到临界荷载表达式

${N_{{\rm{cr}}}} = \frac{{\left( {n_1^2 + n_2^2} \right){D_{11}}{{\rm{ \mathsf{ π} }}^2}}}{{{l^2}}} + \frac{{2{m^2}\left( {{D_{12}} + 2{D_{66}}} \right)}}{{{R^2}}}$

(24)

(24)式与不考虑转动惯量时用分离变量得到的结果相同，此时n₁=n=1, 2, 3, …; m=1, 2, 3, …; n₂=n+2。同理可得当边界条件为一端简支另一端固支时的临界荷载表达式，与(24)式相同，此时n₁=n=1, 2, 3, …; m=1, 2, 3, …; n₂=n+1。

3. 算例分析

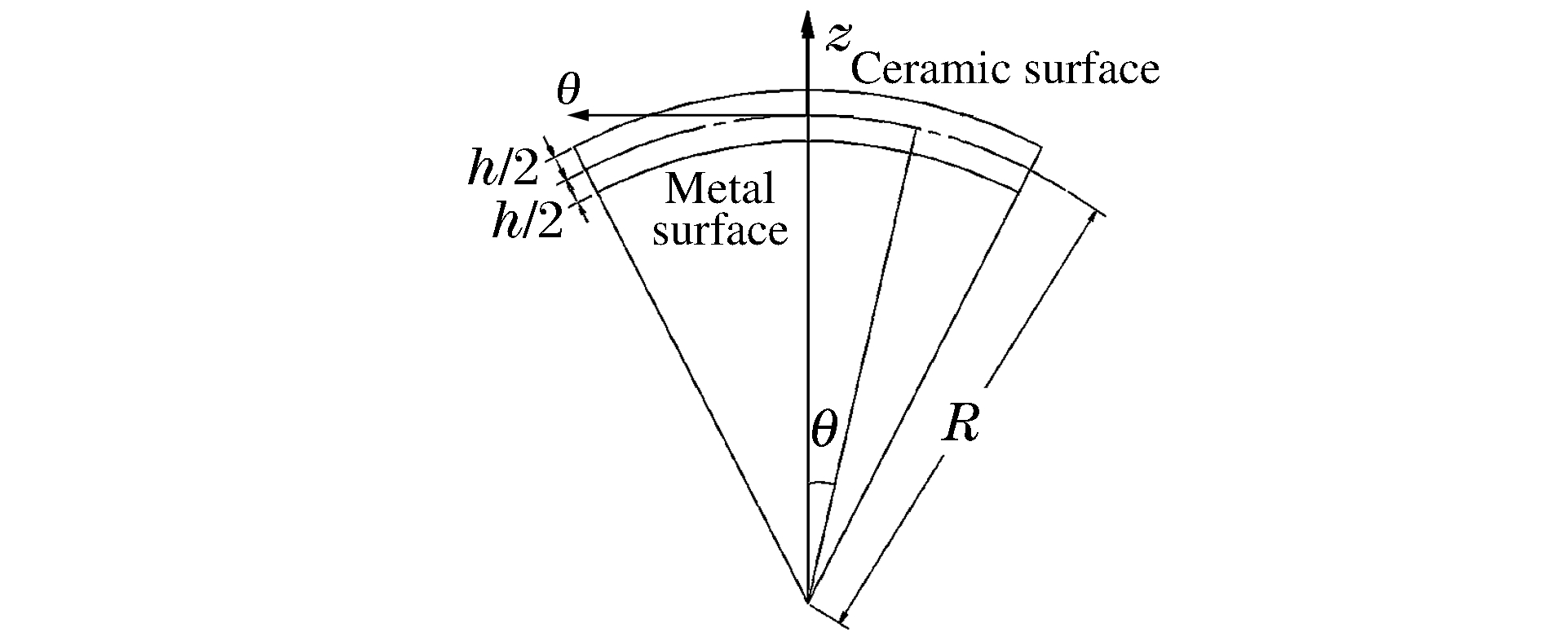
采用MATLAB软件编程，对FGM圆柱壳动力屈曲临界荷载进行计算。讨论由不同材料(陶瓷-钛、陶瓷-铁、陶瓷-铜)组成的FGM圆柱壳(见图 2)的径厚比(R/h)、梯度指数(k)、环向模态数(m)、轴向模态数(n)对临界荷载N_cr的影响。基本材料参数如表 1所示。

图 2 材料沿壁厚分布

Figure 2. Distribution of material along wall thickness

下载: 全尺寸图片幻灯片

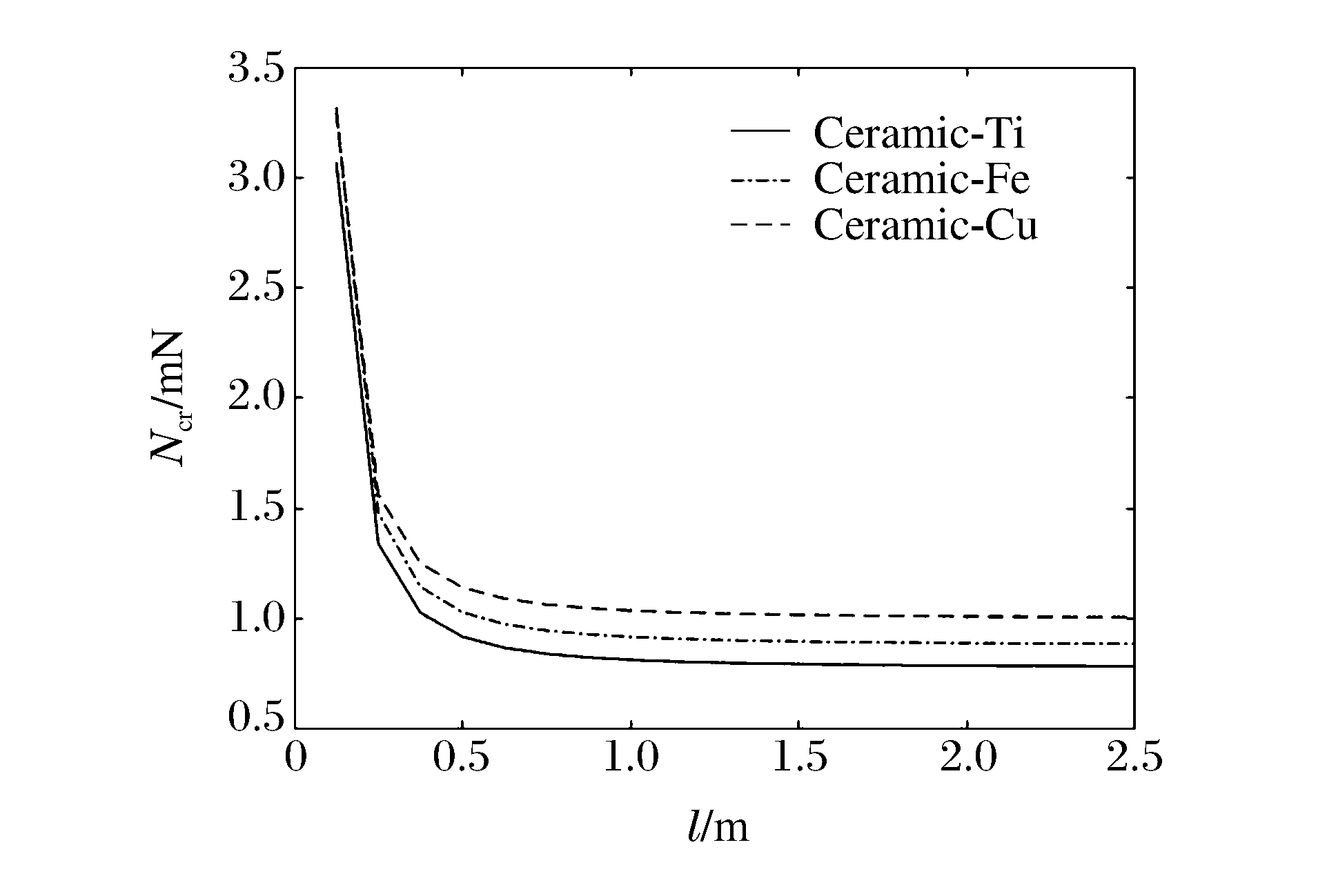
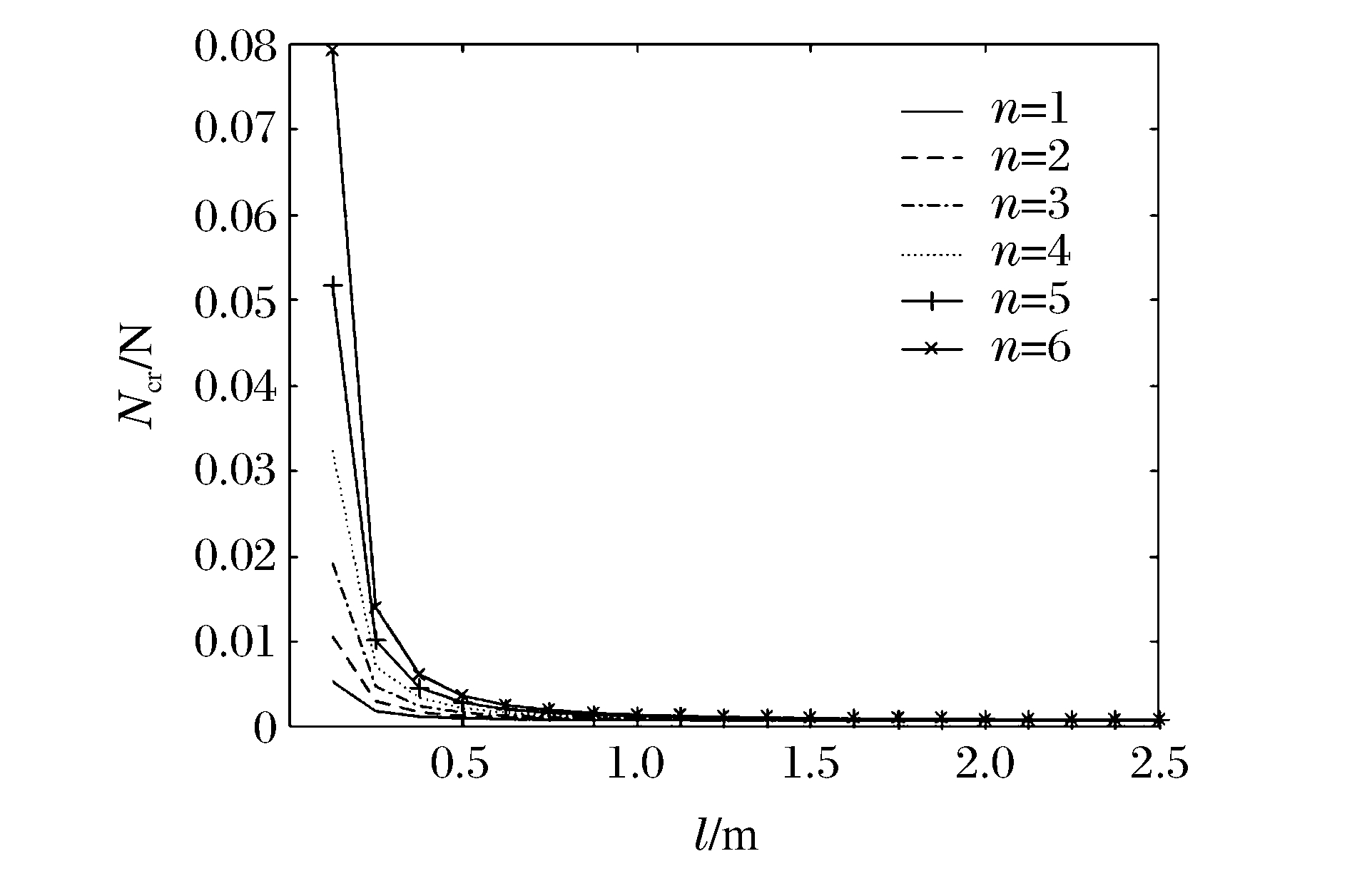
表 1 材料参数

Table 1. Material parameters

Material	E/GPa	ρ/(g·cm^-3)	μ
Ceramic	385	3.96	0.230
Ti	109	4.54	0.410
Fe	155	7.86	0.291
Cu	119	8.96	0.326

下载: 导出CSV

| 显示表格

图 3表示n=1、m=2、k=1、R/h=20时，不同材料组成下N_cr与临界长度l(本研究中临界长度即为圆柱壳长度)的关系曲线。从图 3可以看出：N_cr随l的增加而减小；当l＜0.5 m时，N_cr随l的增加而迅速减小；当l＞0.5 m时，N_cr随l的增大缓慢减小，且逐渐趋于常数；同一l下，陶瓷-铜的N_cr最大，陶瓷-铁次之，陶瓷-钛的N_cr最小。以下均以陶瓷-钛为例进行讨论。

图 3 不同材料组成下临界荷载与临界长度的关系

Figure 3. Critical load vs.critical length under different material composition conditions

下载: 全尺寸图片幻灯片

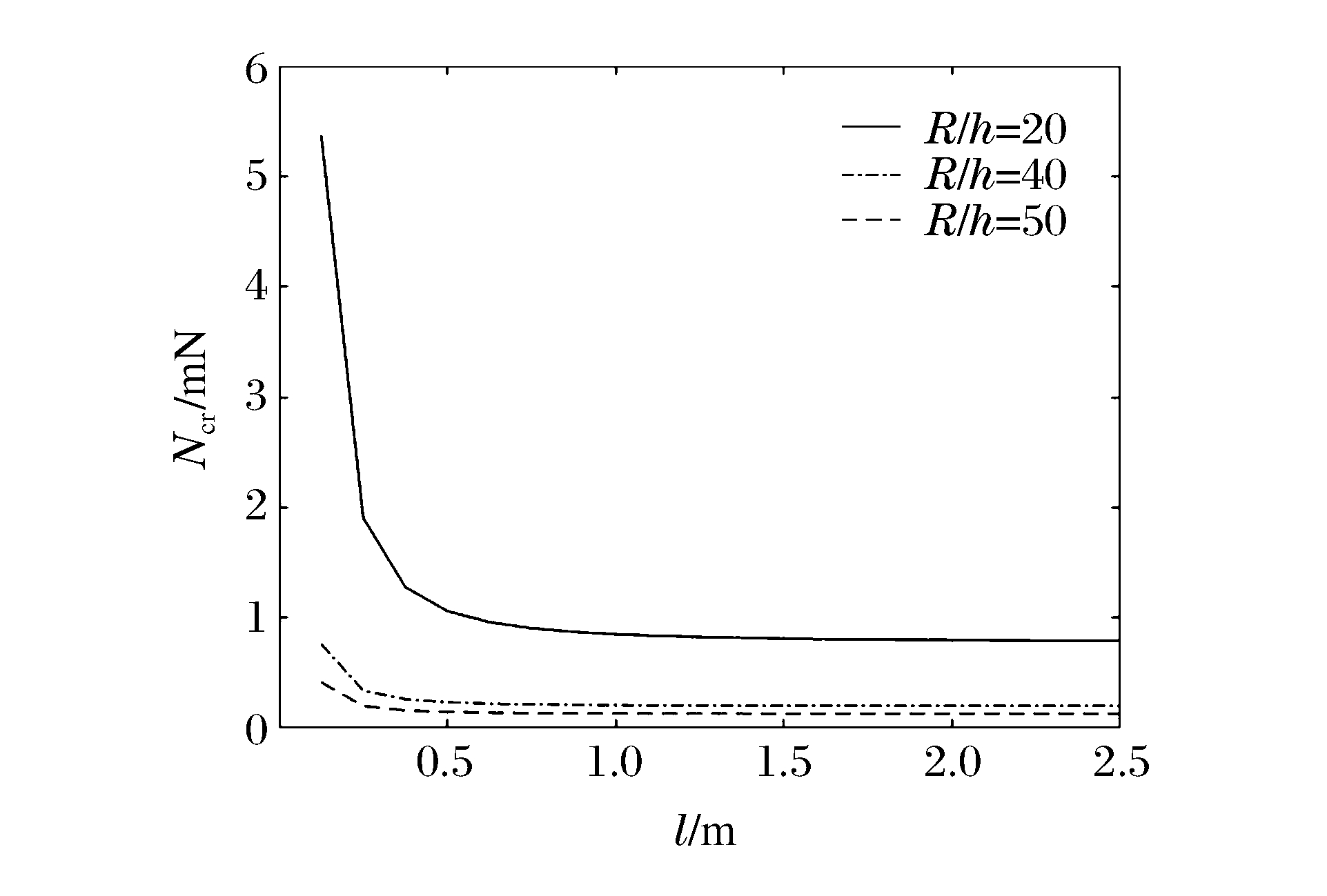
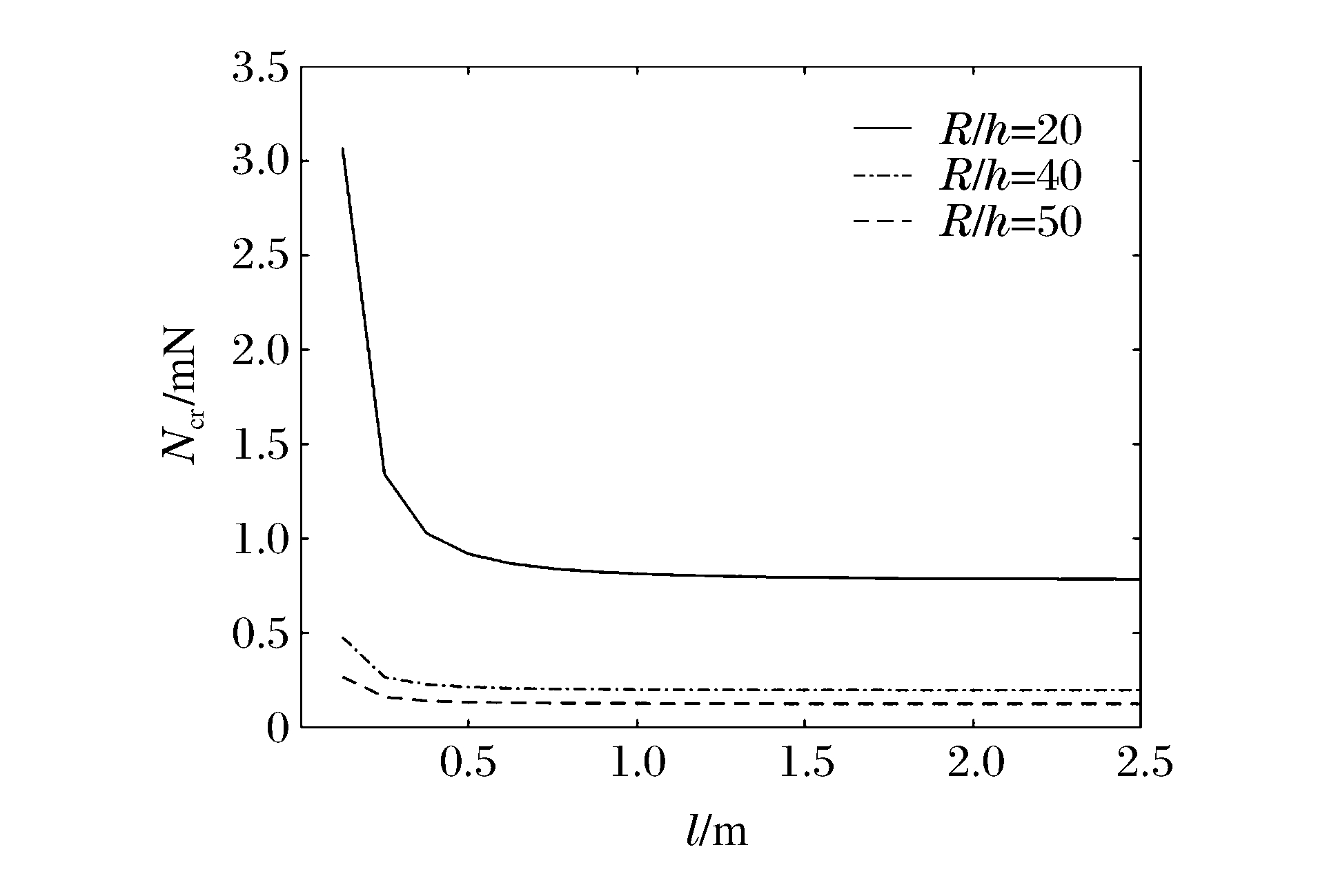
图 4和图 5分别表示冲击端为夹支和简支时n=1、m=2、k=1时不同R/h下N_cr与l的关系曲线。可以看出：当l增加时，N_cr减小，且逐渐趋于常数；在同一l下圆柱壳的N_cr随着R/h的增大而减小；当R/h和l一定时，冲击端为夹支时的N_cr明显比冲击端为简支时的N_cr大，说明约束条件对N_cr有较大影响。

图 4 冲击端为夹支时不同径厚比下临界荷载与临界长度的关系

Figure 4. Critical load vs.critical length under clamped edge and different diameter-thickness ratios conditions

下载: 全尺寸图片幻灯片

图 5 冲击端为简支时不同径厚比下临界荷载与临界长度的关系

Figure 5. Critical load vs.critical length under simple support and different diameter-thickness ratios conditions

下载: 全尺寸图片幻灯片

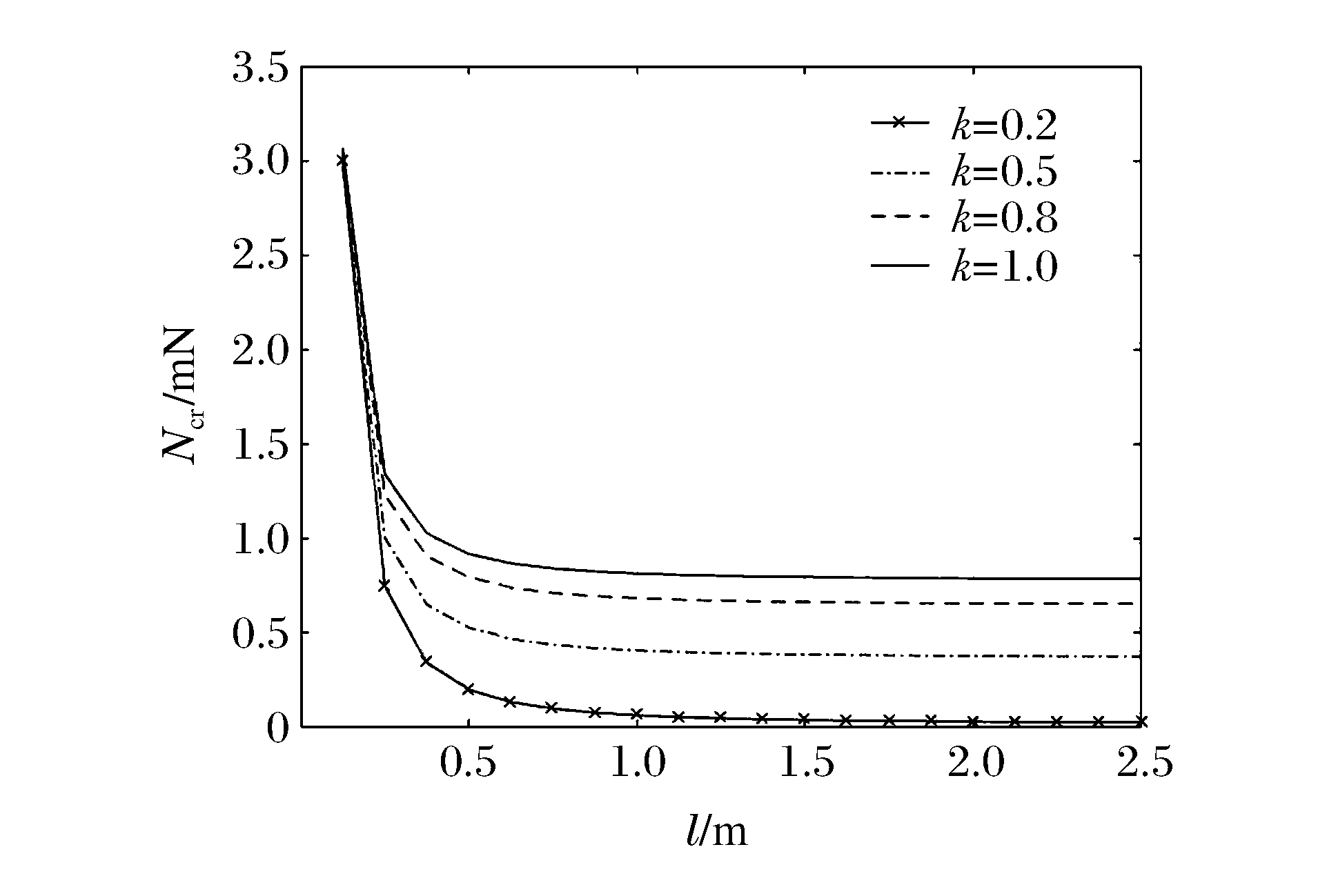
图 6和图 7分别表示冲击端为夹支和简支条件下n=1、m=2、R/h=20时不同k下N_cr与l的关系曲线。可见：FGM圆柱壳的N_cr随着l的增加而减小；在同一l下，FGM圆柱壳的N_cr随着k的增加而增加；当l＜0.5 m时，N_cr随l的增加迅速减小，当l＞0.5 m时，N_cr随l的增加缓慢减小并逐渐趋于常数；当k=1且l一定时，冲击端为夹支时的N_cr明显比冲击端为简支时的N_cr大，再次说明约束条件对N_cr的影响较大。

图 6 冲击端为夹支时不同梯度指数下临界荷载与临界长度的关系

Figure 6. Critical load vs.critical length under clamped edge and different gradient indexes conditions

下载: 全尺寸图片幻灯片

图 7 冲击端为简支时不同梯度指数下临界荷载与临界长度的关系

Figure 7. Critical load vs.critical length under simple support and different gradient indexes conditions

下载: 全尺寸图片幻灯片

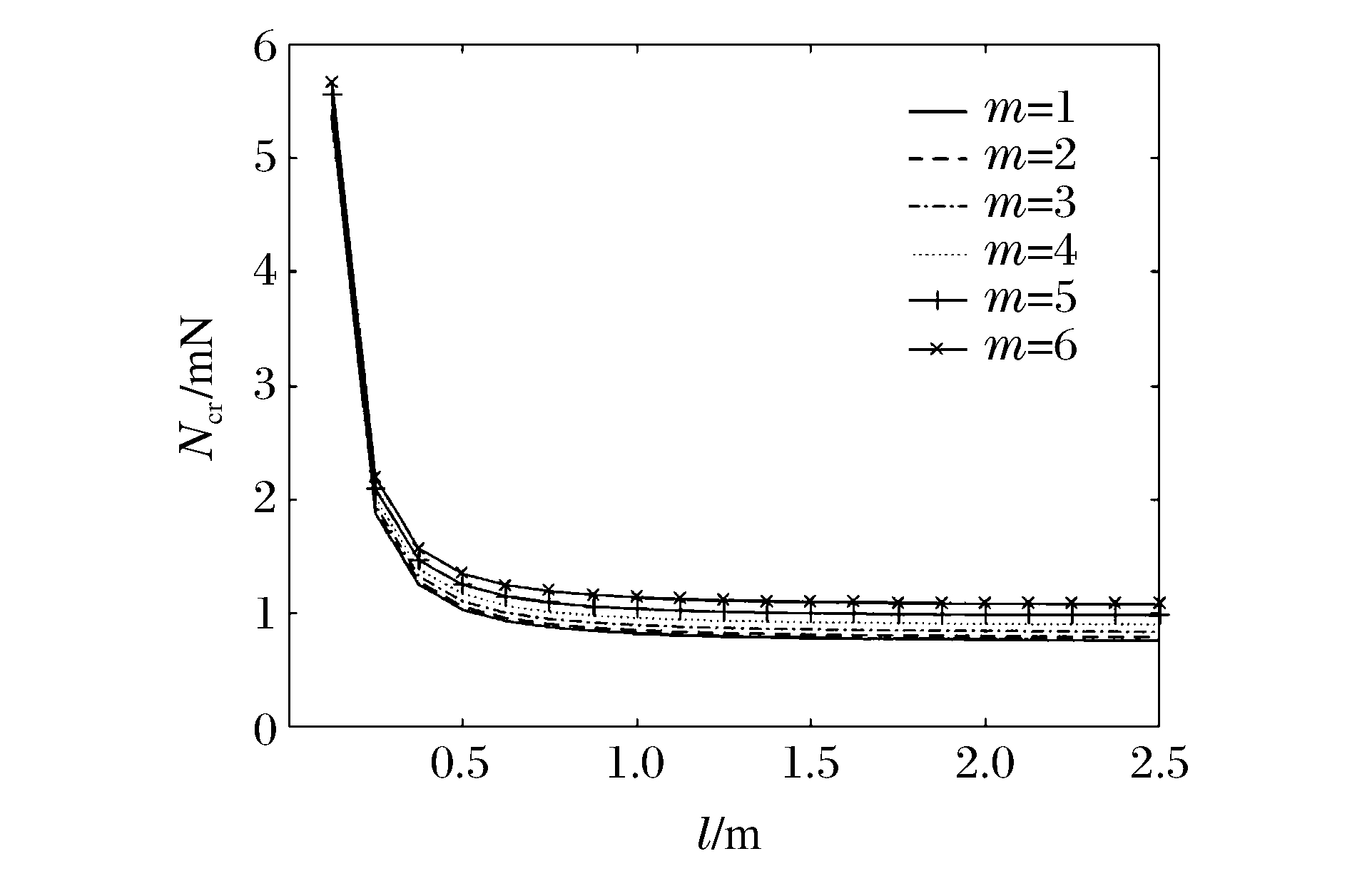
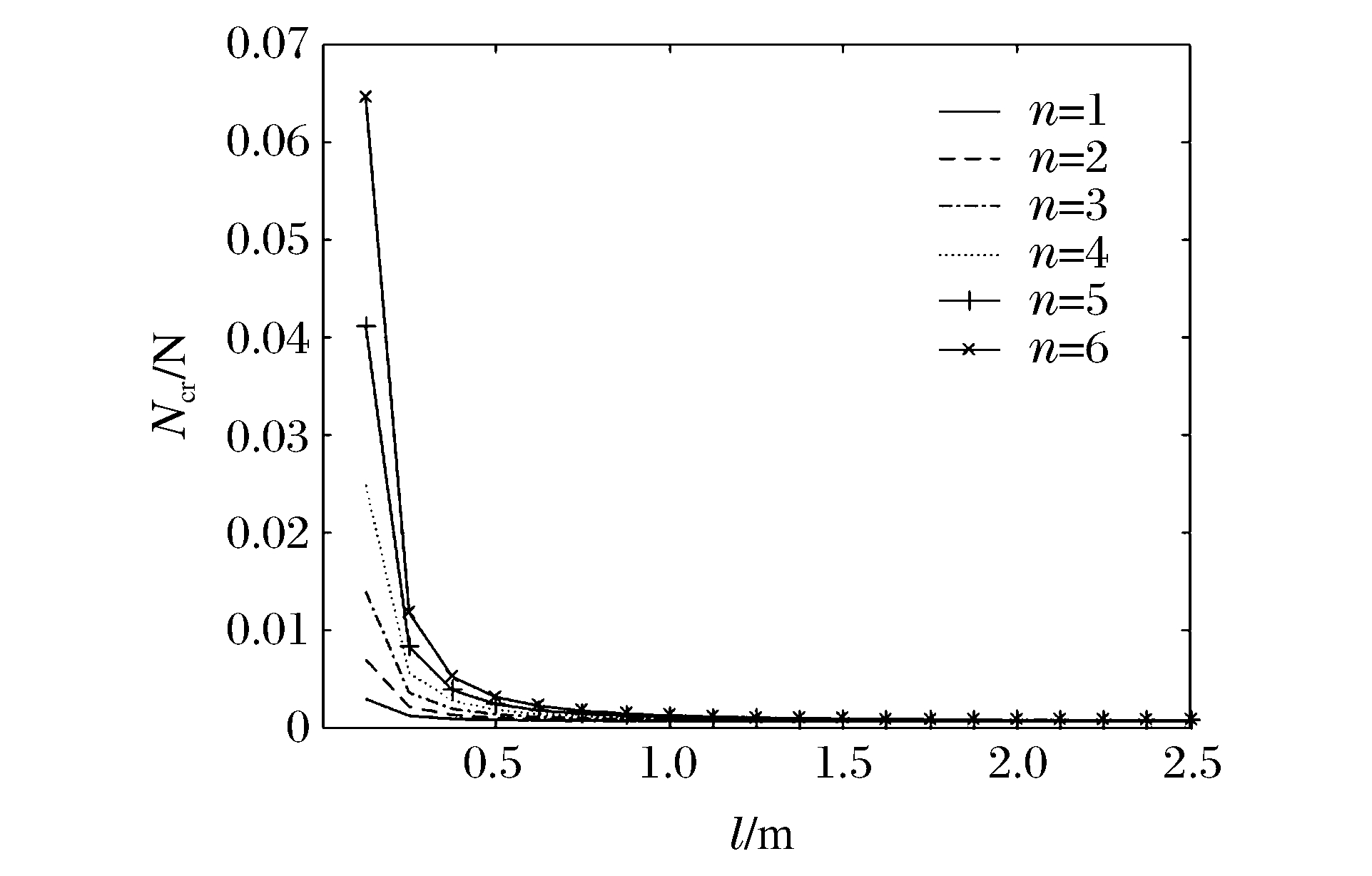
图 8和图 9分别表示冲击端为夹支和简支，n=1、k=1、R/h=20时不同m下N_cr与l的关系曲线。可以看出：当l在一定范围内时，N_cr随l的增加而迅速减小，超出这一范围后N_cr随l的增加而缓慢减小且逐渐趋于常数；同一l下，随着m的增大，FGM圆柱壳的N_cr增大，表明N_cr越大，高阶模态越易被激发。当m=6且l一定时，冲击端为夹支时的N_cr明显比冲击端为简支时的N_cr大，表明约束条件对N_cr有较大影响。

图 8 冲击端为夹支时不同环向模态数下临界荷载与临界长度的关系

Figure 8. Critical load vs.critical length under clamped edge and different circumferential modal number conditions

下载: 全尺寸图片幻灯片

图 9 冲击端为简支时不同环向模态数下临界荷载与临界长度的关系

Figure 9. Critical load vs.critical length under simple support and different circumferential modal number conditions

下载: 全尺寸图片幻灯片

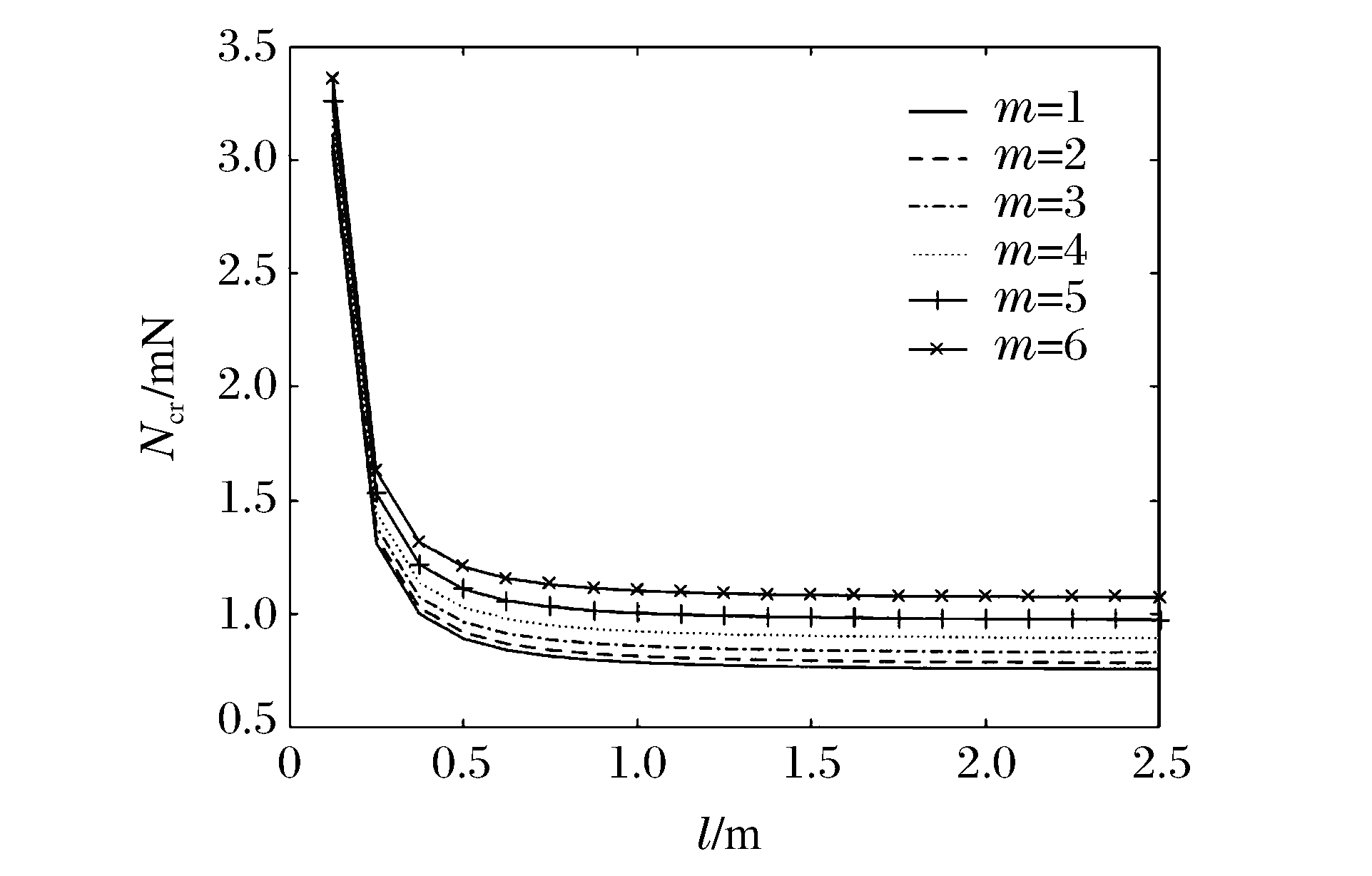
图 10和图 11分别表示冲击端为夹支和简支，m=1、k=1、R/h=20时不同n下N_cr与l的关系曲线。图 10和图 11显示：N_cr随着l的增加而减小；不同n条件下，N_cr随l的增加逐渐趋于同一值；当l＜1 m时，在同一l下N_cr随n的增加而增加，说明N_cr越大，高阶模态越容易被激发。

图 10 冲击端为夹支时不同轴向模态数下临界荷载与临界长度的关系

Figure 10. Critical load vs.critical length under clamped edge and different axial modal number conditions

下载: 全尺寸图片幻灯片

图 11 冲击端为简支时不同轴向模态数下临界荷载与临界长度的关系

Figure 11. Critical load vs.critical length under simple support and different axial modal number conditions

下载: 全尺寸图片幻灯片

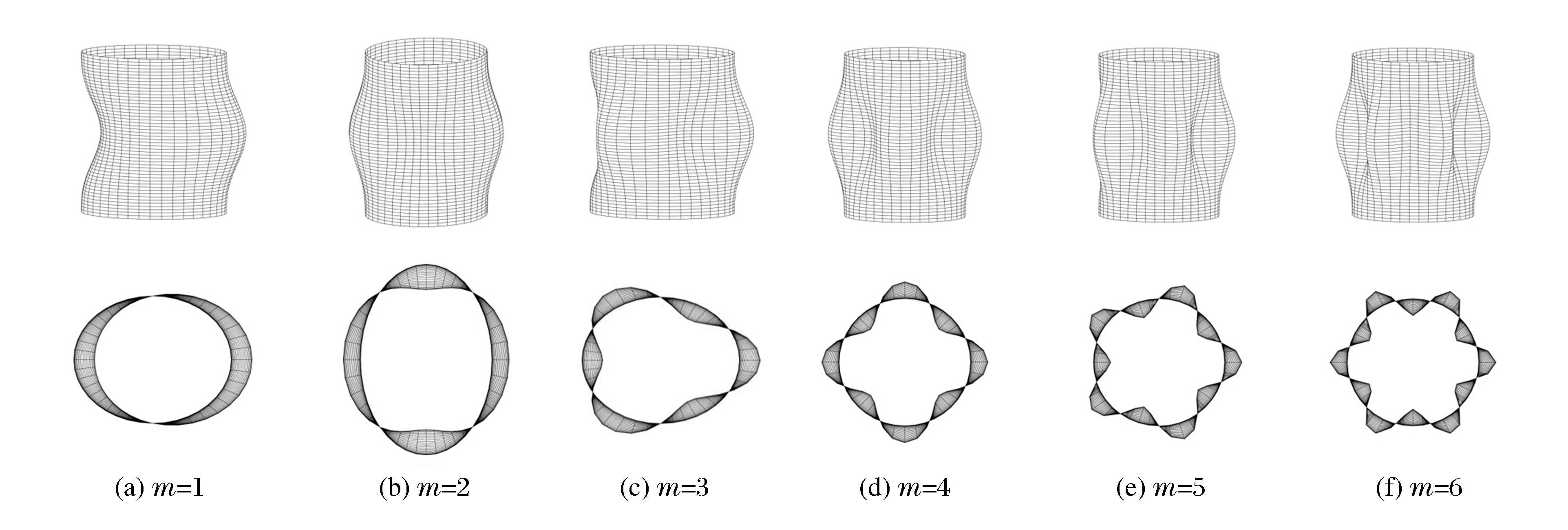
图 12为不同环向模态数m下FGM圆柱壳的动力屈曲模态。可以看出：随着m的增大，圆柱壳的模态变得越来越复杂，俯视图由单一形变为多瓣形；当m=6时，俯视图为12瓣形。

图 12 不同环向屈曲模态图(n=1;m=1, 2, 3, 4, 5, 6)

Figure 12. Different circumferential buckling modes (n=1;m=1, 2, 3, 4, 5, 6)

下载: 全尺寸图片幻灯片

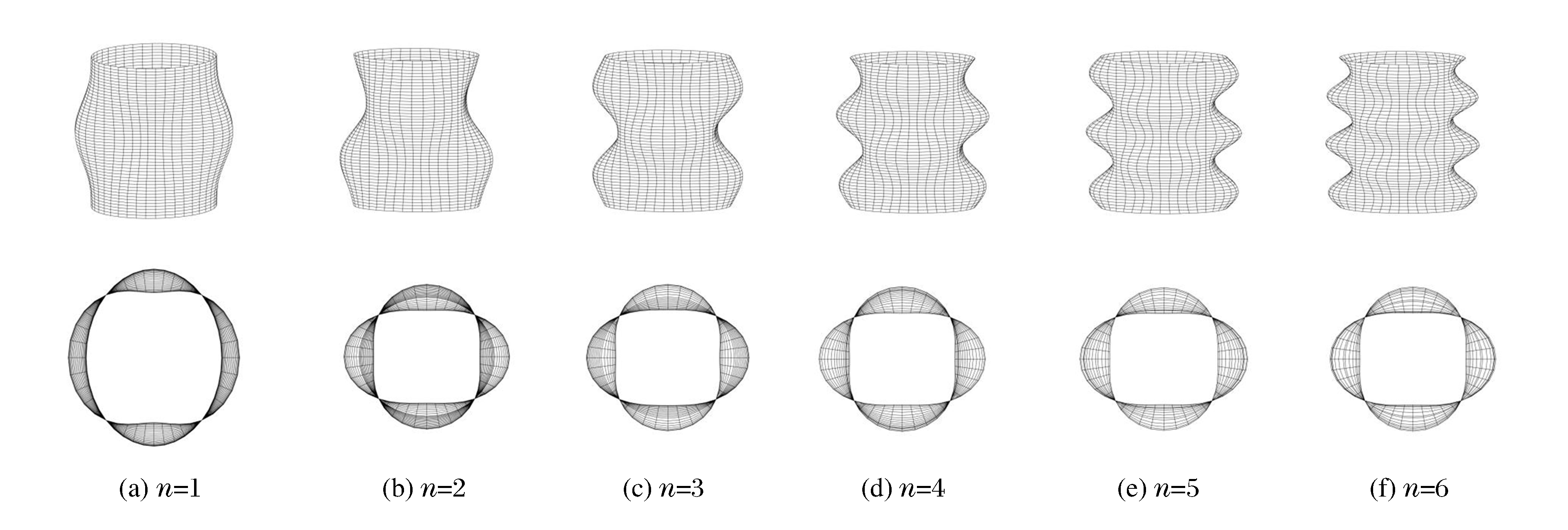
图 13为不同轴向模态数n下FGM圆柱壳的动力屈曲模态图。由图 13可知：随着n的增加，模态图变得越来越复杂。由FGM圆柱壳的俯视图可知，各阶模态数下动力屈曲模态图为轴对称。

图 13 不同轴向屈曲模态图(m=2;n=1, 2, 3, 4, 5, 6)

Figure 13. Different axial buckling modes (m=2;n=1, 2, 3, 4, 5, 6)

下载: 全尺寸图片幻灯片

4. 结论

(1) 根据Donnell壳体理论和经典板壳理论，由Hamilton变分原理得到轴向荷载作用下FGM圆柱壳的动力屈曲控制方程。

(2) 由圆柱壳周向连续性设出径向位移的周向形式，并用分离变量法得到不同约束条件下FGM圆柱壳动力屈曲临界荷载的表达式和屈曲解式。

(3) 利用MATLAB对临界荷载进行计算，得到：在轴向模态数(n)、环向模态数(m)、梯度指数(k)、径厚比(R/h)一定的情况下，同种材料组成的圆柱壳的临界荷载随着临界长度的增加而减小；在n、m、k、l一定的情况下，临界荷载随着径厚比的增大而减小；在n、m、R/h、l一定的情况下，临界荷载随着梯度指数k的增加而增加；不同约束条件下，冲击端为夹支的临界荷载大于冲击端为简支的临界荷载，表明约束条件对临界荷载有较大影响；圆柱壳的临界荷载随模态数的增加而增大，表明临界荷载越大，越容易激发高阶模态；圆柱壳的动力屈曲模态随模态数的增加变得更为复杂。

图 1 R3m、P3₁和Pmmn相相对于Pnma相的热力学焓差曲线

Figure 1. Calculated enthalpy difference of R3m, P3₁ and Pmmn phases relative to Pnma as a function of pressure

下载: 全尺寸图片幻灯片

图 2 R3m、Pnma、P3₁和Pmmn相的体积随压强的变化

Figure 2. Calculated volume of R3m, Pnma, P3₁ and Pmmn phases as a function of pressure

下载: 全尺寸图片幻灯片

图 3 R3m、Pnma和Pmmn相的晶体结构

Figure 3. Crystal structures of R3m, Pnma, and Pmmn phase

下载: 全尺寸图片幻灯片

图 4 R3m、Pnma和Pmmn相的声子色散曲线以及声子态密度

Figure 4. Phonon-dispersion curves and the PHDOS of R3m, Pnma and Pmmn phase

下载: 全尺寸图片幻灯片

图 5 R3m、Pnma和Pmmn相的电子局域函数

Figure 5. Electron localization function of R3m, Pnma and Pmmn phase

下载: 全尺寸图片幻灯片

图 6 R3m、Pnma和Pmmn相的COHP

Figure 6. Crystal orbital Hamilton populations of R3m, Pnma and Pmmn phase

下载: 全尺寸图片幻灯片

图 7 R3m、Pnma和Pmmn相的能带结构和电子态密度

Figure 7. Band structures and partial densities of states of R3m, Pnma and Pmmn phases

下载: 全尺寸图片幻灯片

表 1 RbNO₃-Ⅳ相的晶格常数的理论值与实验值的比较

Table 1. Comparison between experimental and calculated lattice parameters of RbNO₃-Ⅳ phase

Method	Condition	a/Å	c/Å	Volume/Å³
Simulation	PBE	10.68(+1.62%)	7.60(+1.88%)	867.56(+5.29%)
	vdW-DF	10.72(+2.00%)	7.62(+2.14%)	876.29(+6.35%)
	PBEsol	10.39(−1.14%)	7.39(−0.94%)	797.69(−3.19%)
	RPBE	11.14(+5.99%)	7.91(+6.03%)	982.63(+19.26%)
Experiment	T=296 K^[24]	10.47	7.44	815.58
	T=298 K^[25]	10.55	7.47	831.43
	Average	10.51	7.46	823.96

下载: 导出CSV

表 2 高压下R3m、Pnma和Pmmn相的晶格参数和原子位置

Table 2. Lattice parameters and atomic coordinates of R3m, Pnma and Pmmn phases at different pressures

Phase	Pressure/GPa	Lattice parameters	Wyckoff positions
R3m	0	a=b=5.64 Å, c=9.48 Å, α=β=90°, γ=120°	Rb1: 3a(0.3333, 0.6667, 0.1699) N1: 3a(0.3333, 0.6667, 0.7275) O1: 9b(0.5373, 0.0746, 0.3953)
Pnma	4	a=7.18 Å, b=5.61 Å, c=6.77 Å, α=β=γ=90°	Rb1: 4c(0.483, 0.250, 0.686) N1: 4c(0.350, 0.250, 0.122) O1: 4c(0.448, 0.250, 0.275) O5: 8d(0.302, 0.444, 0.043)
Pmmn	12	a=4.67 Å, b=5.34 Å, c=4.63 Å, α=β=γ=90°	Rb1: 2b(0.500, 0, 0.400) N1: 2a(0, 0, 0.992) O1: 2a(0, 0, 0.721) O3: 4e(0, 0.204, 0.128)

下载: 导出CSV

表 3 高压下R3m、Pnma和Pmmn相的弹性常数和弹性模量

Table 3. Elastic constant and elastic moduli of R3m, Pnma and Pmmn phase at high pressure GPa

Phase	Pressure	C₁₁	C₁₂	C₁₃	C₁₄	C₂₂	C₂₃	C₃₃	C₄₄	C₅₅	C₆₆	B	G	E
R3m	0	37	14	11	−4			13	7		12	15	7	19
	4	75	28	21	−8			39	6		23	35	11	29
	12	124	46	42	−15			94	12		39	66	19	52
Pnma	0	22	7	9		41	9	32	7	10	9	16	10	24
	4	50	19	30		73	19	69	12	17	19	36	17	44
	12	96	43	59		129	35	119	19	−5	34	69	−57	−236
Pmmn	0	26	9	9		50	16	54	15	5	5	21	10	26
	4	60	16	25		84	32	83	33	20	9	40	21	53
	12	113	25	53		143	59	129	60	37	16	72	35	91

下载: 导出CSV

表 4 高压下R3m、Pnma和Pmmn相的元胞内的Bader电荷转移

Table 4. Calculated Bader charges within R3m, Pnma and Pmmn phases primitive cells at different pressures

Phase	Pressure/GPa	Atoms	Number	Charge/e	Charge transfer/e
R3m	0	Rb	1	8.10	0.90
		N	1	4.16	0.84
		O	3	6.58	−0.58
Pnma	4	Rb	4	8.13	0.87
		N	4	4.13	0.87
		O1	4	6.57	−0.57
		O2	4	6.58	−0.58
		O3	4	6.59	−0.59
Pmmn	12	Rb	2	8.14	0.86
		N	2	4.07	0.93
		O1	2	6.58	−0.58
		O2	2	6.59	−0.59
		O3	2	6.62	−0.62

下载: 导出CSV

参考文献(30)

[1]	LAUE W, THIEMANN M, SCHEIBLER E, et al. Nitrates and nitrites [J]. Ullmann’s Encyclopedia of Industrial Chemistry, 2000, 24: 153–157.
[2]	MIAO M S, SUN Y H, ZUREK E, et al. Chemistry under high pressure [J]. Nature Reviews Chemistry, 2020, 4(10): 508–527. doi: 10.1038/s41570-020-0213-0
[3]	SANTOS S S M, MARCONDES M L, JUSTO J F, et al. Calcium carbonate at high pressures and high temperatures: a first-principles investigation [J]. Physics of the Earth and Planetary Interiors, 2020, 299: 106327. doi: 10.1016/j.pepi.2019.106327
[4]	LEE W E, GILBERT M, MURPHY S T, et al. Opportunities for advanced ceramics and composites in the nuclear sector [J]. Journal of the American Ceramic Society, 2013, 96(7): 2005–2030. doi: 10.1111/jace.12406
[5]	SOLEIMANPOUR S, SADRAMELI S M, MOUSAVI S A H S, et al. Preparation and characterization of high temperature shape stable NaNO₃/diatomite phase change materials with nanoparticles for solar energy storage applications [J]. Journal of Energy Storage, 2022, 45: 103735. doi: 10.1016/j.est.2021.103735
[6]	DIÉGUEZ O, VANDERBILT D. Theoretical study of ferroelectric potassium nitrate [J]. Physical Review B, 2007, 76(13): 134101. doi: 10.1103/PhysRevB.76.134101
[7]	RAO C N R, PRAKASH B, NATARAJAN M. Crystal structure transformations in inorganic nitrites, nitrates, and carbonates: NSRDS-NBS 53 [R]. Washington, USA: National Bureau of Standards, 1975.
[8]	AHTEE M, HEWAT A W. Structures of the high temperature phases of rubidium nitrate [J]. Physica Status Solidi (A), 1980, 58(2): 525–531. doi: 10.1002/pssa.2210580224
[9]	LIU J J, DUAN C G, OSSOWSKI M M, et al. Molecular dynamics simulation of structural phase transitions in RbNO₃ and CsNO₃ [J]. Journal of Solid State Chemistry, 2001, 160(1): 222–229. doi: 10.1006/jssc.2001.9226
[10]	DEAN C, HAMBLEY T W, SNOW M R. Structures of phase Ⅳ rubidium nitrate, RbNO₃, and phase Ⅱ caesium nitrate, CsNO₃ [J]. Acta Crystallographica Section C, 1984, 40(9): 1512–1515.
[11]	WANG Y C, LV J, ZHU L, et al. Crystal structure prediction via particle-swarm optimization [J]. Physical Review B, 2010, 82(9): 094116. doi: 10.1103/PhysRevB.82.094116
[12]	WANG H, TSE J S, TANAKA K, et al. Superconductive sodalite-like clathrate calcium hydride at high pressures [J]. Proceedings of the National Academy of Sciences of the United States of America, 2012, 109(17): 6463–6466.
[13]	PERDEW J P, BURKE K, ERNZERHOF M. Generalized gradient approximation made simple [J]. Physical Review Letters, 1996, 77(18): 3865–3868. doi: 10.1103/PhysRevLett.77.3865
[14]	DION M, RYDBERG H, SCHRÖDER E, et al. Van der Waals density functional for general geometries [J]. Physical Review Letters, 2004, 92(24): 246401. doi: 10.1103/PhysRevLett.92.246401
[15]	PERDEW J P, RUZSINSZKY A, CSONKA G I, et al. Restoring the density-gradient expansion for exchange in solids and surfaces [J]. Physical Review Letters, 2008, 100(13): 136406. doi: 10.1103/PhysRevLett.100.136406
[16]	HAMMER B, HANSEN L B, NØRSKOV J K. Improved adsorption energetics within density-functional theory using revised Perdew-Burke-Ernzerhof functionals [J]. Physical Review B, 1999, 59(11): 7413–7421. doi: 10.1103/PhysRevB.59.7413
[17]	KRESSE G, FURTHMÜLLER J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set [J]. Physical Review B, 1996, 54(16): 11169–11186. doi: 10.1103/PhysRevB.54.11169
[18]	BLÖCHL P E. Projector augmented-wave method [J]. Physical Review B, 1994, 50(24): 17953–17979. doi: 10.1103/PhysRevB.50.17953
[19]	TOGO A, TANAKA I. First principles phonon calculations in materials science [J]. Scripta Materialia, 2015, 108: 1–5. doi: 10.1016/j.scriptamat.2015.07.021
[20]	HILL R. The elastic behaviour of a crystalline aggregate [J]. Proceedings of the Physical Society Section A, 1952, 65(5): 349–354. doi: 10.1088/0370-1298/65/5/307
[21]	OUADHA I, RACHED H, AZZOUZ-RACHED A, et al. Study of the structural, mechanical and thermodynamic properties of the new MAX phase compounds (Zr_1−xTi_x)₃AlC₂ [J]. Computational Condensed Matter, 2020, 23: e00468. doi: 10.1016/j.cocom.2020.e00468
[22]	MOMMA K, IZUMI F. VESTA 3 for three-dimensional visualization of crystal, volumetric and morphology data [J]. Journal of Applied Crystallography, 2011, 44(6): 1272–1276. doi: 10.1107/S0021889811038970
[23]	MISHRA K K, CHANDRA S, SALKE N P, et al. Soft modes and anharmonicity in H₃[Co(CN)₆]: Raman spectroscopy and first-principles calculations [J]. Physical Review B, 2015, 92(13): 134112. doi: 10.1103/PhysRevB.92.134112
[24]	POHL J, POHL D, ADIWIDJAJA G. Phase transition in rubidium nitrate at 346 K and structure at 296, 372, 413 and 437 K [J]. Acta Crystallographica Section B, 1992, 48(2): 160–166. doi: 10.1107/S0108768191013459
[25]	SHAMSUZZOHA M, LUCAS B W. Structure (neutron) of phase Ⅳ rubidium nitrate at 298 and 403 K [J]. Acta Crystallographica Section B, 1982, 38(9): 2353–2357. doi: 10.1107/S0567740882008772
[26]	SMITH D, LAWLER K V, MARTINEZ-CANALES M, et al. Postaragonite phases of CaCO₃ at lower mantle pressures [J]. Physical Review Materials, 2018, 2(1): 013605. doi: 10.1103/PhysRevMaterials.2.013605
[27]	WU Z J, ZHAO E J, XIANG H P, et al. Crystal structures and elastic properties of superhard IrN₂ and IrN₃ from first principles [J]. Physical Review B, 2007, 76(5): 054115. doi: 10.1103/PhysRevB.76.054115
[28]	MOUHAT F, COUDERT F X. Necessary and sufficient elastic stability conditions in various crystal systems [J]. Physical Review B, 2014, 90(22): 224104. doi: 10.1103/PhysRevB.90.224104
[29]	BORN M, HUANG K, LAX M. Dynamical theory of crystal lattices [J]. American Journal of Physics, 1955, 23(7): 474.
[30]	UKITA M, TOYOURA K, NAKAMURA A, et al. Pressure-induced phase transition of calcite and aragonite: a first principles study [J]. Journal of Applied Physics, 2016, 120(14): 142118. doi: 10.1063/1.4961723