High-Pressure Phase Transitions Kinetics and Physical Properties on Second-to-Microsecond Time Scales: Review, Progress and Prospects
-
摘要: 近年来,基于金刚石对顶砧的快速加载技术(如动态金刚石对顶砧)和时间分辨探测技术的快速发展为高压科学研究开辟了新的研究方向和思路。这些技术使得科学家能够探索微秒到秒级时间尺度的高压非平衡物理过程,填补了静高压与动态冲击波实验之间的空白。本文回顾并总结了近年来在快速加载和时间分辨探测技术上的进展,详细探讨了依赖于加载速率的结构相变动力学、相变途径、亚稳相的形成、微结构、力致发光等现象。希望通过深入思考和系统归纳微秒至秒级时间尺度的高压科学问题和技术挑战,为高压科学领域的研究者提供新的启示和参考。Abstract: In recent years, the development of rapid loading techniques (such as dynamic diamond anvil cell, dDAC) and time-resolved detection technologies based on diamond anvil presses has opened up new research directions in high-pressure science. This involves exploring the evolution of material structures and physical properties under pressure (or over time) in high-pressure non-equilibrium physical processes that lie between static high-pressure and shock-wave experiments in terms of time scale and loading rate. By reviewing and summarizing the rapid loading and time-resolved probe techniques that have emerged in recent years, this paper attempts to think about and generalize high-pressure science issues and technical challenges on the microsecond to second time scale. It starts from aspects such as structure phase transition dynamics that depend on loading rate, phase transition pathways, the formation of metastable phases, microstructures, and mechanoluminescence, aiming to provide some inspiration and reference for researchers in this field.
-
研究金属靶板在弹丸冲击作用下的响应和破坏对军用武器和防护结构的设计和评估有重要的意义。单层金属靶板在刚性平头弹丸正撞击下的破坏模式可分为:带有整体变形的简单剪切破坏和局部化的绝热剪切冲塞破坏,从能量吸收的角度而言前者优于后者。在工程实践中,常用双层板结构代替单层板。对于单层金属板,很多学者做了相关的理论、实验和数值模拟研究。Wen和Jones[1-2]对刚性平头弹丸低速正撞击下固支的软钢圆板和铝合金圆板的响应和破坏进行了系统的实验研究,并根据实验结果和理论分析提出了刚性平头弹丸正撞下金属靶板低速穿透的Wen-Jones模型。BØrvik等[3]通过实验、理论分析和数值模拟研究了不同厚度固支Weldox460E圆板在平头弹撞击下的变形和穿透,通过初始速度和残余速度求出不同厚度靶板的弹道极限值。Chen等[4]利用刚塑性分析方法研究了平头弹撞击金属圆板的问题,考虑了结构的整体响应和局部剪切破坏。这些研究能够较好地描述和预测平头弹撞击下单层金属靶板的破坏模式和抗弹性能。
相比于单层板冲击失效响应方面的大量实验、数值和理论的研究文献,在公开发表的文章中仅有少量文章研究了多层板的抗弹性能。理论方面只提出了简单模型,大部分都是实验和数值模拟研究,且得出的结论也大相径庭。Radin等[5]做了很多平头弹穿透单层和多层2024-0铝板的实验,发现单层板的弹道极限均高于等厚度的多层板,同时用理论分析模型计算了弹丸的弹道极限,分析结果和实验一致。张伟等[6]通过实验研究发现厚度较小时,同样厚度的单层钢板的弹道极限要高于等厚接触式双层板的弹道极限。Teng等[7]通过ABAQUS/Explicit研究了金属单层板和等厚双层板的抗弹性能,数值模拟结果表明:双层板弹道极限高于单层板7%~25%。对平头弹而言,Dey等[8]实验得到的等厚接触式双层板的弹道极限约高于单层板弹道极限47.2%,与实验结果相比,数值模拟结果(数值模拟结果约高22.9%)大大低估了双层板的抗弹性能。
目前对接触式双层板在平头弹撞击下的抗弹性能和破坏模式尚未有统一的结论,仍需要进行大量的研究工作。我们对等厚接触式双层金属板在平头弹撞击下的穿透破坏进行了理论研究,基于先前单层金属板的穿透理论和实验观察提出一个等厚接触式双层金属板穿透的新模型,并与相关实验数据和其他理论模型进行比较和讨论。
1. 等厚接触式双层板穿透的理论模型
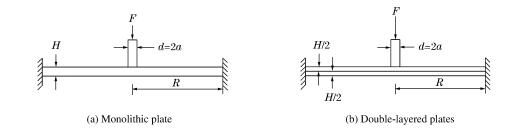
图 1给出了单层板和等厚接触式双层板在平头弹撞击下的示意图。单层板厚度为H,双层板总厚为H,上、下板等厚度,各为H/2,板的半径为R,平头弹弹径为d(d=2a)。由于靶板间的相互作用,双层板的变形和破坏相较单层板要复杂很多。
1.1 第一层板的能量吸收
令F为平头弹作用在双层板上时所受的总作用力,F1为第一层板所受的作用力,F2为第二层板所受的作用力,则F=F1+F2;第一层板的总体变形Wo1,第二层板的总体变形为Wo2。根据文献[1],有
对第一层板:
F1=K1mWo1+F1c (1) 对第二层板:
F2=K2mWo2+F2c (2) 式中:K1m和K2m分别为第一层和第二层板的薄膜刚度,且有K1m=K2m=Km=2πN0/ln(R/a),N0为靶板单位长度的薄膜力,且有N0=σyH/2,σy为靶板材料的屈服应力;F1c和F2c为两层板的静态极限载荷,且有F1c=F2c=Fc=(4/√3)πM0[1+(1+√3/2)/ln(R/a)],M0=σy(H/2)2/4为靶板单位长度的极限塑性弯矩。第一层板穿透破坏之前,第二层板紧贴第一层板,该过程第一层板和第二块板的整体变形基本相同,可以表示为
Wo1≈Wo2=Wo (3) 结合F=F1+F2和方程(1)、方程(2),有
F=2KmWo+2Fc (4) 对于固支金属圆板,等效应变εe[9]可以表达为
ε2e=43(ε2r+εrεθ+ε2θ)+13γ2rz (5) 式中:εr、εθ、γrz分别为径向应变、周向应变和横向剪应变,对于厚度为H/2的靶板,其值可表示为
εr=W202a2ln2(a/R)+W0(H/2)2a2ln(a/R) (6) εθ=−W0(H/2)2a2ln(a/R) (7) 第一层板横向剪应变[1]可以表示为
γrz=Δ/e=(F/Fu)1/nγc (8) 式中: γc为临界剪应变;Δ为弹丸压入深度,Δc为临界压入深度;e为剪切带半宽度;Fu为发生剪切冲塞破坏的临界剪切力,Fu=τuAs,τu=σu[0.41H/(2d)+0.42],As=πdH/2,σu为极限拉伸应力。令(5)式中的等效应变εe等于拉伸破坏应变εf(εe=εf),就可以从方程(5)~方程(8)求得第一层板穿透破坏时的最大整体变形Wo1f
ε2f=163(H2d)4[(2Wo1f/H)4ln4(a/R)+(2Wo1f/H)3ln3(a/R)+(2Wo1f/H)2ln2(a/R)]+13{2λ[0.41H/(2d)+0.42]{2Wo1f/dln(R/a)+1√3[1+√3/2ln(R/a)]H2d}}2/nγ2c (9) 将由(9)式求得的不同总厚度H对应的第一层板的最大整体变形Wo1f代入方程(10)、方程(11)即可求得第一层板的整体变形耗能(Ebm1)
Ebm1=∫Wo1f0F1dS=∫Wo1f0(KmW01+Fc)dWo1+Km2W2o1f+FcWo1f (10) 和局部剪切耗能(Es1)
Es1=∫Δc0FsdΔ=FuΔcn+1(KmWo1f+FcFu)(n+1)/n (11) 第一层板穿透破坏吸收的总能量Ep1为整体变形耗能Ebm1和局部剪切耗能Es1之和,即Ep1=Ebm1+Es1。
1.2 第二层板的能量吸收
在贯穿第一层板后,平头弹前端附着第一层板的塞块撞击第二层板。图 2给出了第二层板组合弹丸(平头弹+塞块)作用下破坏示意图。第一层板的塞块在平头弹和第二层板作用下边缘厚度变薄,剖面近似于四分之一圆,中间部分近似成平面。在组合弹丸(平头弹+塞块)作用下,第二层板的整体变形增大,因薄膜拉伸造成局部厚度变薄。
如图 2所示,根据实验和数值模拟结果第一层板塞块的厚度约为初始厚度的0.9倍,即H1≈0.45H。第二层板的初始厚度为H0=H/2,发生破坏时破坏处的厚度为H2,由塑性变形体积不变可以有πr20H0=πr22H2,即r2/r0=√H0/H2,根据工程应变和真实应变的关系可得第二层圆板在破坏处的径向真实应变,即εr1=ln√H0/H2=(−1/2)ln(2H2/H)。
根据文献[9],将第二层板破坏处的径向应变近似表示为εr2=W2o2f2r21ln2(r1/R)+Wo2fH4r21ln(r1/R),其中r1为破坏处距离弹丸中心的距离,根据本模型可得r1=a-H1+H1sin(π/4),由εr1=εr2可求得第二层板破坏时的最大整体变形Wo2f与总厚度H及第二层板破坏时最终厚度H2之间的函数关系
−12ln(2H2H)=W2o2f2r21ln2(r1/R)+Wo2fH4r21ln(r1/R) (12) 而厚度为H/2的单层板在平头弹撞击下的最大整体变形Wof[8]可以表示为
ε2f=163(H2d)4[2(W0f/H)4ln4(a/R)+(2W0f/H)3ln3(a/R)+(2W0f/H)2ln2(a/R)]+13{1λ[0.41H/(2d)+0.42]{2W0f/dln(R/a)+1√3[1+1+√3/2ln(R/a)]H2d}}2/nγ2c (13) 对于不同厚度的双层板,靶板的厚度越小,第一层板的塞块厚度H1越小,r1=a-H1+H1sin(π/4)的值与平头弹半径a越接近。当靶板厚度趋于零时,有r1|H→0=a,此时第二层板与平头弹穿透厚为H/2单层板的速度场和整体变形场相同[1],有εr2=εr,即(Wo2f=Wof)|H→0,文字表述为当靶板厚度趋于零时第二层板的整体变形和平头弹穿透厚度为H/2的单层靶板的整体变形相同。由于公式较为复杂且包含隐式形式,求解较为复杂,用Matlab软件求解隐式方程组(12)式、(13)式,得到第二层板的最终厚度H2以及(12)式、(13)式对应的函数关系,(12)式、(13)式的函数图像在H=0处相交。将求得的H2代入(12)式即可求得双层靶第二层靶板的最大整体变形Wo2f与总厚度H间的关系,进而可求得第二层板的总体变形耗能Ebm2
Ebm2=Km2W2o2f+FcWo2f (14) 和局部拉伸耗能Et2的近似值
Et2=EV≈σyεr1πr21H2 (15) 第二层板穿透的能量消耗Ep2=Ebm2+Et2,则可以得到平头弹穿透双层板所消耗的总能量Ep为
Ep=Ep1+Ep2=Ebm1+Es1+Ebm2+Et2 (16) 1.3 应变率效应
以上得到的是准静态条件下的穿透能量,动态冲击下需要考虑材料的应变率效应。材料的应变率效应可以用Cowper-Symonds经验公式来描述, 即
σd=σy[1+(˙εm/D)1/q] (17) 式中: σd为材料的动态屈服应力,D和q为描述材料应变率的敏感性常数,˙εm为靶板的平均应变率。对双层靶而言,第一、二层板的平均应变率[1]可分别写为
˙εm1=2Wo1fvbl3√2Raln2(a/R) (18) ˙εm2=2Wo2fvbl3√2Rr1ln2(r1/R) (19) 式中: vbl为弹道极限。将能量公式中的静态屈服应力σy用动态屈服应力σd代替,可得到动态情况下平头弹穿透靶板所消耗的能量Edp。令Edp=Mv2bl/2,可以得到平头弹撞击下双层靶的弹道极限, 即
vbl=√2EdpM (20) 式中: M为平头弹质量。
2. 结果与讨论
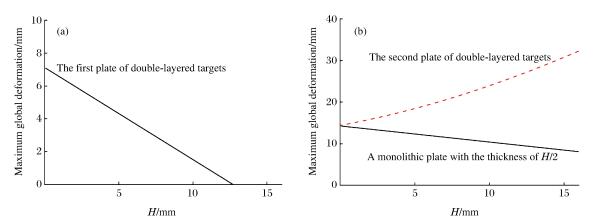
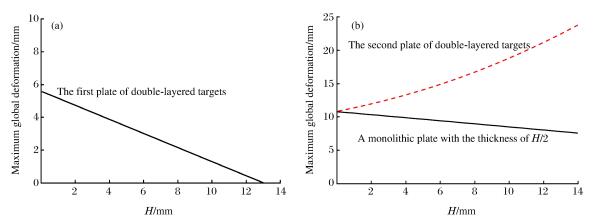
将本研究的理论模型结果与文献中的相关实验结果进行比较和讨论。针对Dey等[8]做的平头弹撞击等厚接触式双层Weldox700E钢板的实验,模型中的相关参数值见表 1,根据本研究模型可以求得不同厚度的第一层板和第二层板的最大整体变形(见图 3),并得到第二层板破坏处的最终厚度为H2≈0.41H。从图 3(a)可以看出,双层靶中的第一层板的总体变形随着总厚度的增加而减少,而第二层板正好相反,其总体变形随着厚度的增加而增加。图 3(b)给出了单层板在平头弹作用下总体变形随厚度的变化情况, 即其总体变形随着厚度的增加而逐步减少。
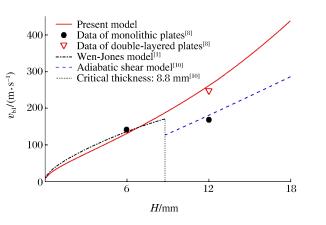
图 4给出了本研究双层板理论模型求得的等厚接触式双层Weldox700E钢板的弹道极限与Dey等的实验结果及单层板的Wen-Jones模型[1]和绝热剪切模型[10]的对比。从图 4可以看出, 本研究模型能较好地预测等厚接触式双层板的弹道极限值。且对于单层板,当发生局部化的绝热剪切破坏时,等厚接触式双层板的弹道极限要明显大于单层板的弹道极限;当发生带有整体变形的简单剪切破坏时,等厚接触式双层板和单层板的弹道极限几乎相同。
针对张伟等[6]的平头弹撞击等厚接触式双层Q235钢板的实验,模型中的相关参数值见表 2,用同样的方法可以求得不同厚度的第一层板和第二层板的最大整体变形(见图 5),根据本研究理论可得第二层板破坏处的最终厚度为H2≈0.315H。接触式双层Q235钢板中第一层板和第二层板的总体变形规律与接触式双层Weldox700E钢板类似,见图 5。
图 6给出了用本研究能量模型求得的等厚接触式双层Q235钢板的弹道极限与张伟等的实验结果及单层板的Wen-Jones模型[1]和绝热剪切模型[10]的对比。从图 4可以发现, 本研究模型与张伟等的等厚接触式双层板的实验弹道极限值吻合得很好。且对于单层板,当发生带有整体变形的简单剪切破坏时,等厚接触式双层板和单层板的弹道极限几乎相同;当发生局部化的绝热剪切破坏时,等厚接触式双层板的弹道极限要明显大于单层板的弹道极限。
3. 结论
通过分析等厚接触式双层板的破坏模式,基于Wen-Jones模型和应变失效准则得到了接触式双层板穿透的理论模型。结果表明:理论预测与有限的实验数据结果吻合得很好;当总厚度大于单层板绝热剪切冲塞临界厚度值时,双层板的弹道极限明显高于单层板的弹道极限;小于该值时,双层板的弹道极限与单层板的弹道极限差别不大。
-
-
[1] MAO H K, BELL P M. High-pressure physics: the 1-megabar mark on the ruby R1 static pressure scale [J]. Science, 1976, 191(4229): 851–852. doi: 10.1126/science.191.4229.851 [2] MAO H K. High-pressure physics: sustained static generation of 1.36 to 1.72 megabars [J]. Science, 1978, 200(4346): 1145–1147. doi: 10.1126/science.200.4346.1145 [3] DUBROVINSKY L, DUBROVINSKAIA N, BYKOVA E, et al. The most incompressible metal osmium at static pressures above 750 gigapascals [J]. Nature, 2015, 525(7568): 226–229. doi: 10.1038/nature14681 [4] DUBROVINSKY L, DUBROVINSKAIA N, PRAKAPENKA V B, et al. Implementation of micro-ball nanodiamond anvils for high-pressure studies above 6 Mbar [J]. Nature Communications, 2012, 3: 1163. doi: 10.1038/ncomms2160 [5] MAO H K, CHEN X J, DING Y, et al. Solids, liquids, and gases under high pressure [J]. Reviews of Modern Physics, 2018, 90(1): 015007. doi: 10.1103/RevModPhys.90.015007 [6] WEHRENBERG C E, MCGONEGLE D, BOLME C, et al. In situ X-ray diffraction measurement of shock-wave-driven twinning and lattice dynamics [J]. Nature, 2017, 550(7677): 496–499. doi: 10.1038/nature24061 [7] TURNEAURE S J, SINCLAIR N, GUPTA Y M. Real-time examination of atomistic mechanisms during shock-induced structural transformation in silicon [J]. Physical Review Letters, 2016, 117(4): 045502. doi: 10.1103/PhysRevLett.117.045502 [8] PANDOLFI S, BROWN S B, STUBLEY P G, et al. Atomistic deformation mechanism of silicon under laser-driven shock compression [J]. Nature Communications, 2022, 13(1): 5535. doi: 10.1038/s41467-022-33220-0 [9] MCMAHON M I. Synchrotron and FEL studies of matter at high pressures [M]//JAESCHKE E J, KHAN S, SCHNEIDER J R, et al. Synchrotron Light Sources and Free-Electron Lasers: Accelerator Physics, Instrumentation and Science Applications. 2nd ed. Cham: Springer, 2020: 1857−1896. [10] HEMLEY R J, MAO H K, STRUZHKIN V V. Synchrotron radiation and high pressure: new light on materials under extreme conditions [J]. Journal of Synchrotron Radiation, 2005, 12(2): 135–154. doi: 10.1107/S0909049504034417 [11] HIRAO N, KAWAGUCHI S I, HIROSE K, et al. New developments in high-pressure X-ray diffraction beamline for diamond anvil cell at SPring-8 [J]. Matter and Radiation at Extremes, 2020, 5(1): 018403. doi: 10.1063/1.5126038 [12] LIERMANN H P, KONÔPKOVÁ Z, MORGENROTH W, et al. The extreme conditions beamline P02.2 and the extreme conditions science infrastructure at PETRA Ⅲ [J]. Journal of Synchrotron Radiation, 2015, 22(4): 908–924. doi: 10.1107/S1600577515005937 [13] LIERMANN H P, KONÔPKOVÁ Z, APPEL K, et al. Novel experimental setup for megahertz X-ray diffraction in a diamond anvil cell at the High Energy Density (HED) instrument of the European X-ray Free-Electron Laser (EuXFEL) [J]. Journal of Synchrotron Radiation, 2021, 28(3): 688–706. doi: 10.1107/S1600577521002551 [14] CHANDRA SHEKAR N V, RAJAN K G. Kinetics of pressure induced structural phase transitions: a review [J]. Bulletin of Materials Science, 2001, 24(1): 1–21. doi: 10.1007/BF02704834 [15] HABERL B, GUTHRIE M, SINOGEIKIN S V, et al. Thermal evolution of the metastable r8 and bc8 polymorphs of silicon [J]. High Pressure Research, 2015, 35(2): 99–116. doi: 10.1080/08957959.2014.1003555 [16] LEE G W, EVANS W J, YOO C S. Dynamic pressure-induced dendritic and shock crystal growth of ice Ⅵ [J]. Proceedings of the National Academy of Sciences of the United States of America, 2007, 104(22): 9178–9181. doi: 10.1073/pnas.0609390104 [17] KIM Y J, LEE Y H, LEE S, et al. Shock growth of ice crystal near equilibrium melting pressure under dynamic compression [J]. Proceedings of the National Academy of Sciences of the United States of America, 2019, 116(18): 8679–8684. doi: 10.1073/pnas.1818122116 [18] LIN C L, LIU X Q, YONG X, et al. Temperature-dependent kinetic pathways featuring distinctive thermal-activation mechanisms in structural evolution of ice Ⅶ [J]. Proceedings of the National Academy of Sciences of the United States of America, 2020, 117(27): 15437–15442. doi: 10.1073/pnas.2007959117 [19] BASTEA M, BASTEA S, BECKER R. High pressure phase transformation in iron under fast compression [J]. Applied Physics Letters, 2009, 95(24): 241911. doi: 10.1063/1.3275797 [20] BASTEA M, BASTEA S, REAUGH J E, et al. Freezing kinetics in overcompressed water [J]. Physical Review B, 2007, 75(17): 172104. doi: 10.1103/PhysRevB.75.172104 [21] DOLAN D H, GUPTA Y M. Nanosecond freezing of water under multiple shock wave compression: optical transmission and imaging measurements [J]. The Journal of Chemical Physics, 2004, 121(18): 9050–9057. doi: 10.1063/1.1805499 [22] DOLAN D H, JOHNSON J N, GUPTA Y M. Nanosecond freezing of water under multiple shock wave compression: continuum modeling and wave profile measurements [J]. The Journal of Chemical Physics, 2005, 123(6): 064702. doi: 10.1063/1.1993556 [23] DOLAN D H, KNUDSON M D, HALL C A, et al. A metastable limit for compressed liquid water [J]. Nature Physics, 2007, 3(5): 339–342. doi: 10.1038/nphys562 [24] BOETTGER J C, WALLACE D C. Metastability and dynamics of the shock-induced phase transition in iron [J]. Physical Review B, 1997, 55(5): 2840–2849. doi: 10.1103/PhysRevB.55.2840 [25] KNUDSON M D, GUPTA Y M. Transformation kinetics for the shock wave induced phase transition in cadmium sulfide crystals [J]. Journal of Applied Physics, 2002, 91(12): 9561–9571. doi: 10.1063/1.1478790 [26] KNUDSON M D, DESJARLAIS M P, BECKER A, et al. Direct observation of an abrupt insulator-to-metal transition in dense liquid deuterium [J]. Science, 2015, 348(6242): 1455–1460. doi: 10.1126/science.aaa7471 [27] YU P, WANG W H, WANG R J, et al. Understanding exceptional thermodynamic and kinetic stability of amorphous sulfur obtained by rapid compression [J]. Applied Physics Letters, 2009, 94(1): 011910. doi: 10.1063/1.3064125 [28] HU Y, SU L, LIU X R, et al. Preparation of high-density nanocrystalline bulk selenium by rapid compressing of melt [J]. Chinese Physics Letters, 2010, 27(3): 038101. doi: 10.1088/0256-307X/27/3/038101 [29] BIWER C M, QUAN A, HUSTON L Q, et al. Cinema: snap: real-time tools for analysis of dynamic diamond anvil cell experiment data [J]. Review of Scientific Instruments, 2021, 92(10): 103901. doi: 10.1063/5.0057878 [30] DOU X M, DING K, SUN B Q. Development and application of piezoelectric driving diamond anvil cell device [J]. Review of Scientific Instruments, 2017, 88(12): 123105. doi: 10.1063/1.4996063 [31] EVANS W J, YOO C S, LEE G W, et al. Dynamic diamond anvil cell (dDAC): a novel device for studying the dynamic-pressure properties of materials [J]. Review of Scientific Instruments, 2007, 78(7): 073904. doi: 10.1063/1.2751409 [32] JENEI Z, LIERMANN H P, HUSBAND R, et al. New dynamic diamond anvil cells for tera-pascal per second fast compression X-ray diffraction experiments [J]. Review of Scientific Instruments, 2019, 90(6): 065114. doi: 10.1063/1.5098993 [33] MÉNDEZ A S J, MARQUARDT H, HUSBAND R J, et al. A resistively-heated dynamic diamond anvil cell (RHdDAC) for fast compression X-ray diffraction experiments at high temperatures [J]. Review of Scientific Instruments, 2020, 91(7): 073906. doi: 10.1063/5.0007557 [34] SMITH J S, SINOGEIKIN S V, LIN C L, et al. Developments in time-resolved high pressure X-ray diffraction using rapid compression and decompression [J]. Review of Scientific Instruments, 2015, 86(7): 072208. doi: 10.1063/1.4926887 [35] SINOGEIKIN S V, SMITH J S, ROD E, et al. Online remote control systems for static and dynamic compression and decompression using diamond anvil cells [J]. Review of Scientific Instruments, 2015, 86(7): 072209. doi: 10.1063/1.4926892 [36] LIN C L, SMITH J S, LIU X Q, et al. Venture into water’s no man’s land: structural transformations of solid H2O under rapid compression and decompression [J]. Physical Review Letters, 2018, 121(22): 225703. doi: 10.1103/PhysRevLett.121.225703 [37] LIN C L, LIU X Q, YANG D L, et al. Temperature- and rate-dependent pathways in formation of metastable silicon phases under rapid decompression [J]. Physical Review Letters, 2020, 125(15): 155702. doi: 10.1103/PhysRevLett.125.155702 [38] LIN C L, SMITH J S, SINOGEIKIN S V, et al. Kinetics of the B1-B2 phase transition in KCl under rapid compression [J]. Journal of Applied Physics, 2016, 119(4): 045902. doi: 10.1063/1.4940771 [39] LIN C L, TSE J S. High-pressure nonequilibrium dynamics on second-to-microsecond time scales: application of time-resolved X-ray diffraction and dynamic compression in ice [J]. The Journal of Physical Chemistry Letters, 2021, 12(33): 8024–8038. doi: 10.1021/acs.jpclett.1c01623 [40] CHEN X H, ZHANG Y, YE S J, et al. Time-resolved Raman spectroscopy for monitoring the structural evolution of materials during rapid compression [J]. Review of Scientific Instruments, 2023, 94(12): 123901. doi: 10.1063/5.0172530 [41] ZHANG L, SHI K Y, WANG Y L, et al. Unraveling the anomalous mechanoluminescence intensity change and pressure-induced red-shift for manganese-doped zinc sulfide [J]. Nano Energy, 2021, 85: 106005. doi: 10.1016/j.nanoen.2021.106005 [42] WANG H, CHEN X H, LI J L, et al. Pressure- and rate-dependent mechanoluminescence with maximized efficiency and tunable wavelength in ZnS: Mn2+, Eu3+ [J]. ACS Applied Materials & Interfaces, 2023, 15(23): 28204–28214. doi: 10.1021/acsami.3c04093 [43] DOLAN D H, GUPTA Y M. Time-dependent freezing of water under dynamic compression [J]. Chemical Physics Letters, 2003, 374(5/6): 608–612. doi: 10.1016/S0009-2614(03)00777-2 [44] CHEN J Y, YOO C S. High density amorphous ice at room temperature [J]. Proceedings of the National Academy of Sciences of the United States of America, 2011, 108(19): 7685–7688. doi: 10.1073/pnas.1100752108 [45] KONG J, SHI K Y, DONG X B, et al. Expanding the pressure frontier in Grüneisen parameter measurement: study of sodium chloride [J]. Physical Review Letters, 2023, 131(26): 266101. doi: 10.1103/PhysRevLett.131.266101 [46] TOMASINO D A, YOO C S. Solidification and crystal growth of highly compressed hydrogen and deuterium: time-resolved study under ramp compression in dynamic-diamond anvil cell [J]. Applied Physics Letters, 2013, 103(6): 061905. doi: 10.1063/1.4818311 [47] TOMASINO D A, YOO C S. Probing dynamic crystal growth of compressed hydrogen using dynamic-DAC, time-resolved spectroscopy and highspeed micro-photography [J]. Journal of Physics: Conference Series, 2014, 500(3): 032019. doi: 10.1088/1742-6596/500/3/032019 [48] CHEN J Y, YOO C S, EVANS W J, et al. Solidification and fcc to metastable hcp phase transition in krypton under variable compression rates [J]. Physical Review B, 2014, 90(14): 144104. doi: 10.1103/PhysRevB.90.144104 [49] OTZEN C, LIERMANN H P, LANGENHORST F. Evidence for a rosiaite-structured high-pressure silica phase and its relation to lamellar amorphization in quartz [J]. Nature Communications, 2023, 14(1): 606. doi: 10.1038/s41467-023-36320-7 [50] CHEN J Y, YOO C S. Formation and phase transitions of methane hydrates under dynamic loadings: compression rate dependent kinetics [J]. The Journal of Chemical Physics, 2012, 136(11): 114513. doi: 10.1063/1.3695212 [51] HONG S M, CHEN L Y, LIU X R, et al. High pressure jump apparatus for measuring Grüneisen parameter of NaCl and studying metastable amorphous phase of poly (ethylene terephthalate) [J]. Review of Scientific Instruments, 2005, 76(5): 053905. doi: 10.1063/1.1899443 [52] JIA R, SHAO C G, SU L, et al. Rapid compression induced solidification of bulk amorphous sulfur [J]. Journal of Physics D: Applied Physics, 2007, 40(12): 3763–3766. doi: 10.1088/0022-3727/40/12/030 [53] ZHANG D D, LIU X R, HE Z, et al. Pressure and time dependences of the supercooled liquid-to-liquid transition in sulfur [J]. Chinese Physics Letters, 2016, 33(2): 026301. doi: 10.1088/0256-307X/33/2/026301 [54] LIU X R, ZHANG L J, YUAN C S, et al. A study of the pressure-induced solidification of polymers [J]. Polymers, 2018, 10(8): 847. doi: 10.3390/polym10080847 [55] CHENG H, ZHANG J R, LI Y C, et al. A convenient dynamic loading device for studying kinetics of phase transitions and metastable phases using symmetric diamond anvil cells [J]. High Pressure Research, 2018, 38(1): 32–40. doi: 10.1080/08957959.2017.1396326 [56] HUSBAND R J, HAGEMANN J, O’BANNON E F, et al. Simultaneous imaging and diffraction in the dynamic diamond anvil cell [J]. Review of Scientific Instruments, 2022, 93(5): 053903. doi: 10.1063/5.0084480 [57] 王碧涵, 李冰, 刘旭强, 等. 毫秒时间分辨同步辐射X射线衍射和高压快速加载装置及应用 [J]. 物理学报, 2022, 71(10): 100702. doi: 10.7498/aps.71.20212360WANG B H, LI B, LIU X Q, et al. Millisecond time-resolved synchrotron radiation X-ray diffraction and high-pressure rapid compression device and its application [J]. Acta Physica Sinica, 2022, 71(10): 100702. doi: 10.7498/aps.71.20212360 [58] SU L, SHI K Y, ZHANG L, et al. Static and dynamic diamond anvil cell (s-dDAC): a bidirectional remote controlled device for static and dynamic compression/decompression [J]. Matter and Radiation at Extremes, 2022, 7(1): 018401. doi: 10.1063/5.0061583 [59] ZHANG L, SHI K Y, WANG Y L, et al. Compression rate-dependent crystallization of pyridine [J]. The Journal of Physical Chemistry C, 2021, 125(12): 6983–6989. doi: 10.1021/acs.jpcc.1c01163 [60] YAN J W, LIU X D, GORELLI F A, et al. Compression rate of dynamic diamond anvil cells from room temperature to 10 K [J]. Review of Scientific Instruments, 2022, 93(6): 063901. doi: 10.1063/5.0091102.doi:10.1063/5.0007557 [61] SINGH A K. The kinetics of some pressure-induced transformations [J]. Materials Science Forum, 1985, 3: 291–306. doi: 10.4028/www.scientific.net/MSF.3.291 [62] GLASSTONE S, LAIDLER K J, EYRING H. The theory of rate processes: the kinetics of chemical reactions, viscosity, diffusion and electrochemical phenomena [M]. New York: McGraw-Hill, 1941. [63] CHRISTIAN J W. The theory of transformations in metals and alloys [M]. Oxford: Pergamon, 2002. [64] FANFONI M, TOMELLINI M. The Johnson-Mehl-Avrami-Kohnogorov model: a brief review [J]. Nuovo Cimento D, 1998, 20(7/8): 1171–1182. doi: 10.1007/BF03185527 [65] AVRAMI M. Kinetics of phase change. Ⅰ general theory [J]. The Journal of Chemical Physics, 1939, 7(12): 1103–1112. doi: 10.1063/1.1750380 [66] AVRAMI M. Kinetics of phase change. Ⅱ transformation-time relations for random distribution of nuclei [J]. The Journal of Chemical Physics, 1940, 8(2): 212–224. doi: 10.1063/1.1750631 [67] AVRAMI M. Granulation, phase change, and microstructure kinetics of phase change. Ⅲ [J]. The Journal of Chemical Physics, 1941, 9(2): 177–184. doi: 10.1063/1.1750872 [68] TSE J S, KLUG D D. Pressure amorphized ices: an atomistic perspective [J]. Physical Chemistry Chemical Physics, 2012, 14(23): 8255–8263. doi: 10.1039/c2cp40201g [69] MISHIMA O. Relationship between melting and amorphization of ice [J]. Nature, 1996, 384(6609): 546–549. doi: 10.1038/384546a0 [70] MISHIMA O, STANLEY H E. The relationship between liquid, supercooled and glassy water [J]. Nature, 1998, 396(6709): 329–335. doi: 10.1038/24540 [71] MISHIMA O. Volume of supercooled water under pressure and the liquid-liquid critical point [J]. The Journal of Chemical Physics, 2010, 133(14): 144503. doi: 10.1063/1.3487999 [72] MISHIMA O, CALVERT L D, WHALLEY E. ‘Melting ice’ Ⅰ at 77 K and 10 kbar: a new method of making amorphous solids [J]. Nature, 1984, 310(5976): 393–395. doi: 10.1038/310393a0 [73] MISHIMA O, CALVERT L D, WHALLEY E. An apparently first-order transition between two amorphous phases of ice induced by pressure [J]. Nature, 1985, 314(6006): 76–78. doi: 10.1038/314076a0 [74] LOERTING T, SALZMANN C, KOHL I, et al. A second distinct structural “state” of high-density amorphous ice at 77 K and 1 bar [J]. Physical Chemistry Chemical Physics, 2001, 3(24): 5355–5357. doi: 10.1039/b108676f [75] TULK C A, BENMORE C J, URQUIDI J, et al. Structural studies of several distinct metastable forms of amorphous ice [J]. Science, 2002, 297(5585): 1320–1323. doi: 10.1126/science.1074178 [76] NELMES R J, LOVEDAY J S, STRÄSSLE T, et al. Annealed high-density amorphous ice under pressure [J]. Nature Physics, 2006, 2(6): 414–418. doi: 10.1038/nphys313 [77] GALLO P, AMANN-WINKEL K, ANGELL C A, et al. Water: a tale of two liquids [J]. Chemical Reviews, 2016, 116(13): 7463–7500. doi: 10.1021/acs.chemrev.5b00750 [78] AMANN-WINKEL K, BÖHMER R, FUJARA F, et al. Colloquium: water’s controversial glass transitions [J]. Reviews of Modern Physics, 2016, 88(1): 011002. doi: 10.1103/RevModPhys.88.011002 [79] FISHER M, DEVLIN J P. Defect activity in amorphous ice from isotopic exchange data: insight into the glass transition [J]. The Journal of Physical Chemistry, 1995, 99(29): 11584–11590. doi: 10.1021/j100029a041 [80] SHEPHARD J J, SALZMANN C G. Molecular reorientation dynamics govern the glass transitions of the amorphous ices [J]. The Journal of Physical Chemistry Letters, 2016, 7(12): 2281–2285. doi: 10.1021/acs.jpclett.6b00881 [81] HABERL B, STROBEL T A, BRADBY J E. Pathways to exotic metastable silicon allotropes [J]. Applied Physics Reviews, 2016, 3(4): 040808. doi: 10.1063/1.4962984 [82] ZHANG H D, LIU H Y, WEI K Y, et al. BC8 silicon (Si-Ⅲ) is a narrow-gap semiconductor [J]. Physical Review Letters, 2017, 118(14): 146601. doi: 10.1103/PhysRevLett.118.146601 [83] WONG S, HABERL B, JOHNSON B C, et al. Formation of an r8-dominant Si material [J]. Physical Review Letters, 2019, 122(10): 105701. doi: 10.1103/PhysRevLett.122.105701 [84] BRAZHKIN V V, LYAPIN A G, POPOVA S V, et al. Nonequilibrium phase transitions and amorphization in Si, Si/GaAs, Ge, and Ge/GaSb at the decompression of high-pressure phases [J]. Physical Review B, 1995, 51(12): 7549–7554. doi: 10.1103/PhysRevB.51.7549 [85] FENG A, SMET P F. A review of mechanoluminescence in inorganic solids: compounds, mechanisms, models and applications [J]. Materials, 2018, 11(4): 484. doi: 10.3390/ma11040484 [86] ZHANG J C, WANG X S, MARRIOTT G, et al. Trap-controlled mechanoluminescent materials [J]. Progress in Materials Science, 2019, 103: 678–742. doi: 10.1016/j.pmatsci.2019.02.001 [87] JHA P, CHANDRA B P. Survey of the literature on mechanoluminescence from 1605 to 2013 [J]. Luminescence, 2014, 29(8): 977–993. doi: 10.1002/bio.2647 [88] TIWARI G, BRAHME N, SHARMA R, et al. Fracto-mechanoluminescence and thermoluminescence properties of UV and γ-irradiated Ca2Al2SiO7: Ce3+ phosphor [J]. Luminescence, 2016, 31(3): 793–801. doi: 10.1002/bio.3025 [89] CHANDRA V K, CHANDRA B P, JHA P. Models for intrinsic and extrinsic elastico and plastico-mechanoluminescence of solids [J]. Journal of Luminescence, 2013, 138: 267–280. doi: 10.1016/j.jlumin.2013.01.024 [90] XIE Y J, LI Z. Triboluminescence: recalling interest and new aspects [J]. Chem, 2018, 4(5): 943–971. doi: 10.1016/j.chempr.2018.01.001 [91] CHANDRA B P, CHANDRA V K, JHA P. Elastico-mechanoluminescence of thermoluminescent crystals [J]. Defect and Diffusion Forum, 2013, 347: 139–177. doi: 10.4028/www.scientific.net/DDF.347.139 [92] XU C N, ZHENG X G, AKIYAMA M, et al. Dynamic visualization of stress distribution by mechanoluminescence image [J]. Applied Physics Letters, 2000, 76(2): 179–181. doi: 10.1063/1.125695 [93] XU C N, WATANABE T, AKIYAMA M, et al. Direct view of stress distribution in solid by mechanoluminescence [J]. Applied Physics Letters, 1999, 74(17): 2414–2416. doi: 10.1063/1.123865 [94] MOON JEONG S, SONG S, LEE S K, et al. Mechanically driven light-generator with high durability [J]. Applied Physics Letters, 2013, 102(5): 051110. doi: 10.1063/1.4791689 [95] CHANDRA B P, CHANDRA V K, JHA P. Microscopic theory of elastic-mechanoluminescent smart materials [J]. Applied Physics Letters, 2014, 104(3): 031102. doi: 10.1063/1.4862655 [96] CHANDRA B P, CHANDRA V K, JHA P. Piezoelectrically-induced trap-depth reduction model of elastico-mechanoluminescent materials [J]. Physica B: Condensed Matter, 2015, 461: 38–48. doi: 10.1016/j.physb.2014.12.007 [97] CHANDRA V K, CHANDRA B P, JHA P. Self-recovery of mechanoluminescence in ZnS: Cu and ZnS: Mn phosphors by trapping of drifting charge carriers [J]. Applied Physics Letters, 2013, 103(16): 161113. doi: 10.1063/1.4825360 [98] ZHAO Y J, PENG D F, BAI G X, et al. Multiresponsive emissions in luminescent ions doped quaternary piezophotonic materials for mechanical-to-optical energy conversion and sensing applications [J]. Advanced Functional Materials, 2021, 31(22): 2010265. doi: 10.1002/adfm.202010265 [99] SANG J K, ZHOU J Y, ZHANG J C, et al. Multilevel static-dynamic anticounterfeiting based on stimuli-responsive luminescence in a niobate structure [J]. ACS Applied Materials & Interfaces, 2019, 11(22): 20150–20156. doi: 10.1021/acsami.9b03562 [100] ZHANG J C, GAO N, LI L, et al. Discovering and dissecting mechanically excited luminescence of Mn2+ activators via matrix microstructure evolution [J]. Advanced Functional Materials, 2021, 31(19): 2100221. doi: 10.1002/adfm.202100221 [101] ZHOU S, CHENG Y, XU J, et al. Design of ratiometric dual-emitting mechanoluminescence: lanthanide/transition-metal combination strategy [J]. Laser & Photonics Reviews, 2022, 16(5): 2100666. doi: 10.1002/lpor.202100666 [102] ZHANG J C, ZHAO L Z, LONG Y Z, et al. Color manipulation of intense multiluminescence from CaZnOS: Mn2+ by Mn2+ concentration effect [J]. Chemistry of Materials, 2015, 27(21): 7481–7489. doi: 10.1021/acs.chemmater.5b03570 [103] YANG Y L, YANG X C, YUAN J Y, et al. Time-resolved bright red to cyan color tunable mechanoluminescence from CaZnOS: Bi3+, Mn2+ for anti-counterfeiting device and stress sensor [J]. Advanced Optical Materials, 2021, 9(16): 2100668. doi: 10.1002/adom.202100668 [104] ZHANG L, LIU Z T, SUN X N, et al. Retainable bandgap narrowing and enhanced photoluminescence in Mn-doped and undoped Cs2NaBiCl6 double perovskites by pressure engineering [J]. Advanced Optical Materials, 2022, 10(2): 2101892. doi: 10.1002/adom.202101892 [105] CAO Y, QI G Y, SUI L, et al. Pressure-induced emission enhancements of Mn2+-doped cesium lead chloride perovskite nanocrystals [J]. ACS Materials Letters, 2020, 2(4): 381–388. doi: 10.1021/acsmaterialslett.0c00033 -








 下载:
下载:






 下载:
下载: