B-C-N-Ti四元超硬复合材料的高压烧结

黄鸿东; 于晓辉; 贺端威

doi:10.11858/gywlxb.20240769

B-C-N-Ti四元超硬复合材料的高压烧结

doi: 10.11858/gywlxb.20240769

黄鸿东^1,,
于晓辉^2,*, ,,
贺端威^1,*, ,

1.
四川大学原子与分子物理研究所, 四川成都　610065
2.
中国科学院物理研究所, 北京　100190

基金项目: 国家重点研发计划（2023YFA1406200）

详细信息

作者简介:
黄鸿东（1997－），男，硕士研究生，主要从事高压下超硬材料的合成研究. E-mail：hongdonghuangsss@163.com

通讯作者:
于晓辉（1981－），男，博士，研究员，主要从事高压物理及材料研究. E-mail：yuxh@iphy.ac.cn

贺端威（1969－），男，博士，教授，主要从事高压物理、大腔体静高压技术以及超硬材料研究. E-mail：duanweihe@scu.edu.cn

中图分类号: O521.3; O521.2
计量
- 文章访问数: 221
- HTML全文浏览量: 137
- PDF下载量: 42
出版历程
- 收稿日期: 2024-03-29
- 修回日期: 2024-04-25
- 网络出版日期: 2024-07-08
- 刊出日期: 2024-07-25

High Pressure Sintering of B-C-N-Ti Quaternary Superhard Composites

HUANG Hongdong^1
,,
YU Xiaohui^{2,*
, ,},
HE Duanwei^{1,*
, ,}

1.
Institute of Atomic and Molecular Physics, Sichuan University, Chengdu 610065, Sichuan, China
2.
Institute of Physics, Chinese Academy of Sciences, Beijing 100190, China

摘要

摘要: 以金刚石、立方氮化硼（cBN）和钛（Ti）为初始材料，通过高温高压反应烧结制备了B-C-N-Ti四元超硬复合材料。结果表明：在高温高压下，Ti与金刚石及cBN反应生成TiC_0.7N_0.3和TiB₂；TiC_0.7N_0.3作为黏结相以键合金刚石和cBN晶粒，适量Ti的加入可以有效地提高烧结体的韧性；反应生成的TiC_0.7N_0.3和TiB₂等陶瓷相以及cBN对金刚石晶粒的包裹提高了烧结体的抗氧化性。当金刚石、cBN和Ti的摩尔比为2∶1∶0.10时，在压力为12 GPa、温度为2000℃、保温5 min的条件下得到的烧结样品性能较好，其维氏硬度达到 (49.0±1.2) GPa，韧性为(14.2±0.6) MPa·m^1/2，空气氛围下的起始氧化温度为921 ℃。
- 金刚石 /
- 立方氮化硼 /
- 钛 /
- 超硬复合材料 /
- 高温高压 /
- 烧结
Abstract: In this work, the B-C-N-Ti quaternary superhard composite was prepared by high pressure and high temperature sintering with diamond, cubic boron nitride (cBN) and titanium (Ti) as starting materials. The characterization results show that Ti reacts with diamond and cBN to form TiC_0.7N_0.3 and TiB₂ under high pressure and high temperature, which acts as the binding additives for diamond and cBN grains. The addition of appropriate amount of Ti can effectively improve the toughness of the sintered composite specimen. The formation of TiC_0.7N_0.3 and TiB₂ ceramics and cBN coated the diamond grains and greatly enhanced the oxidation ability. When the molar ratio of diamond, cBN and Ti is 2∶1∶0.10 and sintered at 12 GPa and 2000 ℃ for 5 min, the specimen exhibits the best performance with Vickers hardness of (49.0±1.2) GPa, fracture toughness of (14.2±0.6) MPa·m^1/2 and oxidation temperature of 921 ℃ under air condition.
- diamond /
- cBN /
- titanium /
- superhard composites /
- high pressure and high temperature /
- sintering

HTML全文

功能梯度材料(Functionally Graded Materials, FGM)的概念是1984年在航空飞机计划中首次提出的^[1]，FGM的特性在于它的组成和结构随着体积的变化而变化，从而导致材料相应性质发生改变。因其材料特性呈幂律分布^[2-3]，FGM被广泛应用于工程领域，如航空航天、机械工程、生物医学等。圆柱壳在联合荷载作用下的屈曲分析备受学术界关注^[4-5]。目前，对FGM板壳的研究较为深入^[6-8]。Beni等^[9]利用改进的偶应力理论，对FGM圆柱壳在不同边界条件下的动力屈曲进行了分析；Kargarnovin等^[10]研究了轴向荷载作用下FGM圆柱壳的动力屈曲；Sofiyev等^[11]研究了横向压力下功能梯度正交各向异性圆柱壳的动力屈曲，推导出基于一阶剪切变形理论的功能梯度正交各向异性圆柱壳的稳定性和相容性方程；Khazaeinejad等^[12]研究了弹性模量在厚度方向上连续变化的FGM圆柱壳在复合外压和轴向压缩载荷作用下的动力屈曲；Khalili等^[13]研究了横向冲击载荷作用下FGM圆柱壳的动力屈曲；Alashti等^[14]对变厚度FGM圆柱壳外压和轴向压缩的动力屈曲问题进行了分析。

基于以上研究，本研究讨论了FGM圆柱壳在轴向荷载作用下的动力屈曲。根据Donnell壳体理论和经典板壳理论，利用Hamilton变分原理得到FGM圆柱壳的动力屈曲控制方程；采用分离变量法求得动力屈曲临界荷载表达式；通过MATLAB软件计算动力屈曲临界荷载，讨论由不同材料(陶瓷和钛、陶瓷和铁、陶瓷和铜)组成的FGM圆柱壳的径厚比(R/h)、梯度指数(k)、环向模态数(m)、轴向模态数(n)等对临界荷载的影响。

1. FGM圆柱壳的屈曲控制方程

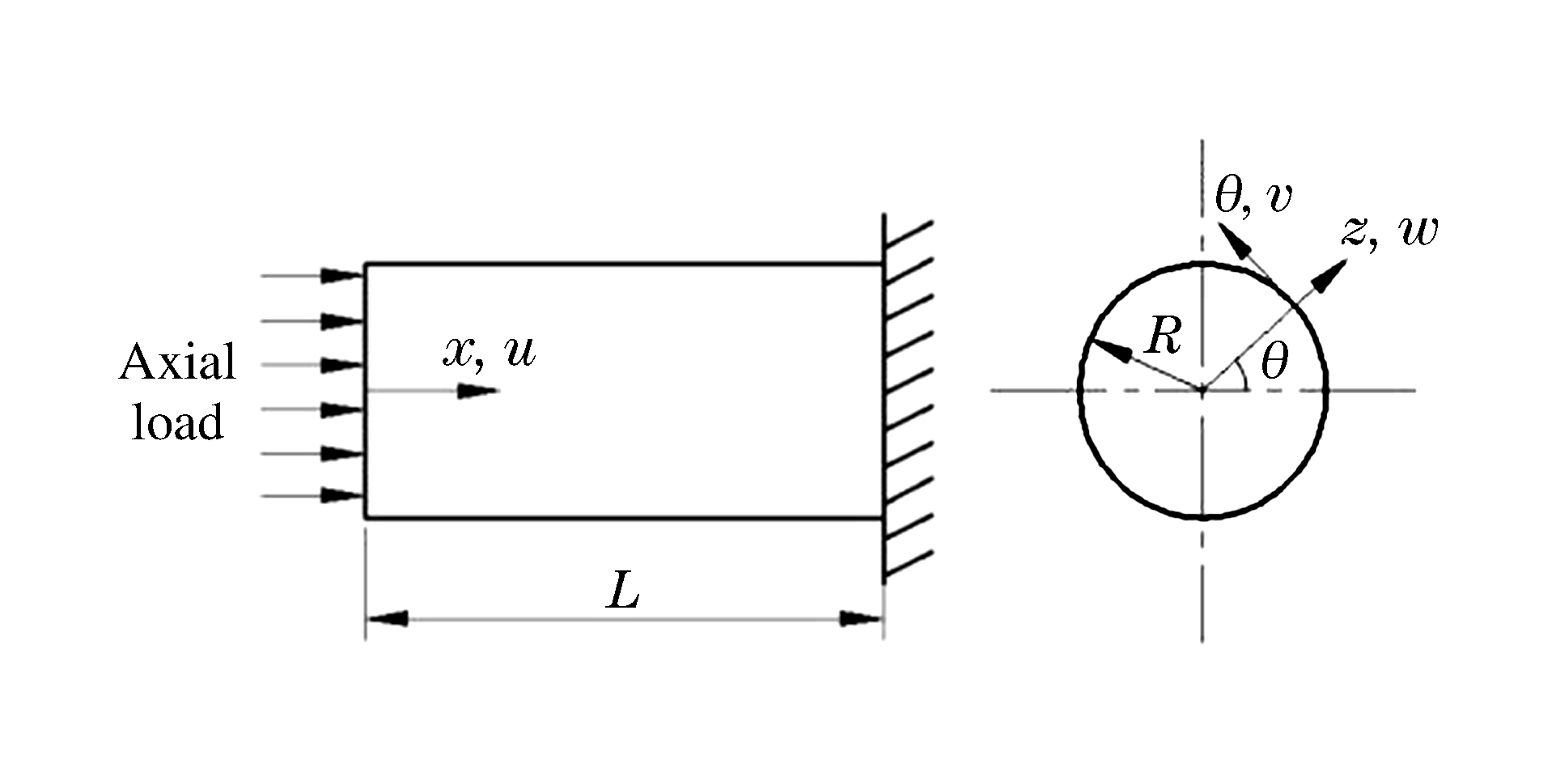
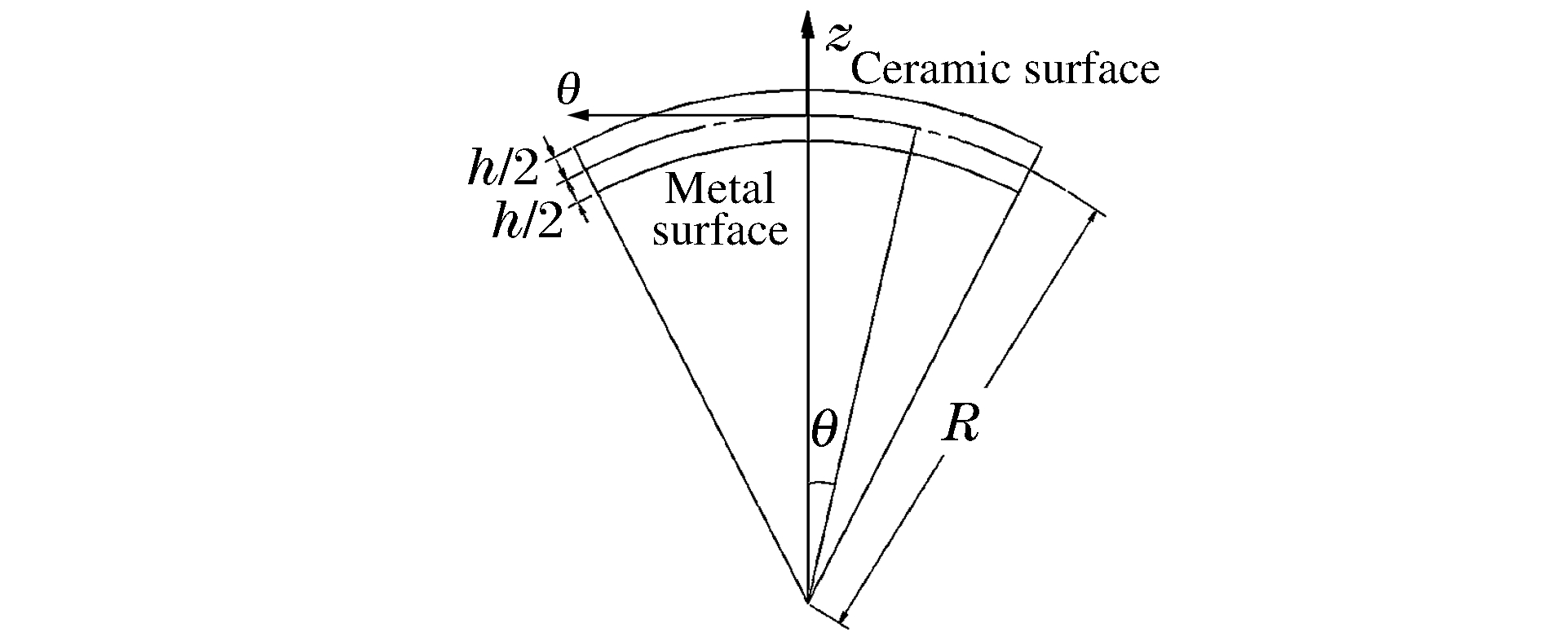
如图 1所示，FGM圆柱壳长度为l，半径为R，总厚度为h，选取柱坐标系(x, θ, z)，其相应位移为(u, v, w)。FGM的材料属性(弹性模量E、密度ρ、泊松比μ等)呈幂律分布^[2-3]，表示为

$P\left( z \right) = \left( {{P_1} - {P_2}} \right){\left( {\frac{{2z + h}}{{2h}}} \right)^k} + {P_2}$

(1)

图 1 圆柱壳坐标系统

Figure 1. Cylindrical shell coordinates

下载: 全尺寸图片幻灯片

式中：P为物性参数，下标“1”和“2”分别代表组分1和组分2；k为梯度指数，k∈(0, ∞)。圆柱壳内任意点的物性参数为

$\left\{ \begin{array}{l} E\left( z \right) = \left( {{E_1} - {E_2}} \right){\left( {\frac{{2z + h}}{{2h}}} \right)^k} + {E_2}\\ \rho \left( z \right) = \left( {{\rho _1} - {\rho _2}} \right){\left( {\frac{{2z + h}}{{2h}}} \right)^k} + {\rho _2}\\ \mu \left( z \right) = \left( {{\mu _1} - {\mu _2}} \right){\left( {\frac{{2z + h}}{{2h}}} \right)^k} + {\mu _2} \end{array} \right.$

(2)

根据Donnell壳体理论，圆柱壳的小挠度几何方程为

$\begin{array}{*{20}{c}} {\left\{ \begin{array}{l} {\varepsilon _x} = \varepsilon _x^0 + z{K_x}\\ {\varepsilon _\theta } = \varepsilon _\theta ^0 + z{K_\theta }\\ {\gamma _{x\theta }} = \gamma _{x\theta }^0 + z{K_{x\theta }} \end{array} \right.,}&{\left\{ \begin{array}{l} u = {u_0} - z\frac{{\partial w}}{{\partial x}}\\ v = {v_0} - z\frac{{\partial w}}{{R\partial \theta }}\\ w = {w_0} \end{array} \right.,}&{\left\{ \begin{array}{l} \varepsilon _x^0 = \frac{{\partial {u_0}}}{{\partial x}}\\ \varepsilon _\theta ^0 = \frac{{\partial {v_0}}}{{R\partial \theta }} - \frac{{{w_0}}}{R}\\ \gamma _{x\theta }^0 = \frac{{\partial {u_0}}}{{R\partial \theta }} - \frac{{\partial {v_0}}}{{\partial x}} \end{array} \right.,}&{\left\{ \begin{array}{l} {K_x} = - \frac{{{\partial ^2}{w_0}}}{{\partial {x^2}}}\\ {K_\theta }{\rm{ = }} - \frac{{{\partial ^2}{w_0}}}{{{R^2}\partial {\theta ^2}}}\\ {K_{x\theta }}{\rm{ = }} - \frac{{2{\partial ^2}{w_0}}}{{R\partial x\partial \theta }} \end{array} \right.} \end{array}$

(3)

式中：ε为正应变，γ为切应变，上、下标“0”表示壳体中面，K为壳体曲率。

根据经典板壳理论，FGM圆柱壳的内力N与内力矩M可表示为

$\left( {\begin{array}{*{20}{c}} {{N_x}}\\ {{N_\theta }}\\ {{N_{x\theta }}}\\ {{M_x}}\\ {{M_\theta }}\\ {{M_{x\theta }}} \end{array}} \right) = \left( {\begin{array}{*{20}{c}} {{A_{11}}}&{{A_{12}}}&{{A_{16}}}&{{B_{11}}}&{{B_{12}}}&{{B_{16}}}\\ {{A_{21}}}&{{A_{22}}}&{{A_{26}}}&{{B_{21}}}&{{B_{22}}}&{{B_{26}}}\\ {{A_{16}}}&{{A_{26}}}&{{A_{66}}}&{{B_{16}}}&{{B_{26}}}&{{B_{66}}}\\ {{B_{11}}}&{{B_{12}}}&{{B_{16}}}&{{D_{11}}}&{{D_{12}}}&{{D_{16}}}\\ {{B_{21}}}&{{B_{22}}}&{{B_{26}}}&{{D_{21}}}&{{D_{22}}}&{{D_{26}}}\\ {{B_{16}}}&{{B_{26}}}&{{B_{66}}}&{{D_{16}}}&{{D_{26}}}&{{D_{66}}} \end{array}} \right)\left( {\begin{array}{*{20}{c}} {\varepsilon _x^0}\\ {\varepsilon _\theta ^0}\\ {\gamma _{x\theta }^0}\\ {{K_x}}\\ {{K_\theta }}\\ {{K_{x\theta }}} \end{array}} \right)$

(4)

式中：A_ij、B_ij、D_ij(i, j=1, 2, 6)分别为FGM圆柱壳的拉伸刚度、耦合刚度和弯曲刚度系数矩阵分量。 ${A_{11}} = {A_{22}} = \int_{ - h/2}^{h/2} {\frac{{E\left( z \right)}}{{1 - {\mu ^2}\left( z \right)}}} {\rm{d}}z$ , ${A_{12}} = {A_{21}} = \int_{ - h/2}^{h/2} {\frac{{\mu \left( z \right)E\left( z \right)}}{{1 - {\mu ^2}\left( z \right)}}} {\rm{d}}z$ , ${A_{66}} = \int_{ - h/2}^{h/2} {\frac{{E\left( z \right)}}{{2\left[ {1 + \mu \left( z \right)} \right]}}} {\rm{d}}z$ , ${B_{11}} = {B_{22}} = \int_{ - h/2}^{h/2} {\frac{{E\left( z \right)}}{{1 - {\mu ^2}\left( z \right)}}z\;}{\rm{d}}z$ , ${B_{12}} = {B_{21}} = \int_{ - h/2}^{h/2} {\frac{{\mu \left( z \right)E\left( z \right)}}{{1 - {\mu ^2}\left( z \right)}}z\;} {\rm{d}}z$ , ${B_{66}} = \int_{ - h/2}^{h/2} {\frac{{E\left( z \right)}}{{2\left[ {1 + \mu \left( z \right)} \right]}}z\;} {\rm{d}}z$ , ${D_{11}} = {D_{22}} = \int_{ - h/2}^{h/2} {\frac{{E\left( z \right)}}{{1 - {\mu ^2}\left( z \right)}}{z^2}\;} {\rm{d}}z$ , ${D_{12}} = {D_{21}} = \int_{ - h/2}^{h/2} {\frac{{\mu \left( z \right)E\left( z \right)}}{{1 - {\mu ^2}\left( z \right)}}{z^2}\;} {\rm{d}}z$ , ${D_{66}} = \int_{ - h/2}^{h/2} {\frac{{E\left( z \right)}}{{2\left[ {1 + \mu \left( z \right)} \right]}}{z^2}\;} {\rm{d}}z$ 。FGM圆柱壳的力学性能为各向同性^[15]，那么：A₁₆=A₂₆=B₁₆=B₂₆=D₁₆=D₂₆=0。

对于圆柱壳，系统的应变能(不考虑剪力)为

$U = \frac{1}{2}\int_0^{2{\rm{ \mathsf{ π} }}} {\int_0^l {\left( {{N_x}\varepsilon _x^0 + {N_\theta }\varepsilon _\theta ^0 + {N_{x\theta }}\gamma _{x\theta }^0 + {M_x}{K_x} + {M_\theta }{K_\theta } + {M_{x\theta }}{K_{x\theta }}} \right)} R{\rm{d}}x{\rm{d}}\theta }$

(5)

动能为

$T = \frac{1}{2}\int_{ - h/2}^{h/2} {\int_0^{2{\rm{ \mathsf{ π} }}} {\int_0^l {\rho \left( z \right)\left[ {{{\left( {\frac{{\partial u}}{{\partial t}}} \right)}^2} + {{\left( {\frac{{\partial v}}{{\partial t}}} \right)}^2} + {{\left( {\frac{{\partial w}}{{\partial t}}} \right)}^2}} \right]R{\rm{d}}x{\rm{d}}\theta {\rm{d}}z} } }$

(6)

外力功为

$W = \frac{1}{2}\int_0^{2{\rm{ \mathsf{ π} }}} {\int_0^l {N\left( t \right){{\left( {\frac{{\partial {w_0}}}{{\partial x}}} \right)}^2}} R{\rm{d}}x{\rm{d}}\theta }$

(7)

Hamilton变分原理为

${\rm{ \mathsf{ δ} }}\int_{{t_2}}^{{t_1}} {\left( {T - U + W} \right){\rm{d}}t} = 0$

(8)

将(3)式~(7)式代入(8)式中，由Donnell壳体理论可知，圆柱壳内力沿环向均匀分布，忽略中面位移^[16]，由u₀、v₀、w₀的变分系数为零，整理得到FGM圆柱壳的动力屈曲控制方程为

$\begin{array}{l} 4{I_0}\frac{{{\partial ^2}{w_0}}}{{\partial t}} - {I_2}\left( {\frac{{{\partial ^4}{w_0}}}{{{R^2}\partial {\theta ^2}\partial {t^2}}} + \frac{{{\partial ^4}{w_0}}}{{\partial {x^2}\partial {t^2}}}} \right) = - 4{A_{22}}\frac{{{w_0}}}{{{R^2}}} - 4\frac{{{B_{12}}}}{{{R^2}}}\frac{{{\partial ^2}{w_0}}}{{\partial {x^2}}} - 4\frac{{{B_{22}}}}{R}\frac{{{\partial ^2}{w_0}}}{{{R^2}\partial {\theta ^2}}} - {D_{11}}\frac{{{\partial ^4}{w_0}}}{{\partial {x^4}}} - \\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;2\left( {{D_{12}} + 2{D_{66}}} \right)\frac{{{\partial ^4}{w_0}}}{{{R^2}\partial {x^2}\partial {\theta ^2}}} - {D_{22}}\frac{{{\partial ^4}{w_0}}}{{{R^4}\partial {\theta ^4}}} + N\left( t \right)\frac{{{\partial ^2}{w_0}}}{{\partial {x^2}}} \end{array}$

(9)

2. 控制方程的求解

设径向位移表示为^[17]

$w = Y\left( x \right)T\left( t \right){{\rm{e}}^{{\rm{i}}m\theta }}$

(10)

将(10)式代入(9)式中，分离变量得

$\left\{ \begin{array}{l} {Y^{\left( 4 \right)}} = {\alpha ^2}Y'' + {\beta ^2}Y = 0\\ \ddot T - \lambda T = 0 \end{array} \right.$

(11)

其中

${\alpha ^2} = \frac{1}{{{D_{11}}}}\left[ {4\frac{{{B_{12}}}}{{{R^2}}} + \frac{2}{{{R^2}}}\left( {{D_{12}} + 2{D_{66}}} \right){m^2} - N\left( t \right) - {I_2}\lambda } \right]$

(12)

${\beta ^2} = \frac{1}{{{D_{11}}}}\left[ {4\frac{{{A_{22}}}}{{{R^2}}} + 4\frac{{{B_{22}}}}{{{R^3}}} + \frac{{{D_{22}}}}{{{R^4}}}{m^4} + 4{I_0}\lambda - \frac{{{I_2}}}{{{R^2}}}{m^2}\lambda } \right]$

(13)

当α⁴＞4β²＞0且λ＞0时，圆柱壳屈曲^[18-20]，其动力屈曲解为

$Y\left( x \right) = {C_1}\sin \left( {{k_1}x} \right) + {C_2}\cos \left( {{k_1}x} \right) + {C_3}\sin \left( {{k_2}x} \right) + {C_4}\cos \left( {{k_2}x} \right)$

(14)

式中：C₁~C₄为系数， ${k_1} = \sqrt {\frac{{{\alpha ^2} - \sqrt {{\alpha ^4} - 4{\beta ^2}} }}{2}} , {k_2} = \sqrt {\frac{{{\alpha ^2} + \sqrt {{\alpha ^4} - 4{\beta ^2}} }}{2}}$ 。

(14)式满足下列两种边界条件：

(1) 对于一端夹支另一端固支的圆柱壳，其边界条件为

$\left\{ \begin{array}{l} Y\left( 0 \right) = Y'\left( 0 \right) = 0\\ Y\left( l \right) = Y'\left( l \right) = 0 \end{array} \right.$

(15)

(2) 对于一端简支另一端固支的圆柱壳，其边界条件为

$\left\{ \begin{array}{l} Y\left( 0 \right) = Y''\left( 0 \right) = 0\\ Y\left( l \right) = Y'\left( l \right) = 0 \end{array} \right.$

(16)

将(14)式代入(15)式中，整理得到如下齐次线性方程组

$\left( {\begin{array}{*{20}{c}} 0&1&0&1\\ {{k_1}}&0&{{k_2}}&0\\ {\sin \left( {{k_1}l} \right)}&{\cos \left( {{k_1}l} \right)}&{\sin \left( {{k_2}l} \right)}&{\cos \left( {{k_2}l} \right)}\\ {{k_1}\cos \left( {{k_1}l} \right)}&{ - {k_1}\sin \left( {{k_1}l} \right)}&{{k_2}\cos \left( {{k_2}l} \right)}&{ - {k_2}\sin \left( {{k_2}l} \right)} \end{array}} \right)\left( {\begin{array}{*{20}{c}} {{C_1}}\\ {{C_2}}\\ {{C_3}}\\ {{C_4}} \end{array}} \right) = 0$

(17)

若要(17)式有非平凡解，其系数行列式必为零，于是

$2{k_1}{k_2} - 2{k_1}{k_2}\cos \left( {{k_1}l} \right)\cos \left( {{k_2}l} \right) - \left( {k_1^2 + k_2^2} \right)\sin \left( {{k_1}l} \right)\sin \left( {{k_2}l} \right) = 0$

(18)

由k₁和k₂可得

$\left\{ \begin{array}{l} k_1^2 + k_2^2 = \left( {n_1^2 + n_2^2} \right){{\rm{ \mathsf{ π} }}^2}/{l^2} = {\alpha ^2}\\ k_1^2k_2^2 = n_1^2n_2^2{{\rm{ \mathsf{ π} }}^4}/{l^4} = {\beta ^2} \end{array} \right.$

(19)

将(19)式代入(12)式和(13)式中，得一端夹支另一端固支时FGM圆柱壳动力屈曲临界荷载N_cr，即

${N_{{\rm{cr}}}} = \frac{{{D_{11}}{{\rm{ \mathsf{ π} }}^2}\left( {n_1^2 + n_2^2} \right)}}{{{l^2}}} - \frac{{{B_{12}}}}{{{R^2}}} + \frac{{2\left( {{D_{12}} + 2{D_{66}}} \right){m^2}}}{{{R^2}}} + \frac{{{h^2}\left( {{D_{11}}n_1^2n_2^2{{\rm{ \mathsf{ π} }}^4}{R^4} - {A_{22}}{l^4}{R^2} + 4{B_{22}}{m^2}{R^2}{l^4} - {D_{22}}{l^4}{m^4}} \right)}}{{{l^4}{R^2}\left( {{h^2}{m^2} - 12{R^2}} \right)}}$

(20)

式中：n₁=n=1, 2, 3, …；m=1, 2, 3, …；n₂=n+2。

同理可得一端简支另一端固支时FGM圆柱壳动力屈曲临界荷载

(21)

此时，n₁=n=1, 2, 3, …；m=1, 2, 3, …；n₂=n+1。

将FGM退化成金属材料，得到金属材料圆柱壳动力屈曲临界荷载

${N_{{\rm{cr}}}} = \frac{{{D_{11}}{{\rm{ \mathsf{ π} }}^2}\left( {n_1^2 + n_2^2} \right)}}{{{l^2}}} + \frac{{2{D_{12}}{m^2}}}{{{R^2}}} + \frac{{{h^2}\left( {{D_{11}}n_1^2n_2^2{{\rm{ \mathsf{ π} }}^4}{R^4} - {A_{22}}{l^4}{R^2} - {D_{22}}{l^4}{m^4}} \right)}}{{{l^4}{R^2}\left( {{h^2}{m^2} - 12{R^2}} \right)}}$

(22)

(22)式与文献[16]中的表达式相同。

根据(10)式，取一端夹支另一端固支时圆柱壳动力屈曲解的表达式^[16]

$w = T\left( t \right)\left[ {\sin \left( {\frac{{{n_1}{\rm{ \mathsf{ π} }}x}}{l}} \right) - \frac{{{n_1}}}{{{n_2}}}\sin \left( {\frac{{{n_2}{\rm{ \mathsf{ π} }}x}}{l}} \right)} \right]\sin \left( {m\theta } \right)$

(23)

将(23)式代入控制方程(9)式中，计算并化简整理得到临界荷载表达式

${N_{{\rm{cr}}}} = \frac{{\left( {n_1^2 + n_2^2} \right){D_{11}}{{\rm{ \mathsf{ π} }}^2}}}{{{l^2}}} + \frac{{2{m^2}\left( {{D_{12}} + 2{D_{66}}} \right)}}{{{R^2}}}$

(24)

(24)式与不考虑转动惯量时用分离变量得到的结果相同，此时n₁=n=1, 2, 3, …; m=1, 2, 3, …; n₂=n+2。同理可得当边界条件为一端简支另一端固支时的临界荷载表达式，与(24)式相同，此时n₁=n=1, 2, 3, …; m=1, 2, 3, …; n₂=n+1。

3. 算例分析

采用MATLAB软件编程，对FGM圆柱壳动力屈曲临界荷载进行计算。讨论由不同材料(陶瓷-钛、陶瓷-铁、陶瓷-铜)组成的FGM圆柱壳(见图 2)的径厚比(R/h)、梯度指数(k)、环向模态数(m)、轴向模态数(n)对临界荷载N_cr的影响。基本材料参数如表 1所示。

图 2 材料沿壁厚分布

Figure 2. Distribution of material along wall thickness

下载: 全尺寸图片幻灯片

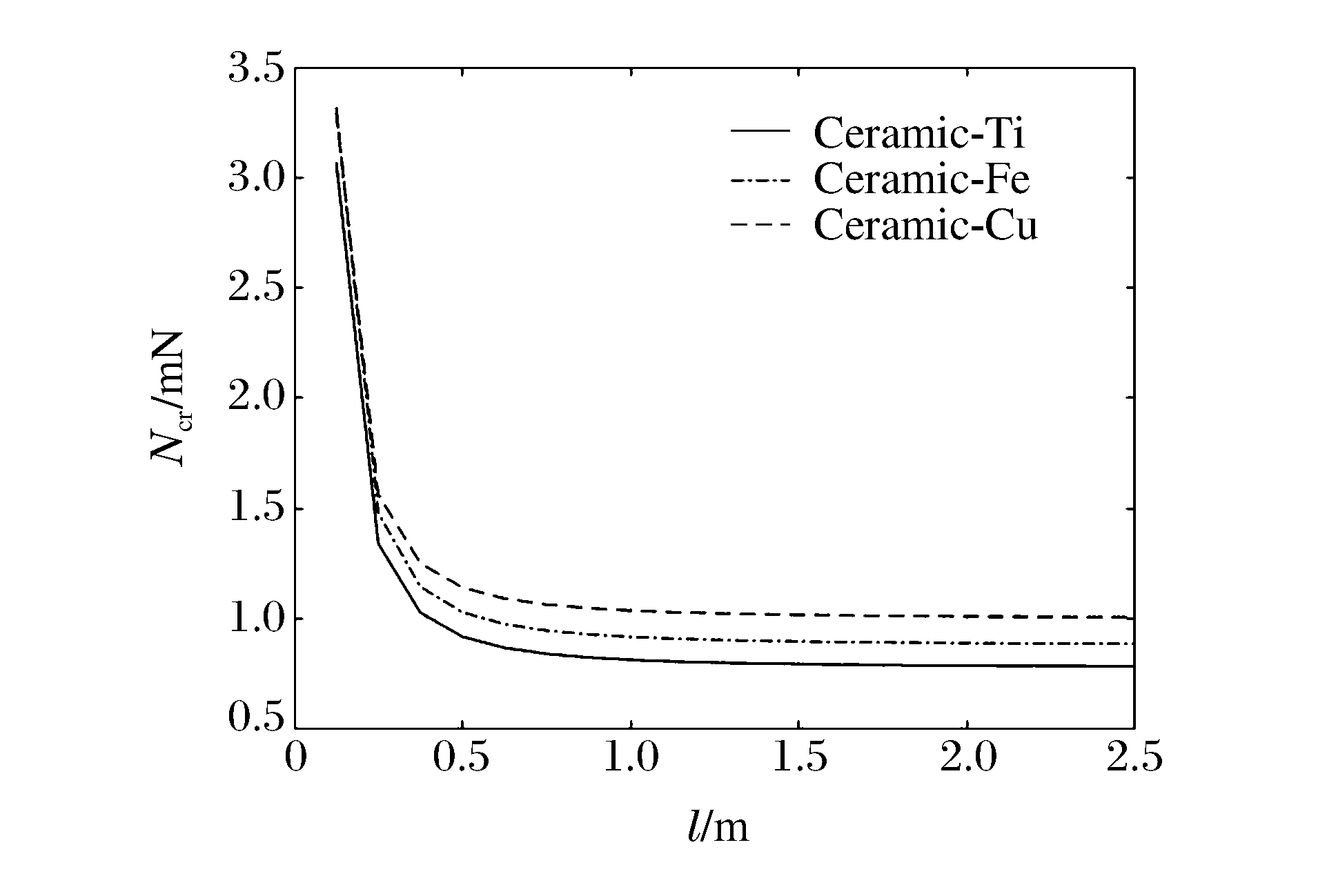
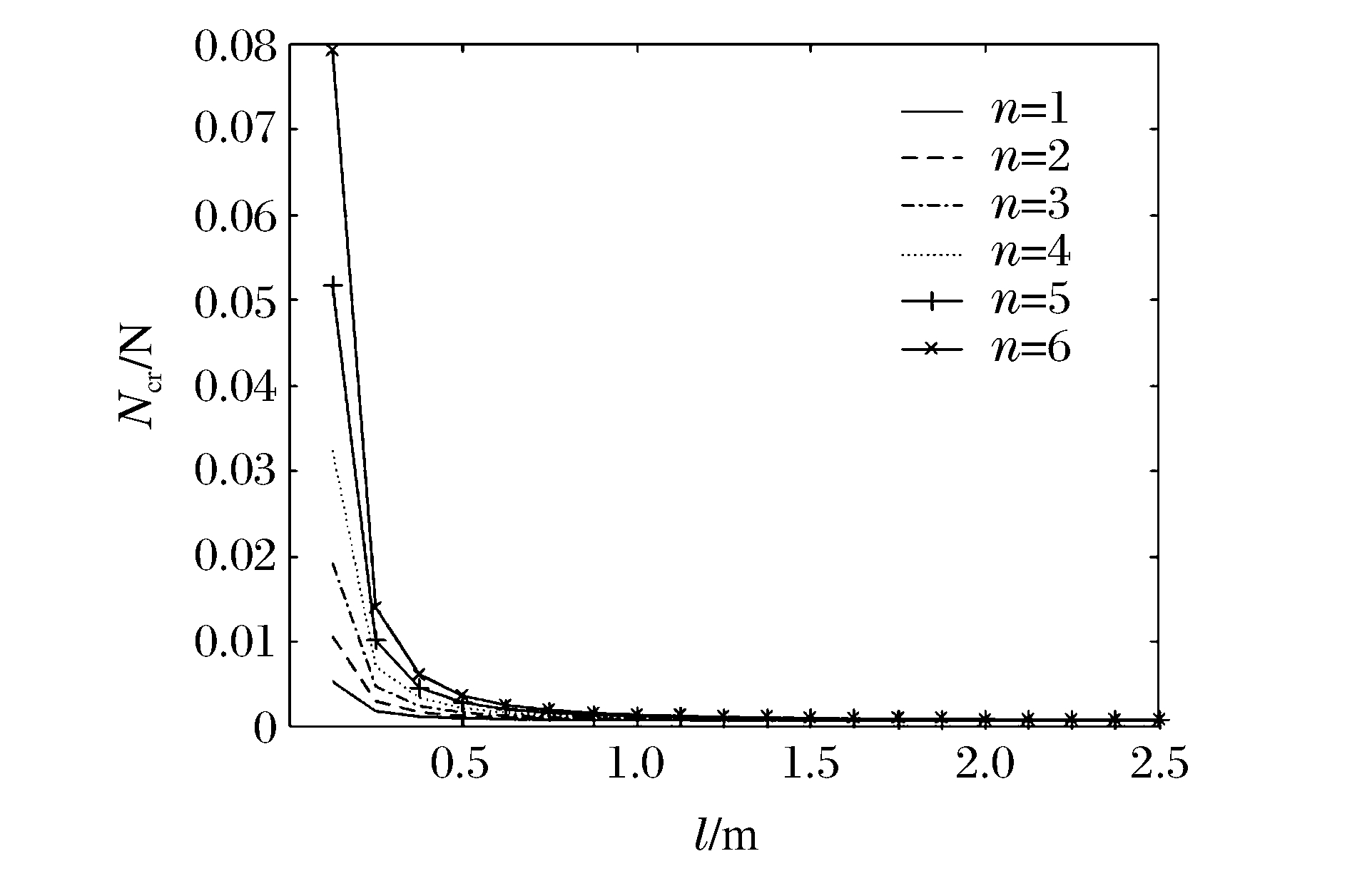
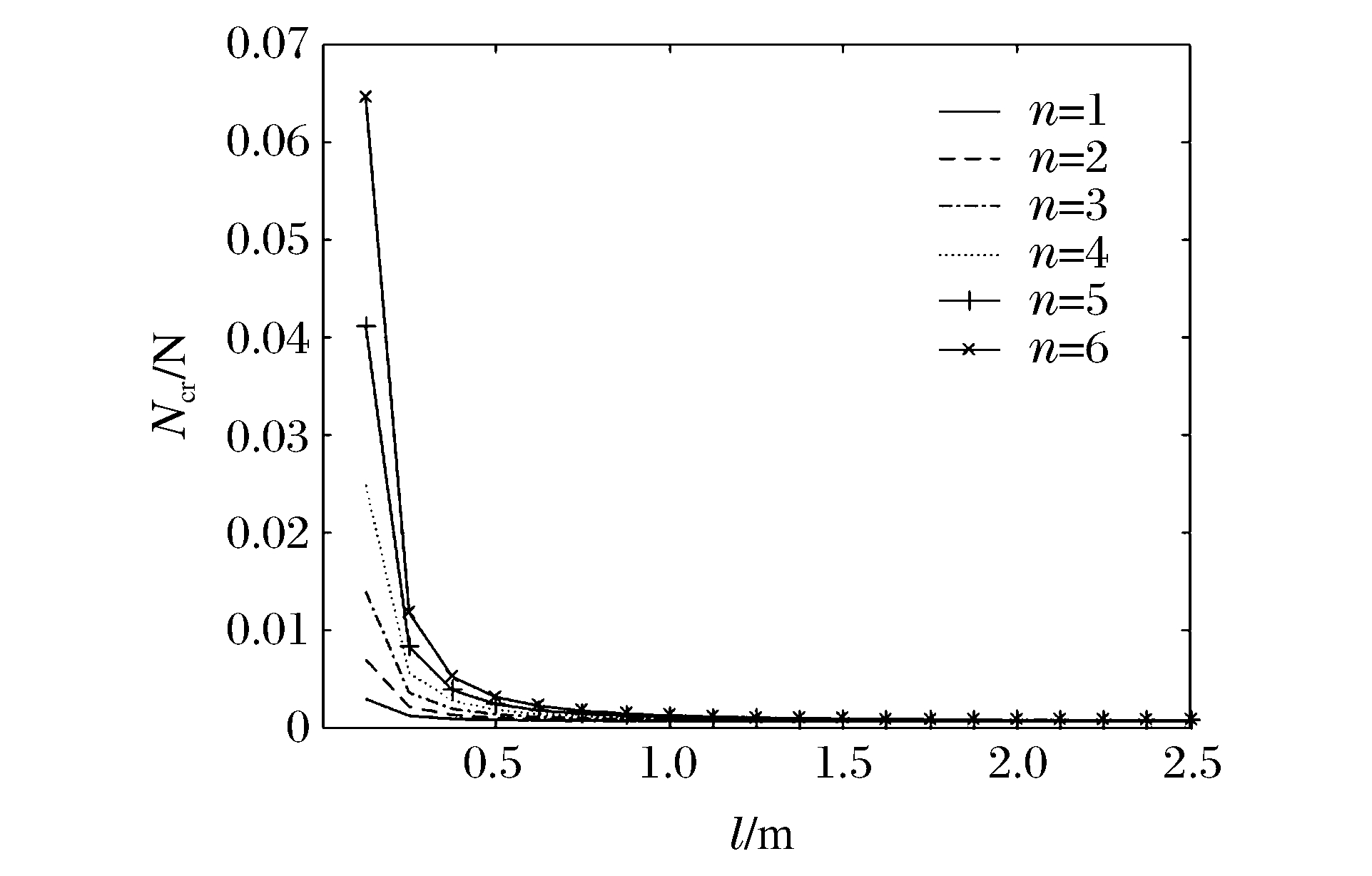
表 1 材料参数

Table 1. Material parameters

Material	E/GPa	ρ/(g·cm^-3)	μ
Ceramic	385	3.96	0.230
Ti	109	4.54	0.410
Fe	155	7.86	0.291
Cu	119	8.96	0.326

下载: 导出CSV

| 显示表格

图 3表示n=1、m=2、k=1、R/h=20时，不同材料组成下N_cr与临界长度l(本研究中临界长度即为圆柱壳长度)的关系曲线。从图 3可以看出：N_cr随l的增加而减小；当l＜0.5 m时，N_cr随l的增加而迅速减小；当l＞0.5 m时，N_cr随l的增大缓慢减小，且逐渐趋于常数；同一l下，陶瓷-铜的N_cr最大，陶瓷-铁次之，陶瓷-钛的N_cr最小。以下均以陶瓷-钛为例进行讨论。

图 3 不同材料组成下临界荷载与临界长度的关系

Figure 3. Critical load vs.critical length under different material composition conditions

下载: 全尺寸图片幻灯片

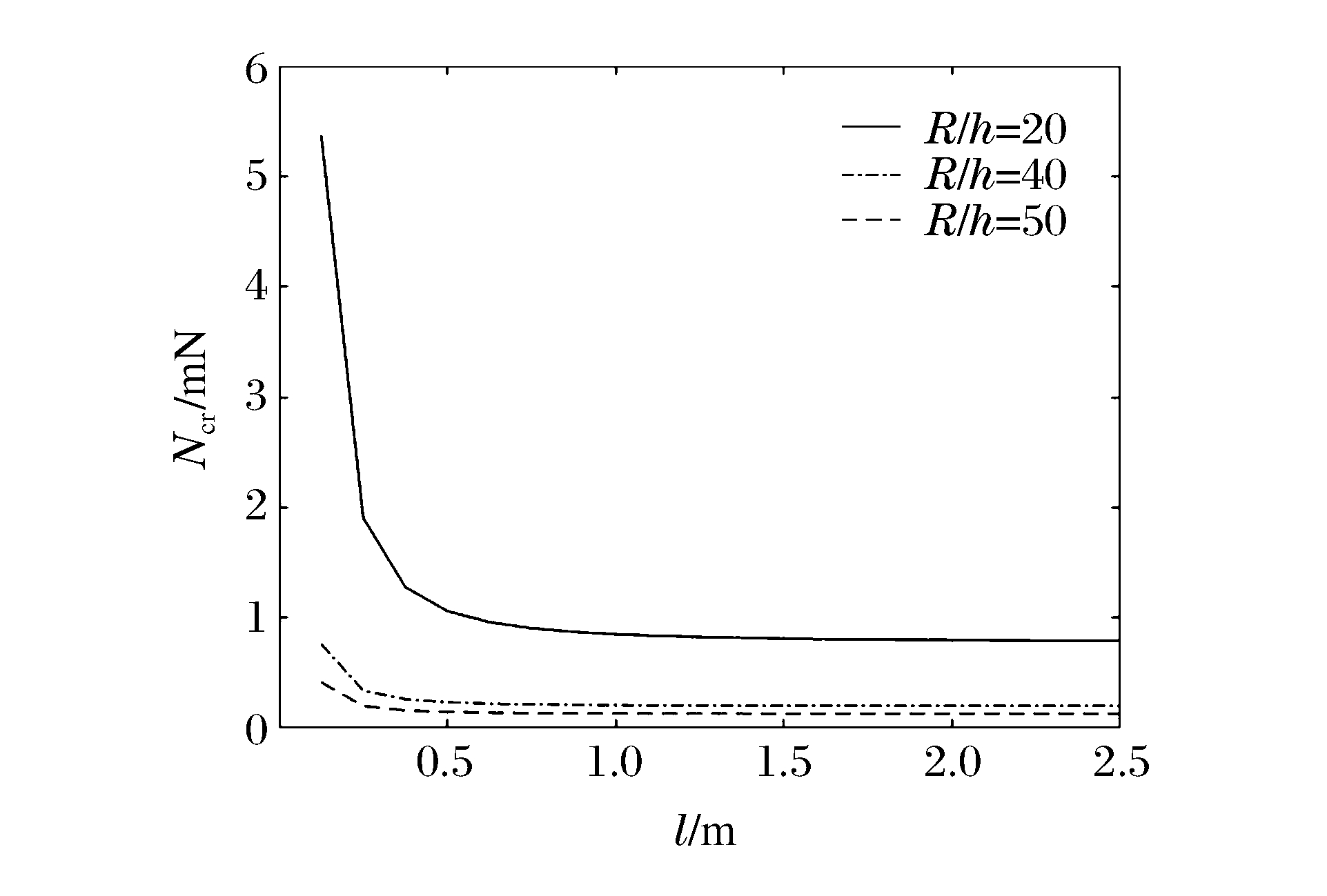
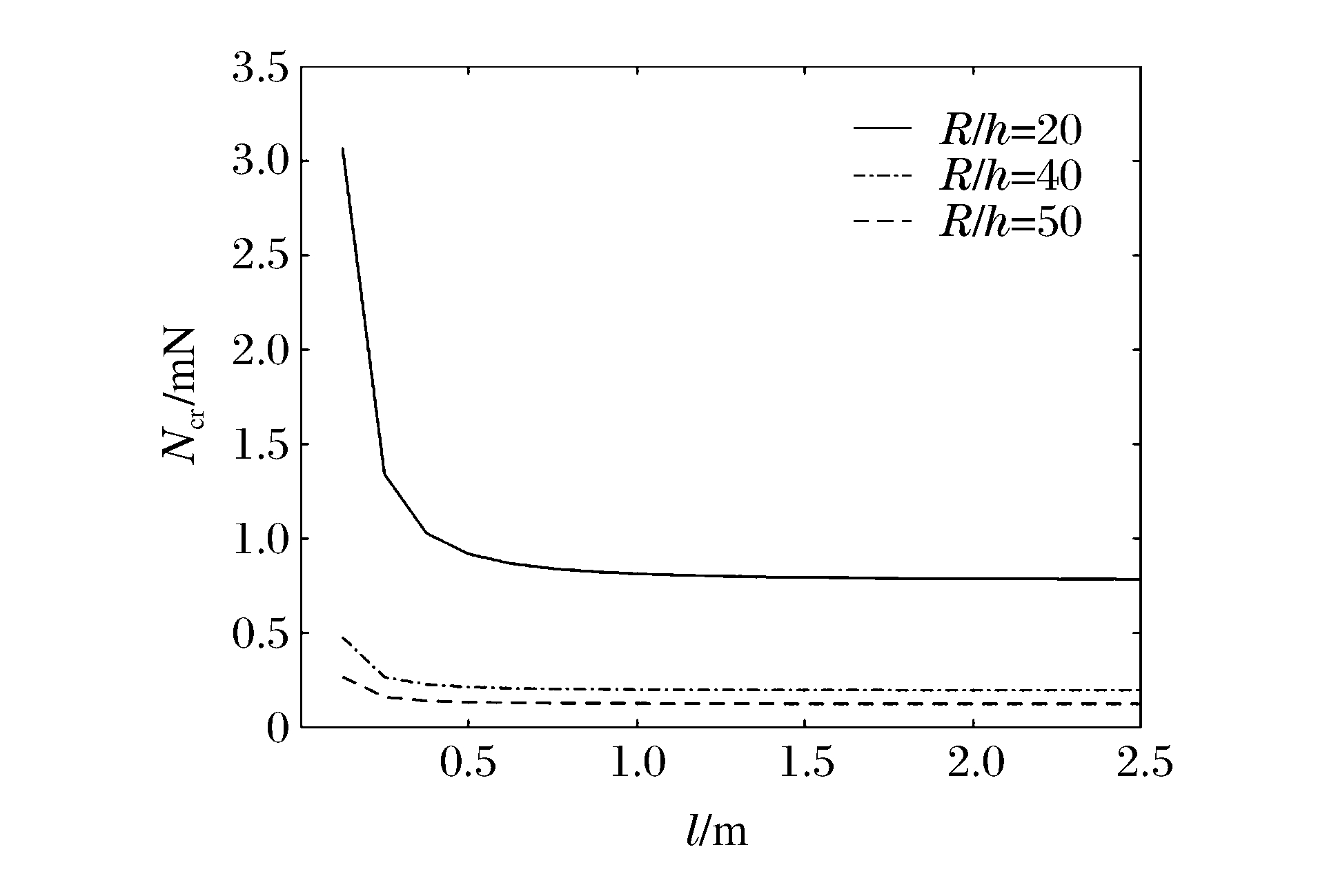
图 4和图 5分别表示冲击端为夹支和简支时n=1、m=2、k=1时不同R/h下N_cr与l的关系曲线。可以看出：当l增加时，N_cr减小，且逐渐趋于常数；在同一l下圆柱壳的N_cr随着R/h的增大而减小；当R/h和l一定时，冲击端为夹支时的N_cr明显比冲击端为简支时的N_cr大，说明约束条件对N_cr有较大影响。

图 4 冲击端为夹支时不同径厚比下临界荷载与临界长度的关系

Figure 4. Critical load vs.critical length under clamped edge and different diameter-thickness ratios conditions

下载: 全尺寸图片幻灯片

图 5 冲击端为简支时不同径厚比下临界荷载与临界长度的关系

Figure 5. Critical load vs.critical length under simple support and different diameter-thickness ratios conditions

下载: 全尺寸图片幻灯片

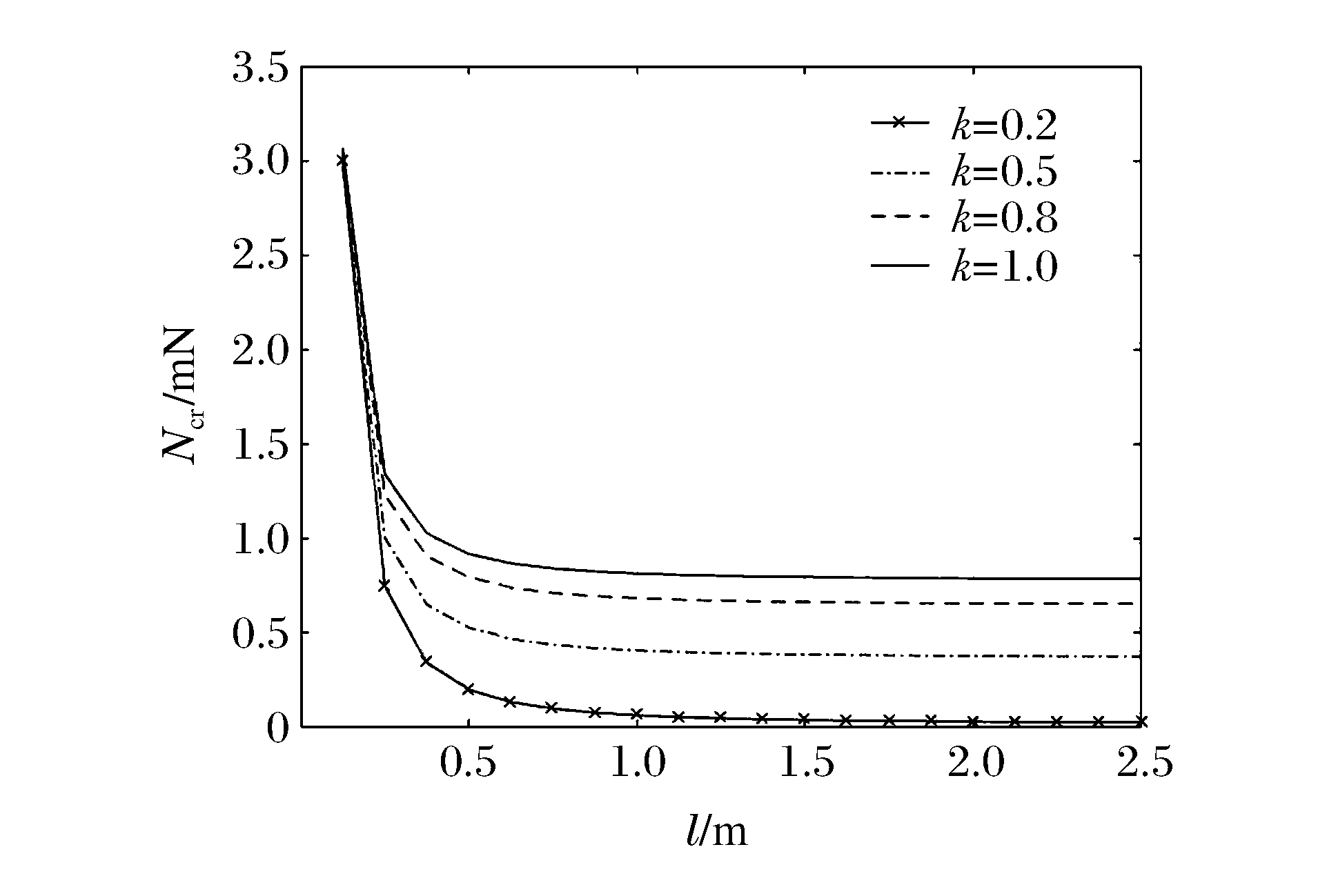
图 6和图 7分别表示冲击端为夹支和简支条件下n=1、m=2、R/h=20时不同k下N_cr与l的关系曲线。可见：FGM圆柱壳的N_cr随着l的增加而减小；在同一l下，FGM圆柱壳的N_cr随着k的增加而增加；当l＜0.5 m时，N_cr随l的增加迅速减小，当l＞0.5 m时，N_cr随l的增加缓慢减小并逐渐趋于常数；当k=1且l一定时，冲击端为夹支时的N_cr明显比冲击端为简支时的N_cr大，再次说明约束条件对N_cr的影响较大。

图 6 冲击端为夹支时不同梯度指数下临界荷载与临界长度的关系

Figure 6. Critical load vs.critical length under clamped edge and different gradient indexes conditions

下载: 全尺寸图片幻灯片

图 7 冲击端为简支时不同梯度指数下临界荷载与临界长度的关系

Figure 7. Critical load vs.critical length under simple support and different gradient indexes conditions

下载: 全尺寸图片幻灯片

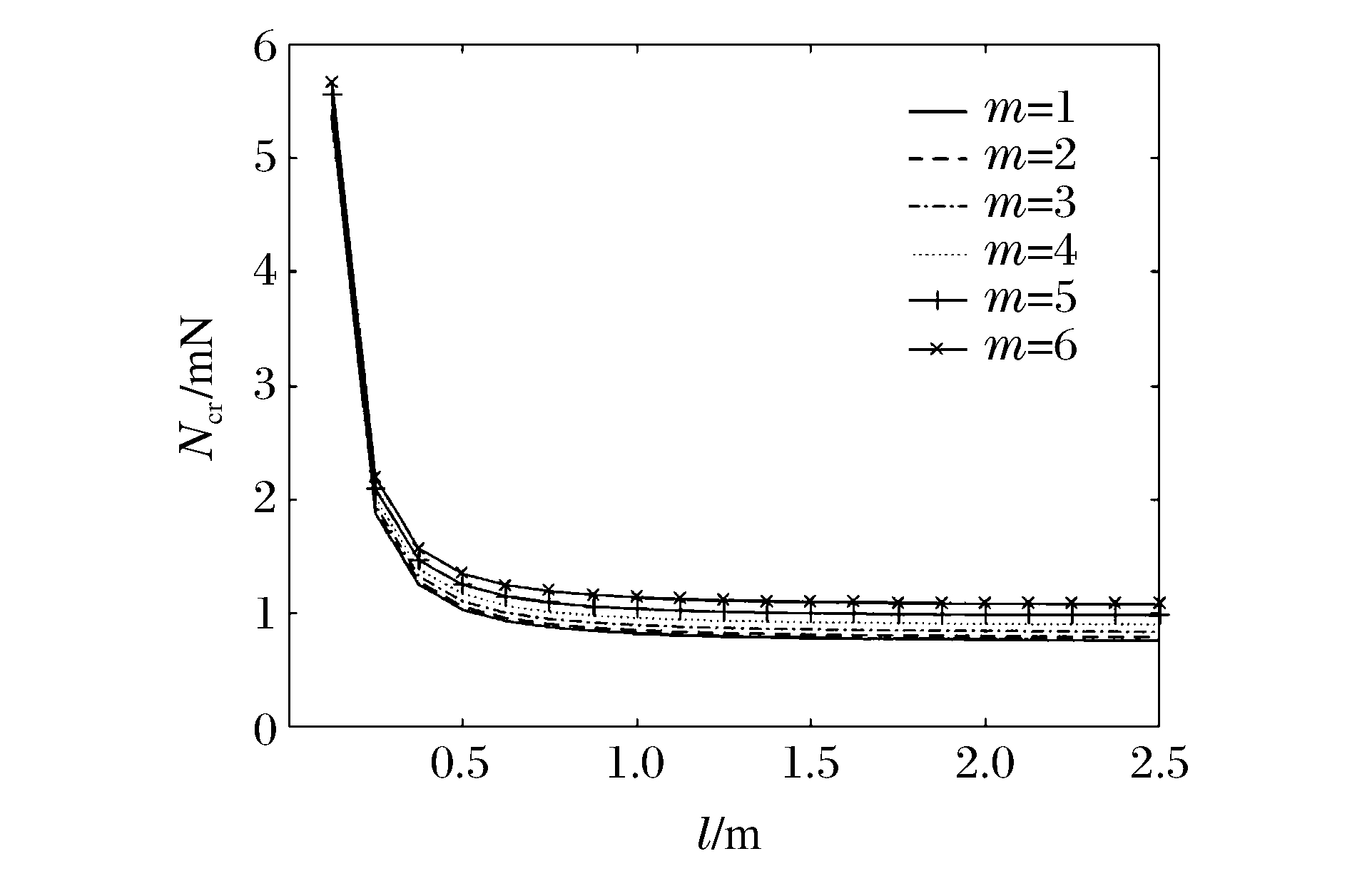
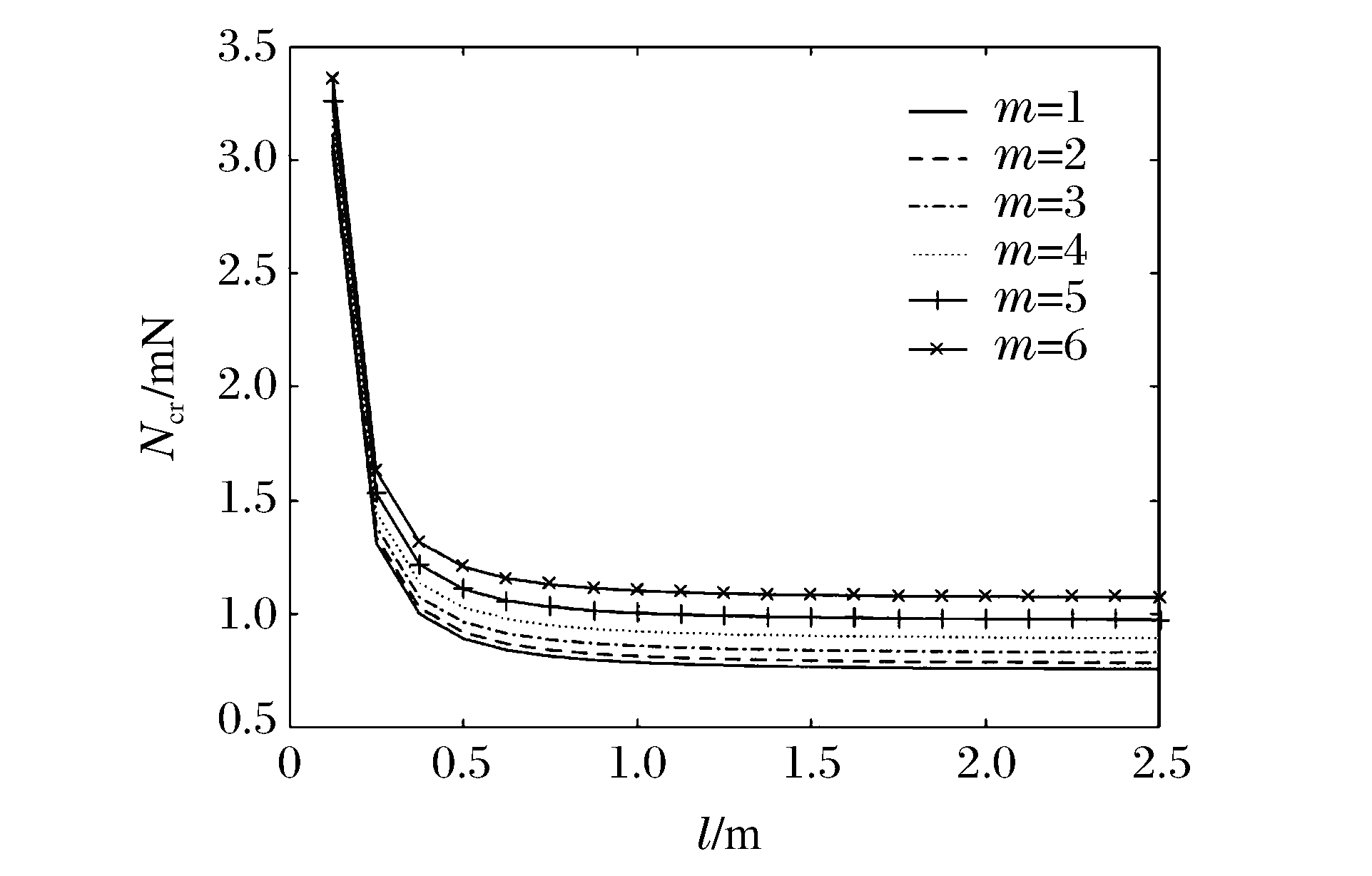
图 8和图 9分别表示冲击端为夹支和简支，n=1、k=1、R/h=20时不同m下N_cr与l的关系曲线。可以看出：当l在一定范围内时，N_cr随l的增加而迅速减小，超出这一范围后N_cr随l的增加而缓慢减小且逐渐趋于常数；同一l下，随着m的增大，FGM圆柱壳的N_cr增大，表明N_cr越大，高阶模态越易被激发。当m=6且l一定时，冲击端为夹支时的N_cr明显比冲击端为简支时的N_cr大，表明约束条件对N_cr有较大影响。

图 8 冲击端为夹支时不同环向模态数下临界荷载与临界长度的关系

Figure 8. Critical load vs.critical length under clamped edge and different circumferential modal number conditions

下载: 全尺寸图片幻灯片

图 9 冲击端为简支时不同环向模态数下临界荷载与临界长度的关系

Figure 9. Critical load vs.critical length under simple support and different circumferential modal number conditions

下载: 全尺寸图片幻灯片

图 10和图 11分别表示冲击端为夹支和简支，m=1、k=1、R/h=20时不同n下N_cr与l的关系曲线。图 10和图 11显示：N_cr随着l的增加而减小；不同n条件下，N_cr随l的增加逐渐趋于同一值；当l＜1 m时，在同一l下N_cr随n的增加而增加，说明N_cr越大，高阶模态越容易被激发。

图 10 冲击端为夹支时不同轴向模态数下临界荷载与临界长度的关系

Figure 10. Critical load vs.critical length under clamped edge and different axial modal number conditions

下载: 全尺寸图片幻灯片

图 11 冲击端为简支时不同轴向模态数下临界荷载与临界长度的关系

Figure 11. Critical load vs.critical length under simple support and different axial modal number conditions

下载: 全尺寸图片幻灯片

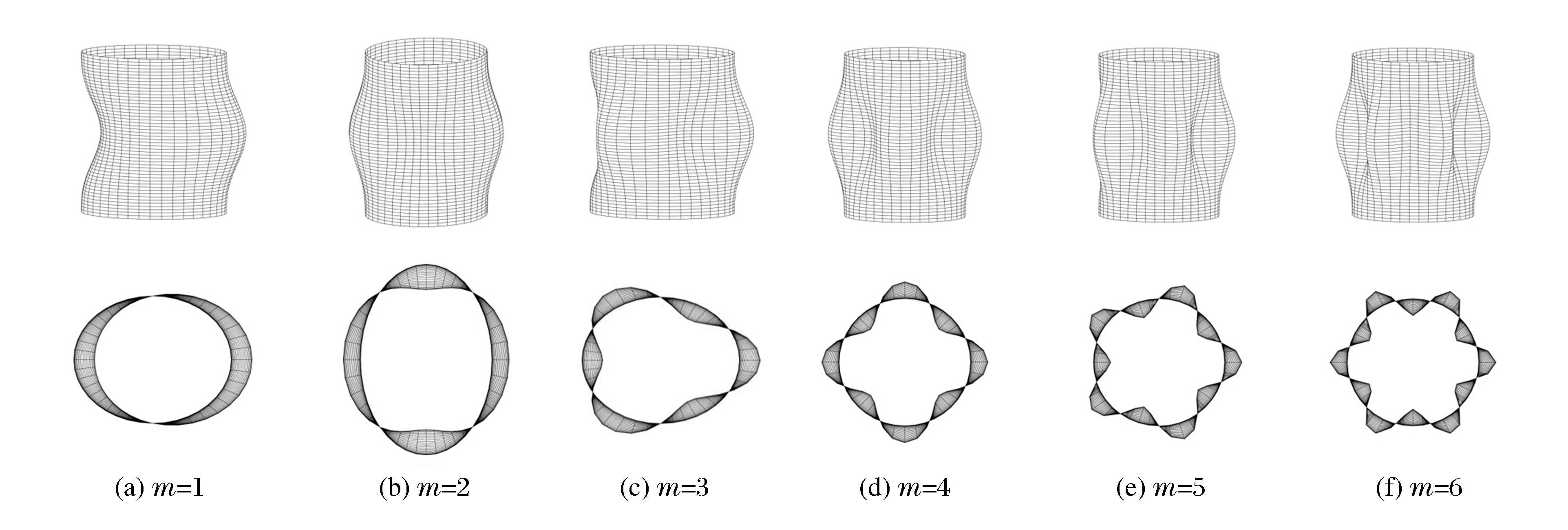
图 12为不同环向模态数m下FGM圆柱壳的动力屈曲模态。可以看出：随着m的增大，圆柱壳的模态变得越来越复杂，俯视图由单一形变为多瓣形；当m=6时，俯视图为12瓣形。

图 12 不同环向屈曲模态图(n=1;m=1, 2, 3, 4, 5, 6)

Figure 12. Different circumferential buckling modes (n=1;m=1, 2, 3, 4, 5, 6)

下载: 全尺寸图片幻灯片

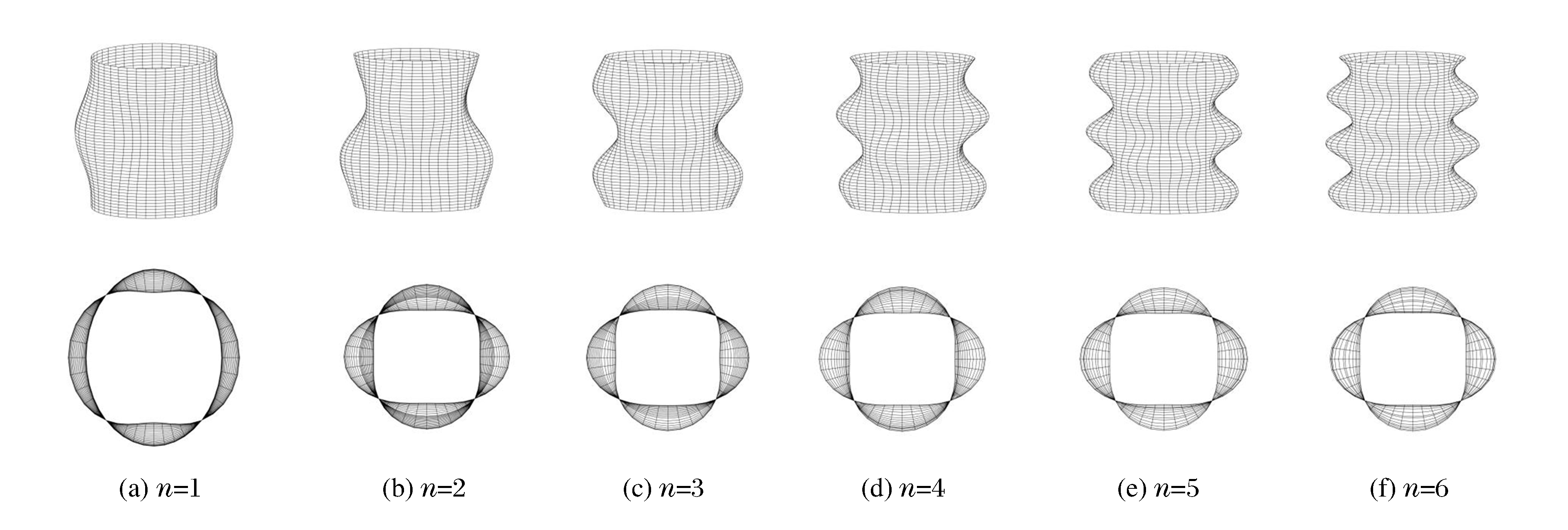
图 13为不同轴向模态数n下FGM圆柱壳的动力屈曲模态图。由图 13可知：随着n的增加，模态图变得越来越复杂。由FGM圆柱壳的俯视图可知，各阶模态数下动力屈曲模态图为轴对称。

图 13 不同轴向屈曲模态图(m=2;n=1, 2, 3, 4, 5, 6)

Figure 13. Different axial buckling modes (m=2;n=1, 2, 3, 4, 5, 6)

下载: 全尺寸图片幻灯片

4. 结论

(1) 根据Donnell壳体理论和经典板壳理论，由Hamilton变分原理得到轴向荷载作用下FGM圆柱壳的动力屈曲控制方程。

(2) 由圆柱壳周向连续性设出径向位移的周向形式，并用分离变量法得到不同约束条件下FGM圆柱壳动力屈曲临界荷载的表达式和屈曲解式。

(3) 利用MATLAB对临界荷载进行计算，得到：在轴向模态数(n)、环向模态数(m)、梯度指数(k)、径厚比(R/h)一定的情况下，同种材料组成的圆柱壳的临界荷载随着临界长度的增加而减小；在n、m、k、l一定的情况下，临界荷载随着径厚比的增大而减小；在n、m、R/h、l一定的情况下，临界荷载随着梯度指数k的增加而增加；不同约束条件下，冲击端为夹支的临界荷载大于冲击端为简支的临界荷载，表明约束条件对临界荷载有较大影响；圆柱壳的临界荷载随模态数的增加而增大，表明临界荷载越大，越容易激发高阶模态；圆柱壳的动力屈曲模态随模态数的增加变得更为复杂。

图 1 不同Ti含量下金刚石-Ti-cBN烧结样品（12 GPa、2000 ℃）的XRD谱

Figure 1. XRD patterns of diamond-Ti-cBN composites sintered at 12 GPa and 2000 ℃ with different contents of Ti

下载: 全尺寸图片幻灯片

图 2 金刚石-Ti-cBN烧结样品断裂面的SEM图像

Figure 2. SEM images of the fracture surfaces of diamond-Ti-cBN sintered composites

下载: 全尺寸图片幻灯片

图 3 BND₂Ti_0.10 的SEM图像以及B、C、N和 Ti 元素的EDS图谱

Figure 3. SEM image and the corresponding EDS mapping of B, C, N, and Ti element for BND₂Ti_0.10 specimen

下载: 全尺寸图片幻灯片

图 4 不同Ti含量的金刚石-Ti-cBN烧结样品的维氏硬度和韧性

Figure 4. Vickers hardness and fracture toughness of diamond-Ti-cBN specimens with different contents of Ti

下载: 全尺寸图片幻灯片

图 5 BND₂Ti_0.10 样品在49 N加载下的压痕SEM图像

Figure 5. SEM images of the Vickers hardness indentation for BND₂Ti_0.10 specimens at the applied load of 49 N

下载: 全尺寸图片幻灯片

图 6 空气氛围下加热至1400 ℃的BND₂Ti_0.10烧结样品的TG-DSC曲线

Figure 6. TG-DSC curve of BND₂Ti_0.10 specimen to 1400 ℃ under air condition

下载: 全尺寸图片幻灯片

参考文献(31)

[1]	VEPŘEK S. The search for novel, superhard materials [J]. Journal of Vacuum Science & Technology A: Vacuum, Surfaces, and Films, 1999, 17(5): 2401–2420.
[2]	IRIFUNE T, KURIO A, SAKAMOTO S, et al. Ultrahard polycrystalline diamond from graphite [J]. Nature, 2003, 421(6923): 599–600. doi: 10.1038/421599b
[3]	MONTEIRO S N, SKURY A L D, DE AZEVEDO M G, et al. Cubic boron nitride competing with diamond as a superhard engineering material: an overview [J]. Journal of Materials Research and Technology, 2013, 2(1): 68–74. doi: 10.1016/j.jmrt.2013.03.004
[4]	SOLOZHENKO V L, ANDRAULT D, FIQUET G, et al. Synthesis of superhard cubic BC₂N [J]. Applied Physics Letters, 2001, 78(10): 1385–1387. doi: 10.1063/1.1337623
[5]	ZHAO Y, HE D W, DAEMEN L L, et al. Superhard B-C-N materials synthesized in nanostructured bulks [J]. Journal of Materials Research, 2002, 17(12): 3139–3145. doi: 10.1557/JMR.2002.0454
[6]	DONG H N, HE D W, DUFFY T S, et al. Elastic moduli and strength of nanocrystalline cubic BC₂N from X-ray diffraction under nonhydrostatic compression [J]. Physical Review B, 2009, 79(1): 014105. doi: 10.1103/PhysRevB.79.014105
[7]	TANG M J, HE D W, WANG W D, et al. Superhard solid solutions of diamond and cubic boron nitride [J]. Scripta Materialia, 2012, 66(10): 781–784. doi: 10.1016/j.scriptamat.2012.02.006
[8]	WANG P, HE D W, WANG L P, et al. Diamond-cBN alloy: a universal cutting material [J]. Applied Physics Letters, 2015, 107(10): 101901. doi: 10.1063/1.4929728
[9]	LIU Y J, HE D W, KOU Z L, et al. Hardness and thermal stability enhancement of polycrystalline diamond compact through additive hexagonal boron nitride [J]. Scripta Materialia, 2018, 149: 1–5. doi: 10.1016/j.scriptamat.2018.01.034
[10]	LI B Z, YING P, GAO Y F, et al. Heterogeneous diamond-cBN composites with superb toughness and hardness [J]. Nano Letters, 2022, 22(12): 4979–4984. doi: 10.1021/acs.nanolett.2c01716
[11]	DRORY M D, DAUSKARDT R H, KANT A, et al. Fracture of synthetic diamond [J]. Journal of Applied Physics, 1995, 78(5): 3083–3088. doi: 10.1063/1.360060
[12]	DUB S, LYTVYN P, STRELCHUK V, et al. Vickers hardness of diamond and cBN single crystals: AFM approach [J]. Crystals, 2017, 7(12): 369. doi: 10.3390/cryst7120369
[13]	TIAN Y J, XU B, YU D L, et al. Ultrahard nanotwinned cubic boron nitride [J]. Nature, 2013, 493(7432): 385–388. doi: 10.1038/nature11728
[14]	HUANG Q, YU D L, XU B, et al. Nanotwinned diamond with unprecedented hardness and stability [J]. Nature, 2014, 510(7504): 250–253. doi: 10.1038/nature13381
[15]	LI J, SHAO G, MA Y, et al. Processing and properties of polycrystalline cubic boron nitride reinforced by SiC whiskers [J]. International Journal of Applied Ceramic Technology, 2019, 16(1): 32–38. doi: 10.1111/ijac.13077
[16]	ZHAO Y S, QIAN J, DAEMEN L L, et al. Enhancement of fracture toughness in nanostructured diamond-SiC composites [J]. Applied Physics Letters, 2004, 84(8): 1356–1358. doi: 10.1063/1.1650556
[17]	HONG S M, AKAISHI M, YAMAOKA S. High-pressure synthesis of heat-resistant diamond composite using a diamond-TiC_0.6 powder mixture [J]. Journal of the American Ceramic Society, 1999, 82(9): 2497–2501. doi: 10.1111/j.1151-2916.1999.tb02109.x
[18]	WANG H K, HE D W, XU C, et al. Nanostructured diamond-TiC composites with high fracture toughness [J]. Journal of Applied Physics, 2013, 113(4): 043505. doi: 10.1063/1.4789004
[19]	ZHOU L, LI Y Y, KOU Z L, et al. Heterogeneous diamond-TiC composites with high fracture toughness and electrical conductivity [J]. Journal of the European Ceramic Society, 2024, 44(8): 4887–4894. doi: 10.1016/J.JEURCERAMSOC.2024.02.042
[20]	LI K, MO P C, CHEN J R, et al. Phase composition, microstructure, and mechanical properties of PcBN composites with Ti and Ti-Al binders: effects of holding time and synthesis pressure [J]. International Journal of Refractory Metals and Hard Materials, 2024, 118: 106434. doi: 10.1016/j.ijrmhm.2023.106434
[21]	WU J H, ZHANG H L, ZHANG Y, et al. The role of Ti coating in enhancing tensile strength of Al/diamond composites [J]. Materials Science and Engineering: A, 2013, 565: 33–37. doi: 10.1016/j.msea.2012.11.124
[22]	SHA X H, YUE W, ZHANG H C, et al. Enhanced oxidation and graphitization resistance of polycrystalline diamond sintered with Ti-coated diamond powders [J]. Journal of Materials Science & Technology, 2020, 43: 64–73. doi: 10.1016/j.jmst.2020.01.031
[23]	SHA X H, FENG B, YUE W, et al. Comparison of tribological behaviors of polycrystalline diamonds synthesized by titanium- and boron-coated diamond particles [J]. Diamond and Related Materials, 2022, 128: 109242. doi: 10.1016/j.diamond.2022.109242
[24]	CHEN Z R, MA D J, WANG S M, et al. Wear resistance and thermal stability enhancement of PDC sintered with Ti-coated diamond and cBN [J]. International Journal of Refractory Metals and Hard Materials, 2020, 92: 105278. doi: 10.1016/j.ijrmhm.2020.105278
[25]	KLIMCZYK P, BENKO E, LAWNICZAK-JABLONSKA K, et al. Cubic boron nitride: Ti/TiN composites: hardness and phase equilibrium as function of temperature [J]. Journal of Alloys and Compounds, 2004, 382(1/2): 195–205. doi: 10.1016/j.jallcom.2004.04.140
[26]	CHEN C, MO P C, CHEN J R, et al. Effects of different binder systems on the reaction mechanism, microstructure and mechanical properties of PcBN composites [J]. Diamond and Related Materials, 2023, 134: 109797. doi: 10.1016/j.diamond.2023.109797
[27]	ARAMIAN A, SADEGHIAN Z, NARIMANI M, et al. A review on the microstructure and properties of TiC and Ti (C,N) based cermets [J]. International Journal of Refractory Metals and Hard Materials, 2023, 115: 106320. doi: 10.1016/J.IJRMHM.2023.106320
[28]	PENG Y, MIAO H Z, PENG Z J. Development of TiCN-based cermets: mechanical properties and wear mechanism [J]. International Journal of Refractory Metals and Hard Materials, 2013, 39: 78–89. doi: 10.1016/j.ijrmhm.2012.07.001
[29]	RITCHIE R O. The conflicts between strength and toughness [J]. Nature Materials, 2011, 10(11): 817–822. doi: 10.1038/nmat3115
[30]	SOLOZHENKO V L, KURAKEVYCH O O, LE GODEC Y. Creation of nanostuctures by extreme conditions: high-pressure synthesis of ultrahard nanocrystalline cubic boron nitride [J]. Advanced Materials, 2012, 24(12): 1540–1544. doi: 10.1002/adma.201104361
[31]	CHEN Z R, MA D J, WANG S M, et al. Enhanced thermal and mechanical performance of polycrystalline diamond compact by introducing polycrystalline cubic boron nitride at the grain boundaries [J]. International Journal of Refractory Metals and Hard Materials, 2021, 96: 105468. doi: 10.1016/j.ijrmhm.2020.105468