〈111〉方向单轴应变下金刚石和硅的广义层错能

黄丽丽; 彭丽; 陈实; 张红平; 李牧

doi:10.11858/gywlxb.20240765

〈111〉方向单轴应变下金刚石和硅的广义层错能

doi: 10.11858/gywlxb.20240765

黄丽丽^1,,
彭丽¹,
陈实¹,
张红平²,
李牧^1,*, ,

1.
深圳技术大学工程物理学院, 超强激光应用技术研究中心, 深圳市超强激光与先进材料重点实验室, 广东深圳　518118
2.
深圳技术大学大数据与互联网学院, 广东深圳　518118

基金项目: 国家自然科学基金（12204317，11974321，11972330）

详细信息

作者简介:
黄丽丽（1989－），女，博士，助理教授，主要从事位错结构的第一性原理计算研究. E-mail：huanglili@sztu.edu.cn

通讯作者:
李　牧（1979－），男，博士，教授，主要从事动高压物理研究. E-mail：limu@sztu.edu.cn

中图分类号: O521.2; O344.1
计量
- 文章访问数: 225
- HTML全文浏览量: 77
- PDF下载量: 28
出版历程
- 收稿日期: 2024-03-28
- 修回日期: 2024-04-16
- 网络出版日期: 2024-05-28
- 刊出日期: 2024-06-03

Generalized Stacking Fault Energies of Diamond and Silicon under ⟨111⟩ Uniaxial Loading

HUANG Lili^1
,,
PENG Li¹,
CHEN Shi¹,
ZHANG Hongping²,
LI Mu^{1,*
, ,}

1.
College of Engineering Physics, Center for Intense Laser Application Technology, Shenzhen Key Laboratory of Ultraintense Laser and Advanced Material Technology, Shenzhen Technology University, Shenzhen 518118, Guangdong, China
2.
College of Big Data and Internet, Shenzhen Technology University, Shenzhen 518118, Guangdong, China

摘要

摘要: 晶体中原子层面剪切所带来的能量称为广义层错能，它是描述晶体中纳米尺度塑性变形的关键参数，如位错分解、成核和孪晶。在冲击加载过程中，弹塑性转变发生在一维弹性应变之后，因此，单轴应变下的广义层错能对于理解塑性流动的发生具有重要意义。应用基于密度泛函理论的第一性原理，计算了在 $\text{[}\text{111}\text{]}$ 方向单轴应变下硅和金刚石的glide (111)面的广义层错能面。基于广义层错能面的平移对称性，通过傅里叶级数展开，拟合得到了广义层错能面的表达式，并给出了 $[\overline{\text{1}}\text{10}\text{]}$ (111)和 $\text{[}\text{11}\overline{\text{2}}\text{]}$ (111)方向的广义层错能曲线。结果表明，随着应变的增加，本征层错能和不稳定层错能出现明显的变化，且不稳定层错能与本征层错能之比减小，说明在 $\left\langle{\text{111}}\right\rangle$ 方向的单轴应变下晶体中的位错不容易发生分解。该结果解释了在四代光源上开展的位错演化动态实验结果，即沿 $\left\langle{\text{111}}\right\rangle$ 方向加载的层错信号出现的速度和强度均远不如沿 $\left\langle{\text{110}}\right\rangle$ 方向和 $\left\langle{\text{100}}\right\rangle$ 方向加载的结果。
- 广义层错能 /
- 第一性原理计算 /
- 冲击加载 /
- 位错
Abstract: The energy caused by atomic level shear in a crystal is called generalized fault energy (GSFE), This is an important material property for describing nanoscale plastic phenomena in crystalline materials, such as dislocation decomposition, nucleation, and twinning. During the shock loading process, the elastoplastic transition occurs after one-dimensional elastic strain, so the generalized stacking fault energy is of great signiﬁcance in understanding the occurrence of plastic ﬂow. Here, we calculate the generalized GSFE surface of glide (111) surface of silicon and diamond under uniaxial strain in [111] direction by using the ﬁrst principles of density functional theory. Based on the translation symmetry of GSFE surface, we ﬁt the GSFE surface expression obtained by Fourier series expansion and the generalized stacking fault energy curves for the $[{\overline{1}10}]$ (111) and $[ 11\overline{2}]$ (111) directions are given. With the increase of strain, the intrinsic fault energy (γ_I) and the unstable fault energy (γ_us) have obvious changes, and the ratio of the unstable stacking fault energy to the intrinsic stacking fault energy (γ_us/γ_I) decreases indicating that dislocations in crystals are not easily decomposed under uniaxial strain in the $\left\langle{111}\right\rangle$ direction. This result explains the results of dynamic experiments of dislocation evolution at four generations of light sources that the speed and strength of fault signals loaded along $\left\langle{111}\right\rangle$ direction are much lower than those loaded along $\left\langle{110}\right\rangle$ direction and $\left\langle{100}\right\rangle$ direction.
- generalized stacking fault energy /
- first principle calculations /
- shock loading /
- dislocation

HTML全文

The corrugated interface between different fluids grow when accelerated from a low-density fluid to a high-density fluid, which is called Rayleigh-Taylor (RT) instability^[1-2].This phenomenon may also occur in metals, but usually under a high pressure and at a high strain-rate, but differs most distinctly from the RT instability in fluids in its strength effect of the metal, which can stabilize the perturbation growth^[3-4] and make the metallic RT instability more complex and difficult.Here, it is also affected by the loading state and the properties of the metallic materials.The metallic RT instability at high pressure and strain-rate can be observed in inertial confinement fusion^[5], supernova explosion^[6], asteroid collision^[7], the motion of earth's inner core and plate tectonics^[8], and so on.Therefore, the metallic RT instability is currently a major concern for researchers and receives a great deal of academic attention.

In theoretical studies about the metallic interface instability, dispersion relations of the perturbation growth are derived mainly based on the energy^[9-11] or force equilibrium^[12-13].However, the previous linear analysis cannot predict the perturbation growth accurately just by applying the perfect plastic constitutive relation and constant pressure loading state.Based on the energy balance, a perturbation growth equation using Steinberg-Guinan (SG) and the Johnson-Cook constitutive models, as well as a variable pressure loading process consistent with experiments, has been derived that precisely predicts the growth of metallic RT instabilities driven by detonation and laser plasma.However, the linear analysis still has its limitations and does not take full account of the loading procedure.

Experimental studies of the metallic RT instability started in the 1970s.The pioneering experimental research^[14] was the perturbation growth of a flat aluminum plate accelerated by the expansion of detonation products, which was observed using a high-energy X-ray facility.What was achieved then inspired researchers, and the similar equipment was utilized in later research^[15-17].In the USA and Russia particularly, numerous numerical simulations and experimental investigations for the metallic RT instability have been carried out, but have mainly concentrated on the perturbation growth and such influencing factors as the initial amplitude, the wavelength and material properties.Igonin and Ignatova et al.^[18-19] experimentally and numerically studied the dynamic behaviors of copper (Cu) and tantalum (Ta) subjected to both shock and shockless loading by employing a perturbation growth method.They observed that the formation of the bi-periodic twin structures resulted in an initial loss of the shear strength of Cu, but failed to observe localization in Ta.Olson et al.^[16] experimentally studied the effects of the grain size and material processing on the RT perturbation growth of Cu.They found that both the single-crystal orientation and the strain hardening due to the material processing can affect the perturbation growth, but the polycrystalline grain size cannot.For the plane detonation, the loading pressure is generally about 30 GPa.To enhance the loading pressure, Henry de Frahan et al.^[17] studied the beryllium RT instability using an iron flyer plate to impact the second high explosive (HE) to raise the pressure to 50 GPa in their experiments, and combined numerical simulations to calibrate the feasibility of different constitutive models.When the sample is driven by electromagnetism^[20-21] or laser^{[4, 22]}, the loading pressure can be further increased.Very extreme conditions of pressures over 1 000 GPa and strain rates of 10⁸ s^-1 have been achieved at the National Ignition Facility, USA, where the RT instability experiment in vanadium was carried out, and constitutive models in solid phase were tested by comparing simulations with experiments measuring the perturbation growth^[23] under the extreme conditions mentioned.

In the metallic interface instability, the perturbation growth is related to and arrested by the material strength.Moreover, some investigations have demonstrated that the material strength increases under these extreme conditions.Results from the metallic RT experiments and computations by Barnes et al.^[14] show that the yield strength of 1100-0 aluminum is over 10 times larger than the standard parameter, and the yield strength of 304 stainless steel also increases by more than three times.Using the SG constitutive strength model, calculations of plasma-driven quasi-isentropic RT experiments of Al-6061-T6 using the Omega laser at a peak drive pressure of 20 GPa indicate that its yield strength is a factor of about 3.6 times over the ambient value^[22].In Park et al.'s^[4] plasma-driven quasi-isentropic polycrystalline vanadium RT experiments using the Omega laser with a peak drive pressure of 100 GPa, the measured RT growth was substantially lower than predictions using the existing constitutive models (SG and Preston-Tonks-Wallace) that work well at low pressures and long timescales.Using the SG model, the simulations agree with the RT experimental data when the initial strength is raised by a factor of 2.3.Therefore, the SG and Preston-Tonks-Wallace models underestimate the strength of vanadium under very high pressures and strain rates.Belof et al.^[24] first measured the dynamic strength of iron undergoing solid-solid phase transition by using RT instability.In conjunction with detailed hydrodynamic simulations, the analysis results revealed significant strength enhancement of the dynamically generated ε-Fe and reverted α′-Fe, comparable in magnitude to the strength of austenitic stainless steels.Therefore, the metallic RT instability was suggested and used as a tool for evaluating the material strength of solids at high pressures and high strain rates^{[3, 25]}, and then for modifying or developing new constitutive models for these conditions^[26-27].

In view of the dominant role of the material strength in metallic interface instabilities, and the limitations of existing constitutive models at high pressures and high strain rates, we aimed to investigate the material strength and its effects on metallic interface instabilities.In this paper, we also conducted an RT instability experiment in explosion-driven aluminum, and measured the perturbation growth using X-ray radiography.In combination with elastic-plastic hydrodynamic simulations, we investigated the dynamic behavior of metallic RT instabilities and the role of the material strength in these.

1. Experimental Setup

Following that of Barnes et al., ^[14]our experiment used the setup as shown in Fig. 1(a), where we have a sketch of the experimental setup consisting of a detonator, a booster, plane wave lens, JO-9159 HE (100 mm in diameter and 50 mm in thickness), an aluminum sample, and a vacuum.Fig. 1(b) shows the experimental sample of aluminum with a diameter of 65 mm and a thickness of 1.5 mm in the central region.An initial sinusoidal perturbation was machined on the side of the aluminum sample facing the HE.The perturbation amplitude and wavelength were 0.25 mm and 6 mm, respectively.The HE products crossed the void of 3.5 mm between the sample and HE and accumulated on the perturbation interface of the sample, providing a smooth rise to peak pressure and a quasi-isentropic drive.Moreover, the void between the sample and HE can ensure that the temperature of the sample at high pressures remain below the melting point^[22].

Figure 1. Sketch of the experimental setup and sample

DownLoad: Full-Size Img PowerPoint

In the experiment, X-ray radiography was used to record the evolution of the perturbation interface from the JO-9159 explosive detonation at zero time.A Doppler pin system was used to measure the history of the free surface velocity, which can be integrated to obtain the corresponding free surface displacement.Fig. 1(c) shows the distribution of measurement points of the free surface velocity, where both points 1 and 2 correspond to the wave trough positions with one wavelength interval, and point 3 corresponds to the wave crest position with 1.5 wavelength intervals from point 2.

2. Numerical Methods

Based on our indoor hydrodynamic code of compressible multi-viscous flow and turbulence (MVFT)^[28-30], we developed a detonation and shock dynamics code with high fidelity by considering the explosive detonation and the elastic-plastic behavior of the material.This code can be used to study the physical problem of multi-materials, large deformation, and strong shock.The governing equations in conserved form are as follows

$\left\{ \begin{array}{l} \frac{\partial }{{\partial t}}\int_V {\rho {\rm{d}}V} =-\oint_S {\rho {u_i}{n_i}{\rm{d}}S} \\ \frac{\partial }{{\partial t}}\int_V {\rho {u_j}{\rm{d}}V} =-\oint_S {P{n_j}{\rm{d}}S}-\oint_S {\rho {u_i}{u_j}{n_i}{\rm{d}}S} + \oint_S {{s_{ij}}{n_i}{\rm{d}}S} \\ \frac{\partial }{{\partial t}}\int_V {\rho E{\rm{d}}V} = - \oint_S {{u_i}P{n_i}{\rm{d}}S} - \oint_S {\rho {u_i}E{n_i}{\rm{d}}S} + \oint_S {{s_{ij}}{u_j}{n_i}{\rm{d}}S} \end{array} \right.$

(1)

where i and j represent the x, y, and z directions; V is the control volume, S the surface of control volume, n the unit vector of the external normal direction, ρ, u_k (where k=i, j), p, and E are the density, velocity, pressure, and total energy of per unit mass; and s_ij the deviation stress tensor.

The physical problem as described by Eq.(1) was decomposed into three one-dimensional problems.For each of them, the physical quantities in a cell were interpolated and reconstructed using a piecewise parabolic method (PPM).The one-dimensional problem was then resolved using a two-step Euler algorithm:First the physical quantities were solved by the Lagrange matching, and then remapped back to the stationary Euler meshes.The effect of material strength, explosive detonation, and artificial viscosity were implemented in the Lagrange step.The multi-material interface was captured by applying a volume-of-fluid (VOF) method.

2.1 Equation of State

In our numerical simulations, the equation of state (EOS) for the explosive and aluminum are the Jones-Wilkins-Lee (JWL) and Mie-Grüneisen equations of state, respectively.The Jones-Wilkins-Lee equation of state is

$p\left( {\rho, T} \right) = A\left( {1-\frac{\omega }{{{R_1}v}}} \right){{\rm{e}}^{-{R_1}v}} + B\left( {1-\frac{\omega }{{{R_2}v}}} \right){{\rm{e}}^{ - {R_2}v}} + \frac{{\omega \bar E}}{v}$

(2)

where v=ρ₀/ρ is specific volume; A, B, R₁, R₂, and ω are constants; and E is the internal energy per unit volume.Table 1 lists the JWL EOS parameters of the JO-9159 explosive.The Mie-Grüneisen equation of state is

Table 1. Equation of state parameters of JO-9159 explosive

ρ/(g·cm^-3)	p_CJ/GPa	D_CJ/(km·s^-1)	A/GPa	B/GPa	R₁	R₂	ω
1.86	36	8.862	934.8	12.7	4.6	1.1	0.37

| Show Table

DownLoad: CSV

$p = \frac{{{\rho _0}{c^2}\mu \left[{1 + \left( {1-{\gamma _0}/2} \right)\mu-a{\mu ^2}/2} \right]}}{{{{\left[{1-\left( {{S_1}-1} \right)\mu-\frac{{{S_2}{\mu ^2}}}{{\mu + 1}} - \frac{{{S_3}{\mu ^3}}}{{{{\left( {\mu + 1} \right)}^2}}}} \right]}^2}}} + \left( {{\gamma _0} + a\mu } \right)\bar E$

(3)

where μ=ρ/ρ₀-1 is the relative compression, ρ₀ the initial density, c the sound velocity at zero pressure, γ₀ the Grüneisen coefficient, and a, S₁, S₂, and S₃ are constants (in Table 2).

Table 2. Mie-Grüneisen equation of state parameters of aluminum

ρ/(g·cm^-3)	c/(km·s^-1)	γ₀	a	S₁	S₂	S₃
2.703	5.22	1.97	0.47	1.37	0	0

| Show Table

DownLoad: CSV

2.2 Constitutive Model

In our simulations, the elastic-plastic behavior of aluminum at high pressures and high strain rates was described using the SG constitutive model.The SG model introduces pressure, temperature, and strain-rate terms into the elastic-plastic constitutive equation, while the coupling effect of pressure and strain rate on flow stress was characterized by the separating variables.Additionally, as the flow stress in the SG model relies on pressure, there is a coupling relationship between the material constitutive equation and the equation of state, which indicates the feature of pressure hardening of metal under high pressure.The dynamic yield strength Y_SG and the shear modulus G determined by the SG model are expressed as

${Y_{{\rm{SG}}}} = {Y_0}{\left[{1 + \beta \left( {{\varepsilon _p} + {\varepsilon _i}} \right)} \right]^n}\left[{1 + Ap{\eta ^{-1/3}}-B\left( {T-300} \right)} \right]$

(4)

$G = {G_0}\left[{1 + Ap{\eta ^{-1/3}}-B\left( {T-300} \right)} \right]$

(5)

where Y₀ and G₀ are the initial yield strength and the shear modulus, respectively; β and n are the material strain hardening coefficient and the hardening index, respectively; A is the pressure hardening coefficient; η=ρ/ρ₀ is the material compression ratio; and B is the temperature softening coefficient (in Table 3).

Table 3. Steinberg-Guinan constitutive model parameters of aluminum

Y₀/GPa	Y_max/GPa	G₀/GPa	β	n	A/GPa^-1	B/(10^-3K^-1)
0.29	0.68	27.6	125	0.1	0.0652	0.616

| Show Table

DownLoad: CSV

3. Results and Discussions

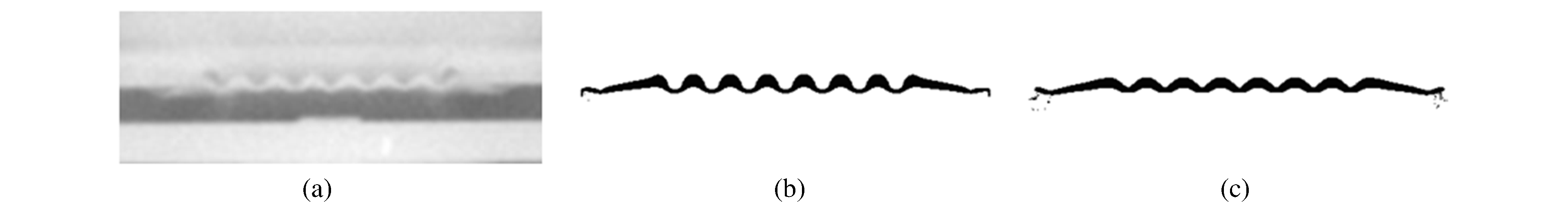
In our experiment, X-ray radiography recorded an image of the perturbed interface at 7.78 μs, as shown in Fig. 2(a), from which we obtained the amplitude of 0.77 mm simultaneously by image processing.In the simulations, the mesh size was 15.6 μm, and Tables 2 and 3 list the parameters of the Mie-Grüneisen EOS and the SG constitutive model of aluminum, respectively.

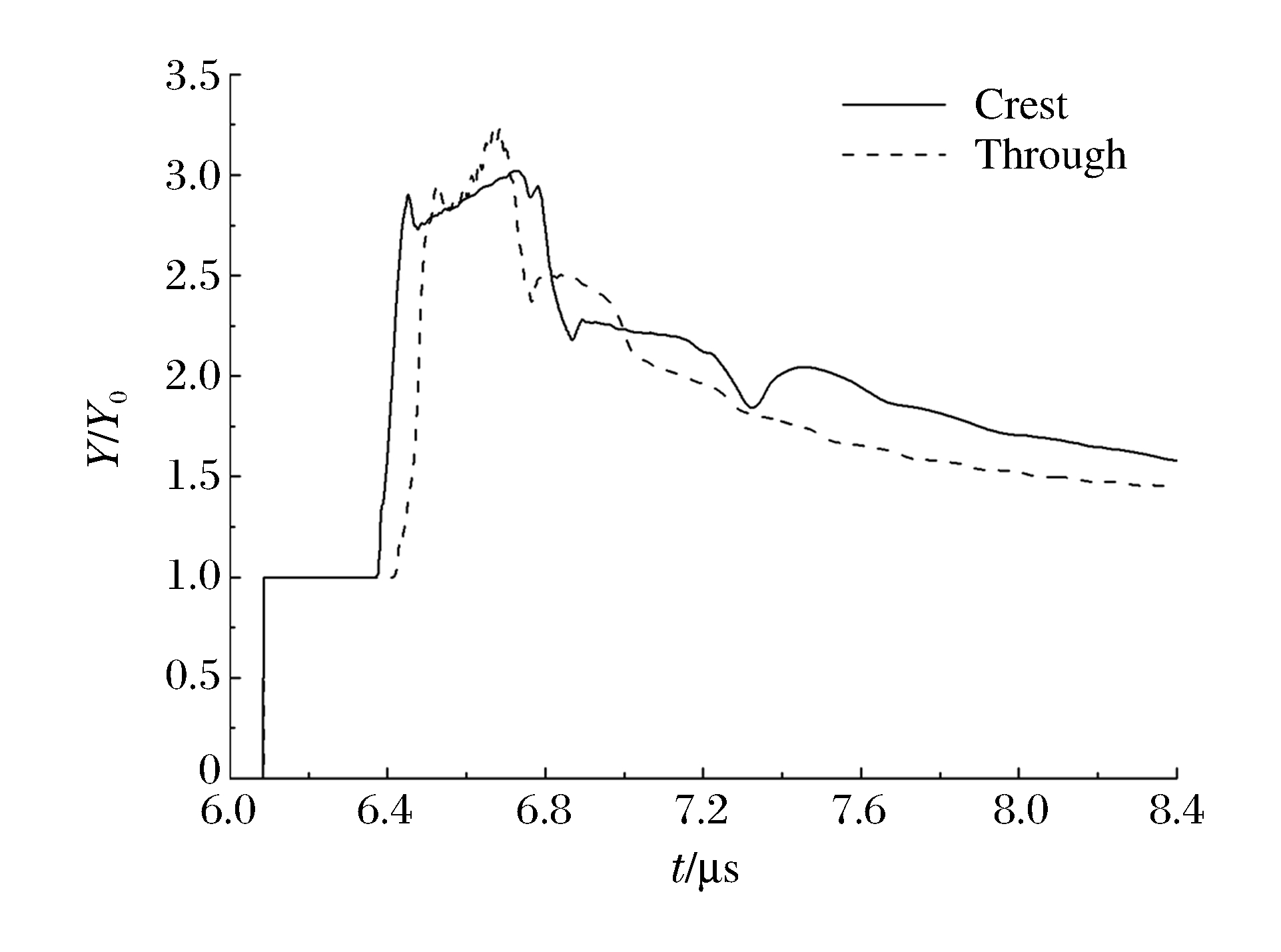
Figure 2. Comparisons of the perturbed interface between experiment and numerical simulations ((a) Experimental image, (b) Simulated image at normal strengths Y₀ and G₀, (c) Simulated image at 10 times the normal strengths Y₀ and G₀)

DownLoad: Full-Size Img PowerPoint

Fig. 3 shows the pressure histories of the crest (solid line) and the trough (dashed line) on the loading surface, which increase continuously and smoothly in a short time and form an approximate quasi-isentropic drive.Afterwards, the expansion of the detonation products decelerates gradually, and the loading pressure on the interface rises slowly.However, the pressure at the trough ascends faster than that at the crest, and the peak pressure at the trough is also relatively larger, because the detonation products converges at the trough and diverges at the crest.The average peak pressure is about 25 GPa, and the strain rate reaches 10⁶ s^-1.The loading pressure then reduces gradually, which is attributed to the decrease of the expansion pressure of the detonation products and the unloading effect of the rarefaction wave reflecting from the free surface.

Figure 3. Pressure histories of crest and trough at the loading surface

DownLoad: Full-Size Img PowerPoint

Fig. 4 shows several contours of local pressure (a), density (b), and temperature (c) at 6 different times after the arrival of the detonation products at the loading surface, from left to right and top to bottom, at 6.36, 6.5, 6.7, 6.9, 7.1 and 7.3 μs.They exhibit an evolution process of the perturbed interface and the interaction of the wave and the interface.The blue part of the temperature contour is the aluminum sample, and the sample temperature remains below 500 K and far below the melting point of 1 200 K, which indicates that the sample is in the elastic-plastic state all the time.

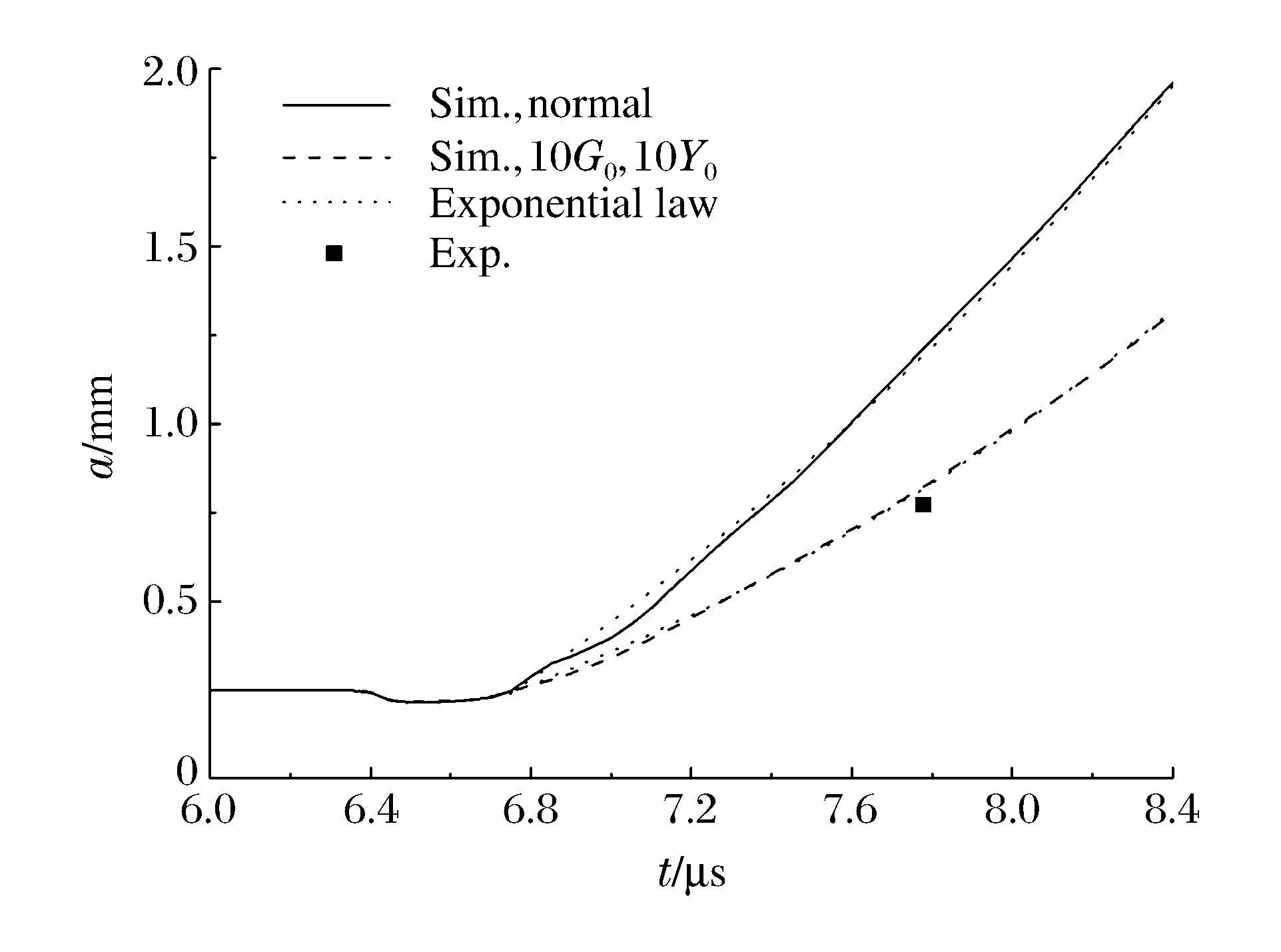
Figure 4. Contours of local pressure (a), density (b), and temperature (c) at 6.36, 6.5, 6.7, 6.9, 7.1, and 7.3 μs from left to right and top to bottom after the arrival of detonation products at the loading surface

DownLoad: Full-Size Img PowerPoint

Fig. 2(b) shows an image of the sample at 7.78 μs when the initial yield strength Y₀ and the shear modulus G₀ are normal values.Fig. 5 shows a comparison of the perturbation amplitudes between the experiment and numerical simulations, where the square symbol corresponds to the experimental result, and the solid black line corresponds to the numerical results when Y₀ and G₀ are normal.The simulated amplitude is much larger than that in the experiment when using normal values of Y₀ and G₀.This is because the aluminum strengthens under such conditions, and the SG constitutive model underestimates its strength, which can suppress the perturbation growth.

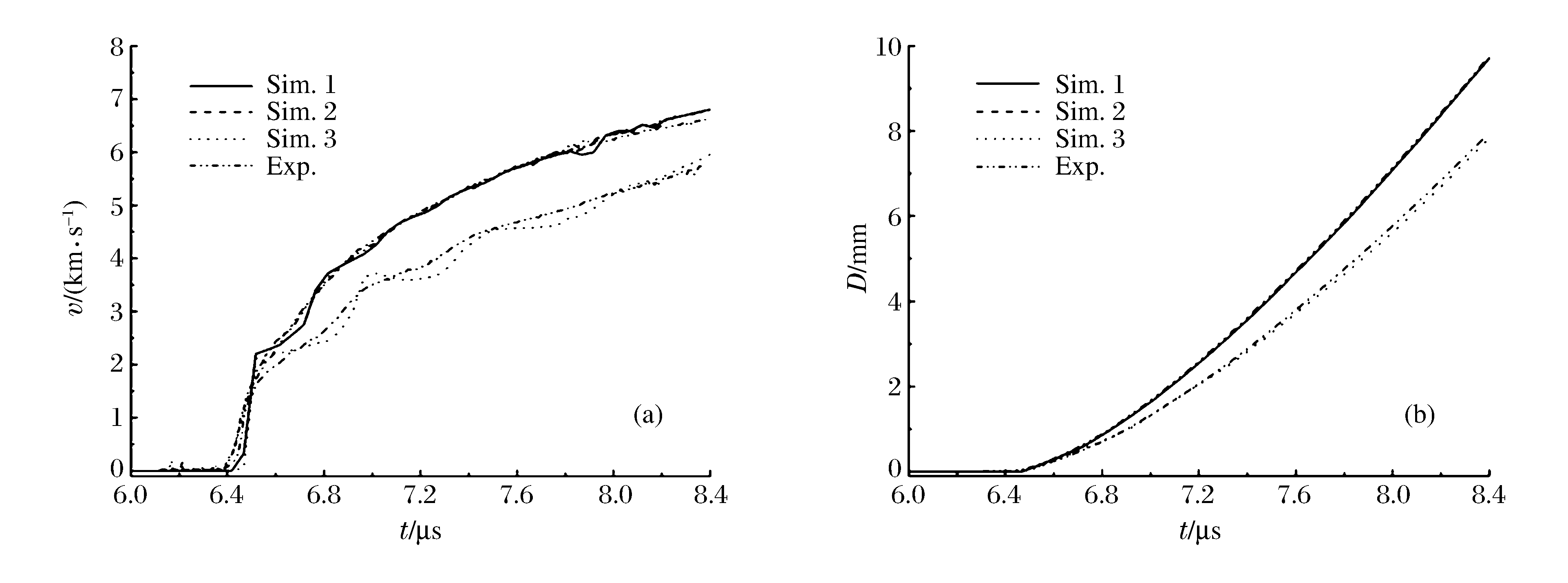
Figure 5. Growth histories of the perturbation amplitude

DownLoad: Full-Size Img PowerPoint

Fig. 6 shows the time histories of the free surface velocity (a) and displacement (b) at 3 measurement points (dot-dot-dashed lines:experiment; solid, dashed, and dotted lines:simulations), which agree well with each other.Therefore, the calculations of detonation of the explosive and the thermodynamic state of the sample are exact.

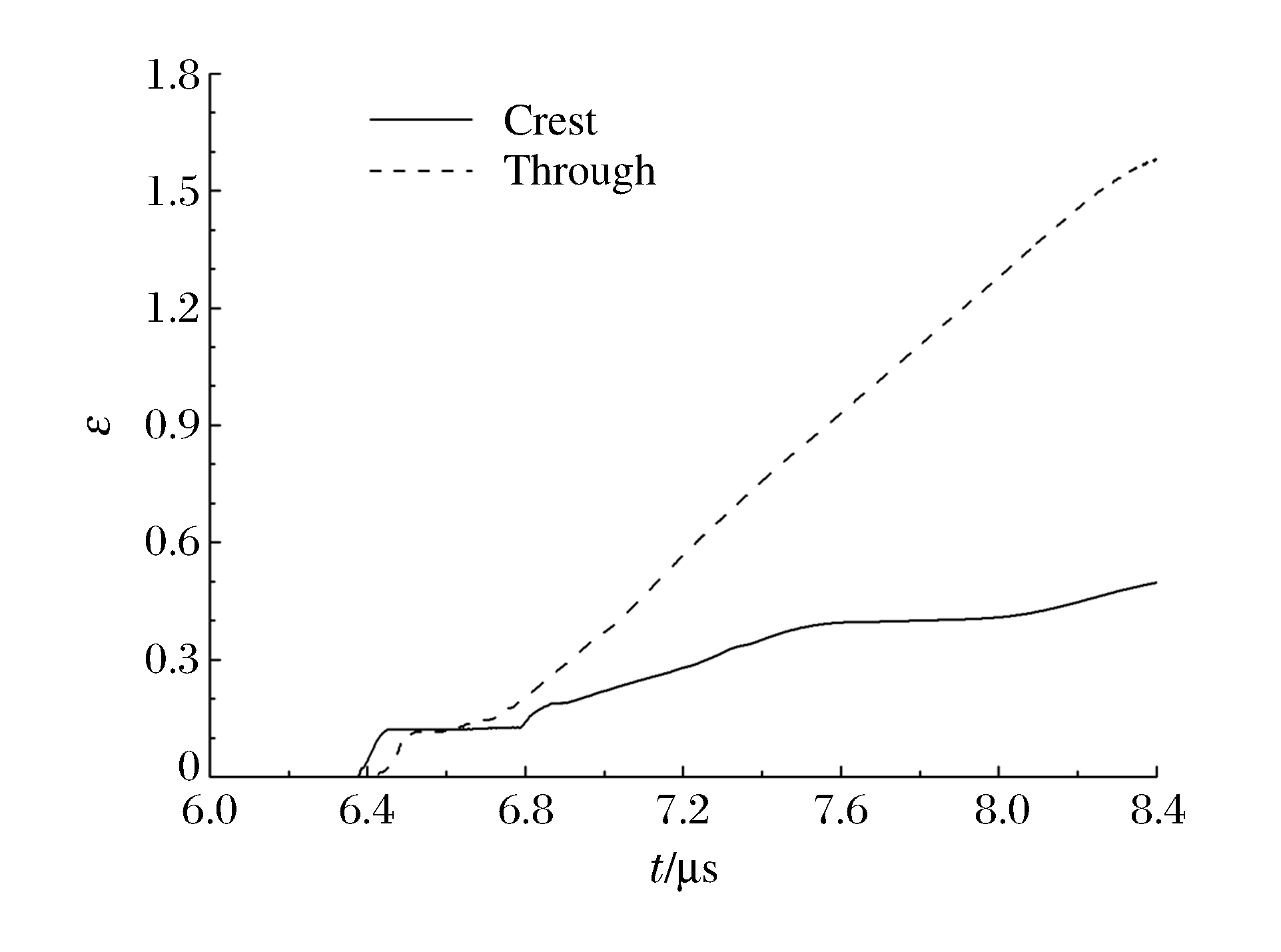
Figure 6. Time histories of the free surface velocity (a) and displacement (b)

DownLoad: Full-Size Img PowerPoint

Fig. 7 shows the calculated time histories of the strain at the crest (solid line) and trough (dashed line) of the loading surface.The deformation at the trough is much larger than that at the crest because the trough of the sample is in a stronger tensile stress state, which is the main mechanism for the deformation of the perturbation interface.Fig. 8 shows the time histories of the dynamic yield strength at the crest (solid line) and trough (dashed line) of the loading surface, calculated using the SG constitutive model, similar to the profile of the loading pressure, which demonstrate that the material strength increased as did the loading pressure under a certain condition.

Figure 7. Time histories of strain at the crest and trough of the loading surface

DownLoad: Full-Size Img PowerPoint

Figure 8. Time histories of yield strength at the crest and trough of the loading surface

DownLoad: Full-Size Img PowerPoint

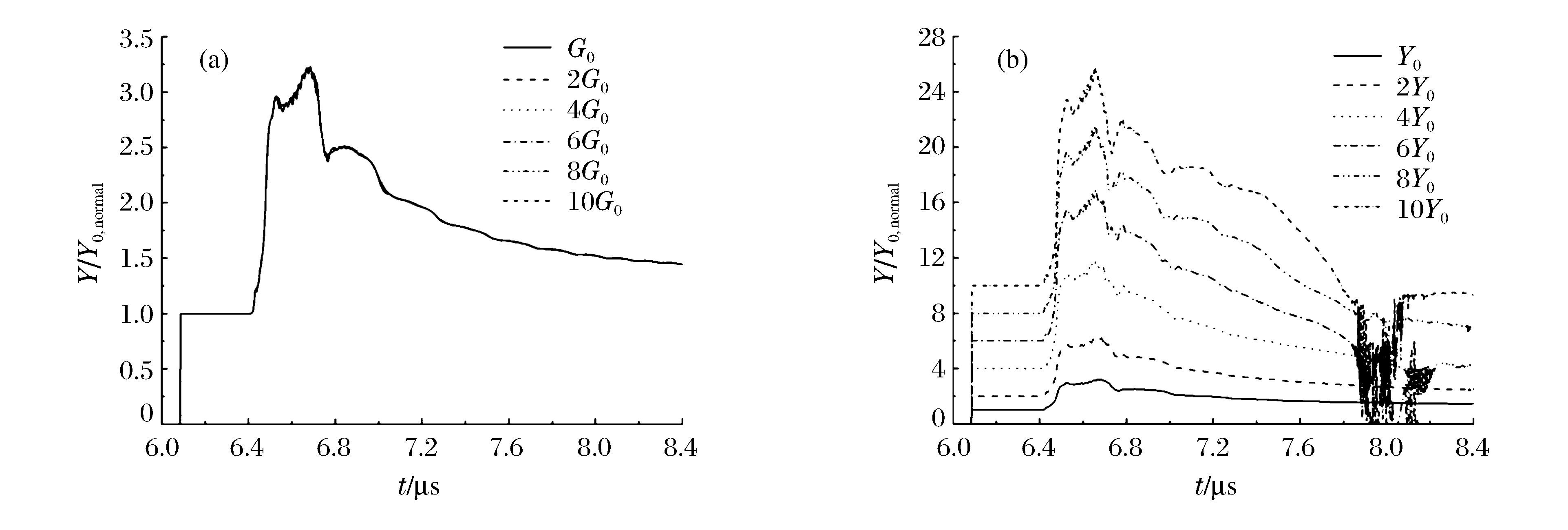
Moreover, when the loading pressure reaches a peak, the dynamic yield strength was up to 3 times that of the initial value.In fact, the normal SG model is generally calibrated by conventional Hopkinson and Taylor impacts experiment with a lower strain rate.Under the current loading conditions (loading pressure of 25 GPa and strain rate of 10⁶ s^-1), the strength is not great enough to suppress the perturbation growth.However, when the initial yield strength Y₀ and the shear modulus G₀ increase to 10 times that of the normal values, good agreement between the experiment and simulation is achieved, as shown in Fig. 2(c) for the perturbation interface and in Fig. 5 for the perturbation amplitude with the dashed line.Therefore, the material strength intensively stabilizes the perturbation growth.The dotted lines in Fig. 5 are fitted lines from the simulated results, indicating that the perturbation amplitude grows exponentially over time.

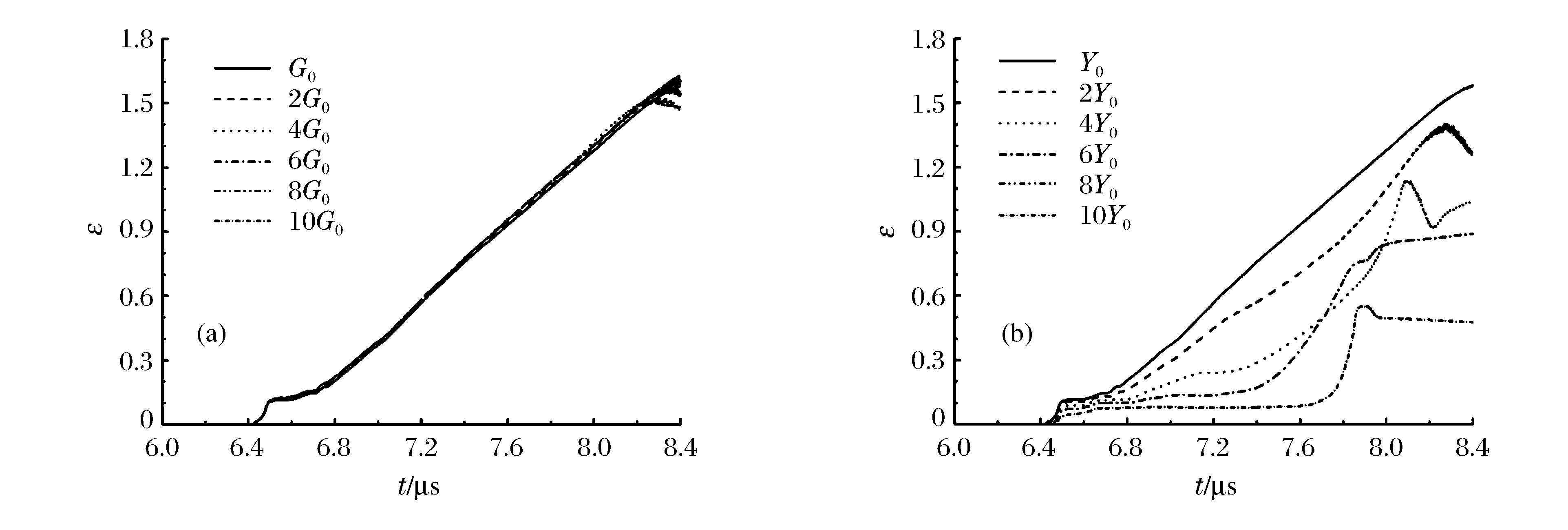
We studied the effect of the initial yield strength and the initial shear modulus of the material on the evolution and growth of the perturbed interface.Figs. 9(a), 10(a), and 11(a) show the calculated growth histories of perturbation amplitude, strain histories, and dynamic yield strength histories at the trough of the loading surface, respectively, when the initial yield strength is fixed at the normal value and as the initial shear modulus increases gradually.The growth of the perturbation amplitude exhibits no change even when the initial shear modulus increases to 10 times that of the normal value, which means that the initial shear modulus has no influence on the material deformation and does not affect the dynamic yield strength.

Figure 9. Growth histories of the perturbation amplitude for different values of initial shear modulus (a) and yield strength (b)

DownLoad: Full-Size Img PowerPoint

Figure 10. Time histories of strain at the trough of the loading surface for different values of initial shear modulus (a) and yield strength (b)

DownLoad: Full-Size Img PowerPoint

Figure 11. Time histories of yield strength at the trough of the loading surface for different values of initial shear modulus (a) and yield strength (b)

DownLoad: Full-Size Img PowerPoint

Figs. 9(b), 10(b), and 11(b) show the numerical results when the initial shear modulus is fixed and the initial yield strength gradually increases to 10 times that of the normal value.These indicate that, with the increase of the initial yield strength, the dynamic yield strength also increases, the material deformation is retarded, and the perturbation growth is suppressed markedly.Therefore, the initial shear modulus of the material exerts no effect on the growth of the metallic RT instability within a certain range, while the initial yield strength has an obvious effect on it.

4. Conclusions

We have established an experimental setup and developed a numerical simulation method to investigate the RT instability in metallic materials driven by explosion.We also studied the RT instability in aluminum, and drew the following conclusions:

(1) The perturbation amplitude grows following an exponential law over time.

(2) When using the normal physical property parameters of aluminum, simulated evolution of the perturbed interface agrees with experiment only qualitatively, and there is a big quantitative difference between them because the aluminum strengthens under high pressures and at high strain rates, and the SG constitutive model underestimates its strength as being not great enough to suppress the perturbation growth.

(3) When the initial yield strength and the initial shear modulus increase to 10 times their normal values, the numerical and experimental results are in good agreement both qualitatively and quantitatively.The underlying physical mechanism is the stabilization effect of material strength on the perturbation growth.Moreover, the initial shear modulus has no influence on the perturbation growth within a certain range whereas the initial yield strength does influence it strongly.Therefore, the material strength dominates the evolution of the metallic RT instability.

图 1 glide (111)面示意图（蓝色原子为下半无限大晶体的最上层原子，灰色原子为上半无限大晶体的最下层原子，R为OB的距离，C点为本征层错位置，D点为不稳定层错位置）

Figure 1. Schematic diagram of glide (111) surface (Blue atoms are the topmost layer of atoms in the lower half of the infinity crystal, and gray atoms are the bottom layer of atoms in the upper half of the infinity crystal; R is the distance of OB, the point C is the location of intrinsic stacking fault, and the point D is the location of unstable stacking fault.)

下载: 全尺寸图片幻灯片

图 2 基于DFT的第一性原理计算理想超胞模型

Figure 2. Ideal supercell model for the first principle computations based on DFT

下载: 全尺寸图片幻灯片

图 3 不同应变下由式(4)拟合得到的硅的glide (111)面的GSFE面（图中的点为第一性原理计算结果，[ $11\overline{2}$ ]方向上的第1个能量峰值为不稳定层错能，第1个能量最小值为本征层错能，GSFE面沿[ $\overline{1}10$ ]方向的投影是对称的）

Figure 3. GSFE surface of the glide (111) surface of silicon fitted by Eq. (4) for different strains (The points in the figures are the results of first principles calculations, the first energy peak in the [ $11\overline{2}$ ] direction is the unstable stacking fault energy, the first energy minimum is the intrinsic stacking fault energy, and the GSFE surface is symmetric along the projection in the [ $\overline{1}10$ ] direction.)

下载: 全尺寸图片幻灯片

图 4 不同应变下由式(4)拟合得到的金刚石的glide (111)面的GSFE面（图中的点为第一性原理计算结果，[ $11\overline{2}$ ]方向上的第1个能量峰值为不稳定层错能，第1个能量最小值为本征层错能，GSFE面沿[ $\overline{1}10$ ]方向的投影是对称的）

Figure 4. GSFE surface of the glide (111) surface of diamond fitted by Eq. (4) for different strains (The points in the figures are the results of first principles calculations, the first energy peak in the [ $11\overline{2}$ ] direction is the unstable stacking fault energy, the first energy minimum is the intrinsic stacking fault energy, and the GSFE surface is symmetric along the projection in the [ $\overline{1}10$ ] direction.)

下载: 全尺寸图片幻灯片

图 5 不同应变下glide (111)面的GSFE面在 $\text{[}\text{11}\overline{\text{2}}\text{]}$ 滑移方向上的投影

Figure 5. Projections of the GSFE surface on the $\text{[}\text{11}\overline{\text{2}}\text{]}$ slip direction for different strains on the glide (111) surface

下载: 全尺寸图片幻灯片

图 6 不同应变下glide (111)面的GSFE面在[ $\overline{1}10$ ]滑移方向上的投影

Figure 6. Projections of the GSFE surface on the [ $\overline{1}10$ ] slip direction for different strains on the glide (111) surface

下载: 全尺寸图片幻灯片

图 7 不同应变下硅的glide (111)面的本征层错能（γ_I）、不稳定层错能（γ_us）以及两者的比值

Figure 7. Intrinsic stacking fault energy (γ_I) and unstable stacking fault energy (γ_us) and the ratio of them for the glide (111) surface of silicon at different strains

下载: 全尺寸图片幻灯片

图 8 不同应变下金刚石的glide (111)面的本征层错能（γ_I ）、不稳定层错能（γ_us）以及两者的比值

Figure 8. Intrinsic stacking fault energy (γ_I) and unstable stacking fault energy (γ_us) and the ratio of them for the glide (111) surface of dimond at different strains

下载: 全尺寸图片幻灯片

表 1 不同超胞的硅的本征层错能和不稳定层错能

Table 1. Intrinsic stacking faults energies and unstable stacking faults energies for silicon with different supercells

Layers	γ_I/(eV·Å⁻²)	γ_us/(eV·Å⁻²)	Layers	γ_I/(eV·Å⁻²)	γ_us/(eV·Å⁻²)
6	0.002	0.085	18	0.003	0.106
10	0.004	0.098	22	0.003	0.107
14	0.004	0.104

下载: 导出CSV

表 2 不同应变下由式(4)描述的GSFE面的拟合参数

Table 2. Fitting parameters of the GSFE surface described by Eq. (4) for different strains

Material	ε	Fitting parameters/(J·m⁻²)
Material	ε	c₀	c₁	c₂	c₃	c₄	c₅
Silicon	0	24.085	−12.658	7.490	−2.221	−0.471	0.257
	0.03	25.623	−13.568	8.163	−2.439	−0.521	0.295
	0.06	27.235	−14.531	8.882	−2.674	−0.573	0.337
	0.09	28.911	−15.538	9.646	−2.924	−0.631	0.383
	0.12	30.631	−16.577	10.446	−3.183	−0.696	0.432
	0.15	32.372	−17.634	11.265	−3.445	−0.764	0.482
	0.18	34.143	−18.720	12.112	−3.720	−0.834	0.537

Material	ε	Fitting parameters/(J·m⁻²)
Material	ε	c₆	s₁	s₂	s₃	s₄	s₅
Silicon	0	0.046	21.901	−3.797	0.849	−0.020	−0.013
	0.03	0.050	23.465	−4.160	0.944	−0.026	−0.016
	0.06	0.054	25.114	−4.548	1.046	−0.033	−0.020
	0.09	0.059	26.835	−4.960	1.157	−0.041	−0.023
	0.12	0.064	28.604	−5.391	1.275	−0.046	−0.025
	0.15	0.069	30.395	−5.833	1.397	−0.048	−0.027
	0.18	0.077	32.225	−6.289	1.527	−0.054	−0.032

Material	ε	Fitting parameters/(J·m⁻²)
Material	ε	c₀	c₁	c₂	c₃	c₄	c₅
Diamond	0	55.327	−29.945	20.276	−6.548	−1.668	0.852
	0.03	62.026	−34.033	23.583	−7.701	−1.944	1.084
	0.06	69.519	−38.668	27.417	−9.054	−2.268	1.371
	0.09	77.951	−43.959	31.877	−10.654	−2.641	1.717
	0.12	87.438	−50.002	37.078	−12.544	−3.070	2.129
	0.15	97.852	−56.793	43.166	−14.733	−3.638	2.648
	0.18	109.070	−64.368	50.293	−17.270	−4.382	3.312

Material	ε	Fitting parameters/(J·m⁻²)
Material	ε	c₆	s₁	s₂	s₃	s₄	s₅
Diamond	0	0.260	51.709	−11.059	3.080	−0.125	−0.059
	0.03	0.278	58.586	−12.938	3.634	−0.175	−0.079
	0.06	0.297	66.292	−15.122	4.294	−0.238	−0.102
	0.09	0.318	74.966	−17.651	5.078	−0.325	−0.133
	0.12	0.335	84.742	−20.561	5.996	−0.439	−0.167
	0.15	0.369	95.601	−23.980	7.099	−0.515	−0.208
	0.18	0.441	107.721	−28.001	8.429	−0.528	−0.264

下载: 导出CSV

表 3 在不同应变下式(6)描述的 $[\overline{{\boldsymbol{1}}}{\boldsymbol{10}}]$ (111)方向的GSFE面的拟合参数

Table 3. Fitting parameters of the GSFE surface for $[\overline{{\boldsymbol{1}}}{\boldsymbol{10}}]$ (111) slip direction described by Eq. (6) at different strains

Material	ε	μ_γ/(GJ·m⁻³)	$\varDelta_1$	$\varDelta_2$	Material	ε	μ_γ/(GJ·m⁻³)	$\varDelta_1$	$\varDelta_2$
Silicon	0	16.297	−1.002	0.495	Dimond	0	121.297	−1.149	0.850
	0.03	16.612	−1.013	0.496		0.03	125.646	−1.150	0.825
	0.06	16.898	−1.025	0.496		0.06	129.464	−1.150	0.797
	0.09	17.151	−1.035	0.495		0.09	132.670	−1.148	0.768
	0.12	17.369	−1.045	0.492		0.12	135.093	−1.145	0.735
	0.15	17.545	−1.053	0.489		0.15	136.433	−1.141	0.694
	0.18	17.665	−1.060	0.481		0.18	136.151	−1.129	0.629

下载: 导出CSV

参考文献(31)

[1]	JOHNSTON W G, GILMAN J J. Dislocation multiplication in lithium ﬂuoride crystals [J]. Journal of Applied Physics, 1960, 31(4): 632–643. doi: 10.1063/1.1735655
[2]	HIRTH J P, KUBIN L. Preface [J]. Dislocations in Solids, 2009.
[3]	MADEC R, DEVINCRE B, KUBIN L, et al. The role of collinear interaction in dislocation-induced hardening [J]. Science, 2003, 301(5641): 1879–1882. doi: 10.1126/science.1085477
[4]	SHEHADEH M A, BRINGA E M, ZBIB H M, et al. Simulation of shock-induced plasticity including homogeneous and heterogeneous dislocation nucleations [J]. Applied Physics Letters, 2006, 89(17): 171918. doi: 10.1063/1.2364853
[5]	GERMANN T C, HOLIAN B L, LOMDAHL P S, et al. Dislocation structure behind a shock front in fcc perfect crystals: atomistic simulation results [J]. Metallurgical and Materials Transactions A, 2004, 35(9): 2609–2615. doi: 10.1007/s11661-004-0206-5
[6]	KANEL G I, FORTOV V E, RAZORENOV S V. Shock-wave phenomena and the properties of condensed matter [M]. New York: Springer, 2004.
[7]	MACDONALD M J, MCBRIDE E E, GALTIER E, et al. Using simultaneous X-ray diffraction and velocity interferometry to determine material strength in shock-compressed diamond [J]. Applied Physics Letters, 2020, 116(23): 234104. doi: 10.1063/5.0013085
[8]	ZEPEDA-RUIZ L A, STUKOWSKI A, OPPELSTRUP T, et al. Probing the limits of metal plasticity with molecular dynamics simulations [J]. Nature, 2017, 550(7677): 492–495. doi: 10.1038/nature23472
[9]	FAN H D, WANG Q Y, EL-AWADY J A, et al. Strain rate dependency of dislocation plasticity [J]. Nature Communications, 2021, 12(1): 1845. doi: 10.1038/s41467-021-21939-1
[10]	GUMBSCH P, GAO H J. Dislocations faster than the speed of sound [J]. Science, 1999, 283(5404): 965–968. doi: 10.1126/science.283.5404.965
[11]	TEUTONICO L J. Dynamical behavior of dislocations in anisotropic media [J]. Physical Review, 1961, 124(4): 1039–1045. doi: 10.1103/PhysRev.124.1039
[12]	TEUTONICO L J. Uniformly moving dislocations of arbitrary orientation in anisotropic media [J]. Physical Review, 1962, 127(2): 413–418. doi: 10.1103/PhysRev.127.413
[13]	BLASCHKE D N, CHEN J, FENSIN S, et al. Clarifying the definition of ‘transonic’ screw dislocations [J]. Philosophical Magazine, 2021, 101(8): 997–1018. doi: 10.1080/14786435.2021.1876269
[14]	KATAGIRI K, PIKUZ T, FANG L C, et al. Transonic dislocation propagation in diamond [J]. Science, 2023, 382(6666): 69–72. doi: 10.1126/science.adh5563
[15]	WESSEL K, ALEXANDER H. On the mobility of partial dislocations in silicon [J]. Philosophical Magazine, 1977, 35(6): 1523–1536. doi: 10.1080/14786437708232975
[16]	RABIER J, DEMENET J L. Low temperature, high stress plastic deformation of semiconductors: the silicon case [J]. Physica Status Solidi B, 2000, 222(1): 63–74. doi: 10.1002/1521-3951(200011)222:1<63::AID-PSSB63>3.0.CO;2-E
[17]	CAI W, BULATOV V V, CHANG J P, et al. Dislocation core effects on mobility [M]//NABARRO F R N, HIRTH J P. Dislocations in Solids, Amsterdam: Elsevier, 2004: 1–80.
[18]	VITEK V. Intrinsic stacking faults in body-centred cubic crystals [J]. Philosophical Magazine, 1968, 18(154): 773–786. doi: 10.1080/14786436808227500
[19]	VITEK V. Theory of the core structures of dislocations in body-centered-cubic metals [J]. Cryst Lattice Defects, 1974, 5: 1–34.
[20]	THOMSON R. Intrinsic ductility criterion for interfaces in solids [J]. Physical Review B, 1995, 52(10): 7124–7134. doi: 10.1103/PhysRevB.52.7124
[21]	SUN Y M, KAXIRAS E. Slip energy barriers in aluminium and implications for ductile-brittle behaviour [J]. Philosophical Magazine A, 1997, 75(4): 1117–1127. doi: 10.1080/01418619708214014
[22]	ANDRIC P, YIN B L, CURTIN W A. Stress-dependence of generalized stacking fault energies [J]. Journal of the Mechanics and Physics of Solids, 2019, 122: 262–279. doi: 10.1016/j.jmps.2018.09.007
[23]	PERDEW J P, BURKE K, ERNZERHOF M. Generalized gradient approximation made simple [J]. Physical Review Letters, 1996, 77(18): 3865–3868. doi: 10.1103/PhysRevLett.77.3865
[24]	PERDEW J P, BURKE K, ERNZERHOF M. Generalized gradient approximation made simple [Phys. Rev. Lett. 77, 3865 (1996)] [J]. Physical Review Letters, 1997, 78(7): 1396. doi: 10.1103/PhysRevLett.78.1396
[25]	BLÖCHL P E. Projector augmented-wave method [J]. Physical Review B, 1994, 50(24): 17953–17979. doi: 10.1103/PhysRevB.50.17953
[26]	KRESSE G, JOUBERT D. From ultrasoft pseudopotentials to the projector augmented-wave method [J]. Physical Review B, 1999, 59(3): 1758–1775. doi: 10.1103/PhysRevB.59.1758
[27]	KRESSE G, HAFNER J. Ab initio molecular dynamics for liquid metals [J]. Physical Review B, 1993, 47(1): 558–561. doi: 10.1103/PhysRevB.47.558
[28]	KRESSE G, FURTHMÜLLER J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set [J]. Physical Review B, 1996, 54(16): 11169–11186. doi: 10.1103/PhysRevB.54.11169
[29]	HU X S, HUANG M S, LI Z H, et al. Study on lattice discreteness effect on superdislocation core properties of Ni₃Al by improved semi-discrete variational peierls-Nabarro model [J]. Intermetallics, 2022, 151: 107695. doi: 10.1016/j.intermet.2022.107695
[30]	ANDERSON P M, HIRTH J P, LOTHE J. Theory of dislocations [M]. 3rd ed. Cambridge: Cambridge University Press, 2017.
[31]	VAN SWYGENHOVEN H, DERLET P M, FRØSETH A G. Stacking fault energies and slip in nanocrystalline metals [J]. Nature Materials, 2004, 3(6): 399–403. doi: 10.1038/nmat1136