Study on Failure Mode and Energy Evolution of Fractured Rock Body under Triaxial Compression
-
摘要: 为研究不同围压条件下含不同长度单裂隙岩体的裂纹扩展特征和能量演化规律,基于室内三轴压缩试验结果标定细观参数,开展了PFC2D颗粒流数值模拟试验。结果表明:拉伸裂纹先于剪切裂纹产生,两者呈指数增长,裂隙长度减小和围压增大使拉伸裂纹和剪切裂纹快速增长时间滞后;最终破坏时,随裂隙长度增加,拉伸裂纹和剪切裂纹减少。应力集中于裂隙两端,裂纹周围存在应力集中现象。相同围压下,裂隙长度增加,岩样破坏时块体数减少。岩体破坏本质为能量储存、耗散与释放的过程,在加载过程中,岩体能量转化被分为4个阶段。裂隙长度增加削弱岩样储存应变能的能力,总能量减少,围压增强岩样储存应变能的能力。岩样破坏时,耗散能大于应变能,随裂隙增长,耗散能减少。Abstract: To study the crack extension characteristics and energy evolution law of the rock body with different lengths of single fissure under different confining pressures, the mesoscopic parameters were calibrated by use of the indoor triaxial compression test, and the numerical simulation test of PFC2D particle flow was carried out. The results show that tensile cracks are generated before shear cracks, and both of them grow exponentially; the decrease of the fissure length and the increase of the confining pressure restrain the rapid growth of tensile and shear cracks; when the final failure occurs, the tensile and shear cracks decrease with the increase of the fissure length. The stress is concentrated at both ends of the crack, and there is stress concentration around the crack. Under the same confining pressure, the number of failure blocks of the rock sample decreases with the increment of fissure length. The nature of rock failure is the process of energy storage, dissipation and release, and the rock energy transformation is divided into four stages during the loading process. The increase in fissure length weakens the ability of the rock samples to store strain energy, the total energy decreases, and the confining pressure enhances the ability of the rock samples to store strain energy. The dissipated energy is greater than the strain energy when the rock sample fails, and the dissipated energy decreases with the fissure growth.
-
Ceramic materials have been increasingly used in armors, personal armor system, aeronautic engineering and vehicle engineering due to its excellent ballistic performance. An understanding of the response and failure of ceramic materials under impact loading is of great significance for the design and assessment of military hardware and protective structures.
As ballistic tests are expensive to perform and time consuming, numerical simulations have been widely employed in the design and optimization of ceramic armors. However, the accuracy of numerical simulations depends on the dynamic constitutive model for ceramic materials to a large extent. To this end, many models have been put forward[1-8]. Fahrenthold[1] proposed a continuum damage model for fracture of brittle solids under dynamic loading in which Weibull strength distribution was employed to account for the effects of flaw size distribution on the damage accumulation rate. The model was used to compute the depth of penetration in a steel plate impacted by a sphere of alumina without being applied to simulate the behavior of ceramic armors subjected to projectile impact. Rajendran[2] modeled the impact behavior of AD85 ceramic under multiaxial loading by assuming an existing distribution of micro-cracks and employing a criterion for crack growth based on theories of dynamic fracture mechanics. The strength of the intact material was rate dependent, and the inelastic deformation was modeled using an elastic-plastic cracking rule. The model predictions were found to be in good agreement with the test data obtained from plate impact tests. The most widely used constitutive model for ceramic materials in many commercial codes is the Johnson-Holmquist model (JH-2)[3]. The model employs two strength surfaces to describe the pressure-dependent compressive strength of the intact and failed materials, respectively. However, in the model strain rate effect is described by the same form of function adopted in the constitutive models for metals and concrete materials, which has been found to be inconsistent with the dynamic mechanical behavior in recent studies[9-12]. Furthermore, tensile softening cannot be accurately predicted, and no differentiation was made between the effects of strain rate and inertia (containment).
The main objective of this paper is to establish a macroscopic dynamic constitutive model for ceramic materials by closely following the previous work on concrete[9] and on the basis of the experimental observations for some ceramic materials. Various equations of the constitutive model are given and compared with some available experimental data for ceramic materials in terms of pressure-volumetric response, quasi-static strength surface and strain rate effect. Furthermore, the model is also verified against the data for triaxial test by single element simulation approach.
1. A Macroscopic Constitutive Model for Ceramics
A dynamic macroscopic constitutive model for ceramic materials is developed in the following sections based on the computational constitutive model for concrete subjected to dynamic loadings[9] with some modifications being made. There are two points which should be highlighted here, namely, (1) the pressure-volumetric response is described by polynomial equation and the phase transition (e.g. AlN ceramics) as the pressure increases to a certain value is considered; (2) a hyperbolic tangent function is employed to describe the pressure dependent shear strength surfaces of ceramic materials which level out at very high pressures.
1.1 Equation of State
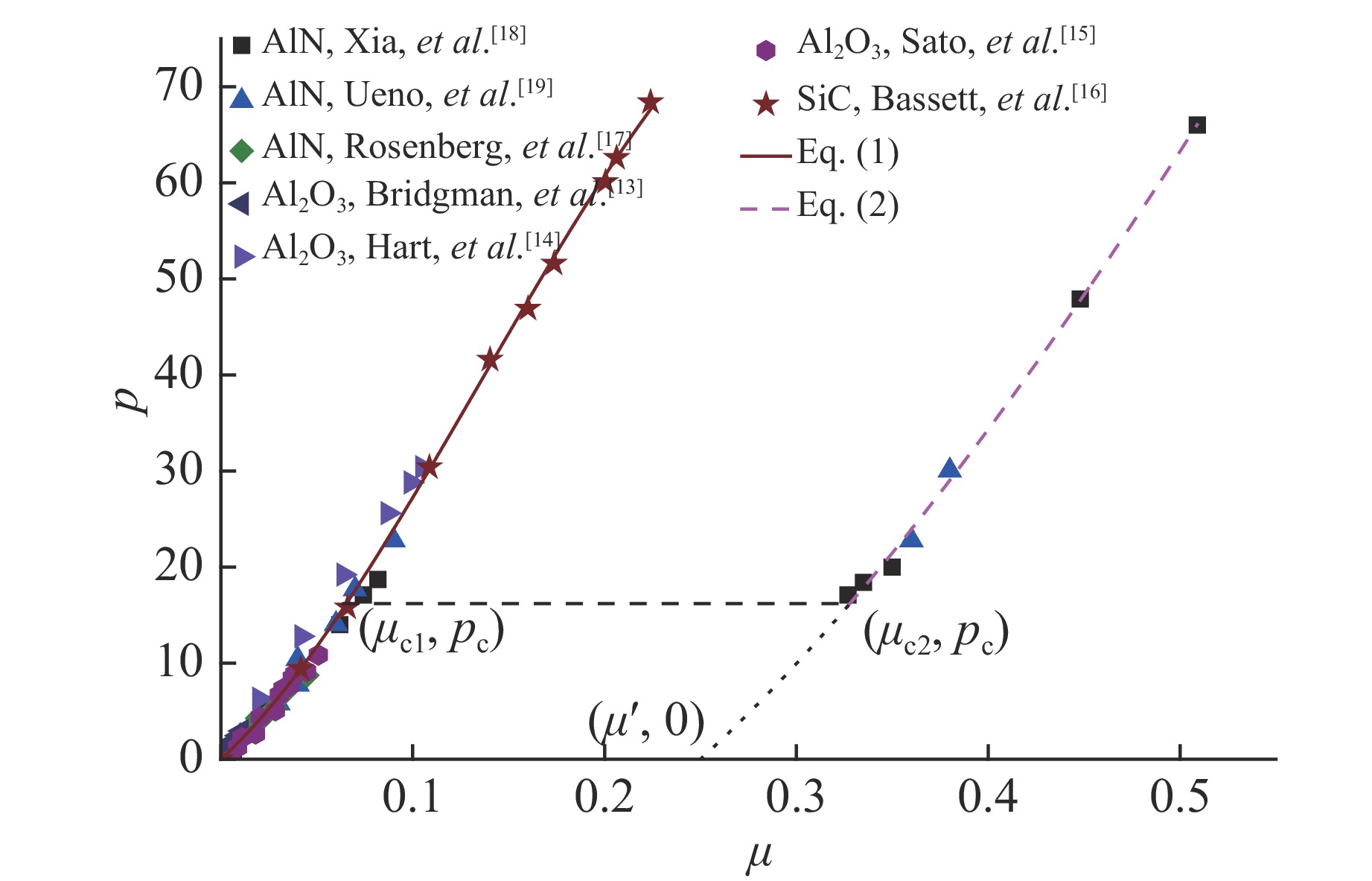
Ceramics are complex granular materials, which contain a large number of micro cracks and voids just as concrete material. On the one hand, it has been observed experimentally that the volumetric strains of Al2O3 and SiC increase with increasing pressure[13-16] and the equation of state for this category of ceramic materials can be expressed as polynomial equation, viz.
{p=K1μ+K2μ2+K3μ3μ=V0/V−1=ρ/ρ0−1 (1) where p is pressure;
μ is volumetric strain; K1, K2, K3 are bulk moduli for ceramic material;ρ0=1/V0 andρ=1/V indicate initial and current densities of ceramic material, respectively. On the other hand, the pressure-volumetric response of AlN has been observed experimentally to be quite different[17-19] and a phase transformation occurs from wurtzite structure to salt structure as pressure reaches a critical value. Thus, the equation of state for such ceramics as AlN can be written in the following form, namelyp={K1μ+K2μ2+K3μ3μ<μc1pcμc1⩽μ⩽μc2K4(μ−μ′)+K5(μ−μ′)2+K6(μ−μ′)3μ>μc2 (2) where pc represents a constant pressure at which phase transformation occurs; K4, K5, K6 are bulk moduli for ceramic material after the phase transformation;
μ′ is an offset in volumetric strain due to phase transformation;μc1 andμc2 are volumetric strains at the beginning and end of phase transformation, respectively. For μ < 0, ceramic material is under tension condition. Thus, one obtainsp=K1μ (3) 1.2 Strength Model
On the basis of the previous studies on concrete[9-10] and the experimental observations for ceramic materials[17, 20-24], it is suggested that the strength surface of ceramic materials can be cast into the following form, i.e.
Y={3(p+ftt)r(θ,e)p<0[3ftt+(fcc−3ftt)×3p/fcc]r(θ,e)0⩽p⩽fc/3[fcc+Bf′ctanh(p/f′c−fcc/(3f′c))]r(θ,e)p>fc/3 (4) in which Y is shear strength;
fcc=f′cψcηc andftt=ftψtηt are respective dynamic strengths of ceramic materials in uniaxial compression and tension, which take account of shear damage in the form of damage shape functions (η ) and strain rate effects by dynamic increase factor (DIF); B is an empirical constant;r(θ,e) represents Lode effect. The dynamic increase factor in compression (ψc) can be obtained by the following equation[9-10], viz.ψc=fcdf′c=(ψt−1)ftf′c+1 (5) where fcd and
f′c are the dynamic and static uniaxial compressive strength, respectively, ft is the static uniaxial tensile strength, and ψt represents the dynamic increase factor in tension which is expressed as followsψt=ftdft={(FmWy−1)tanh[(lg˙ε˙ε0−Wx)S]+1}Wy (6) in which ftd is the dynamic uniaxial tensile strength, parameters Wx, Fm, Wy and S are constants to be determined experimentally,
˙ε is the strain rate,˙ε0 is the reference strain rate usually taken to be˙ε0 = 1.0 s−1.The multi-axial tests of ceramics show that the effective strain at maximum load (
εmax ) increases almost linearly with increasing pressure. Furthermore, these tests also show ceramic compressive strength has no significant influence on this behavior. According to Eq.(18) in Ref.[9], the failure strain of ceramic is written asεf=εmλm(˙ε˙ε0)0.02=1λm0.0065max[1,1+λs(pfc−13)](˙ε˙ε0)0.02 (7) where
εf is the failure strain of ceramic material,εm is the strain corresponding to the maximum compressive strength,λm is the shear damage at which strength reaches its maximum value under compression, andλs is an empirical constant.The residual strength of the crushed ceramics is still high under the confining pressure. The residual strength surface for ceramic materials can be obtained from Eq.(4) by setting ftt = 0 and fcc =
f′c × r, namelyY={3p×r(θ,e)0<p⩽f′c×r/3[f′c×r+Bf′ctanh(pf′c−f′c×r3f′c)]×r(θ,e)p>f′c×r/3 (8) where r is a constant,
f′c × r represents the residual strength of concrete under quasi-static uniaxial compression. Meanwhile, the initial yield strength of concrete under quasi-static uniaxial compression is defined asf′c × l.2. Verification of the Newly-Developed Constitutive Model
The present model is verified against some available experimental data in terms of pressure-volumetric response, quasi-static strength surface and strain rate effect.
Values of various parameters in equation of state can be determined by hydrostatic compression experiment and they are listed in Table 1. For Al2O3 and SiC ceramic materials, the relationship between pressure and volumetric strain (Eq.(1)) can be rewritten as
Table 1. Values of parameters for BeO in the present modelEquation of state parameters Constitutive model parameters K1/GPa K2/GPa K3/GPa ρc/(kg·m−3) Fm Wx Wy S 181.5 1 207.9 −2 991 3 030 3 3.8 2 1.25 Constitutive model parameters f′c/GPa ft/GPa B G/GPa λm λs l r 1.5 0.15 1.2 125 0.3 7.5 0.8 0.3 p=1.815×1011μ+1.208×1012μ2−2.991×1012μ3 (9) and, for AlN ceramic material, the relationship between pressure and volumetric strain (Eq.(2)) can be recast into the following form
p={1.815×1011μ+1.208×1012μ2−2.991×1012μ3μ<0.0671.668×10100.067⩽μ⩽0.3301.819×1011(μ−0.250)+3.556×1011(μ−0.250)2−2.830×1011(μ−0.250)3μ>0.330 (10) Fig.1 shows comparison of the present model predictions (Eq.(9) and Eq.(10)) with the experimental data for Al2O3 and SiC[13-16] and for AlN[17-19]. It can be seen from Fig.1 that good agreement is obtained.
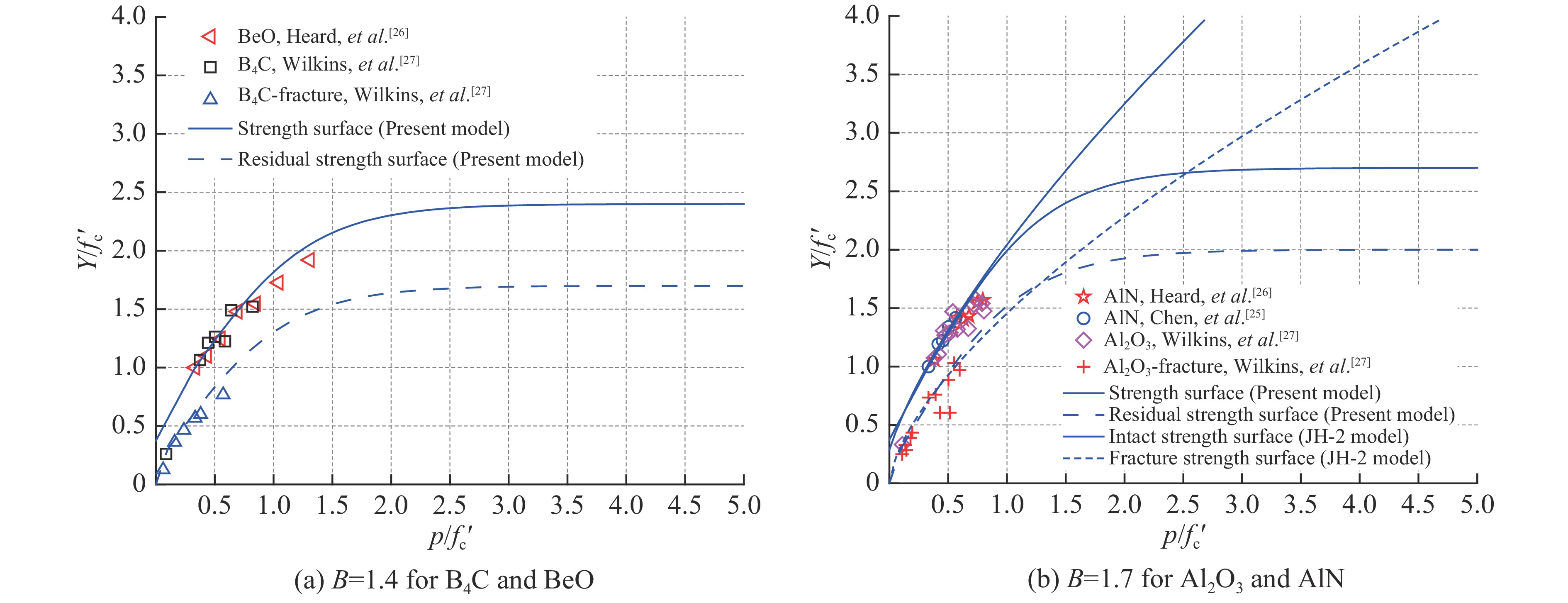
Fig.2(a) and Fig.2(b) show comparisons between the present model predictions (Eq.(4) with B = 1.4 for B4C and BeO and Eq.(9) with B = 1.7 for Al2O3 and AlN) and the test data[25-27] for the strength surfaces. The strength surfaces of Al2O3 and AlN obtained from the JH-2 model[28] are also shown in Fig.2(b). It is clear from Fig.2 that the present model predictions are in good agreement with available test results for ceramic materials. It is also clear from Fig.2(b) that the JH-2 model produces similar results to those of the present model for relatively low pressures whilst for higher pressure the present model levels out and the JH-2 model increases with increasing pressure.
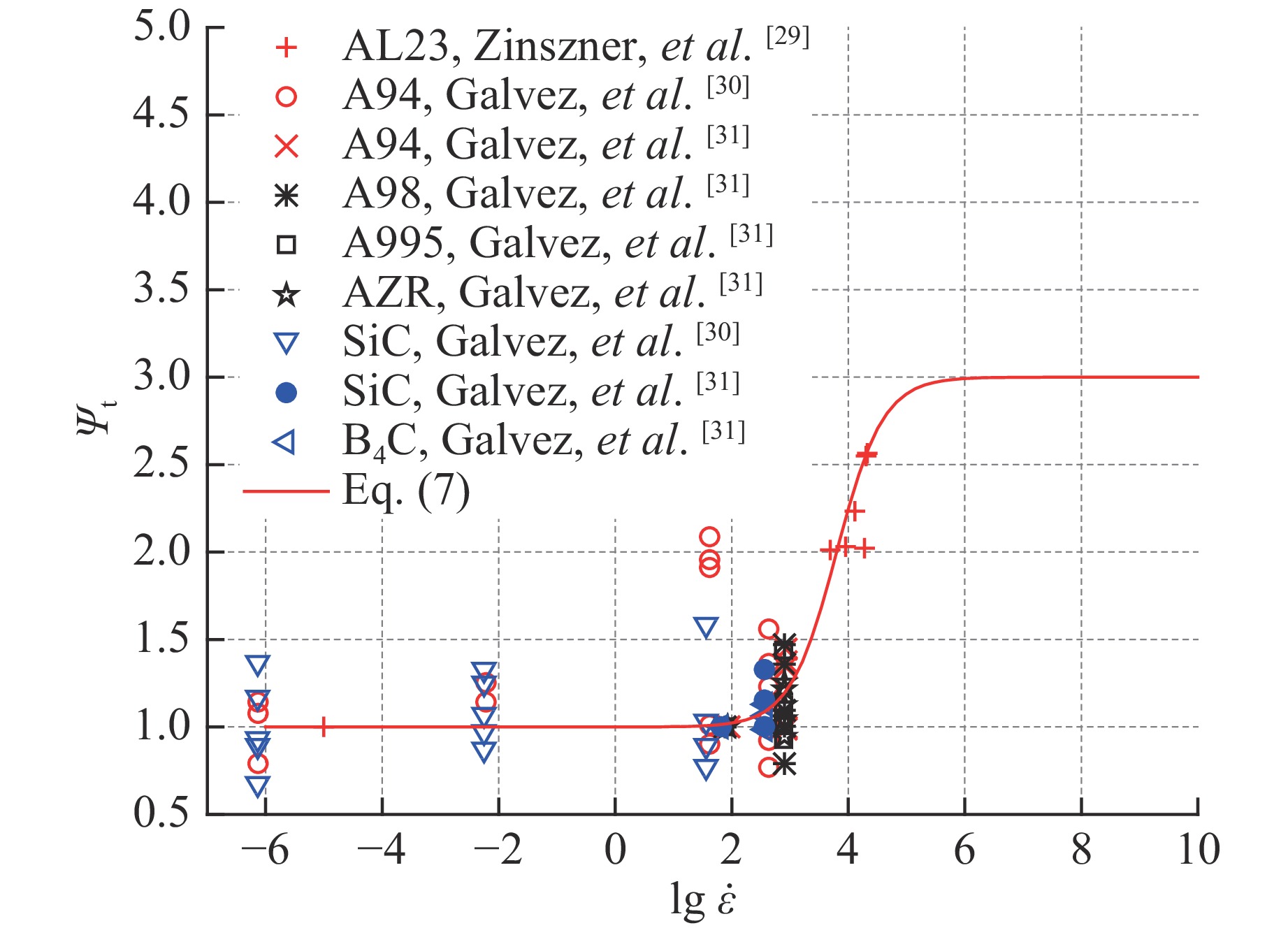
Values of parameters Fm, Wx and S in Eq.(6) for the dynamic increase factor in tension can be determined from tensile test data for ceramic materials at different strain rates. Fig.3 shows comparisons between the present model predictions (Eq.(6) with Fm = 3, Wx = 3.8 and S = 1.25) and available experimental data[29-31] for different ceramic materials at different strain rates. It is evident from Fig.3 that reasonable agreement is obtained.
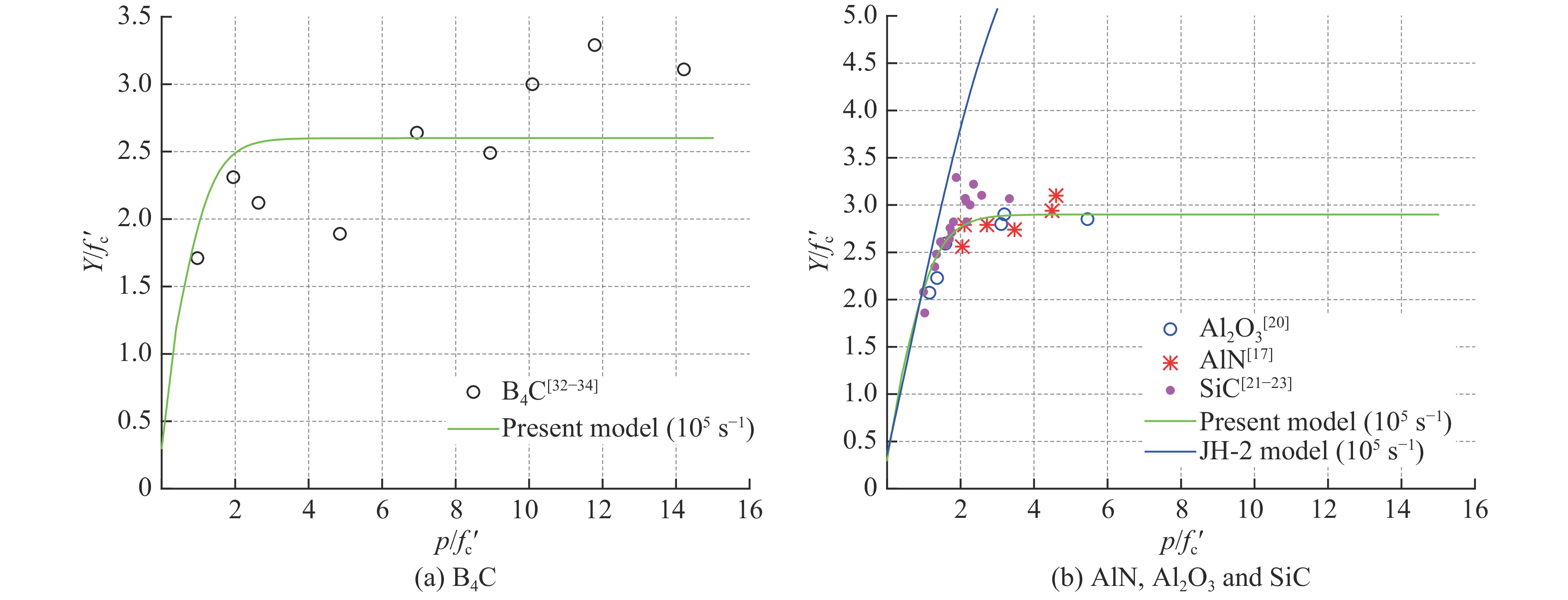
Fig.4(a) and Fig.4(b) show comparisons between the theoretically predicted strength surfaces (Eq.(4)) and some experimental data obtained from plate impact tests on B4C[32-34], AlN[17], Al2O3[20] and SiC[21-23]. In the calculations, a strain rate of 105 s−1 is taken which is of a typical value in a plate impact test. Also shown in Fig.4(b) are the predictions from the JH-2 model. It is clear from Fig.4(a) that reasonable agreement is obtained between the present model predictions and the tests results for B4C which are somehow scattered whilst good agreement is achieved between Eq.(4) and the test data for AlN, Al2O3 and SiC as can be seen from Fig.4(b). It is also clear from Fig.4(b) that the JH-2 model has failed to predict the dynamic mechanical behavior of ceramic materials at higher confining pressure.
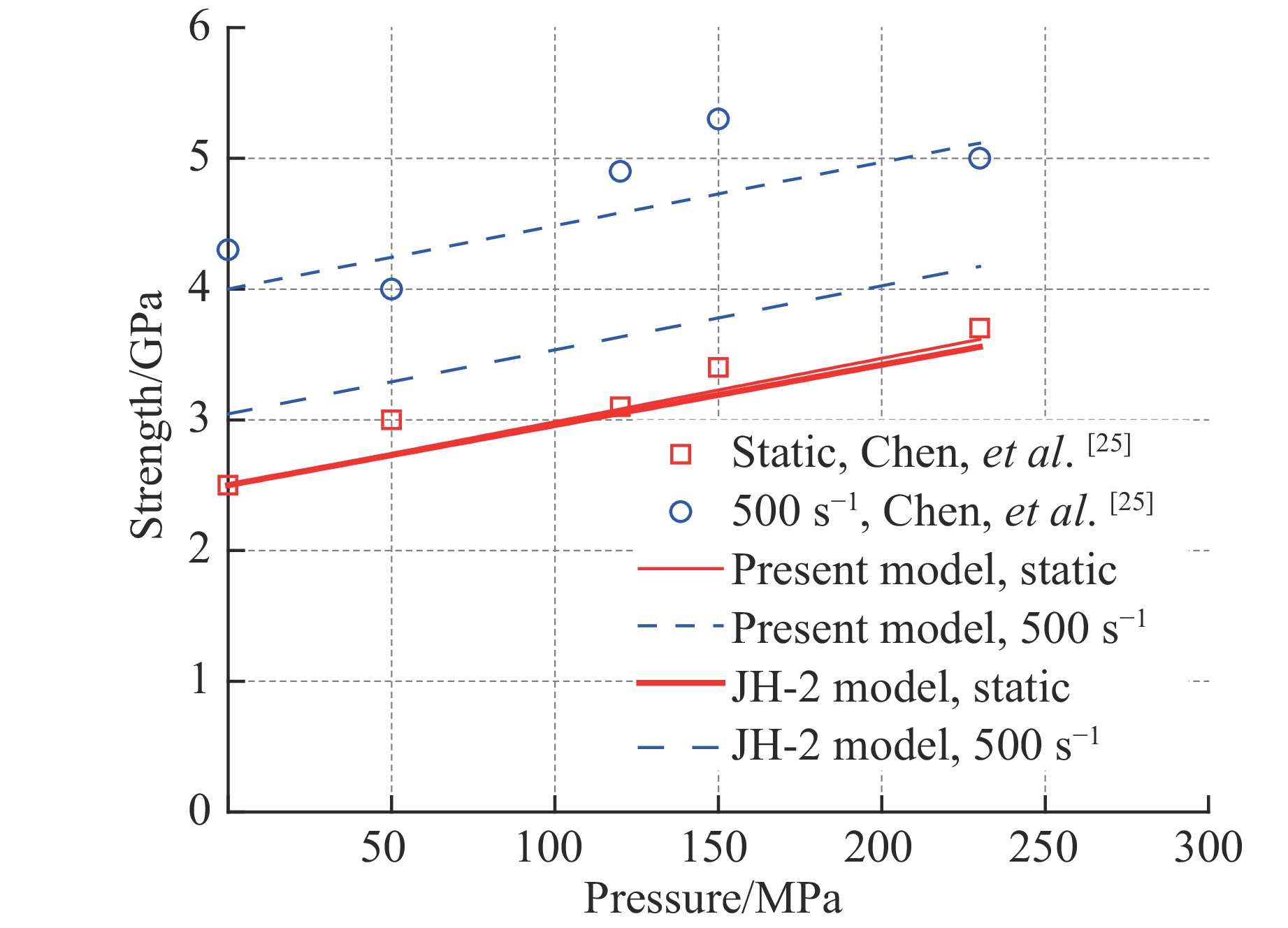
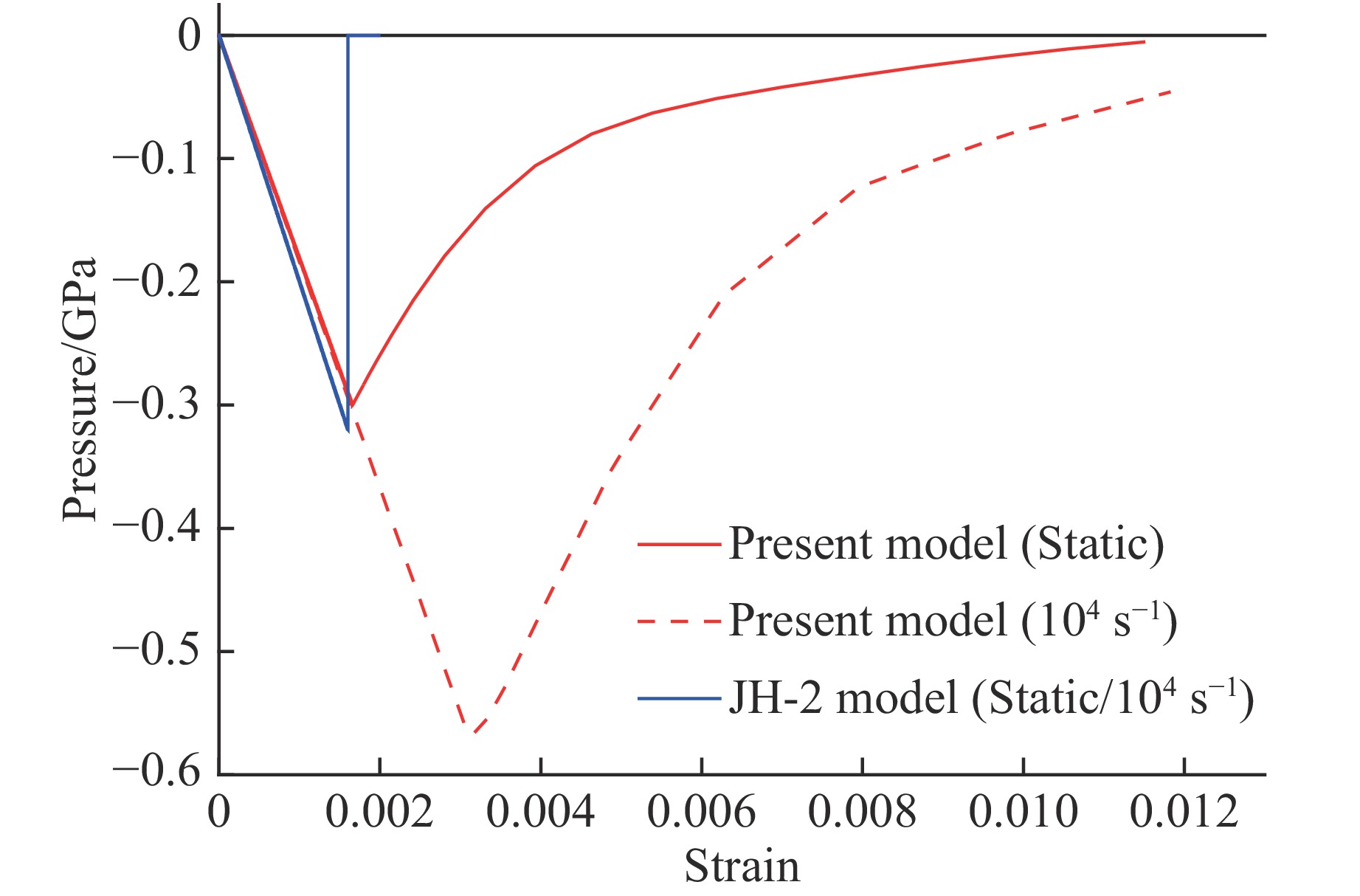
Fig.5 shows comparison of the present model predictions and the experimental data obtained from SHPB (split Hopkinson pressure bar) tests[25]. Also shown in the figure are the predictions from the JH-2 model. In the tests, confining pressures of up to 230 MPa were applied, and a constant strain rate was employed (i.e. 500 s−1). The solid line indicates theoretically predicted quasi-static strength surface, and the broken line designates dynamic strength surface from the present model with a strain rate of 500 s−1. As can be seen from Fig.5 that a good agreement is obtained between the present model predictions and the experimental data whilst the JH-2 model underestimates the strength surface of AlN ceramic under the strain rate of 500 s−1. It should be stressed here that the present model predicts that the quasi-static and dynamic strength surfaces are parallel to each other, which has been confirmed/verified by the experimental data. It leads to further support for the accuracy and validity of the present constitutive model for ceramics.
 Figure 5. Comparison between the experimental data[25] and the predictions by the present model of strength varies with pressure at different strain rates of AlN
Figure 5. Comparison between the experimental data[25] and the predictions by the present model of strength varies with pressure at different strain rates of AlNTo demonstrate the quasi-static behavior of the present constitutive model, numerical tests are performed to evaluate stress-strain relationships for ceramics under various loading conditions, which includes: (a) triaxial compression with different confining pressures, (b) uniaxial tension, (c) biaxial tension and (d) triaxial tension. The numerical tests are carried out using single element simulation approach. Table 1 lists the values of various parameters in the present constitutive model for BeO ceramic. Table 2 and Table 3 list the values of various parameters for AlN ceramic employed in the present model and JH-2 model, respectively. Parameters in the present model are clearly defined in the previous paragraphs, and in JH-2 model. K1, K2, K3 are bulk moduli, and ρc is the density for ceramic material; a, b, C, m, n are strength surface parameters; pHEL, σHEL and μHEL are respectively the pressure, the equivalent stress and the volumetric strain at HEL (Hugoniot elastic limit); T is the maximum tensile hydrostatic pressure the material can withstand; β is strain rate parameter; d1, d2 are damage parameters.
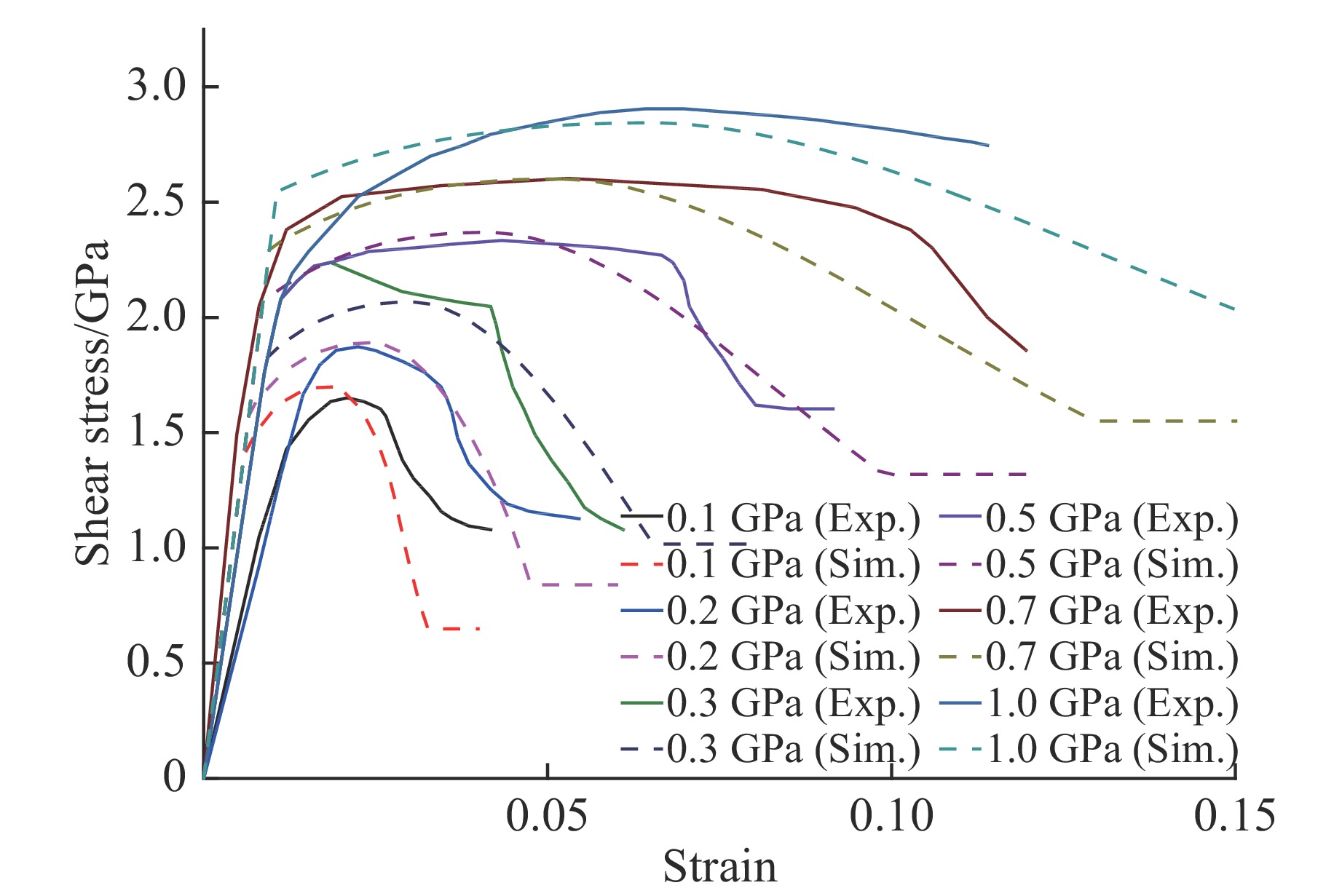
Table 2. Values of parameters for AlN in the present modelEquation of state parameters Constitutive model parameters ρc/(kg·m−3) K1/GPa K2/GPa K3/GPa K4/GPa K5/GPa K6/GPa Fm Wx Wy 3 229 181.5 1 207.9 −2 991 181.9 335.6 −283 3 3.8 2 Constitutive model parameters f′c/GPa ft/GPa B G/GPa S λm λs l r 3 0.3 1.7 127 1.25 0.3 7.5 0.8 0.3 Table 3. Values of various parameters for AlN ceramic (JH-2 model)Equation of state parameters Constitutive model parameters ρc/(kg·m−3) K1/GPa K2/GPa K3/GPa a b C n m 3 229 201 260 0 1.36 1.0 0.013 0.75 0.65 Constitutive model parameters HEL/GPa pHEL/GPa σHEL/GPa μHEL T/GPa β d1 d2 9.0 5.0 6.0 0.024 2 0.32 1.0 0.02 1.85 Fig.6 shows the comparison of the numerically predicted stress-strain curves and the experimental data for BeO under triaxial compression with confinement pressures ranging from 0.1 GPa up to 1.0 GPa, as reported by Heard and Cline[26]. It is clear from Fig.6 that the numerical results are in reasonable agreement with the experimental observations.
 Figure 6. Comparison of stress-strain curves for BeO under triaxial compression between the present model and experimental data[22]
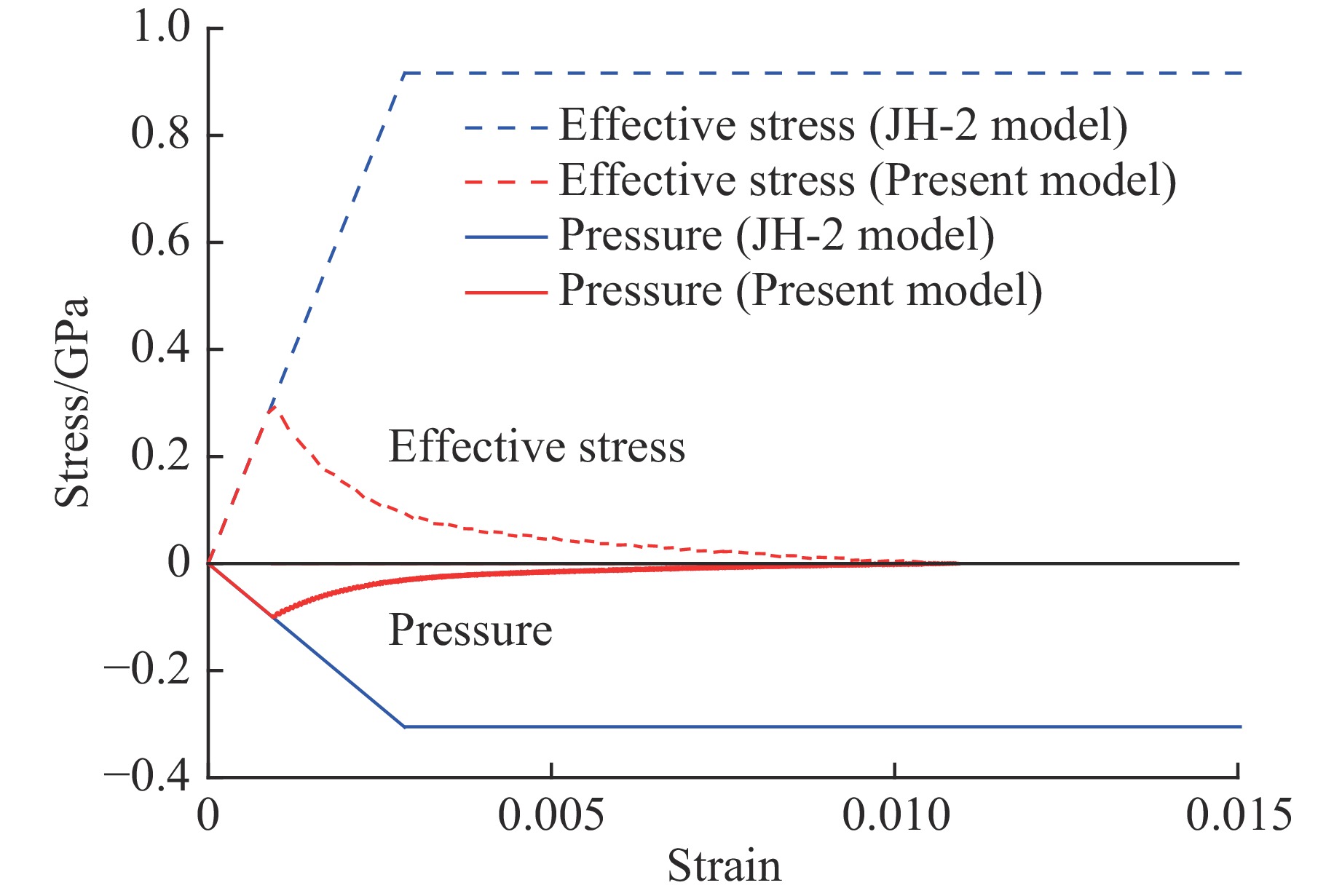
Figure 6. Comparison of stress-strain curves for BeO under triaxial compression between the present model and experimental data[22]Fig.7 shows variations of pressure and effective stress with maximum principal strain of AlN under quasi-static uniaxial tension. It is evident from Fig.7 that the numerical results from the present model give an elastic-brittle softening response of AlN under uniaxial tension with a principal tensile strength of 0.3 GPa for AlN. It is also evident from the figure that the mechanic behavior of the ceramic under uniaxial tension predicted numerically by the JH-2 model is elastic-perfectly plastic with the minimum pressure of 0.31 GPa and the maximum effective stress of 0.93 GPa, which are obviously not in compliance with the actual tensile behavior of the ceramic.
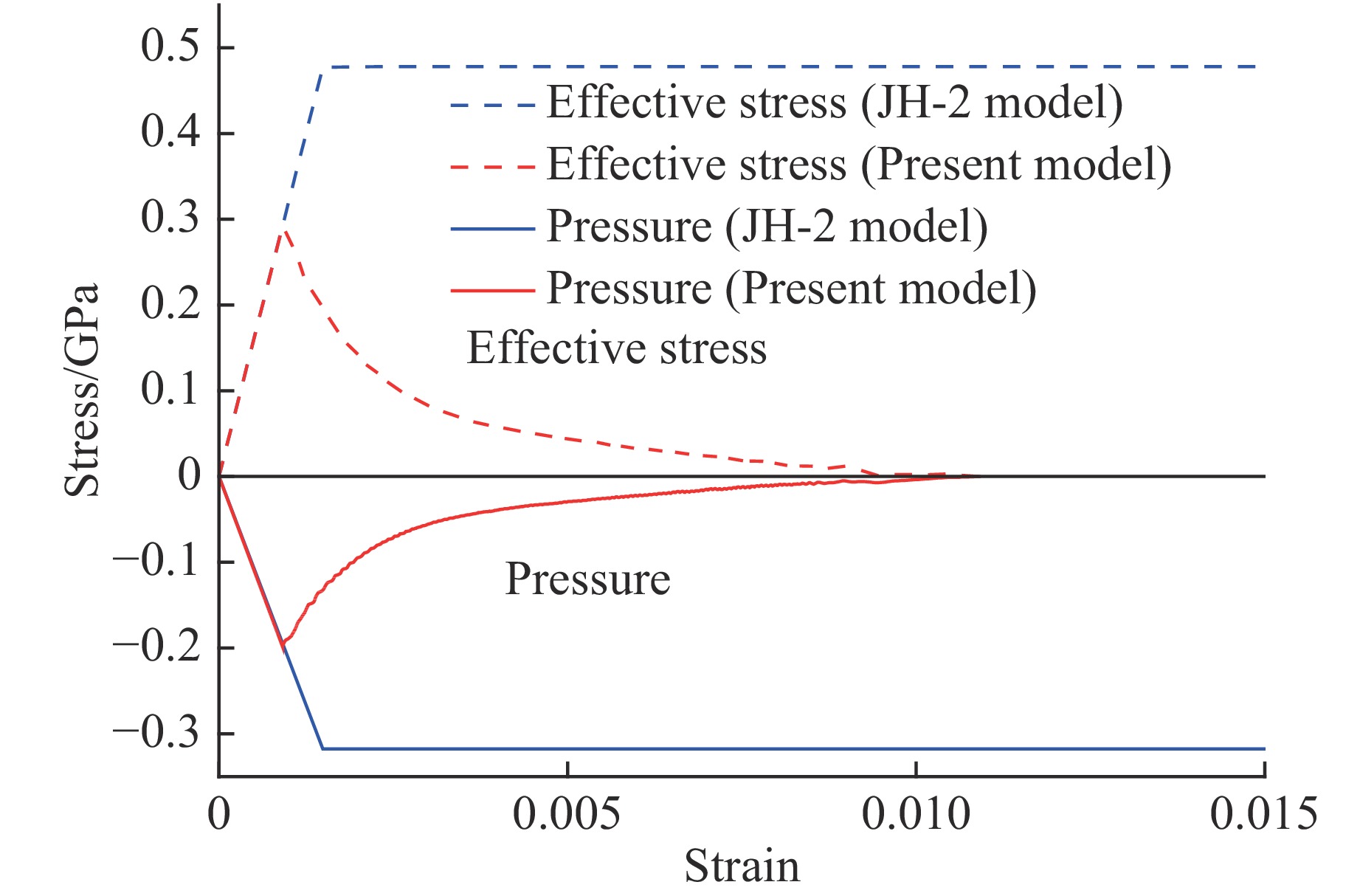
Fig.8 shows variations of pressure and effective stress with maximum principal strain of AlN under quasi-static biaxial tension. Elastic-brittle softening response of the ceramic is predicted numerically using the present model under biaxial tension with a principal tensile strength of 0.3 GPa. On the other hand, an elastic-perfectly plastic response of the ceramic under biaxial tension predicted numerically by the JH-2 model have the minimum pressure of 0.31 GPa and the maximum effective stress of 0.48 GPa.
Fig.9 shows numerically predicted relationship between pressure and the maximum principal strain of AlN under both quasi-static triaxial tension. It can be seen from the figure that the present model predicts the elastic-brittle softening response of AlN under triaxial tension with quasi-static principal tensile strength of 0.3 GPa. Whilst the material is no longer able to withstand any loads after the maximum pressure reached according to the JH-2 model predictions. Furthermore, no strain rate effect is found under hydrostatic tension in the JH-2 model since it hasn’t taken into account the strain rate effect in tension.
3. Penetration of AD99.7/RHA Target
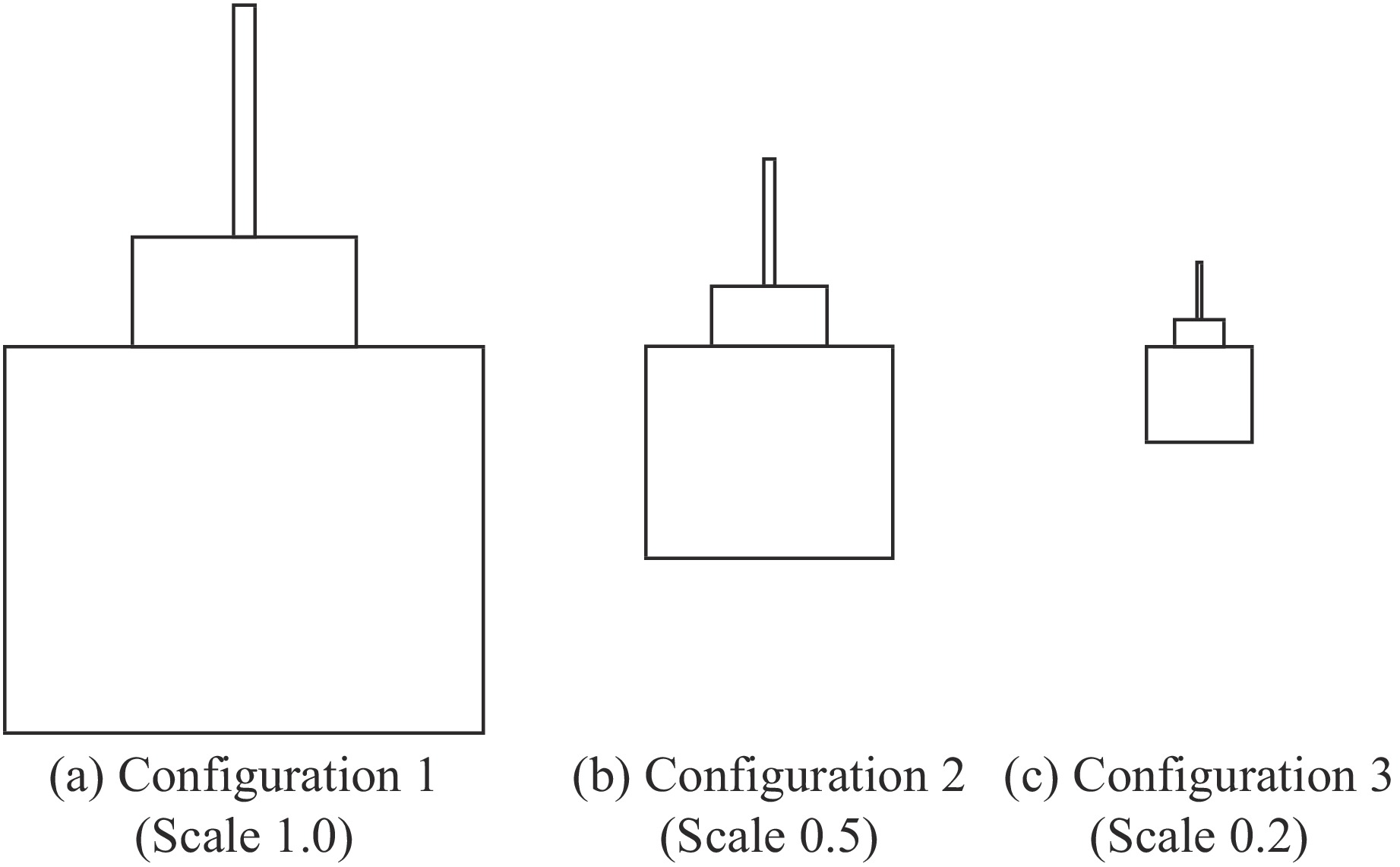
Numerical simulations are conducted in this section for the penetration of AD99.7/RHA target struck by flat-nosed projectiles as reported by Lundberg[35]. In the experiments, long tungsten projectiles with length-to-diameter ratio 15 were fired against the unconfined alumina with steel backing. The tests were carried out in three different scales with projectile lengths 30, 75 and 150 mm (corresponding diameters 2, 5 and 10 mm), respectively. The impact velocities were 1 500 m/s and 2 500 m/s. Fig.10 shows the schematic diagrams of the geometric dimensions of the projectile-target combination. Table 4, Table 5 and Table 6 list the values of various parameters for AD99.7 ceramic material in the present model, tungsten alloy and RHA materials in the JC model. Parameters A1 in the JC model is the initial yield strength under reference strain rate and reference temperature; B1, N1 are respectively the strain hardening modulus and index; C1 is strain rate hardening parameter; M1 is temperature softening index;
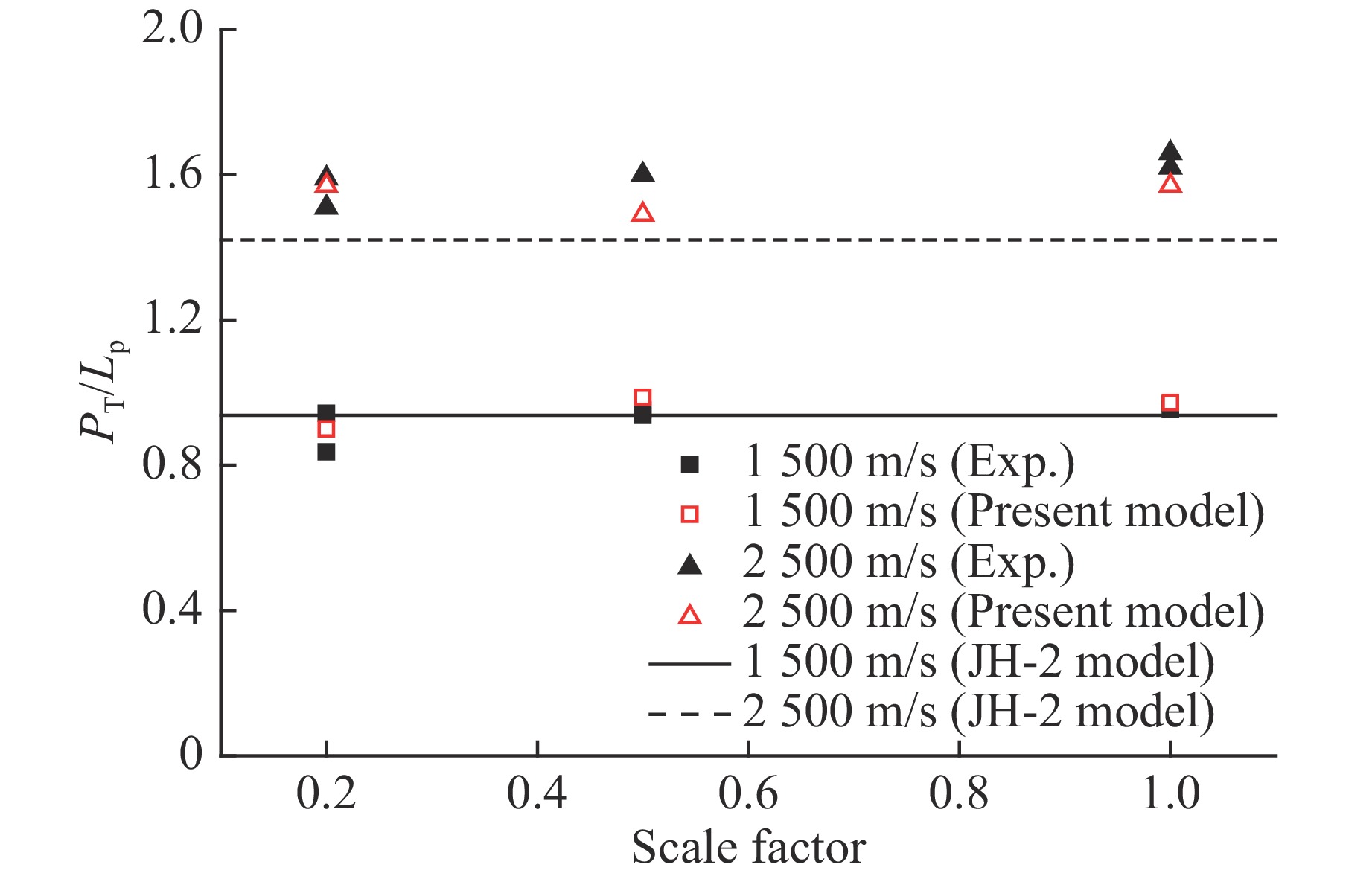
˙ε0 is the reference strain rate; cp is heat capacity; Tm and Tr are the ambient temperature and the reference temperature (melting point), respectively; D1, D2, D3, D4 and D5 are failure criteria parameters; Cs is the intercept of shock wave velocity-particle velocity (us-up) curve; S1, S2, S3 are us-up curveslope coefficient; γ0 is Grüneisen parameter, A0 is the first order volume correction of γ0.Table 4. Values of various parameters for AD99.7 ceramic[35] (The present model)Equation of state parameters Constitutive model parameters ρc/(kg·m−3) K1/GPa K1/GPa K3/GPa Fm Wx Wy S 3 809 181.5 1 207.9 −2 991 3 3.8 2 1.25 Constitutive model parameters f′c/GPa ft/GPa B G/GPa λm λs l r 3 0.3 1.4 135 0.3 7.5 0.8 0.3 Table 5. Values of various parameters for tungsten alloy[35] (JC model)Constitutive model parameters ρc/(kg·m−3) G/GPa A1/GPa B1/GPa N1 C1 M1 ˙ε0/s−1 17 600 122 1.506 0.177 0.12 0.016 1.0 1.0 Constitutive model parameters cp/(J·kg−1·K−1) Tm/K Tr/K D1 D2 D3 D4 D5 134 1 723 300 2.0 0 0 0 0 Equation of state parameters Cs/(m·s−1) S1 S2 S3 γ0 A0 4 029 1.23 0 0 1.54 0.4 Table 6. Values of various parameters for RHA[35] (JC model)Constitutive model parameters ρc/(kg·m−3) G/GPa A1/GPa B1/GPa N1 C1 M1 ˙ε0/s−1 7 800 77 0.792 0.51 0.26 0.014 1.03 1.0 Constitutive model parameters cp/(J·kg−1·K−1) Tm/K Tr/K D1 D2 D3 D4 D5 477 1 793 294 0.05 3.44 −2.12 0.002 0.61 Equation of state parameters Cs/(m·s−1) S1 S2 S3 γ0 A0 4 569 1.49 0 0 2.17 0.460 Fig.11 shows the comparison of total penetration depth between the numerical predictions and the experimental data[35]. PT represents the sum of thickness of the ceramic and penetration depth in the RHA back plate, and Lp is the length of the projectile. In the figure, the solid symbols indicate the experimental data and the hollow symbols represent the numerical results by the present model proposed in this paper. The data of different scales under the impact velocity of 1 500 m/s and 2 500 m/s are designated by square and triangle, respectively. For comparison, the numerical results obtained by Lundberg[35] with JH-2 model are also shown in Fig.11. The solid and broken lines represent the numerical results by JH-2 model at 1 500 m/s and 2 500 m/s respectively. It can be seen from Fig.11 that the numerical results from the present model are in good agreement with the experimental data. As can be seen from the figure that the numerical results for impact velocity of 1 500 m/s are in good agreement with the test data, whilst the results for impact velocity of 2 500 m/s are below the experimental observations[35]. Thus, the JH-2 model has failed to produce consistent results as compared to the experiments.
 Figure 11. Comparison between the numerical results and the test data for the depth of penetration in AD99.7/RHA targets by flat-nosed tungsten alloy penetrators[35]
Figure 11. Comparison between the numerical results and the test data for the depth of penetration in AD99.7/RHA targets by flat-nosed tungsten alloy penetrators[35]4. Conclusions
A dynamic macroscopic constitutive model for ceramic materials has been developed by closely following the previous work on concrete. The model captures the basic features of the mechanical response of ceramic materials including pressure hardening behavior, strain rate effects, strain softening behavior, path dependent behavior (Lode angle), and failure in both low and high confining pressures. In particular, a new function is used to characterize the pressure dependent shear strength surface of ceramic materials which levels out at very high pressures, and strain rate effect is taken into consideration by dynamic increase factor which excludes inertial effect.
The present model has been compared with some available experimental data for ceramic materials. It transpires that the present model predictions are in good agreement with the experimental observations in terms of pressure-volumetric response, triaxial compression, quasi-static strength surface, dynamic strength surface, strain rate effect and depth of penetration. It also transpires that the present model is much more improved than the JH-2 model.
-
表 1 岩石PFC模型细观参数
Table 1. Mesoscopic parameters of the rock PFC model
Minimum radius of particles/mm Ratio of maximum to minimum of radius Density of the particle/
(kg·m−3)Friction coefficient Bond friction angle/(º) 0.3 1.5 2 950 0.17 40 Parallel bonding stiffness ratio Particle stiffness ratio Effective modulus of bonding/GPa Tangential bond strength/MPa Normal bond strength/MPa 1.1 1.1 11 76.6 69.6 Method Deviatoric stress/MPa Elastic modulus/MPa Indoor test[16] 144.782 17.147 Numerical simulation 144.670 17.109 Error/% 0.077 0.222 表 3 不同裂隙长度的岩样在不同围压下的接触力链演化过程
Table 3. Evolution of contact force chain of rock samples under different confining pressures and fissure lengths
L/mm Confining pressure/MPa Pre-peak period Peak value Post-peak period 0 5.0 


10.0 


5 5.0 


10.0 


10 5.0 


10.0 


表 4 不同裂隙长度岩样的峰值点能量指标
Table 4. Indexes of peak point energy of rock samples with different fissure lengths
L/mm Confining pressure/MPa Total energy/kJ Dissipated energy Energy/kJ Proportion/% 0 2.5 288.67 9.54 3.30 5.0 324.79 10.41 3.21 10.0 415.48 14.47 3.48 15.0 474.29 21.31 4.49 5 2.5 248.58 8.24 3.31 5.0 281.97 9.11 3.23 10.0 348.54 12.51 3.59 15.0 355.56 12.34 3.47 10 2.5 172.37 4.89 2.84 5.0 205.55 7.32 3.56 10.0 279.90 9.16 3.27 15.0 293.19 10.24 3.49 15 2.5 153.98 5.86 3.8 5.0 169.91 5.02 2.95 10.0 215.02 7.95 3.70 15.0 240.84 10.34 4.29 -
[1] 李泓颖, 刘晓辉, 郑钰, 等. 深埋锦屏大理岩渐进破坏过程中的特征能量分析 [J]. 岩石力学与工程学报, 2022, 41(Suppl 2): 3229–3239.LI H Y, LIU X H, ZHENG Y, et al. Analysis of characteristic energy during the progressive failure of deep-buried marble in Jinping [J]. Chinese Journal of Rock Mechanics and Engineering, 2022, 41(Suppl 2): 3229–3239. [2] 刘鹏飞, 范俊奇, 郭佳奇, 等. 三轴应力下花岗岩加载破坏的能量演化和损伤特征 [J]. 高压物理学报, 2021, 35(2): 024102. doi: 10.11858/gywlxb.20200622LIU P F, FAN J Q, GUO J Q, et al. Damage and energy evolution characteristics of granite under triaxial stress [J]. Chinese Journal of High Pressure Physics, 2021, 35(2): 024102. doi: 10.11858/gywlxb.20200622 [3] LI E B, GAO L, JIANG X Q, et al. Analysis of dynamic compression property and energy dissipation of salt rock under three-dimensional pressure [J]. Environmental Earth Sciences, 2019, 78(14): 388. doi: 10.1007/s12665-019-8389-7 [4] DU X H, XUE J H, SHI Y, et al. Triaxial mechanical behaviour and energy conversion characteristics of deep coal bodies under confining pressure [J]. Energy, 2023, 266: 126443. doi: 10.1016/j.energy.2022.126443 [5] 刘之喜, 孟祥瑞, 赵光明, 等. 真三轴压缩下砂岩的能量和损伤分析 [J]. 岩石力学与工程学报, 2023, 42(2): 327–341.LIU Z X, MENG X R, ZHAO G M, et al. Energy and damage analysis of sandstone under true triaxial compression [J]. Chinese Journal of Rock Mechanics and Engineering, 2023, 42(2): 327–341. [6] ZHANG Y, FENG X T, ZHANG X W, et al. Strain energy evolution characteristics and mechanisms of hard rocks under true triaxial compression [J]. Engineering Geology, 2019, 260: 105222. doi: 10.1016/j.enggeo.2019.105222 [7] 王星辰, 王志亮, 黄佑鹏, 等. 预制裂隙岩样宏细观力学行为颗粒流数值模拟 [J]. 水文地质工程地质, 2021, 48(4): 86–92.WANG X C, WANG Z L, HUANG Y P, et al. Particle flow simulation of macro- and meso-mechanical behavior of the prefabricated fractured rock sample [J]. Hydrogeology & Engineering Geology, 2021, 48(4): 86–92. [8] 方前程, 周科平, 刘学服. 不同围压下断续节理岩体破坏机制的颗粒流分析 [J]. 中南大学学报(自然科学版), 2014, 45(10): 3536–3543.FANG Q C, ZHOU K P, LIU X F. Failure mechanism of discontinuous joint rock mass under different confining pressures based on particle flow code [J]. Journal of Central South University (Science and Technology), 2014, 45(10): 3536–3543. [9] 黄明智, 李新平, 王刚, 等. 三轴压缩条件下单裂隙花岗岩破坏特性研究 [J]. 地下空间与工程学报, 2022, 18(4): 1208–1218.HUANG M Z, LI X P, WANG G, et al. Study on the failure characteristics of single-fissured granite under triaxial compression condition [J]. Chinese Journal of Underground Space and Engineering, 2022, 18(4): 1208–1218. [10] SONG L B, WANG G, WANG X K, et al. The influence of joint inclination and opening width on fracture characteristics of granite under triaxial compression [J]. International Journal of Geomechanics, 2022, 22(5): 04022031. doi: 10.1061/(ASCE)GM.1943-5622.0002372 [11] 陈鹏宇, 孔莹, 余宏明. 岩石单轴压缩PFC2D模型细观参数标定研究 [J]. 地下空间与工程学报, 2018, 14(5): 1240–1249.CHEN P Y, KONG Y, YU H M. Research on the calibration method of microparameters of a uniaxial compression PFC2D model for rock [J]. Chinese Journal of Underground Space and Engineering, 2018, 14(5): 1240–1249. [12] 张亮, 王桂林, 雷瑞德, 等. 单轴压缩下不同长度单裂隙岩体能量损伤演化机制 [J]. 中国公路学报, 2021, 34(1): 24–34. doi: 10.3969/j.issn.1001-7372.2021.01.003ZHANG L, WANG G L, LEI R D, et al. Energy damage evolution mechanism of single jointed rock mass with different lengths under uniaxial compression [J]. China Journal of Highway and Transport, 2021, 34(1): 24–34. doi: 10.3969/j.issn.1001-7372.2021.01.003 [13] 周杰, 汪永雄, 周元辅. 基于颗粒流的砂岩三轴破裂演化宏-细观机理 [J]. 煤炭学报, 2017, 42(Suppl 1): 76–82.ZHOU J, WANG Y X, ZHOU Y F. Macro-micro evolution mechanism on sandstone failure in triaxial compression test based on PFC2D [J]. Journal of China Coal Society, 2017, 42(Suppl 1): 76–82. [14] 龙恩林, 陈俊智. 花岗岩颗粒流模型循环压缩作用下能量特征分析 [J]. 中国安全生产科学技术, 2019, 15(10): 95–100. doi: 10.11731/j.issn.1673-193x.2019.10.015LONG E L, CHEN J Z. Analysis on energy characteristics of granite particle flow model under cyclic compression [J]. Journal of Safety Science and Technology, 2019, 15(10): 95–100. doi: 10.11731/j.issn.1673-193x.2019.10.015 [15] 李晓锋, 李海波, 夏祥, 等. 类节理岩石直剪试验力学特性的数值模拟研究 [J]. 岩土力学, 2016, 37(2): 583–591.LI X F, LI H B, XIA X, et al. Numerical simulation of mechanical characteristics of jointed rock in direct shear test [J]. Rock and Soil Mechanics, 2016, 37(2): 583–591. [16] 刘剑. 闪长岩加卸荷失稳破裂前兆信息研究 [D]. 昆明: 昆明理工大学, 2022.LIU J. Study on the precursor information of diorite plus unloading instability rupture [D]. Kunming: Kunming University of Science and Technology, 2022. [17] CHEN Z Q, HE C, MA G Y, et al. Energy damage evolution mechanism of rock and its application to brittleness evaluation [J]. Rock Mechanics and Rock Engineering, 2019, 52(4): 1265–1274. doi: 10.1007/s00603-018-1681-0 [18] WANG Q S, CHEN J X, GUO J Q, et al. Acoustic emission characteristics and energy mechanism in karst limestone failure under uniaxial and triaxial compression [J]. Bulletin of Engineering Geology and the Environment, 2019, 78(3): 1427–1442. doi: 10.1007/s10064-017-1189-y [19] YANG S Q, LIU X R, JING H W. Experimental investigation on fracture coalescence behavior of red sandstone containing two unparallel fissures under uniaxial compression [J]. International Journal of Rock Mechanics and Mining Sciences, 2013, 63: 82–92. doi: 10.1016/j.ijrmms.2013.06.008 [20] ZHANG X P, JIANG Y J, WANG G, et al. Mechanism of shear deformation, failure and energy dissipation of artificial rock joint in terms of physical and numerical consideration [J]. Geosciences Journal, 2019, 23(3): 519–529. doi: 10.1007/s12303-018-0043-y [21] FENG Q, JIN J C, ZHANG S, et al. Study on a damage model and uniaxial compression simulation method of frozen-thawed rock [J]. Rock Mechanics and Rock Engineering, 2022, 55(1): 187–211. doi: 10.1007/s00603-021-02645-2 [22] 张志镇, 高峰. 受载岩石能量演化的围压效应研究 [J]. 岩石力学与工程学报, 2015, 34(1): 1–11.ZHANG Z Z, GAO F. Confining pressure effect on rock energy [J]. Chinese Journal of Rock Mechanics and Engineering, 2015, 34(1): 1–11. -







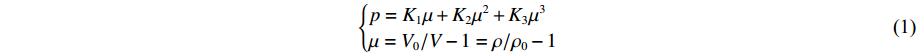
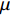
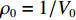
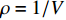
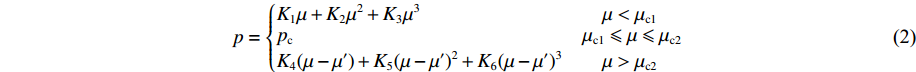
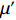
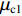
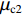
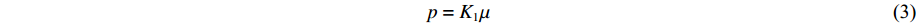
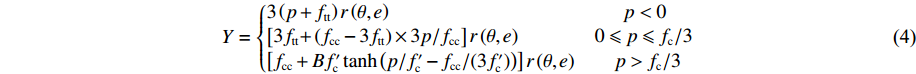
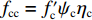
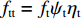
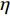
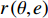

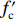
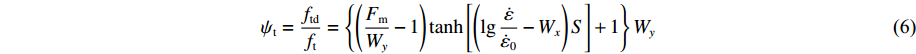
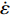
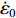
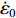
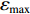

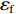
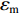
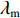
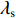
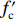
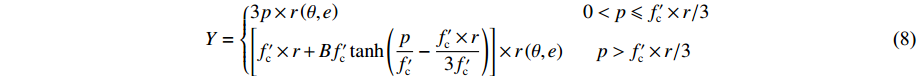
 DownLoad:
DownLoad:
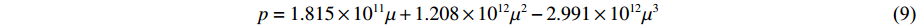
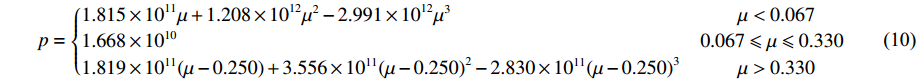

 DownLoad:
DownLoad:








 下载:
下载: