含损伤的无机防弹玻璃的JH2本构模型

史刘彤; 黄友奇; 高玉波; 贾哲; 李志豪

doi:10.11858/gywlxb.20240704

含损伤的无机防弹玻璃的JH2本构模型

doi: 10.11858/gywlxb.20240704

史刘彤^1,,
黄友奇²,
高玉波^1,*, ,,
贾哲³,
李志豪¹

1.
中北大学航空宇航学院, 山西太原　030051
2.
中国建筑材料科学研究总院有限公司, 北京　100024
3.
中国航天科工集团第六研究院, 内蒙古呼和浩特　010010

基金项目: 国家自然科学基金（12172337）；山西省基础研究计划（20210302123022）；中北大学研究生科技立项（20231976）

详细信息

作者简介:
史刘彤（1999－），女，硕士研究生，主要从事防弹玻璃的本构关系及损伤机理研究. E-mail：1456185422@qq.com

通讯作者:
高玉波（1986－），男，博士，副教授，主要从事穿甲动力学、材料本构关系与损伤机理研究. E-mail：gaoyb@nuc.edu.cn

中图分类号: O345; TB321
计量
- 文章访问数: 171
- HTML全文浏览量: 86
- PDF下载量: 29
出版历程
- 收稿日期: 2024-01-03
- 修回日期: 2024-02-04
- 录用日期: 2024-03-15
- 刊出日期: 2024-07-25

JH2 Constitutive Model of Inorganic Bulletproof Glass with Damage

SHI Liutong^1
,,
HUANG Youqi²,
GAO Yubo^{1,*
, ,},
JIA Zhe³,
LI Zhihao¹

1.
School of Aerospace Engineering, North University of China, Taiyuan 030051, Shanxi, China
2.
China Building Materials Academy, Beijing 100024, China
3.
The Sixth Academy of China Aerospace Science and Industry Corporation Limited, Hohhot 010010, Inner Mongolia, China

摘要

摘要: 防弹玻璃具有良好的抗冲击性能，能够抵御枪弹、爆炸碎片以及其他高速飞行物体的攻击性威胁，广泛应用于安全防护领域。为探究防弹玻璃的无机玻璃层在冲击加载下的动态力学性能及本构关系，首先，采用电子万能试验机和分离式霍普金森压杆（split Hopkinson pressure bar, SHPB）试验装置，获得了不同应变率下材料的拉伸和压缩力学性能，结果表明，无机玻璃具有明显的应变率效应，材料强度随应变率的升高而增大。其次，借鉴土力学三轴围压试验，设计了适用于本研究的高强围压套筒，测试了完全损伤条件下玻璃颗粒的力学性能，发现其强度明显低于完整状态下无机玻璃的强度。最后，结合试验数据构建了含损伤无机玻璃的JH2本构模型，采用非线性有限元软件 LS-DYNA 模拟了材料在SHPB加载下的压缩过程，通过对比试验结果与模拟结果，验证了本构模型的有效性。
- 无机玻璃 /
- 力学性能 /
- 本构关系 /
- 损伤
Abstract: Bulletproof glass exhibits excellent impact resistance and protective capabilities against bullets, explosive fragments, high-speed projectiles, and various other aggressive threats, making it extensively utilized in the field of safety and security. To investigate the dynamic mechanical properties and constitutive relation of the inorganic glass layers in bulletproof glass under impact loading, we firstly employed an electronic universal testing machine and a split Hopkinson pressure bar (SHPB) test setup to obtain the tensile and compressive mechanical properties of the material at different strain rates. Results reveal a noticeable strain rate effect that the material’s strength increases with the strain rate. Secondly, drawing on the experience of geotechnical triaxial compression tests, we designed a high-strength confinement sleeve suitable for assessing the mechanical properties of glass particles under conditions of complete damage. Results show a significantly lower strength compared to that of the intact state of inorganic glass. Finally, by integrating test data, an JH2 constitutive model for inorganic glass with damage was established. By using the non-linear finite element software LS-DYNA, the SHPB test process was simulated. The effectiveness of the constitutive model was verified by comparing test and simulated results.
- bulletproof glass /
- mechanical properties /
- constitutive relation /
- damage

HTML全文

功能梯度材料(Functionally Graded Materials, FGM)的概念是1984年在航空飞机计划中首次提出的^[1]，FGM的特性在于它的组成和结构随着体积的变化而变化，从而导致材料相应性质发生改变。因其材料特性呈幂律分布^[2-3]，FGM被广泛应用于工程领域，如航空航天、机械工程、生物医学等。圆柱壳在联合荷载作用下的屈曲分析备受学术界关注^[4-5]。目前，对FGM板壳的研究较为深入^[6-8]。Beni等^[9]利用改进的偶应力理论，对FGM圆柱壳在不同边界条件下的动力屈曲进行了分析；Kargarnovin等^[10]研究了轴向荷载作用下FGM圆柱壳的动力屈曲；Sofiyev等^[11]研究了横向压力下功能梯度正交各向异性圆柱壳的动力屈曲，推导出基于一阶剪切变形理论的功能梯度正交各向异性圆柱壳的稳定性和相容性方程；Khazaeinejad等^[12]研究了弹性模量在厚度方向上连续变化的FGM圆柱壳在复合外压和轴向压缩载荷作用下的动力屈曲；Khalili等^[13]研究了横向冲击载荷作用下FGM圆柱壳的动力屈曲；Alashti等^[14]对变厚度FGM圆柱壳外压和轴向压缩的动力屈曲问题进行了分析。

基于以上研究，本研究讨论了FGM圆柱壳在轴向荷载作用下的动力屈曲。根据Donnell壳体理论和经典板壳理论，利用Hamilton变分原理得到FGM圆柱壳的动力屈曲控制方程；采用分离变量法求得动力屈曲临界荷载表达式；通过MATLAB软件计算动力屈曲临界荷载，讨论由不同材料(陶瓷和钛、陶瓷和铁、陶瓷和铜)组成的FGM圆柱壳的径厚比(R/h)、梯度指数(k)、环向模态数(m)、轴向模态数(n)等对临界荷载的影响。

1. FGM圆柱壳的屈曲控制方程

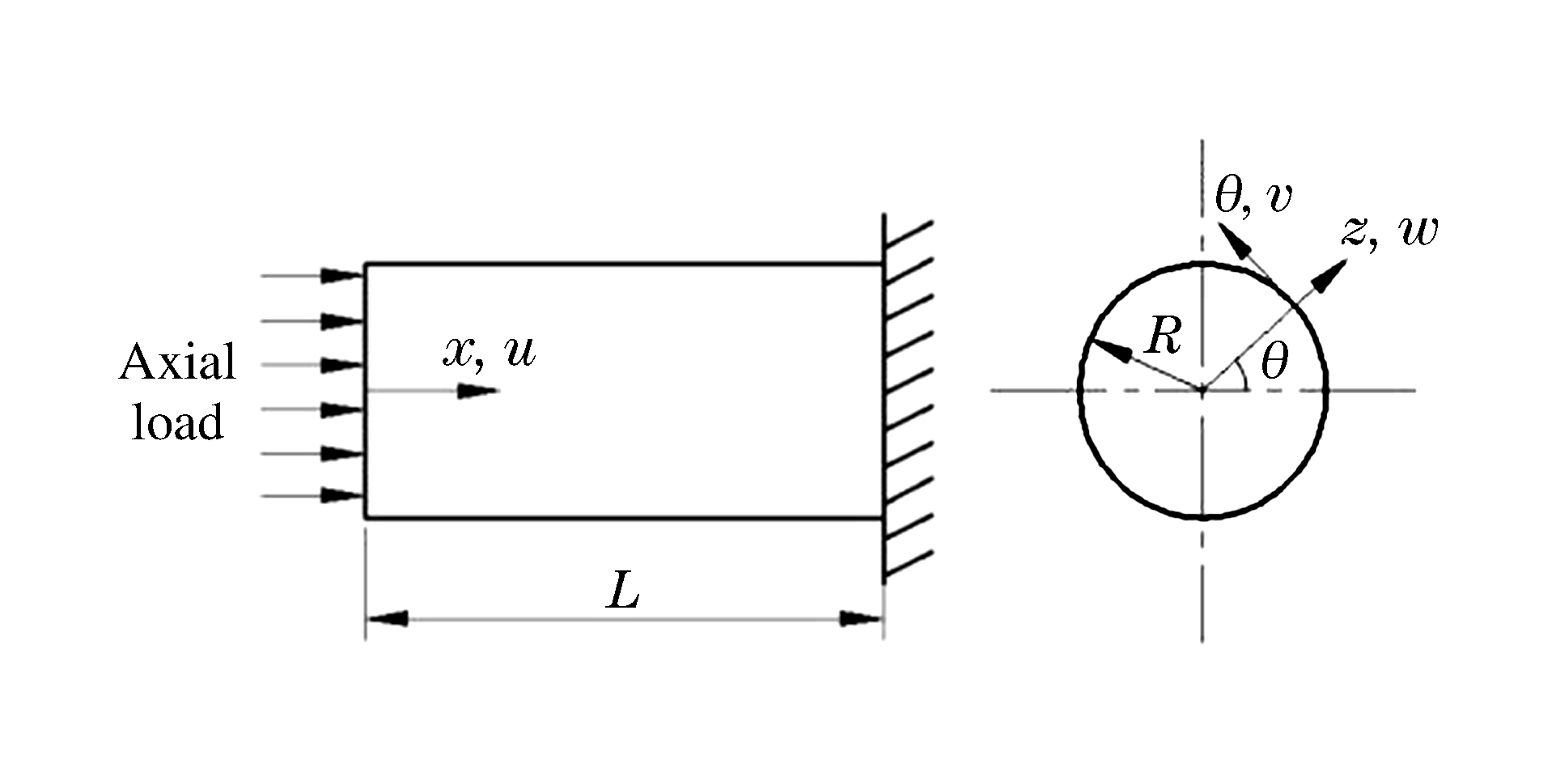
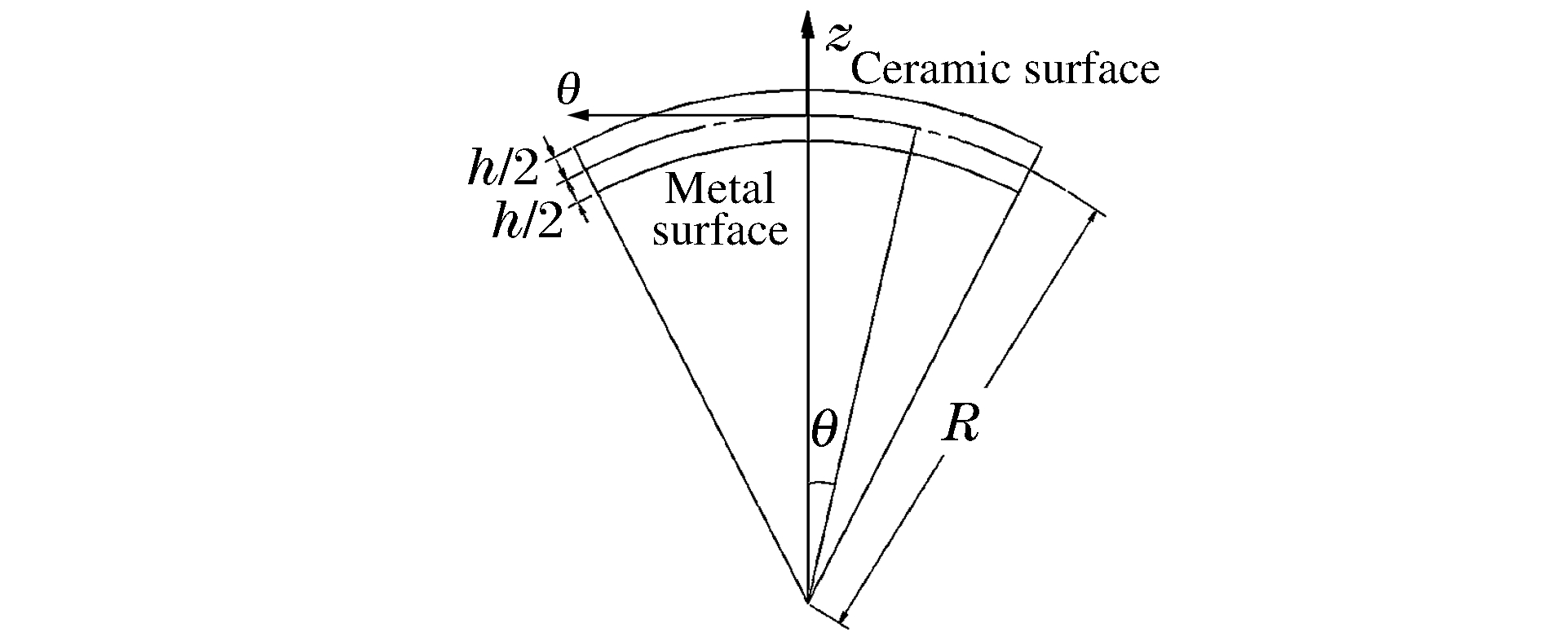
如图 1所示，FGM圆柱壳长度为l，半径为R，总厚度为h，选取柱坐标系(x, θ, z)，其相应位移为(u, v, w)。FGM的材料属性(弹性模量E、密度ρ、泊松比μ等)呈幂律分布^[2-3]，表示为

$P\left( z \right) = \left( {{P_1} - {P_2}} \right){\left( {\frac{{2z + h}}{{2h}}} \right)^k} + {P_2}$

(1)

图 1 圆柱壳坐标系统

Figure 1. Cylindrical shell coordinates

下载: 全尺寸图片幻灯片

式中：P为物性参数，下标“1”和“2”分别代表组分1和组分2；k为梯度指数，k∈(0, ∞)。圆柱壳内任意点的物性参数为

$\left\{ \begin{array}{l} E\left( z \right) = \left( {{E_1} - {E_2}} \right){\left( {\frac{{2z + h}}{{2h}}} \right)^k} + {E_2}\\ \rho \left( z \right) = \left( {{\rho _1} - {\rho _2}} \right){\left( {\frac{{2z + h}}{{2h}}} \right)^k} + {\rho _2}\\ \mu \left( z \right) = \left( {{\mu _1} - {\mu _2}} \right){\left( {\frac{{2z + h}}{{2h}}} \right)^k} + {\mu _2} \end{array} \right.$

(2)

根据Donnell壳体理论，圆柱壳的小挠度几何方程为

$\begin{array}{*{20}{c}} {\left\{ \begin{array}{l} {\varepsilon _x} = \varepsilon _x^0 + z{K_x}\\ {\varepsilon _\theta } = \varepsilon _\theta ^0 + z{K_\theta }\\ {\gamma _{x\theta }} = \gamma _{x\theta }^0 + z{K_{x\theta }} \end{array} \right.,}&{\left\{ \begin{array}{l} u = {u_0} - z\frac{{\partial w}}{{\partial x}}\\ v = {v_0} - z\frac{{\partial w}}{{R\partial \theta }}\\ w = {w_0} \end{array} \right.,}&{\left\{ \begin{array}{l} \varepsilon _x^0 = \frac{{\partial {u_0}}}{{\partial x}}\\ \varepsilon _\theta ^0 = \frac{{\partial {v_0}}}{{R\partial \theta }} - \frac{{{w_0}}}{R}\\ \gamma _{x\theta }^0 = \frac{{\partial {u_0}}}{{R\partial \theta }} - \frac{{\partial {v_0}}}{{\partial x}} \end{array} \right.,}&{\left\{ \begin{array}{l} {K_x} = - \frac{{{\partial ^2}{w_0}}}{{\partial {x^2}}}\\ {K_\theta }{\rm{ = }} - \frac{{{\partial ^2}{w_0}}}{{{R^2}\partial {\theta ^2}}}\\ {K_{x\theta }}{\rm{ = }} - \frac{{2{\partial ^2}{w_0}}}{{R\partial x\partial \theta }} \end{array} \right.} \end{array}$

(3)

式中：ε为正应变，γ为切应变，上、下标“0”表示壳体中面，K为壳体曲率。

根据经典板壳理论，FGM圆柱壳的内力N与内力矩M可表示为

$\left( {\begin{array}{*{20}{c}} {{N_x}}\\ {{N_\theta }}\\ {{N_{x\theta }}}\\ {{M_x}}\\ {{M_\theta }}\\ {{M_{x\theta }}} \end{array}} \right) = \left( {\begin{array}{*{20}{c}} {{A_{11}}}&{{A_{12}}}&{{A_{16}}}&{{B_{11}}}&{{B_{12}}}&{{B_{16}}}\\ {{A_{21}}}&{{A_{22}}}&{{A_{26}}}&{{B_{21}}}&{{B_{22}}}&{{B_{26}}}\\ {{A_{16}}}&{{A_{26}}}&{{A_{66}}}&{{B_{16}}}&{{B_{26}}}&{{B_{66}}}\\ {{B_{11}}}&{{B_{12}}}&{{B_{16}}}&{{D_{11}}}&{{D_{12}}}&{{D_{16}}}\\ {{B_{21}}}&{{B_{22}}}&{{B_{26}}}&{{D_{21}}}&{{D_{22}}}&{{D_{26}}}\\ {{B_{16}}}&{{B_{26}}}&{{B_{66}}}&{{D_{16}}}&{{D_{26}}}&{{D_{66}}} \end{array}} \right)\left( {\begin{array}{*{20}{c}} {\varepsilon _x^0}\\ {\varepsilon _\theta ^0}\\ {\gamma _{x\theta }^0}\\ {{K_x}}\\ {{K_\theta }}\\ {{K_{x\theta }}} \end{array}} \right)$

(4)

式中：A_ij、B_ij、D_ij(i, j=1, 2, 6)分别为FGM圆柱壳的拉伸刚度、耦合刚度和弯曲刚度系数矩阵分量。 ${A_{11}} = {A_{22}} = \int_{ - h/2}^{h/2} {\frac{{E\left( z \right)}}{{1 - {\mu ^2}\left( z \right)}}} {\rm{d}}z$ , ${A_{12}} = {A_{21}} = \int_{ - h/2}^{h/2} {\frac{{\mu \left( z \right)E\left( z \right)}}{{1 - {\mu ^2}\left( z \right)}}} {\rm{d}}z$ , ${A_{66}} = \int_{ - h/2}^{h/2} {\frac{{E\left( z \right)}}{{2\left[ {1 + \mu \left( z \right)} \right]}}} {\rm{d}}z$ , ${B_{11}} = {B_{22}} = \int_{ - h/2}^{h/2} {\frac{{E\left( z \right)}}{{1 - {\mu ^2}\left( z \right)}}z\;}{\rm{d}}z$ , ${B_{12}} = {B_{21}} = \int_{ - h/2}^{h/2} {\frac{{\mu \left( z \right)E\left( z \right)}}{{1 - {\mu ^2}\left( z \right)}}z\;} {\rm{d}}z$ , ${B_{66}} = \int_{ - h/2}^{h/2} {\frac{{E\left( z \right)}}{{2\left[ {1 + \mu \left( z \right)} \right]}}z\;} {\rm{d}}z$ , ${D_{11}} = {D_{22}} = \int_{ - h/2}^{h/2} {\frac{{E\left( z \right)}}{{1 - {\mu ^2}\left( z \right)}}{z^2}\;} {\rm{d}}z$ , ${D_{12}} = {D_{21}} = \int_{ - h/2}^{h/2} {\frac{{\mu \left( z \right)E\left( z \right)}}{{1 - {\mu ^2}\left( z \right)}}{z^2}\;} {\rm{d}}z$ , ${D_{66}} = \int_{ - h/2}^{h/2} {\frac{{E\left( z \right)}}{{2\left[ {1 + \mu \left( z \right)} \right]}}{z^2}\;} {\rm{d}}z$ 。FGM圆柱壳的力学性能为各向同性^[15]，那么：A₁₆=A₂₆=B₁₆=B₂₆=D₁₆=D₂₆=0。

对于圆柱壳，系统的应变能(不考虑剪力)为

$U = \frac{1}{2}\int_0^{2{\rm{ \mathsf{ π} }}} {\int_0^l {\left( {{N_x}\varepsilon _x^0 + {N_\theta }\varepsilon _\theta ^0 + {N_{x\theta }}\gamma _{x\theta }^0 + {M_x}{K_x} + {M_\theta }{K_\theta } + {M_{x\theta }}{K_{x\theta }}} \right)} R{\rm{d}}x{\rm{d}}\theta }$

(5)

动能为

$T = \frac{1}{2}\int_{ - h/2}^{h/2} {\int_0^{2{\rm{ \mathsf{ π} }}} {\int_0^l {\rho \left( z \right)\left[ {{{\left( {\frac{{\partial u}}{{\partial t}}} \right)}^2} + {{\left( {\frac{{\partial v}}{{\partial t}}} \right)}^2} + {{\left( {\frac{{\partial w}}{{\partial t}}} \right)}^2}} \right]R{\rm{d}}x{\rm{d}}\theta {\rm{d}}z} } }$

(6)

外力功为

$W = \frac{1}{2}\int_0^{2{\rm{ \mathsf{ π} }}} {\int_0^l {N\left( t \right){{\left( {\frac{{\partial {w_0}}}{{\partial x}}} \right)}^2}} R{\rm{d}}x{\rm{d}}\theta }$

(7)

Hamilton变分原理为

${\rm{ \mathsf{ δ} }}\int_{{t_2}}^{{t_1}} {\left( {T - U + W} \right){\rm{d}}t} = 0$

(8)

将(3)式~(7)式代入(8)式中，由Donnell壳体理论可知，圆柱壳内力沿环向均匀分布，忽略中面位移^[16]，由u₀、v₀、w₀的变分系数为零，整理得到FGM圆柱壳的动力屈曲控制方程为

$\begin{array}{l} 4{I_0}\frac{{{\partial ^2}{w_0}}}{{\partial t}} - {I_2}\left( {\frac{{{\partial ^4}{w_0}}}{{{R^2}\partial {\theta ^2}\partial {t^2}}} + \frac{{{\partial ^4}{w_0}}}{{\partial {x^2}\partial {t^2}}}} \right) = - 4{A_{22}}\frac{{{w_0}}}{{{R^2}}} - 4\frac{{{B_{12}}}}{{{R^2}}}\frac{{{\partial ^2}{w_0}}}{{\partial {x^2}}} - 4\frac{{{B_{22}}}}{R}\frac{{{\partial ^2}{w_0}}}{{{R^2}\partial {\theta ^2}}} - {D_{11}}\frac{{{\partial ^4}{w_0}}}{{\partial {x^4}}} - \\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;2\left( {{D_{12}} + 2{D_{66}}} \right)\frac{{{\partial ^4}{w_0}}}{{{R^2}\partial {x^2}\partial {\theta ^2}}} - {D_{22}}\frac{{{\partial ^4}{w_0}}}{{{R^4}\partial {\theta ^4}}} + N\left( t \right)\frac{{{\partial ^2}{w_0}}}{{\partial {x^2}}} \end{array}$

(9)

2. 控制方程的求解

设径向位移表示为^[17]

$w = Y\left( x \right)T\left( t \right){{\rm{e}}^{{\rm{i}}m\theta }}$

(10)

将(10)式代入(9)式中，分离变量得

$\left\{ \begin{array}{l} {Y^{\left( 4 \right)}} = {\alpha ^2}Y'' + {\beta ^2}Y = 0\\ \ddot T - \lambda T = 0 \end{array} \right.$

(11)

其中

${\alpha ^2} = \frac{1}{{{D_{11}}}}\left[ {4\frac{{{B_{12}}}}{{{R^2}}} + \frac{2}{{{R^2}}}\left( {{D_{12}} + 2{D_{66}}} \right){m^2} - N\left( t \right) - {I_2}\lambda } \right]$

(12)

${\beta ^2} = \frac{1}{{{D_{11}}}}\left[ {4\frac{{{A_{22}}}}{{{R^2}}} + 4\frac{{{B_{22}}}}{{{R^3}}} + \frac{{{D_{22}}}}{{{R^4}}}{m^4} + 4{I_0}\lambda - \frac{{{I_2}}}{{{R^2}}}{m^2}\lambda } \right]$

(13)

当α⁴＞4β²＞0且λ＞0时，圆柱壳屈曲^[18-20]，其动力屈曲解为

$Y\left( x \right) = {C_1}\sin \left( {{k_1}x} \right) + {C_2}\cos \left( {{k_1}x} \right) + {C_3}\sin \left( {{k_2}x} \right) + {C_4}\cos \left( {{k_2}x} \right)$

(14)

式中：C₁~C₄为系数， ${k_1} = \sqrt {\frac{{{\alpha ^2} - \sqrt {{\alpha ^4} - 4{\beta ^2}} }}{2}} , {k_2} = \sqrt {\frac{{{\alpha ^2} + \sqrt {{\alpha ^4} - 4{\beta ^2}} }}{2}}$ 。

(14)式满足下列两种边界条件：

(1) 对于一端夹支另一端固支的圆柱壳，其边界条件为

$\left\{ \begin{array}{l} Y\left( 0 \right) = Y'\left( 0 \right) = 0\\ Y\left( l \right) = Y'\left( l \right) = 0 \end{array} \right.$

(15)

(2) 对于一端简支另一端固支的圆柱壳，其边界条件为

$\left\{ \begin{array}{l} Y\left( 0 \right) = Y''\left( 0 \right) = 0\\ Y\left( l \right) = Y'\left( l \right) = 0 \end{array} \right.$

(16)

将(14)式代入(15)式中，整理得到如下齐次线性方程组

$\left( {\begin{array}{*{20}{c}} 0&1&0&1\\ {{k_1}}&0&{{k_2}}&0\\ {\sin \left( {{k_1}l} \right)}&{\cos \left( {{k_1}l} \right)}&{\sin \left( {{k_2}l} \right)}&{\cos \left( {{k_2}l} \right)}\\ {{k_1}\cos \left( {{k_1}l} \right)}&{ - {k_1}\sin \left( {{k_1}l} \right)}&{{k_2}\cos \left( {{k_2}l} \right)}&{ - {k_2}\sin \left( {{k_2}l} \right)} \end{array}} \right)\left( {\begin{array}{*{20}{c}} {{C_1}}\\ {{C_2}}\\ {{C_3}}\\ {{C_4}} \end{array}} \right) = 0$

(17)

若要(17)式有非平凡解，其系数行列式必为零，于是

$2{k_1}{k_2} - 2{k_1}{k_2}\cos \left( {{k_1}l} \right)\cos \left( {{k_2}l} \right) - \left( {k_1^2 + k_2^2} \right)\sin \left( {{k_1}l} \right)\sin \left( {{k_2}l} \right) = 0$

(18)

由k₁和k₂可得

$\left\{ \begin{array}{l} k_1^2 + k_2^2 = \left( {n_1^2 + n_2^2} \right){{\rm{ \mathsf{ π} }}^2}/{l^2} = {\alpha ^2}\\ k_1^2k_2^2 = n_1^2n_2^2{{\rm{ \mathsf{ π} }}^4}/{l^4} = {\beta ^2} \end{array} \right.$

(19)

将(19)式代入(12)式和(13)式中，得一端夹支另一端固支时FGM圆柱壳动力屈曲临界荷载N_cr，即

${N_{{\rm{cr}}}} = \frac{{{D_{11}}{{\rm{ \mathsf{ π} }}^2}\left( {n_1^2 + n_2^2} \right)}}{{{l^2}}} - \frac{{{B_{12}}}}{{{R^2}}} + \frac{{2\left( {{D_{12}} + 2{D_{66}}} \right){m^2}}}{{{R^2}}} + \frac{{{h^2}\left( {{D_{11}}n_1^2n_2^2{{\rm{ \mathsf{ π} }}^4}{R^4} - {A_{22}}{l^4}{R^2} + 4{B_{22}}{m^2}{R^2}{l^4} - {D_{22}}{l^4}{m^4}} \right)}}{{{l^4}{R^2}\left( {{h^2}{m^2} - 12{R^2}} \right)}}$

(20)

式中：n₁=n=1, 2, 3, …；m=1, 2, 3, …；n₂=n+2。

同理可得一端简支另一端固支时FGM圆柱壳动力屈曲临界荷载

(21)

此时，n₁=n=1, 2, 3, …；m=1, 2, 3, …；n₂=n+1。

将FGM退化成金属材料，得到金属材料圆柱壳动力屈曲临界荷载

${N_{{\rm{cr}}}} = \frac{{{D_{11}}{{\rm{ \mathsf{ π} }}^2}\left( {n_1^2 + n_2^2} \right)}}{{{l^2}}} + \frac{{2{D_{12}}{m^2}}}{{{R^2}}} + \frac{{{h^2}\left( {{D_{11}}n_1^2n_2^2{{\rm{ \mathsf{ π} }}^4}{R^4} - {A_{22}}{l^4}{R^2} - {D_{22}}{l^4}{m^4}} \right)}}{{{l^4}{R^2}\left( {{h^2}{m^2} - 12{R^2}} \right)}}$

(22)

(22)式与文献[16]中的表达式相同。

根据(10)式，取一端夹支另一端固支时圆柱壳动力屈曲解的表达式^[16]

$w = T\left( t \right)\left[ {\sin \left( {\frac{{{n_1}{\rm{ \mathsf{ π} }}x}}{l}} \right) - \frac{{{n_1}}}{{{n_2}}}\sin \left( {\frac{{{n_2}{\rm{ \mathsf{ π} }}x}}{l}} \right)} \right]\sin \left( {m\theta } \right)$

(23)

将(23)式代入控制方程(9)式中，计算并化简整理得到临界荷载表达式

${N_{{\rm{cr}}}} = \frac{{\left( {n_1^2 + n_2^2} \right){D_{11}}{{\rm{ \mathsf{ π} }}^2}}}{{{l^2}}} + \frac{{2{m^2}\left( {{D_{12}} + 2{D_{66}}} \right)}}{{{R^2}}}$

(24)

(24)式与不考虑转动惯量时用分离变量得到的结果相同，此时n₁=n=1, 2, 3, …; m=1, 2, 3, …; n₂=n+2。同理可得当边界条件为一端简支另一端固支时的临界荷载表达式，与(24)式相同，此时n₁=n=1, 2, 3, …; m=1, 2, 3, …; n₂=n+1。

3. 算例分析

采用MATLAB软件编程，对FGM圆柱壳动力屈曲临界荷载进行计算。讨论由不同材料(陶瓷-钛、陶瓷-铁、陶瓷-铜)组成的FGM圆柱壳(见图 2)的径厚比(R/h)、梯度指数(k)、环向模态数(m)、轴向模态数(n)对临界荷载N_cr的影响。基本材料参数如表 1所示。

图 2 材料沿壁厚分布

Figure 2. Distribution of material along wall thickness

下载: 全尺寸图片幻灯片

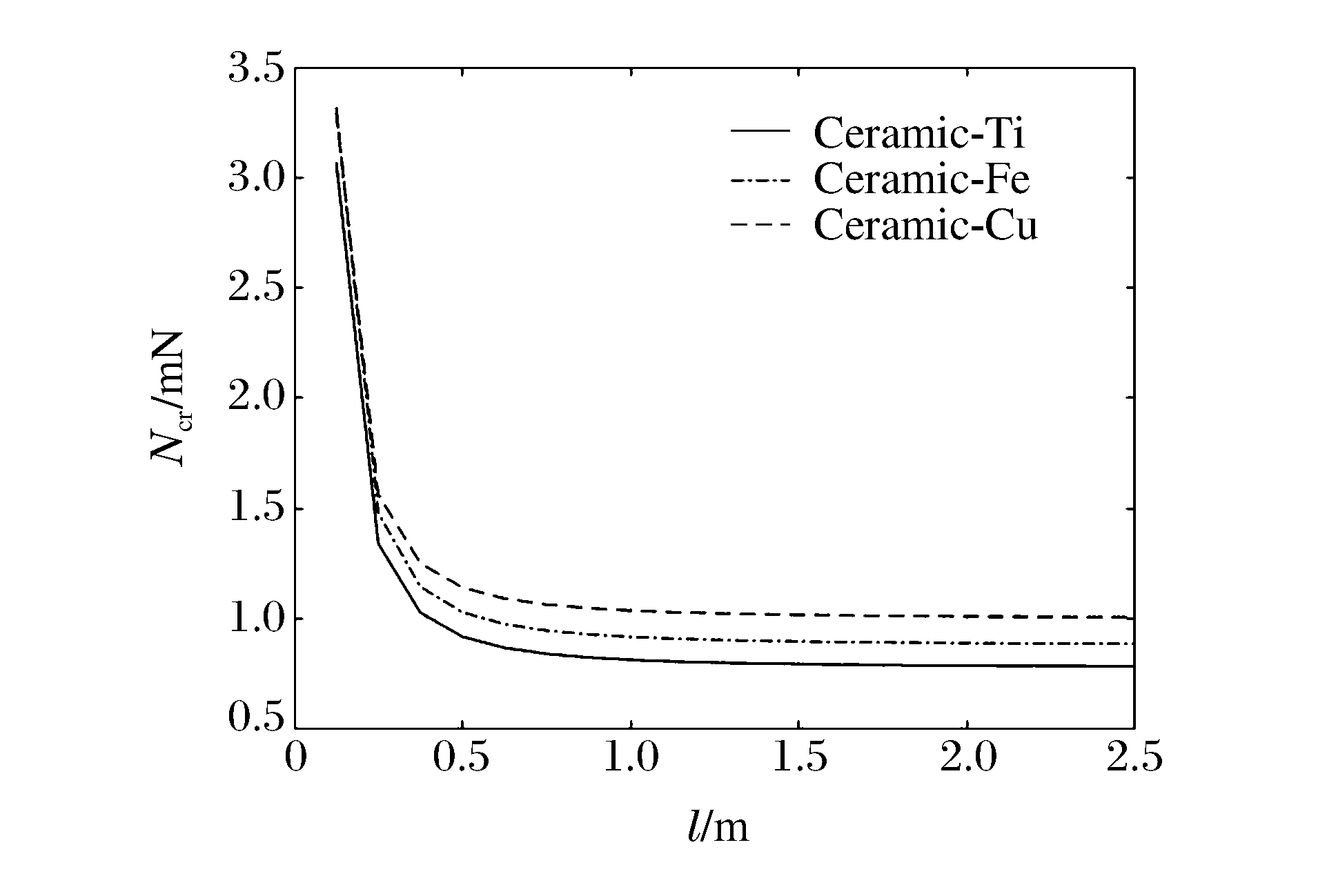
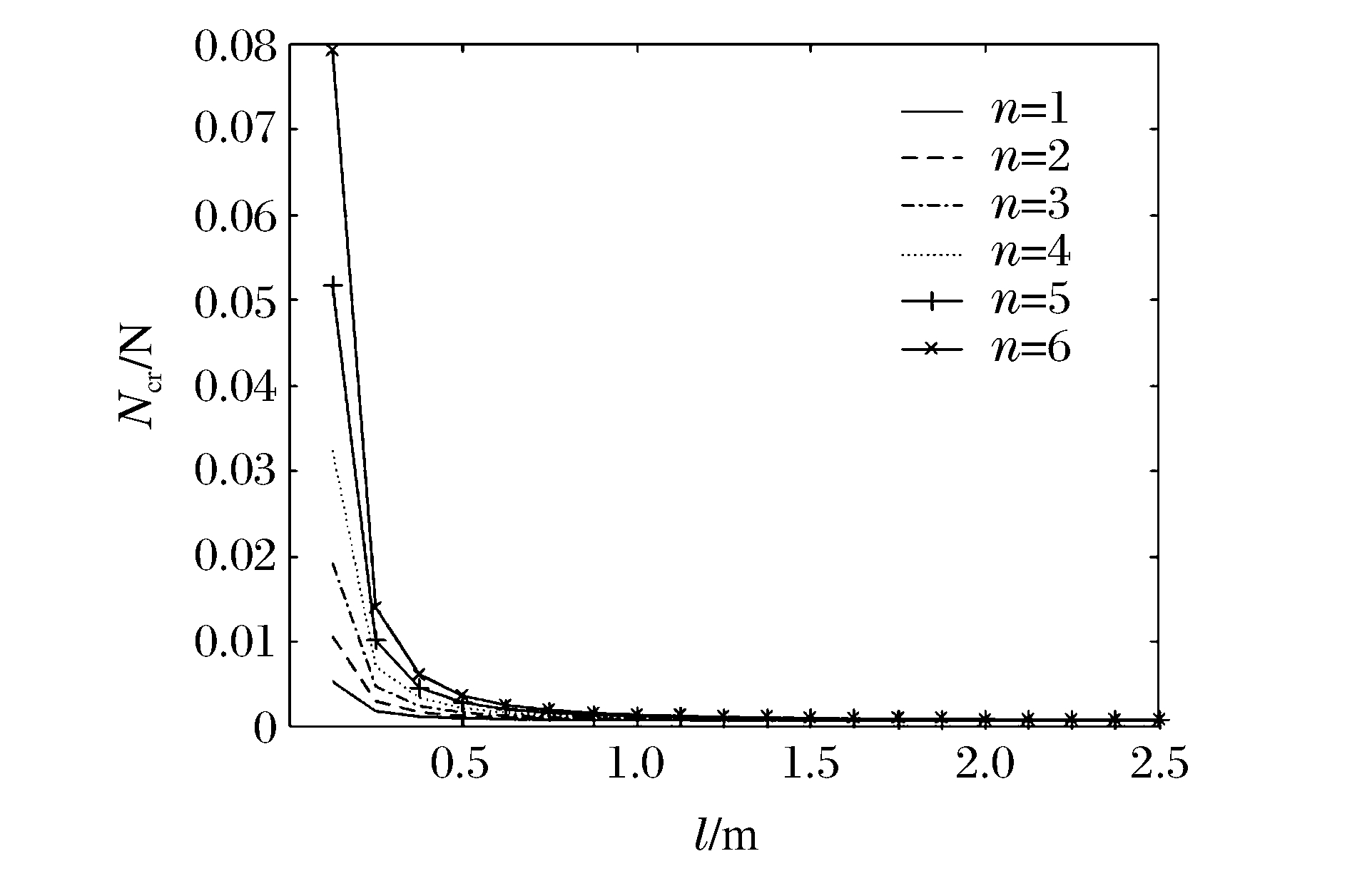
表 1 材料参数

Table 1. Material parameters

Material	E/GPa	ρ/(g·cm^-3)	μ
Ceramic	385	3.96	0.230
Ti	109	4.54	0.410
Fe	155	7.86	0.291
Cu	119	8.96	0.326

下载: 导出CSV

| 显示表格

图 3表示n=1、m=2、k=1、R/h=20时，不同材料组成下N_cr与临界长度l(本研究中临界长度即为圆柱壳长度)的关系曲线。从图 3可以看出：N_cr随l的增加而减小；当l＜0.5 m时，N_cr随l的增加而迅速减小；当l＞0.5 m时，N_cr随l的增大缓慢减小，且逐渐趋于常数；同一l下，陶瓷-铜的N_cr最大，陶瓷-铁次之，陶瓷-钛的N_cr最小。以下均以陶瓷-钛为例进行讨论。

图 3 不同材料组成下临界荷载与临界长度的关系

Figure 3. Critical load vs.critical length under different material composition conditions

下载: 全尺寸图片幻灯片

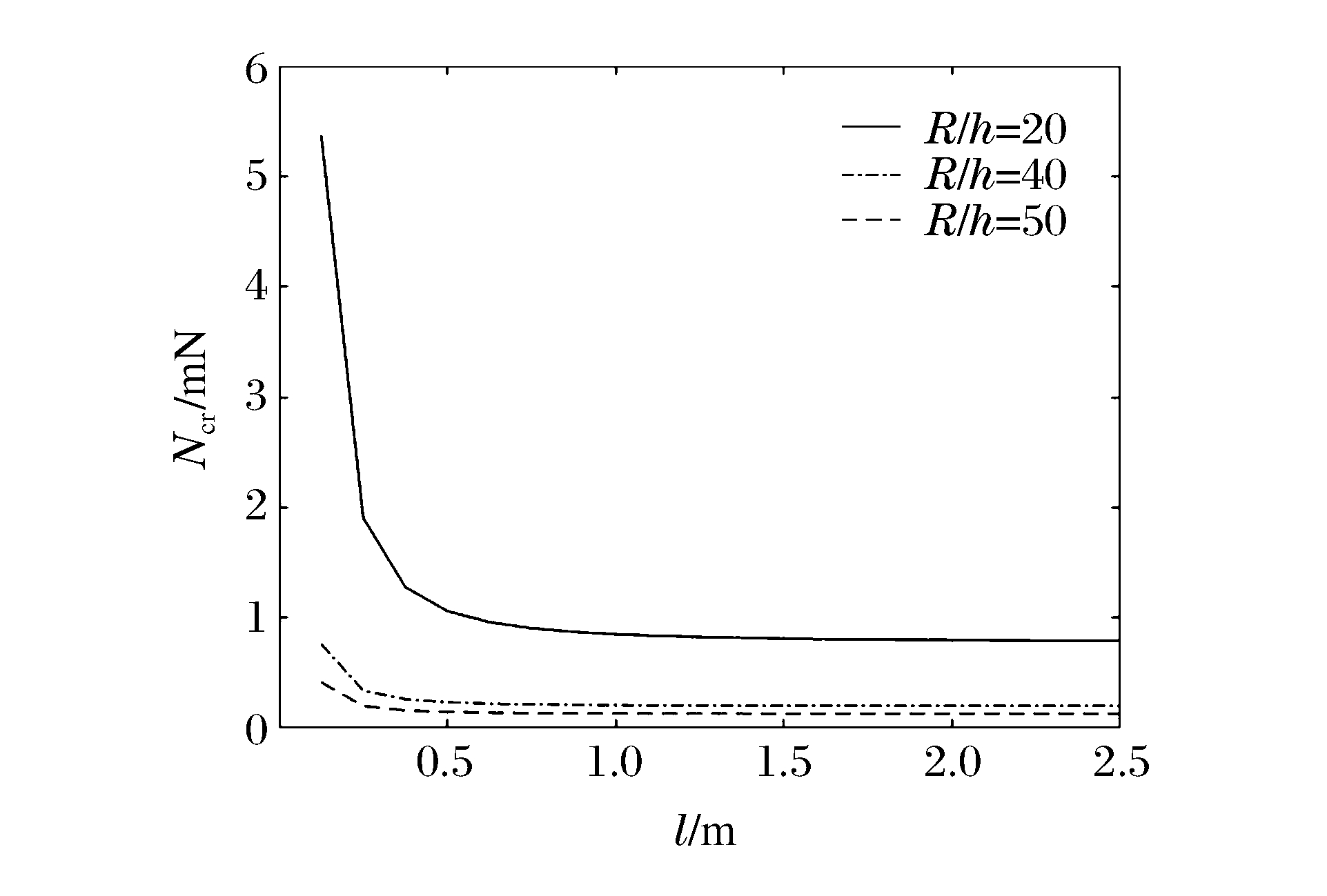
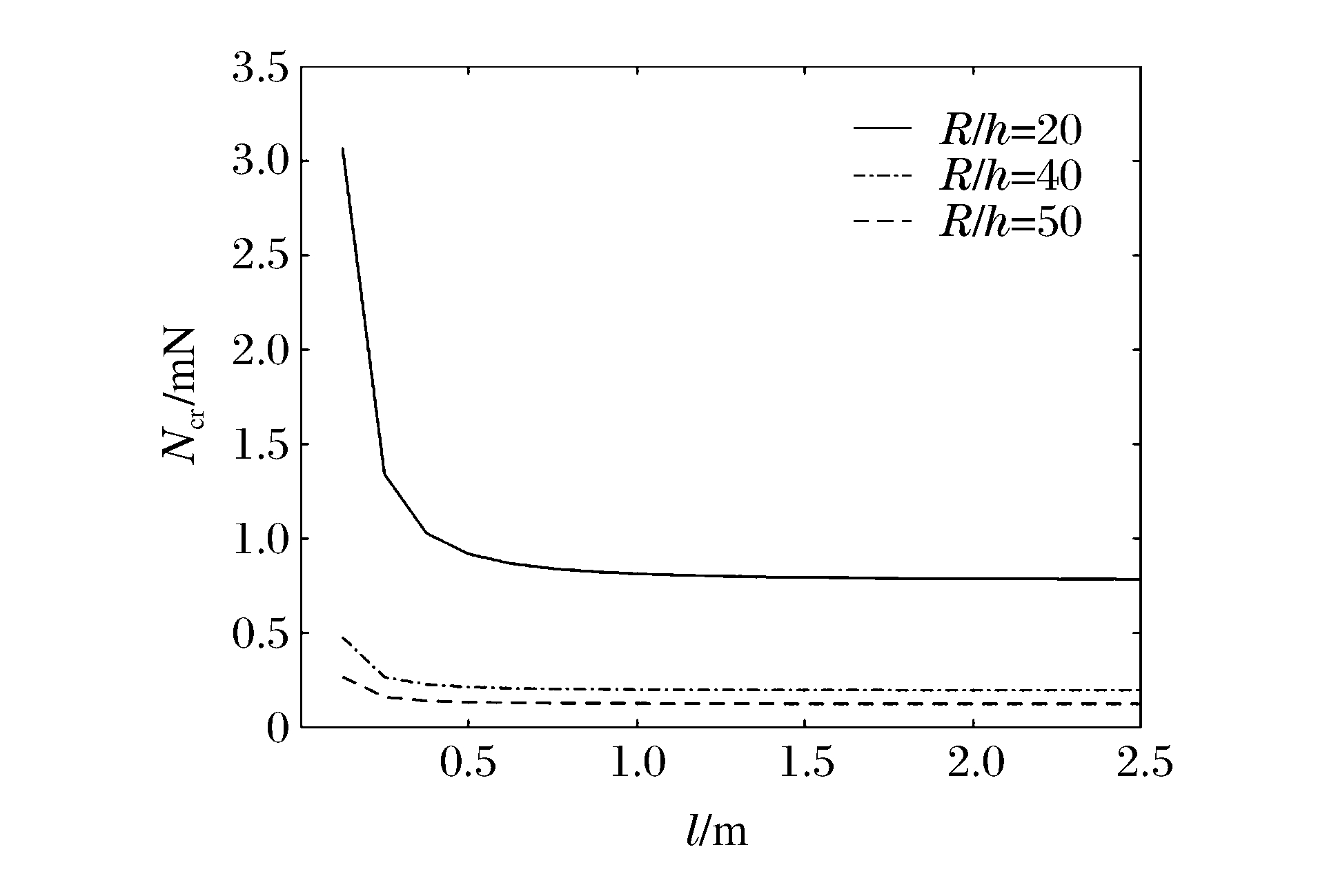
图 4和图 5分别表示冲击端为夹支和简支时n=1、m=2、k=1时不同R/h下N_cr与l的关系曲线。可以看出：当l增加时，N_cr减小，且逐渐趋于常数；在同一l下圆柱壳的N_cr随着R/h的增大而减小；当R/h和l一定时，冲击端为夹支时的N_cr明显比冲击端为简支时的N_cr大，说明约束条件对N_cr有较大影响。

图 4 冲击端为夹支时不同径厚比下临界荷载与临界长度的关系

Figure 4. Critical load vs.critical length under clamped edge and different diameter-thickness ratios conditions

下载: 全尺寸图片幻灯片

图 5 冲击端为简支时不同径厚比下临界荷载与临界长度的关系

Figure 5. Critical load vs.critical length under simple support and different diameter-thickness ratios conditions

下载: 全尺寸图片幻灯片

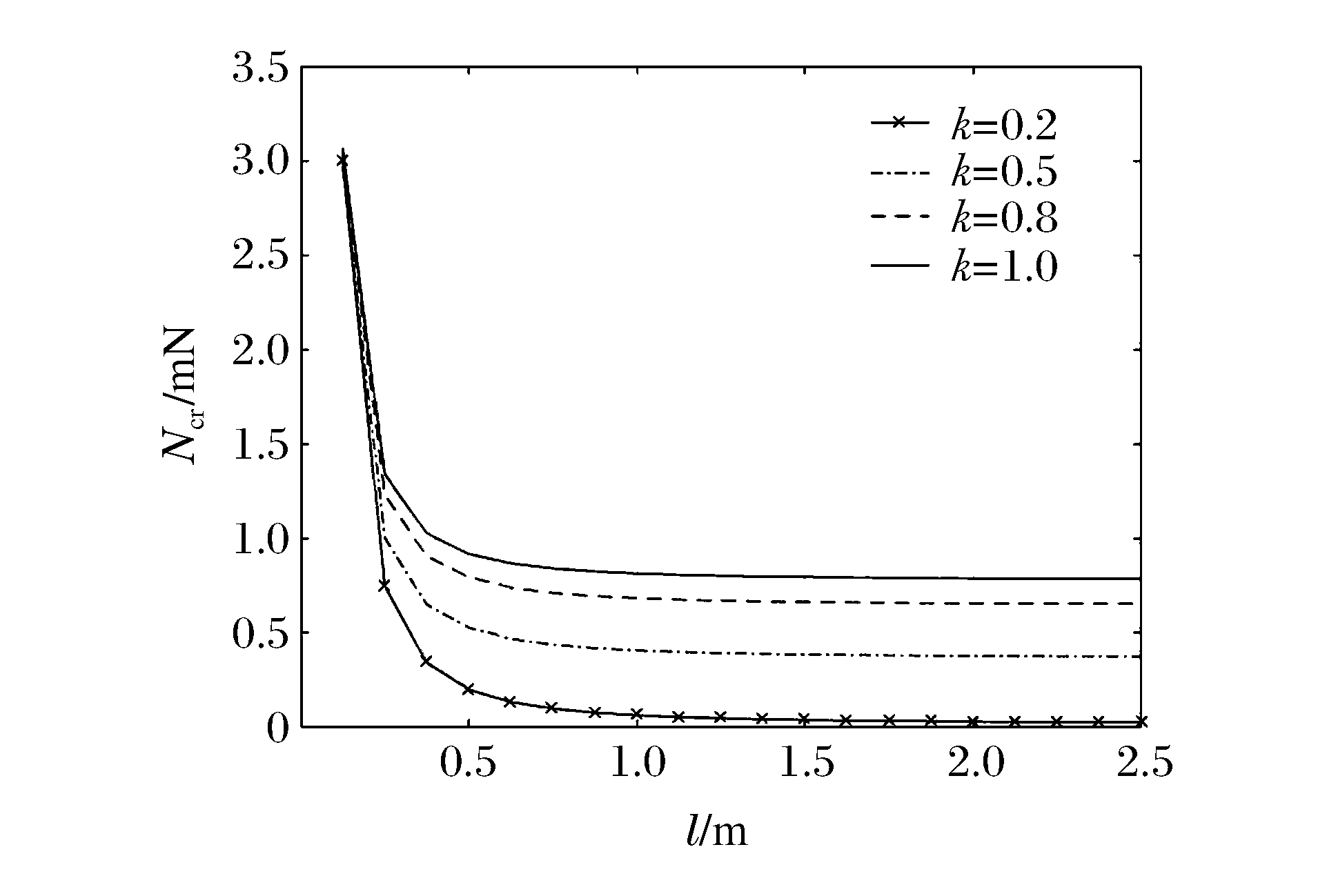
图 6和图 7分别表示冲击端为夹支和简支条件下n=1、m=2、R/h=20时不同k下N_cr与l的关系曲线。可见：FGM圆柱壳的N_cr随着l的增加而减小；在同一l下，FGM圆柱壳的N_cr随着k的增加而增加；当l＜0.5 m时，N_cr随l的增加迅速减小，当l＞0.5 m时，N_cr随l的增加缓慢减小并逐渐趋于常数；当k=1且l一定时，冲击端为夹支时的N_cr明显比冲击端为简支时的N_cr大，再次说明约束条件对N_cr的影响较大。

图 6 冲击端为夹支时不同梯度指数下临界荷载与临界长度的关系

Figure 6. Critical load vs.critical length under clamped edge and different gradient indexes conditions

下载: 全尺寸图片幻灯片

图 7 冲击端为简支时不同梯度指数下临界荷载与临界长度的关系

Figure 7. Critical load vs.critical length under simple support and different gradient indexes conditions

下载: 全尺寸图片幻灯片

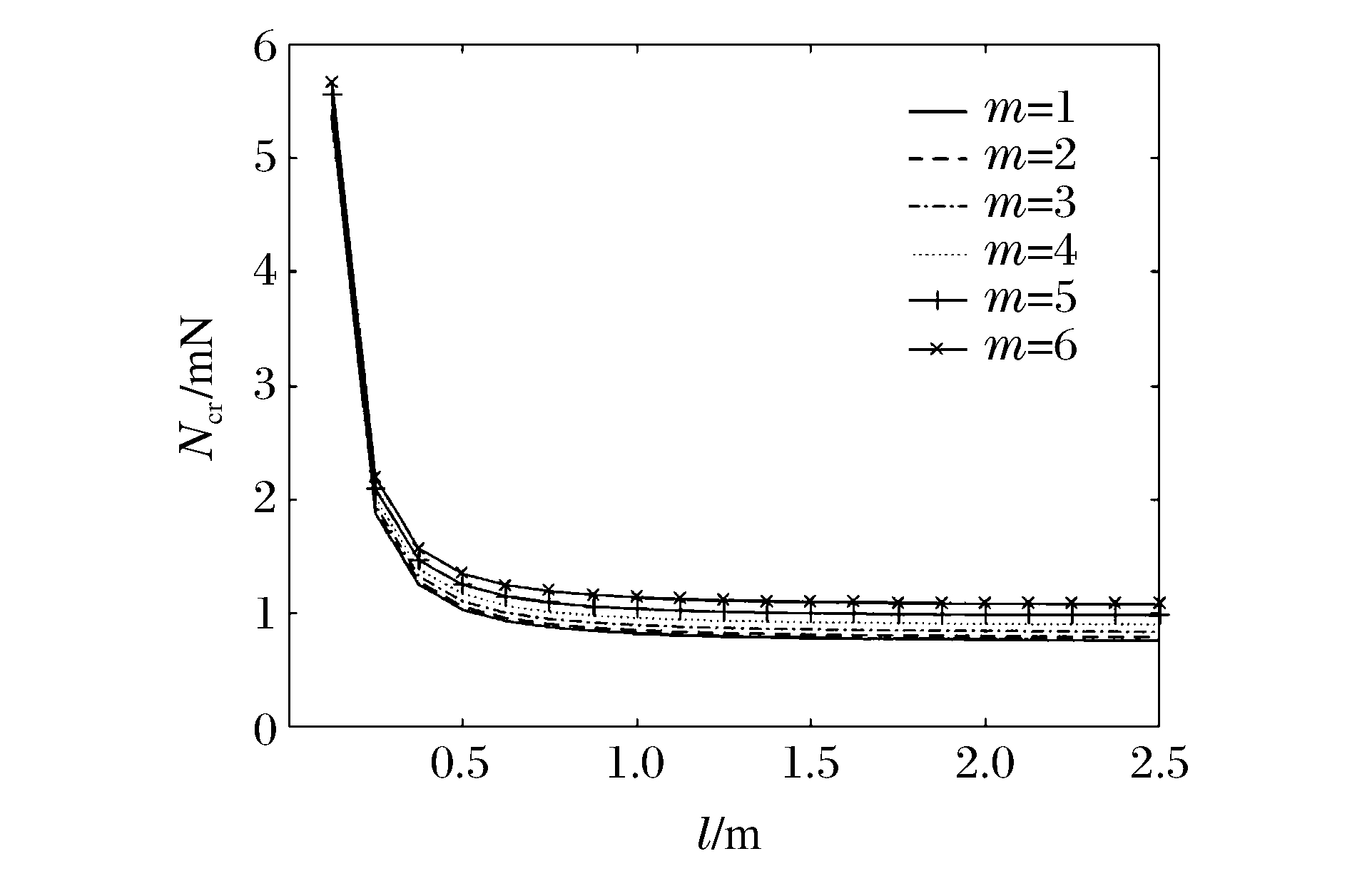
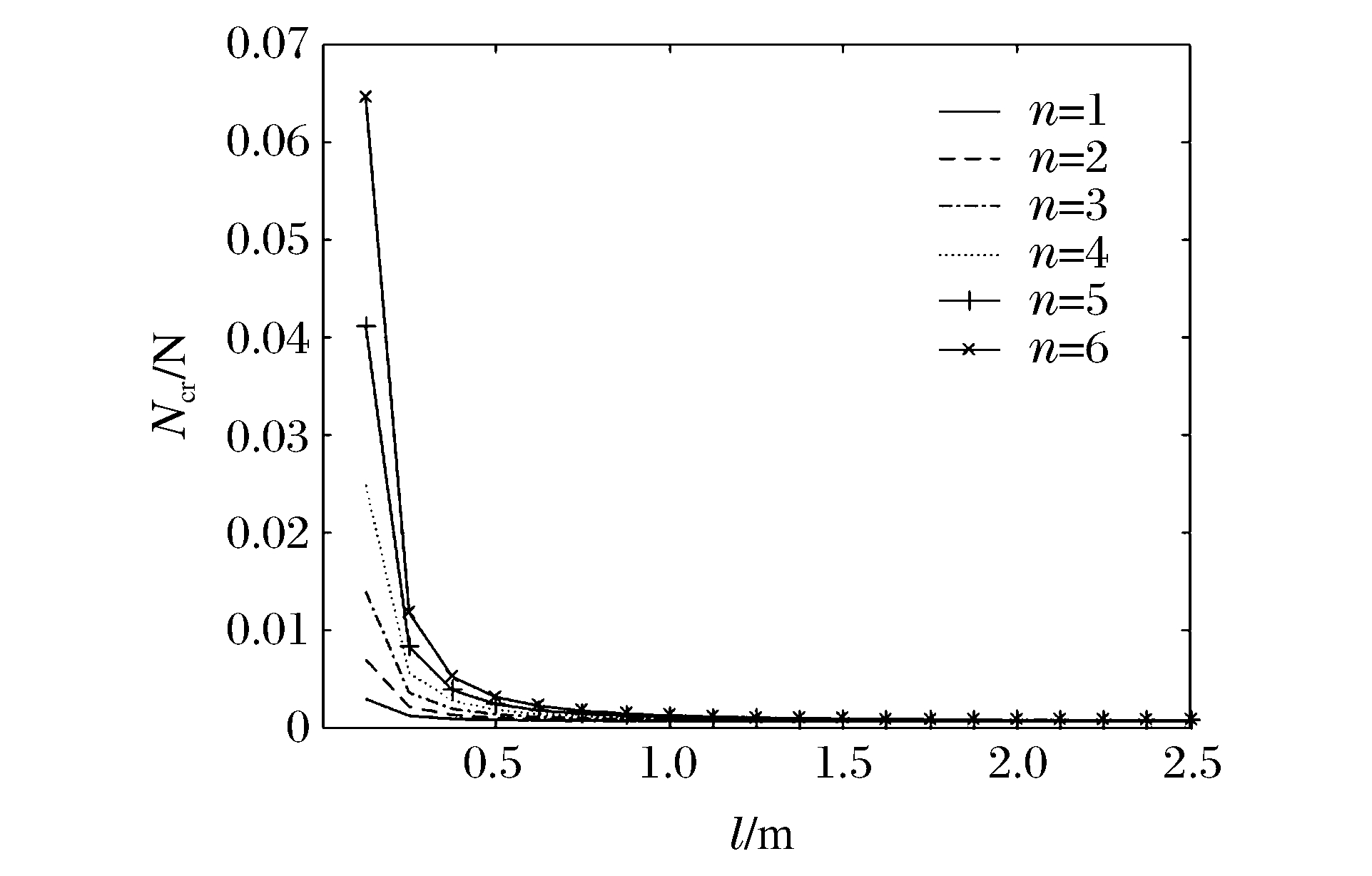
图 8和图 9分别表示冲击端为夹支和简支，n=1、k=1、R/h=20时不同m下N_cr与l的关系曲线。可以看出：当l在一定范围内时，N_cr随l的增加而迅速减小，超出这一范围后N_cr随l的增加而缓慢减小且逐渐趋于常数；同一l下，随着m的增大，FGM圆柱壳的N_cr增大，表明N_cr越大，高阶模态越易被激发。当m=6且l一定时，冲击端为夹支时的N_cr明显比冲击端为简支时的N_cr大，表明约束条件对N_cr有较大影响。

图 8 冲击端为夹支时不同环向模态数下临界荷载与临界长度的关系

Figure 8. Critical load vs.critical length under clamped edge and different circumferential modal number conditions

下载: 全尺寸图片幻灯片

图 9 冲击端为简支时不同环向模态数下临界荷载与临界长度的关系

Figure 9. Critical load vs.critical length under simple support and different circumferential modal number conditions

下载: 全尺寸图片幻灯片

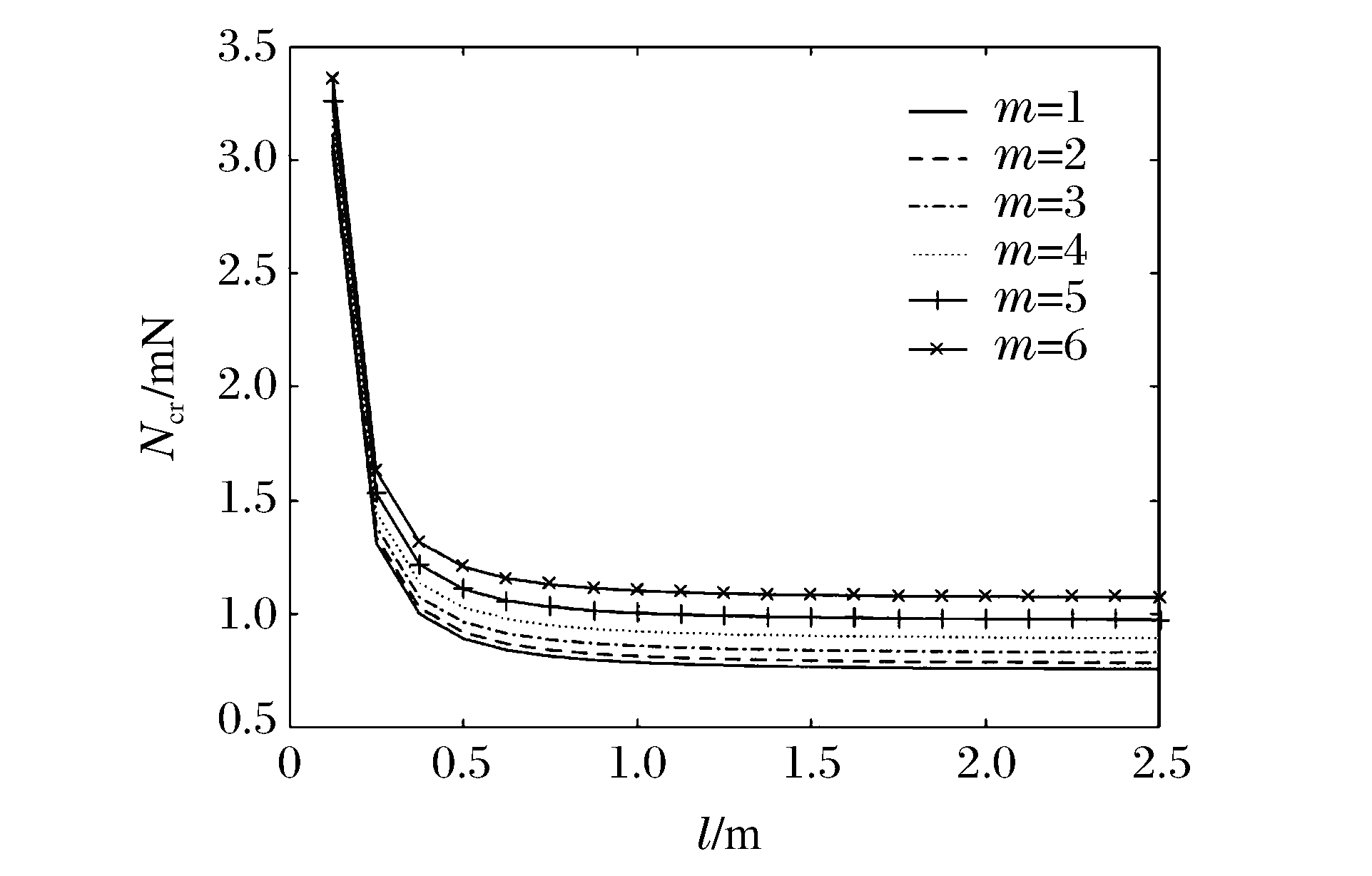
图 10和图 11分别表示冲击端为夹支和简支，m=1、k=1、R/h=20时不同n下N_cr与l的关系曲线。图 10和图 11显示：N_cr随着l的增加而减小；不同n条件下，N_cr随l的增加逐渐趋于同一值；当l＜1 m时，在同一l下N_cr随n的增加而增加，说明N_cr越大，高阶模态越容易被激发。

图 10 冲击端为夹支时不同轴向模态数下临界荷载与临界长度的关系

Figure 10. Critical load vs.critical length under clamped edge and different axial modal number conditions

下载: 全尺寸图片幻灯片

图 11 冲击端为简支时不同轴向模态数下临界荷载与临界长度的关系

Figure 11. Critical load vs.critical length under simple support and different axial modal number conditions

下载: 全尺寸图片幻灯片

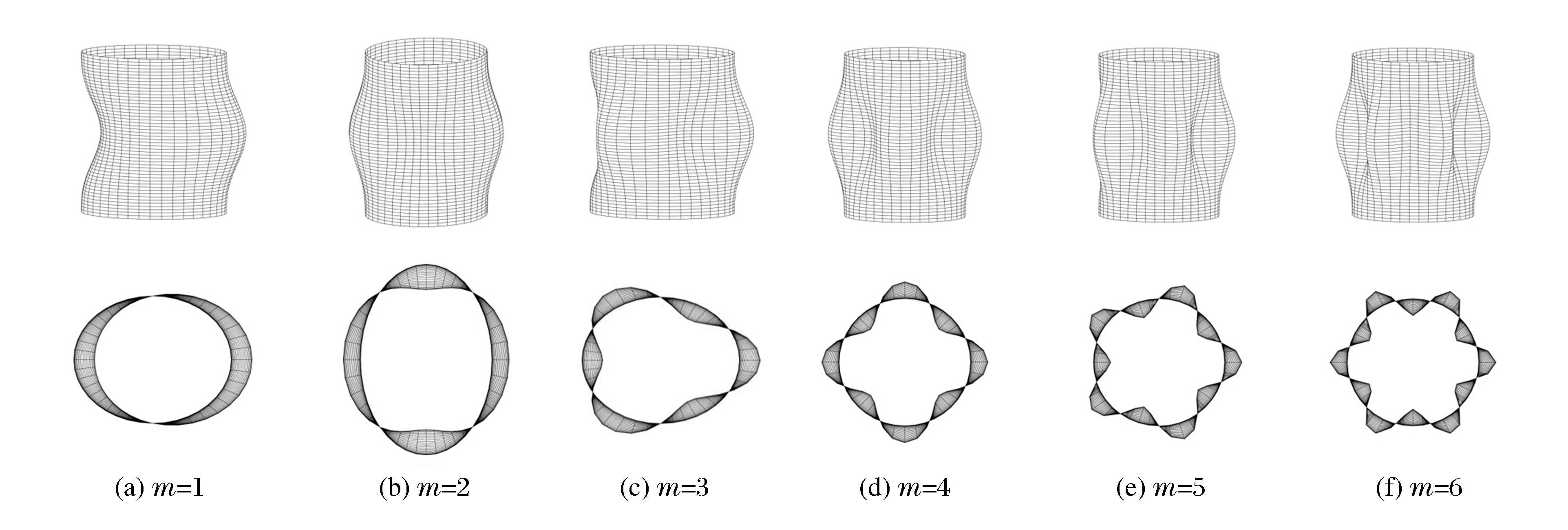
图 12为不同环向模态数m下FGM圆柱壳的动力屈曲模态。可以看出：随着m的增大，圆柱壳的模态变得越来越复杂，俯视图由单一形变为多瓣形；当m=6时，俯视图为12瓣形。

图 12 不同环向屈曲模态图(n=1;m=1, 2, 3, 4, 5, 6)

Figure 12. Different circumferential buckling modes (n=1;m=1, 2, 3, 4, 5, 6)

下载: 全尺寸图片幻灯片

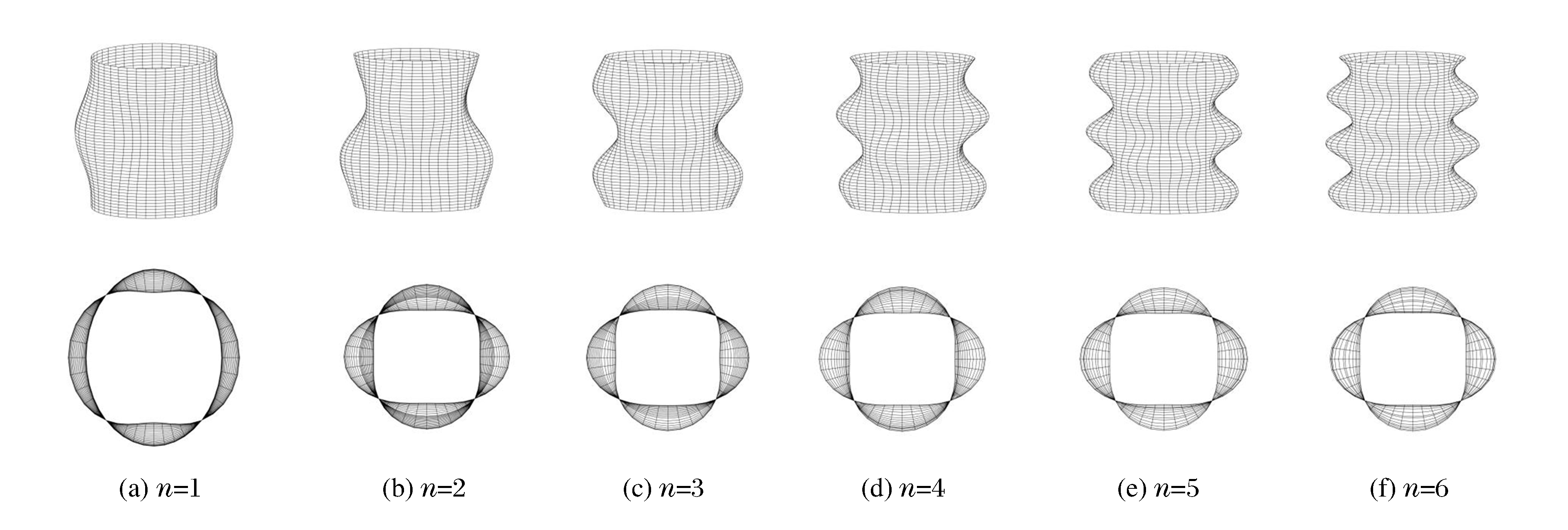
图 13为不同轴向模态数n下FGM圆柱壳的动力屈曲模态图。由图 13可知：随着n的增加，模态图变得越来越复杂。由FGM圆柱壳的俯视图可知，各阶模态数下动力屈曲模态图为轴对称。

图 13 不同轴向屈曲模态图(m=2;n=1, 2, 3, 4, 5, 6)

Figure 13. Different axial buckling modes (m=2;n=1, 2, 3, 4, 5, 6)

下载: 全尺寸图片幻灯片

4. 结论

(1) 根据Donnell壳体理论和经典板壳理论，由Hamilton变分原理得到轴向荷载作用下FGM圆柱壳的动力屈曲控制方程。

(2) 由圆柱壳周向连续性设出径向位移的周向形式，并用分离变量法得到不同约束条件下FGM圆柱壳动力屈曲临界荷载的表达式和屈曲解式。

(3) 利用MATLAB对临界荷载进行计算，得到：在轴向模态数(n)、环向模态数(m)、梯度指数(k)、径厚比(R/h)一定的情况下，同种材料组成的圆柱壳的临界荷载随着临界长度的增加而减小；在n、m、k、l一定的情况下，临界荷载随着径厚比的增大而减小；在n、m、R/h、l一定的情况下，临界荷载随着梯度指数k的增加而增加；不同约束条件下，冲击端为夹支的临界荷载大于冲击端为简支的临界荷载，表明约束条件对临界荷载有较大影响；圆柱壳的临界荷载随模态数的增加而增大，表明临界荷载越大，越容易激发高阶模态；圆柱壳的动力屈曲模态随模态数的增加变得更为复杂。

图 1 准静态试验设置

Figure 1. Quasi-static test setup

下载: 全尺寸图片幻灯片

图 2 动态压缩试验装置

Figure 2. Setup of dynamic compression tests

下载: 全尺寸图片幻灯片

图 3 三轴围压试验装置

Figure 3. Triaxial confining pressure test device

下载: 全尺寸图片幻灯片

图 4 无机玻璃的拉伸应力-应变曲线

Figure 4. Tensile stress-strain curves of inorganic glass

下载: 全尺寸图片幻灯片

图 5 不同应变率下典型应力-应变曲线

Figure 5. Typical stress-strain curves at different strain rates

下载: 全尺寸图片幻灯片

图 6 三轴围压试验轴向应力-应变曲线

Figure 6. Axial stress-strain curves of triaxial confining pressure test

下载: 全尺寸图片幻灯片

图 7 无机玻璃的Hugoniot曲线

Figure 7. Hugoniot curve of inorganic glass

下载: 全尺寸图片幻灯片

图 8 应变率敏感系数C的拟合过程

Figure 8. Fitting process of strain rate sensitivity coefficient C

下载: 全尺寸图片幻灯片

图 9 材料完整强度模型的拟合曲线

Figure 9. Fitting curve of complete strength model of material

下载: 全尺寸图片幻灯片

图 10 无机玻璃颗粒的无量纲静水压力与无量纲强度的关系

Figure 10. Relation between dimensionless hydrostatic pressure and dimensionless strength for inorganic glass particles

下载: 全尺寸图片幻灯片

图 11 玻璃试样和SHPB的有限元模型

Figure 11. Glass specimen and finite element model of SHPB

下载: 全尺寸图片幻灯片

图 12 模拟得到的无机玻璃的失效历程

Figure 12. Failure process of inorganic glass obtained by simulation

下载: 全尺寸图片幻灯片

图 13 试验与数值模拟结果对比

Figure 13. Comparison of test and simulation results

下载: 全尺寸图片幻灯片

表 1 玻璃的基本力学性能参数

Table 1. Basic mechanical parameters of glass

$\overline{\rho}$ /(kg∙m⁻³)	E_p/GPa	ν	G/GPa	K₁/GPa
2468	72	0.23	29.3	44.4

下载: 导出CSV

表 2 准静态和动态压缩试验得到的参考参数

Table 2. Parameters obtained by quasi-static and dynamic compression test

Test	${ \dot{{ \varepsilon }}}\text{/}{\text{s}}^{{-1}}$	σ/GPa	p/GPa	$\sigma^{*}$	$p^{*}$
Quasi-static compression	10⁻⁴	0.760	0.253	0.127	0.0719
SHPB	420	0.930	0.310	0.156	0.0881

下载: 导出CSV

表 3 根据动态压缩试验数据拟合得到的完整强度参数

Table 3. Complete strength parameters obtained by dynamic compression test data fitting

No.	${ \dot{{ \varepsilon }}}^{*}$	σ/GPa	${\sigma}^{{*}}$	$p^{{*}}$	X	Y
1	230	0.864	0.1447	0.0818	−2.0393	−1.9576
2	280	0.893	0.1496	0.0846	−2.0184	−1.9255
3	350	0.917	0.1536	0.0868	−2.0015	−1.9000
4	420	0.933	0.1563	0.0884	−1.9903	−1.8835

下载: 导出CSV

表 4 三轴围压试验数据

Table 4. Triaxial confining pressure test data

Particle size/mm	${\sigma _{\textit{zz}}}$ /GPa	${{\varepsilon} _{\text{c}}}/10^{-3}$	${E_{\text{c}}}$ /GPa	${\sigma _{{\theta \theta }}}$ /GPa	p/GPa	${\sigma _{\rm f}}$ /GPa	${p ^*}$	$\sigma_{\mathrm{f}}^*$
0–0.1	0.533	7.00	192	0.089	0.237	0.222	0.067	0.037
0.3–0.5	0.408	4.75	192	0.058	0.175	0.175	0.050	0.029

下载: 导出CSV

表 5 钢杆和垫块的线弹性材料参数

Table 5. Linear elastic material property parameters of steel bar and block

ρ_s/(kg∙m⁻³)	E_p/GPa	ν
7850	200	0.30

下载: 导出CSV

表 6 试验拟合得到的玻璃材料的JH2本构模型参数

Table 6. Parameters of JH2 constitutive model of glass material fitted by the test

ρ/(kg∙m⁻³)	G/GPa	K₁/GPa	K₂/GPa	K₃/GPa	T/GPa	σ_HEL/GPa	p_HEL/GPa
2468	29.3	44.4	−145	244	0.07	7.5	3.52

A	B	C	M	N	D₁	D₂	S_fmax
3.10	0.36	0.00256	0.83	1.51	0.005	0.85	0.2

下载: 导出CSV

表 7 数值模拟和试验获得的不同应变率下的单轴压缩强度

Table 7. Uniaxial compression strength at different strain rates obtained by simulation and test

${ \dot{{ \varepsilon }}}\text{/}{\text{s}}^{{-1}}$	Uniaxial compression strength/MPa		Error/%
${ \dot{{ \varepsilon }}}\text{/}{\text{s}}^{{-1}}$	Test	Simulation	Error/%
220	874	837	4.2
350	917	885	3.5
425	939	911	3.0

下载: 导出CSV

参考文献(21)

[1]	刘志海. 夹层玻璃的发展现状及趋势 [J]. 中国建材, 2003(9): 64–66. LIU Z H. Current situation and future trends of laminated glass [J]. China Building Materials, 2003(9): 64–66.
[2]	SHIM G I, KIM S H, EOM H W, et al. Improvement in ballistic impact resistance of a transparent bulletproof material laminated with strengthened soda-lime silicate glass [J]. Composites Part B: Engineering, 2015, 77: 169–178. doi: 10.1016/j.compositesb.2015.03.035
[3]	SHIM G I, EOM H W, KIM S H, et al. Fabrication of lightweight and thin bulletproof windows using borosilicate glass strengthened by ion exchange [J]. Composites Part B: Engineering, 2015, 69: 44–49. doi: 10.1016/j.compositesb.2014.09.023
[4]	ZHANG X H, HAO H, MA G W. Dynamic material model of annealed soda-lime glass [J]. International Journal of Impact Engineering, 2015, 77: 108−119.
[5]	葛彦鑫. Al₂O₃/SiC复相陶瓷冲击失效机理研究 [D]. 太原: 中北大学, 2022: 52−54. GE Y X. Study on impact failure mechanism of Al₂O₃/SiC composite [D]. Taiyuan: North University of China, 2022: 52−54.
[6]	JOHNSON G R, HOLMQUIST T J. An improved computational constitutive model for brittle materials [J]. AIP Conference Proceedings, 1994, 309(1): 981–984.
[7]	HOLMQUIST T J, JOHNSON G R, GRADY D E, et al. High strain rate properties and constitutive modeling of glass [C]//The 15th International Symposium on Ballistics. Jerusalem, Isreal, 1995: T14929.
[8]	ALEXANDER C S, CHHABILDAS L C, TEMPLETON D W. The hugoniot elastic limit of soda-lime glass [J]. AIP Conference Proceedings, 2007, 955(1): 733–738.
[9]	RENGANATHAN P, DUFFY T S, GUPTA Y M. Hugoniot states and optical response of soda lime glass shock compressed to 120 GPa [J]. Journal of Applied Physics, 2020, 127(20): 205901.
[10]	杨震琦, 庞宝君, 王立闻, 等. JH-2模型及其在Al₂O₃陶瓷低速撞击数值模拟中的应用 [J]. 爆炸与冲击, 2010, 30(5): 463–471. YANG Z Q, PANG B J, WANG L W, et al. JH-2 model and its application to numerical simulation on Al₂O₃ ceramic under low-velocity impact [J]. Explosion and Shock Waves, 2010, 30(5): 463–471.
[11]	高玉波. TiB₂-B₄C复合材料动态力学性能及抗侵彻机理研究 [D]. 哈尔滨: 哈尔滨工业大学, 2016: 29−42. GAO Y B. Study of dynamic mechanical property and anti-penetration mechanism of TiB₂-B₄C composites [D]. Harbin: Harbin Institute of Technology, 2016: 29−42.
[12]	MEYER L W, FABER I. Investigations on granular ceramics and ceramic powder [J]. Journal De Physique Ⅳ, 1997, 7: C3-565–C3-570.
[13]	ASTM. Standard test method for tensile strength estimate by disc compression of manufactured graphite: D8289-2020 [S]. 2020.
[14]	贾哲. 弹道冲击下防弹玻璃的损伤机理和抗侵彻性能研究 [D]. 太原: 中北大学, 2023: 12−36. JIA Z. Study on damage mechanism and anti-penetration performance of bulletproof glass under ballistic impact [D]. Taiyuan: North University of China, 2023: 12−36.
[15]	JOHNSON G R, COOK W H. A constitutive model and data for metals subjected to large strains, high strain rates, and high temperatures [C]//The 7th International Symposium on Ballistics. The Hague, The Netherlands, 1983.
[16]	JOHNSON G R, COOK W H. Fracture characteristics of three metals subjected to various strains, strain rates, temperatures and pressures [J]. Engineering Fracture Mechanics, 1985, 21(1): 31–48. doi: 10.1016/0013-7944(85)90052-9
[17]	HOLMQUIST T J, JOHNSON G R, GERLACH C A. An improved computational constitutive model for glass [J]. Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences, 2017, 375(2085): 20160182. doi: 10.1098/rsta.2016.0182
[18]	OSNES K, HOLMEN J K, GRUE T, et al. Perforation of laminated glass: an experimental and numerical study [J]. International Journal of Impact Engineering, 2021, 156: 103922. doi: 10.1016/j.ijimpeng.2021.103922
[19]	GRADY D E, CHHABILDAS L C. Shock-wave properties of soda-lime glass [R]. Albuquerque: Sandia National Laboratories, 1996: 4−6.
[20]	DANDEKAR D P. Index of refraction and mechanical behavior of soda lime glass under shock and release wave propagations [J]. Journal of Applied Physics, 1998, 84(12): 6614−6622.
[21]	SIMHA C H M, GUPTA Y M. Time-dependent inelastic deformation of shocked soda-lime glass [J]. Journal of Applied Physics, 2004, 96(4): 1880−1890.