Adiabatic Shear Failure Behavior of 30CrMnMo Steel under Pulse Stress Impact
-
摘要: 为研究30CrMnMo钢在脉冲应力冲击载荷下的绝热剪切失效及演化特性，利用分离式霍普金森压杆对一种轴对称帽型试件进行冲击剪切实验，并运用LS-DYNA动力学有限元软件对不同入射脉冲应力载荷下的剪切失效演化及剪切区温度分布进行数值模拟。结果表明，帽型试件的绝热剪切失效与脉冲应力比冲量相关，对于30CrMnMo钢帽型试件，其绝热剪切失效对应的脉冲应力比冲量近似为常量。数值模拟中，当网格尺寸小于剪切带宽度时，能够有效模拟剪切带内的局部温升热点特性。绝热剪切演化表现为失稳由帽型试件剪切区拐角处启动并同时向中心传播，剪切带内外材料主要经历均匀剪切变形和失稳快速扩展2个阶段。Abstract: In order to study the adiabatic shear failure behavior and evolution characteristics of 30CrMnMo steel under pulse stress impact, a split Hopkinson pressure bar was used to conduct an axisymmetric cap shaped specimen for impact shear experiments. The shear failure evolution and temperature distribution in the shear zone under different incident pulse stress loads were numerically simulated using LS-DYNA dynamic finite element software. The results indicate that the adiabatic shear failure of the cap shaped specimen is related to the specific impulse of the pulse stress. For cap shaped specimen of 30CrMnMo steel, the specific impulse of pulse stress corresponding to the adiabatic shear failure is approximately constant. In numerical simulation, when the grid size is smaller than the width of the shear band, the local temperature rise of hot spot within the shear band can be effectively simulated. The evolution of adiabatic shear instability is characterized by simultaneous propagation from the corner of the shear zone to the center, and the materials inside and outside the shear zone mainly undergo two stages: uniform shear deformation and rapid expansion of instability.
-
稀土六硼化物LaB6具有较低的逸出功和低蒸发率等优异的电子发射性能，同时还具有熔点高、化学稳定性强、硬度高等特点，因此自Lafferty发现LaB6的热发射性能以来一直是电子材料及器件领域的研究热点[1-3]。人们对LaB6晶体材料的制备和表征及其多元硼化物开展了较多的研究工作，例如：张宁等[4]通过悬浮区熔法制备了高质量LaB6单晶体；包黎红等[5]制备了La0.6Ce0.4B6材料，硬度达到2.31 GPa，1873 K下的最高发射电流密度达40.7 A/cm2；刘洪亮等[6]通过测试发现，LaB6晶体材料(100)晶面具有最佳的发射性能，最大发射电流密度在1773 K时可达40.4 A/cm2。然而，高致密度的LaB6晶体材料的烧结制备较为困难（致密度较低，80%～92%），所得材料的力学性能较差[7]。当前，关于LaB6晶体材料力学性能的理论研究报道较少，为此本研究基于密度泛函理论，结合Birch-Murnaghan物态方程，系统研究LaB6晶体材料的弹性性质及其他力学性能，以期为LaB6的深入研究和应用提供参考。
1. 计算方法与过程
计算中，将芯电子及核视为原子核，用Vanderbilt超软势近似其对外层电子的作用，外层电子设为La（5s25p65d16s2）、B（2s2 2p1），电子波函数采用平面波基矢组[8]。电子交换关联项采用广义梯度近似法（General Gradient Approximation，GGA）中的PBE（Perdew Burke Ernzerhof）泛函近似。首先对晶格结构进行充分弛豫，在此过程中固定晶体的对称性，允许原子在3个方向上弛豫。考虑到La d电子的在位库仑相互作用，计算时将其作用项设置为2.5 eV。计算弹性常数时，每个原子的能量收敛精度设置为2×10–6 eV，最大受力收敛精度设置为0.06 eV/nm；应力-应变计算中，原子最大位移的收敛精度设置为2×10–5 nm；电子结构计算中，电子平面波矢组基矢截止能量设为240 eV，布里渊区k点的自动生成采用Monkhorst-Pack法，k点网格密度为4×4×4。
根据胡克定律，固体材料在弹性形变范围内所受的应力与应变之间符合
S=Cε(1) (1) 式中：S为应力，
C 为弹性常数，ε 为应变。广义上晶体材料中的应力和应变都是二阶张量，因此弹性常数c 为六阶张量，其中独立分量数为36个，即[S1S2S3S4S5S6]=[C11C12C13C14C15C16C21C22C23C24C25C26C31C32C33C34C35C36C41C42C43C44C45C46C51C52C53C54C55C56C61C62C63C64C65C66][ε1ε2ε3ε4ε5ε6](2) (2) 由于晶体具有结构对称性（见图1），因此弹性常数张量矩阵可化为一个具有21个独立分量的矩阵[8]，考虑到LaB6为立方晶系，空间群为
Pmˉ3m ，因此其弹性常数张量矩阵具有3个独立分量，即C11 、C44 、C12 。采用密度泛函理论和B-M物态方程研究LaB6的弹性常数，基于Voigt方法、Reuss方法和Hill方法[9]对体弹性模量和剪切弹性模量进行计算分析，采用压缩各向因子衡量弹性的各向异性，采用Tian等[10]的维氏硬度方法分析硬度，并采用泊松比和各向异性因子对其延脆性进行分析[10-11]。2. 结果与讨论
计算分析得到的LaB6晶体材料的晶格参数列于表1。从表1中可以看出，初始结构经过充分弛豫之后，计算得到的晶格参数与实验值之间的相对误差均小于3%，说明计算分析过程所用参数较为合理。
表 1 LaB6晶体材料的晶格参数Table 1. Structural parameters of LaB6 crystalline materialMethod a/nm b/nm c/nm α/(°) β/(°) γ/(°) Experiment 0.415 49 0.415 49 0.415 49 90 90 90 Calculation 0.420 205 0.420 205 0.420 205 90 90 90 通过计算得到LaB6晶体材料的弹性常数参数C11、C12和C44分别为436.92、22.37和47.64 GPa，
C11 较大，表明在此主轴应力方向上LaB6晶体具有较大的弹性常数和体弹性模量。根据立方晶体的力学稳定性判定公式[12]，经过计算分析得出LaB6晶体的弹性常数参数满足C11>0,C44>0,C11>|C12|,C11+2C12>0 (3) 表明本研究所用晶格结构为力学稳定的晶体结构。
分别采用Voigt法、Reuss法和Hill法，根据以下公式，计算LaB6晶体材料的体弹性模量和剪切弹性模量
BV=(C11+2C12)/3 (4) GV=(C11−C12+3C44)/5 (5) BR=(C11+2C12)/3 (6) GR=5(C11−C12)C44/[4C44+3(C11−C12)] (7) BH=(BR+BV)/2 (8) GH=(GR+GV)/2 (9) 式中：B为体弹性模量，G为剪切弹性模量，下标V、R、H分别表示Voigt法、Reuss法和Hill法。计算结果列于表2，可见LaB6晶体具有较大的体弹性模量。由于LaB6晶体在a、b、c方向上具有各向同性，因此采用Voigt方法和Reuss方法计算所得体弹性模量具有相同的数值。
表 2 LaB6晶体材料的体弹性模量和剪切弹性模量Table 2. Bulk modulus and shear modulus of LaB6 crystalline materialBV/GPa BR/GPa BH/GPa GV/GPa GR/GPa GH/GPa 160.55 160.55 160.55 111.49 68.85 90.17 杨氏模量E可用于衡量固体材料的刚度，其值越大，刚性越强。泊松比
γ 可用于衡量固体材料抵抗切应变的能力，数值一般在–1～0.5之间，值越大，延性越好，一般认为γ <1/3时材料呈脆性，γ >1/3时呈韧性。体弹性模量与剪切弹性模量的比值λ可用于衡量固体材料的脆性和延展性，其分界值一般是1.75，小于此值时材料明显呈脆性，大于此值时则呈延性。弹性各向异性因子A在0～1之间，A=0表明材料具有各向同性，A=1表明具有最大的各向异性。根据微观原子结合键强度理论计算出的硬度适合分析含有d态电子体系的抗剪性，且其值越高，表明抵抗变形的能力越强[11]。采用以下公式计算LaB6晶体材料的杨氏模量E、泊松比
γ 、体剪弹性模量比λ（λ=B/G）、体弹性模量各向异性因子AB、剪切弹性模量各向异性因子AG和硬度H。E=9BG/(3B+G) (10) γ=(3B−2G)/(6B+2G) (11) AB=(BV−BR)/(BV+BR) (12) AG=(GV−GR)/(GV+GR) (13) H=0.92λ−1.137G0.708 (14) λ=B/G (15) 表3列出了计算分析结果，可以看出：LaB6晶体材料的杨氏模量为227.85 GPa，远大于一些金属的杨氏模量，与钢材的杨氏模量接近，表明LaB6不易发生弹性形变，刚性较强；其泊松比为0.26，表明LaB6具有一定的脆性；体剪弹性模量上限值的比值λ为1.44，小于1.75，与泊松比计算分析结果吻合；体弹性模量各向异性因子AB=0，剪切弹性模量各向异性因子AG=0.24，表明LaB6的体弹性具有各向同性，而剪切弹性具有一定的各向异性；理论硬度H达到11.56 GPa，表明LaB6抵抗剪切形变的能力较强。
表 3 LaB6晶体材料的力学性能参数和弹性波速Table 3. Mechanical parameters and elastic velocities of LaB6 crystalline materialE/GPa γ λ AB AG H/GPa vl/(km·s–1) vt/(km·s–1) vm/(km·s–1) 227.85 0.26 1.44 0 0.24 11.56 7.72 4.38 4.87 采用以下公式[13-14]计算LaB6晶体材料的纵波弹性波速vl、剪切弹性波速vt和平均弹性波速vm
vl=√(B+43G)1ρ,vt=√Gρ,vm=[13(2v3t+1v3l)]−13 (16) 计算结果如表3所示。LaB6晶体材料的3支弹性波中，有1支纵波和2支横波。从表3可以看出，LaB6晶体的纵波弹性波速（7.72 km/s）较大，剪切弹性波速（4.38 km/s）相对较小，平均波速达到4.87 km/s。在LaB6晶体材料的长波极限，声学波的纵波支速度较大，横波支速度相对较小，表明原子相对运动的振动波速较大。
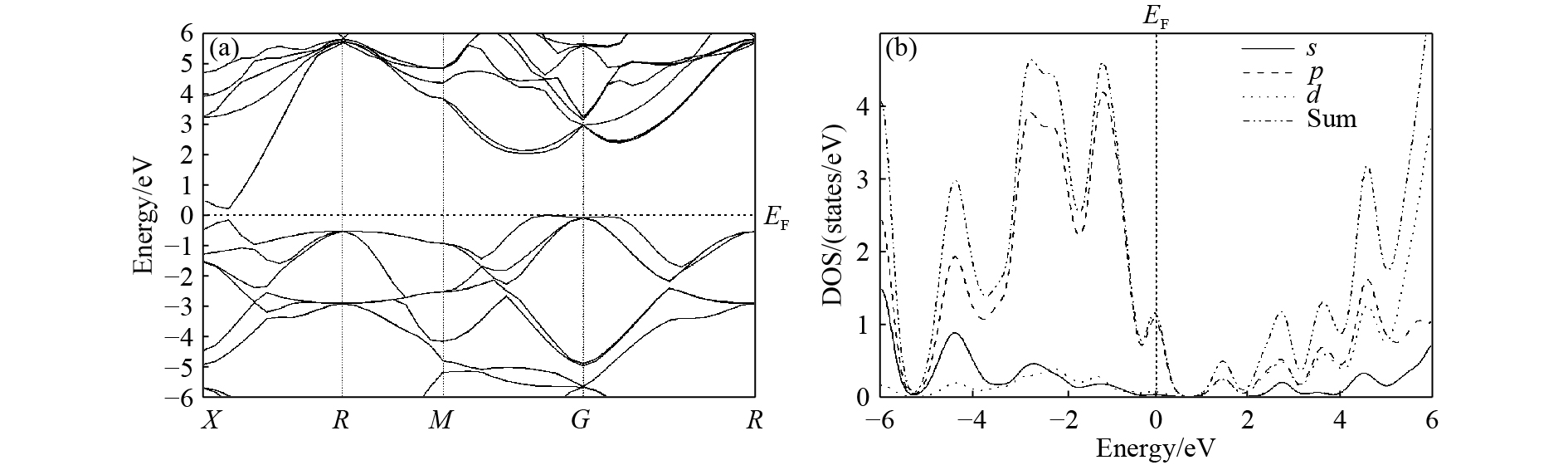
计算得到的LaB6晶体材料的能带结构和分态密度如图2所示。从图2中可以看出，LaB6具有较窄的带隙，带隙宽度为0.20 eV，费米能级穿过价带，表明LaB6呈金属性。从分态密度图可以看出，曲线具有多个峰值，表明LaB6内部电子具有较强的局域性，这也是稀土La化合物特有的性质。结合能带结构和分态密度可以看出，LaB6材料价带顶的能带由p、d和s电子形成，导带底的能带由p和d电子形成，其中p态电子对价带顶和导带底的形成起最重要的作用。计算分析得到的LaB6晶体材料各轨道的电荷转移分布情况如表4所示。可见，La的s轨道和p轨道的电子转移至d轨道和B原子上，B得到La的电子，其s轨道的电子转移至p轨道，因此La呈2.53价而B呈–0.42价，表明La和B之间具有较强的共价键成分。La和B的这种结合也表明LaB6具有较高的体弹性模量、杨氏模量和硬度，同时其离子键成分使其具有一定的脆性。
图 2 LaB6晶体材料的能带结构和分态密度Figure 2. Band structure and partial density of states for the LaB6 crystalline material表 4 LaB6晶体材料的电荷转移Table 4. Charge distributions of LaB6 crystalline materialAtom Charge distribution/e s orbital p orbital d orbital Total charge B 0.88 2.54 0.00 –0.42 La 1.50 5.46 1.51 2.53 3. 结 论
基于密度泛函理论和Birch-Murnaghan物态方程，系统分析了LaB6晶体材料的弹性常数参数、体弹性模量、剪切弹性模量和力学性能。结果表明：LaB6晶体材料具有较大的
C11 ，表明在此主轴应力方向上具有较大的弹性常数；LaB6晶体具有较大的体弹性模量，并且体弹性模量呈各向同性，而剪切弹性模量呈各向异性；LaB6晶体的杨氏模量为227.85 GPa，泊松比为0.26，体剪弹性模量比值λ达到1.44，表明其脆性较强，不易发生弹性形变；LaB6晶体的硬度达到11.56 GPa，平均弹性波速达4.87 km/s；LaB6晶体呈金属性，带隙宽度为0.20 eV，内部电子具有较强的局域性，La和B之间具有较强的共价键成分。 -
图 2 30CrMnMo钢的动态真应力-真应变曲线
Figure 2. Dynamic true stress-strain curves of 30CrMnMo steel
图 3 帽型试件SHPB冲击剪切夹持端状态
Figure 3. State of the SHPB impact shear clamping end of the cap shaped specimen
图 4 不同长度子弹撞击产生的脉冲应力波形
Figure 4. Pulse stress waveforms generated by bullet impacts of different lengths
图 5 不同速度冲击时帽型试件的脉冲应力比冲量
Figure 5. Pulse stress specific impulse of hat shaped specimens under different impact velocities
图 6 采用不同网格尺寸模拟的绝热剪切带的温度云图
Figure 6. Temperature nephogram of simulated adiabatic shear bands with different grid sizes
图 7 脉冲应力幅值-撞击速度曲线的模拟结果与实验结果的对比
Figure 7. Comparison of simulation and experimental results of pulse stress amplitude-impact velocity curves
图 9 典型单元的等效应力及温度随时间变化曲线
Figure 9. Variations of equivalent stress and temperature of typical elements with time
图 11 绝热剪切带形成过程中典型时刻的温度云图
Figure 11. Temperature nephogram at typical moments during the formation of adiabatic shear bands
表 1 帽型试件的冲击剪切实验
Table 1. Impact shear experiment on cap shaped specimens
Case L/mm v0/(m·s−1) σim/MPa Δt/μs ts/μs As/mm2 I/(MPa·μs·mm−2) 1 100 43.00 900 39 34 54.6 560.4 2 200 19.00 370 77 54.6 528.6 3 200 24.33 506 77 61 54.6 565.3 4 300 19.12 384 115 81 54.6 569.7 5 300 20.12 412 115 75 54.6 565.9 
下载: 导出CSV
表 2 采用3种网格尺寸模拟得到的剪切区
Table 2. Results of shear zone simulated with three grid sizes
Mesh size/(μm×μm) Calculation time/h Data file/GB CPU number ASB formation 8×8 90.00 180 8 Yes 15×15 1.36 54 8 Yes 30×30 0.24 30 8 Yes
下载: 导出CSV
-
[1] KURIYAMA S, MEYERS M A. Numerical modeling of the propagation of an adiabatic shear band [J]. Metallurgical Transactions A, 1986, 17(3): 443–450. doi: 10.1007/BF02643951 [2] BONNET-LEBOUVIER A S, MOLINARI A, LIPINSKI P, et al. Analysis of the dynamic propagation of adiabatic shear bands [J]. International Journal of Solids and Structures, 2002, 39(16): 4249–4269. doi: 10.1016/S0020-7683(02)00244-5 [3] MEDYANIK S N, LIU W K, LI S F. On criteria for dynamic adiabatic shear band propagation [J]. Journal of the Mechanics and Physics of Solids, 2007, 55(7): 1439–1461. doi: 10.1016/j.jmps.2006.12.006 [4] TENG X, WIERZBICKI T, COUQUE H. On the transition from adiabatic shear banding to fracture [J]. Mechanics of Materials, 2007, 39(2): 107–125. doi: 10.1016/j.mechmat.2006.03.001 [5] 付应乾, 董新龙. 帽型试样动态绝热剪切破坏演化分析 [J]. 固体力学学报, 2015, 36(5): 392–400. doi: 10.19636/j.cnki.cjsm42-1250/o3.2015.05.004FU Y Q, DONG X L. Study of evolution of adiabatic shear failure in hat-shaped specimen under dynamic loading [J]. Chinese Journal of Solid Mechanics, 2015, 36(5): 392–400. doi: 10.19636/j.cnki.cjsm42-1250/o3.2015.05.004 [6] GUO Y Z, RUAN Q C, ZHU S X, et al. Dynamic failure of titanium: temperature rise and adiabatic shear band formation [J]. Journal of the Mechanics and Physics of Solids, 2020, 135: 103811. doi: 10.1016/j.jmps.2019.103811 [7] ZHU S X, GUO Y Z, CHEN H S, et al. Formation of adiabatic shear band within Ti-6Al-4V: effects of stress state [J]. Mechanics of Materials, 2019, 137: 103102. doi: 10.1016/j.mechmat.2019.103102 [8] 王财源, 余丰. 复杂应力状态下GCr15轴承钢的动态剪切及熔融特性研究 [J]. 力学季刊, 2022, 43(1): 84–92. doi: 10.15959/j.cnki.0254-0053.2022.01.009WANG C Y, YU F. Research on dynamic shearing and melting characteristics of GCr15 bearing steel under complex stress state [J]. Chinese Quarterly of Mechanics, 2022, 43(1): 84–92. doi: 10.15959/j.cnki.0254-0053.2022.01.009 [9] MEYER L W, MANWARING S. Critical adiabatic shear strength of low alloyed steel under compressive loading [M]//MURR L E, STAUDHAMMER K P, MEYERS M A. Metallurgical Applications of Shock Wave and High-Strain-Rate Phenomena. New York: Marcel Dekker Inc., 1985: 657–674. [10] 王礼立. 应力波基础 [M]. 2版. 北京: 国防工业出版社, 2005.WANG L L. Foundation of stress waves [M]. 2nd ed. Beijing: National Defense Industry Press, 2005. [11] 杨扬, 程信林. 绝热剪切的研究现状及发展趋势 [J]. 中国有色金属学报, 2002, 12(3): 401–408. doi: 10.3321/j.issn:1004-0609.2002.03.001YANG Y, CHENG X L. Current status and trends in researches on adiabatic shearing [J]. The Chinese Journal of Nonferrous Metals, 2002, 12(3): 401–408. doi: 10.3321/j.issn:1004-0609.2002.03.001 [12] TRESCA M H. On further applications of the flow of solids [J]. Proceedings of the Institution of Mechanical Engineers, 1878, 29(1): 301–345. doi: 10.1243/PIME_PROC_1878_029_017_02 [13] RULE W K, JONES S E. A revised form for the Johnson-Cook strength model [J]. International Journal of Impact Engineering, 1998, 21(8): 609–624. doi: 10.1016/S0734-743X(97)00081-X [14] BØRVIK T, DEY S, CLAUSEN A H. Perforation resistance of five different high-strength steel plates subjected to small-arms projectiles [J]. International Journal of Impact Engineering, 2009, 36(7): 948–964. doi: 10.1016/j.ijimpeng.2008.12.003 [15] 周刚毅. TA2钛合金绝热剪切破坏特性及应力状态、晶粒度影响 [D]. 宁波: 宁波大学, 2018.ZHOU G Y. Adiabatic shearing behavior of TA2 titanium alloy and its influence of stress state, grain size [D]. Ningbo: Ningbo University, 2018. [16] LI J R, YU J L, WEI Z G. Influence of specimen geometry on adiabatic shear instability of tungsten heavy alloys [J]. International Journal of Impact Engineering, 2003, 28(3): 303–314. doi: 10.1016/S0734-743X(02)00022-2 [17] WEI Z G, YU J L, LI J, et al. Influence of stress condition on adiabatic shear localization of tungsten heavy alloys [J]. International Journal of Impact Engineering, 2001, 26(1): 843–852. [18] RANC N, TARAVELLA L, PINA V, et al. Temperature field measurement in titanium alloy during high strain rate loading-adiabatic shear bands phenomenon [J]. Mechanics of Materials, 2008, 40(4/5): 255–270. doi: 10.1016/j.mechmat.2007.08.002 [19] WRIGHT T W, OCKENDON H. A model for fully formed shear bands [J]. Journal of the Mechanics and Physics of Solids, 1992, 40(6): 1217–1226. doi: 10.1016/0022-5096(92)90013-R [20] BRONKHORST C A, CERRETA E K, XUE Q, et al. An experimental and numerical study of the localization behavior of tantalum and stainless steel [J]. International Journal of Plasticity, 2006, 22(7): 1304–1335. doi: 10.1016/j.ijplas.2005.10.002 -
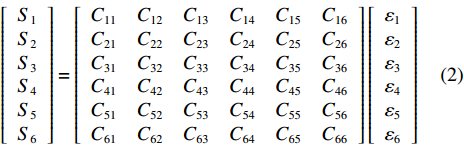
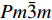
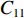
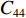
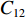
下载:
下载:
点击查看大图
计量
- 文章访问数: 163
- HTML全文浏览量: 60
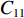
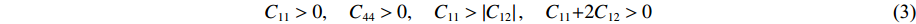
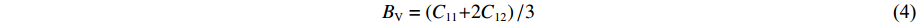
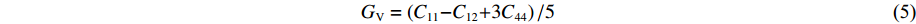
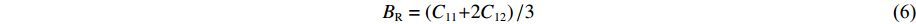
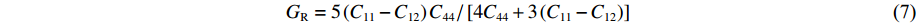
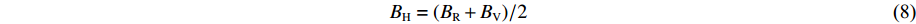
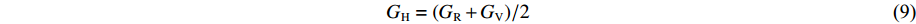
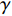
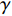
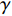
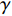
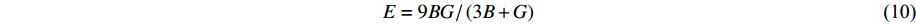
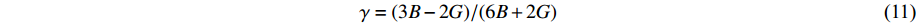
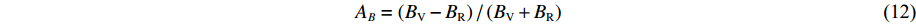
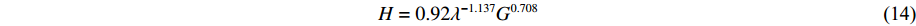
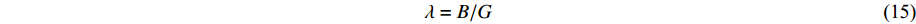
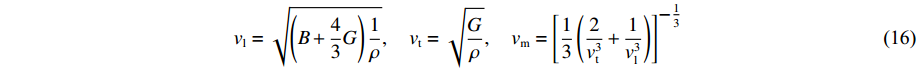
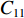
- PDF下载量: 29
