Physico-Mechanical Behavior and Size Effect of Nano-Tungsten under High Pressure
-
摘要: 探究尺寸效应对材料高压物性的影响规律有助于开发具有新颖或者改良特性的新材料。采用金刚石对顶砧结合同步辐射X射线衍射技术,研究了平均晶粒尺寸分别为30和65 nm的多晶钨粉在高压下的静态压缩行为。通过分析每个压力点下X射线衍射图谱的峰位和半高宽等,得到了纳米金属钨在高压下的晶胞体积、晶粒尺寸和微观应变等。通过拟合三阶Birch-Murnaghan方程,得到了30和65 nm钨的体弹模量,分别为257(7) GPa和343(8) GPa。结合前人的研究结果发现,当晶粒尺寸从微米降低至10 nm,钨的屈服强度逐渐增大,10 nm钨的屈服强度较微米晶样品的屈服强度提高了3.5倍;体弹模量呈现先增大后减小的趋势,30 nm钨的体弹模量较65 nm钨减小了25%。Abstract: Exploring the influence of size effect on the physical properties of materials under high pressure is helpful for the development of new materials with novel or improved properties. The static compression behaviors of polycrystalline tungsten powder with average grain sizes of 30 and 65 nm under high pressure were studied by using diamond anvil cell (DAC) combined with synchrotron radiation X-ray diffraction respectively. By analyzing the peak position and the half-height width of the X-ray diffraction spectrum at each pressure, the unit cell volume, grain size, and microscopic strain of nano-tungsten metal under high pressure were obtained. By fitting the third Birch-Murnaghan equation, the bulk moduli of 30 and 65 nm tungsten are obtained to be 257(7) GPa and 343(8) GPa, respectively. Combined with the results of previous studies, it is found that when the gain size decreases from micron to 10 nm, the yield strength of tungsten at 10 nm increases by 3.5 times compared with that of microcrystal samples; the bulk modulus shows a tendency of increasing firstly and then decreasing, and the bulk elastic modulus of tungsten at 30 nm decreases by 25% compared with that of tungsten at 65 nm.
-
Key words:
- equation of state /
- yield strength /
- bulk modulus /
- high pressure /
- nanocrystalline
-
自1999年美国圣地亚国家实验室开展磁驱动平面实验以来,磁驱动飞片发射、磁驱动冲击压缩、磁驱动准等熵压缩、磁驱动样品研究等平面实验取得了飞速发展,促进了高压状态方程、高能量密度物理和高功率脉冲技术等研究的发展[1–6]。随着磁驱动平面实验的开展,磁驱动平面实验理论取得了巨大进展。2001年,Reisman等[7]首次采用磁流体力学程序对磁驱动飞片实验进行了数值模拟。2003年,Lemke等[8]提出了磁驱动平面实验中边界磁场的初始公式;2011年,他们进一步发展了该公式,认为边界磁场强度与电极宽度和阴阳电极之间的间隙两者之和成反比[9]。基于上述边界磁场的磁流体力学程序并不能完全正确模拟磁驱动双侧飞片发射实验[10],在磁驱动实验后期,模拟的飞片自由面速度与实验测量结果的偏差较大。2018年,Kan等[11]指出,电流不是从阴、阳电极电流加载端的端面通过,而是从阴、阳电极电流加载端的液/气界面通过,从而提出具有烧蚀影响的边界磁场公式,解决了磁驱动双侧飞片实验后期模拟与测量不一致的问题。
磁驱动样品实验主要用于样品材料的状态方程参量、强度等研究。本研究将采用考虑烧蚀影响的边界磁场公式和磁流体力学程序,对大电流脉冲功率装置上的磁驱动样品实验进行模拟研究,分析磁驱动样品实验结构系数的影响因素,确定结构系数与样品材料、样品厚度、电极宽度的依赖关系,以期为磁驱动样品实验的数值设计和预测提供理论支撑。
1. 负载结构
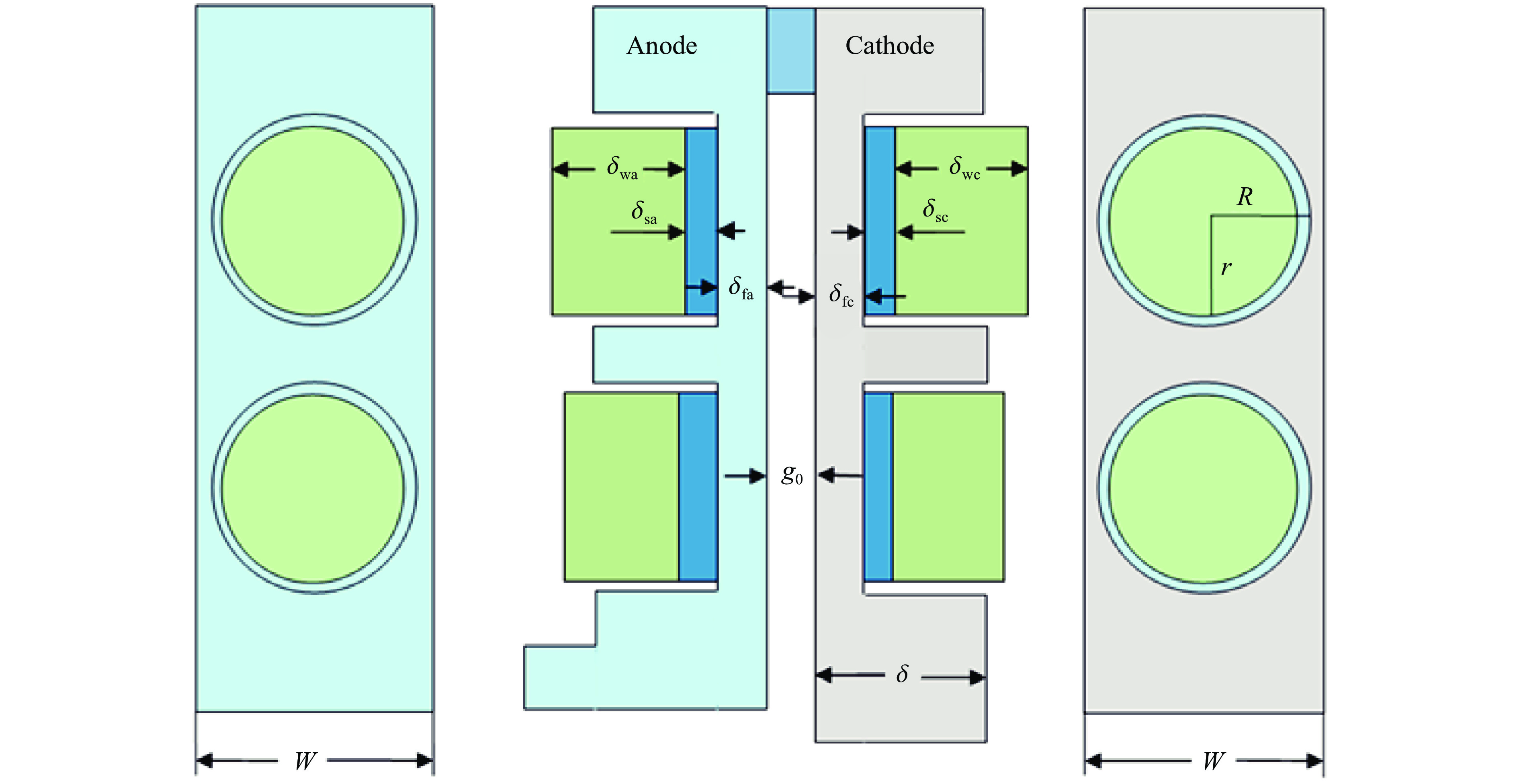
磁驱动样品实验的负载结构如图1所示,其中:阴极和阳极采用铝金属长方体,厚度和宽度相同,设
δ 为初始厚度,W为初始宽度,g0 为阴、阳极板之间的初始间隙。磁驱动样品实验采用上下结构,在阴阳极上下部嵌入两对半径为R的圆柱形飞片,飞片后界面紧贴半径为r(r<R)的圆柱体样品,样品后界面紧贴半径为r的圆柱体LiF窗口,设δfc、δfa分别为阴、阳极上飞片的厚度,δsc、δsa分别为阴、阳极上样品的厚度,δwc、δwa分别为阴、阳极上窗口的厚度。采用激光速度干涉仪测量样品/窗口界面速度。若样品厚度为零,即飞片与窗口之间无样品,则实验测量的速度为飞片/窗口界面速度。磁驱动样品实验的负载参数列于表1。表 1 磁驱动样品实验负载参数Table 1. Load parameters for magnetically driven sample experimentsExp. No. Sample material δfa/mm δsa/mm δwa/mm δfc/mm δsc/mm δwc/mm g0/mm W/mm Exp1-top MgAl 0.970 0 8 0.983 0.565 8 1.200 13 Exp1-bottom MgAl 0.984 0.968 8 0.988 0.788 8 1.200 13 Exp2-top Sn 1.000 0.386 8 1.000 0 8 1.200 11 Exp2-bottom Sn 1.000 0.386 8 1.000 0 8 1.200 11 Exp3-top Sn 0.993 0.417 8 0.990 0.451 8 1.200 13 Exp3-bottom Sn 0.993 0 8 0.990 0.643 8 1.200 13 Exp4-top Sn 0.995 0.433 8 0.998 0.650 8 1.175 15 Exp4-bottom Sn 0.990 0.817 8 0.991 0 8 1.175 15 2. 数值模拟
二维磁驱动数值模拟程序(two-dimensional magnetically driven simulation code,MDSC2)是基于二维四边形网格的拉格朗日磁流体力学程序,包含rz平面[12]和xy平面[13]两种程序模式。rz平面模式用于求解磁驱动柱对称问题,xy平面模式用于求解磁驱动飞片等平面实验问题。MDSC2程序已成功用于Z箍缩[14]、磁驱动飞片发射[11]、带窗口磁驱动准等熵压缩[15]等磁驱动实验的模拟、设计和研究。磁驱动柱面实验和磁驱动平面实验的边界磁感应强度公式是不同的。对于磁驱动平面实验,MDSC2中的电极电流加载端的边界磁感应强度B为
B=fμ0I(t)/[g(t)+gm(t)+W] (1) 式中:f为结构系数,
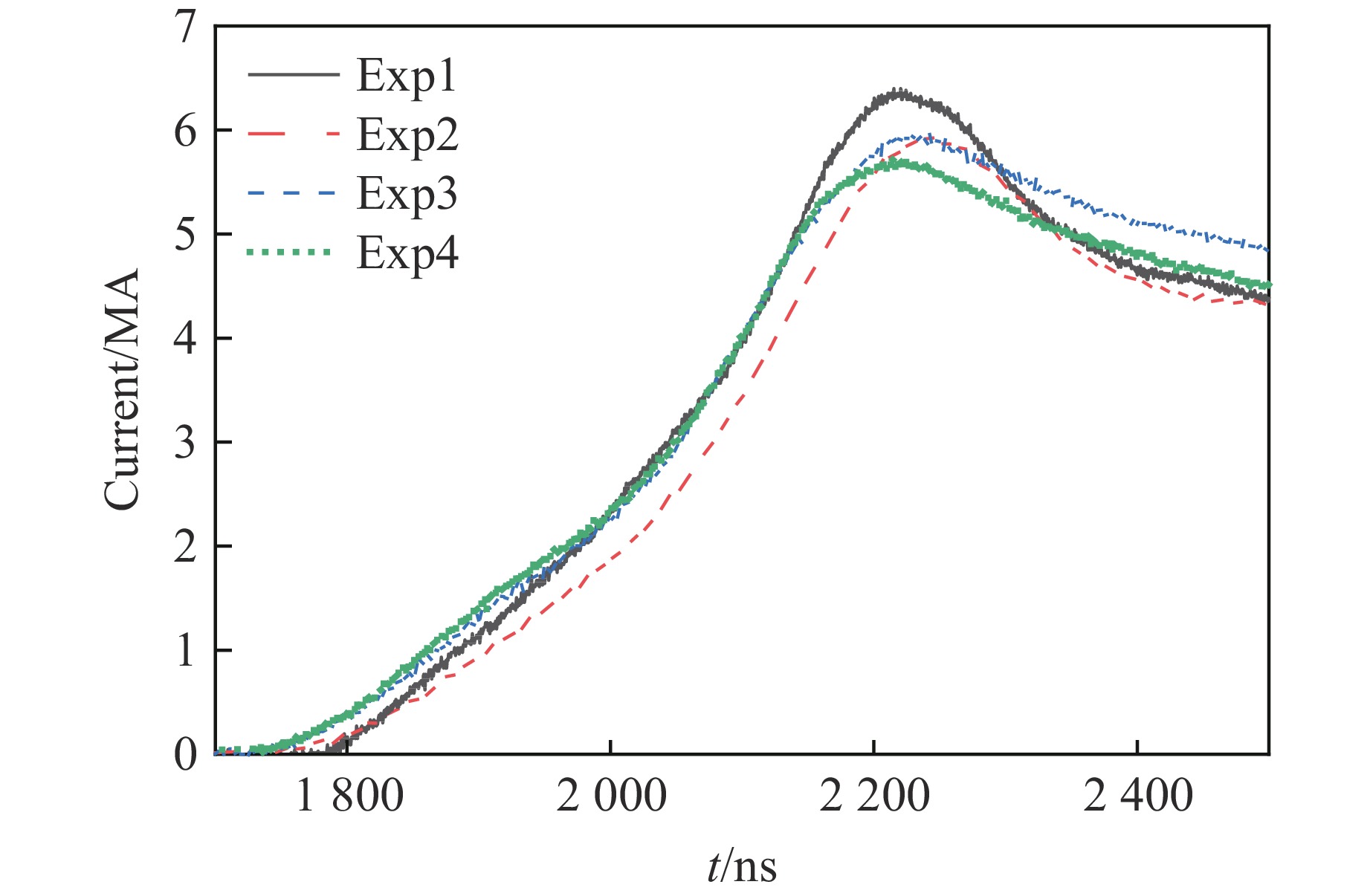
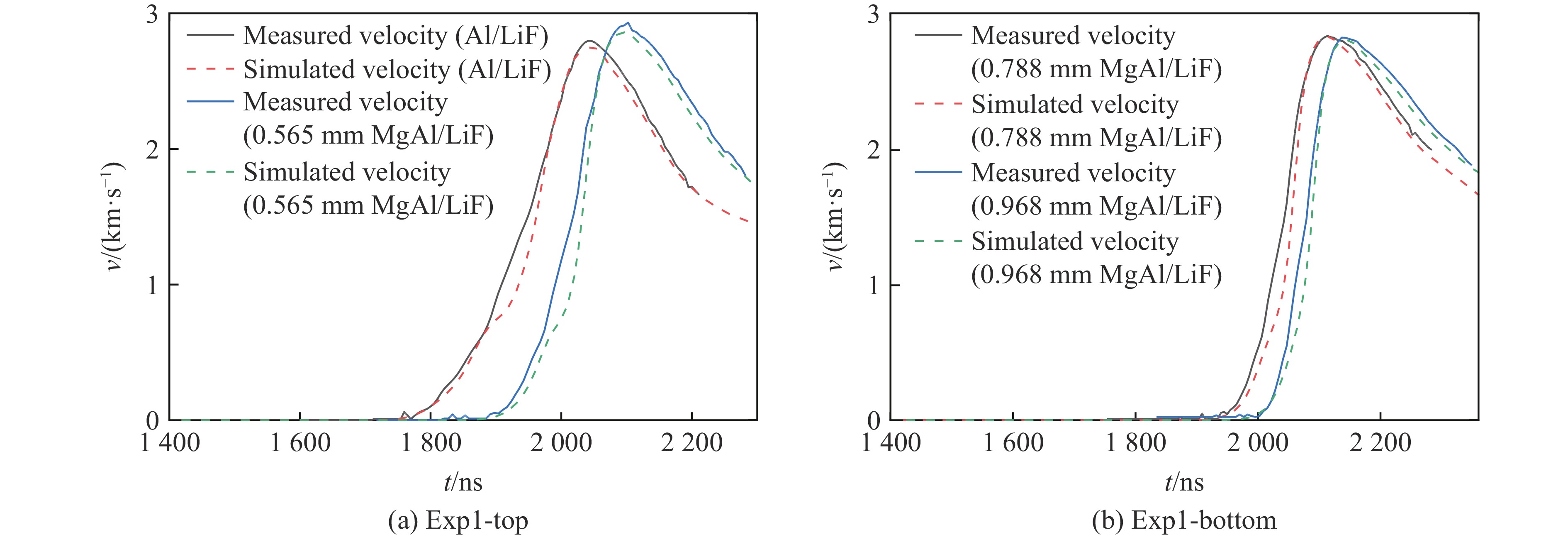
μ0 为真空磁导率,I(t)为实验电流,g(t)为阴、阳电极之间的间隙,gm(t) 为阴、阳电极电流加载端厚度方向上气体宽度之和[11]。采用MDSC2程序,对大电流脉冲装置上的磁驱动样品实验进行模拟。Exp1-top和Exp1-bottom为同一电流驱动上下两组MgAl样品的磁驱动实验(Exp1),阴阳极的极板宽度为13 mm。Exp1-top为磁驱动单侧MgAl样品实验:阳极上飞片与窗口直接相连,实验测量的是飞片/窗口界面速度;阴极上飞片与窗口之间嵌入0.565 mm厚的MgAl样品,实验测量的是MgAl/窗口界面速度。Exp1-bottom为磁驱动双侧MgAl样品实验,阴、阳极上飞片与窗口之间嵌入不同厚度的MgAl样品,厚度分别为0.788和0.968 mm,实验测量的速度为样品/窗口界面速度。磁驱动样品实验Exp1的电流变化曲线见图2。图3为磁驱动单侧MgAl样品实验Exp1-top和磁驱动双侧MgAl样品实验Exp1-bottom的界面速度。可见,MDSC2程序模拟的界面速度与实验测量的界面速度基本一致,说明MDSC2程序能正确模拟磁驱动MgAl样品实验Exp1。由图2和图3可知,磁驱动MgAl样品实验Exp1的结构系数为0.78。
Exp2-top和Exp2-bottom为同一电流驱动上下两组Sn样品的磁驱动实验(Exp2),初始结构完全相同,阴阳极的极板宽度均为11 mm。阳极上飞片与窗口之间都嵌入0.386 mm厚的Sn样品,阴极上飞片与窗口直接相连。Exp2实验中,仅对Exp2-bottom的界面进行了速度测量。磁驱动Sn样品实验Exp2的实测电流见图2。图4为Exp2-bottom磁驱动单侧Sn样品实验的界面速度。由图4可知,MDSC2程序能正确模拟磁驱动Sn样品实验Exp2。结合图2可得,Exp2的结构系数为0.84。
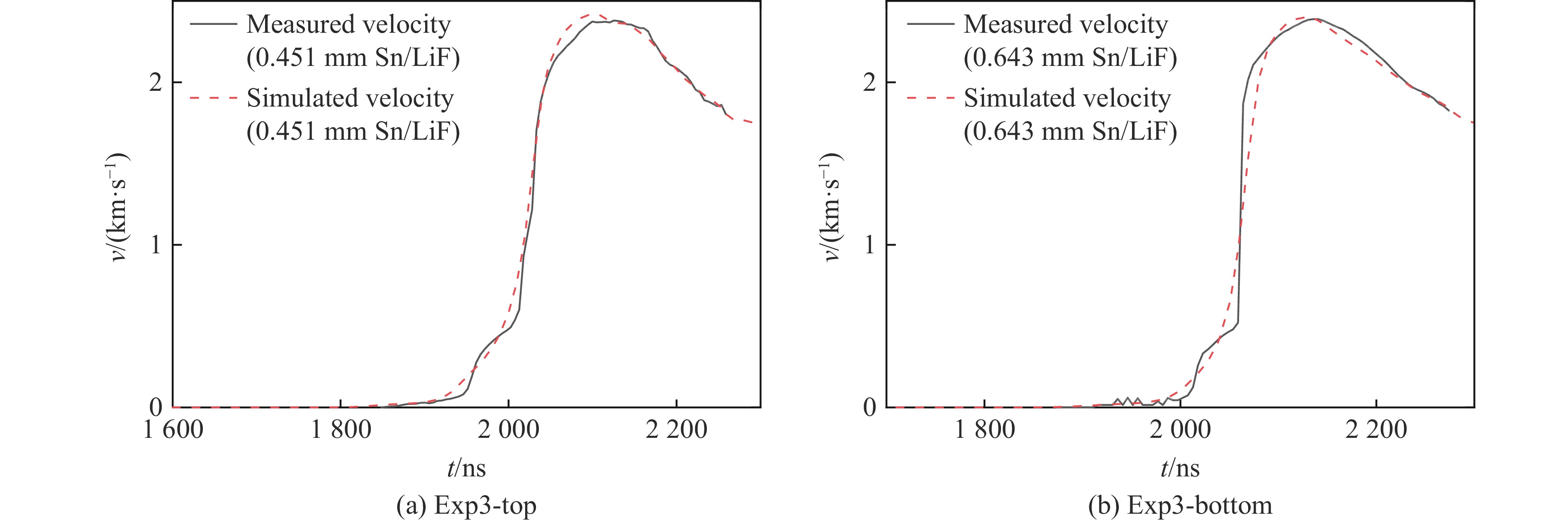
Exp3-top和Exp3-bottom为同一电流驱动上下两组Sn样品的磁驱动样品实验(Exp3),阴阳极的极板宽度为13 mm。其中:Exp3-top为磁驱动双侧Sn样品实验,阴、阳电极上飞片与窗口之间嵌入不同厚度的Sn样品,阴、阳极上Sn样品的厚度分别为0.451和0.417 mm,实验测量的是0.451 mm Sn/LiF界面速度;Exp3-bottom为磁驱动单侧Sn样品实验,阳极上飞片与窗口直接相连,阴极上飞片与窗口之间嵌入0.643 mm厚的Sn样品,实验测量的是0.643 mm Sn/LiF界面速度。Exp3的实测电流见图2。图5给出了Exp3-top和Exp3-bottom的界面速度,可见,MDSC2程序能正确模拟Exp3。结合图2可得,Exp3的结构系数为0.85。
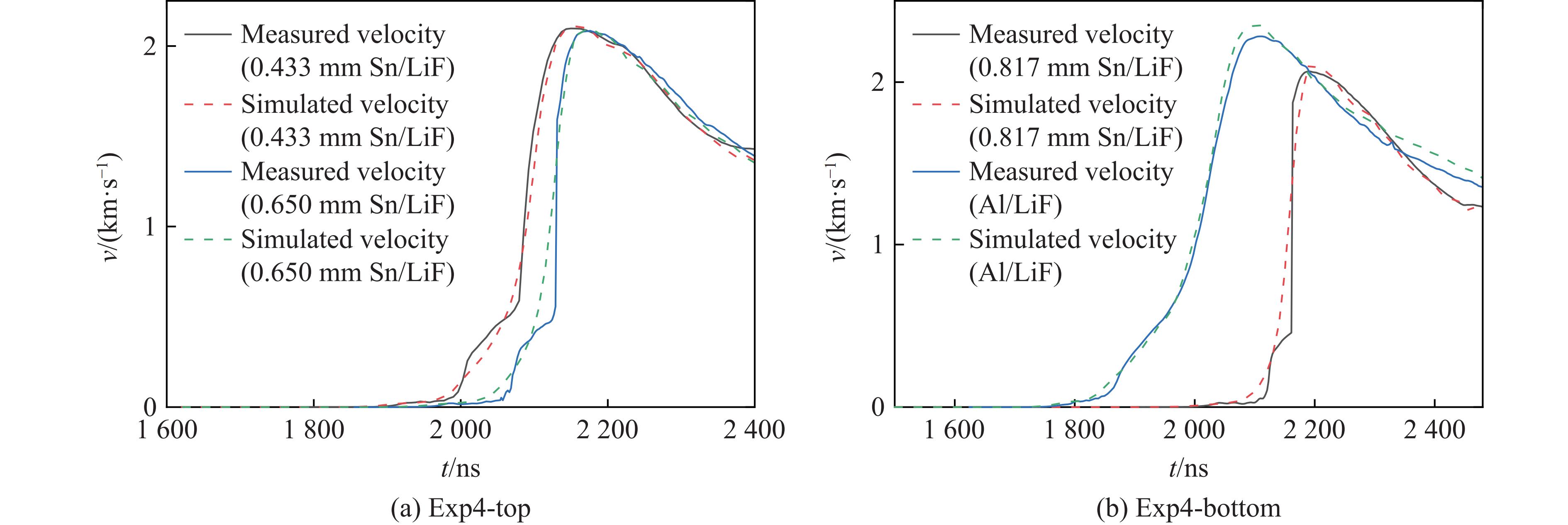
Exp4-top和Exp4-bottom为同一电流驱动上下两组Sn样品的磁驱动样品实验(Exp4),阴阳电极的极板宽度为15 mm。其中:Exp4-top为磁驱动双侧Sn样品实验,阴、阳电极上飞片与窗口之间嵌入不同厚度的Sn样品(厚度分别为0.433和0.650 mm),实测速度为Sn/LiF界面速度;Exp4-bottom为磁驱动单侧Sn样品实验,阳极上飞片与窗口之间嵌入0.817 mm厚的Sn样品,实验测量的是0.817 mm Sn/LiF界面速度,阴极上飞片与窗口直接相连,实验测量的是Al/LiF界面速度。Exp4的实测电流见图2。Exp4-top和Exp4-bottom的界面速度如图6所示,可见,MDSC2程序能正确模拟Exp4。结合图2可知,Exp4的结构系数为0.88。
综合以上结果,MDSC2程序能正确模拟大电流脉冲功率装置磁驱动样品实验,所模拟的磁驱动样品/窗口界面速度与实验测量的界面速度一致。
3. 结构系数
尽管利用式(1)的磁流体力学程序MDSC2能正确模拟磁驱动样品实验,但是,对于不同的磁驱动样品实验,其结构系数不同。由于结构系数不同时,飞片的边界磁场强度也会不同,致使飞片所受的洛伦兹力不同,因此,同一模型的磁流体力学模拟结果显然不同。磁驱动样品实验的结构系数直接影响磁驱动样品实验模拟的正确性,也影响磁驱动样品实验设计、预测以及实验数据解读的准确性,因而确定结构系数的影响因素和变化规律非常重要。
由第2节可知,磁驱动样品实验的结构系数是一个常数,仅由磁驱动样品实验的初始条件决定。磁驱动样品实验的初始条件包括:电极板的宽度、阴阳电极之间的间隙、飞片材料类型、飞片厚度、样品材料类型、样品厚度、窗口材料类型、窗口厚度等。对于表1中的磁驱动样品实验:飞片均为金属铝,飞片厚度约1 mm,相对偏差不大于3%,可以看成同一厚度;窗口材料均为LiF,窗口厚度均为8 mm。因此,初始条件中变化的因素包括电极板宽度、阴阳极间隙、样品材料类型和样品厚度。表2列出了磁驱动样品实验的结构系数。
表 2 磁驱动样品实验的结构系数Table 2. Structure coefficients of magnetically driven sample experimentsExp. No. Sample material δsa/mm δsc/mm g0/mm W/mm f Exp1-top MgAl 0 0.565 1.200 13 0.78 Exp1-bottom MgAl 0.968 0.788 1.200 13 0.78 Exp2-top Sn 0.386 0 1.200 11 0.84 Exp2-bottom Sn 0.386 0 1.200 11 0.84 Exp3-top Sn 0.417 0.451 1.200 13 0.85 Exp3-bottom Sn 0 0.643 1.200 13 0.85 Exp4-top Sn 0.433 0.650 1.175 15 0.88 Exp4-bottom Sn 0.817 0 1.175 15 0.88 Exp1-top和Exp1-bottom的样品材料、阴阳极间隙、电极板宽度都相同,尽管样品厚度明显不同,但结构系数相同,Exp3、Exp4也有相似的结果,说明样品厚度对结构系数的影响很小。
在Exp1和Exp3中,极板宽度均为13 mm,阴阳极间隙均为1.200 mm,样品分别为MgAl合金和Sn,结构系数分别为0.78和0.84,考虑到样品厚度对结构系数的影响很小,因此结构系数与样品材料有关。
在Exp2、Exp3和Exp4中,样品材料均为Sn,阴阳极间隙分别为 1.200、1.200和1.175 mm(相对偏差很小,可看作同一间隙),电极宽度分别为11、13、15 mm(相对偏差很大),结构系数分别为0.84、0.85和0.88,说明结构系数的影响因素主要是阴阳电极板宽度,极板宽度越宽,结构系数越大。
4. 结 论
采用考虑烧蚀影响的边界磁场公式和MDSC2程序,对磁驱动样品实验进行了模拟,并分析了磁驱动样品实验结构系数的影响因素和规律。结果表明:考虑烧蚀影响的磁流体力学程序能正确模拟磁驱动样品实验,结构系数与实验过程无关,仅由实验的负载结构确定。结构系数与样品材料相关,样品材料不同,结构系数不同;样品厚度对结构系数的影响很小;电极宽度对结构系数的影响较大,电极宽度越宽,结构系数越大。研究结果为磁驱动样品实验的设计、预测等提供了理论依据。
-
图 8 钨的晶胞体积压缩率随压力的变化(实线是基于30和65 nm钨晶粒的晶胞体积压缩率-压力数据点,通过三阶Birch-Murnaghan状态方程拟合的曲线;离散的数据点为其他研究人员所得结果[8–9, 22–26])
Figure 8. Volume compression ratio of unit cell for tungsten as a function of pressure (The solid lines are fitting curves of unit cell volume compression ratio and pressure for tungsten crystals with sizes of 30 and 65 nm by the third Birch-Murnaghan equation of state; the discrete data points are obtained by other researchers[8–9, 22–26])
表 1 各种晶粒尺寸下钨的体弹模量
Table 1. Bulk modulus of tungsten with a series of particle sizes
Grain size Pressure/GPa B0/GPa B′0 Pressure transmitting
mediumMethod Ref. 30 nm
65 nm
50 nm
4−6 μm
<4 μm
4−6 μm
4−6 μm
4−6 μm
Bulk
Bulk0−20
0−30
16−53
0−69
0−153
0−96
0−96
0−96
264−676
0−300257(7)
343(8)
338
312(36)
295(4)
312(10)
256(2)
342(1)
280(9)
3094.32 (fixed)
4.32 (fixed)
4.32 (fixed)
4.32 (fixed)
4.32 (0.11)
4.32 (fixed)
4.32 (fixed)
4.32 (fixed)
4.32 (fixed)
4.32 (fixed)None
None
None
None
Helium
NoneAXRD
AXRD
RXRD (ψ=54.7°)
RXRD (ψ=54.7°)
AXRD
RXRD (ψ=54.7°)
RXRD (ψ=0°)
RXRD (ψ=90°)
Shock compression
Shock compressionThis work
This work
Ref. [7]
Ref. [8]
Ref. [22]
Ref. [9]
Ref. [9]
Ref. [9]
Ref. [23]
Ref. [24] -
[1] CORDERO Z C, KNIGHT B E, SCHUH C A. Six decades of the Hall-Petch effect: a survey of grain-size strengthening studies on pure metals [J]. International Materials Reviews, 2016, 61(8): 495–512. doi: 10.1080/09506608.2016.1191808 [2] SCHIØTZ J, DI TOLLA F D, JACOBSEN K W. Softening of nanocrystalline metals at very small grain sizes [J]. Nature, 1998, 391(6667): 561–563. doi: 10.1038/35328 [3] YIP S. The strongest size [J]. Nature, 1998, 391(6667): 532–533. doi: 10.1038/35254 [4] SCHIØTZ J, JACOBSEN K W. A maximum in the strength of nanocrystalline copper [J]. Science, 2003, 301(5638): 1357–1359. doi: 10.1126/science.1086636 [5] ZHOU X L, FENG Z Q, ZHU L L, et al. High-pressure strengthening in ultrafine-grained metals [J]. Nature, 2020, 579(7797): 67–72. doi: 10.1038/s41586-020-2036-z [6] GU Q F, KRAUSS G, STEURER W, et al. Unexpected high stiffness of Ag and Au nanoparticles [J]. Physical Review Letters, 2008, 100(4): 045502. doi: 10.1103/PhysRevLett.100.045502 [7] YANG J, DENG W, LI Q, et al. Strength enhancement of nanocrystalline tungsten under high pressure [J]. Matter and Radiation at Extremes, 2020, 5(5): 058401. doi: 10.1063/5.0005395 [8] HE D W, DUFFY T S. X-ray diffraction study of the static strength of tungsten to 69 GPa [J]. Physical Review B, 2006, 73(13): 134106. doi: 10.1103/PhysRevB.73.134106 [9] XIONG L, LI B, TANG Y M, et al. Radial X-ray diffraction study of the static strength and texture of tungsten to 96 GPa [J]. Solid State Communications, 2018, 269: 83–89. doi: 10.1016/j.ssc.2017.10.016 [10] 邹勇, 陈立溁. 贵金属金的状态方程 [J]. 高压物理学报, 2006, 20(3): 308–312. doi: 10.3969/j.issn.1000-5773.2006.03.015ZOU Y, CHEN L Y. Equation of state for gold [J]. Chinese Journal of High Pressure Physics, 2006, 20(3): 308–312. doi: 10.3969/j.issn.1000-5773.2006.03.015 [11] MUSTAPHA S, NDAMITSO M M, ABDULKAREEM A S, et al. Comparative study of crystallite size using Williamson-Hall and Debye-Scherrer plots for ZnO nanoparticles [J]. Advances in Natural Sciences: Nanoscience and Nanotechnology, 2019, 10(4): 045013. doi: 10.1088/2043-6254/ab52f7 [12] WU Z Q, LIN F. Evaluation of Pt and Au pressure scales based on MgO absolute pressure scale [J]. Science China Earth Sciences, 2017, 60(1): 114–123. doi: 10.1007/s11430-015-0232-4 [13] WANG Y, AHUJA R, JOHANSSON B. Reduction of shock-wave data with mean-field potential approach [J]. Journal of Applied Physics, 2002, 92(11): 6616–6620. doi: 10.1063/1.1518781 [14] HAMMERSLEY A P, SVENSSON S O, HANFLAND M, et al. Two-dimensional detector software: from real detector to idealised image or two-theta scan [J]. High Pressure Research, 1996, 14(4): 235–248. doi: 10.1080/08957959608201408 [15] SINGH T B, REY L, GARTIA R K. Applications of PeakFit software in thermoluminescence studies [J]. Indian Journal of Pure & Applied Physics, 2011, 49(5): 297–302. [16] 李春, 刘泽民, 陈恒杰, 等. PeakFit、1stOpt在弗兰克-赫兹实验数据处理中的应用 [J]. 大学物理实验, 2018, 31(5): 117–123. doi: 10.14139/j.cnki.cn22-1228.2018.05.028LI C, LIU Z M, CHEN H J, et al. Application of PeakFit and 1stOpt in data processing of the Frank-Hertz experiment [J]. Physical Experiment of College, 2018, 31(5): 117–123. doi: 10.14139/j.cnki.cn22-1228.2018.05.028 [17] LANGFORD J. X-ray powder diffraction studies of vitromet samples [J]. Journal of Applied Crystallography, 1971, 4: 164–168. doi: 10.1107/S002188987100654X [18] 余建新, 王晓鹏, 崔喜平. 高温环境下材料泊松比测试方法研究[J]. 实验科学与技术, 2022, 20(1): 28–33.YU J X, WANG X P, CUI X P, Material poisson’s ratio measurement method at elevated temperatures [J]. Experiment Science and Technology, 2022, 20(1): 28–33. [19] EINARSDOTTER K, SADIGH B, GRIMVALL G, et al. Phonon instabilities in fcc and bcc tungsten [J]. Physical Review Letters, 1997, 79(11): 2073–2076. doi: 10.1103/PhysRevLett.79.2073 [20] KATAHARA K, MANGHNANI M, FISHER E. Pressure derivatives of the elastic-moduli of bcc Ti-V-Cr, Nb-Mo and Ta-W alloys [J]. Journal of Physics F: Metal Physics, 1979, 9(5): 773–790. doi: 10.1088/0305-4608/9/5/006 [21] ZHANG J Z, ZHAO Y S, PALOSZ B. Comparative studies of compressibility between nanocrystalline and bulk nickel [J]. Applied Physics Letters, 2007, 90(4): 043112. doi: 10.1063/1.2435325 [22] DEWAELE A, LOUBEYRE P, MEZOUAR M. Equations of state of six metals above 94 GPa [J]. Physical Review B, 2004, 70(9): 094112. doi: 10.1103/PhysRevB.70.094112 [23] HIXSON R S, FRITZ J N. Shock compression of tungsten and molybdenum [J]. Journal of Applied Physics, 1992, 71(4): 1721–1728. doi: 10.1063/1.351203 [24] CHIJIOKE A D, NELLIS W J, SILVERA I F. High-pressure equations of state of Al, Cu, Ta, and W [J]. Journal of Applied Physics, 2005, 98(7): 073526. doi: 10.1063/1.2071449 [25] MING L C, MANGHNANI M H. Isothermal compression of bcc transition metals to 100 kbar [J]. Journal of Applied Physics, 1978, 49(1): 208–212. [26] MA Y Z, CUI Q L, SHEN L H, et al. X-ray diffraction study of nanocrystalline tungsten nitride and tungsten to 31 GPa [J]. Journal of Applied Physics, 2007, 102(1): 013525. [27] CHEN B, PENWELL D, BENEDETTI L R, et al. Particle-size effect on the compressibility of nanocrystalline alumina [J]. Physical Review B, 2002, 66(14): 144101. doi: 10.1103/PhysRevB.66.144101 [28] GLEASON A E, MARQUARDT H, CHEN B, et al. Anomalous sound velocities in polycrystalline MgO under non-hydrostatic compression [J]. Geophysical Research Letters, 2011, 38: L03304. [29] LE GODEC Y, KURAKEVYCH O O, MUNSCH P, et al. Effect of nanostructuration on compressibility of cubic BN [J]. Journal of Superhard Materials, 2012, 34(5): 336–338. doi: 10.3103/S1063457612050085 [30] AL-KHATATBEH Y, LEE K K M, KIEFER B. Compressibility of nanocrystalline TiO2 anatase [J]. Journal of Physical Chemistry C, 2012, 116(40): 21635–21639. doi: 10.1021/jp3075699 [31] JIANG J Z, OLSEN J S, GERWARD L, et al. Enhanced bulk modulus and reduced transition pressure in γ-Fe2O3 nanocrystals [J]. Europhysics Letters, 1999, 45(2): 275–275. doi: 10.1209/epl/i1999-00158-3 [32] TOLBERT S H. High-pressure structural transformations in semiconductor nanocrystals [J]. Annual Review of Physical Chemistry, 1995, 46(1): 595–625. doi: 10.1146/annurev.pc.46.100195.003115 [33] HONG X G, DUFFY T S, EHM L, et al. Pressure-induced stiffness of Au nanoparticles to 71 GPa under quasi-hydrostatic loading [J]. Journal of Physics: Condensed Matter, 2015, 27(48): 485303. doi: 10.1088/0953-8984/27/48/485303 [34] MIKHEYKIN A S, DMITRIEV V P, CHAGOVETS S V, et al. The compressibility of nanocrystalline Pt [J]. Applied Physics Letters, 2012, 101(17): 173111. doi: 10.1063/1.4758000 [35] CHEN B, PENWELL D, KRUGER M B, et al. Nanocrystalline iron at high pressure [J]. Journal of Applied Physics, 2001, 89(9): 4794–4796. doi: 10.1063/1.1357780 [36] WANG Z W, PISCHEDDA V, SAXENA S K, et al. X-ray diffraction and Raman spectroscopic study of nanocrystalline CuO under pressures [J]. Solid State Communications, 2002, 121(5): 275–279. doi: 10.1016/S0038-1098(01)00509-9 [37] GU Q F, KRAUSS G, GRAMM F, et al. On the compressibility of TiC in microcrystalline and nanoparticulate form [J]. Journal of Physics: Condensed Matter, 2008, 20(44): 445226. doi: 10.1088/0953-8984/20/44/445226 [38] WANG Q M, HE D W, PENG F, et al. Compression behavior of nanocrystalline TiN [J]. Solid State Communications, 2014, 182: 26–29. doi: 10.1016/j.ssc.2013.12.015 -









 下载:
下载:
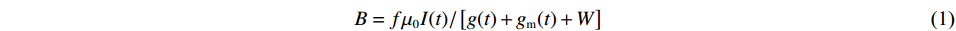
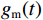






 下载:
下载: