Mechanical Properties and Energy Evolution Characteristics of Fracture-Bearing Rocks under Uniaxial Compression
-
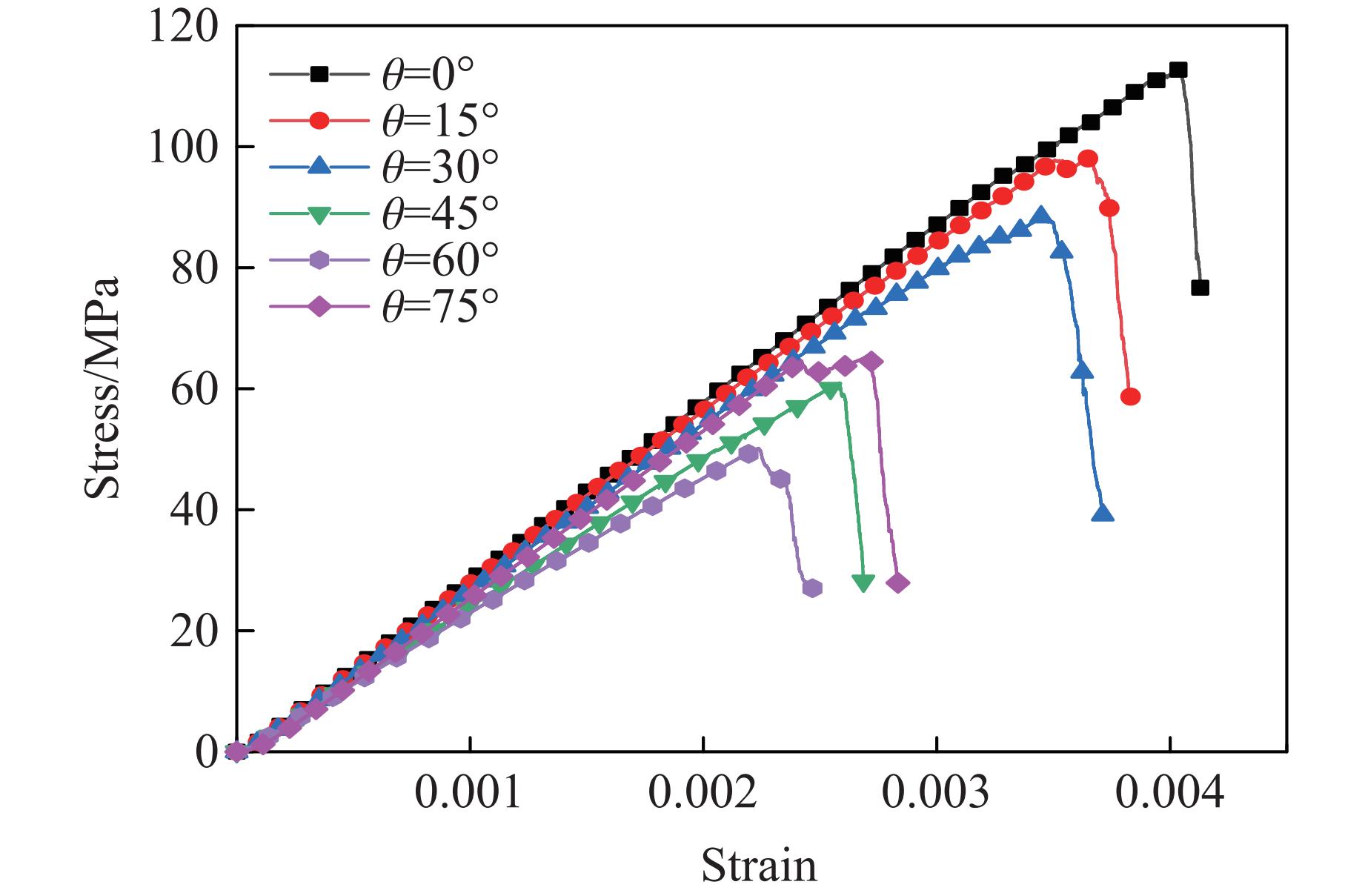
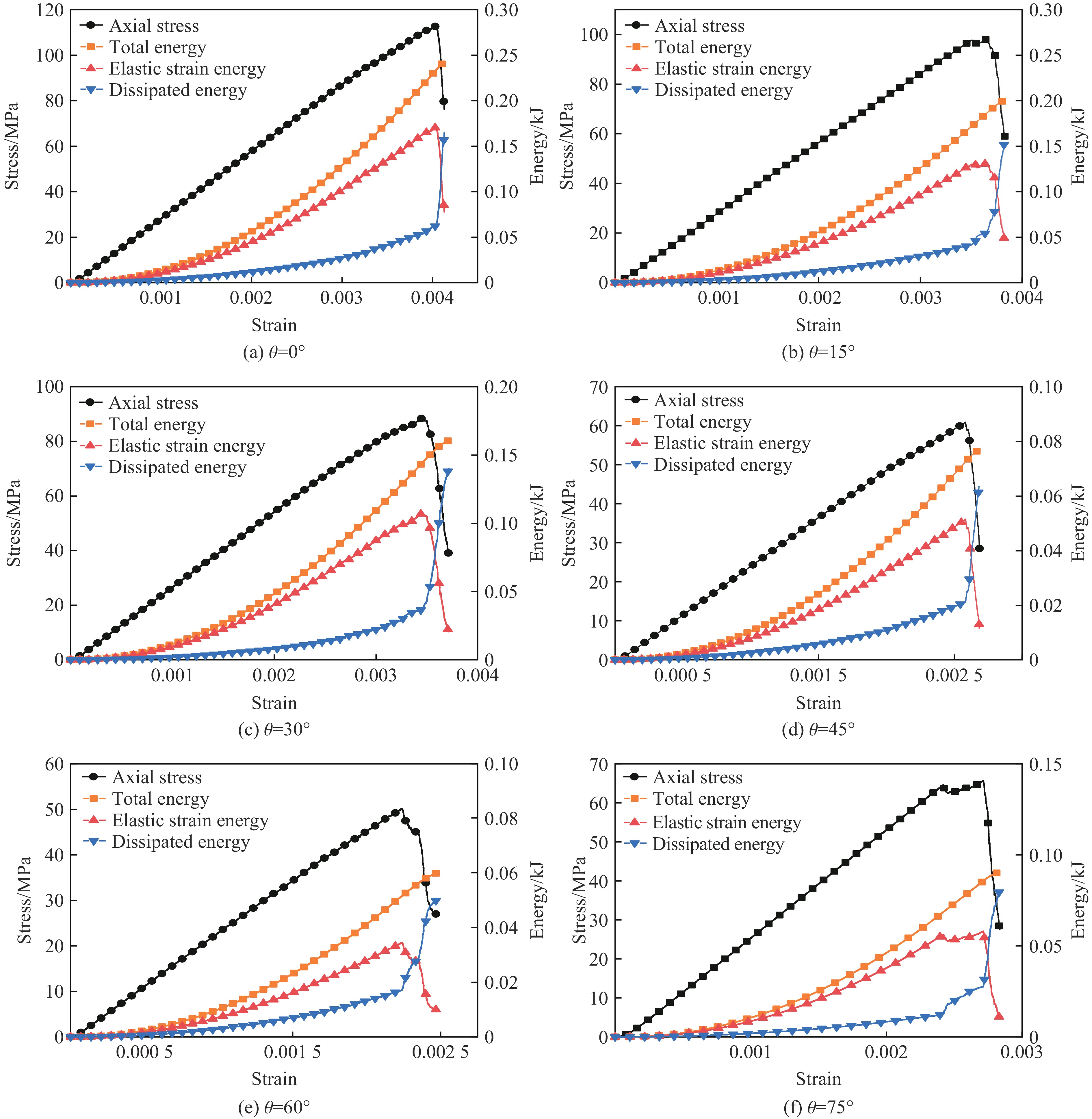
摘要: 为了研究裂隙倾角对岩石力学性能以及破坏过程中能量演化机制的影响,基于颗粒流离散元数值平台,构建了具有不同裂隙倾角的岩石的计算模型,开展了含不同裂隙倾角岩石的单轴压缩数值试验研究。结果表明:随着裂隙倾角的增大,裂隙岩石的峰值强度和弹性模量均呈先减小后增大的“V”形变化趋势;当裂隙倾角较小时,岩石试样主要发生剪切破坏和竖向劈裂破坏,拉剪裂纹数主要呈台阶式增长;裂隙倾角越大,岩石破坏模式将过渡为竖向劈裂和剪切的混合破坏,拉剪裂纹数变化曲线呈指数增长;随着裂隙倾角的增大,岩石试样的总输入能量和弹性应变能呈先减小后增大的变化趋势;裂隙角度越大,耗散能上升越快,但试样破坏时的最终耗散能则越低。裂隙结构的存在对试样在受压破坏时的储能极限均有明显的弱化作用,削弱了岩石吸收和储存弹性应变能的能力,增强了其在峰值应力处的能量耗散能力。Abstract: To study the influence of crack inclination angle on the mechanical properties and the energy evolution mechanism during the rock failure, a calculation model was constructed based on the particle flow dispersion element numerical platform, and uniaxial compression numerical experiments were conducted on rock samples with different crack inclination angles. The research results indicate that as the crack inclination angle increases, the peak strength and elastic modulus of fractured rocks show a “V” shaped trend of first decreasing and then increasing. When the crack inclination angle is small, the rock sample mainly undergoes shear failure and vertical splitting failure, and the number of tensile and shear cracks mainly increases in a stepped pattern. The larger the crack inclination angle, the more the rock failure mode will transition to a mixture of vertical splitting and shear failure, and the curve of the number of tensile and shear cracks will increase exponentially. As the crack inclination angle increases, the total input energy and elastic strain energy of the rock sample show a trend of first decreasing and then increasing. The larger the crack inclination angle, the faster the increase in dissipated energy, but the lower the final dissipated energy when the rock sample fails. The existence of cracks significantly weakens the energy storage limit, weakens the ability of the rock to absorb and store elastic strain energy, and enhances its energy dissipation ability at peak stress in the rock specimen during compressive failure.
-
It is very important for mining and civil construction to predict the morphology distribution of cracks induced by blasting. Hence, many researchers have paid their attention to dynamic fracture behavior of rocks due to drilling and blasting operations[1-3]. A number of experiments and numerical simulations have been conducted to investigate the blasting-induced fractures in the near borehole zone as well as in the far field[4-5]. In order to gain high fidelity in simulating the complex responses of rocks subjected to blasting loading, a realistic constitutive model is required. In the last 20 years, various macro-scale material models have been proposed, from relatively simple ones to more sophisticated, and their capabilities in describing actual nonlinear behavior of material under different loading conditions have been evaluated[6].
During blasting operation, chemical reactions of explosive in borehole occur rapidly, and instantaneously a shock/stress wave applies to borehole wall. Initially, a crushed zone around the borehole is developed by the shock/stress wave. Then, a radial shock/stress wave propagates away from borehole, and its magnitude decreases. Once the radial shock/stress drops below the local dynamic compressive strength, no shear damage occurs. At the same time, a tensile tangential stress with enough strength can be developed behind the radial compressive stress wave, which results in an extension of the existing flaws or a creation of new radial cracks. If there is a nearby free boundary, the incident compressive stress wave changes to a tensile stress wave upon reflection, and reflects back into the rock. In this case, if the dynamic tensile strength of rock is exceeded, spall cracks appear close to the free boundary.
The purpose of this paper is to conduct a numerical study on borehole blasting-induced fractures in rocks. First, a dynamic constitutive model for rocks based on the previous work of concrete[7] is briefly described, and the values of various parameters in the model for granite are estimated. The model is then employed to simulate the borehole blasting-induced fractures in granitic rocks. Comparisons between the numerical results and the experimental observations are made, and a discussion is given.
1. Dynamic Constitutive Model for Rocks
A number of models for concrete-like materials, such as TCK model[8], HJC model[9], RHT model[10], K&C model[11], have been developed. The sophisticated numerical models are increasingly used as they are capable of describing the material behavior under high strain rate loading. However, these models have been found to have some serious flaws, and cannot predict the experimentally observed crack patterns or exhibit improper behavior under certain loading conditions[7, 12-15].
In the following, a dynamic constitutive model for rocks is briefly described according to equation of state (EOS) and strength model, based on the previous work on concrete[7].
1.1 EOS
A typical form of EOS is the so-called p-
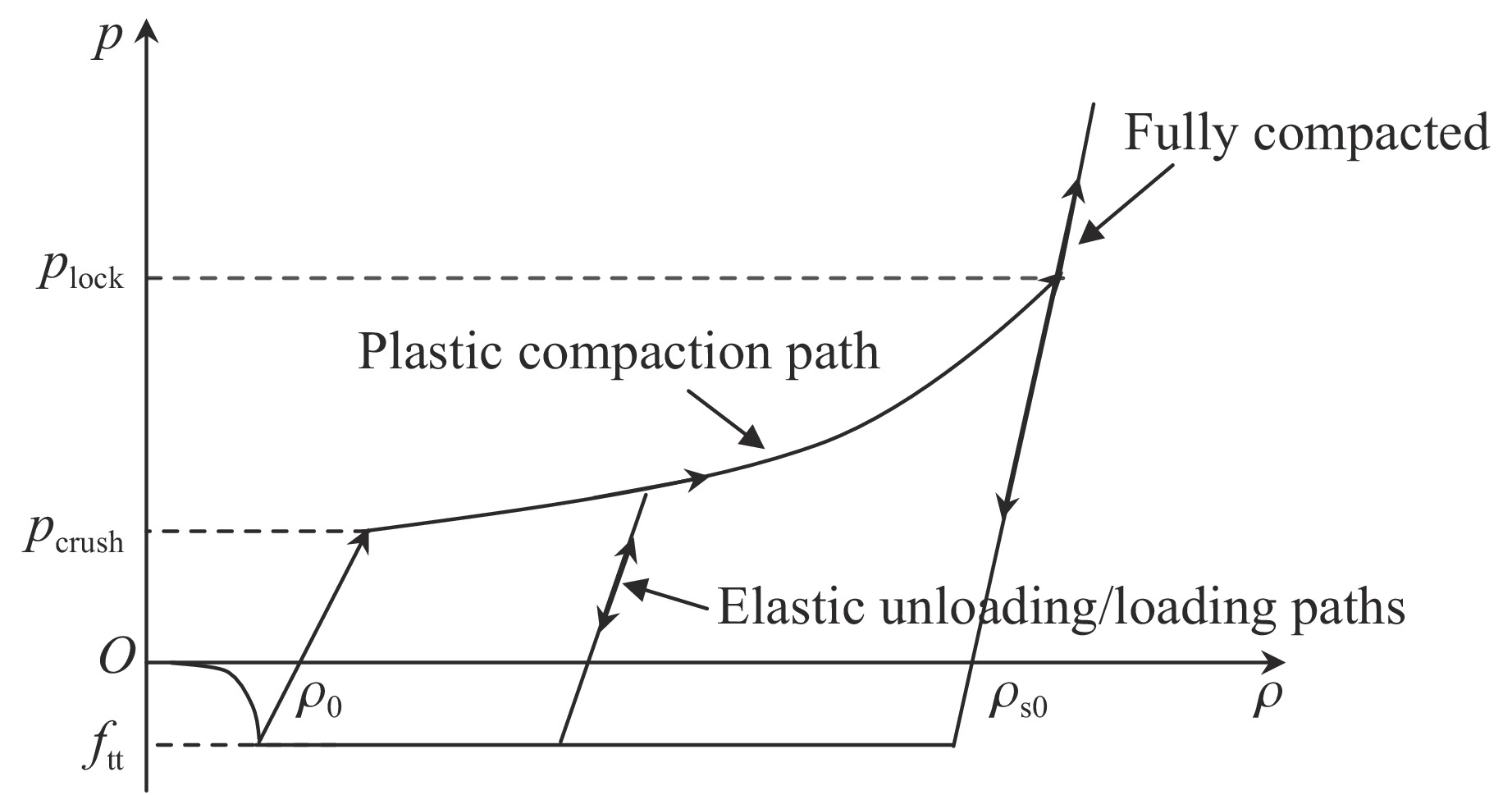
α relation, which is proved to be capable of representing brittle material’s response behavior at high pressures, and it allows for a reasonably detailed description of the compaction behavior at low pressure ranges as well, as shown schematically in Fig.1.pcrush corresponds to the pore collapse pressure beyond which plastic compaction occurs, andplock is the pressure when porosityα reaches 1,ftt is the tensile strength,ρ0 is the initial density,ρs0 refers to the density of the initial solid. Figure 1. Schematic diagram of EOS[7]
Figure 1. Schematic diagram of EOS[7]The EOS for compression (p≥0) is given by
p=K1ˉμ+K2ˉμ2+K3ˉμ3 (1) where p denotes pressure, K1, K2, K3 are constants, and
ˉμ is defined byˉμ=ραρ0α0−1=αα0(1+μ)−1 (2) where
ρ is the current density,μ=ρ/ρ0−1 specifies the volumetric strain,α0 =ρ s0/ρ 0 andα=ρs/ρ represent the initial porosity and the current porosity, respectively.ρs refers to the density of fully compacted solid. Physically,α is a function of the hydro-static pressure p, and is expressed asα=1+(α0−1)(plock−pplock−pcrush)n (3) where n is the compaction exponent.
When material withstands hydro-static tension, the EOS for tension (p<0) is given by
p=K1ˉμ (4) α=α0(1+p/K1)/(1+μ) (5) 1.2 Strength Model
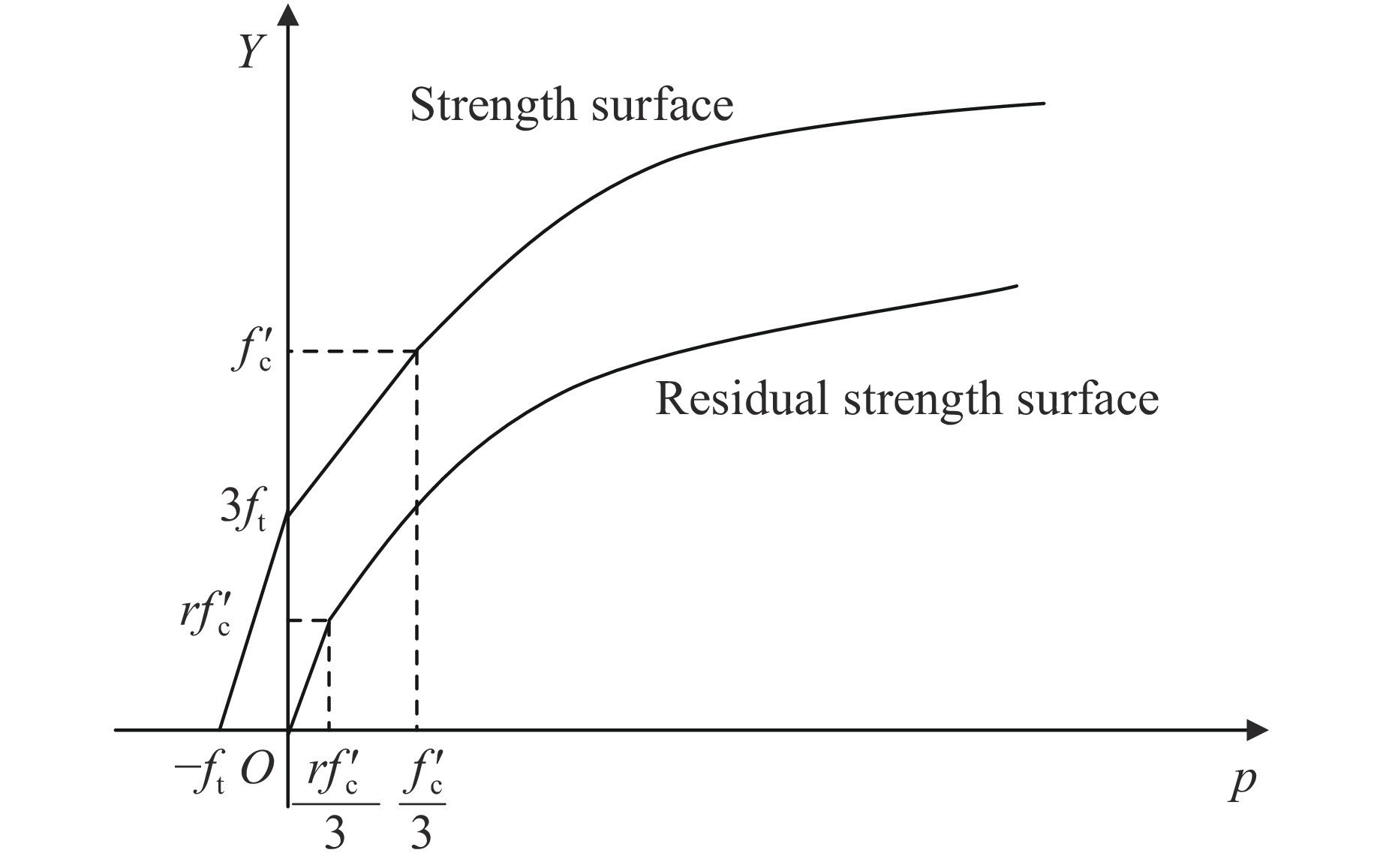
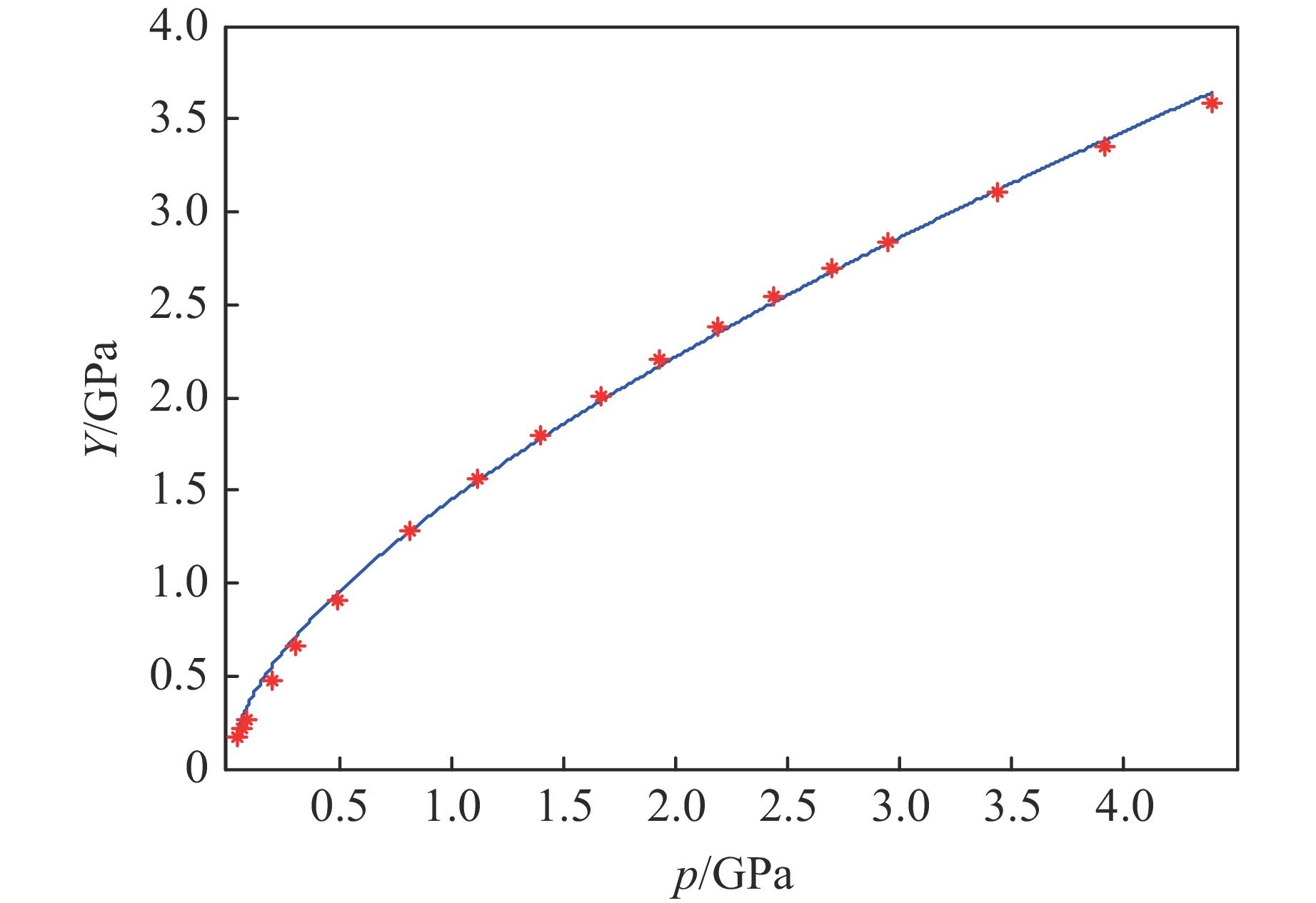
The strength model takes into account various effects, such as pressure hardening, damage softening, third stress invariant (Lode angle) and strain rate. The strength surface Y, shown schematically in Fig.2, can be written as[7]
Y={3(p+ftt)R(θ,e) p<0[3ftt + 3p(fcc−3ftt)/fcc]R(θ,e) 0⩽p⩽fcc/3{fcc+Bfc′[p/fc′−fcc/(3fc′)]N}R(θ,e) p>fcc/3 (6) where p is the hydro-static pressure, parameters B and N are constants, R(θ,e) is a function of the Lode angle θ and the tensile-to-compressive meridian ratio e,
fc′ is the static uni-axial compressive strength, the compressive strengthfcc and the tensile strengthftt are defined byfcc=fc′Dm_tηc (7) ftt=ftDtηt (8) where
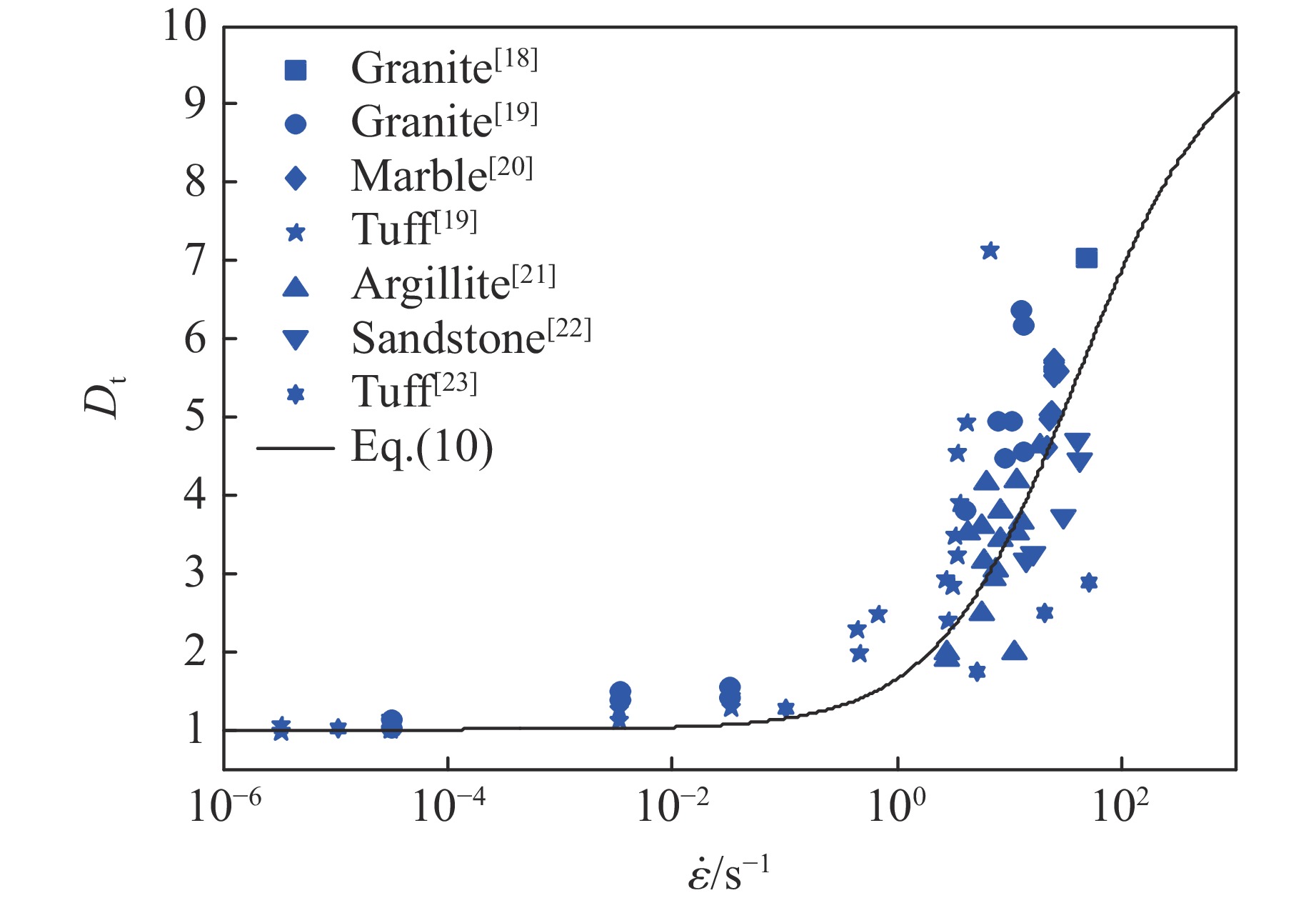
ft is the static uni-axial tensile strength.Dm_t is the compression dynamic increase factor due to strain rate effect only, and can be expressed as[7, 16]Dm_t=(Dt−1)ft/fc′+1 (9) where
Dt is the tension dynamic increase factor determined byDt={tanh[(lg˙ε˙ε0−Wx)S][FmWy−1]+1}Wy (10) where
Fm ,Wx ,Wy andS are experimental constants,˙ε is the strain rate, and˙ε0 is the reference strain rate, usually taken˙ε0=1.0 s−1 .ηc is the damage function for compression, which can be expressed asηc={l+(1−l)η(λ)λ⩽λmr+(1−r)η(λ)λ>λm (11) where l and r are constants[7],
λm is the value of shear damage (λ ) when strength reaches its maximum value under compression.η(λ) is defined asη(λ)=aλ(λ−1)exp(−bλ) (12) in which a and b can be determined by setting
η(λ) = 1 and∂η∂λ=0 whenλ =λ m.ηt is the damage function for tension which can be written asηt=[1+(c1εtεfrac)3]exp(−c2εtεfrac)−εtεfrac(1+c31)exp(−c2) (13) where c1 and c2 are constants[7],
εt denotes the tensile strain andεfrac is the fracture strain.The residual strength (
rfc′ ) surface for rocks, shown schematically in Fig.2, can be obtained from Eq.(6) by settingftt=0 andfcc=rfc′ , vizY={3pR(θ,e) 0<p⩽rfc′/3{rfc′+Bfc′[p/fc′−rfc′/(3fc′)]N}R(θ,e) p>rfc′/3 (14) 2. Numerical Simulations
Granite is selected for investigating the dynamic fractures which result from borehole blast loading.
2.1 Evaluation of Various Parameters in the Model
Table 1 lists the values of the various parameters used in the dynamic constitutive model for granite. As to how to determine the values of the various parameters in the model, more details are presented in [7, 15-17].
p-α relation ρ0/(kg∙m−3) pcrush/MPa plock/GPa n K1/GPa K2/TPa K3/TPa 2660 50.5 3 3 25.7 −3 150 Strength surface Strain rate effect fc′/MPa ft/MPa B N G/GPa Fm Wx 161.5 7.3 2.59 0.66 21.9 10 1.6 Strain rate effect Shear damage Wy S ˙ε0/s−1 λs λm l r 5.5 0.8 1.0 4.6 0.3 0.45 0.3 Lode effect Tensile damage e1 e2 e3 c1 c2 εfrac 0.65 0.01 5 3 6.93 0.007 Fig.3 shows the comparison of the strength surface between Eq.(6) (with B=2.59, N=0.66) and the triaxial test data for granite[17]. It can be seen from Fig.3 that a good agreement is obtained. Similarly, Fig.4 shows the tensile strengths/dynamic increase factor obtained by Eq.(10) and the test results of various rocks at different strain rates[18-23]. It is clear from Fig.4 that a good agreement is achieved.
 Figure 3. Comparison of the strength surface between Eq.(6) (with B=2.59, N=0.66) and the triaxial test data for granite[17]
Figure 3. Comparison of the strength surface between Eq.(6) (with B=2.59, N=0.66) and the triaxial test data for granite[17]2.2 Numerical Results
In the following, numerical simulations are carried out for the response of the granite targets subjected to borehole blasting loading. The dynamic fracture behavior of two kinds of granite samples are studied, namely, cylindrical sample as reported in the literature and square sample as examined in our own laboratory.
2.2.1 Cylindrical Rock Sample
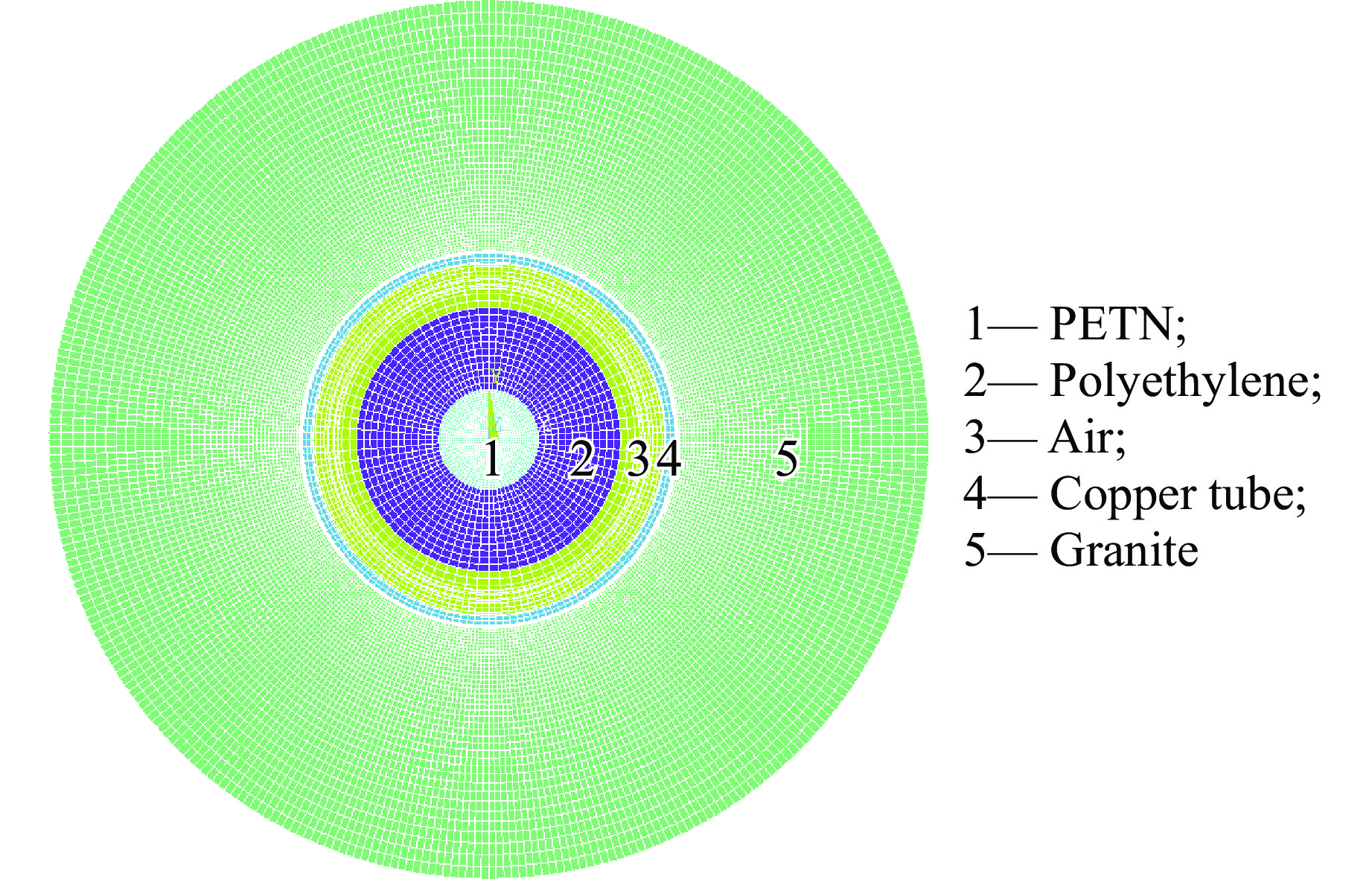
In consideration of the sizes of the cylindrical granite samples prepared for laboratory-scale blasting experiments by Dehghan Banadaki and Mohanty[17] (with a diameter of 144 mm, a height of 150 mm and a borehole diameter of 6.45 mm), a circular plane strain model with an outer diameter of 144 mm is made in our simulation, as shown in Fig.5, being a scaled close-up view of the borehole region. Multi-material Euler solver is used for modeling PETN explosive, polyethylene and air. Lagrangian descriptions are used for modeling the copper tube and granite.
The material model and the properties of PETN explosive, polyethylene, air and copper tube used in the simulation are given in Ref.[17]. The values of various parameters in the constitutive model for granite are listed in Table 1.
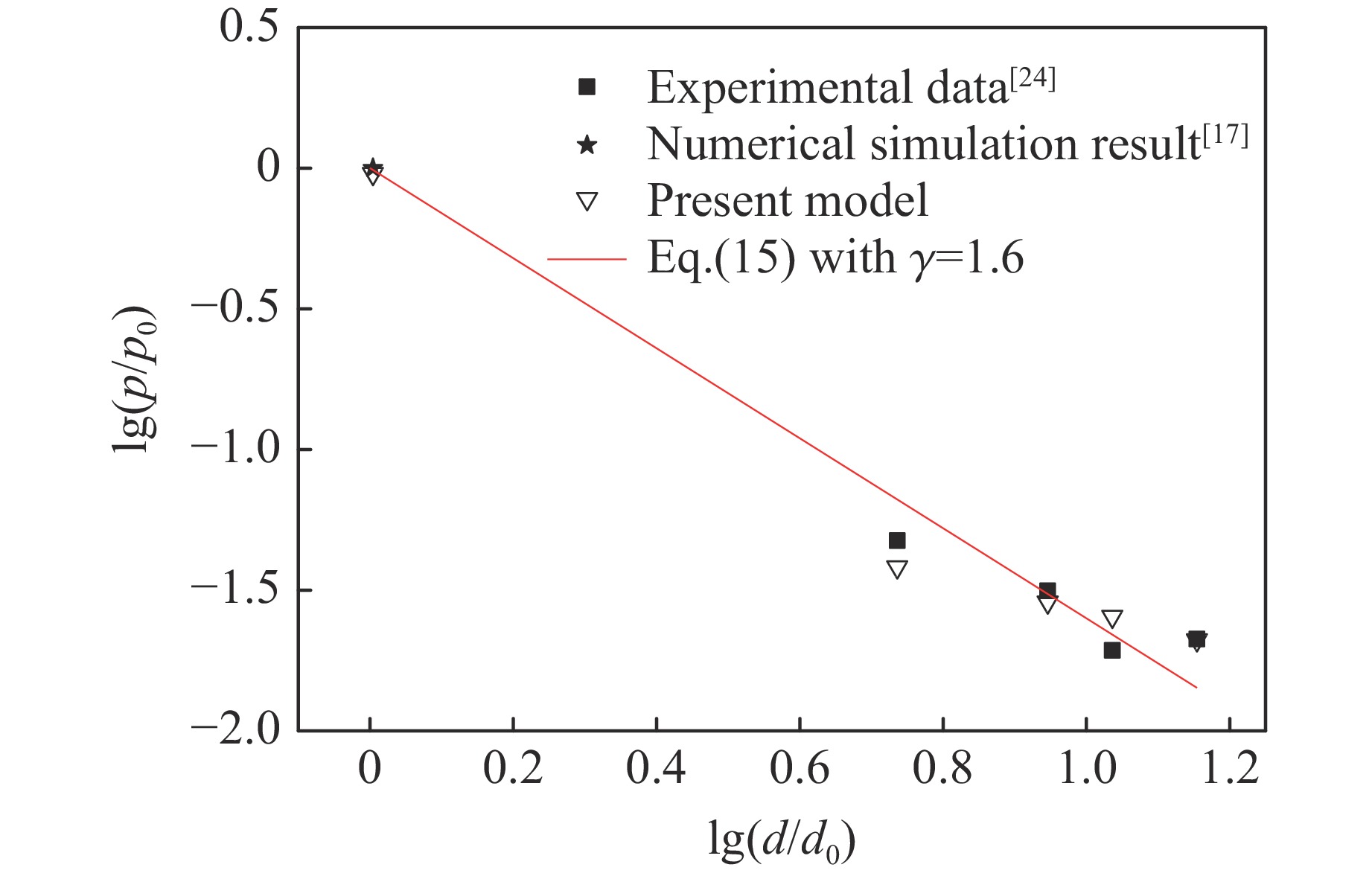
Fig.6 shows the comparison of the peak pressures between our simulation results of the present model, the numerical results[17], and the experimental results by Dehghan Banadaki and Mohanty[24]. It can be seen from Fig.6 that a good agreement is obtained.
In order to characterize the damping behavior of stress in granite, the peak pressure p in granite is expressed in an exponential form as
pp0=(dd0)−γ (15) where p0 is the peak pressure on the borehole wall, d0 is the initial radius of the borehole, d is the distance from the center point of the borehole,
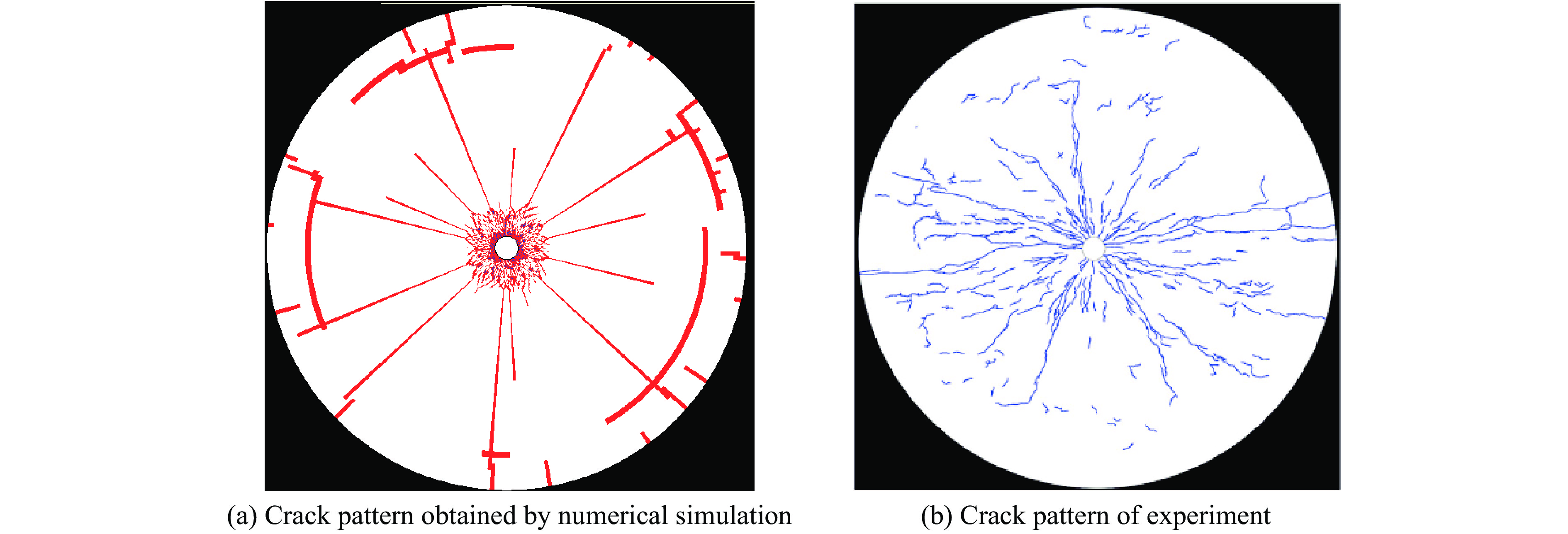
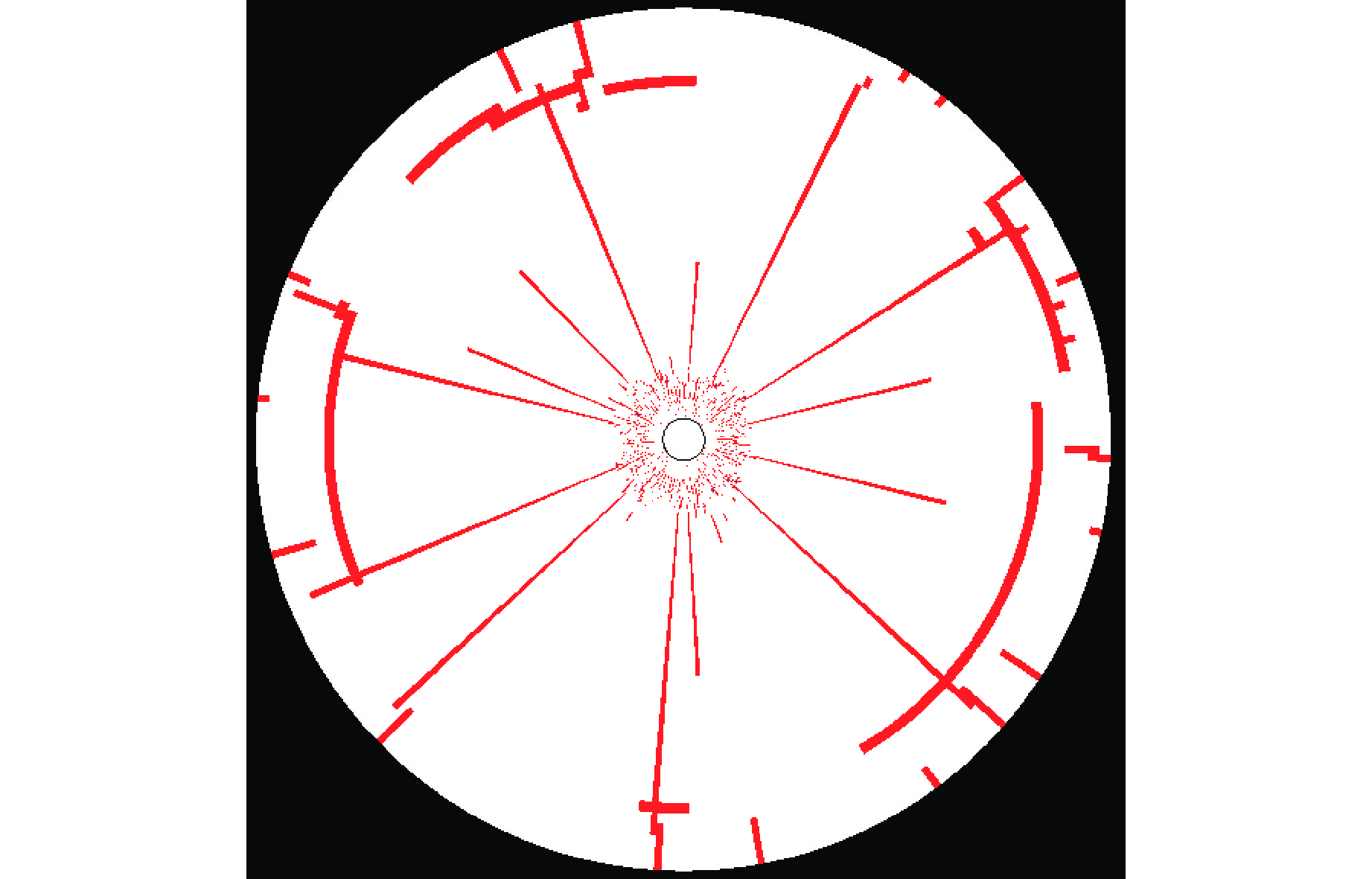
γ is an index. It is evident from Fig.6 that Eq.(15) withγ =1.6 correlates well with the experimental results.Fig.7 shows the comparison between the crack patterns predicted numerically based on the present model and the one observed experimentally in the cylindrical granite sample[17]. It is clear that a relatively good agreement on the crack pattern is obtained. It is also clear that the stress waves produce three distinct crack regions in the cylindrical granite sample: densely populated smaller cracks around the borehole, a few large radial cracks propagating towards the outer boundary, and circumferential cracks close to the sample boundary which are due to the reflected tension stress.
 Figure 7. Comparison of the crack patterns between the numerical prediction and the experiment of the cylindrical granite sample[17]
Figure 7. Comparison of the crack patterns between the numerical prediction and the experiment of the cylindrical granite sample[17]In order to make an assessment of the contributions of both the compression/shear stress and the tensile stress to the crack patterns, Fig.8 shows the numerically predicted crack pattern which results from the tension stress only. Fom Fig.8 and Fig.7(a), it is apparent that there are virtually few changes in crack patterns, both having the large radial and circumferential cracks caused by the same tensile stress, however, the smaller cracks around the borehole in Fig.8 are much less than those in Fig.7(a). In another word, crack patterns are mainly caused by tensile stress, and smaller cracks around borehole are created largely by compression/shear stress.
2.2.2 Square Granite Sample
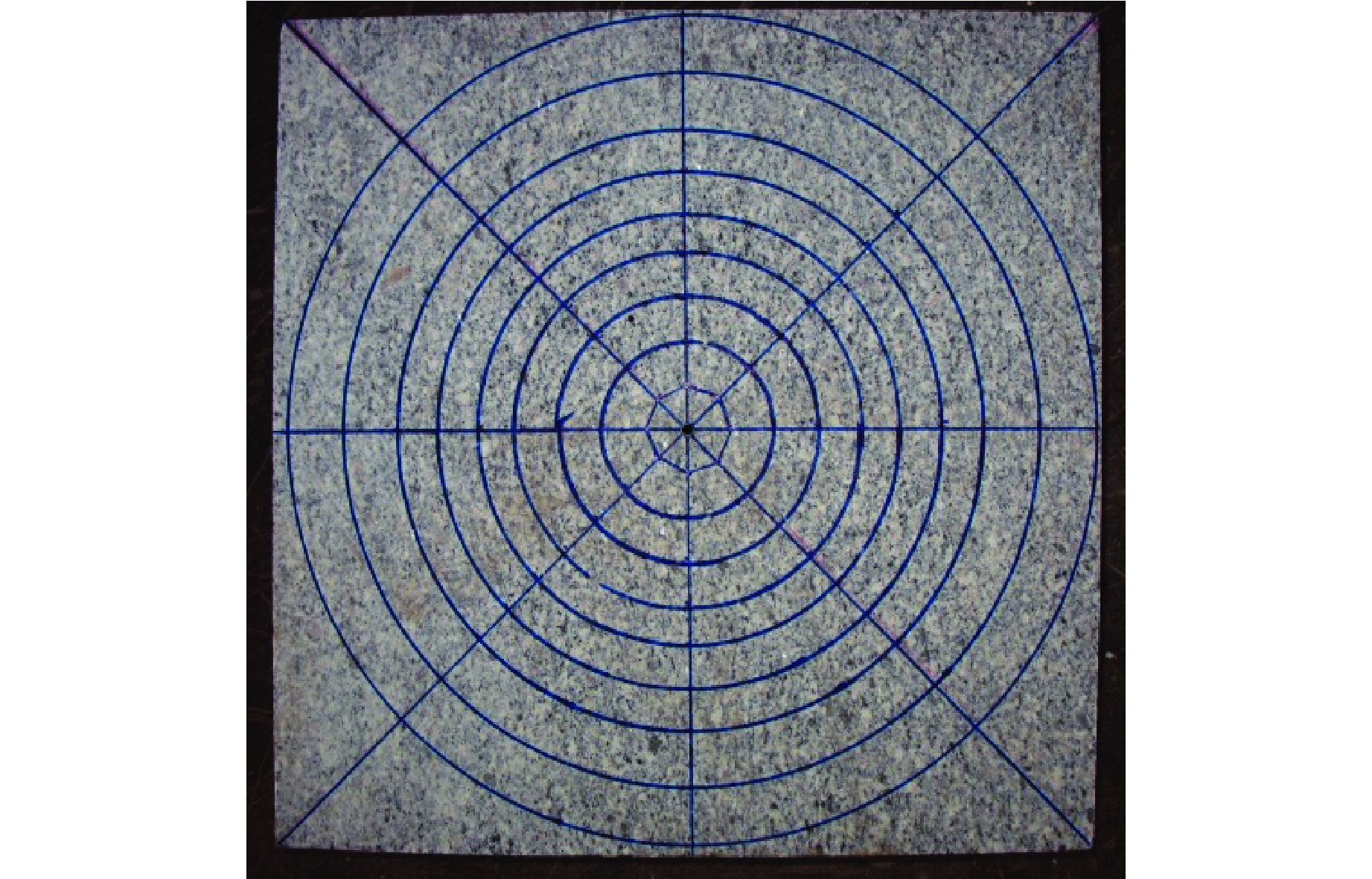
The laboratory-scale single-hole blasting tests are also carried out in order to validate further the accuracy and the reliability of the present model. Two square granite samples with a side length of 400 mm and a height of 100 mm are employed in the experiments. The borehole diameter is 4 mm and a series of concentric rings is drawn on the top surface of the samples (see Fig.9), so that the damage regions induced by detonation can be assessed visually.
A cylindrical RDX explosive enclosed by an aluminum sheath (see Fig.10) is tightly installed in the borehole of the No.1 sample, while an unwrapped RDX explosive is inserted into the borehole of the No.2 sample. The density of the RDX is 1700 kg/m3, and the material model and properties of the RDX explosive and the aluminum sheath used in the experiments are given in ref.[25]. The values of the various parameters in the constitutive model for granite are listed in Table 1.
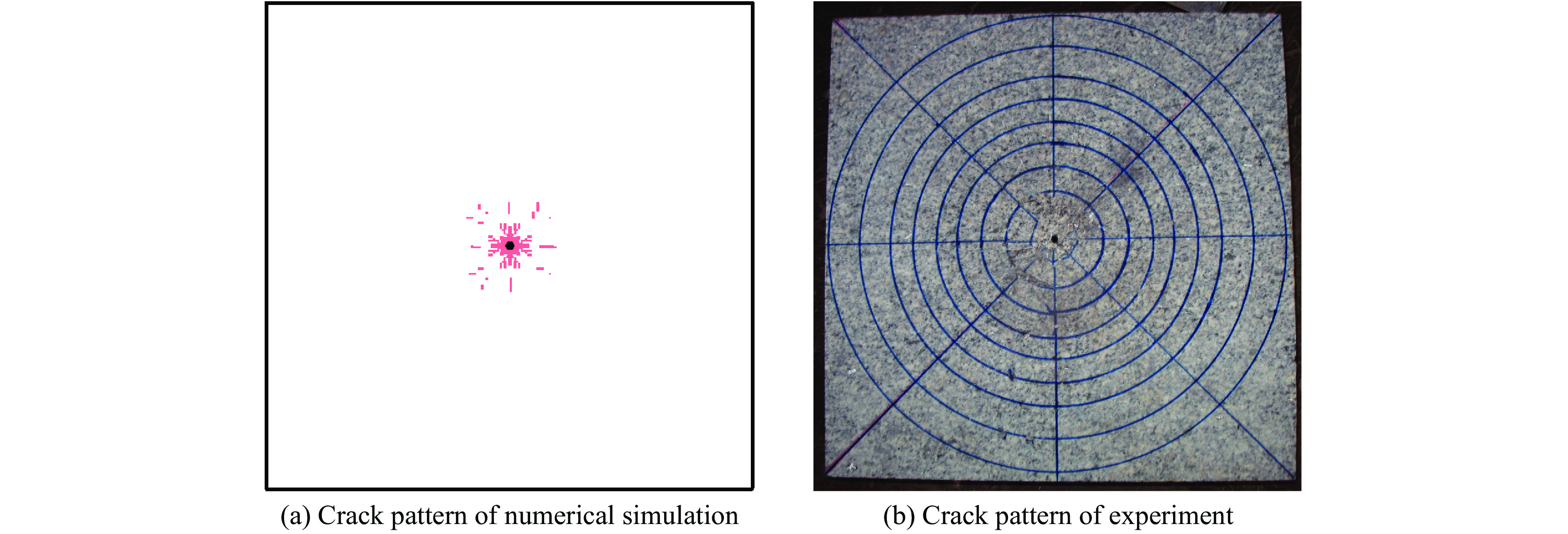
Fig.11 and Fig.12 show the comparisons of the crack patterns between the numerical predictions from the present model and the ones observed experimentally in the square granite samples. It can be seen from Fig.11 and Fig.12 that good agreements are obtained. It should be mentioned here that No.1 sample receives less damage due to less RDX explosive used in the test, and that No.2 sample is broken up into four major pieces due to more RDX explosive employed in the experiment. Severe damages and small cracks are induced in the vicinity of the boreholes of both samples, as can be seen clearly from Fig.11(b) and Fig.12(b).
3. Conclusions
A numerical study on the borehole blasting-induced fractures in rocks is conducted in this paper, using a dynamic constitutive model developed previously for concrete. Two kinds of granite rocks are simulated numerically, one in the cylindrical form and the other in the square form. The numerical results are compared with the corresponding experiments. Main conclusions can be drawn as follows.
(1) The crack patterns predicted numerically from the present model are found to be in good agreement with the experimental observations, both in cylindrical and square granite samples subjected to borehole blasting loading.
(2) The peak pressures predicted numerically based on the present model are found to be in good agreement with the test data.
(3) Crack pattern observed experimentally in the rock sample is mainly caused by the tensile stress, while the smaller cracks in the vicinity of the borehole are created largely by compression/shear stress.
(4) The consistency between the numerical results and the experimental observations demonstrates the accuracy and reliability of the present model. Thus the model can be used in the numerical simulations of the response and the failure of rocks under blasting loading.
-
表 1 岩石的宏观力学参数
Table 1. Macromechanical parameters of rocks
Material Rock density/
(g·cm−3)Compressive
strength/MPaTensile
strength/MPaElastic
modulus/GPaPoisson’s
ratioCohesive
force/MPaFriction
angle/(°)Rock materials[15] 2.63 155.1 11.06 37.63 0.21 Structural plane 0.5 70 表 2 岩石的细观参数
Table 2. Mesoscopic parameters of rocks
Effective modulus
of parallel
bonding/GPaStiffness
ratioLinear contact
effective
modulus/GPaTangential bonding
strength/MPaNormal bonding
strength/MPaFriction
angle/(°)Frictional
coefficient98.6 1.5 29.2 28 7.6 70 0.5 -
[1] 佘诗刚, 林鹏. 中国岩石工程若干进展与挑战 [J]. 岩石力学与工程学报, 2014, 33(3): 433–457. doi: 10.13722/j.cnki.jrme.2014.03.001SHE S G, LIN P. Some developments and challenging issues in rock engineering field in China [J]. Chinese Journal of Rock Mechanics and Engineering, 2014, 33(3): 433–457. doi: 10.13722/j.cnki.jrme.2014.03.001 [2] LI Y P, CHEN L Z, WANG Y H. Experimental research on pre-cracked marble under compression [J]. International Journal of Solids and Structures, 2005, 42(9/10): 2505–2516. doi: 10.1016/j.ijsolstr.2004.09.033 [3] WANG S Y, SLOAN S W, SHENG D C, et al. Numerical study of failure behavior of pre-cracked rock specimens under conventional triaxial compression [J]. International Journal of Solids and Structures, 2014, 51(5): 1132–1148. doi: 10.1016/j.ijsolstr.2013.12.012 [4] 陈卫忠, 杨建平, 邹喜德, 等. 裂隙岩体宏观力学参数研究 [J]. 岩石力学与工程学报, 2008, 27(8): 1569–1575.CHEN W Z, YANG J P, ZOU X D, et al. Research on micromechanical parameters of fractured rock mass [J]. Chinese Journal of Rock Mechanics and Engineering, 2008, 27(8): 1569–1575. [5] 易婷, 唐建新, 王艳磊. 裂隙倾角及数目对岩体强度和破坏模式的影响 [J]. 地下空间与工程学报, 2021, 17(1): 98–106, 134.YI T, TANG J X, WANG Y L. The influence of the inclination and number of cracks on the strength and failure mode of rock mass [J]. Chinese Journal of Underground Space and Engineering, 2021, 17(1): 98–106, 134. [6] 朱泽奇, 肖培伟, 盛谦, 等. 基于数字图像处理的非均质岩石材料破坏过程模拟 [J]. 岩土力学, 2011, 32(12): 3780–3786. doi: 10.3969/j.issn.1000-7598.2011.12.040ZHU Z Q, XIAO P W, SHENG Q, et al. Numerical simulation of fracture propagation of heterogeneous rock material based on digital image processing [J]. Rock and Soil Mechanics, 2011, 32(12): 3780–3786. doi: 10.3969/j.issn.1000-7598.2011.12.040 [7] 张仕林, 杜贻腾, 李延春, 等. 单轴压缩荷载下红砂岩不同张开度三维通透裂隙扩展机理研究 [J]. 西安科技大学学报, 2016, 36(4): 548–553. doi: 10.13800/j.cnki.xakjdxxb.2016.0415ZHANG S L, DU Y T, LI Y C, et al. Propagation mechanism of 3D through fracture with under uniaxial load [J]. Journal of Xi’an University of Science and Technology, 2016, 36(4): 548–553. doi: 10.13800/j.cnki.xakjdxxb.2016.0415 [8] 唐春安, 刘红元, 秦四清, 等. 非均质性对岩石介质中裂纹扩展模式的影响 [J]. 地球物理学报, 2000, 43(1): 116–121.TANG C A, LIU H Y, QIN S Q, et al. Influence of heterogeneity on crack propagation modes in brittle rock [J]. Chinese Journal of Geophysics, 2000, 43(1): 116–121. [9] 谢和平, 鞠杨, 黎立云. 基于能量耗散与释放原理的岩石强度与整体破坏准则 [J]. 岩石力学与工程学报, 2005, 24(17): 3003–3010. doi: 10.3321/j.issn:1000-6915.2005.17.001XIE H P, JU Y, LI L Y. Rock strength and global failure criteria based on the principle of energy dissipation and release [J]. Chinese Journal of Rock Mechanics and Engineering, 2005, 24(17): 3003–3010. doi: 10.3321/j.issn:1000-6915.2005.17.001 [10] 谢和平, 鞠杨, 黎立云, 等. 岩体变形破坏过程的能量机制 [J]. 岩石力学与工程学报, 2008, 27(9): 1729–1740.XIE H P, JU Y, LI L Y, et al. Energy mechanism of rock deformation and failure process [J]. Chinese Journal of Rock Mechanics and Engineering, 2008, 27(9): 1729–1740. [11] 武晋文, 赵阳升, 万志军, 等. 高温均匀压力花岗岩热破裂声发射特性实验研究 [J]. 煤炭学报, 2012, 37(7): 1111–1117. doi: 10.13225/j.cnki.jccs.2012.07.016WU J W, ZHAO Y S, WAN Z J, et al. Experimental study of acoustic emission of granite due to thermal cracking under high temperature and isostatic stress [J]. Journal of China Coal Society, 2012, 37(7): 1111–1117. doi: 10.13225/j.cnki.jccs.2012.07.016 [12] 孙友杰, 戚承志, 朱华挺, 等. 岩石动态断裂过程的能量分析 [J]. 地下空间与工程学报, 2020, 16(1): 43–49.SUN Y J, QI C Z, ZHU H T, et al. Energy analysis of rock dynamic fracture process [J]. Chinese Journal of Underground Space and Engineering, 2020, 16(1): 43–49. [13] POTYONDY D O, CUNDALL P A. A bonded-particle model for rock [J]. International Journal of Rock Mechanics & Mining Sciences, 2004, 41(8): 1329–1364. [14] 耿萍, 卢志楷, 丁梯. 基于颗粒流的围岩注浆动态过程模拟研究 [J]. 铁道工程学报, 2017, 34(3): 34–40. doi: 10.3969/j.issn.1006-2106.2017.03.007GENG P, LU Z K, DING T. Research on the dynamic process simulation of rock grouting based on particle Flow [J]. Journal of Railway Engineering Society, 2017, 34(3): 34–40. doi: 10.3969/j.issn.1006-2106.2017.03.007 [15] 王春萍, 王璐, 刘建锋, 等. 单轴压缩条件下单裂隙花岗岩力学特性及破坏特征 [J/OL]. 西南交通大学学报 (2023-07-10)[2024-01-18]. http://kns.cnki.net/kcms/detail/51.1277.u.20230706.1836.007.html.WANG C P, WANG L, LIU J F, et al. Investigations on the mechanical behavior and failure mechanism of single fractured granite under uniaxial compression [J/OL]. Journal of Southwest Jiaotong University (2023-07-10)[2024-01-18]. http://kns.cnki.net/kcms/detail/51.1277.u.20230706.1836.007.html. [16] 郭奇峰, 武旭, 蔡美峰, 等. 预制裂隙花岗岩的强度特征与破坏模式试验 [J]. 工程科学学报, 2019, 41(1): 43–52.GUO Q F, WU X, CAI M F, et al. Experiment on the strength characteristics and failure modes of granite with pre-existing cracks [J]. Chinese Journal of Engineering, 2019, 41(1): 43−52. [17] 郭奇峰, 武旭, 蔡美峰, 等. 预制裂隙花岗岩的裂纹起裂机理试验研究 [J]. 煤炭学报, 2019, 44(Suppl 2): 476–483. doi: 10.13225/j.cnki.jccs.2019.1212GUO Q F, WU X, CAI M F, et al. Crack initiation mechanism of pre-existing cracked granite [J]. Journal of China Coal Society, 2019, 44(Suppl 2): 476–483. doi: 10.13225/j.cnki.jccs.2019.1212 [18] 蒋明镜, 张宁, 申志福, 等. 含裂隙岩体单轴压缩裂纹扩展机制离散元分析 [J]. 岩土力学, 2015, 36(11): 3293–3300. doi: 10.16285/j.rsm.2015.11.034JIANG M J, ZHANG N, SHEN Z F, et al. DEM analyses of crack propagation in flawed rock mass under uniaxial compression [J]. Rock and Soil Mechanics, 2015, 36(11): 3293–3300. doi: 10.16285/j.rsm.2015.11.034 [19] BROBERG K B. Cracks and fracture [M]. New York, NY: Academic Press, 1999. [20] WANG Q S, CHEN J X, GUO J Q, et al. Acoustic emission characteristics and energy mechanism in karst limestone failure under uniaxial and triaxle compression [J]. Bulletin of Engineering Geology & the Environment, 2019, 78: 1427–1442. [21] NING J, WANG J, JIANG J Q, et al. Estimation of crack initiation and propagation thresholds of confined brittle coal specimens based on energy dissipation theory [J]. Rock Mechanics & Rock Engineering. 2018, 51: 119–134. [22] 黄达, 黄润秋, 张永兴. 粗晶大理岩单轴压缩力学特性的静态加载速率效应及能量机制试验研究 [J]. 岩石力学与工程学报, 2012, 31(2): 245–255. doi: 10.3969/j.issn.1000-6915.2012.02.003HUANG D, HUANG R Q, ZHANG Y X. Experimental investigations on static loading rate effects on mechanical properties and energy mechanism of coarse crystal grain marble under uniaxial compression [J]. Chinese Journal of Rock Mechanics and Engineering, 2012, 31(2): 245–255. doi: 10.3969/j.issn.1000-6915.2012.02.003 -







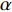


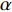
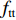
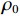
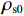
 DownLoad:
DownLoad:


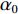
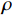
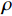
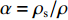
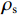
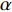
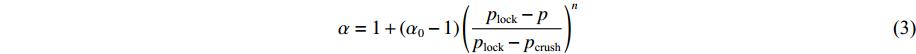
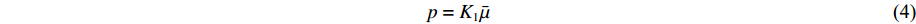
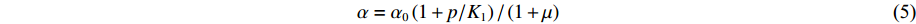

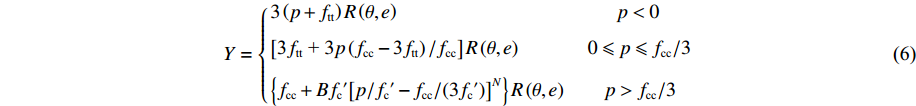


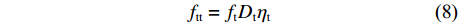
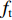
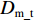
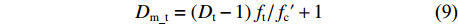
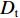
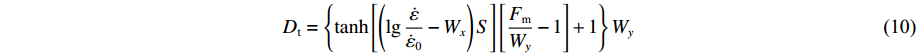
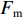
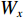
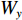

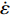
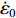
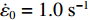
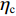
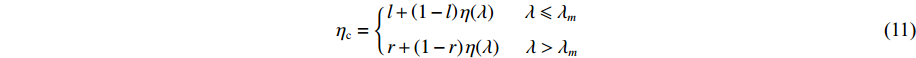
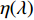
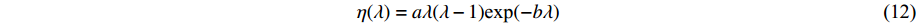
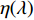
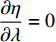
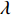
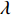
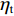
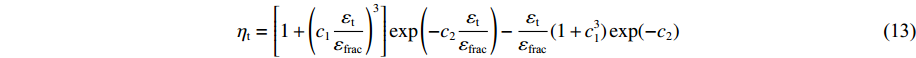
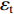
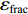
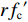
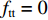
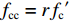
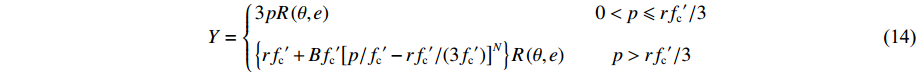
 DownLoad:
DownLoad:



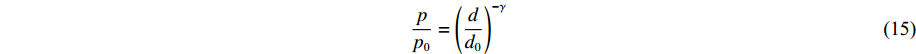
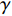







 下载:
下载: