Influence of Penetration Deflection by the Shape of Tail Oblique Cone of Projectile
-
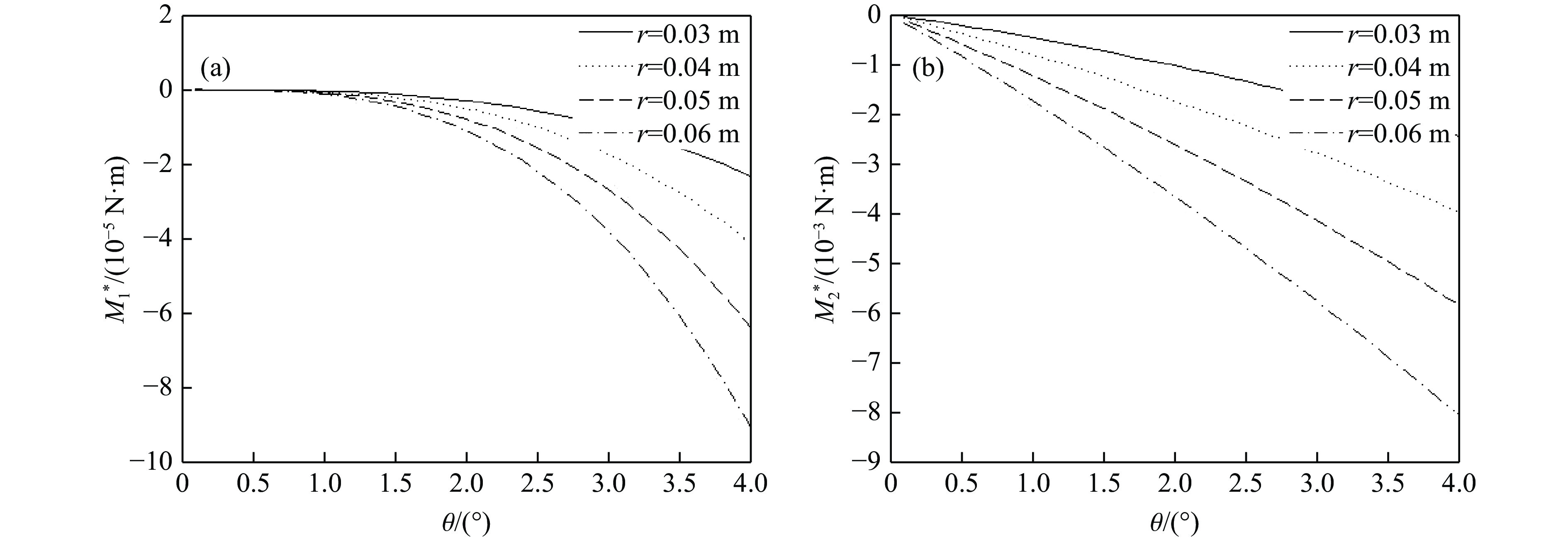
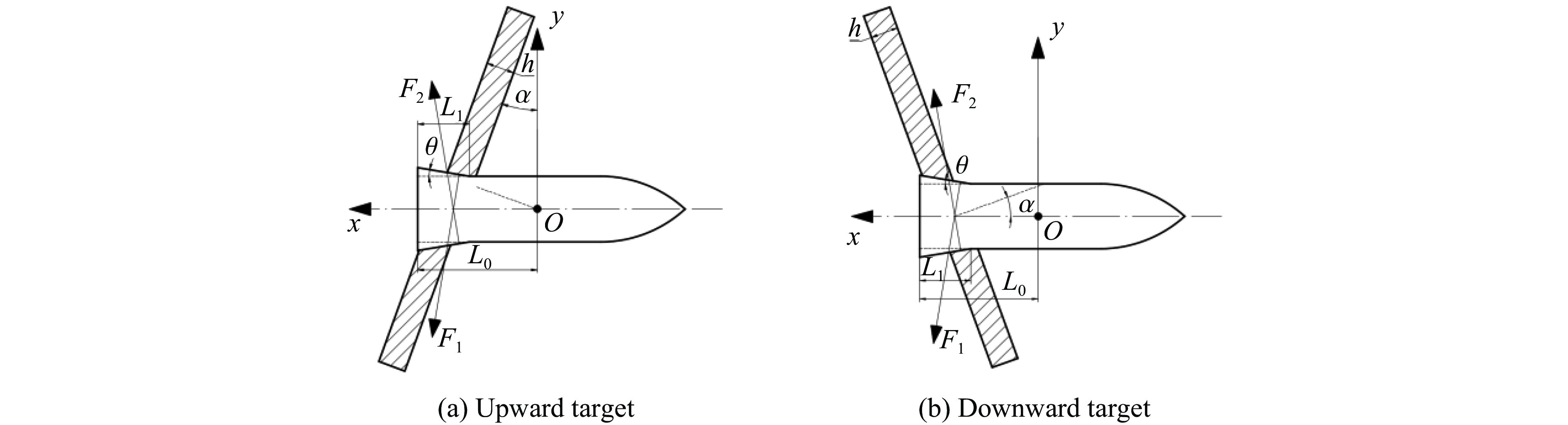
摘要: 针对弹体斜侵彻多层靶时弹道发生的偏转问题,建立了弹体尾部为斜锥面结构的弹体侵彻偏转理论计算模型,获得了当速度为0.2~1.2 km/s、着角为−30°~20°、弹体半径为30~60 mm、尾飘斜面与弹轴的夹角为0°~4°时偏转随尾部结构的变化规律,通过与试验结果进行对比,验证了模型的准确性。结果表明:弹体侵彻仰靶时,弹尾形成负的偏转力矩,弹体产生“低头”效果;弹体侵彻俯靶时,弹尾形成正的偏转力矩,弹体产生“抬头”效果;弹体尾部斜锥面产生的垂直弹轴的偏转力矩约为平行弹轴的偏转力矩的100倍;增大尾飘斜面与弹轴的夹角、尾飘长度、弹体半径均可增大偏转力矩;与增大尾飘长度相比,增大尾飘斜面与弹轴的夹角对增大偏转力矩更有效。Abstract: Aiming at the problem of deflection when the projectile obliquely penetrates a multi-layer target, a theoretical calculation model of the projectile deflection caused by the oblique cone structure of the projectile tail is established, and the change rule of the deflection with the tail structure is obtained at the working conditions of the velocity of 0.2−1.2 km/s, the angle of attraction of −30°−20°, the radius of the projectile body of 30–60 mm, and the angle between bevel plane of tail and the projectile shaft of 0°−4°. Moreover, the accuracy of the model is verified by comparing with the test results. The results showed that the tail of the projectile forms a negative deflection moment and the projectile produces a “head down” effect when the tail of the projectile penetrated upward target, and the tail of the projectile forms a positive deflection moment and the projectile produces a “head up” effect when the tail of the projectile penetrated downward target; the deflection moment of vertical axis is about 100 times greater than that of parallel axis. The deflecting moment can be increased by increasing the angle between bevel plane of tail and the projectile shaft, the length of the tail, and the radius of the projectile. Increasing the angle between bevel plane of tail and the projectile shaft is more effective in increasing the deflection moment than increasing the tail length.
-
Key words:
- multi-layer target /
- penetration /
- deflection moment /
- tail shape /
- oblique cone structure
-
硝酸肼(hydrazinium nitrate,HN,分子式为N2H4·HNO3)是一种典型的含能材料,不易挥发、无毒、无腐蚀性,且易于合成,便于使用,在军事和工业领域被广泛应用。美国根据HN的性质,将其作为氧化剂,开发了Astrolite系列液体及塑胶炸药[1]。近年来,HN作为潜在推进剂组元受到了越来越多的关注[2–4]。此外,在乏燃料后处理的普雷克斯(PUREX)萃取工艺中,水相HN作为稳定剂还可用于催化还原钚和铀,以达到提纯和浓缩的目的[5]。在上述应用中,HN通常在高压环境下发生反应,因此,其高压稳定性和反应机制是研究人员的关注重点。
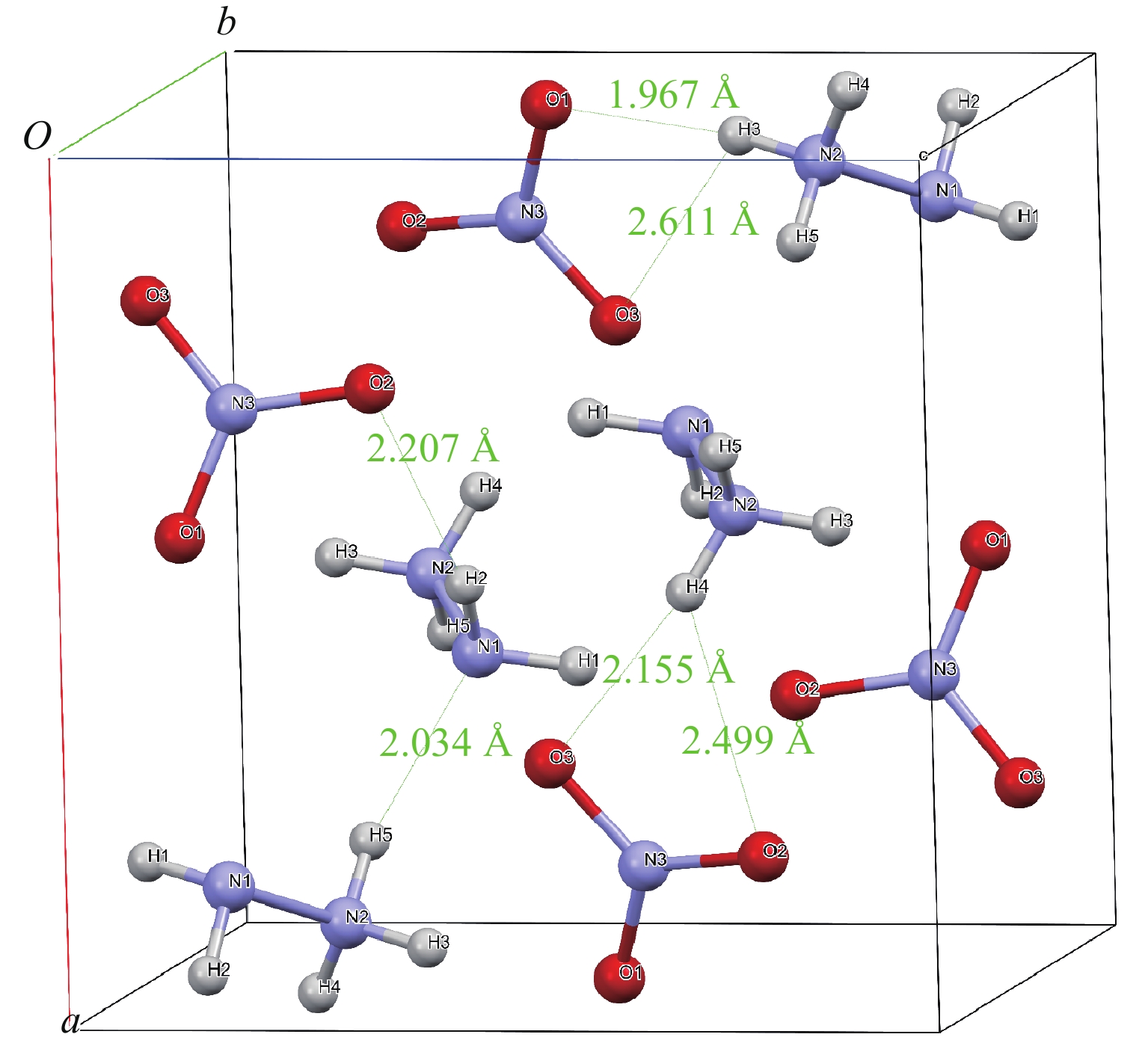
HN作为一种分子晶体,有α-HN和β-HN2种晶型[6]。其中,α-HN为常温常压稳定相[7–9],为单斜晶系,空间群为P21/n,每个晶胞中包含4个[
N2H+5 ]阳离子、4个[NO−3 ]阴离子(共44个原子),如图1所示(晶胞结构取自文献[8],可视化及其中的氢键显示通过Mercury软件[10]实现)。HN晶体内含有丰富的氢键(为了使图示简洁,图1仅显示部分氢键),如肼基上的氢原子H3与硝基上的氧原子O1形成较强的氢键(NH···O),键长为1.967 Å,肼基上的另一个氢原子H5与相邻肼基氮原子N1形成稍弱的氢键(NH···N),键长为2.034 Å[8]。这些氢键与范德瓦尔斯弱相互作用形成复杂的三维网络,将阴阳离子结合,形成稳定的分子晶体。与HN结构类似的硝酸铵(ammonium nitrate,AN,分子式为NH4·NO3)在高温高压下表现出丰富的相结构变化,不同晶相结构在较宽的温度压力区间具有不同的化学分解反应路径[11–12];另外,阴阳离子基团的非简谐效应会使结构在高压下产生负热膨胀行为。高压下这类材料的结构和性质对于含能材料的稳定性和反应机理研究具有重要意义。然而,HN作为这类含能材料的典型代表,缺乏高压下结构变化研究,使得HN在高压下的稳定性及反应机理等后续研究变得十分困难。拉曼光谱是研究含能材料高压结构和物性的常规手段,通过探测含能材料的晶格振动与分子振动,可以表征材料在高压下的结构变化[13]。然而,高压下部分含能材料(如TATB)具有较强的荧光,从而覆盖样品的拉曼信号。与拉曼光谱互补的红外吸收光谱能有效地规避该问题。但是,受光源和探测技术的限制,目前,商用红外吸收光谱仪较少用于高压下含能材料的性质研究。近年来,随着同步辐射光源及相关技术的发展,利用光源线站提供的红外光源开展含能材料研究成为可能。例如,Ciezak[14]、Pravica等[15]利用美国布鲁克海文国家实验室同步辐射光源的真空紫外环境下的红外光源,研究了TATB、DMATNT等含能材料在高压环境下的中远红外振动光谱。考虑到利用同步辐射红外光源需要专门的线站和申请机时,为了能在常规光学实验室使用涵盖太赫兹波段的宽谱带红外光谱,中国工程物理研究院流体物理研究所的Zhu等[16]利用基于飞秒激光双色场与空气等离子体相互作用产生中远红外超连续机制,搭建了可用于含能材料高压实验、涵盖30~1700 cm−1范围的红外光谱系统。
鉴于HN在军事和工业中的重要作用和应用前景,探讨肼基与硝基堆垛形成的分子间相互作用网络系统具有代表性意义,为此,本研究围绕HN的高压结构性质开展实验和理论研究。利用自主搭建的中远红外宽谱带光谱探测系统,测量HN的变高压中远红外光谱;同时,基于密度泛函理论的第一性原理方法,计算HN晶体的晶格参数和振动性质,采用相互作用区域指示(interaction region indicator,IRI)函数[17]研究HN晶体中的化学键、氢键、范德瓦尔斯作用以及空间位阻效应;最后,结合高压下中远红外振动光谱,分析HN晶体结构相变的部分规律。
1. 中远红外高压实验
1.1 HN样品制备
在低温下通过无水肼与硝酸的中和反应制备HN。具体操作步骤如下:将无水肼(1.0 mol,32 mL)加入100 mL甲醇中,冷却至−20 ℃,缓慢滴加预先冷却至0 ℃的66 mL浓硝酸溶液(质量分数68%)并不断搅拌,控制反应温度不超过0 ℃;滴加完毕后,继续搅拌1 h;当温度缓慢回升至室温后,抽滤,滤饼用甲醇重结晶,获得76 g无色片状HN晶体。熔点仪测试结果显示,样品的熔点为70 ℃,与文献[1]一致。
1.2 高压中远红外光谱系统
采用超连续中远红外光谱技术测量样品的高压中远红外光谱,该技术的细节在文献[18–19]中有详细描述。简单地说,将波长为800 nm、脉宽为100 fs的飞秒激光脉冲分成两束:一束经过倍频后与基频光在空气中聚焦形成等离子体,焦点处飞秒激光双色场与空气等离子体相互作用产生中远红外超连续光,中远红外超连续光通过由金刚石对顶砧(diamond anvil cell,DAC)加载的样品后进入光谱探测系统;另一束光先展宽成皮秒脉冲,再与中远红外激光四波混频成可见光,并由可见光光谱仪直接记录,这种方法记录的混频光可以通过频移直接获得中远红外光谱。因为空气为非线性介质,对红外激光没有吸收和色散,所以该方法可以实现红外全谱探测。
高压实验中,选用100 μm厚的不锈钢垫片作为密封垫片,将其预压至50 μm左右,利用电火花打孔机打孔,样品腔直径为300 μm,金刚石砧面直径为600 μm。通过红宝石R1荧光峰确定压力[20–21]。首先,将传压介质与粉末样品混合均匀后,与红宝石一起装入不锈钢垫片的样品腔内。对于中红外光谱探测,由于HN在中红外区间的吸收很强,为了避免其吸收饱和,需要填充传压介质,考虑到KBr在中红外没有吸收,不会干扰HN的中红外光谱,因此,选用KBr作为传压介质。对于远红外光谱探测,由于HN在远红外区的吸收远小于在中红外区的吸收,且样品粉末本身可作为传压介质,因此,无需额外填充其他传压介质。装样前,在研钵中研磨HN晶体至粉末状;对于混合装样,压制前,对KBr与HN的混合粉末研磨30 min,整个研磨过程在烤灯下完成,以保证KBr和HN不被潮解。制作混合压片时,KBr和HN的质量比为10∶1,可以得到较好的中红外吸收谱。
高压下稳态红外光谱实验分成远红外和中红外2组实验。每组实验都以1 GPa的间隔进行加压,纯HN样品组的最高压力约12 GPa,KBr和HN混合样品组的最高压力约为15 GPa。每次加压后,弛豫10 min,然后采集该压力下的红外吸收光谱。
2. 第一性原理计算
采用基于密度泛函理论(density of functional theory,DFT)的第一性原理软件CP2K[22]完成几何结构优化及振动光谱计算。初始结构采用实验测定值[8],扩胞为2×2×2(352个原子),进行分子结构和晶胞优化。利用高斯缀加平面波方法(Gaussian and augmented plane wave method,GAPW)进行全电子计算[23]:对于高斯基组,采用pob-TZVP-rev2基组[24];对于平面波,截断能取800 Ry。交换关联能采用PBE(Perdew-Burke-Ernzerhof)泛函[25]描述,色散相互作用采用Grimme等[26–27]提出的DFT-D3色散校正,采用Becke-Johnson阻尼,计算多体相互作用的高阶项。变胞优化收敛标准中,最大位移设置为1×10−4 Bohr,根均方位移设为1×10−4 Bohr,最大受力为1×10−5 Hartree/Bohr,根均方受力设为1×10−4 Hartree/Bohr。在结构优化的基础上,通过有限差分法计算Hessian矩阵,得到各振动模式的正则坐标,据此得到常压下的振动模式和振动光谱。结构优化及振动光谱的计算均在国家超级计算天津中心完成。振动模式指认及可视化通过MFakeG程序[28]将CP2K产生的molden文件转换后由VMD[29]实现。分子间相互作用的IRI函数分析则采用Multiwfn[30]计算,并由VMD完成可视化。
3. 结果与讨论
3.1 HN晶体的结构和分子间相互作用性质
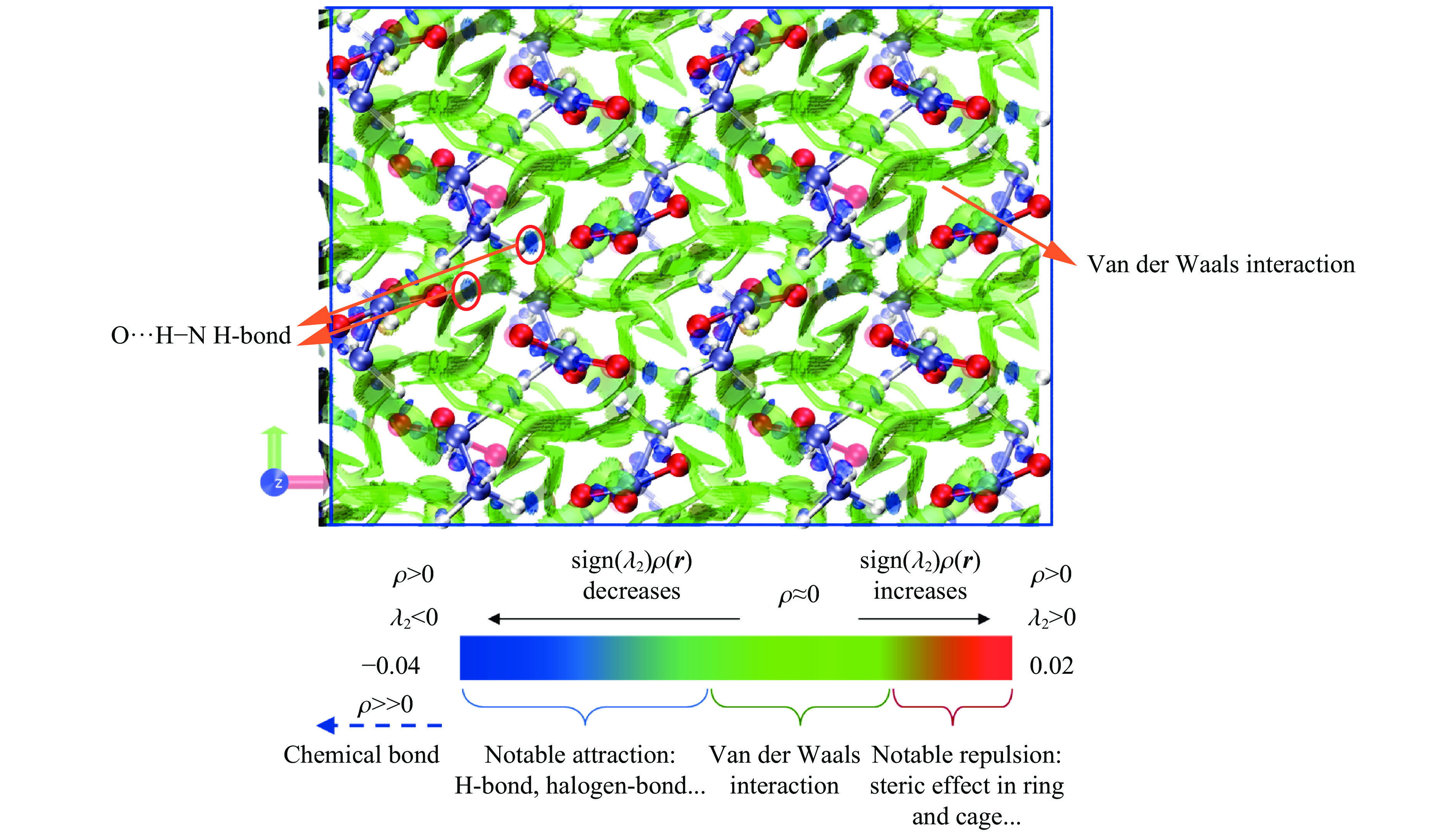
优化后的α-HN的晶格参数及其与实验数据的相对误差(δ(Expt.[8])和δ(Expt.[9]))见表1,其中:a、b、c、β为晶格参数,V为晶胞体积。整体上看,理论计算结果与实验测量结果[8–9]吻合,但是与早期Robinson等[7]测得的晶胞参数相差较大,推测与晶体质量(Robinson等未说明是否使用大尺寸单晶)、仪器性能(X射线源的稳定性)、X射线衍射精修(早期未发展出完善的数据精修方法)等因素有关。在结构优化的基础上,通过IRI指示函数研究HN晶体中基团内的化学键以及基团之间的相互作用,如图2所示。将IRI函数定义为约化电子密度梯度[16]。在计算IRI函数的基础上,将sign(λ2)ρ(r)函数投影到IRI等值面上,通过不同颜色将弱相互作用的位置、强度、类型表示出来,其中:ρ(r)代表电子密度,λ2代表电子密度Hessian矩阵第二大本征值,而sign(x)为符号函数。比如,绿色区域表示电子密度趋于零,相互作用强度较弱,为范德瓦尔斯作用区域;蓝色区域表示吸引相互作用,在HN体系中体现为氢键和化学键;红色区域表示排斥作用,体现为位阻。可以看到,HN晶体中肼基团[
N2H+5 ]和硝酸基团[NO−3 ]周围都充满范德瓦尔斯相互作用,维持着晶体的堆垛结构。其中,氢原子与邻近硝酸基团原子之间存在电子密度较大、sign(λ2)ρ(r)函数为负的蓝色区域,表明形成了较强的氢键作用。另外,硝酸基团与氢原子附近还存在一定的位阻作用(偏红色区域)。表 1 HN晶格参数的计算结果与实验结果比较Table 1. Calculated crystal lattice parameters of HN compared with experimental data3.2 HN晶体的高压中远红外振动光谱
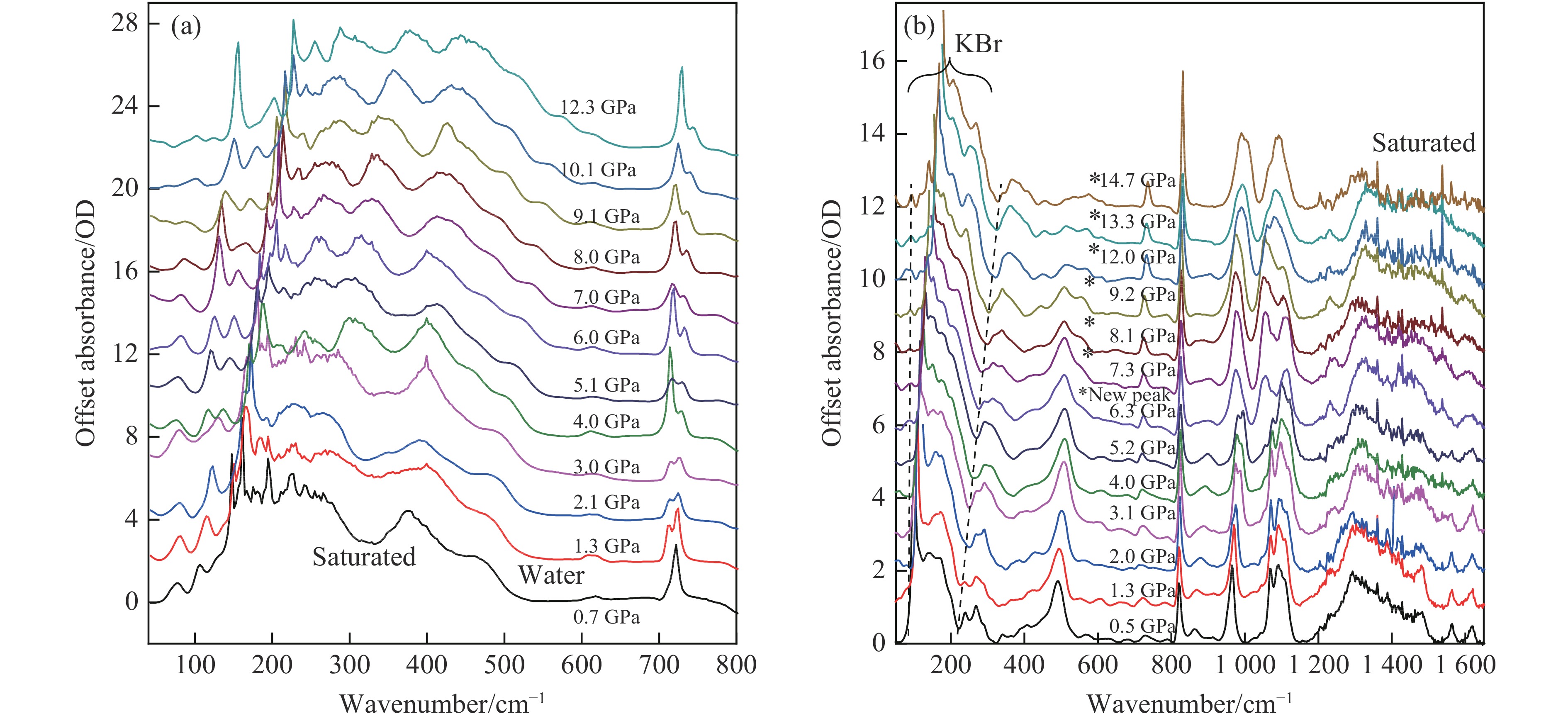
图3是利用高压超宽谱带中远红外探测系统测量的HN的高压红外吸收光谱,其中:远红外光谱的探测范围为30~800 cm−1,为纯HN样品的变压光谱,如图3(a)所示;中红外光谱的探测范围为50~1700 cm−1,为HN和KBr混合压片样品的变压光谱,如图3(b)所示。可以发现:随着压力的升高,大部分红外吸收峰蓝移,多个吸收峰有从单峰劈裂为多峰的趋势,并且非静水压效应增强,吸收峰展宽,部分吸收峰强度减弱。对于纯HN样品实验,由于无法完全消除水蒸气的影响,因此,在150~500 cm−1范围内结合水的红外吸收影响样品信号;对于混合样品实验,KBr在100~300 cm−1范围内有较强的吸收,也会影响样品信号,同时在1200~1500 cm−1范围内样品信号出现饱和。因此,对这些区域的信号不做定量分析。在实验所探测的波数范围内,可以观察到HN经历了多个非静水压下的结构变化。
与AN的结构类似,HN由2种离子构成,随着压力升高,分子间的相互作用和分子内的化学键逐渐增强,导致红外吸收峰蓝移。对于分子晶体,压力对分子间相互作用的影响比对分子内化学键的影响更强。因此,本研究主要考察肼基团[
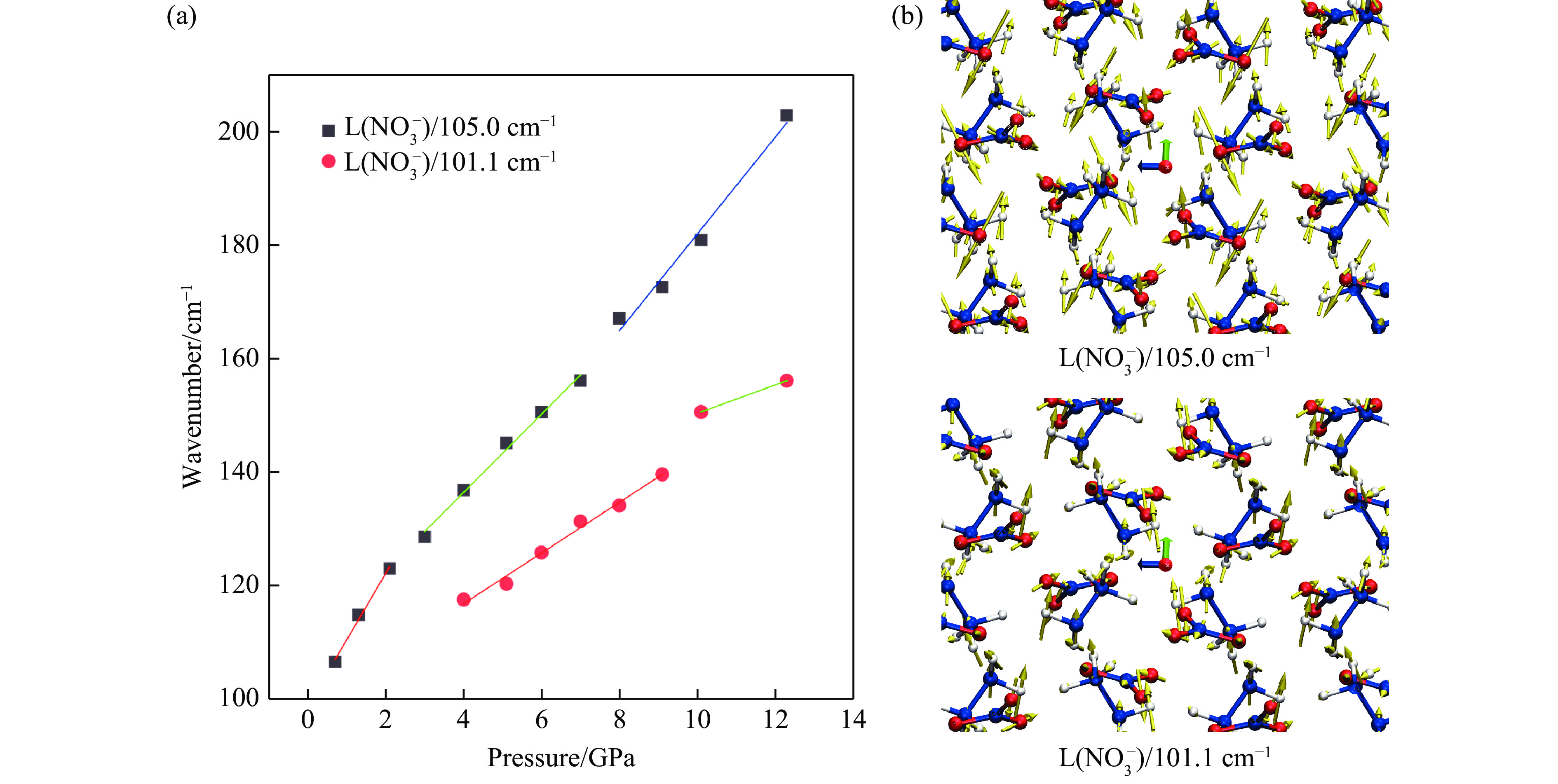
N2H+5 ]和硝酸基团[NO−3 ]的红外吸收声学模。图4显示了硝酸基团平动(libration)L(
NO−3 )对应的吸收峰随压力升高而蓝移的规律。首先,在3 GPa附近吸收峰从单峰分裂为双峰(图3),预示着结构发生变化;3~9 GPa压力范围内L(NO−3 ) 对应的位于101.1 和105.0 cm−1处的吸收峰频率随压力的升高而单调变化;从9 GPa开始,频移出现突变,预示着结构出现第2次变化。从第一性原理计算指认的模式上看,这2个红外吸收峰主要对应硝酸基团平动,同时也耦合肼基团平动。低频振动(L(NO−3 )/101.1 cm−1)中2种基团同相位转动,高频振动(L(NO−3 )/105.0 cm−1)中两者反相位转动。在3 GPa以上,L(NO−3 )/105.0 cm−1的蓝移随着压力的升高而快速增加,说明2种基团的相向旋转随压力的变化较同相旋转更敏感。结合前述分子间弱相互作用的IRI分析,在此压力范围内,分子间相互作用留给2种基团堆垛的“空间”足够大,近似“平面”的硝酸基团和近似“哑铃”的肼基团更容易通过相向转动调整位置。图5显示了肼基团平动L(
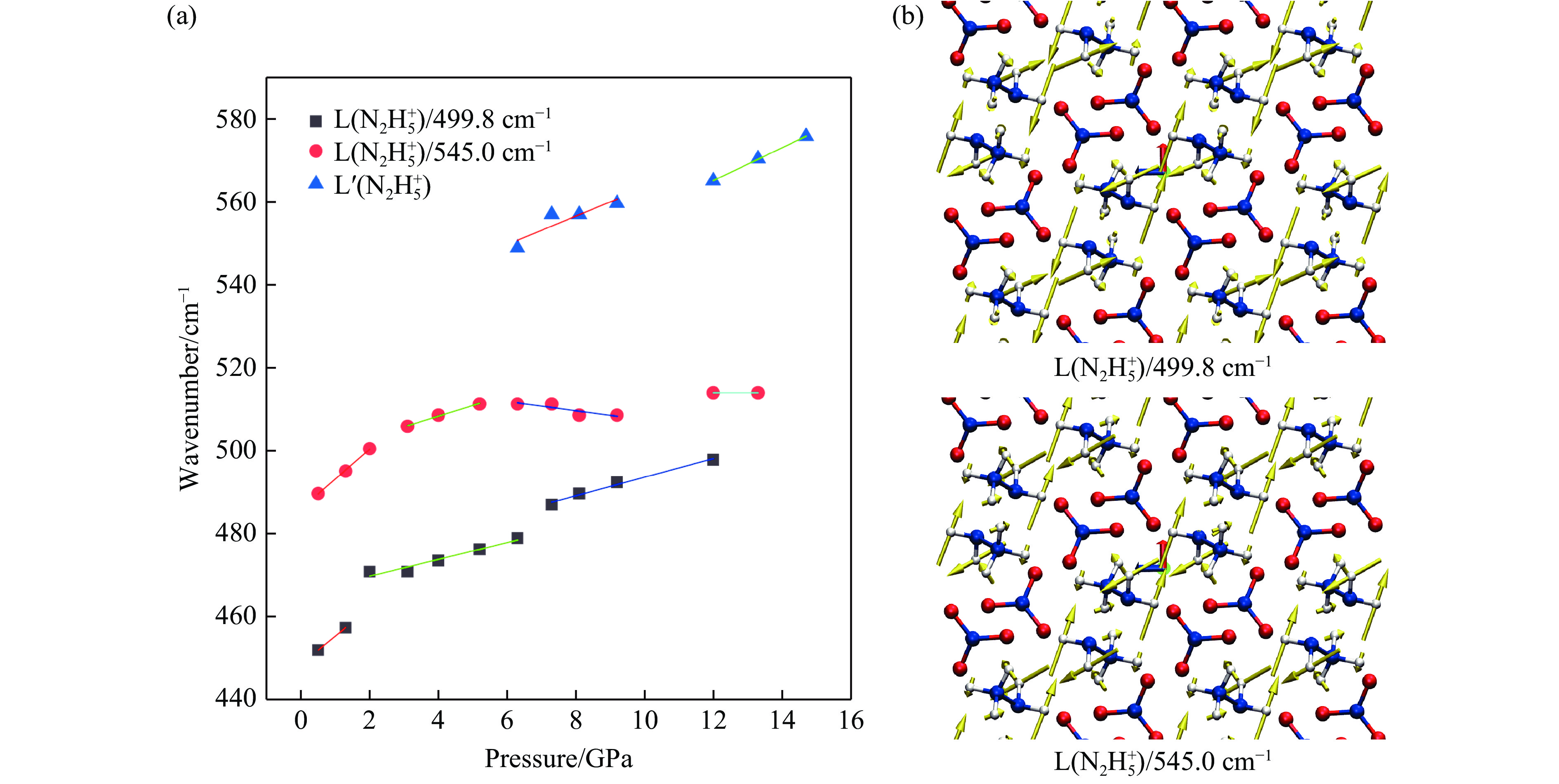
N2H+5 )对应的吸收峰频率随压力的变化规律。肼基团平动与硝酸基团平动(耦合肼基团)有显著区别:随着压力升高,其振动模式的变化更加复杂,并且在某些压力下部分振动模式有软化现象。根据第一性原理计算的指认结果,L(N2H+5 )/499.8 cm−1和L(N2H+5 )/545.0 cm−1均为纯粹的肼基团平动,没有耦合其他振动模式,因此,指认伴随L(N2H+5 )平动而产生的新峰L'(N2H+5 )也是肼基的平动振动,如图3(b)和图5所示。在约3 GPa时,2种振动模式所对应的频率随压力的升高出现突变,预示着结构变化。在6 GPa附近,产生新峰,见图3(b)中“New peak”。其中,L(N2H+5 )/545.0 cm−1模式出现软化现象。L(N2H+5 )/545.0 cm−1模式中NH2基团的转动方向偏向a轴和c轴,说明在当前实验条件的非静水压环境下,随着压力升高,a轴和c轴方向的分子间相互作用减弱,振动模式更倾向于向这2个方向调整。这种振动模式随压力升高而软化的现象也在AN的压致相变中出现[11]。随着压力继续升高,在12 GPa附近出现第3次频移突变,预示着HN又发生了一次结构变化。根据HN晶体中2种基团振动模式随压力的变化,推测HN在非静水压条件下经历了至少2次结构变化。这样的结构变化往往与压致相变关联[31–32]。第1次结构变化发生在3 GPa附近,2种基团的平动振动模式频率随压力升高的变化率出现突变,将可能的高压相标记为γ-HN;第2次结构变化发生在6 GPa(硝酸基团平动模式)至9 GPa(肼基团平动模式),2种基团协同变化,将可能的高压相标记为δ-HN。HN晶体压力相变的进一步确定还需开展后续实验进行持续深入的研究。今后的研究重点将放在改进高压超宽谱带中远红外光谱系统探测范围、分辨率和信噪比上,同时利用高温高压原位测量确定HN的相边界以及高温高压下的化学性质。
4. 结 论
为了满足军事和工业应用的需求,利用自主搭建的基于空气等离子体的超宽谱带中远红外光谱探测系统,成功获得了含能材料HN在高压下的红外振动光谱,得到如下主要结论:
(1) 基于空气等离子体的超宽谱中远红外光谱系统在含能材料高压性质研究领域具有独特作用;
(2) 基于GAPW基组、PBE泛函以及DFT-D3色散矫正的DFT能够合理地计算HN晶体的几何结构和振动光谱;
(3) 在0~15 GPa的非静水压范围内,随着压力的升高,HN中2种基团的平动振动模式经历了2次突变,预示着发生了2次压致相变。
本工作为后续建立HN这类重要含能材料在高温高压下的相图提供了初步的实验依据。考虑到HN与AN具有类似的结构,参考已发表的AN相图实验工作[11–12],有理由相信,HN也具有丰富的高温高压相。为此,在后续工作中,将通过进一步提高实验系统的分辨率和信噪比,采用原位高温高压系统与第一性原理结构演化搜索算法[33]相结合的方式,有望确定HN在高温高压下的相结构、相边界及化学反应活性。
-
表 1 计算工况
Table 1. Calculation conditions
No. v0/(m·s−1) α/(°) r/m L1/m Variable 1 800 10 0.06 0.08, 0.09, 0.13, 0.16 θ 2 200, 400, 800, 1200 10 0.06 0.13 θ 3 800 10, 20, −15, −30 0.06 0.13 θ 4 800 10 0.03, 0.04, 0.05, 0.06 0.13 θ 5 400 10 0.06 θ, L1 表 2 弹体结构参数
Table 2. Structural parameters of projectiles
Projectile r/mm L0/mm L1/mm θ/(°) I/(kg·m2) A 60 230 130 2.2 0.5035 B 60 230 95 4.5 0.5098 C 60 230 130 1.8 0.5029 表 3 侵彻试验结果
Table 3. Penetration test results
Projectile v0/(m·s−1) β0/(°) β5/(°) (β5–β0)/(°) ˉβ/(°) A 795 0.3 0.1 −0.2 −0.20 A 802 0 −0.2 −0.2 B 791 0.5 0 −0.5 −0.45 B 796 0.2 −0.2 −0.4 C 806 0 −0.1 −0.1 –0.10 C 801 0.1 0 −0.1 表 4 弹体尾部结构引起的偏转角度的计算结果
Table 4. Calculated results of deflection angle by change of tail projectile
Projectile β1/(°) β2/(°) β3/(°) β4/(°) β5/(°) δ/% A −0.018 −0.041 −0.077 −0.140 −0.250 25 B −0.029 −0.079 −0.160 −0.300 −0.530 18 C −0.008 −0.016 −0.028 −0.049 −0.081 8 -
[1] 李鹏飞, 吕永柱, 周涛, 等. 弹头形状对侵彻多层靶弹道的影响 [J]. 含能材料, 2021, 29(2): 124–131. doi: 10.11943/CJEM2020291LI P F, LYU Y Z, ZHOU T, et al. Influence of nose shape of projectile on the penetration trajectory of multilayer target [J]. Chinese Journal of Energetic Materials, 2021, 29(2): 124–131. doi: 10.11943/CJEM2020291 [2] 葛超, 董永香, 陆志超, 等. 弹丸头部对斜侵彻弹道偏转影响研究 [J]. 兵工学报, 2015, 36(2): 255–262. doi: 10.3969/j.issn.1000-1093.2015.02.010GE C, DONG Y X, LU Z C, et al. Ballistic deflection on oblique penetration of projectiles with different noses [J]. Acta Armamentarii, 2015, 36(2): 255–262. doi: 10.3969/j.issn.1000-1093.2015.02.010 [3] 张丁山, 谷鸿平, 徐笑, 等. 截卵形头部平台直径对初始侵彻弹道偏转的影响 [J]. 高压物理学报, 2021, 35(5): 055102. doi: 10.11858/gywlxb.20200655ZHANG D S, GU H P, XU X, et al. Effects of truncated ovate nose diameter of the penetration warhead on the ballistic deflection [J]. Chinese Journal of High Pressure Physics, 2021, 35(5): 055102. doi: 10.11858/gywlxb.20200655 [4] 杜华池, 张先锋, 刘闯, 等. 弹体斜侵彻多层间隔钢靶的弹道特性 [J]. 兵工学报, 2021, 42(6): 1204–1214. doi: 10.3969/j.issn.1000-1093.2021.06.010DU H C, ZHANG X F, LIU C, et al. Trajectory characteristics of projectile obliquely penetrating into steel target with multi-layer space structure [J]. Acta Armamentarii, 2021, 42(6): 1204–1214. doi: 10.3969/j.issn.1000-1093.2021.06.010 [5] 薛建锋, 沈培辉, 王晓鸣. 弹体斜侵彻混凝土过程中弹道偏转仿真分析 [J]. 系统仿真学报, 2017, 29(8): 1801–1808. doi: 10.16182/j.issn1004731x.joss.201708021XUE J F, SHEN P H, WANG X M. Simulation analysis on ballistic deflection of projectile obliquely penetrating into concrete [J]. Journal of System Simulation, 2017, 29(8): 1801–1808. doi: 10.16182/j.issn1004731x.joss.201708021 [6] 康海峰, 代廷静, 沈培辉, 等. 弹体形状对侵彻弹道的影响分析 [J]. 弹箭与制导学报, 2012, 32(2): 73–76. doi: 10.3969/j.issn.1673-9728.2012.02.020KANG H F, DAI T J, SHEN P H, et al. The analysis of the influence of projectile’s shape on penetration trajectory [J]. Journal of Projectiles, Rockets, Missiles and Guidance, 2012, 32(2): 73–76. doi: 10.3969/j.issn.1673-9728.2012.02.020 [7] 段卓平, 李淑睿, 马兆芳, 等. 刚性弹体斜侵彻贯穿混凝土靶的姿态偏转理论模型 [J]. 爆炸与冲击, 2019, 39(6): 063302. doi: 10.11883/bzycj-2018-0411DUAN Z P, LI S R, MA Z F, et al. Analytical model for attitude deflection of rigid projectile during oblique perforation of concrete targets [J]. Explosion and Shock Waves, 2019, 39(6): 063302. doi: 10.11883/bzycj-2018-0411 [8] 高旭东, 李庆明. 带攻角斜侵彻混凝土的弹道偏转分析 [J]. 兵工学报, 2014, 35(Suppl 2): 33–39.GAO X D, LI Q M. Trajectory analysis of projectile obliquely penetrating into concrete target at attack angle [J]. Acta Armamentarii, 2014, 35(Suppl 2): 33–39. [9] 吴普磊, 李鹏飞, 董平, 等. 攻角对弹体斜侵彻多层混凝土靶弹道偏转影响的数值模拟及试验验证 [J]. 火炸药学报, 2018, 41(2): 202–207. doi: 10.14077/j.issn.1007-7812.2018.02.017WU P L, LI P F, DONG P, et al. Numerical simulation and experimental verification on the influence of angle of attack on ballistic deflection of oblique penetrating multi-layer concrete targets for projectile [J]. Chinese Journal of Explosives & Propellants, 2018, 41(2): 202–207. doi: 10.14077/j.issn.1007-7812.2018.02.017 [10] 邓勇军, 陈小伟, 姚勇, 等. 基于细观混凝土模型的刚性弹体正侵彻弹道偏转分析 [J]. 爆炸与冲击, 2017, 37(3): 377–386. doi: 10.11883/1001-1455(2017)03-0377-10DENG Y J, CHEN X W, YAO Y, et al. On ballistic trajectory of rigid projectile normal penetration based on a meso-scopic concrete model [J]. Explosion and Shock Waves, 2017, 37(3): 377–386. doi: 10.11883/1001-1455(2017)03-0377-10 [11] 成丽蓉, 汪德武, 贺元吉, 等. 弹体斜侵彻多层间隔混凝土薄靶姿态偏转机理研究 [J]. 北京理工大学学报, 2022, 42(10): 1009–1016. doi: 10.15918/j.tbit1001-0645.2021.299CHENG L R, WANG D W, HE Y J, et al. Effect of projectile oblique penetrating into multi-layered thin concrete slabs on attitude deflection [J]. Transactions of Beijing Institute of Technology, 2022, 42(10): 1009–1016. doi: 10.15918/j.tbit1001-0645.2021.299 [12] 马兆芳, 段卓平, 欧卓成, 等. 尾裙对弹体斜侵彻混凝土薄靶弹道的影响规律研究 [J]. 北京理工大学学报, 2015, 35(6): 576–579, 584. doi: 10.15918/j.tbit1001-0645.2015.06.006MA Z F, DUAN Z P, OU Z C, et al. Investigation on the influence rules of the tapered tail projectile penetration into thin concrete target [J]. Transactions of Beijing Institute of Technology, 2015, 35(6): 576–579, 584. doi: 10.15918/j.tbit1001-0645.2015.06.006 [13] 柴传国, 皮爱国, 武海军, 等. 卵形弹体侵彻混凝土开坑区侵彻阻力计算 [J]. 爆炸与冲击, 2014, 34(5): 630–634. doi: 10.11883/1001-1455(2014)05-0630-06CHAI C G, PI A G, WU H J, et al. A calculation of penetration resistance during cratering for ogive-nose projectile into concrete [J]. Explosion and Shock Waves, 2014, 34(5): 630–634. doi: 10.11883/1001-1455(2014)05-0630-06 [14] 张爽, 武海军, 黄风雷. 刚性弹正侵彻钢筋混凝土靶阻力模型 [J]. 兵工学报, 2017, 38(11): 2081–2092. doi: 10.3969/j.issn.1000-1093.2017.11.001ZHANG S, WU H J, HUANG F L. Resistance model of rigid projectile penetrating into reinforced concrete target [J]. Acta Armamentarii, 2017, 38(11): 2081–2092. doi: 10.3969/j.issn.1000-1093.2017.11.001 -









 下载:
下载:












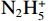
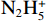

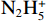
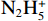


 下载:
下载: