Progress on Physical and Chemical Processes Deep Inside Ice Giants
-
摘要: 宇宙中诸如天王星、海王星等冰巨行星的数量繁多,理解冰巨行星的内部结构与局部反应过程对于建立统一的行星演化体系具有重要意义。近几十年来,随着模拟计算方法、实验加载与诊断技术的不断发展,与冰巨行星内部相关的多个物理问题研究取得了突破性进展,如“超离子态水”、“钻石雨”等现象不再不可捉摸。聚焦冰巨行星相关物理问题,简要介绍并讨论了极端状态下的高压状态方程和微观物理过程的理论及实验研究进展,包括相关实验平台与配套技术的发展情况,并对该领域的未来发展方向提出了展望。Abstract: Ice giants such as Uranus and Neptune are numerous in the universe. Understanding the internal structure and local reaction processes of ice giants is of great significance for establishing a unified planetary evolution system. In recent decades, with the continuous development of simulation methods and experimental driving as well as diagnostic techniques, breakthroughs have been made in multiple physical problems related to the interior of ice giants, such as “superionic water” and “diamond rain”, which are no longer unpredictable. Starting from the physical issues related to ice giants, this article briefly introduces and discusses the theoretical and experimental research progress in high-pressure equations of state and microscopic physical processes under extreme conditions, as well as the development of related experimental platforms and supporting technologies. It also proposes prospects for the future direction of this field.
-
Key words:
- ice giants /
- superionic water /
- diamond rain /
- dynamic in situ X-ray diagnosis
-
It is very important for mining and civil construction to predict the morphology distribution of cracks induced by blasting. Hence, many researchers have paid their attention to dynamic fracture behavior of rocks due to drilling and blasting operations[1-3]. A number of experiments and numerical simulations have been conducted to investigate the blasting-induced fractures in the near borehole zone as well as in the far field[4-5]. In order to gain high fidelity in simulating the complex responses of rocks subjected to blasting loading, a realistic constitutive model is required. In the last 20 years, various macro-scale material models have been proposed, from relatively simple ones to more sophisticated, and their capabilities in describing actual nonlinear behavior of material under different loading conditions have been evaluated[6].
During blasting operation, chemical reactions of explosive in borehole occur rapidly, and instantaneously a shock/stress wave applies to borehole wall. Initially, a crushed zone around the borehole is developed by the shock/stress wave. Then, a radial shock/stress wave propagates away from borehole, and its magnitude decreases. Once the radial shock/stress drops below the local dynamic compressive strength, no shear damage occurs. At the same time, a tensile tangential stress with enough strength can be developed behind the radial compressive stress wave, which results in an extension of the existing flaws or a creation of new radial cracks. If there is a nearby free boundary, the incident compressive stress wave changes to a tensile stress wave upon reflection, and reflects back into the rock. In this case, if the dynamic tensile strength of rock is exceeded, spall cracks appear close to the free boundary.
The purpose of this paper is to conduct a numerical study on borehole blasting-induced fractures in rocks. First, a dynamic constitutive model for rocks based on the previous work of concrete[7] is briefly described, and the values of various parameters in the model for granite are estimated. The model is then employed to simulate the borehole blasting-induced fractures in granitic rocks. Comparisons between the numerical results and the experimental observations are made, and a discussion is given.
1. Dynamic Constitutive Model for Rocks
A number of models for concrete-like materials, such as TCK model[8], HJC model[9], RHT model[10], K&C model[11], have been developed. The sophisticated numerical models are increasingly used as they are capable of describing the material behavior under high strain rate loading. However, these models have been found to have some serious flaws, and cannot predict the experimentally observed crack patterns or exhibit improper behavior under certain loading conditions[7, 12-15].
In the following, a dynamic constitutive model for rocks is briefly described according to equation of state (EOS) and strength model, based on the previous work on concrete[7].
1.1 EOS
A typical form of EOS is the so-called p-
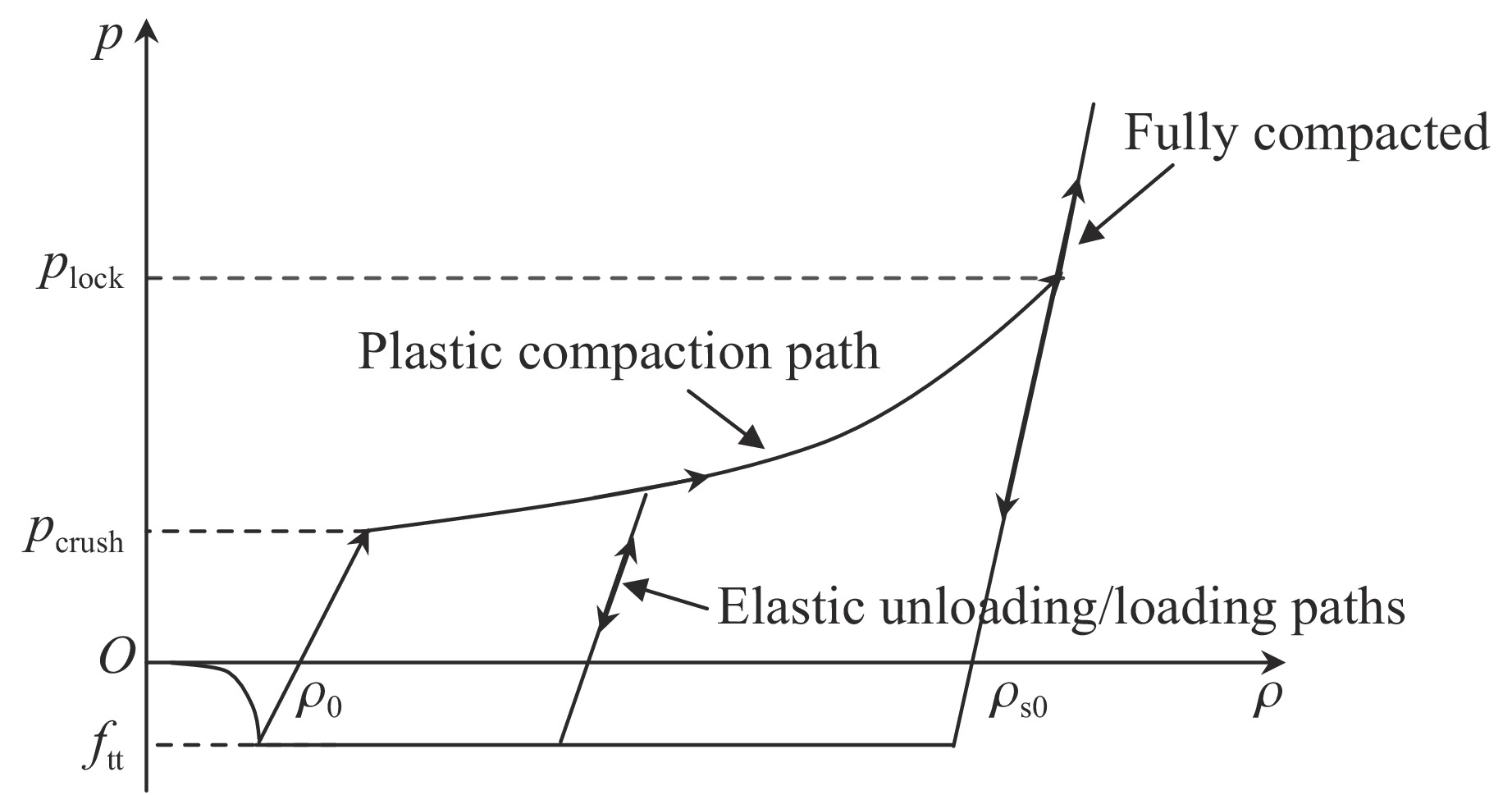
α relation, which is proved to be capable of representing brittle material’s response behavior at high pressures, and it allows for a reasonably detailed description of the compaction behavior at low pressure ranges as well, as shown schematically in Fig.1.pcrush corresponds to the pore collapse pressure beyond which plastic compaction occurs, andplock is the pressure when porosityα reaches 1,ftt is the tensile strength,ρ0 is the initial density,ρs0 refers to the density of the initial solid. Figure 1. Schematic diagram of EOS[7]
Figure 1. Schematic diagram of EOS[7]The EOS for compression (p≥0) is given by
p=K1ˉμ+K2ˉμ2+K3ˉμ3 (1) where p denotes pressure, K1, K2, K3 are constants, and
ˉμ is defined byˉμ=ραρ0α0−1=αα0(1+μ)−1 (2) where
ρ is the current density,μ=ρ/ρ0−1 specifies the volumetric strain,α0 =ρ s0/ρ 0 andα=ρs/ρ represent the initial porosity and the current porosity, respectively.ρs refers to the density of fully compacted solid. Physically,α is a function of the hydro-static pressure p, and is expressed asα=1+(α0−1)(plock−pplock−pcrush)n (3) where n is the compaction exponent.
When material withstands hydro-static tension, the EOS for tension (p<0) is given by
p=K1ˉμ (4) α=α0(1+p/K1)/(1+μ) (5) 1.2 Strength Model
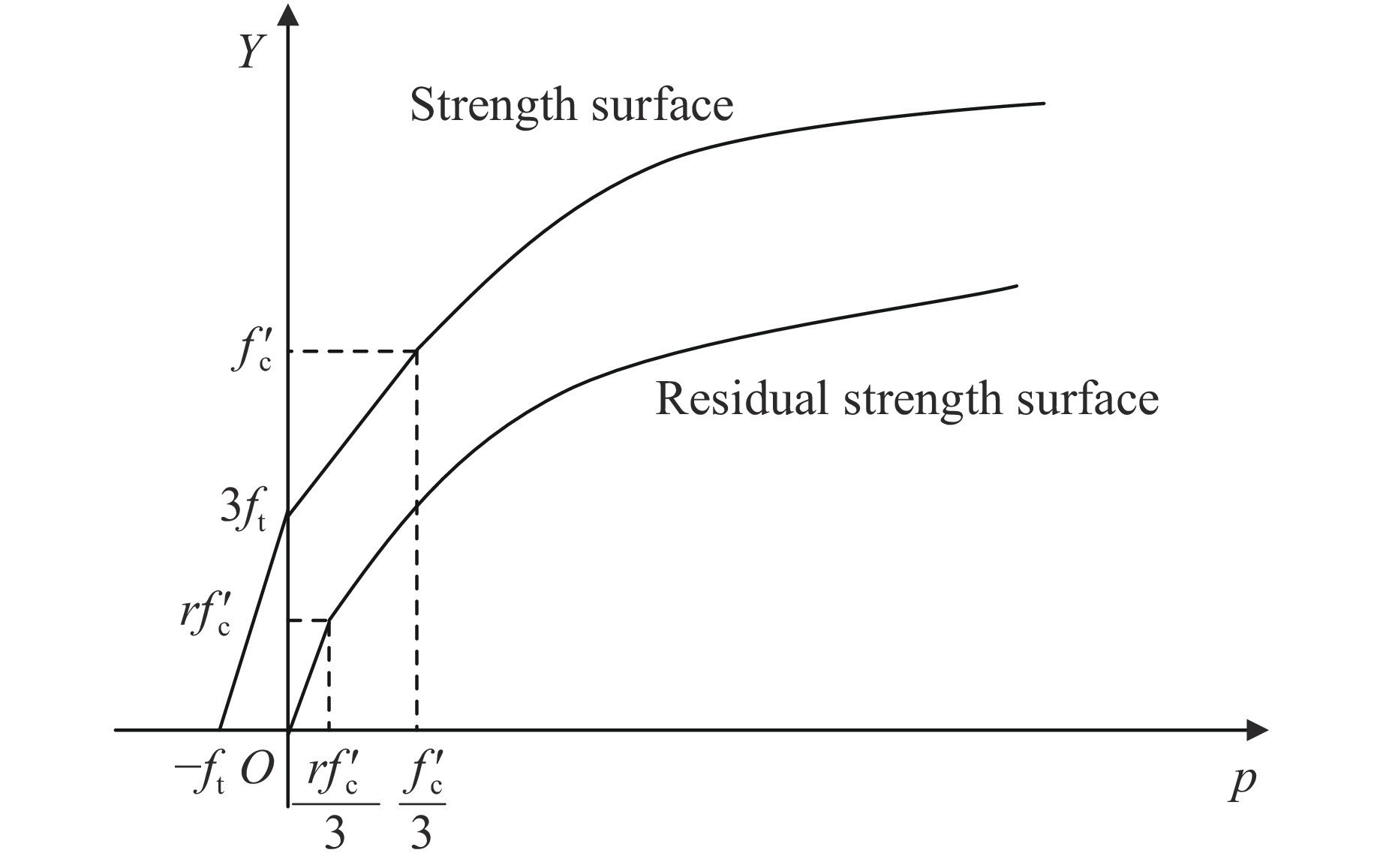
The strength model takes into account various effects, such as pressure hardening, damage softening, third stress invariant (Lode angle) and strain rate. The strength surface Y, shown schematically in Fig.2, can be written as[7]
Y={3(p+ftt)R(θ,e) p<0[3ftt + 3p(fcc−3ftt)/fcc]R(θ,e) 0⩽p⩽fcc/3{fcc+Bfc′[p/fc′−fcc/(3fc′)]N}R(θ,e) p>fcc/3 (6) where p is the hydro-static pressure, parameters B and N are constants, R(θ,e) is a function of the Lode angle θ and the tensile-to-compressive meridian ratio e,
fc′ is the static uni-axial compressive strength, the compressive strengthfcc and the tensile strengthftt are defined byfcc=fc′Dm_tηc (7) ftt=ftDtηt (8) where
ft is the static uni-axial tensile strength.Dm_t is the compression dynamic increase factor due to strain rate effect only, and can be expressed as[7, 16]Dm_t=(Dt−1)ft/fc′+1 (9) where
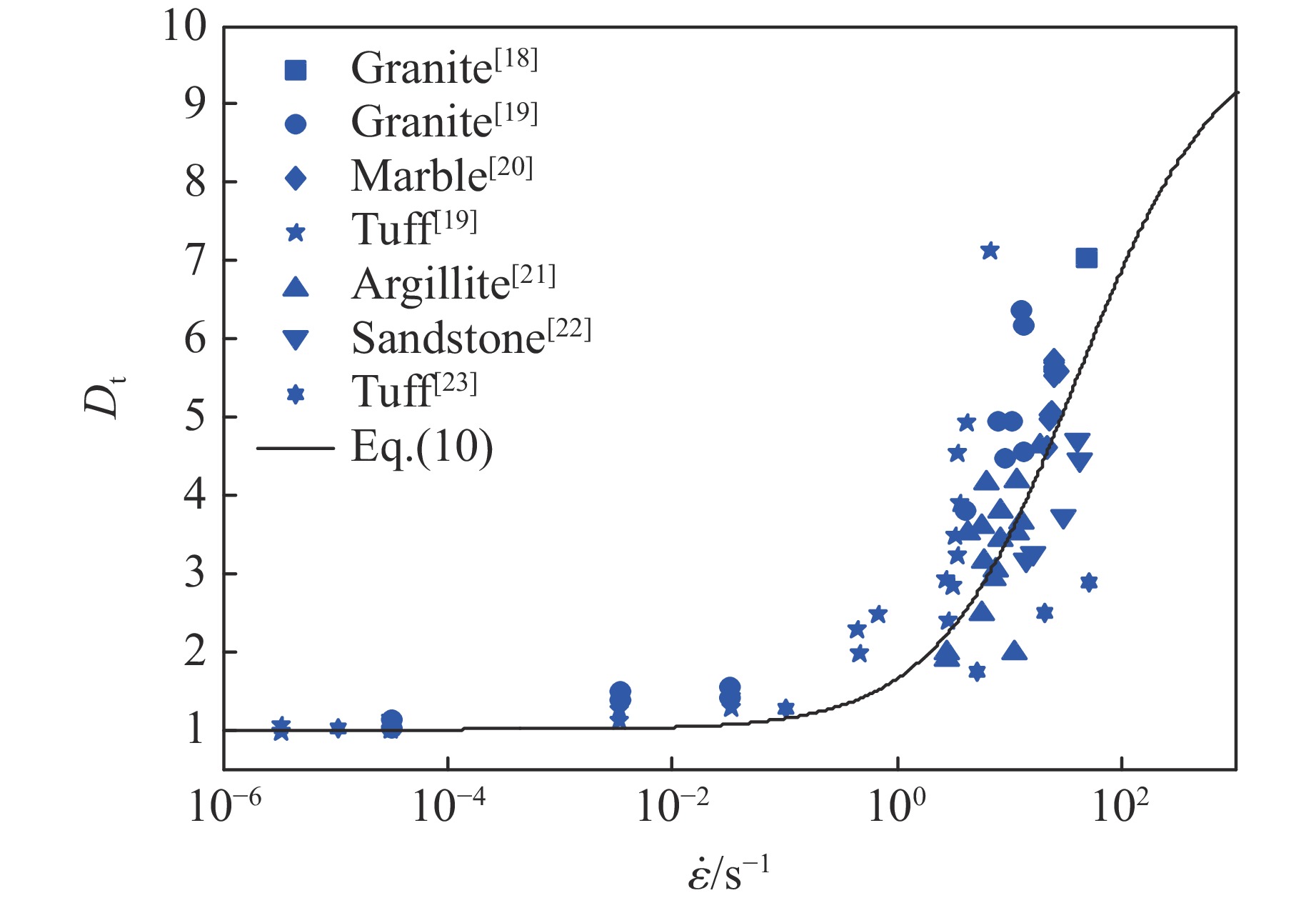
Dt is the tension dynamic increase factor determined byDt={tanh[(lg˙ε˙ε0−Wx)S][FmWy−1]+1}Wy (10) where
Fm ,Wx ,Wy andS are experimental constants,˙ε is the strain rate, and˙ε0 is the reference strain rate, usually taken˙ε0=1.0 s−1 .ηc is the damage function for compression, which can be expressed asηc={l+(1−l)η(λ)λ⩽λmr+(1−r)η(λ)λ>λm (11) where l and r are constants[7],
λm is the value of shear damage (λ ) when strength reaches its maximum value under compression.η(λ) is defined asη(λ)=aλ(λ−1)exp(−bλ) (12) in which a and b can be determined by setting
η(λ) = 1 and∂η∂λ=0 whenλ =λ m.ηt is the damage function for tension which can be written asηt=[1+(c1εtεfrac)3]exp(−c2εtεfrac)−εtεfrac(1+c31)exp(−c2) (13) where c1 and c2 are constants[7],
εt denotes the tensile strain andεfrac is the fracture strain.The residual strength (
rfc′ ) surface for rocks, shown schematically in Fig.2, can be obtained from Eq.(6) by settingftt=0 andfcc=rfc′ , vizY={3pR(θ,e) 0<p⩽rfc′/3{rfc′+Bfc′[p/fc′−rfc′/(3fc′)]N}R(θ,e) p>rfc′/3 (14) 2. Numerical Simulations
Granite is selected for investigating the dynamic fractures which result from borehole blast loading.
2.1 Evaluation of Various Parameters in the Model
Table 1 lists the values of the various parameters used in the dynamic constitutive model for granite. As to how to determine the values of the various parameters in the model, more details are presented in [7, 15-17].
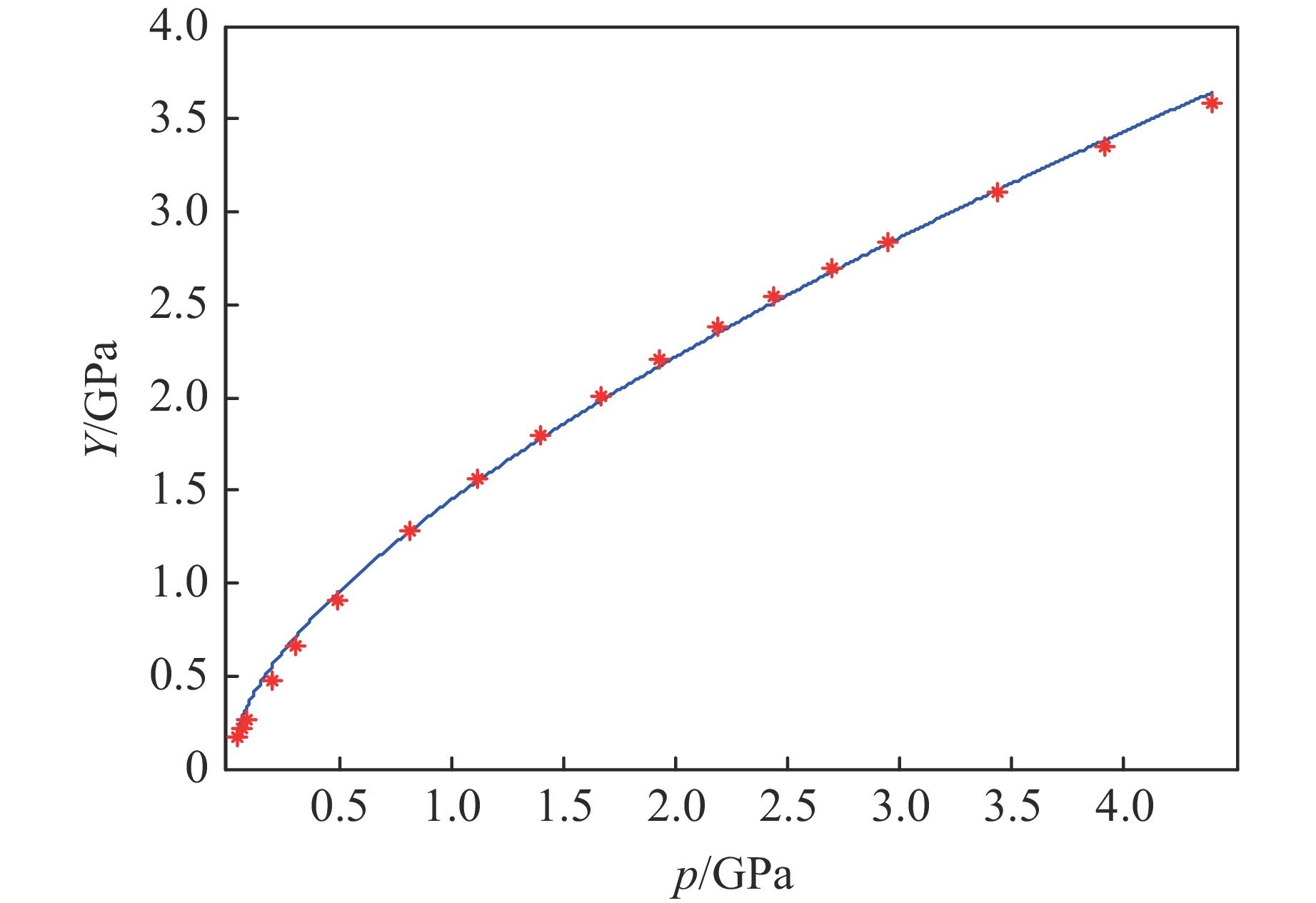
p-α relation ρ0/(kg∙m−3) pcrush/MPa plock/GPa n K1/GPa K2/TPa K3/TPa 2660 50.5 3 3 25.7 −3 150 Strength surface Strain rate effect fc′/MPa ft/MPa B N G/GPa Fm Wx 161.5 7.3 2.59 0.66 21.9 10 1.6 Strain rate effect Shear damage Wy S ˙ε0/s−1 λs λm l r 5.5 0.8 1.0 4.6 0.3 0.45 0.3 Lode effect Tensile damage e1 e2 e3 c1 c2 εfrac 0.65 0.01 5 3 6.93 0.007 Fig.3 shows the comparison of the strength surface between Eq.(6) (with B=2.59, N=0.66) and the triaxial test data for granite[17]. It can be seen from Fig.3 that a good agreement is obtained. Similarly, Fig.4 shows the tensile strengths/dynamic increase factor obtained by Eq.(10) and the test results of various rocks at different strain rates[18-23]. It is clear from Fig.4 that a good agreement is achieved.
 Figure 3. Comparison of the strength surface between Eq.(6) (with B=2.59, N=0.66) and the triaxial test data for granite[17]
Figure 3. Comparison of the strength surface between Eq.(6) (with B=2.59, N=0.66) and the triaxial test data for granite[17]2.2 Numerical Results
In the following, numerical simulations are carried out for the response of the granite targets subjected to borehole blasting loading. The dynamic fracture behavior of two kinds of granite samples are studied, namely, cylindrical sample as reported in the literature and square sample as examined in our own laboratory.
2.2.1 Cylindrical Rock Sample
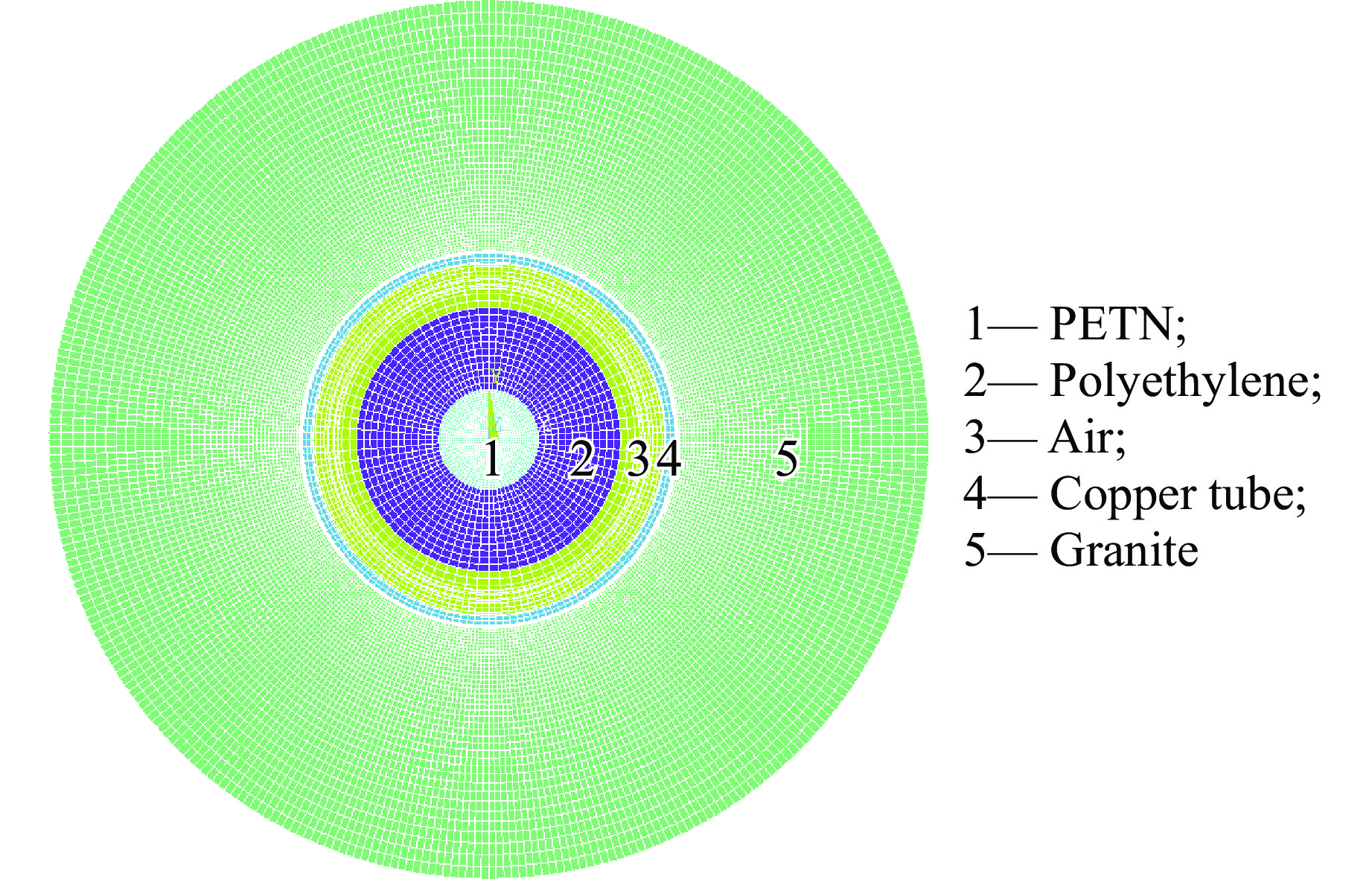
In consideration of the sizes of the cylindrical granite samples prepared for laboratory-scale blasting experiments by Dehghan Banadaki and Mohanty[17] (with a diameter of 144 mm, a height of 150 mm and a borehole diameter of 6.45 mm), a circular plane strain model with an outer diameter of 144 mm is made in our simulation, as shown in Fig.5, being a scaled close-up view of the borehole region. Multi-material Euler solver is used for modeling PETN explosive, polyethylene and air. Lagrangian descriptions are used for modeling the copper tube and granite.
The material model and the properties of PETN explosive, polyethylene, air and copper tube used in the simulation are given in Ref.[17]. The values of various parameters in the constitutive model for granite are listed in Table 1.
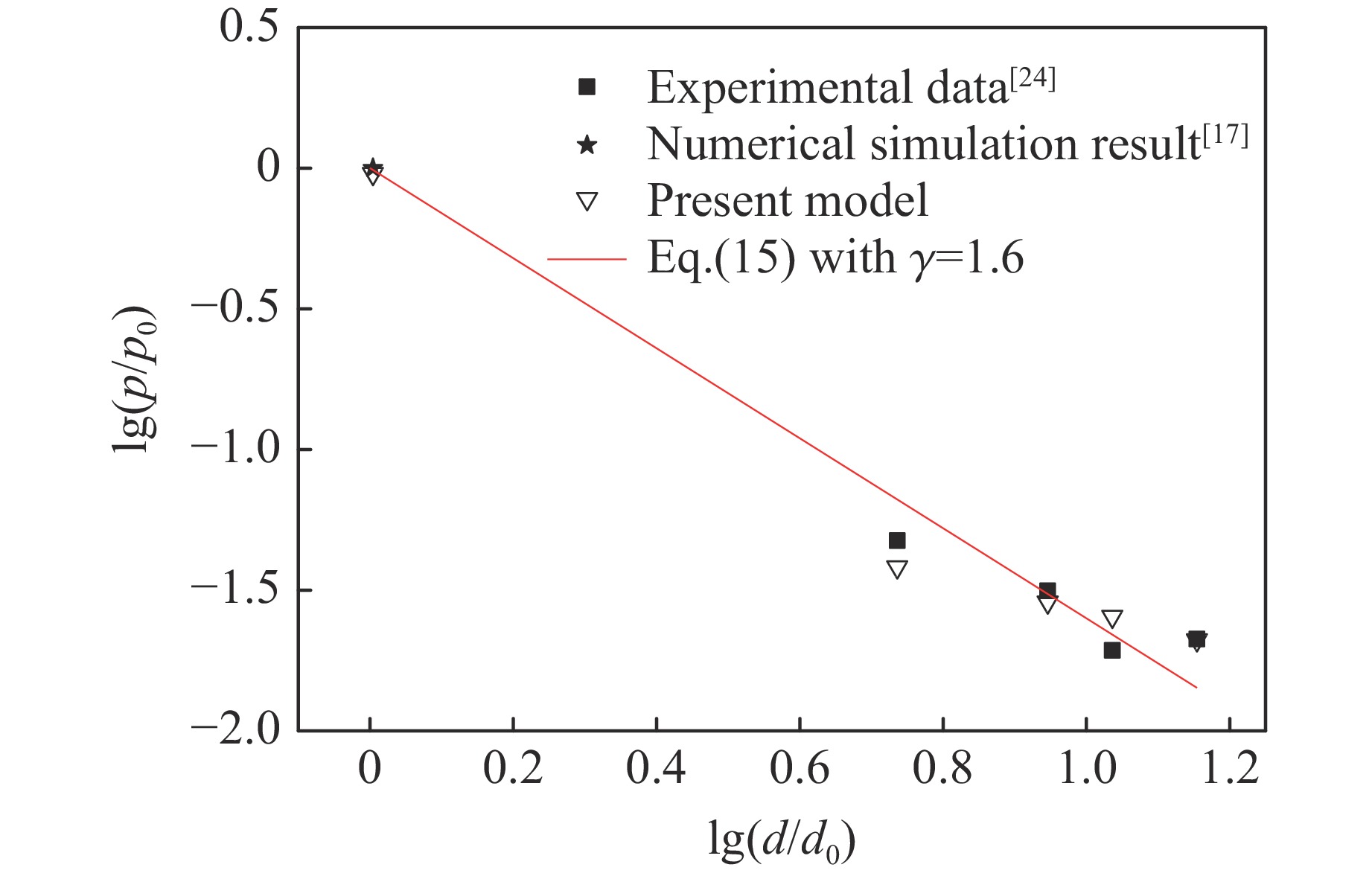
Fig.6 shows the comparison of the peak pressures between our simulation results of the present model, the numerical results[17], and the experimental results by Dehghan Banadaki and Mohanty[24]. It can be seen from Fig.6 that a good agreement is obtained.
In order to characterize the damping behavior of stress in granite, the peak pressure p in granite is expressed in an exponential form as
pp0=(dd0)−γ (15) where p0 is the peak pressure on the borehole wall, d0 is the initial radius of the borehole, d is the distance from the center point of the borehole,
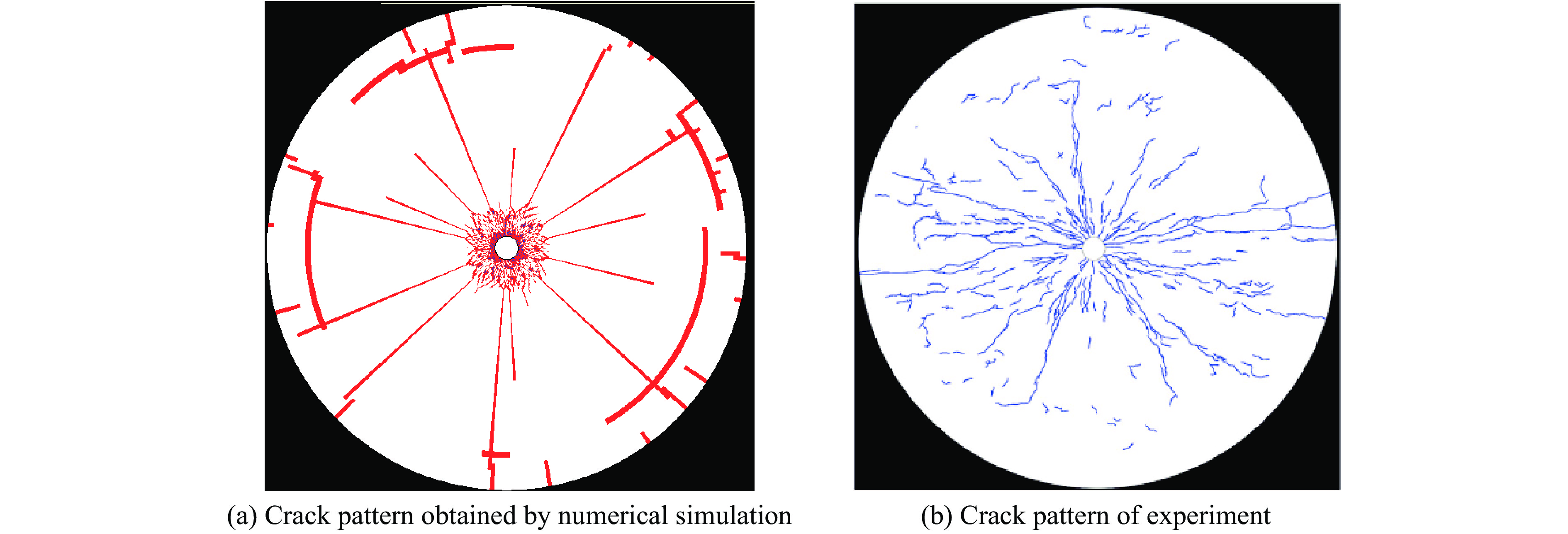
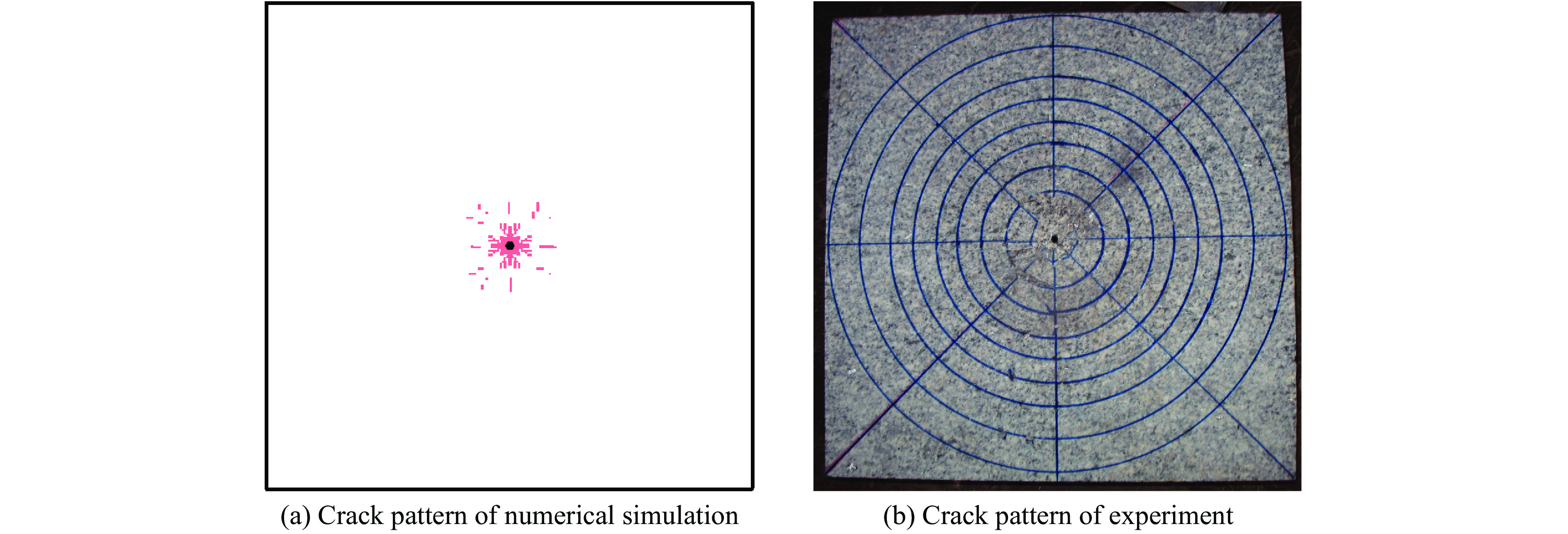
γ is an index. It is evident from Fig.6 that Eq.(15) withγ =1.6 correlates well with the experimental results.Fig.7 shows the comparison between the crack patterns predicted numerically based on the present model and the one observed experimentally in the cylindrical granite sample[17]. It is clear that a relatively good agreement on the crack pattern is obtained. It is also clear that the stress waves produce three distinct crack regions in the cylindrical granite sample: densely populated smaller cracks around the borehole, a few large radial cracks propagating towards the outer boundary, and circumferential cracks close to the sample boundary which are due to the reflected tension stress.
 Figure 7. Comparison of the crack patterns between the numerical prediction and the experiment of the cylindrical granite sample[17]
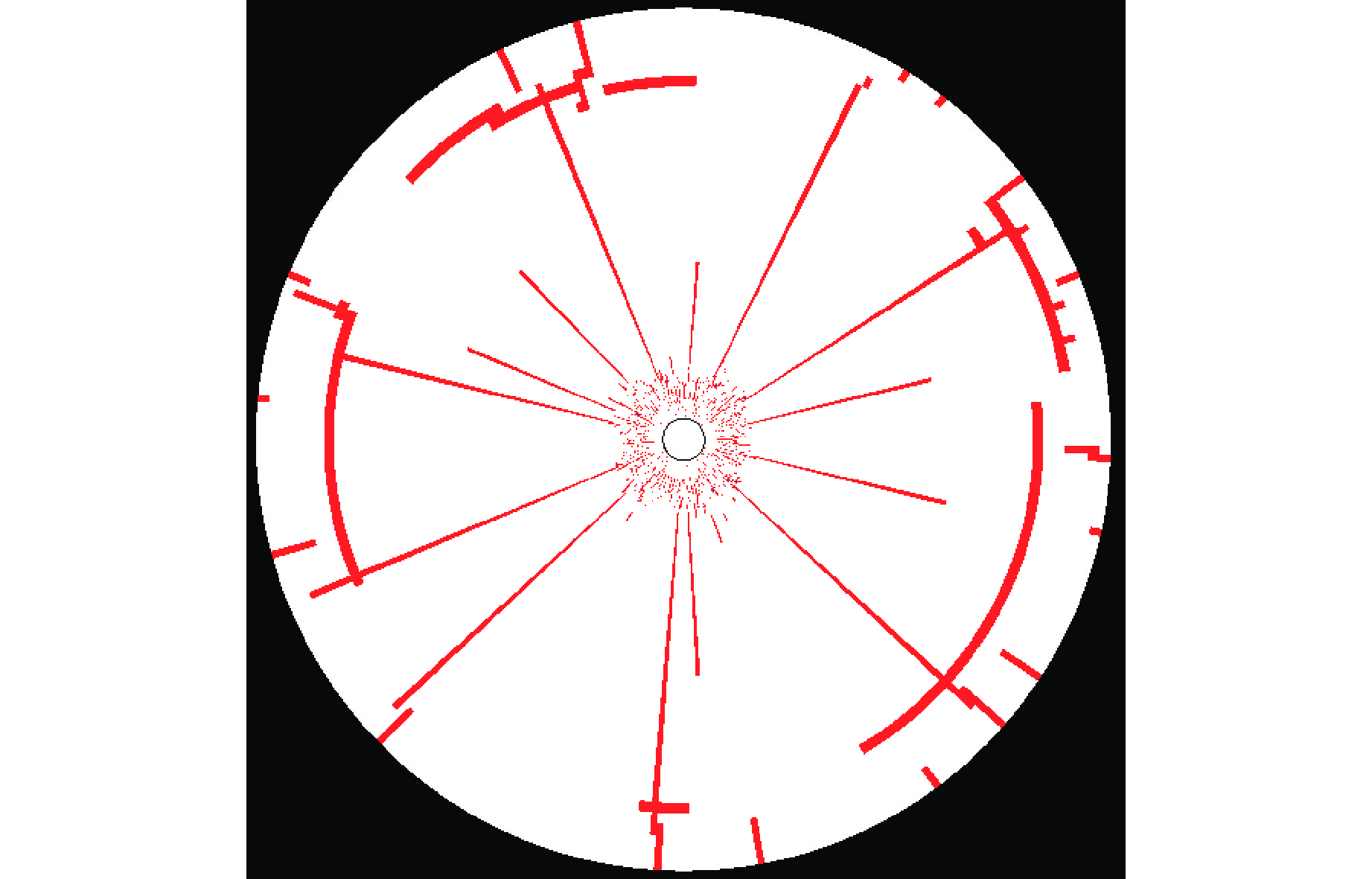
Figure 7. Comparison of the crack patterns between the numerical prediction and the experiment of the cylindrical granite sample[17]In order to make an assessment of the contributions of both the compression/shear stress and the tensile stress to the crack patterns, Fig.8 shows the numerically predicted crack pattern which results from the tension stress only. Fom Fig.8 and Fig.7(a), it is apparent that there are virtually few changes in crack patterns, both having the large radial and circumferential cracks caused by the same tensile stress, however, the smaller cracks around the borehole in Fig.8 are much less than those in Fig.7(a). In another word, crack patterns are mainly caused by tensile stress, and smaller cracks around borehole are created largely by compression/shear stress.
2.2.2 Square Granite Sample
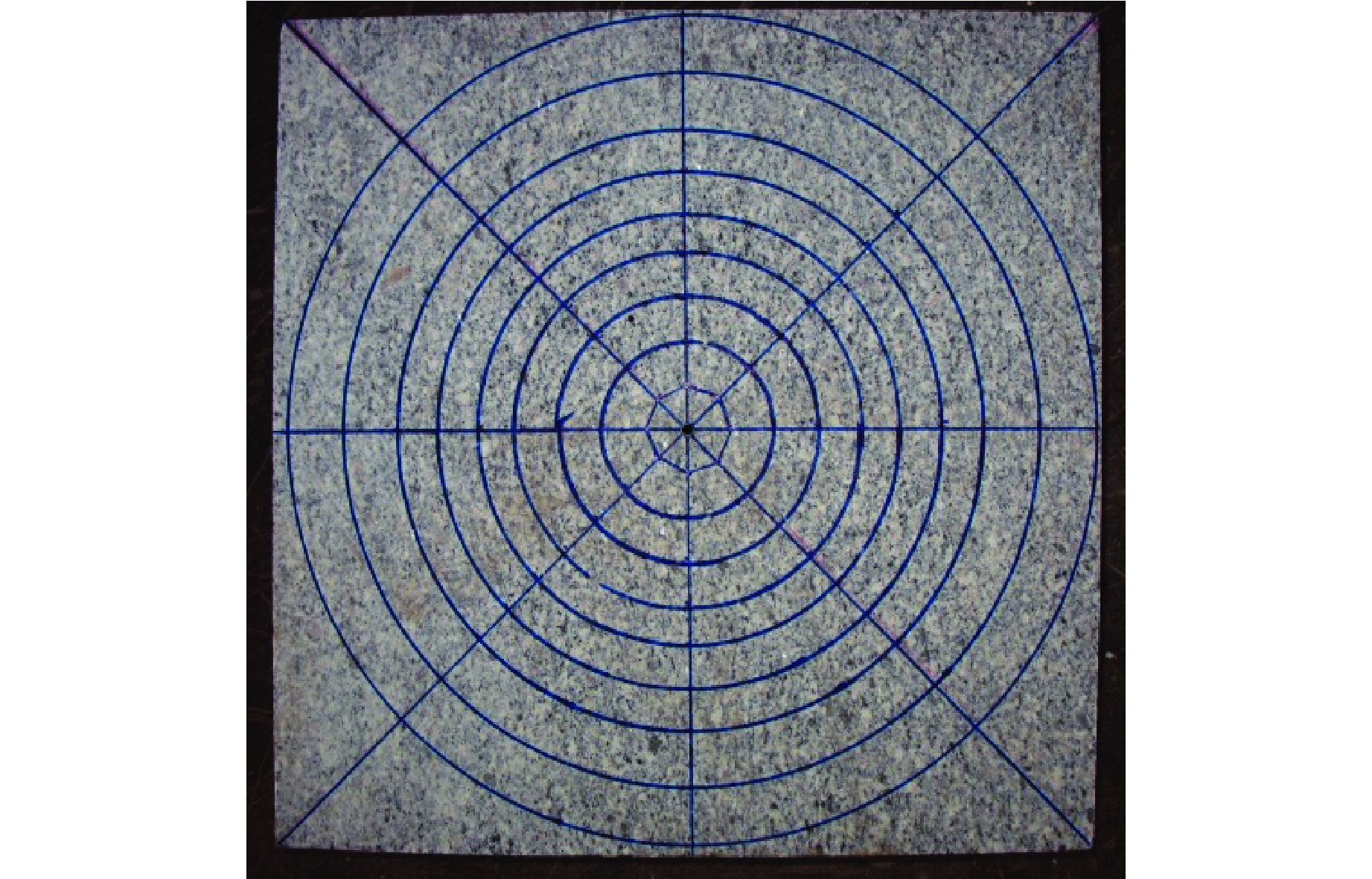
The laboratory-scale single-hole blasting tests are also carried out in order to validate further the accuracy and the reliability of the present model. Two square granite samples with a side length of 400 mm and a height of 100 mm are employed in the experiments. The borehole diameter is 4 mm and a series of concentric rings is drawn on the top surface of the samples (see Fig.9), so that the damage regions induced by detonation can be assessed visually.
A cylindrical RDX explosive enclosed by an aluminum sheath (see Fig.10) is tightly installed in the borehole of the No.1 sample, while an unwrapped RDX explosive is inserted into the borehole of the No.2 sample. The density of the RDX is 1700 kg/m3, and the material model and properties of the RDX explosive and the aluminum sheath used in the experiments are given in ref.[25]. The values of the various parameters in the constitutive model for granite are listed in Table 1.
Fig.11 and Fig.12 show the comparisons of the crack patterns between the numerical predictions from the present model and the ones observed experimentally in the square granite samples. It can be seen from Fig.11 and Fig.12 that good agreements are obtained. It should be mentioned here that No.1 sample receives less damage due to less RDX explosive used in the test, and that No.2 sample is broken up into four major pieces due to more RDX explosive employed in the experiment. Severe damages and small cracks are induced in the vicinity of the boreholes of both samples, as can be seen clearly from Fig.11(b) and Fig.12(b).
3. Conclusions
A numerical study on the borehole blasting-induced fractures in rocks is conducted in this paper, using a dynamic constitutive model developed previously for concrete. Two kinds of granite rocks are simulated numerically, one in the cylindrical form and the other in the square form. The numerical results are compared with the corresponding experiments. Main conclusions can be drawn as follows.
(1) The crack patterns predicted numerically from the present model are found to be in good agreement with the experimental observations, both in cylindrical and square granite samples subjected to borehole blasting loading.
(2) The peak pressures predicted numerically based on the present model are found to be in good agreement with the test data.
(3) Crack pattern observed experimentally in the rock sample is mainly caused by the tensile stress, while the smaller cracks in the vicinity of the borehole are created largely by compression/shear stress.
(4) The consistency between the numerical results and the experimental observations demonstrates the accuracy and reliability of the present model. Thus the model can be used in the numerical simulations of the response and the failure of rocks under blasting loading.
-
图 2 不同混合物的BACF:(a) 不同温度下4种键的BACF,(b) 4000 K下的分子动力学模拟快照,(c) 不同混合物在4000 K、176 GPa下的BACF[13]
Figure 2. BACF of different mixtures: (a) BACF of four types of bond at different temperatures; (b) a snapshot of the molecular dynamics simulation at 4000 K; (c) BACF of different mixtures at 4000 K and 176 GPa[13]
图 6 (a)激光脉冲诱导液氨样品激波压缩实验装置示意图,(b) VISAR信号和 (c) SOP数据以及提取的速度和温度测量值,(d) 纯液态NH3样品的拉曼光谱,(e) NH3沿Hugoniot(黑色方块)的直流电导率[35]
Figure 6. (a) Schematic experimental setup of the laser pulse inducing shock compression in the liquid ammonia sample; (b) VISAR signal and (c) SOP data together with the extracted velocity and temperature measurements; (d) Raman spectrum of the sample indicative of pure liquid NH3; (e) calculated DC electrical conductivity of NH3 along the Hugoniot (black square)[35]
-
[1] BORUCKI W J. Kepler mission: development and overview [J]. Reports on Progress in Physics, 2016, 79(3): 036901. doi: 10.1088/0034-4885/79/3/036901 [2] ROSS M. The ice layer in Uranus and Neptune: diamonds in the sky? [J]. Nature, 1981, 292(5822): 435–436. doi: 10.1038/292435a0 [3] NELLIS W J, HOLMES N C, MITCHELL A C, et al. Equation of state and electrical conductivity of “synthetic Uranus”, a mixture of water, ammonia, and isopropanol, at shock pressure up to 200 GPa (2 Mbar) [J]. The Journal of Chemical Physics, 1997, 107(21): 9096–9100. [4] STANLEY S, BLOXHAM J. Numerical dynamo models of Uranus’ and Neptune’s magnetic fields [J]. Icarus, 2006, 184(2): 556–572. doi: 10.1016/j.icarus.2006.05.005 [5] REDMER R, MATTSSON T R, NETTELMANN N, et al. The phase diagram of water and the magnetic fields of Uranus and Neptune [J]. Icarus, 2011, 211(1): 798–803. doi: 10.1016/j.icarus.2010.08.008 [6] NETTELMANN N, WANG K, FORTNEY J J, et al. Uranus evolution models with simple thermal boundary layers [J]. Icarus, 2016, 275: 107–116. doi: 10.1016/j.icarus.2016.04.008 [7] BETHKENHAGEN M, MEYER E R, HAMEL S, et al. Planetary ices and the linear mixing approximation [J]. The Astrophysical Journal, 2017, 848(1): 67. doi: 10.3847/1538-4357/aa8b14 [8] MILLOT M, COPPARI F, RYGG J R, et al. Nanosecond X-ray diffraction of shock-compressed superionic water ice [J]. Nature, 2019, 569(7755): 251–255. doi: 10.1038/s41586-019-1114-6 [9] KRAUS D, RAVASIO A, GAUTHIER M, et al. Nanosecond formation of diamond and lonsdaleite by shock compression of graphite [J]. Nature Communications, 2016, 7(1): 10970. doi: 10.1038/ncomms10970 [10] KRAUS D, VORBERGER J, PAK A, et al. Formation of diamonds in laser-compressed hydrocarbons at planetary interior conditions [J]. Nature Astronomy, 2017, 1(9): 606–611. doi: 10.1038/s41550-017-0219-9 [11] KRAUS D, HARTLEY N J, FRYDRYCH S, et al. High-pressure chemistry of hydrocarbons relevant to planetary interiors and inertial confinement fusion [J]. Physics of Plasmas, 2018, 25(5): 056313. doi: 10.1063/1.5017908 [12] STANLEY S, BLOXHAM J. Convective-region geometry as the cause of Uranus’ and Neptune’s unusual magnetic fields [J]. Nature, 2004, 428(6979): 151–153. doi: 10.1038/nature02376 [13] CHAU R, HAMEL S, NELLIS W J. Chemical processes in the deep interior of Uranus [J]. Nature Communications, 2011, 2(1): 203. doi: 10.1038/ncomms1198 [14] CHEN B, ZENG Q Y, YU X X, et al. Three-step formation of diamonds in shock-compressed hydrocarbons: decomposition, species separation, and nucleation [EB/OL]. (2022-08-03)[2023-08-18]. https://arxiv.org/abs/2208.01830v1. [15] LIU C, GAO H, WANG Y, et al. Multiple superionic states in helium-water compounds [J]. Nature Physics, 2019, 15(10): 1065–1070. doi: 10.1038/s41567-019-0568-7 [16] GAO H, LIU C, HERMANN A, et al. Coexistence of plastic and partially diffusive phases in a helium-methane compound [J]. National Science Review, 2020, 7(10): 1540–1547. doi: 10.1093/nsr/nwaa064 [17] LIU C, GAO H, HERMANN A, et al. Plastic and superionic helium ammonia compounds under high pressure and high temperature [J]. Physical Review X, 2020, 10(2): 021007. doi: 10.1103/PhysRevX.10.021007 [18] LIU C, SHI J Y, GAO H, et al. Mixed coordination silica at megabar pressure [J]. Physical Review Letters, 2021, 126(3): 035701. doi: 10.1103/PhysRevLett.126.035701 [19] GAO H, LIU C, SHI J Y, et al. Superionic silica-water and silica-hydrogen compounds in the deep interiors of Uranus and Neptune [J]. Physical Review Letters, 2022, 128(3): 035702. doi: 10.1103/PhysRevLett.128.035702 [20] HUANG T H, LIU C, WANG J J, et al. Metallic aluminum suboxides with ultrahigh electrical conductivity at high pressure [J]. Research, 2022, 2022: 9798758. doi: 10.34133/2022/9798758 [21] PAN S N, HUANG T H, VAZAN A, et al. Magnesium oxide-water compounds at megabar pressure and implications on planetary interiors [J]. Nature Communications, 2023, 14(1): 1165. doi: 10.1038/s41467-023-36802-8 [22] SHI J M, CUI W W, HAO J, et al. Formation of ammonia-helium compounds at high pressure [J]. Nature Communications, 2020, 11(1): 3164. doi: 10.1038/s41467-020-16835-z [23] ZHANG P, SHI J M, CUI W W, et al. Formation of NH3-Xe compound at the extreme condition of planetary interiors [J]. Physical Review B, 2022, 105(21): 214109. doi: 10.1103/PhysRevB.105.214109 [24] ZHANG J R, LV J, LI H F, et al. Rare helium-bearing compound FeO2 He stabilized at deep-earth conditions [J]. Physical Review Letters, 2018, 121(25): 255703. doi: 10.1103/PhysRevLett.121.255703 [25] BENEDETTI L R, NGUYEN J H, CALDWELL W A, et al. Dissociation of CH4 at high pressures and temperatures: diamond formation in giant planet interiors? [J]. Science, 1999, 286(5437): 100–102. doi: 10.1126/science.286.5437.100 [26] HIRAI H, KONAGAI K, KAWAMURA T, et al. Polymerization and diamond formation from melting methane and their implications in ice layer of giant planets [J]. Physics of the Earth and Planetary Interiors, 2009, 174(1): 242–246. doi: 10.1016/j.pepi.2008.06.011 [27] ZERR A, SERGHIOU G, BOEHLER R, et al. Decomposition of alkanes at high pressures and temperatures [J]. High Pressure Research, 2006, 26(1): 23–32. doi: 10.1080/08957950600608931 [28] ANCILOTTO F, CHIAROTTI G L, SCANDOLO S, et al. Dissociation of methane into hydrocarbons at extreme (planetary) pressure and temperature [J]. Science, 1997, 275(5304): 1288–1290. doi: 10.1126/science.275.5304.1288 [29] GAO G Y, OGANOV A R, MA Y M, et al. Dissociation of methane under high pressure [J]. The Journal of Chemical Physics, 2010, 133(14): 144508. doi: 10.1063/1.3488102 [30] LOBANOV S S, CHEN P N, CHEN X J, et al. Carbon precipitation from heavy hydrocarbon fluid in deep planetary interiors [J]. Nature Communications, 2013, 4(1): 2446. doi: 10.1038/ncomms3868 [31] NETTELMANN N, HELLED R, FORTNEY J J, et al. New indication for a dichotomy in the interior structure of Uranus and Neptune from the application of modified shape and rotation data [J]. Planetary and Space Science, 2013, 77: 143–151. doi: 10.1016/j.pss.2012.06.019 [32] LEE M S, SCANDOLO S. Mixtures of planetary ices at extreme conditions [J]. Nature Communications, 2011, 2(1): 185. doi: 10.1038/ncomms1184 [33] KADOBAYASHI H, OHNISHI S, OHFUJI H, et al. Diamond formation from methane hydrate under the internal conditions of giant icy planets [J]. Scientific Reports, 2021, 11(1): 8165. doi: 10.1038/s41598-021-87638-5 [34] NELLIS W J, HAMILTON D C, MITCHELL A C. Electrical conductivities of methane, benzene, and polybutene shock compressed to 60 GPa (600 kbar) [J]. The Journal of Chemical Physics, 2001, 115(2): 1015–1019. doi: 10.1063/1.1379537 [35] RAVASIO A, BETHKENHAGEN M, HERNANDEZ J A, et al. Metallization of shock-compressed liquid ammonia [J].Physical Review Letters, 2021, 126(2): 025003. doi: 10.1103/PhysRevLett.126.025003 [36] CELLIERS P M, BRADLEY D K, COLLINS G W, et al. Line-imaging velocimeter for shock diagnostics at the Omega laser facility [J]. Review of Scientific Instruments, 2004, 75(11): 4916–4929. doi: 10.1063/1.1807008 [37] MILLER J E, BOEHLY T R, MELCHIOR A, et al. Streaked optical pyrometer system for laser-driven shock-wave experiments on Omega [J]. Review of Scientific Instruments, 2007, 78(3): 034903. doi: 10.1063/1.2712189 [38] BARRIOS M A, HICKS D G, BOEHLY T R, et al. High-precision measurements of the equation of state of hydrocarbons at 1–10 Mbar using laser-driven shock waves [J]. Physics of Plasmas, 2010, 17(5): 056307. doi: 10.1063/1.3358144 [39] BARRIOS M A, BOEHLY T R, HICKS D G, et al. Precision equation-of-state measurements on National Ignition Facility ablator materials from 1 to 12 Mbar using laser-driven shock waves [J]. Journal of Applied Physics, 2012, 111(9): 093515. doi: 10.1063/1.4712050 [40] LÜTGERT J, VORBERGER J, HARTLEY N J, et al. Measuring the structure and equation of state of polyethylene terephthalate at megabar pressures [J]. Scientific Reports, 2021, 11(1): 12883. doi: 10.1038/S41598-021-91769-0 [41] GORMAN M G, BRIGGS R, MCBRIDE E E, et al. Direct observation of melting in shock-compressed bismuth with femtosecond X-ray diffraction [J]. Physical Review Letters, 2015, 115(9): 095701. doi: 10.1103/PhysRevLett.115.095701 [42] HE Z Y, RÖDEL M, LÜTGERT J, et al. Diamond formation kinetics in shock-compressed C-H-O samples recorded by small-angle X-ray scattering and X-ray diffraction [J]. Science Advances, 2022, 8(35): eabo0617. doi: 10.1126/sciadv.abo0617 [43] 李俊, 陈小辉, 吴强, 等. 基于原位X射线衍射技术的动态晶格响应测量方法研究 [J]. 物理学报, 2017, 66(10): 136101. doi: 10.7498/aps.66.136101LI J, CHEN X H, WU Q, et al. Experimental investigation on dynamic lattice response by in-situ Xray diffraction method [J]. Acta Physica Sinica, 2017, 66(10): 136101. doi: 10.7498/aps.66.136101 [44] KRAUS R G, HEMLEY R J, ALI S J, et al. Measuring the melting curve of iron at super-Earth core conditions [J].Science, 2022, 375(6577): 202–205. doi: 10.1126/science.abm1472 [45] 陈小辉, 谭伯仲, 薛桃, 等. 高压高应变率加载下多晶相变的原位X射线衍射 [J]. 物理学报, 2020, 69(24): 246201. doi: 10.7498/aps.69.20200929CHEN X H, TAN B Z, XUE T, et al. In situ observation of phase transition in polycrystalline under high-pressure high-strain-rate shock compression by X-ray diffraction [J]. Acta Physica Sinica, 2020, 69(24): 246201. doi: 10.7498/aps.69.20200929 [46] DUNAEVA A N, ANTSYSHKIN D V, KUSKOV O L. Phase diagram of H2O: thermodynamic functions of the phase transitions of high-pressure ices [J]. Solar System Research, 2010, 44(3): 202–222. doi: 10.1134/S0038094610030044 [47] BARTELS-RAUSCH T, BERGERON V, CARTWRIGHT J H E, et al. Ice structures, patterns, and processes: a view across the icefields [J]. Reviews of Modern Physics, 2012, 84(2): 885–944. doi: 10.1103/RevModPhys.84.885 [48] GONCHAROV A F, STRUZHKIN V V, SOMAYAZULU M S, et al. Compression of ice to 210 gigapascals: infrared evidence for a symmetric hydrogen-bonded phase [J]. Science, 1996, 273(5272): 218–220. doi: 10.1126/science.273.5272.218 [49] LOUBEYRE P, LETOULLEC R, WOLANIN E, et al. Modulated phases and proton centring in ice observed by X-ray diffraction up to 170 GPa [J]. Nature, 1999, 397(6719): 503–506. doi: 10.1038/17300 [50] BENOIT M, BERNASCONI M, FOCHER P, et al. New high-pressure phase of ice [J]. Physical Review Letters, 1996, 76(16): 2934–2936. doi: 10.1103/PhysRevLett.76.2934 [51] CAVAZZONI C, CHIAROTTI G L, SCANDOLO S, et al. Superionic and metallic states of water and ammonia at giant planet conditions [J]. Science, 1999, 283(5398): 44–46. doi: 10.1126/science.283.5398.44 [52] SUN J M, CLARK B K, TORQUATO S, et al. The phase diagram of high-pressure superionic ice [J]. Nature Communications, 2015, 6(1): 8156. doi: 10.1038/ncomms9156 [53] FRENCH M, DESJARLAIS M P, REDMER R. Ab initio calculation of thermodynamic potentials and entropies for superionic water [J]. Physical Review E, 2016, 93(2): 022140. doi: 10.1103/PhysRevE.93.022140 [54] HERNANDEZ J A, CARACAS R. Superionic-superionic phase transitions in body-centered cubic H2O ice [J]. Physical Review Letters, 2016, 117(13): 135503. doi: 10.1103/PhysRevLett.117.135503 [55] KNUDSON M D, DESJARLAIS M P, LEMKE R W, et al. Probing the interiors of the ice giants: shock compression of water to 700 GPa and 3.8 g/cm³ [J]. Physical Review Letters, 2012, 108(9): 091102. doi: 10.1103/PhysRevLett.108.091102 [56] CELLIERS P M, COLLINS G W, HICKS D G, et al. Electronic conduction in shock-compressed water [J]. Physics of Plasmas, 2004, 11(8): L41–L44. doi: 10.1063/1.1758944 [57] MILLOT M, HAMEL S, RYGG J R, et al. Experimental evidence for superionic water ice using shock compression [J]. Nature Physics, 2018, 14(3): 297–302. doi: 10.1038/s41567-017-0017-4 [58] GLATTER V O, KRATKY O. Small angle X-ray scattering [M]. London: Academic Press, 1982. [59] MCWILLIAMS R S, DALTON D A, MAHMOOD M F, et al. Optical properties of fluid hydrogen at the transition to a conducting state [J]. Physical Review Letters, 2016, 116(25): 255501. doi: 10.1103/PhysRevLett.116.255501 [60] WEIR S T, MITCHELL A C, NELLIS W J. Metallization of fluid molecular hydrogen at 140 GPa (1.4 Mbar) [J]. Physical Review Letters, 1996, 76(11): 1860–1863. doi: 10.1103/PhysRevLett.76.1860 [61] CELLIERS P M, MILLOT M, BRYGOO S, et al. Insulator-metal transition in dense fluid deuterium [J]. Science, 2018, 361(6403): 677–682. doi: 10.1126/science.aat0970 [62] KNUDSON M D, DESJARLAIS M P, BECKER A, et al. Direct observation of an abrupt insulator-to-metal transition in dense liquid deuterium [J]. Science, 2015, 348(6242): 1455–1460. doi: 10.1126/science.aaa7471 [63] FORTOV V E, ILKAEV R I, ARININ V A, et al. Phase transition in a strongly nonideal deuterium plasma generated by quasi-isentropical compression at megabar pressures [J]. Physical Review Letters, 2007, 99(18): 185001. doi: 10.1103/PhysRevLett.99.185001 [64] MOCHALIN V N, SHENDEROVA O, HO D, et al. The properties and applications of nanodiamonds [J]. Nature Nanotechnology, 2012, 7(1): 11–23. doi: 10.1038/nnano.2011.209 [65] MARSHALL M C, GORMAN M M, POLSIN D N, et al. Diamond formation in double-shocked epoxy to 150 GPa [J]. Journal of Applied Physics, 2022, 131(8): 085904. doi: 10.1063/5.0082237 [66] MONSHI A, FOROUGHI M R, MONSHI M R. Modified Scherrer equation to estimate more accurately nano-crystallite size using XRD [J]. World Journal of Nano Science and Engineering, 2012, 2(3): 154–160. doi: 10.4236/wjnse.2012.23020 [67] WATKINS E B, HUBER R C, CHILDS C M, et al. Diamond and methane formation from the chemical decomposition of polyethylene at high pressures and temperatures [J]. Scientific Reports, 2022, 12(1): 631. doi: 10.1038/s41598-021-04206-7 [68] HARTLEY N J, BROWN S, COWAN T E, et al. Evidence for crystalline structure in dynamically-compressed polyethylene up to 200 GPa [J]. Scientific Reports, 2019, 9(1): 4196. doi: 10.1038/s41598-019-40782-5 -









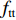
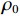
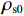
 DownLoad:
DownLoad:
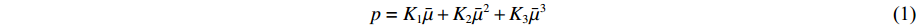
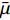


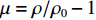
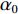
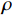
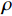
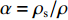
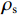
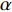
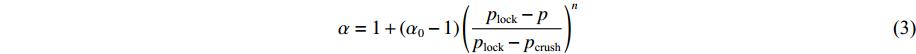
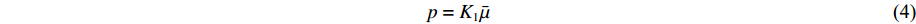
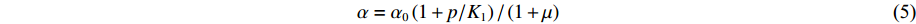

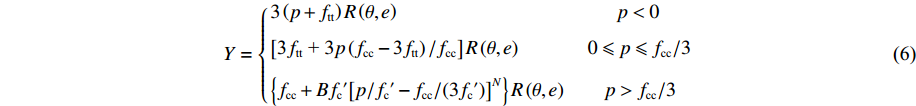
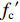
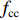


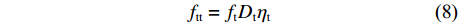
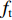
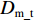
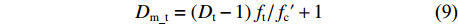
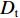
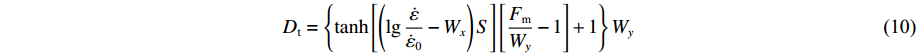
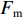
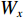
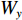

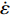
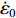
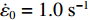
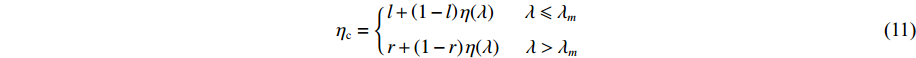
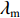
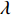
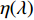
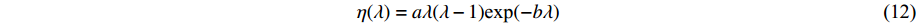
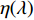
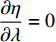
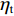
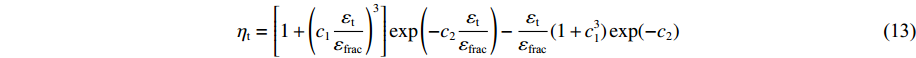
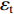
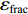
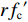
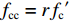
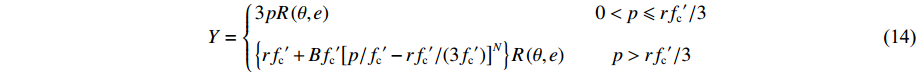
 DownLoad:
DownLoad:



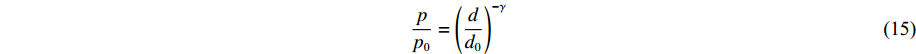







 下载:
下载: