基于原子力显微镜的压痕技术及其应用

王晓萌; 高扬

doi:10.11858/gywlxb.20230694

基于原子力显微镜的压痕技术及其应用

doi: 10.11858/gywlxb.20230694

王晓萌^1,,
高扬^{1, 2,*, ,}

1.
浙江大学航空航天学院, 交叉力学中心, 浙江杭州　310027
2.
浙江省软体机器人与智能器件研究重点实验室, 浙江杭州　310027

基金项目: 国家自然科学基金（12102386，12192211）

详细信息

作者简介:
王晓萌（1999－），男，硕士研究生，主要从事二维材料力学性质研究. E-mail：22124005@zju.edu.cn

通讯作者:
高　扬（1989－），男，博士，研究员，主要从事微纳米力学及二维材料研究. E-mail：ygao96@zju.edu.cn

中图分类号: O521.3; O521.2
计量
- 文章访问数: 319
- HTML全文浏览量: 120
- PDF下载量: 59
出版历程
- 收稿日期: 2023-07-20
- 修回日期: 2023-09-04
- 网络出版日期: 2023-11-20
- 刊出日期: 2023-12-15

Atomic Force Microscope Based Indentation Techniques and Their Applications

WANG Xiaomeng^1
,,
GAO Yang^{1, 2,*
, ,}

1.
Center for X-Mechanics, School of Aeronautics and Astronautics, Zhejiang University, Hangzhou 310027, Zhejiang, China
2.
Key Laboratory of Soft Machines and Smart Devices of Zhejiang Province, Hangzhou 310027, Zhejiang, China

摘要

摘要: 基于原子力显微镜的压痕技术具有高分辨、高精度等优点，是表征材料力学性质的重要手段，在材料科学、纳米科学、生物力学等领域具有广泛应用。首先，介绍了原子力显微镜及相关压痕技术的基本工作原理；然后，回顾总结了基于原子力显微镜的压痕技术在低维材料、软材料等领域的应用；接着，简要综述了原子力显微镜技术在二维材料高压相变方面的最新研究进展，着重介绍基于原子力显微镜的新型埃压痕技术，该技术可施加亚纳米级压痕深度，有效表征与调控二维材料的层间耦合作用；最后，对基于原子力显微镜的压痕技术在未来的发展和应用进行了展望。
- 原子力显微镜 /
- 压痕技术 /
- 二维材料 /
- 高压相变
Abstract: Indentation techniques based on atomic force microscopy (AFM) have been widely used in characterizing the mechanical properties of various types of materials, due to their high lateral resolution and vertical precision. This review article begins with a concise introduction to the fundamental principles of AFM and related indentation techniques. Subsequently, it extensively discusses and reviews the applications of AFM-based indentation techniques in measuring the mechanical properties of low-dimensional materials, biological materials, etc. This article also reviews the latest progress of the applications of AFM in the research of high-pressure phase transition in two-dimensional materials. Particularly, we introduce a novel angstrom-indentation technique, which enables atomic-level deformation on the samples. Angstrom-indentation allows for highly effective characterization and tuning of the interlayer coupling in two-dimensional materials. Finally, we make an outlook on the development of AFM-based indentation techniques.
- atomic force microscopy /
- indentation /
- two-dimensional materials /
- high-pressure phase transition

HTML全文

1. 引言

自1996年German-French Research Institute Saint Louis (ISL)实验室第一次提出横向效应增强型侵彻体(Penetrator with Enhanced Lateral Effect, PELE)概念以来, 国内外学者对PELE弹丸的作用过程进行了广泛研究。在PELE弹丸壳体断裂的机理方面, Kesberg等人^[1]认为壳体侵彻目标的内核因密度较低而被压缩并沿径向膨胀, 导致壳体内壁压力剧增至吉帕级, 弹丸穿透目标后能量瞬间释放, 导致壳体破裂成大量破片。Paulus等人^[2]结合Recht-Ipson模型^[3]和Mott模型^[4]计算出弹体的剩余速度、装填物的压力以及壳体破片的径向飞散速度, 并且用弱激波理论描述了PELE弹丸壳体的断裂过程。对于PELE弹丸壳体断裂的研究, 除了可从力学和能量的角度定性地解释PELE弹丸壳体断裂过程外, 还可以从损伤断裂的角度, 具体分析其在侵彻过程中的断裂机理。

在研究材料动态拉伸断裂方面有许多模型, Seaman、Curran和Shockey^[5]从实验出发, 基于微损伤的统计性分析结果, 提出了成核与长大模型(Nucleation and Growth, NAG)。但是NAG模型计算需要大量的实验参数, 实用性比较差。封加波等人^[6-7]在NAG模型的基础上利用单孔洞近似, 从能量平衡的角度出发, 提出了简单实用的损伤度函数模型。本研究基于损伤度模型, 结合PELE弹丸侵彻过程, 将PELE弹丸壳体的膨胀断裂过程分为加速膨胀和匀速膨胀两个阶段, 推导出这两个阶段PELE弹丸壳体的损伤断裂方程, 并根据损伤断裂方程分析影响PELE弹丸横向效应的内在机理。

2. 金属壳体动态损伤模型

2.1 金属壳体的动态本构关系

PELE弹丸壳体一般为高密度金属或合金。金属或合金材料在高应变率下的变形过程比较复杂, 理论上通常把动态变形过程划分为几个阶段, 如弹性阶段、塑性阶段和失效阶段, 对不同变形阶段需要用不同的本构关系表达式进行描述。动态塑性本构方程可以写为

$\sigma=\varphi\left(e^{\mathrm{p}}, \dot{e}^{\mathrm{p}}\right)$

(1)

式中:σ为材料的动态屈服应力, e^p为径向塑性自然应变, 为径向塑性自然应变率。

根据文献[7], 金属壳体的动态本构关系可以表示为

$\sigma=\sigma_{0}+K e^{\mathrm{p}}+\eta \dot{e}^{\mathrm{p}}$

(2)

式中:σ₀为静态屈服强度, K为材料强化系数, η为材料黏度系数。

对于柱壳结构有

$e^{\mathrm{p}}=\ln \left(\frac{R(t)}{R_{0}}\right)$

(3)

$\dot{e}^{\mathrm{p}}=u(t) / R(t)$

(4)

$\varepsilon^{\mathrm{p}}=\frac{R(t)-R_{0}}{R_{0}}$

(5)

式中:R₀为壳体初始半径, R(t)为壳体实时半径, R₀、R(t)均是壳体内径和外径的平均值, ε^p为径向塑性应变, u(t)为壳体径向膨胀速度。结合(3)式和(5)式可以得到e^p与ε^p的关系

$e^{\mathrm{p}}=\ln \left(1+\varepsilon^{\mathrm{p}}\right)$

(6)

将(6)式代入(2)式, 可以将动态本构关系改写成以下形式

$\sigma=\sigma_{0}+K \ln \left(1+\varepsilon^{\mathrm{P}}\right)+\eta \dot{e}^{\mathrm{p}}$

(7)

2.2 金属壳体的动态损伤模型

本研究所采用的断裂准则建立在封加波提出的损伤度模型的基础上。金属材料的损伤度包括在应力波作用下材料初始损伤缺陷及其发展, 以及新产生的损伤缺陷及其发展, 而且材料的损伤度具有累积效应。在PELE弹丸壳体动态断裂的过程中, 为了考虑材料的损伤累积效应, 引入损伤变量D(t), D(t)定义为材料某一截面上微孔洞所占的面积比。参照文献[6], 有如下形式的方程

$\frac{\mathrm{d} D}{\mathrm{d} t}=\frac{\left[(1-D)\left(\sigma-\sigma_{0}\right)\right]^{2}}{\eta K}$

(8)

求解方程(8)式需要知道σ(或ε^p和随时间变化的规律。从(4)式和(5)式看出, 需要知道壳体膨胀的实时半径R(t)和壳体径向膨胀速度u(t)的函数形式。在处理实际问题时, 很难求出解析表达式, 一般需要结合实验结果求解。

3. PELE弹丸壳体膨胀规律

3.1 PELE作用机理

PELE弹丸是一种基于物理效应、不需要装填炸药和引信的新概念弹药, 主要用于防空、反导、城市作战等领域。PELE弹丸由壳体和内核组成, 壳体一般为密度较大的金属材料, 内核是密度及强度比较低的金属或聚合物。高密度金属壳体对靶板具有良好的侵彻性能, 而内核材料在侵彻过程中被封闭压缩, 巨大的压力在内核中迅速积聚, 弹丸穿透靶板后能量瞬间释放导致外壳破裂解体。

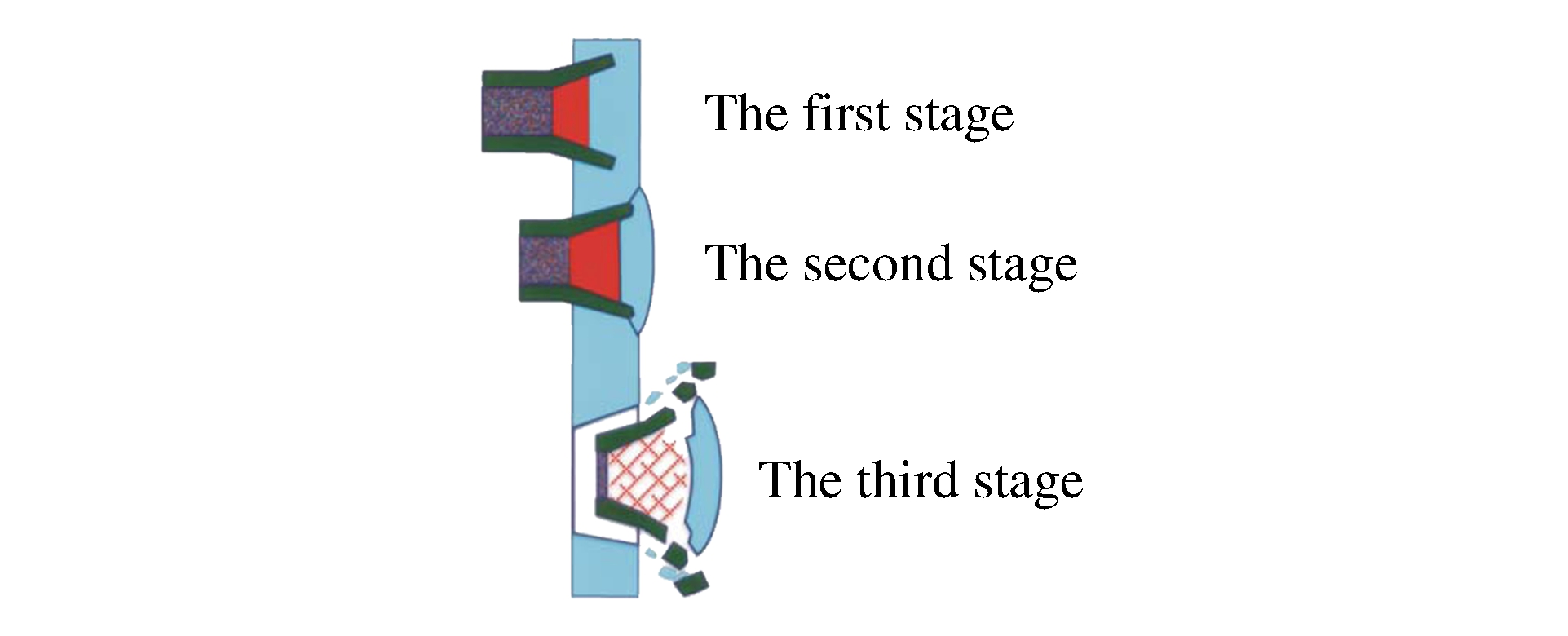
PELE的横向增强效应作用过程分为3个主要时段和一个后效作用时段^[1]。如图 1所示, 第1时段, 当弹丸击中目标后, 外层高密度壳体侵彻目标, 内核因材料强度低而无法侵彻, 将会在靶前停滞而被压缩; 第2时段, 封闭压缩过程中产生的高压将使内核材料沿径向膨胀, 导致壳体内壁压力急剧增加, 可达到数吉帕; 第3时段, 当应力应变达到壳体的破坏极限时, 出靶后的卸载应力波致使外层弹体破裂成大小不同的破片。后效作用时段则是壳体破片的径向飞散以及内核和塞块间继续相互作用的过程。

图 1 PELE的3个阶段

Figure 1. Three stages of penetration process of the PELE

下载: 全尺寸图片幻灯片

3.2 PELE侵彻过程数值计算

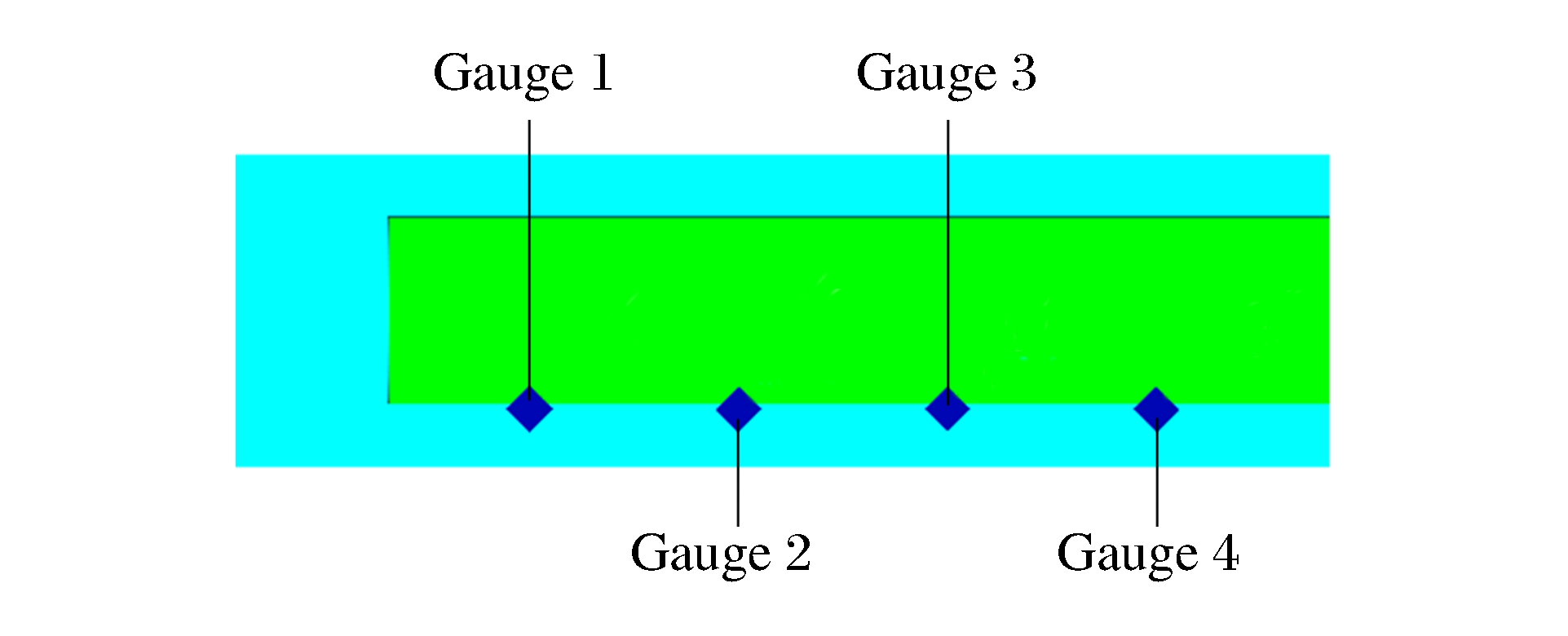
图 2给出了PELE弹丸计算模型, 其中壳体材料为35CrMnSi, 长度为105 mm, 外径为30 mm, 内径为18 mm, 弹底部厚为15 mm; 内核材料为聚乙烯, 长度为90 mm, 直径为18 mm; 靶板材料为装甲钢, 厚度为8 mm。网格平均尺寸为壳体1 mm, 内核1.2 mm, 靶板采用变步长网格(0.5~10 mm)以提高计算效率。为了获得侵彻过程中的时间历程参数, 在弹丸模型的壳体中心线沿轴向均匀分布4个观测点(Gauge点), 每个观测点间隔20 mm, 如图 2所示。

图 2 PELE弹丸计算模型

Figure 2. Calculation model of the PELE

下载: 全尺寸图片幻灯片

表 1、表 2分别为材料模型和材料参数。数值计算采用拉格朗日方法, 材料均采用侵蚀算法, 靶板采用材料应变失效模型, 壳体使用有效应力失效模型。采用Stochastic模型^[8]模拟壳体随机断裂, 其中材料弱化点的破坏概率在[0, 1]范围为

表 1 材料模型

Table 1. Material model

Component	Material species	Equation of state	Strength model	Invalidation model
Shell	35CrMnSi	Linear	Johnson-Cook	Principal stress
Core	Polyethylene	Shock	von-Mises	-
Target plate	RHA	Shock	von-Mises	Material strain

下载: 导出CSV

| 显示表格

表 2 材料参数

Table 2. Material parameters

Material	ρ/(g/cm³)	E/(GPa)	σ/(MPa)	G/(GPa)	ν
35CrMnSi	7.8	210	1 270	81	0.33
Polyethylene	0.94	1.5	0.26	0.55	0.45
RHA	7.8	171	1400	64.1	0.33

下载: 导出CSV

| 显示表格

$P=1-\exp \left(-c \mathrm{e}^{\gamma \varepsilon} / \gamma\right)$

(9)

式中:P为应变为ε时的网格破坏概率, c、γ取决于材料性质, 根据文献[8], 取c=0.467, γ=10。

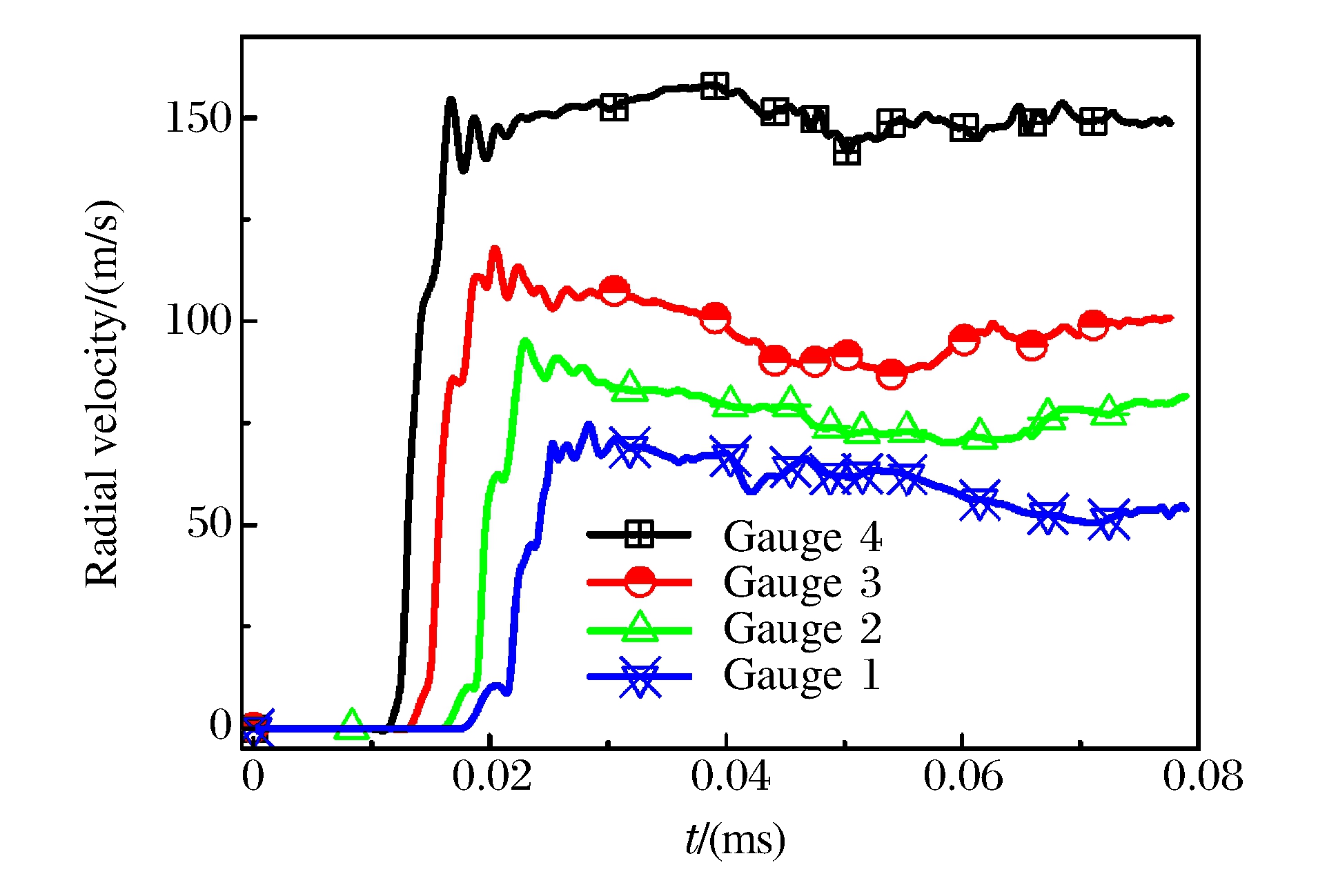
PELE弹丸以700 m/s的着靶速度垂直侵彻8 mm厚的装甲钢靶板。壳体中Gauge点沿壳体径向速度变化曲线如图 3所示。从图 3中看出, PELE侵彻过程第2时段, 壳体径向先经历短暂加速膨胀阶段, 膨胀速度达到一个较大值; PELE侵彻过程第3时段, 壳体膨胀速度小幅震荡并趋于稳定。

图 3 PELE弹丸壳体Gauge点的径向速度

Figure 3. The radical velocity of gauge points in the PELE

下载: 全尺寸图片幻灯片

3.3 实验过程与结果

PELE实验弹丸参数:外径为30 mm, 内径为18 mm, 长度为105 mm; 壳体材料采用35CrMnSi合金钢, 内核为聚乙烯, 长度为90 mm, 靶板为装甲钢, 厚度为8 mm; 装配全弹质量为350 g。

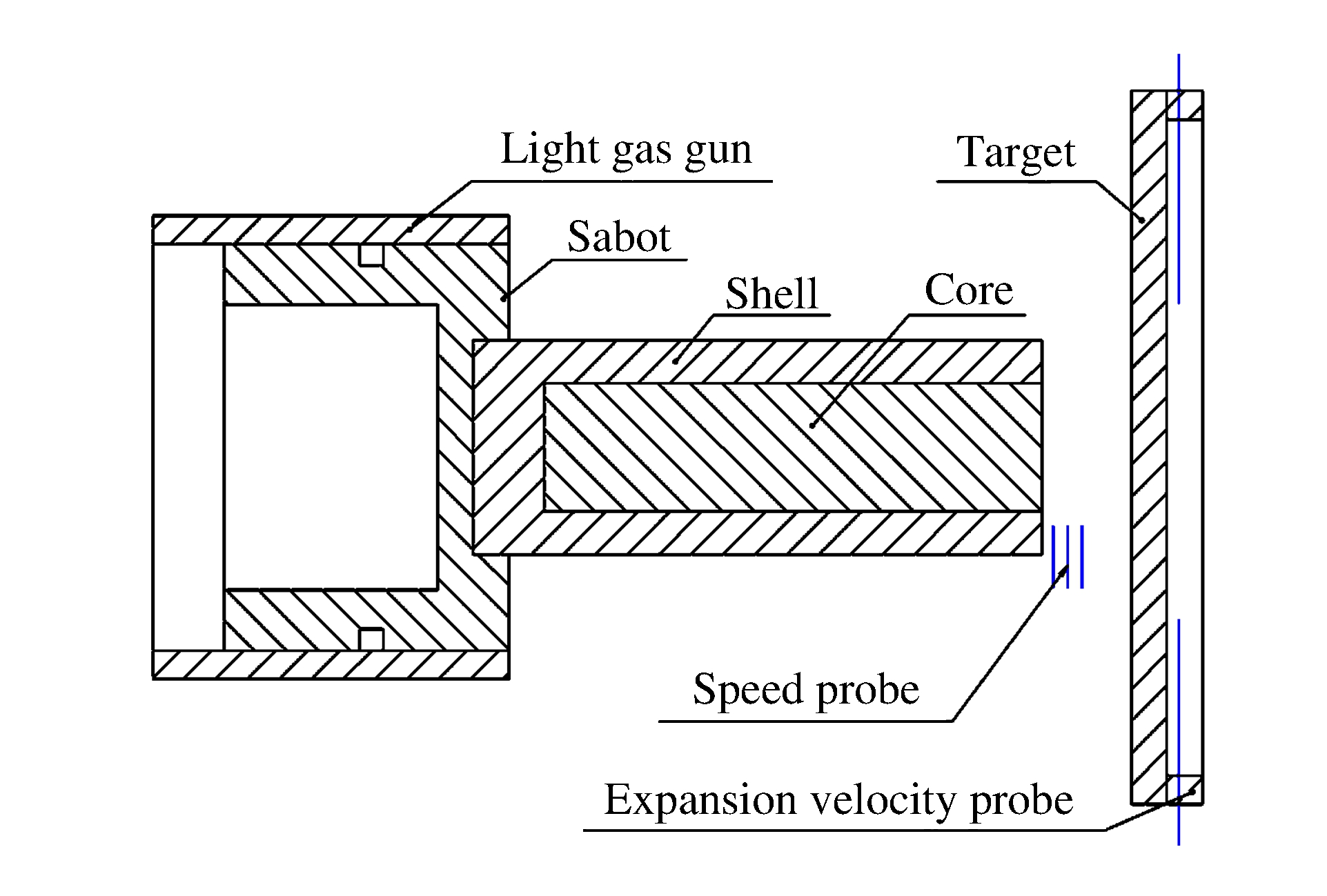
实验弹采用轻气炮发射。弹丸着靶和出靶分别设置了测速点探针, 以测量着靶速度和弹丸穿透靶板的时间。为了获得弹丸头部出靶时的膨胀速度, 设计了一套环形电探针测量装置。实验装置示意图如图 4所示, 实验装置各部分如图 5所示。

图 4 实验装置示意图

Figure 4. Experimental setup

下载: 全尺寸图片幻灯片

图 5 实验装置

Figure 5. Experimental device

下载: 全尺寸图片幻灯片

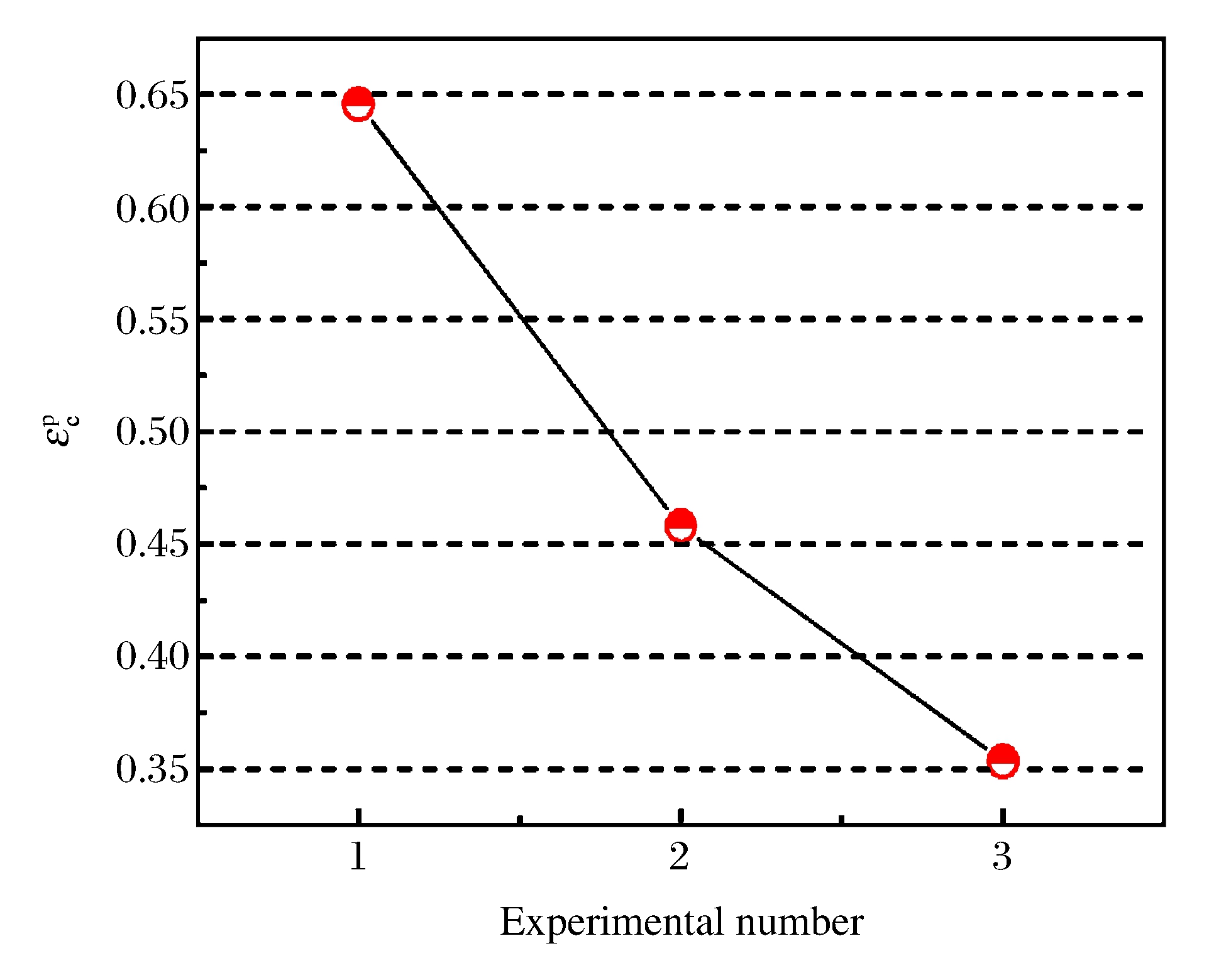
表 3分别统计了3发有效实验的弹丸着靶速度、弹丸头部从着靶到出靶的穿靶时间, 以及出靶时弹丸头部的径向速度。应该指出的是, 由于弹丸穿靶时间极短, 弹丸出靶时头部的径向速度比较大, 探针测试存在一定的误差。

表 3 着靶速度与穿靶时间统计

Table 3. Statistics of the impact velocity and the penetrating time

Experimental No.	Velocity/(m/s)	Time/(μs)	Radical velocity/(m/s)
1	643	8.4	127
2	694	7.2	142
3	762	6.5	153

下载: 导出CSV

| 显示表格

3.4 PELE侵彻过程壳体的膨胀规律

壳体膨胀断裂主要处于PELE侵彻过程的第2时段和第3时段。壳体动态断裂过程可以描述为:应力波从内核传入壳体内壁, 壳壁开始加速膨胀, 在应力波多次反射效应下膨胀速度振荡衰减, 直至最终趋于平稳。其衰减规律基本上按余弦函数变化^[9-10], 所以径向速度公式可以写为

$u(t)=\left\{\begin{array}{ll} C t & t_{0} \leqslant t \lt t_{1} \\ A \mathrm{e}^{-\lambda t} \cos (\psi t)+u_{0} & t_{1} \leqslant t \lt t_{c} \end{array}\right.$

(10)

式中:A、ψ、C、λ为与总体模型有关的参数, u₀为稳态时的径向速度值。t₀为应力波传到壳体外壁的时刻, t₁为壳体膨胀加速结束的时刻, t_c为壳体发生贯穿性断裂的时刻。

根据侵彻过程壳体的膨胀规律, 可以将PELE壳体断裂过程分为壳体加速膨胀阶段和壳体匀速膨胀阶段。调整数值模拟中弹丸的着靶速度, 使其工况与3发实验相吻合, 对比数值模拟结果与实验结果发现, PELE壳体加速膨胀的时间与壳体从着靶到出靶的时间相吻合。可以看出当壳体处于靶板内时, 壳体处于加速状态; 当壳体出靶后, 壳体匀速膨胀。

4. PELE弹丸壳体断裂过程求解

根据PELE侵彻过程壳体的膨胀规律, 将PELE壳体断裂过程分为两个阶段:壳体加速膨胀阶段和壳体匀速膨胀阶段。

第1阶段:壳体加速膨胀阶段。假定自然应变率可以近似看作常数

$\dot{e}^{\mathrm{p}}=u(t) / R(t)=c$

(11)

另外

$\mathrm{d} t=\mathrm{d} e^{\mathrm{p}} / \dot{e}^{\mathrm{p}}$

(12)

对(12)式积分得

$t_{1}-t_{0}=e_{1}^{\mathrm{p}} / \dot{e}^{\mathrm{p}}$

(13)

将(7)代入(8), 在[t₀, t₁]内积分得

$D_{1}=1-\frac{3 \omega_{1}}{\left(\omega_{1}+e_{1}^{\mathrm{p}}\right)^{3}+3 \omega_{1}-\omega_{1}^{3}}$

(14)

式中:D₁为t₁时刻的损伤度。(14)式中

$\omega_{1}=(K / \eta) \dot{e}^{\mathrm{p}}$

(15)

通过实验结果可以得到代入(13)式可以求出将代入(14)式求出D₁。

第2阶段:壳体匀速膨胀阶段。第1阶段结束时刻即为第2阶段初始时刻。依据PELE壳体膨胀规律, 给出描述壳体膨胀速度的变化规律为

$u(t)=A \mathrm{e}^{-\lambda t} \cos (\psi t)+u_{0}$

(16)

(16) 式反映应力波多次反射引起的衰减振荡, 代表了壳体膨胀速度的真实情况, 但直接代入损伤度方程难以求解。为了方便积分, 这里按照能量等效原理取均值, 假设柱壳膨胀速度u(t)等于常数

$u(t)=u_{0}$

(17)

由于

$\begin{aligned} \dot{e}^{\mathrm{p}} =u(t) / R(t) \end{aligned}$

(18)

$\begin{aligned} \dot{e}_{2}^{\mathrm{p}} & =u(t) / R_{2} \end{aligned}$

(19)

$\begin{aligned} \\ u(t) & =\mathrm{d} R(t) / \mathrm{d} t \end{aligned}$

(20)

将(5)式、(18)式、(19)式代入(20)式, 得

$\mathrm{d} t=\mathrm{d} R(t) / u(t)=\mathrm{d} \varepsilon^{\mathrm{p}} / \dot{e}_{2}^{\mathrm{p}}$

(21)

式中: 为第2阶段的初始径向塑性自然应变率, R₂为第2阶段壳体的初始半径。

将(7)式、(17)式、(21)式代入(8)式, 在[t₁, t_c]积分得

$\int_{D_{1}}^{D_{c}} \frac{\mathrm{d} D}{(1-D)^{2}}=\int_{\varepsilon_{1}^{\mathrm{p}}}^{\varepsilon_{\mathrm{c}}^{\mathrm{p}}} \frac{1}{\omega_{2}}\left[\ln \left(1+\varepsilon^{\mathrm{p}}\right)+\frac{\omega_{2}}{1+\varepsilon^{\mathrm{p}}}\right] \mathrm{d} \varepsilon^{\mathrm{p}}$

(22)

式中: 为壳体发生贯穿性破坏的损伤度。经过整理, 最终得

$\begin{array}{l} \frac{\varepsilon_{\mathrm{c}}^{\mathrm{p}}-\varepsilon_{1}^{\mathrm{p}}}{\left(1+\varepsilon_{\mathrm{c}}^{\mathrm{p}}\right)\left(1+\varepsilon_{1}^{\mathrm{p}}\right)} \omega_{2}^{2}+\left[\left(e_{\mathrm{c}}^{\mathrm{p}}\right)^{2}-\left(e_{1}^{\mathrm{p}}\right)^{2}-\frac{D_{\mathrm{c}}-D_{1}}{\left(1-D_{\mathrm{c}}\right)\left(1-D_{1}\right)}\right] \omega_{2}+ \\ \left(1+\varepsilon_{\mathrm{c}}^{\mathrm{p}}\right)\left[\left(e_{\mathrm{c}}^{\mathrm{p}}\right)^{2}-2 e_{\mathrm{c}}^{\mathrm{p}}+2\right]-\left(1+\varepsilon_{1}^{\mathrm{p}}\right)\left[\left(e_{1}^{\mathrm{p}}\right)^{2}-2 e_{1}^{\mathrm{p}}+2\right]=0 \end{array}$

(23)

式中: 为第一阶段壳体材料径向塑形应变。由(6)式知与满足以下关系式

$e_{1}^{\mathrm{p}}=\ln \left(1+\varepsilon_{1}^{\mathrm{p}}\right)$

(24)

(23) 式为PELE弹丸壳体的损伤断裂方程。第1阶段的自然应变率和第2阶段的初始半径R₂、径向速度u₀可以通过实验得到, 通过R₂和u₀可以求出而壳体材料参数σ₀、K、η和D_c均可以通过查找手册和文献获得, 最终可以得到一个确定的临界应变作为损伤断裂判据。

将(17)式、(19)式代入(21)式, 在[t₁, t_c]内积分可得壳体发生贯穿断裂的时刻t_c

$t_{\mathrm{c}}-t_{1}=R_{2}\left(\varepsilon_{\mathrm{c}}^{\mathrm{p}}-\varepsilon_{1}^{\mathrm{p}}\right) / u_{0}$

(25)

5. PELE壳体断裂实例

对于35CrMnSi, 参照文献[10], 壳体特征参数取η=-12 kPa·s, K=1.66 GPa, ω_λ=3.5 kJ/m², D₀=10^-5, D_c=0.2, σ₀=0.72 GPa。当初始半径R₀=15 mm的PELE弹丸以1 km/s速度着靶时, 结合实验数据, 得到第1阶段的自然应变率和第2阶段的初始半径R₂及径向膨胀速度u₀, 见表 4。

表 4 3个时刻膨胀断裂过程的参数

Table 4. Parameters of expansion and fracture at three time points

t/(μs)	$\dot{e}^{\mathrm{p}} /\left(10^{4} \mathrm{~s}^{-1}\right)$	R₂/(mm)	u₀/(m/s)
8.4	2.62	17.7	127
7.2	1.91	18.6	142
6.5	1.71	19.9	153

下载: 导出CSV

| 显示表格

将第2阶段的初始半径R₂及径向膨胀速度u₀代入(19)式, 得到第2阶段的初始应变率将第一阶段代入(13)式可以求出将代入(14)式求出D₁; 同时将代入(24)式求出将代入(23)式, 得到3个典型时刻临界应变如图 6所示。从图 6中可以看出, 在一定速度范围内, 随着弹丸着靶速度的增加, 壳体断裂的临界应变明显降低。

图 6 PELE壳体断裂的临界应变

Figure 6. Critical strain of the PELE

下载: 全尺寸图片幻灯片

6. 结论

(1) PELE弹丸在侵彻过程中壳体膨胀断裂可以分为加速膨胀和匀速膨胀两个阶段, 在封加波提出的损伤度模型的基础上, 得到了PELE弹丸壳体的损伤断裂方程和断裂判据。

(2) 根据PELE弹丸壳体损伤断裂方程, 计算出PELE弹丸壳体膨胀断裂过程的参数, 分析了弹丸着靶速度与壳体断裂之间的关系, 可为PELE弹丸设计提供理论指导。

致谢: 感谢胡玉涛博士和曹雷博士在公式推导和实验方面给予的帮助。

图 1 基于AFM的压痕技术的应用

Figure 1. Applications of indentation techniques based on AFM

下载: 全尺寸图片幻灯片

图 2 AFM和压痕技术

Figure 2. AFM and indentation techniques

下载: 全尺寸图片幻灯片

图 3 二维材料示意图^[12–13]

Figure 3. Schematic of 2D materials^[12–13]

下载: 全尺寸图片幻灯片

图 4 悬空二维材料的纳米压痕实验^{[3, 46, 48]}

Figure 4. Nanoindentation experiment of suspended two-dimensional materials^{[3, 46, 48]}

下载: 全尺寸图片幻灯片

图 5 悬空金属薄膜的纳米压痕实验^[11]

Figure 5. Nanoindentation experiment of suspended metal nanosheets^[11]

下载: 全尺寸图片幻灯片

图 6 埃压痕实验装置、弹性力学模型以及实验结果^[59]

Figure 6. Å-indentation experimental set-up, elastic mechanics model and experimental results^[59]

下载: 全尺寸图片幻灯片

图 7 氧化石墨烯的层间力学性质^[60]

Figure 7. Interlayer elasticity of graphene oxide^[60]

下载: 全尺寸图片幻灯片

图 8 双层石墨烯-单层金刚石相变^[71–72]

Figure 8. Bilayer graphene-monolayer diamond phase transition^[71–72]

下载: 全尺寸图片幻灯片

图 9 六方氮化硼-金刚石氮化硼相变^[81]

Figure 9. Hexagonal-to-diamond phase transition of boron nitride^[81]

下载: 全尺寸图片幻灯片

图 10 生物材料的纳米压痕实验^[92–94]

Figure 10. AFM-based indentation on biomaterials^[92–94]

下载: 全尺寸图片幻灯片

表 1 基于悬空纳米压痕技术获得的部分常见二维材料的力学性能

Table 1. Mechanical properties of some typical 2D materials by suspended nanoindentation

Materials	Elastic modulus/GPa	Strength/GPa
Monolayer graphene^[3]	1000±100	130
Monolayer boron nitride^[45]	865±73	70.5±5.5
Monolayer MoS₂^[43]	270±100	23
Multilayer MoS₂^[46]	210–370
Monolayer WSe₂^[56]	258.6±38.3	38.0±6.0
Multilayer WSe₂^[55]	167.3±6.7	12.4
Monolayer WS₂^[56]	302.4±24.1	47.0±8.6
Monolayer WTe₂^[56]	149.1±9.4	6.4±3.3
Multilayer black phosphorous^[54]	276±32.4	25

下载: 导出CSV

参考文献(94)

[1]	BINNIG G, QUATE C F, GERBER C. Atomic force microscope [J]. Physical Review Letters, 1986, 56(9): 930–933. doi: 10.1103/PhysRevLett.56.930
[2]	STYLIANOU A, KONTOMARIS S V, GRANT C, et al. Atomic force microscopy on biological materials related to pathological conditions [J]. Scanning, 2019: 8452851. doi: 10.1155/2019/8452851
[3]	LEE C, WEI X D, KYSAR J W, et al. Measurement of the elastic properties and intrinsic strength of monolayer graphene [J].Science, 2008, 321(5887): 385–388. doi: 10.1126/science.1157996
[4]	LIU K, WU J Q. Mechanical properties of two-dimensional materials and heterostructures [J]. Journal of Materials Research, 2016, 31(7): 832–844. doi: 10.1557/jmr.2015.324
[5]	AKINWANDE D, BRENNAN C J, BUNCH J S, et al. A review on mechanics and mechanical properties of 2D materials: graphene and beyond [J]. Extreme Mechanics Letters, 2017, 13: 42–77. doi: 10.1016/j.eml.2017.01.008
[6]	PAPAGEORGIOU D G, KINLOCH I A, YOUNG R J. Mechanical properties of graphene and graphene-based nanocomposites [J]. Progress in Materials Science, 2017, 90: 75–127. doi: 10.1016/j.pmatsci.2017.07.004
[7]	CAO G X, GAO H J. Mechanical properties characterization of two-dimensional materials via nanoindentation experiments [J].Progress in Materials Science, 2019, 103: 558–595. doi: 10.1016/j.pmatsci.2019.03.002
[8]	LI B W, YIN J, LIU X F, et al. Probing van der Waals interactions at two-dimensional heterointerfaces [J]. Nature Nanotechnology, 2019, 14(6): 567–572. doi: 10.1038/s41565-019-0405-2
[9]	YU Q, ZHANG J Y, LI J, et al. Strong, ductile, and tough nanocrystal-assembled freestanding gold nanosheets [J]. Nano Letters, 2022, 22(2): 822–829. doi: 10.1021/acs.nanolett.1c04553
[10]	QIN H L, JIN J, PENG X S, et al. Mechanical properties of free-standing single layers of metallic nanocrystals [J]. Journal of Materials Chemistry, 2010, 20(5): 858–861. doi: 10.1039/B923745N
[11]	PARK M, YU Q, WANG Q, et al. Ultrastrong yet ductile 2D titanium nanomaterial for on-skin conformal triboelectric sensing [J]. Nano Letters, 2023, 23(12): 5802–5810. doi: 10.1021/acs.nanolett.3c01776
[12]	GUO B, XIAO Q L, WANG S H, et al. 2D layered materials: synthesis, nonlinear optical properties, and device applications [J].Laser & Photonics Reviews, 2019, 13(12): 1800327. doi: 10.1002/lpor.201800327
[13]	GEIM A K, GRIGORIEVA I V. Van der Waals heterostructures [J]. Nature, 2013, 499(7459): 419–425. doi: 10.1038/nature12385
[14]	NOVOSELOV K S, GEIM A K, MOROZOV S V, et al. Electric field effect in atomically thin carbon films [J]. Science, 2004, 306(5696): 666–669. doi: 10.1126/science.1102896
[15]	NOVOSELOV K S, GEIM A K, MOROZOV S V, et al. Two-dimensional gas of massless Dirac fermions in graphene [J].Nature, 2005, 438(7065): 197–200. doi: 10.1038/nature04233
[16]	BERGER C, SONG Z M, LI T B, et al. Ultrathin epitaxial graphite: 2D electron gas properties and a route toward graphene-based nanoelectronics [J]. The Journal of Physical Chemistry B, 2004, 108(52): 19912–19916. doi: 10.1021/jp040650f
[17]	GEIM A K, NOVOSELOV K S. The rise of graphene [J]. Nature Materials, 2007, 6(3): 183–191. doi: 10.1038/nmat1849
[18]	ZHANG Y B, TAN Y W, STORMER H L, et al. Experimental observation of the quantum Hall effect and Berry’s phase in graphene [J]. Nature, 2005, 438(7065): 201–204. doi: 10.1038/nature04235
[19]	GEIM A K, NOVOSELOV K S. The rise of graphene [M]//Nanoscience and Technology. World Scientific, 2007: 11−19.
[20]	GEIM A K. Graphene: status and prospects [J]. Science, 2009, 324(5934): 1530–1534. doi: 10.1126/science.1158877
[21]	CASTRO NETO A H, GUINEA F, PERES N M R, et al. The electronic properties of graphene [J]. Reviews of Modern Physics, 2009, 81(1): 109–62. doi: 10.1103/RevModPhys.81.109
[22]	SPLENDIANI A, SUN L, ZHANG Y B, et al. Emerging photoluminescence in monolayer MoS₂ [J]. Nano Letters, 2010, 10(4): 1271–1275. doi: 10.1021/nl903868w
[23]	LEE C, LI Q Y, KALB W, et al. Frictional characteristics of atomically thin sheets [J]. Science, 2010, 328(5974): 76–80. doi: 10.1126/science.1184167
[24]	ZHAO P D, DESAI S, TOSUN M, et al. 2D layered materials: from materials properties to device applications[C]//2015 IEEE International Electron Devices Meeting (IEDM). Washington, USA: IEEE, 2015: 27.
[25]	BAI F, XU L, ZHAI X Y, et al. Vacancy in ultrathin 2D nanomaterials toward sustainable energy application [J]. Advanced Energy Materials, 2020, 10(11): 1902107. doi: 10.1002/aenm.201902107
[26]	MA J H, LIU H F, YANG N, et al. Circuit-level memory technologies and applications based on 2D materials [J]. Advanced Materials, 2022, 34(48): 2202371. doi: 10.1002/adma.202202371
[27]	NAIR R R, BLAKE P, GRIGORENKO A N, et al. Fine structure constant defines visual transparency of graphene [J].Science, 2008, 320(5881): 1308. doi: 10.1126/science.1156965
[28]	CAO Y, FATEMI V, DEMIR A, et al. Correlated insulator behaviour at half-filling in magic-angle graphene superlattices [J].Nature, 2018, 556(7699): 80–84. doi: 10.1038/nature26154
[29]	CAO Y, FATEMI V, FANG S A, et al. Unconventional superconductivity in magic-angle graphene superlattices [J].Nature, 2018, 556(7699): 43–50. doi: 10.1038/nature26160
[30]	ZHOU Y, SUNG J, BRUTSCHEA E, et al. Bilayer Wigner crystals in a transition metal dichalcogenide heterostructure [J].Nature, 2021, 595(7865): 48–52. doi: 10.1038/s41586-021-03560-w
[31]	DECKER R, WANG Y, BRAR V W, et al. Local electronic properties of graphene on a BN substrate via scanning tunneling microscopy [J]. Nano Letters, 2011, 11(6): 2291–2295. doi: 10.1021/nl2005115
[32]	TRAN K, MOODY G, WU F C, et al. Evidence for moiré excitons in van der Waals heterostructures [J]. Nature, 2019, 567(7746): 71–75. doi: 10.1038/s41586-019-0975-z
[33]	SEYLER K L, RIVERA P, YU H Y, et al. Signatures of moiré-trapped valley excitons in MoSe₂/WSe₂ heterobilayers [J]. Nature, 2019, 567(7746): 66–70. doi: 10.1038/s41586-019-0957-1
[34]	JIN C H, REGAN E C, YAN A M, et al. Observation of moiré excitons in WSe₂/WS₂ heterostructure superlattices [J].Nature, 2019, 567(7746): 76–80. doi: 10.1038/s41586-019-0976-y
[35]	高扬. 原子力显微镜在二维材料力学性能测试中的应用综述 [J]. 力学学报, 2021, 53(4): 929–943. doi: 10.6052/0459-1879-20-354 GAO Y. Review of the application of atomic force microscopy in testing the mechanical properties of two-dimensional materials [J]. Chinese Journal of Theoretical and Applied Mechanics, 2021, 53(4): 929–943. doi: 10.6052/0459-1879-20-354
[36]	FRANK I W, TANENBAUM D M, VAN DER ZANDE A M, et al. Mechanical properties of suspended graphene sheets [J].Journal of Vacuum Science & Technology B: Microelectronics and Nanometer Structures, 2007, 25(6): 2558–2561. doi: 10.1116/1.2789446
[37]	LIU F, MING P B, LI J. Ab initio calculation of ideal strength and phonon instability of graphene under tension [J]. Physical Review B, 2007, 76(6): 064120. doi: 10.1103/PhysRevB.76.064120
[38]	WEI Y J, YANG R G. Nanomechanics of graphene [J]. National Science Review, 2019, 6(2): 324–348. doi: 10.1093/nsr/nwy067
[39]	ZHAO H, MIN K, ALURU N R. Size and chirality dependent elastic properties of graphene nanoribbons under uniaxial tension [J]. Nano Letters, 2009, 9(8): 3012–3015. doi: 10.1021/nl901448z
[40]	王慧, 徐萌, 郑仁奎. 二维材料/铁电异质结构的研究进展 [J]. 物理学报, 2020, 69(1): 017301. doi: 10.7498/aps.69.20191486 WANG H, XU M, ZHENG R K. Research progress and device applications of multifunctional materials based on two-dimensional film/ferroelectrics heterostructures [J]. Acta Physica Sinica, 2020, 69(1): 017301. doi: 10.7498/aps.69.20191486
[41]	WAN K T, GUO S, DILLARD D A. A theoretical and numerical study of a thin clamped circular film under an external load in the presence of a tensile residual stress [J]. Thin Solid Films, 2003, 425(1/2): 150–162. doi: 10.1016/S0040-6090(02)01103-3
[42]	KOMARAGIRI U, BEGLEY M R, SIMMONDS J G. The mechanical response of freestanding circular elastic films under point and pressure loads [J]. Journal of Applied Mechanics, 2005, 72(2): 203–212. doi: 10.1115/1.1827246
[43]	BERTOLAZZI S, BRIVIO J, KIS A. Stretching and breaking of ultrathin MoS₂ [J]. ACS Nano, 2011, 5(12): 9703–9709. doi: 10.1021/nn203879f
[44]	CASTELLANOS-GOMEZ A, POOT M, STEELE G A, et al. Elastic properties of freely suspended MoS₂ nanosheets [J]. Advanced Materials, 2012, 24(6): 772–775. doi: 10.1002/adma.201103965
[45]	FALIN A, CAI Q R, SANTOS E J G, et al. Mechanical properties of atomically thin boron nitride and the role of interlayer interactions [J]. Nature Communications, 2017, 8: 15815. doi: 10.1038/ncomms15815
[46]	LIPATOV A, LU H D, ALHABEB M, et al. Elastic properties of 2D Ti₃C₂T_x MXene monolayers and bilayers [J]. Science Advances, 2018, 4(6): eaat0491. doi: 10.1126/sciadv.aat0491
[47]	CHITARA B, YA’AKOBOVITZ A. Elastic properties and breaking strengths of GaS, GaSe and GaTe nanosheets [J].Nanoscale, 2018, 10(27): 13022–13027. doi: 10.1039/C8NR01065J
[48]	SUN Y F, WANG Y J, WANG E Z, et al. Determining the interlayer shearing in twisted bilayer MoS₂ by nanoindentation [J].Nature Communications, 2022, 13(1): 3898. doi: 10.1038/s41467-022-31685-7
[49]	GUO L L, YAN H M, MOORE Q, et al. Elastic properties of van der Waals epitaxy grown bismuth telluride 2D nanosheets [J].Nanoscale, 2015, 7(28): 11915–11921. doi: 10.1039/C5NR03282B
[50]	LI C, BANDO Y, ZHI C Y, et al. Thickness-dependent bending modulus of hexagonal boron nitride nanosheets [J].Nanotechnology, 2009, 20(38): 385707. doi: 10.1088/0957-4484/20/38/385707
[51]	LI Y H, YU C B, GAN Y Y, et al. Elastic properties and intrinsic strength of two-dimensional InSe flakes [J]. Nanotechnology,2019, 30(33): 335703. doi: 10.1088/1361-6528/ab1a96
[52]	SUN Y F, PAN J B, ZHANG Z T, et al. Elastic properties and fracture behaviors of biaxially deformed, polymorphic MoTe₂ [J].Nano Letters, 2019, 19(2): 761–769. doi: 10.1021/acs.nanolett.8b03833
[53]	WANG H, SANDOZ-ROSADO E J, TSANG S H, et al. Elastic properties of 2D ultrathin tungsten nitride crystals grown by chemical vapor deposition [J]. Advanced Functional Materials, 2019, 29(31): 1902663. doi: 10.1002/adfm.201902663
[54]	WANG J Y, LI Y, ZHAN Z Y, et al. Elastic properties of suspended black phosphorus nanosheets [J]. Applied Physics Letters, 2016, 108(1): 013104. doi: 10.1063/1.4939233
[55]	ZHANG R, KOUTSOS V, CHEUNG R. Elastic properties of suspended multilayer WSe₂ [J]. Applied Physics Letters, 2016, 108(4): 042104. doi: 10.1063/1.4940982
[56]	FALIN A, HOLWILL M, LV H F, et al. Mechanical properties of atomically thin tungsten dichalcogenides: WS₂, WSe₂, and WTe₂ [J]. ACS Nano, 2021, 15(2): 2600–2610. doi: 10.1021/acsnano.0c07430
[57]	WU B, HEIDELBERG A, BOLAND J J. Mechanical properties of ultrahigh-strength gold nanowires [J]. Nature Materials, 2005, 4(7): 525–529. doi: 10.1038/nmat1403
[58]	KIM Y J, SON K, CHOI I C, et al. Exploring nanomechanical behavior of silicon nanowires: AFM bending versus nanoindentation [J]. Advanced Functional Materials, 2011, 21(2): 279–286. doi: 10.1002/adfm.201001471
[59]	CELLINI F, GAO Y, RIEDO E. Å-indentation for non-destructive elastic moduli measurements of supported ultra-hard ultra-thin films and nanostructures [J]. Scientific Reports, 2019, 9(1): 4075. doi: 10.1038/s41598-019-40636-0
[60]	GAO Y, KIM S, ZHOU S, et al. Elastic coupling between layers in two-dimensional materials [J]. Nature Materials, 2015, 14(7): 714–720. doi: 10.1038/nmat4322
[61]	GAO Y. Force microscopy of two-dimensional materials [D]. Atlanta: Georgia Insititute of Technology, 2017: 44−51.
[62]	KELLY B T. Physics of graphite [M]. London: Applied Science, 1981.
[63]	PAN Z, HE L, QIU L, et al. Mechanical properties and microstructure of a graphene oxide-cement composite [J]. Cement and Concrete Composites, 2015, 58: 140–147. doi: 10.1016/j.cemconcomp.2015.02.001
[64]	MOUHAT F, COUDERT F X, BOCQUET M L. Structure and chemistry of graphene oxide in liquid water from first principles [J].Nature Communications, 2020, 11(1): 1566. doi: 10.1038/s41467-020-15381-y
[65]	RAJASEKARAN S, ABILD-PEDERSEN F, OGASAWARA H, et al. Interlayer carbon bond formation induced by hydrogen adsorption in few-layer supported graphene [J]. Physical Review Letters, 2013, 111(8): 085503. doi: 10.1103/PhysRevLett.111.085503
[66]	KVASHNIN A G, CHERNOZATONSKII L A, YAKOBSON B I, et al. Phase diagram of quasi-two-dimensional carbon, from graphene to diamond [J]. Nano Letters, 2014, 14(2): 676–681. doi: 10.1021/nl403938g
[67]	MARTINS L G P, MATOS M J S, PASCHOAL A R, et al. Raman evidence for pressure-induced formation of diamondene [J].Nature Communications, 2017, 8(1): 96. doi: 10.1038/s41467-017-00149-8
[68]	BAKHAREV P V, HUANG M, SAXENA M, et al. Chemically induced transformation of chemical vapour deposition grown bilayer graphene into fluorinated single-layer diamond [J]. Nature Nanotechnology, 2020, 15(1): 59–66. doi: 10.1038/s41565-019-0582-z
[69]	KE F, CHEN Y B, YIN K T, et al. Large bandgap of pressurized trilayer graphene [J]. Proceedings of the National Academy of Sciences of the United States of America, 2019, 116(19): 9186–9190. doi: 10.1073/pnas.1820890116
[70]	KE F, ZHANG L K, CHEN Y B, et al. Synthesis of atomically thin hexagonal diamond with compression [J]. Nano Letters, 2020, 20(8): 5916–5921. doi: 10.1021/acs.nanolett.0c01872
[71]	GAO Y, CAO T F, CELLINI F, et al. Ultrahard carbon film from epitaxial two-layer graphene [J]. Nature Nanotechnology, 2018, 13(2): 133–138. doi: 10.1038/s41565-017-0023-9
[72]	CELLINI F, LAVINI F, CAO T F, et al. Epitaxial two-layer graphene under pressure: diamene stiffer than diamond [J].FlatChem, 2018, 10: 8–13. doi: 10.1016/j.flatc.2018.08.001
[73]	CHAI P, LI S, LI Y, et al. Mechanical behavior investigation of 4H-SiC single crystal at the micro-nano scale [J]. Micromachines (Basel), 2020, 11(1).
[74]	LIN J, CHEN H, GAO Y, et al. Pressure-induced semiconductor-to-metal phase transition of a charge-ordered indium halide perovskite [J]. Proceedings of the National Academy of Sciences, 2019, 116(47): 23404. doi: 10.1073/pnas.1907576116
[75]	REJHON M, ZHOU X, LAVINI F, et al. Giant increase of hardness in silicon carbide by metastable single layer diamond-like coating [J]. Advanced Science (Weinh), 2023, 10(6): e2204562. doi: 10.1002/advs.202204562
[76]	BRAZHKIN V V, LYAPIN A G, HEMLEY R J. Harder than diamond: dreams and reality [J]. Philosophical Magazine A, 2002, 82(2): 231–253. doi: 10.1080/01418610208239596
[77]	SZCZEFANOWICZ B, KUWAHARA T, FILLETER T, et al. Formation of intermittent covalent bonds at high contact pressure limits superlow friction on epitaxial graphene [J]. Physical Review Research, 2023, 5(1): L012049. doi: 10.1103/PhysRevResearch.5.L012049
[78]	HOFMANN T, REN X G, WEYMOUTH A J, et al. Evidence for temporary and local transition of sp² graphite-type to sp³ diamond-type bonding induced by the tip of an atomic force microscope [J]. New Journal of Physics, 2022, 24(8): 083018. doi: 10.1088/1367-2630/ac8570
[79]	ARES P, PISARRA M, SEGOVIA P, et al. Tunable graphene electronics with local ultrahigh pressure [J]. Advanced Functional Materials, 2019, 29(8): 1806715. doi: 10.1002/adfm.201806715
[80]	SUN J H, CHANG K K, MEI D H, et al. Mutual identification between the pressure-induced superlubricity and the image contrast inversion of carbon nanostructures from AFM technology [J]. The Journal of Physical Chemistry Letters, 2019, 10(7): 1498–1504. doi: 10.1021/acs.jpclett.9b00155
[81]	CELLINI F, LAVINI F, CHEN E, et al. Pressure-induced formation and mechanical properties of 2D diamond boron nitride [J].Advanced Science, 2021, 8(2): 2002541. doi: 10.1002/advs.202002541
[82]	WANG D, RUSSELL T P. Advances in atomic force microscopy for probing polymer structure and properties [J]. Macromolecules, 2018, 51(1): 3–24. doi: 10.1021/acs.macromol.7b01459
[83]	关东石, 李航宇, 童彭尔. 原子力显微镜的生物力学实验方法和研究进展 [J]. 实验流体力学, 2020, 34(2): 57–66. doi: 10.11729/syltlx20200026 GUAN D S, LI H Y, TONG P E. Experimental methods and recent progress in biomechanics using atomic force microscopy [J].Journal of Experiments in Fluid Mechanics, 2020, 34(2): 57–66. doi: 10.11729/syltlx20200026
[84]	ZHOU G Q, ZHANG B K, TANG G L, et al. Cells nanomechanics by atomic force microscopy: focus on interactions at nanoscale [J]. Advances in Physics: X, 2021, 6(1): 1866668. doi: 10.1080/23746149.2020.1866668
[85]	CHAUDHURI O, COOPER-WHITE J, JANMEY P A, et al. Effects of extracellular matrix viscoelasticity on cellular behaviour [J]. Nature, 2020, 584(7822): 535–546. doi: 10.1038/s41586-020-2612-2
[86]	LI M, XI N, WANG Y C, et al. Advances in atomic force microscopy for single-cell analysis [J]. Nano Research, 2019, 12(4): 703–718. doi: 10.1007/s12274-018-2260-0
[87]	MÜLLER D J, DUMITRU A C, LO GIUDICE C, et al. Atomic force microscopy-based force spectroscopy and multiparametric imaging of biomolecular and cellular systems [J]. Chemical Reviews, 2021, 121(19): 11701–11725. doi: 10.1021/acs.chemrev.0c00617
[88]	BOSE K, NANDI T, AINAVARAPU S R K. Applications of atomic force microscopy in modern biology [J]. Emerging Topics in Life Sciences, 2021, 5(1): 103–111. doi: 10.1042/ETLS20200255
[89]	PINTO G, DANTE S, ROTONDI S M C, et al. Spectroscopic ellipsometry investigation of a sensing functional interface: DNA SAMs hybridization [J]. Advanced Materials Interfaces, 2022, 9(19): 2200364. doi: 10.1002/admi.202200364
[90]	BANERJEE S, LYUBCHENKO Y L. Topographically smooth and stable supported lipid bilayer for high-resolution AFM studies [J]. Methods, 2022, 197: 13–19. doi: 10.1016/j.ymeth.2021.02.010
[91]	ABAY A, SIMIONATO G, CHACHANIDZE R, et al. Glutaraldehyde: a subtle tool in the investigation of healthy and pathologic red blood cells [J]. Frontiers in Physiology, 2019, 10: 514. doi: 10.3389/fphys.2019.00514
[92]	ARGATOV I, JIN X Q, MISHURIS G. AFM-based spherical indentation of a brush-coated soft material: modeling the bottom effect [J]. Soft Matter, 2023, 19(26): 4891–4898. doi: 10.1039/D3SM00432E
[93]	SHEN Y S, GUAN D S, SERIEN D, et al. Mechanical characterization of microengineered epithelial cysts by using atomic force microscopy [J]. Biophysical Journal, 2017, 112(2): 398–409. doi: 10.1016/j.bpj.2016.12.026
[94]	DUMITRU A C, PONCIN M A, CONRARD L, et al. Nanoscale membrane architecture of healthy and pathological red blood cells [J]. Nanoscale Horizons, 2018, 3(3): 293–304. doi: 10.1039/C7NH00187H