Density Generalized Function Theory Study on New MAX Phase M2SeC (M=Zr, Hf) under High Pressure
-
摘要: 基于密度泛函理论的第一性原理,研究了压力对新型MAX相Zr2SeC和Hf2SeC晶体结构、弹性、电子和热力学性质的影响。弹性常数和声子计算表明,两种化合物在0~40 GPa压力范围内具有稳定结构。与大多数MAX相不同,Zr2SeC和Hf2SeC沿a轴方向比沿c轴方向更容易被压缩,外部压力对Zr2SeC晶体结构的影响比Hf2SeC更显著。电子结构计算表明,Zr2SeC和Hf2SeC具有金属性质,压力的升高降低了Zr2SeC和Hf2SeC在费米能级处的电子态密度,因此提高了Zr2SeC和Hf2SeC的稳定性。此外,弹性模量、泊松比和各向异性指数等均随着压力的升高而增大。在0~40 GPa压力范围内,相同压力下Hf2SeC的弹性模量大于Zr2SeC,表明高压下Hf2Se具有比Zr2SeC更强的抗断裂和抗变形能力。热力学性质计算表明,Zr2SeC和Hf2SeC在0~40 GPa压力范围内具有较高的熔化温度。Abstract: The effects of pressure on the crystal structure, elasticity, electronic and thermodynamic properties of the new MAX phases Zr2SeC and Hf2SeC were investigated by employing the first principle of density generalized function theory. Elastic constants and phonon calculations show that both compounds have stable structure in the pressure range of 0–40 GPa. Unlike most MAX phases, Zr2SeC and Hf2SeC are more easily compressed along the a-axis than along the c-axis, and the effect of external pressure on the crystal structure of Zr2SeC is more significant than Hf2SeC. Electronic structure calculations show that Zr2SeC and Hf2SeC have metallic properties, and the electronic density of states at the Fermi energy level decrease gradually with increasing pressure, thus improving the stability of Zr2SeC and Hf2SeC. In addition, the elastic modulus, the Poisson’s ratio and the anisotropy index show an enhancement with increasing pressure. In the pressure range of 0–40 GPa, the elastic modulus of Hf2SeC is greater than that of Zr2SeC at the same pressure, indicating that Hf2SeC has stronger resistance to fracture and deformation than Zr2SeC at high pressure. Thermodynamic property calculations show that Zr2SeC and Hf2SeC have higher melting temperatures in the pressure range of 0–40 GPa.
-
Key words:
- M2SeC /
- high pressure /
- first principles /
- crystal structure /
- electronic structure
-
针对现代战场环境毁伤目标多样化的需求,具备多毁伤元打击能力的复合战斗部(Composite warhead)应运而生。复合战斗部将多种不同的战斗部(如破片战斗部、爆炸成型弹丸和空心装药战斗部等)结合在一起,可以同时对多种目标进行有效打击,战斗部利用效率高、通用化程度高、适应性强,已成为目前战斗部技术发展的一个重要方向。
近年来,针对复合毁伤战斗部的研究逐渐增多。王利侠等[1]利用新型薄型波形控制器、半预制壳体和精密破甲战斗部技术,设计了破甲/杀伤多用途战斗部,使单兵战斗部在基本保持原有穿深威力的基础上,增加了杀伤功能。李兴隆等[2]则在此基础上,进一步研究了战斗部装填系数(装药质量与壳体质量之比)对破片杀伤半径和成型射流破甲深度的影响。然而,更多的复合战斗部还是通过在原有聚能战斗部上增设预制破片,实现聚能侵彻和破片杀伤相结合。张俊等[3]提出了一种轴向爆炸成型弹丸(Explosively formed projectile,EFP)、周向预制破片的新型破甲杀伤复合战斗部结构,并仿真分析了该结构下EFP的成型性能与破片场的杀伤威力。龚柏林等[4]提出了一种将预制破片周向分布在药型罩端面的复合战斗部结构,使预制破片与EFP均沿轴线飞行。基于此类结构,常江等[5]对药型罩设计参数进行了针对性的优化;董晓亮等[6]分析了预制破片类型、直径、材料、间距等对复合毁伤元战斗部发散角的影响;Ji等[7]分析了Ta-2.5W合金药型罩和钨铬合金预制破片的可行性及性能。此外,陈闯等[8]基于战斗部毁伤威力可控技术,进一步提出了一种外围采用刻槽壳体且可展开不同角度的EFP战斗部,并对EFP和预控破片群相结合的复合破片场成型过程进行了数值模拟研究,分析了中心与外围装药量、外围装药起爆位置、展开角度对复合破片场成型特性的影响。总体而言,当前研究多集中于战斗部结构本身的影响及优化,较少从起爆能量的角度分析其毁伤效能。
为了进一步提高复合战斗部的能量输出效率,本研究利用LS-DYNA数值仿真软件,研究起爆点位置、起爆直径和起爆点数量对复合战斗部所形成的聚能侵彻体、自然破片和预制破片3种毁伤元成型及性能的影响,讨论实现战斗部毁伤威力可调的技术路径。
1. 战斗部计算模型及研究方案
1.1 战斗部结构模型
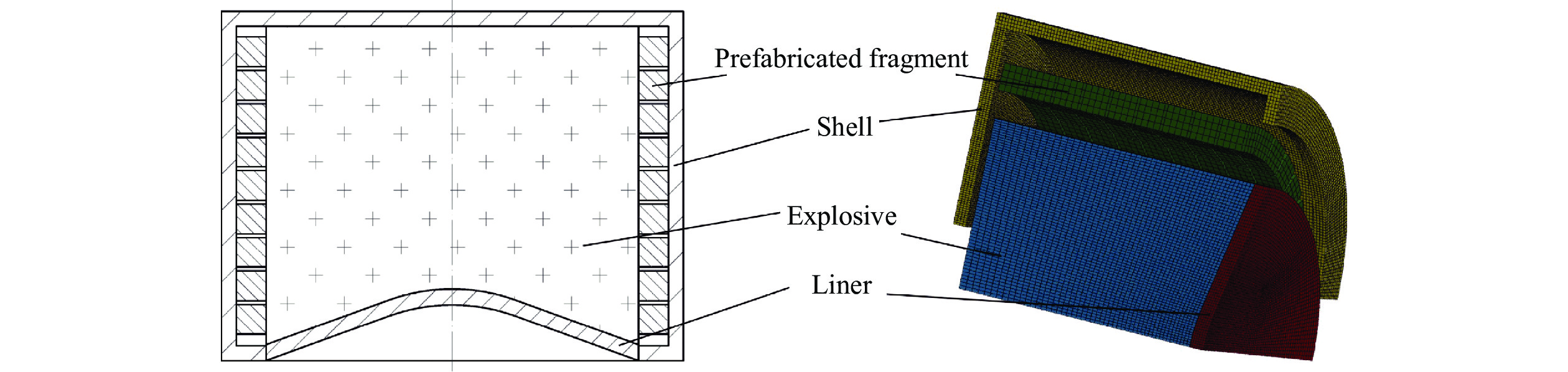
本研究中的复合战斗部主要包括主装药、药型罩、预制破片、壳体等部分,可形成聚能侵彻体、预制破片和自然破片3种毁伤元。其装药口径Dk为100 mm,装药高度为90 mm。药型罩采用弧锥结合结构,锥角155°,曲率半径55 mm,罩厚4.3 mm;预制破片为方块,边长为8 mm,共9排324枚;壳体厚度为4 mm,具体结构如图1所示。
主装药为JH-2炸药,采用High_Explosive_Burn模型和JWL状态方程描述。JWL状态方程表示为
p=A(1−ωR1V)e−R1V+B(1−ωR2V)e−R2V+ωe0V 式中:A、B、R1、R2和
ω 为输入参数,V为相对体积,e0为炸药材料的初始比内能。炸药材料参数如表1[9]所示,其中: ρe 为炸药密度,D为爆速,pC-J为C-J爆轰压力。空气选用Null材料模型和Grüneisen状态方程描述。对于压缩材料,Grüneisen状态方程表示为
p=ρ0C2μ[1+(1−γ02)μ−a2μ2][1−(S1−1)μ−S2μ2μ+1−S3μ3(μ+1)2]2 对于膨胀材料,则式(2)进一步表征为
p=ρ0C2μ+(γ0+aμ)E 式中:C为材料的静态体积声速;S1、S2、S3为系数;
γ0 为Grüneisen常数;μ=ρa/ρ0−1 ,ρa 为空气密度,ρ0 为空气初始密度;a为γ0 的一阶体积修正,E为空气的初始比内能。空气材料参数如表2[9]所示。金属部件主要包括药型罩、预制破片和壳体,所用材料分别为紫铜、钨合金和45钢。毁伤元成型过程属于典型的高温、高应变过程,因此选择Johnson-Cook本构模型和Grüneisen状态方程描述。Johnson-Cook本构方程式表示为
σy=(σ+b¯εpn)(1+cln˙ε∗)(1−T∗m) 式中:
σy 为材料的流动屈服强度,σ 为准静态下的屈服应力,b为应变硬化系数,c为应变率相关系数,m为温度相关系数,n为应变硬化指数,¯εp 为等效塑性应变,˙ε∗ 为无量纲化后的等效塑性应变率,T∗ 为无量纲温度。金属材料参数见表3[10],其中:ρ 为材料密度,G为剪切模量。1.2 数值仿真方案
利用ANSYS/LS-DYNA软件对复合毁伤元战斗部的成型过程进行数值模拟。考虑到模型为轴对称结构,取1/4结构建立三维模型。数值模拟过程采用ALE算法,其中药型罩、炸药、空气选择Euler网格;预制破片及壳体采用Lagrange网格,置于Euler网格中。Euler网格与Lagrange网格之间采用流体/结构耦合方式[9]。具体的网格划分为:药型罩径向60等分,厚度划分为6份,共16 200个网格;装药径向同样60等分,轴线划分为50份,共135 000个网格;空气与装药、药型罩相接位置划分方式一致;其他位置网格大小沿EFP成型方向分别取1.5、2.0和3.0 mm,共1 035 600个网格;预制破片网格大小为0.2 mm,共5 184个网格;考虑自然破片的形成,壳体网格大小取1.0 mm,共62 928个网格。
预制破片和壳体之间采用自动面-面接触方式,钨球定义为自动单面接触[6]。为避免压力在边界面反射,在整个空气计算域的周围表面定义无反射边界。考虑到战斗部作用过程属于典型爆炸问题,时间步长取0.67 μs。
本研究主要分析起爆点位置、起爆直径和起爆点数量对复合战斗部毁伤元成型及性能的影响,涉及到的起爆形式主要包括:端面单点起爆、端面多点起爆、轴线多点同步起爆、轴线阵列多点同步起爆等,起爆方式如图2所示。
单点起爆指装药端面中心点处起爆,多点起爆除特别说明外默认为8点起爆。起爆位置如图2(a)所示,分别取装药顶点(P1)、装药高度60 mm处(P2)、装药高度30 mm处(P3)和药型罩顶点(P4)4个位置;对于起爆直径,如图2(b)所示,分别取装药端面中心点、Dk/4、 Dk/2、3Dk/4和装药直径Dk处;对于起爆数量,如图2(c)所示,分别取端面单点、4点、6点、8点和轴线1 × 3点、4 × 3点、6 × 3点、8 × 3点。
2. 起爆方式对复合毁伤元的影响
对于该复合战斗部结构,其毁伤元主要包括聚能侵彻体、自然破片和预制破片3种形式。对于聚能侵彻体,主要成型参数为头部速度、头尾速度差和长径比,此处长径比为侵彻体总长与最大直径之比;对于破片,则主要分析其成型状态及速度分布,以探究起爆方式对毁伤元成型的影响。
2.1 起爆点位置的影响
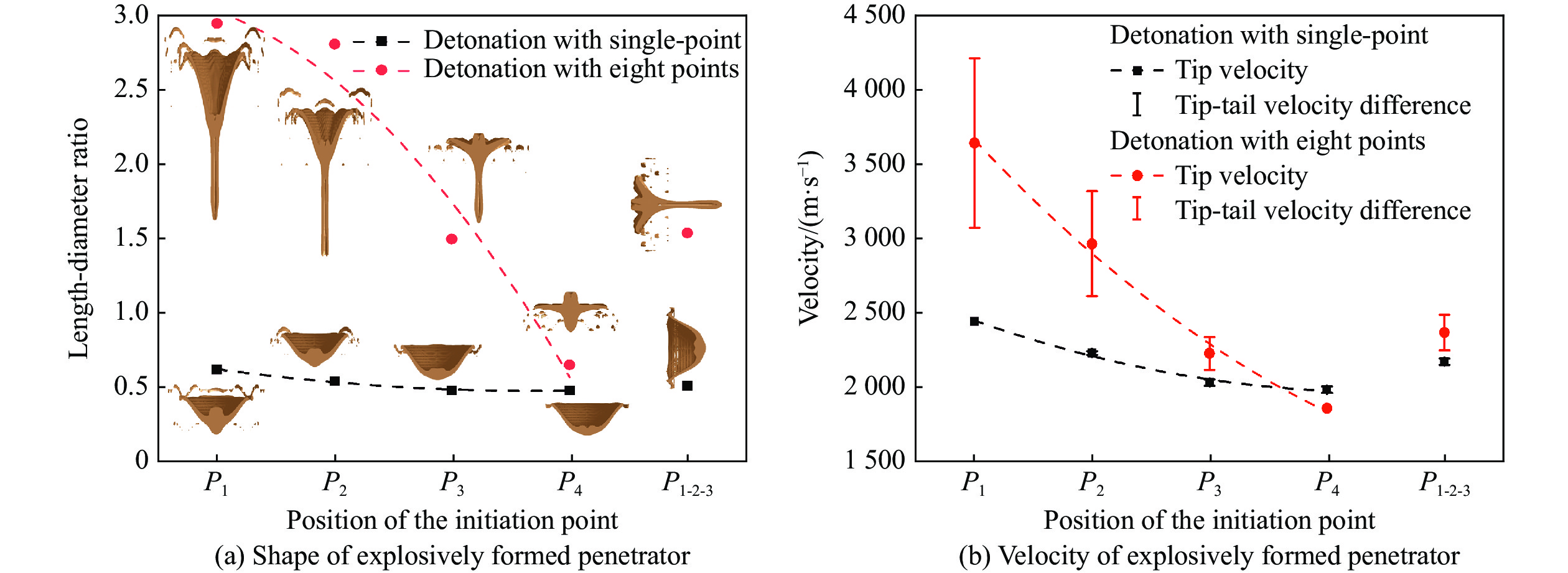
为了研究起爆点位置对毁伤元成型的影响,分别研究起爆点位置为P1、P2、P3、P4时中心单点起爆及端面多点起爆对复合战斗部毁伤元成型的影响。端面多点起爆采用8点同步起爆,且起爆直径为装药直径;轴线多点起爆为P1、P2、P3处3点同步起爆,采用P1-2-3来表示。通过数值模拟计算得到150 μs时刻各方案聚能侵彻体成型参数及形态,如图3所示,此时聚能侵彻体成型已基本稳定,形态不再发生明显变化。
由图3可知,随着起爆点位置逐渐向药型罩靠近,单点起爆形成的聚能侵彻体头部速度由2 434 m/s减小至2 165 m/s,而多点起爆形成的聚能侵彻体头部速度则由3 640 m/s减小至不足2 000 m/s。单点起爆时聚能侵彻体头尾速度差较小,始终保持在50 m/s以下,形成了典型的EFP;多点起爆时形成的聚能侵彻体头尾速度差更大,甚至形成了头尾速度差约为1 100 m/s的聚能杆式侵彻体(Jetting projectile charge,JPC)。起爆点位置离药型罩越远,形成的聚能侵彻体头部长度越大,其头尾速度差也越大,越有利于JPC的成型。轴线多点起爆时,其聚能侵彻体成型状态与P3处相近。这说明对于聚能侵彻体,轴线多点起爆时,距药型罩最近的起爆点受到的影响更大。在进行聚能毁伤元形态仿真研究的同时,对预制破片的飞散情况也进行了研究,150 μs时刻预制破片的速度参数如图4所示。
由图4可知,不论中心单点起爆还是端面多点起爆,起爆点位置由装药端面向药型罩逼近的过程中,预制破片的最高速度均呈现出先增大后减小的规律。整体来看,虽然多点起爆与单点起爆的最低速度相近,但多点起爆的速度分布带更大,均在200 m/s以上,对预制破片最高速度的提升作用显著,速度最高可提升100 m/s以上。对于复合战斗部,壳体形成的自然破片也至关重要,150 μs时刻自然破片成型参数及形态如图5所示。
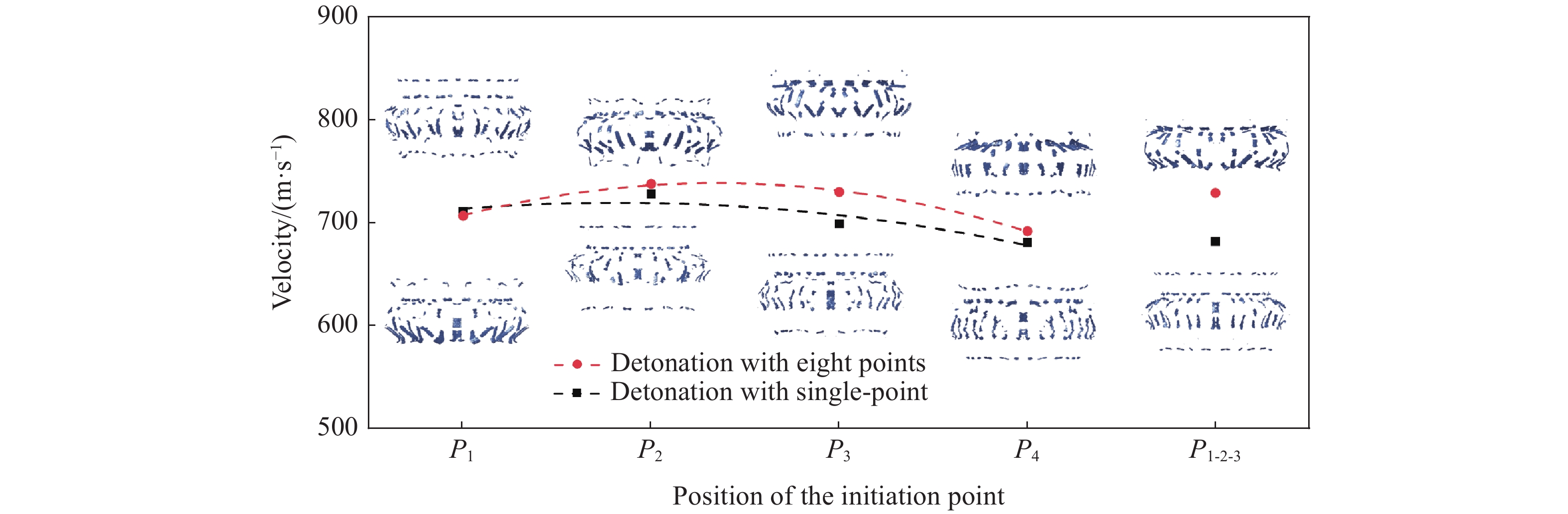
由图5可知,与预制破片的规律相似,起爆点位置由装药端面向药型罩逼近的过程中,自然破片平均速度也呈现出先增大后减小的规律,且两种起爆模式下仍然是P2处起爆时速度最高,分别达到728和738 m/s。对于破片质量分布,相较于端面多点起爆,中心单点起爆时壳体破碎形成的自然破片分布更均匀;而端面起爆时破片质量更大,也更完整,有利于提高破片威力。
2.2 起爆直径的影响
为了研究起爆直径对毁伤元成型的影响,分别研究了不同起爆直径时端面8点起爆和轴线阵列多点起爆对复合战斗部毁伤元成型的影响,轴线阵列起爆时P1、P2、P3同步24点(3 × 8)起爆。起爆直径分别取0 mm(中心单点)、Dk/4、Dk/2、3Dk/4和Dk。通过数值模拟计算得到150 μs时刻各方案聚能侵彻体成型参数及形态,如图6所示。
由图6可知,端面起爆时形成的侵彻体头尾速度均大于轴向阵列起爆时所形成的侵彻体,相较于轴向阵列起爆形成的EFP,其更易形成JPC。随着起爆直径的增大,两种起爆模式下形成的聚能侵彻体头部速度和头尾速度差均增大,杆型头部逐渐增长,长径比大幅提高,毁伤元由EFP形态逐渐转变为JPC形态。而端面起爆增益效果更为明显,头部速度从2 434 m/s提高到3 640 m/s的同时,头尾速度差也从零增大至570 m/s;侵彻体长径比达到2.94,是中心点起爆时长径比的4.8倍。150 μs时刻预制破片速度参数如图7所示。
由图7可知,不论是装药端面起爆还是轴向阵列起爆,当起爆直径增大时,预制破片的最高速度均增大,分别提高63和86 m/s;但端面起爆时预制破片的最小速度逐渐减小至585 m/s,轴向阵列起爆时预制破片的最小速度却逐渐增大至621 m/s,且轴向阵列起爆的速度分布带更大,对预制破片最高速度的提升作用明显,最高提升速度达到100 m/s以上。150 μs时刻自然破片成型参数及形态如图8所示。
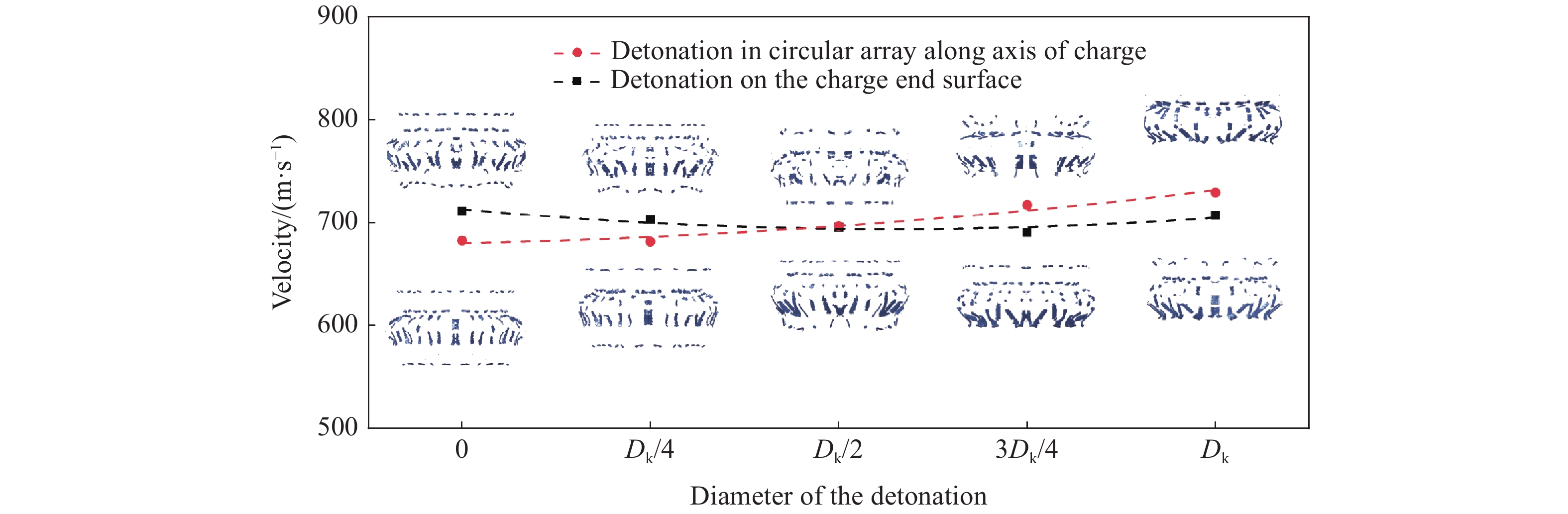
由图8可知,与预制破片不同,随着起爆直径的增大,端面起爆自然破片速度先减小后增大,3Dk/4处速度最低约690 m/s;轴向3处圆形阵列起爆自然破片的速度逐渐增大,速度提高约50 m/s,达到729 m/s。起爆直径为Dk/2时,两种起爆方式下自然破片的速度近乎一致,均为696 m/s。装药端面起爆直径存在一个最值,此时破片平均速度最低,且随着起爆直径的增大,大尺寸破片增加。
2.3 起爆点数量的影响
为了研究起爆点数量对毁伤元成型的影响,分别研究了不同起爆点数量时装药端面及轴向阵列起爆对复合战斗部毁伤元成型的影响。装药端面起爆时,起爆点数量分别取中心单点、端面4点、6点和8点;轴向阵列起爆时,取3点(1 × 3)、12点(4 × 3)、18点(6 × 3)和24点(8 × 3)。通过数值模拟计算得到150 μs时刻各方案聚能侵彻体成型参数及形态,如图9所示。
由图9可知,端面单点和轴向阵列起爆容易形成EFP,而端面多点起爆更易形成JPC。随着起爆点数量的增加,聚能侵彻体头部速度增大,端面起爆和轴向阵列起爆的速度增益分别达到1 000 m/s以上和200 m/s;长径比也随之增大,分别由0.62和0.51增大至2.58和0.96,但随着起爆点数量增加,长径比增大速度逐渐放缓,端面起爆点数量由4个增加到8个时,长径比仅提高约10%;轴向阵列起爆形成的侵彻体头尾速度增长较为缓和,头尾速度和长度均远小于端面起爆形成的侵彻体,最大头尾速度差不超过120 m/s,只能形成较典型的EFP。150 μs时刻预制破片的速度参数如图10所示。
由图10可知,随着起爆点数量的增加,端面起爆时预制破片的最大速度由743 m/s逐渐提高到803 m/s,而最小速度则由620 m/s减小到585 m/s;轴向阵列起爆时预制破片的最大速度及最小速度均先增大后减小,最大时分别为831和626 m/s。整体来看,端面起爆预制破片速度区间随着起爆点数量的增加而增大,速度区间由123 m/s增大至211 m/s,增长70%以上;但轴向阵列起爆的最大速度更大,达到831 m/s,对预制破片的最高速度提高了10%,作用更加显著。壳体自然破片在150 μs时刻的成型参数及形态如图11所示。
由图11可知,与预制破片的规律相似,随着起爆点数量增加,轴向阵列起爆时破片速度先增大后缓慢减小。但端面起爆时形成的自然破片平均速度变化不大,始终为710 m/s左右,上下浮动在10 m/s以内。壳体质量分布方面,随着起爆点数量增加,壳体破片质量增大,且破片更完整,更有利于破片威力的提高。总体来看,端面起爆时起爆点数量的作用不明显,轴线阵列起爆时增加起爆点数量有利于破片速度的提高。
3. 结 论
通过数值仿真研究了起爆点位置、直径和起爆点数量对复合战斗部所形成的聚能侵彻体、自然破片和预制破片3种毁伤元成型及性能的影响,得出以下主要结论。
(1)随着起爆点位置向药型罩靠近,聚能侵彻体长径比和头部速度均呈现减小趋势,端面8点起爆时尤为明显,且头尾速度差明显减小;单点起爆时位置的影响较小,P1-2-3同时起爆的效果并不明显,与P3处起爆形成的EFP相近。预制破片均在P2处起爆时达到最大破片速度,且端面8点起爆的速度增益更为明显。对于壳体形成的自然破片,其平均速度也在P2处达到最大;多点起爆位置对应壳体处形成的破片最大;P1-2-3同时起爆虽不能提高破片速度,但有助于使自然破片的断裂更均匀。
(2)随着起爆直径的增大,端面起爆和轴线阵列起爆对聚能侵彻体的影响一致,其长径比、头部速度和头尾速度差均随之增大,速度增益最高可达50%;端面起爆更有利于聚能侵彻体的拉伸,甚至可以形成JPC。预制破片的最大速度均呈上升趋势,但最小速度呈相反的变化趋势。对于壳体形成的自然破片,装药端面起爆直径存在一个最值,此时破片平均速度最低,且随着起爆直径的增大,大尺寸破片增加;而轴线阵列起爆直径的增大有利于破片速度的提高。
(3)随着起爆点数量的增加,聚能侵彻体长径比、头部速度和头尾速度差同样随之增大,速度增益与起爆直径的影响一致,也形成了JPC,但随着起爆点数量的增加,速度增大的趋势逐渐减缓。预制破片的速度变化与起爆直径的影响相近,但具有平台现象,整体来看,两种起爆方式对预制破片速度的增益相近。对于壳体形成的自然破片,端面起爆点数量的变化对破片平均速度的影响不大,仅对破片质量分布有一定影响,轴线阵列起爆点数量的增加有利于破片速度的提高。
-
表 1 Zr2SeC和Hf2SeC的结构参数
Table 1. Structural parameters of Zr2SeC and Hf2SeC
表 2 M2SeC的弹性常数和弹性模量随压力的变化
Table 2. Elastic constant and elastic moduli change of M2SeC with pressure
M2AX Pressure/GPa C11/GPa C12/GPa C13/GPa C33/GPa C44/GPa B/GPa G/GPa E/GPa Zr2SeC 0
4
8
12
16
20
24
28
32
36
40272
290
305
320
337
354
366
378
393
404
41784
91
99
106
113
121
128
135
144
152
16194
106
118
130
144
157
165
176
187
197
207293
313
331
349
368
386
397
416
430
446
462128
140
153
165
178
191
200
212
222
232
243153
166
179
191
204
217
227
238
249
259
270106
113
120
126
132
139
143
148
153
157
162259
277
294
309
326
343
354
368
381
392
404Hf2SeC 0
4
8
12
16
20
24
28
32
36
40288
307
325
341
358
374
391
406
422
437
45185
93
100
107
115
123
132
141
150
159
167101
114
127
140
150
161
171
181
192
202
212307
327
347
366
383
401
418
435
454
470
487129
144
159
172
185
198
211
223
234
246
257161
176
189
202
214
226
238
250
262
274
285111
119
126
133
140
147
153
159
165
170
176271
291
310
328
345
362
378
393
409
423
437表 3 0~40 GPa压力下M2SeC的横向声速、纵向声速、平均声速和德拜温度
Table 3. Transverse, longitudinal, mean sound velocities, and Debye’s temperaturefor the M2SeC phase at 0–40 GPa pressure
M2AX Pressure/GPa vt/(km·s−1) vl/(km·s−1) vm/(km·s−1) ΘD/K Tm/K Ref. Zr2SeC 0
4
8
12
16
20
24
28
32
36
403.935
4.013
4.079
4.132
4.200
4.263
4.289
4.335
4.372
4.402
4.4376.555
6.714
6.859
6.979
7.125
7.260
7.331
7.432
7.527
7.604
7.6924.353
4.441
4.517
4.578
4.654
4.726
4.756
4.809
4.852
4.886
4.926508.8
523.4
536.3
547.2
560.0
571.9
578.8
588.3
596.4
603.4
611.11 609
1 693
1 767
1 837
1 916
1 995
2 046
2 113
2 178
2 237
2 299Hf2SeC 0
4
8
12
16
20
24
28
32
36
403.083
3.156
3.218
3.273
3.325
3.374
3.419
3.458
3.498
3.531
3.5645.149
5.289
5.412
5.523
5.624
5.719
5.811
5.893
5.979
6.056
6.1273.412
3.493
3.563
3.625
3.683
3.738
3.790
3.833
3.879
3.917
3.954403.6
416.5
427.8
438.2
447.9
457.1
465.9
473.5
481.4
488.3
495.01 678
1 767
1 848
1 927
2 003
2 078
2 154
2 224
2 301
2 371
2 438Zr2AlC 0 4.22 6.89 4.66 544 [51] Hf2AlC 0 3.38 5.50 3.73 439 [52] -
[1] BARSOUM M W. The M N+1AXN phases: a new class of solids: thermodynamically stable nanolaminates [J]. Progress in Solid State Chemistry, 2000, 28(1): 201–281. [2] WANG X H, ZHOU Y C. Layered machinable and electrically conductive Ti2AlC and Ti3AlC2 ceramics: a review [J]. Journal of Materials Science and Technology, 2010, 26(5): 385–416. [3] EKLUND P, BECKERS M, JANSSON U, et al. The M n+1AXn phases: materials science and thin-film processing [J]. Thin Solid Films, 2010, 518: 1851–1878. doi: 10.1016/j.tsf.2009.07.184 [4] BARSOUM M W, EL-RAGHY T. The MAX phases: unique new carbide and nitride materials [J]. American Scientist, 2001, 89: 334–343. doi: 10.1511/2001.28.736 [5] BARSOUM M W, EL-RAGHY T. Synthesis and characterization of a remarkable ceramic: Ti3SiC2 [J]. Journal of the American Ceramic Society, 1996, 79: 1953–1956. doi: 10.1111/j.1151-2916.1996.tb08018.x [6] TZENOV N V, BARSOUM M W. Synthesis and characterization of Ti3AlC2 [J]. Journal of the American Ceramic Society, 2000, 83(4): 825–832. [7] HAJAS D E, TO BABEN M, HALLSTEDT B, et al. Oxidation of Cr2AlC coatings in the temperature range of 1 230 to 1 410 °C [J]. Surface and Coatings Technology, 2011, 206(4): 591–598. doi: 10.1016/j.surfcoat.2011.03.086 [8] SMIALEK J L. Oxidation of Al2O3 scale-forming MAX phases in turbine environments [J]. Metallurgical and Materials Transactions A, 2018, 49: 782–792. doi: 10.1007/s11661-017-4346-9 [9] FU J, ZHANG T F, XIA Q X, et al. Oxidation and corrosion behavior of nanolaminated MAX-phase Ti2AlC film synthesized by high-power impulse magnetron sputtering and annealing [J]. Journal of Nanomaterials, 2015, 16: 411. [10] GUPTA S, FILIMONOV D A, PALANISAMY T G, et al. Tribological behavior of select MAX phases against Al2O3 at elevated temperatures [J]. Wear, 2008, 265: 560–565. doi: 10.1016/j.wear.2007.11.018 [11] HOPFELD M, GRIESELER R, VOGEL A, et al. Tribological behavior of selected M n+1AXn phase thin films on silicon substrates [J]. Surface and Coatings Technology, 2014, 257: 286–294. [12] SHEIN I R, IVANOVSKII A L. Elastic properties of superconducting MAX phases from first-principles calculations [J]. Physica Status Solidi B. Basic Research, 2010, 248(1): 228–232. [13] LEE W E, GIORGI E, HARRISON R, et al. Nuclear applications for ultra-high temperature ceramics and MAX phases [M]//FAHRENHOLTZ W G, WUCHINA E J, LEE W E. Ultra-High Temperature Ceramics: Materials for Extreme Environment Applications. The American Ceramic Society, 2014. [14] SUN D D, HU Q K, CHEN J F, et al. Structural transformation of MXene (V2C, Cr2C, and Ta2C) with O groups during lithiation: a first-principles investigation [J]. ACS Applied Materials and Interfaces, 2015, 8(1): 74–81. [15] LIN Z, BARBARA D, TABEMA P-L, et al. Capacitance of Ti3C2Tx MXene in ionic liquid electrolyte [J]. Journal of Power Sources, 2016, 326: 575–579. doi: 10.1016/j.jpowsour.2016.04.035 [16] WANG Y H, MA C, MA W Q, et al. Enhanced low-temperature Li-ion storage in MXene titanium carbide by surface oxygen termination [J]. 2D Materials, 2019, 6(4): 045025. doi: 10.1088/2053-1583/ab30f9 [17] LAPAUW T, TUNCA B, CABIOCH T, et al. Synthesis of MAX phases in the Hf-Al-C system [J]. Inorganic Chemistry, 2016, 55(21): 10922–10927. [18] PIECHOWIAK M A, HENON J, DURAND-PANTEIX O, et al. Growth of dense Ti3SiC2 MAX phase films elaborated at room temperature by aerosol deposition method [J]. Journal of the European Ceramic Society, 2014, 34(5): 1063–1072. doi: 10.1016/j.jeurceramsoc.2013.11.019 [19] MOCKUTE A, PERSSON P O Å, MAGNUS F, et al. Synthesis and characterization of arc deposited magnetic (Cr, Mn)2AlC MAX phase films [J]. Physica Status Solidi, 2014, 8(5): 420–423. [20] HOFFMAN E N, VINSON D W, SINDELAR R L, et al. MAX phase carbides and nitrides: properties for future nuclear power plant in-core applications and neutron transmutation analysis [J]. Nuclear Engineering and Design, 2012, 244: 17–24. doi: 10.1016/j.nucengdes.2011.12.009 [21] ROMEO M, ESCAMILLA R. Pressure effect on the structural, elastic and electronic properties of Nb2AC (A=S and In) phases; ab initio study [J]. Computational Materials Science, 2014, 81: 184–190. doi: 10.1016/j.commatsci.2013.08.010 [22] ROMEO M, ESCAMILLA R. First-principles calculations of structural, elastic and electronic properties of Nb2SnC under pressure [J]. Computational Materials Science, 2012, 55: 142–146. doi: 10.1016/j.commatsci.2011.11.038 [23] BOUHEMADOU A, KHENATA R, KHAROUBI M, et al. First-principles study of structural and elastic properties of Sc2AC (A=Al, Ga, In, Tl) [J]. Solid State Communications, 2008, 146(3): 175–180. [24] BOUHEMADOU A. Calculated structural and elastic properties of M2InC (M=Sc, Ti, V, Zr, Nb, Hf, Ta) [J]. Modern Physics Letters B, 2008, 22(22): 2063–2076. doi: 10.1142/S0217984908016807 [25] BOUHEMADOU A. Structural, electronic and elastic properties of MAX phases M2GaN (M = Ti, V and Cr) [J]. Solid State Sciences, 2009, 11: 1875–1881. doi: 10.1016/j.solidstatesciences.2009.08.002 [26] PENG M J, WANG R F, WU Y J, et al. Elastic anisotropies, thermal conductivities and tensile properties of MAX phases Zr2AlC and Zr2AlN: a first-principles calculation [J]. Vacuum, 2022, 196: 110715. doi: 10.1016/j.vacuum.2021.110715 [27] UDDIN M, ALI M A, HOSSAIN M M, et al. Comparative study of predicted MAX phase Hf2AlN with recently synthesized Hf2AlC: a first principle calculations [J]. Indian Journal of Physics, 2022, 96(5): 1321–1333. doi: 10.1007/s12648-021-02050-z [28] MIAO N X, WANG J J, GONG Y T, et al. Computational prediction of boron-based MAX phases and MXene derivatives [J]. Chemistry of Materials, 2020, 32(16): 6947–6957. [29] LUO F, GUO Z C, ZHANG X L, et al. Ab initio predictions of structural and thermodynamic properties of Zr2AlC under high pressure and high temperature [J]. Chinese Journal of Chemical Physics, 2015, 28(3): 263–268. doi: 10.1063/1674-0068/28/cjcp1503032 [30] FU H Z, TENG M, LIU W F, et al. The axial compressibility, thermal expansion and elastic anisotropy of Hf2SC under pressure [J]. The European Physical Journal B-Condensed Matter and Complex Systems, 2010, 78(1): 37–42. doi: 10.1140/epjb/e2010-10332-5 [31] QURESHI M W, MA X X, TANG G Z, et al. Structural stability, electronic, mechanical, phonon, and thermodynamic properties of the M2GaC (M=Zr, Hf) MAX phase: an ab initio calculation [J]. Materials, 2020, 13(22): 1–18. [32] ALI M A, QURESHI M W. Newly synthesized MAX phase Zr2SeC: DFT insights into physical properties towards possible applications [J]. RSC Advances, 2021, 11: 16892–16905. doi: 10.1039/D1RA02345D [33] ALI M A, QURESHI M W. DFT insights into the new Hf-based chalcogenide MAX phase Hf2SeC [J]. Vacuum, 2022, 201: 111072. doi: 10.1016/j.vacuum.2022.111072 [34] KRESSE G, FURTHMÜLLER J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set [J]. Physical Review B, 1996, 54(16): 11169–11186. doi: 10.1103/PhysRevB.54.11169 [35] KRESSE G, HAFNER J. Ab initio molecular dynamics for open-shell transition metals [J]. Physical Review B, 1993, 48(17): 13115–13118. doi: 10.1103/PhysRevB.48.13115 [36] BLÖCHL P E. Projector augmented-wave method [J]. Physical Review B, 1994, 50(24): 17953–17979. doi: 10.1103/PhysRevB.50.17953 [37] WU Z G, COHEN R E. More accurate generalized gradient approximation for solids [J]. Physical Review B, 2006, 73(23): 235116. doi: 10.1103/PhysRevB.73.235116 [38] MONKHORST H J, PACK J D. Special points for Brillouin-zone integrations [J]. Physical Review B, 1976, 13(12): 5188–5192. doi: 10.1103/PhysRevB.13.5188 [39] CHEN K, BAI X J, MU X L, et al. MAX phase Zr2SeC and its thermal conduction behavior [J]. Journal of the European Ceramic Society, 2021, 41(8): 4447–4451. doi: 10.1016/j.jeurceramsoc.2021.03.013 [40] WANG X D, CHEN K, WU E X, et al. Synthesis and thermal expansion of chalcogenide MAX phase Hf2SeC [J]. Journal of the European Ceramic Society, 2022, 42(5): 2084–2088. doi: 10.1016/j.jeurceramsoc.2021.12.062 [41] KANG D B. Influence of different a elements on bonding and elastic properties of Zr2AC (A=Al, Si, P, S): a theoretical investigation [J]. Bulletin of the Korean Chemical Society, 2013, 34(2): 609–614. doi: 10.5012/bkcs.2013.34.2.609 [42] YANG Z J, GUO Y, LINGHU R F, et al. First-principles calculation of the lattice compressibility, elastic anisotropy and thermodynamic stability of V2GeC [J]. Chinese Physics B, 2012, 21: 036301. doi: 10.1088/1674-1056/21/3/036301 [43] WU Z J, ZHAO E J, XIANG H P, et al. Crystal structures and elastic properties of superhard IrN2 and IrN3 from first principles [J]. Physical Review B, 2007, 76(5): 054115. doi: 10.1103/PhysRevB.76.054115 [44] HILL R. The elastic behaviour of a crystalline aggregate [J]. Proceedings of the Physical Society Section A, 1952, 65(5): 349–354. doi: 10.1088/0370-1298/65/5/307 [45] OUADHA I, RACHED H, AZZOUZ-RACHED A, et al. Study of the structural, mechanical and thermodynamic properties of the new MAX phase compounds (Zr1 − Tix)3AlC2 [J]. Computational Condensed Matter, 2020, 23: e00468. doi: 10.1016/j.cocom.2020.e00468x [46] AYDIN S, SIMSEK M. First-principles calculations of elemental crystalline boron phases under high pressure: orthorhombic B28 and tetragonal B48 [J]. Journal of Alloys and Compounds, 2011, 509(17): 5219–5229. doi: 10.1016/j.jallcom.2011.02.070 [47] FRANTSEVICH I N. Elastic constants and elastic moduli of metals and insulators [J]. Reference Book, 1982. [48] CHEN Q, HUANG Z W, ZHAO Z D, et al. Thermal stabilities, elastic properties and electronic structures of B2-MgRE (RE=Sc, Y, La) by first-principles calculations [J]. Computational Materials Science, 2013, 67: 196–202. doi: 10.1016/j.commatsci.2012.08.010 [49] XU Y, HU C Y, ZHOU S G, et al. Theoretical insights on structural, mechanical and thermodynamic properties of MCoB (M=Nb, Mo, and W) ternary borides under high pressure [J]. Solid State Sciences, 2022, 130: 106931. doi: 10.1016/j.solidstatesciences.2022.106931 [50] ANDERSON O L. A simplified method for calculating the debye temperature from elastic constants [J]. Journal of Physics and Chemistry of Solids, 1963, 24(7): 909–917. doi: 10.1016/0022-3697(63)90067-2 [51] BOUHEMADOU A, KHENATA R, CHEGAAR M. Structural and elastic properties of Zr2AlX and Ti2AlX (X = C and N) under pressure effect [J]. The European Physical Journal B, 2007, 56: 209–215. doi: 10.1140/epjb/e2007-00115-6 [52] BOUHEMADOU A. Structural and elastic properties under pressure effect of Hf2AlN and Hf2AlC [J]. High Pressure Research, 2008, 28: 45–53. doi: 10.1080/08957950701882872 -








 下载:
下载:
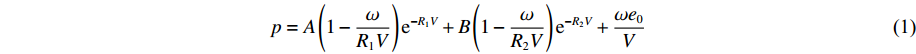
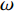
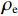
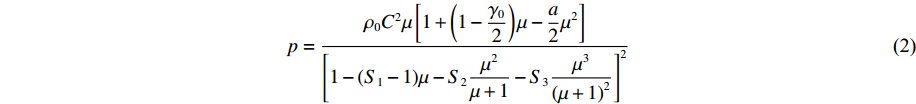
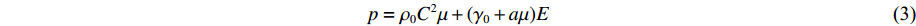
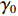
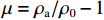
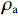
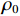
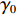
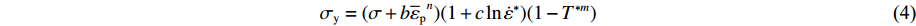
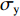
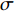

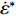

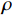











 下载:
下载: