Quasi-Static Axial Energy Absorption Characteristics and Optimization of Sunflower-Like Sandwich Cylindrical Shells
-
摘要: 采用实验、理论和数值模拟方法研究了类向日葵夹芯圆柱壳在准静态轴向加载下的吸能特性。首先,对3种内径的夹芯圆柱壳及其构件进行了准静态轴向压缩实验和数值模拟。结果表明:所有夹芯圆柱壳的比吸能和压溃力效率都大于其各个组成构件以及构件之和;芯壳的波浪形结构比圆柱壳结构具有更好的能量吸收能力;圆柱壳和波浪形曲线芯壳的联合使用可以有效地提高薄壁金属结构的吸能效率。其次,基于简化的超级折叠单元理论,推导了夹芯圆柱壳的轴向平均压溃力的理论公式。理论预测的平均压溃力与实验结果及数值模拟结果间的相对误差均在10%以内。最后,以比吸能最大和峰值压溃力最小为目标,进行了类向日葵夹芯圆柱壳吸能特性的多目标优化设计,得到了夹芯圆柱壳比吸能和峰值压溃力的Pareto前沿,优化后的夹芯圆柱壳的比吸能和平均压溃力均有提高,与此同时,质量减小。Abstract: Experiments, theoretical analysis, and numerical simulations were conducted to investigate the energy absorption characteristics of sunflower-like sandwich cylindrical shells under quasi-static axial loading. Firstly, quasi-static axial compression experiments and numerical simulations were conducted for sandwich cylindrical shells with three inner diameters and for their components. It was found that the specific energy absorptions and crushing force efficiencies of all sandwich cylindrical shells are greater than those of their individual components, and those of the sums of the individual components. The combination of cylindrical shell and corrugated core shell can effectively improve the energy absorption efficiency of thin-walled metal structure. Then, the theoretical formula of the axial average crushing force for the sandwich cylindrical shell was derived based on the simplified super folding element theory. The axial average crushing force predicted by the theoretical model was compared with the experimental and simulation results. It was found that the errors are within 10%. Finally, a multi-objective optimization design, with the objectives of maximum specific energy absorption and minimum peak crushing force for the sunflower-like sandwich cylindrical shell, was carried out. The Pareto front of specific energy absorption and peak crushing force of the sandwich cylindrical shell was obtained. The optimized sandwich cylindrical shell structure was improved in terms of specific energy absorption, average crushing force, and mass.
-
薄壁结构具有轻质性和优异的吸能特性,被广泛应用于汽车、飞机、轮船等领域。在最近几十年里,人们对薄壁结构进行了大量的研究。薄壁圆管的结构简单、能量吸收效率高,研究人员首先对其进行了研究。Johnson等[1]推导了压溃过程中理想刚性圆管的平均压溃载荷与吸收能量之间关系的简单表达式。Andrews等[2]研究了管长度对铝合金圆管的轴向压溃方式和能量吸收的影响。此外,还有研究发现,截面形状对薄壁结构的力学性能有重要影响。例如,李志斌等[3]通过准静态轴向压缩实验,比较了金属薄壁方管和圆管的变形模式和吸能能力,发现圆管的吸能特性指标优于方管。Ali等[4]比较了具有不同横截面形状的薄壁管的吸能特性,结果表明,截面特性对单层壳结构的力学性能有重要影响。荆友录等[5]采用数值模拟方法比较了方形管、圆形管、圆锥形管和方锥形管在轴向吸能方面的性能,发现圆形截面的管件在吸能方面表现出较优异的性能。随着研究的深入,多层壳结构受到关注。Vinayagar等[6]通过实验方法研究了双截面双层壳结构的轴向压溃性能,发现双层壳结构比单圆管具有更高的平均压溃载荷和比吸能,其能量吸收效率提升了132%~213%。Zheng等[7]研究了多边形单层壳和多边形多层壳在轴向压溃载荷下的能量吸收特性,发现平均压溃力和吸收的能量随着双层壳中多边形边数的增加而增大,并且圆形双层壳结构具有更加出色的能量吸收特性。Goel[8]研究了单层、双层和多层圆壳结构的轴向变形模式和能量吸收特性,发现双层圆壳和3层圆壳表现出更利于能量吸收的变形模式。近年来,为了获得更高的吸能效率,夹芯薄壁金属管逐渐成为研究热点。Chen等[9]对三角形、四边形和五边形波纹夹芯壳进行了理论分析和数值模拟,发现五边形波纹夹芯壳具有最高的吸能性能。马梦娇等[10]研究了轴向载荷下四边形、五边形和六边形波纹夹芯壳的力学响应,发现六边形夹芯壳具有最优的吸能能力,并对3种结构进行了多目标优化,得到了比吸能和初始峰值力的Pareto前沿。葛平政等[11]采用数值模拟方法研究了一种波纹薄壁圆柱壳结构,分析了不同截面形状对薄壁结构吸能特性的影响。闫栋等[12]研究了径向加载下类向日葵薄壁夹芯结构的耐撞性能。为了进一步探究类向日葵薄壁夹芯结构在轴向加载下的吸能特性,本研究从实验、理论和数值模拟3方面对不同内径、壁厚的类向日葵夹芯圆柱壳及其构件的轴向准静态吸能特性进行系统性研究。
1. 问题描述
1.1 几何描述
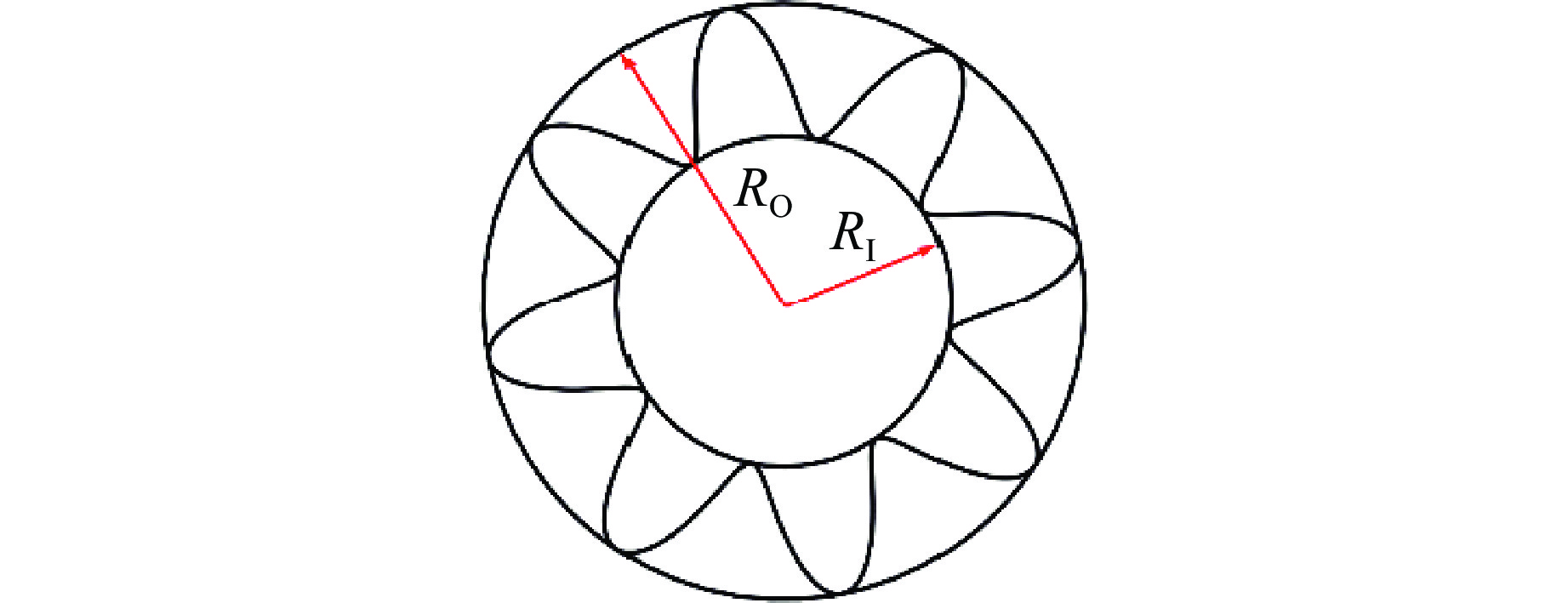
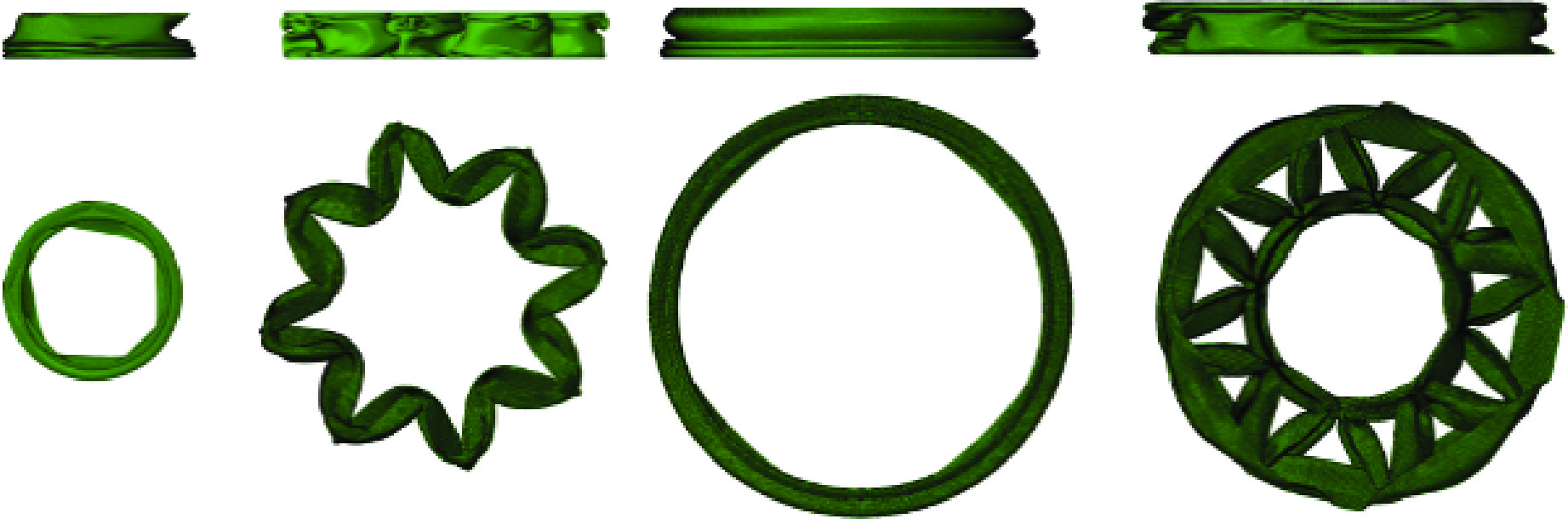
典型的类向日葵夹芯圆柱壳的横截面如图1所示。由图1可知,夹芯圆柱壳由两个同心圆和一条向日葵曲线围合组成。RI为内圆的半径,RO为外圆的半径。本研究中,外圆半径保持为45 mm不变,内圆半径选取15、25 和35 mm 3种尺寸。向日葵曲线的表达式为
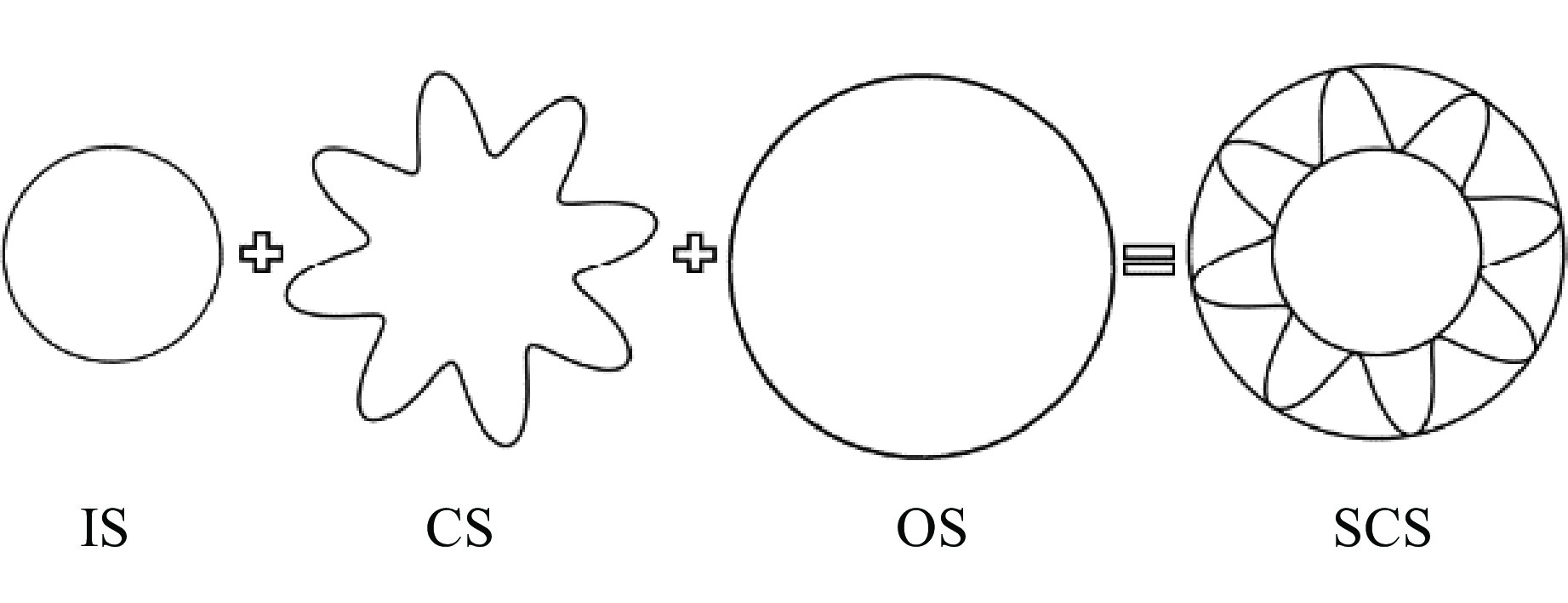
X(θ)=[RO+RI2+RO−RI2sin(Nθπ180)]cos(θπ180) (1) Y(θ)=[RO+RI2+RO−RI2sin(Nθπ180)]sin(θπ180) (2) 式中:N为花瓣数,(RO+RI)/2为向日葵曲线振幅基准轴,(RO−RI)/2为向日葵曲线振幅。内外径尺寸和花瓣数共同决定了曲线的几何特征。图1所示的夹芯圆柱壳的内圆半径RI=25 mm,花瓣数N=8。夹芯圆柱壳(SCS)中,外圆壳(OS)、芯壳(CS)和内圆壳(IS)的壁厚保持相等。夹芯圆柱壳的高度H=45 mm。图2为夹芯圆柱壳的组成结构示意图。
1.2 吸能特性指标
为了量化夹芯圆柱壳的吸能特性,定义如下吸能特性指标。
能量吸收(energy absorption,EA),是夹芯圆柱壳在受压过程中的总吸能。能量吸收是力和位移曲线的积分,表示为
EA=∫l0F(s)ds (3) 式中:l为压溃过程的总位移,F(s)为压溃距离为s时的压溃力。能量吸收是基本的吸能特性指标。
比能量吸收(specific energy absorption,SEA)表示单位质量的薄壁结构的能量吸收,定义为
ESA=EA/m (4) 式中:m为夹芯壳的质量。比能量吸收越大,结构的吸能能力越好。
峰值压溃力(peak crushing force,PCF)是压溃过程中的最大压溃力,用pp表示。
平均压溃力(mean crushing force,MCF)是圆柱壳整个压溃过程中压溃力的平均值,表达式为
pm=EA/l (5) 压溃力效率(crushing force efficiency,CFE)为平均压溃力与峰值压溃力的比值,是评价压溃力均匀性的一个指标。压溃力效率越高,表明压溃力均匀度越好,压溃力效率的表达式为
ηc=pm/pp (6) 2. 实验研究
2.1 材料属性
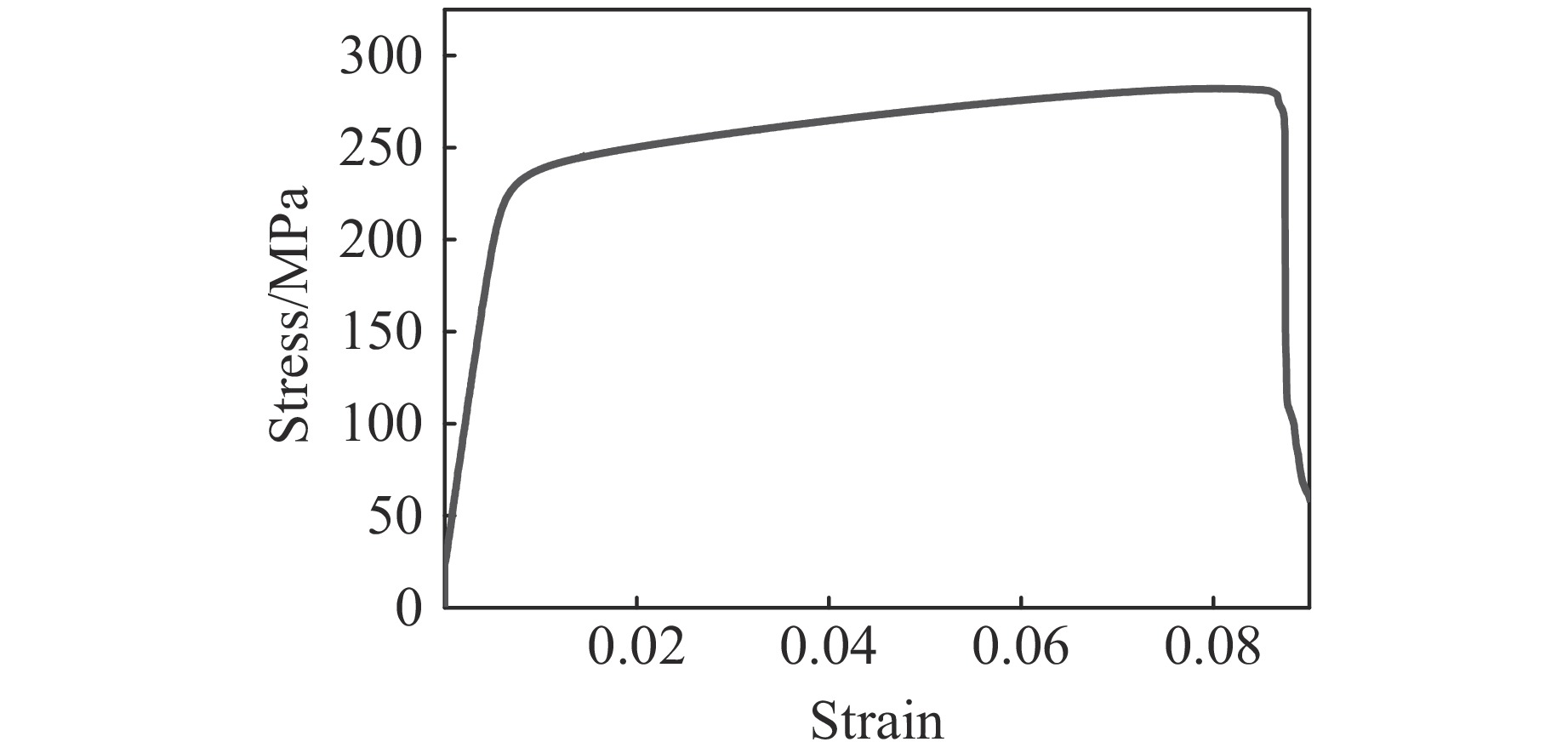
夹芯圆柱壳的材料为AA6061铝合金。首先,采用电火花线切割方法从AA6061铝合金块体上切割出试件。然后,运用万能材料试验机测量试样在准静态拉伸下的力学性能。图3给出了实验获得的应力-应变曲线。从拉伸曲线获得的材料力学性能结果列于表1中。
表 1 AA6061铝合金的材料属性Table 1. Material properties of AA6061 aluminium alloyDensity/(kg·m−3) Young’s modulus/GPa Initial yield stress/MPa Ultimate strength/MPa Poison’s ratio 2.7×103 68.2 227 282 0.3 2.2 实验研究
采用电火花线切割方法对AA6061铝合金进行切割,制备出内径为15、25和35 mm的类向日葵曲线夹芯圆柱壳及其构件,如图4所示。在设计过程中,夹芯圆柱壳的内壳、外壳和芯壳的厚度保持一致,但由于加工误差和磨损,实际测得的壁厚存在一定差异。调整壁厚,使不同尺寸的类向日葵夹芯圆柱壳的质量都尽可能接近0.1 kg。调整后,夹芯圆柱壳及其构件的壁厚t和质量m分别由游标卡尺和电子秤测量得到,结果列于表2中。
表 2 夹芯圆柱壳及其构件的壁厚和质量Table 2. Wall thickness and mass of sandwich cylindrical shells and its componentsItem t/mm m/kg RI=15 mm RI=25 mm RI=35 mm RI=15 mm RI=25 mm RI=35 mm SCS 1.00 1.08 1.11 0.102 0.103 0.103 IS 0.96 0.98 1.04 0.010 0.018 0.025 CS 0.96 1.04 1.10 0.058 0.046 0.037 OS 0.92 1.06 1.08 0.029 0.032 0.032 在准静态压缩条件下进行夹芯圆柱壳及其构件的压缩实验。压缩实验在图5所示的万能材料试验机Zwick Roell Z250上进行。将试件放置在支撑平台与压头之间,压头以5 mm/min的恒定速度压缩试件。当压缩位移为试件高度的70%时,压缩过程自动终止。整个压缩变形过程由数码相机记录,万能材料试验机上的传感器记录夹芯圆柱壳及其构件的力和位移数据。
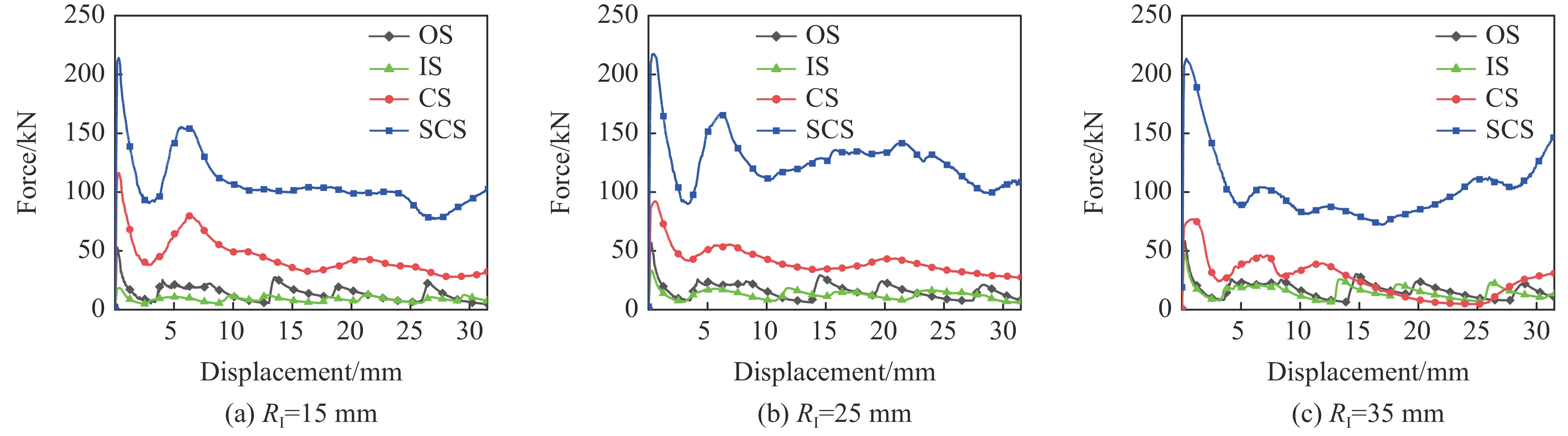
图6给出了实验得到的夹芯圆柱壳及其构件的力-位移曲线,可以看到,夹芯圆柱壳的力-位移曲线远高于构件的力-位移曲线。随着内圆柱壳尺寸的增大,内、外圆柱壳尺寸逐渐接近,内、外圆柱壳的力-位移曲线也逐渐接近。
3. 数值模拟
3.1 夹芯圆柱壳及其构件的有限元模型
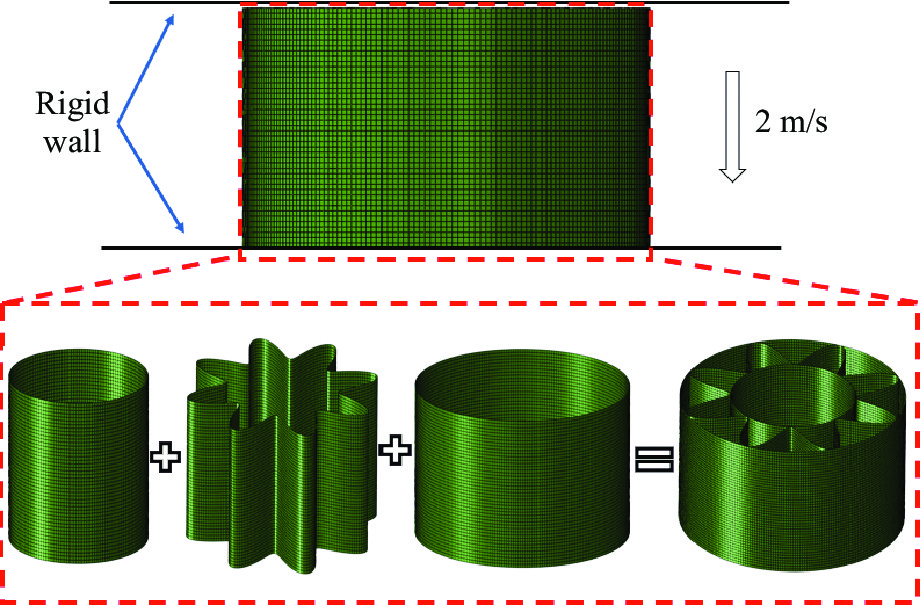
使用有限元分析软件ABAQUS建立夹芯圆柱壳及其构件在轴向压缩下的有限元模型,如图7所示。有限元模型由上、下两个刚性板和中间的壳体组成,上、下板采用离散刚体,上端刚性板以2 m/s的速度向下压缩夹芯圆柱壳,下端刚性板完全固定,上板与夹芯圆柱壳的初始间隙为1 mm。模拟过程采用显式求解器,夹芯圆柱壳与上下板之间设置为通用接触,接触属性为硬接触,摩擦系数设置为0.2[13]。
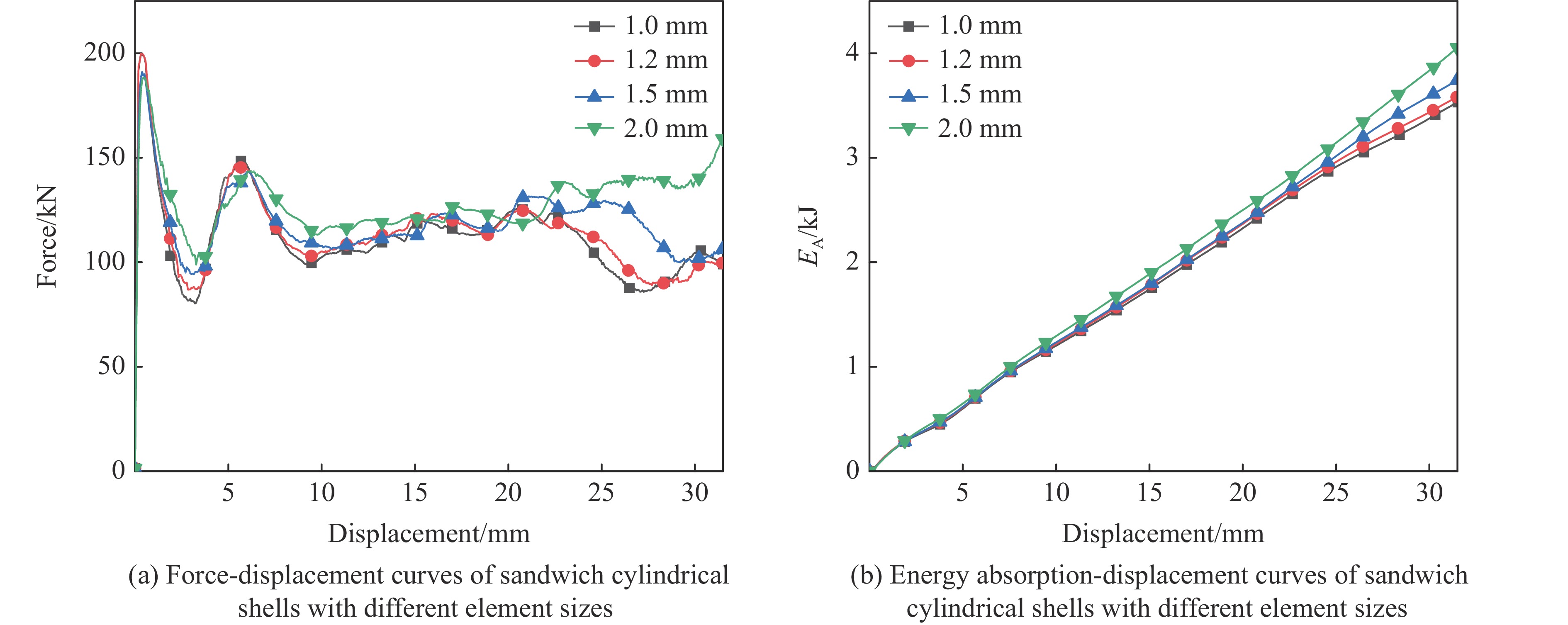
夹芯圆柱壳及其构件使用四节点壳单元(S4R)[14]进行网格划分,S4R壳单元对不同厚度的壳体具有良好的适应性。为了提高精度,在厚度方向设置5个积分点。为了平衡计算成本和模拟精度,以RI=25 mm的夹芯圆柱壳为例进行收敛分析,建立4种不同网格尺寸(边长分别为1.0、1.2、1.5和2.0 mm)的模型,模型计算结果如图8所示,其中:图8(a)为不同网格尺寸的夹芯圆柱壳的力-位移曲线,图8(b)为夹芯圆柱壳的能量吸收-位移曲线。综合考虑计算精度和计算成本,后续算例全部采用边长为1.0 mm的单元网格。
3.2 有限元模型验证
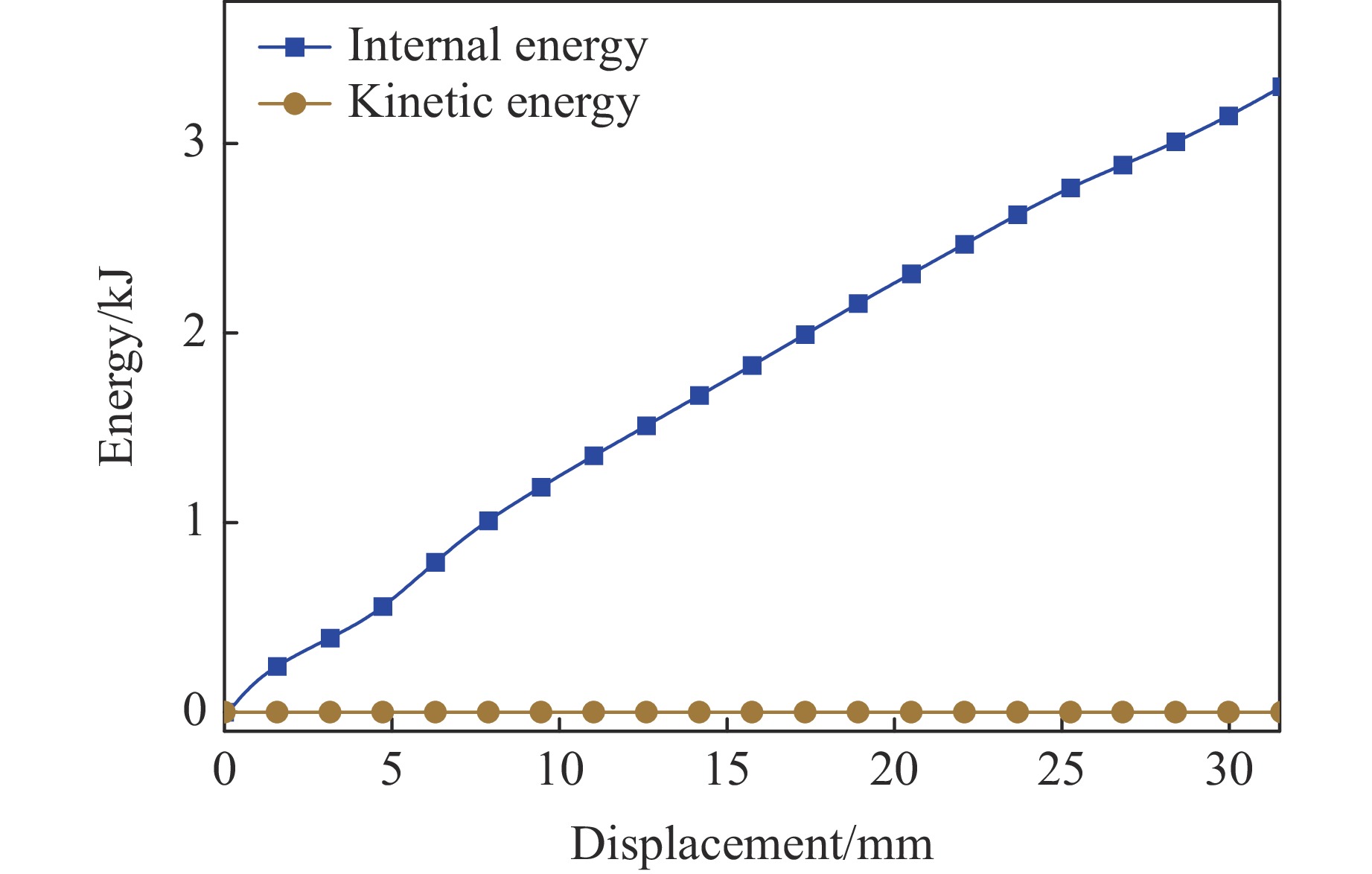
为验证有限元模型的可靠性,依据试件的几何参数(见2.2节),在ABAQUS中建立类向日葵夹芯圆柱壳及其组成构件的有限元模型,并进行了数值模拟。由于在中低速工况下铝合金的应变率敏感性低(应变率对铝合金力学性能的影响可以忽略不计),因此,采用准静态压缩实验方法验证有限元模型的准确性是可靠的。为了降低计算成本,将有限元模型的压缩速度设置为2 m/s。图9给出了压缩速度为2 m/s时内径为25 mm的夹芯圆柱壳的动能与内能的比较。可以看出,在整个压缩过程中,动能小于内能的1%,因此,模拟过程可视为准静态压缩过程[15]。
图10展示了实验和有限元模拟获得的内径为25 mm的夹芯圆柱壳及其构件在不同压缩位移处的变形情况,其中:图10(a)、图10(b)、图10(c)和图10(d)分别为夹芯圆柱壳、内壳、芯壳和外壳的变形情况。可以看出,实验和有限元模拟显示的夹芯圆柱壳的变形过程均为自下端或上端开始的渐进折叠,实验与模拟获得的夹芯圆柱壳的变形模式吻合很好。
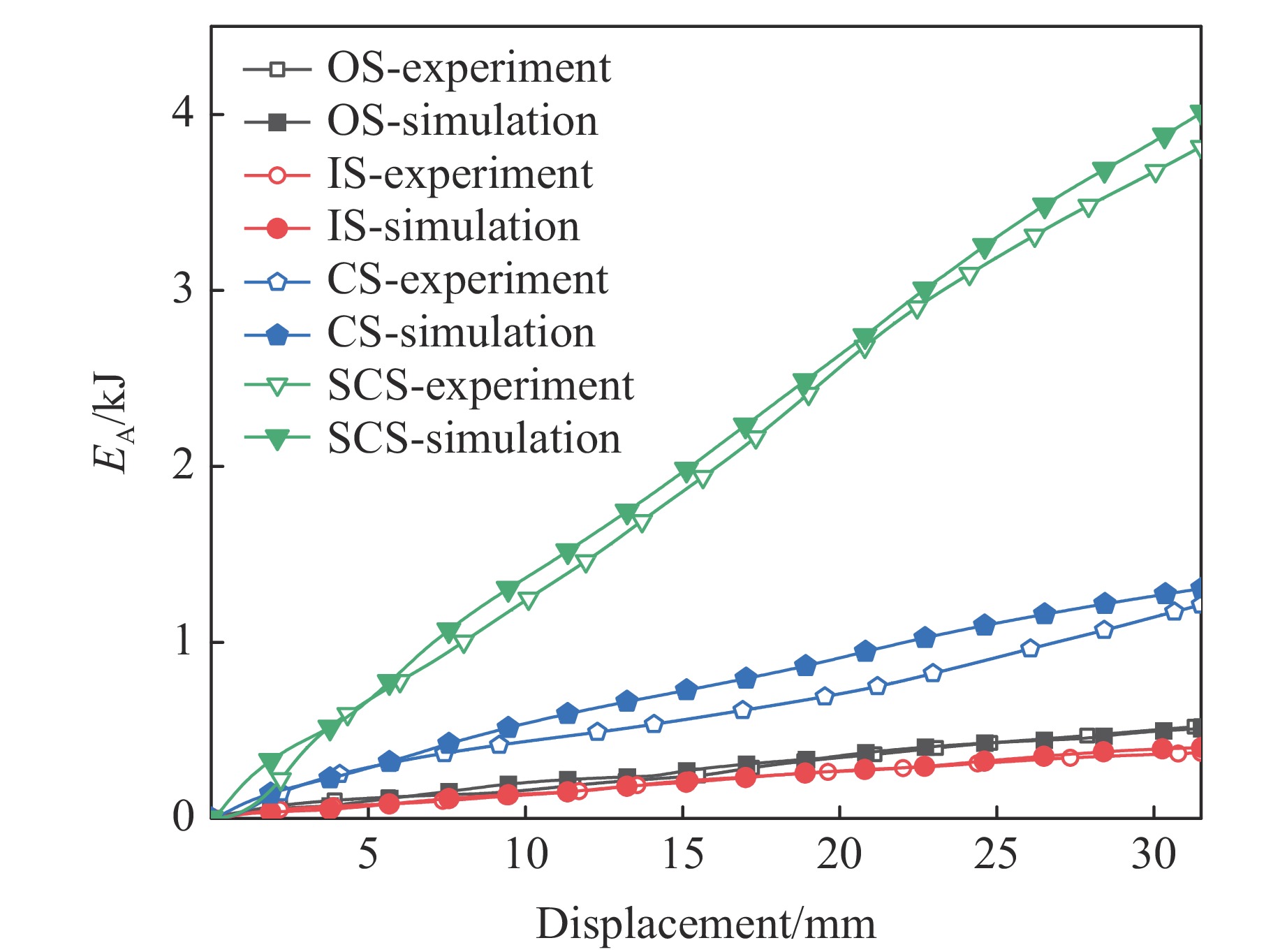
图11为实验和有限元模拟获得的内径为25 mm的类向日葵夹芯圆柱壳及其构件的力-位移曲线,可以看到,在压缩过程中,对于同种结构,实验与模拟获得的力-位移曲线波动幅度比较接近。图12给出了与图11的力-位移曲线对应的能量吸收-位移曲线,可以看出,实验结果与模拟结果同样吻合较好。
表3给出了实验和有限元模拟获得的3种内径尺寸的夹芯圆柱壳及其构件的比能量吸收结果,可以发现建立的有限元模型非常可靠。
表 3 实验和有限元模拟获得的夹芯圆柱壳及其构件的SEA对比Table 3. SEA comparison of experiments and finite element simulaitons of sandwich cylindrical shells and its componentsName ESA for RI=15 mm ESA for RI=25 mm ESA for RI=35 mm Exp./(J·g−1) Sim./(J·g−1) Error/% Exp./(J·g−1) Sim./(J·g−1) Error/% Exp./(J·g−1) Sim./(J·g−1) Error/% SCS 34.82 31.70 −8.95 37.14 37.87 1.93 32.91 30.50 −7.91 IS 30.43 29.69 −2.43 21.16 22.93 7.73 18.54 18.89 1.84 OS 15.23 15.01 −1.46 16.49 16.14 −2.17 15.48 16.45 5.93 CS 21.18 24.44 13.36 26.12 28.10 7.05 23.85 22.63 −5.39 4. 夹芯圆柱壳及其组成构件的吸能能力比较
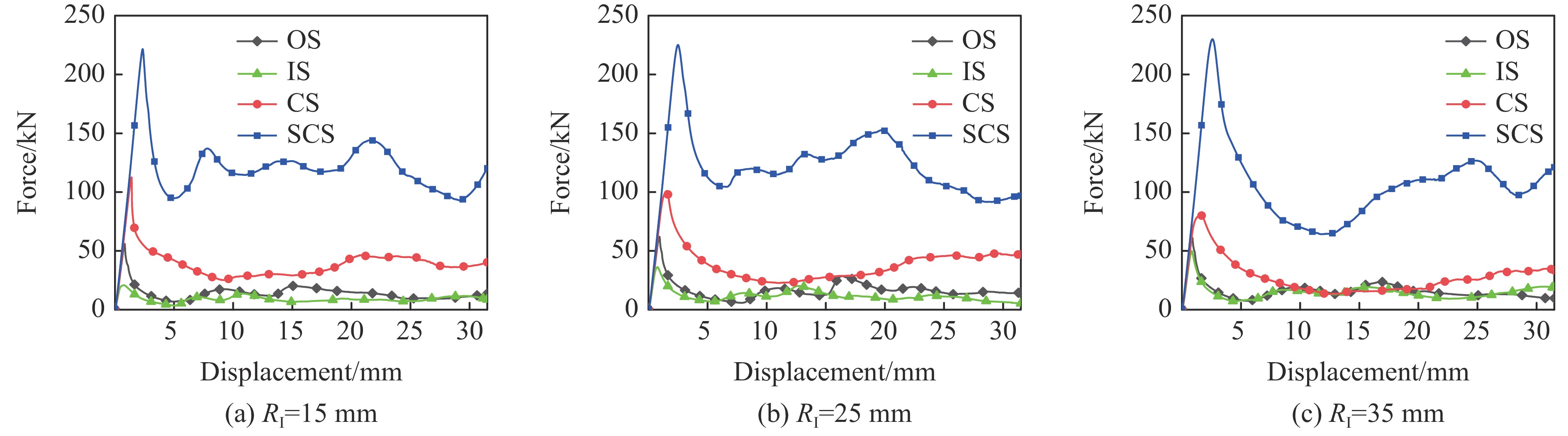
对3种内径的类向日葵曲线夹芯圆柱壳及其构件在轴向压缩过程中的吸能能力进行分析。图13显示了模拟得到的夹芯圆柱壳及其构件的力-位移曲线。可以看出,每条曲线在初始阶段迅速达到峰值力,然后,力逐渐减小并伴随一定的波动。与实验结果一致,模拟结果显示,在压缩过程中,不同内径的夹芯圆柱壳的力-位移曲线远高于对应的芯壳、内壳和外壳,意味着夹芯圆柱壳比其组成构件具有更高的能量吸收能力。
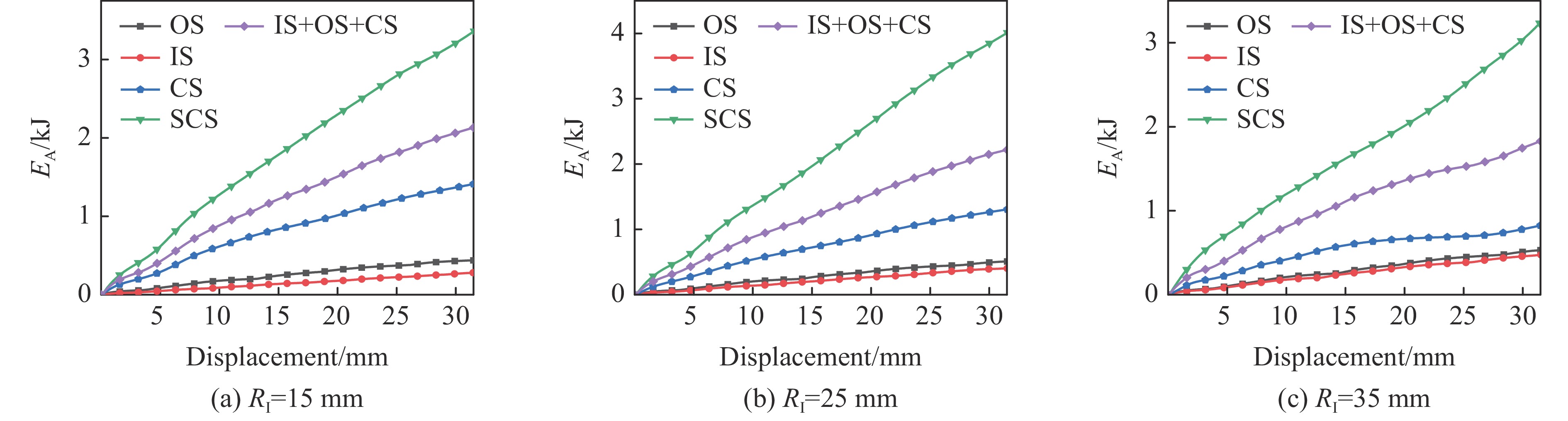
图14给出了有限元模拟得到的夹芯圆柱壳及其构件的能量吸收-位移曲线,可以看出,3种尺寸的夹芯圆柱壳的能量吸收均大于对应的内壳、外壳和芯壳,也大于构件之和。
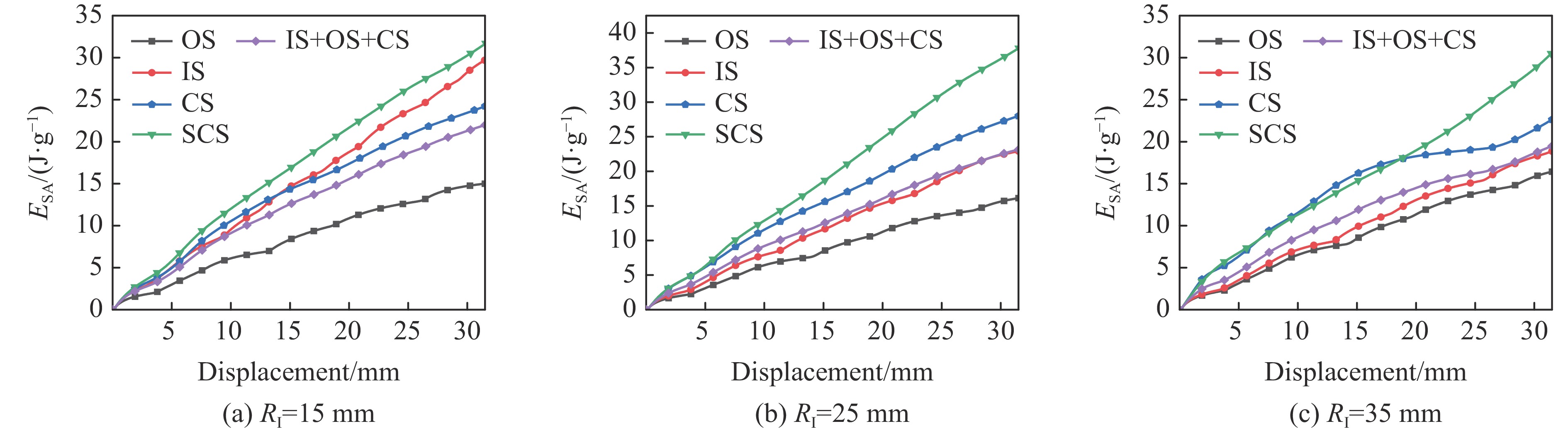
图15比较了有限元模拟得到的夹芯圆柱壳及其构件的比能量吸收,可以看出,夹芯圆柱壳的比能量吸收明显高于各个构件和3个构件之和,所以,夹芯圆柱壳相比其构件具有更好的吸能能力。
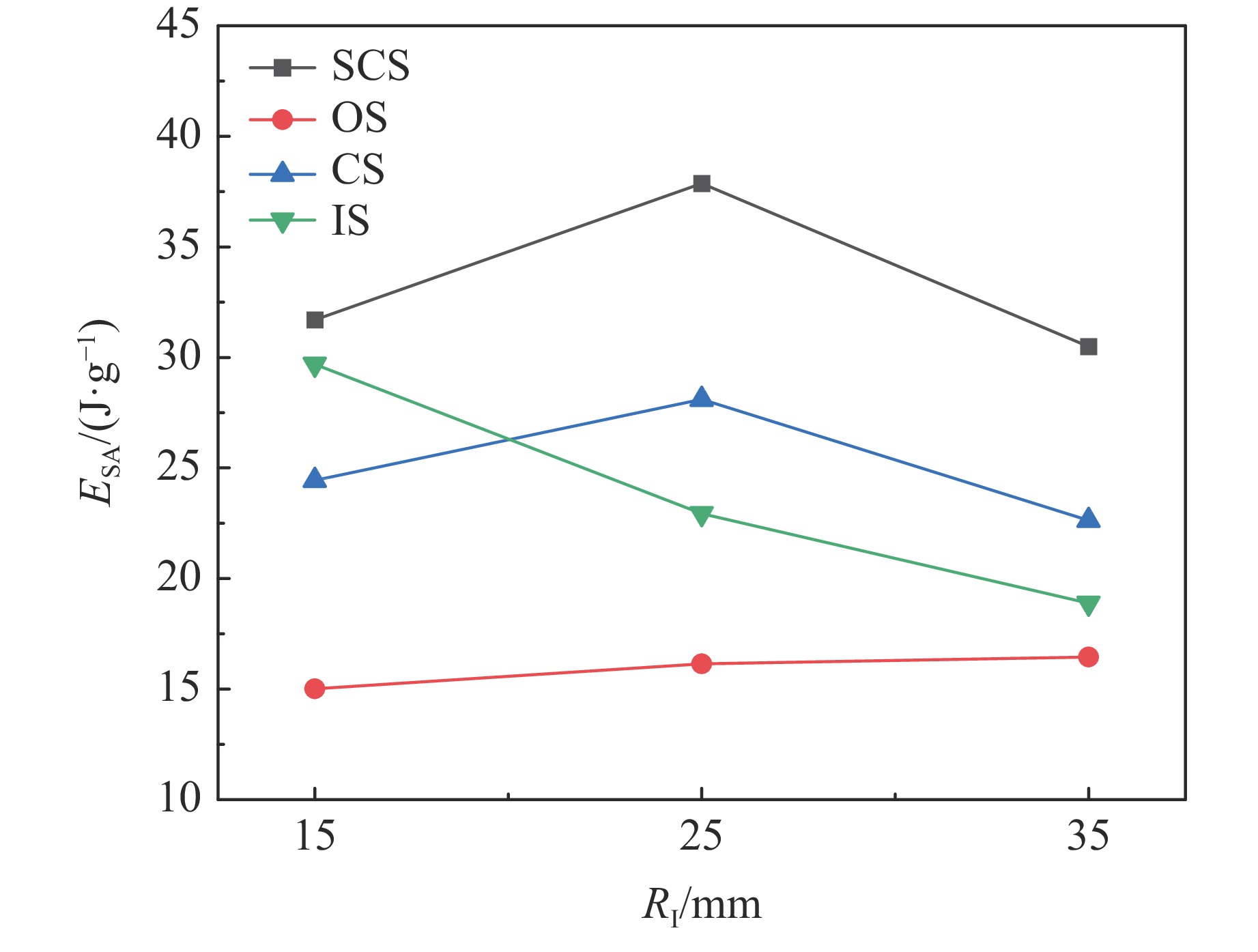
图16为模拟获得的不同内径的夹芯圆柱壳及其构件的比能量吸收。总体来看,夹芯圆柱壳的比能量吸收最高,芯壳和内壳次之,外壳最低。夹芯圆柱壳和芯壳的比能量吸收在内径为25 mm时比内径为15 和35 mm时更高。内壳的比能量吸收在内径为15 mm时最高,因为内径为15 mm的内壳比其他内壳多产生了一个折叠,说明长细比影响变形模式,进而影响吸能效率。
表4给出了内径为25 mm的夹芯圆柱壳及其构件在31.5 mm压缩位移下的吸能特性指标。对于比能量吸收,夹芯圆柱壳比内壳、外壳、芯壳以及构件之和分别高65.0%、134.0%、34.7%和63.2%,芯壳比内壳和外壳分别高22.6%和74.1%。对于压溃力效率,内径为25 mm的夹芯圆柱壳比内壳、外壳、芯壳以及构件之和分别高53.0%、107.0%、30.0%和51.8%,芯壳比内壳和外壳高17.4%和59.0%。这说明夹芯圆柱壳在吸能能力方面优于内壳、外壳和芯壳,也说明芯壳在吸能能力方面优于标准圆柱壳。图17为夹芯圆柱壳及其构件在31.5 mm压缩位移处的变形,结合图10中构件的变形模式可以看出,与内壳和外壳的变形模式相比,芯壳的变形模式更加复杂,产生的塑性折叠占比更大,这是芯壳吸能优于内、外壳的主要原因。
表 4 内径为25 mm的夹芯圆柱壳及其构件的能量吸收性能指标Table 4. Energy absorption performance indicators of sandwich cylindrical shell with the inner diameter of 25 mm and its componentsItem m/kg EA/kJ ESA/(J·g−1) pp/kN ηc SCS 0.106 4.02 37.87 217.36 0.59 IS 0.018 0.40 22.93 33.27 0.38 OS 0.032 0.51 16.14 57.10 0.28 CS 0.047 1.69 28.11 92.15 0.45 综合上述实验和数值模拟结果可以看出,与内壳、外壳和芯壳相比,夹芯圆柱壳具有更好的吸能能力。壳体结构之间的相互作用是吸能性能提高的主要原因。因而,夹芯设计是提高薄壁结构吸能特性的有效方法。芯壳的比能量吸收高于3个构件之和,说明波浪形的芯壳具有更高的吸能密度。所以,波浪形壳体作为夹芯结构的芯体可以提高整个结构的吸能效率。
5. 平均压溃力的理论预测
根据Chen等[16]提出的简化的超级折叠单元理论对夹芯圆柱壳的轴向平均压溃力进行预测。简化的超级折叠单元理论可以很好地应用于多层壳薄壁结构。
当薄壁结构受到载荷作用时,塑性变形是能量吸收的主要途径,包括弯曲变形和薄膜变形两种形式。根据瞬时能量平衡原理,夹芯圆柱壳的瞬时载荷为
pm⋅2λ=Eb+Emη (7) 式中:
2λ 为折叠波长,Eb 为弯曲能,Em 为膜能,η 为有效压溃位移系数。根据Abramowicz等[17]的实验结果,角单元在压缩过程中并未完全平展,导致折叠波长实际上小于2λ 。因此,系数η 被纳入式(7)中。在该理论模型中,系数η 取0.8。5.1 弯曲变形能
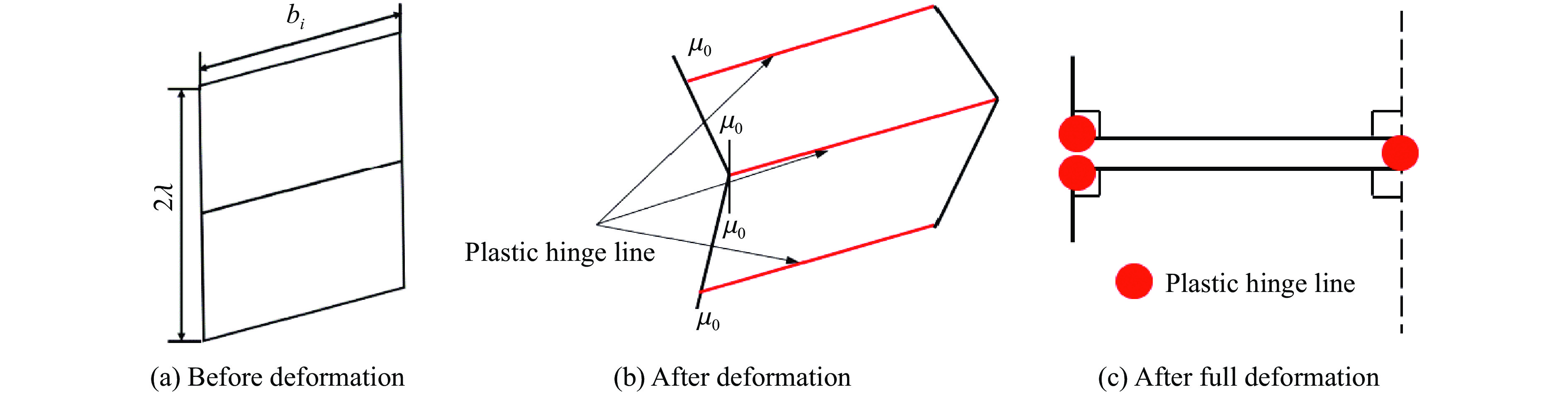
根据简化的超级折叠单元理论,每个平面弯曲变形所耗散的能量为塑性铰链弯曲所需的能量。图18为一个基本折叠单元的折叠过程,在每个平面中,弯曲变形所耗散的能量等于塑性铰链的弯曲能。
弯曲能
Eb 可以表示为Eb=z∑i=1M0biμ0 (8) 式中:z为折叠单元的个数,
μ0 为弯曲过程中铰链的旋转角;bi 为折叠单元的边长;弯矩M0=t2σ04 ,其中,σ0 为材料的流动应力。本研究中,使用屈服强度σu 和极限强度σy 的平均值[18]来表示流动应力σ0=(σu+σy)2 (9) 因此,AA6061铝合金材料的流动应力
σ0 为254.6 MPa。在基本折叠单元的折叠过程中,假定其完全折叠,即图18(c)所示的4个旋转角都为90°。假定各平面的变形相似,则总的弯曲能可以表示为
Eb=2πBM0 (10) 式中:B为夹芯圆柱壳横截面上每个构件的边长总和。结合式(1)和式(2),根据几何特性可知B的表达式为
B=2π(RI+RO)+∫2π0√X′2(θ)+Y′2(θ)dθ (11) 式中:
X′(θ) 和Y′(θ) 分别为X(θ) 和Y(θ) 对θ 的导数。5.2 膜变形能
膜变形能
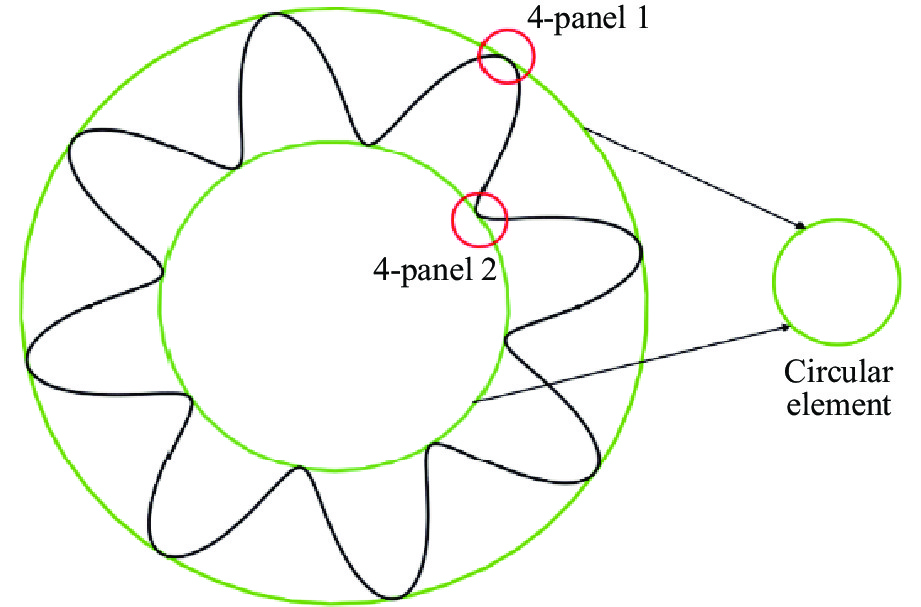
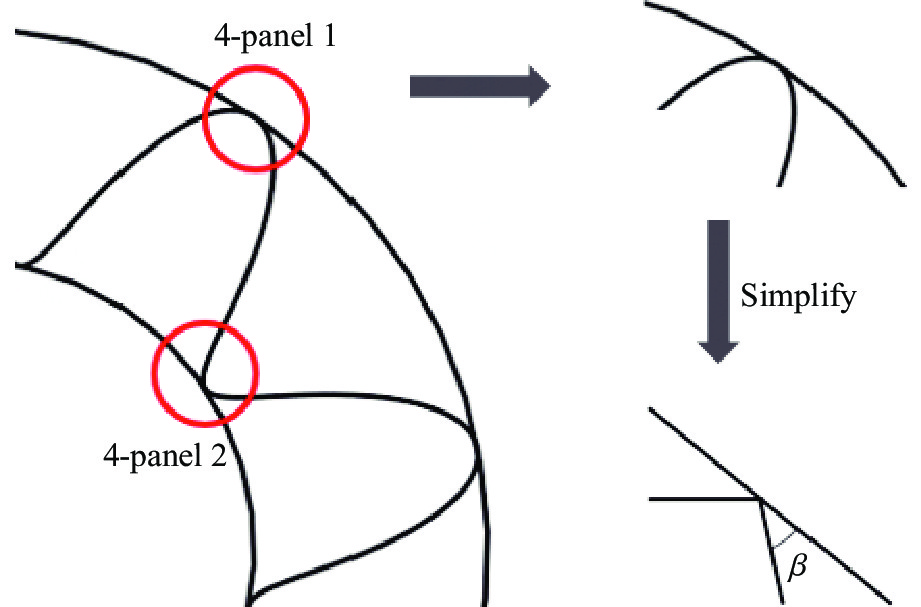
Em 与角单元的类型有关。为表征不同角单元的膜变形能,根据夹芯圆柱壳的截面特性,将角单元划分为4面板角单元(4-panel)和圆形单元(circular element)两种基本类型。在一个夹芯圆柱壳中,4面板角单元在内壳和外壳处的夹角不同。根据夹角的不同,将4面板角单元分为4面板角单元1(4-panel 1)和4面板角单元2(4-panel 2)。不同类型的角单元如图19所示。(1) 4面板角单元的膜变形能
4面板角单元由两个弯曲面板和两个圆弧面板组成。薄壁结构的折叠特性决定了膜能量的耗散主要发生在角单元相交的区域,并且折叠区域的范围主要与相交面板的角度有关。因此,将弯曲面板用曲面板交点处的切平面代替的简化方法是可行的[19]。简化过程如图20所示,简化后的4面板角单元由4个直面板组成。
4面板角单元的膜能量公式[20]为
E4−panelm=8M0λ2t(1+1cosβ) (12) 式中:β为图20所示的两个板之间的夹角,可在建模软件中直接测量。
(2) 圆形单元的膜变形能
对于圆形单元,膜能通过对圆形单元进行压缩或拉伸而产生,在整个褶皱形成过程中,圆形单元的膜能量耗散公式[14]为
ECircularm=8πM0λ2t (13) 5.3 平均压溃力
根据夹芯圆柱壳的几何特征,将每个夹芯圆柱壳分为2个圆形单元、N个4-panel 1和N个4-panel 2,所以,总的膜能表示为
Em=2ECircularm+NEm1+NEm2 (14) 将式(10)和式(14)代入式(7),得
pm⋅2λ=1η(2πBM0+cM0λ2t) (15) 式中:
c=16π+16N+8N(cosβ1+cosβ2)cosβ1cosβ2 ,β1 和β2 分别为4-panel 1和4-panel 2对应的夹角。假设所有折叠波长相等,根据静态平衡条件,可得
∂pm∂λ=0 (16) 求解式(16)得到
λ=(2πtBc)0.5 (17) 将
λ 代入式(15),得到夹芯圆柱壳在准静态条件下的平均压溃力理论公式为pm=1η√π8B0.5t1.5σ0c0.5 (18) 5.4 理论公式的验证
为了验证理论公式的准确性,将理论预测的平均压溃力分别与模拟和实验的平均压溃力比较,对比结果列于表5中。不难发现,理论预测结果与有限元模拟及实验结果都非常接近,理论预测与模拟的最大误差为5.03%,与实验的最大误差为8.17%。因此,可以认为推导的夹芯圆柱壳的平均压溃力的理论公式具有足够高的准确性。
表 5 理论预测与实验及模拟结果的对比Table 5. Comparison of theoretical predictions with experiments and simulationsRI/mm t/mm pm/kN Error/% Theory Sim. Exp. Theory relative to simulation Theory relative to experiment 15 1.00 109.1 106.8 112.2 2.15 −2.76 25 1.08 115.1 121.2 121.2 −5.03 −5.03 35 1.11 116.5 114.2 107.7 2.01 8.17 6. 类向日葵夹芯圆柱壳的多目标优化
优化设计流程通常包含如下步骤:明确优化问题,进行试验设计,得到样本点并进行数值模拟,基于样本点的数值模拟结果构建近似模型,对近似模型进行误差分析。如果近似模型的精度不满足要求,则需要重新进行试验设计;如果精度满足要求,则可以使用适当的优化算法对近似模型进行优化,最终得到优化目标的Pareto前沿。
为了得到吸能性能更好且峰值压溃力更低的类向日葵夹芯圆柱壳结构,将峰值压溃力和比吸能设置为目标函数,将壁厚t、内径RI和外径RO定义为设计变量,以N=12的夹芯圆柱壳为研究对象,进行多目标优化。优化问题定义为
minf(x)=[pp(x),−ESA(x)],x=[RI,RO,t]Ts.t.{0.5mm⩽t⩽1.5mm,22mm⩽RI⩽32mm,36mm⩽RO⩽46mm (19) 最优拉丁超立方设计(optimal Latin hypercube design,OLHD)被用来获取采样点。该方法具有效率高和准确性高的优点,结合了拉丁超立方设计和优化算法,可以避免设计中的随机性和重复性,当试验因素的水平数比较多时,该方法所需的试验次数较少,并且能得到满足要求的高精度结果。尽管试验次数较少,该方法仍能获得准确的响应曲面。采用最优拉丁超立方设计方法[21]均匀地划分式(19)的设计空间,并随机组合划分出的水平值,采集了20个样本点。该方法采集到的样本点在设计空间中非常均匀。根据选取的样本点的参数信息建立有限元模型 ,使用ABAQUS软件进行数值模拟,得到20个夹芯圆柱壳的比吸能和峰值压溃力,见表6。
表 6 夹芯圆柱壳的样本点及响应值Table 6. Sample points and response values of sandwich cylindrical shellsNo. RI/mm RO/mm t/mm pp/kN ESA/(J·g−1) 1 23.05 37.05 0.816 154.1 43.7 2 23.58 46.00 1.079 273.3 41.6 3 32.00 38.11 0.763 129.5 40.4 4 27.79 36.53 0.711 119.2 41.9 5 26.74 43.37 0.974 217.1 42.5 6 31.47 42.32 0.868 170.9 40.5 7 28.32 44.42 1.500 360.6 58.7 8 28.84 44.95 0.658 142.6 34.7 9 22.00 38.63 1.237 269.4 52.7 10 22.53 41.79 0.921 208.2 40.6 11 30.95 41.26 1.289 262.3 53.8 12 25.16 39.68 0.553 104.7 33.3 13 24.63 43.89 0.605 132.9 30.4 14 29.89 37.58 1.184 213.8 51.7 15 25.68 36.00 1.342 249.7 60.6 16 29.37 40.74 0.500 91.6 30.7 17 30.42 45.47 1.132 258.8 47.3 18 24.11 42.84 1.395 337.4 52.7 19 27.26 40.21 1.447 307.0 62.0 20 26.21 39.16 1.026 203.3 48.0 构建近似模型是指利用已有数据,通过数学方法建立一个能够准确描述数据规律的模型,这个模型称为代理模型,可以用于预测新的数据。构建代理模型可以替代有限元模拟过程中大量的迭代计算,既可以满足计算精度要求,又可以节省时间和人力成本。使用表6中样本点的设计变量和响应结果构建近似模型。吸能问题主要采用的近似建模技术有多项式响应面模型(response surface methodology,RSM)、克里金模型(Kriging,KRG)、径向基函数神经网络模型(radial basis function,RBF)。然而,这些不同的代理技术往往提供不同的建模精度、梯度信息和设计结果,适用于不同的优化问题。为此,将对这3种近似建模技术的精度进行对比,选择最优的代理模型。
一般常用统计量确定性系数(R2)、均方根(P)和最大相对误差(Q)评价近似模型的精度,表达式如下
R2=1−n∑i=1(yi−ˆyi)2n∑i=1(yi−ˉyi)2 (20) P=√n∑i=1(ˆyi−yi)2n (21) Q=maxi=1,2,…,n(|ˆyi−yi||yi|) (22) 式中:n为样本点数,
yi 为样本点的数值模拟结果,ˆyi 为近似模型的预测结果,ˉyi 为模拟结果的平均值。采用R2、P和Q共同评价近似模型的精度。其中R2和P用来评估近似模型的整体精度,而Q主要用来评估模型的局部精度。一般来说,当R2>0.9,P<0.2且Q<0.3时,认为构造的代理模型可靠性较高,满足工程预测精度要求。求。
基于表6中采集的样本点以及响应值,构建夹芯圆柱壳的比吸能和峰值压溃力的RSM(阶数为2阶)、KRG和RBF 3种近似模型。表7显示,3种近似模型的确定性系数R2都超过0.9,均方根误差都小于0.1,并且最大相对误差都小于0.2,精度都在工程预测精度范围要求内,因此,3种代理模型都满足精度要求。综合比较3种代理模型的评价指标后发现,RSM代理模型更优,所以选用RSM代理模型进行后续的优化分析。采用RSM代理模型建立的响应变量的二次多项式为
表 7 夹芯圆柱壳近似模型的预测精度评估Table 7. Evaluation of prediction accuracy of the approximation models for sandwich cylindrical shellModel R2 P Q pp ESA pp ESA pp ESA KRG 0.9742 0.9687 0.0458 0.0520 0.1501 0.1354 RBF 0.9951 0.9737 0.0198 0.0476 0.0683 0.1088 RSM 0.9993 0.9718 0.0076 0.0494 0.0171 0.1137 ESA=155.55+0.04x1−5.99x2+23.52x3−0.07x21+0.03x22+2.09x23+0.09x1x2+0.09x1x3−0.02x2x3 (23) pp=13.06−2.98x1+1.51x2−9.23x3+0.09x21−0.02x22+26.89x23−0.02x1x2−4.33x1x3+7.53x2x3 (24) 式中:x1、x2和x3分别为RI、RO和t。
NSGA-Ⅱ非支配排序遗传算法改进了传统的遗传算法,在求解多目标优化问题时,计算速度更快并且能更好地满足精度要求。因此,使用NSGA-Ⅱ非支配排序遗传算法求解上述RSM代理模型。NSGA-Ⅱ算法的具体参数设置列于表8。经过482次计算,得到136组最优解。
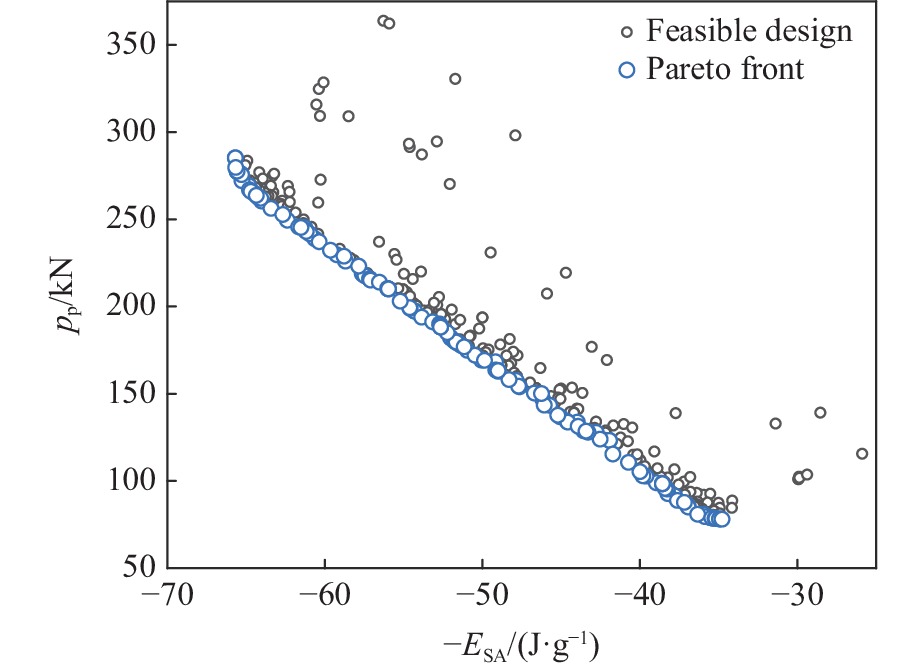
表 8 NSGA-Ⅱ算法参数设置Table 8. Parameters of NSGA-Ⅱ algorithmPopulation size Number of iterations Crossover probability Crossover distribution index Mutation distribution index 12 40 0.9 10 20 图21为NSGA-Ⅱ算法得到的最优解集的Pareto前沿,可以观察到峰值压溃力和比吸能是两个相互制约的量,若想得到高的比能量吸收,便会引起峰值压溃力的增加。Pareto前沿上的任何一个点都是最优结果,可以根据实际情况进行选取。例如,选取峰值压溃力在205 kN附近的最优解,对应的夹芯圆柱壳的几何参数为RI=28.94 mm,RO=36.80 mm, t=1.16 mm。
对选取的最优解进行模拟验证。在ABAQUS软件中,采用选取的最优解对应的参数进行建模和模拟计算,将得到的峰值压溃力和比能量吸收结果与选取的最优解对应的峰值压溃力和比能量吸收进行比较,如表9所示。可以发现,相比于最优解,模拟计算获得的峰值压溃力和比能量吸收的误差分别为1.0%和2.9%,说明优化结果精度较高。
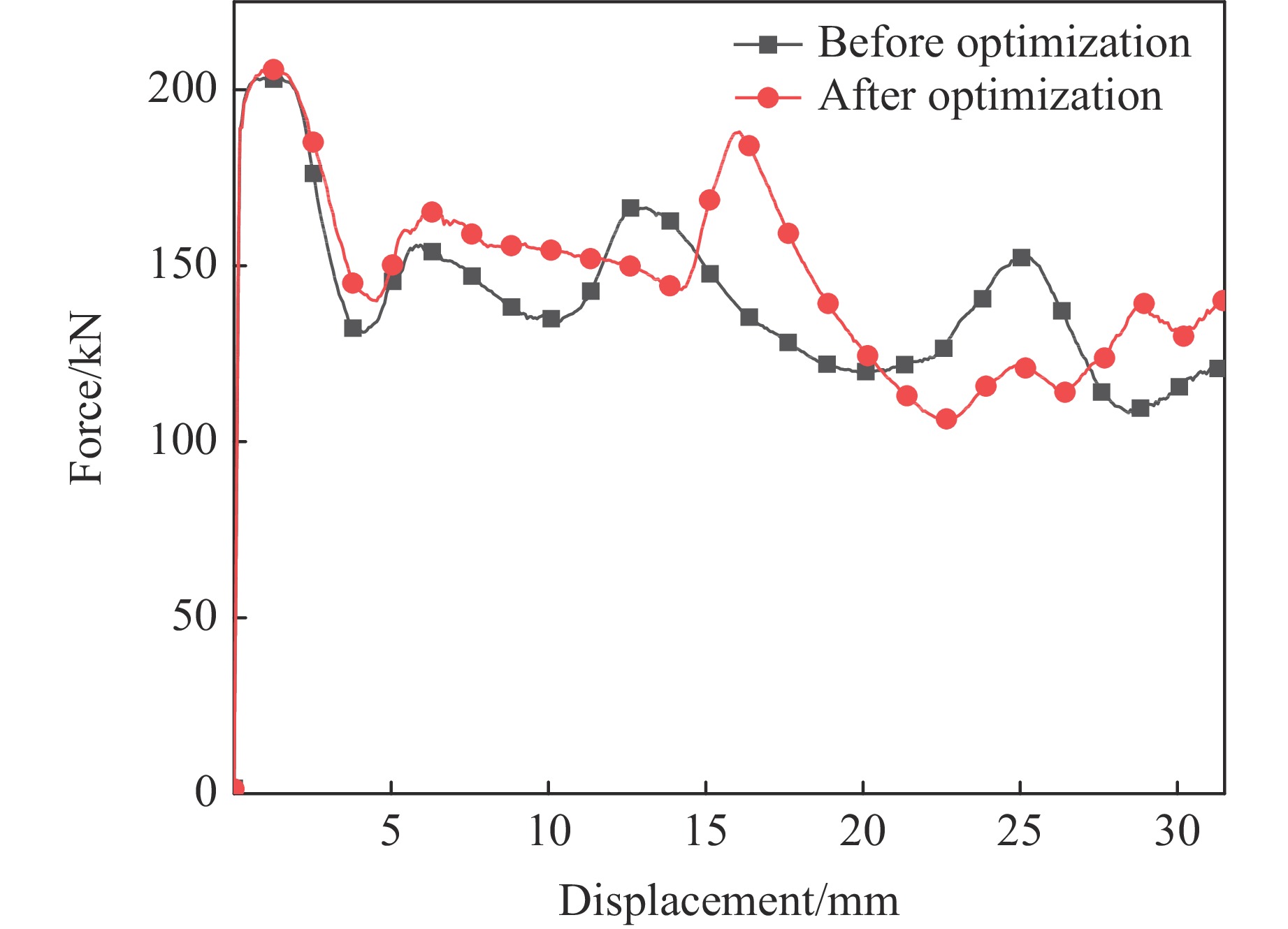
表 9 优化获得的PCF和 SEA 与有限元结果对比Table 9. PCF and SEA comparison between optimization results and finite element resultspp/kN ESA/(J·g−1) Error/% Optimization Simulation Optimization Simulation pp ESA 204.1 206.2 53.7 52.2 1.0 2.9 为了直观地呈现优化后的夹芯圆柱壳的能量吸收能力的提升和结构轻量化效果,选取峰值压溃力在205 kN附近的优化前后的夹芯圆柱壳的模拟结果进行对比,即将最优解对应的夹芯圆柱壳与表6中No.20夹芯圆柱壳的模拟结果进行对比。优化前后夹芯圆柱壳的力-位移曲线显示在图22中,具体的数值列于表10中。图22显示,优化前后峰值压溃力基本不变,优化后的力-位移曲线的波动范围有所扩大。从表10可以看出,在同等峰值压溃力下,相比于优化前,优化后夹芯圆柱壳的比能量吸收提高了8.75%,平均压溃力提高了4.43%,质量减轻了4.35%。所以,优化后的夹芯圆柱壳在提高能量吸收能力的同时达到了减重的目标。
表 10 优化前后模拟结果的对比Table 10. Comparison of simulation results before and after optimizationCase pp/kN ESA/(J·g−1) pm/kN m/kg Before optimization 203.3 48.0 140.0 0.092 After optimization 206.2 52.2 146.2 0.088 Change/% 1.43 8.75 4.43 −4.35 7. 结 论
通过实验、理论和有限元模拟,系统研究了类向日葵夹芯圆柱壳及其组成构件在轴向准静态压缩下的吸能特性,得到以下结论。
(1)在薄壁圆柱壳的能量吸收能力方面,夹芯圆柱壳结构比单个构件具有更大的潜力。波浪形芯壳比内壳和外壳具有更好的能量吸收能力,因此,夹芯圆柱壳和波浪形曲线芯壳的联合使用可以有效地提高薄壁金属结构的吸能效率。
(2)随着内径尺寸的增加,夹芯圆柱壳和芯壳的比能量吸收先增大后减小,而内壳由于变形模式的变化,内壳的比能量吸收单调减小。内径尺寸为25 mm的夹芯圆柱壳相比其他内径的夹芯圆柱壳具有更高的比能量吸收,同时,内径尺寸为25 mm的芯壳相比其他内径的芯壳具有更高的比能量吸收。
(3)基于简化的超级折叠单元理论建立的理论模型可以准确地预测夹芯圆柱壳的平均压溃力。理论预测的平均压溃力与实验结果相比,最大误差为8.17%;理论预测的平均压溃力与有限元模拟结果相比,最大误差为5.03%。理论预测的最大误差都在10%以内。
(4)以RI、RO和t为设计变量,将比吸能最大和峰值压溃力最小作为优化目标,得到了夹芯圆柱壳的Pareto前沿,优化后的夹芯圆柱壳同时实现了比吸能的提升和结构的轻量化。
-
表 1 AA6061铝合金的材料属性
Table 1. Material properties of AA6061 aluminium alloy
Density/(kg·m−3) Young’s modulus/GPa Initial yield stress/MPa Ultimate strength/MPa Poison’s ratio 2.7×103 68.2 227 282 0.3 表 2 夹芯圆柱壳及其构件的壁厚和质量
Table 2. Wall thickness and mass of sandwich cylindrical shells and its components
Item t/mm m/kg RI=15 mm RI=25 mm RI=35 mm RI=15 mm RI=25 mm RI=35 mm SCS 1.00 1.08 1.11 0.102 0.103 0.103 IS 0.96 0.98 1.04 0.010 0.018 0.025 CS 0.96 1.04 1.10 0.058 0.046 0.037 OS 0.92 1.06 1.08 0.029 0.032 0.032 表 3 实验和有限元模拟获得的夹芯圆柱壳及其构件的SEA对比
Table 3. SEA comparison of experiments and finite element simulaitons of sandwich cylindrical shells and its components
Name ESA for RI=15 mm ESA for RI=25 mm ESA for RI=35 mm Exp./(J·g−1) Sim./(J·g−1) Error/% Exp./(J·g−1) Sim./(J·g−1) Error/% Exp./(J·g−1) Sim./(J·g−1) Error/% SCS 34.82 31.70 −8.95 37.14 37.87 1.93 32.91 30.50 −7.91 IS 30.43 29.69 −2.43 21.16 22.93 7.73 18.54 18.89 1.84 OS 15.23 15.01 −1.46 16.49 16.14 −2.17 15.48 16.45 5.93 CS 21.18 24.44 13.36 26.12 28.10 7.05 23.85 22.63 −5.39 表 4 内径为25 mm的夹芯圆柱壳及其构件的能量吸收性能指标
Table 4. Energy absorption performance indicators of sandwich cylindrical shell with the inner diameter of 25 mm and its components
Item m/kg EA/kJ ESA/(J·g−1) pp/kN ηc SCS 0.106 4.02 37.87 217.36 0.59 IS 0.018 0.40 22.93 33.27 0.38 OS 0.032 0.51 16.14 57.10 0.28 CS 0.047 1.69 28.11 92.15 0.45 表 5 理论预测与实验及模拟结果的对比
Table 5. Comparison of theoretical predictions with experiments and simulations
RI/mm t/mm pm/kN Error/% Theory Sim. Exp. Theory relative to simulation Theory relative to experiment 15 1.00 109.1 106.8 112.2 2.15 −2.76 25 1.08 115.1 121.2 121.2 −5.03 −5.03 35 1.11 116.5 114.2 107.7 2.01 8.17 表 6 夹芯圆柱壳的样本点及响应值
Table 6. Sample points and response values of sandwich cylindrical shells
No. RI/mm RO/mm t/mm pp/kN ESA/(J·g−1) 1 23.05 37.05 0.816 154.1 43.7 2 23.58 46.00 1.079 273.3 41.6 3 32.00 38.11 0.763 129.5 40.4 4 27.79 36.53 0.711 119.2 41.9 5 26.74 43.37 0.974 217.1 42.5 6 31.47 42.32 0.868 170.9 40.5 7 28.32 44.42 1.500 360.6 58.7 8 28.84 44.95 0.658 142.6 34.7 9 22.00 38.63 1.237 269.4 52.7 10 22.53 41.79 0.921 208.2 40.6 11 30.95 41.26 1.289 262.3 53.8 12 25.16 39.68 0.553 104.7 33.3 13 24.63 43.89 0.605 132.9 30.4 14 29.89 37.58 1.184 213.8 51.7 15 25.68 36.00 1.342 249.7 60.6 16 29.37 40.74 0.500 91.6 30.7 17 30.42 45.47 1.132 258.8 47.3 18 24.11 42.84 1.395 337.4 52.7 19 27.26 40.21 1.447 307.0 62.0 20 26.21 39.16 1.026 203.3 48.0 表 7 夹芯圆柱壳近似模型的预测精度评估
Table 7. Evaluation of prediction accuracy of the approximation models for sandwich cylindrical shell
Model R2 P Q pp ESA pp ESA pp ESA KRG 0.9742 0.9687 0.0458 0.0520 0.1501 0.1354 RBF 0.9951 0.9737 0.0198 0.0476 0.0683 0.1088 RSM 0.9993 0.9718 0.0076 0.0494 0.0171 0.1137 表 8 NSGA-Ⅱ算法参数设置
Table 8. Parameters of NSGA-Ⅱ algorithm
Population size Number of iterations Crossover probability Crossover distribution index Mutation distribution index 12 40 0.9 10 20 表 9 优化获得的PCF和 SEA 与有限元结果对比
Table 9. PCF and SEA comparison between optimization results and finite element results
pp/kN ESA/(J·g−1) Error/% Optimization Simulation Optimization Simulation pp ESA 204.1 206.2 53.7 52.2 1.0 2.9 表 10 优化前后模拟结果的对比
Table 10. Comparison of simulation results before and after optimization
Case pp/kN ESA/(J·g−1) pm/kN m/kg Before optimization 203.3 48.0 140.0 0.092 After optimization 206.2 52.2 146.2 0.088 Change/% 1.43 8.75 4.43 −4.35 -
[1] JOHNSON W, SODEN P D, AL-HASSANI S T S. Inextensional collapse of thin-walled tubes under axial compression [J]. The Journal of Strain Analysis for Engineering Design, 1977, 12(4): 317–330. doi: 10.1243/03093247V124317 [2] ANDREWS K R F, ENGLAND G L, GHANI E. Classification of the axial collapse of cylindrical tubes under quasi-static loading [J]. International Journal of Mechanical Sciences, 1983, 25(9/10): 687–696. [3] 李志斌, 虞吉林, 郑志军, 等. 薄壁管及其泡沫金属填充结构耐撞性的实验研究 [J]. 实验力学, 2012, 27(1): 77–86.LI Z B, YU J L, ZHENG Z J, et al. An experimental study on the crashworthiness of thin-walled tubes and their metallic foam-filled structures [J]. Journal of Experimental Mechanics, 2012, 27(1): 77–86. [4] ALI M, OHIOMA E, KRAFT F, et al. Theoretical, numerical, and experimental study of dynamic axial crushing of thin walled pentagon and cross-shape tubes [J]. Thin-Walled Structures, 2015, 94: 253–272. doi: 10.1016/j.tws.2015.04.007 [5] 荆友录, 温卫东, 魏民祥. 不同截面结构薄壁直梁的轴向耐撞性研究 [J]. 机械科学与技术, 2009, 28(4): 455–459. doi: 10.3321/j.issn:1003-8728.2009.04.008JING Y L, WEN W D, WEI M X. A study of the axial crashworthiness of thin-walled straight beams with different shapes [J]. Mechanical Science and Technology for Aerospace Engineering, 2009, 28(4): 455–459. doi: 10.3321/j.issn:1003-8728.2009.04.008 [6] VINAYAGAR K, SENTHIL KUMAR A. Crashworthiness analysis of double section bi-tubular thin-walled structures [J]. Thin-Walled Structures, 2017, 112: 184–193. doi: 10.1016/j.tws.2016.12.008 [7] ZHENG G, WU S Z, SUN G Y, et al. Crushing analysis of foam-filled single and bitubal polygonal thin-walled tubes [J]. International Journal of Mechanical Sciences, 2014, 87: 226–240. doi: 10.1016/j.ijmecsci.2014.06.002 [8] GOEL M D. Deformation, energy absorption and crushing behavior of single-, double- and multi-wall foam filled square and circular tubes [J]. Thin-Walled Structures, 2015, 90: 1–11. doi: 10.1016/j.tws.2015.01.004 [9] CHEN H C, ZHANG Y, LIN J M, et al. Crushing responses and optimization of novel sandwich columns [J]. Composite Structures, 2021, 263: 113682. doi: 10.1016/j.compstruct.2021.113682 [10] 马梦娇, 刘志芳, 李世强. 波纹芯层夹芯管的轴向压缩吸能特性与多目标优化 [J]. 高压物理学报, 2022, 36(6): 064201.MA M J, LIU Z F, LI S Q. Energy absorption and multi-objective optimization for sandwich tubes with a corrugated core under axial compression [J]. Chinese Journal of High Pressure Physics, 2022, 36(6): 064201. [11] 葛平政, 张勇. 新颖波纹截面薄壁圆管的耐撞性 [J]. 华侨大学学报(自然科学版), 2016, 37(5): 531–535.GE P Z, ZHANG Y. Crashworthiness of novel thin-walled circular tube with novel corrugated cross section [J]. Journal of Huaqiao University (Natural Science), 2016, 37(5): 531–535. [12] 闫栋, 王根伟, 宋辉, 等. 类向日葵夹芯圆柱壳径向冲击数值模拟 [J]. 高压物理学报, 2020, 34(5): 054201.YAN D, WANG G W, SONG H, et al. Numerical simulation of radial impact on sunflower-like sandwich cylindrical shell [J]. Chinese Journal of High Pressure Physics, 2020, 34(5): 054201. [13] YIN H F, WEN G L, HOU S J, et al. Crushing analysis and multiobjective crashworthiness optimization of honeycomb-filled single and bitubular polygonal tubes [J]. Materials & Design, 2011, 32(8/9): 4449−4460. [14] ZHANG Y, XU X, WANG J, et al. Crushing analysis for novel bio-inspired hierarchical circular structures subjected to axial load [J]. International Journal of Mechanical Sciences, 2018, 140: 407–431. doi: 10.1016/j.ijmecsci.2018.03.015 [15] LI W W, LUO Y H, LI M, et al. A more weight-efficient hierarchical hexagonal multi-cell tubular absorber [J]. International Journal of Mechanical Sciences, 2018, 140: 241–249. doi: 10.1016/j.ijmecsci.2018.03.006 [16] CHEN W G, WIERZBICKI T. Relative merits of single-cell, multi-cell and foam-filled thin-walled structures in energy absorption [J]. Thin-Walled Structures, 2001, 39(4): 287–306. doi: 10.1016/S0263-8231(01)00006-4 [17] ABRAMOWICZ W, JONES N. Dynamic axial crushing of square tubes [J]. International Journal of Impact Engineering, 1984, 2(2): 179–208. doi: 10.1016/0734-743X(84)90005-8 [18] LANGSETH M, HOPPERSTAD O S, HANSSEN A G. Crash behaviour of thin-walled aluminium members [J]. Thin-Walled Structures, 1998, 32(1/2/3): 127–150. [19] ZHANG Y, CHEN T T, XU X, et al. Out-of-plane mechanical behaviors of a side hierarchical honeycomb [J]. Mechanics of Materials, 2020, 140: 103227. doi: 10.1016/j.mechmat.2019.103227 [20] TRAN T, HOU S J, HAN X, et al. Crushing analysis and numerical optimization of angle element structures under axial impact loading [J]. Composite Structures, 2015, 119: 422–435. doi: 10.1016/j.compstruct.2014.09.019 [21] LI Z C, RAKHEJA S, SHANGGUAN W B. Study on crushing behaviors of foam-filled thin-walled square tubes with different types and number of initiators under multiple angle loads [J]. Thin-Walled Structures, 2019, 145: 106376. doi: 10.1016/j.tws.2019.106376 -








 下载:
下载:









































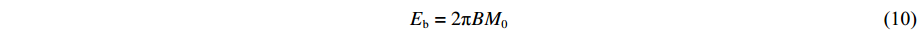
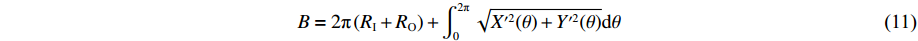
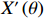

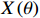
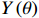
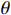
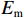


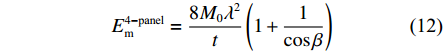

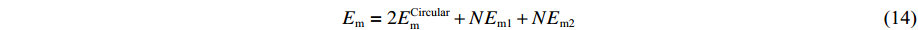
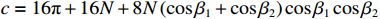
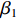
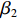
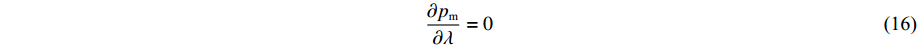
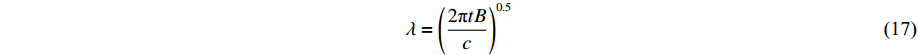
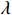
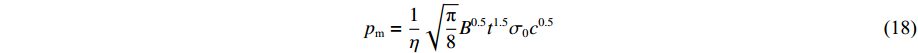
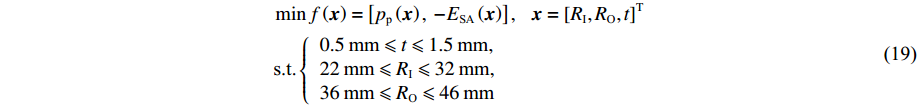
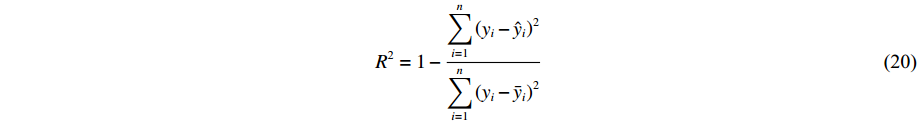
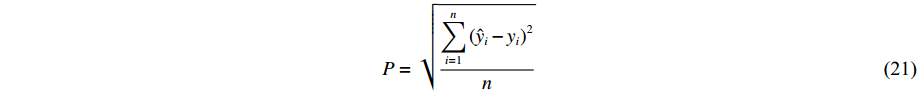
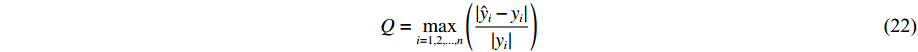
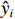
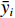
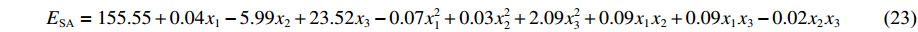
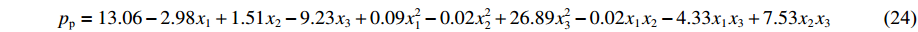



 下载:
下载: