Dynamic Response and Multi-Objective Optimization of Aluminum Foam-Filled Sandwich Tube under Lateral Blast Loading
-
摘要: 基于动力显式有限元法,研究了泡沫铝夹芯管在横向爆炸载荷下的动态响应,以芯层能量吸收及外管刚度为目标,对结构的抗爆性能进行了多目标优化设计。系统研究了泡沫铝夹芯管结构的几何参数、泡沫铝芯层相对密度和爆炸加载条件等对其变形规律和能量吸收性能的影响。结果表明:横向爆炸载荷下,泡沫铝夹芯管的变形区域集中在跨中位置,内外管通过跨中位置塑性变形和变形区域左右两端的弯曲变形吸收能量,泡沫铝芯层主要依靠芯层压缩吸收能量;减小外管壁厚或泡沫铝芯层相对密度能有效提高结构的比吸能,但会影响泡沫铝夹芯管内外管的变形程度;外管几何参数对结构吸能性能和内外管变形的影响程度远大于内管;基于泡沫铝夹芯管的数值模拟结果,构造了响应面模型并对其进行了多目标优化,给出了Pareto前沿图,可根据实际工程应用来确定泡沫铝夹芯管结构中内外管的壁厚与芯层的相对密度。Abstract: The dynamic response of aluminum foam-filled sandwich tubes subjected to lateral blast loading was investigated numerically using the dynamic explicit finite element method. Based on numerical simulation, the structural blast resistance was optimized with the core energy absorption and outer tube stiffness as the optimization objectives. The effects of structural geometric parameters, the relative density of the aluminum foam core layer, and blast loading conditions on the deformation patterns and energy absorption properties of aluminum foam-filled sandwich tube have been systematically investigated. The study results indicate that the deformation region of the aluminum foam-filled sandwich tube under lateral blast loading is mainly concentrated in the middle span. Energy absorption occurs through plastic deformation in the middle of the span and bending deformation at the left and right ends of the deformed region for both the inner and outer tubes. In contrast, the energy absorption of the aluminum foam core layer relies primarily on core compression. Reducing of the thickness of the outer tube or the relative density of the aluminum foam core layer can effectively improve the specific energy absorption of the structure and increase the deformation of the inner and outer tubes. The effect of the geometry parameters of the outer tube on the energy absorption properties of the structure and the deformation of the inner and outer tubes is much larger than that of the inner tube. A response surface model is constructed based on the numerical simulation results of aluminum foam-filled sandwich tube. Subsequently, multi-objective optimization is performed and the resulting Pareto front graph is provided. The determination of the wall thickness of the inner and outer tubes, together with the relative density of the aluminum foam core layers in the aluminum foam-filled sandwich tube, can be based on the specific engineering application requirements.
-
随着人们对安全要求的不断提高和人为爆炸事故的频繁发生,发生爆炸时工程的安全可靠性已逐渐成为人们关注的重要问题,深入了解爆炸载荷下结构的动态响应具有重要意义。与圆管相比,填充可压缩泡沫芯层的夹芯管能够通过冲击衰减和塑性功耗散来吸收大量爆炸能量,具有更好的抗爆性能。因此,泡沫夹芯结构作为抗爆吸能装置,引起了研究者们的广泛关注[1-3]。
Fan等[4]研究了短空心圆管和夹芯管在横向冲击载荷下的动态响应,发现结构的能量耗散主要通过塑性铰的形成来实现。Hall等[5]研究了横向冲击载荷下泡沫铝、泡沫黄铜和泡沫钛填充管的能量吸收性能,结果表明,泡沫铝填充管的能量吸收特性最佳。Song等[6]提出了横向爆炸载荷作用下钢圆管塑性变形的解析模型,预测了钢管的挠度和变形角,发现理论预测结果与实验结果吻合较好。Yuen等[7]对横向爆炸载荷作用下的圆管进行了实验和数值模拟分析,并与Wierzbicki等[8]提出的理论模型进行了对比,发现实验结果与数值模拟结果吻合良好,但理论模型预测的最终挠度偏低。Jing等[9-11]研究了爆炸载荷下泡沫铝夹芯壳结构的动态响应和吸能特性,结果表明,载荷强度和几何构型影响结构的变形/破坏模式和吸能特性,增加面板厚度或泡沫铝芯层的密度可提高其抗爆性能,并建立了流固耦合、芯层压缩和整体弯曲拉伸的三阶段框架理论模型预测结构的动态响应。Hoo Fatt等[12]考虑了泡沫芯层的弹塑性行为,对外爆炸载荷作用下的复合材料夹芯壳进行了应力波分析,结果表明,高峰值、低持续时间的冲击波作用下结构能量吸收最高,低峰值、长持续时间的冲击波反之。Liu等[13]通过实验和数值模拟研究了两端固支的泡沫铝填充管在横向外爆炸载荷作用下的动力响应和能量吸收性能,结果表明,泡沫铝芯层的引入减小了结构跨中挠度,提高了其能量吸收能力。上述研究表明,填充泡沫的管状结构具有良好的抗冲击性能,是一种理想的吸能装置。
多目标优化可以平衡各个目标,使目标之间作出协调和折中处理,最终总体的目标尽可能达到最优。Baroutaji等[14-15]通过多目标优化设计方法对准静态横向压缩下的圆管和泡沫铝夹芯管进行了优化,发现圆管在最大壁厚和最小直径下耐撞性最好,而夹芯管在最大芯层厚度和最小内管直径下耐撞性最好。Qi等[16]对爆炸载荷下的泡沫铝夹芯板进行了多目标优化,通过非支配排序遗传算法(non-dominated sorting genetic algorithm, NSGA-Ⅱ)对结构的几何尺寸和芯层的相对密度进行优化,得到了最佳参数,平衡了结构的吸能能力和结构变形量。
综上所述,已有的研究主要集中于横向冲击载荷下薄壁圆管、填充管和夹芯壳结构的动态响应和能量吸收性能的研究。但是,关于泡沫金属夹芯管结构在横向爆炸载荷作用下的变形失效模式、吸能机制及抗冲击性能等方面的研究比较有限,已有的研究也局限于带曲率的夹芯壳结构,针对爆炸载荷下夹芯管结构的多目标优化的研究尚不多见。本研究基于动力显式有限元法,探究泡沫铝夹芯管在横向爆炸载荷下的动态响应,并对其抗爆性能进行多目标优化。建立横向爆炸作用下泡沫铝夹芯管的有限元模型,研究泡沫铝夹芯管的几何参数、芯层的相对密度和爆炸加载条件对内外管跨中挠度和吸能性能的影响;以内管、外管壁厚和芯层相对密度为设计变量,以芯层比吸能和外管跨中挠度为优化指标,采用响应面法(response surface method, RSM)和NSGA-Ⅱ算法实现泡沫铝夹芯管抗爆性能多目标优化设计,为抗爆结构设计提供技术指导。
1. 有限元模拟
1.1 有限元模型
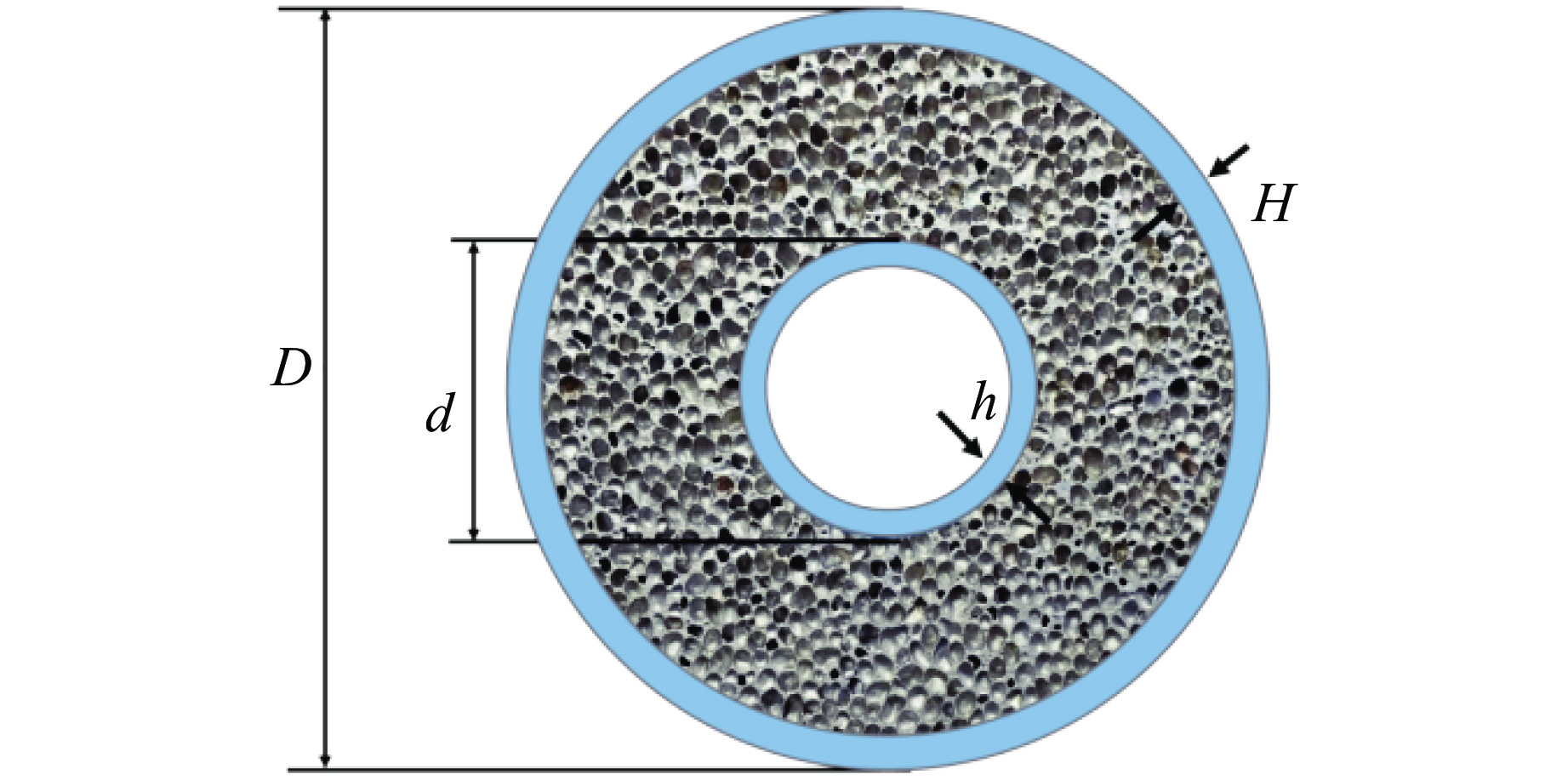
泡沫铝夹芯管由内、外两个同心的薄壁圆管和泡沫铝芯层3部分组成,内、外薄壁圆管采用钢材,泡沫铝芯层采用相对密度分别为10%、15%和20%的泡沫铝材料。图1为泡沫铝夹芯管横截面的示意图,其中:D为外管外径,H为外管壁厚,d为内管外径,h为内管壁厚。试件长度为L。
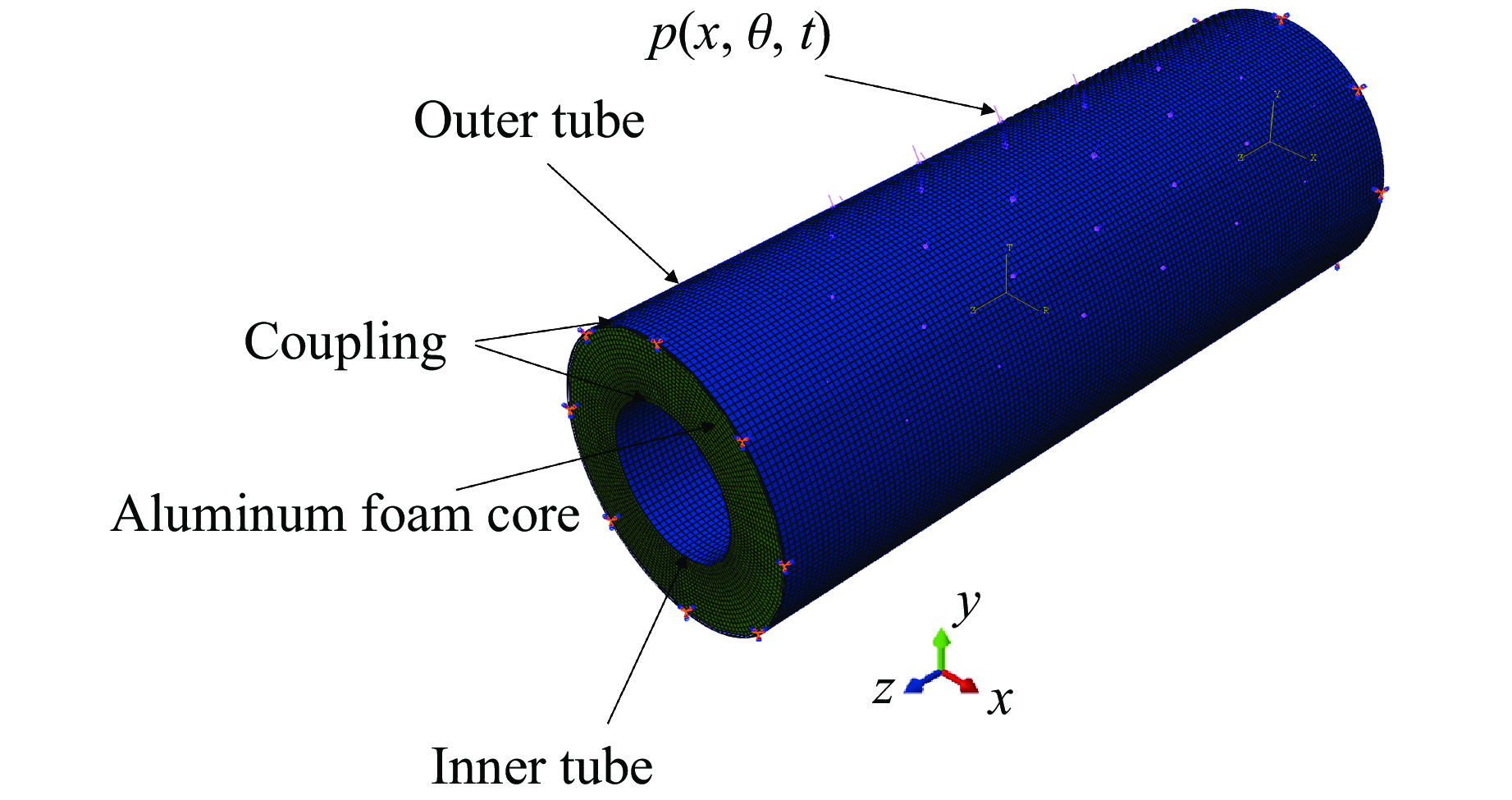
采用有限元软件ABAQUS/EXPLICIT建立泡沫铝夹芯管的有限元模型,如图2所示。泡沫铝夹芯管的中线与z轴重合,内管和外管采用常规的四节点壳单元(S4R),泡沫铝芯层采用八节点线性实体单元(C3D8R),采用中性轴算法划分网格。内、外管与泡沫芯层之间定义为绑定约束,试件两端约束全部自由度来模拟两端固支的边界条件,分析过程采用动态显式算法。
内外管采用各向同性硬化双线性本构模型,密度ρ=7 830 kg/m3,杨氏模量E=193 GPa,泊松比μ=0.25,屈服应力σ0=205 MPa,在最大应变εUTS=0.4时,最大拉伸强度σUTS =520 MPa,应变率敏感性参数C和P分别取100 s−1和10[17]。泡沫铝芯层采用各向同性硬化塑性模型定义,用可压缩泡沫材料模拟泡沫铝芯层。不同相对密度泡沫铝的材料参数如表1[18]所示,其中:ρ*为相对密度,μc、μp分别为压缩屈服应力比和塑性泊松比。
1.2 爆炸载荷的假设
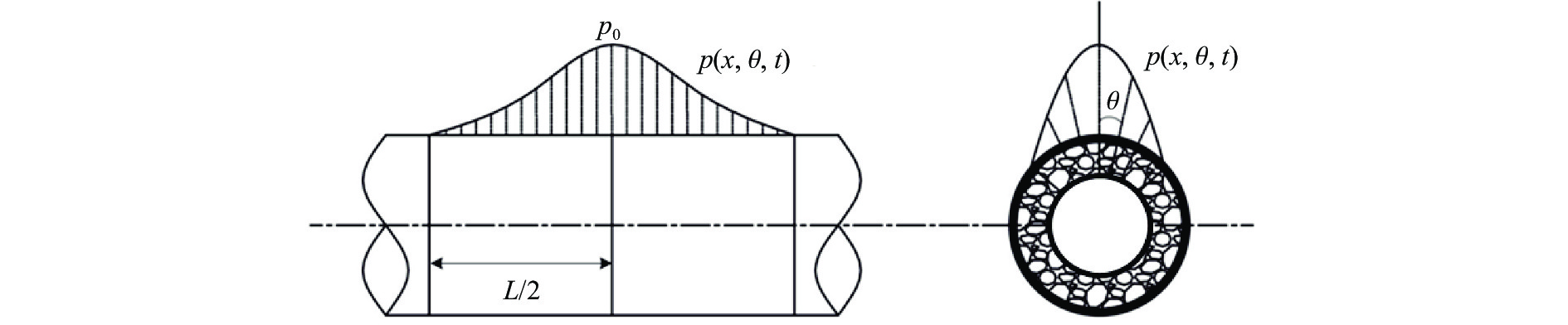
球形炸药作用在试件上形成了脉冲载荷,假定作用在泡沫铝夹芯管上的脉冲载荷分布如图3所示,其脉冲近似方程可写为
p(x,θ,t)={p0(1−t/τ)cos2(πx/L)sinθt⩽τ0t>τ (1) 式中:τ为爆炸载荷响应时间,取τ=50 μs[17];L为试件总长,L=280 mm;p0为作用在泡沫铝夹芯管迎爆面处的超压峰值。
根据TNT球形炸药在无限大气中的超压峰值计算公式,结合实验中的实际炸药质量和起爆距离,超压峰值[19](单位:105 Pa)可以表示为
p0=0.085Z+0.3Z2+0.8Z3 (2) Z=R/3√WTNT (3) 式中:WTNT为TNT炸药质量,kg;R为起爆距离,m。实验中所采用的乳化炸药与TNT炸药的换算关系为
WTNT=0.7Ws (4) 式中:Ws为乳化炸药质量。结合式(1)~式(4),可得出作用在试件上的总冲量IT为
IT=4∫L/20∫π/20∫τ0p(x,θ,t)D2dxdθdt=14p0DLτ (5) 表2列出了横向爆炸载荷下两端固支的两组泡沫铝填充管试件的理论冲量与文献[13]中实验冲量的对比,其中m为泡沫铝填充管的质量,冲量IE为实验结果,超压峰值p0由式(2)计算得到,总冲量IT由式(5)给出。由表2可知,理论计算冲量与实验冲量吻合较好,验证了爆炸载荷假设的合理性。
表 2 理论冲量与实验冲量的对比Table 2. Comparison of impulse between theoretical solution and experimentTest piece Ws/g R/mm D/mm H/mm m/kg p0/MPa IE/(N·s) IT/(N·s) IT−IEIE/% No.1 30 40 76 0.7 96.8 27.7 7.1 7.4 4.2 No.2 50 40 76 0.7 96.7 45.8 12.9 12.2 −5.4 1.3 数值模拟验证及泡沫铝夹芯管网格敏感性验证
为了验证本研究中有限元模型的有效性,根据文献[13]中的实验模型,利用ABAQUS建立有限元模型,爆炸载荷以式(1)的形式施加在泡沫铝填充管上。图4给出了试件1的数值模拟和实验的最终变形模式,其中泡沫铝夹芯管截面中的4.1 mm为最终变形区域的径向厚度尺寸。由图4可知,结构的整体变形模式与有限元结果吻合较好。表2中试件1的外管跨中挠度的实验值与模拟值分别为14.0和12.6 mm,试件2的外管跨中挠度的实验值和模拟值分别为22.1和29.0 mm,数值模拟结果与实验测试结果吻合较好,表明所建立的有限元模型是合理的。
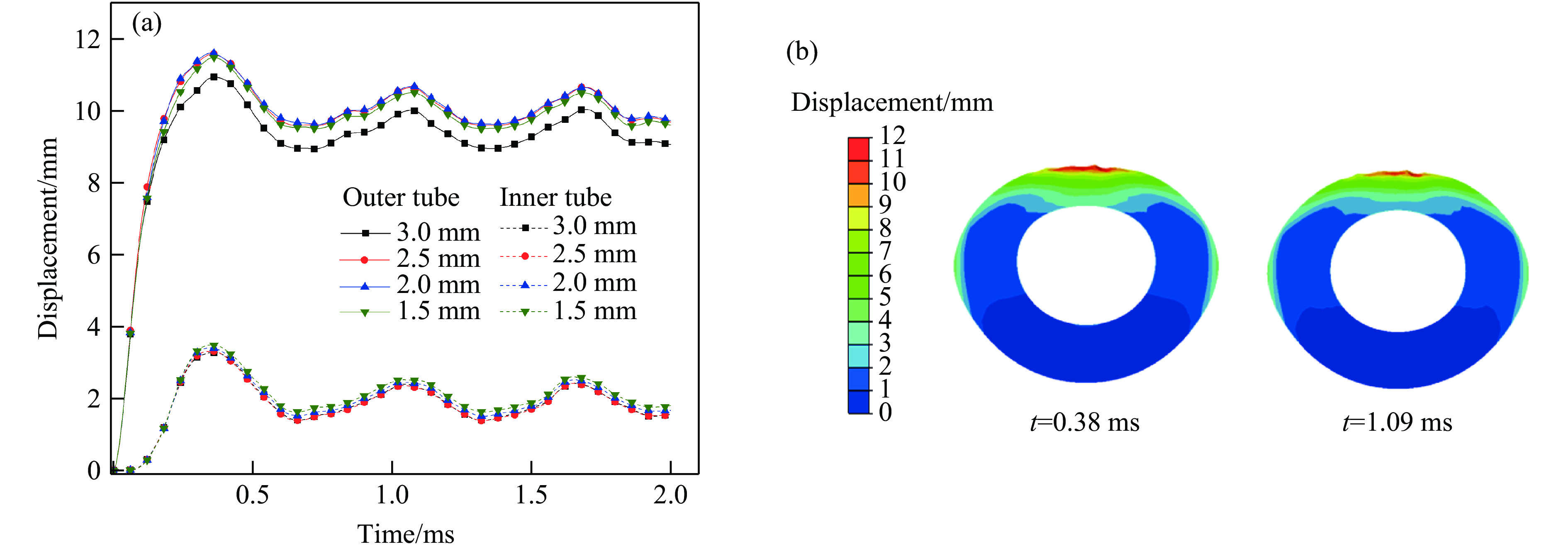
为研究横向爆炸载荷下泡沫铝夹芯管的动态响应,对其有限元模型的网格敏感性进行了验证。采用乳化炸药的质量为30 g,起爆位置距离试件跨中40 mm,载荷以式(1)给出的脉冲载荷函数形式施加在泡沫铝夹芯管上。试件的几何参数:外管外径为101 mm,壁厚为0.7 mm;内管外径为54 mm,壁厚为0.7 mm,泡沫铝芯层的相对密度为15%。泡沫铝芯层和内、外管分别采用1.5、2.0、2.5和3.0 mm共4种不同的单元网格尺寸。图5给出了在横向爆炸载荷下4种不同单元网格尺寸泡沫铝夹芯管试件内外管跨中挠度时程曲线,其中虚线和实线分别代表内、外管挠度曲线,外管的挠度大于内管挠度,内外管跨中挠度随时间上下波动,取第一个波谷点后的挠度曲线的平均值作为泡沫铝夹芯管的最终挠度。当网格尺寸为1.5、2.0和2.5 mm时,三者显示了较好的一致性,而网格尺寸为3.0 mm时,数值模拟与其他网格尺寸结果相差较大。综合考虑计算精度和效率,数值模拟中采用2.0 mm的网格尺寸。
2. 模拟结果与分析
为研究爆炸载荷下泡沫铝夹芯管结构的动态响应和能量吸收特性,对结构的几何参数、爆炸加载条件进行分析,研究结构的内外管直径与壁厚、泡沫铝芯层的相对密度、炸药起爆距离及炸药质量对泡沫铝夹芯管动态响应的影响。考虑9组不同几何参数的泡沫铝夹芯管试件在横向爆炸载荷下的抗爆性能,其中泡沫芯层的相对密度为15%,试件详细的几何参数如表3所示。
表 3 试件几何参数Table 3. Geometric parameter of specimensTest piece D/mm d/mm H/mm h/mm L/mm m/g NS01 101 76 0.7 0.7 280 1 242.62 NS02 101 65 0.7 0.7 280 1 326.02 NS03 101 54 0.7 0.7 280 1 388.13 NS04 90 54 0.7 0.7 280 1 150.28 NS05 79 54 0.7 0.7 280 933.72 NS06 101 76 0.8 0.7 280 1 312.19 NS07 101 76 0.9 0.7 280 1 381.75 NS08 101 76 0.7 0.8 280 1 294.97 NS09 101 76 0.7 0.9 280 1 347.31 泡沫铝夹芯管对于爆炸冲击波能量的吸收主要靠内外管的变形与泡沫铝芯层的坍塌塑性变形来实现,定义比吸能Ea为单位质量泡沫铝夹芯管所吸收的能量
Ea=Etm (6) 式中:Et为泡沫铝夹芯管吸收的总能量,在数值模拟中取2 ms时的塑性能量耗散值为结构整体的吸能,m为泡沫铝夹芯管的质量。
2.1 外管直径和壁厚的影响
为研究泡沫铝夹芯管结构中外管直径和壁厚对泡沫铝夹芯管抗爆性能的影响,对2组试样共计6个试件进行了横向爆炸载荷下的动态响应和能量吸收性能研究,其中:第一组试样NS03、NS04和NS05的内外管壁厚和内管直径保持不变,仅改变外管的直径;另一组试样NS01、NS06和NS07的内外管直径和内管壁厚保持不变,仅改变外管的壁厚。加载条件为:乳化炸药质量30 g,起爆位置距离试件跨中40 mm。表4给出了6个不同几何参数试件吸收的总能量、比吸能和内、外管跨中挠度(wi和wo)。由表4可知,随着外管直径的减小,试件的总吸能、比吸能和外管跨中挠度减小,内管跨中挠度增大。原因是随着外管直径的减小,泡沫铝夹芯管迎爆面积减小,结构承受的爆炸载荷降低,外管塑性变形减小,因而结构的总吸能减小;而内管跨中挠度增大是由于泡沫铝芯层厚度减小导致芯层密实化使内管吸收了较多的能量。随着外管壁厚的增大,试件整体结构刚度增加,内外管的塑性变形和芯层的压缩量减小,因此试件的总吸能、比吸能和内、外管跨中挠度均减小。
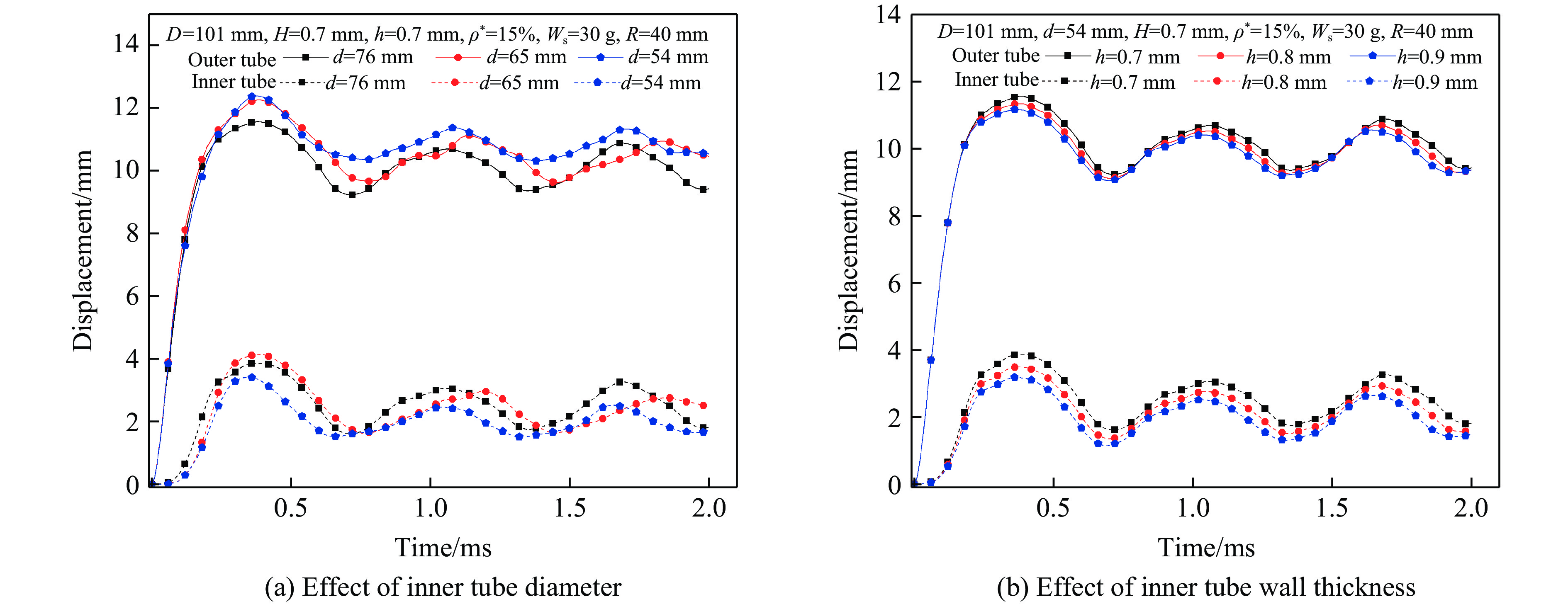
表 4 外管直径和壁厚的影响Table 4. Effect of outer tube diameter and wall thicknessTest piece Et/J m/g Ea/(J·g−1) wo/mm wi/mm NS03 539.878 1 388.13 0.389 10.78 1.95 NS04 442.605 1 150.28 0.385 9.06 2.21 NS05 354.622 933.72 0.380 8.88 2.87 NS01 542.923 1 242.62 0.437 10.06 2.45 NS06 456.645 1 312.19 0.348 7.61 2.16 NS07 388.472 1 381.75 0.281 5.81 1.76 图6给出了不同外管直径和壁厚的泡沫铝夹芯管试件内外管的跨中挠度时程曲线,其中虚线和实线分别代表内、外管跨中挠度。显然,外管跨中挠度大于内管跨中挠度。外管壁厚对外管跨中挠度的影响程度大于外管直径的影响;随着外管直径的增大及外管壁厚的减小,泡沫铝夹芯管芯层压缩量增大,结构吸收的总能量增加。
2.2 内管直径和壁厚的影响
为研究泡沫铝夹芯管结构中内管直径和壁厚对泡沫铝夹芯管动态响应的影响,对2组试样共计6个试件进行了横向爆炸载荷下的力学性能研究,其中:第一组试样NS01、NS02和NS03的外管直径和内、外管壁厚保持不变,仅改变内管的直径;另一组试样NS01、NS08和NS09的内外管直径和外管壁厚保持不变,仅改变内管的壁厚。试件的加载条件为:乳化炸药质量30 g,起爆位置距离试件跨中40 mm。表5给出了试件的总吸能、比吸能及内、外管跨中挠度。由表5可知,随着内管直径的减小,试件的比吸能及内管跨中挠度减小,外管跨中挠度增大。内管直径的减小对泡沫铝夹芯管迎爆面积无影响,因而对结构吸收总能量的影响很小,芯层厚度的增大使内管吸能减少,内管跨中挠度减小。随着内管壁厚的增大,试件整体结构刚度增加,内外管的塑性变形和芯层的压缩量减小,因此试件的总吸能、比吸能和内、外管跨中挠度均减小。
表 5 内管直径和壁厚的影响Table 5. Effect of inner tube diameter and wall thicknessTest piece Et/J m/g Ea/(J·g−1) wo/mm wi/mm NS01 542.923 1 242.62 0.437 10.06 2.45 NS02 546.692 1 326.02 0.412 10.39 2.32 NS03 539.878 1 388.13 0.389 10.78 1.95 NS08 542.470 1 294.97 0.419 9.92 2.16 NS09 542.161 1 347.31 0.402 9.81 1.92 图7给出了不同内管直径和不同内管壁厚的泡沫铝夹芯管试件内外管跨中挠度时程曲线,其中虚线和实线分别代表内管和外管跨中挠度时程曲线,外管挠度大于内管挠度。由图7(b)可知,内管壁厚对外管跨中挠度的影响程度小于对内管跨中挠度的影响;对比图6,外管的直径和壁厚对内、外管跨中挠度的影响程度大于内管直径和壁厚的影响。
2.3 芯层相对密度的影响
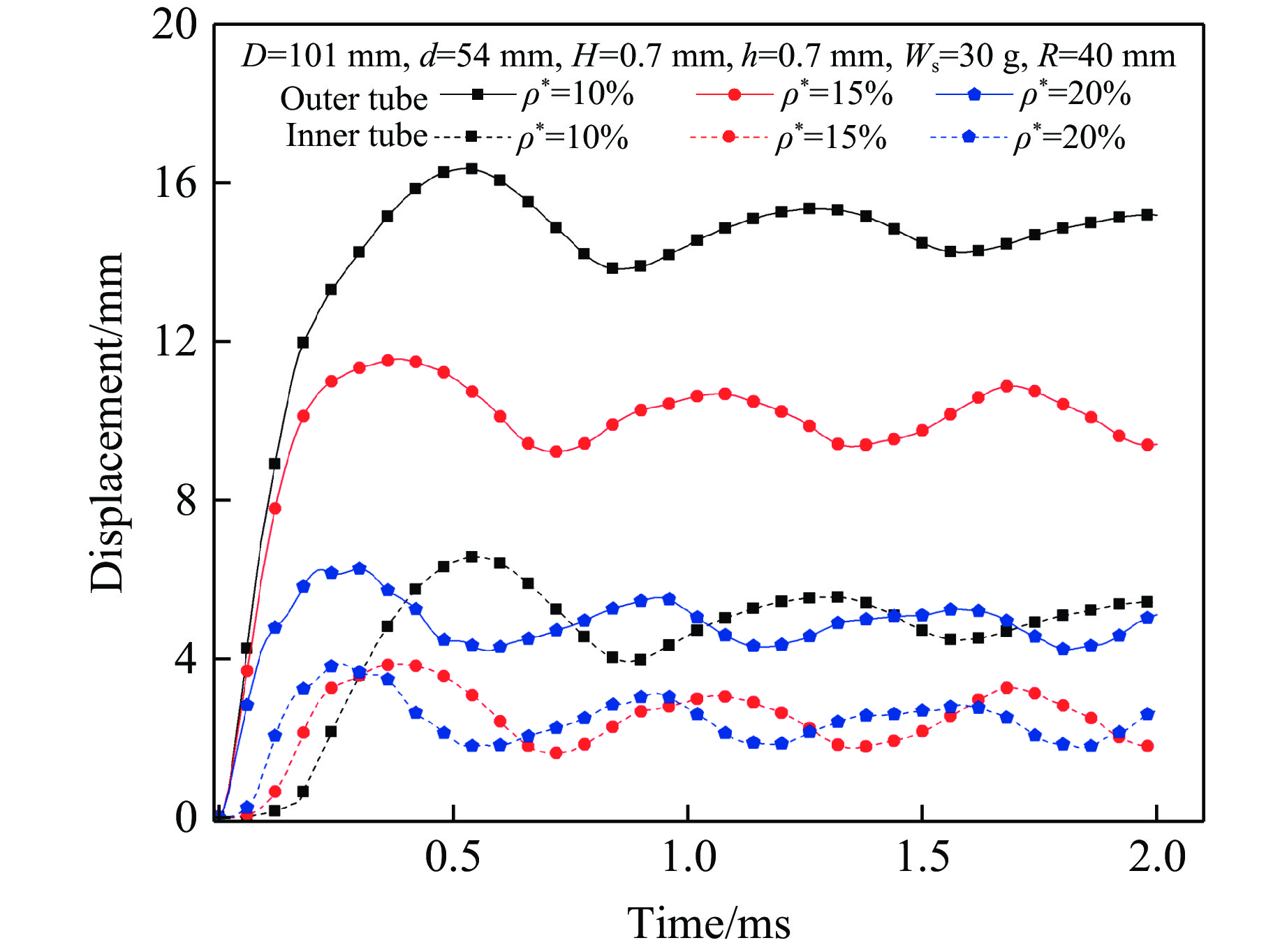
为研究泡沫铝夹芯管结构中泡沫铝夹芯层的相对密度对泡沫铝夹芯管动态性能的影响,对以NS01试件为基础分别填充相对密度为10%、15%和20%的泡沫铝芯层的3个试件进行横向爆炸载荷下的力学性能研究,试件的加载条件为:乳化炸药质量30 g,起爆位置距离试件跨中40 mm。表6给出了不同相对密度芯层的泡沫铝夹芯管结构的总能量、比吸能和内外管跨中挠度。由表6可知,随着泡沫铝芯层相对密度的增大,结构的整体刚度增大,内、外管的塑性变形和芯层压缩量减小。因此,结构的总吸能、比吸能和内、外管跨中挠度均减小。图8给出了不同相对密度芯层的泡沫铝夹芯管试件的内外管跨中挠度时程曲线,其中虚线和实线分别代表内、外管跨中挠度随时间的变化规律,外管挠度大于内管挠度。由图8可知,相对密度越小,泡沫铝芯层的压缩量越大,结构的总吸能和比吸能越大。
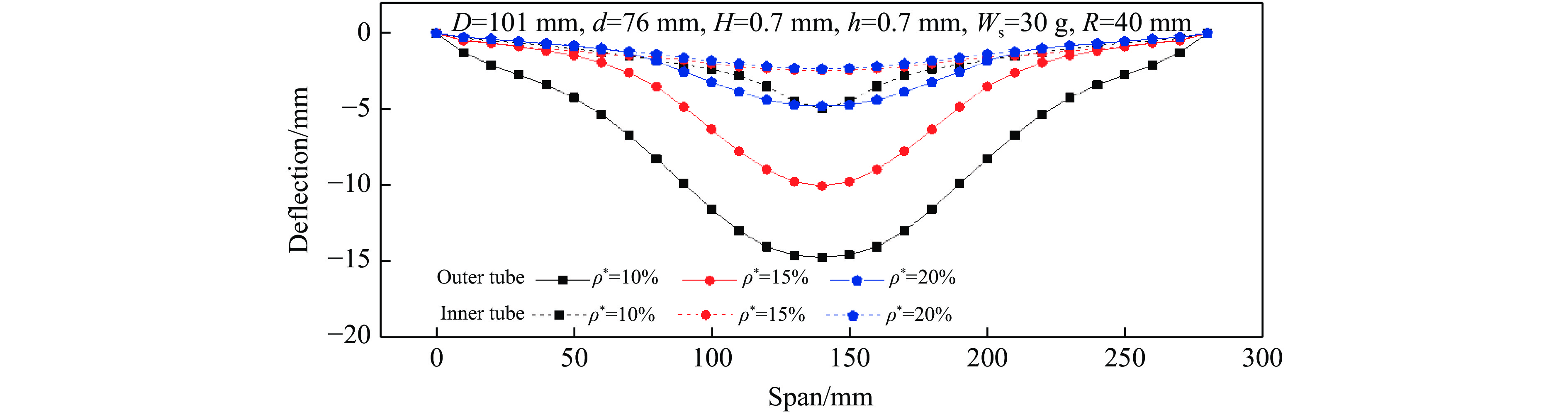
表 6 泡沫铝相对密度的影响Table 6. Effect of relative density of aluminum foamρ*/% p0/MPa Et/J m/g Ea/(J·g−1) wo/mm wi/mm 10 36.8 614.301 1 116.12 0.550 14.77 4.99 15 36.8 542.923 1 242.62 0.437 10.06 2.45 20 36.8 379.921 1 378.86 0.276 4.82 2.35 图9给出了填充不同相对密度泡沫铝夹芯管内、外管的轴向变形轮廓,其中虚线和实线分别代表内、外管变形轮廓。由图9可知,内、外管的变形可分为3个区域,包括中心部位的塑性大变形区域、围绕塑性铰移动的刚性截面和未变形边界区域[17]。
2.4 爆炸加载条件的影响
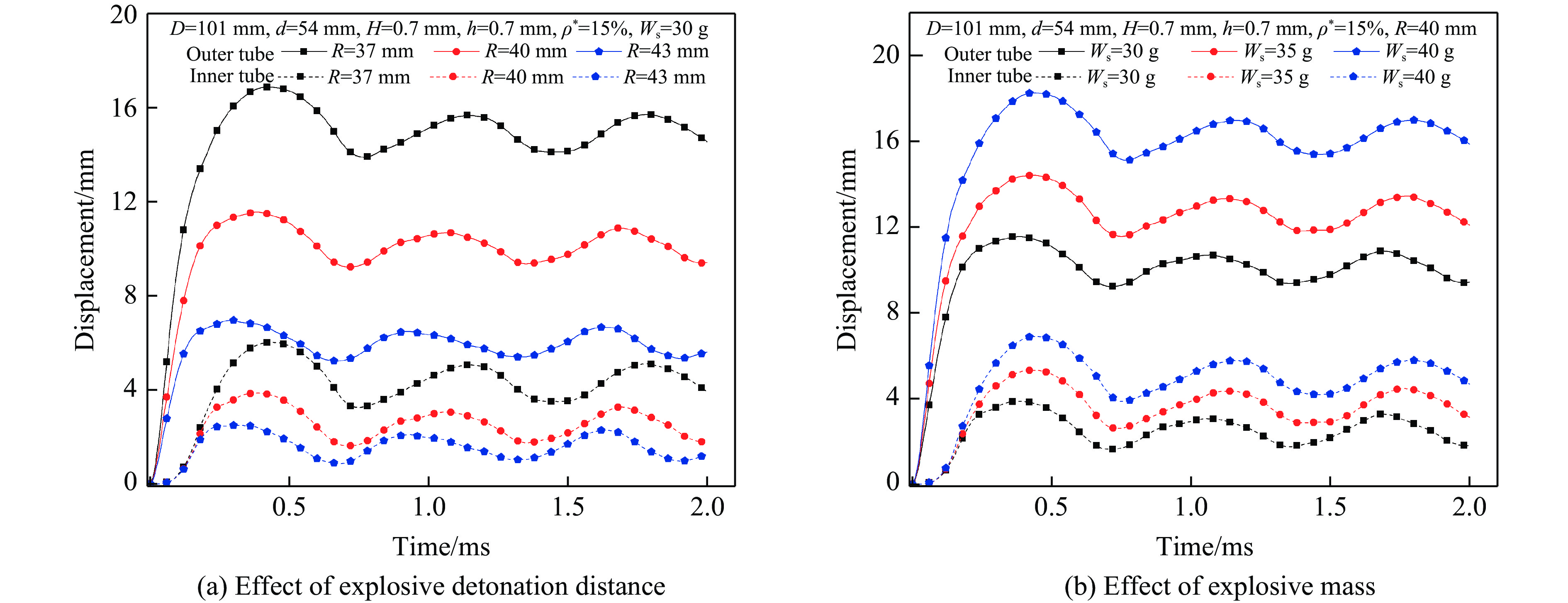
由2.3节可知,横向爆炸载荷下泡沫铝夹芯管NS01试件在所研究的试件中抗爆性能最佳,因此以NS01试件为研究对象,在距离试件跨中37、40和43 mm处施加炸药质量为30 g的爆炸载荷,研究炸药起爆距离对试件动态响应和吸能特性的影响。在距离跨中40 mm处分别施加乳化炸药质量为30、35和40 g的爆炸载荷,研究炸药质量对试件动态响应和吸能特性的影响。表7给出了不同爆炸加载条件下试件NS01的总能量、比吸能和内、外管跨中挠度。由表7可知,随着炸药起爆距离的减小和炸药质量的增加,泡沫铝夹芯管承受的爆炸载荷增大,内外管的塑性变形区域增大。因此,结构的总吸能、比吸能和内、外管跨中挠度均增大。
表 7 爆炸加载条件的影响Table 7. Effect of blast loading conditionsR/mm Ws/g p0/MPa Et/J Ea/(J·g−1) wo/mm wi/mm 37 30 34.9 935.594 0.753 14.96 4.34 40 30 27.7 542.923 0.437 10.06 2.45 40 35 32.3 776.974 0.625 12.60 3.62 40 40 36.8 1058.910 0.852 16.20 4.98 43 30 22.4 312.212 0.251 5.92 1.54 图10给出了炸药起爆距离与炸药质量对泡沫铝夹芯管试件内外管跨中挠度的影响,其中虚线和实线分别代表内、外管跨中挠度时程曲线,外管的挠度大于内管的挠度。由图10可知,炸药起爆距离和炸药药量对泡沫铝夹芯管的内、外管跨中挠度影响显著。
泡沫铝夹芯管各组成部分的吸能占比如表8所示。由表8可知,外管吸收的能量最多,吸能百分比均超过了50%,其次是泡沫铝芯层,吸能最少的是内管。原因是外管作为迎爆面,首先承受爆炸载荷,之后外管压缩芯层,芯层部分密实化后与外管共同压缩内管变形。随着外管壁厚的减小,外管的吸能百分比减小,泡沫铝芯层的吸能百分比增大;内管壁厚对各部分吸能百分比的影响较小;当夹芯管外管直径或内管直径一定时,随着芯层厚度的增加,内管的吸能百分比减小;芯层的相对密度对泡沫铝夹芯管的吸能百分比影响显著,随着芯层相对密度的减小,芯层和内管的吸能百分比减小,外管的吸能百分比增加。
表 8 泡沫铝夹芯管组成部分的吸能百分比Table 8. Energy absorption percentage of aluminum foam-filled sandwich tube componentsTest piece ρ∗/% R/mm Ws/g p0/MPa Energy absorption percentage/% Outer tube Foam core Inner tube NS01 15 40 30 27.7 59.9 32.0 8.1 NS02 15 40 30 27.7 62.6 30.5 6.9 NS03 15 40 30 27.7 61.8 33.7 4.5 NS04 15 40 30 27.7 62.4 30.7 6.9 NS05 15 40 30 27.7 59.7 32.3 8.0 NS06 15 40 30 27.7 64.0 28.1 7.9 NS07 15 40 30 27.7 66.3 25.6 8.2 NS08 15 40 30 27.7 59.5 32.4 8.0 NS09 15 40 30 27.7 59.2 32.9 7.9 NS01 10 40 30 27.7 79.8 17.5 2.7 NS01 20 40 30 27.7 52.0 32.2 15.8 NS01 15 37 30 34.9 61.7 31.0 7.3 NS01 15 43 30 22.4 61.5 29.6 8.9 NS01 15 40 35 32.3 62.0 30.5 7.5 NS01 15 40 40 36.8 61.5 31.2 7.3 3. 抗爆性能的多目标优化
3.1 抗爆性能的定义及优化方法
由参数分析可知,内、外管壁厚和泡沫铝芯层的相对密度对泡沫铝夹芯管的抗爆性能具有一定影响,期望芯层比吸能最大化的同时,尽可能将外管跨中挠度控制在合理的范围内。因此,将最大化芯层比吸能ECSA和最小化外管跨中挠度wo作为优化目标,通过多目标优化方法研究泡沫铝夹芯管的抗爆性能。以泡沫铝夹芯管NS01内、外管壁厚以及芯层的相对密度作为设计参数(壁厚单位:mm),则多目标优化问题表示为
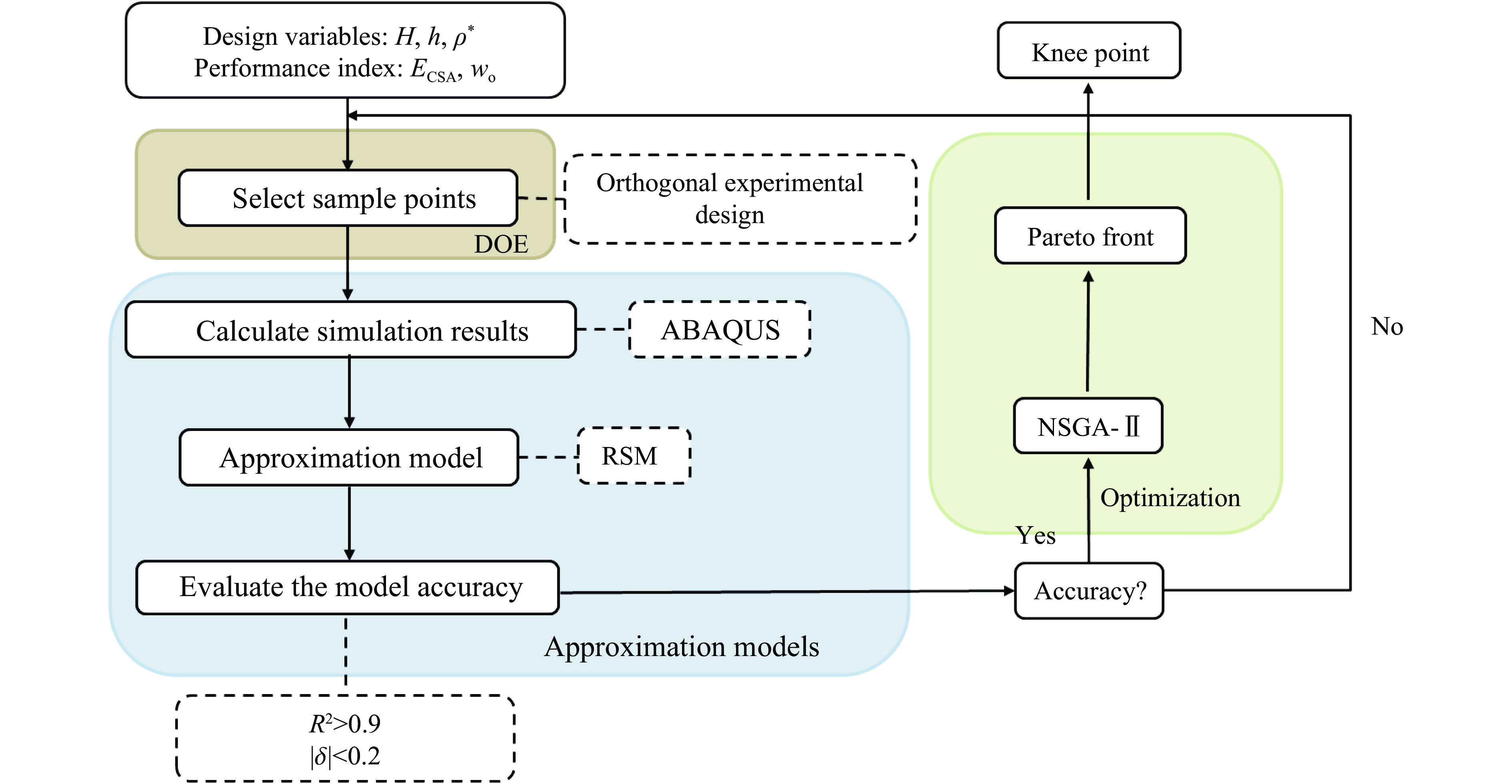
{f(x)=min(1/1ECSA(H,h,ρ∗)ECSA(H,h,ρ∗),wo(H,h,ρ∗))s.t. 10%⩽ρ∗⩽20% 0.7⩽H⩽1.780.7⩽h⩽1.78 (7) 多目标优化方案如图11所示。首先,采用正交试验设计方法,在设计变量的范围内选取样本点;然后,采用响应面法(RSM),根据有限元模拟得到样本点数据建立ECSA和wo的代理模型,并对代理模型进行评估;最后,基于非支配排序遗传算法(NSGA-Ⅱ)对参数进行优化,得到多目标优化问题的Pareto前沿解集。
采用正交试验设计的方法选取试验点[20],将外管与内管壁厚、泡沫铝芯层的相对密度作为3个影响因子,内、外管壁厚采用4个水平,内、外管壁厚水平分别采用0.70、1.06、1.42、1.78 mm,相对密度采用3个水平,相对密度水平采用10%、15%和20%,按照正交试验设计方法选取48个样本点进行数值计算,乳化炸药的质量为30 g,起爆位置距离试件跨中40 mm,分别得到不同样本点的外管跨中挠度和泡沫铝芯层的比吸能,并以此建立响应面模型。
响应面法具有简单易行、效果良好的特点,在建立高度非线性的代理模型中得到了广泛应用,使用三次多项式构建代理模型,得到48个样本点对应的ECSA和wo,通过数据拟合和最小二乘法确定响应面代理模型的系数。由设计变量H、h、
ρ∗ 构造的ECSA和wo三次多项式响应面函数为1/ECSA=−6.4773+49.5197H−23.3641h+122.0080ρ∗−8.8641H2−4.1417h2−481.0666ρ∗2+18.8084Hh−739.6430Hρ∗+301.3099hρ∗+0.3891H3−0.2289h3−224.4299ρ∗3−4.4783H2h+116.4278H2ρ∗+2.4124Hh2+21.4824h2ρ∗+2533.4736Hρ∗2−847.8994hρ∗2−127.9660Hhρ∗ (8) wo=74.4479−95.2639H−19.7206h−162.4696ρ∗+44.2081H2+3.0028h2−324.6523ρ∗2+11.9992Hh+151.6219Hρ∗+97.5556hρ∗−6.0282H3−0.0447h3−135.3097ρ∗3−2.0022H2h−75.7620H2ρ∗−1.3057Hh2−4.6779h2ρ∗+430.9722Hρ∗2−201.8056hρ∗2−18.2485Hhρ∗ (9) 为了评估代理模型的准确性,采用拟合优度R2和相对误差的绝对值
|δ| 两种评价指标对多项式响应面进行误差分析,这两种指标的计算方法为R2=1−n∑i=1(yi−ˆyi)2n∑i=1(yi−ˉy)2 (10) |δ|=n∑i=1|yi−ˆyi|n∑i=1|yi−ˉy| (11) 式中:
yi 和ˆyi 分别为第i个样本点的有限元模拟和多项式响应面的响应值;ˉy 为yi 的平均值;n为样本点的个数;R2为评价响应面函数与数值计算数据拟合准确度的指标,|δ| 用于评估模型的整体精度,R2>0.9 、|δ| <0.2表示代理模型有足够高的精确度。结果显示:ECSA和wo的R2分别为0.992 6和0.993 8,均大于0.99,接近1,ECSA和wo的|δ| 分别为0.096 9和0.084 7,均小于0.1,表明建立的多项式代理模型精度满足要求。3.2 优化结果分析
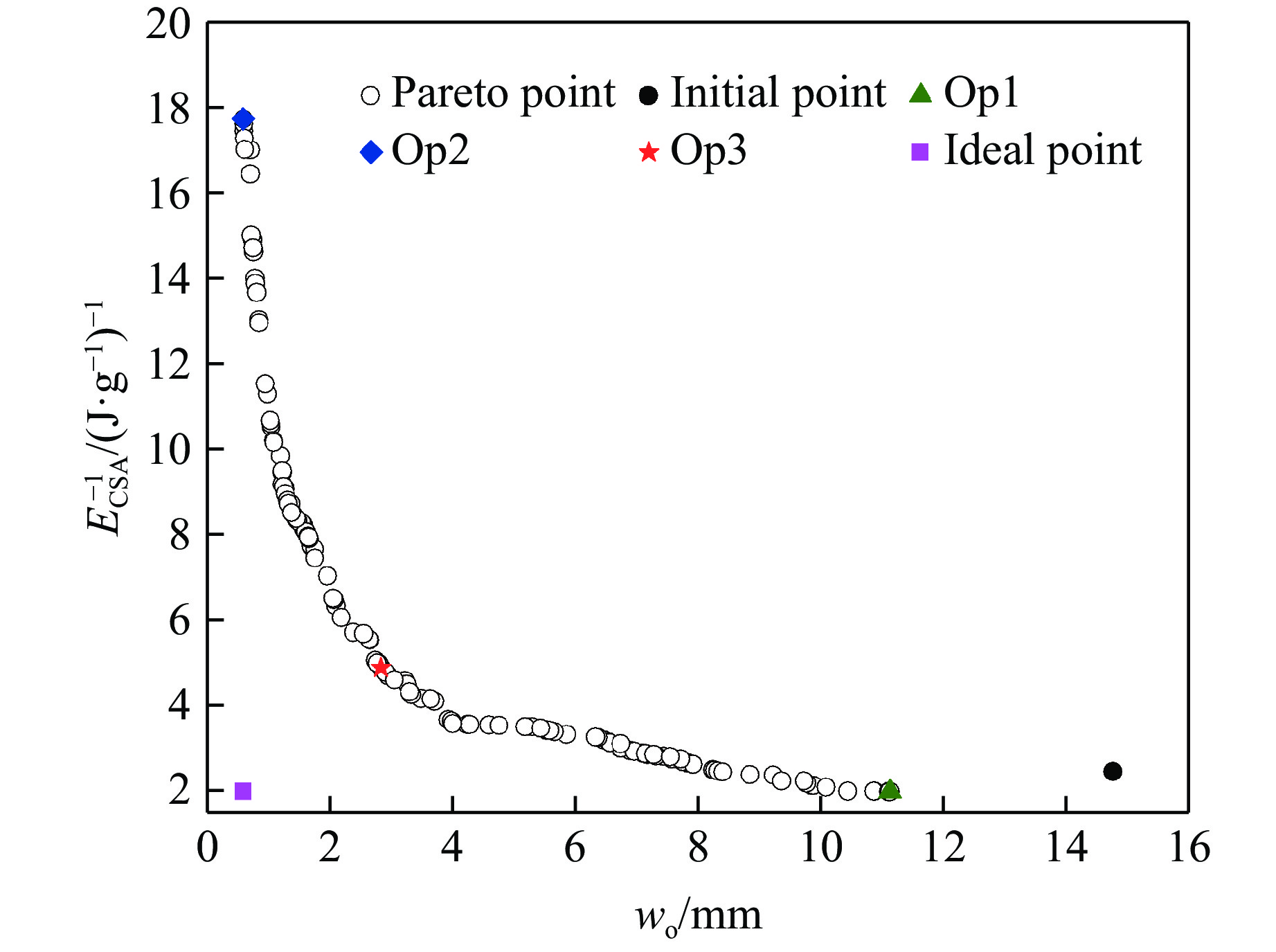
最终得到的Pareto前沿如图12所示,空心点为NSGA-Ⅱ得到的数据。当ECSA增大时,wo也增大,两个优化目标相互冲突。由图12可知,所有的解集中wo都小于初始设计点。表9给出了设计点的结构参数,并将有限元模拟结果与响应面函数得到的结果进行比较,误差均小于9.5%,验证了代理模型的准确性。当仅要求芯层比吸能最大化时,最佳参数设计点为Op1,与初始设计点相比,外管跨中挠度减小了28.9%,芯层比吸能提高了11.7%。当仅要求外管跨中挠度最小化时,最佳参数设计点为Op2,与初始设计点相比,外管跨中挠度减小了95.7%,芯层比吸能降低了87.5%。为使ECSA和wo达到平衡,采用最小距离选择法设计Op3,最小距离选择法的公式为
表 9 最佳设计点Table 9. Optimum design pointDesign point H/mm h/mm ρ∗/% wo ECSA FEM/mm RSM/mm Error/% FEM/(J·g−1) RSM/(J·g−1) Error/% Op1 0.70 0.76 13.7 0.457 0.505 −9.5 10.50 11.14 −5.7 Op2 1.78 1.75 18.8 0.051 0.056 −8.9 0.64 0.59 8.5 Op3 0.84 1.76 20.0 0.194 0.205 −5.4 2.85 2.83 0.7 minS=√[(1/ECSA(H,h,ρ∗))−(1/ECSA(H,h,ρ∗))min(1/ECSA(H,h,ρ∗))max−(1/ECSA(H,h,ρ∗))min]2+[wo(H,h,ρ∗)−wo(H,h,ρ∗)minwo(H,h,ρ∗)max−wo(H,h,ρ∗)min]2 (12) 式中:S为Pareto解集中每个非劣解到理想最优解(ideal point)的距离,
(1/1ECSA(H,h,ρ∗)ECSA(H,h,ρ∗)) 和wo(H,h,ρ∗) 为Pareto解集中每个非劣解对应芯层比吸能的倒数和外管跨中挠度的值,(1/1ECSA(H,h,ρ∗)ECSA(H,h,ρ∗))max 和(1/1ECSA(H,h,ρ∗)ECSA(H,h,ρ∗))min 分别为所有Pareto解集中芯层比吸能的倒数的最大值和最小值,wo(H,h,ρ∗)max 和wo(H,h,ρ∗)min 分别为所有Pareto解集中外管跨中挠度的最大值和最小值。最佳参数设计点为Op3,与初始设计点相比,外管跨中挠度减小了80.7%,芯层比吸能降低了52.6%。4. 结 论
采用数值模拟方法研究了泡沫铝夹芯管在横向爆炸载荷下的动态响应,对比分析了泡沫铝夹芯管的几何参数、芯层的相对密度和爆炸加载条件对泡沫铝夹芯管的总吸能、比吸能和内、外管跨中挠度的影响。以泡沫铝夹芯管内管壁厚、外管壁厚和芯层相对密度为设计变量进行多目标优化,以提高泡沫铝夹芯管结构的抗爆性能,得到以下主要结论。
(1) 泡沫铝夹芯管结构的几何参数和芯层的相对密度对其抗爆性能存在显著影响,相对于内管的直径和壁厚,外管直径和壁厚对内外管跨中挠度的影响更显著。随着外管直径的增大和内管直径的减小,泡沫铝夹芯管内管跨中挠度减小,外管跨中挠度增大,比吸能随内外管直径的减小而减小;随着内管和外管壁厚的增大,泡沫铝夹芯管的比吸能和内外管跨中挠度减小。泡沫铝芯层的相对密度越大,泡沫铝夹芯管的比吸能和内、外管跨中挠度越小。
(2) 随着炸药质量的增加和起爆距离的减小,泡沫铝夹芯管的变形区域增大,比吸能和内、外管跨中挠度增大。分析了不同工况下泡沫铝夹芯管各组成部分的吸能情况,其中外管吸收的能量最多,均超过了50%,其次是泡沫铝芯层,吸能最少的是内管;内管壁厚对各部分吸能百分比的影响较小;芯层的厚度和相对密度对泡沫铝夹芯管的吸能百分比影响显著。
(3) 以内管壁厚、外管壁厚和芯层相对密度为设计变量,以芯层的比吸能和外管跨中挠度为优化指标,采用响应面法和非支配排序遗传算法可以实现泡沫铝夹芯管抗爆性多目标优化设计,得到了Pareto前沿图,发现外管跨中挠度取最小值时,对应的芯层比吸能最小。因此,当确定了外管跨中挠度和芯层比吸能的偏好时,可确定泡沫铝夹芯管结构的内外管壁厚和芯层的相对密度。
-
ρ*/% ρ/(g·cm−3) E/MPa μ μc μp 10 0.27 48 0 1.41 0.17 15 0.40 71 0 1.12 0.29 20 0.54 143 0 1.10 0.30 表 2 理论冲量与实验冲量的对比
Table 2. Comparison of impulse between theoretical solution and experiment
Test piece Ws/g R/mm D/mm H/mm m/kg p0/MPa IE/(N·s) IT/(N·s) IT−IEIE/% No.1 30 40 76 0.7 96.8 27.7 7.1 7.4 4.2 No.2 50 40 76 0.7 96.7 45.8 12.9 12.2 −5.4 表 3 试件几何参数
Table 3. Geometric parameter of specimens
Test piece D/mm d/mm H/mm h/mm L/mm m/g NS01 101 76 0.7 0.7 280 1 242.62 NS02 101 65 0.7 0.7 280 1 326.02 NS03 101 54 0.7 0.7 280 1 388.13 NS04 90 54 0.7 0.7 280 1 150.28 NS05 79 54 0.7 0.7 280 933.72 NS06 101 76 0.8 0.7 280 1 312.19 NS07 101 76 0.9 0.7 280 1 381.75 NS08 101 76 0.7 0.8 280 1 294.97 NS09 101 76 0.7 0.9 280 1 347.31 表 4 外管直径和壁厚的影响
Table 4. Effect of outer tube diameter and wall thickness
Test piece Et/J m/g Ea/(J·g−1) wo/mm wi/mm NS03 539.878 1 388.13 0.389 10.78 1.95 NS04 442.605 1 150.28 0.385 9.06 2.21 NS05 354.622 933.72 0.380 8.88 2.87 NS01 542.923 1 242.62 0.437 10.06 2.45 NS06 456.645 1 312.19 0.348 7.61 2.16 NS07 388.472 1 381.75 0.281 5.81 1.76 表 5 内管直径和壁厚的影响
Table 5. Effect of inner tube diameter and wall thickness
Test piece Et/J m/g Ea/(J·g−1) wo/mm wi/mm NS01 542.923 1 242.62 0.437 10.06 2.45 NS02 546.692 1 326.02 0.412 10.39 2.32 NS03 539.878 1 388.13 0.389 10.78 1.95 NS08 542.470 1 294.97 0.419 9.92 2.16 NS09 542.161 1 347.31 0.402 9.81 1.92 表 6 泡沫铝相对密度的影响
Table 6. Effect of relative density of aluminum foam
ρ*/% p0/MPa Et/J m/g Ea/(J·g−1) wo/mm wi/mm 10 36.8 614.301 1 116.12 0.550 14.77 4.99 15 36.8 542.923 1 242.62 0.437 10.06 2.45 20 36.8 379.921 1 378.86 0.276 4.82 2.35 表 7 爆炸加载条件的影响
Table 7. Effect of blast loading conditions
R/mm Ws/g p0/MPa Et/J Ea/(J·g−1) wo/mm wi/mm 37 30 34.9 935.594 0.753 14.96 4.34 40 30 27.7 542.923 0.437 10.06 2.45 40 35 32.3 776.974 0.625 12.60 3.62 40 40 36.8 1058.910 0.852 16.20 4.98 43 30 22.4 312.212 0.251 5.92 1.54 表 8 泡沫铝夹芯管组成部分的吸能百分比
Table 8. Energy absorption percentage of aluminum foam-filled sandwich tube components
Test piece ρ∗/% R/mm Ws/g p0/MPa Energy absorption percentage/% Outer tube Foam core Inner tube NS01 15 40 30 27.7 59.9 32.0 8.1 NS02 15 40 30 27.7 62.6 30.5 6.9 NS03 15 40 30 27.7 61.8 33.7 4.5 NS04 15 40 30 27.7 62.4 30.7 6.9 NS05 15 40 30 27.7 59.7 32.3 8.0 NS06 15 40 30 27.7 64.0 28.1 7.9 NS07 15 40 30 27.7 66.3 25.6 8.2 NS08 15 40 30 27.7 59.5 32.4 8.0 NS09 15 40 30 27.7 59.2 32.9 7.9 NS01 10 40 30 27.7 79.8 17.5 2.7 NS01 20 40 30 27.7 52.0 32.2 15.8 NS01 15 37 30 34.9 61.7 31.0 7.3 NS01 15 43 30 22.4 61.5 29.6 8.9 NS01 15 40 35 32.3 62.0 30.5 7.5 NS01 15 40 40 36.8 61.5 31.2 7.3 表 9 最佳设计点
Table 9. Optimum design point
Design point H/mm h/mm ρ∗/% wo ECSA FEM/mm RSM/mm Error/% FEM/(J·g−1) RSM/(J·g−1) Error/% Op1 0.70 0.76 13.7 0.457 0.505 −9.5 10.50 11.14 −5.7 Op2 1.78 1.75 18.8 0.051 0.056 −8.9 0.64 0.59 8.5 Op3 0.84 1.76 20.0 0.194 0.205 −5.4 2.85 2.83 0.7 -
[1] MIRFENDERESKI L, SALIMI M, ZIAEIRAD S. Parametric study and numerical analysis of empty and foam-filled thin-walled tubes under static and dynamic loadings [J]. International Journal of Mechanical Sciences, 2008, 50(6): 1042–1057. doi: 10.1016/j.ijmecsci.2008.02.007 [2] ZHANG J X, DU J L, MIAO F X, et al. Plastic behavior of slender circular metal foam-filled tubes under transverse loading [J]. Thin-Walled Structures, 2022, 171: 108768. doi: 10.1016/j.tws.2021.108768 [3] 苏兴亚, 敬霖, 赵隆茂. 爆炸载荷下分层梯度泡沫铝夹芯板的失效模式与抗冲击性能 [J]. 爆炸与冲击, 2019, 39(6): 063103. doi: 10.11883/bzycj-2018-0198SU X Y, JING L, ZHAO L M. Failure modes and shock resistance of sandwich panels with layered-gradient aluminum foam cores under air-blast loading [J]. Explosion and Shock Waves, 2019, 39(6): 063103. doi: 10.11883/bzycj-2018-0198 [4] FAN Z H, LU G X, RUAN D, et al. Dynamic lateral crushing of empty and sandwich tubes [J]. International Journal of Impact Engineering, 2013, 53: 3–16. doi: 10.1016/j.ijimpeng.2012.09.006 [5] HALL I W, GUDEN M, CLAAR T D. Transverse and longitudinal crushing of aluminum-foam filled tubes [J]. 2002, 46(7): 513−518. [6] SONG K J, LONG Y, JI C, et al. Plastic deformation of metal tubes subjected to lateral blast loads [J]. Mathematical Problems in Engineering. 2014, 2014: 250379. [7] YUEN S C K, NURICK G N, BRINCKMANN H B, et al. Response of cylindrical shells to lateral blast load [J]. International Journal of Protective Structure. 2013, 4(3): 209−230. [8] WIERZBICKI T, HOOFATT M S. Damage assessment of cylinders due to impact and explosive loading [J]. International Journal of Impact Engineering, 1993, 13(2): 215–241. doi: 10.1016/0734-743X(93)90094-N [9] JING L, WANG Z H, ZHAO L M. Dynamic response of cylindrical sandwich shells with metallic foam cores under blast loading-numerical simulations [J]. Composite Structures, 2013, 99: 213–223. doi: 10.1016/j.compstruct.2012.12.013 [10] JING L, WANG Z H, ZHAO L M. An approximate theoretical analysis for clamped cylindrical sandwich shells with metallic foam cores subjected to impulsive loading [J]. Composites Part B: Engineering, 2014, 60: 150–157. doi: 10.1016/j.compositesb.2013.12.047 [11] JING L, WANG Z H, SHIM V P W. An experimental study of the dynamic response of cylindrical sandwich shells with metallic foam cores subjected to blast loading [J]. International Journal of Impact Engineering, 2014, 71: 60–72. doi: 10.1016/j.ijimpeng.2014.03.009 [12] HOO FATT M S, SURABHI H. Blast resistance and energy absorption of foam-core cylindrical sandwich shells under external blast [J]. Composite Structures, 2012, 94(11): 3174–3185. doi: 10.1016/j.compstruct.2012.05.013 [13] LIU Z F, ZHANG T H, LI S Q, et al. Experiment and numerical simulation on the dynamic response of foam-filled tubes under lateral blast loading [J]. Acta Mechanica Solida Sinica, 2021, 34(6): 937–953. doi: 10.1007/s10338-021-00285-1 [14] BAROUTAJI A, GILCHRIST M D, SMYTH D, et al. Crush analysis and multi-objective optimization design for circular tube under quasi-static lateral loading [J]. Thin-Walled Structures, 2015, 86: 121–131. doi: 10.1016/j.tws.2014.08.018 [15] BAROUTAJI A, GILCHRIST M D, SMYTH D, et al. Analysis and optimization of sandwich tubes energy absorbers under lateral loading [J]. International Journal of Impact Engineering, 2015, 82: 74–88. doi: 10.1016/j.ijimpeng.2015.01.005 [16] QI C, YANG S, YANG L J, et al. Blast resistance and multi-objective optimization of aluminum foam-cored sandwich panels [J]. Composite Structures, 2013, 105: 45–57. doi: 10.1016/j.compstruct.2013.04.043 [17] LI S Q, YU B L, KARAGIOZOVA D, et al. Experimental, numerical, and theoretical studies of the response of short cylindrical stainless steel tubes under lateral air blast loading [J]. International Journal of Impact Engineering, 2019, 124: 48–60. doi: 10.1016/j.ijimpeng.2018.10.004 [18] LI S Q, WANG Z H, WU G Y, et al. Dynamic response of sandwich spherical shell with graded metallic foam cores subjected to blast loading [J]. Composites Part A: Applied Science and Manufacturing, 2014, 56: 262–271. doi: 10.1016/j.compositesa.2013.10.019 [19] GOEL M D, MATSAGAR V A, GUPTA A K, et al. An abridged review of blast wave parameters [J]. Defence Science Journal, 2012, 62(5): 300–306. doi: 10.14429/dsj.62.1149 [20] 李磊, 马宏昊, 沈兆武. 基于正交设计方法的双锥罩结构优化设计 [J]. 爆炸与冲击, 2013, 33(6): 567–573. doi: 10.3969/j.issn.1001-1455.2013.06.002LI L, MA H H, SHEN Z W. Optimal design of biconical liner structure based on orthogonal design method [J]. Explosion and Shock Waves, 2013, 33(6): 567–573. doi: 10.3969/j.issn.1001-1455.2013.06.002 -








 下载:
下载:



















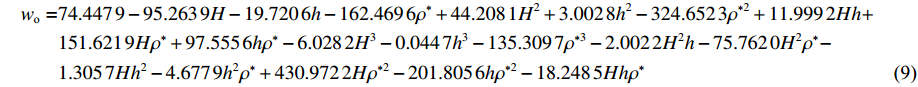
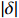
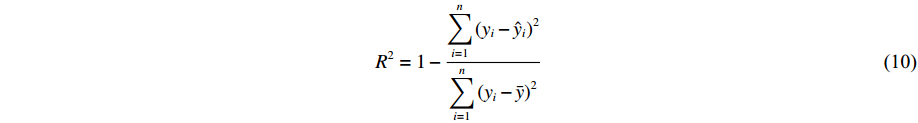
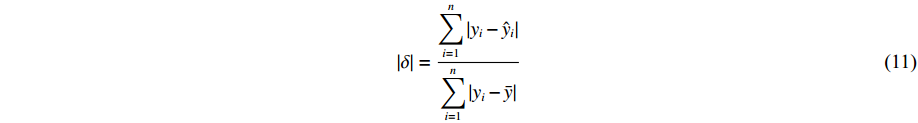
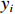
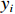
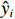
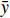
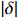
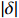
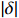
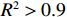


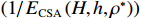
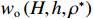
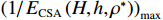
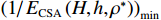
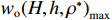
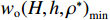

 下载:
下载: