Design and Mechanical Properties of Star-Shaped Structure with Double Stress Plateaus
-
摘要: 为实现多孔格栅类结构平台应力和能量吸收的可调控,提出了一种双应力平台星形结构的设计方法,设计并制备了3种双应力平台星形结构。采用实验、理论分析与数值模拟相结合的方法研究了结构在面内压缩载荷下的力学行为和能量吸收性能。结果表明,双应力平台星形结构的载荷-位移曲线呈现两个明显的平台阶段,结构的几何参数和肋板数对结构变形的稳定性以及平台应力的大小存在显著影响。平台应力的理论预测结果与实验、数值模拟结果吻合较好。通过调整相应的设计参数,能够有效地调控结构在压缩过程中的平台应力和能量吸收能力。为了进一步提高双应力平台星形结构的能量吸收性能,以结构的质量和比吸能为设计变量,进行了多目标优化。采用基于径向基耦合多项式函数代理模型和遗传算法(NSGA-Ⅱ),使结构比吸能最大化的同时质量最小化。与最初设计的结构相比,优化后结构的质量减小了6.0%,比吸能提高了21.5%。Abstract: To achieve the tunable plateau stress and energy absorption in porous grid structures, a design method for star-shaped structures with double stress plateaus was proposed. Three kinds of star-shaped structures with double stress plateaus were designed and fabricated. The mechanical behavior and energy absorption properties under in-plane compressive load were investigated through experimental tests, theoretical analysis and numerical simulations. It was shown that the star-shaped structures with double stress plateaus exhibit two distinct plateau steps in the load-displacement curves. The geometric parameters of the structure and the number of ribs have a significant effect on the structural deformation stability and the plateau stress. The theoretical predictions, experimental results and numerical simulations were in good agreement with each other. The plateau stress and energy absorption capacity of star-shaped structures can be effectively controlled by tuning the corresponding design parameters. To further improve the energy absorption capacity of the star-shaped structure with double stress plateaus, a multi-objective optimization method was performed using the mass and specific absorption energy of the structure as design variables. The radial basis coupling polynomial function proxy model and genetic algorithm (NSGA-Ⅱ) were used to maximize the specific energy absorption and minimize the mass of the structure. Compared to the original structure, the optimized structure has a 6.0% reduction in mass and a 21.5% increase in specific energy absorption.
-
侵彻战斗部主装药广泛采用PBX炸药,由于服役环境可能同时存在多种外界刺激,炸药自身的力学响应会影响侵彻过程中装药的安全性和战斗部的毁伤能力[1],因此PBX装药对弹药的整体性能有着决定性意义。而在目标侵彻过程中,侵彻战斗部的内部装药面临复杂的力学环境[2],同时复杂的装药结构也会对内部装药产生影响。在这些外部刺激下,炸药中的局部损伤处可能产生热点,导致意外点火的发生,进而可能转化成为更剧烈的爆燃或者爆轰,引发多种事故。因此研究各种复杂侵彻环境下装药的力学性能与损伤响应,对保障弹药在整个服役过程中的安全性与可靠性具有重要意义。
对于侵彻过程中内部装药的安全性而言,建立能够准确描述炸药力学响应和损伤行为的本构模型,是通过数值模拟方法研究侵彻装药安全性问题的关键。PBX炸药动态损伤本构模型可以划分为两类:第一类是基于黏弹性、黏塑性等连续介质损伤力学基础理论,考虑应变率、温度对PBX炸药力学行为的影响而建立的宏观唯象经验本构模型[3-9];第二类则考虑微缺陷是PBX炸药中一类重要的细观结构,是基于材料力学损伤-点火行为和细观缺陷演化与热点形成之间的联系而建立的PBX炸药细观损伤力学本构模型[10-13]。近年来,国内外学者基于以上模型对侵彻装药安全性进行了广泛的研究。张馨予等[14]将孔隙压塌损伤、炸药晶体破碎损伤、黏结剂脱黏等多种细观损伤形式耦合到炸药宏观本构模型中,研究了侵彻环境下弹体装药的损伤分布情况。石啸海等[15-16]基于内聚裂纹模型,模拟了战斗部侵彻半无限大混凝土过程中PBX装药的动态力学响应及损伤演化,并且对某种缩比弹侵彻混凝土靶板进行了数值模拟,从过载、裂纹宽度、裂纹含量等角度比较了弹头形状对装药损伤的影响,结果表明控制弹头曲径比有利于减小装药损伤。成丽蓉等[17]基于裂纹摩擦、孔洞塌缩两种热点生成机制细观模型,开展了侵彻单层和多层典型靶板时战斗部装药的动态响应、损伤演化及热点生成对比研究。Li等[18]基于微裂纹动态损伤模型,通过数值模拟方法预测了实弹侵彻实验中PBX1314的损伤分布、点火位置与点火区域的反应进程,结果表明,侵彻过程中经多次撞击载荷作用,PBX1314尾端面局部区域达到点火临界条件而发生点火,反复撞击过程中PBX1314内部微裂纹摩擦引起的能量局部化是引发点火的重要原因。
上述模型往往仅考虑了剪切裂纹热点等单一缺陷机制的细观力热响应过程,在研究复杂载荷条件下不同类型PBX炸药损伤-热点主导机制的自适应能力方面,模型还需要进一步改进。此外,上述研究工作多围绕压装型炸药PBX展开,针对浇注型PBX炸药的研究较少,由于浇注类PBX炸药和压装类PBX炸药的材料组分、物理状态和成型工艺不同,力学性能与点火特性也存在差异,因此对比其在侵彻环境下的响应特性,对于战斗部装药材料的选取和装药结构设计具有重要意义。基于此,本研究应用前期发展的PBX炸药微裂纹-微孔洞力热化学耦合细观模型[18],考虑微裂纹-微孔洞两种细观缺陷演化对炸药损伤-热点形成的影响,通过动态分离式霍普金森压杆(SHPB)实验校核模型参数,并利用数值模拟方法分析两类典型装药(压装PBX04和浇注GOFL-5)在弹体侵彻混凝土薄板过程中的应力波传播、损伤演化和温升响应情况,为深入理解侵彻过程中装药的力学-损伤-点火响应提供参考。
1. 本构模型与参数校核
1.1 PBX炸药微裂纹-微孔洞力热化学耦合细观模型
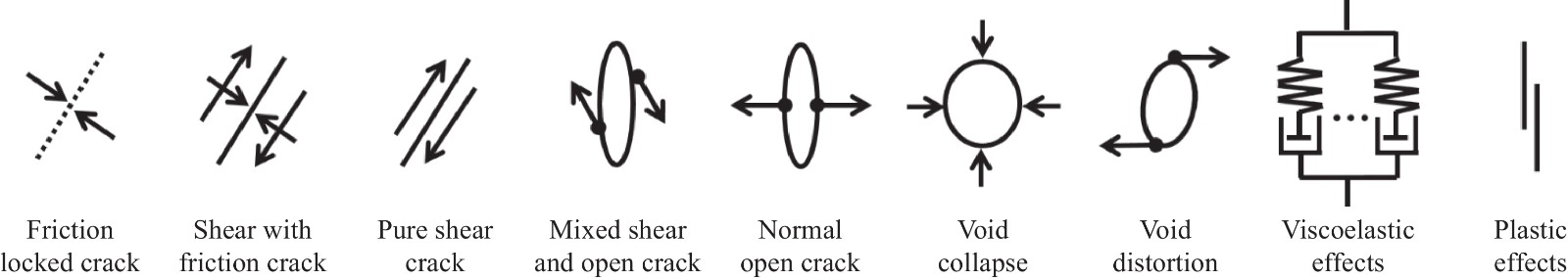
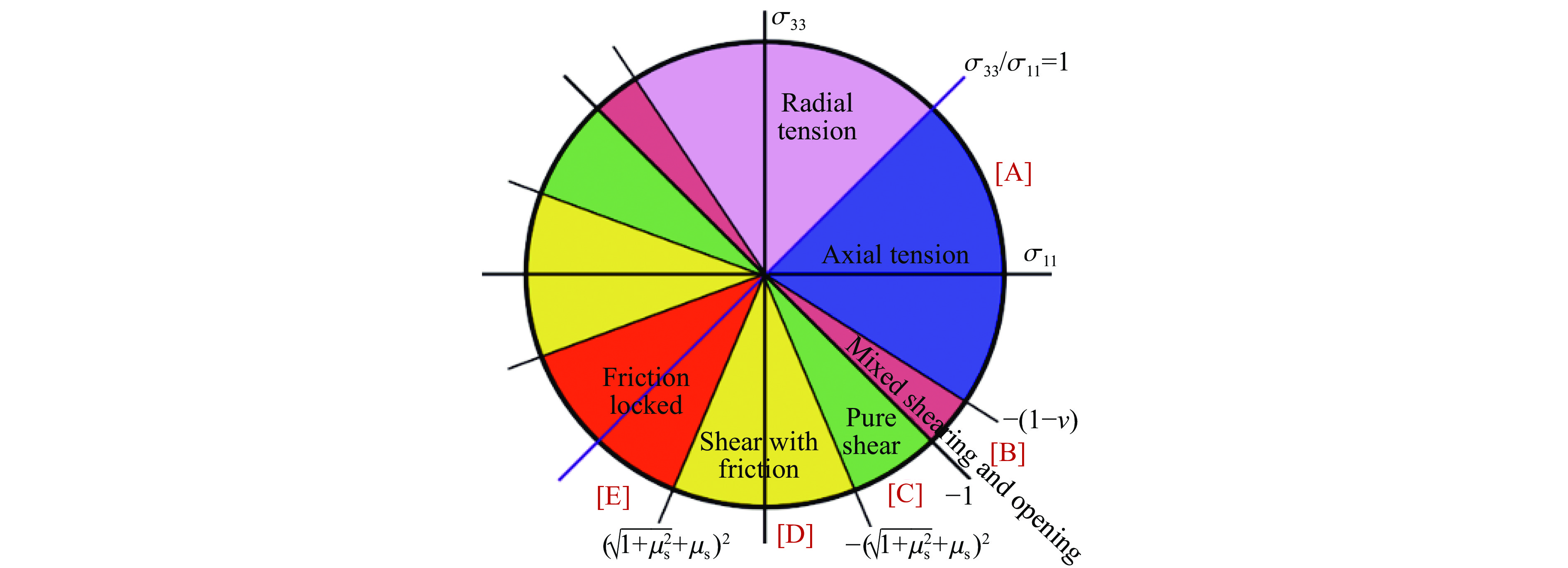
PBX炸药微裂纹-微孔洞力热化学耦合细观模型(CMM)中考虑了拉伸张开、剪切张开、纯剪切、剪切摩擦、摩擦自锁5种微裂纹演化模式,以及微孔洞坍塌与扭曲变形两种演化模式,如图1所示。同时,CMM模型还囊括了剪切裂纹热点与孔洞坍塌热点子模型,具有复杂应力状态-微缺陷演化模式自判断能力,以及两种微缺陷热点自启动能力。CMM模型中总体应力、应变分别分解为偏量部分与体量部分,依次建立微裂纹相关偏量本构关系与微孔洞相关体量本构关系,二者通过Gurson屈服准则进行耦合,通过微裂纹、微孔洞演化方程更新相关变量,建立剪切裂纹摩擦热点与孔洞坍塌热点子模型,模型细节与算法实现详见文献[19–20]。
将总体偏应变(
ε )分解为黏弹性应变(ε ve)、塑性应变(ε p)以及由于微裂纹扩展所引起的微裂纹应变(ε cr) 3部分,分别描述PBX炸药内黏结剂基体材料所表现出的黏弹性变形、塑性变形以及由于微裂纹扩展对材料力学行为的影响。PBX炸药黏弹性变形由广义Maxwell模型描述,微裂纹张开/剪切扩展引起的裂纹应变由SCRAM模型描述,材料的偏量本构关系可表示为
˙S=2GA0⋅(˙ε−˙εp)−B0⋅(S+C0) (1) 式中:
S 为应力偏量,G为剪切模量,A0、B0、C0表示为 A0=11+αe3(ˉc/a)3,B0=αe(ˉc/a)2˙ˉc/a1+αe3(ˉc/a)3,C0=N∑n=1Sn/τnαe(ˉc/a)2˙ˉc/a (2) 式中:
ˉc 为微裂纹平均尺寸;a为微裂纹初始特征尺寸,a−3=6Gβ ;Sn和τn 为广义黏弹性体元中第n个Maxwell黏弹性单元中的偏应力和松弛时间。αe={3p⩾05−νp<0,β=64π(1−ν)N015(2−ν)G (3) 式中:p为压力,N0为初始裂纹密度,
ν 为泊松比。为描述材料内微孔洞演化所发生的不可逆损伤对PBX炸药体积变形的影响,采用孔隙率相关状态方程
p(ρ,e,f)=(1−f)[ρsc20ηs(1−sηs)2(1−Γsηs2)+Γsρses] (4) 式中:f为孔隙率;
ρ 、e分别为孔隙材料的密度与比内能,密实材料对应的ρ s =ρ /(1 − f),es = e,η s = 1 −ρs0 /ρs ,ρs0为密实材料的初始密度;Γ s为Grüneisen系数;c0、s为材料参数。含孔隙PBX材料的塑性变形采用经典Gurson模型描述,模型中材料屈服面与von-Mises等效应力
σe 和压力p相关F(σe,p,f)=(σeYM)2+2fcosh(−3p2YM)−f2−1=0 (5) 考虑动态加载下材料硬化效应与应变率效应,密实材料(f = 0)的屈服强度可表示为
YM=[σ0+h(ˉεpM)n][1+Cln(1+˙ε∗)] (6) 式中:
σ0 为˙ε0 =10 s−1加载下所对应的初始屈服应力,ˉεpM 为等效塑性应变,˙ε∗=˙ε/˙ε0 为等效应变率,h为硬化模量,C为应变率相关系数。基于Griffith能量释放率裂纹扩展准则,微裂纹扩展方程可表示为
˙ˉc=˙cmax (7) 式中:
{\dot c_{\max }} 为最大扩展速度,\bar \gamma 为材料比表面能,{g_{\rm{dom}}}\left( {{{\sigma}},\bar c} \right) 为主裂纹对应的能量释放率。由于材料内微裂纹方向分布具有随机性,存在临界微裂纹方向,其对应的能量释放率最大,即该方向的微裂纹在最小施加应力下最先发生失稳扩展,因此定义为主裂纹。主裂纹方向的确定与当前应力状态相关(
\sigma 1~\sigma 3平面),微裂纹扩展对应的材料整体损伤度定义为{d_{\rm{cr}}} = {{{{\bar c}^3}} / {\left( {{a^3} + {{\bar c}^3}} \right)}} 。考虑孔洞坍塌引起的孔隙率减小,以及孔洞扭曲引起的孔隙率增加两种变形机制,孔隙率演化方程可表示为
\dot f = \left( {1 - f} \right)\dot \varepsilon _V^{\rm{p}} + f{k_{\rm{w}}}\omega \left( \sigma \right)\frac{{{s_{ij}}\dot e_{ij}^{\rm{p}}}}{{{\sigma _{\rm{e}}}}} (8) 式中:
\dot \varepsilon _V^{\rm{p}} = {{\dot \varepsilon _{\rm{kk}}^{\rm{p}}} / 3} ,为塑性体积应变率;kw为剪切相关材料参数;\omega 与应力状态相关,取值范围为0 ≤\omega ≤ 1。\omega \left( {{\sigma}} \right) = 1 - {\left( {\frac{{27{{{J}}_3}}}{{2{{\sigma}} _{\rm{e}}^3}}} \right)^2} (9) 式中:J3为表示应力张量的第三不变量。
采用一维热传导方程来描述剪切裂纹表面及其周围区域摩擦生热、熔化、点火与传热等热力学过程
\rho {c_V}{\dot T_{\rm{hs}}} = \frac{\partial }{{\partial x}}\left( {\kappa \frac{{\partial {T_{\rm{hs}}}}}{{\partial x}}} \right) + \rho {Q_{\rm{r}}}Z{{\rm{e}}^{ - E/(R{T_{\rm{hs}}})}} + \varphi {\mu _v}{\dot \varepsilon_{\rm{m}} ^2} (10) 式中:x为沿微裂纹法向的坐标轴;等式右端3项分别表示热传导项、化学反应释放热量以及熔化区域(Ths ≥ Tm)液相黏性流动生热;Ths、cV、κ和Qr分别为微裂纹热点温度、比定容热容、热传导系数以及单位质量化学反应放热;Z和E为Arrhenius反应速率方程参数;
\varphi (0 ≤\varphi ≤ 1)为熔化百分数;{\;\mu _v} 为熔化区域黏性系数;\dot \varepsilon_{\rm{m}}= \varphi {v_{\rm{c}}}/l 为熔化区域的剪切应变率,vc为微裂纹滑动速度,熔化区域宽度l由熔化区域的传播速度确定,\dot l(t) = \Delta \left( {k{T_x}} \right)/\rho L ,其中\Delta \left( {k{T_x}} \right) 表示固液分界面热流量梯度。微孔洞周围材料温度(Tvo)分布情况由一维球形热传导方程进行描述
\rho {c_V}{\dot T_{\rm{vo}}} = k\left[ {\frac{{{\partial ^2}{T_{\rm{vo}}}}}{{\partial {r^2}}} + \frac{2}{r}\frac{{\partial {T_{\rm{vo}}}}}{{\partial r}}} \right] + \dot w_{\rm{vp}}^* + \rho {Q_{\rm{r}}}Z{{\rm{e}}^{ - E/(R{T_{\rm{vo}}})}} (11) 其中,孔洞周围单位体黏塑性功生成速率可表示为
\dot w_{\rm{vp}}^*\left( r \right) = s_{ij}^*\dot \varepsilon _{ij}^* = \frac{{ - 2{Y_{\rm{M}}}\dot \varepsilon _V^{\rm{p}}}}{{{r^3}}}\frac{{1 - {f_0}}}{{1 - {f_{\rm{vc}}}}}b_0^3 + \frac{{4\eta {{\left( {\dot \varepsilon _V^{\rm{p}}} \right)}^2}}}{{{r^6}}}{\left( {\frac{{1 - {f_0}}}{{1 - {f_{\rm{vc}}}}}} \right)^2}b_0^6 (12) 式中:等号右边两项分别表示基体材料的塑性效应与黏性效应所产生的功率,b0为微孔洞初始外径,
\eta 为微孔洞周围基体材料相关的黏性系数。1.2 模型参数标定
应用SHPB方法对中国工程物理研究院化工材料研究所提供的压装和浇注两类PBX炸药进行动态力学性能测试,浇注类复合炸药选取GOFL-5炸药(HMX、FOX-7、黏结剂的质量分数分别为50%、35%、15%),样品尺寸为20 mm × 20 mm,密度为1.75 g/cm3,初始孔隙率为1.01%。压装类复合炸药选取PBX04炸药(HMX、黏结剂的质量分数分别为95%、5%),密度为1.82 g/cm3,初始孔隙率为1.08%。在中国工程物理研究院化工材料研究所物理与力学性能实验室开展SHPB实验,实验中采用直径为20 mm的铝杆,子弹长度为300 mm,入射杆、透射杆长度分别为2 000、1 500 mm。Yang等[19]在前期研究了CMM模型中微缺陷参数(
{\bar c_0} , N0,\bar \gamma , f0, kw)对材料力学性能的影响规律。基于此,依次对CMM模型中的广义Maxwell黏弹性参数(G1~G5, τ1~τ5)、塑性参数({\sigma _0} , h, C, n)、微裂纹模型参数({\bar c_0} , N0,\bar \gamma , m)、微孔洞模型参数(f0, kw)以及Mie-Grüneisen状态方程参数(c0, s, Г)进行优化调整,使得标定的参数能够最大程度地符合计算与实验应力-应变曲线。压装炸药PBX04与浇注炸药GOFL-5标定曲线如图2所示,相关材料参数如表1所示,其中{\mu _{\rm{s}}} 为静摩擦系数。由表1可知:两种炸药材料的黏弹性模量G1~G5存在数量级差异,GOFL-5炸药的塑性参数({\sigma _0} , h, C)均远小于PBX-5炸药,GOFL-5炸药的初始微裂纹密度与微裂纹尺寸均小于PBX-5炸药。表 1 GOFL-5与PBX04材料参数Table 1. Material parameters for GOFL-5 and PBX04Material \;\rho 0/(kg·m−3) G/GPa \nu G1/MPa G2/MPa G3/MPa G4/MPa G5/MPa {\tau{_1^{-1} } } /{\rm{s} }{^{-1} } GOFL-5 1 750 0.55 0.3 167 30.45 90.03 185.6 120.0 0 PBX04 1 820 8.25 0.3 1 940 1 175.00 1 521.00 1 909.0 1 688.0 0 Material {\tau{_2^{-1} } } /{\rm{s} }{^{-1} } {\tau{_3^{-1} } } /{\rm{s} }{^{-1} } {\tau{_4^{-1} } } /{\rm{s} }{^{-1} } {\tau{_5^{-1} } } /{\rm{s} }{^{-1} } \sigma{{_0}}/MPa C h/MPa n {\bar c}0/μm GOFL-5 7.32 × 103 7.32 × 104 7.32 × 105 7.32 × 106 2.2 0.76 4.5 0.45 30 PBX04 9.00 × 103 9.00 × 104 9.00 × 105 2.00 × 106 40.0 0.10 1500.0 1.00 30 Material N0/cm−3 \bar \gamma /(J·m−2) {\dot c_{\max }}/(m·s–1) \;\mu_{\rm{s}} m f0/(m·s–1) kw c0/(m·s–1) s \varGamma GOFL-5 3 0.5 300 0.3 5.0 0.01 2.0 1 000 0.46 0.89 PBX04 300 1.4 300 0.5 5.0 0.01 2.0 2 500 2.26 1.50 对比压装炸药与浇注炸药的应力-应变曲线,可以看到PBX04炸药峰值应力远高于GOFL-5炸药,但其破坏应变远小于GOFL-5炸药。两种材料的力学行为差异主要与其内部微缺陷数量和黏结剂含量相关。压装类PBX04炸药内微裂纹含量较多,且黏结剂含量较少,因此材料表现为准脆性材料破坏特征。浇注类GOFL-5炸药内部微缺陷数量较少,且对材料起到增韧效应的黏结剂含量较多,表现为韧性材料破坏特征。
2. 侵彻算例分析
2.1 有限元模型
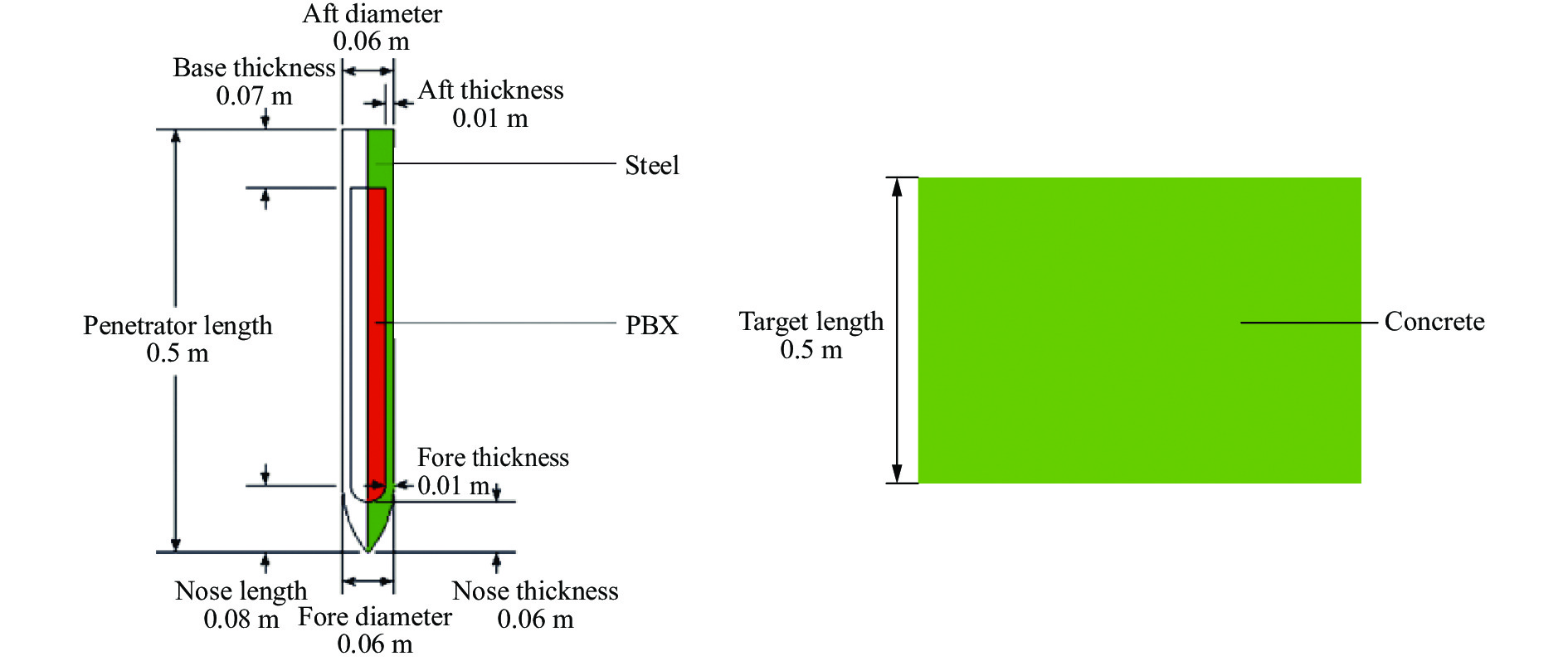
建立二维侵彻混凝土靶板的计算模型,设置轴对称边界条件和加载条件,建立1/2模型进行计算,选取侵彻速度为800 m/s,弹体尺寸如图3所示,侵彻的混凝土靶板厚度为0.5 m。计算过程中,由于侵彻过程中靶体内温度升高的区域是有限的,靶体外边界的温度不会受到侵彻过程中靶体温度变化区域的影响,因此在靶体边界处设置温度边界条件,边界温度设置成294 K。
弹体材料本构模型使用Johnson-Cook模型。该模型是一个经验型的黏塑性本构模型,由Johnson和Cook在1983年首先提出,多用于描述金属材料在高载荷、高应变率和高温下的应力-应变关系,模型形式简单,本构参数少,能较好地描述材料的加工硬化效应、应变率效应和温度软化效应。在侵彻速度不高时弹体变形较小,因此本研究运用Johnson-Cook模型来描述壳体材料4340钢的力学响应,其主要材料参数见表2,其中:
\rho 为密度,c为比热容,α为热膨胀系数,Tm为熔化温度,\kappa 为导热系数,M为温度软化系数,N为应变强化参数,C1~C4 、D1~D5为材料常数。混凝土靶板的材料模型使用HJC本构模型[21]。该模型是针对混凝土材料提出的一种率相关损伤型本构模型,适用于大应变、高应变率和高压情况,其主要材料参数见表3,其中:A、B、N为材料硬化参数,FC为材料准静态单轴抗压强度,K1~K3为材料体积模量参数,pC、pL、UC、UL为压力参数,D1、D2为损伤参数,\varepsilon_{\rm{min}} 为最小断裂应变。表 2 弹体材料参数Table 2. Parameters of projectile materialPhysical properties Johnson-Cook model \;\rho /(g·cm−3) c/(J·kg−1·K−1) \kappa /(kW·m−1·K−1) \alpha /(m·K−1) Tm/℃ G/GPa N M 7.82 478 38.11 3.24 × 10−5 1 793.15 774.97 0.26 1.03 Johnson-Cook model Damage model C1/MPa C2/MPa C3 C4 D1 D2 D3 D4 D5 792.21 509.52 1.4 0 –0.8 2.1 –0.5 20.0 0.61 表 3 混凝土靶板材料参数Table 3. Material parameters of concrete plate targetPhysical properties HJC model \;\rho /(g·cm−3) c/(J·kg−1·K−1) κ/(kW·m−1·K−1) α/(m·K−1) G/GPa FC/MPa A B N C 2.28 654 1.76 4.32 × 10−5 16.40 40.68 0.75 1.65 0.76 7.0 × 10−3 HJC model Damage model pC/MPa UC pL/GPa UL K1/GPa K2/GPa K3/GPa D1 D2 \varepsilon min 13.56 5.80 × 10−4 1.05 0.10 17.40 38.80 29.80 0.03 1.0 0.01 2.2 压缩波传播情况
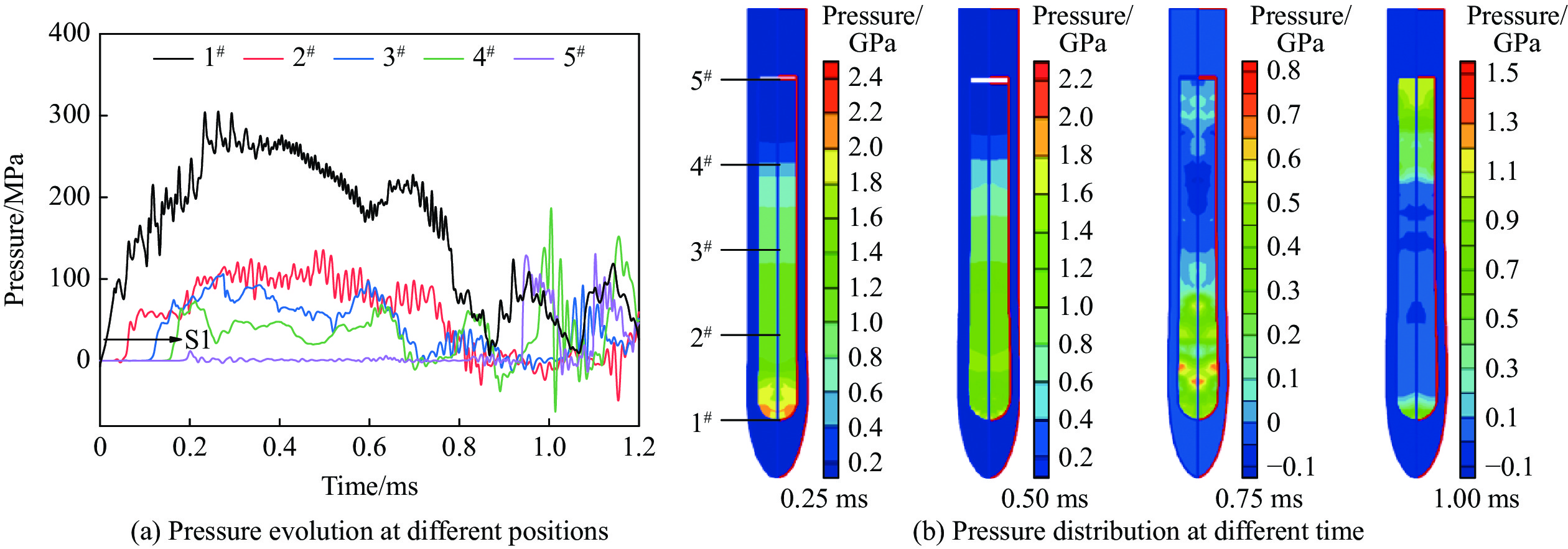
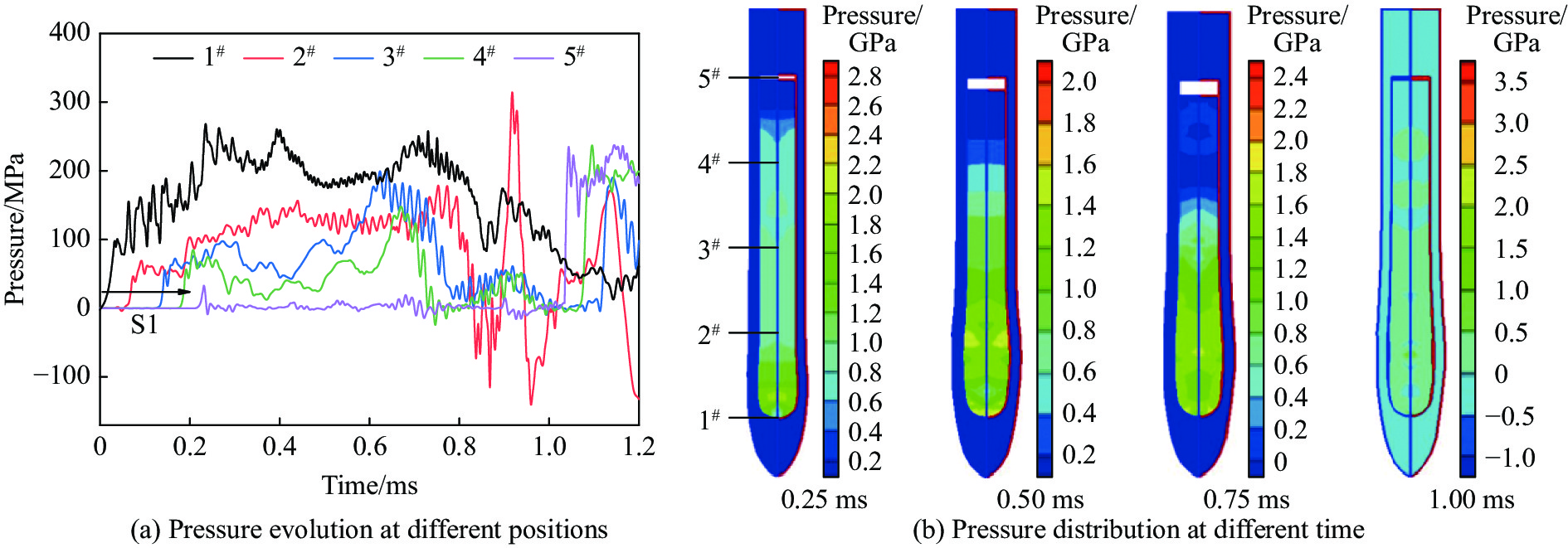
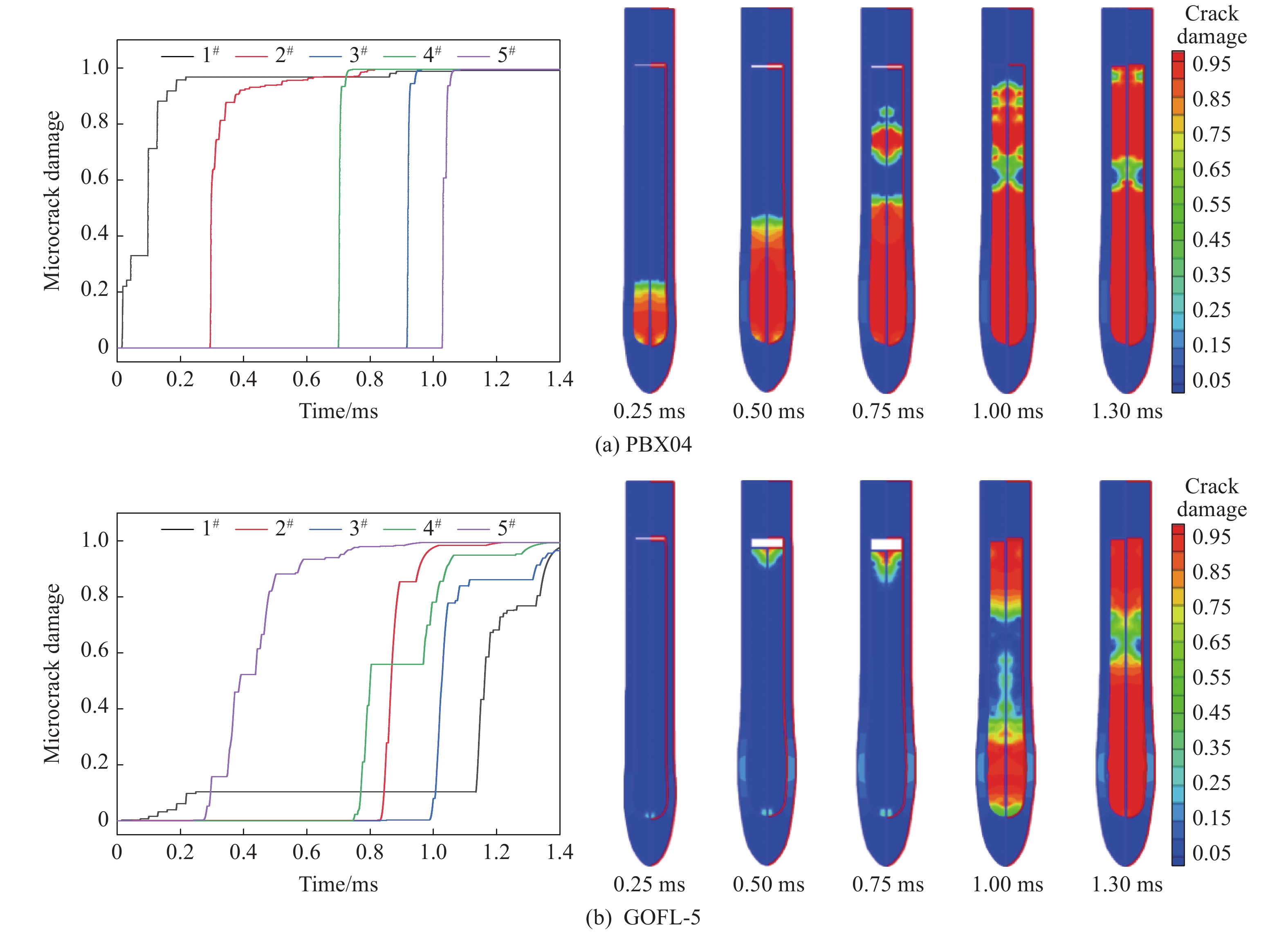
为分析药柱内压缩波传播情况,初始时刻沿药柱中心线由装药头部至尾部等间隔依次选取1#~5#位置,如图4(b)所示,图4(a)和图5(a)分别为压装(PBX04)与浇注炸药(GOFL-5)内1#~5#位置处的压力变化曲线。由图4(a)可知,弹体与混凝土靶板初始接触时,弹体受到强烈的压缩波作用,装药头部压力最大,入射压缩波S1分别于104和179 μs到达装药中部(3#位置)和装药尾部(5#位置),当应力波传播至尾部自由端面时反射拉伸波,两种应力波相互抵消,装药尾部压力值最低。930 μs时,装药尾部在弹性恢复与拉伸波的作用下与弹体内壁面发生撞击,尾部压力迅速升高。由图5(a)可知,入射压缩波S1到达装药中部和装药尾部的时刻分别为123和213 μs,对比图4(a)和图5(a)可知,相同侵彻速度下,浇注药内压缩波S1的波速(约2.11 km/s)小于压装药(约2.57 km/s)。
不同时刻压装、浇注药内压力云图演化如图4(b)和图5(b)所示。随着压缩波的传播,药柱整体受压区域逐渐扩大,装药尾部与弹体内壁产生相对间隙,浇注GOFL-5炸药由于材料强度较低,抵抗变形的能力较弱,侵彻过程中装药尾部的最大间隙(dmax)较压装药更大(GOFL-5,dmax = 1.61 cm;PBX04,dmax = 0.68 cm)。结合图4(a)和图5(a)可知,浇注GOFL-5炸药在侵彻过程中产生了较大的变形,装药尾部与弹体内壁发生撞击时尾部压力峰值(pmax)也更高(GOFL-5,pmax = 234 MPa;PBX04,pmax = 128 MPa)。
2.3 微缺陷损伤演化
图6为压装、浇注药柱内微裂纹损伤(dcr)演化情况。由图6(a)可知,加载初期压装药微裂纹损伤主要集中在头部,随着压缩波的传播,微裂纹损伤逐渐扩展至装药中部,装药尾部与弹体内壁发生撞击后尾部微裂纹损伤较严重。由图6(b)可知,浇注药的流动性较好,在侵彻过程中产生了较大的变形,尾部装药受到向药柱中心的拉应力作用产生裂纹扩展损伤。整个侵彻过程中,两类炸药微裂纹损伤较严重区域均为装药头部和尾部,由于开始侵彻时压装药内入射压缩波强度高于浇注药,同时压装药初始微裂纹密度较高,加载初期压装药头部微裂纹损伤高于浇注药。
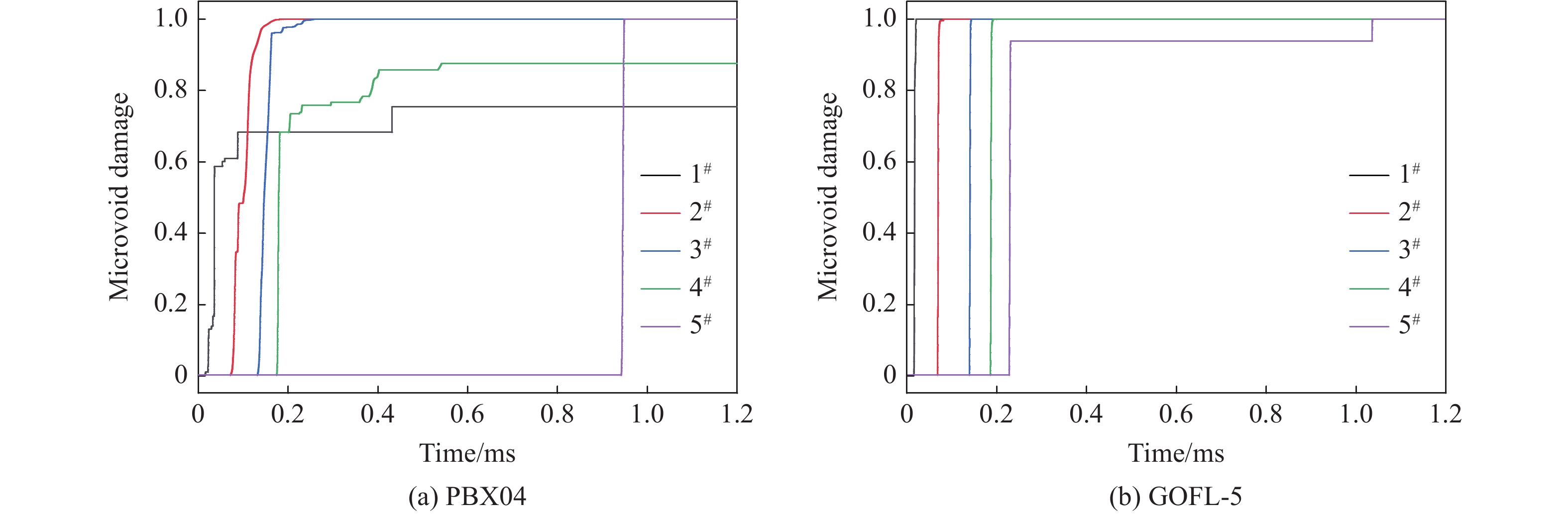
图7为不同时刻压装、浇注药柱内不同位置处微孔洞损伤(dvo = f0−ft)随时间演化曲线。对比图6和图7可知,微孔洞坍塌时间尺度相对于微裂纹扩展时间尺度明显更小,微孔洞随着入射压缩波传播而发生坍塌,孔洞坍塌损伤发生的时刻与入射压缩波到达的时刻基本一致,而由于裂纹扩展损伤主要受拉伸和剪切状态影响,入射压缩波刚到达装药对应位置时并未发生明显的微裂纹扩展损伤。
2.4 温升情况分析
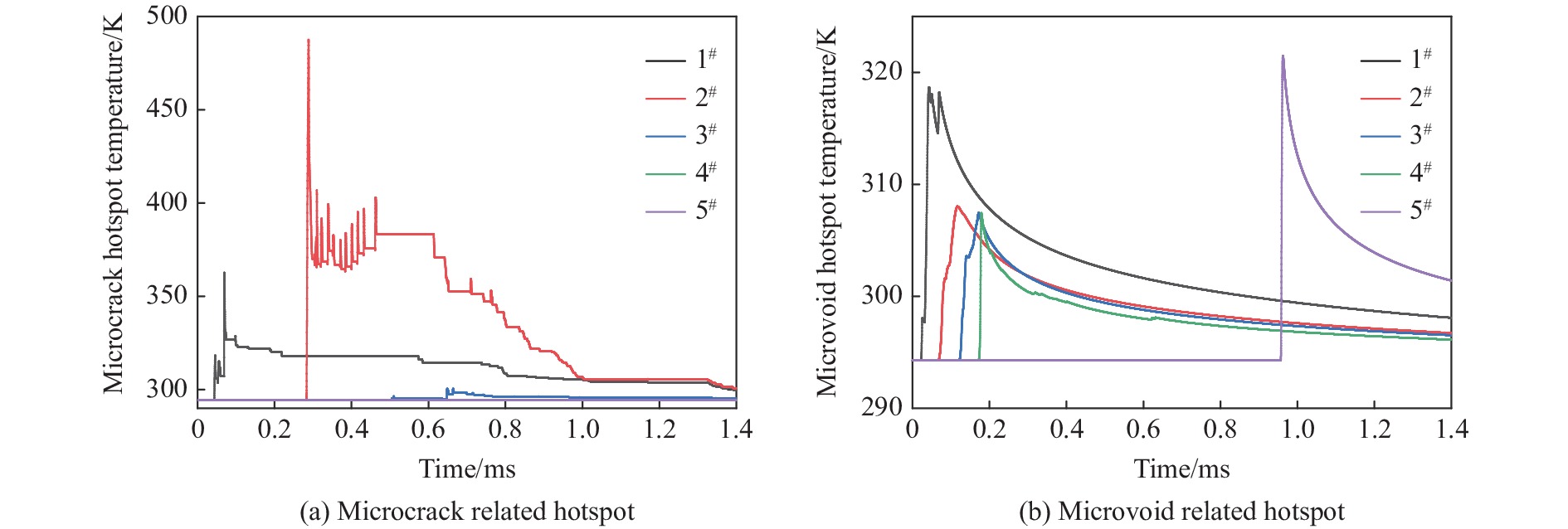
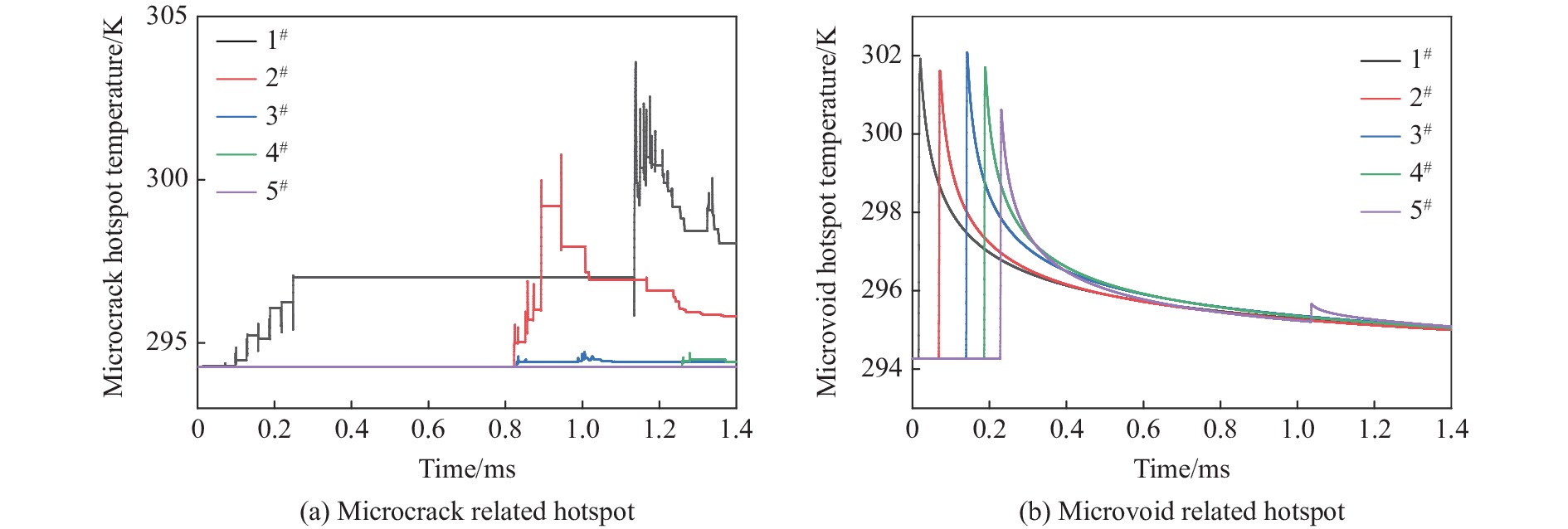
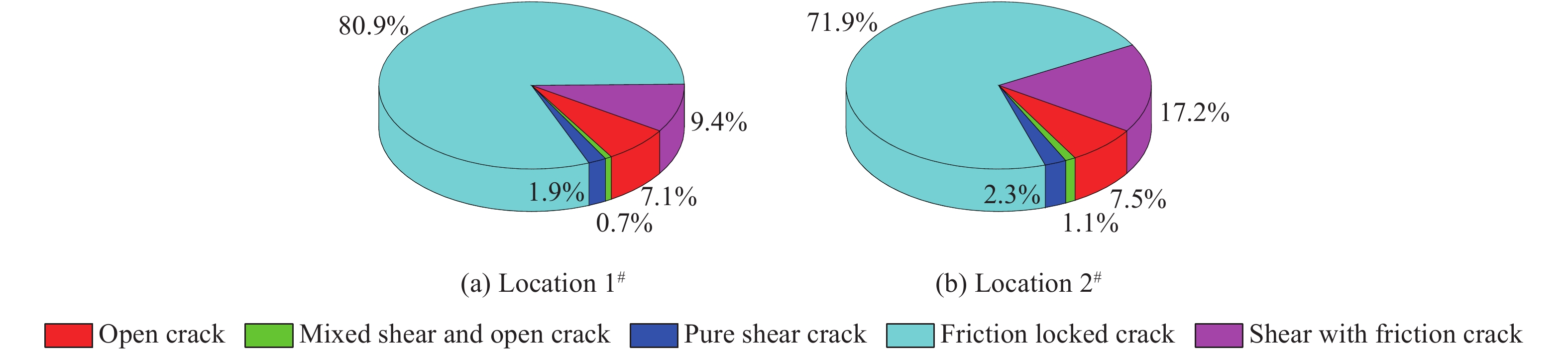
PBX04和GOFL-5内不同位置微裂纹与微孔洞热点温度随时间演化曲线分别如图8和图9所示。由图8(a)可知,压装药微裂纹热点温升主要集中在装药头部和装药前端,1#和2#处的微裂纹热点峰值分别为362和487 K。根据CMM模型中由应力二轴度可确定5种微裂纹状态(拉伸张开、剪切张开、纯剪切、剪切摩擦、摩擦自锁),5种微裂纹演化模式如图10所示,由此可进一步分析温升较高位置处的微裂纹状态。整个侵彻过程中,1#和2#处5种微裂纹状态的频率分布如图11所示。侵彻过程中弹体内装药处于压力主导的应力状态,微裂纹大多处于摩擦自锁状态而非剪切裂纹扩展状态,同时热点密度较小可能引起热点湮灭现象,因此微裂纹热点温度达到峰值后逐渐降低。对比图8(a)和图9(a)可知,由于GOFL-5材料的初始微裂纹密度、微裂纹尺寸以及微裂纹扩展速率较低,因此GOFL-5材料中剪切裂纹引起的热点温升较PBX04更低。结合图8(b)和图9(b),随着入射压缩波的传播孔洞发生坍塌,与GOFL-5不同的是,PBX04装药尾部微孔洞温升峰值主要来自弹体撞击造成的孔洞坍塌,而坍塌孔洞周围黏塑性功引起的温升较小(约20 K),不足以引起点火。对比图8和图9可知:对于压装药而言,裂纹摩擦相比孔洞坍塌引起的温升更高,剪切裂纹热点为压装药主导的温升机制;对于浇注药而言,两种热点机制引起的温升差别不大。
3. 结 论
应用PBX炸药微裂纹-微孔洞力热化学耦合细观模型,研究了压装PBX04和浇注GOFL-5两类典型装药在弹体侵彻混凝土薄板过程中应力波的传播、损伤演化和温升响应情况,对比分析了相同侵彻条件下两类炸药力学-损伤-温升响应的差异性,得到以下主要结论。
(1) 根据实验曲线标定了PBX04和GOFL-5微裂纹-微孔洞本构模型参数,两种炸药材料的弹性模量存在数量级差异,GOFL-5炸药的屈服强度、硬化模量、初始微裂纹密度和微裂纹尺寸均小于PBX04炸药。
(2) 加载初期压装药头部微裂纹损伤高于浇注药,而浇注药的流动性较好,侵彻过程中产生了较大的变形,当装药尾部和壳体内表面发生撞击时形成高压区,整个侵彻过程中两类炸药微裂纹损伤较严重的区域均为装药头部和尾部,装药损伤较严重的区域往往容易引起能量聚集,进而在这些局部高温区形成热点,在侵彻弹体设计时应作为重点防护区域。
(3) 通过计算可知,800 m/s速度侵彻混凝土薄板条件下,两种装药材料均未发生点火,裂纹摩擦热点为压装药主导的温升机制,而对于浇注药而言,两种热点机制引起的温升差别不大,且浇注药GOFL-5在侵彻过程中的温升较压装药PBX04更低。
-
表 1 27组试验设计样本点及其响应值
Table 1. Design sample points and their response values of 27 group experimental tests
No. a/mm b/mm \overline m \overline e 1 0 11.61 0.52 0.49 2 0.97 5.81 0.77 0.14 3 1.94 26.13 1.13 0.96 4 2.90 16.45 0.97 1.15 5 3.87 0.97 0.82 0.34 6 5.81 10.65 0.93 1.07 7 7.74 4.84 0.88 0.65 8 9.68 14.52 1.07 0.76 9 11.61 8.71 1.01 0.88 10 13.55 2.90 0.96 0.71 11 15.48 12.58 1.15 0.50 12 17.42 6.77 1.09 0.86 13 19.35 0 1.07 0.89 14 23.23 3.87 1.10 0.40 15 28.06 1.94 1.25 0.42 16 5.25 2.73 0.79 0.54 17 8.18 16.36 1.07 1.17 18 13.64 8.18 1.04 0.84 19 8.57 4.29 0.89 0.61 20 17.14 8.57 1.12 0.60 21 3.33 3.33 0.77 0.29 22 6.67 13.33 0.99 0.93 23 12.86 12.86 1.10 0.63 24 10.00 10.00 1.00 1.00 25 3.45 15.25 0.94 1.20 26 18.14 7.31 1.11 0.80 27 20.71 4.31 1.11 0.26 表 2 4组检验点的参数、响应及相对误差
Table 2. Parameters, responses and relative errors of four test points
No. a/mm b/mm {\overline m {_{\rm{RBF} } }} {\overline e {_{\rm{RBF} } }} {\overline m {_{\rm{FEA} } }} {\overline e {_{\rm{FEA} } }} \delta{_ {\overline m}}/% \delta {_{\overline e}}/% 1 4.78 19.04 1.0501 1.2601 1.0481 1.269 0 −0.19 0.71 2 8.57 6.46 0.9189 0.7180 0.9131 0.7292 −0.63 1.50 3 12.86 15.14 1.1264 0.6601 1.1262 0.6300 −0.39 −4.50 4 18.01 7.11 1.1084 0.8011 1.1141 0.8406 0.51 4.90 -
[1] YU X L, ZHOU J, LIANG H Y, et al. Mechanical metamaterials associated with stiffness, rigidity and compressibility: a brief review [J]. Progress in Materials Science, 2018, 94: 114–173. doi: 10.1016/j.pmatsci.2017.12.003 [2] LV W T, LI D, DONG L. Study on mechanical properties of a hierarchical octet-truss structure [J]. Composite Structures, 2020, 249(8): 112640. [3] WANG Z G, LI Z D, SHI C, et al. Mechanical performance of vertex-based hierarchical vs square thin-walled multi-cell structure [J]. Thin Walled Structures, 2019, 134: 102–110. doi: 10.1016/j.tws.2018.09.017 [4] WEN G L, CHEN G X, LONG K, et al. Stacked-origami mechanical metamaterial with tailored multistage stiffness [J]. Research Square, 2021, 212(11): 110203. [5] LU H, WANG X P, CHEN T N. Design and quasi-static responses of a hierarchical negative Poisson’s ratio structure with three plateau stages and three-step deformation [J]. Composite Structures, 2022, 291(2): 115591. [6] ZHANG Z W, TIAN R L, ZHANG X L, et al. A novel butterfly-shaped auxetic structure with negative Poisson’s ratio and enhanced stiffness [J]. Journal of Materials Science, 2021, 56(25): 1–18. [7] FU Y M, YU T B, WANG X. Study on a Chiral structure with tunable Poisson’s ratio [J]. Materials, 2021, 14(12): 3338. doi: 10.3390/ma14123338 [8] WANG H, LU Z X, YANG Z Y, et al. In-plane dynamic crushing behaviors of a novel auxetic honeycomb with two plateau stress regions [J]. International Journal of Mechanical Sciences, 2019, 151: 746–759. doi: 10.1016/j.ijmecsci.2018.12.009 [9] WANG H, HE Y, YU A, et al. Origami-inspired structures with programmable multi-step deformation [J]. Mechanics of Advanced Materials and Structures, 2022, 137(18): 2133197. [10] AN M R, WANG L, LIU H T, et al. In-plane crushing response of a novel bidirectional re-entrant honeycomb with two plateau stress regions [J]. Thin-Walled Structures, 2022, 170: 108530. [11] LIU H T, WANG L. Design 3D improved star-shaped honeycomb with different tip angles from 2D analytical star-shaped model [J]. Composite Structures, 2022, 283(5): 115154. [12] LI X, FAN R, FAN Z J, et al. Programmable mechanical metamaterials based on hierarchical rotating structures [J]. International Journal of Solids and Structures, 2021, 216(1): 145–155. [13] WEI L L, ZHAO X, YU Q, et al. A novel star auxetic honeycomb with enhanced in-plane crushing strength [J]. Thin-Walled Structures, 2020, 149(19): 106623. [14] HECTOR K W, RESTREPO D, BONILLA C T, et al. Mechanics of chiral honeycomb architectures with phase transformations [J]. Journal of Applied Mechanics, 2019, 86(11): 111014. doi: 10.1115/1.4044024 [15] MENG Z Q, LIU M C, ZHANG Y F, et al. Multi-step deformation mechanical metamaterials [J]. Journal of the Mechanics and Physics of Solids, 2020, 144: 104095. doi: 10.1016/j.jmps.2020.104095 [16] AHMAD Z, THAMBIRATNAM D P. Crushing response of foam-filled conical tubes under quasi-static axial loading [J]. Materials & Design, 2009, 30(7): 2393–2403. [17] 王中钢. 轻质蜂窝结构力学 [M] 北京: 科学出版社, 2019. [18] ABRAMOWICZ W, WIERZBICKI T. Axial crushing of multicorner sheet metal columns [J]. Journal of applied mechanics, 1989, 56(1): 113–120. [19] MICHAEL M. Choosing basis functions and shape parameters for radial basis function methods [J]. SIAM Undergraduate Research Online, 2011, 4(4): 190–209. -








 下载:
下载:


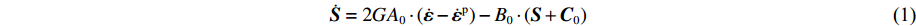
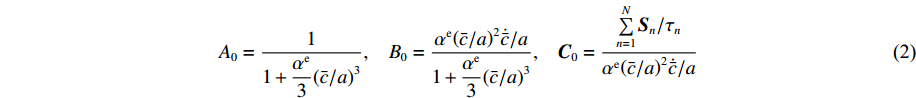
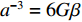
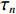

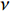
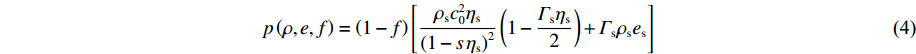
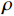
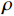
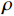
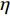
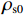
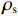
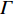
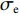
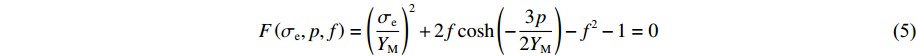
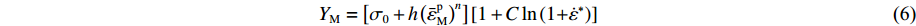

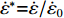
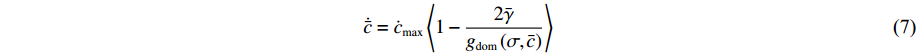
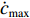
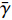
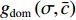
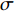
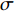
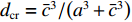
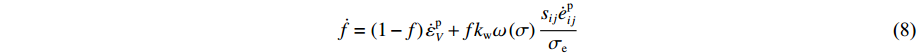
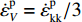
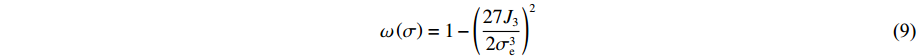
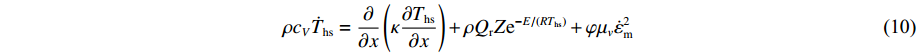

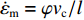
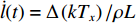
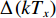
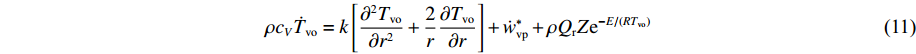
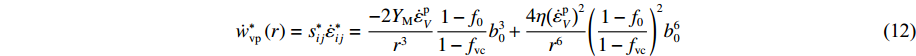
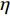
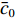
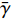
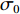
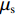


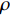
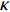
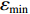









 下载:
下载: