四方相PbTeO<sub>3</sub>晶体在高压下的压缩行为

法志湘; 王文丹; 黎傲; 于少楠; 王李平

doi:10.11858/gywlxb.20220646

四方相PbTeO₃晶体在高压下的压缩行为

doi: 10.11858/gywlxb.20220646

法志湘^1,,
王文丹^1,*, ,,
黎傲¹,
于少楠¹,
王李平²

1.
西南交通大学物理科学与技术学院, 四川成都　610031
2.
南方科技大学前沿与交叉科学研究院, 广东深圳　518055

基金项目: 国家自然科学基金（51402245）；四川省中央引导地方科技发展专项（2021ZYD0027）；西南交通大学原创性科研仪器设备研制项目（XJ2021KJZK055，XJ2021KJZK052）

详细信息

作者简介:
法志湘（1998－），女，硕士研究生，主要从事高温高压下材料的物性研究. E-mail：afxxya@163.com

通讯作者:
王文丹（1981－），男，博士，副教授，主要从事高压下新材料的合成及物性测试研究. E-mail：wendan.wang@swjtu.edu.cn

中图分类号: O521.2
计量
- 文章访问数: 373
- HTML全文浏览量: 224
- PDF下载量: 45
出版历程
- 收稿日期: 2022-08-29
- 修回日期: 2022-09-19
- 网络出版日期: 2023-02-24
- 刊出日期: 2023-02-05

Compression Behavior of Tetragonal PbTeO₃ Crystals under High Pressure

FA Zhixiang^1
,,
WANG Wendan^{1,*
, ,},
LI Ao¹,
YU Shaonan¹,
WANG Liping²

1.
School of Physical Science and Technology, Southwest Jiaotong University, Chengdu 610031, Sichuan, China
2.
Academy for Advanced Interdisciplinary Studies, South University of Science and Technology, Shenzhen 518055, Guangdong, China

摘要

摘要: 利用水热法在230 ℃以及水的饱和蒸汽压条件下合成出毫米尺寸的四方相PbTeO₃单晶样品，并研究了其晶体结构、微观形貌、热稳定性等性质。利用金刚石对顶砧和同步辐射原位X射线衍射技术，探讨了该四方相PbTeO₃晶体在高压下的压缩行为，发现在0～37 GPa的压力范围内四方相PbTeO₃无相变发生。拟合该PbTeO₃样品的压力-体积数据，得到其体弹模量为B₀=42(1) GPa，体弹模量的一阶导数 $B_0'$ =5.5(0.2)。晶格参数随压力的变化显示，该晶体在c轴方向更易压缩。
- 四方相PbTeO₃ /
- 晶体结构 /
- 状态方程 /
- 体弹模量
Abstract: The millimeter-size tetragonal PbTeO₃ single crystal was synthesized by hydrothermal method under saturated vapor pressure of water at 230 °C. Crystal structure, microscopic morphology, thermal stability and other properties of the sample were studied. The compression behavior of the tetragonal PbTeO₃ crystal under high pressure was investigated by diamond anvil cell (DAC) with in-situ synchrotron X-ray diffraction. The results show there is no phase transition observed in the tetragonal PbTeO₃ up to 37 GPa. Using the Birch-Murnaghan equation of state to fit the observed pressure-volume data of the tetragonal PbTeO₃ sample, a bulk modulus B₀=42(1) GPa, $B_0'$ =5.5(0.2) for tetragonal phase was obtained. The variation of lattice parameters with pressure shows that the crystal is easier to compress in the c-axis direction.
- tetragonal PbTeO₃ /
- crystal stracture /
- equation of state /
- bulk modulus

HTML全文

光滑粒子流体动力学(Smoothed Particle Hydrodynamics, SPH)方法中的搜索算法较耗时，即每个时间步都要对领域粒子进行搜索，粒子越多，耗时情况越突出，与有限元法相比，SPH方法的计算效率要低得多。为了解决SPH方法计算效率低的问题，Johnson等^[1-2]和Attaway等^[3]将有限元与SPH方法相结合，提出了SPH-FEM耦合的算法，即:在小变形区域使用有限元法，大变形区域仍使用SPH方法。该方法不仅提高了计算效率，而且适应性较强。

目前，采用SPH方法对爆炸焊接进行数值模拟的相关报道较少，而且多采用二维SPH方法。Tanaka^[4]采用SPH方法对爆炸焊接的斜碰撞过程进行了数值模拟，成功地模拟出射流、波形和涡旋，波长的模拟结果相对实验结果偏大。李晓杰等^[5]采用SPH方法及热塑性流体力学模型对爆炸复合板的斜碰撞过程中出现的界面波进行了数值模拟，模拟结果与张登霞等^[6-7]实验结果的一致性较好。刘江等^[8]利用AUTODYN软件中的SPH方法模拟了爆炸复合的斜碰撞，结合模拟中有效塑性变形、温度及剪切应力呈现的变化规律发现，爆炸复合的结合机理集塑性变形、熔化和扩散为一体。本研究将采用三维SPH方法对双面爆炸焊接过程进行模拟，将其结果与实验及理论结果进行对比，分析SPH-FEM耦合方法对爆炸焊接模拟的有效性。

1. 计算模型及参数选取

1.1 计算模型

以前期45钢/Q235钢双面爆炸焊接实验^[9]为基础，考虑到计算效率，利用LS-DYNA建立如图 1及图 2所示的两组双面爆炸焊接SPH-FEM耦合的三维真实计算模型，选用的炸药为乳化炸药(玻璃微球的质量分数为5%)，计算模型中基板和复板的材料、尺寸、间隙(δ)及药厚如表 1所示。起爆方式为点起爆。

图 1 计算模型Ⅰ(10 mm药厚)

Figure 1. Calculation model Ⅰ with explosive thickness of 10 mm

下载: 全尺寸图片幻灯片

图 2 计算模型Ⅱ(5 mm药厚)

Figure 2. Calculation model Ⅱ with explosive thickness of 5 mm

下载: 全尺寸图片幻灯片

表 1 计算模型中材料的相关参数

Table 1. Related parameters of materials in calculation models

Calculationmodel	Flyer plate		Base plate		Gap δ/mm	Size of explosive/(mm×mm×mm)
Calculationmodel	Material	Size/(mm×mm×mm)	Material	Size/(mm×mm×mm)	Gap δ/mm	Size of explosive/(mm×mm×mm)
Ⅰ	45 steel	300×150×2	Q235	300×150×16	6	300×150×10
Ⅱ	45 steel	300×150×2	Q235	300×150×16	6	300×150×5

下载: 导出CSV

| 显示表格

基、复板采用3D Solid 164实体单元，单元边长为0.1 cm；炸药划分为光滑粒子，粒子的大小Δr取为0.1 cm。考虑到模型的对称性，为了提高计算效率，采用1/2模型进行计算。单位制为cm-g-μs。

1.2 材料模型及参数设定

数值计算中乳化炸药采用高能燃烧模型^[10-11]及JWL状态方程^[12]。JWL状态方程表达式为

$p={{A}_{\text{JWL}}}\left( 1-\frac{\omega }{{{R}_{1}}v} \right){{\text{e}}^{-{{R}_{1}}v}}+{{B}_{\text{JWL}}}\left( 1-\frac{\omega }{{{R}_{2}}v} \right){{\text{e}}^{-{{R}_{2}}v}}+\frac{\omega {{E}_{0}}}{v}$

(1)

式中：A_JWL、B_JWL、R₁、R₂和ω为材料参数；p为爆轰产物压力，GPa；E₀为初始比内能，kJ/cm³；v为爆轰气体产物的相对比容，为无量纲量。炸药的相关参数见表 2，其中：ρ为密度，D为炸药爆速。

表 2 乳化炸药的JWL状态参数^[13]

Table 2. JWL equation-of-state parameters of emulsion explosives^[13]

ρ/(g·cm^-3)	D/(m·s^-1)	A_JWL/GPa	B_JWL/GPa	R₁	R₂	ω	E₀/(kJ·cm^-3)
1.12	4 510	326.42	5.808 9	5.80	1.56	0.57	3.323

下载: 导出CSV

| 显示表格

数值计算中，基、复板均采用Mie-Grüneisen状态方程^[14]和Johnson-Cook材料模型^[15]。Johnson-Cook材料模型的形式如下

$\sigma =\left( A+B\varepsilon _{\text{p}}^{n} \right)\left( 1+C\ln \dot{\varepsilon }_{\text{p}}^{*} \right)\left( 1-{{T}^{*m}} \right)$

(2)

式中：ε_p为有效塑性应变； $\dot{\varepsilon }_{\text{p}}^{*}={{\dot{\varepsilon }}_{\text{p}}}/\dot{\varepsilon }_{\text{p}}^{0}$ 为有效塑性应变率，其中 $\dot{\varepsilon }_{\text{p}}^{0}$ 为参考应变率；A、B、C、m及n为与材料相关的常数；无量纲温度T^*表示为T^*=(T-T_r)/(T_m-T_r)，其中T_r为室温, T_m为熔点。45钢选用与Q235钢相同的Johnson-Cook材料模型参数，具体参数如表 3所示。

表 3 Q235钢的Johnson-Cook模型参数^[16]

Table 3. Johnson-Cook parameters of Q235 steel^[16]

ρ/(g·cm^-3)	G/GPa	A/GPa	B/GPa	C	n	m	T_m/K	T_r/K
7.83	77	0.792	0.51	0.014	0.26	1.03	1 793	294

下载: 导出CSV

| 显示表格

2. 模拟结果与分析

2.1 10 mm药厚的模拟结果

2.1.1 碰撞点位移

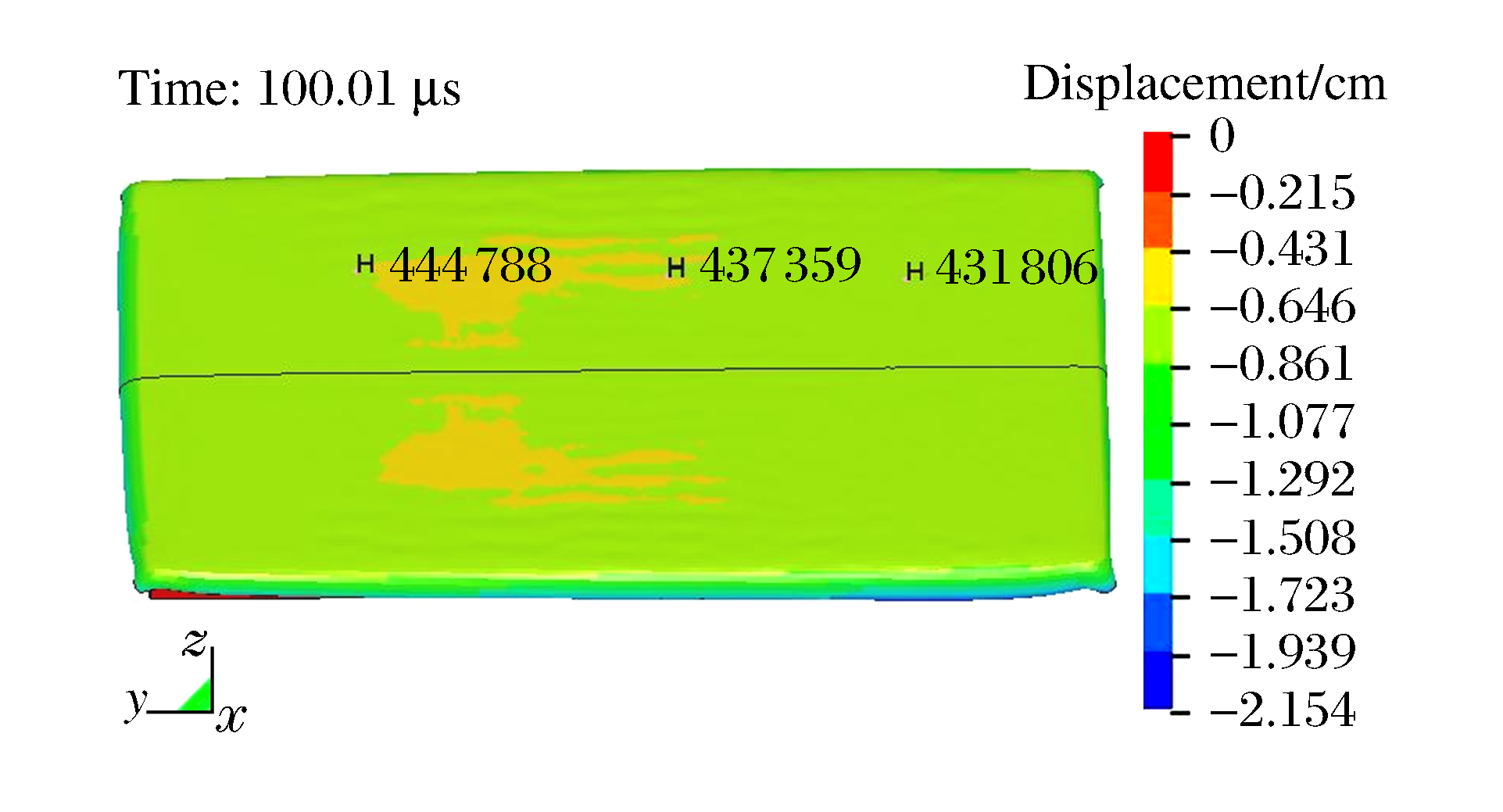
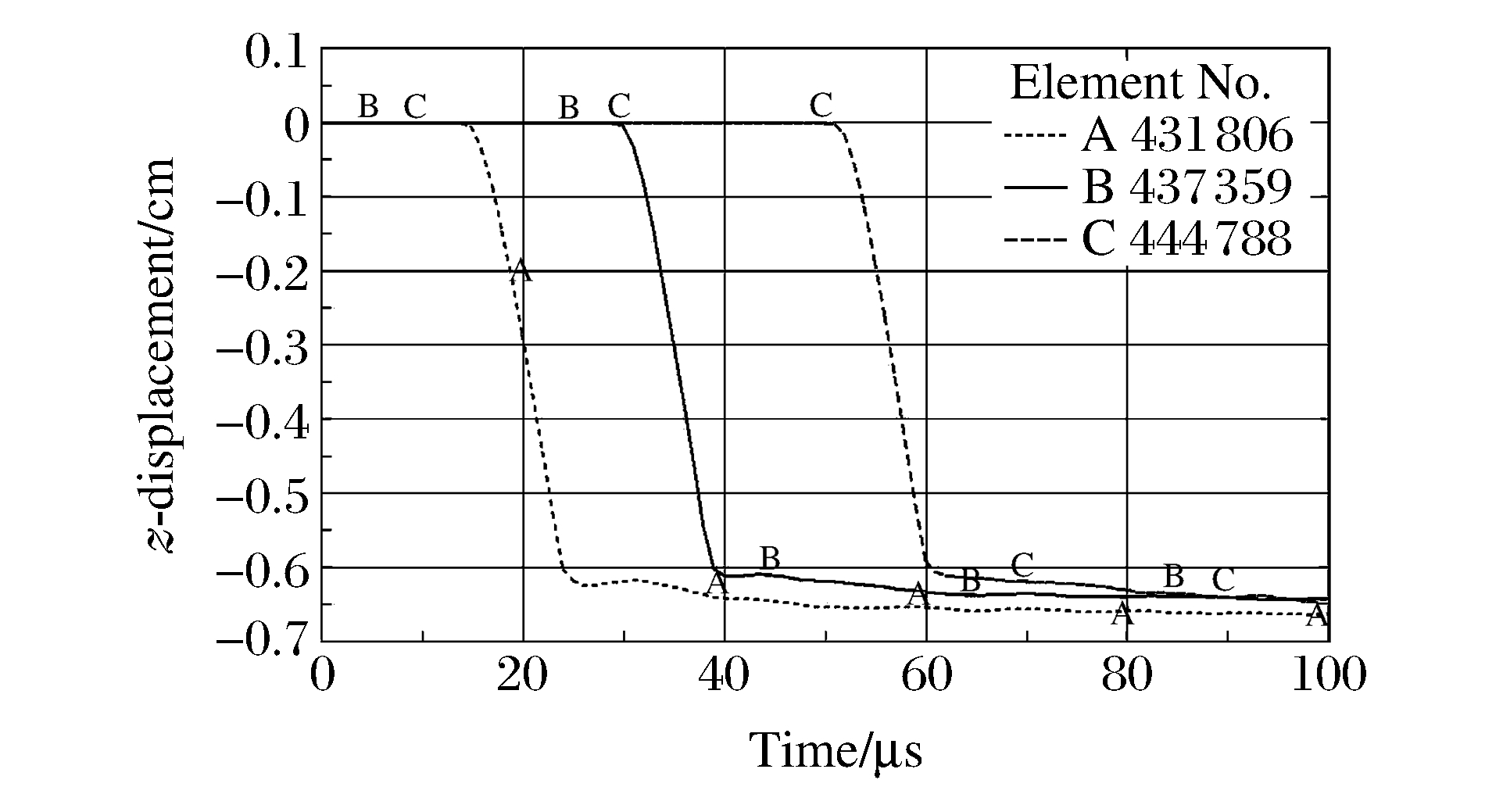
图 3所示是爆炸焊接结束时复板的竖向位移云图。由图 3可看出，复板的位移大致均为6 mm，表明基、复板已完全复合。为了更直观地观察复板单元位移的变化情况，在复板上选择3个特征单元(431 806、437 359、444 788)，输出其位移-时间曲线，如图 4所示。由图 4可看出，特征单元的竖向位移均略大于间隙(6 mm)，这是由于在爆炸载荷作用下复板有一定程度的减薄率所致。

图 3 10 mm药厚下爆炸焊接结束时复板的z向位移云图

Figure 3. z-direction displacement contour of flyer plate with explosive thickness of 10 mm at the end of explosive welding

下载: 全尺寸图片幻灯片

图 4 10 mm药厚下复板上3个特征单元的z向位移-时间历程

Figure 4. z-direction displacement histories of 3 characteristic elements with explosive thickness of 10 mm

下载: 全尺寸图片幻灯片

2.1.2 复板碰撞速度

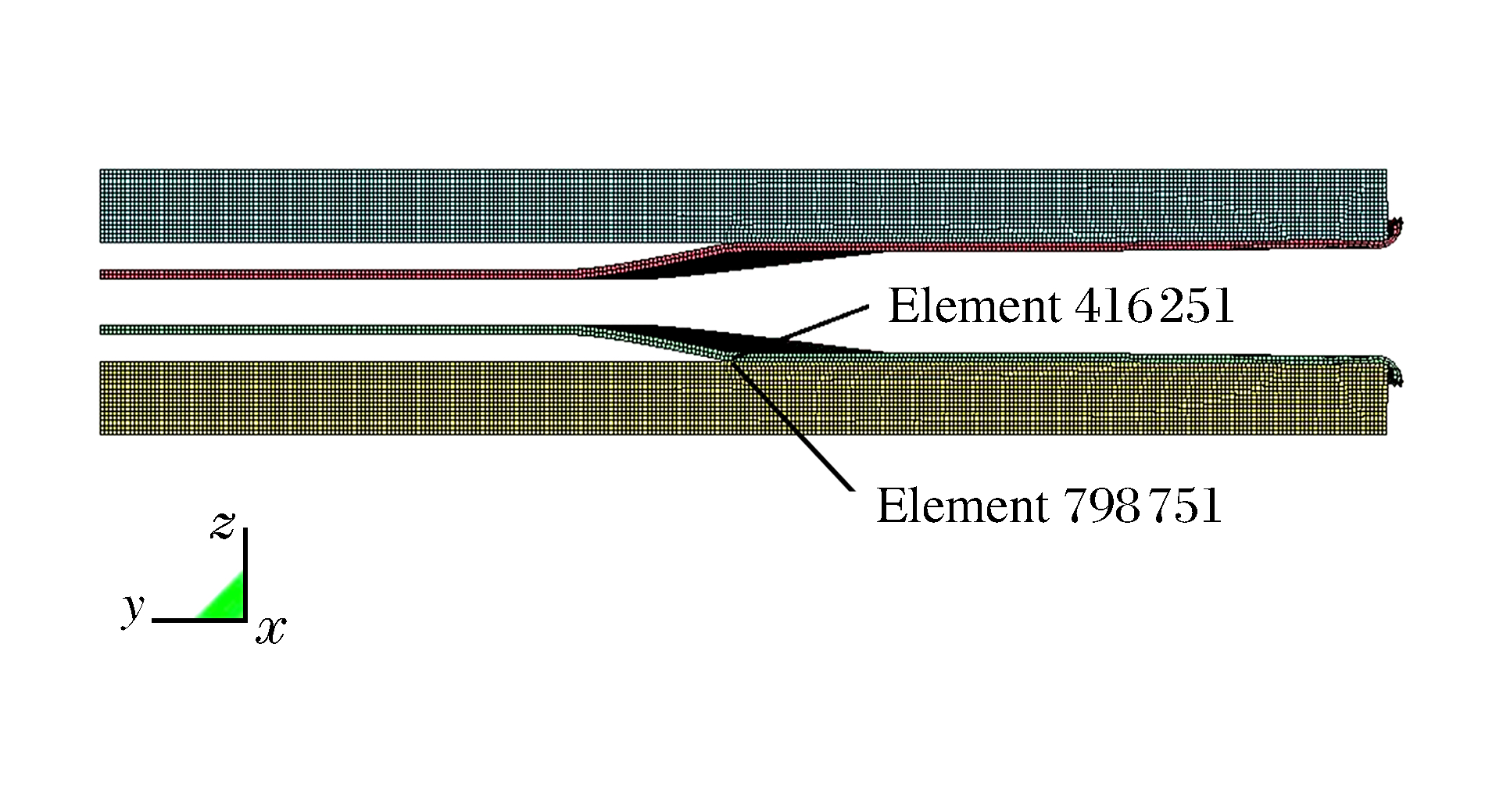
图 5所示是一对分别取自基板与复板结合界面处的特征单元(基板单元：798 751；复板单元：416 251)，特征单元的选取与前期实验^[9]中金相试样的取样位置一致。

图 5 10 mm药厚下的一对特征单元

Figure 5. A pair of characteristic elements with explosive thickness of 10 mm

下载: 全尺寸图片幻灯片

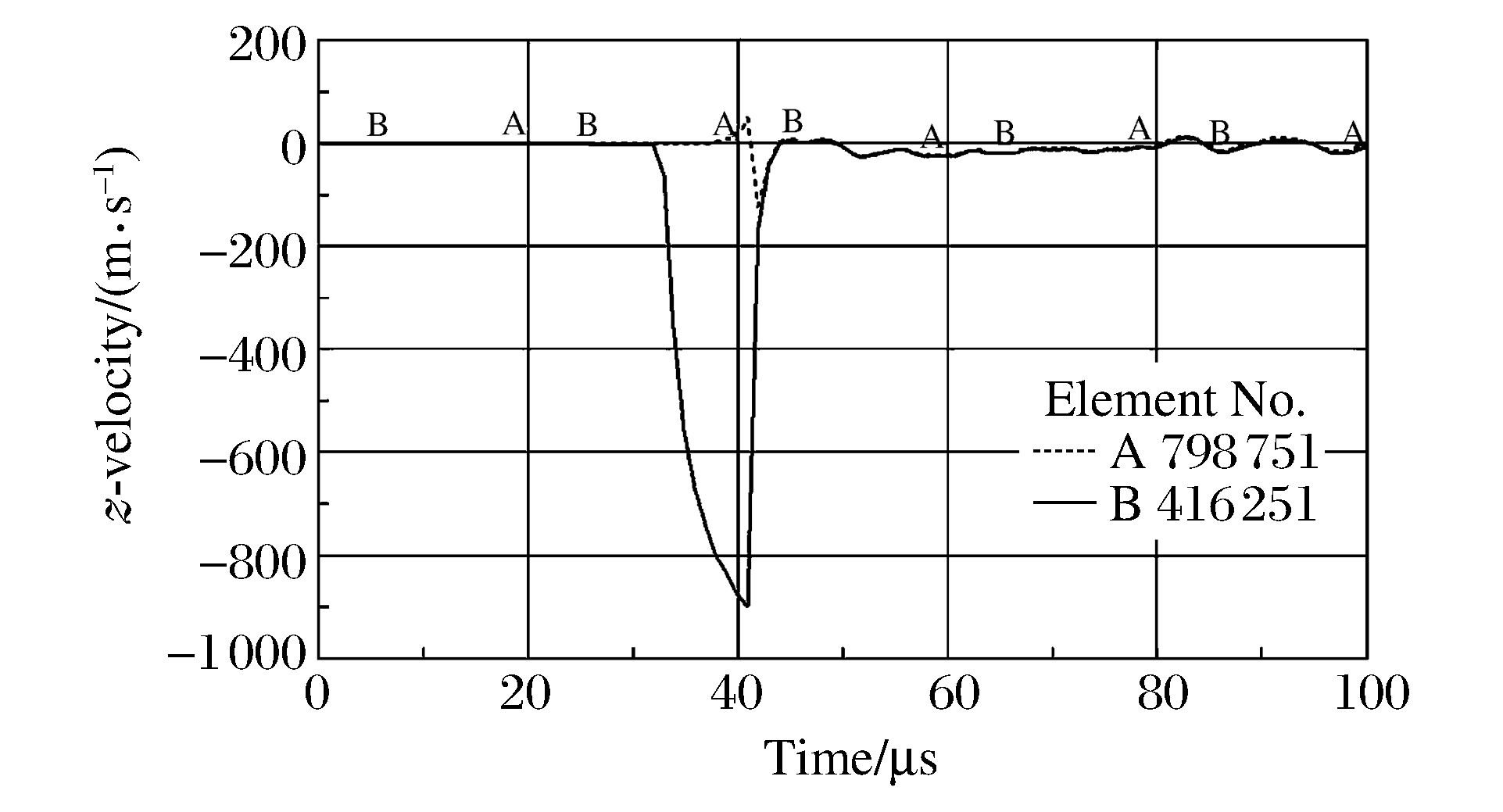
图 6所示是这对特征单元的速度-时间曲线。可以看出，基板在碰撞前有一个正的速度峰；该现象的产生如文献[17]所述，是由于爆轰产物不断堆积以及前碰撞点在待复合区产生的振动能所致。复板上所取单元的最大碰撞速度为897 m/s。

图 6 一对特征单元(见图 5)的速度-时间曲线

Figure 6. Velocity-time curves of the pair of characteristic elements (see Fig. 5)

下载: 全尺寸图片幻灯片

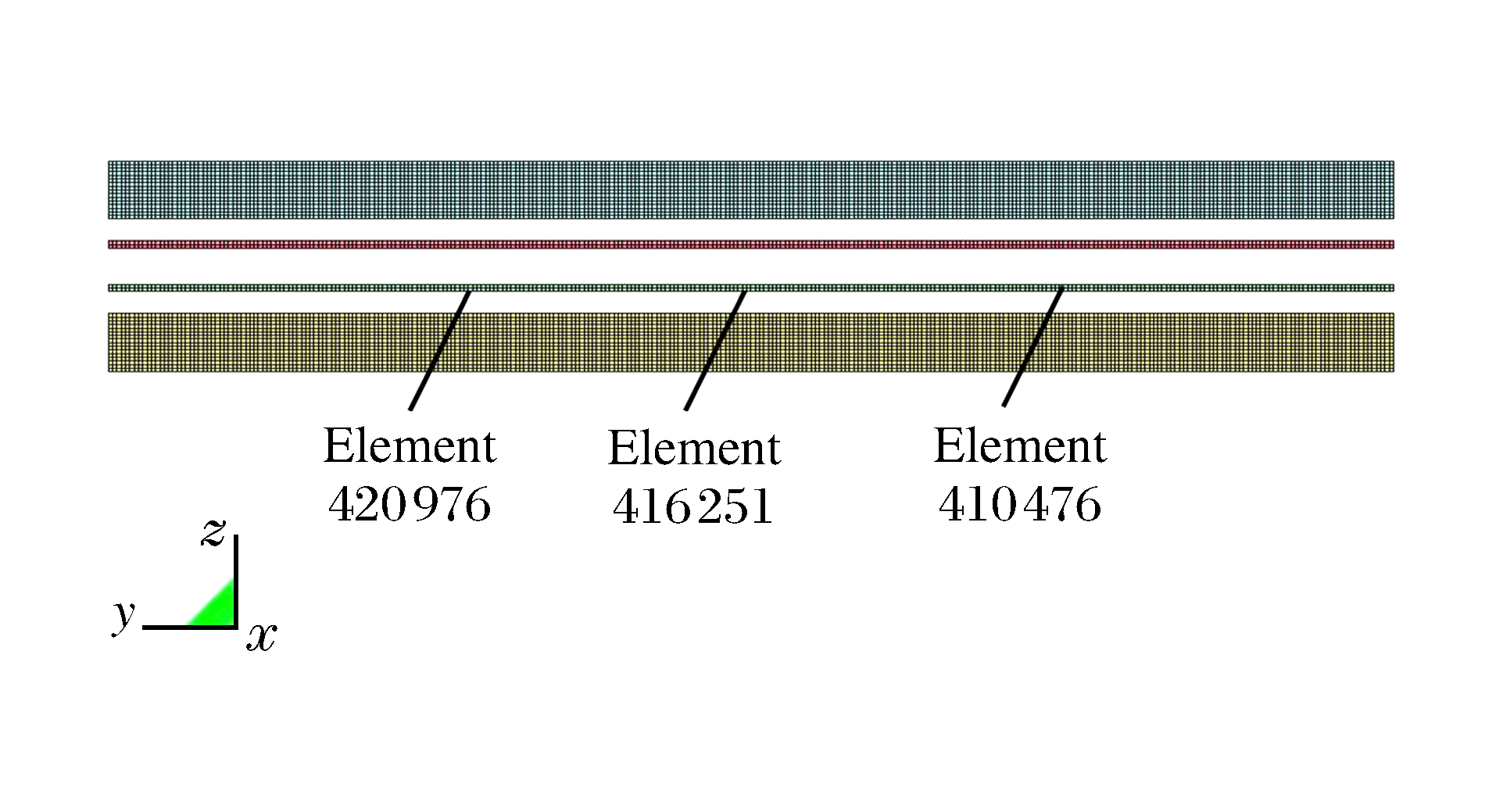
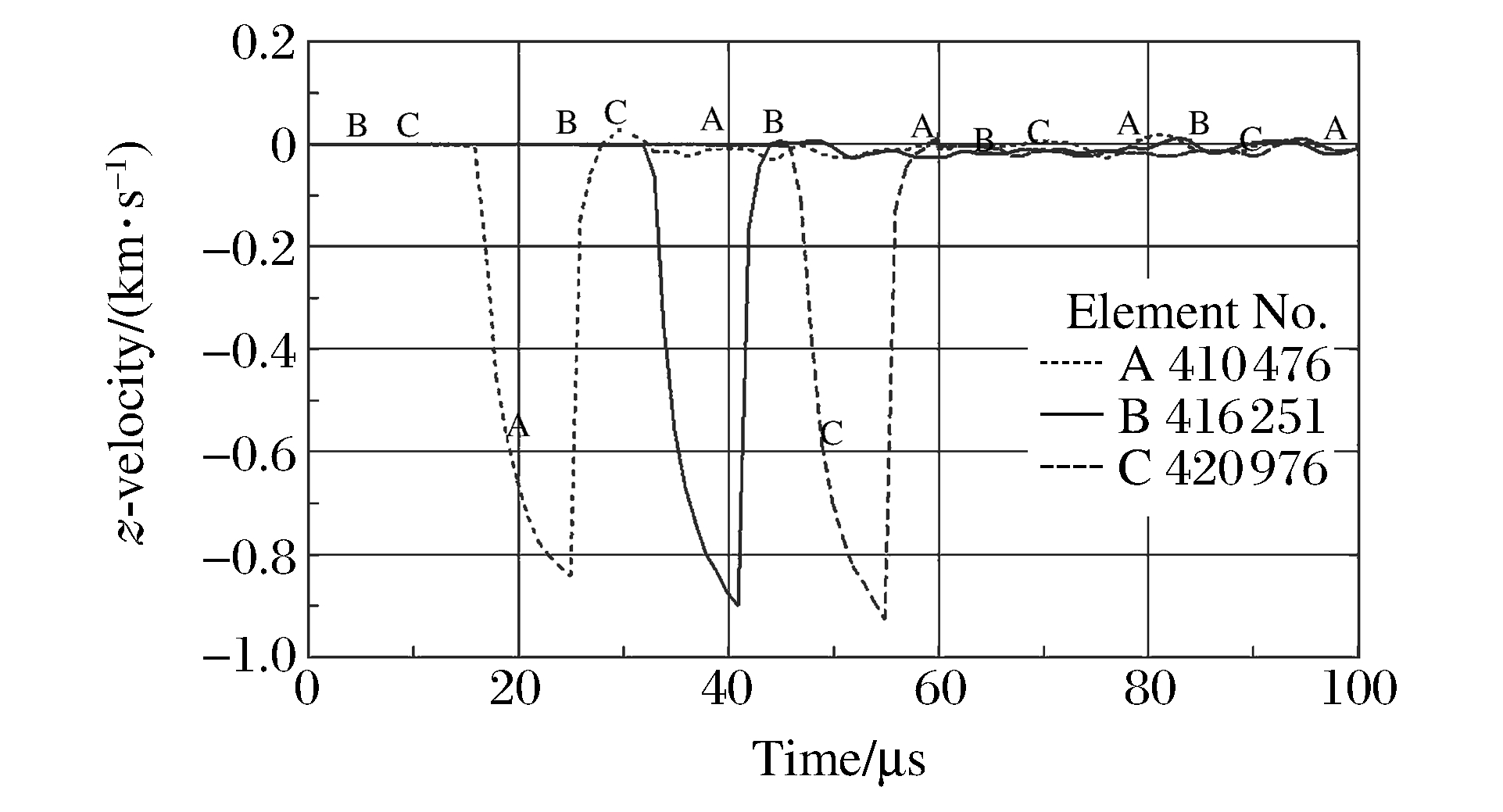
图 7所示是在复板结合界面处所选取的3个特征单元(410 476、416 251、420 976)。图 8所示是这3个特征单元的速度-时间曲线。

图 7 10 mm药厚下复板结合界面处的3个特征单元

Figure 7. 3 characteristic elements at the bonding interface of flyer plate with explosive thickness of 10 mm

下载: 全尺寸图片幻灯片

图 8 10 mm药厚下3个特征单元的速度-时间曲线

Figure 8. Velocity-time curves of 3 characteristic elements with explosive thickness of 10 mm

下载: 全尺寸图片幻灯片

由图 8可以看出，随着距起爆端距离的增加，复板的碰撞速度增大。由文献[17]的结论可知，该现象是由于基板与复板的碰撞在金属板的待复合区产生了强烈振动引起的。

2.1.3 碰撞点压力分布

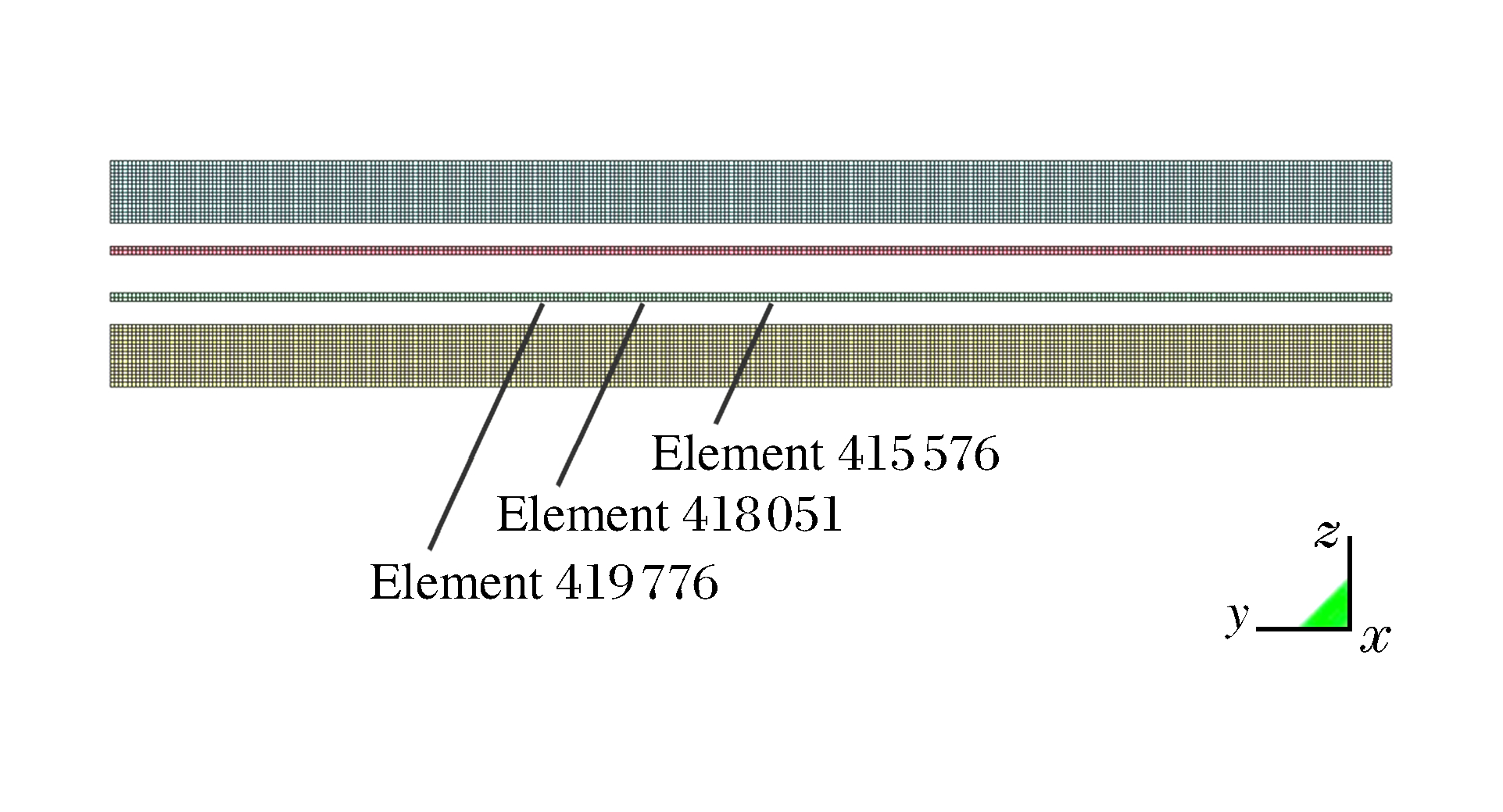
图 9所示是在结合界面处选取的3个特征单元(415 576、418 051、419 776)，单元415 576取在复板中心处，与前期实验^[7]中取样做金相观察的位置一致。图 10所示是3个特征单元的压力历程。

图 9 10 mm药厚下复板结合界面处的3个特征单元

Figure 9. 3 characteristic elements at the bonding interface of flyer plate with explosive thickness of 10 mm

下载: 全尺寸图片幻灯片

图 10 10 mm药厚下3个特征单元的压力-时间曲线

Figure 10. Pressure-time curves of 3 characteristic elements with explosive thickness of 10 mm

下载: 全尺寸图片幻灯片

由图 10可以看出，随着距起爆端距离的增加，复板的碰撞压力增大。由文献[17]的结论可知，该现象是爆轰产物不断堆积以及前碰撞点在金属板待复合区振动能不断增加的共同作用结果。

2.2 5 mm药厚的模拟结果

2.2.1 碰撞点位移

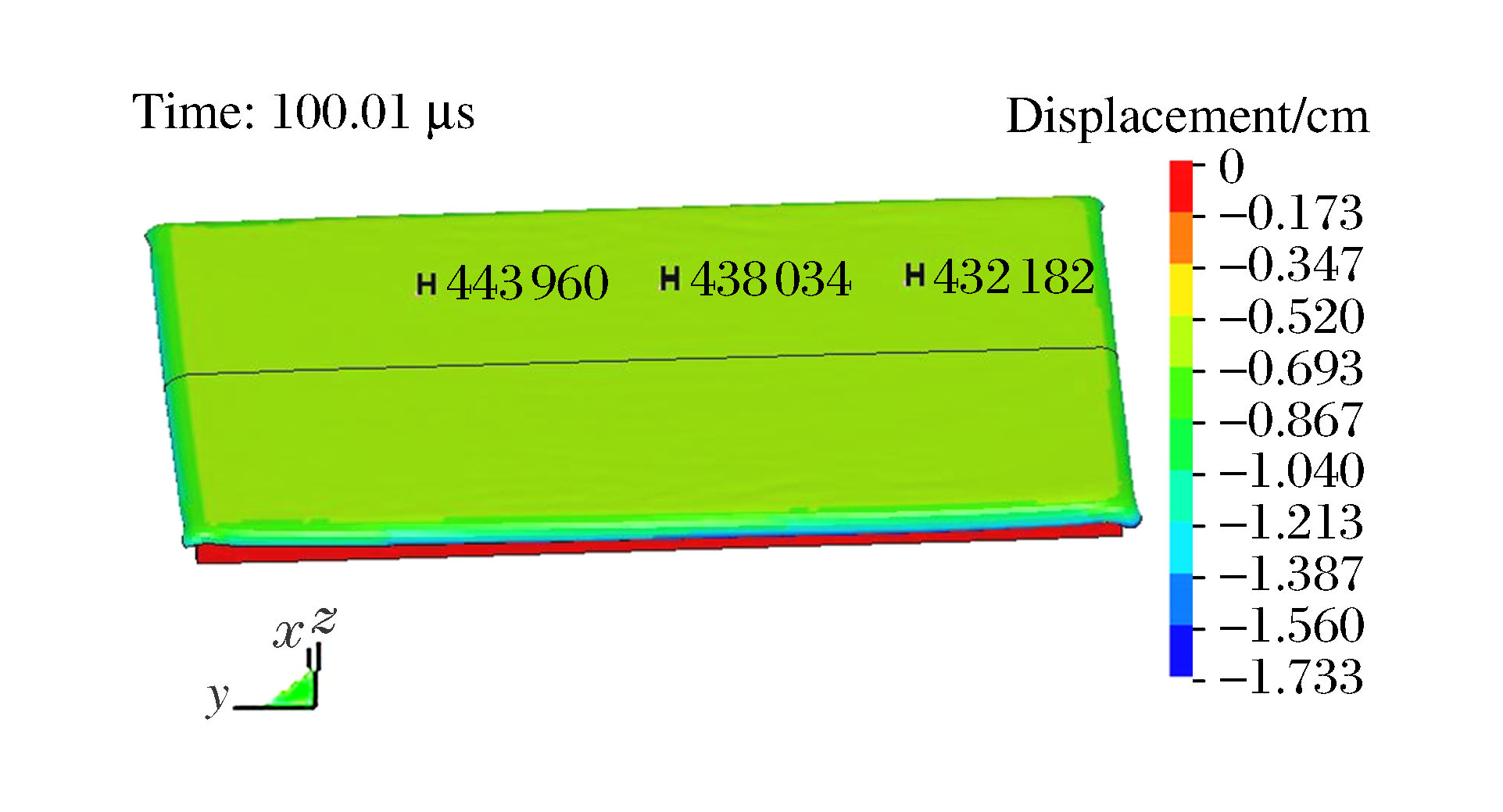
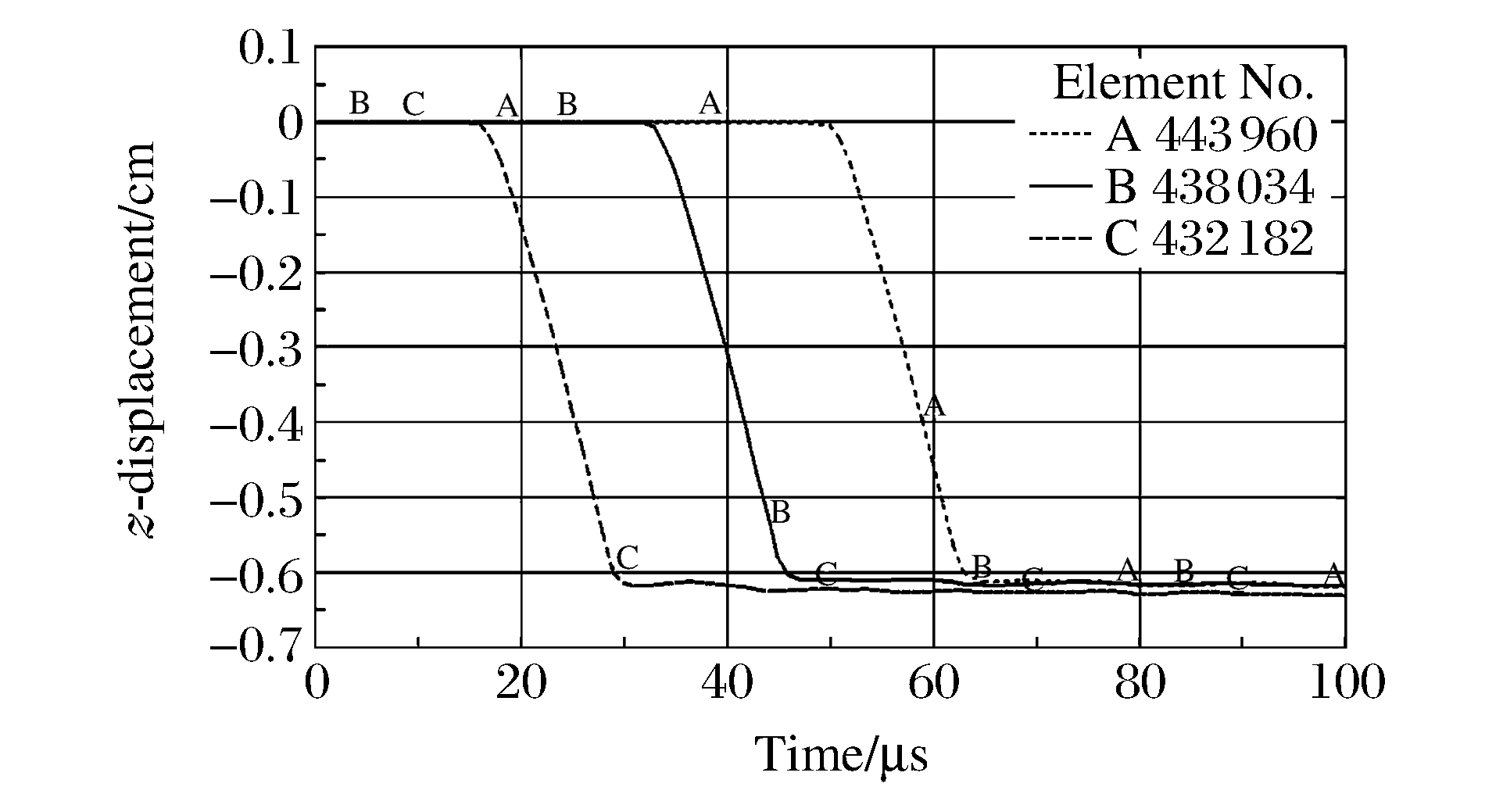
图 11所示是爆炸焊接结束时复板的竖向位移云图。由图 11可看出，复板的位移大致均为6 mm，表明基、复板已完全复合。为了更加直观地观察复板单元位移的变化情况，在复板上选择3个特征单元(432 182、438 034、443 960)，输出其位移-时间曲线，如图 12所示。由图 12可看出，特征单元的竖向位移均略大于6 mm，但较10 mm药厚下的竖向位移小。这是由于5 mm药厚下的爆炸载荷作用比10 mm药厚下小，导致5 mm药厚下的复板减薄率比10 mm药厚下低。

图 11 5 mm药厚下爆炸复合结束时复板的z向位移云图

Figure 11. z-displacement contour of flyer plate with explosive thickness of 5 mm at the end of explosive welding

下载: 全尺寸图片幻灯片

图 12 5 mm药厚下复板上特征单元的z向位移-时间历程

Figure 12. z-displacement histories of 3 characteristic elements with explosive thickness of 5 mm

下载: 全尺寸图片幻灯片

2.2.2 复板碰撞速度

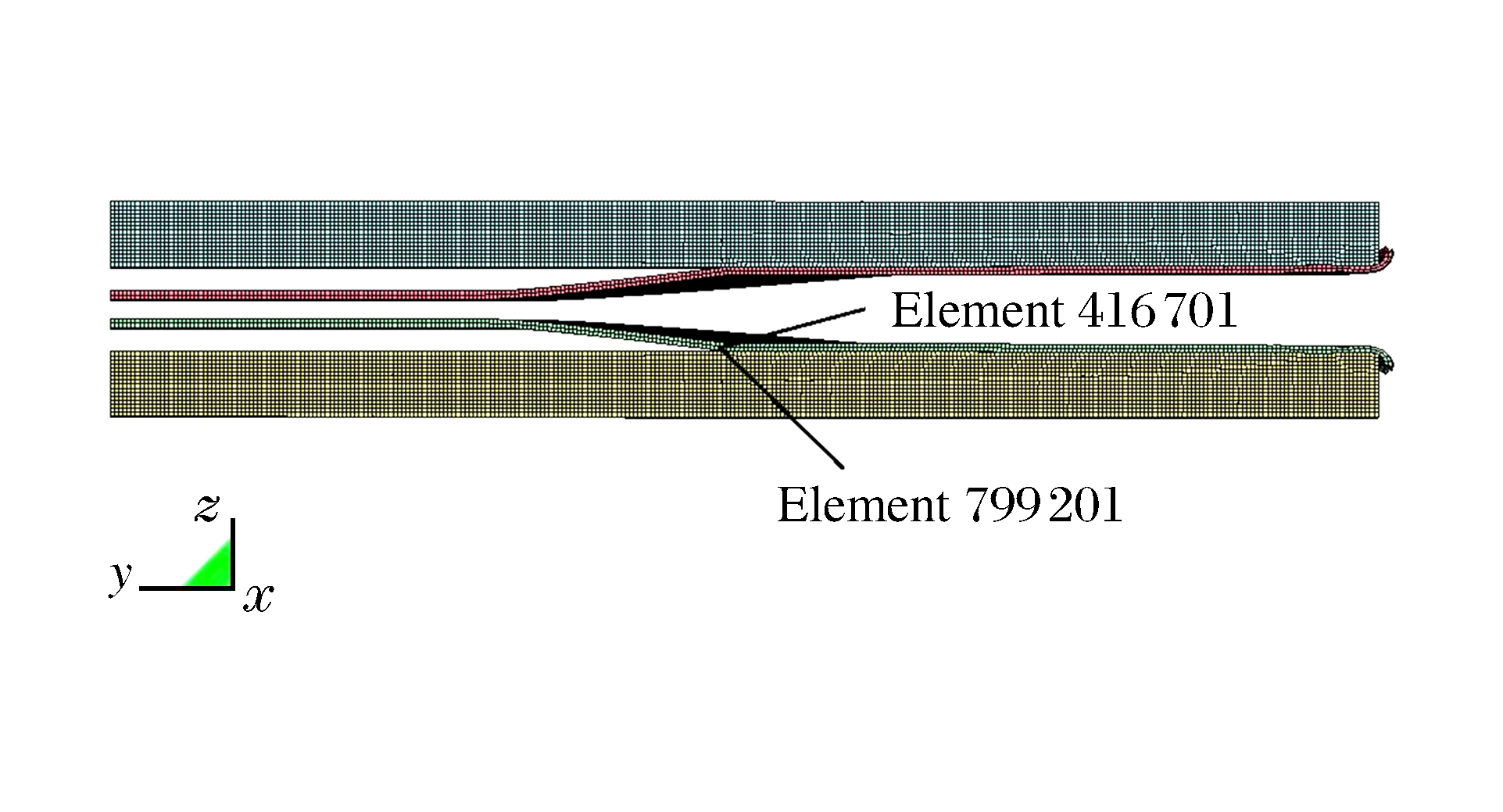
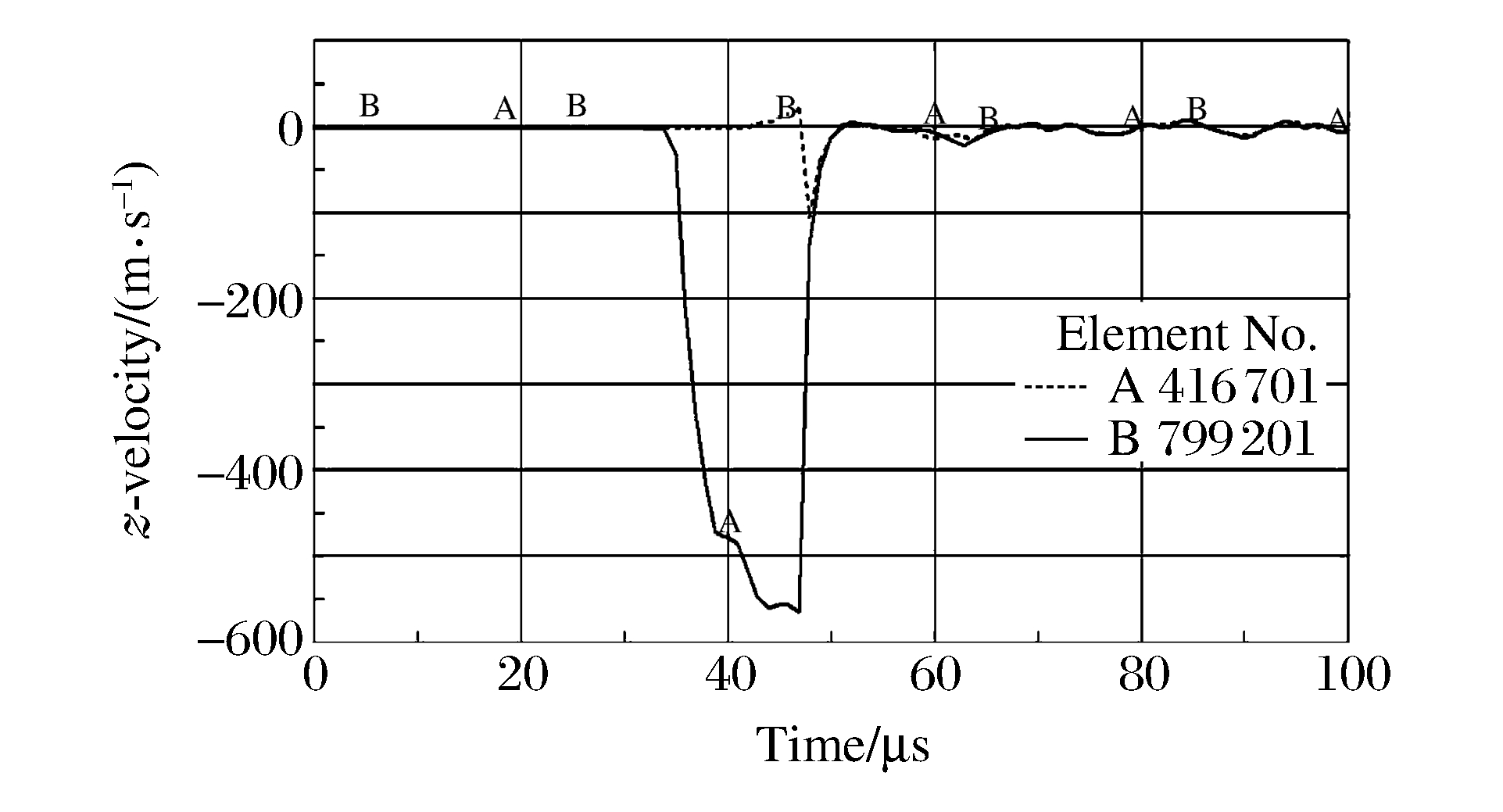
图 13所示是一对分别取自基板与复板结合界面处的特征单元(基板单元：799 201；复板单元：416 701)，特征单元的选取与前期实验^[9]中金相试样的取样位置一致。

图 13 5 mm药厚下的一对特征单元

Figure 13. A pair of characteristic elements with explosive thickness of 5 mm

下载: 全尺寸图片幻灯片

图 14所示是这对特征单元的速度-时间曲线, 可以看出，基板在碰撞前也有一个正的速度峰。复板上所取单元的最大碰撞速度为565 m/s。

图 14 一对特征单元(见图 13)的速度-时间曲线

Figure 14. Velocity-time curves of the pair of characteristic elements (see Fig. 13)

下载: 全尺寸图片幻灯片

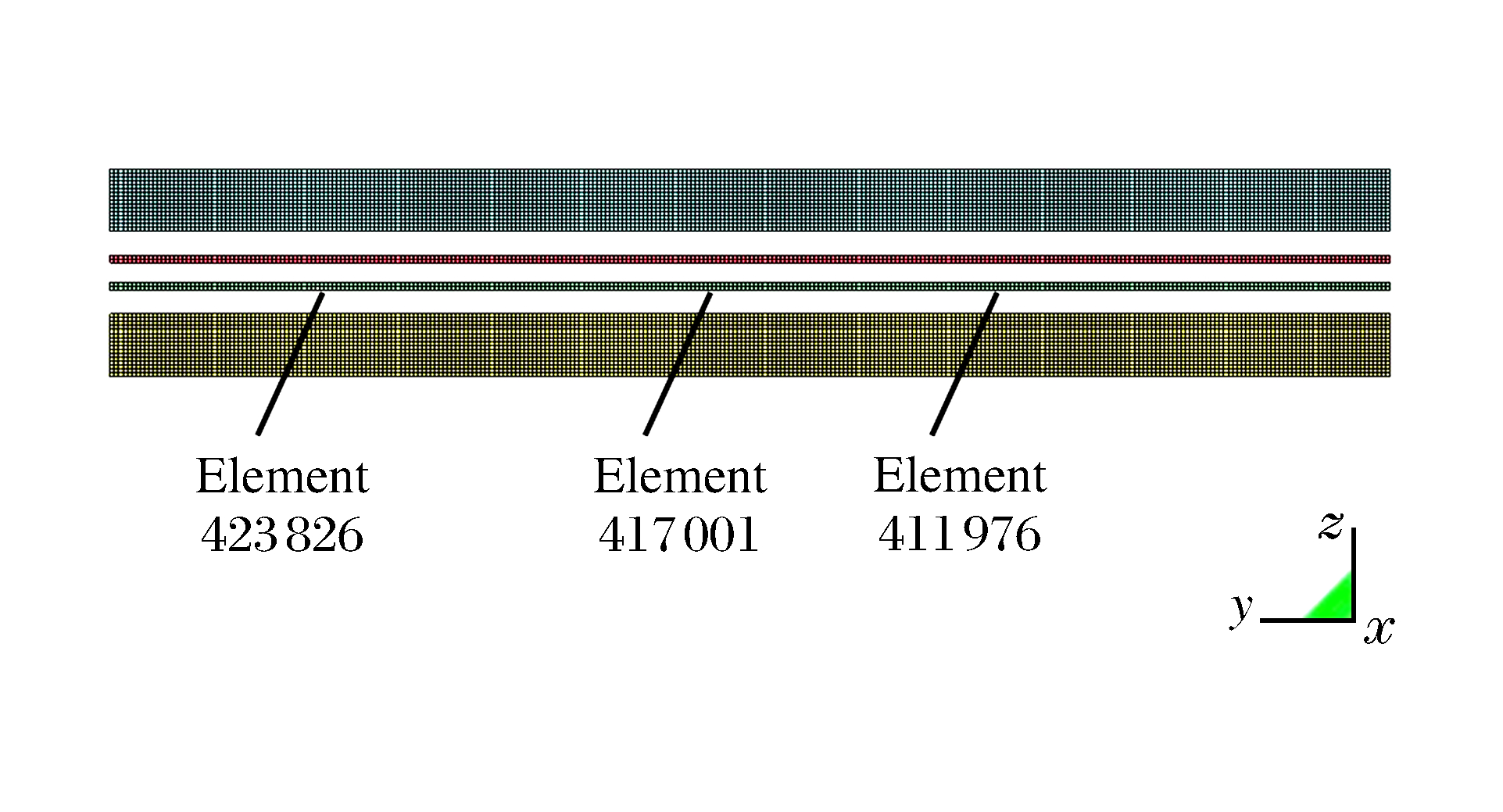
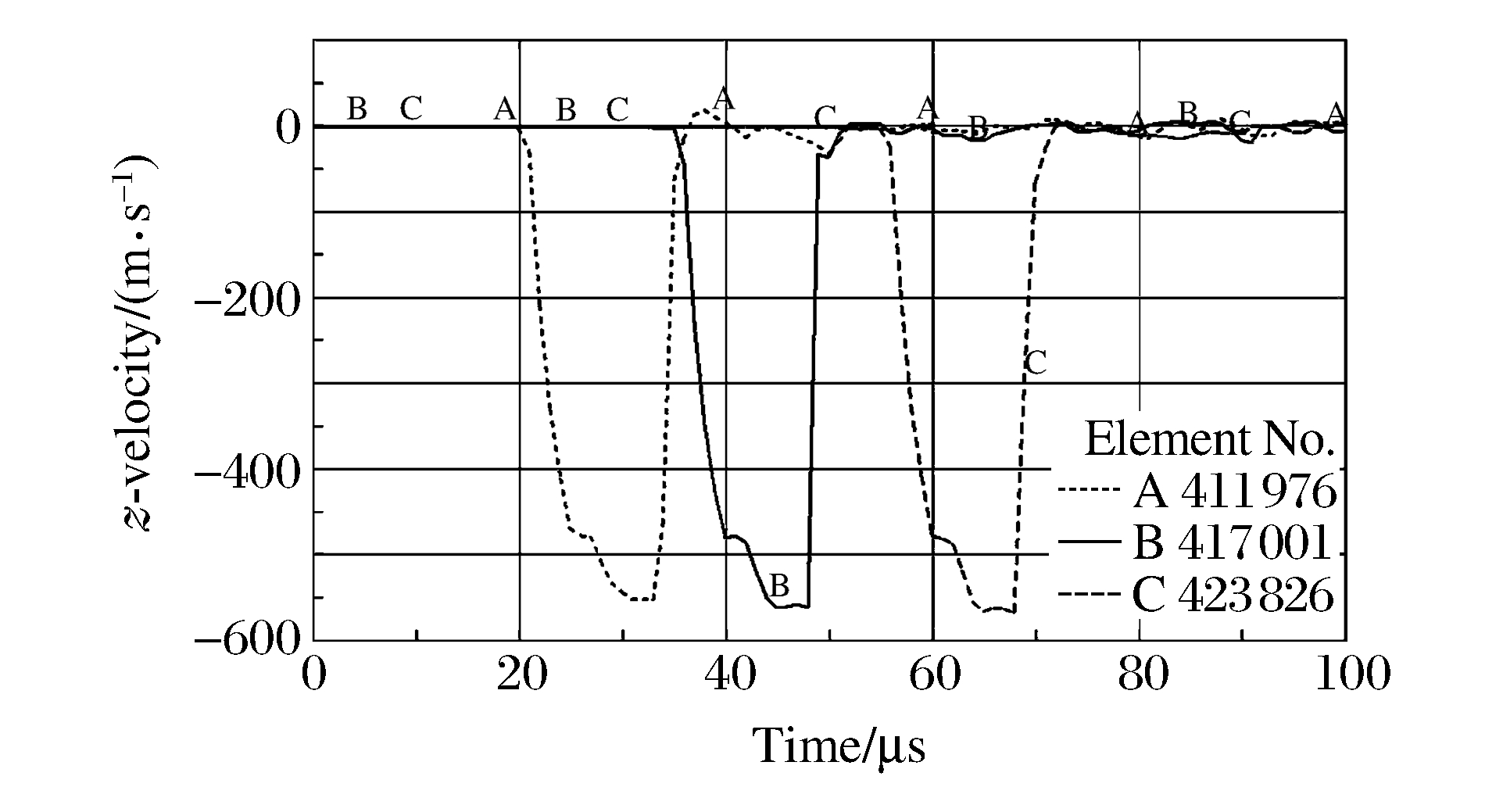
图 15所示是在复板结合界面处所选取的3个特征单元(411 976、417 001、423 826)。图 16所示是这3个特征单元的速度-时间曲线。由图 16可以看出，随着距起爆端距离的增加，复板的碰撞速度增大。

图 15 5 mm药厚下复板结合界面处的3个特征单元

Figure 15. 3 characteristic elements at the bonding interface of flyer plate with explosive thickness of 5 mm

下载: 全尺寸图片幻灯片

图 16 5 mm药厚下3个特征单元的速度-时间曲线

Figure 16. Velocity-time curves of 3 characteristic elements with explosive thickness of 5 mm

下载: 全尺寸图片幻灯片

2.2.3 碰撞点压力分布

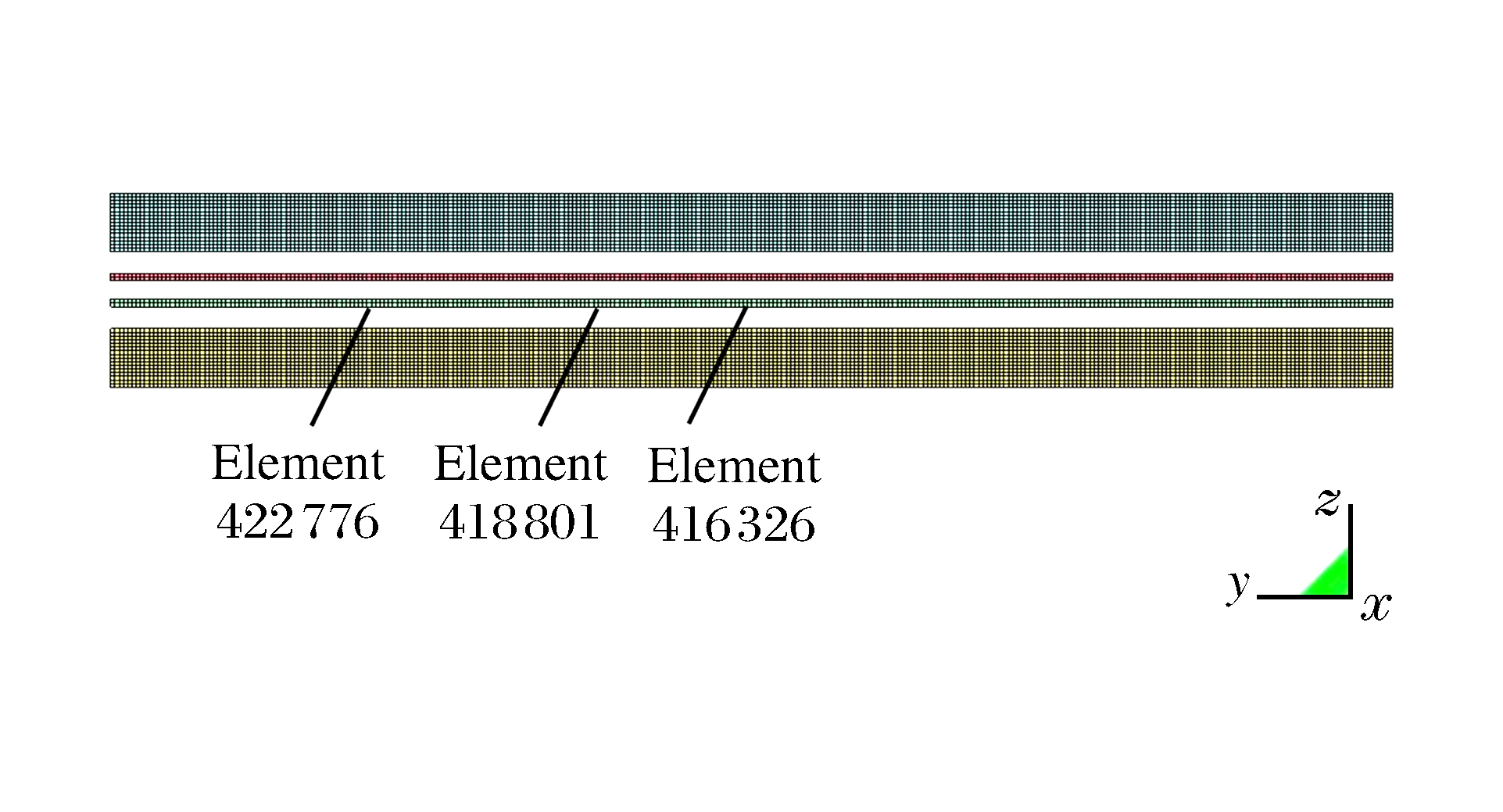
图 17所示是在结合界面处选取的3个特征单元(416 326、418 801、422 776)，单元416 326取在复板中心处，与前期实验^[7]中取样做金相观察的位置一致。图 18所示是这3个特征单元的压力历程。由图 18可以看出，随着距起爆端距离的增加，复板的碰撞压力增大。

图 17 5 mm药厚下复板结合界面处的3个特征单元

Figure 17. 3 characteristic elements at the bonding interface of flyer plate with explosive thickness of 5 mm

下载: 全尺寸图片幻灯片

图 18 5 mm药厚下3个特征单元的压力历程

Figure 18. Pressure histories of 3 characteristic elements with explosive thickness of 5 mm

下载: 全尺寸图片幻灯片

2.3 分析与讨论

由图 6可以看出，10 mm药厚下复板的最大碰撞速度为897 m/s。由图 14可以看出，5 mm药厚下复板的最大碰撞速度为565 m/s。利用前期工作^[18]中提到的3种理论公式(Gurney公式、Aziz公式、Deribas公式)计算了复板的碰撞速度，如表 4、表 5所示，并与数值模拟结果进行了比较。由表 4和表 5可以看出：Gurney公式和Aziz公式的计算结果均存在较大的偏差；而由Deribas公式计算的两组结果与数值模拟结果较接近，误差均未超过5%，且与前期实验结果较吻合，证明了SPH-FEM耦合算法的可靠性。

表 4 10 mm药厚下碰撞速度理论计算结果与数值模拟结果的比较

Table 4. Comparison of collision velocity between theoretical calculation and numerical simulation with explosive thickness of 10 mm

Theoreticalformula	Massfraction	Collision velocity/(m·s^-1)		Error/%
Theoreticalformula	Massfraction	Theoretical calculation^[18]	Simulation	Error/%
Gurney	0.75	1 089	897	-21.0
Aziz	0.75	711	897	20.0
Deribas	0.75	853	897	4.9

下载: 导出CSV

| 显示表格

表 5 5 mm药厚下碰撞速度理论计算结果与数值模拟结果的比较

Table 5. Comparison of collision velocity between theoretical calculation and numerical simulation with explosive thickness of 5 mm

Theoreticalformula	Massfraction	Collision velocity/(m·s^-1)		Error/%
Theoreticalformula	Massfraction	Theoretical calculation^[18]	Simulation	Error/%
Gurney	0.45	863	565	-52.7
Aziz	0.45	480	565	15.0
Deribas	0.45	576	565	-1.9

下载: 导出CSV

| 显示表格

由图 10可以看出，10 mm药厚下复板单元415 576处的碰撞压力为17.08 GPa。由图 18可以看出，5 mm药厚下复板单元416 326处的碰撞压力为11.25 GPa。

Ezra等提出的碰撞压力的计算公式为^[16]

$p=\frac{{{\rho }_{1}}{{v}_{\text{s}, 1}}{{v}_{\text{p}}}}{1+\frac{{{\rho }_{1}}{{v}_{\text{s}, 1}}}{{{\rho }_{2}}{{v}_{\text{s, }2}}}}$

(3)

式中：v_{s, 1}、v_{s, 2}分别表示复板、基板的声速，m·s^-1；ρ₁、ρ₂分别表示复板、基板的密度，g·cm^-3；v_p表示复板的碰撞速度，m·s^-1。

结合表 4和表 5中3种理论公式计算得到的碰撞速度，通过(3)式可得到复板的碰撞压力，表 6及表 7为其理论计算值与数值模拟结果的比较。可见：Gurney公式和Aziz公式的计算结果均存在较大的偏差；而由Deribas公式计算的两组结果与数值模拟结果较接近，误差均未超过5%，说明Deribas公式和SPH-FEM耦合方法对双面爆炸焊接具有较好的指导意义。

表 6 10 mm药厚下碰撞压力理论计算结果与数值模拟结果的比较

Table 6. Comparison of collision pressure betweentheoretical calculation and numerical simulationwith explosive thickness of 10 mm

Theoreticalformula	Collision pressure/GPa		Error/%
Theoreticalformula	Calculation	Simulation	Error/%
Gurney	22.08	17.08	-29.3
Aziz	14.42	17.08	15.6
Deribas	17.30	17.08	-1.3

下载: 导出CSV

| 显示表格

表 7 5 mm药厚下碰撞压力理论计算结果与数值模拟结果的比较

Table 7. Comparison of collision pressure betweentheoretical calculation and numerical simulationwith explosive thickness of 5 mm

Theoreticalformula	Collision pressure/GPa		Error/%
Theoreticalformula	Calculation	Simulation	Error/%
Gurney	17.50	11.25	-55.6
Aziz	9.73	11.25	13.5
Deribas	11.68	11.25	-3.8

下载: 导出CSV

| 显示表格

3. 结论

利用LS-DYNA软件和SPH-FEM耦合方法对前期双面爆炸焊接实验进行了三维数值模拟，并将模拟结果与实验及理论计算结果进行了对比，得到如下结论。

(1) 10 mm药厚和5 mm药厚下复板位移均略大于间隙值6 mm，这是由于爆轰载荷作用下复板有一定的减薄率所致。

(2) 10 mm药厚下，复板中部的最大碰撞速度为897 m/s，碰撞压力为17.08 GPa；5 mm药厚下，复板中部的最大碰撞速度为565 m/s，碰撞压力为11.25 GPa。通过与3种理论公式(Gurney公式、Aziz公式、Deribas公式)计算得到的碰撞速度进行比较发现，数值模拟结果与Deribas公式的计算结果较接近，误差较小，且与实验结果较吻合，证明了SPH-FEM耦合方法用于双面爆炸复合模拟的有效性，同时Deribas公式和SPH-FEM耦合方法对双面爆炸复合具有较好的指导意义。

(3) 10 mm药厚和5 mm药厚下复板的碰撞速度及碰撞压力均随着距起爆端距离的增加而增大，该现象是由于爆轰产物的不断堆积和前碰撞点在金属板待复合区振动能的不断增加共同作用的结果。

图 1 四方相PbTeO₃晶体的光学照片(a)及SEM图像(b)

Figure 1. Optical photograph (a) and SEM image (b) of tetragonal PbTeO₃

下载: 全尺寸图片幻灯片

图 2 四方相PbTeO₃在常温常压下的XRD谱（λ=1.59059 Å；黑色“×”、红色实线、蓝色实线、绿色“ | ”分别表示实验数据、计算出的衍射图、细化残差和衍射峰位置；插图显示常温常压XRD谱中存在较微弱的(010)和(111)衍射峰）

Figure 2. XRD pattern of tetragonal PbTeO₃ under room temperature and ambient pressure (λ=1.59059 Å. Black “×”, red solid line, blue solid line and green “|” represent experimental data, calculated diffraction patterns, residuals of the refinement and peak positions, respectively. The illustration shows that the XRD spectra collected at room temperature and ambient pressure has week diffraction peaks at position (010) and (111).)

下载: 全尺寸图片幻灯片

图 3 室温下四方相PbTeO₃的晶体结构

Figure 3. Crystal structure of tetragonal PbTeO₃ at room temperature

下载: 全尺寸图片幻灯片

图 4 常压下四方相PbTeO₃的DSC曲线

Figure 4. DSC curves of tetragonal PbTeO₃ at ambient pressure

下载: 全尺寸图片幻灯片

图 5 四方相PbTeO₃ 样品在不同温度下再烧结的粉末XRD谱

Figure 5. Powder XRD patterns of tetragonal PbTeO₃ samples reheated at different temperatures

下载: 全尺寸图片幻灯片

图 6 室温下采集的不同压力下四方相PbTeO₃的XRD谱

Figure 6. XRD patterns of tetragonal PbTeO₃ collected at room temperature and different pressures

下载: 全尺寸图片幻灯片

图 7 四方相PbTeO₃在0.71 GPa下的XRD谱（λ=0.4133 Å，黑色“×”、红色实线、蓝色实线、绿色“ | ”分别表示实验数据、计算出的衍射图、细化残差和衍射峰位置）

Figure 7. XRD pattern of tetragonal PbTeO₃ at 0.71 GPa (λ=0.4133 Å. Black “×”, red solid line, blue solid line and green “|” represent experimental data, calculated diffraction patterns, residuals of the refinement and peak positions, respectively.)

下载: 全尺寸图片幻灯片

图 8 四方相PbTeO₃在37.5 GPa下的XRD谱（λ=0.4133 Å，黑色“×”、红色实线、蓝色实线、绿色 “ | ”分别表示实验数据、计算出的衍射图、细化残差和衍射峰位置）

Figure 8. XRD pattern of tetragonal PbTeO₃ at 37.5 GPa (λ=0.4133 Å. Black “×”, red solid line, blue solid line and green “|” represent experimental data, calculated diffraction patterns, residuals of the refinement and peak positions, respectively.)

下载: 全尺寸图片幻灯片

图 9 四方相PbTeO₃的晶格参数随压力的变化

Figure 9. Variations of lattice parameters of tetragonal PbTeO₃ with pressure

下载: 全尺寸图片幻灯片

图 10 四方相PbTeO₃的相对体积随压力的变化

Figure 10. Variation of relative volume of tetragonal PbTeO₃ with pressure

下载: 全尺寸图片幻灯片

表 1 室温下四方相PbTeO₃的结构参数

Table 1. Structural parameters of tetragonal PbTeO₃ at room temperature

Atom	Site	x	y	z	U_iso/Å²
Pb1	4a	0.745(5)	0.752(6)	−0.057(2)	0.007(1)
Te1	4a	0.753(4)	0.246(6)	0.200(7)	0.003(5)
O2	4a	0.648(4)	0.014(5)	0.152(9)	0.071(9)
O1	4a	0.879(3)	0.485(8)	0.125(2)	0.038(2)
O3	4a	0.976(4)	0.220(3)	0.287(2)	0.056(8)
Note: P4₁, a=b=5.318(2) Å, c=11.946(9) Å, V=337.906(3) Å³, α=β=γ=90°, R_wp=7.14%, R_p=5.56%.

下载: 导出CSV

表 2 0.71 GPa下四方相PbTeO₃的结构参数

Table 2. Structural parameters of tetragonal PbTeO₃ at 0.71 GPa

Atom	Site	x	y	z	U_iso/Å²
Pb1	4a	0.752(1)	0.746(8)	0.391(1)	0.010(6)
Te1	4a	0.789(8)	0.238(8)	0.618(7)	0.006(5)
O2	4a	1.059(8)	0.676(9)	0.225(7)	0.080(1)
O1	4a	0.419(4)	0.068(8)	0.155(3)	0.080(3)
O3	4a	0.917(8)	0.386(4)	0.251(9)	0.297(8)
Note: P4₁(76), a=b=5.2388(1) Å, c=11.7442(1) Å, V=322.3211(2) Å³, α=β=γ=90°, R_wp=8.47%, R_p=6.36%.

下载: 导出CSV

表 3 37.5 GPa下四方相PbTeO₃的结构参数

Table 3. Structural parameters of tetragonal PbTeO₃ at 37.5 GPa

Atom	Site	x	y	z	U_iso/Å²
Pb1	4a	0.802(8)	0.725(2)	0.396(9)	0.282(7)
Te1	4a	0.806(5)	0.263(9)	0.606(4)	0.037(6)
O2	4a	1.568(1)	0.675(5)	0.278(3)	0.088(5)
O1	4a	0.605(6)	0.008(2)	0.071(4)	0.090(1)
O3	4a	1.078(5)	0.345(7)	0.228(4)	0.008(1)
Note: P4₁(76), a=b=4.7167(6) Å, c=10.538(3) Å, V=234.455(3)Å³, α=β=γ=90°, R_wp=4.36%, R_p=3.43%.

下载: 导出CSV

表 4 四方相PbTeO₃及一些相关铅氧化物的体弹模量 $B{_0}$

Table 4. Bulk modulus $B{_0}$ of PbTeO₃ and several related lead oxides

Compound	B₀/GPa
Compound	PhaseⅠ	Phase Ⅱ（perovskite）
PbNiO₃	184(3)^[4]	166(8)^[4]
PbCrO₃	59(5)^[5]	187(4)^[5]
PbTiO₃	104(4)^[32]	141(5)^[32]
PbVO₃	61(2)^[33]	155(3)^[33]
PbTeO₃	42(1)

下载: 导出CSV

参考文献(33)

[1]	SHIMONI-LIVNY L, GLUSKER J P, BOCK C W. Lone pair functionality in divalent lead compounds [J]. Inorganic Chemistry, 1998, 37(8): 1853–1867. doi: 10.1021/ic970909r
[2]	HAMANI D, MASSON O, THOMAS P. Localization and steric effect of the lone electron pair of the tellurium Te⁴⁺ cation and other cations of the p-block elements: a systematic study [J]. Journal of Applied Crystallography, 2020, 53(5): 1243–1251. doi: 10.1107/S1600576720010031
[3]	PYYKKO P. Relativistic effects in structural chemistry [J]. Chemical Reviews, 1988, 88(3): 563–594. doi: 10.1021/cr00085a006
[4]	WANG W D, WANG S M, HE D W, et al. Pressure induced phase transition of PbNiO₃ from LiNbO₃-type to perovskite [J]. Solid State Communications, 2014, 196(1): 8–12.
[5]	XIAO W S, TAN D Y, XIONG X L, et al. Large volume collapse observed in the phase transition in cubic PbCrO₃ perovskite [J]. Proceedings of the National Academy of Sciences of the United States of America, 2010, 107(32): 14026–14029. doi: 10.1073/pnas.1005307107
[6]	WANG W D, HE D W, XIAO W S, et al. Electrical characterization in the phase transition between cubic PbCrO₃ perovskites at high pressures [J]. Chinese Physics Letters, 2013, 30(11): 117201. doi: 10.1088/0256-307X/30/11/117201
[7]	KOHN K, INOUE K, HORIE O, et al. Crystal chemistry of MSeO₃and MTeO₃ (M= Mg, Mn, Co, Ni, Cu, and Zn) [J]. Journal of Solid State Chemistry, 1976, 18(1): 27–37. doi: 10.1016/0022-4596(76)90075-X
[8]	LI Y, HAN Y H, MA Y Z, et al. Pressure effects on grain boundary, electrical and vibrational properties of the polycrystalline BaTeO₃ [J]. Europhysics Letters, 2012, 98(6): 66006. doi: 10.1209/0295-5075/98/66006
[9]	BALDINOZZI G, SCIAU P, MORET J, et al. A new incommensurate phase in a lead ordered perovskite: Pb₂MgTeO₆ [J]. Solid State Communications, 1994, 89(5): 441–445. doi: 10.1016/0038-1098(94)90209-7
[10]	RAI R S, SHARMA S, CHOUDHARY R N P. Structural and electrical properties of magnesium tellurite ceramics [J]. Ferroelectrics, 2002, 275(1): 11–18. doi: 10.1080/00150190214284
[11]	YAMADA T, AHAREN T, KANEMITSU Y. Near-band-edge optical responses of CH₃NH₃PbCl₃ single crystals: photon recycling of excitonic luminescence [J]. Physical Review Letters, 2018, 120(5): 057404. doi: 10.1103/PhysRevLett.120.057404
[12]	DITYATIEV O A, BERDONOSOV P S, DOLGIKH V A, et al. On the crystal structures of SrTeO₃ [J]. Solid State Sciences, 2006, 8(7): 830–835. doi: 10.1016/j.solidstatesciences.2006.03.003
[13]	RAI R, SHARMA S, CHOUDHARY R N P. Ferroelectric phase transition in calcium tellurite ceramics [J]. Journal of Materials Science Letters, 2002, 21(4): 297–299. doi: 10.1023/A:1017923820691
[14]	POUPON M, BARRIER N, PETIT S, et al. Hydrothermal synthesis and dehydration of CaTeO₃(H₂O): an original route to generate new CaTeO₃ polymorphs [J]. Inorganic Chemistry, 2015, 54(12): 5660–5670. doi: 10.1021/acs.inorgchem.5b00037
[15]	DITYAT’EV O A, STEFANOVICH S Y, PRITUZHALOV V A, et al. Dielectric and nonlinear optical properties of SrTeO₃-based solid solutions [J]. Inorganic Materials, 2004, 40(7): 740–743. doi: 10.1023/B:INMA.0000034774.16227.cb
[16]	BERGMAN J G, BOYD G D, ASHKIN A, et al. New nonlinear optical materials: metal oxides with nonbonded electrons [J]. Journal of Applied Physics, 1969, 40(7): 2860–2863. doi: 10.1063/1.1658089
[17]	WILLIAMS S A. Schieffelinite, a new lead tellurate-sulphate from Tombstone, Arizona [J]. Mineralogical Magazine, 1980, 43(330): 771–773. doi: 10.1180/minmag.1980.043.330.11
[18]	WEIL M, SHIRKHANLOU M, FÜGLEIN E, et al. Determination of the correct composition of “hydrous lead (Ⅱ) oxotellurate (Ⅳ)” as PbTeO₃, crystallizing as a new polymorph [J]. Crystals, 2018, 8(1): 51. doi: 10.3390/cryst8010051
[19]	MARIOLACOS K. Die kristallstruktur von PbTeO₃ [J]. Anzeiger der Österreichische Akademie der Wissenschaften Mathematisch-Naturwissenschatliche Klasse, 1969, 106(1): 129–130.
[20]	ROBERTSON D S, SHAW N, YOUNG I M. A study of crystals in the lead oxide/tellurium dioxide system [J]. Journal of Physics D: Applied Physics, 1976, 9(8): 1257. doi: 10.1088/0022-3727/9/8/012
[21]	KOSSE L, POLITOVA E, BUSH A, et al. Growth and some peculiarities of beta-PbTeO₃ single-crystals [J]. Kristallografiya, 1983, 28(3): 510–513.
[22]	YOUNG I M. The central region of the PbO-TeO₂ phase diagram [J]. Journal of Materials Science, 1979, 14(7): 1579–1585. doi: 10.1007/BF00569277
[23]	KOSSE L, POLITOVA E, ASTAF’EV A, et al. Growth and electrophysical properties of some Pb-Te-O single crystals [J]. Soviet Physics Solid State, 1983, 25(7): 1170–1171.
[24]	SCIAU P, LAPASSET J, MORET J. Structure de la phase quadratique de PbTeO₃ [J]. Acta Crystallographica Section C: Crystal Structure Communications, 1986, 42(12): 1688–1690. doi: 10.1107/S0108270186090923
[25]	GAITÁN M, JEREZ A, NOGUERALES P, et al. New methods of synthesis of mixed oxides of Te and Pb: characterization of the new phases PbTeO₃(cubic) and PbTeO₄(orthorhombic) [J]. Synthesis and Reactivity in Inorganic and Metal-Organic Chemistry, 1987, 17(5): 479–490. doi: 10.1080/00945718708070212
[26]	STAVRAKIEVA D, IVANOVA Y, PYROV J. On the composition of the crystal phases in the PbO-TeO₂ system [J]. Journal of Materials Science, 1988, 23(5): 1871–1876. doi: 10.1007/BF01115733
[27]	MA Y, WANG W D, LIU Q J, et al. Raman studies in tetragonal structure PbTeO₃ [J]. Solid State Communications, 2017, 260(1): 1–5.
[28]	MAO H K, XU J, BELL P M. Calibration of the ruby pressure gauge to 800 kbar under quasi-hydrostatic conditions [J]. Journal of Geophysical Research, 1986, 91(5): 4673–4676.
[29]	HEMLEY R J, ZHA C S, JEPHCOAT A P, et al. X-ray diffraction and equation of state of solid neon to 110 GPa [J]. Physical Review B: Condensed Matter and Materials Physics, 1989, 39(16): 11820–11827. doi: 10.1103/PhysRevB.39.11820
[30]	FINGER L W, HAZEN R M, ZOU G, et al. Structure and compression of crystalline argon and neon at high pressure and room temperature [J]. Applied Physics Letters, 1981, 39(11): 892–894. doi: 10.1063/1.92597
[31]	LEVY D, PAVESE A, SANI A, et al. Structure and compressibility of synthetic ZnAl₂O₄ (gahnite) under high-pressure conditions, from synchrotron X-ray powder diffraction [J]. Physics and Chemistry of Minerals, 2001, 28(9): 612–618. doi: 10.1007/s002690100194
[32]	ZHU J L, XU H W, ZHANG J Z, et al. Thermal equations of state and phase relation of PbTiO₃: a high P-T synchrotron X-ray diffraction study [J]. Journal of Applied Physics, 2011, 110(8): 084103. doi: 10.1063/1.3651377
[33]	ZHOU W, TAN D, XIAO W, et al. Structural properties of PbVO₃ perovskites under hydrostatic pressure conditions up to 10.6 GPa [J]. Journal of Physics: Condensed Matter, 2012, 24(43): 435403. doi: 10.1088/0953-8984/24/43/435403

施引文献

资源附件(0)

访问统计

点击查看大图

图(10) / 表(4)

计量

文章访问数: 373
HTML全文浏览量: 224
PDF下载量: 45

1. 计算模型及参数选取
1.1 计算模型
1.2 材料模型及参数设定
2. 模拟结果与分析
2.1 10 mm药厚的模拟结果
2.2 5 mm药厚的模拟结果
2.3 分析与讨论
3. 结论

四方相PbTeO3晶体在高压下的压缩行为

doi: 10.11858/gywlxb.20220646

作者简介: 法志湘（1998－），女，硕士研究生，主要从事高温高压下材料的物性研究. E-mail：afxxya@163.com

通讯作者: 王文丹（1981－），男，博士，副教授，主要从事高压下新材料的合成及物性测试研究. E-mail：wendan.wang@swjtu.edu.cn

计量

出版历程

Compression Behavior of Tetragonal PbTeO3 Crystals under High Pressure

1. 计算模型及参数选取

1.1 计算模型

1.2 材料模型及参数设定

2. 模拟结果与分析

2.1 10 mm药厚的模拟结果

2.1.1 碰撞点位移

2.1.2 复板碰撞速度

2.1.3 碰撞点压力分布

2.2 5 mm药厚的模拟结果

2.2.1 碰撞点位移

2.2.2 复板碰撞速度

2.2.3 碰撞点压力分布

2.3 分析与讨论

3. 结论

计量

出版历程

目录

1. 计算模型及参数选取

1.1 计算模型

1.2 材料模型及参数设定

2. 模拟结果与分析

2.1 10 mm药厚的模拟结果

2.2 5 mm药厚的模拟结果

2.3 分析与讨论

3. 结论

四方相PbTeO₃晶体在高压下的压缩行为

作者简介:
法志湘（1998－），女，硕士研究生，主要从事高温高压下材料的物性研究. E-mail：afxxya@163.com

通讯作者:
王文丹（1981－），男，博士，副教授，主要从事高压下新材料的合成及物性测试研究. E-mail：wendan.wang@swjtu.edu.cn

Compression Behavior of Tetragonal PbTeO₃ Crystals under High Pressure