Crystal Plasticity Finite Element Simulation of Polycrystal Aluminum under Shock Loading
-
摘要: 在多晶材料中,不同取向晶粒间的晶界往往对材料在冲击加载下的动力学响应有极大的影响。在单晶晶体塑性模型的基础上,通过考虑晶界与位错相互作用的微观机理,建立了一个包含晶界阻力、几何必需位错以及背应力的多晶晶体塑性模型,并模拟研究了基于Voronoi几何模型的多晶铝在冲击加载下的力学响应。结果表明:冲击波后的晶界单元存在极高的残余剪应力,而晶粒内部单元的剪应力趋近于零;晶界附近存在较大的塑性变形梯度,产生大量沿晶界分布的几何必需位错和背应力;由滑移不连续性引起的晶界阻力是造成冲击波后大量残余剪应力的主要因素,而几何必需位错和背应力对剪应力松弛程度的影响较小。Abstract: In polycrystalline materials, the grain boundaries between grains with different orientations have a great influence on the dynamic response of material under shock loading. On the basis of the single crystal plasticity model, a polycrystal plasticity model containing grain boundary obstacle, geometrically necessary dislocations (GND) and back-stress is established by considering the microscopic mechanism of the interaction between grain boundaries and dislocations. Based on this model, the mechanical response of polycrystalline aluminum with Voronoi geometry under shock loading was studied through simulations. The results show that: (1) the grain boundary elements after the shock loading have a very high residual shear stress, while the shear stress of the element within the grain tends to be zero; (2) a large plastic deformation gradient is found near the grain boundary, resulting in a large number of GND and back stress distributed along the grain boundary; (3) the grain boundary obstacle caused by the slip discontinuity is the main factor causing the large amount of residual shear stress, while the GND and back stress have little influence on the extent of shear stress relaxation.
-
熔铸装药法具有成本低廉、自动化程度高且不受药室形状限制等优点[1-3],在军用混合炸药铸装中具有不可替代的地位。但是,传统铸装产品容易出现密度不均匀、缩孔、气孔和底隙等[4-6]质量缺陷,不仅影响爆轰性能,而且影响弹药的使用安全性[7-9]。目前国内外对一些先进的熔铸装药工艺尚缺乏探索,因此,对熔铸装药工艺优化的研究尤为重要。
Wang等[10]利用软件模拟了加压凝固工艺下炸药的成型过程,发现外加压力可改善药柱缩孔。李敬明等[11]通过模拟炸药的熔铸成型过程,预测了药柱的缩孔形态与位置,并加以实验验证,软件预测的缩孔与实验结果近似,表明软件适用于炸药熔铸成型计算。黄勇等[12]分别模拟了不同冒口保温温度和不同外加压力下炸药的熔铸成型过程,得出了可基本消除药柱缩孔的最佳冒口保温温度和最低外加压力。田勇[13]对炸药熔铸成型过程中的各项参数进行了数值模拟,得出了降低药液黏度或加大冒口漏斗尺寸可降低药柱缩孔率,降低换热系数可改善药柱热应力的结论。然而,目前的研究大多局限于对已有的工艺进行验证或得到某种工艺的最佳参数,缺乏创新性。因此,利用数值仿真对熔铸装药工艺进行优化研究是可行且必要的[14-15]。
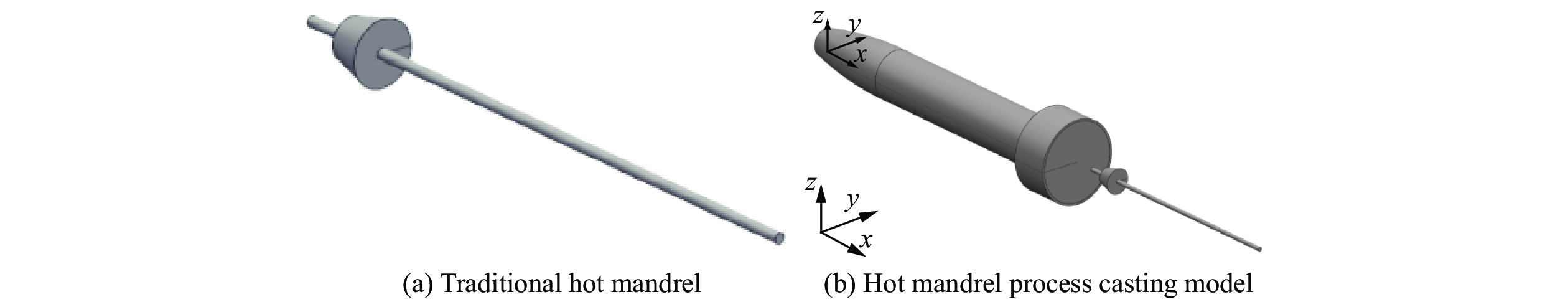
液态炸药从注入弹体到降至室温的过程中伴随着液态收缩、凝固收缩(相变收缩)和固态收缩,如果收缩的体积得不到补充,将在最后凝固部位形成缩孔[16]。热芯棒工艺是通过使其周围局部炸药受热重新熔化,保持补缩通道畅通来避免缩孔的产生[17]。
本研究基于传统熔铸装药工艺,对炸药成型过程中的流场和温度场进行耦合计算,验证缩孔形成机理以及传统热芯棒作用机理,通过分析数值模拟结果发现传统热芯棒对于大长径比战斗部装药的不足并对其进行改良,设计出一种优化温度控制的热芯棒系统,通过数值模拟对缩孔缩松形成位置和大小进行预测,证明改良热芯棒的优越性,以期为改良熔铸装药成型工艺提供一个新的方向,对提高熔铸装药质量起到推动和指导作用。
1. 熔铸装药工艺仿真与分析
1.1 铸装模型建立
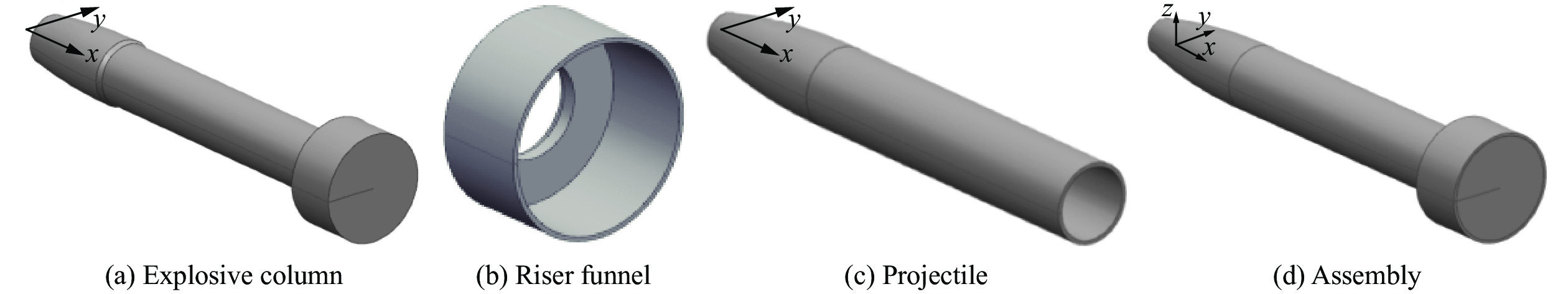
本研究中用于数值模拟的弹丸直径为300 mm,长径比为6∶1,为典型的大长径比装药战斗部。利用三维建模软件UG建立炸药柱、冒口漏斗、弹体3部分的模型,分别如图1(a)、图1(b)、图1(c)所示,装配得到的熔铸装药模型如图1(d)所示。
将模型导入铸造软件ProCAST的Visual-Mesh模块中进行画网操作,由于此模型结构并不规则,因此在划分面网格时选择三角形网格,然后划分体网格生成四面体单元。为提高仿真精度,网格尺寸应设置得尽可能小,这里网格尺寸设定为30 mm,总单元数为29 405 180。网格检查无误后保存为.vdb格式,进入Visual-Cast模块中进行前处理操作。所用炸药为B炸药,材料的热物性参数如表1所示,其中:
θm 为材料熔点,L为内能,C为比热容,ρ 为密度,μ 为黏度,α 为线膨胀系数。弹体材料为H13钢,冒口漏斗材料为铝。炸药、弹体和冒口的初始温度分别设置为95、60和60 ℃,冷却方式为30 ℃水冷,边界条件在装药伊始进行加载,并作用于整个工艺过程。Material θm/℃ L/(kJ·kg–1) C/(J·kg–1·K–1) ρ/(g·cm–3) μ/(Pa·s) α/K–1 Explosive B 78–80 33.5 1 063 (25 ℃)
1 276 (50 ℃)
1 573 (75 ℃)
1 481 (85 ℃)
1 427 (90 ℃)
1 035 (100 ℃)1.680 0.31 (83 ℃)
0.27 (90 ℃)
0.27 (95 ℃)7.69 × 10–5 (23 ℃)
2.00 × 10–5 (50 ℃)
5.00 × 10–6 (75 ℃)
1.00 × 10–6 (80 ℃)H13 steel 0.73 7.800 4.45 × 10–8 (22–500 ℃) Al 0.90 7.625 1.2 传统铸装工艺仿真
在熔铸装药数值模拟过程中,通过完全的Navier-Stocks流动方程对流体流动和传热进行耦合计算,炸药充型过程中紊流的模拟采用k-
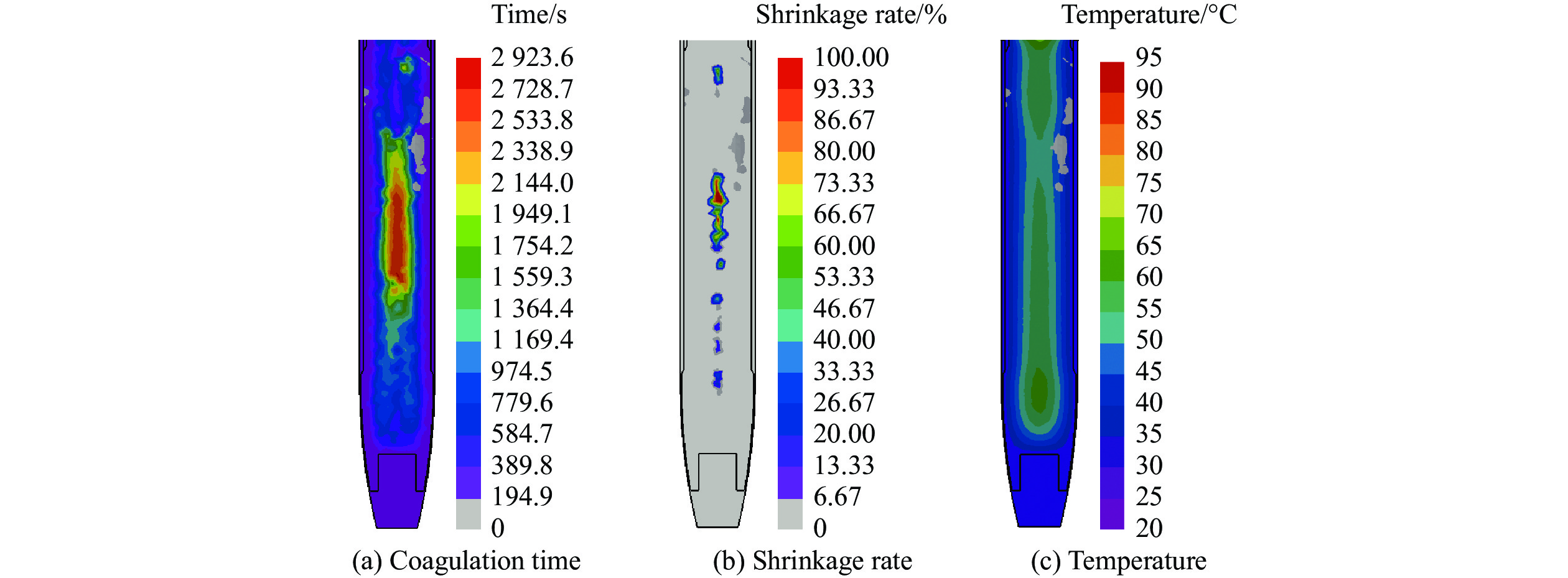
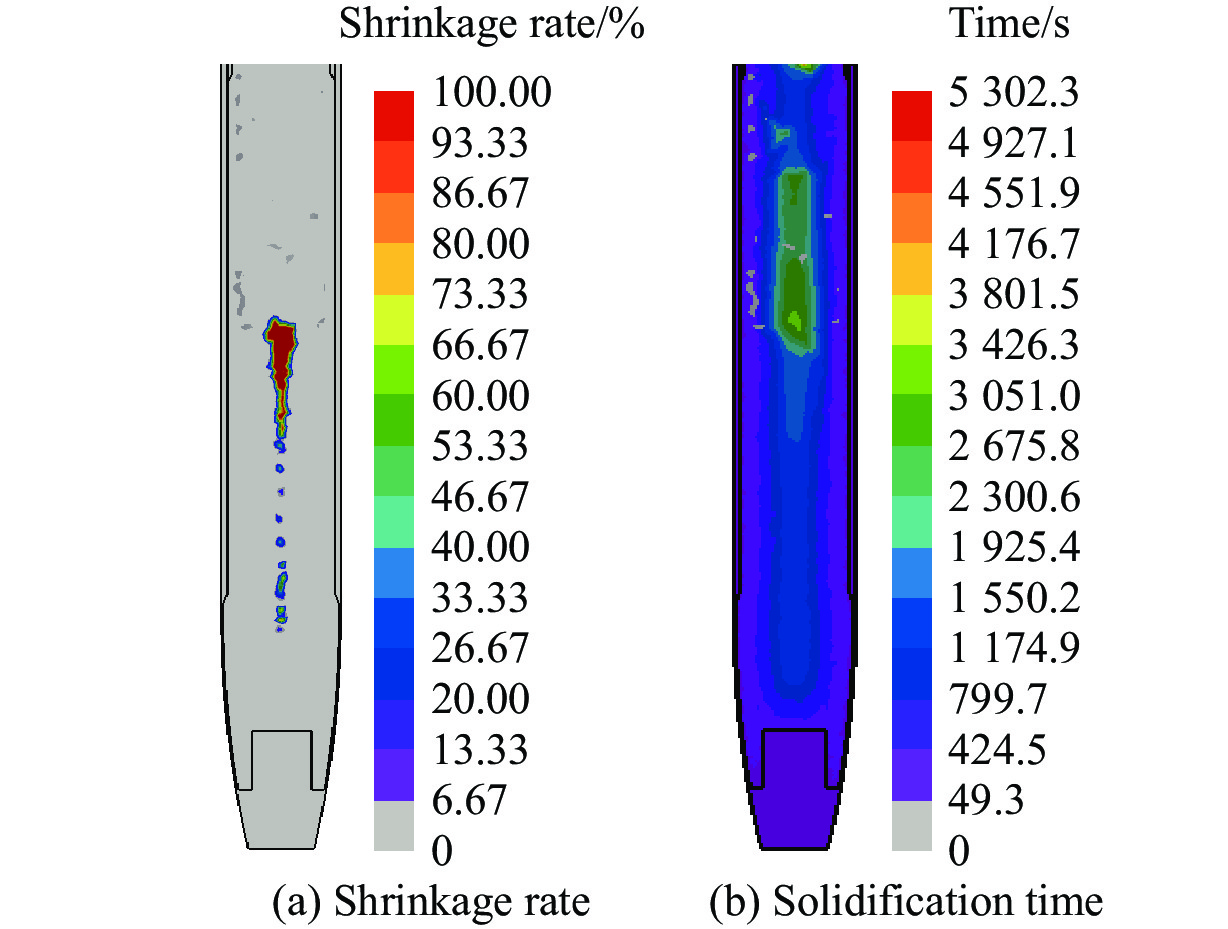
ε 双方程模型,自由表面的处理采用SOLA-VOF法;利用热焓法处理凝固过程中的相变潜热,采用双线性强化弹塑性模型描述凝固过程中的应力和应变;用临界固相率与补缩距离法相结合的方式预测熔铸药柱内部的缩孔缩松,并采用Gurson修正模型来预测裂纹[21]。基于以上计算模型,根据文献[11]中的熔铸装药实验设计仿真模型模拟炸药的熔铸装药过程,从而实现对装药缺陷的预测,并与文献[11]中的实验结果进行对比,如图2所示。实验结果的CT扫描显示:装药缺陷出现在炸药顶部且呈漏斗状,与数值模拟得到的缺陷云图显示的缺陷大小和位置基本一致。对炸药进行传统铸装时,流速设置为0.8 kg/s,充型后自然凝固,后处理中药柱凝固时间、温度以及缩松率云图如图3所示。图3中的凝固时间是指凝固过程持续的时间,即各部分的温度保持在固液相线之间的时间。
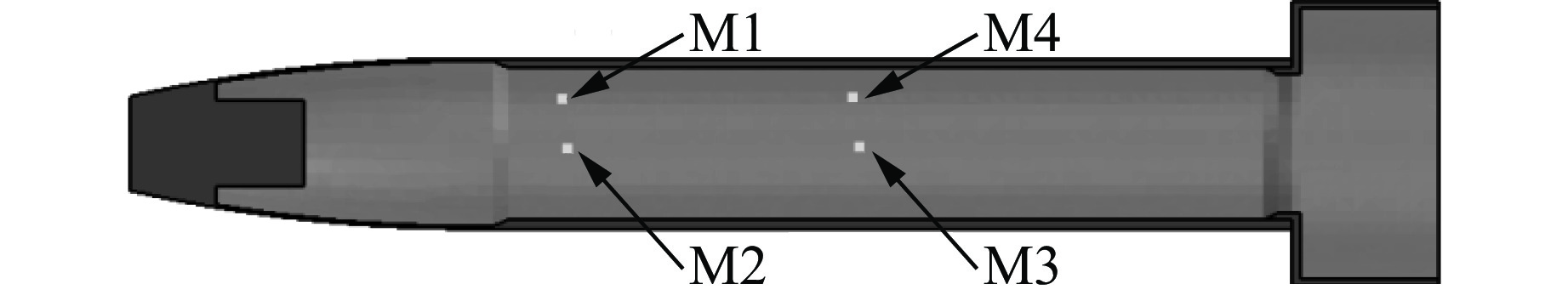
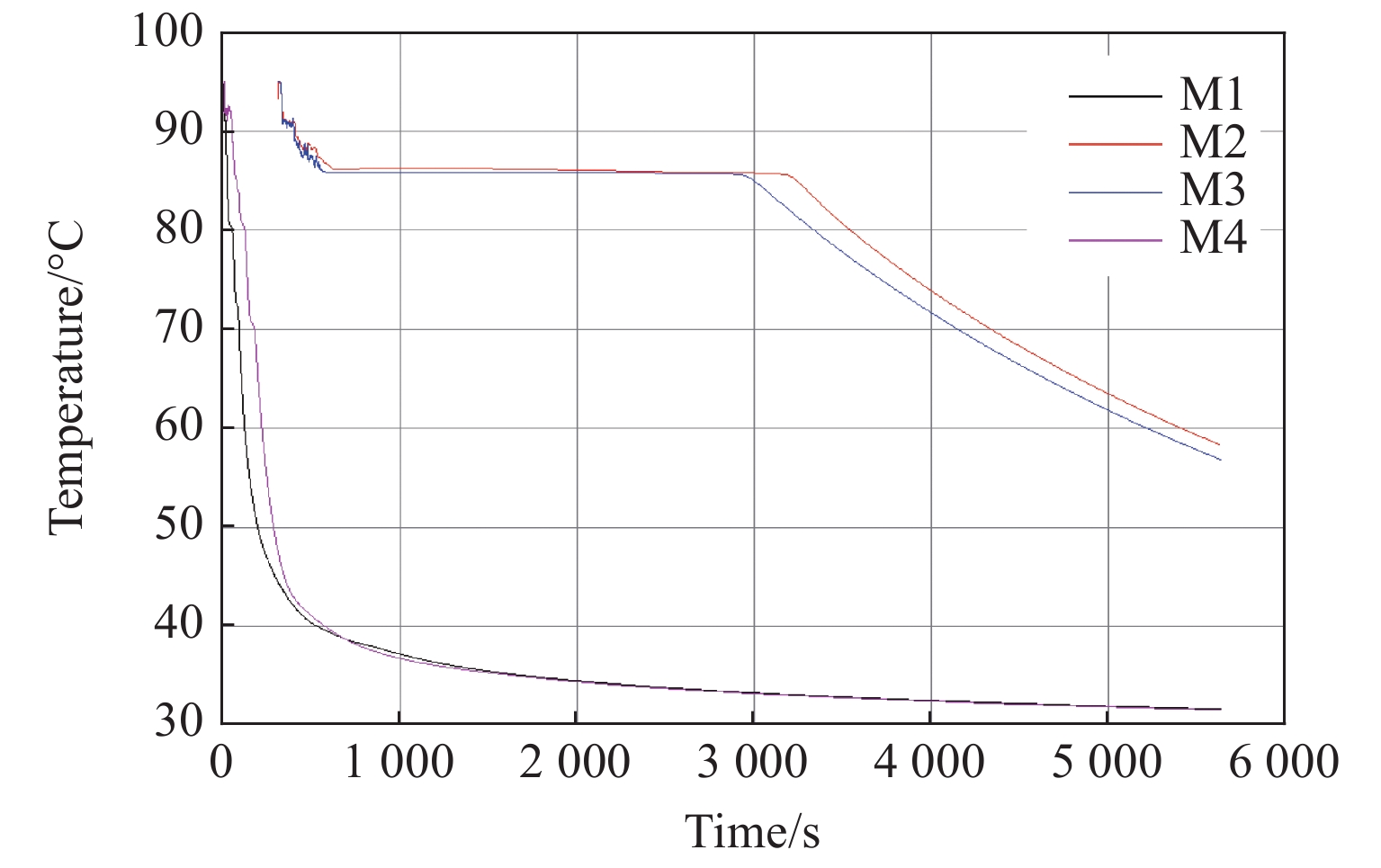
为了更好地了解炸药柱内部温度的变化情况,选取4个温度测试点,其分布如图4所示。其中:M1和M4、M2和M3分别位于靠近弹体和靠近炸药柱中心的不同高度,且M1和M2、M3和M4分别处于同一高度。4个测温点处的温度-时间曲线如图5所示。
由图3(a)可以看出,自然凝固时,传统铸装药柱的凝固顺序为由外向内平行层凝固,药柱最中心需要2 000 s以上才可凝固,靠近中心的一层和靠近弹体的一层需要的凝固时间分别为800 和200 s左右。由图3(b)可以看出,在药柱的中心轴线上分布着7处缩松率达20%以上的疵病,在药柱中段甚至出现了最大直径20 mm以上、不规则形状的缩孔,这必然会影响弹丸发射的安全性。结合图3(c)和图5进行分析可知,靠近弹体的外层炸药经过热量传导能够快速冷凝,而外层的凝固阻碍了中心热量向外传导,导致药柱直径最大处温度居高不下,降低到熔点以下需要的时间就会比外层长得多。由于药柱中心的温度长时间高于药柱其他位置,形成了非常大的径向温度梯度,药柱中心会产生巨大的收缩量,而轴向温度梯度很小,补缩通道在凝固之前被堵塞,该收缩量得不到相应的补充,因此形成了图3(b)所示的缩孔缩松。
2. 传统热芯棒工艺
2.1 热芯棒工艺设计
实际生产中热芯棒工艺的传热过程很复杂,为了提高仿真效率,应作适当的简化。考虑到热芯棒的工作原理是通过不断发热影响炸药柱的温度场分布,从而影响炸药柱的固液相分布,因此将热芯棒部分看作热量源,建立热芯棒模型,如图6所示。
热芯棒和热芯杯的温度设置为90 ℃,根据图3(a)中自然状态下的凝固时间云图来设计热芯棒的位移-时间(x-t)关系,如表2所示。
表 2 热芯棒位移-时间关系Table 2. Displacement-time relationship of the hot mandrelt/s x/mm 0 0 5 600 500 600 1 000 0 2.2 仿真结果分析
由图7(a)的铸装后处理云图可以看出,在药柱中心处仍然散布着缩松率为20%左右的疵病,在药柱中部甚至有最大直径为28 mm左右、不规则形状的缩孔,这都是由于补缩通道堵塞引起的补缩不充足导致的。由图7(b)可以看出,药柱最中心需要2 600 s以上才可凝固,靠近药柱中心的一层和靠近弹体的一层凝固时间分别为1 000 和400 s左右。这表明加入热芯棒工艺后,由外到内的凝固顺序没有发生改变,因而缩孔缩松仍然难以完全消除。
3. 热芯棒优化
3.1 多层次热芯棒的作用机理
炸药熔铸装药在自然状态下由外到内层层凝固,传统的热芯棒工艺只能在一定程度上保持补缩通道畅通,而不能改变药柱径向的凝固顺序。针对传统热芯棒在预防缩孔上的局限性,设计了一种新型的多层次优化的热芯棒,在更大程度上改变熔融态炸药的凝固顺序,从根本上预防缩孔的形成。
3.2 多层次热芯棒的设计
3.2.1 模型结构设计
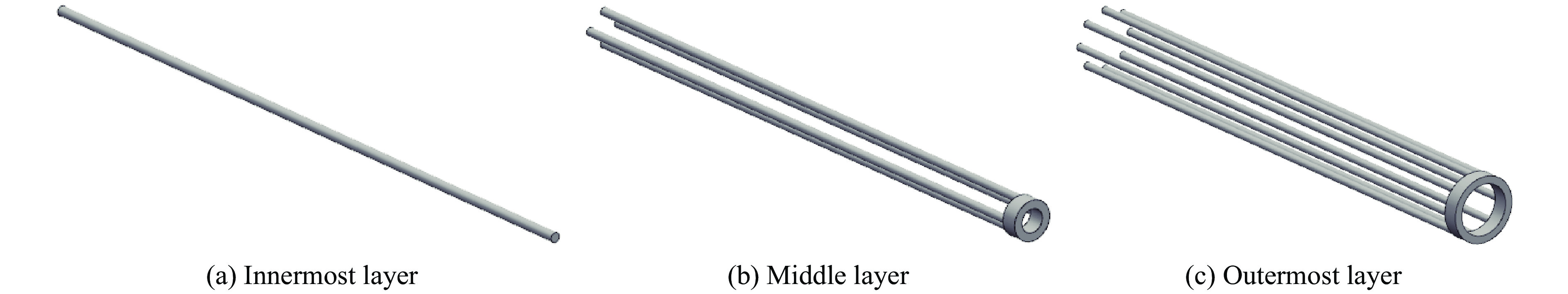
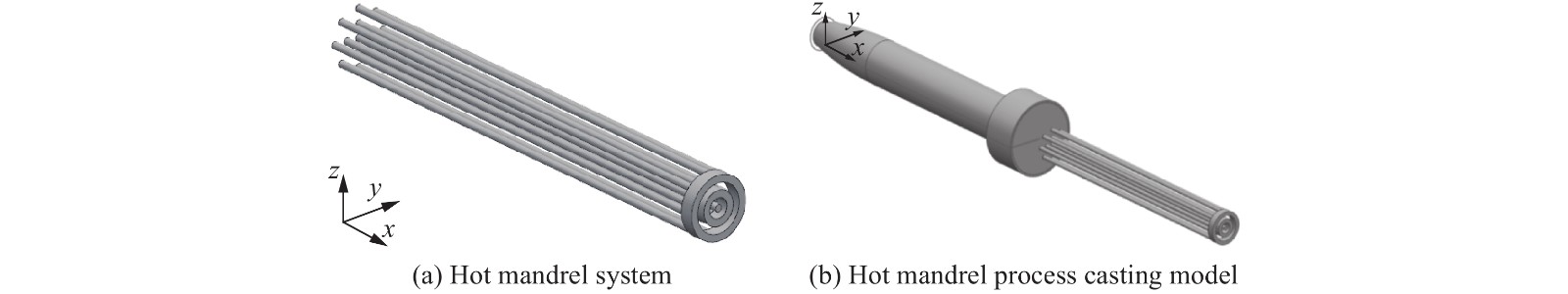
改良后的热芯棒由3部分组成:系统最内层、中间层和最外层,分别由1支、4支和8支热芯棒组成,每支热芯棒直径均为10 mm,如图8所示,分别作用于装药的不同部位,3部分同时作用,在一定程度上将由外到内的凝固顺序改变为自下而上。将3部分装配起来得到热芯棒系统,与熔铸装药模型装配后得到改良的铸装模型,如图9所示。
3.2.2 工艺参数设计
为了改变炸药的凝固顺序,需要降低药柱的径向温度差,由图5可以看出,熔铸装药在自然凝固过程中,越靠近弹体位置的温度下降越快。为了降低同一高度的内外层温度差,热芯棒作为热源,由外层到内层需要有一定的温度降。炸药液体的初始温度为95 ℃,为了保证炸药的安全性,设置温度不宜过高,而由表1可知,炸药的熔点为80 ℃,温度太低会使炸药液过早凝固。因此3组热芯棒系统的温度设置由外而内分别为:95、89和86 ℃,95、91和86 ℃,95、92和86 ℃。
3.3 结果对比分析
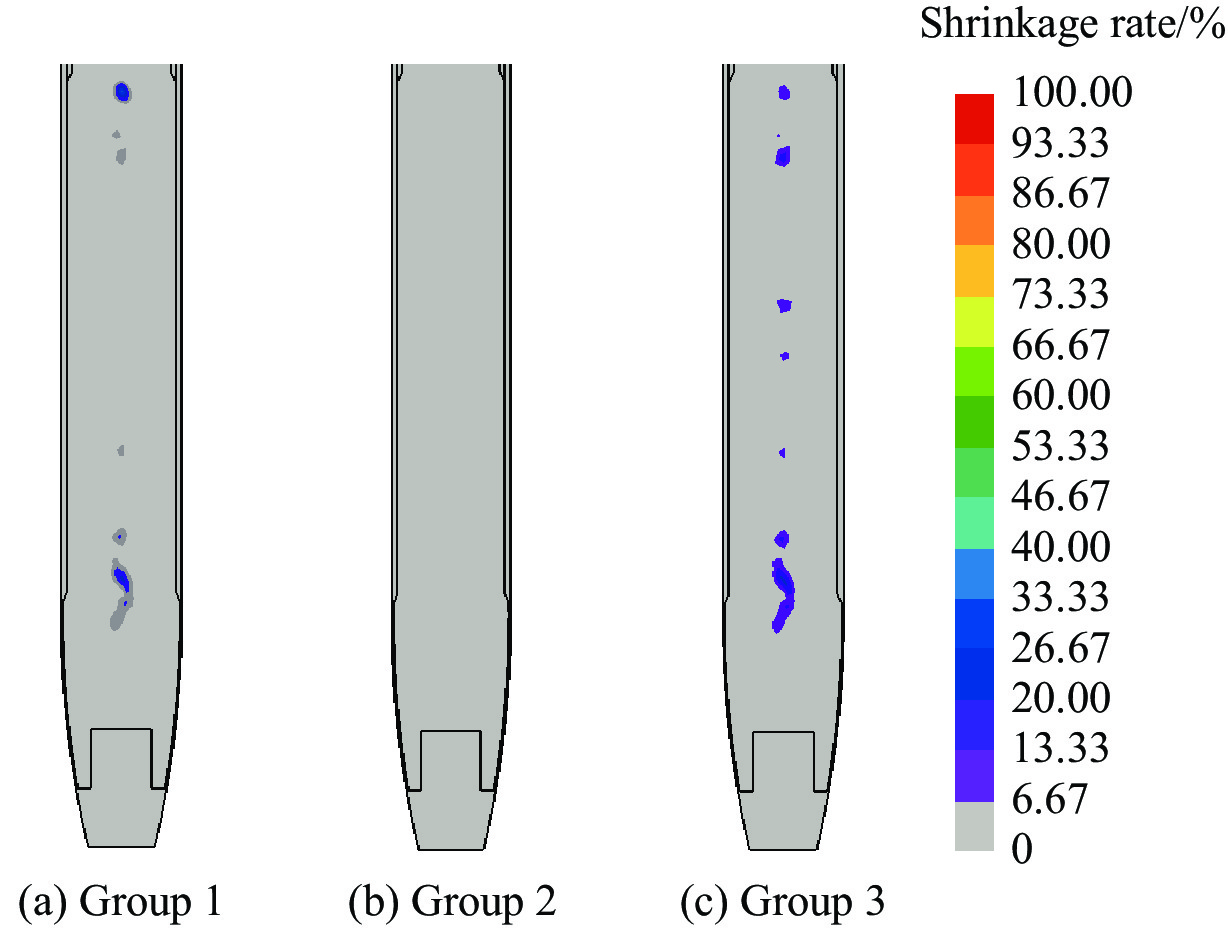
3组温度设置的改良热芯棒工艺铸装缩松率结果如图10所示。由图10(a)可以看出,在药柱中心直径最大处仍然分布着缩松率约为13%的疵病,说明大直径处的大收缩量没有得到足够的补充,表明热芯棒系统并没有使同一高度炸药的温度保持一致,因而第1组温度设置并不合理;由图10(b)可以看出,整个药柱的缩松率均为零,热芯棒系统使得药柱同一高度的径向温度差降低,改变了熔融态炸药由外到内的凝固顺序,缩孔缩松被完全消除,说明此时的热芯棒系统温度设置是合理的;由图10(c)可以看出,第3组与第1组的缩松分布结果基本一致,但缩松率明显高于第1组,因此第3组的温度设置也不合理。
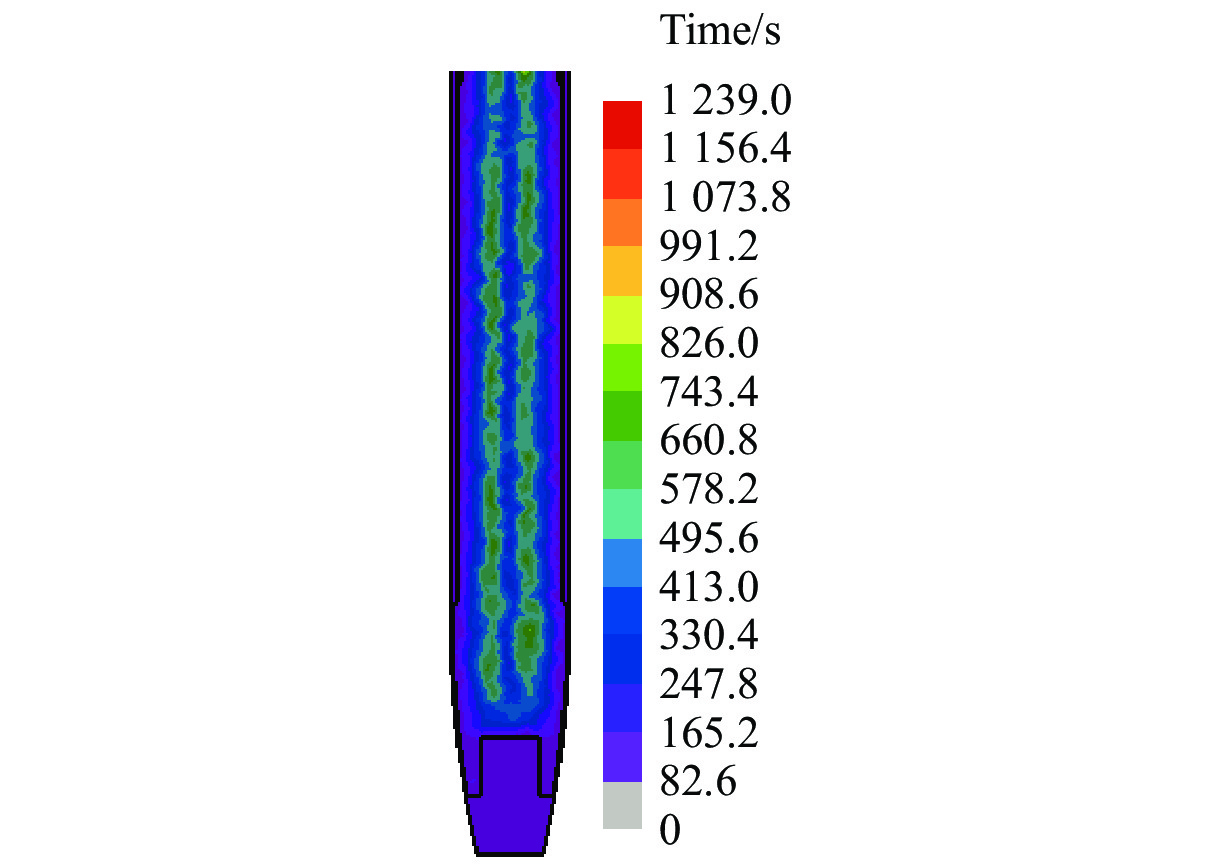
第2组铸造仿真的凝固时间云图如图11所示。改良热芯棒系统与传统热芯棒的作用原理不同,改良热芯棒系统中炸药的凝固顺序发生变化,凝固时间最长的位置出现在冒口内部而不是药柱中心,忽略壳体外部炸药,只给出冒口以下凝固时间云图。对比图3(a)和图11可以看出,改良热芯棒工艺比传统工艺铸装的凝固时间大大缩短,这是由于传统工艺自然冷却时没有外部干预,中心位置传热受阻,长时间处于80 ~ 82 ℃,因此在图3(a)中表现为整体凝固时间较久。而改良工艺后,药液在热芯棒系统作用下有序传热、顺序冷凝,温度很快从液相线以上降到固相线以下,因此整个凝固过程持续的时间相比自然凝固大大缩短。药柱中心层凝固时间为100 s左右,靠近弹体的一层凝固时间为60 s左右。对比图3(a)和图7(b)可以看出:药柱自然凝固时,内部凝固时间极差达到1 200 s以上;采用传统热芯棒工艺时,药柱内部的凝固时间极差可达2 200 s;而采用改良热芯棒工艺后,药柱内外层的凝固时间差仅为40 s左右。这表明改良热芯棒工艺大大缩短了药柱内外层的凝固时间差。
对第2组铸造仿真进行测温,仍然选取图4中所示的4个测温点,得到图12所示的温度-时间曲线,对比图5可以看出,0 s时测温点M1~ M4处的初始温度一致,均为95 ℃左右。
对于靠近弹体的测温点M1和M4,在熔铸装药自然凝固时,M1和M4处温度迅速下降,1 000 s之内温度从95 ℃下降到40 ℃以下;改良热芯棒工艺铸装时,M1和M4处温度下降较慢,降温速率平稳,5 000 s时温度仍在50 ℃以上。这表明改良热芯棒工艺减缓了靠近弹体装药的降温速率。
对于药柱中心的测温点M2和M3,在熔铸装药自然凝固时,从0 s开始,M2和M3处温度一直保持平稳,3 200 s之后温度才开始下降;而改良热芯棒工艺铸装时,M2和M3处温度从0 s就开始下降,并且整个过程中M2和M3处温度一直呈下降趋势。这表明改良热芯棒工艺使药柱中心温度持续下降。
总体来看,改良热芯棒工艺缩小了药柱中心和靠近弹体炸药液的温度差,改变了熔铸装药自然状态下由外到内的凝固顺序,达到了预期目的。
4. 结 论
(1)熔铸装药自然凝固时,凝固顺序为由外层到内层逐层凝固,药柱中心最晚凝固且无液态炸药补充,在中心轴线处产生多处20%以上的缩松以及一处最大直径为20 mm以上的缩孔。
(2)热芯棒工艺可以在一定程度上保证位于药柱中心的补缩通道畅通,但是在弹体最大直径处,径向温度梯度大导致收缩量大,狭细的补缩通道来不及补充巨大的收缩量,补缩不充分同样会导致装药出现缩孔缩松。
(3)改良热芯棒工艺降低了装药内外层温度差,减小了内外层凝固时间差,改变了装药凝固顺序,极大地改善了药柱内部的缩孔缩松问题。当热芯棒系统温度设置由外到内分别为95、82、86 ℃时,缩孔缩松完全消除,达到了预期目的。
-
表 1 单晶铝的晶体塑性本构参数
Table 1. Parameters of crystal plasticity model for single-crystal Al
-
[1] ZHAKHOVSKY V V, BUDZEVICH M M, INOGAMOV N A, et al. Two-zone elastic-plastic single shock waves in solids [J]. Physical Review Letters, 2011, 107(13): 135502. doi: 10.1103/PhysRevLett.107.135502 [2] ZARETSKY E B, KANEL G I. Tantalum and vanadium response to shock-wave loading at normal and elevated temperatures. non-monotonous decay of the elastic wave in vanadium [J]. Journal of Applied Physics, 2014, 115(24): 243502. doi: 10.1063/1.4885047 [3] KOSITSKI R, STEINBERGER D, SANDFELD S, et al. Shear relaxation behind the shock front in <110> molybdenum: from the atomic scale to continuous dislocation fields [J]. Computational Materials Science, 2018, 149: 125–133. doi: 10.1016/j.commatsci.2018.02.058 [4] AUSTIN R A. Elastic precursor wave decay in shock-compressed aluminum over a wide range of temperature [J]. Journal of Applied Physics, 2018, 123(3): 035103. doi: 10.1063/1.5008280 [5] 刘静楠, 叶常青, 陈开果, 等. <100>LiF高速冲击变形过程的晶体塑性有限元模拟 [J]. 高压物理学报, 2019, 33(1): 014101. doi: 10.11858/gywlxb.20180551LIU J N, YE C Q, CHEN K G, et al. Crystal plasticity finite element simulation of high-rate shock deformation process of <100> LiF [J]. Chinese Journal of High Pressure Physics, 2019, 33(1): 014101. doi: 10.11858/gywlxb.20180551 [6] GURRUTXAGA-LERMA B, BALINT D S, DINI D, et al. Attenuation of the dynamic yield point of shocked aluminum using elastodynamic simulations of dislocation dynamics [J]. Physical Review Letters, 2015, 114(17): 174301. doi: 10.1103/PhysRevLett.114.174301 [7] 刘静楠, 叶常青, 刘桂森, 等. 高温、高压、高应变速率动态过程晶体塑性有限元理论模型及其应用 [J]. 高压物理学报, 2020, 34(3): 030102. doi: 10.11858/gywlxb.20190874LIU J N, YE C Q, LIU G S, et al. Crystal plasticity finite element theoretical models and applications for high temperature, high pressure and high strain-rate dynamic process [J]. Chinese Journal of High Pressure Physics, 2020, 34(3): 030102. doi: 10.11858/gywlxb.20190874 [8] 郑松林. 晶体塑性有限元在材料动态响应研究中的应用进展 [J]. 高压物理学报, 2019, 33(3): 030108. doi: 10.11858/gywlxb.20190725ZHENG S L. Advances in the study of dynamic response of crystalline materials by crystal plasticity finite element modeling [J]. Chinese Journal of High Pressure Physics, 2019, 33(3): 030108. doi: 10.11858/gywlxb.20190725 [9] LUSCHER D J, ADDESSIO F L, CAWKWELL M J, et al. A dislocation density-based continuum model of the anisotropic shock response of single crystal α-cyclotrimethylene trinitramine [J]. Journal of the Mechanics and Physics of Solids, 2017, 98: 63–86. doi: 10.1016/j.jmps.2016.09.005 [10] LLOYD J T, CLAYTON J D, BECKER R, et al. Simulation of shock wave propagation in single crystal and polycrystalline aluminum [J]. International Journal of Plasticity, 2014, 60: 118–144. doi: 10.1016/j.ijplas.2014.04.012 [11] AUSTIN R A, MCDOWELL D L. A dislocation-based constitutive model for viscoplastic deformation of fcc metals at very high strain rates [J]. International Journal of Plasticity, 2011, 27(1): 1–24. doi: 10.1016/j.ijplas.2010.03.002 [12] MANDAL A, GUPTA Y M. Elastic-plastic deformation of molybdenum single crystals shocked along [100] [J]. Journal of Applied Physics, 2017, 121(4): 045903. doi: 10.1063/1.4974475 [13] BECKER R. Effects of crystal plasticity on materials loaded at high pressures and strain rates [J]. International Journal of Plasticity, 2004, 20(11): 1983–2006. doi: 10.1016/j.ijplas.2003.09.002 [14] VOGLER T J, CLAYTON J D. Heterogeneous deformation and spall of an extruded tungsten alloy: plate impact experiments and crystal plasticity modeling [J]. Journal of the Mechanics and Physics of Solids, 2008, 56(2): 297–335. doi: 10.1016/j.jmps.2007.06.013 [15] HILL R, RICE J R. Constitutive analysis of elastic-plastic crystals at arbitrary strain [J]. Journal of the Mechanics and Physics of Solids, 1972, 20(6): 401–413. doi: 10.1016/0022-5096(72)90017-8 [16] ASARO R J, NEEDLEMAN A. Overview No. 42 texture development and strain hardening in rate dependent polycrystals [J]. Acta Metallurgica, 1985, 33(6): 923–953. doi: 10.1016/0001-6160(85)90188-9 [17] PEIRCE D, ASARO R J, NEEDLEMAN A. An analysis of nonuniform and localized deformation in ductile single crystals [J]. Acta Metallurgica, 1982, 30(6): 1087–1119. doi: 10.1016/0001-6160(82)90005-0 [18] HUANG Y. A user-material subroutine incorporating single crystal plasticity in the ABAQUS finite element program [M]. Cambridge: Harvard University, 1991. [19] LUSCHER D J, BRONKHORST C A, ALLEMAN C N, et al. A model for finite-deformation nonlinear thermomechanical response of single crystal copper under shock conditions [J]. Journal of the Mechanics and Physics of Solids, 2013, 61(9): 1877–1894. doi: 10.1016/j.jmps.2013.05.002 [20] TYUTEREV V G, VAST N. Murnaghan’s equation of state for the electronic ground state energy [J]. Computational Materials Science, 2006, 38(2): 350–353. doi: 10.1016/j.commatsci.2005.08.012 [21] SHYUE K M. A fluid-mixture type algorithm for compressible multicomponent flow with Mie-Grüneisen equation of state [J]. Journal of Computational Physics, 2001, 171(2): 678–707. doi: 10.1006/jcph.2001.6801 [22] HANSEN B L, BEYERLEIN I J, BRONKHORST C A, et al. A dislocation-based multi-rate single crystal plasticity model [J]. International Journal of Plasticity, 2013, 44: 129–146. doi: 10.1016/j.ijplas.2012.12.006 [23] SHAHBA A, GHOSH S. Crystal plasticity FE modeling of Ti alloys for a range of strain-rates. part Ⅰ: a unified constitutive model and flow rule [J]. International Journal of Plasticity, 2016, 87: 48–68. doi: 10.1016/j.ijplas.2016.09.002 [24] KUKSIN A Y, YANILKIN A V. Atomistic simulation of the motion of dislocations in metals under phonon drag conditions [J]. Physics of the Solid State, 2013, 55(5): 1010–1019. doi: 10.1134/S1063783413050193 [25] BLASCHKE D N, BURAKOVSKY L, PRESTON D L. On the temperature and density dependence of dislocation drag from phonon wind [J]. Journal of Applied Physics, 2021, 130(1): 015901. doi: 10.1063/5.0054536 [26] KANEL G I, RAZORENOV S V, BAUMUNG K, et al. Dynamic yield and tensile strength of aluminum single crystals at temperatures up to the melting point [J]. Journal of Applied Physics, 2001, 90(1): 136–143. doi: 10.1063/1.1374478 [27] CHOUDHURI D, GUPTA Y M. Shock compression of aluminum single crystals to 70 GPa: role of crystalline anisotropy [J]. Journal of Applied Physics, 2013, 114(15): 153504. doi: 10.1063/1.4824825 [28] 王礼立, 胡时胜, 杨黎明, 等. 材料动力学 [M]. 合肥: 中国科学技术大学出版社, 2017. [29] 胡建波, 俞宇颖, 谭华, 等. 铝的动态屈服强度测量 [C]//第四届全国爆炸力学实验技术学术会议论文集. 武夷山: 安徽省力学学会, 2006. [30] ANDERSSON S, BACKSTROM G. Thermal conductivity and heat capacity of single-crystal LiF and CaF2 under hydrostatic pressure [J]. Journal of Physics C: Solid State Physics, 1987, 20(35): 5951–5952. doi: 10.1088/0022-3719/20/35/011 [31] MILLER R A, SMITH C S. Pressure derivatives of the elastic constants of LiF and NaF [J]. Journal of Physics and Chemistry of Solids, 1964, 25(12): 1279–1292. doi: 10.1016/0022-3697(64)90043-5 [32] RODRÍGUEZ-MARTÍNEZ J A, RODRÍGUEZ-MILLÁN M, RUSINEK A, et al. A dislocation-based constitutive description for modeling the behavior of fcc metals within wide ranges of strain rate and temperature [J]. Mechanics of Materials, 2011, 43(12): 901–912. doi: 10.1016/j.mechmat.2011.09.008 [33] TALLON J L, WOLFENDEN A. Temperature dependence of the elastic constants of aluminum [J]. Journal of Physics and Chemistry of Solids, 1979, 40(11): 831–837. doi: 10.1016/0022-3697(79)90037-4 [34] THOMAS JR J F. Third-order elastic constants of aluminum [J]. Physical Review, 1968, 175(3): 955–962. doi: 10.1103/PhysRev.175.955 [35] NIX F C, MACNAIR D. The thermal expansion of pure metals: copper, gold, aluminum, nickel, and iron [J]. Physical Review, 1941, 60(8): 597–605. doi: 10.1103/PhysRev.60.597 [36] RAVAJI B, JOSHI S P. A crystal plasticity investigation of grain size-texture interaction in magnesium alloys [J]. Acta Materialia, 2021, 208: 116743. doi: 10.1016/j.actamat.2021.116743 [37] 刘晶, 刘韧, 季忠, 等. 基于晶粒晶界有限元的激光微冲击成形数值分析 [J]. 中国激光, 2010, 37(1): 291–295. doi: 10.3788/CJL20103701.0291LIU J, LIU R, JI Z, et al. Numerical analysis of micro laser peen forming based on grain and grain boundary element [J]. Chinese Journal of Lasers, 2010, 37(1): 291–295. doi: 10.3788/CJL20103701.0291 [38] LIM H, LEE M G, KIM J H, et al. Simulation of polycrystal deformation with grain and grain boundary effects [J]. International Journal of Plasticity, 2011, 27(9): 1328–1354. doi: 10.1016/j.ijplas.2011.03.001 [39] SHEN Z, WAGONER R H, CLARK W A T. Dislocation pile-up and grain boundary interactions in 304 stainless steel [J]. Scripta Metallurgica, 1986, 20(6): 921–926. doi: 10.1016/0036-9748(86)90467-9 [40] YUAN W, PANIGRAHI S K, SU J Q, et al. Influence of grain size and texture on Hall-Petch relationship for a magnesium alloy [J]. Scripta Materialia, 2011, 65(11): 994–997. doi: 10.1016/j.scriptamat.2011.08.028 [41] ZHOU G, JEONG W, HOMER E R, et al. A predictive strain-gradient model with no undetermined constants or length scales [J]. Journal of the Mechanics and Physics of Solids, 2020, 145: 104178. doi: 10.1016/j.jmps.2020.104178 -








 下载:
下载:


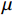
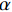
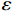












 下载:
下载: