Blasting Damage and Energy Characteristics of Rock Mass under High in-Situ Stress
-
摘要: 在深层岩体地下工程中，岩体的爆破效果与地应力密切相关。基于岩体-空气流固耦合模型，采用理论分析与LS-DYNA有限元数值模拟相结合的方式，研究了不同地应力和侧压系数条件下岩体爆破损伤效果以及岩体非弹性边界处能量及质点峰值振动速度阈值的变化规律。结果表明：岩体的损伤范围和裂纹扩展在一定程度上受到地应力的抑制作用，地应力越大，损伤范围和裂纹长度越小；不同地应力下，非弹性区与弹性区边界处的能量差随侧压系数的增大而减小，当侧压系数一定时，能量随地应力的增大而增大；高地应力状态下，利用质点峰值振动速度阈值判据进行岩体爆破安全控制是不可靠的。Abstract: In blasting excavation of deep rock mass engineering, the blasting effect is closely related to ground stress. Based on the rock mass-air fluid-solid coupling model, the blasting damage effect of rock mass under different in-situ stress and lateral pressure coefficient, and the variation rule of energy and peak particle velocity (PPV) threshold at inelastic boundary of rock mass were studied by combining theoretical analysis and LS-DYNA finite element numerical simulation. The results show that the damage range and crack propagation of rock mass are restrained by in-situ stress to a certain extent. The larger the in-situ stress is, the smaller the damage range and crack length will be. Under different ground stresses, the energy difference between the inelastic zone and the elastic zone decreases with the increase of the lateral pressure coefficient. When the lateral pressure coefficient is constant, the energy increases with the increase of ground stress. Under the condition of high in-situ stress, it is inaccurate to use the PPV threshold to determine the safety control of rock blasting.
-
Key words:
- in-situ stress /
- peak particle velocity /
- fluid-structure interaction /
- blasting effect /
- damage area
-
聚脲是由异氰酸酯组分和氨基化合物快速交联加聚反应而形成的一类高分子材料，因具有优异的力学性能[1-3]，受到国内外专家和学者的广泛关注，并开展了爆炸防护研究。相关研究[4-6]表明，聚脲涂层能够减弱钢板在爆炸荷载作用下的损坏程度，增强整体结构的抗爆能力。而对于混凝土结构，无论是爆破拆除，还是反恐防爆，减少混凝土破碎导致的损害是目前研究的热点。在聚脲加固钢筋混凝土结构方面，美国空军实验室研究聚合物加固墙体时发现，相较于碳纤维、芳纶纤维等其他众多高分子聚合物材料，聚脲在墙体结构爆炸防护中表现出更好的抗爆效果[7]。Parniani等[8]在此基础上研究发现，聚脲能够提高钢筋混凝土梁的抗弯能力和延性。Raman等[9]和Ghaderi等[10]通过数值模拟发现，聚脲涂层可以提升钢筋混凝土板的位移控制和能量耗散，降低结构的应变能及动能，通过降低位移和能量吸收、减弱爆炸能量传递来提高抗爆能力。Iqbal等[11]通过聚脲涂覆混凝土爆炸实验，发现聚脲能够包覆混凝土爆炸后产生的碎片，减少碎片对周围人员及物体造成的伤害。王军国[12]、尚伟等[13]对聚脲加固墙体进行了爆炸试验，发现有聚脲涂层加固的墙体具有良好的抗爆能力，且背爆面涂覆墙体在爆炸作用下没有产生爆炸碎片，表明聚脲的包覆效果良好。聚脲涂层厚度对结构的抗爆能力有着巨大影响，为了深入研究聚脲涂层厚度对墙体抗爆能力的影响，Tao等[14]开展了不同工况下聚脲加固墙体的爆炸实验，发现喷涂3 mm厚聚脲的墙体在爆炸荷载下损伤较小，只有轻微的凹陷变形，而喷涂4 mm厚聚脲的墙体没有破坏，显示出良好的防爆性能。田力等[15]、Gu等[16]运用ANASYS/LS-DYNA软件模拟了在爆炸荷载作用下不同厚度聚脲涂覆墙体的破坏情况，结果表明，随着聚脲涂层厚度的增加，墙体中心点位移明显减小，聚脲加固后墙体的抗爆能力提高。
目前，人们已经开展了聚脲涂层的抗爆研究，并取得了良好的效果，但是对于聚脲涂层抗爆机理的研究尚不够完善，需要对爆炸过程中聚脲的力学性能及爆炸后涂层的微观及宏观破坏形式进行深入研究，以确定两者之间的关联。为此，本研究选择青岛理工大学功能材料研究所自主研发的抗爆型T26聚脲，分析不同应变率下T26聚脲的力学性能；通过广角X射线衍射（wide-angle X-ray diffraction，WXRD）实验，研究材料的微观结构，并利用差示扫描量热（differential scanning calorimetry，DSC）实验分析其热性能，研究T26聚脲在高温条件下的形态及其特点；在此基础上，对喷涂T26聚脲前后的钢筋混凝土板进行接触爆炸实验，通过1.4 kg当量TNT下混凝土板的宏观破坏形貌，分析其破坏模式，并借助电子显微镜观察涂层的微观形态，结合其热性能结果，探讨涂层的爆炸防护规律，分析其抗爆机理。
1. 聚脲涂层材料制备与性能
1.1 涂层试样制备
T26聚脲是由青岛理工大学功能材料研究所针对抗爆要求专门研发的高强抗爆型聚脲。喷涂设备包括PHX-40主机和AP-2喷枪（美国聚氨酯机械公司生产），喷雾温度为65 ℃，压力为17.24 MPa。由于材料力学性能是缓慢提升的，因此实验前需要将试样在常温下养护7 d，保证其力学强度稳定。
1.2 涂层性能实验
1.2.1 涂层力学性能
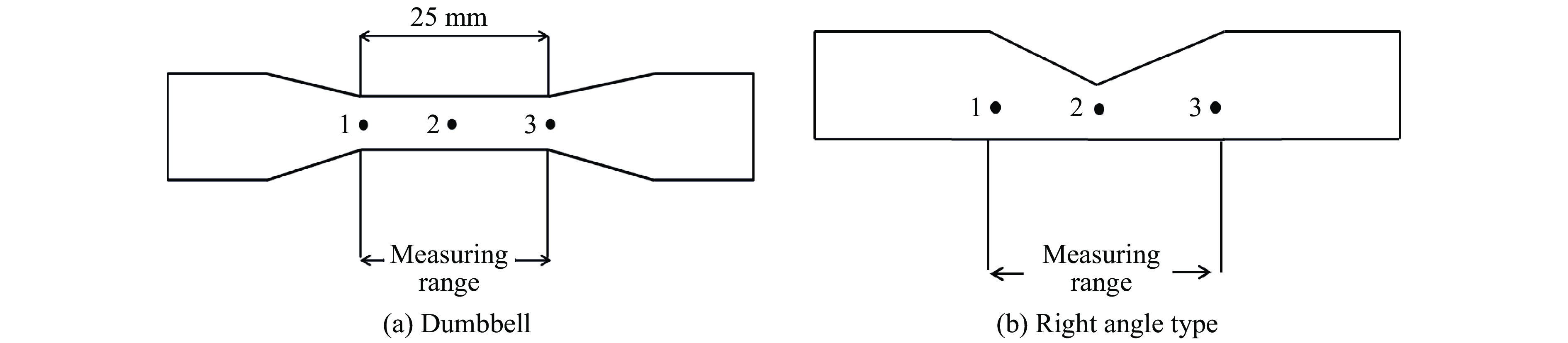
根据ASTM D412《硫化橡胶和热塑性弹性体拉伸试验方法》，将T26聚脲试样分别裁成无割口直角形与哑铃形。裁取哑铃形试样中间25 mm长区域作为测量区，在测量区内取两端及中间3点1、2、3（见图1(a)）进行厚度测量，取平均值并输入计算机端。将待测哑铃形试样两端用万能试验机夹具夹住，使受力方向与试样测量区间保持重合，夹具间距离设置为70 mm，调零后运行。拉伸试样直至断裂，得到其拉伸强度和断裂伸长率。取直角形样片中心及两边3点进行厚度测试（见图1(b)），求平均值，进行撕裂强度测试。
1.2.2 涂层WXRD实验
选取尺寸为2 cm×2 cm的聚脲样片，将其固定在测角仪的载玻片上，采用RINT2000 vertical goniometer测角仪，对样片表面进行测试，得到衍射峰，并计算结晶度。扫描速度为10°/min，精度为 ± 0.02°，扫描范围为5°～80°。
1.2.3 涂层热性能
称取T26聚脲样品12.2 mg，利用NETZSCH DSC 204 F1差示扫描量热仪（见图2）对样品进行测量。为减小实验误差，在氮气条件下进行DSC测试，升温速率为10 ℃/min，温度范围为−100～800 ℃。
2. 涂层抗爆特性预估
2.1 涂层密度和力学性能
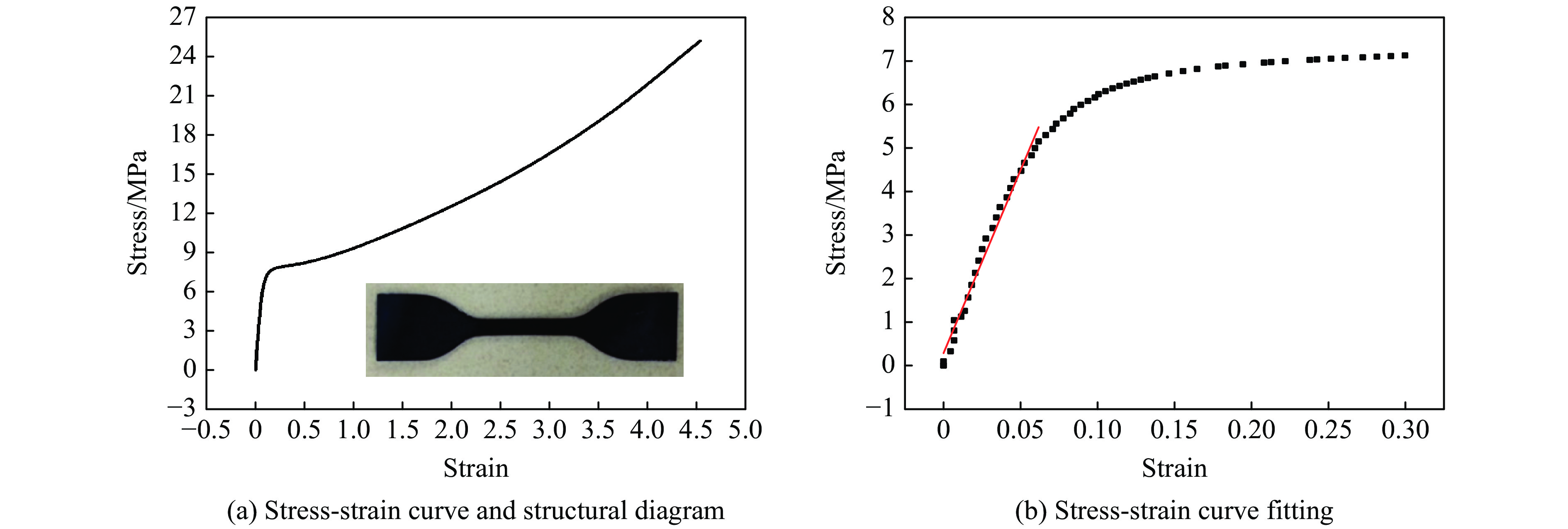
T26聚脲是传统聚脲配方优化调整后的高强抗爆型聚脲材料，T26聚脲的密度在1 g/cm3以下（详见表1）。对T26聚脲进行准静态拉伸实验，其应力-应变曲线及材料试件如图3(a)所示；对于该曲线的弹性阶段，采用一次函数y=0.493+79.729x进行拟合，得到材料的应力-应变曲线拟合情况，如图3(b)所示。所测得的T26聚脲的基本力学性能如表1所示。
表 1 T26聚脲与其他聚脲材料的物理性能对比Table 1. Physical properties comparison of T26 polyurea with other polyureasMaterial Density/
(g·cm−3)Elastic modulus/
MPaTensile strength/
MPaPeel strength/
(N·mm−1)Breaking elongation/
%T26 0.977 84.05 25.4 75.5 451.9 T1[13] 1.020 19.2 391.7 T2[17] 1.02−1.07 18.0 350.0 Note: (1) T1 is energy absorbing polyurea for strengthening masonry wall explosion test;
(2) T2 is common polyurea for explosion test of reinforced autoclaved aerated concrete plate.
图 3 准静态下T26聚脲的应力-应变曲线及试件结构Figure 3. Stress-strain curve of T26 polyurea under quasi-static loading and the specimen structure由准静态拉伸实验结果可知，T26聚脲的拉伸强度达到25.4 MPa，断裂伸长率为451.9%，相较于T1、T2两种聚脲，其抗拉强度和断裂伸长率均大幅增加。炸药产生的爆炸荷载可看作均布荷载作用到板表面，由于混凝土板的抗压强度远大于其抗拉强度，需要涂层承受较大的拉力作用，T26涂层的抗拉强度高，可以起到更好的保护作用；同时T26聚脲具有较高的断裂伸长率，说明T26聚脲涂层具有良好的柔性，可以更好地包裹混凝土板，降低碎片飞溅的速度。
2.2 涂层WXRD分析
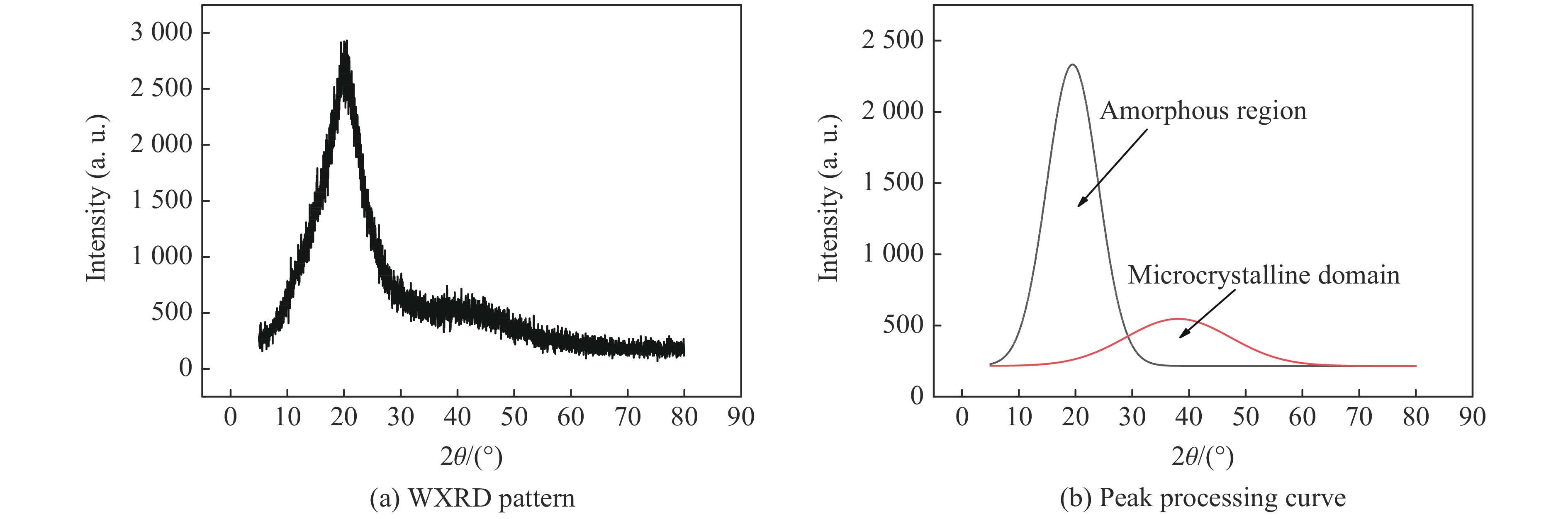
图4为T26聚脲的WXRD图谱及分峰处理结果。从图4(a)中可以发现，在2
θ =20.52°附近出现较强的软段非晶漫散射峰，材料链段更趋向于规整有序排列或空间折叠结构，表明材料中的脲键作为分子链中的硬段部分，达到了远程有序，产生结晶。WXRD分峰处理结果如图4(b)所示，具体数据列于表2。T26聚脲微晶区位于38°附近，无定形区位于20°附近，结晶度（结晶峰面积与总的峰面积的比值）较高，达到24.11%，说明材料分子链中氢键较多。氢键具有易断裂、易形成的特点，并且氢键的作用力大于原子间作用力，小于化学键的作用力。随着氢键的断裂与形成，材料表现出优异的吸能效率，从宏观上看，有序规整的分子结构更有利于材料形成优异的力学性能。表 2 T26聚脲的WXRD分峰处理结果Table 2. WXRD peak separation results of T26 polyureaSpecimen Peak position/(°) Peak area Crystallinity/% Amorphous region Microcrystalline domain Amorphous region Microcrystalline domain T26 20.52 38.16 24042.85 7638.57 24.11 2.3 涂层热性能分析
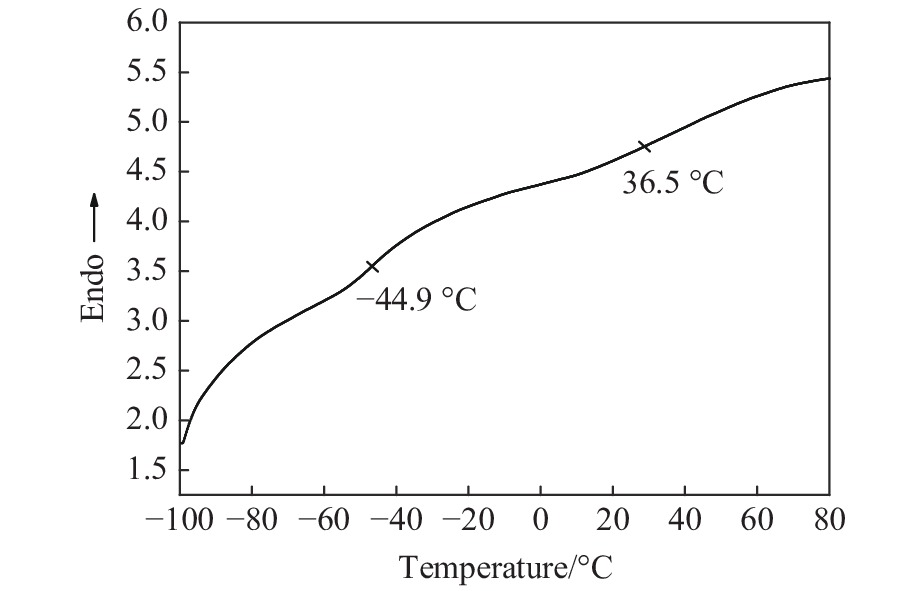
图5为T26聚脲在−100～80 ℃范围内的DSC曲线。从图5中可以得到，材料的软段玻璃化转变温度（Tgs）为−44.9 ℃，硬段玻璃化转变温度（Tgh）为36.5 ℃，说明T26聚脲分子链为一定的微相分离结构，软段与硬段之间排列有序。在−100～80 ℃温度范围内，T26聚脲材料没有出现明显的吸热和放热峰，说明在较高的温度环境下未发生较大的变化，对高温环境的适应性较强。
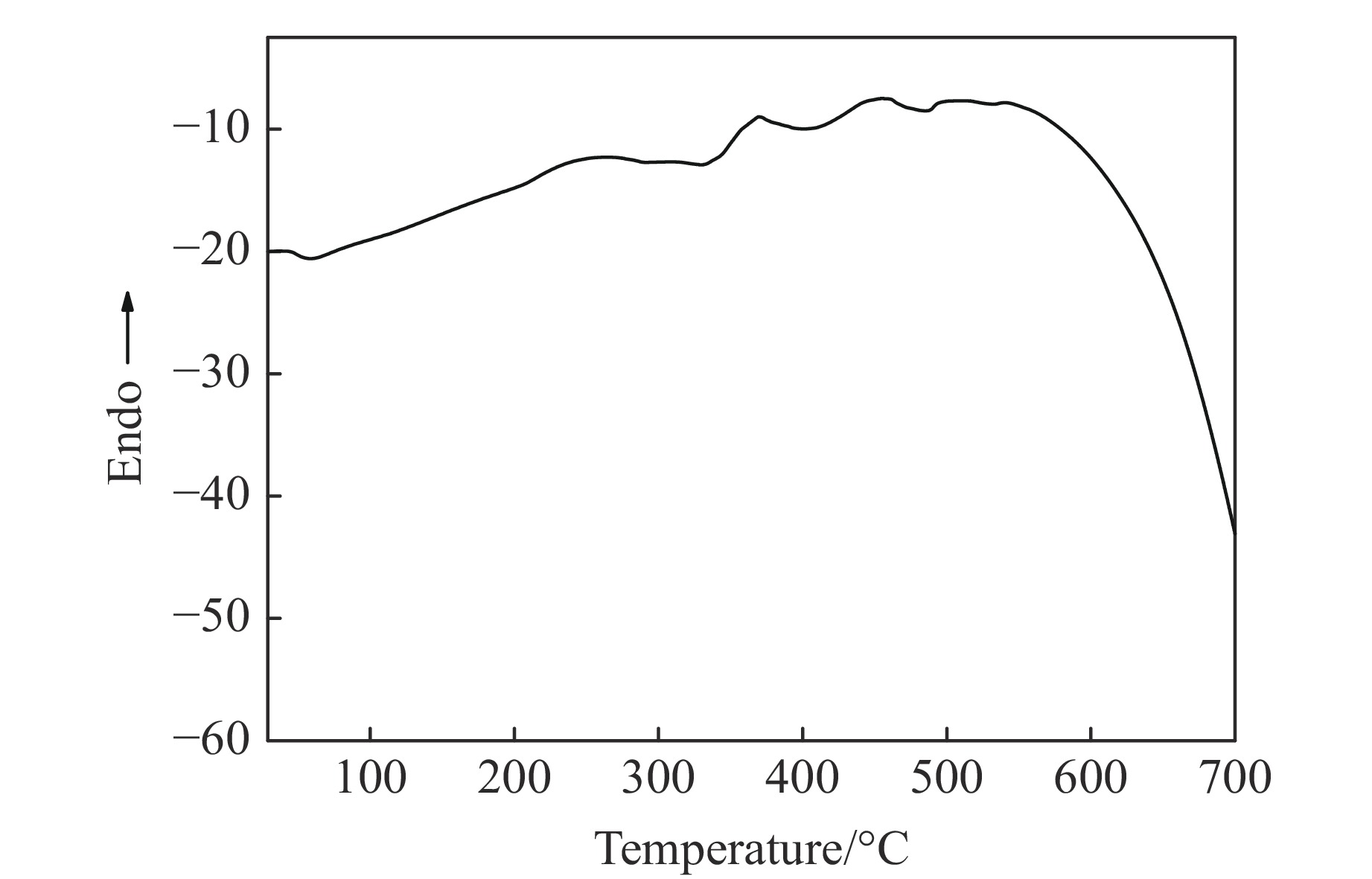
由于爆炸产生的热量极高，因此需要对T26聚脲在更高的温度下进行测试，为此选择30～700 ℃的温度范围进行DSC实验，如图6所示。从图6中可以看出，随着温度的升高，375 ℃附近出现结晶熔融吸热峰，说明T26聚脲中存在结晶，与WXRD实验结果一致，因此材料可以在高温下通过硬段域内氢键的断裂吸收能量，产生结晶熔融吸热峰。材料分子链中的软段决定着材料的柔性，硬段决定着材料自身的强度。在爆炸过程中，炸药会瞬间释放能量，产生高温高热，T26聚脲在高温下会发生结晶熔融，通过涂层的结晶熔融吸收能量。同时，在高温环境下，T26聚脲材料的自由体积开始膨胀，分子链段运动变得活跃并逐渐舒展，材料更加柔软。在爆炸中，良好的柔韧性使T26聚脲能够包覆混凝土板表面，黏结爆炸碎片，减少爆炸碎片对周围环境造成的危害。
3. 抗爆实验验证
3.1 聚脲涂覆钢筋混凝土板的爆炸实验
3.1.1 实验方案及试样喷涂
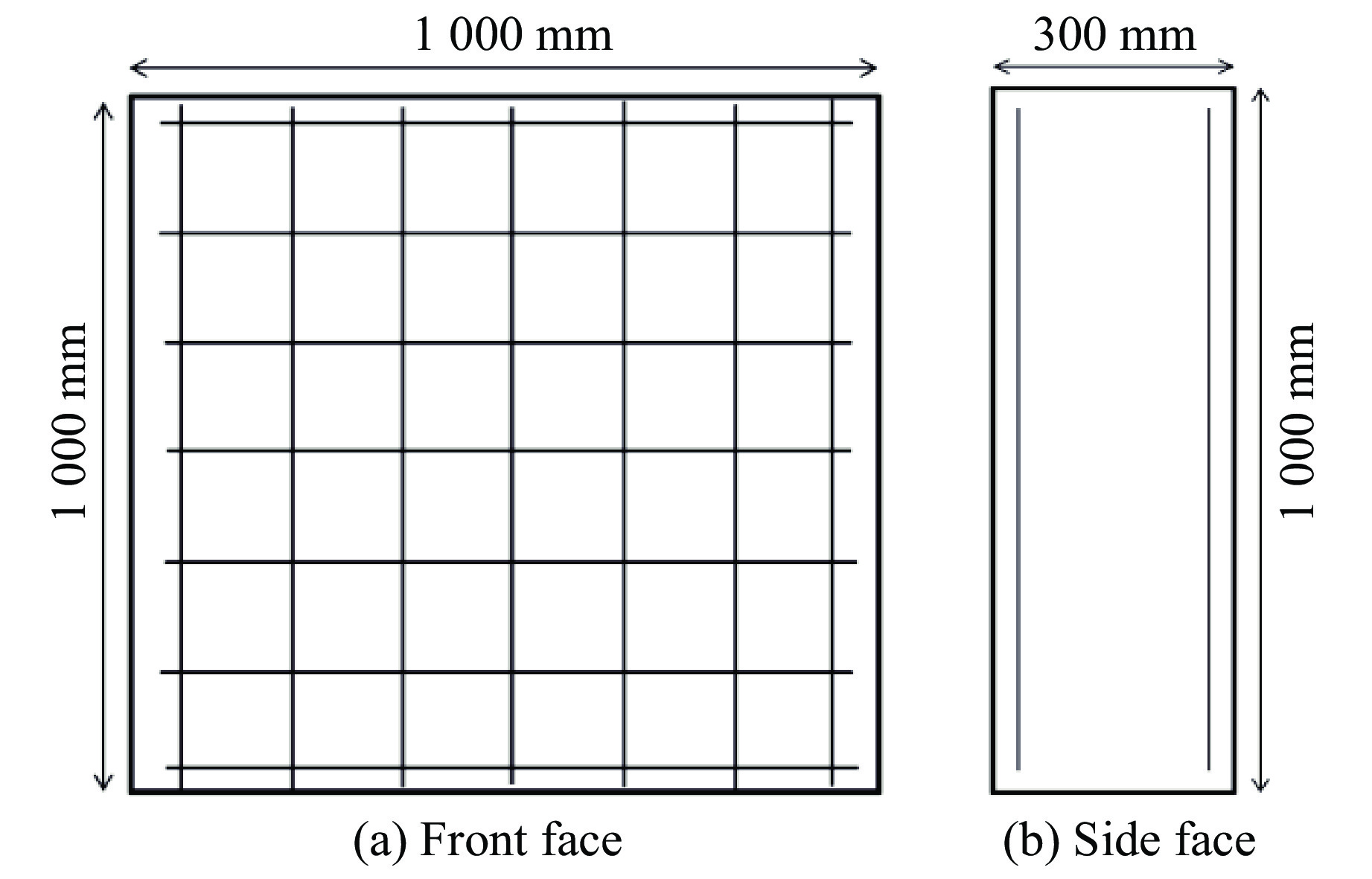
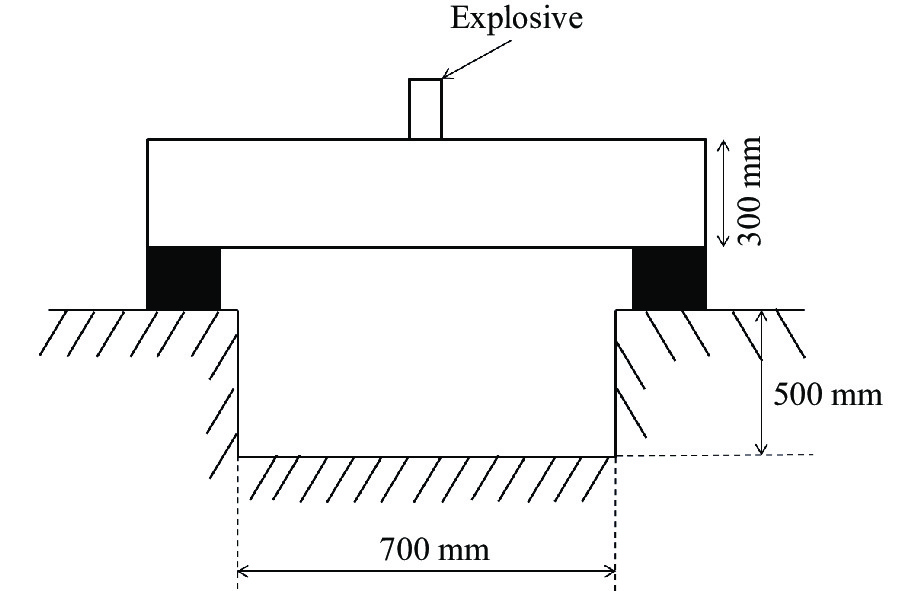
按照GB50010—2010《混凝土结构设计规范》，混凝土强度等级为C40，板采用双层配筋，钢筋间距为150 mm，直径为10 mm，保护层厚度为15 mm，钢筋混凝土板的尺寸为1000 mm×1000 mm×300 mm，如图7所示。
T26聚脲涂层与混凝土基材良好的附着至关重要。在喷涂之前，需要将环氧改性聚氨酯底漆涂抹在基材表面上，这是由于：(1) 底漆的封闭能力较好，可以降低基材中的针眼缺陷；(2) 底漆可以将残留在基材表面的灰尘牢固地粘在基材表面，避免因灰尘导致的涂层缺陷；(3) 底漆的渗透能力较强，能同时与混凝土和聚脲涂层紧密黏结，增强涂层的附着力。底漆选用青岛理工大学功能材料研究所自主研发的环氧改性聚氨酯底漆，附着力强度可达到5 MPa。
利用喷涂设备将聚脲喷涂到钢筋混凝土板表面，采用全面喷涂方式，涂层厚度10 mm。将经T26聚脲喷涂处理后的钢筋混凝土板养护7 d后，安置在约束固定架上，背爆面侧挖深度为0.5 m、宽度为0.7 m的爆炸坑，炸药放置在钢筋混凝土板的上表面，进行有无涂层钢筋混凝土板的爆炸实验，TNT药量为1.4 kg，如图8所示。
3.1.2 爆炸后钢筋混凝土宏观形貌
根据实验方案（见表3），比较有无涂层钢筋混凝土板爆炸后迎爆面与背爆面的损伤形式。设置工况3为对照组，分析钢筋混凝土板迎爆面与背爆面T26涂层的防护效果。
表 3 宏观形貌分析方法Table 3. Analysis method of macroscopic morphologyExp. condition Coating Coating thickness/mm Failure plane Macroscopic morphology comparison 1 None 0 Contact blast face Failure mode and diameter Back burst face Failure mode and diameter 2 T26 10 Contact blast face Failure mode and diameter Back burst face Failure mode and diameter 3.1.3 爆炸后涂层的微观形貌
选取爆炸后不同爆炸区域的T26涂层，利用JSM-7500F扫描电子显微镜对爆炸后样品进行放大4000倍的微观形貌观察。该仪器的分辨率为1.0 nm（15 kV）和1.4 nm（1 kV），加速电压为0.1～30 kV，电子枪使用钨丝灯，放大倍数为2000。
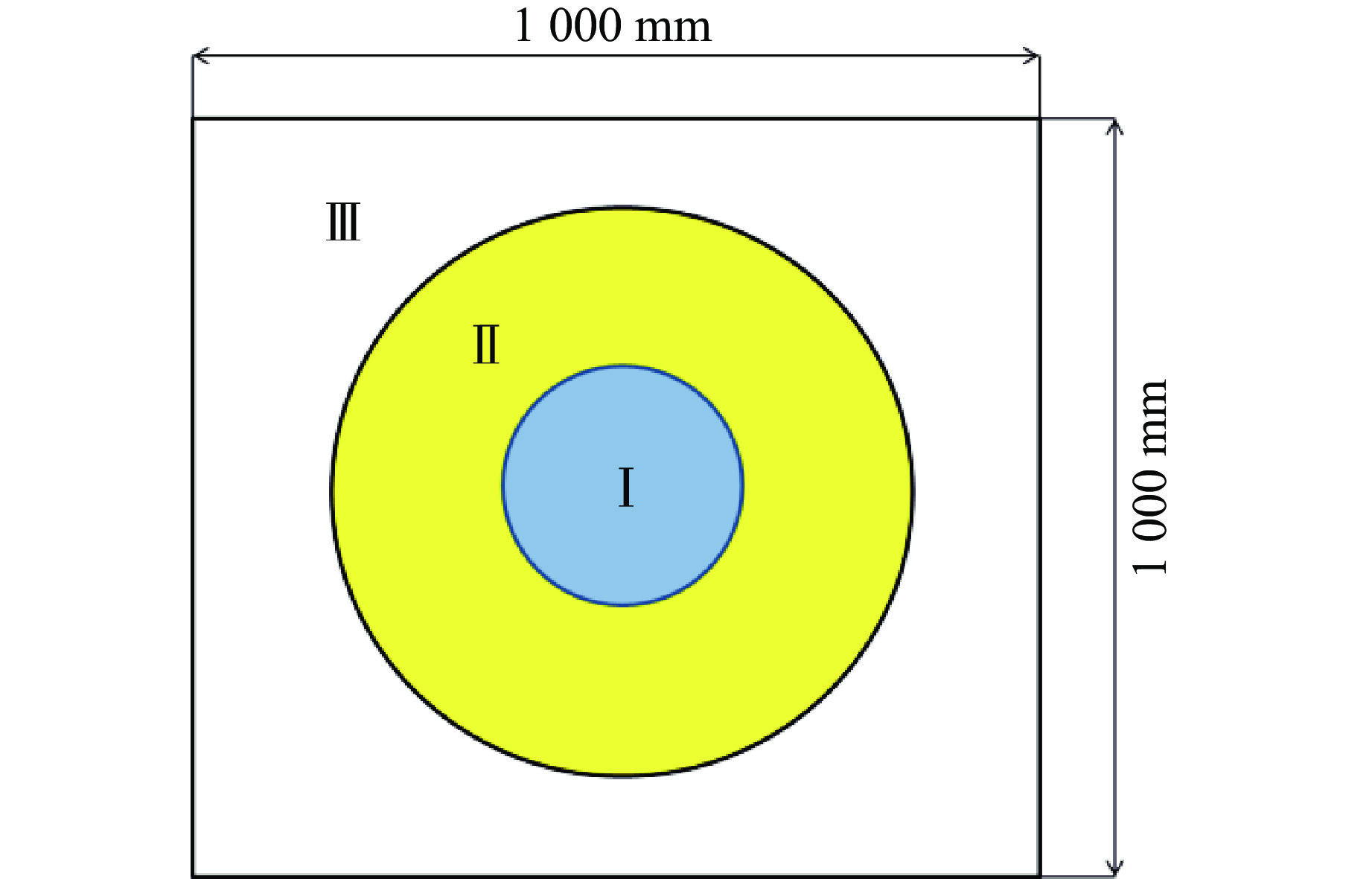
将爆炸破坏后的T26聚脲涂层划分为3个区域，分别为断面区域Ⅰ、爆炸源中心区域Ⅱ及涂层边缘区域Ⅲ，如图9所示。分别裁取3个区域涂层，借助扫描电子显微镜进行微观形貌观察，研究爆炸冲击波在涂层爆炸核心区及边缘区域的传播规律以及对涂层微观结构的影响。
3.2 爆炸后形貌
3.2.1 宏观形貌
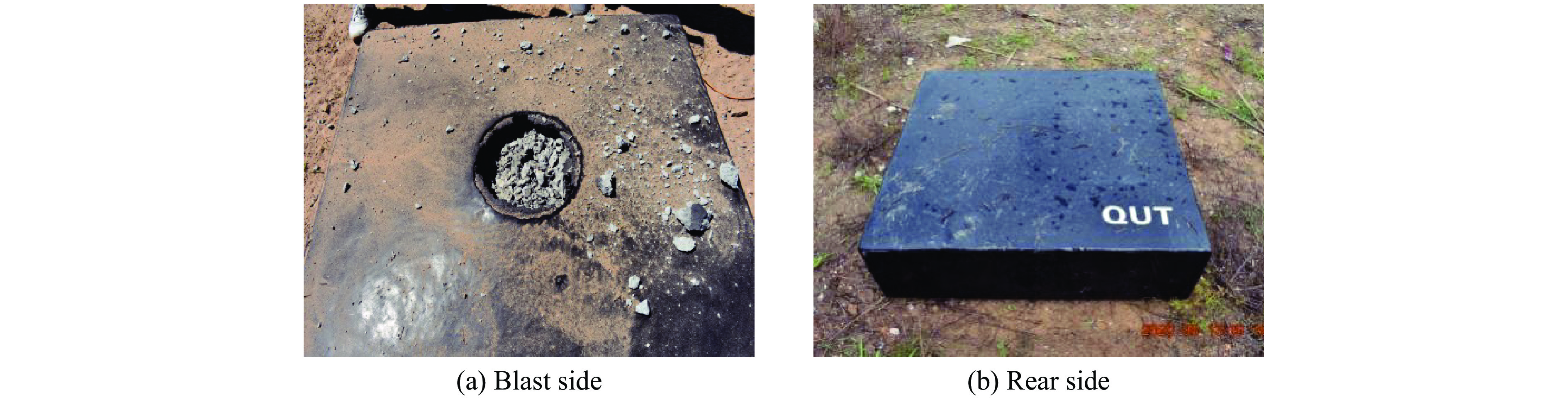
图10为无涂层钢筋混凝土板的破坏情况。通过对比可以发现，无涂层钢筋混凝土板迎爆面（图10(a)）中心区域发生严重破坏，出现爆炸凹坑，混凝土破碎并裸露出钢筋，沿凹坑边缘向四周扩散大量裂缝；观察图10(b)发现，无涂层板背爆面表面混凝土发生大面积破碎，一些破片发生脱落，钢筋发生弯曲并凸出混凝土保护层，内部混凝土损坏成块，出现大量裂缝沿破坏区向四周扩散，无涂层板发生大面积震塌破坏，完全失去承载力。爆炸冲击波可近似看成由中心点向外扩散，在板迎爆面中心处产生极大能量，使板产生较大凹坑后，穿透整个混凝土板，导致板内部破碎，背爆面震塌。
图 10 无涂层钢筋混凝土板的破坏形貌Figure 10. Failure morphology of uncoated reinforced concrete plate图11为有涂层钢筋混凝土板背爆面破坏情况。从图11中可以发现，T26涂层钢筋混凝土板迎爆面（图11(a)）中心区域发生破坏，凹坑边缘涂层向上凸起，破坏区域近似圆形，凹坑内混凝土破碎，并且从凹坑内飞溅出爆炸碎片。在破坏凹坑边缘，涂层发生剥离，涂层出现向上凸起的现象；而有涂层板背爆面完好（图11(b)），聚脲涂层没有出现破坏，涂层没有明显凸起，结构背爆面平整。相较于无涂层钢筋混凝土板，T26涂层钢筋混凝土板的凹坑破坏较规则，破坏程度减轻，并且背爆面的聚脲涂层能完全包覆住钢筋混凝土板的破坏碎片，极大地减少爆炸碎片飞溅造成的伤害。综合来看，对于迎爆面涂层，除了瞬间高温导致聚脲软化，爆炸反射波的稀疏拉伸作用也会使聚脲材料发生破坏，导致涂层材料发生撕裂；而对于背爆面涂层，由于聚脲涂层削弱了爆炸冲击拉伸波的作用，从而保护混凝土材料不被破碎，防止爆炸碎片飞溅。
为了深入探讨T26聚脲涂层的抗爆效果，对有无涂层钢筋混凝土板的破坏区域进行测量，结果见表4。从表4中可以得到：无涂层板迎爆面凹坑最大破坏区域直径达到41.0 cm，破坏深度为11.2 cm，而有涂层板迎爆面破坏直径为24.5 cm，破坏深度为9.4 cm。相较于无涂层板，有涂层板的迎爆面破坏程度大大减弱，破坏直径减小了40.24%，破坏深度减小了16.07%。无涂层板背爆面破坏直径达到60.0 cm，破坏凸起高度为5.0 cm，而有涂层板背爆面则表现良好，没有发生破坏和凸起。从碎片情况来看，有涂层板无论是迎爆面还是背爆面，碎片均减少，并且背爆面没有产生碎片。可见，T26聚脲涂层能够减弱钢筋混凝土板的破坏程度，背爆面涂层的抗爆能力比迎爆面更好，减少碎片的能力也更优。
表 4 有无涂层板的破坏结果对比Table 4. Comparison of failure results of coated plates with uncoated platesPlate Damage diameter Failure depth or bulge height Uncoating/cm Coating/cm Error/% Uncoating/cm Coating/cm Error/% Blast side 41.0 24.5 40.24 60.0 0 100 Rear side 11.2 9.4 16.07 5.0 0 100 Debris situation More and wide
dispersion rangeLess and more
concentrated
in pitsLarger, larger
splash rangeNo debris 3.2.2 微观形貌
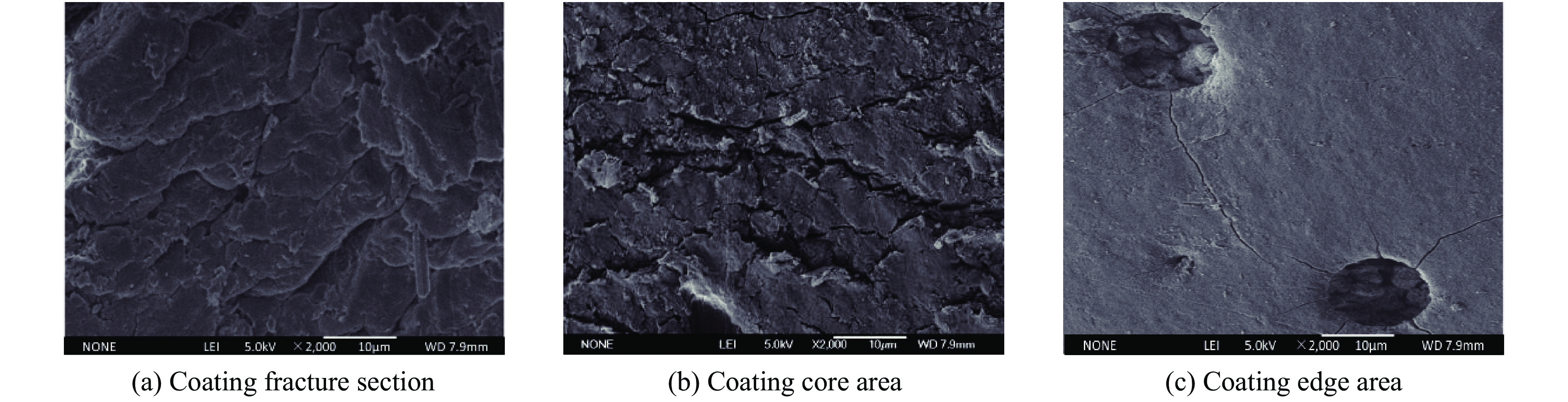
图12显示了爆炸后T26聚脲涂层区域Ⅰ、区域Ⅱ和区域Ⅲ的局部微观形貌（放大2000倍）。从图12(a)可以看出，断面区域表面呈鳞片状分散式分布，表面排布错乱不平整，并且伴有较多撕裂层及少量的脆性破坏。可见，聚脲涂层部分区域因爆炸产生的高温而发生熔融，同时强大的冲击波使聚脲涂层产生撕裂破坏，爆炸结束后，温度瞬间降低，导致聚脲从玻璃态转变为黏弹态，断裂的聚脲固化成鳞片状附着在断面表层。观察图12(b)发现，聚脲涂层断面不均匀，组织致密，发生因较大塑性变形导致的明显的撕裂破坏，并且伴有较多裂缝，部分区域出现熔融现象。可见，聚脲在抵抗爆炸作用时受到高温和强大的冲击波作用，导致聚脲涂层表面发生不同程度的撕裂破坏及大量裂缝。从图12(c)可以看出，涂层边缘区域表面较光滑，没有发生熔融及撕裂破坏，说明边缘涂层受爆炸荷载作用较小，爆炸产生的大部分能量均在中心处释放，但是出现较多微小裂纹，并且裂纹由涂层凹陷处向四周延伸。图12(c)中凹陷处形状较规则，可能是由于原料中的气泡在喷涂过程中被破坏，导致涂层表面形成圆孔缺陷。在爆炸形成的冲击力下，圆孔处强度较低，导致裂纹从圆孔处向四周扩展，因此，在聚脲的喷涂过程中应尽量减少气泡的产生。
图 12 爆炸后T26聚脲涂层的微观形貌Figure 12. Micromorphology of T26 polyurea coating after explosion4. 抗爆机理验证分析
当爆炸冲击波从钢筋混凝土板迎爆面传播至背爆面时，板的破坏以爆炸凹坑、爆炸震塌、爆炸贯穿和爆炸冲切4种破坏模式为主[18-19]。本研究中钢筋混凝土板的破坏以迎爆面爆炸凹坑及背爆面爆炸震塌为主：无涂层板的迎爆面出现爆炸凹坑破坏，背爆面则完全震塌，混凝土成块脱落；有涂层板的迎爆面出现爆炸凹坑，而背爆面没有破坏，涂层完好无损。
爆炸能够瞬间产生大量的热量和强大的冲击能量，以波的形式向周围传播，可以穿透钢筋混凝土板造成其局部或整体破坏。观察爆炸后涂层的微观形貌可以发现，在强大的爆炸冲击波下涂层的破坏以撕裂破坏为主，并伴有少量脆性破坏，可见材料在高温高热下会转变为玻璃态，表现为刚性。通过对T26聚脲的WXRD和DSC分析可知，T26聚脲的分子链是一种微相分离结构，软段和硬段之间的分离程度较大并且排列有序，导致聚脲既有足够的力学强度，又能表现出类似于橡胶的柔软性。同时，随着温度的不断升高，T26聚脲会结晶和熔融，从而吸收热量，导致材料的自由体积逐渐膨胀，分子链段运动变得活跃并逐渐舒展；此外，T26聚脲的断裂伸长率较高，对于背爆面上的涂层来说，涂层承受的温度相对较低，仍保持其黏弹性行为，能够在爆炸荷载作用下通过自身的变形起到防护作用，包覆产生的爆炸碎片，达到“以柔克刚”的效果。
由爆炸应力波的破岩过程可知，在炸药爆炸初期，强烈的高温高压作用在迎爆面涂层，使涂层产生熔融和破坏，导致混凝土板形成粉碎区，此时主要表现为强冲击波的超高压对结构施加冲击压缩破坏效应[20]。由于粉碎区消耗冲击波的部分能量，使冲击波衰减成压应力波，压应力波继续传播使背爆面涂层主要承受拉应力，产生拉伸变形。因此，对于迎爆面涂层，需要承受更大的爆炸能量，由有涂层钢筋混凝土的接触爆炸实验可以知道，迎爆面涂层会出现穿孔破坏，通过自身的大变形吸收能量，首次减弱冲击波的强度，降低传播的能量。当爆炸冲击波经混凝土传播至背爆面涂层时，能量再次衰减，背爆面涂层能够承受剩余的能量，通过自身的变形使能量完全耗散，并能完全包裹混凝土破坏产生的碎片，起到良好的防震塌效果。迎爆面涂层和背爆面涂层的两次作用使钢筋混凝土板保持良好的完整性及稳定性，对钢筋混凝土板的抗爆能力具有重要意义。
综上研究可以发现，聚脲材料的抗爆机理与其自身热性能及力学性能密切相关。材料分子链段中硬段与软段的分离程度决定其力学性能，分离程度越高，力学性能越优异，抗爆效果越明显。此外，在抵抗爆炸荷载的过程中，迎爆面涂层和背爆面涂层分别依赖于其不同的力学特点：迎爆面涂层通过更高的强度和吸能效率吸收爆炸应力波，减弱反射波，从而抵抗爆炸能量；而背爆面涂层则需要更加柔软，通过橡胶态的性质包裹爆炸碎片，极大地减弱混凝土板的震塌损伤。
5. 结 论
(1) 对T26聚脲进行了准静态力学实验，得到T26聚脲的抗拉强度为25.4 MPa，撕裂强度为75.5 N/mm，断裂伸长率为451.9%，表现出更高的力学强度。通过WXRD和DSC得到，材料分子链是微相分离结构，软段与硬段之间排列规整有序，结晶度为24.11%；软段玻璃化转变温度为−44.9 ℃，硬段玻璃化转变温度为36.5 ℃。
(2) 对10 mm厚T26聚脲涂层进行1.4 kg TNT当量的接触爆炸，结果表明：无涂层钢筋混凝土板出现爆炸凹坑及爆炸震塌破坏，凹坑的最大直径为41.0 cm，最大深度为11.2 cm，背爆面破坏区域的最大直径为60.0 cm，板结构失去整体稳定性，并产生大量的爆炸碎片。T26聚脲涂覆钢筋混凝土板出现爆炸凹坑破坏，凹坑中心区的最大破坏直径为24.5 cm，最大深度为9.4 cm，背爆面涂层没有发生破坏和凸起，T26涂层能够良好地包裹爆炸碎片，降低碎片飞溅的伤害。综合来看，对于迎爆面涂层，除了瞬间高温导致聚脲软化，还有爆炸反射波的稀疏拉伸作用也使聚脲材料发生破坏，导致涂层材料发生撕裂；对于背爆面涂层，由于聚脲涂层削弱了爆炸冲击拉伸波的作用，从而保护混凝土材料不被破碎，防止爆炸碎片飞溅。
(3) 涂层的抗爆机理与其力学性能及热性能密切相关。T26聚脲具有优异的力学性能、良好的热稳定性以及较高的结晶度，能够在温度升高时产生熔融吸热峰，导致材料内部分子链断裂及重新组合，消耗部分能量，最终使其能够承受爆炸产生的荷载与能量的多方面耦合作用。迎爆面和背爆面涂层表现出不同的防护方式，迎爆面涂层通过变形吸收能量，背爆面涂层则展现出更好的包覆能力。
-
图 2 t=2 ms时无地应力岩体爆破损伤区域示意图
Figure 2. Schematic diagram of blasting damage area of rock mass without in-situ stress at t=2 ms
图 3 不同地应力下岩体的损伤云图
Figure 3. Damage cloud map of rock mass under different in-situ stresses
图 7 损伤度与爆心距的关系
Figure 7. Relationship between damage degree and the distance to the blast center
图 9 不同地应力下损伤度与PPV的关系
Figure 9. Relationship between damage degree and PPV under different in-situ stresses
表 1 岩石模型材料参数
Table 1. Rock material parameters in model
ρ/(g⋅cm−3) E0/GPa μ σ/GPa Etan/GPa βr 2.7 6.2 0.22 0.5 0.02 0.6 
下载: 导出CSV
表 2 炸药的力学参数
Table 2. Mechanical parameters of explosive
ρ0/(g·cm−3) D/(m·s−1) pmax/GPa A/GPa B/GPa R1 R2 ω e0/GPa 1.18 5122 9.53 276.2 8.44 5.2 2.1 0.5 3.87
下载: 导出CSV
表 3 工况参数
Table 3. Parameters of working conditions
Case No. ξ σx/MPa σy/MPa Case No. ξ σx/MPa σy/MPa 1 0 0 0 8 2 45 22.5 2 1 15 15.0 9 2 60 30.0 3 1 30 30.0 10 3 15 5.0 4 1 45 45.0 11 3 30 10.0 5 1 60 60.0 12 3 45 15.0 6 2 15 7.5 13 3 60 20.0 7 2 30 15.0
下载: 导出CSV
-
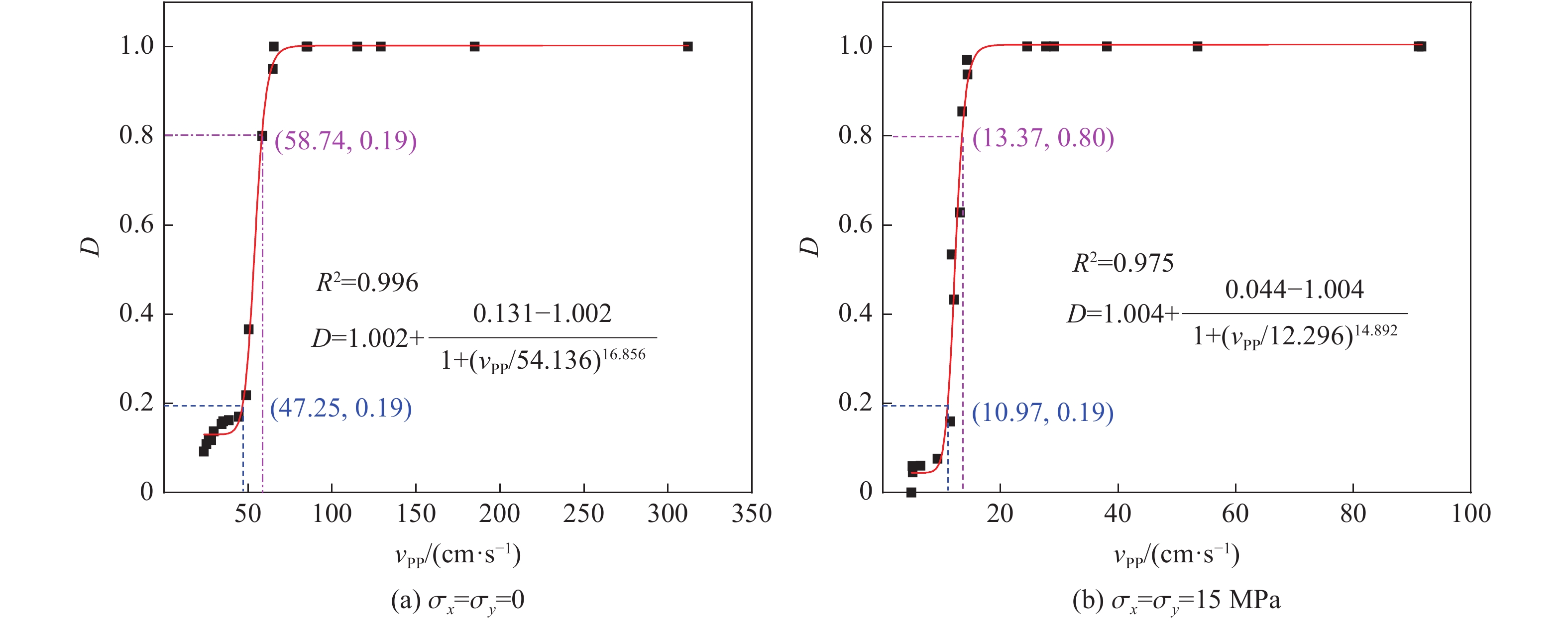
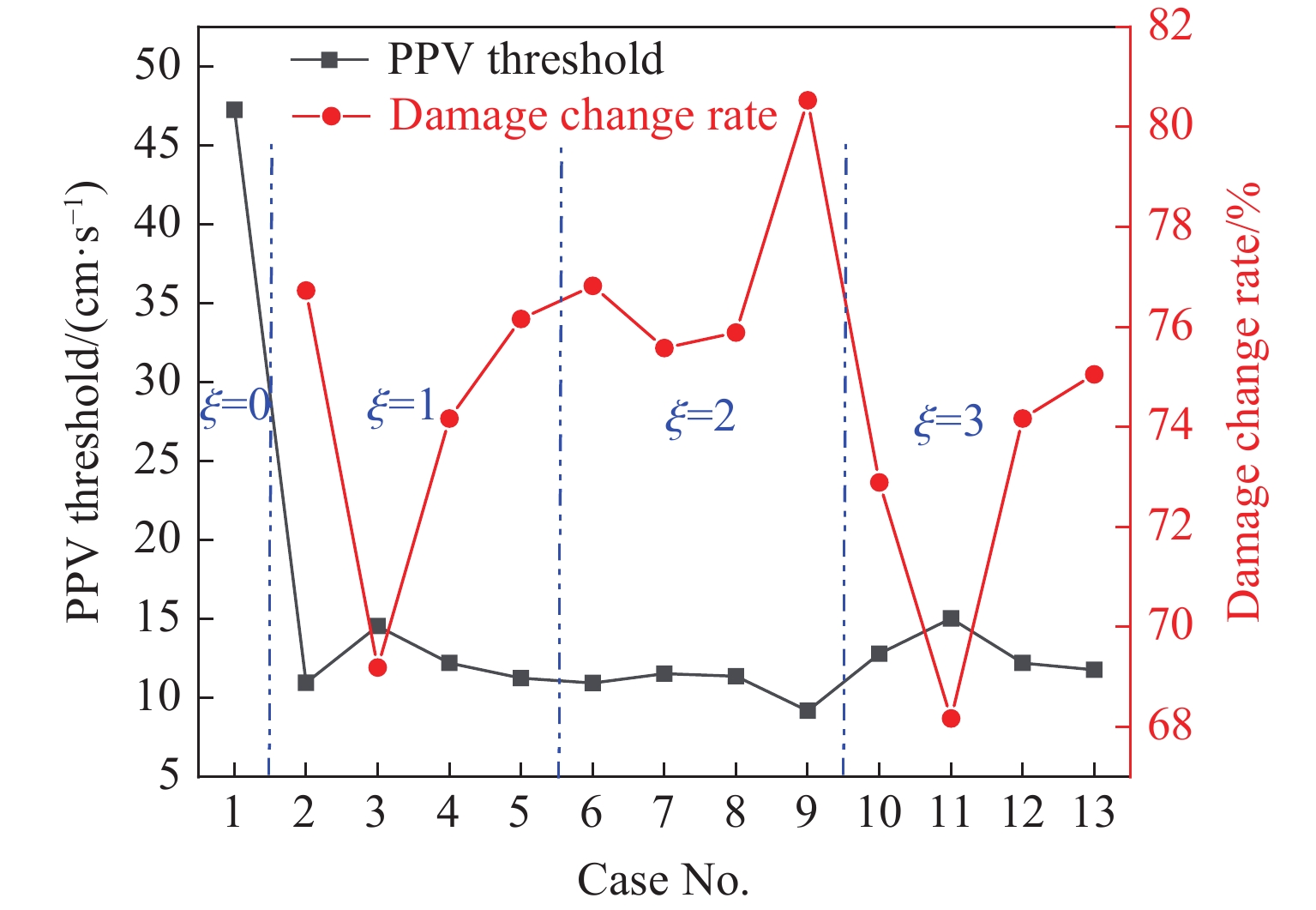
[1] BHANDARI S. On the role of stress waves and quasi-static gas pressure in rock fragmentation by blasting [J]. Acta Astronautica, 1979, 6(3/4): 365–383. doi: 10.1016/0094-5765(79)90104-8 [2] PAINE A S, PLEASE C P. An improved model of fracture propagation by gas during rock blasting: some analytical results [J]. International Journal of Rock Mechanics and Mining Sciences & Geomechanics Abstracts, 1994, 31(6): 699–706. doi: 10.1016/0148-9062(94)90009-4 [3] 刘殿书, 王万富, 杨吕俊, 等. 初始应力条件下爆破机理的动光弹实验研究 [J]. 煤炭学报, 1999, 24(6): 612–614. doi: 10.3321/j.issn:0253-9993.1999.06.012LIU D S, WANG W F, YANG L J, et al. Holophotoelasticity study on mechanism of blasting under initiative stress field [J]. Journal of China Coal Society, 1999, 24(6): 612–614. doi: 10.3321/j.issn:0253-9993.1999.06.012 [4] 肖正学, 张志呈, 李端明. 初始应力场对爆破效果的影响 [J]. 煤炭学报, 1996, 21(5): 497–501. doi: 10.13225/j.cnki.jccs.1996.05.011XIAO Z X, ZHANG Z C, LI D M. The influence of initial stress field on blasting [J]. Journal of China Coal Society, 1996, 21(5): 497–501. doi: 10.13225/j.cnki.jccs.1996.05.011 [5] 肖正学, 张志呈, 郭学彬. 断裂控制爆破裂纹发展规律的研究 [J]. 岩石力学与工程学报, 2002, 21(4): 546–549. doi: 10.3321/j.issn:1000-6915.2002.04.019XIAO Z X, ZHANG Z C, GUO X B. Research on crack developing law of rock fracture controlled blasting [J]. Chinese Journal of Rock Mechanics and Engineering, 2002, 21(4): 546–549. doi: 10.3321/j.issn:1000-6915.2002.04.019 [6] 陈明, 卢文波, 周创兵, 等. 初始地应力对隧洞开挖爆生裂隙区的影响研究 [J]. 岩土力学, 2009, 30(8): 2254–2258. doi: 10.3969/j.issn.1000-7598.2009.08.009CHEN M, LU W B, ZHOU C B, et al. Influence of initial in-situ stress on blasting-induced cracking zone in tunnel excavation [J]. Rock and Soil Mechanics, 2009, 30(8): 2254–2258. doi: 10.3969/j.issn.1000-7598.2009.08.009 [7] 戴俊. 深埋岩石隧洞的周边控制爆破方法与参数确定 [J]. 爆炸与冲击, 2004, 24(6): 493–498. doi: 10.3321/j.issn:1001-1455.2004.06.003DAI J. The controlled contour blasting technique and its parameter determination for rock tunnel at depth [J]. Explosion and Shock Waves, 2004, 24(6): 493–498. doi: 10.3321/j.issn:1001-1455.2004.06.003 [8] 王长柏, 李海波, 谢冰, 等. 岩体爆破裂纹扩展影响因素分析 [J]. 煤炭科学技术, 2010, 38(10): 31–34, 61. doi: 10.13199/j.cst.2010.10.38.wangzhb.036WANG C B, LI H B, XIE B, et al. Analysis on influencing factors of blasting crack expansion [J]. Coal Science and Technology, 2010, 38(10): 31–34, 61. doi: 10.13199/j.cst.2010.10.38.wangzhb.036 [9] 高全臣, 赫建明, 冯贵文, 等. 高应力岩巷的控制爆破机理与技术 [J]. 爆破, 2003, 20(Suppl 1): 52–55.GAO Q C, HE J M, FENG G W, et al. Mechanism and technology of controlled blasting for high stress rock tunneling [J]. Blasting, 2003, 20(Suppl 1): 52–55. [10] DALLY J W, FOURNEY W L, HOLLOWAY D C. Influence of containment of the bore hole pressures on explosive induced fracture [J]. International Journal of Rock Mechanics and Mining Sciences & Geomechanics Abstracts, 1975, 12(1): 5–12. doi: 10.1016/0148-9062(75)90737-8 [11] 穆朝民, 潘飞. 煤体在爆炸荷载和地应力耦合作用下裂纹扩展的数值模拟 [J]. 高压物理学报, 2013, 27(3): 403–410. doi: 10.11858/gywlxb.2013.03.014MU C M, PAN F. Numerical study on the damage of the coal under blasting loads coupled with geostatic stress [J]. Chinese Journal of High Pressure Physics, 2013, 27(3): 403–410. doi: 10.11858/gywlxb.2013.03.014 [12] 朱万成, 左宇军, 尚世明, 等. 动态扰动触发深部巷道发生失稳破裂的数值模拟 [J]. 岩石力学与工程学报, 2007, 26(5): 915–921. doi: 10.3321/j.issn:1000-6915.2007.05.007ZHU W C, ZUO Y J, SHANG S M, et al. Numerical simulation of instable failure of deep rock tunnel triggered by dynamic disturbance [J]. Chinese Journal of Rock Mechanics and Engineering, 2007, 26(5): 915–921. doi: 10.3321/j.issn:1000-6915.2007.05.007 [13] 杨栋, 李海波, 夏祥, 等. 高地应力条件下爆破开挖诱发围岩损伤的特性研究 [J]. 岩土力学, 2014, 35(4): 1110–1116, 1122. doi: 10.16285/j.rsm.2014.04.012YANG D, LI H B, XIA X, et al. Study of blasting-induced dynamic damage of tunnel surrounding rocks under high in-situ stress [J]. Rock and Soil Mechanics, 2014, 35(4): 1110–1116, 1122. doi: 10.16285/j.rsm.2014.04.012 [14] 李新平, 陈俊桦, 李友华, 等. 溪洛渡电站地下洞室群爆破地震效应的研究 [J]. 岩石力学与工程学报, 2010, 29(3): 493–501.LI X P, CHEN J H, LI Y H, et al. Study of blasting seismic effects of underground chamber group in Xiluodu hydropower station [J]. Chinese Journal of Rock Mechanics and Engineering, 2010, 29(3): 493–501. [15] 刘艳, 许金余. 地应力场下岩体爆体的数值模拟 [J]. 岩土力学, 2007(11): 2485–2488. doi: 10.16285/j.rsm.2007.11.042LIU Y, XU J Y. Numerical simulation of rock mass explosion under in-situ stress field [J]. Geotechnical Mechanics, 2007(11): 2485–2488. doi: 10.16285/j.rsm.2007.11.042 [16] 李真珍, 于建新, 杨小林, 等. 深部煤层水压爆破裂纹扩展规律 [J]. 高压物理学报, 2022, 36(3): 035301. doi: 10.11858/gywlxb.20210912LI Z Z, YU J X, YANG X L, et al. Crack propagation regularity of hydraulic blasting in deep coal seam [J]. Chinese Journal of High Pressure Physics, 2022, 36(3): 035301. doi: 10.11858/gywlxb.20210912 [17] 王文龙. 钻眼爆破 [M]. 北京: 煤炭工业出版社, 1984.WANG W L. Drilling and blasting [M]. Beijing: China Coal Industry Publishing House, 1984. [18] 杨善元. 岩石爆破动力学基础 [M]. 北京: 煤炭工业出版社, 1993.YANG S Y. The foundation of rock blasting dynamics [M]. Beijing: China Coal Industry Publishing House, 1993. [19] 冷振东, 卢文波, 陈明, 等. 岩石钻孔爆破粉碎区计算模型的改进 [J]. 爆炸与冲击, 2015, 35(1): 101–107. doi: 10.11883/1001-1455(2015)01-0101-07LENG Z D, LU W B, CHEN M, et al. Improved calculation model for the size of crushed zone around blasthole [J]. Explosion and Shock Waves, 2015, 35(1): 101–107. doi: 10.11883/1001-1455(2015)01-0101-07 [20] 戴俊. 岩石动力学特性与爆破理论 [M]. 2版. 北京: 冶金工业出版社, 2013.DAI J. Dynamic behaviors and blasting theory of rock [M]. 2nd ed. Beijing: Metallurgical Industry Press, 2013. [21] 李洪超, 刘殿书, 赵磊, 等. 大理岩RHT模型参数确定研究 [J]. 北京理工大学学报, 2017, 37(8): 801–806. doi: 10.15918/j.tbit1001-0645.2017.08.006LI H C, LIU D S, ZHAO L, et al. Study on parameter determination of marble RHT model [J]. Journal of Beijing Institute of Technology, 2017, 37(8): 801–806. doi: 10.15918/j.tbit1001-0645.2017.08.006 [22] SIERRA C, TSENG E, JAIN A, et al. Cornering stiffness estimation based on vehicle lateral dynamics [J]. Vehicle System Dynamics, 2006, 44(Suppl 1): 24–38. doi: 10.1080/00423110600867259 [23] 钮强. 岩石爆破机理 [M]. 北京: 中国建筑工业出版社, 1992.NIU Q. Rock blasting mechanism [M]. Beijing: China Architecture & Building Press, 1992. [24] LU W B, CHEN M, GENG X, et al. A study of excavation sequence and contour blasting method for underground powerhouses of hydropower stations [J]. Tunnelling and Underground Space Technology, 2012, 29: 31–39. doi: 10.1016/j.tust.2011.12.008 [25] 李芳涛, 胡志平, 陈南南, 等. 爆破荷载作用下隧道围岩裂隙范围计算方法研究 [J]. 振动与冲击, 2022, 41(8): 260–269. doi: 10.13465/j.cnki.jvs.2022.08.032LI F T, HU Z P, CHEN N N, et al. A study of fracture range of tunnel surrounding rock under blasting [J]. Journal of Vibration and Shock, 2022, 41(8): 260–269. doi: 10.13465/j.cnki.jvs.2022.08.032 [26] SANCHIDRIÁN J A, SEGARRA P, LÓPEZ L M. Energy components in rock blasting [J]. International Journal of Rock Mechanics and Mining Sciences, 2007, 44(1): 130–147. doi: 10.1016/j.ijrmms.2006.05.002 [27] WAN D Y, ZHU Z M, LIU R F, et al. Measuring method of dynamic fracture toughness of mode Ⅰ crack under blasting using a rectangle specimen with a crack and edge notches [J]. International Journal of Rock Mechanics and Mining Sciences, 2019, 123: 104104. doi: 10.1016/j.ijrmms.2019.104104 [28] YUE Z W, QIU P, YANG R S, et al. Stress analysis of the interaction of a running crack and blasting waves by caustics method [J]. Engineering Fracture Mechanics, 2017, 184: 339–351. doi: 10.1016/j.engfracmech.2017.08.037 [29] 杨建华, 吴泽南, 姚池, 等. 地下洞室爆破开挖诱发围岩损伤特性及PPV阈值研究 [J]. 振动与冲击, 2019, 38(2): 131–139. doi: 10.13465/j.cnki.jvs.2019.02.020YANG J H, WU Z N, YAO C, et al. Characteristics and PPV thresholds of rock damages under underground blasting excavation [J]. Journal of Vibration and Shock, 2019, 38(2): 131–139. doi: 10.13465/j.cnki.jvs.2019.02.020 -
下载:
下载:
点击查看大图
计量
- 文章访问数: 454
- HTML全文浏览量: 179
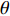
- PDF下载量: 54
