Response Characteristics of Spring-Mass System under Impact of Momentum Ball
-
摘要: 冲量是爆炸空气冲击波的重要威力参数,基于动量块的测试方法是获取冲量的途径之一。通过开展弹簧-质量块系统在动量球撞击下的响应特性研究,尝试将动量球的动量转换为弹簧的定量压缩位移。研究过程中,选定一种弹簧-质量块组合,通过开展聚甲醛、聚四氟乙烯、铝、钢4种不同动量球撞击试验,获得了弹簧-质量块压缩响应特性和动量转化效率。结果表明,在所试验的测试范围内,弹簧的最大压缩位移与动量球的加载速度线性相关,聚四氟乙烯动量球与弹簧-质量块系统耦合最稳定,适合作为冲击波冲量测试的转换载体。该系统可以为冲击波测量提供一种新方法。Abstract: Impulse is an important power parameter of explosive air shock wave, and the momentum-block test method is one of the ways to measure the impulse. This paper attempts to convert the momentum of the momentum-ball into the quantitative compression displacement of the spring by investigating the impact response characteristics of spring-mass system. Four different momentum-balls impact tests, such as polyformaldehyde, polytetrafluoron, aluminum and steel, were conducted to obtain the spring-mass compression response characteristics and momentum/energy conversion efficiency. The results show that the maximum compression displacement of the spring is linearly related to the loading velocity of the momentum-ball. The polyformaldehyde momentum-ball spring coupling system shows the most stable properties, which is suitable for the transfer carrier of shock wave impulse measurement. This work can provide a new method for shock wave measurement.
-
Key words:
- explosive shock wave /
- momentum block /
- spring-mass system /
- impact stiffness /
- flutter wave
-
炸药在空气中爆炸产生的冲击波是爆破/杀爆类战斗部对目标进行毁伤的重要因素之一,对冲击波的研究一直以来都是非常热门的课题[1-2]。对于空气冲击波来说,超压峰值、正压作用时间和比冲量被认为是反映威力的3个重要参数。要准确评估爆炸冲击波的破坏效应,就要对这些参数进行测量。在比冲量测试方面,目前常用的方法包括传感器电测法、等效靶板法、冲击摆法、等高线法等[3-4]。其中,电测法是利用各种传感器测试获得冲击波压力时程曲线,再积分获得比冲量,因其测试结果准确,是应用最广泛的形式,但其也存在易受电磁干扰、成本高和布设困难等不足,在部分场合难以应用。后3种测量方法主要是利用爆炸冲击波与周围物体的作用进行测试,在定量方面不如电测传感器,但突出的优点是不需要用电。其中等效靶板法[5]是利用效应靶在冲击波作用下的变形和破碎情况来估算冲击波参数,敏感性较好,但是可能存在变形不规则、破裂不均匀等问题,易带来较大的不确定性。冲击摆法[6]是将待测冲量转换成摆锤的角位移,具有抗干扰能力强和无需现场标定等优点,但该方法在实验过程中难以确定中心点位置,容易造成较大的测量误差。等高线法[7-8]是根据炸药爆炸驱动动量块飞散,通过测量动量块落点反推动量块速度,从而评价冲击波比冲量或炸药当量,该方法存在动量块第一落点的选择主观性较强、精度较差等问题。最近,本研究团队[9]发展了利用收集仓接收动量球的方法,但是仍然存在体积较大、安装要求高等问题。本工作拟改进收集仓接收动量球方式,探索利用弹簧-质量块系统定量测试动量块的动量,以支撑形成装置小巧、环境适应性更强的爆炸冲击波比冲量无源测量装置。
弹簧是一种技术比较成熟的机械元件,其中螺旋弹簧是应用最广泛的弹簧结构,其在准静态条件下的力学特性相对比较简单,但是在受到冲击加载时,由于波的作用和弹簧径向扩张等影响,其响应会比较复杂。国内外许多学者对弹簧的动态冲击响应开展了研究,主要包括弹簧中力的分析和波的传播[10]、在冲击载荷作用下的响应[11-12]以及数值计算算法[13-14]和弹簧设计等。近年来,随着弹簧在减震、隔振和储能等方面的突出表现,针对动载条件下弹簧的刚度变化研究日益增加。薛瑞娟等[15]通过对环形弹簧的静刚度和冲击刚度进行数值模拟研究,得出了环形弹簧冲击刚度远大于静刚度的结论。在对空气弹簧的研究中,赵亚敏等[16]建立了约束模式空气弹簧的改进刚度模型,并对影响其刚度变化的因素进行了数值模拟研究,提出了实现低刚度隔振特性的结构和材料参数。
本研究选取一种弹簧-质量块组合,通过气体炮驱动的方式,开展聚甲醛、聚四氟乙烯、铝、钢4种动量球撞击试验(后续分别记为Pom球、Ptfe球、Al球和Steel球),以获得该弹簧-质量块系统在不同质量球和不同加载速度下的动态响应特性,并分析利用这种方式进行动量球速度测试的可行性。
1. 实验设计
1.1 实验装置
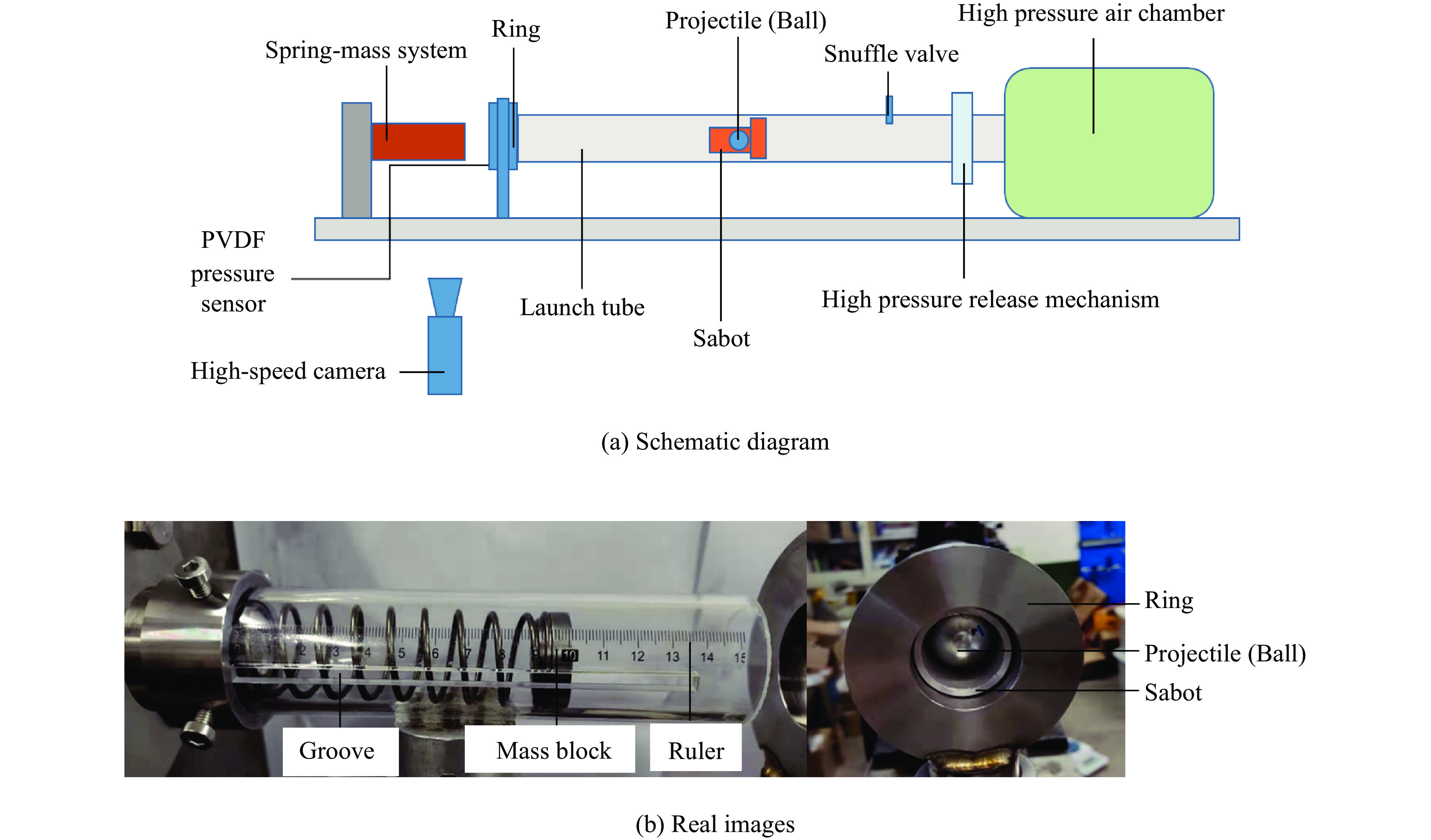
本研究设计利用气体炮来模拟冲击波加载和驱动动量球,整体装置如图1所示。气体炮发射管的内径为50 mm,长度为2 m。实验过程中,通过改变气压和动量球在发射管中的位置实现不同的加载速度。同时,为保证动量球单独被加速,且后续气体不会继续加载到弹簧-质量块系统上,设计了弹托分离装置,将弹托设计成T形,运动到挡弹环时底部被阻挡从而使弹托停止,里面的动量球飞出,单独撞击弹簧-质量块系统,如图1(a)所示。
弹簧-质量块系统安装在透明的有机玻璃管(内径32 mm,长度220 mm)内,玻璃管起限位作用,防止弹簧碰撞后弯曲和偏移,同时便于利用高速相机拍摄整个撞击过程。通过前期加工安装可使玻璃管内侧下沿与弹托内侧下沿处于同一高度,以较好地完成对心碰撞。弹簧与质量块之间通过卡槽连接,即在质量块上开设2 mm深的槽,将弹簧卡入其中,为了不影响弹簧的压缩运动,槽的深度以刚好卡进簧丝即可;弹簧与底部支撑之间的连接方式与质量块相同。为了避免管内的气体影响质量块的运动,在管侧壁开设了两条宽度为4 mm、长度为140 mm的通槽。另外,为方便定量记录质量块和弹簧的运动情况,在管壁粘贴了刻度尺。
1.2 主要部件
4种动量球的参数如表1所示。弹簧选取普通圆柱螺旋压缩弹簧,参数如表2所示。质量块采用光轴切割的圆柱形,参数如表3所示。
表 1 动量球参数Table 1. Parameters of momentum ballMaterial Shape Diameter/mm ρ/(g·cm−3) Mass/g Al Sphere 30.02 2.71 38.27 Pom Sphere 30.02 1.35 18.98 Steel Sphere 30.01 7.91 111.71 Ptfe Sphere 29.98 2.20 30.25 表 2 弹簧参数Table 2. Parameters of springMaterial Wire diameter/mm Diameter/mm Height/mm Turns Shear modulus/GPa Mass/g Spring steel 2.20 30 100 10 80 27.85 表 3 质量块参数Table 3. Parameters of mass blockMaterial Shape Diameter/mm Height/mm Mass/g 45 steel Cylinder 30 10 50.50 2. 实验结果及分析
2.1 碰撞过程和实验数据
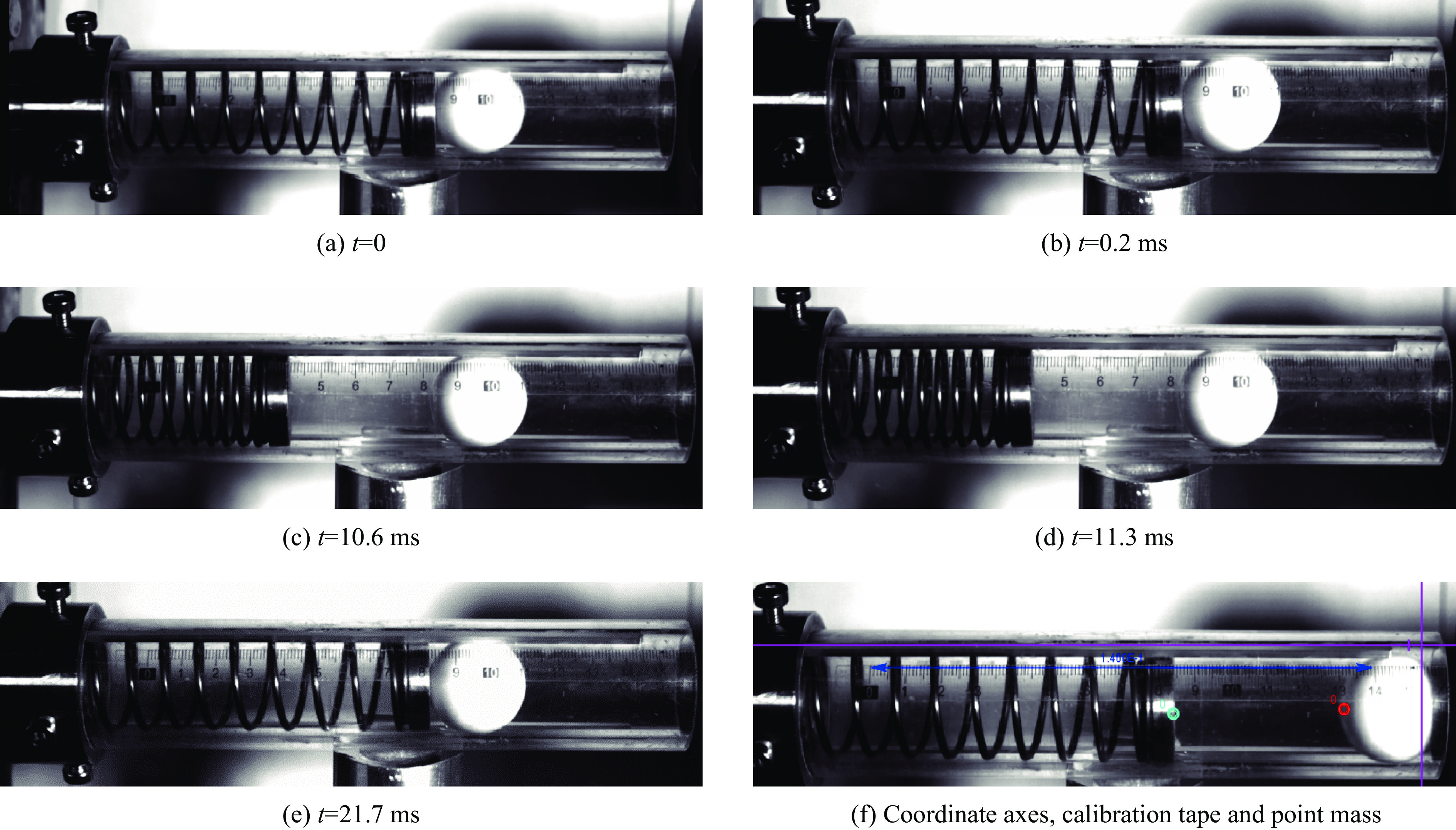
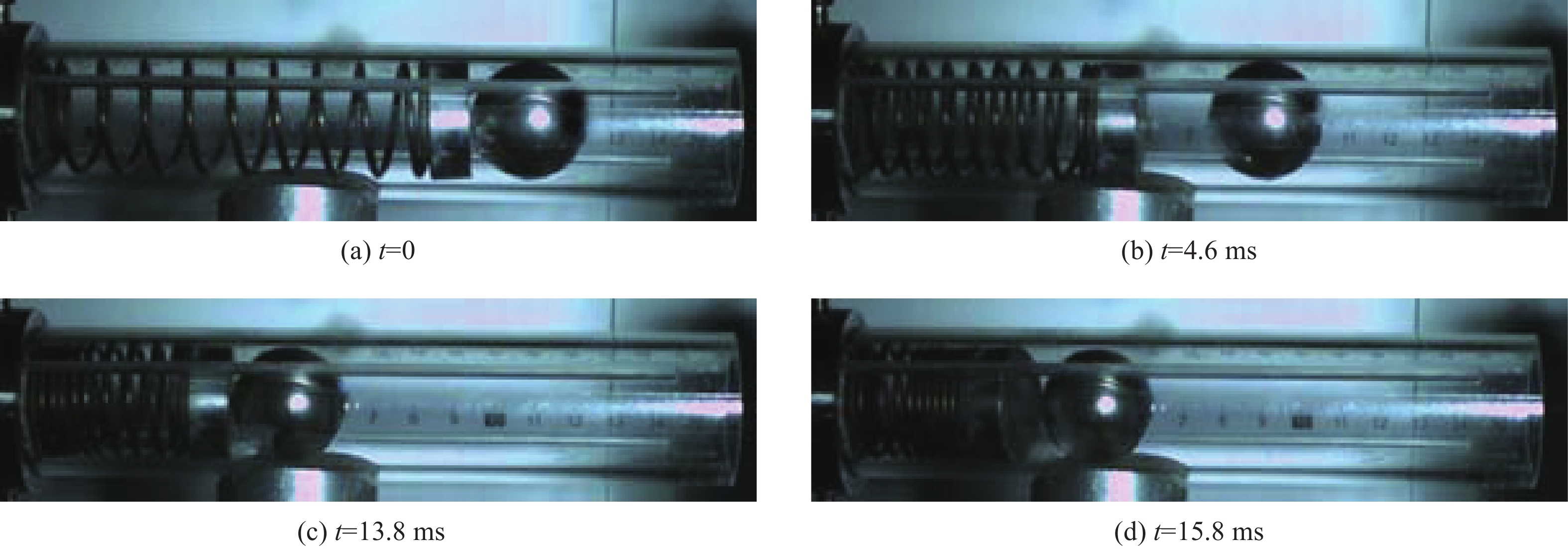
通过高速相机拍摄可得到动量球碰撞质量块的全过程,这里以Ptfe球为例,对碰撞过程进行阐述。高速相机的型号为FASTCAM Nova S12,拍摄过程中设置拍摄速率为10000帧/秒,并通过LED灯进行补光,典型结果如图2所示。对所拍摄图片进行判读,并设定动量球与质量块刚刚接触时为零时刻,如图2(a)所示;动量球与质量块短暂接触后,t=0.2 ms时两者分离,如图2(b)所示;质量块与质量球短暂接触后获得速度并压缩弹簧前进,而Ptfe球几乎维持在原位,t=10.6 ms时,质量块压缩弹簧至最大位移,如图2(c)所示;然后质量块在最大位移处保持几乎静止状态,至t=11.3 ms,持续了约0.7 ms,如图2(d)所示;随后质量块在弹簧驱动下开始反弹,t=21.7 ms时,弹簧驱动质量块反弹后再次与Ptfe球相撞,如图2(e)所示。
另外,在多次实验中均发现动量球撞击质量块后的一小段时间内质量块有一定的倾斜,其产生的原因是弹簧与质量块的接触在弹簧圆周方向上不是均匀的,而设计有机玻璃管道与质量块匹配尺寸时,预留了一定的空间,稍微倾斜的质量块不会与管壁发生接触而影响质量块的运动。同时,随着质量块的运动,偏斜逐渐减小,因此在后续分析时不考虑倾斜的影响。
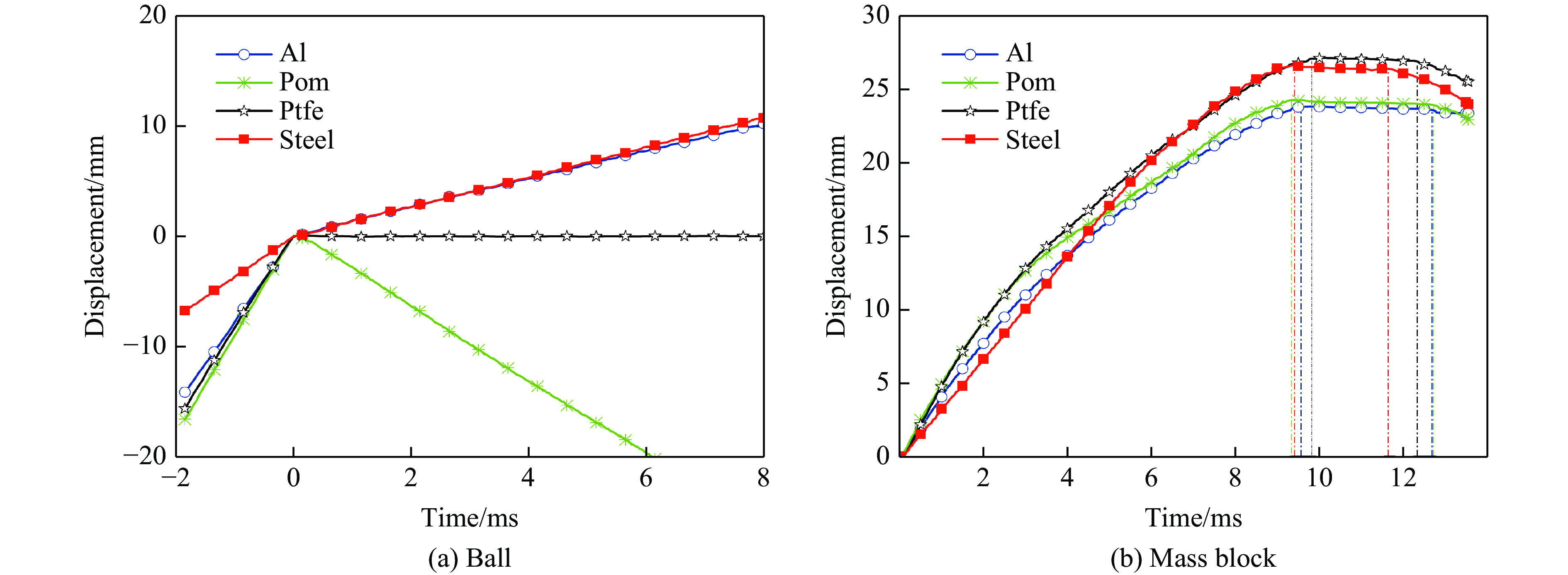
获得碰撞图像后,通过图像处理软件可得到动量球的运动情况(包括碰撞速度、继续运动的速度或者反弹速度等)、质量块的运动情况(包括位移、速度等),如图2(f)所示。同样设置碰撞点时刻为零时刻,图3给出了4种动量球碰撞质量块后两者的位移时程曲线。从图3(a)可以看出,密度大的动量球(Al球和Steel球)在碰撞后还会继续跟随质量块进行运动,Ptfe球几乎留在碰撞位置,密度小的Pom球则会发生反弹。从图3(b)可以看出,在4种球的碰撞下,质量块压缩弹簧达到最大位移后均可以维持一定的时间,且最大位移是稳定的,这对于通过质量块-弹簧系统的最大压缩反推动量球的运动速度是非常有利的。
另外,在前期工作[9]中发现,上面提到的4种动量球在距离爆点一定距离后获得的速度一般在几米每秒到十几米每秒之间,因此在本实验中也控制加载速度在此范围。提取不同加载速度下4种动量球碰撞的典型实验结果,得到的速度和位移结果如表4所示,其中v为动量球的加载速度,v1为动量球碰撞后的速度,v2为碰撞后质量块的最大速度,x为弹簧的最大压缩位移。
表 4 不同工况下的实验结果Table 4. Experimental results under different working conditionsMaterial Mass of ball/g Mass of block/g v/(m·s−1) v1/(m·s−1) v2/(m·s−1) x/mm Ptfe 30.25 50.50 3.77 0 2.07 14.30 Ptfe 30.25 50.50 4.36 0 2.13 15.40 Ptfe 30.25 50.50 4.82 0 2.61 16.40 Ptfe 30.25 50.50 5.91 0 2.98 19.60 Ptfe 30.25 50.50 6.64 0 3.33 20.30 Ptfe 30.25 50.50 7.32 0 4.31 24.60 Ptfe 30.28 50.50 10.77 0 6.21 35.45 Ptfe 30.28 50.50 11.92 0 6.61 39.37 Ptfe 30.28 50.50 13.43 0 7.42 44.20 Al 38.26 50.50 4.82 0.25 2.57 15.70 Al 38.26 50.50 7.25 1.47 3.92 22.73 Al 38.35 50.50 7.78 2.04 3.86 23.95 Al 38.35 50.50 8.81 2.72 3.70 28.60 Al 38.32 50.50 9.28 2.40 3.67 28.23 Al 38.32 50.50 9.70 2.62 4.59 29.95 Al 38.32 50.50 10.02 2.93 4.61 32.40 Al 38.32 50.50 11.37 3.35 5.24 34.68 Steel 111.71 50.50 2.67 0.78 3.66 19.80 Steel 111.71 50.50 2.93 0.91 3.88 21.10 Steel 111.71 50.50 3.86 1.26 3.85 25.27 Steel 111.71 50.50 4.86 2.06 6.25 33.30 Steel 111.71 50.50 6.70 2.87 6.55 41.10 Steel 111.71 50.50 7.13 2.74 6.31 44.00 Steel 111.71 50.50 8.42 3.81 9.40 55.30 Steel 111.71 50.50 9.66 4.07 11.10 64.40 Pom 19.07 50.50 4.11 −1.28 2.03 12.37 Pom 19.07 50.50 4.70 −1.74 2.39 13.80 Pom 19.07 50.50 8.36 −3.38 4.41 23.93 Pom 19.07 50.50 9.07 −3.97 4.82 24.20 2.2 动量球速度与弹簧最大压缩位移的关系
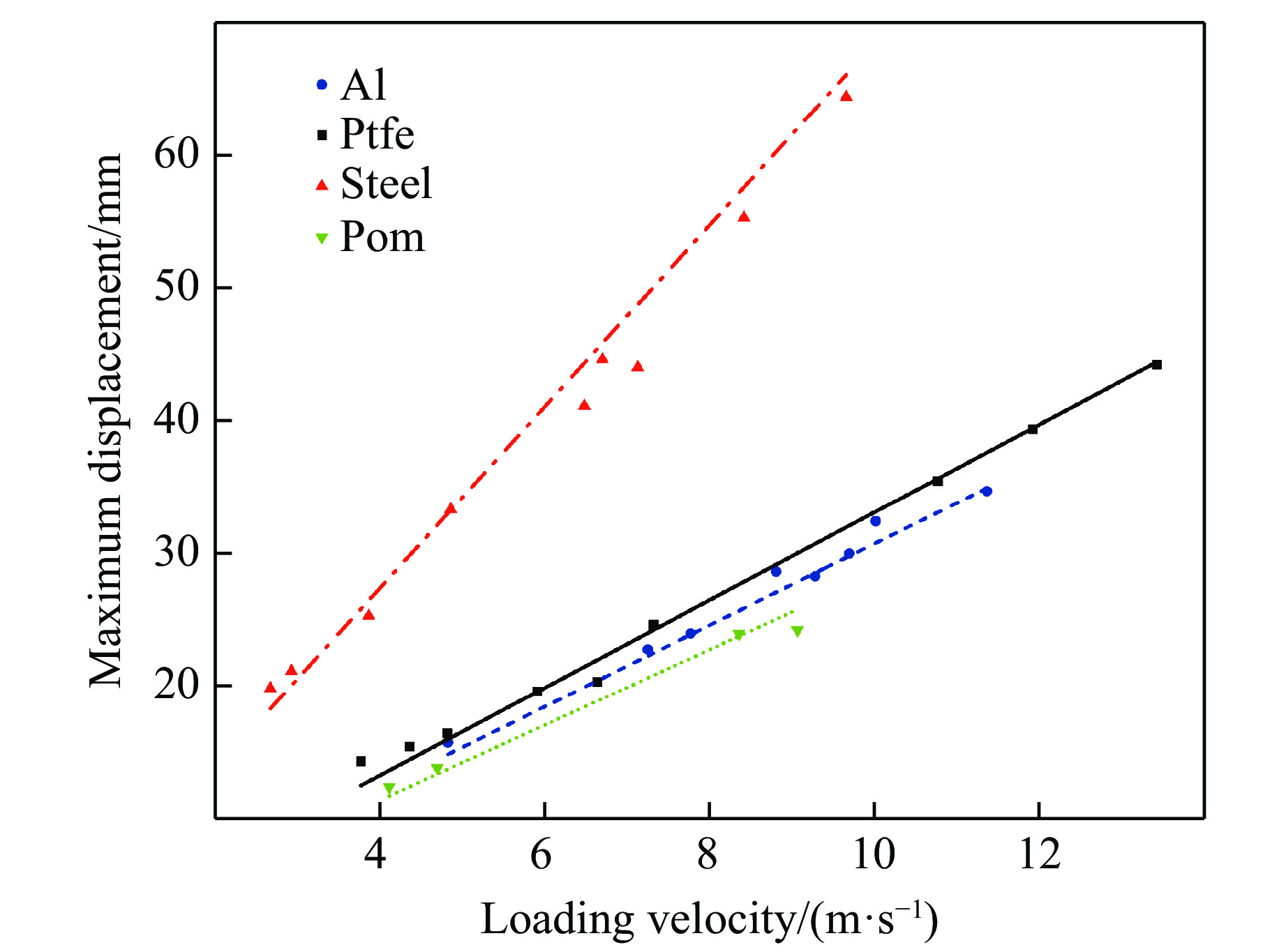
根据表4的数据,可以得到如图4所示的动量球碰撞速度与弹簧最大压缩位移的关系曲线。可以看出,在本实验的加载速度范围内,两者具有较好的线性关系。对于Steel、Al、Ptfe和Pom球,拟合曲线的斜率分别为6.840±0.211、3.070±0.025、3.306±0.036、2.840±0.062,拟合度R2分别为0.995、0.999、0.999、0.998。对于实验工况较多的Al球和Ptfe球,线性度非常高,说明如果标定动量球速度与弹簧最大压缩位移之间的关系,可以利用位移得到动量球的速度。
2.3 动量球与质量块的动量转化
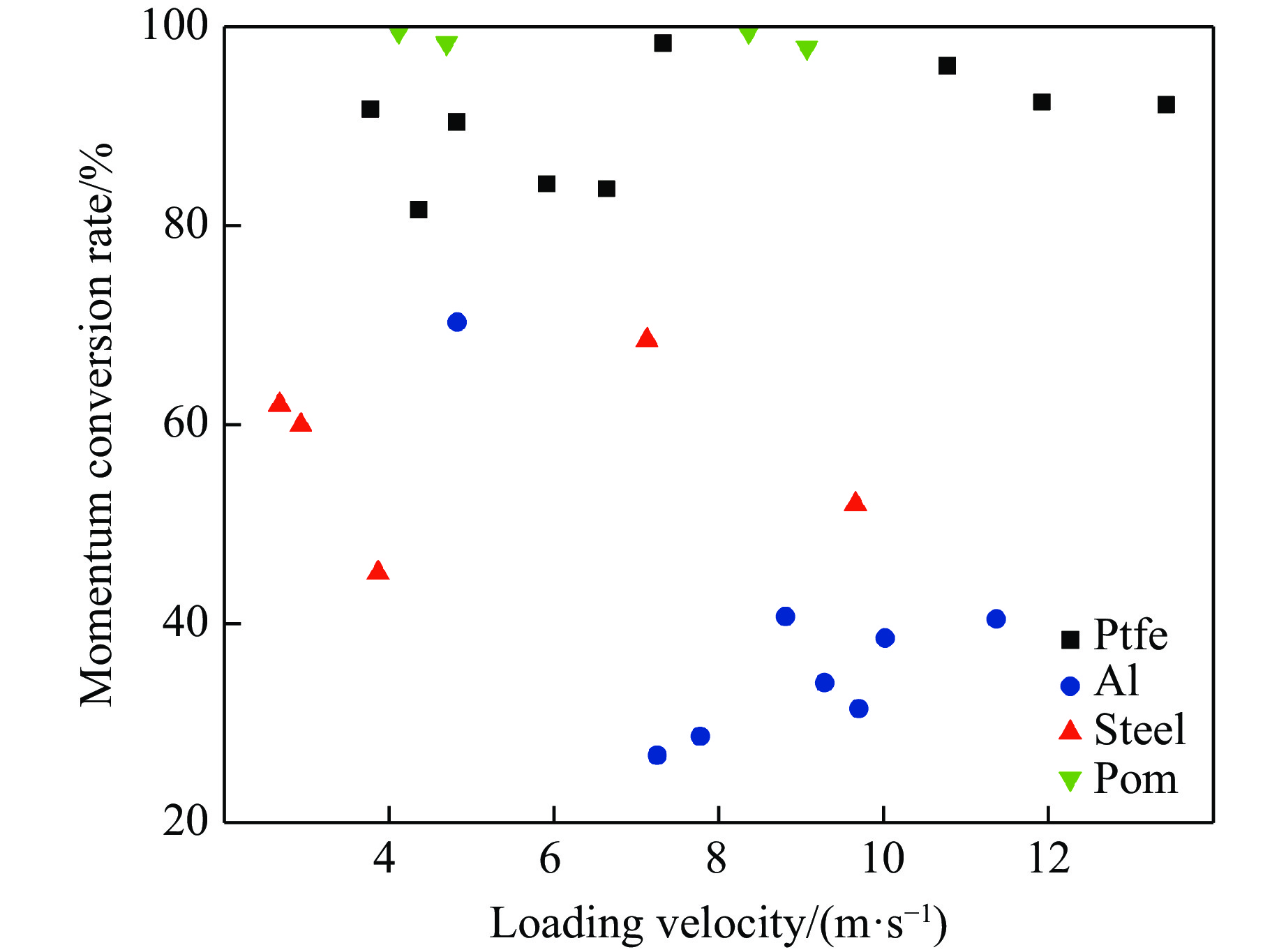
根据动量守恒定律,碰撞后动量球的动量将全部转化为质量块的动量和动量球的剩余动量。但在本实验中,质量块实际上是与弹簧组成了一个系统,弹簧的存在会影响动量的转换,同时弹簧的质量如何等效也是一个较难解决的问题。鉴于动量球与质量块的碰撞时间较短(基本在0.2 ms以内),不考虑弹簧对碰撞过程的影响,只分析动量球与质量块之间的动量转化情况。计算时,若动量球第1次碰撞不反弹,则转化率为质量块动量与动量球动量之比;若动量球存在反弹,则将动量球加载动量与反弹动量取绝对值求和后作为分母,质量块的动量作为分子,以此来计算转化率。分析4种动量球在不同速度下的撞击结果,可以得到如图5所示的图像,数据如表5所示。
表 5 4种动量球动量转化率对比Table 5. Comparison of momentum conversion rate of four kinds of momentum ballsMaterial Momentum conversion range/% Average/% Stdev Steel 40.01−61.97 51.77 0.094 Al 26.72−70.24 34.35 0.057 Ptfe 81.56−98.30 90.05 0.057 Pom 97.88−99.51 98.80 0.008 从图5和表5可以看到,对于所选取的质量块和弹簧组合:由于Al球和Steel球碰撞后仍有向前的速度,因此动量转化率较低; Ptfe球由于碰撞后速度基本降为零,动量转化率比较高,稳定在90%左右。另外,单从转化率数值的偏差程度上看,Pom球和Ptfe球均具有较好的稳定性,Al球在加载速度为6~12 m/s时稳定性较好。
2.4 动量球对质量块二次撞击的影响
若动量球碰撞后停止或仍有向前的速度,均会与质量块发生两次以上的碰撞。如果第2次及后续碰撞会导致弹簧产生比第1次碰撞时的最大压缩位移还大的压缩,会对利用弹簧的最大压缩位移计算动量球的速度带来困难(图4的线性关系只能反映第1次撞击结果)。对于本实验的4种动量球来说,需要考虑二次碰撞的主要是Al球和Steel球,为此对Al球(碰撞速度为9.85 m/s)和Steel球(碰撞速度为9.66 m/s)的碰撞结果进行分析,图6和图7分别给出了Al球和Steel球的二次撞击过程。
从图6可以看到:t=0时,Al球与质量块第1次碰撞;t=9.7 ms时,弹簧压缩至最大位移,Al球也刚好接近质量块; t=9.9 ms时,Al球再次与质量块发生碰撞,从图6(b)和图6(c)可以看到,质量块前沿对应的刻度没有变化;经过二次碰撞后,弹簧被再次压缩约2 mm,如图6(d)所示,随后系统反推动量球离开。由此可知,在此加载速度下,Al球二次碰撞产生的最大位移超过第1次碰撞约2 mm。
同样地,如图7所示:t=0时,Steel球与质量块第1次碰撞;t=13.8 ms时,弹簧压缩至最大位移,动量球与质量块发生二次碰撞;二次碰撞导致质量块继续前进了10 mm,且偏移较大,如图7(d)所示。分析Al球和Steel球两种动量球的二次撞击可知,二次撞击在冲击波测试中的影响不可忽略。因此,在设置工况时,要尽可能防止出现二次碰撞情况。
2.5 弹簧在冲击加载下的刚度
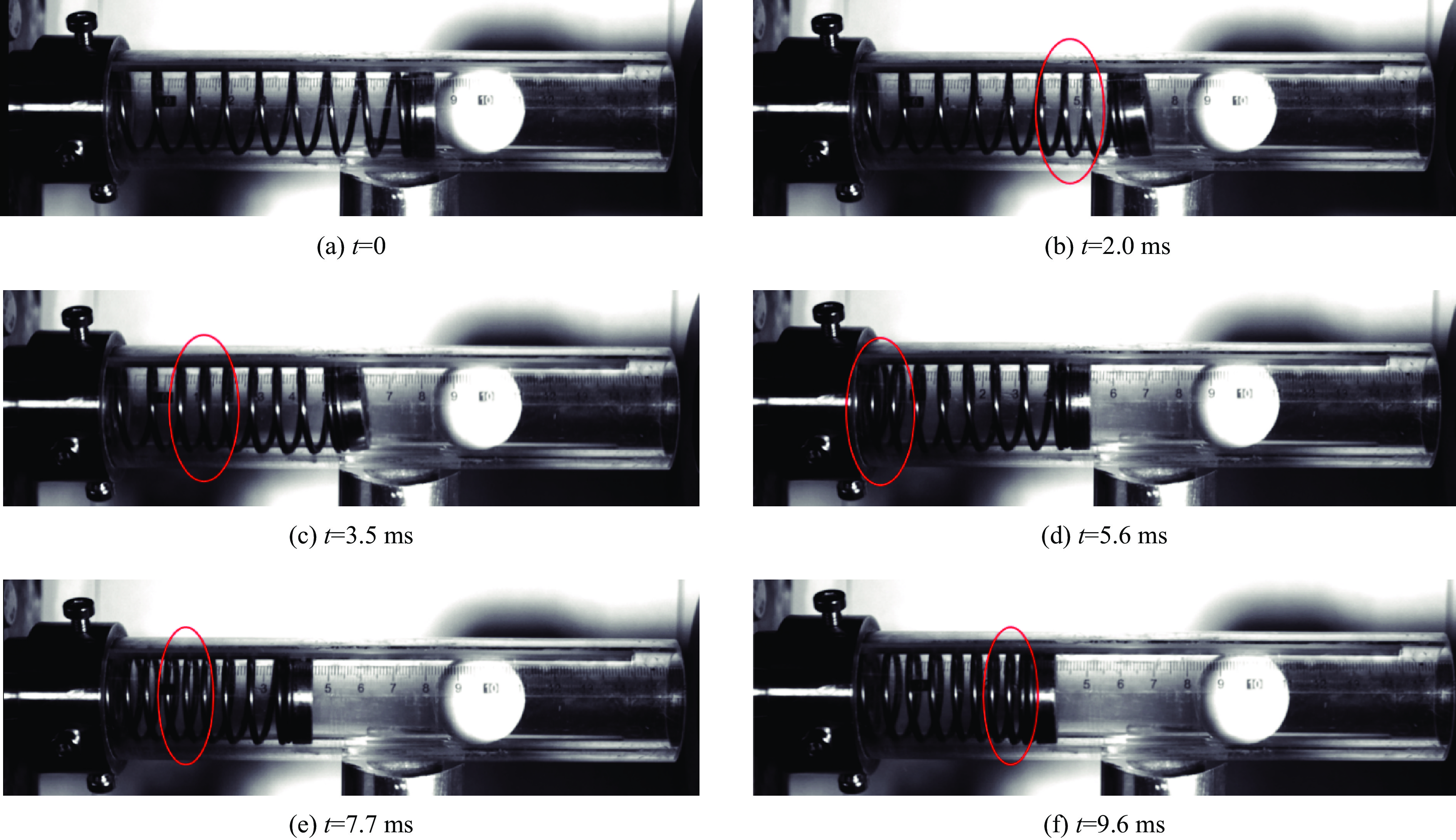
在静态或者准静态加载下,线性弹簧的静刚度为定值,但从高速相机拍摄的弹簧动态冲击压缩过程来看,虽然加载速度仅为10 m/s左右,但还是能观察到明显的颤振波[17]传播现象,冲击刚度可能发生变化。图8给出了Ptfe动量球以13.24 m/s的速度撞击弹簧-质量块系统的过程。从图8(b)可以看到,t=2.0 ms时,撞击过程只引起弹簧的前面几圈响应,产生的颤振波传播到刻度4.69 cm处;t=3.5 ms时,颤振波继续传播至刻度1.35 cm处,如图8(c)所示;随后颤振波作用于固支底座,继而反射,如图8(d)所示。当颤振波经过支撑底座反弹再次作用于质量块时,出现卸载过程,因此弹簧受到质量块的压缩和卸载的叠加作用,致使某段时间内速度为零,位移不改变,如图3(b)所示。根据图8所示结果,可以得到Ptfe动量球以13.24 m/s的速度碰撞所引起的弹簧颤振波速度v0为22.27 m/s。同理,分析4.45和7.32 m/s的撞击结果,可以得到所对应的颤振波速度分别为20.88和21.35 m/s。
弹簧-质量块系统是一个典型的单自由度系统,其无阻尼系统振动的圆频率只与系统的质量和刚度有关[18],表达式为
(1) 式中:K为系统的刚度;me为系统的等效质量,弹簧质量很小时,可将me近似等于质量块的质量m。在本实验中,系统是有阻尼的,不妨设阻尼比为
(2) 在阻尼比不变的情况下,其圆频率只与系统的质量和刚度有关。
本实验中,系统的质量是一定的,需要进一步考察系统的刚度。已知弹簧刚度与弹簧有效圈数成反比,从前面的分析结果来看,弹簧在冲击作用下的影响并非整体响应。如初始时,只有前面的部分簧圈被压缩,后面的簧圈来不及响应,这就相当于在这一段时间内响应的弹簧刚度比整个弹簧大。考虑到分段描述弹簧的刚度比较复杂,此处通过弹簧圆频率与响应周期的关系整体分析弹簧在动态撞击下的冲击刚度。圆频率与系统周期(T)的关系为
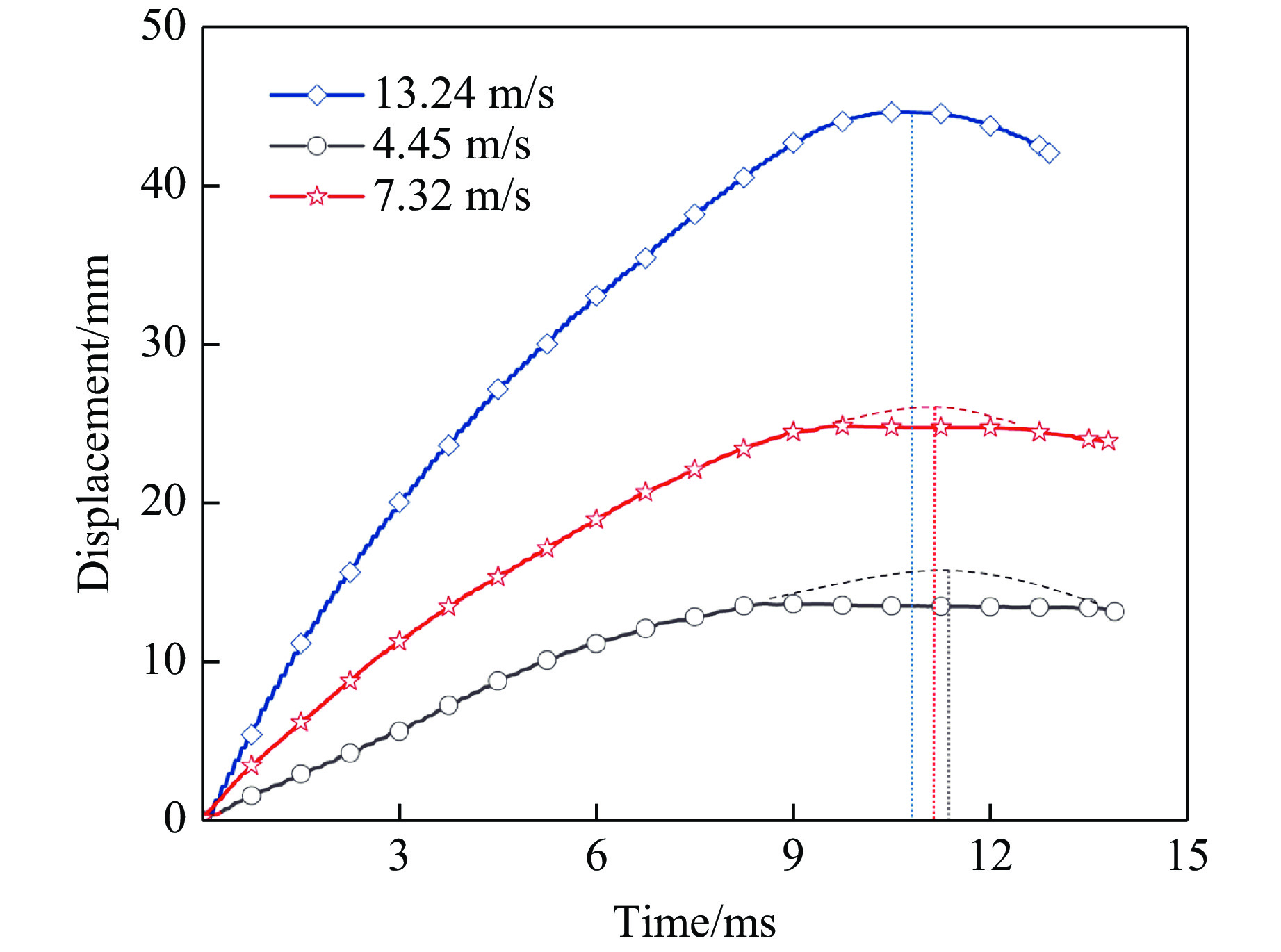
(3) 图9显示了Ptfe球以不同速度(4.45、7.32、13.24 m/s)碰撞后弹簧-质量块系统1/4周期的响应曲线。前面提到,由于颤振波的影响,在质量块运动到最大振幅时会有一段平整的曲线,为了考察其周期,将其拟合补齐(按照正弦函数的对称性,直接取平台的中点作为1/4周期点)。通过提取周期值,可以得到3种撞击速度下弹簧-质量块系统的响应周期分别为44.6、44.3、43.0 ms。根据式(2)和式(3),可以计算得到7.32 m/s撞击时弹簧的冲击刚度比4.45 m/s时高6.14%,13.32 m/s撞击时弹簧的冲击刚度比4.45 m/s时高7.58%。
2.6 弹簧-质量块系统适用的动量球速度范围
本研究的目的是考察和建立动量球加载速度与弹簧最大压缩位移之间的关系。从测量的角度来看,为提高精度,应尽量使弹簧获得明显的压缩,但又不能接近被压实(线性度变差)。定义弹簧压缩率为弹簧的最大压缩长度与弹簧原长度之比,本研究希望获得压缩率保持在10%~60%之间的工况。表6给出了4种不同材料动量球在不同加载速度下的弹簧压缩率区间。从表6可以看到,除了Steel球有个别较大加载速度的弹簧压缩率超过60%以外,其他均在10%~60%之间。
表 6 4种不同材料动量球加载下弹簧的压缩率Table 6. Compressibility of the spring under 4 momentum ball loadsMaterial Loading velocity/(m·s−1) Compression ratio/% Remark Steel 2−13 19−70 A few more than 60% Al 3−13 12−37 Ptfe 3−14 14−45 Pom 3−11 11−25 综合分析动量转化率、二次碰撞等的影响,可以看到,针对本研究设计的弹簧-质量块系统,Ptfe球最适合作为冲击测量的动量球。对于其他3种动量球和更高的加载速度,可以通过改变质量块质量和弹簧的规格(尺寸、刚度等),以实现更合适的相互匹配。
3. 结 论
采用气体炮驱动4种不同密度动量球,对选定的弹簧-质量块系统进行冲击加载实验,获得了弹簧-质量块的响应特性,主要结论如下:
(1) 弹簧的最大压缩位移与动量球的加载速度呈较好的线性关系,表明弹簧-质量块系统作为冲击波比冲量测试辅助装置是可行的;
(2) 对于本实验的弹簧-质量块系统,在10 m/s左右的加载速度下,Ptfe动量球的动量转化率较高且稳定,适合用于冲击波比冲量的测量;
(3) 在动量球冲击加载下,弹簧中会出现颤振波,颤振波的速度与加载速度正相关;
(4) 随着加载速度的提高,弹簧的等效刚度会小幅增加,Ptfe球以13.32 m/s的速度撞击时弹簧的整体动刚度比4.45 m/s时高7.58%。
-
表 1 动量球参数
Table 1. Parameters of momentum ball
Material Shape Diameter/mm ρ/(g·cm−3) Mass/g Al Sphere 30.02 2.71 38.27 Pom Sphere 30.02 1.35 18.98 Steel Sphere 30.01 7.91 111.71 Ptfe Sphere 29.98 2.20 30.25 表 2 弹簧参数
Table 2. Parameters of spring
Material Wire diameter/mm Diameter/mm Height/mm Turns Shear modulus/GPa Mass/g Spring steel 2.20 30 100 10 80 27.85 表 3 质量块参数
Table 3. Parameters of mass block
Material Shape Diameter/mm Height/mm Mass/g 45 steel Cylinder 30 10 50.50 表 4 不同工况下的实验结果
Table 4. Experimental results under different working conditions
Material Mass of ball/g Mass of block/g v/(m·s−1) v1/(m·s−1) v2/(m·s−1) x/mm Ptfe 30.25 50.50 3.77 0 2.07 14.30 Ptfe 30.25 50.50 4.36 0 2.13 15.40 Ptfe 30.25 50.50 4.82 0 2.61 16.40 Ptfe 30.25 50.50 5.91 0 2.98 19.60 Ptfe 30.25 50.50 6.64 0 3.33 20.30 Ptfe 30.25 50.50 7.32 0 4.31 24.60 Ptfe 30.28 50.50 10.77 0 6.21 35.45 Ptfe 30.28 50.50 11.92 0 6.61 39.37 Ptfe 30.28 50.50 13.43 0 7.42 44.20 Al 38.26 50.50 4.82 0.25 2.57 15.70 Al 38.26 50.50 7.25 1.47 3.92 22.73 Al 38.35 50.50 7.78 2.04 3.86 23.95 Al 38.35 50.50 8.81 2.72 3.70 28.60 Al 38.32 50.50 9.28 2.40 3.67 28.23 Al 38.32 50.50 9.70 2.62 4.59 29.95 Al 38.32 50.50 10.02 2.93 4.61 32.40 Al 38.32 50.50 11.37 3.35 5.24 34.68 Steel 111.71 50.50 2.67 0.78 3.66 19.80 Steel 111.71 50.50 2.93 0.91 3.88 21.10 Steel 111.71 50.50 3.86 1.26 3.85 25.27 Steel 111.71 50.50 4.86 2.06 6.25 33.30 Steel 111.71 50.50 6.70 2.87 6.55 41.10 Steel 111.71 50.50 7.13 2.74 6.31 44.00 Steel 111.71 50.50 8.42 3.81 9.40 55.30 Steel 111.71 50.50 9.66 4.07 11.10 64.40 Pom 19.07 50.50 4.11 −1.28 2.03 12.37 Pom 19.07 50.50 4.70 −1.74 2.39 13.80 Pom 19.07 50.50 8.36 −3.38 4.41 23.93 Pom 19.07 50.50 9.07 −3.97 4.82 24.20 表 5 4种动量球动量转化率对比
Table 5. Comparison of momentum conversion rate of four kinds of momentum balls
Material Momentum conversion range/% Average/% Stdev Steel 40.01−61.97 51.77 0.094 Al 26.72−70.24 34.35 0.057 Ptfe 81.56−98.30 90.05 0.057 Pom 97.88−99.51 98.80 0.008 表 6 4种不同材料动量球加载下弹簧的压缩率
Table 6. Compressibility of the spring under 4 momentum ball loads
Material Loading velocity/(m·s−1) Compression ratio/% Remark Steel 2−13 19−70 A few more than 60% Al 3−13 12−37 Ptfe 3−14 14−45 Pom 3−11 11−25 -
[1] 赵传荣, 孔德仁. 基于量纲分析的平面冲击波经验模型研究 [J]. 高压物理学报, 2016, 30(6): 526–530. doi: 10.11858/gywlxb.2016.06.014ZHAO C R, KONG D R. Empirical model of plane shock wave on the impact surface of target based on dimensional analysis [J]. Chinese Journal of High Pressure Physics, 2016, 30(6): 526–530. doi: 10.11858/gywlxb.2016.06.014 [2] 孟令存, 闫明, 杜志鹏, 等. 外界条件对中空结构物内爆冲击波的影响 [J]. 高压物理学报, 2020, 34(4): 79–88. doi: 10.11858/gywlxb.20190849MENG L C, YAN M, DU Z P, et al. Influence of external conditions on implosion shock wave of hollow structure [J]. Chinese Journal of High Pressure Physics, 2020, 34(4): 79–88. doi: 10.11858/gywlxb.20190849 [3] 郭炜, 俞统昌, 王建林. 空气冲击波压力的地面测量技术 [C]//第三届全国爆炸力学实验技术交流会. 合肥, 2004: 7.GUO W, YU T C, WANG J L. Surface measurement of air shock wave pressure [C]//The Third National Explosion Mechanics Experiment Technology Exchange Conference. Hefei, 2004: 7 [4] 翟永. 冲击波存储测试系统的同步技术研究 [D]. 太原: 中北大学, 2016: 18−19.ZHAI Y. Research on synchronization technology of stored testing system for shock wave [D]. Taiyuan: North University of China, 2016: 18−19. [5] 叶希洋, 苏健军, 姬建荣. 冲击波测试效应靶法综述 [J]. 兵器装备工程学报, 2019, 40(12): 55−61, 124.YE X Y, SU J J, JI J R. Review of effect target method for shock wave measurement [J]. Journal of Ordnance Equipment Engineering, 2019, 40(12): 55−61, 124. [6] CLOETE T J, NURICK G N, PALMER R N. The deformation and shear failure of peripherally clamped centrally supported blast loaded circular plates [J]. International Journal of Impact Engineering, 2005, 32(1): 92–117. doi: 10.1016/j.ijimpeng.2005.06.002 [7] 仲倩. 燃料空气炸药爆炸参数测量及毁伤效应评估 [D]. 南京: 南京理工大学, 2012: 15.ZHONG Q. Research on measurement of explosion parameters and evaluation of damage effect of fuel air explosive [D]. Nanjing: Nanjing University of Science and Technology, 2012: 15. [8] MANFRED H. Momentum method to measure the blast contour [C]//17th International Symposium on Ballistics. Midrand, South Africa, 1998: 23−27. [9] 李钰钢. 基于动量块的冲击波比冲量测试装置 [D]. 长沙: 国防科技大学, 2021: 50−57.LI Y G. Research on shock wave impulse measurement method based on momentum block [D]. Changsha: National University of Defense Technology, 2021: 50−57. [10] WITTRICK W H. On elastic wave propagation in helical springs [J]. International Journal of Mechanical Sciences, 1966, 8(1): 25–47. doi: 10.1016/0020-7403(66)90061-0 [11] PHILLIPS J W, COSTELLO G A. Large deflections of impacted helical springs [J]. The Journal of the Acoustical Society of America, 1972, 51(3B): 967–973. doi: 10.1121/1.1912946 [12] COSTELLO G A. Radial expansion of impacted helical springs [J]. Journal of Applied Mechanics, 1975, 42(4): 789–792. doi: 10.1115/1.3423707 [13] SINHA S K, COSTELLO G A. The numerical solution of the dynamic response of helical springs [J]. International Journal for Numerical Methods in Engineering, 1978, 12(6): 949–961. doi: 10.1002/nme.1620120607 [14] 赵宗昌, 何水清, 何大同, 等. 螺旋弹簧质量系统动态响应的特征线法[J]. 哈尔滨工程大学学报, 1989(1): 62−73.ZHAO Z C, HE S Q, HE D T, et al. The characteristics method of the dynamic response of the spring-mass systems[J]. Journal of Harbin Shipbuilding Engineering Institute, 1989(1): 62−73. [15] 薛瑞娟, 郭敬彬, 王君, 等. 环形弹簧静刚度与冲击性能有限元分析 [J]. 舰船科学技术, 2018, 40(10): 67–71. doi: 10.3404/j.issn.1672-7649.2018.10.013XUE R J, GUO J B, WANG J, et al. Finite element analysis of static stiffness and shock performance of ring spring [J]. Ship Science and Technology, 2018, 40(10): 67–71. doi: 10.3404/j.issn.1672-7649.2018.10.013 [16] 赵亚敏, 崔俊宁, 邹丽敏, 等. 约束膜式空气弹簧的刚度建模与分析 [J]. 振动与冲击, 2022, 41(1): 60−67, 115.ZHAO Y M, CUI J N, ZOU L M, et al. Stiffness modeling and analysis of constrained membrane air spring [J]. Journal of Vibration and Shock, 2022, 41(1): 60−67, 115. [17] 张英会, 刘辉航, 王德成. 弹簧手册 [M]. 北京: 机械工业出版社, 2017: 389.ZHANG Y H, LIU H H, WANG D C. Spring manual [M]. Beijing: China Machine Press, 2017: 389. [18] 盛宏玉. 结构动力学 [M]. 合肥: 合肥工业大学出版社, 2012: 17−19.SHENG H Y. Structural dynamics [M]. Hefei: Hefei University of Technology Press, 2012: 17−19. -








 下载:
下载:







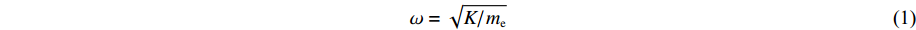

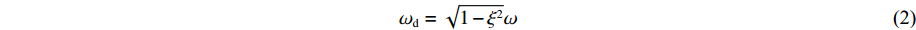
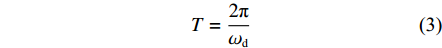


 下载:
下载: