Topological Optimization and Dynamic Response of Periodic Porous Sandwich Structure under Impact Load
-
摘要: 在等效静力方法框架下基于双向渐进结构优化硬杀法构建了冲击载荷下周期性多孔夹芯结构的拓扑优化方法。采用ABAQUS有限元软件，研究了周期性优化夹芯结构与梯形波纹芯层、矩形波纹芯层和随机Voronoi芯层夹芯梁在刚体以100 m/s的速度撞击下的变形失效模式。在载荷作用前期，优化夹芯结构的上半部分芯层被完全压缩，能量吸收优于其他3种结构；在载荷作用后期，由于优化夹芯结构的最终塑性变形较小，在整个响应过程中总能量吸收略小于其他3种结构。为检验单一载荷工况下优化夹芯结构在其他载荷作用下的性能，采用不同速度的刚体以3种不同类型的脉冲载荷加载，比较了4种夹芯结构的能量吸收性能。综合考虑夹芯结构上下面板的跨中挠度、比吸能、芯层吸能占比和平均冲击力后发现：在刚体冲击下，优化夹芯结构具有更好的能量吸收性能和抗冲击性能；在矩形脉冲下，优化夹芯结构的比吸能小于矩形波纹芯夹芯结构，未能体现结构优化的优势。研究表明，单一工况下优化所得的结构不能在任意载荷下均表现出最优异的性能，因此，针对不同的载荷工况，需要进一步的研究。Abstract: In this study, under the frame of equivalent static loads (ESL) method structural optimization and based on hard-kill bi-directional evolutionary structural optimization (hard-kill BESO), the topological optimization method for periodic porous sandwich structure under impact load was carried out. The commercial software ABAQUS was used to investigate the deformation patterns of the optimized periodic sandwich structure and the sandwich structures with trapezoidal, rectangular and random Voronoi cores under the impact load imposed by a rigid body with an initial velocity of 100 m/s. In the early stage of load, the upper half of the core layer of the optimized periodic sandwich structure is completely compressed and the energy absorption is higher than the other three structures. However, the total energy absorption of the optimal sandwich structure is slightly less than the other three due to the small plastic deformation at the end stage of load. To study the capabilities of the topologically optimized structure under different load conditions, the energy absorption performance of the four sandwich structures subjected to the rigid body impact loads at different velocities and three impulse loads were compared. After comprehensively considering the deflection at the centers of top and bottom panels, the specific energy absorption, the ratio of energy absorption of core layer, as well as the mean impact load, it shows that the optimized sandwich periodic structure performs higher energy absorption capability and resistance under the rigid body impact. The specific energy absorption of the optimal sandwich structure is less than the sandwich structure with rectangular core under rectangular impulse, losing advantages of the structural optimization. It indicates that the optimization design obtained under a single load condition cannot get the best performance for any load condition, and further research is required for different load conditions.
-
Key words:
- topology optimization /
- impact load /
- sandwich periodic structure /
- energy absorption
-
钽作为药型罩材料在聚能战斗部中的应用是当前成型装药技术研究的热点问题之一，其研究的核心内容是确定毁伤元成型较佳的药型罩结构参数，实现聚能战斗部的高效毁伤。目前国外灵巧弹药中已成功应用了钽药型罩，如美国的SADARM末敏弹、德国的SMART末敏弹和瑞典的BONUS灵巧炮弹等[1]。针对钽的动力学性能及钽罩侵彻体的应用研究，Zerilli等[2]基于位错动力学建立了钽材料Zerilli-Armstrong本构模型；Bergh等[3]研究发现钽罩爆炸成型弹丸(Explosively Formed Projectile，EFP)的拉伸长度较传统紫铜罩明显提高，并通过X射线试验验证了钽形成EFP毁伤元的可行性。在侵彻行为中，影响侵彻效率最关键的因素为弹靶材料密度比。钽的高密度决定了钽罩EFP的高效毁伤能力，弹道试验表明，Ta的侵彻性能较Cu高30%~35%[4]。Weimann等[5]通过试验和数值模拟对比了钽、铁EFP的侵彻威力，得出同质量下长径比为3的钽EFP毁伤能力可等效长径比为6的铁EFP。Rondot[6]对比研究了空腔钽EFP、密实钽EFP及优化的铁EFP的终点效应，结果表明，相较于铁EFP的侵彻能力，空腔及密实的钽EFP分别提高了14.2%和34.9%。国内前期主要对钽及钽合金的本构关系开展了大量研究，如张廷杰等[7-8]研究了钽-钨合金在高压加载下的动态响应和塑性变形机制；郭扬波等[9]通过改进Z-A模型建立了一种可描述动态应变时效现象的本构模型。上述文献未涉及钽罩结构参数对毁伤元成型及侵彻影响的研究，结合国内外目前对钽罩EFP战斗部的研究现状，开展钽药型罩结构参数对EFP成型及侵彻的控制研究，揭示各结构参数对EFP成型及侵彻的控制规律，确定毁伤性能较佳的钽罩结构参数匹配方案很有必要，以期为钽罩EFP的靶后效应研究提供参考依据。
本研究选取钽作为药型罩材料，采用LS-DYNA仿真软件研究弧锥结合形钽药型罩结构参数对EFP毁伤元成型及侵彻性能的影响规律，确定了EFP毁伤元成型及侵彻性能均较佳的钽罩结构参数组合。
1. 结构设计及方案选取
1.1 钽罩EFP战斗部结构设计
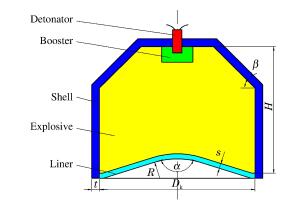
基于对弧锥结合形药型罩及船尾型装药结构的大量研究[10-11]，设计如图 1所示的钽罩EFP战斗部结构，起爆方式为装药中心单点起爆。影响规律研究所涉及的结构参数有药型罩锥角α、药型罩壁厚s和药型罩圆弧半径R，装药及壳体结构参数(装药口径Dk、装药高度H、船尾倾角β和壳体厚度t)设计如表 1所示。
表 1 装药及壳体结构参数设计Table 1. Design of charge and shell’s structural parametersDk/mm H/mm β/(°) t/mm 100 90 45 5 1.2 仿真模型及研究方案
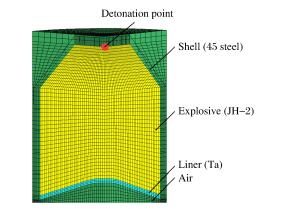
本研究所建立的有限元三维仿真模型如图 2所示。由于成型装药毁伤元的形成存在高应变率、高过载过程，因此仿真中采用ALE算法来计算涉及网格大变形、材料流动问题的聚能侵彻体形成过程，炸药、药型罩、空气采用欧拉算法，炸药、药型罩、空气和壳体间的相互作用采用流固耦合算法。药型罩和壳体材料分别选用钽和45钢，本构方程选用Johnson-Cook模型，状态方程为Grüneisen方程；主装药采用JH-2炸药，状态方程选取JWL (Jones-Wilkins-Lee)方程。钽材料的Johnson-Cook本构方程关键参数见表 2[12]，炸药、空气和壳体的具体参数见表 3[13], 其中：C、S1、S2、S3、γ0为材料特性参数，A为材料准静态下屈服应力，B为应变硬化系数，n为应变硬化指数，m为温度系数，D为爆速，pCJ为Chapman-Jouguet压力。
A/MPa B/MPa n C m 142 164 0.314 8 0.057 0.883 6 Air C γ0 S1 S2 S3 ρ/(kg·m-3) 0.344 1.4 0 0 0 1.25 Shell (45 steel) A/MPa B/MPa C n m ρ/(g·cm-3) 496 434 0.014 0.26 1.03 7.83 Explosive (JH-2) D/(km·s-1) pCJ/GPa A/GPa B/GPa G ρ/(g·cm-3) 8.425 29.66 854.5 2.05 - 1.845 为了获得钽药型罩结构参数对EFP毁伤元成型及侵彻的影响规律，采取保持其余参数值不变，研究单一参数变化的影响规律的方法。选取药型罩锥角α的变化范围为135°~155°(参量增量为4°)、药型罩壁厚s的变化范围为2.0~3.0 mm(参量增量为0.2 mm)、药型罩圆弧半径R的变化范围为40~85 mm(参量增量为5 mm)。
1.3 EFP成型性能指标
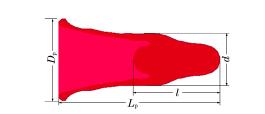
为了便于后续的研究，根据文献[14]，在图 3中给出了EFP成型性能指标的定义。其中：l为绝对实心长度，是指EFP实心部总长；d为绝对实心直径，是指EFP实心部最大直径；Lp为EFP长度，是指EFP总长; Dp为EFP直径，是指EFP最大直径；相对实心长度用l/Lp表示，相对实心直径用d/Dp表示。
2. 钽罩结构参数对EFP性能的控制规律
2.1 成型性能的控制
2.1.1 药型罩锥角的影响
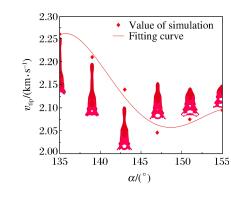
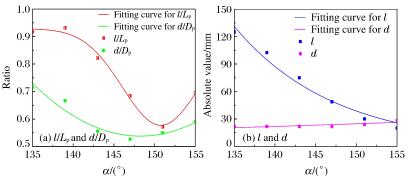
选取s=2.4 mm、R=50 mm，对药型罩锥角α的6个方案进行数值仿真，得出每个方案在200 μs时刻EFP的头部速度vtip及其成型形态，如图 4所示。计算各方案下EFP毁伤元的成型性能指标，得出绝对实心长度l、绝对实心直径d、相对实心长度l/Lp、相对实心直径d/Dp随锥角的变化规律曲线，如图 5所示。
图 5 EFP成型指标随药型罩锥角的变化曲线Figure 5. Variation of molding performance indicator of EFP along α由图 4可知：当药型罩锥角大于143°后，由于药型罩由压垮翻转作用逐渐转变为翻转变形，导致EFP的拉伸长度逐渐减小。在锥角为147°时，侵彻体头部速度变化趋势发生改变的原因也是由于药型罩成型模式转为翻转变形后，随着锥角的增加并接近于爆轰波对平板的作用机理，EFP用于拉伸变形的能量减弱。
由图 5可知：当药型罩锥角α由135°增加至150°时，随着药型罩压合作用的减弱，毁伤元整体的轴向拉伸及径向收缩能力减小，故其相对实心长度及直径逐渐降低；当药型罩锥角α大于150°时，药型罩成型模式转变为翻转变形，毁伤元整体的轴向拉伸及径向收缩能力大幅削弱，导致EFP长度及直径锐减，而绝对实心长度小幅下降，绝对实心直径基本不变，故其相对实心长度及直径略有增加。因此，药型罩锥角主要通过控制药型罩的成型模式，进而控制毁伤元轴向拉伸及径向收缩的能力。
综合分析图 4和图 5：当药型罩锥角小于143°时，虽然侵彻体头部速度及相对实心长度、直径较大，但其头尾速度差过大，易拉断；当药型罩锥角大于147°时，毁伤元绝对实心长度过小，不利于侵彻。因此，综合考虑毁伤元的成型性能，选取钽药型罩锥角α为143°~147°。
2.1.2 药型罩壁厚的影响
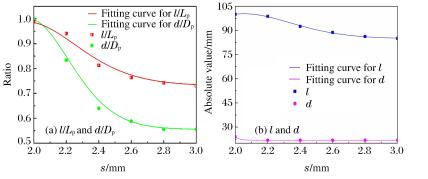
选取α=143°、R=50 mm，对药型罩壁厚的6个方案进行数值仿真，得出各方案在200 μs时刻EFP的头部速度vtip及其成型形态，如图 6所示。计算各方案下EFP毁伤元的成型指标，得出绝对实心长度l、绝对实心直径d、相对实心长度l/Lp、相对实心直径d/Dp随壁厚的变化规律曲线，如图 7所示。
图 7 EFP成型指标随药型罩壁厚的变化曲线Figure 7. Variation of molding performance indicator of EFP along s由图 6可知：随着药型罩壁厚的增加，EFP尾部的断裂现象逐渐减弱，且当药型罩壁厚s由2.0 mm增加至3.0 mm时，EFP的头部速度呈线性减小的变化趋势，且下降了23.8%。
分析图 7：当药型罩壁厚s由2.0 mm增加至2.6 mm时，毁伤元尾部断裂现象不断减弱，尾部外张现象逐渐增强，导致EFP长度、直径逐渐增大，而绝对实心长度及直径小幅减小，故EFP相对实心长度、直径呈现快速下降的趋势；当药型罩壁厚s大于2.6 mm时，毁伤元尾部断裂现象微弱，尾部外张现象不再增强，实心部的轴向拉伸及径向收缩基本不变，故其相对实心长度、直径变化趋势趋于平缓。因此，药型罩壁厚主要控制EFP毁伤元的头部速度及其尾部的断裂与外张情况。
综合分析图 6和图 7：在保证EFP的相对实心长度、直径适中的情况下，考虑选取头部速度相对较大及尾部断裂质量相对较小的毁伤元为较佳毁伤元。形成较佳毁伤元的钽药型罩壁厚s的取值范围为：0.024Dk~0.026Dk。
2.1.3 药型罩圆弧半径的影响
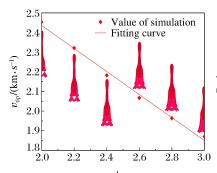
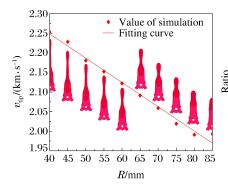
选取α=143°、s=2.4 mm，对药型罩圆弧半径的10个方案进行数值仿真，得出每个方案在200 μs时刻EFP的头部速度vtip及其成型形态，如图 8所示。计算各方案下EFP毁伤元的成型指标，得出相对实心长度l/Lp、相对实心直径d/Dp随圆弧半径的变化规律曲线，如图 9所示。
图 9 EFP成型指标随药型罩圆弧半径的变化曲线Figure 9. Variation of molding performance indicator of EFP along R观察图 8可知：当药型罩圆弧半径大于75 mm后，由于药型罩的成型模式已转变为完全翻转型，故EFP毁伤元的头部射滴现象逐渐减弱至消失，毁伤元形貌变为整体较均匀的长杆形。当药型罩圆弧半径R由40 mm增加至85 mm时，EFP的头部速度呈线性减小的变化趋势，且下降了11.5%。
由图 9可知：当药型罩圆弧半径R由40 mm增加至85 mm时，爆轰波对罩顶部压垮面积逐渐增大，使药型罩圆弧部分各微元获得的初始运动速度不断相近，拉伸时间逐渐缩短，进而导致EFP绝对实心长度大幅减小；在爆轰波对罩顶部压垮面积不断增大的同时，爆轰波对罩锥部的高压加载作用基本不变，使药型罩整体初始运动速度梯度逐渐下降，导致EFP总的拉伸长度有所减小。因此，圆弧半径R由40 mm增加至85 mm时，主要削弱了EFP实心部的轴向拉伸，且其绝对实心长度减小了72.8%。故，EFP相对实心长度随圆弧半径的增加呈现快速下降的变化趋势。当药型罩圆弧半径R大于50 mm后，毁伤元径向收缩能力基本不变，故其相对实心直径变化趋势趋于平缓。因此，药型罩圆弧半径主要控制EFP的头部形态及其绝对实心长度。
综合分析图 8和图 9：在保证EFP的头部速度适中的情况下，考虑选取成型形态相对较好及绝对实心长度相对较大的毁伤元为较佳毁伤元。形成较佳毁伤元的钽药型罩圆弧半径R的取值范围为0.7Dk~0.8Dk。
2.2 侵彻性能的控制
基于上述钽罩结构参数对EFP成型性能的控制研究，针对毁伤元成型较佳的钽罩结构参数取值范围，对EFP侵彻钢靶性能进行正交设计，靶板采用45钢，尺寸为160 mm×160 mm，厚度为200 mm。通过研究钽罩结构参数对EFP侵彻性能的控制规律，得出EFP成型及侵彻性能均较佳的钽罩结构参数组合方案。
2.2.1 正交设计方案
将钽药型罩结构参数(药型罩锥角α、药型罩壁厚s、药型罩圆弧半径R)作为正交设计[15]的3个因素，各因素选取3个水平，得到各因素水平方案见表 4。
表 4 正交设计各因素水平表Table 4. Orthogonal design at each levelLevel Factor α s R 1 143 2.4 70 2 145 2.5 75 3 147 2.6 80 2.2.2 计算结果及分析
正交设计就是从选优区全面水平组合中挑选出具有代表性的部分水平组合进行分析。针对表 4中3因素3水平的情况，可利用正交表L9(其中L表示正交表，9表示表中安排的9种水平组合)进行计算分析。L9保证了因素α的每个水平与因素s、R的每个水平各搭配一次，分布均衡、代表性强，能够较为全面地反映选优区的基本情况。L9及EFP毁伤元侵彻性能指标(侵彻深度P、侵彻孔径D)见表 5。
表 5 正交计算表(200 μs)Table 5. Orthogonal table (200 μs)Project Factor Indicator of penetration performance α s R P/mm D/mm 1 1 1 1 143.26 50.16 2 1 2 2 137.37 52.44 3 1 3 3 135.91 49.36 4 2 1 2 131.38 49.24 5 2 2 3 130.88 49.98 6 2 3 1 148.01 50.12 7 3 1 3 129.46 50.78 8 3 2 1 131.77 52.06 9 3 3 2 131.38 50.88 选取同一时刻的EFP侵彻性能指标进行比较，利用极差分析法[16]对9次仿真结果进行分析，计算各列水平下的极差S，通过S的大小可得到各因素对各指标影响的主次顺序，极差分析结果见表 6，其中：KN (N=1，2，3)表示正交表中各因素下9个方案中所有第N水平对应组合的侵彻性能指标之和。
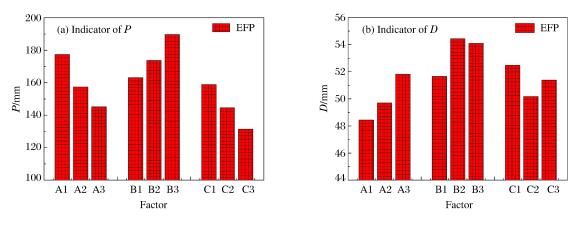
表 6 极差分析表Table 6. Polar difference analysisResult of analysis Indicator of P Indicator of D α s R α s R K1 416.54 404.1 423.04 151.96 150.18 152.34 K2 410.27 400.02 400.13 149.34 154.48 152.56 K3 392.61 415.3 396.25 153.72 150.36 150.12 K1/3 138.85 134.7 141.01 50.65 50.06 50.78 K2/3 136.76 133.34 133.38 49.78 51.49 50.85 K3/3 130.87 138.43 132.08 51.24 50.12 50.04 S 7.98 5.09 8.93 1.46 1.43 0.81 分析可得：药型罩圆弧半径R是影响钽罩EFP毁伤元侵彻深度的最主要因素，各结构参数对钽EFP侵彻深度影响的主次顺序分别为：R、α、s。同样，采用极差分析法计算各因素对钽罩EFP毁伤元侵彻孔径的影响规律。结果表明，药型罩锥角α是影响钽罩EFP毁伤元侵彻孔径的最主要因素，各结构参数对钽EFP侵彻深度影响的主次顺序分别为：α、s、R。
为了分析每个因素中各水平对两个侵彻性能指标的影响情况，现将各指标随因素水平变化的情况用图形表示，如图 10所示。其中，A、B、C分别代表药型罩锥角α、药型罩壁厚s、药型罩圆弧半径R等3个药型罩结构参数，1、2、3分别代表各参数下3个水平，这样可以清楚地表明各因素对每个侵彻性能指标的影响规律和不同因素之间对同一指标的影响差异。
图 10 不同因素水平下的EFP侵彻性能指标Figure 10. Indicator of penetration performance from EFP along factors由于药型罩锥角是影响评价指标最重要的因素，故优先确定锥角的取值。观察图 10可知：药型罩锥角与侵彻深度指标具有负相关性，与侵彻孔径指标具有正相关性，因此综合考虑侵彻体对靶板的侵彻性能，选取药型罩锥角α为145°；药型罩壁厚过小会导致侵彻体尾部断裂现象严重、侵彻深度降低，因此选取药型罩壁厚s为2.6 mm；药型罩圆弧半径与侵彻深度指标具有负相关性，而侵彻孔径在圆弧半径大于70 mm后小幅减小，因此选取药型罩圆弧半径R为70 mm。但考虑到影响侵彻深度指标最重要的因素为头部速度、实心长度，而药型罩锥角及壁厚分别控制了侵彻体的轴向拉伸及头部速度，故两者不可同时选取较大值。综合分析各结构参数对两项侵彻性能指标的影响，选取药型罩锥角α为145°、药型罩壁厚s为2.5 mm、药型罩圆弧半径R为70 mm。故最终确定的参数组合方案为“A2B2C1”，由于正交设计表中不存在此组合方案，因此按照优化后的方案重新进行数值计算，得出该方案下钽罩EFP毁伤元的成型及侵彻性能指标如表 7所示。
表 7 优化方案下钽EFP的成型及侵彻性能参数Table 7. Formation and penetration performance parameters of Ta EFP in optimizationFormulation picture Parameter of forming performance Parameter of penetration performance vtip/(m·s-1) l/Lp d/Dp P/Dk D/Dk 
1 973 0.55 0.67 1.46 0.51 3. 结论
通过仿真研究钽药型罩结构参数对EFP成型及侵彻性能的控制，得到以下结论。
(1) 揭示了钽药型罩结构参数对EFP成型性能的控制规律，其中，药型罩锥角控制EFP的轴向拉伸及径向收缩的能力，药型罩壁厚控制EFP的头部速度及尾部断裂与外张情况，药型罩圆弧半径控制EFP的头部形态及其绝对实心长度。
(2) 获得了EFP成型性能较佳的钽罩结构参数取值范围，其中药型罩锥角为143°~147°，药型罩壁厚、圆弧半径分别为0.024Dk~0.026Dk和0.7Dk~0.8Dk。
(3) 利用正交设计的方法得到了钽罩结构参数对EFP侵彻深度及侵彻孔径影响的主次顺序分别为R、α、s和α、s、R；确定了EFP成型及侵彻性能最佳的钽罩结构参数组合：药型罩锥角为145°，药型罩壁厚、圆弧半径分别为0.025Dk、0.70Dk。
-
图 2 典型的具有m1× m2个单胞的二维周期结构
Figure 2. Representative two dimensional periodic structure with m1× m2 cells
图 3 冲击载荷下固支梁结构示意图
Figure 3. Schematic diagram of fixed sandwich structure subjected to impact load
图 6 不同芯层的夹芯结构示意图
Figure 6. Schematic diagram of sandwich structures with different cores
图 8 冲击载荷下不同夹芯结构的吸能时程曲线
Figure 8. Energy absorption of differnet sandwich structures versus time under impact load
图 9 不同速度的刚体撞击下不同结构上下面板的跨中挠度
Figure 9. Deflections at the center points of top and bottom panels of different sandwich structures under the rigid body impact at different velocities
图 10 不同速度的刚体撞击下夹芯结构的比吸能和芯层吸能占比EC/EW
Figure 10. Comparison of specific energy absorption and EC/EW of sandwich structures under the rigid body impact at different velocities
图 11 不同速度刚体撞击下结构受到的平均冲击力
Figure 11. Mean impact load received by sandwich structures under the rigid body impact at different velocities
图 12 不同脉冲下夹芯结构的变形
Figure 12. Deformations of sandwich structures under different impulse loads
图 13 不同脉冲下不同夹芯结构上下面板的跨中挠度
Figure 13. Deflections at the center points of top and bottom panels of sandwich structures under different impulse loads
图 14 不同脉冲下夹芯结构的吸能性能比较
Figure 14. Comparison of energy absorption of sandwich structures under different impulse loads
表 1 优化结构的上面板挠度与其他3种结构中上面板挠度的最大值的对比
Table 1. Comparison of top panel deflection of optimal structure and the maximum value of other three structures
v0/(m∙s−1) Core with the maximum deflection of the
top panel for other three structuresUt,max/mm Uopt/mm (Ut,max−Uopt)/mm Error/% 30 Rectangular core 5.20 4.17 1.03 19.8 50 Trapezoidal core 11.57 9.84 1.73 15.0 70 Rectangular core 21.28 16.73 4.55 21.4 100 Trapezoidal core 41.90 27.36 14.54 34.7 120 Trapezoidal core 54.28 35.86 18.42 33.9 150 Trapezoidal core 75.40 51.56 23.84 31.6 
下载: 导出CSV
表 2 优化结构的下面板挠度与其他3种结构中最大值的对比
Table 2. Comparison of bottom panel deflection of optimal structure and the maximum value of other three structures
v0/(m∙s−1) Core with the maximum deflection of the
bottom panel for other three structuresUb,max/mm Uopt/mm (Ub,max−Uopt)/mm Error/% 30 Rectangular core 5.05 1.32 3.73 73.9 50 Rectangular core 10.96 3.46 7.50 68.4 70 Rectangular core 18.20 7.08 11.12 61.1 100 Trapezoidal core 30.62 14.86 15.76 51.5 120 Trapezoidal core 49.75 21.47 28.28 56.8 150 Rectangular core 63.48 34.96 28.52 44.9
下载: 导出CSV
-
[1] LI S Q, WU G X, WANG Z H, et al. Finite element simulation of metallic cylindrical sandwich shells with graded aluminum tubular cores subjected to internal blast loading [J]. International Journal of Mechanical Sciences, 2015, 96/97: 1–12. doi: 10.1016/j.ijmecsci.2015.03.011 [2] 张振聪, 张旭, 黄辉秀, 等. 风电叶片夹芯结构的疲劳性能 [J]. 塑料工业, 2021, 49(11): 79–84. doi: 10.3969/j.issn.1005-5770.2021.11.017ZHANG Z C, ZHANG X, HUANG H X, et al. Fatigue behavior of sandwich structure of wind turbine blade [J]. China Plastics Industry, 2021, 49(11): 79–84. doi: 10.3969/j.issn.1005-5770.2021.11.017 [3] 陈昕, 朱锡, 张力军, 等. 雷达防弹天线罩夹芯结构设计与性能研究 [J]. 兵工学报, 2010, 31(10): 1298–1302.CHEN X, ZHU X, ZHANG L J, et al. Design and properties of sandwich structure for ballistic-resistant radome [J]. Acta Armamentarii, 2010, 31(10): 1298–1302. [4] 徐平, 石瑞瑞, 阮文松, 等. 泡沫铝夹芯结构汽车顶板的研究 [J]. 机械科学与技术, 2016, 35(10): 1636–1640. doi: 10.13433/j.cnki.1003-8728.2016.1027XU P, SHI R R, RUAN W S, et al. Studying car roof of aluminum foam sandwich structure [J]. Mechanical Science and Technology for Aerospace Engineering, 2016, 35(10): 1636–1640. doi: 10.13433/j.cnki.1003-8728.2016.1027 [5] MUKHERJEE G S, SARAF M N. Studies on a fiber reinforced plastics honeycomb structure [J]. Polymer Composites, 1994, 15(3): 217–222. doi: 10.1002/pc.750150307 [6] GIBSON L J, ASHBY M F. Cellular solids: structure and properties [M]. Cambridge: Cambridge University Press, 1997: 546. [7] YU J L, WANG X, WEI Z G, et al. Deformation and failure mechanism of dynamically loaded sandwich beams with aluminum-foam core [J]. International Journal of Impact Engineering, 2003, 28(3): 331–347. doi: 10.1016/S0734-743X(02)00053-2 [8] RUAN D, LU G X, WONG Y C. Quasi-static indentation tests on aluminium foam sandwich panels [J]. Composite Structures, 2010, 92(9): 2039–2046. doi: 10.1016/j.compstruct.2009.11.014 [9] JANDAGHI SHAHI V, MARZBANRAD J. Analytical and experimental studies on quasi-static axial crush behavior of thin-walled tailor-made aluminum tubes [J]. Thin-Walled Structures, 2012, 60: 24–37. doi: 10.1016/j.tws.2012.05.015 [10] HANSSEN A G, LANGSETH M, HOPPERSTAD O S. Static and dynamic crushing of circular aluminium extrusions with aluminium foam filler [J]. International Journal of Impact Engineering, 2000, 24(5): 475–507. doi: 10.1016/S0734-743X(99)00170-0 [11] SUN G Y, ZHANG J T, LI S Q, et al. Dynamic response of sandwich panel with hierarchical honeycomb cores subject to blast loading [J]. Thin-Walled Structures, 2019, 142: 499–515. doi: 10.1016/j.tws.2019.04.029 [12] SUN Y L, LI Q M. Dynamic compressive behaviour of cellular materials: a review of phenomenon, mechanism and modelling [J]. International Journal of Impact Engineering, 2018, 112: 74–115. doi: 10.1016/j.ijimpeng.2017.10.006 [13] NURICK G N, LANGDON G S, CHI Y, et al. Behaviour of sandwich panels subjected to intense air blast: part 1: experiments [J]. Composite Structures, 2009, 91(4): 433–441. doi: 10.1016/j.compstruct.2009.04.009 [14] LI S Q, LI X, WANG Z H, et al. Sandwich panels with layered graded aluminum honeycomb cores under blast loading [J]. Composite Structures, 2017, 173: 242–254. doi: 10.1016/j.compstruct.2017.04.037 [15] LI S Q, LI X, WANG Z H, et al. Finite element analysis of sandwich panels with stepwise graded aluminum honeycomb cores under blast loading [J]. Composites Part A: Applied Science and Manufacturing, 2016, 80: 1–12. doi: 10.1016/j.compositesa.2015.09.025 [16] YAHAYA M A, RUAN D, LU G, et al. Response of aluminium honeycomb sandwich panels subjected to foam projectile impact: an experimental study [J]. International Journal of Impact Engineering, 2015, 75: 100–109. doi: 10.1016/j.ijimpeng.2014.07.019 [17] CHEN W J, TONG L Y, LIU S T. Concurrent topology design of structure and material using a two-scale topology optimization [J]. Computers & Structures, 2017, 178: 119–128. [18] BENDSØE M P. Optimal shape design as a material distribution problem [J]. Structural Optimization, 1989, 1(4): 193–202. doi: 10.1007/BF01650949 [19] XIE Y M, STEVEN G P. A simple evolutionary procedure for structural optimization [J]. Computers & Structures, 1993, 49(5): 885–896. [20] HUANG X, XIE Y M. Convergent and mesh-independent solutions for the bi-directional evolutionary structural optimization method [J]. Finite Elements in Analysis and Design, 2007, 43(14): 1039–1049. doi: 10.1016/j.finel.2007.06.006 [21] XIA L, XIA Q, HUANG X D, et al. Bi-directional evolutionary structural optimization on advanced structures and materials: a comprehensive review [J]. Archives of Computational Methods in Engineering, 2018, 25(2): 437–478. doi: 10.1007/s11831-016-9203-2 [22] GUO X, ZHANG W S, ZHANG J, et al. Explicit structural topology optimization based on moving morphable components (MMC) with curved skeletons [J]. Computer Methods in Applied Mechanics and Engineering, 2016, 310: 711–748. doi: 10.1016/j.cma.2016.07.018 [23] ZHOU Y, ZHANG W H, ZHU J H, et al. Feature-driven topology optimization method with signed distance function [J]. Computer Methods in Applied Mechanics and Engineering, 2016, 310: 1–32. doi: 10.1016/j.cma.2016.06.027 [24] WANG M Y, WANG X M, GUO D M. A level set method for structural topology optimization [J]. Computer Methods in Applied Mechanics and Engineering, 2003, 192(1/2): 227–246. [25] LI H, LUO Z, GAO L, et al. Topology optimization for functionally graded cellular composites with metamaterials by level sets [J]. Computer Methods in Applied Mechanics and Engineering, 2018, 328: 340–364. doi: 10.1016/j.cma.2017.09.008 [26] 彭细荣, 隋允康. 考虑破损-安全的连续体结构拓扑优化ICM方法 [J]. 力学学报, 2018, 50(3): 611–621. doi: 10.6052/0459-1879-17-366PENG X R, SUI Y K. ICM method for fail-safe topology optimization of continuum structures [J]. Chinese Journal of Theoretical and Applied Mechanics, 2018, 50(3): 611–621. doi: 10.6052/0459-1879-17-366 [27] YOO S H, CHANG S H, SUTCLIFFE M P F. Compressive characteristics of foam-filled composite egg-box sandwich panels as energy absorbing structures [J]. Composites Part A: Applied Science and Manufacturing, 2010, 41(3): 427–434. doi: 10.1016/j.compositesa.2009.11.010 [28] WANG L S, BASU P K, LEIVA J P. Automobile body reinforcement by finite element optimization [J]. Finite Elements in Analysis and Design, 2004, 40(8): 879–893. doi: 10.1016/S0168-874X(03)00118-5 [29] CAVAZZUTI M, BALDINI A, BERTOCCHI E. High performance automotive chassis design: a topology optimization based approach [J]. Structural and Multidisciplinary Optimization, 2011, 44(1): 45–56. doi: 10.1007/s00158-010-0578-7 [30] MRZYGŁÓD M, KUCZEK T. Uniform crashworthiness optimization of car body for high-speed trains [J]. Structural and Multidisciplinary Optimization, 2014, 49(2): 327–336. doi: 10.1007/s00158-013-0972-z [31] HUANG X, XIE Y M, LU G. Topology optimization of energy-absorbing structures [J]. International Journal of Crashworthiness, 2007, 12(6): 663–675. doi: 10.1080/13588260701497862 [32] DUDDECK F, HUNKELER S, LOZANO P, et al. Topology optimization for crashworthiness of thin-walled structures under axial impact using hybrid cellular automata [J]. Structural and Multidisciplinary Optimization, 2016, 54(3): 415–428. doi: 10.1007/s00158-016-1445-y [33] PATEL N M, KANG B S, RENAUD J E, et al. Crashworthiness design using topology optimization [J]. Journal of Mechanical Design, 2009, 131(6): 061013. doi: 10.1115/1.3116256 [34] SOTO C A. Structural topology optimization for crashworthiness [J]. International Journal of Crashworthiness, 2004, 9(3): 277–283. doi: 10.1533/ijcr.2004.0288 [35] PARK G J. Technical overview of the equivalent static loads method for non-linear static response structural optimization [J]. Structural and Multidisciplinary Optimization, 2011, 43(3): 319–337. doi: 10.1007/s00158-010-0530-x [36] NELSON M F, WOLF JR J A. The use of inertia relief to estimate impact loads [C]//Proceeding of the 2nd International Conference on Vehicle Structural Mechanics. SAE, 1977: 149−155. [37] WU S Z, ZHENG G, SUN G Y, et al. On design of multi-cell thin-wall structures for crashworthiness [J]. International Journal of Impact Engineering, 2016, 88: 102–117. doi: 10.1016/j.ijimpeng.2015.09.003 [38] 孙晓辉, 丁晓红. 结构多目标拓扑优化设计 [J]. 机械设计与研究, 2018, 28(4): 1–4, 9. doi: 10.13952/j.cnki.jofmdr.2012.04.016SUN X H, DING X H. Research on multi-objective topology optimization design methods for structure [J]. Machine Design and Research, 2018, 28(4): 1–4, 9. doi: 10.13952/j.cnki.jofmdr.2012.04.016 [39] DAVIS J R, JOSEPH R. Metals handbook [M]. Ohio: Materials Park, 1998: 878. [40] KARAGIOZOVA D, NURICK G N, LANGDON G S. Behaviour of sandwich panels subject to intense air blasts: part 2: numerical simulation [J]. Composite Structures, 2009, 91(4): 442–450. doi: 10.1016/j.compstruct.2009.04.010 -
下载:
下载:
点击查看大图
计量
- 文章访问数: 453
- HTML全文浏览量: 348
- PDF下载量: 58
