Effects of Heating-Induced Phase Transition on Damage for HMX Crystal
-
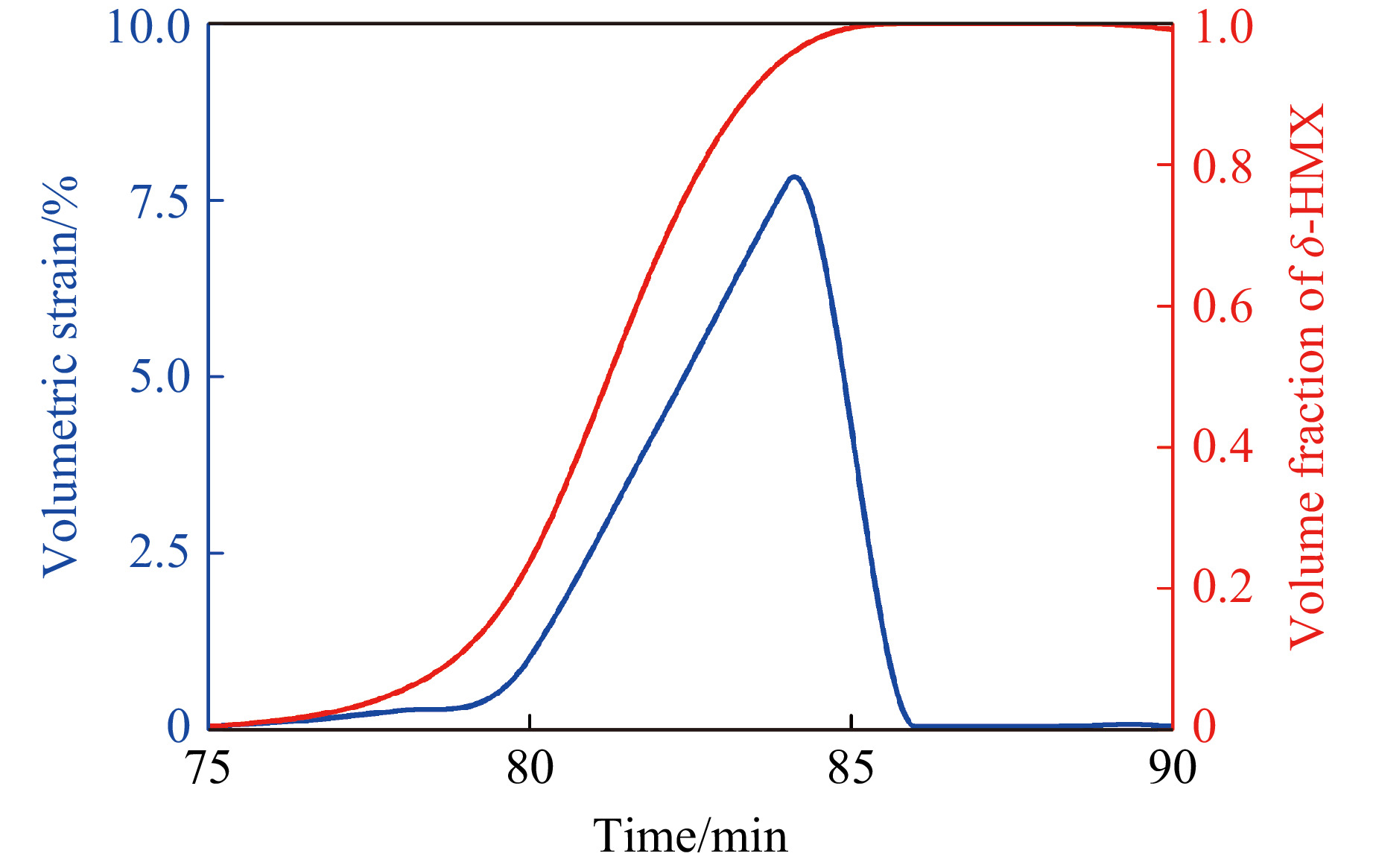
摘要: HMX基PBX炸药混合体系中炸药晶体在发生高温熔化和分解反应之前,会率先发生非均匀热膨胀和固相晶型转变,使材料的力学性能和安全性能发生突变。为探究HMX晶体的热致相变对材料内部损伤演化的影响机制,发展了考虑HMX晶体热膨胀和相变等变形机制的热力耦合晶体本构模型,从力学角度揭示了黏结剂包覆HMX晶体相变对体积变形、应力状态以及裂纹成核演化过程的影响机理,量化分析了升温速率对材料相变和裂纹损伤状态的影响规律。结果表明:随着加载温度升高,HMX晶体的热膨胀和
-
Key words:
- HMX single crystal /
- heating-induced phase transition /
- thermal expansion /
- crack growth /
- damage
-
表 1
Table 1. Elastic constants and temperature coefficients of
C11/GPa C12/GPa C13/GPa C15/GPa C22/GPa C23/GPa C25/GPa 22.2 9.6 13.2 −0.1 23.9 13.0 4.7 C33/GPa C44/GPa C55/GPa C66/GPa C35/GPa C46/GPa /(GPa·K−1) 23.4 9.2 11.1 10.1 1.6 2.5 −0.030 /(GPa·K−1) /(GPa·K−1) /(GPa·K−1) /(GPa·K−1) /(GPa·K−1) /(GPa·K−1) /(GPa·K−1) −0.001 −0.012 0.002 −0.023 −0.018 0.005 −0.003 /(GPa·K−1) /(GPa·K−1) /(GPa·K−1) /(GPa·K−1) /(GPa·K−1) −0.009 −0.004 −0.007 0.003 0.004 表 2
Table 2. Elastic constants and temperature coefficients of
C11/GPa C12/GPa C13/GPa C22/GPa C23/GPa C33/GPa 14.5 10.6 10.3 14.0 10.3 18.0 C44/GPa C55/GPa C66/GPa /(GPa·K−1) /(GPa·K−1) /(GPa·K−1) 4.4 4.4 2.3 −0.014 −0.007 −0.009 /(GPa·K−1) /(GPa·K−1) /(GPa·K−1) /(GPa·K−1) /(GPa·K−1) /(GPa·K−1) −0.014 −0.009 −0.019 −0.006 −0.006 −0.007 表 3 HMX单晶模型的物理参数
Table 3. Physical parameters for HMX single crystal model
Phase /(kg∙m−3) cV/(J∙kg−1∙K−1) α11/K−1 α22/K−1 α33/K−1 α13/K−1 1900 667 −2.90×10−6 1.16×10−4 1.79×10−5 −1.26×10−5 1760 667 6.18×10−5 6.18×10−5 2.47×10−5 表 4 HMX的
Table 4. Parameters related to
i Q/(m3∙mol−1) S*/(J∙mol−1∙K−1) H*/(kJ∙mol−1) V*/(10−5 m3∙mol−1) 1 1.00 144.44 207.691 1.14 −1 1.00 121.68 197.891 0 2 3.0×10−10 149.85 79.700 2.33 −2 3.0×10−10 127.09 69.900 1.19 表 5 界面与HMX晶体内双线性内聚力模型参数
Table 5. Bilinear cohesive model parameters for interface and HMX granular
Position K11/(MPa∙m−1) K22/(MPa∙m−1) K33/(MPa∙m−1) T11/MPa T22/MPa T33/MPa G/(J∙m−2) Interface 1.556 1.556 1.556 1.66 1.66 1.66 80.0 Intra-HMX 106 106 106 2.0 3.0 3.0 100.0 -
[1] ASAY B W. Non-shock initiation of explosives [M]. New York: Springer, 2010. [2] DAI X G, WEN Y S, WEN M P, et al. Projectile impact ignition and reaction violent mechanism for HMX-based polymer bonded explosives at high temperature [J]. Propellants, Explosives, Pyrotechnics, 2017, 42(7): 799–808. doi: 10.1002/prep.201600130 [3] 文玉史, 文雯, 代晓淦, 等. 相变与微裂纹对HMX晶体高温下撞击感度的影响机制 [J]. 含能材料, 2019, 27(3): 184–189. doi: 10.11943/CJEM2018116WEN Y S, WEN W, DAI X G, et al. Influence mechanism of phase transition and micro cracks on impact sensitivity of HMX crystal at high temperature [J]. Chinese Journal of Energetic Materials, 2019, 27(3): 184–189. doi: 10.11943/CJEM2018116 [4] 郜婵, 孙晓宇, 梁文韬, 等. RDX, HMX及CL-20晶体的高温高压相变研究进展 [J]. 含能材料, 2020, 28(9): 902–914. doi: 10.11943/CJEM2020088GAO C, SUN X Y, LIANG W T, et al. Review on phase transition of RDX, HMX and CL-20 crystals under high temperature and high pressure [J]. Chinese Journal of Energetic Materials, 2020, 28(9): 902–914. doi: 10.11943/CJEM2020088 [5] HENSON B F, ASAY B W, SANDER R K, et al. Dynamic measurement of the HMX β-δ phase transition by second harmonic generation [J]. Physical Review Letters, 1999, 82(6): 1213–1216. doi: 10.1103/PhysRevLett.82.1213 [6] HU W J, WU Y Q, HUANG F L, et al. Numerical simulation analyses of β↔δ phase transition for a finite-sized HMX single crystal subjected to thermal loading [J]. RSC Advances, 2018, 8(44): 24873–24882. doi: 10.1039/C8RA02649A [7] WANG X J, WU Y Q, HU W J, et al. Anisotropic mechanical-thermal-phase transformation response of cyclotetramethylene tetranitramine (HMX) single crystal under ramp loading [J]. International Journal of Solids and Structures, 2020, 200: 170–187. doi: 10.1016/j.ijsolstr.2020.05.024 [8] 胡惟佳. 高温下炸药晶体尺度相变效应及损伤点火响应研究 [D]. 北京: 北京理工大学, 2020.HU W J. Phase transition and damage ignition response of explosives under high temperature at the crystal scale [D]. Beijing: Beijing Institute of Technology, 2020. [9] XUE C, SUN J, KANG B, et al. The β-δ phase transition and thermal expansion of octahydro-1, 3, 5, 7-tetranitro-1, 3, 5, 7-tetrazocine [J]. Propellants, Explosives, Pyrotechnics, 2010, 35(4): 333–338. doi: 10.1002/prep.200900036 [10] WILLEY T M, LAUDERBACH L, GAGLIARDI F, et al. Mesoscale evolution of voids and microstructural changes in HMX-based explosives during heating through the β-δ phase transition [J]. Journal of Applied Physics, 2015, 118(5): 055901. doi: 10.1063/1.4927614 [11] 代晓淦. 高温下HMX基PBX炸药撞击响应规律及影响机制研究 [D]. 北京: 北京理工大学, 2018.DAI X G. Impact responses and influence mechanisms of HMX-based polymer-bonded explosives subjected to elevated temperature [D]. Beijing: Beijing Institute of Technology, 2018. [12] HENSON B F, SMILOWITZ L, ASAY B W, et al. The β–δ phase transition in the energetic nitramine octahydro-1, 3, 5, 7-tetranitro-1, 3, 5, 7-tetrazocine: thermodynamics [J]. The Journal of Chemical Physics, 2002, 117(8): 3780–3788. doi: 10.1063/1.1495398 [13] 范正杰, 刘占芳. 升温和降温引起TATB基PBX炸药脱黏的数值分析 [J]. 应用数学和力学, 2020, 41(9): 956–973. doi: 10.21656/1000-0887.410062FAN Z J, LIU Z F. Numerical analysis on debonding of crystal-binder interface in TATB-based polymer-bonded explosive caused by heating and cooling processes [J]. Applied Mathematics and Mechanics, 2020, 41(9): 956–973. doi: 10.21656/1000-0887.410062 [14] TAN H, LIU C, HUANG Y, et al. The cohesive law for the particle/matrix interfaces in high explosives [J]. Journal of the Mechanics and Physics of Solids, 2005, 53(8): 1892–1917. doi: 10.1016/j.jmps.2005.01.009. [15] XIA Q Z, WU Y Q, HUANG F L. Effect of interface behaviour on damage and instability of PBX under combined tension–shear loading [J]. Defence Technology, 2022. -












 下载:
下载: