动能块超高速碰撞多层防护结构的毁伤特性数值模拟

杨玉好; 郭香华; 张庆明

doi:10.11858/gywlxb.20220533

动能块超高速碰撞多层防护结构的毁伤特性数值模拟

doi: 10.11858/gywlxb.20220533

北京理工大学机电学院, 北京　100081

详细信息

作者简介:
杨玉好（1996－），男，硕士研究生，主要从事材料与结构冲击动力学研究.E-mail：15952102687@163.com

通讯作者:
郭香华（1974－），男，副教授，主要从事爆炸与冲击仿真、材料与结构冲击动力学研究.E-mail：guoxh@bit.edu.cn

中图分类号: O385
计量
- 文章访问数: 353
- HTML全文浏览量: 423
- PDF下载量: 56
出版历程
- 收稿日期: 2022-03-15
- 修回日期: 2022-04-13
- 网络出版日期: 2022-07-27
- 刊出日期: 2022-07-28

Numerical Simulation of Damage Characteristics of Multi-Layer Protective Structure under Hypervelocity Impact of Kinetic Energy Block

School of Mechatronical Engineering, Beijing Institute of Technology, Beijing 100081, China

摘要

摘要: 基于有限元-光滑粒子流体动力学（FEM-SPH）自适应算法，采用有限元软件LS-DYNA对动能块超高速碰撞多层防护结构的毁伤特性进行了数值模拟，并结合量纲分析方法，分析了动能块的质量和撞击速度对多层防护结构穿孔特性的影响。结果表明：保持其他参数不变，在所研究的质量和撞击速度范围内，所有的动能块均可以穿透全部17层铝合金板，并在靶后形成碎片云，在撞击过程中动能块和铝合金板内部出现层裂现象；第1层铝合金板的穿孔直径随着动能块质量的增大近似呈幂函数增大，拟合误差在5%以内；第2层铝合金板的穿孔直径随着撞击速度的提升也呈幂函数增大，拟合误差在10%以内；碎片云的头部速度随着撞击速度的提升近似呈线性增大。研究结果可为后期分析靶后碎片云的质量与速度分布、建立冲击载荷模型奠定基础。
- 多层防护结构 /
- 超高速碰撞 /
- 量纲分析 /
- 穿孔特性 /
- 碎片云 /
- 层裂
Abstract: Based on finite element method-smoothed particle hydrodynamics (FEM-SPH) adaptive algorithm of finite element software LS-DYNA, the damage characteristics of a multi-layer protective structure caused by the hypervelocity impact of a kinetic energy block are numerically simulated. Combined with the dimensional analysis method, the effects of the mass and the impact velocity of the kinetic energy block on the perforation characteristics of the multi-layer protective structure are analyzed. The results show that when other parameters remain unchanged and within the range of mass and impact velocity studied in this paper, all kinetic energy blocks can penetrate 17 layers of aluminum alloy plates and form debris clouds behind the target. During the impact process, spallation occurs in the kinetic energy blocks and the aluminum alloy plates. The perforation diameter of the first layer of the aluminum alloy plate increases approximately as a power function with the increase of the mass of the kinetic energy block, and the fitting error is within 5%. The perforation diameter of the second layer of the aluminum alloy plate also increases approximately as another power function with the increase of impact velocity, and the fitting error is less than 10%. The head velocity of the debris cloud increases linearly with the increase of impact velocity. The research results can lay a foundation for analyzing mass and velocity distribution of debris cloud behind target and establishing impact load model.
- multi-layer protective structure /
- hypervelocity impact /
- dimensional analysis /
- perforation characteristics /
- debris cloud /
- spallation

HTML全文

为应对日益复杂的战场环境，需要不断创新毁伤模式。横向效应增强体(Penetrator with Enhanced Lateral Effect, PELE)是一种新型侵彻弹，最早由法德圣路易斯研究所于1996年提出^[1]。PELE弹的典型结构特点是采用密度不同的外壳和内芯，无需引信和装药，其中：外壳一般为高密度材料，如合金钢、钨合金；弹芯为低密度材料，如铝、尼龙、聚乙烯等。弹丸侵彻目标时，由于低密度弹芯的侵彻能力弱，弹芯被压缩产生径向膨胀，弹体内部压力迅速上升；弹丸穿过目标后，内外压力差使壳体断裂，形成破片。由此可见，PELE弹兼具穿甲和破片杀伤效果，具有结构简单、低成本、弹体使用安全等优点，可出色地完成高效毁伤^[2-3]。

传统侵彻弹的毁伤机理和作用特点研究已经相当成熟^[4]，而PELE弹不同于一般的侵彻弹，需要在靶后形成有效破片，其弹体结构的侵彻规律也有自己的特点，因此学者们开展了大量的研究工作。张谋等^[5]通过数值仿真技术分析了PELE弹内芯与横向效应的关联；朱建生等^[6]对PELE弹的破碎机理和弹体结构进行了理论分析；杜忠华等^[7]通过理论分析与数值仿真，认为PELE弹撞击金属薄板时，壳体的横向速度随着弹丸着靶速度和装填材料声阻抗的增大而增大。

在现代战场上，随着目标防护性能的增强，单一功能的弹丸很难达到理想的破坏效果，这就要求进攻模式多元化。为此，本研究提出分段式PELE弹的概念，期望通过分段式PELE弹体结构增强毁伤能力，尤其是应对多层目标。然而，分段式PELE弹的穿靶过程受多种因素影响，本研究采用数值模拟方法重点探讨弹体的侵彻速度和靶板厚度对其终点效应的影响规律，以实现分段式PELE弹应对不同目标时发挥出最强的优势性能。

1. 数值方法与模型

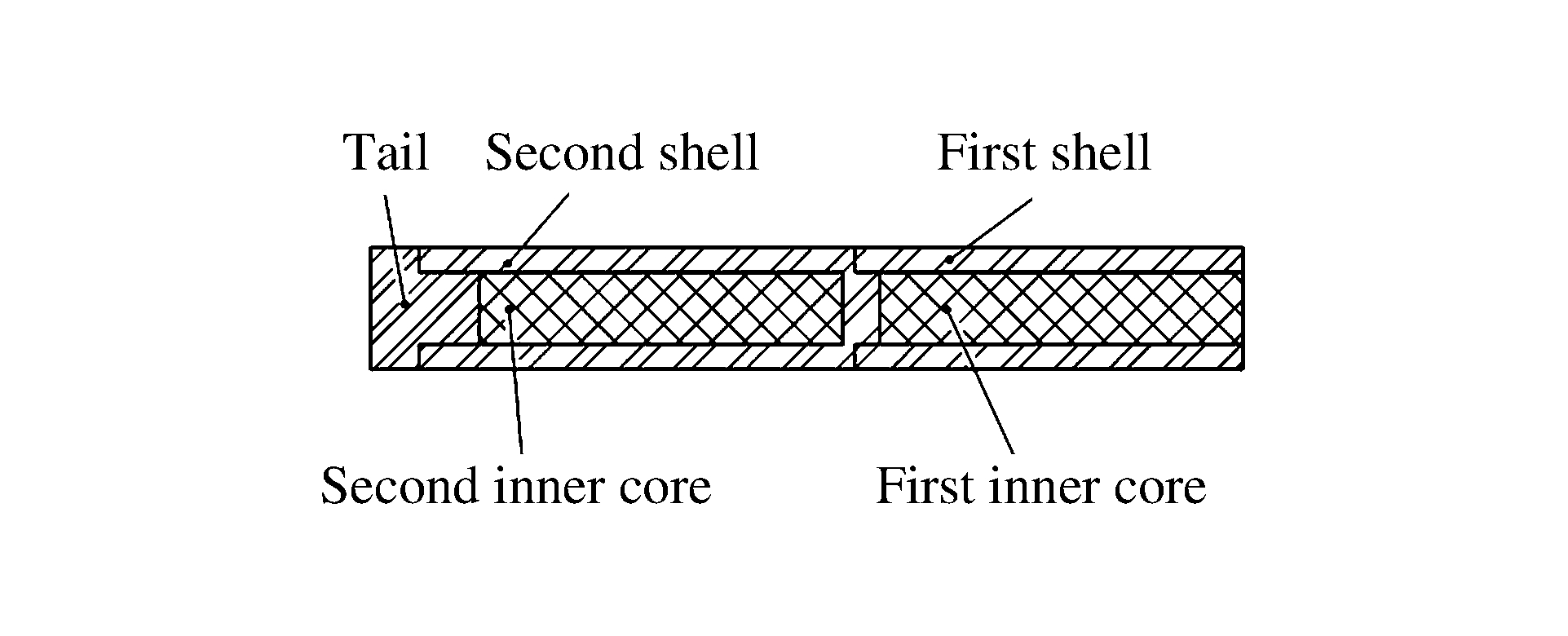
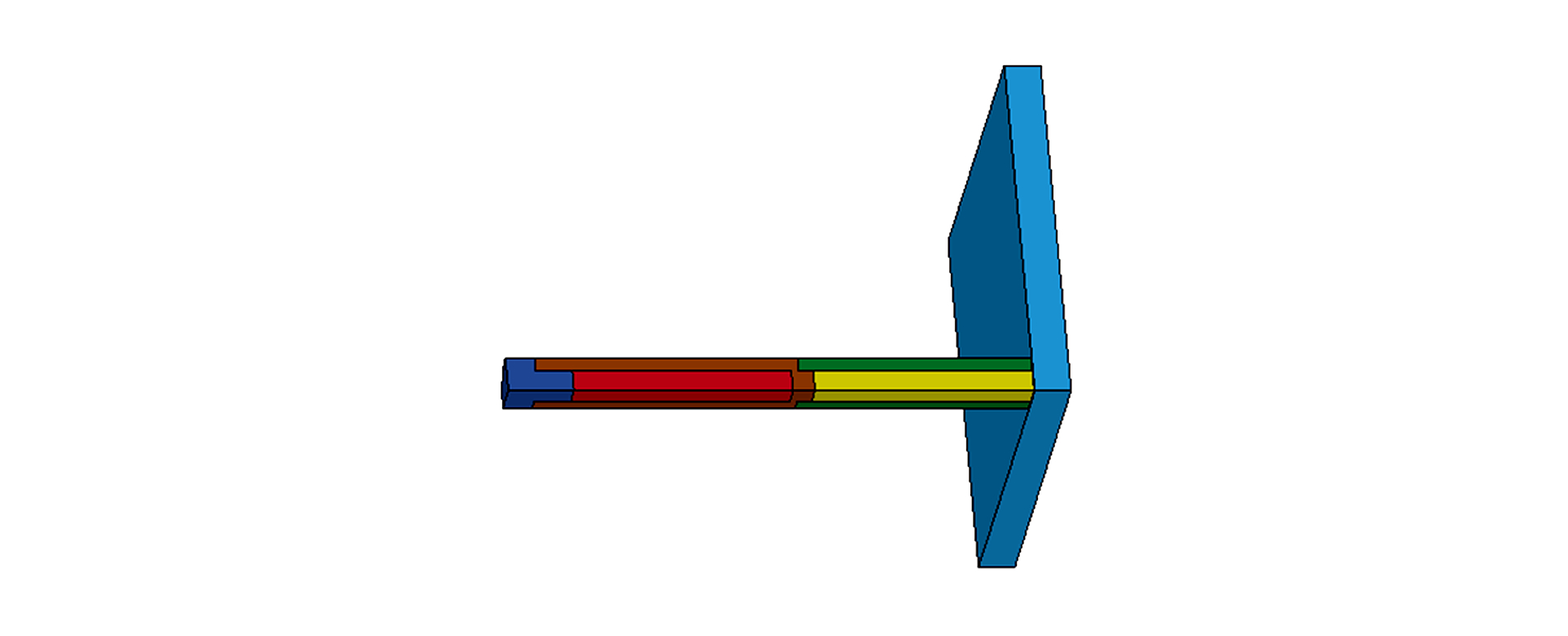
利用非线性动力学软件LS-DYNA进行数值模拟。弹体采用两段PELE结构，用螺纹连接，其结构如图 1所示，有限元模型见图 2。第1段和第2段弹体的长度分别为32和40 mm，外径10 mm，弹芯尺寸为Ø6 mm×30 mm。靶板为4层，每层靶的材料和厚度均相同，设靠近弹丸一侧为第1层。弹壳均采用钨合金，内芯材料为铝，弹尾采用钨合金。弹体材料参数列于表 1，其中ρ₀为密度，E为弹性模量，G为剪切模量，ν为泊松比。

图 1 弹体结构

Figure 1. Projectile structure

下载: 全尺寸图片幻灯片

图 2 有限元模型

Figure 2. Finite element model

下载: 全尺寸图片幻灯片

表 1 材料参数

Table 1. Material parameters

Material	ρ₀/(g·cm^-3)	E/GPa	G/GPa	ν	Constitutive model
Tungsten alloy	17.67	354	138	0.28	Johnson-Cook
Aluminum	2.70	69		0.33	Plastic_Kinematic
921 steel	7.85	210	78	0.30	Johnson-Cook

下载: 导出CSV

| 显示表格

为了提高计算效率，采用1/4模型模拟，约束对称面的位移和转动，设置为轴对称状态。靶板周围边界采用固定约束，限制所有方向的运动。网格尺寸为0.1~0.5 mm，弹体经过区域网格加密，其他区域采用渐变网格。网格单元均采用SOLID164单元，为八节点六面体^[8]。靶板和壳体均采用Johnson-Cook模型和Grüneisen状态方程描述，弹芯采用Plastic_Kinematic模型描述。同时添加Add_Erosion，以控制材料的失效。弹体内部采用Contact_Automatic_Surface_To_Surface接触算法，弹体与靶板之间采用Contact_Eroding_Surface_To_Surface接触算法。

2. 模拟结果与分析

2.1 分段PELE弹与一般PELE弹对比

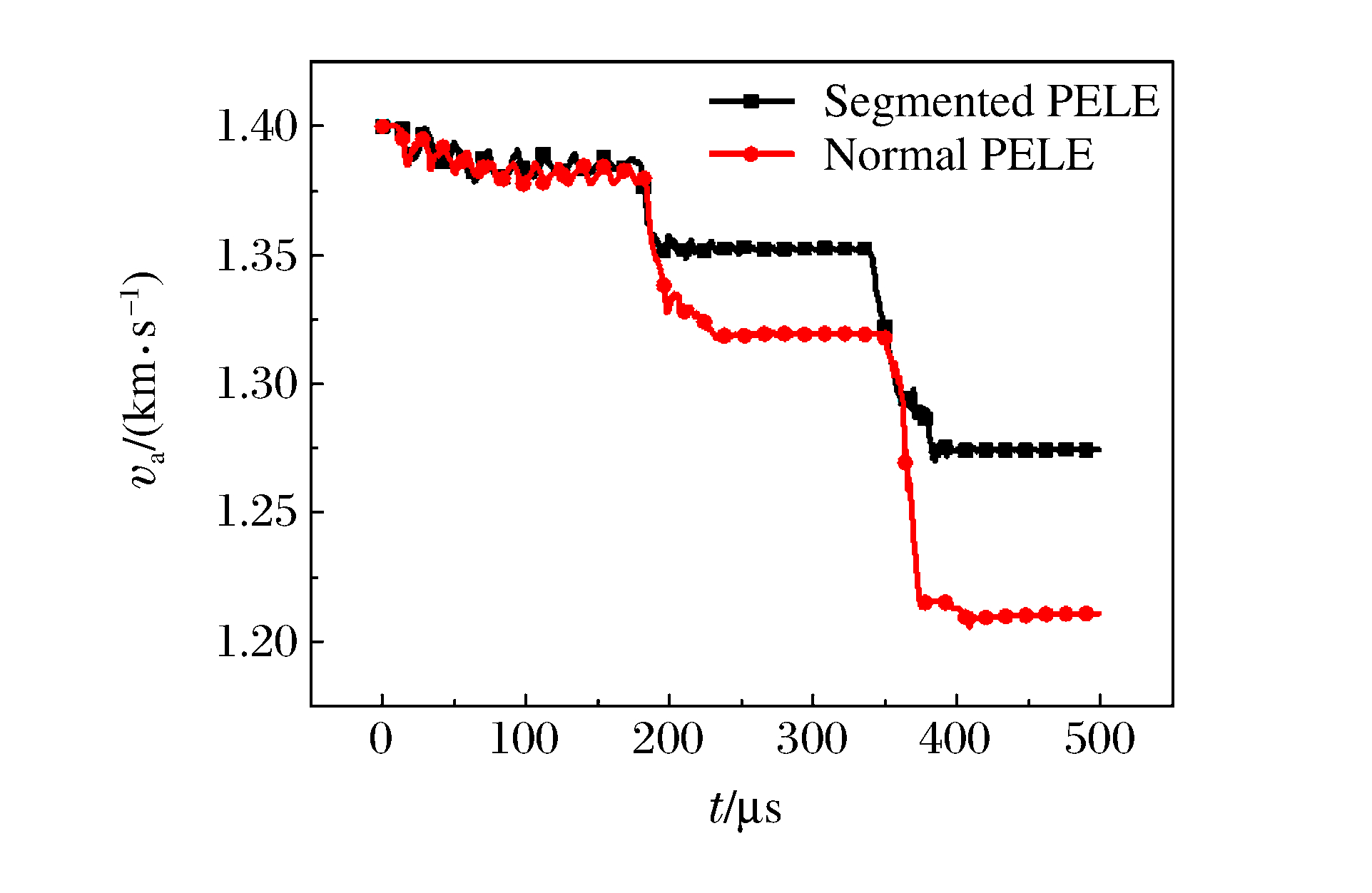
设计一个尺寸与分段PELE弹一致的普通PELE弹进行模拟，入射速度为1.4 km/s，单层靶板厚度为8 mm。两类弹体的轴向速度(v_a)变化曲线如图 3所示。可见：穿过第1层靶时，两种弹的轴向速度变化基本一致；180 μs左右两类弹体穿过第2层靶，轴向速度产生分离；此后，普通PELE弹的轴向速度降幅明显大于分段PELE弹。两类弹穿过4层靶板后，弹壳未破碎长度L_r如表 2所示。结果显示，分段PELE弹主要在第1层与第2层靶板之间以及第3层与第4层靶板之间形成破片。在侵彻多层靶板过程中，由于分段PELE弹在弹芯之间设置壳体间隔保护，能够在一定程度上限制每次穿靶后弹体的破碎长度，保留其后续侵彻和产生破片的能力，因此分段PELE弹在侵彻多层靶过程中的破片分配更合理。相比之下，普通结构的PELE弹在穿过前两层靶板后，弹体基本全部破碎，难以对后续目标产生有效毁伤。对比表明：分段PELE弹相较普通PELE弹，具有更强的侵彻能力。

图 3 弹体轴向速度-时间曲线

Figure 3. Axial velocity-time curve for projectile

下载: 全尺寸图片幻灯片

表 2 弹壳未破裂长度

Table 2. Length of unbroken PELE shell after penetration

Projectile type	L_r/mm
Projectile type	1st layer	2nd layer	3rd layer	4th layer
Segmented PELE	39.75	22.82	5.12	0
Normal PELE	31.49	8.71	0	0

下载: 导出CSV

| 显示表格

2.2 靶板厚度对分段PELE弹终点效应的影响

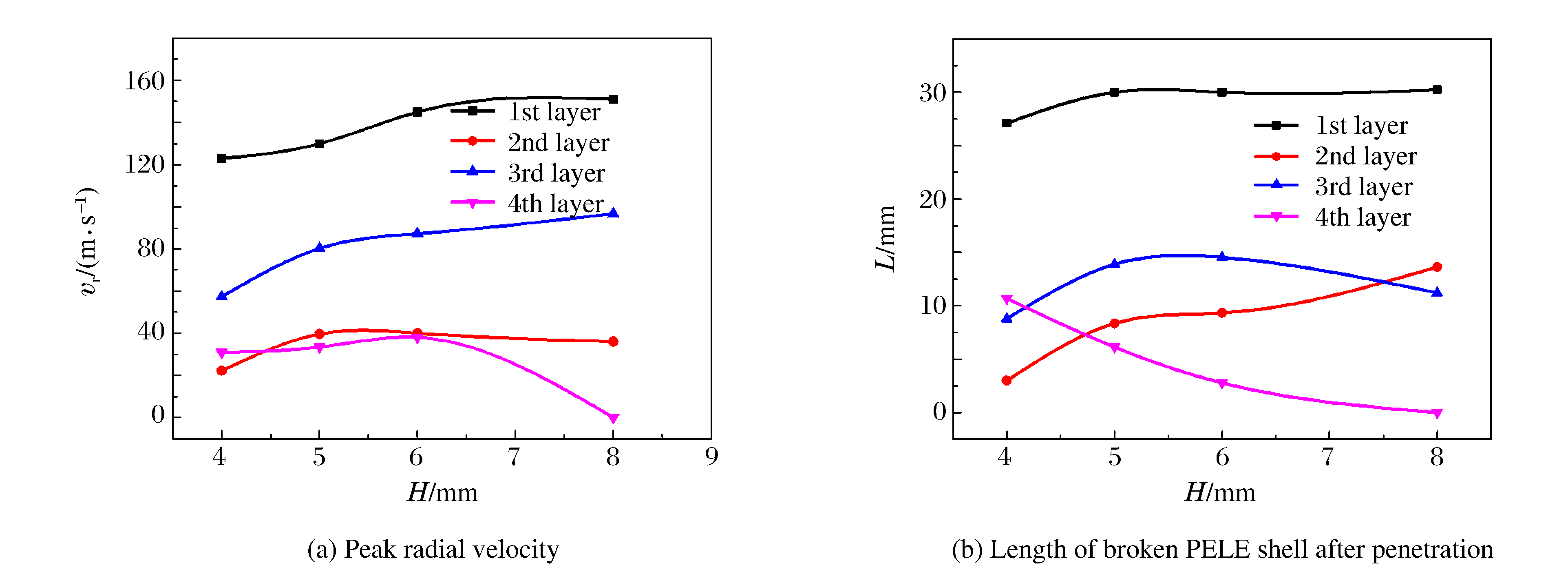
一般来说，PELE弹主要打击较薄的防护壳体及其后方的目标，而靶板厚度对弹丸的终点效应有重要影响^[9]。设弹丸的初始侵彻速度为1.4 km/s，改变单层靶板厚度H(4、5、6和8 mm)，进行数值模拟。不同H下，弹丸穿过各层靶板后壳体径向速度峰值v_r以及壳体破裂长度L如图 4所示。

图 4 不同靶板厚度条件下壳体的径向速度峰值和破裂长度

Figure 4. Peak radial velocity and crushing length of shell under different thicknesses of target plate

下载: 全尺寸图片幻灯片

如图 4(a)所示，随着H的增加，分段PELE弹穿过各层靶板后壳体的径向速度峰值呈现增加趋势；只有当靶板厚度H为8 mm时，弹丸在侵彻第4层靶板过程中完全被侵蚀消耗，无破片产生，速度为零。在H不同的条件下，弹丸穿过多层靶板后壳体径向速度峰值基本逐层递减，但穿过第2层靶时例外，其壳体径向速度峰值最低。由图 4(b)可知，弹丸穿过第1层靶板后第1段弹体几乎完全破碎，表明该阶段产生的破片最多。故而弹体侵彻第2层靶板时，由于破片提前对靶板进行破坏，导致穿靶后弹壳的径向速度峰值相对其他3次较低。

H对弹壳破碎的影响主要体现在后3层靶板的侵彻过程中。增加H会使弹丸在穿过第2层靶板后的破碎长度L增加；穿过第3层靶板后，L多数情况大于穿过第2层靶后，且此时L先随H的增加而增加，当H=8 mm时，因前两次穿靶时L较大，导致第3次穿靶后L反而减少；穿过第4层靶后，L随着H的增加而逐渐变小。由此可见，当H处于5~6 mm区间时，弹丸穿透各层靶后L的分配比较均匀合理。

虽然增加H可使穿靶后壳体的径向速度峰值更大，但也会使弹体在前几次穿靶过程中壳体破碎过多，不利于后续侵彻和毁伤目标。总体而言，H在5~6 mm时，本弹体结构每次穿靶后的壳体径向速度峰值较高，破碎长度均匀，效果最好。

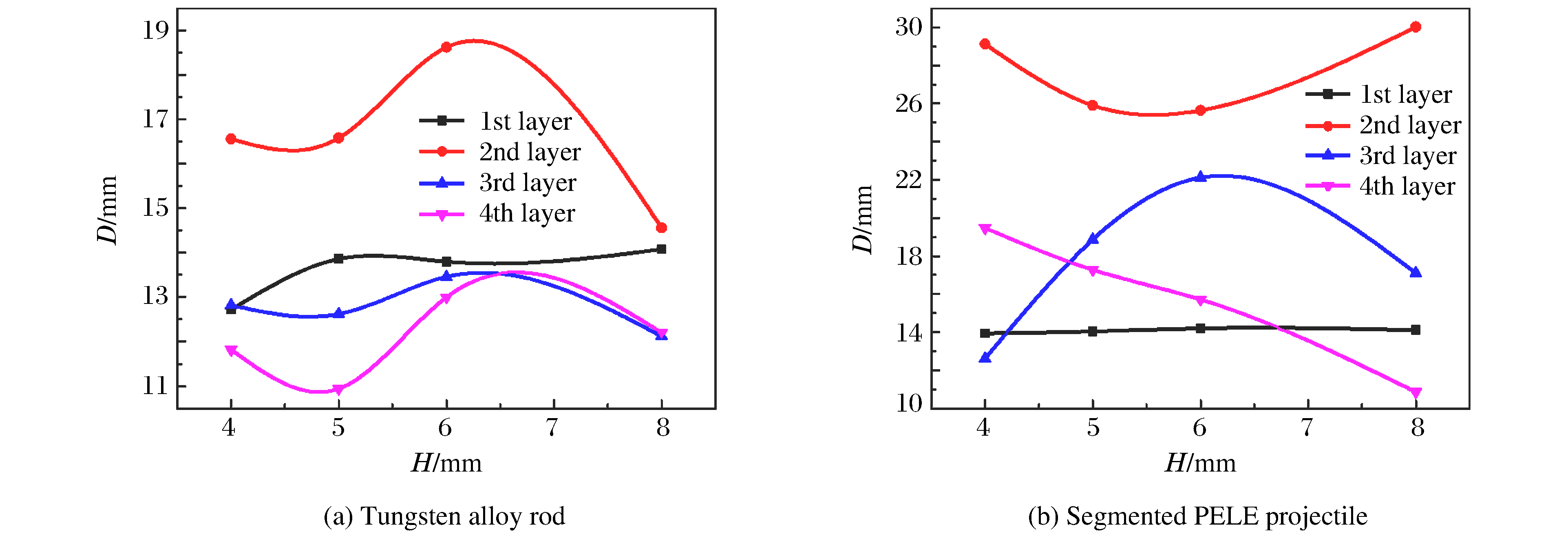
设计一个与分段PELE弹相同尺寸的钨合金杆，以相同的速度侵彻多层靶板。得到两种弹体侵彻不同厚度靶板后的弹孔直径(D)，如图 5所示。从图 5(b)中可以看到，分段PELE弹侵彻不同厚度靶板时，在第2层靶上的弹孔最大。随着H的增加，分段PELE弹在每层靶板上的开孔规律并不一致：对于第1层靶，D随着H的增加略微增大；第2层靶中，H在5~6 mm时D有极小值；第3层靶板中，靶板厚度为6 mm时D达到极大值；而第4层靶板中，D随着H的增加而减小。

图 5 两种弹体侵彻不同厚度靶板的弹孔直径

Figure 5. Layer thickness effect on diameter of crater on multi-layer targets

下载: 全尺寸图片幻灯片

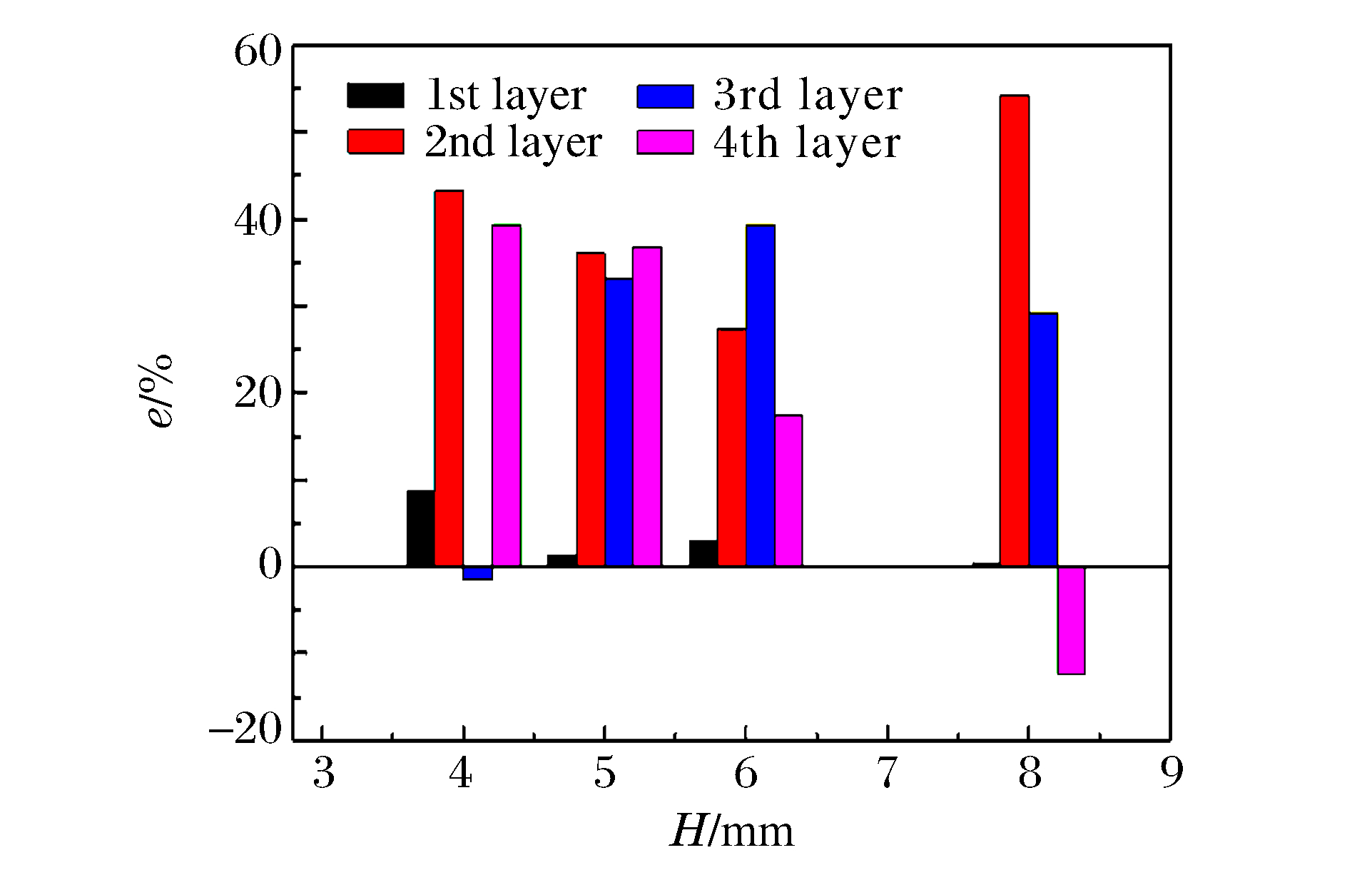
图 6中e表示分段PELE弹与钨合金杆侵彻靶板后弹孔直径的相对偏差，e＞0表示分段PELE弹的弹孔直径更大。数据显示：第1层靶板中的e值均较低，H=4 mm时达到最大值，但此时分段PELE弹的D相对钨合金杆仅有8.67%的提升；对于不同厚度的靶板，分段PELE弹侵彻第2层靶板的D值均远大于钨合金杆，即使对于e最低的6 mm厚靶板，D也增加27.68%，而H=8 mm时，D的增幅达到54.1%；第3层靶板的e值在H=6 mm时取极大值；第4层靶板的e值随着H的增加而减小。

图 6 钨合金杆和分段PELE杆在多层靶中造成的开孔大小差异

Figure 6. Relative difference in diameters of craters caused by tungsten alloy rod and segmented PELE penetration

下载: 全尺寸图片幻灯片

综合来看，当H为5~6 mm时，分段PELE弹侵彻各层靶板时的弹孔均大于钨合金杆，即就相对开孔能力而言，本研究中的分段PELE弹适应的最佳靶板厚度依然是5~6 mm。

2.3 侵彻速度对分段PELE弹终点效应的影响

根据以上分析结果，选定靶板厚度为5 mm，改变弹丸初始速度v₀(0.8、1.1、1.4和1.7 km/s)，进行数值模拟，所得v_r和L随v₀的变化曲线如图 7所示。

图 7 不同侵彻速度下壳体的径向速度峰值和破裂长度

Figure 7. Peak radial velocity (a) and length of broken PELE shell (b) after different velocity penetration

下载: 全尺寸图片幻灯片

图 7(a)显示，弹丸以不同的v₀侵彻靶板时，穿过第1层和第4层靶板后，壳体径向速度峰值随着v₀的增加而增大；而穿过第2层靶后，壳体径向速度峰值相对较低；穿过第2层和第3层靶板后，壳体径向速度峰值在v₀=1.4 km/s时出现极大值，在v₀=1.7 km/s时反而降低。从图 7(b)中也可以看到，当v₀达到1.7 km/s时，弹丸穿过第2层和第3层靶板后壳体破裂很少，此时弹丸的横向效应不显著。这表明在一定的靶板厚度条件下，分段PELE弹的v₀过高反而不利于其在侵彻多层薄靶后产生适量破片。

当H=5 mm、v₀在0.8~1.7 km/s范围内时：弹丸穿过第1层靶板后前段弹体完全破裂，L均为30 mm，体现分段PELE弹可在一定程度上控制破碎范围；之后的几层靶板侵彻过程中，随着侵彻速度的变化，L与v_r的变化趋势一致，径向速度峰值越高，破碎长度越长。综合考虑后认为弹丸以1.4 km/s的初始速度侵彻最佳。

3. 结论

(1) 相对普通PELE弹，分段PELE弹侵彻多层靶板时，壳体破裂产生的破片分布更合理，侵彻能力更强。

(2) 分段PELE弹在侵彻多层金属薄靶后，各层靶的弹孔直径普遍大于相同金属杆侵彻形成的弹孔直径，特别是第2层靶板，其弹孔直径增大超过26%；分段PELE弹在侵彻不同厚度靶板时，均在第2层靶上的弹孔直径最大。

(3) 随着靶板厚度的增加，弹丸贯穿各层靶板后的壳体径向速度峰值一同增加，弹丸侵彻第2层至第4层靶时壳体随靶板厚度的增加而产生更多破碎。随着弹丸初速度的增加，弹丸在穿过第2层和第3层靶板后壳体径向速度峰值和破碎长度均在初始速度为1.4 km/s时达到极大值。一定范围内改变靶板厚度和弹丸初始速度，弹丸贯穿第2层靶后的壳体径向速度峰值最低，而穿过第1层靶板后的弹体破碎长度基本保持不变。

(4) 此分段PELE弹在单层靶板厚度接近一半弹丸口径、初始速度在1.4 km/s附近时有较好的终点效应。

图 1 FEM-SPH自适应算法的计算过程^[19]

Figure 1. Calculation process of FEM-SPH adaptive algorithm^[19]

下载: 全尺寸图片幻灯片

图 2 15.9 µs时刻碎片云数值模拟与实验结果^[24]的比较

Figure 2. Comparison between numerical simulation and experimental result^[24] of debris cloud at 15.9 µs

下载: 全尺寸图片幻灯片

图 3 数值模拟的有限元模型

Figure 3. Finite element model of numerical simulation

下载: 全尺寸图片幻灯片

图 4 工况A1～A7的数值模拟结果

Figure 4. Numerical simulation results of different conditions (Case A1–A7)

下载: 全尺寸图片幻灯片

图 5 动能块和铝合金板内部的冲击波传播（剖视图）

Figure 5. Propagation of shock wave in kinetic energy block and aluminum alloy plate (sectional view)

下载: 全尺寸图片幻灯片

图 6 各层铝合金板的穿孔直径统计（工况A1～A7）

Figure 6. Statistics of perforation diameter of each layer of aluminum alloy plate (Case A1–A7)

下载: 全尺寸图片幻灯片

图 7 工况B1～B5的数值模拟结果

Figure 7. Numerical simulation results of different conditions (Case B1−B5)

下载: 全尺寸图片幻灯片

图 8 碎片云头部速度随动能块速度的变化曲线

Figure 8. Variation of debris cloud head velocity with impact velocity

下载: 全尺寸图片幻灯片

图 9 各层铝合金板的穿孔直径统计（工况B1～B5）

Figure 9. Statistics of perforation diameter of each layer of aluminum alloy plate (Case B1−B5)

下载: 全尺寸图片幻灯片

表 1 钨合金和铝合金的材料模型参数^[19–22]

Table 1. Material parameters of aluminum and tungsten alloy^[19–22]

Material	$\, \rho$ /(g·cm^–³)	$\,\mu$	E/GPa	G/GPa	A/GPa	B/GPa	n
Tungsten alloy	17.000	0.28	409.6	160.0	1.506	0.177	0.12
Al2024-T351	2.785	0.33	73.4	27.6	0.265	0.426	0.34

Material	C	m	T_m/K	T_r/K	D₁	D₂	D₃
Tungsten alloy	0.016	1.0	1723	300	1.5	0	0
Al2024-T351	0.015	1.0	775	300	1.0	0	0

Material	D₄	D₅	c/(km·s⁻¹)	S₁	${\gamma }{_{0}}$	a	${\sigma }{_{\mathrm{p} }}$ /GPa
Tungsten alloy	0	0	4.029	1.237	1.54	0.134	3.5
Al2024-T351	0	0	5.328	1.338	2.00	0.875	2.6

下载: 导出CSV

表 2 15.9 μs时的数值模拟结果与实验数据^[24]的比较

Table 2. Comparison between numerical simulation and experimental result^[24] at 15.9 μs

Method	d_h/cm	v_a/(m·s⁻¹)	v_r/(m·s⁻¹)	L_d/cm	d_d/cm
Simulation	2.00	5320	1860	8.16	6.57
Experiment	1.89	5296	1913	8.11	6.56
Error/%	5.82	0.45	2.77	0.62	0.15

下载: 导出CSV

表 3 数值模拟工况

Table 3. Conditions of numerical simulation

Group	No.	m_p/g	d_p/mm	v_p/(km·s⁻¹)	Group	No.	m_p/g	d_p/mm	v_p/(km·s⁻¹)
A	1	5	8.16	3	B	1	5	8.16	3
	2	45	16.96	3		2	5	8.16	4
	3	85	20.98	3		3	5	8.16	5
	4	125	23.84	3		4	5	8.16	6
	5	165	26.16	3		5	5	8.16	7
	6	205	28.12	3
	7	250	30.04	3

下载: 导出CSV

表 4 第1层铝合金板的穿孔直径

Table 4. Perforation diameter of the first layer of aluminum alloy plate

Case	${d}{_{\mathrm{p} } }$ /mm	${d}{_{\mathrm{h},\mathrm{m}\mathrm{a}\mathrm{x} }}$ /mm	${d}{_{\mathrm{h},\mathrm{m}\mathrm{i}\mathrm{n} } }$ /mm	${d}{_{\mathrm{h} } }$ /mm	${d}{_{\mathrm{h} } }/{d}{_{\mathrm{p} } }$
A1	8.16	22.72	22.68	22.70	2.7819
A2	16.96	39.08	39.00	39.04	2.3019
A3	20.98	47.12	45.98	46.55	2.2188
A4	23.84	51.34	50.96	51.15	2.1456
A5	26.16	56.80	56.72	56.76	2.1697
A6	28.12	58.50	57.74	58.12	2.0669
A7	30.04	62.08	59.62	60.85	2.0256

下载: 导出CSV

表 5 第1层铝合金穿孔直径的计算数据与数值模拟结果的对比

Table 5. Comparison between calculation and simulation of perforation diameter of the first layer of aluminum alloy plate

m_p/g	${d} {_{\mathrm{h} } }/{d}{_{\mathrm{p} } }$		Error/%
m_p/g	Calc.	Sim.	Error/%
25	2.4437	2.4835	−1.60
65	2.2683	2.2320	1.63
105	2.1854	2.0982	4.16
145	2.1312	2.0571	3.60
185	2.0912	2.1155	−1.15
225	2.0594	2.0255	1.67

下载: 导出CSV

表 6 第2层铝合金板的穿孔直径（ ${d}_{\mathrm{p}}$ =8.16 mm）

Table 6. Perforation diameter of the second layer of aluminum alloy plate ( ${d}_{\mathrm{p}}$ =8.16 mm)

Case	${v}{_{\mathrm{p} } }$ /(km·s⁻¹)	${d}{_{\mathrm{h},\mathrm{m}\mathrm{a}\mathrm{x} } }$ /mm	${d}{_{\mathrm{h},\mathrm{m}\mathrm{i}\mathrm{n} } }$ /mm	${d}{_{\mathrm{h} } }$ /mm	${d}{_{\mathrm{h} }}/{d}{_{\mathrm{p} } }$
B1	3	36.50	36.48	36.49	4.4718
B2	4	46.08	45.94	46.01	5.6385
B3	5	54.86	52.84	53.85	6.5993
B4	6	61.16	57.84	59.50	7.2917
B5	7	65.94	61.54	63.74	7.8113

下载: 导出CSV

表 7 第2层铝合金板穿孔直径的计算结果与数值模拟结果的对比

Table 7. Comparison between calculation and simulation of perforation diameter of the second layer of aluminum alloy plate

${v}{_{\mathrm{p} } }$ /(km·s⁻¹)	${d}{_{\mathrm{h} }}/{d}{_{\mathrm{p} }}$		Error/%
${v}{_{\mathrm{p} } }$ /(km·s⁻¹)	Calc.	Sim.	Error/%
3.5	5.0670	4.7426	6.84
4.5	5.9888	6.0515	−1.04
5.5	6.8405	6.4877	5.44
6.5	7.6414	7.3627	3.79

下载: 导出CSV

参考文献(26)

[1]	张羽. 层间距对多冲击结构超高速撞击损伤特性影响研究 [D]. 哈尔滨: 哈尔滨工业大学, 2012. ZHANG Y. Hypervelocity impact damage characteristics research on the impact of protection spacing on multi-shock shields [D]. Harbin: Harbin Institute of Technology, 2012.
[2]	江增荣, 李向荣, 李世才, 等. 预制破片对战斗部冲击起爆数值模拟 [J]. 弹道学报, 2009, 21(1): 9–13. JIANG Z R, LI X R, LI S C, et al. Numerical simulation on shock initiation of performed fragment to warhead [J]. Journal of Ballistics, 2009, 21(1): 9–13.
[3]	梁斌, 冯高鹏, 魏雪婷. 多枚破片冲击引爆带盖板炸药数值模拟分析 [J]. 弹箭与制导学报, 2013, 33(6): 62–66, 69. doi: 10.3969/j.issn.1673-9728.2013.06.018 LIANG B, FENG G P, WEI X T. Numerical simulation on shock initiation of composition explosive of cover board subjected to multi-fragment [J]. Journal of Projectiles, Rockets, Missiles and Guidance, 2013, 33(6): 62–66, 69. doi: 10.3969/j.issn.1673-9728.2013.06.018
[4]	GUPTA N K, MADHU V. An experimental study of normal and oblique impact of hard-core projectile on single and layered plates [J]. International Journal of Impact Engineering, 1997, 19(5/6): 395–414. doi: 10.1016/S0734-743X(97)00001-8
[5]	董永香, 冯顺山, 段相杰. 弹丸斜侵彻多层金属间隔靶特性研究 [J]. 中北大学学报 (自然科学版), 2010, 31(3): 221–226. doi: 10.3969/j.issn.1673-3193.2010.03.004 DONG Y X, FENG S S, DUAN X J. Oblique penetration characteristics of multi-layered spaced targets by steel projectiles [J]. Journal of North University of China (Natural Science Edition), 2010, 31(3): 221–226. doi: 10.3969/j.issn.1673-3193.2010.03.004
[6]	吕珮毅, 张允航, 张曌. 破片形状、着靶姿态对侵彻多层靶影响的数据模拟研究 [J]. 国外电子测量技术, 2021, 40(1): 27–31. doi: 10.19652/j.cnki.femt.2002343 LYU P Y, ZHANG Y H, ZHANG Z. Numerical simulation research on the influence of fragment shape and posture on penetrating multi-layer target [J]. Foreign Electronic Measurement Technology, 2021, 40(1): 27–31. doi: 10.19652/j.cnki.femt.2002343
[7]	屈科佛, 姚勇, 邓勇军, 等. 多层靶板抗不同形状高速破片侵彻性能研究 [J]. 兵器装备工程学报, 2020, 41(2): 6–9. doi: 10.11809/bqzbgcxb2020.02.002 QU K F, YAO Y, DENG Y J, et al. Numerical study on effect of fragment shape on penetration resistance of multi-layered target [J]. Journal of Ordnance Equipment Engineering, 2020, 41(2): 6–9. doi: 10.11809/bqzbgcxb2020.02.002
[8]	DENG Y F, ZHANG W, CAO Z S. Experimental investigation on the ballistic resistance of monolithic and multi-layered plates against ogival-nosed rigid projectiles impact [J]. Materials & Design, 2013, 44: 228–239. doi: 10.1016/j.matdes.2012.06.048
[9]	赵小峰. 破片质量对钨合金破片侵彻威力的影响 [J]. 科学技术与工程, 2020, 20(10): 3967–3971. doi: 10.3969/j.issn.1671-1815.2020.10.025 ZHAO X F. Impact of fragment mass on the penetration capacity of tungsten alloy fragment [J]. Science Technology and Engineering, 2020, 20(10): 3967–3971. doi: 10.3969/j.issn.1671-1815.2020.10.025
[10]	JOHNSON G R. Linking of Lagrangian particle methods to standard finite element methods for high velocity impact computations [J]. Nuclear Engineering and Design, 1994, 150(2/3): 265–274. doi: 10.1016/0029-5493(94)90143-0
[11]	JOHNSON G R, BEISSEL S R, GERLACH C A. Another approach to a hybrid particle-finite element algorithm for high-velocity impact [J]. International Journal of Impact Engineering, 2011, 38(5): 397–405. doi: 10.1016/j.ijimpeng.2011.01.002
[12]	JOHNSON G R, BEISSEL S R, GERLACH C A. A combined particle-element method for high-velocity impact computations [J]. Procedia Engineering, 2013, 58: 269–278. doi: 10.1016/j.proeng.2013.05.031
[13]	RNÁNDEZ-MÉNDEZ S, BONET J, HUERTA A. Continuous blending of SPH with finite elements [J]. Computers and Structures, 2005, 83(17/18): 1448–1458. doi: 10.1016/j.compstruc.2004.10.019
[14]	DE VUYST T, VIGNJEVIC R, CAMPBELL J C. Coupling between meshless and finite element methods [J]. International Journal of Impact Engineering, 2005, 31(8): 1054–1064. doi: 10.1016/j.ijimpeng.2004.04.017
[15]	SAUER M. Simulation of high velocity impact in fluid-filled containers using finite elements with adaptive coupling to smoothed particle hydrodynamics [J]. International Journal of Impact Engineering, 2011, 38(6): 511–520. doi: 10.1016/j.ijimpeng.2010.10.023
[16]	王吉, 王肖钧, 卞梁. 光滑粒子法与有限元的耦合算法及其在冲击动力学中的应用 [J]. 爆炸与冲击, 2007, 27(6): 522–528. doi: 10.11883/1001-1455(2007)06-0522-07 WANG J, WANG X J, BIAN L. Linking of smoothed particle hydrodynamics method to standard finite element method and its application in impact dynamics [J]. Explosion and Shock Waves, 2007, 27(6): 522–528. doi: 10.11883/1001-1455(2007)06-0522-07
[17]	胡德安, 韩旭, 肖毅华, 等. 光滑粒子法及其与有限元耦合算法的研究进展 [J]. 力学学报, 2013, 45(5): 639–652. doi: 10.6052/0459-1879-13-092 HU D A, HAN X, XIAO Y H, et al. Research developments of smoothed particle hydrodynamics method and its coupling with finite element method [J]. Chinese Journal of Theoretical and Applied Mechanics, 2013, 45(5): 639–652. doi: 10.6052/0459-1879-13-092
[18]	胡德安, 孙占华, 朱婷. 三维自适应FE-SPH耦合算法在多层间隔金属靶侵彻问题中的应用 [J]. 爆炸与冲击, 2015, 35(3): 416–422. doi: 10.11883/1001-1455-(2015)03-0416-07 HU D A, SUN Z H, ZHU T. Application of 3D FE-SPH adaptive coupling algorithm to penetration analysis of spaced multi-layered metallic targets [J]. Explosion and Shock Waves, 2015, 35(3): 416–422. doi: 10.11883/1001-1455-(2015)03-0416-07
[19]	HE Q G, CHEN X W, CHEN J F. Finite element-smoothed particle hydrodynamics adaptive method in simulating debris cloud [J]. Acta Astronautica, 2020, 175: 99–117. doi: 10.1016/j.actaastro.2020.05.056
[20]	ROHR I, NAHME H, THOMA K, et al. Material characterisation and constitutive modelling of a tungsten-sintered alloy for a wide range of strain rates [J]. International Journal of Impact Engineering, 2008, 35(8): 811–819. doi: 10.1016/j.ijimpeng.2007.12.006
[21]	张伟, 庞宝君, 贾斌, 等. 弹丸超高速撞击防护屏碎片云数值模拟 [J]. 高压物理学报, 2004, 18(1): 47–52. doi: 10.11858/gywlxb.2004.01.009 ZHANG W, PANG B J, JIA B, et al. Numerical simulation of debris cloud produced by hypervelocity impact of projectile on bumper [J]. Chinese Journal of High Pressure Physics, 2004, 18(1): 47–52. doi: 10.11858/gywlxb.2004.01.009
[22]	郝伟江, 龙仁荣, 张庆明, 等. 球形弹丸超高速撞击靶板时背表面材料破碎的数值模拟分析 [J]. 高压物理学报, 2019, 33(2): 024102. doi: 10.11858/gywlxb.20180651 HAO W J, LONG R R, ZHANG Q M, et al. Numerical simulation analysis of back fragmentation of sphere by hypervelocity impact [J]. Chinese Journal of High Pressure Physics, 2019, 33(2): 024102. doi: 10.11858/gywlxb.20180651
[23]	汪庆桃, 吴克刚, 陈志阳. 圆柱形长杆超高速正碰撞薄板结构破碎效应 [J]. 振动与冲击, 2017, 36(5): 54–60. doi: 10.13465/j.cnki.jvs.2017.05.009 WANG Q T, WU K G, CHEN Z Y. Fragmentation effect of a long cylindrical rod with a hypervelocity normally impacting a thin plate structure [J]. Journal of Vibration and Shock, 2017, 36(5): 54–60. doi: 10.13465/j.cnki.jvs.2017.05.009
[24]	SIBEAUD J M, HÉREIL P L, ALBOUYS V. Hypervelocity impact on spaced target structures: experimental and Ouranos simulation achievements [J]. International Journal of Impact Engineering, 2003, 29(1): 647−658.
[25]	王礼立. 应力波基础 [M]. 2版. 北京: 国防工业出版社, 2005. WANG L L. Foundation of stress waves [M]. 2nd ed. Beijing: National Defense Industry Press, 2005.
[26]	谈庆明. 量纲分析 [M]. 合肥: 中国科学技术大学出版社, 2005. TAN Q M. Dimensional analysis [M]. Hefei: University of Science and Technology of China Press, 2005.