First-Principles Study on Structural, Electronic and Optical Properties of G2ZT Crystal under High Pressure
-
摘要: 基于密度泛函理论的第一性原理研究了高压下富氮含能材料（双3, 4, 5-三氨基-1, 2, 4-三唑）-5, 5′-偶氮四唑（G2ZT）的几何结构、电子结构和光学性质。结果表明，在考虑范德瓦尔斯色散修正和密度泛函色散修正的情况下, 分子晶体结构数据与实验结果的相对误差均在3%以内。Hirshfeld表面分析结果表明，随着压强增大，分子间氢键的相互作用减弱。G2ZT晶体在零压下的能带带隙为2.03 eV，是一种p型半导体。随着压强增大，带隙变窄，吸收系数可达到3.0×106 cm−1。研究结果为进一步分析高压下G2ZT晶体的特征提供了理论参考。Abstract: Geometric structure, electronic structure and optical properties of nitrogen-rich energetic materials (bis 3, 4, 5-triamino-1, 2, 4-triazole)-5, 5′-azotetrazole (G2ZT) at high pressures are investigated using first-principles based on density functional theory. The calculated results obtained by using vdW-DF2 and PBE-D2 methods show that the crystal structure data fit well with the experimental results, and the error rates are all within 3%. Hirshfeld surface analyses indicate that interactions of the inter-molecular hydrogen bond are weaken with the increasing pressure. G2ZT crystal possesses a band gap of 2.03 eV at zero pressure, and it is a p-type semiconductor. As the pressure increases, the band gap becomes narrower and the absorption coefficient can approach 3.0×106 cm−1. These results provide a theoretical reference for further analysis of G2ZT crystal’s characteristics under high pressure.
-
Key words:
- G2ZT crystal /
- high pressure /
- electronic structure /
- optical properties
-
为应对日益复杂的战场环境，需要不断创新毁伤模式。横向效应增强体(Penetrator with Enhanced Lateral Effect, PELE)是一种新型侵彻弹，最早由法德圣路易斯研究所于1996年提出[1]。PELE弹的典型结构特点是采用密度不同的外壳和内芯，无需引信和装药，其中：外壳一般为高密度材料，如合金钢、钨合金；弹芯为低密度材料，如铝、尼龙、聚乙烯等。弹丸侵彻目标时，由于低密度弹芯的侵彻能力弱，弹芯被压缩产生径向膨胀，弹体内部压力迅速上升；弹丸穿过目标后，内外压力差使壳体断裂，形成破片。由此可见，PELE弹兼具穿甲和破片杀伤效果，具有结构简单、低成本、弹体使用安全等优点，可出色地完成高效毁伤[2-3]。
传统侵彻弹的毁伤机理和作用特点研究已经相当成熟[4]，而PELE弹不同于一般的侵彻弹，需要在靶后形成有效破片，其弹体结构的侵彻规律也有自己的特点，因此学者们开展了大量的研究工作。张谋等[5]通过数值仿真技术分析了PELE弹内芯与横向效应的关联；朱建生等[6]对PELE弹的破碎机理和弹体结构进行了理论分析；杜忠华等[7]通过理论分析与数值仿真，认为PELE弹撞击金属薄板时，壳体的横向速度随着弹丸着靶速度和装填材料声阻抗的增大而增大。
在现代战场上，随着目标防护性能的增强，单一功能的弹丸很难达到理想的破坏效果，这就要求进攻模式多元化。为此，本研究提出分段式PELE弹的概念，期望通过分段式PELE弹体结构增强毁伤能力，尤其是应对多层目标。然而，分段式PELE弹的穿靶过程受多种因素影响，本研究采用数值模拟方法重点探讨弹体的侵彻速度和靶板厚度对其终点效应的影响规律，以实现分段式PELE弹应对不同目标时发挥出最强的优势性能。
1. 数值方法与模型
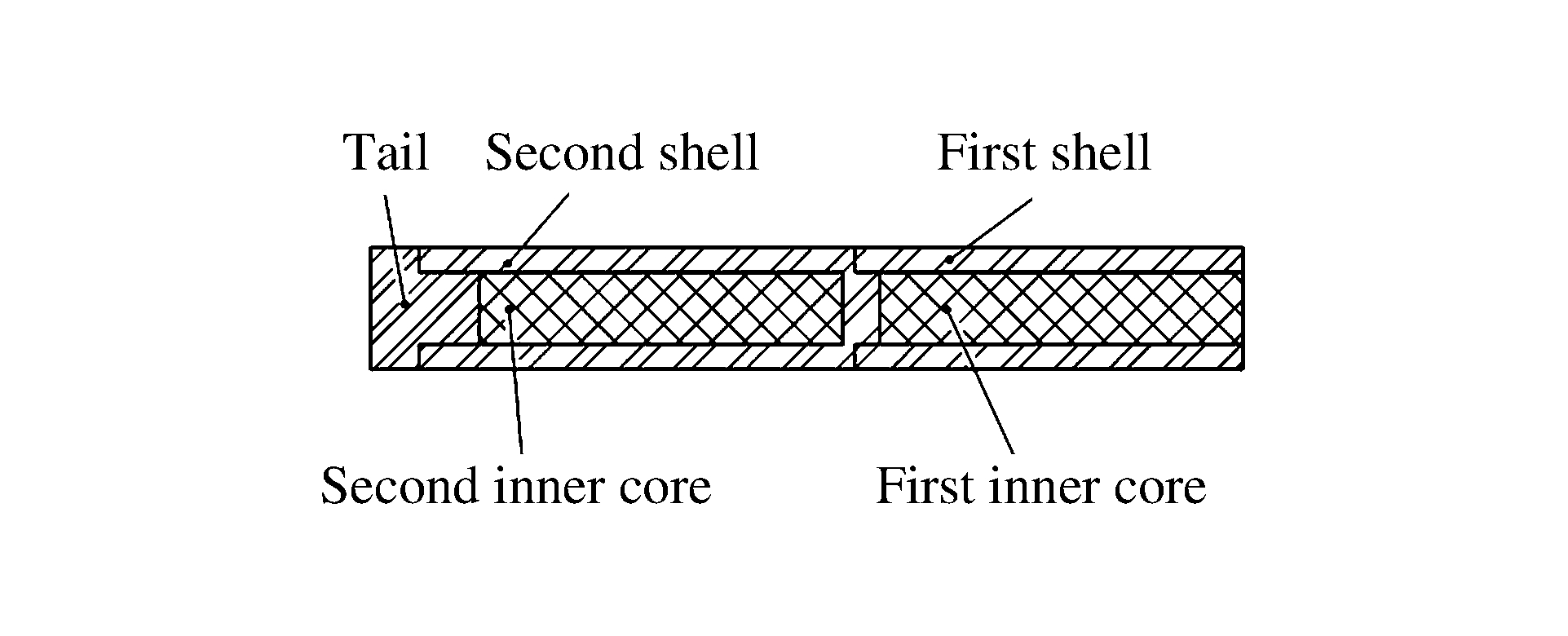
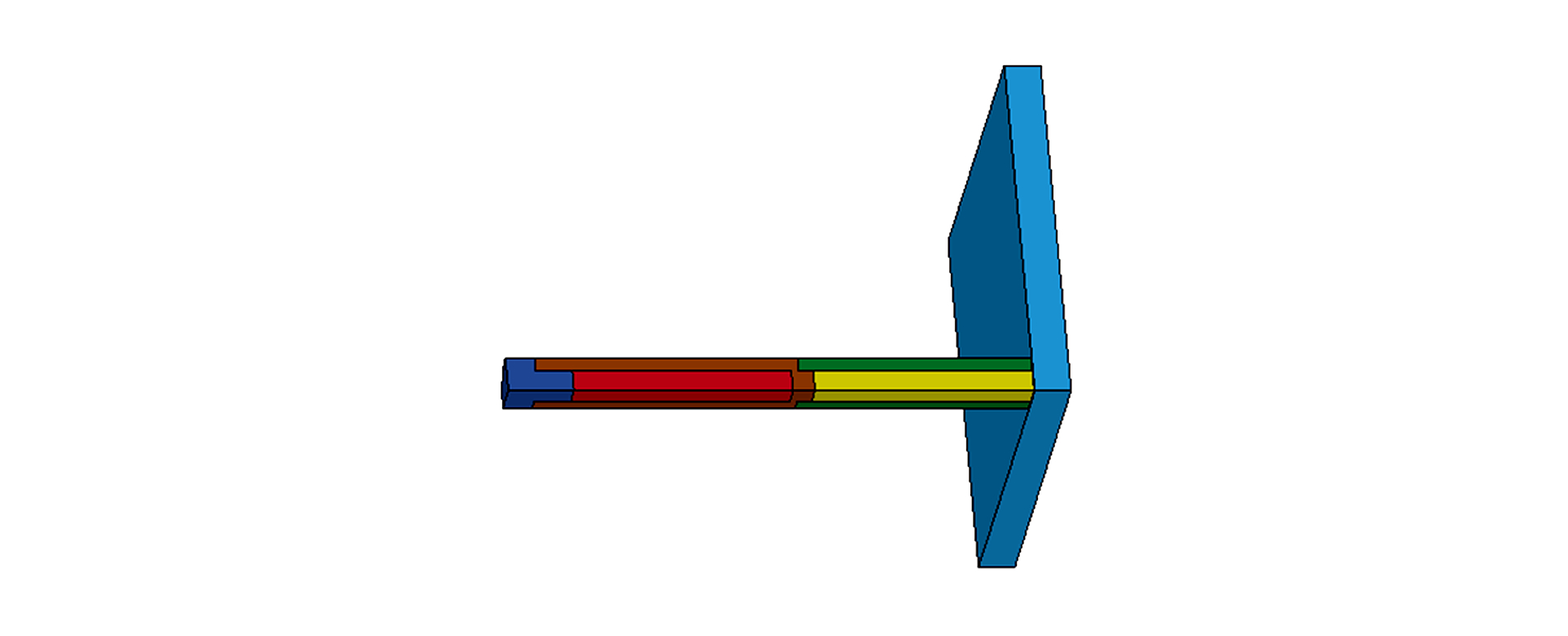
利用非线性动力学软件LS-DYNA进行数值模拟。弹体采用两段PELE结构，用螺纹连接，其结构如图 1所示，有限元模型见图 2。第1段和第2段弹体的长度分别为32和40 mm，外径10 mm，弹芯尺寸为Ø6 mm×30 mm。靶板为4层，每层靶的材料和厚度均相同，设靠近弹丸一侧为第1层。弹壳均采用钨合金，内芯材料为铝，弹尾采用钨合金。弹体材料参数列于表 1，其中ρ0为密度，E为弹性模量，G为剪切模量，ν为泊松比。
表 1 材料参数Table 1. Material parametersMaterial ρ0/(g·cm-3) E/GPa G/GPa ν Constitutive model Tungsten alloy 17.67 354 138 0.28 Johnson-Cook Aluminum 2.70 69 0.33 Plastic_Kinematic 921 steel 7.85 210 78 0.30 Johnson-Cook 为了提高计算效率，采用1/4模型模拟，约束对称面的位移和转动，设置为轴对称状态。靶板周围边界采用固定约束，限制所有方向的运动。网格尺寸为0.1~0.5 mm，弹体经过区域网格加密，其他区域采用渐变网格。网格单元均采用SOLID164单元，为八节点六面体[8]。靶板和壳体均采用Johnson-Cook模型和Grüneisen状态方程描述，弹芯采用Plastic_Kinematic模型描述。同时添加Add_Erosion，以控制材料的失效。弹体内部采用Contact_Automatic_Surface_To_Surface接触算法，弹体与靶板之间采用Contact_Eroding_Surface_To_Surface接触算法。
2. 模拟结果与分析
2.1 分段PELE弹与一般PELE弹对比
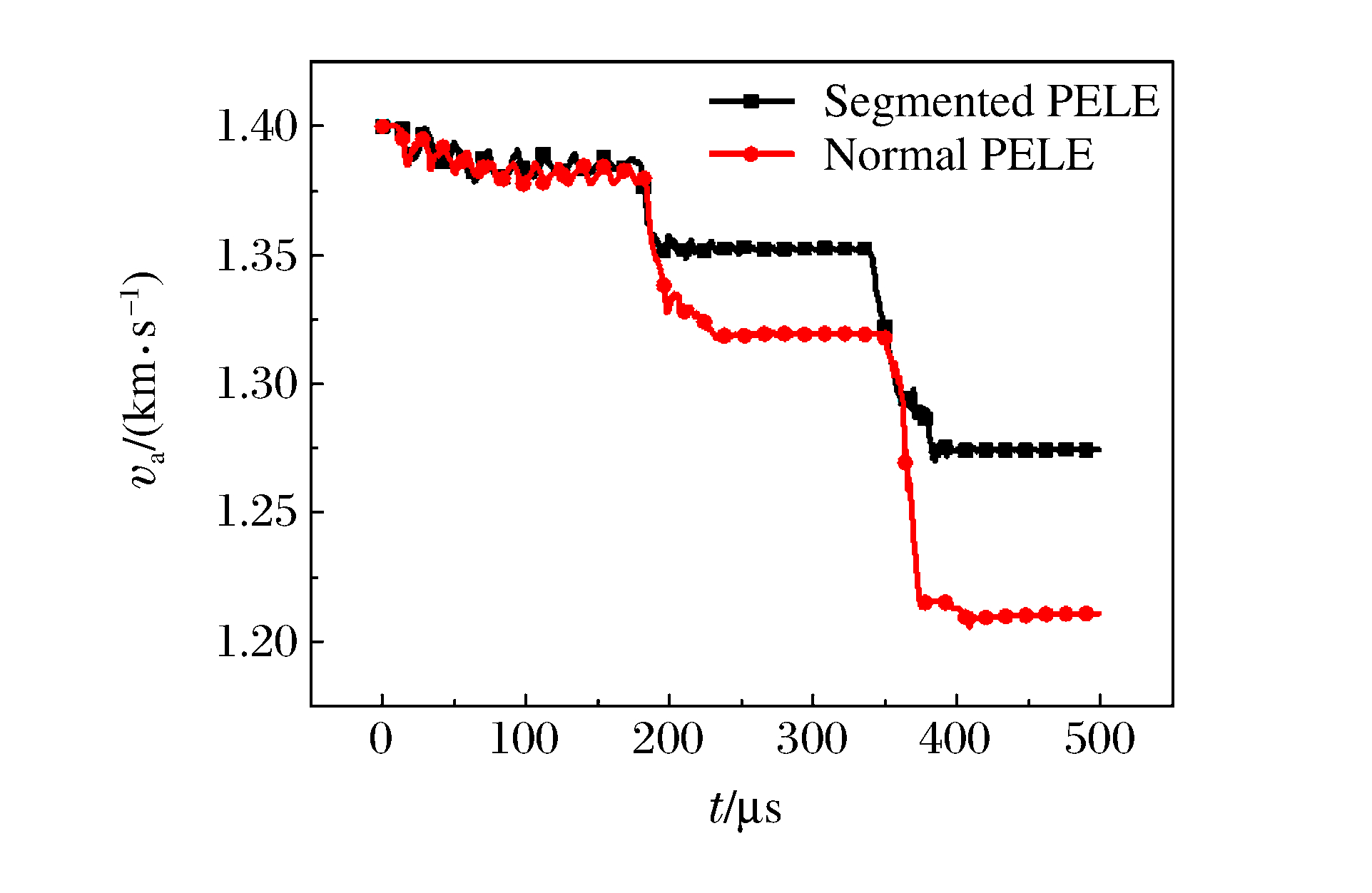
设计一个尺寸与分段PELE弹一致的普通PELE弹进行模拟，入射速度为1.4 km/s，单层靶板厚度为8 mm。两类弹体的轴向速度(va)变化曲线如图 3所示。可见：穿过第1层靶时，两种弹的轴向速度变化基本一致；180 μs左右两类弹体穿过第2层靶，轴向速度产生分离；此后，普通PELE弹的轴向速度降幅明显大于分段PELE弹。两类弹穿过4层靶板后，弹壳未破碎长度Lr如表 2所示。结果显示，分段PELE弹主要在第1层与第2层靶板之间以及第3层与第4层靶板之间形成破片。在侵彻多层靶板过程中，由于分段PELE弹在弹芯之间设置壳体间隔保护，能够在一定程度上限制每次穿靶后弹体的破碎长度，保留其后续侵彻和产生破片的能力，因此分段PELE弹在侵彻多层靶过程中的破片分配更合理。相比之下，普通结构的PELE弹在穿过前两层靶板后，弹体基本全部破碎，难以对后续目标产生有效毁伤。对比表明：分段PELE弹相较普通PELE弹，具有更强的侵彻能力。
表 2 弹壳未破裂长度Table 2. Length of unbroken PELE shell after penetrationProjectile type Lr/mm 1st layer 2nd layer 3rd layer 4th layer Segmented PELE 39.75 22.82 5.12 0 Normal PELE 31.49 8.71 0 0 2.2 靶板厚度对分段PELE弹终点效应的影响
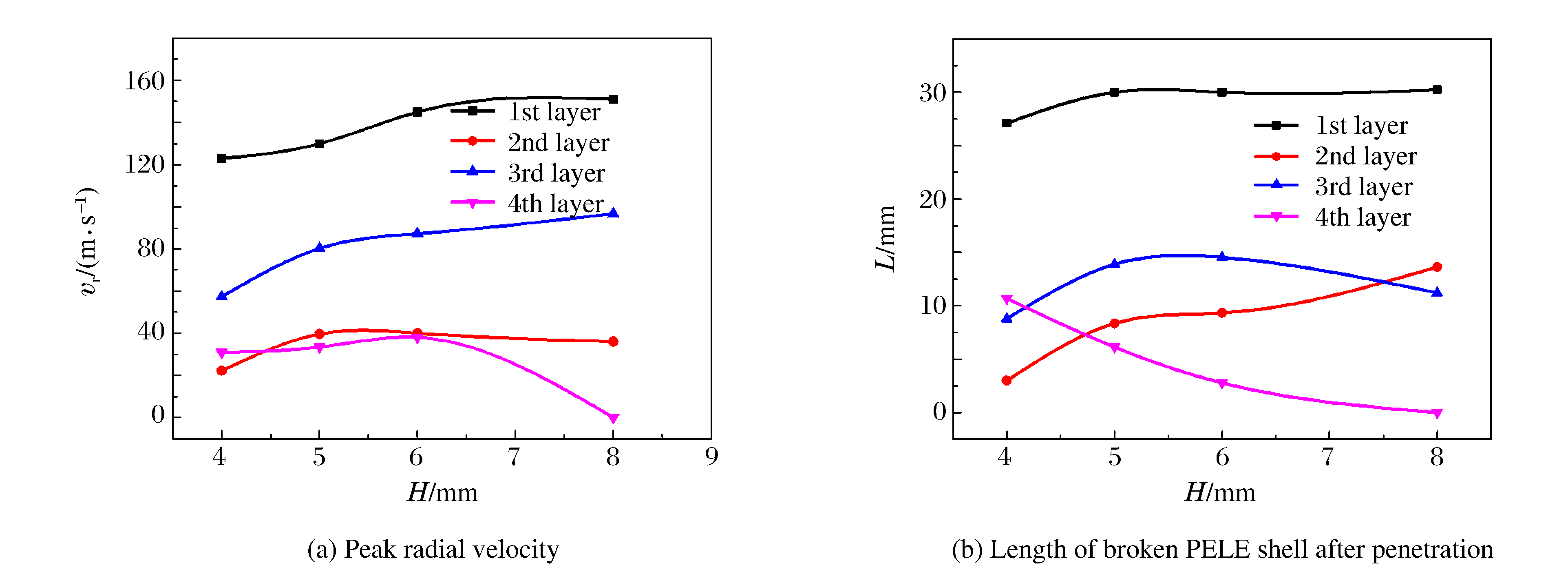
一般来说，PELE弹主要打击较薄的防护壳体及其后方的目标，而靶板厚度对弹丸的终点效应有重要影响[9]。设弹丸的初始侵彻速度为1.4 km/s，改变单层靶板厚度H(4、5、6和8 mm)，进行数值模拟。不同H下，弹丸穿过各层靶板后壳体径向速度峰值vr以及壳体破裂长度L如图 4所示。
图 4 不同靶板厚度条件下壳体的径向速度峰值和破裂长度Figure 4. Peak radial velocity and crushing length of shell under different thicknesses of target plate如图 4(a)所示，随着H的增加，分段PELE弹穿过各层靶板后壳体的径向速度峰值呈现增加趋势；只有当靶板厚度H为8 mm时，弹丸在侵彻第4层靶板过程中完全被侵蚀消耗，无破片产生，速度为零。在H不同的条件下，弹丸穿过多层靶板后壳体径向速度峰值基本逐层递减，但穿过第2层靶时例外，其壳体径向速度峰值最低。由图 4(b)可知，弹丸穿过第1层靶板后第1段弹体几乎完全破碎，表明该阶段产生的破片最多。故而弹体侵彻第2层靶板时，由于破片提前对靶板进行破坏，导致穿靶后弹壳的径向速度峰值相对其他3次较低。
H对弹壳破碎的影响主要体现在后3层靶板的侵彻过程中。增加H会使弹丸在穿过第2层靶板后的破碎长度L增加；穿过第3层靶板后，L多数情况大于穿过第2层靶后，且此时L先随H的增加而增加，当H=8 mm时，因前两次穿靶时L较大，导致第3次穿靶后L反而减少；穿过第4层靶后，L随着H的增加而逐渐变小。由此可见，当H处于5~6 mm区间时，弹丸穿透各层靶后L的分配比较均匀合理。
虽然增加H可使穿靶后壳体的径向速度峰值更大，但也会使弹体在前几次穿靶过程中壳体破碎过多，不利于后续侵彻和毁伤目标。总体而言，H在5~6 mm时，本弹体结构每次穿靶后的壳体径向速度峰值较高，破碎长度均匀，效果最好。
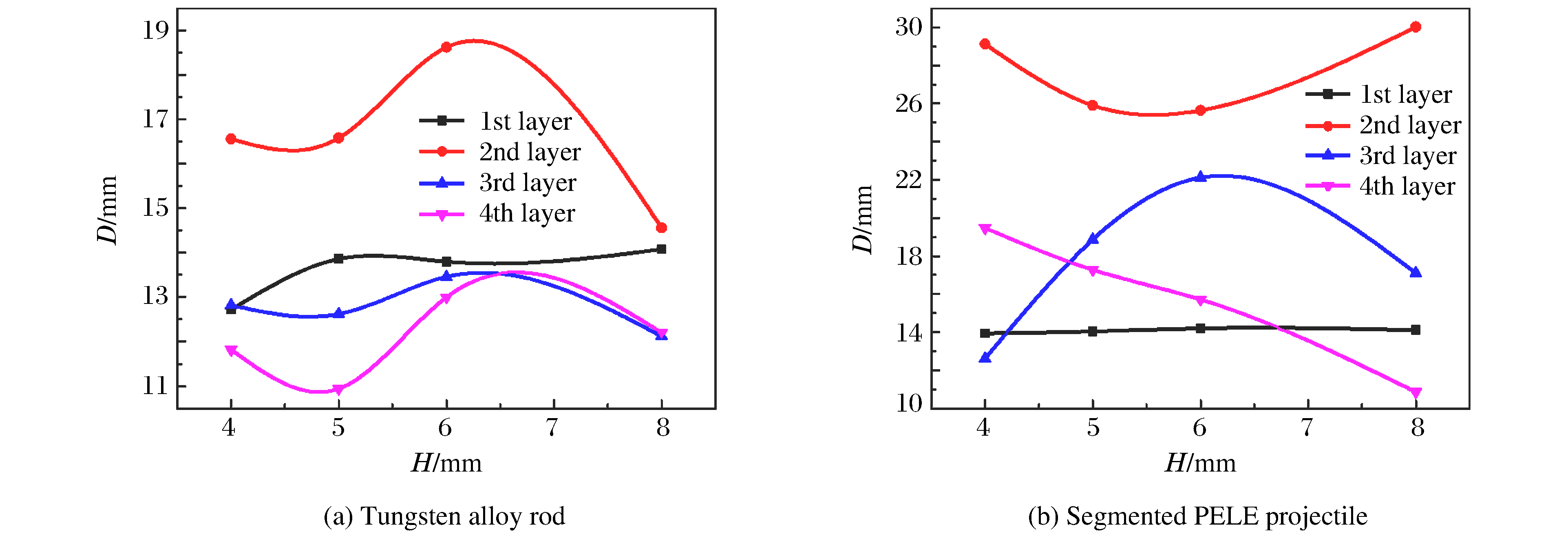
设计一个与分段PELE弹相同尺寸的钨合金杆，以相同的速度侵彻多层靶板。得到两种弹体侵彻不同厚度靶板后的弹孔直径(D)，如图 5所示。从图 5(b)中可以看到，分段PELE弹侵彻不同厚度靶板时，在第2层靶上的弹孔最大。随着H的增加，分段PELE弹在每层靶板上的开孔规律并不一致：对于第1层靶，D随着H的增加略微增大；第2层靶中，H在5~6 mm时D有极小值；第3层靶板中，靶板厚度为6 mm时D达到极大值；而第4层靶板中，D随着H的增加而减小。
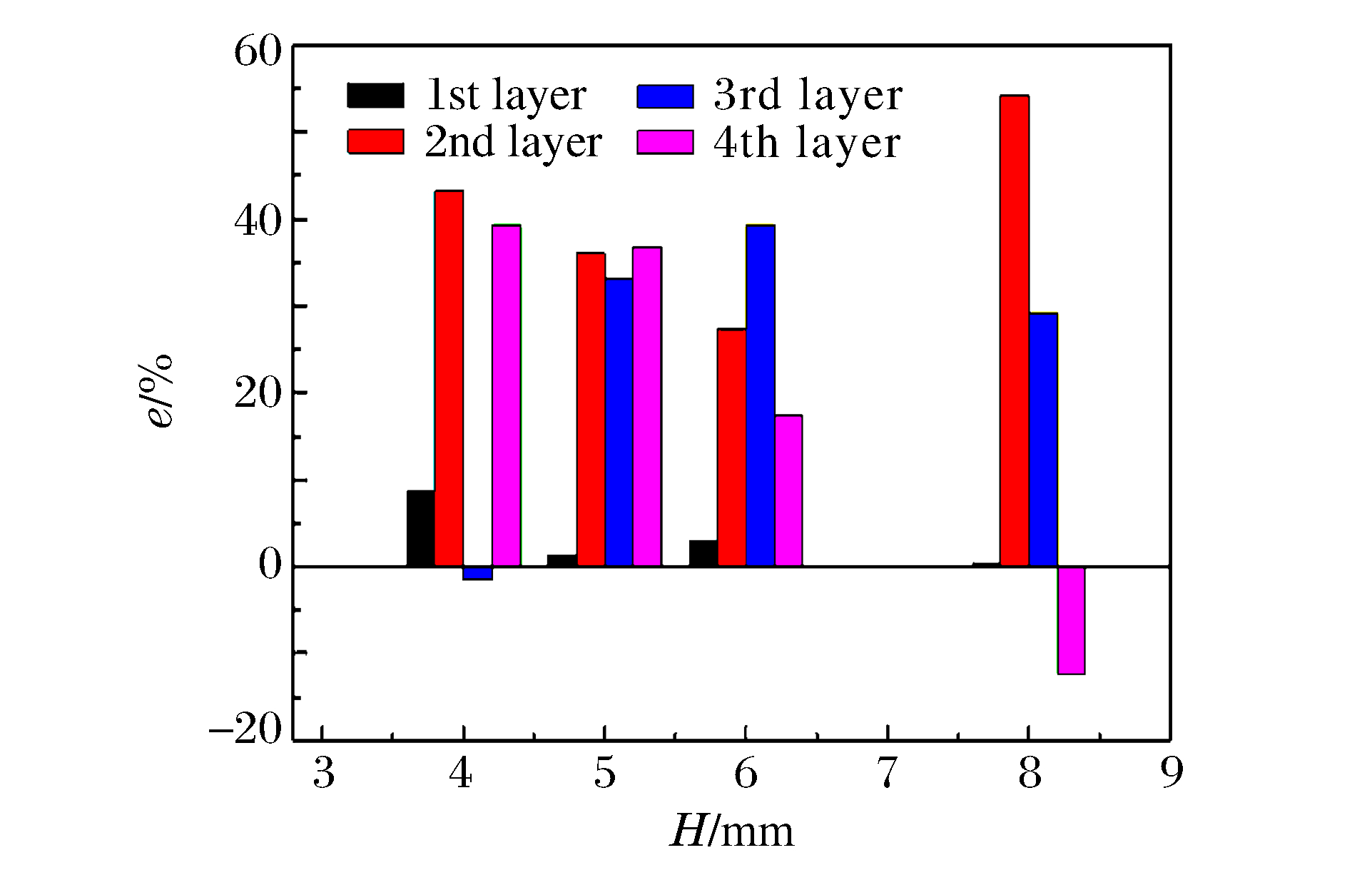
图 5 两种弹体侵彻不同厚度靶板的弹孔直径Figure 5. Layer thickness effect on diameter of crater on multi-layer targets图 6中e表示分段PELE弹与钨合金杆侵彻靶板后弹孔直径的相对偏差，e＞0表示分段PELE弹的弹孔直径更大。数据显示：第1层靶板中的e值均较低，H=4 mm时达到最大值，但此时分段PELE弹的D相对钨合金杆仅有8.67%的提升；对于不同厚度的靶板，分段PELE弹侵彻第2层靶板的D值均远大于钨合金杆，即使对于e最低的6 mm厚靶板，D也增加27.68%，而H=8 mm时，D的增幅达到54.1%；第3层靶板的e值在H=6 mm时取极大值；第4层靶板的e值随着H的增加而减小。
图 6 钨合金杆和分段PELE杆在多层靶中造成的开孔大小差异Figure 6. Relative difference in diameters of craters caused by tungsten alloy rod and segmented PELE penetration综合来看，当H为5~6 mm时，分段PELE弹侵彻各层靶板时的弹孔均大于钨合金杆，即就相对开孔能力而言，本研究中的分段PELE弹适应的最佳靶板厚度依然是5~6 mm。
2.3 侵彻速度对分段PELE弹终点效应的影响
根据以上分析结果，选定靶板厚度为5 mm，改变弹丸初始速度v0(0.8、1.1、1.4和1.7 km/s)，进行数值模拟，所得vr和L随v0的变化曲线如图 7所示。
图 7 不同侵彻速度下壳体的径向速度峰值和破裂长度Figure 7. Peak radial velocity (a) and length of broken PELE shell (b) after different velocity penetration图 7(a)显示，弹丸以不同的v0侵彻靶板时，穿过第1层和第4层靶板后，壳体径向速度峰值随着v0的增加而增大；而穿过第2层靶后，壳体径向速度峰值相对较低；穿过第2层和第3层靶板后，壳体径向速度峰值在v0=1.4 km/s时出现极大值，在v0=1.7 km/s时反而降低。从图 7(b)中也可以看到，当v0达到1.7 km/s时，弹丸穿过第2层和第3层靶板后壳体破裂很少，此时弹丸的横向效应不显著。这表明在一定的靶板厚度条件下，分段PELE弹的v0过高反而不利于其在侵彻多层薄靶后产生适量破片。
当H=5 mm、v0在0.8~1.7 km/s范围内时：弹丸穿过第1层靶板后前段弹体完全破裂，L均为30 mm，体现分段PELE弹可在一定程度上控制破碎范围；之后的几层靶板侵彻过程中，随着侵彻速度的变化，L与vr的变化趋势一致，径向速度峰值越高，破碎长度越长。综合考虑后认为弹丸以1.4 km/s的初始速度侵彻最佳。
3. 结论
(1) 相对普通PELE弹，分段PELE弹侵彻多层靶板时，壳体破裂产生的破片分布更合理，侵彻能力更强。
(2) 分段PELE弹在侵彻多层金属薄靶后，各层靶的弹孔直径普遍大于相同金属杆侵彻形成的弹孔直径，特别是第2层靶板，其弹孔直径增大超过26%；分段PELE弹在侵彻不同厚度靶板时，均在第2层靶上的弹孔直径最大。
(3) 随着靶板厚度的增加，弹丸贯穿各层靶板后的壳体径向速度峰值一同增加，弹丸侵彻第2层至第4层靶时壳体随靶板厚度的增加而产生更多破碎。随着弹丸初速度的增加，弹丸在穿过第2层和第3层靶板后壳体径向速度峰值和破碎长度均在初始速度为1.4 km/s时达到极大值。一定范围内改变靶板厚度和弹丸初始速度，弹丸贯穿第2层靶后的壳体径向速度峰值最低，而穿过第1层靶板后的弹体破碎长度基本保持不变。
(4) 此分段PELE弹在单层靶板厚度接近一半弹丸口径、初始速度在1.4 km/s附近时有较好的终点效应。
-
图 2 G2ZT晶体的晶格参数和体积比随压强的变化
Figure 2. Lattice parameters and V/V0 of G2ZT crystal versus pressure
图 3 C2N10分子在0 GPa下的Hirshfeld面和二维指纹图
Figure 3. Hirshfeld surface and two-dimensional finger patterns of C2N10 molecule at 0 GPa
图 4 C2N10分子在40 GPa下的Hirshfeld面和二维指纹图
Figure 4. Hirshfeld surface and two-dimensional finger patterns of C2N10 molecule at 40 GPa
图 5 G2ZT晶体在不同压强下的能带色散关系
Figure 5. Energy band dispersion of G2ZT crystal at different pressures
图 6 不同压强下G2ZT晶体的态密度分布
Figure 6. Distributions of DOS of G2ZT crystal at different pressures
图 7 不同压强下G2ZT晶体的介电函数随入射能量的变化
Figure 7. Dielectric functions of G2ZT crystal versus incident energy at different pressures
图 8 G2ZT晶体在0和40 GPa压强下的反射率随入射能量的变化
Figure 8. Reflection index of G2ZT crystal versus incident energy at 0 and 40 GPa
图 9 G2ZT晶体在0和40 GPa压强下的折射率随入射能量的变化
Figure 9. Refraction index of G2ZT crystal versus incident energy at 0 and 40 GPa
图 10 G2ZT晶体在0和40 GPa压强下的吸收系数随入射能量的变化
Figure 10. Absorption coefficient of G2ZT crystal versus incident energy at 0 and 40 GPa
表 1 G2ZT晶格参数的计算结果和实验结果比较[2]
Table 1. Calculated crystal lattice parameters of G2ZT compared with experimental data[2]
Method a/Å b/Å c/Å α/(°) β/(°) γ/(°) V/Å3 vdW-DF2 5.3733 6.6044 11.9931 102.36 91.14 109.42 390.1694 PBE-D2 5.3540 6.7920 11.7190 100.23 91.80 110.55 390.6893 Expt.[2] 5.2619 6.6980 11.8840 102.05 90.80 109.96 383.3499 δvdW-DF2/% 2.10 −1.40 0.92 0.30 0.37 −0.49 1.80 δPBE-D2/% 1.70 1.40 −1.40 −1.80 1.10 0.53 1.90 
下载: 导出CSV
表 2 三阶BM和Vinet物态方程拟合得到的G2ZT晶体的体弹模量及其一阶导数
Table 2. Bulk moduli and their pressure-derivatives of G2ZT crystal determined by third-order BM and Vinet equations of state
Method Third-order BM Vinet B0/GPa B′0 B0/GPa B′0 PBE-D2 19.69±0.80 5.50±0.22 18.84±0.63 6.06±0.16 vdW-DF2 27.58±0.76 4.85±0.14 26.48±0.66 5.39±0.13
下载: 导出CSV
表 3 不同压强下第一布里渊区高对称
k 点在价带顶Ev 和导带底Ec 的特征能量Table 3. Characteristic energy values for top of valence band
Ev and bottom of conduction bandEc in highly symmetrick points of the first Brillouin region at different pressuresPressure/GPa Ec/eV Ev/eV R Γ X M R Γ X M 0 2.03 2.25 2.03 2.03 0.00 −0.05 0.00 0.00 3 1.73 2.01 1.73 1.73 0.00 −0.04 0.00 0.00 10 1.33 1.66 1.31 1.33 0.00 −0.09 −0.03 0.00 40 0.93 1.46 0.81 0.91 −0.04 −0.25 −0.18 0.00
下载: 导出CSV
-
[1] KLAPÖTKE T M, SABATÉ C M. Bistetrazoles: nitrogen-rich, high-performing, insensitive energetic compounds [J]. Chemistry of Materials, 2008, 20(11): 3629–3637. doi: 10.1021/cm703657k [2] KLAPÖTKE T M, SABATÉ C M. 5, 5′-Hydrazinebistetrazole: an oxidation-stable nitrogen-rich compound and starting material for the synthesis of 5, 5′-Azobistetrazolates [J]. Zeitschrift für Anorganische und Allgemeine Chemie, 2007, 633(15): 2671–2677. [3] KARAGHIOSOFF K, KLAPÖTKE T M, SABATÉ C M. Nitrogen-rich compounds in pyrotechnics: alkaline earth metal salts of 5, 5′-hydrazine-1, 2-diylbis (1H-tetrazole) [J]. European Journal of Inorganic Chemistry, 2009(2): 238–250. doi: 10.1002/ejic.200800939 [4] EBESPÄCHER M, KLAPÖTKE T M, SABATÉ C M. Nitrogen-rich alkali metal 5, 5′-hydrazinebistetrazolate salts: environmentally friendly compounds in pyrotechnic mixtures [J]. New Journal of Chemistry, 2009, 33(3): 517–527. doi: 10.1039/B818927G [5] DE LUCIA F C JR, GOTTFRIED J L. Characterization of a series of nitrogen-rich molecules using laser induced breakdown spectroscopy [J]. Propellants, Explosives, Pyrotechnics, 2010, 35(3): 268–277. doi: 10.1002/prep.201000009 [6] DREGER Z A, STASH A I, YU Z G, et al. High-pressure structural response of an insensitive energetic crystal: dihydroxylammonium 5, 5′-bistetrazole-1, 1′-diolate (TKX-50) [J]. The Journal of Physical Chemistry C, 2017, 121(10): 5761–5767. doi: 10.1021/acs.jpcc.7b00867 [7] FANG X, STONE M, STENNETT C. Pulsed laser irradiation of a nanoparticles sensitized RDX crystal [J]. Combustion and Flame, 2020, 214: 387–393. doi: 10.1016/j.combustflame.2020.01.009 [8] ISBELL R A, BREWSTER M Q. Optical properties of energetic materials: RDX, HMX, AP, NC/NG, and HTPB [J]. Propellants, Explosives, Pyrotechnics, 1998, 23(4): 218–224. doi: 10.1002/(SICI)1521-4087(199808)23:4<218::AID-PREP218>3.0.CO;2-A [9] ORDEJÓN P, ARTACHO E, SOLER J M. Self-consistent order-N density-functional calculations for very large systems [J]. Physical Review B, 1996, 53(16): R10441–R10444. doi: 10.1103/PhysRevB.53.R10441 [10] SÁNCHEZ-PORTAL D, ORDEJÓN P, ARTACHO E, et al. Density-functional method for very large systems with LCAO basis sets [J]. International Journal of Quantum Chemistry, 1997, 65(5): 453–461. doi: 10.1002/(SICI)1097-461X(1997)65:5<453::AID-QUA9>3.0.CO;2-V [11] SOLER J M, ARTACHO E, GALE J D, et al. The SIESTA method for ab initio order-N materials simulation [J]. Journal of Physics: Condensed Matter, 2002, 14(11): 2745–2779. doi: 10.1088/0953-8984/14/11/302 [12] DION M, RYDBERG H, SCHRÖDER E, et al. Van der Waals density functional for general geometries [J]. Physical Review Letters, 2004, 92(24): 246401. doi: 10.1103/PhysRevLett.92.246401 [13] HAMADA I. Van der Waals density functional made accurate [J]. Physical Review B, 2014, 89(12): 121103. doi: 10.1103/PhysRevB.89.121103 [14] GRIMME S. Semiempirical GGA-type density functional constructed with a long-range dispersion correction [J]. Journal of Computational Chemistry, 2006, 27(15): 1787–1799. doi: 10.1002/jcc.20495 [15] GRIMME S, EHRLICH S, GOERIGK L. Effect of the damping function in dispersion corrected density functional theory [J]. Journal of Computational Chemistry, 2011, 32(7): 1456–1465. doi: 10.1002/jcc.21759 [16] TROULLIER N, MARTINS J L. Efficient pseudopotentials for plane-wave calculations [J]. Physical Review B, 1991, 43(3): 1993–2006. doi: 10.1103/PhysRevB.43.1993 [17] SANKEY O F, NIKLEWSKI D J. Ab initio multicenter tight-binding model for molecular dynamics simulations and other applications in covalent systems [J]. Physical Review B, 1989, 40(6): 3979–3995. doi: 10.1103/PhysRevB.40.3979 [18] JUNQUERA J, PAZ Ó, SÁNCHEZ-PORTAL D, et al. Numerical atomic orbitals for linear-scaling calculations [J]. Physical Review B, 2001, 64(23): 235111. doi: 10.1103/PhysRevB.64.235111 [19] MONKHORST H J, PACK J D. Special points for Brillouin-zone integrations [J]. Physical Review B, 1976, 13(12): 5188–5192. doi: 10.1103/PhysRevB.13.5188 [20] TRAN F, BLAHA P. Accurate band gaps of semiconductors and insulators with a semilocal exchange-correlation potential [J]. Physical Review Letters, 2009, 102(22): 226401. doi: 10.1103/PhysRevLett.102.226401 [21] AMBROSCH-DRAXL C, SOFO J O. Linear optical properties of solids within the full-potential linearized augmented planewave method [J]. Computer Physics Communications, 2006, 175(1): 1–14. doi: 10.1016/j.cpc.2006.03.005 [22] TOLL J S. Causality and the dispersion relation: logical foundations [J]. Physical Review, 1956, 104(6): 1760–1770. doi: 10.1103/PhysRev.104.1760 [23] RIVAS-SILVA J F, BLAS M A, HOAT D M. Theoretical study of electronic and optical properties of antiferromagnetic β-MnS using the modified becke johnson (mBJ) potential [J]. Journal of Physics and Chemistry of Solids, 2017, 128: 310–315. [24] BIRCH F. Finite elastic strain of cubic crystals [J]. Physical Review, 1947, 71(11): 809–824. doi: 10.1103/PhysRev.71.809 [25] VINET P, FERRANTE J, SMITH J R, et al. A universal equation of state for solids [J]. Journal of Physics C: Solid State Physics, 1986, 19(20): L467–L473. doi: 10.1088/0022-3719/19/20/001 [26] MCKINNON J J, JAYATILAKA D, SPACKMAN M A. Towards quantitative analysis of intermolecular interactions with Hirshfeld surfaces [J]. Chemistry Communications, 2007(37): 3814–3816. doi: 10.1039/b704980c [27] SPACKMAN M A, JAYATILAKA D. Hirshfeld surface analysis [J]. CrystEngComm, 2009, 11(1): 19–32. doi: 10.1039/B818330A [28] SPACKMAN P R, TURNER M J, MCKINNON J J, et al. CrystalExplorer: a program for Hirshfeld surface analysis, visualization and quantitative analysis of molecular crystals [J]. Journal of Applied Crystallography, 2021, 54(3): 1006–1011. doi: 10.1107/S1600576721002910 -
下载:
下载:
点击查看大图
计量
- 文章访问数: 400
- HTML全文浏览量: 327
- PDF下载量: 53
