Numerical Study on Wave Effect of the Frictional Interface
-
摘要: 界面摩擦是一种普遍的自然现象。基于摩擦的界面微接触断裂机制,采用线弹性本构关系和D-P破坏准则,建立了包含三角形微凸起的二维界面摩擦模型,采用有限元分析对入射波和摩擦界面的相互作用进行数值模拟。结果表明:在主动加载的微过程中,界面存在显著的应力波动及精细结构特征,波阵面在界面近区域内的演化具有对称扩散性,应力扰动作用于界面微凸起可诱发其断裂,从而以断裂面为中心形成纵波、横波和界面波结构。一个有趣的现象是,在加载的瞬间,界面几乎同步产生了微应力扰动,以纵波形式向基体内传播,更多比较算例和分析证实该扰动产生的物理机制同作用在界面的整体重力微调整有关。该工作揭示了摩擦早期的界面波动效应及其微断裂机制,有望为地震预测提供新的有效途径,从而实现将地震预测时间提前。Abstract: Interface friction is a common natural phenomenon. Based on the micro-contact fracture mechanism of friction, a two-dimensional interface friction model including a triangular micro bulge is established with linear elastic constitutive relationship and D-P failure criterion. The early dynamic behavior of the interface under transient loading is numerically calculated and analyzed by the finite element simulation method. The research shows that in the micro process of loading, there exist significant stress fluctuations and fine structure characteristics at frictional interfaces. The evolution of the wavefront in the near region of the interface has symmetrical diffusion. The interaction of the incoming stress disturbance and the micro bulge will induce the fracture of the bulge, resulting in a three-wave profile centered on the fracture surface: longitudinal wave, transverse wave, and interface wave. A new interesting phenomenon is that at the moment of loading, a micro stress disturbance is generated synchronously from the interface and propagates to the substrate in the form of longitudinal waves. More comparative examples and analysis show that the mechanism of this disturbance is related to the overall gravity micro-adjustment acting on the interface. This work reveals the early wave effect of interface friction and its micro fracture mechanism, which is expected to provide an effective way for earthquake prediction and to advance the earthquake prediction time.
-
Key words:
- wave mechanics /
- interface friction /
- simulation /
- fracture /
- earthquake prediction
-
Metal nitrides have attracted increasing attention in a wide range application due to their fascinating properties, such as hardness, superconductivity, various types of magnetism, etc.[1–2] Recently, extensive efforts have focused on the synthesis and design of metal polynitrides with single and double nitrogen bonds under high pressure, as metal polynitrides are proposed to be promissing candidates for high-energy density materials (HEDMs)[3–5]. The decomposition of these polynitrides is expected to release huge energy due to the conversion from a single/double-bonded polymeric nitrogen (160/418 kJ/mol) into a triple-bonded nitrogen (954 kJ/mol) molecule without producing pollutants. The atomic polymeric nitrogen with cubic gauche structure (cg-N) at a high pressure and high temperature (HPHT) condition (pressure above 110 GPa and temperature greater than 2 000 K) was successfully synthetized by Eremets et al.[6–8]. Motivated by that synthesis, alkali metal azides AN3, constructed by spherical cations and linear molecular
${\mathrm{N}}_3^- $ anions with N=N double bonds, was proposed to be suitable precursor in the formation of polymeric nitrogen under pressure[9]. Experimentally, the polymeric evolutionary behaviors of${\mathrm{N}}_3^- $ anions in LiN3[10], NaN3[11–13], KN3[14–16], RbN3[17–18], and CsN3[19–20] have been fully investigated under high pressure. One of the most interesting findings is the reported polymeric nitrogen nets in NaN3[11] at 160 GPa. More recently, a fully sp2-hybridized layered polymeric nitrogen structure, featuring fused 18-membered rings, in potassium supernitride (K2N16) was successfully synthesized under HPHT condition using a laser heating diamond anvil cell (DAC) technique[21]. In our previous works[22–25], the${\mathrm{N}}_3^- $ anions in LiN3, NaN3, and KN3 all transform firstly to “N6” molecular clusters under compression, and then to a polymerized nitrogen phase at high pressures.Besides the well-known alkali metal azides AN3, alkali metal diazenides A2N2 containing the N=N double bonds, were also proposed as precursors of the polymeric atomic nitrogen under pressure. In 2010, two alkali metal diazenides, Li2N2 and Na2N2, were proposed to have orthorhombic Pmmm structure[26]. However, an orthorhombic Immm structure of Li2N2[27] was then synthesized under HPHT condition. Immediately after that, a serial pressure-induced structural phase transitions of Li2N2 were reported in two independent works[28–29]. Moreover, a high-pressure tetragonal I41/acd phase containing the spiral nitrogen chain was uncovered at 242 GPa[29], indicating that the polymerization of nitrogen is realized from Li2N2.
Compared to Li2N2 and Na2N2, K2N2 have not been reported so far, neither for ambient pressure nor for high pressure. Here, we will extensively explore the high-pressure structures of K2N2 up to 150 GPa by using the first principles swarm structure searching method. The structure evolutions and chemical bonding behaviors of K2N2 within different structures were then fully studied to provide an insight into the formation of polymeric nitrogen in alkali metal diazenides.
1. Computational Method
The variable-cell crystal structure predictions for K2N2 (1–4 formula unit (f. u.) in the simulation cell) up to 150 GPa were conducted by CALYPSO code[30–31]. The effectiveness of this method has been fully confirmed in predicting high-pressure structures of various systems[32–35]. During the structure search process, the 60% structures of each generation with lower enthalpies were selected to generate the structures for the next generation by particle swarm optimization (PSO) technique, and the other structures in new generation were randomly generated to increase the structural diversity. Usually, the structure searching simulation stopped at the 20th generation and 40 structures per generation were generated at each pressure point. After finishing the structure search, the most stable structures were used for further structural relaxation and property calculation, a process that is implemented in the Vienna ab Initio Simulation Package[36]. The Perdew-Burke-Ernzerhof (PBE) exchange-correlation functional under the generalized gradient approximation[37] and the projector-augmented wave (PAW)[38] for ion-electron potentials were adopted herein. The van-der-Waals (vdW) correction was applied in the structural relaxation using Grimme’s method (DFT-D3)[39]. The cutoff energy of 550 eV and Monkhorst-Pack k meshes[40] of 2π×0.032 Å−1 were used to ensure the good convergences of total energy and maximum force on each atom. The lattice dynamic stability of each structure was checked by phonon spectrum obtained by PHONOPY code[41]. The charge transfer behavior of K2N2 was performed using Bader atoms-in-molecules (AIM) method[42].
2. Results and Discussion
2.1 Ground-State and High-Pressure Structures of K2N2
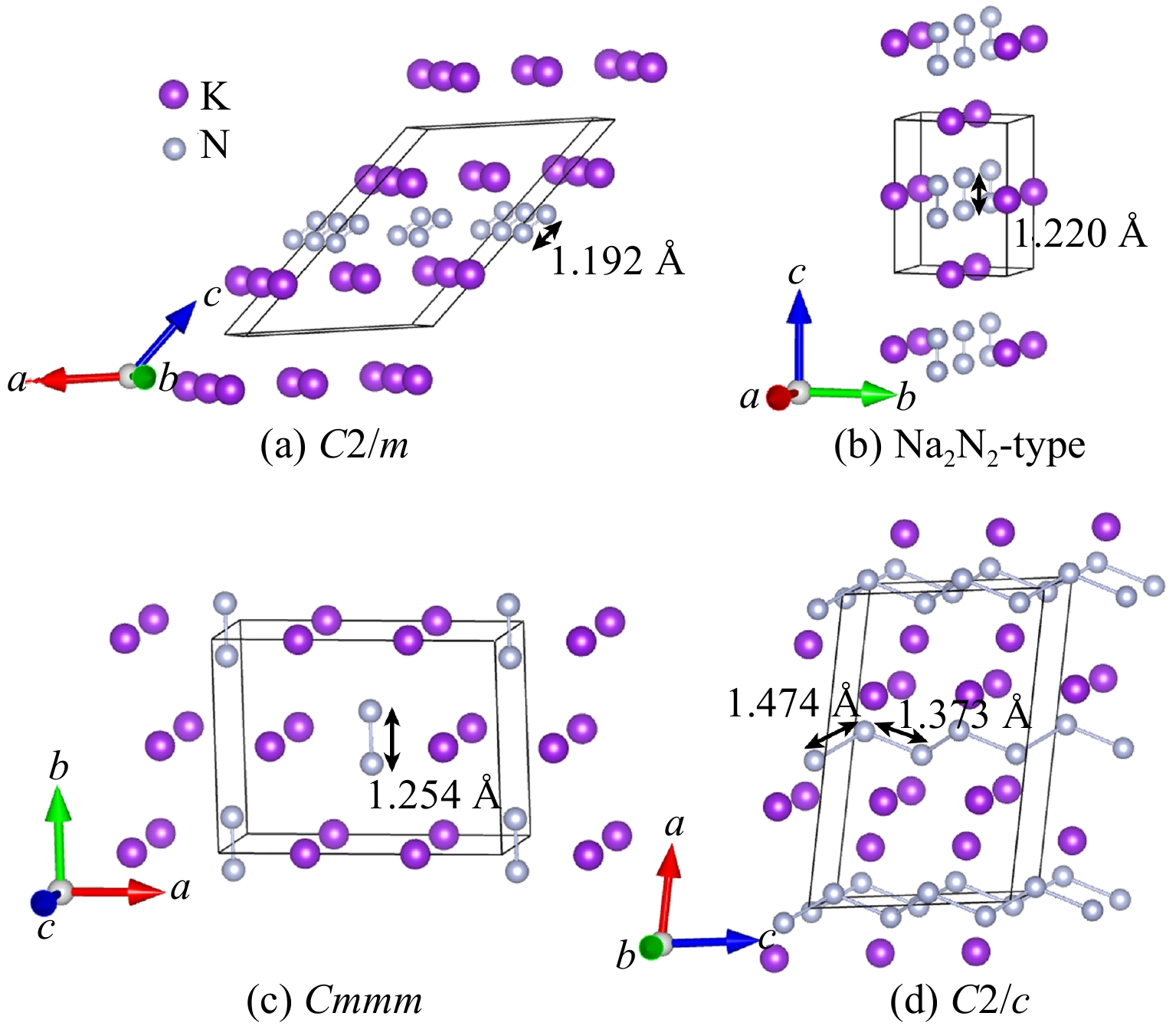
The ground-state structure for K2N2 at ambient pressure in our fully structural search (in Fig. 1(a)) is a monoclinic structure with the space group C2/m, which is different from those reported for Li2N2 and Na2N2[26]. In Fig. 1(a), the N2 quasimolecule dimers in C2/m structure are parallel to each other, and are tilted by a certain angle within a-b plane. The N-N bond length is 1.192 Å, which is a typical value for double bond. Also, the calculated Mayer bond order[43] for the N2 anion in the C2/m is 2.18, being consistent with double bond. Due to the weak interaction between the N2 quasimolecules in the C2/m phase, the application of gigapascal pressure leads to the orientation change of N2 quasimolecules and the structure transformation. Indeed, two stable orthorhombic structures, i.e. Pmmm and Cmmm, for K2N2 were uncovered at 2 and 5 GPa, respectively, as shown in Fig. 1(b) and Fig. 1(c). Remarkably, the unveiled Pmmm phase and the previously reported ground-state structure of Na2N2 are exactly isostructrual (the Pmmm phase denoted as the Na2N2-type phase hereafter), and the Cmmm phase of K2N2 and the high-pressure metastable structures of Na2N2 are isostructrual as well[26]. Furthermore, both Na2N2-type and Cmmm orthorhombic phases have the similar atomic arrangement that the parallel N2 dimers are perpendicular to the K atomic layer. In the Na2N2-type and Cmmm phases, the calculated N-N bond lengths are 1.220 and 1.254 Å, while the characteristics of the N=N double bond are as that of the C2/m structure in the ground-state. As the pressures rises to 150 GPa, a stable monoclinic C2/c structure, containing a folded one-dimensional nitrogen chains (see Fig. 1(d)), is identified for K2N2 for the first time. The lengths of two nonequivalent N-N bond in C2/c structure are 1.474 and 1.373 Å at 135 GPa, indicating genuine N―N single bond, and thus the nature of the structure is similar to that of the high-pressure phase of NaN3[24]. Table 1 lists the structural details of the lattice parameters and the atomic positions of various K2N2 structures at selected pressure points.
Table 1. Optimized structural parameters of the C2/m, Na2N2-type, Cmmm, and C2/c phases of K2N2Phase Pressure/GPa Lattice parameters dN-N/Å Atomic fractional coordinates C2/m 0 a = 7.562 Å, b = 3.912 Å, c = 10.923 Å,
α = γ = 90°, β= 134.955°1.192 K 4i (0.613, 0, 0.260)
N 4i (0.519, 0, 0.456)Na2N2-type 2.5 a = 3.326 Å, b = 4.375 Å, c = 5.694 Å,
α = β = γ = 90°1.220 K1 1e (0, 0.500, 0)
K2 1c (0, 0, 0.500)
N 2t (0.500, 0.500, 0.393)Cmmm 20.0 a = 6.896 Å, b = 5.104 Å, c = 2.855 Å,
α = β = γ = 90°1.254 K 4g (0.694, 0, 0)
N 4j (0, 0.877, 0.500)C2/c 135.0 a = 6.971 Å, b = 4.099 Å, c = 4.510 Å,
α = γ = 90°, β= 81.342°1.474, 1.373 K 8f (0.669, 0.909, 0.198)
N 8f (0.041, 0.887, 0.102)The phonon dispersion curves of the four predicted structures are illustrated in Fig. 2 in order to check their dynamic stabilities. Fig. 2 shows that all the K2N2 phases are dynamically stable as the eigen frequencies of their lattice vibrations are positive for all wavevectors in the Brillouin zone. It should be noted that the predicted C2/m, Na2N2-type, and Cmmm phases exhibit higher eigen frequencies of around 50 THz, which correspond to the vibrations of N2 quasimolecules with stronger N=N double bonds. By contrast, the C2/c phase shows relative lower vibration frequencies of around 30−35 THz, corresponding to the vibration frequencies of weaker N―N single bonds.
Herein, we evaluated the energy involved in the decomposition of the C2/c phase with polymerized N―N single bond chains into metal K and gaseous N2. The energy involved in the C2/c-K2N2 decompostion was determined to be 0.344 kJ/g, which is about three times of that of conventional energetic materials (0.104−0.105 kJ/g)[44], but much smaller than those reported for high-energy density materials (3.61 kJ/g for BeN4, 5.30 kJ/g for GaN5, 4.81 kJ/g for GaN10)[45–46]. This may be due to the low nitrogen content in K2N2, so the nitrogen-rich KNx (x> 1) is expected to be potential HEDM under high pressure.
2.2 Pressure-Induced Structural Phase Transitions of K2N2
To determine the phase transition pressure of K2N2, we plotted in Figs. 3(a) and 3(b) the enthalpy difference of the predicted C2/m, Cmmm, and C2/c structures relative to the Na2N2-type phase in the pressure range of 0−150 GPa. The inset of Fig. 3(a) shows that above 1.7 GPa the Na2N2-type phase becomes energetically preferable with respect to the ground-state C2/m structure and is enthalpically stable up to about 3.6 GPa, above which it transforms into the Cmmm structure. From Fig. 3(b), it can be seen that the Cmmm structure persists up to 122 GPa and then transforms to the C2/c structure according to our structural predictions and enthalpy difference calculations. The wide pressure range of stable Cmmm phase may be attributed to the relative smaller ionic radius and atomic mass of K atom,in contrast with the heavier Rb and Cs atoms. It is thus expected that the chemical precompression exerted by heavier atoms, such as Rb and Cs, in A2N2 (as for Cs2N2[43]) can yield lower pressures for the polymerization of one-dimension N chains. From the calculated pressure dependence of the volume per f. u. in Figs. 3(c) and 3(d), the volume collapses of the first-order transformations C2/m→Na2N2-type, Na2N2-type→Cmmm, and Cmmm→C2/c are 14.4%, 22.5%, and 4.0% respectively. Thus, the Cmmm phase is much stiffer than low-pressure C2/m and Na2N2-type phases under compression. This is consistent with the increasing coordination number of the K atom in the different K2N2 phases under compression in Figs. 3(c) and 3(d). The densification of K2N2 under pressure is realized by increasing the cation coordination number from 5 in ground-state C2/m phase to 8 in Na2N2-type (K2 atoms are coordinated by eight N atoms and K1 atoms are fourfold coordinated by four N atoms) and Cmmm phases, and finally to 10 in C2/c phase.
2.3 Electronic Structures of K2N2 Phases
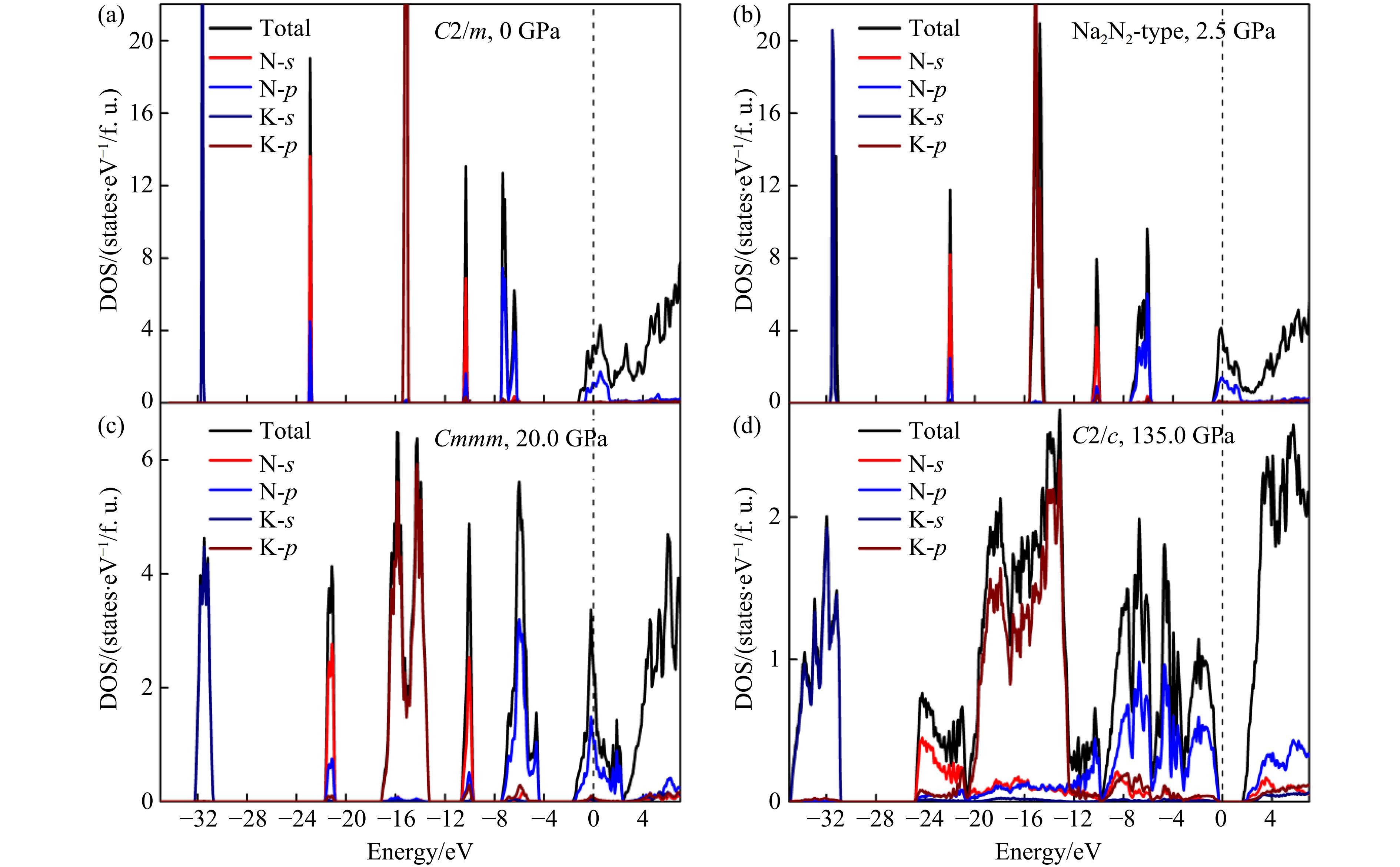
To get deeper insight into the electronic structure evolutions of these K2N2 phases under pressure, we calculated the total density of states (DOS) and the site projected DOS, as shown in Fig. 4, where the Fermi level is the vertical dotted line. In contrast with the semiconducting nature of C2/c phase (Fig. 4(d)), the C2/m, Na2N2-type, and Cmmm phases are all metallic with the N-p electron crossing the Fermi level. Thus, the K2N2 possesses a metal-semiconductor transition, that is similar to that of Li-N system in recent work[28]. From Fig. 4(a)–Fig. 4(c), the C2/m, Na2N2-type, and Cmmm phases exhibit similar projected DOS profiles in the valence band region, the K-s and K-p atomic orbitals are located at the deep energy levels (around −32 and −16 eV, respectively), and the N-s atomic orbitals mainly lie around −22 − −10 eV. Moreover, the interaction between K and N atoms is extremely weak due to the absence of overlapping regions in their atomic projected DOSs. From the inspection of the DOS profiles, a clear broadening of different atomic decomposed states is seen in the high-pressure C2/c phase (Fig. 4(d)), leading to clear hybridizations between K-p and N-p atomic orbitals. In contrast to the first three low-pressure K2N2 phases, this distinction is partially due to the increasing coordination number of K atoms in C2/c phase and to the resulting chemical bonding change that will be illustrated in the following Bader charge transfer analysis.
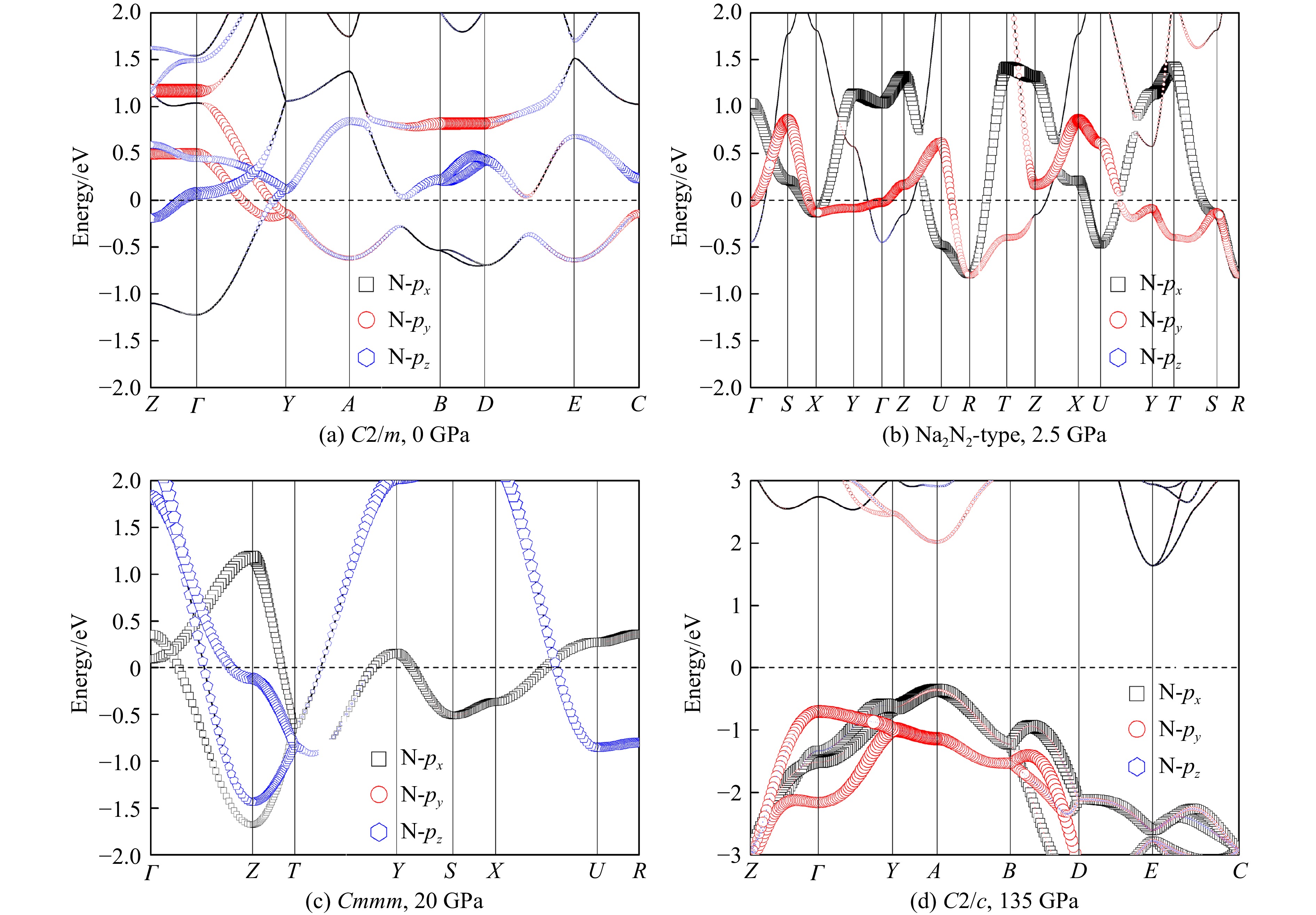
Fig. 5 presents the PBE-resulted energy band structures of the four K2N2 phases, the projected weights of K-s, K-p, and N-s orbital electrons are not taken into account herein because of their exceptionally tiny contributions around the Fermi level in Fig. 4. Compared to the strong metallic nature of the Cmmm phase, which is mainly dominated by N-px/N-pz at the considered energy range of −2 − 2 eV (Fig. 5(c)), the C2/m ( Na2N2-type) phase exhibits a relative weaker metallic behavior that two bands of N-py/N-pz (N-px/N-py) crossing the Fermi level in Fig. 5(a) (Fig. 5(b)). The indirect semiconducting nature of C2/c phase (band gap of 2.0 eV) is demonstrated in Fig. 5(d), with the valence band maximum (VBM) and conduction band minimum (CBM) is located at A and E points, respectively. It is further found that the VBM and CBM of the C2/c phase are principally composed of N-px and N-pz orbitals, respectively.
2.4 Atomic Chemical Bonding of K2N2 Phases
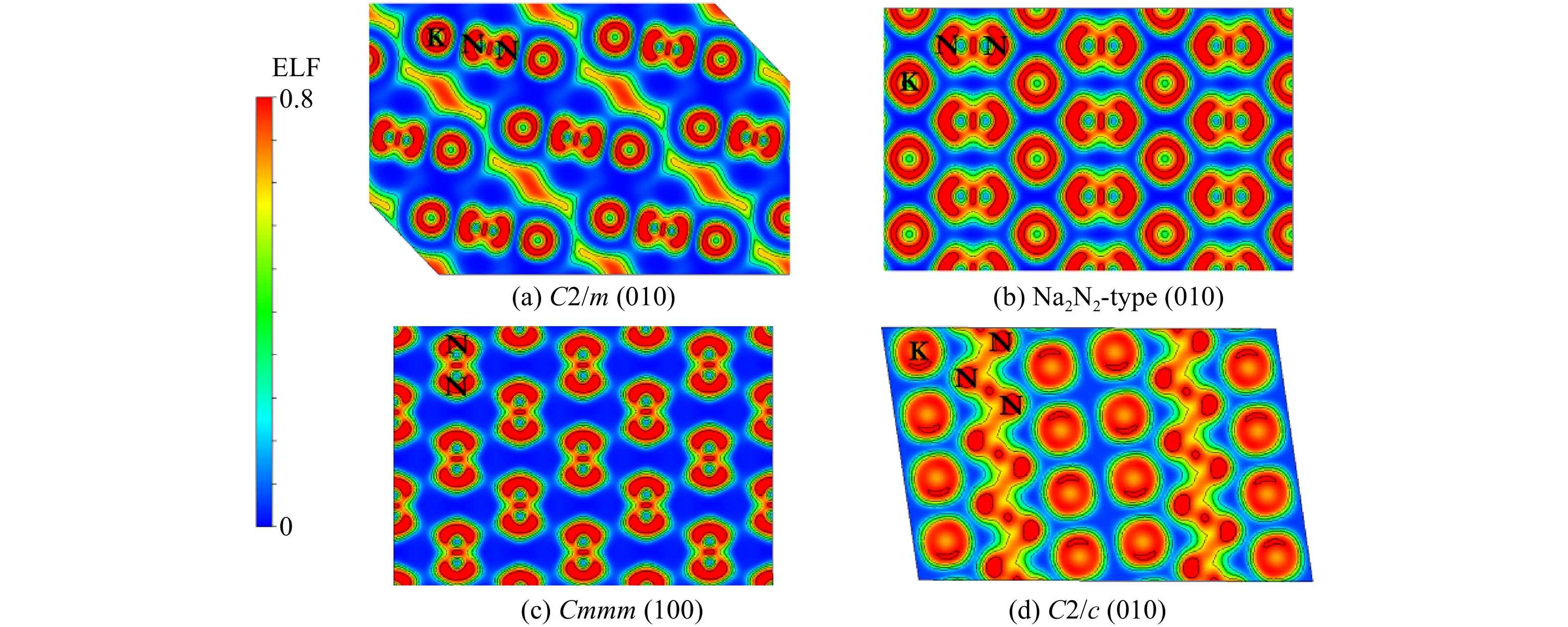
Fig. 6 shows the projected two-dimensional (2D) electron localization functions (ELF) of the four K2N2 phases to further reveal the atomic chemical bonding. The ELF is used to characterize the localized distribution of electrons of different atoms. The ELF values of 1, 0.5, and close to 0 indicate strong electron localization, electron gas, and nonelectron localization, respectively. From Fig. 6(a)–Fig. 6(c), the high-ELF regions are distributed between two N atoms, indicating the strong covalent N-N bonding in the C2/m, Na2N2-type, and Cmmm phases. Lone-pair electrons of K remain from the C2/m to Cmmm phases under pressure, as illustrated by the ELF value of 0 around K atoms, which is in conformity with the projected atomic orbital DOSs described in Fig. 4(a)–Fig. 4(c). For the compressed C2/c phase, some lone-pair electrons of K still remain, yet some of them are transferred to the regions of the K-N bond. Hence, the pressure compels the K-p lone-pair electrons to participate in the chemical bonding with N-p electrons under compression, realizing the high pressure C2/c phase with increased coordination number of K atoms. The electron localization on N-N bond in the C2/c phase (Fig. 6(d)) is weaker than that in the other three low-pressure K2N2 phases, confirming that the N―N single bond of the polymerized one-dimensional nitrogen chains is weaker than the N=N double bond of the N2 quasimolecules.
Finally, the Bader charges in the four phases are listed in Table 2 by using the Bader AIM method. For the ground-state C2/m phase, each N atom accepts 0.491e which corresponds to the electron loss per K atom. When phase transits into the Na2N2-type and Cmmm phases, a relatively stronger ionic bonding feature of K-N bond is disclosed because that the charge transfer is raised to approximately 0.645e − 0.690e as a result of the electron gain of N. Contrarily, it is noted that the atomic charge transfer from the K atom to N atom decrease to 0.623e in the compressed C2/c phase. This variation may be originated from that partial K atoms participate in covalent bonding with the N atom under compression, as indicated by the orbital hybridizations of K-p and N-p in Fig. 4(d). It then can be concluded that the chemical bonding in the C2/c phase containing one-dimensional polymerized nitrogen chains is a complex mixture of covalent and ionic characteristics.
Table 2. Calculated Bader charges of K and N atoms in C2/m, Na2N2-type, Cmmm, and C2/c phasesPhase Pressure/GPa Atom Charge value/e Charge transfer/e C2/m 0 K
N8.509 (×2)
5.491 (×2)+0.491
−0.491Na2N2-type 2.5 K1
K2
N8.407
8.303
5.645 (×2)+0.593
+0.697
−0.645Cmmm 20.0 K
N8.310 (×2)
5.690 (×2)+0.690
−0.690C2/c 135.0 K
N8.377 (×2)
5.623 (×2)+0.623
−0.6233. Conclusions
In summary, we have performed a systematic crystal structure search for alkali metal diazenide K2N2 under pressure using the advanced swarm intelligence structure simulations. The ground-state phase of K2N2 is determined to be a new monoclinic C2/m structure with N2 quasimolecules, and three high-pressure structures of K2N2, including Na2N2-type (1.7−3.6 GPa), Cmmm (3.6−122.0 GPa), and C2/c (above 122.0 GPa), are identified. Remarkably, the pressure-induced polymerization of one-dimensional nitrogen chains in the high-pressure C2/c semiconducting phase is firstly reported, attributed to the participation of K-p lone-pair electrons in the chemical bonding under pressure. The occurrences of the high-pressure phases of Na2N2-type, Cmmm, and C2/c follow the increased coordination numbers (from 5 to 10) of K atoms with the incresed pressures, accompanied by significant N-N bonding modification from N=N dimers to polymerized N―N single bond one-dimensional chains. Furthermore, a metal-semiconductor transition of K2N2 under pressure is demonstrated by the electronic structures calculations. The present findings provide fundamental insights into the high-pressure structures and the polymerization of N atoms of alkali metal diazenides, supplying useful guidance for the syntheses of K2N2 in further experimental efforts.
-
表 1 计算材料参数
Table 1. Material parameters for calculation
Density/
(kg·m−3)Elastic modulus/GPa Shear modulus/GPa P wave velocity/(m·s–1) S wave velocity/(m·s–1) Friction coefficient Internal friction angle/(°) 2300 62.8 24.1 5225 3237 0.1 44 Expansion angle/(°) Hardening coefficient Fracture strain Tensile
strength/MPaCohesion strength/MPa Shear stress ratio Absolute plastic strain 0 6.98 0.0075 3.5 8 0.33 0 -
[1] 许金泉. 界面力学 [M]. 北京: 科学出版社, 2006.XU J Q. The mechanics of interface [M]. Beijing: Science Press, 2006. [2] RUBINSTEIN S M, COHEN G, FINEBERG J. Detachment fronts and the onset of dynamic friction [J]. Nature, 2004, 430(7003): 1005–1009. doi: 10.1038/nature02830 [3] RUBINSTEIN S M, COHEN G, FINEBERG J. Dynamics of precursors to frictional sliding [J]. Physical Review Letters, 2007, 98(22): 226103. doi: 10.1103/PhysRevLett.98.226103 [4] BEN-DAVID O, COHEN G, FINEBERG J. The dynamics of the onset of frictional slip [J]. Science, 2010, 330(6001): 211–214. doi: 10.1126/science.1194777 [5] ZHU Y D, ZHENG Z J, ZHANG Y L, et al. Adhesion of elastic wavy surfaces: interface strengthening/weakening and mode transition mechanisms [J]. Journal of the Mechanics and Physics of Solids, 2021, 151: 104402. doi: 10.1016/j.jmps.2021.104402 [6] PERSSON B N J. Sliding friction: physical principles and applications [M]. 2nd ed. Berlin: Springer, 2000. [7] TA W R, QIU S M, WANG Y L, et al. Volumetric contact theory to electrical contact between random rough surfaces [J]. Tribology International, 2021, 160: 107007. doi: 10.1016/j.triboint.2021.107007 [8] GERDE E, MARDER M. Friction and fracture [J]. Nature, 2001, 413(6853): 285–288. doi: 10.1038/35095018 [9] BAUMBERGER T, BERTHOUD P, CAROLI C. Physical analysis of the state- and rate-dependent friction law. Ⅱ. dynamic friction [J]. Physical Review B, 1999, 60(6): 3928–3939. doi: 10.1103/PhysRevB.60.3928 [10] BRAUN O M, MANINI N, TOSATTI E. Size scaling of static friction [J]. Physical Review Letters, 2013, 110(8): 085503. doi: 10.1103/PhysRevLett.110.085503 [11] BARRAS F, AGHABABAEI R, MOLINARI J F. Onset of sliding across scales: how the contact topography impacts frictional strength [J]. Physical Review Materials, 2021, 5(2): 023605. doi: 10.1103/PHYSREVMATERIALS.5.023605 [12] SHAO R L, WAHLE M, ZIMMERMANN M. A model for the dynamic friction behaviour of rubber-like materials [J]. Tribology International, 2021, 164: 107220. doi: 10.1016/j.triboint.2021.107220 [13] 张磊, 王文帅, 苗春贺, 等. 花岗岩粗糙表面动摩擦形态演化 [J]. 高压物理学报, 2021, 35(3): 031201. doi: 10.11858/gywlxb.20200640ZHANG L, WANG W S, MIAO C H, et al. Rough surface morphology of granite subjected to dynamic friction [J]. Chinese Journal of High Pressure Physics, 2021, 35(3): 031201. doi: 10.11858/gywlxb.20200640 [14] BERMAN N, COHEN G, FINEBERG J. Dynamics and properties of the cohesive zone in rapid fracture and friction [J]. Physical Review Letters, 2020, 125(12): 125503. doi: 10.1103/PhysRevLett.125.125503 [15] WANG P F, JIANG H B, XU S L, et al. Dynamic plastic instability of ring-shaped aluminum alloy with different interface behaviors [J]. International Journal of Impact Engineering, 2021, 155: 103898. doi: 10.1016/j.ijimpeng.2021.103898 [16] 赵剑衡, 孙承纬, 段祝平, 等. 玻璃样品表面对失效波萌生的影响 [J]. 力学学报, 2001, 33(6): 834–838. doi: 10.3321/j.issn:0459-1879.2001.06.014ZHAO J H, SUN C W, DUAN Z P, et al. Effect of impacted surface of K9 glass sample on formation of failure wave [J]. Acta Mechanica Sinica, 2001, 33(6): 834–838. doi: 10.3321/j.issn:0459-1879.2001.06.014 [17] 刘均伟, 张先锋, 刘闯, 等. 考虑摩擦因数变化的弹体高速侵彻混凝土质量侵蚀模型研究 [J]. 爆炸与冲击, 2021, 41(8): 083301. doi: 10.11883/bzycj-2020-0250LIU J W, ZHANG X F, LIU C, et al. Study on mass erosion model of projectile penetrating concrete at high speed considering variation of friction coefficient [J]. Explosion and Shock Waves, 2021, 41(8): 083301. doi: 10.11883/bzycj-2020-0250 [18] POCHIRAJU K V, TANDON G P, PAGANO N J. Analyses of single fiber pushout considering interfacial friction and adhesion [J]. Journal of the Mechanics and Physics of Solids, 2001, 49(10): 2307–2338. doi: 10.1016/S0022-5096(01)00045-X [19] 王蕉, 楚锡华. 冲击载荷下颗粒材料临边界区域的波动行为及变形特征分析 [J]. 力学学报, 2021, 53(9): 2395–2403. doi: 10.6052/0459-1879-21-242WANG J, CHU X H. Analysis of wave behavior and deformation characteristics of granular materials in pro-border zone under impact load [J]. Chinese Journal of Theoretical and Applied Mechanics, 2021, 53(9): 2395–2403. doi: 10.6052/0459-1879-21-242 [20] ZHENG W, ZHANG S Y, XU N. Jamming of packings of frictionless particles with and without shear [J]. Chinese Physics B, 2018, 27(6): 066102. doi: 10.1088/1674-1056/27/6/066102 [21] SCHOLZ C H. Earthquakes and friction laws [J]. Nature, 1998, 391(6662): 37–42. doi: 10.1038/34097 [22] KANAMORI H, ANDERSON D L, HEATON T H. Frictional melting during the rupture of the 1994 Bolivian earthquake [J]. Science, 1998, 279(5352): 839–842. doi: 10.1126/science.279.5352.839 [23] RUBINO V, ROSAKIS A J, LAPUSTA N. Understanding dynamic friction through spontaneously evolving laboratory earthquakes [J]. Nature Communications, 2017, 8: 15991. doi: 10.1038/ncomms15991 [24] PYRAK-NOLTE L J, XU J P, HALEY G M. Elastic interface waves propagating in a fracture [J]. Physical Review Letters, 1992, 68(24): 3650–3653. doi: 10.1103/PhysRevLett.68.3650 [25] XIA K W, ROSAKIS A J, KANAMORI H. Laboratory earthquakes: the sub-Rayleigh-to-supershear rupture transition [J]. Science, 2004, 303(5665): 1859–1861. doi: 10.1126/science.1094022 [26] FERRER C, SALAS F, PASCUAL M, et al. Discrete acoustic emission waves during stick-slip friction between steel samples [J]. Tribology International, 2010, 43(1/2): 1–6. doi: 10.1016/j.triboint.2009.02.009 [27] BRAUN O M, BAREL I, URBAKH M. Dynamics of transition from static to kinetic friction [J]. Physical Review Letters, 2009, 103(19): 194301. doi: 10.1103/PhysRevLett.103.194301 [28] SVETLIZKY I, FINEBERG J. Classical shear cracks drive the onset of dry frictional motion [J]. Nature, 2014, 509(7499): 205–208. doi: 10.1038/nature13202 [29] DI BARTOLOMEO M, MASSI F, BAILLET L, et al. Wave and rupture propagation at frictional bimaterial sliding interfaces: from local to global dynamics, from stick-slip to continuous sliding [J]. Tribology International, 2012, 52: 117–131. doi: 10.1016/j.triboint.2012.03.008 [30] KAMMER D S, MUÑOZ D P, MOLINARI J F. Length scale of interface heterogeneities selects propagation mechanism of frictional slip fronts [J]. Journal of the Mechanics and Physics of Solids, 2016, 88: 23–34. doi: 10.1016/j.jmps.2015.12.014 [31] 李永池. 波动力学 [M]. 合肥: 中国科学技术大学出版社, 2015.LI Y C. Wave mechanics [M]. Hefei: University of Science and Technology of China Press, 2015. -







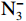
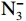
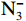

 DownLoad:
DownLoad:
 DownLoad:
DownLoad:






 下载:
下载: