Reliability Optimization Design of Anti-Penetration Perforated Armor
-
摘要: 孔结构装甲在满足抗侵彻性能的同时需实现减重,因而轻量化设计具有工程实际意义。以孔结构装甲的轻量化为设计目标,抗侵彻性能为约束条件,考虑不确定性因素的影响,开展孔结构装甲的可靠性优化设计。样本点采用最优拉丁超立方法设计生成,孔结构装甲抗侵彻仿真的参数化建模及响应计算通过商业软件ANSYS的二次开发实现,引入Kriging代理模型和期望改变量(expected improvement, EI)加点法构建性能函数,最后采用序列优化与可靠性评估方法(sequential optimization and reliabilityassessment, SORA)进行可靠性优化设计。结果表明,可靠性优化后,孔结构装甲在满足抗侵彻性能和相关可靠度指标的前提下,可有效地实现减重11.5%。研究结果可为其他抗侵彻防护结构的可靠性优化设计提供参考。Abstract: Perforated armor can effectively reduce weight while meeting anti-penetration performance. Structural lightweight design of perforated armor has practical engineering significance. Considering the influence of uncertain factors, a reliability optimization design of a perforated armor was realized in this research. In the process of the reliability optimization design, the light weight of the perforated armor was taken as the goal of the design, and the anti-penetration performance was taken as the constraint condition. The optimal Latin hypercube design method was used to generate sample points. Based on a development of the commercial software ANSYS, the parametric modeling and the response calculation of the anti-penetration simulation of the perforated armor were realized. The Kriging surrogate model and the expected improvement maximization method were introduced to construct the performance functions. The sequential optimization and the reliability assessment method were applied in the reliability optimization design of the perforated armor. Under the premise of meeting the anti-penetration performance requirements and having a related reliability indicator of 0.9, the weight of the perforated armor after the reliability optimization can be effectively reduced by 11.5%. The research can provide references for the reliability optimization design of other anti-penetration structures.
-
Key words:
- perforated armor /
- lightweight design /
- Kriging model /
- reliability optimization
-
多孔材料和粉末材料的冲击压缩一直是重点研究方向。早期研究主要通过多孔或粉末材料的冲击压缩,获得在固体材料中无法获得的热力学状态,以研究完全物态方程,此类研究关注高压区域,忽略粉末材料的自身强度[1-2]。粉末的冲击压实利用冲击波作用于非晶或纳米晶粉末,在瞬态高温高压下得到近乎致密的块体,且仍保持原始晶粒尺寸,是制备亚稳态材料的一种非常有潜力和前景的方法。为了避免高压导致的高温和裂纹问题,往往在1~10 GPa的低压范围内实现压实,因此,粉末在低压区的致密化行为对于粉末冲击压实研究非常重要。
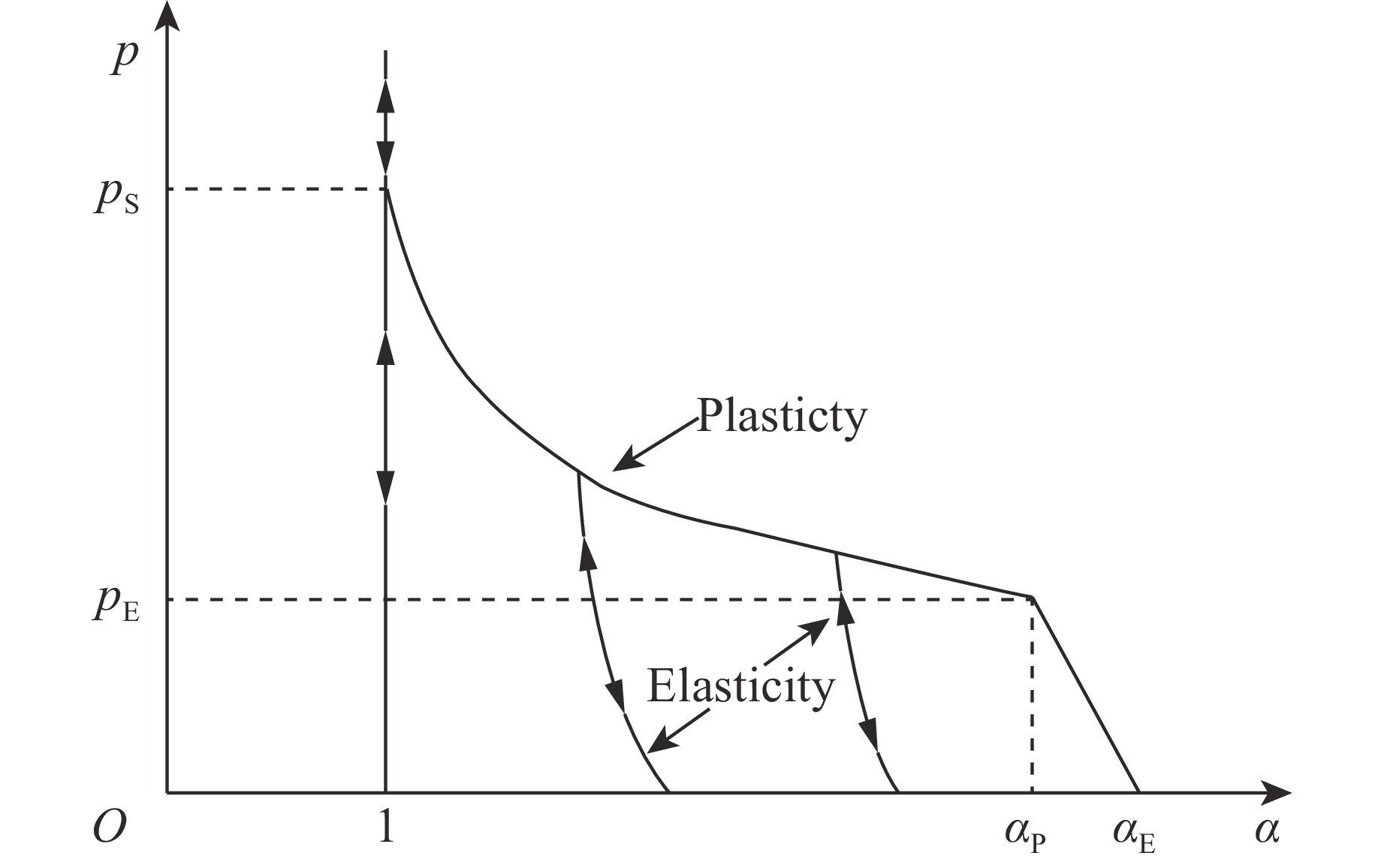
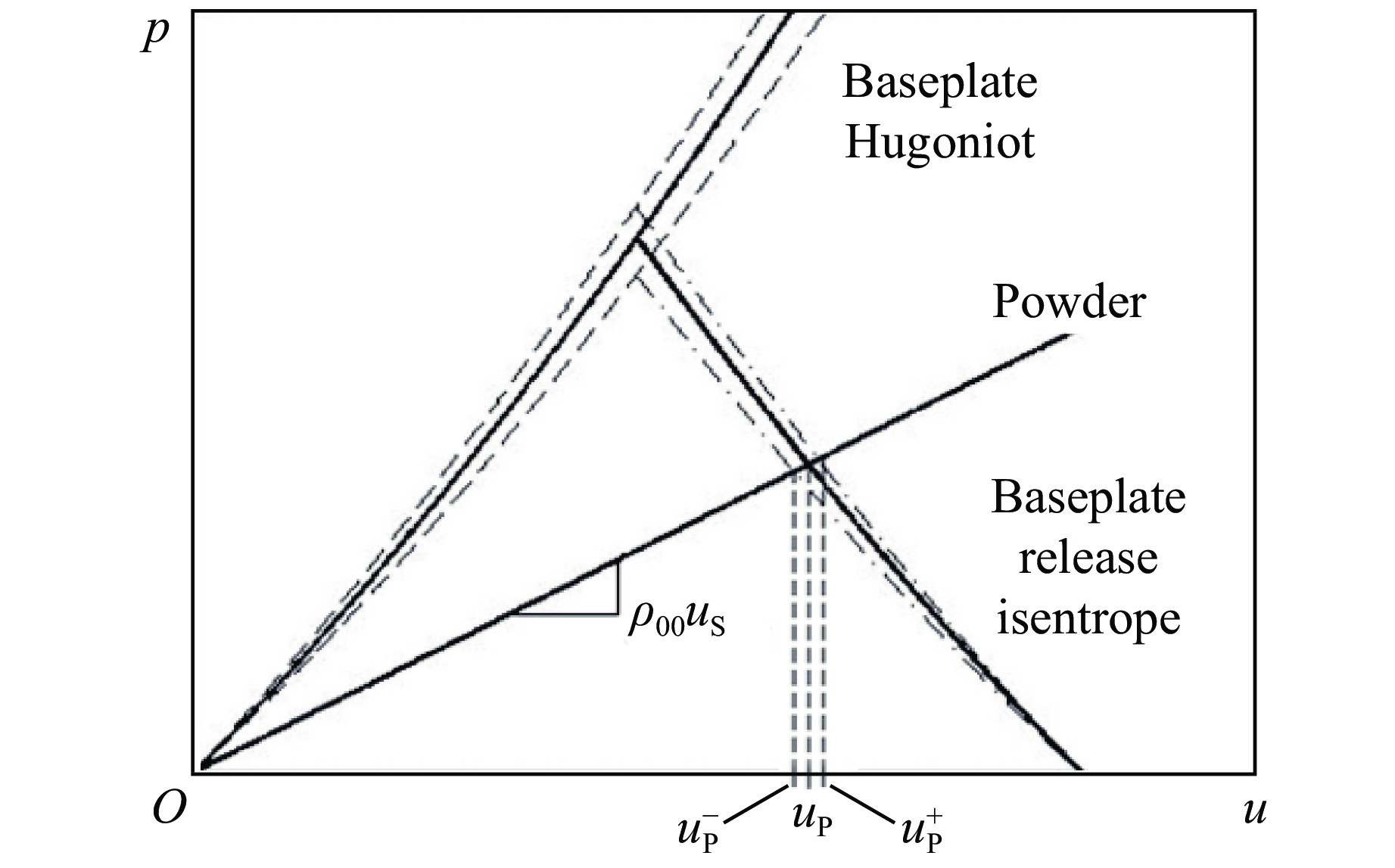
粉末在低压区的冲击压实是一个复杂的过程,受到多种因素的影响,包括粉末材料的弹塑性、初始颗粒的大小和形状等[3]。为了在较宽的压力范围内更好地描述粉末的压实过程,一般将这一过程分解为弹性段和塑性段,如图1所示,其中:
αE 、pE为弹性段结束时的孔隙度和压力,由于在整个压缩过程中,弹性段仅占很小部分,一般都忽略不计,多数模型取αE=α0 ;当压力超过pE后,进入塑性段,随着颗粒形变和孔隙坍缩,孔隙率逐渐降低,压力达到pS时,粉末完全压实,pS也被称为压溃强度。目前已经有较多模型描述粉末压实响应,但存在两个假设前提:(1)忽略了剪切强度;(2)在同等压力、温度条件下,粉末和固体材料的比内能是相等的。假设(1)使得模型只能描述由于孔隙闭合导致的不可逆压实;假设(2)忽略了孔隙和颗粒的表面能,更适合描述颗粒较大的粉末体系。在众多描述颗粒或粉末材料动态压实行为的模型中,以p-
α 模型[4-7]和p-λ 模型[8]应用最为广泛,是典型的描述型模型,这类模型结合了粉末材料本征特性,通过实验数据拟合获得模型的关键参数。尽管模型的具体形式不同,但都包含一个或者多个经验拟合参数。通常情况下这些参数与可测量的物理参数没有直接关系,只是为了使模型更好地与实验数据匹配,这种灵活性使模型可以很好地描述某种特定粉末系统的冲击压实过程。对于由不同性质粉末组成的混合物,粉末冲击压实过程变得更加复杂。目前并没有有效的方法建立混合粉末冲击压缩方程,一般可通过0 K混合法则[9-10]或质量分数加权平均法则[11-12]先得到混合固体Hugoniot线,再经等容Mie-Grüneisen方法计算得到粉体的冲击压缩方程,但这种方法的计算过程繁琐,需要专门程序计算,而且其有效性缺乏实验验证。
本研究测量了W-Cu混合粉末的Hugoniot数据,系统分析了测量误差;然后分别应用p-
α 和p-λ 等模型对测量数据进行拟合;利用Mie-Grüneisen方法[13]得到同孔隙度的W、Cu单质粉末的Hugoniot线,按照Barry等压混合法则[14]预测同孔隙度的W-Cu混合粉末的Hugoniot线,并与测量得到的数据进行对比,探讨p-α 和p-λ 等模型在描述混合粉末冲击压缩行为时的适用性和经单质粉末Hugoniot线预测混合粉末Hugoniot线的可行性。1. 实验测试
1.1 W-Cu混合粉末Hugoniot测量实验装置
实验使用的W和Cu单质粉末均为市购,纯度均为99.9%,粉末粒度均为
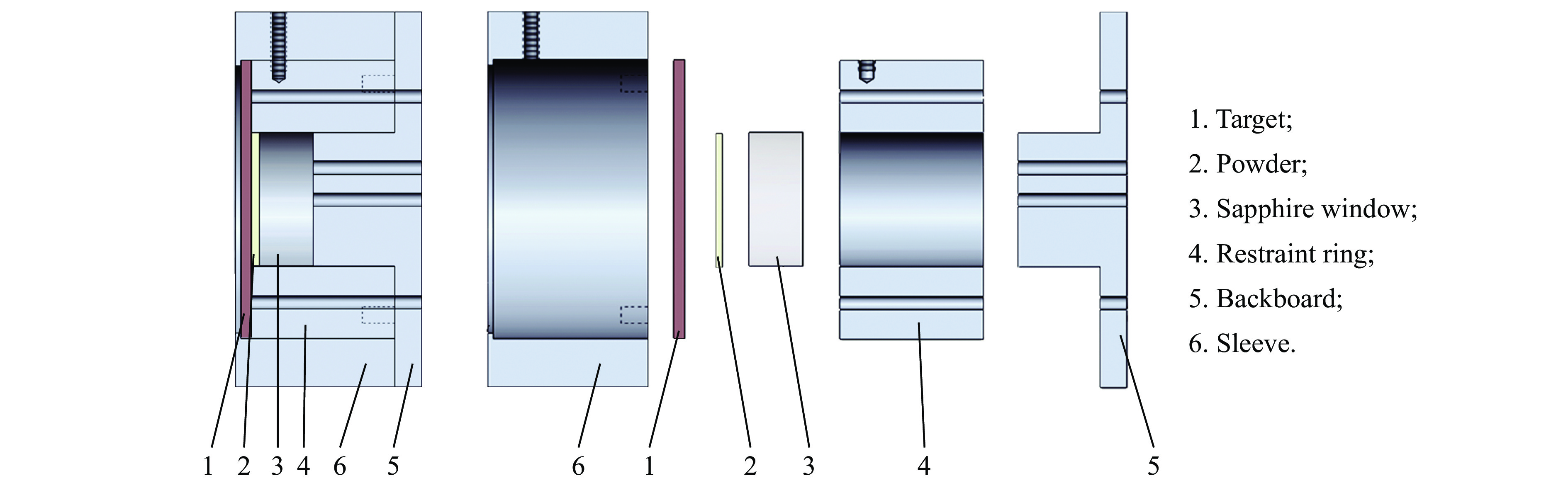
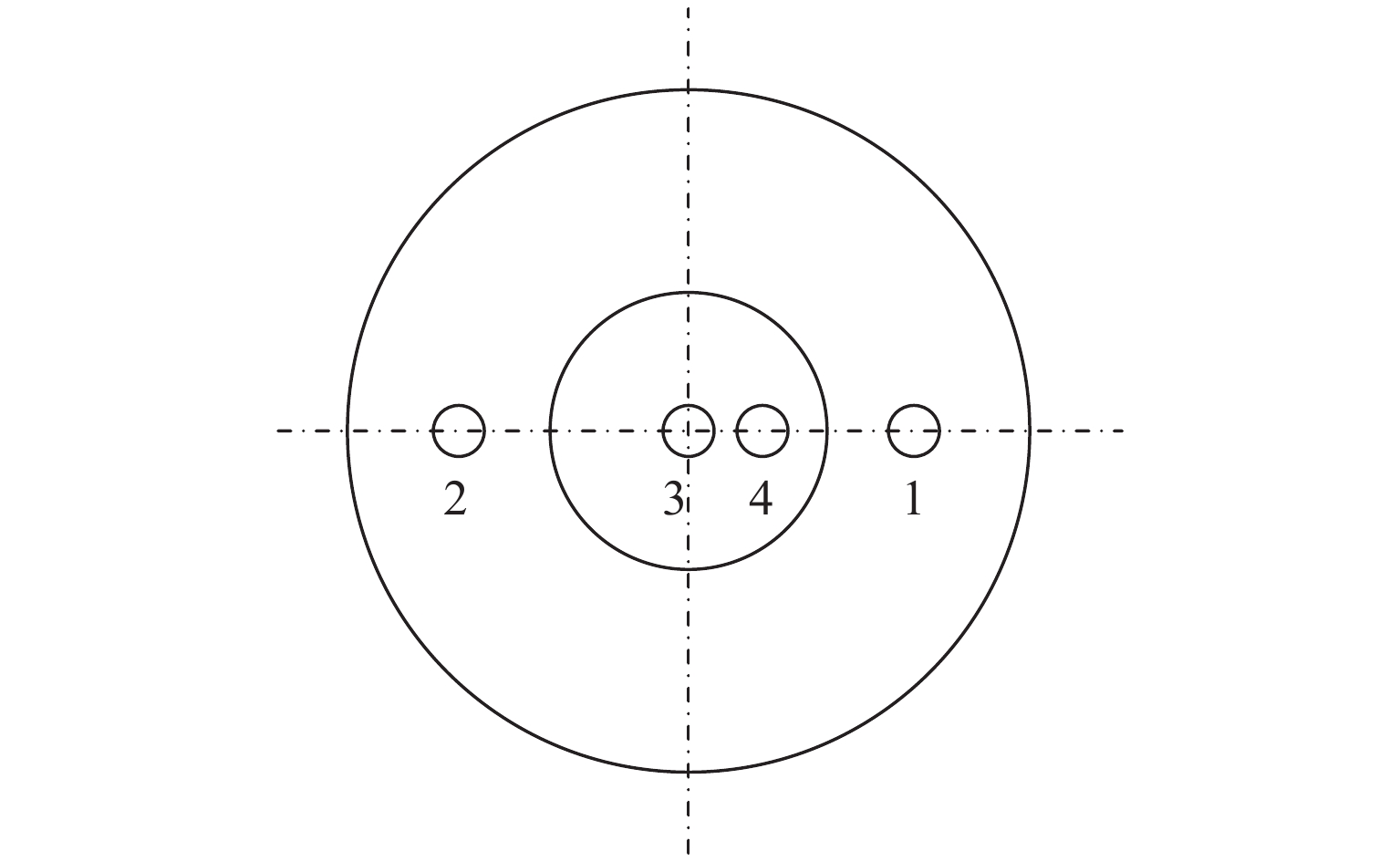
1μm 。W、Cu粉按照质量比76∶24混合,经V型混料机混合均匀得到W-Cu混合粉末。图2为实验测量所用装置的结构示意图,将无氧铜基板和约束环依次安装于中空的304不锈钢套筒后,形成一个容器,再依次装入W-Cu混合粉末和蓝宝石窗口,并通过背板确保粉末后表面与窗口材料紧密接触。实验使用二级轻气炮进行加载,飞片使用与基板同材质的无氧铜,应用光纤位移干涉仪(Displacement interferometer system for any reflector,DISAR)[15],通过基板背面和窗口背面设置的4个光纤探头,分别记录冲击波抵达粉末样品上下表面的时间,探头布置的空间位置如图3所示。2. 参数测量及误差分析
2.1 粉末初始密度
粉末初始密度
ρ00 主要通过调整粉体厚度来控制,粉末厚度由组装件整体厚度减去基板中心、蓝宝石窗口及背板厚度计算得到。厚度测量精度为10–4 mm,粉末质量称量精度为10–3 g。每组取多次测量的平均值,并在初始密度的不确定度分析中加入了每个位置测量的方差。粉末中存在局部的不均匀性,但无法确定不均匀部位的位置和范围,因此初始孔隙密度ρ00 的测量误差取最大值,即直接取粉体厚度测量的最大值和最小值来确定。初始密度的测量误差会在阻抗匹配计算中传递,对Hugoniot参数(uP, p和V)的测量误差影响较大。2.2 飞片速度
飞片速度vI由炮口的电磁线圈测量得到,该系统的测量误差为0.5%。由于飞片在炮膛中会发生偏转,形成的倾角可由图3中1、2号探针确定后扣除。
2.3 冲击波速度
冲击波速度uS根据冲击波分别到达图3中1、2号探针和3、4号探针记录的时间和粉末厚度计算得出,即
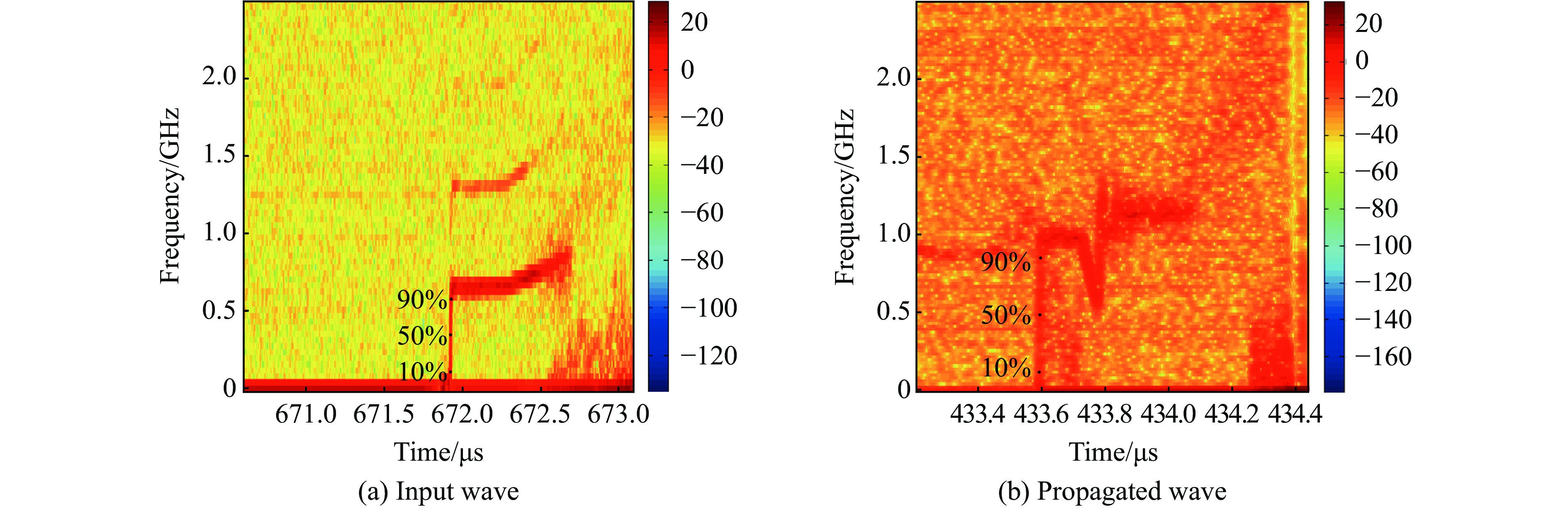
uS=∆xi/∆ti ,其中∆xi 和∆ti 分别是粉末厚度和冲击波传播时间。粉末厚度的标准差为δ(∆xi)=(δx2B+δx2W)1/2 ,其中xB表示基板位移,xW表示粉末厚度[16]。∆ti 即冲击波分别抵达1、2号探针和3、4号探针的时间差,其标准差为δ(∆ti) 。图4所示为粉体上下表面处探针的DISAR信号。由于飞片飞行过程中会发生偏转,1、2号探针信号存在时间差,也会导致冲击波速度测量误差,这一误差也体现在δ(∆ti) 中。在两个标准差范围内一个实验的平均冲击速度总误差为 图 4 典型的入射波(a)和传播波(b)的DISAR轮廓曲线(显示了结构化波形,以及50%的均衡到达时间和10%、90%的不确定到达时间,用于计算冲击波速度)Figure 4. Typical extracted profile for input (a) and propagated waves (b) that illustrating structured waveforms (Locations of 50% equilibrium time of arrival and 10% and 90% uncertainty arrival times used for calculation of shock velocity are also marked.)
图 4 典型的入射波(a)和传播波(b)的DISAR轮廓曲线(显示了结构化波形,以及50%的均衡到达时间和10%、90%的不确定到达时间,用于计算冲击波速度)Figure 4. Typical extracted profile for input (a) and propagated waves (b) that illustrating structured waveforms (Locations of 50% equilibrium time of arrival and 10% and 90% uncertainty arrival times used for calculation of shock velocity are also marked.)δuSuS=[∑i2[δ(∆xi)∆xi]2+∑i2(δ(∆ti)∆ti)2]12 (1) 2.4 粒子速度
粉末的粒子速度uP使用阻抗匹配法计算,计算原理和误差分布如图5所示。根据文献[17],粒子速度的总误差是系统误差和实验误差之和,即
δuP=δuEP+δuSP 。粉末粒子速度的系统误差是δuP=[(δuSP,I−B)2+(δuSP)2]1/2 (2) 式中:
uSP,I−B 表示靶板Hugoniot误差,uSP 表示靶板等熵线上的误差。实验误差为δuEP={[δuP(uS)]2+[δuP(vI)]2+[δuP(ρ00)]2+[δuP(ρ0I)]2[δuP(ρ0B)]2}1/2 (3) 式中:
ρ0I 和ρ0B 分别是飞片和基板的初始密度。2.5 压 力
压力的初始值p0和粉末初始速度u0等于零,则粉末中的压力p可直接由下式计算
p=ρ0(uS−u0)uP (4) 压力的误差
δp/p 也包括系统误差和实验误差。由于p是ρ00 、uS、uP的函数,因此,压力p的系统误差为δσσ=δρ00ρ00+δuSuS+δuPuP (5) 实验误差为[17]
(δσσ)E={(1+uSuP∂uP∂uS)2(∂uSuS)2+(1+ρ00uP∂uP∂ρ00)2(∂ρ00ρ00)2+[∂uP(v1)uP]2+(∂uP(ρ0I)uP)[∂uP(ρ0I)uP]2+(∂uP(ρ0B)uP)[∂uP(ρ0B)uP]2}12 (6) 2.6 冲击压缩比容
粉末中的冲击压缩比容V由
V=V0[1−(uP−u0)/(uS−u0)] 计算得到,其中V=1/ρ 。V的系统误差为(δV/V)S=(η−1)|δuSP|/uP ,其中η 是粉末压缩度,η=ρ/ρ00 。实验误差是(δVV)E={(η−1)2(1−uSuP∂uP∂uS)2(∂uSuS)2+[1+(η−1)ρ00uP∂uP∂ρ00]2(∂ρ00ρ00)2+(η−1)2F2} (7) 式中:
F2=[∂uP(V1)uP]2+[∂uP(ρ0I)uP]2+[∂uP(ρ0B)uP]2。 2.7 测量结果分析
实验测量的所有Hugoniot参数及其误差范围如表1所示。可以看到uS的误差较大,主要是粉体厚度测量误差较大所致,也导致粉末初始密度和压缩密度误差均接近10%。压力超过4 GPa后,粉体已接近致密。
表 1 实验相关参数计算结果及误差范围Table 1. Calculated results and errors of the related experimental parametersNo. ρ00/(g·cm–3) vI/ (km·s–1) uS/(km·s–1) uP/(km·s–1) p/GPa ρ/(g·cm–3) 1 10.696±0.910 0.622±0.003 1.254±0.041 0.457±0.019 6.126±0.434 16.826±1.122 2 10.484±0.731 0.270±0.001 0.913±0.055 0.213±0.013 2.038±0.223 13.668±0.536 3 10.707±1.229 0.784±0.004 1.549±0.089 0.546±0.028 9.056±0.913 16.639±1.324 4 10.237±1.370 0.508±0.003 1.107±0.030 0.388±0.016 4.392±0.211 15.752±1.370 3. 模型拟合
3.1 p-
α 模型p-
α 模型被广泛用于描述均质和非均质粉末的弹塑性压实响应[18]。其中α=V/VS 是一个描述粉末膨胀程度的参数,V是粉末的比容,VS是相应固体材料在相同温度T和压力p下的比容。p-α 模型具有不同的表达形式,本研究仅关注其中最常见的3种。3.1.1 二次改进模型(Modified quadratic,MQ)
p-
α 模型常用于描述动态冲击加载下塑性多孔材料的冲击特性。当应力范围较大时,要分解为弹性和塑性两部分。此模型中忽略了剪切强度的存在,并且假设在等温等压下,多孔材料和固体材料的内能相等。在低压力段,弹性压实响应决定压实行为和从部分压实状态下的释放过程。通常初始的弹性状态αE 很小,在计算中经常会忽略,或设为αE=α0 直到达到阈值压力。塑性变形中的
α 是对Butcher和Karnes方法[6]的二次改进。一般公式如下α=1+(αE−1)(pS−ppS−pE)N (8) 式中:N是经验拟合参数,可以视实验具体情况改变。
3.1.2 静态球面公式(Static spherical,SS)
Carroll和Holt[7]通过将多孔材料中孔洞的坍塌用一个由相同密度的空心球的压实相平衡来补充p-
α 模型。假设应力张量为球形,只考虑压力p。静态球形公式可对压实响应进行预测,因此压实响应由材料性质和孔洞的几何形状决定。假设固体材料不可压缩,将孔洞坍塌分为3个区域。在最初的弹塑性区,观察到在压力达到临界值前孔隙度基本没有发生变化。这使压实过程可以得到简化:在压实过程的前两个区域,压力小于pE时孔隙率的变化可以忽略不计。pE的大小使用下式计算pE=23Ylnα0α0−1 (9) 式中:Y是固体材料的屈服强度,
α0 是初始孔隙度。压力大于pE时为第3个阶段,材料塑性变形,压实由孔洞坍塌造成,此时的孔洞坍塌关系可以用下式简化α=(1−e−3p/2Y)−1 (10) 静态球面的p-
α 模型只考虑了与速率无关的屈服特性,因此可能不适用于高速率敏感粉体材料的压实响应,而且此模型忽略了颗粒形态可能造成的影响。3.1.3 幂律公式(Power law,PL)
Brown等[19]的p-
α 模型与前两种不同,他们用同一种机制描述弹塑性压实,直接描述了脆性微粒系统的动态压实,并没有将弹塑性机制分开研究。幂律公式的表达式如下α=(PS/p)1n (11) 式中:PS是压碎强度,n是经验参数。
3.2 p-
λ 模型p-
λ 模型明确地将单个成分的物质属性包含在一个非均匀混合物中[20]。与p-α 模型类似,该模型使用单内部状态变量λ描述压实过程并忽略粉末混合物的剪切强度,只考察了一般的p-V响应。该模型分为3个不同的区域:一端为不平衡的弹性响应,另一端为单个分量的压力平衡状态,两者之间为过渡区。假设压力较小时变形是弹性的。使用两种不同的附加模型定义混合物的体积弹性模量K和弹性响应:等压和等应变。等压响应是混合模量的下限,等应变响应是上限。在选择合适的混合模量后,通过应变的定义确定了非平衡弹性区混合物的p-V响应;在原方程的基础上,提出了一个简单的质量分数加性方程。在过渡区,假定材料以非平衡弹性状态和压力平衡状态的某种组合形式存在。材料在给定压力值下的相对量通过变量k来分配,k定义为完全压实状态下的物质质量分数。λ取值0~1。当材料完全处于不平衡的弹性状态下λ = 0;当材料处在压力平衡状态时,λ = 1。λ的具体函数关系为λ=1−e−(p/Y)n (12) 式中:p是组件的局部压力差异;Y是固体的屈服强度,当混合物屈服时,会同时在混合物中引发屈服和压实;n无法从单个组件属性中进行定义。
3.3 拟合结果
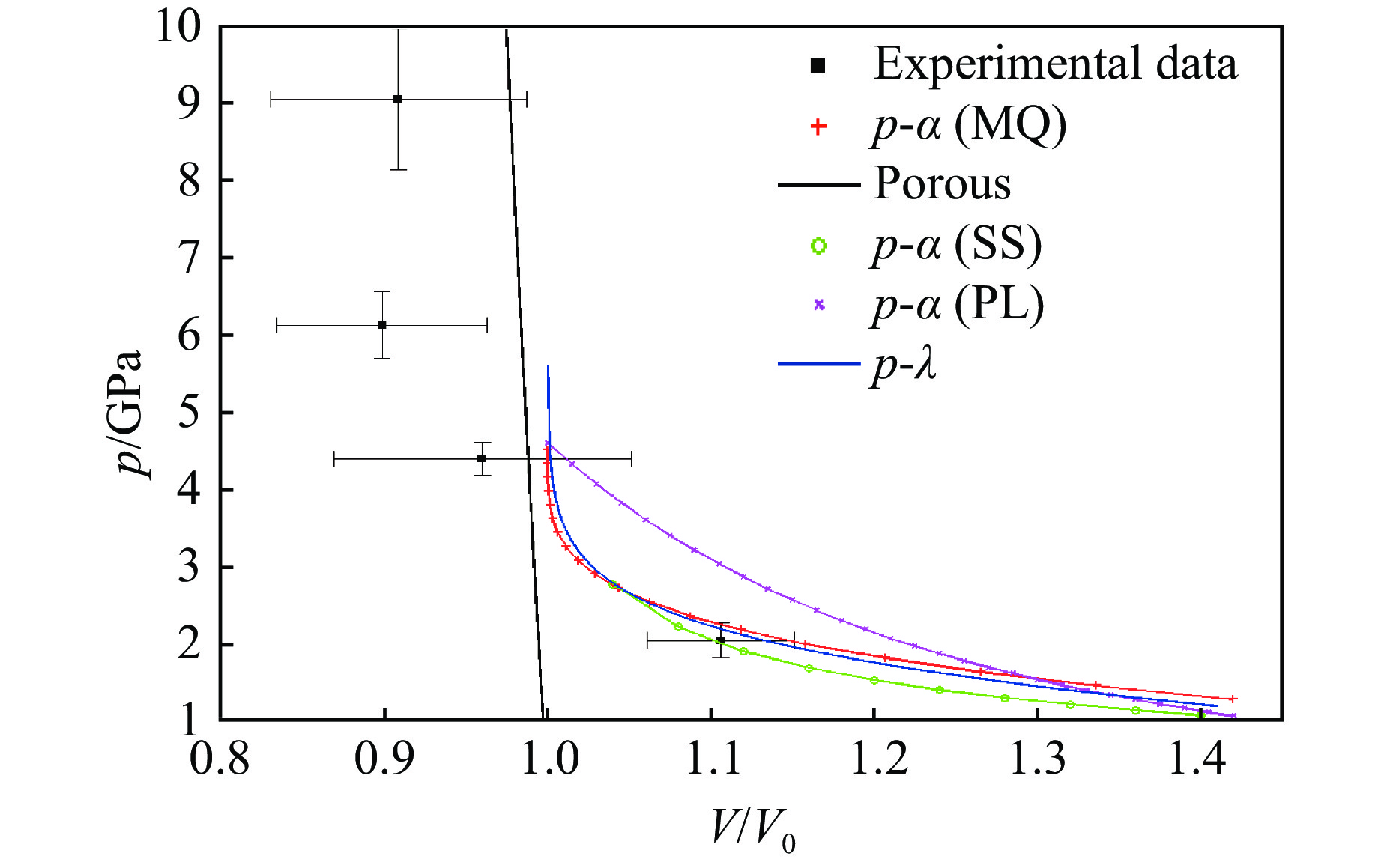
为了检验测量数据与模型拟合的精度,必须给出混合粉末的Hugoniot线,当压力超过粉体的致密压力后,测量数据点应该落在计算的粉末Hugoniot线上。本研究粉末的Hugoniot线使用等容Mie-Grüneisen方法计算得到。首先,基于W、Cu固体Hugoniot数据计算得到相同孔隙度的W、Cu粉体Hugoniot线,再经由Barry提出的两相混合Hugoniot原理计算得到W-Cu混合粉末的Hugoniot线。该原理主要存在两个基本假设:
(1)各组分压力相等;
(2)
V(p)=∑ni=1miVi(p) ,EC(V)=∑ni=1miECi(V) ,∑ni=1mi=1 ,EC是材料比内能。对于p-
α 模型,孔隙度α 可由N和n的值经拟合得到,pE是从弹性压缩转为塑性压缩的临界值,αE 是相应的孔隙度,pS由实验数据和Mie-Grüneisen粉末状态方程的交点确定。p-λ 模型中的曲线拟合与上述方法类似。混合物的屈服强度Y采用体积分数平均法计算。压力计算基于飞片和靶板材料的状态方程(EOS)。所用参数在表2中给出,其中C0和S是线性冲击状态方程uS=C0+SuP参数 , γ0是Grüneisen常数。符合最佳拟合结果的数据在表3中给出。表 2 组分和混合物的相关冲击和材料特性Table 2. Relevant shock and material properties of constituents and mixturesMaterial ρ0/(g·cm–3) C0/(km·s–1) S γ0 V0/(cm3·g–1) Y/GPa W 19.35 4.064 1.204 1.78 0.052 0 1.700 Cu 8.93 3.910 1.510 1.97 0.112 1 0.065 W-Cu 15.117 4.027 1.277 1.83 0.066 4 1.030 表 3 模型拟合参数Table 3. Model fitting parametersModel Parameters pS/GPa pE/GPa αE N p-α(MQ) 4.60 0.057 7 1.359 1.952 p-α(PL) pS/GPa n 4.60 7.80 p-α(SS) Y/GPa 1.273 4 p-λ n 1.51 图6为多个模型的拟合结果。可以看到粉末在4~5 GPa压力下达到致密,压力继续升高后,数据点落到计算得到的粉体Hugoniot线上。除p-
α PL模型外,其他模型均较好地描述了W-Cu混合粉末的冲击压缩响应。但在孔隙度接近零时,预测结果均与计算的粉末Hugoniot线有一定偏差,这是由于Mie-Grüneisen方法忽略了材料强度和实验初始孔隙度测量误差所致。p-α MQ和p-α PL模型中都存在压溃强度pS,经拟合得到pS= 4.6 GPa;参数N影响曲线的曲率,N取值大则曲率增加,p-α PL模型受N取值影响较大,拟合效果较差。p-α SS模型仅受粉末的屈服强度Y的影响,完全忽略了粉末的微观尺度效应(颗粒形状和大小),但得到了较好的拟合结果,预测的压溃强度pS为4~5 GPa,与p-α MQ和p-α PL模型一致。p-λ 模型也可以较好地描述低压段的冲击压实响应,但孔隙度无限接近于零时,在屈服强度Y一定的前提下,参数n的取值决定了曲线的曲率,当n减小时,曲率减小,pS急剧增大,而Y的取值影响pE的值。由于低于4 GPa的数据点仅有一个,所以很难确定其余模型哪个更适合描述W-Cu混合粉末的冲击压缩响应。拟合过程中发现,所有模型通过参数调整都可以获得较好的拟合效果,是典型的描述型模型,预测性较差。如p-α MQ和p-α PL模型中的参数pS无法通过实验测量,p-α SS和p-λ 模型中的Y受混合颗粒特性(如颗粒的尺寸、颗粒级配和颗粒形状等)的影响显著,无法准确预测。此外,除p-α PL模型外,其余模型均能很好地描述粉末压缩中由弹性转塑性的行为,而p-α PL模型经参数调整后也可以描述这一转变,但其曲线曲率变小,如图6所示,与实验数据差别较大。4. 结 论
测量了W-Cu混合粉末的Hugoniot数据,系统分析了测量误差,发现粉末致密度测量精度对结果影响较大。利用Mie-Grüneisen方法和Barry等压混合法,可以利用单质W、Cu粉末的Hugoniot关系很好地预测同孔隙度W-Cu混合粉末的Hugoniot线,但由于忽略了粉体强度和粉体初始密度测量误差较大的原因,预测结果在低压段与实验偏差较大。分别应用3种p-
α 模型和p-λ 模型对实验结果进行拟合,发现除p-α PL模型外,其他模型均能较好地描述W-Cu混合粉末的冲击压缩响应,表明这些原本用于描述单质粉末的冲击压实响应的模型对于更为复杂的混合粉末也是适用的,但所有模型的拟合精度均受经验参数选择的影响,预测能力较差。 -
Component ρ/(kg·m−3) E/GPa G/GPa v A/MPa B/MPa n C ˙ε0/s−1 cp/(J·kg−1·K−1) T0/K Tm/K m Target material 7 850 206 80 0.30 1200 1 580 0.175 0.004 0.000 1 450 300 1 800 1.00 Bullet core 7 850 206 80 0.30 1900 1 100 0.065 0.050 0.001 0 477 300 1 800 1.00 Brass jacket 8 960 124 46 0.34 90 292 0.310 0.025 1.000 0 386 300 1 356 1.09 表 2 孔结构装甲可靠性优化设计相关变量的约束范围
Table 2. Ranges of the relevant variables in reliability optimization design of perforated structure armor
R/mm d/mm v/(m∙s−1) θ/(°) 4.5−6.2 7.8−12.4 834−874 85−95 表 3 最优拉丁超立方法抽取的采样点和响应
Table 3. Sampling points and responses obtained by the optimal Latin hypercube method
R/mm d/mm v/(m·s−1) θ/(°) v′/(m·s−1) m′/g 6.052 10.200 870.522 94.130 811.129 4.662 5.978 8.600 867.043 86.304 808.016 4.889 5.387 8.400 844.435 93.261 760.791 4.854 4.796 12.200 842.696 86.739 675.821 4.112 4.722 8.800 837.478 85.435 603.861 3.958 4.943 11.800 874.000 87.174 710.546 4.245 5.017 11.600 856.609 90.652 667.470 4.005 5.239 10.800 872.261 92.826 780.641 4.506 5.683 11.200 853.130 88.478 754.275 4.529 5.461 9.200 849.652 85.870 747.881 4.622 4.870 9.800 846.174 91.957 691.675 4.366 5.830 11.000 847.913 93.696 762.888 4.720 5.535 12.000 868.783 89.783 761.734 4.493 4.500 12.400 851.391 91.522 568.453 3.551 6.126 10.400 839.217 89.348 782.666 4.794 5.609 7.800 840.957 88.043 788.874 4.941 5.313 10.600 835.739 92.391 719.739 4.375 5.757 9.000 834.000 91.087 759.182 4.708 6.200 8.200 858.348 95.000 814.127 5.034 4.648 11.400 860.087 85.000 701.905 4.282 5.091 9.400 865.304 88.914 725.571 4.170 5.904 10.000 863.565 90.217 800.931 4.745 5.165 8.000 861.826 94.565 767.436 4.786 4.574 9.600 854.870 87.609 648.105 4.038 表 4 剩余速度响应代理模型构建的新增样本和响应
Table 4. Added samples and responses built by residual velocity response surrogate model
R/mm d/mm v/(m·s−1) θ/(°) v′/(m·s−1) 4.668 12.400 835.174 91.508 562.223 6.200 12.400 837.169 92.044 779.260 4.500 9.031 843.095 86.100 604.955 4.501 12.399 857.090 88.573 529.787 4.503 9.796 853.839 86.088 619.838 6.200 12.400 837.029 85.000 757.080 表 5 剩余质量响应代理模型构建的新增样本和响应
Table 5. Added samples and responses built by residual mass response surrogate model
R/mm d/mm v/(m·s−1) θ/(°) m′/g 6.200 12.395 835.626 85.000 4.713 4.500 7.800 873.789 85.000 3.846 4.500 7.800 856.411 88.096 4.159 4.500 12.400 834.180 92.023 3.463 6.200 7.800 834.000 85.000 5.209 4.500 12.400 834.000 94.902 3.448 表 6 校核样本点和结果
Table 6. Check samples and results
R/mm d/mm v/(m·s−1) θ/(°) v′/(m·s−1) Residual velocity
accuracy checkingm′/g Residual mass
accuracy checkingSur. Sim. AAE/% MAE/(m·s−1) Sur. Sim. AAE/% MAE/g 5.860 11.480 874 91 805.051 805.150 0.58 8.394 4.700 4.650 1.58 0.105 4.500 12.400 850 90 555.406 552.960 3.636 3.531 6.200 8.720 842 87 820.845 814.010 5.116 5.048 4.840 9.640 866 85 698.654 690.260 4.159 4.229 5.180 10.560 834 93 697.718 701.010 4.288 4.329 5.520 7.800 858 95 788.991 793.070 4.933 4.929 Note: Sur. means surrogate model. 表 7 随机变量参数的概率分布信息
Table 7. Probability distribution information of random variables and parameters
Symbol Distribution pattern Mean Variance Variable upper limit Variable lower limit R Normal distribution μR 0.02μR 4.5 mm 6.2 mm d Normal distribution μd 0.02μd 7.8 mm 12.4 mm v Normal distribution 854 m/s 17.08 m/s θ Normal distribution 90° 1.8° 表 8 孔结构装甲优化参数结果
Table 8. Parameter results of the optimization of perforated structure armor
Variable R/mm d/mm n1 n2 Initial design 6.00 10.00 10 11 Reliability optimization 5.06 7.83 13 15 Note: n1 is the maximum hole quantity in each row; n2 is the maximum hole quantity in each column. 表 9 输出响应和孔结构装甲质量属性
Table 9. Output responses and the quality attributes of perforated structure armor
Responses v′/(m⋅s−1) m′/g M/g σ/(g⋅cm−2) Lwd/% Initial design 794.460 4.739 331.168 3.31 0 Reliability optimization 739.480 4.557 292.940 2.93 11.50 Note: Mis the mass of the perforated armor; σ is the surface density of the perforated armor; Lwd is the lightweight degree
of the perforated armor. -
[1] CHOCRON S, ANDERSON C E JR, GROSCH D J, et al. Impact of the 7.62 mm APM2 projectile against the edge of a metallic target [J]. International Journal of Impact Engineering, 2001, 25(5): 423–437. doi: 10.1016/S0734-743X(00)00063-4 [2] BEN-MOSHE D, TARSI Y, ROSENBERG G. An armor assembly for armored vehicles: EP 0209221 [P]. 1986. [3] KILIÇ N, BEDIR S, ERDIK A, et al. Ballistic behavior of high hardness perforated armor plates against 7.62 mm armor piercing projectile [J]. Materials & Design, 2014, 63: 427–438. [4] MISHRA B, RAMAKRISHNA B, JENA P K, et al. Experimental studies on the effect of size and shape of holes on damage and microstructure of high hardness armour steel plates under ballistic impact [J]. Materials & Design, 2013, 43: 17–24. [5] RADISAVLJEVIC I, BALOS S, NIKACEVIC M, et al. Optimization of geometrical characteristics of perforated plates [J]. Materials & Design, 2013, 49: 81–89. [6] 王郑. 间隙对穿孔装甲抗弹性能影响的数值分析 [J]. 科技创新与生产力, 2016(2): 87–89. doi: 10.3969/j.issn.1674-9146.2016.02.087WANG Z. Numerical analysis about the influence of intervals on the ballistic performance of perforated armor [J]. Sci-Tech Innovation and Productivity, 2016(2): 87–89. doi: 10.3969/j.issn.1674-9146.2016.02.087 [7] 王建波, 闫慧敏, 范秉源, 等. 弹着点对多孔钢板抗弹性能影响的数值模拟 [J]. 兵器材料科学与工程, 2010, 33(6): 73–75. doi: 10.3969/j.issn.1004-244X.2010.06.022WANG J B, YAN H M, FAN B Y, et al. Numerical simulation analysis about the influence of the hitting position on the ballistic performance of the multi-hole steel plate [J]. Ordnance Material Science and Engineering, 2010, 33(6): 73–75. doi: 10.3969/j.issn.1004-244X.2010.06.022 [8] 胡丽萍, 王智慧, 满红, 等. 孔结构间隙复合装甲位置效应研究 [J]. 兵器材料科学与工程, 2010, 33(1): 89–90. doi: 10.3969/j.issn.1004-244X.2010.01.025HU L P, WANG Z H, MAN H, et al. Study on the spot effect of spaced composite armor with multi-holes [J]. Ordnance Material Science and Engineering, 2010, 33(1): 89–90. doi: 10.3969/j.issn.1004-244X.2010.01.025 [9] 秦庆华, 崔天宁, 施前, 等. 孔结构金属装甲抗弹能力的数值模拟 [J]. 高压物理学报, 2018, 32(5): 055105.QIN Q H, CUI T N, SHI Q, et al. Numerical study on ballistic resistance of metal perforated armor to projectile impact [J]. Chinese Journal of High Pressure Physics, 2018, 32(5): 055105. [10] 肖红亮, 李晓源, 时捷, 等. 倾角效应对高强度钢板抗弹性能的影响 [J]. 兵器材料科学与工程, 2011, 34(6): 36–40.XIAO H L, LI X Y, SHI J, et al. Influence of obliquity effect on the ballistic performance of high strength steel plate [J]. Ordnance Material Science and Engineering, 2011, 34(6): 36–40. [11] 李换芝. 倾角穿孔装甲对14.5 mm穿燃弹防护性能的影响 [J]. 科技创新与生产力, 2016(2): 90–91, 94. doi: 10.3969/j.issn.1674-9146.2016.02.090LI H Z. Influence of oblique perforated armor on the protective performance of the 14.5 mm armor-piercing incendiary [J]. Sci-Tech Innovation and Productivity, 2016(2): 90–91, 94. doi: 10.3969/j.issn.1674-9146.2016.02.090 [12] 彭吉祥, 崔天宁, 金永喜, 等. 斜孔结构装甲设计及抗弹性能研究 [J]. 应用力学学报, 2021, 38(3): 893–901.PENG J X, CUI T N, JIN Y X, et al. Research on design and ballistic resistance of perforated armor with oblique holes [J]. Chinese Journal of Applied Mechanics, 2021, 38(3): 893–901. [13] BURIAN W, ŻOCHOWSKI P, GMITRZUK M, et al. Protection effectiveness of perforated plates made of high strength steel [J]. International Journal of Impact Engineering, 2019, 126: 27–39. doi: 10.1016/j.ijimpeng.2018.12.006 [14] CUI T N, QIN Q H, YAN W M, et al. Ballistic resistance of novel amorphous-alloy-reinforced perforated armor [J]. Acta Mechanica Solida Sinica, 2021, 34(1): 12–26. doi: 10.1007/s10338-020-00180-1 [15] JOHNSON G R, COOK W H. A constitutive model and data for metals subjected to large strains, high strain rates, and high temperatures [C]//Proceedings of the 7th International Symposium on Ballistics. The Hague, 1983: 541−547. [16] 张冬冬, 郭勤涛. Kriging响应面代理模型在有限元模型确认中的应用 [J]. 振动与冲击, 2013, 32(9): 187–191. doi: 10.3969/j.issn.1000-3835.2013.09.036ZHANG D D, GUO Q T. Application of Kriging response surface in finite element model validation [J]. Journal of Vibration and Shock, 2013, 32(9): 187–191. doi: 10.3969/j.issn.1000-3835.2013.09.036 [17] 周宁. ANSYS-APDL高级工程应用实例分析与二次开发 [M]. 北京: 中国水利水电出版社, 2007. [18] JONES D R, SCHONLAU M, WELCH W J. Efficient global optimization of expensive black-box functions [J]. Journal of Global Optimization, 1998, 13(4): 455–492. doi: 10.1023/A:1008306431147 [19] SIMPSON T W, POPLINSKI J D, KOCH P N, et al. Metamodels for computer-based engineering design: survey and recommendations [J]. Engineering with Computers, 2001, 17(2): 129–150. doi: 10.1007/PL00007198 [20] KRIGE D G. A statistical approach to some basic mine valuation problems on the Witwatersrand [J]. Journal of the Southern African Institute of Mining and Metallurgy, 1951, 52(6): 119–139. [21] 郭领. 孔结构金属装甲抗小口径穿甲弹的厚度效应 [D]. 南京: 南京理工大学, 2012.GUO L. The thickness effect of metal armor with poerstructure penetrating by Small caliber projectile [D]. Nanjing: Nanjing University of Science & Technology, 2012. [22] STANAG N. Protection levels for occupants of logistic and light armoured vehicles [S]. 2004. [23] DU X P, CHEN W. Sequential optimization and reliability assessment method for efficient probabilistic design [J]. Journal of Mechanical Design, 2004, 126(2): 225–233. doi: 10.1115/1.1649968 [24] AOUES Y, CHATEAUNEUF A. Benchmark study of numerical methods for reliability-based design optimization [J]. Structural and Multidisciplinary Optimization, 2010, 41(2): 277–294. doi: 10.1007/s00158-009-0412-2 [25] HUANG Z L, JIANG C, ZHOU Y S, et al. An incremental shifting vector approach for reliability-based design optimization [J]. Structural and Multidisciplinary Optimization, 2016, 53(3): 523–543. doi: 10.1007/s00158-015-1352-7 [26] ZHANG Z, DENG W, JIANG C. Sequential approximate reliability-based design optimization for structures with multimodal random variables [J]. Structural and Multidisciplinary Optimization, 2020, 62(2): 511–528. doi: 10.1007/s00158-020-02507-5 -







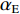
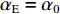

 下载:
下载:
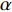
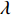
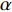
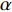
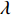
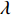
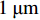



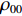
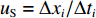

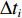
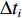
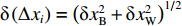


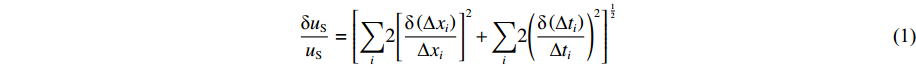
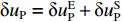

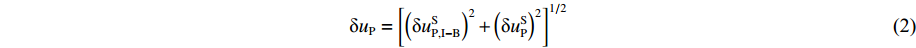

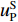
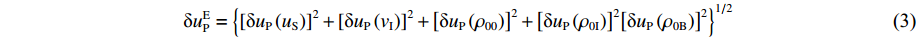


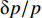
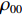
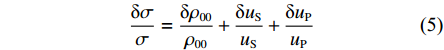

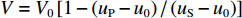
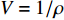

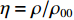
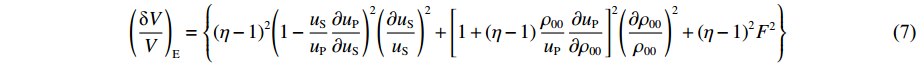
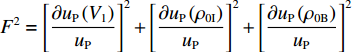

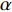
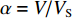
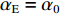
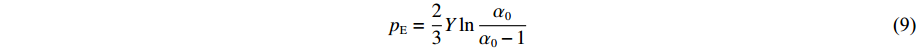
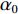

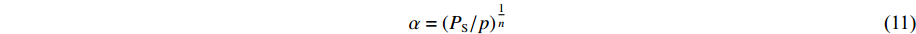

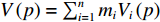

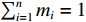
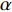
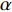
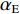
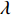
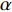
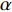

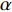
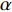
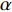
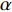
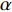
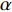
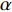
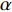
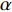
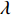
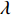

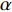
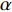
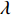

 下载:
下载: