Mechanical Behaviors and Constitutive Model of Polymide under Quasi-Static and Dynamic Compressive Loading
-
摘要: 为研究聚酰亚胺在动静态压缩载荷作用下的力学特性,采用材料试验机(material testing system, MTS)和分离式霍普金森压杆(split Hopkinson pressure bar, SHPB)进行压缩实验,得到了材料在不同应变率下的应力-应变曲线。通过分析回收试样的形貌,得到了聚酰亚胺在裂纹形式、尺寸变形等方面的特性。聚酰亚胺屈服强度的动态增强因子与应变率的关系具有明显的双线性特性,可采用多元线性方程或Cowper-Symonds模型描述。针对聚酰亚胺的动态力学响应特性,阐述了从低应变率到高应变率范围内的压缩变形机理。采用考虑
$\,\beta $ 转变的唯象本构模型,描述了聚酰亚胺在大应变率范围内的弹塑性大变形响应,包括初始黏弹性、屈服、应变软化和应变硬化在内的力学行为。通过贝叶斯方法拟合模型参数,拟合结果与实验结果在各应变率下都具有较好的一致性。-
关键词:
- 聚酰亚胺 /
- 应变率效应 /
- $\alpha $转变 /
- $\,\beta $转变 /
- 本构模型
Abstract: To study the mechanical properties of polyimide, quasi-static and dynamic compression experiments were carried out using the materials testing system (material testing system, MTS) and the split Hopkinson pressure bar (SHPB). The stress-strain curves of the material under different strain rates were obtained. The morphology of the recovered specimens was analyzed, and the characteristics of the polyimide in terms of crack form and size deformation were obtained. A bilinear relationship between the dynamic increase factor of the polyimide and the strain rate was obtained. The bilinear characteristics were described by piecewise fitting model and Cowper-Symonds model. Based on the characteristics of the dynamic mechanical response of the polyimide, its compression deformation mechanism from low to high strain-rates was explained. A phenomenological constitutive model in consideration of the contributions of the$\,\beta $ -transition was modified to describe the large elastic-plastic deformation response of the material, in which initial viscoelasticity, yield, strain softening and strain hardening were all included. Then the constitutive model’s parameters were fitted by Bayesian approach. The Bayesian fitting results at different strain rates were in good agreement with the experimental data.-
Key words:
- polyimide /
- strain rate effect /
- $\alpha $ transition /
- $\,\beta $ transition /
- constitutive model
-
聚酰亚胺(polyimide,PI)因其优异的冲击吸能效果、良好的加工性能和较高的热变形温度,以薄膜、复合材料、泡沫等多种形式广泛应用于封装涂覆、飞行器机身绝热板、液晶显示等[1-3]。在实际使用过程中,聚酰亚胺时常受到各种静态及动态载荷的作用,因而,探究其在不同应变率下的压缩力学行为具有重要的研究意义和实用价值。
随着霍普金森实验技术的发展,国内外学者对不同应变率下聚酰亚胺类高分子聚合物的压缩性能开展了一系列实验和理论研究。聚合物的应力-应变曲线可大致分为两类:应力-应变曲线单调递增,无明显屈服段,如聚乙烯[4]和聚甲基苯烯酸甲酯(polymethyl methacrylate, PMMA)[5]等;应力-应变曲线出现明显的屈服段,包括屈服软化和屈服平台,随后发生应变硬化,如聚氨酯[6]、聚脲[7]和聚碳酸酯[8]等。Chou等[9]在宽应变率范围内对PMMA、聚丙烯和尼龙66等多种聚合物的应力-应变行为进行研究,发现随着应变率的增加,屈服强度与应变率对数
$ \lg \dot \varepsilon $ 存在双线性关系。Walley等[10]研究了大量聚合物在室温下10−2~104 s−1应变率范围内的力学行为,发现聚合物的屈服强度与$ \lg \dot \varepsilon $ 的关系可分为3类:线性关系、存在梯度剧增的双线性关系和高应变率下先降低后增加的关系。Goglio等[11]研究了双组分环氧胶粘剂的抗拉强度和抗压强度,利用与应变率相关的模型对屈服强度和应变进行拟合。于鹏等[12]总结了聚碳酸酯(polycarbonate,PC)类非晶聚合物的力学性能实验研究现状,综述了PC类聚合物的主流本构模型发展现况,阐述了本构方程的基本原理、方法和特点等。陈春晓等[13]进行了聚甲醛在温度为25、50、70 ℃,应变率为800、1400、2300、3100 s−1时的动态压缩实验,采用ZWT本构模型的非线性黏弹性部分替换JC本构模型的应变硬化部分,提出了经验型本构方程,结果显示在应变5%以内时经验本构方程对实验结果的拟合效果较好。Wang等[14-15]研究了PC在宽应变率范围内的力学行为,利用动态力学分析(dynamic mechanical analysis,DMA)观察了PC的玻璃转变和次级转变,在Mulliken-Boyce、G'Sell-Jonas和DSGZ 3个模型的基础上,提出了预测PC力学行为的绝热模型,该模型考虑$\alpha $ 和$\,\beta $ 转变对力学行为的贡献。综上可知,目前,已进行PC、PMMA、尼龙66等普通工程塑料在宽应变率范围内压缩力学特性的一系列研究,针对材料屈服强度与应变率的关系提出了多种本构模型。然而,目前聚酰亚胺特种塑料研究主要集中在理化特性、分子特性等,少有涉及不同应变率下压缩行为和变形机制。基于上述研究现状,本研究在室温条件下采用电子万能材料试验机(material testing system, MTS)和霍普金森压杆(split Hopkinson pressure bar,SHPB)开展聚酰亚胺在准静态和动态下的压缩实验,以得到不同应变率下的应力-应变曲线,通过分析不同应变率下试样的变形特征,揭示相应的变形机理,并提出一种考虑β转变的本构模型。
1. 实 验
1.1 材料和试样
实验材料为上海盛钧塑胶科技有限公司生产的SJ-100纯聚酰亚胺,是通过粉末冶金法模压成型的热固性材料,密度为1420 kg/m3,热变形温度大于360 ℃,具有极好的耐高温性能。
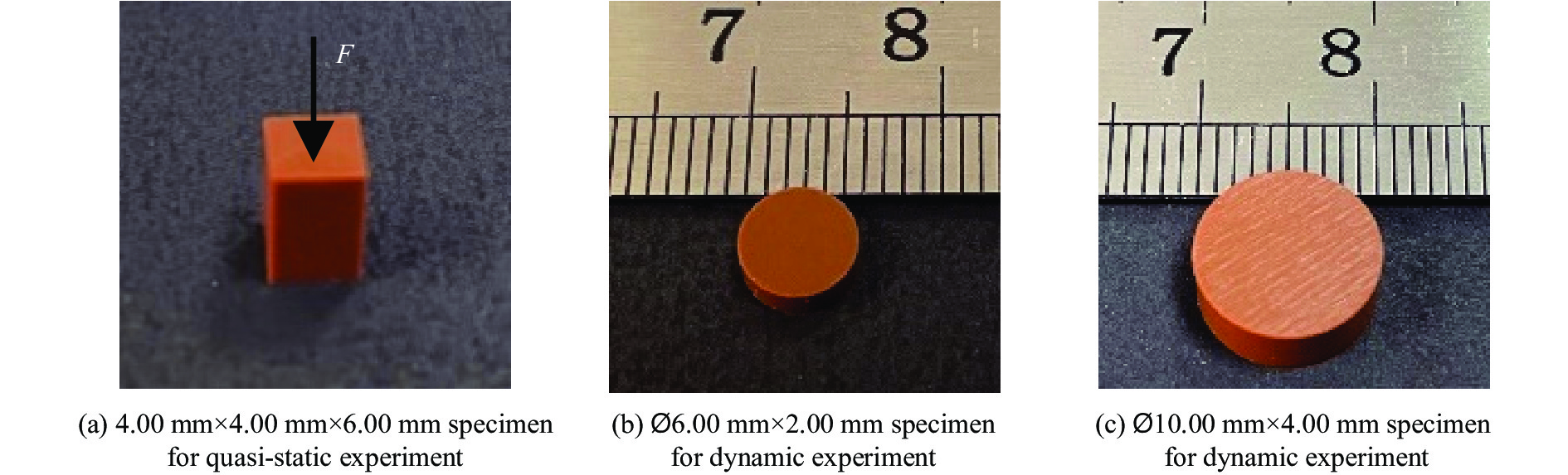
根据GB/T 1041—2008中规定的小试样尺寸标准,设计加工了尺寸为4.00 mm×4.00 mm×6.00 mm的立方体试样用于准静态压缩实验;依据GJB 5982—2007,工程塑料动态压缩实验所用圆柱形试样的长径比取0.3~0.6为宜,为达到动态实验中宽应变率的实验要求,设计加工了尺寸为
$\varnothing $ 6.00 mm×2.00 mm和$\varnothing $ 10.00 mm×4.00 mm的两种薄圆柱试样。使用高精度数控铣床切割试样,保证试样尺寸精度和表面光滑度,试样照片见图1。1.2 实验设备及方法
准静态和动态压缩实验均在室温下进行。准静态压缩实验在爆炸科学与技术国家重点实验室的MTS上完成,利用数据采集软件记录加载力F和试样位移S。根据试样的几何尺寸,将工程应力
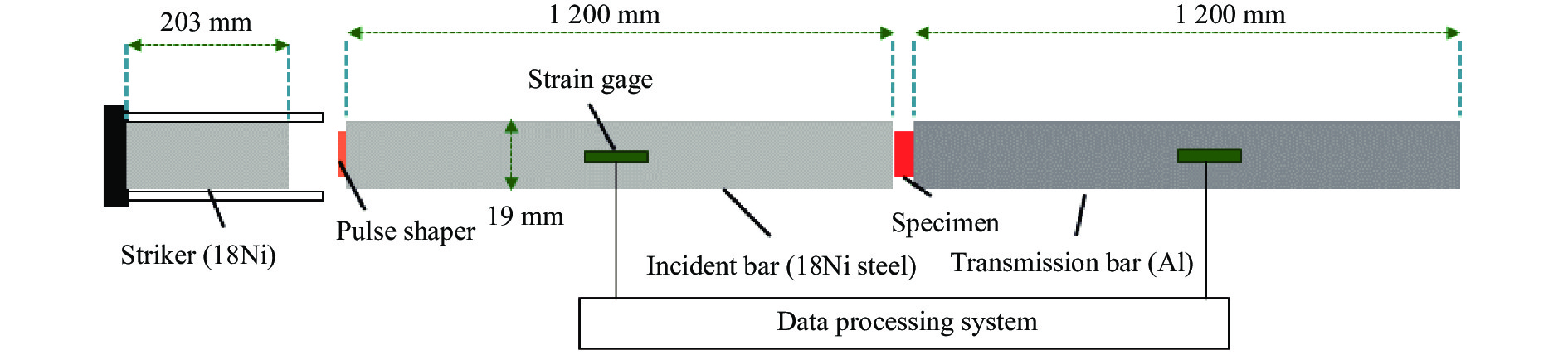
$ {\sigma _{\text{E}}} $ 和工程应变$ {\varepsilon _{\text{E}}} $ 转化为真实应力$ {\sigma _{\text{T}}} $ 和真实应变$ {\varepsilon _{\text{T}}} $ [4]σT=σE(1−εE) (1) εT=−ln(1−εE) (2) 动态压缩实验在爆炸科学与技术国家重点实验室的SHPB上进行。SHPB装置主要由撞击杆、入射杆、透射杆以及数据采集系统组成,如图2所示。撞击杆和入射杆采用18Ni钢,弹性模量E=190 GPa,密度
$\,\rho $ =8000 kg/m3,撞击杆杆长203 mm,入射杆杆长1 200 mm;透射杆采用铝杆,弹性模量E=70 GPa,密度$\,\rho $ =2700 kg/m3,杆长1200 mm;撞击杆、入射杆、反射杆的直径均为19 mm。实验中,将试件置于入射杆与透射杆之间,采用退火铜片作为整形器粘贴在撞击杆侧的入射杆端,以使加载波的上升沿变得平缓,保证样品中的应变率近似恒定[16]。聚酰亚胺的密度远小于18Ni钢,若输出杆同样采用18Ni钢,则杆上的透射信号较弱,所得信号的信噪比较小,难以确定透射波的起跳点,数据处理误差较大,因此选用广义波阻抗比18Ni钢低的铝杆作为透射杆,从而放大透射信号。本研究采用基于绝对时间的简化三波法处理实验数据,应变计算采用三波法[17],应力计算采用透射波[17],得到试件的应变率
$ \dot \varepsilon (t) $ 、应变$ \varepsilon (t) $ 和应力$ \sigma (t) $ ˙ε(t)=CI(εI−εR)−CTεTL (3) ε(t)=1L∫t0[CI(εI−εR)−CTεT]dt (4) σ(t)=ETAεTAS (5) 式中:
$ {E_{\text{T}}} $ 、$ {C_{\text{I}}} $ 、$ {C_{\text{T}}} $ 、$ A $ 、$ {A_{\text{S}}} $ 分别为透射杆的弹性模量、入射杆波速、透射杆波速、杆的横截面积和试样的横截面积,$ {\varepsilon _{\text{I}}} $ 、$ {\varepsilon _{\text{R}}} $ 、$ {\varepsilon _{\text{T}}} $ 分别为入射应变、反射应变和透射应变。2. 实验结果分析
2.1 准静态压缩实验结果分析
2.1.1 准静态压缩下PI的形貌
MTS以0.36、3.60、36.00 mm/min的恒定加载速率压缩试样,对应的应变率分别为0.001、0.010、0.100 s−1。每个加载速率下重复3次实验,回收后的试样宏观状态见表1。试样1、试样2和试样3以0.36 mm/min的加载速率压缩,3个试样表面均未发生宏观破坏;试样4、试样5和试样6是以3.60 mm/min的加载速率压缩,只有试样6的表面出现宏观破坏,且裂纹仅在一个表面产生,未产生贯穿破坏;试样7、试样8和试样9以36.00 mm/min 的加载速率压缩,3个试样的表面均出现了宏观破坏,且试样8中裂纹出现在前、后两个表面,发生贯穿破坏。随着加载速率的增加,聚酰亚胺发生宏观破坏时的应变逐渐减小,破坏程度更加严重,其原因可能是高速加载导致分子链不能及时松弛至合理位置,进而使平均应力与应力集中程度提升。MTS设置的最大压缩位移为4.00 mm,因而,实验后试样在加载方向上的尺寸应为2.00 mm,但实际上在0.001、0.010、0.100 s−1 3种加载速率下试样在加载方向上的平均尺寸分别为2.76、2.72、2.59 mm,可见试件有明显回弹,并且随应变率的提升,发生宏观破坏的不可恢复性变形增加,回弹量减少。
表 1 准静态压缩实验后回收试样的形貌Table 1. Morphology of the recovered samples after quasi-static experimentNo. Strain rate/s−1 Specimens after quasi-static compression Size/(mm×mm×mm) Crack 1 0.001 
6.02×5.83×2.78 No 2 0.001 
6.15×5.72×2.74 No 3 0.001 
6.18×5.80×2.75 No 4 0.010 
6.13×5.83×2.71 No 5 0.010 
6.25×5.78×2.72 No 6 0.010 
6.46×5.70×2.72 Yes 7 0.100 
6.64×5.86×2.58 Yes 8 0.100 
6.76×5.69×2.59 Yes 9 0.100 
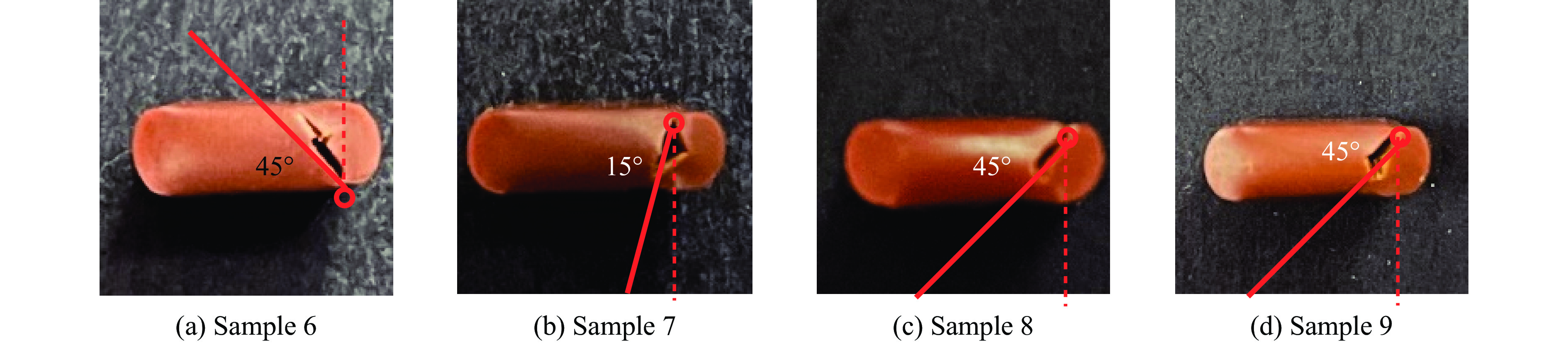
6.54×5.89×2.59 Yes 图3显示了试样6~试样9的裂纹形貌,可见,发生宏观破坏的试样整体未发生分离,部分粘连在一起,仍保持较好的完整性。由于应力集中,裂纹以加载面的顶角为端点,与加载方向呈一定的夹角延伸。试样6、试样8和试样9的断口两侧形状大致相似,呈现约45°角的斜劈裂,这是由于试件在压缩时与轴线成约45°角的斜截面具有最大的剪应力。试样7的断口两侧形状不同,劈裂角度约为15°,可能是因为在缓慢压缩过程中试样一侧产生失稳压溃,影响其沿最大剪应力方向的破坏。
2.1.2 准静态压缩应力-应变曲线
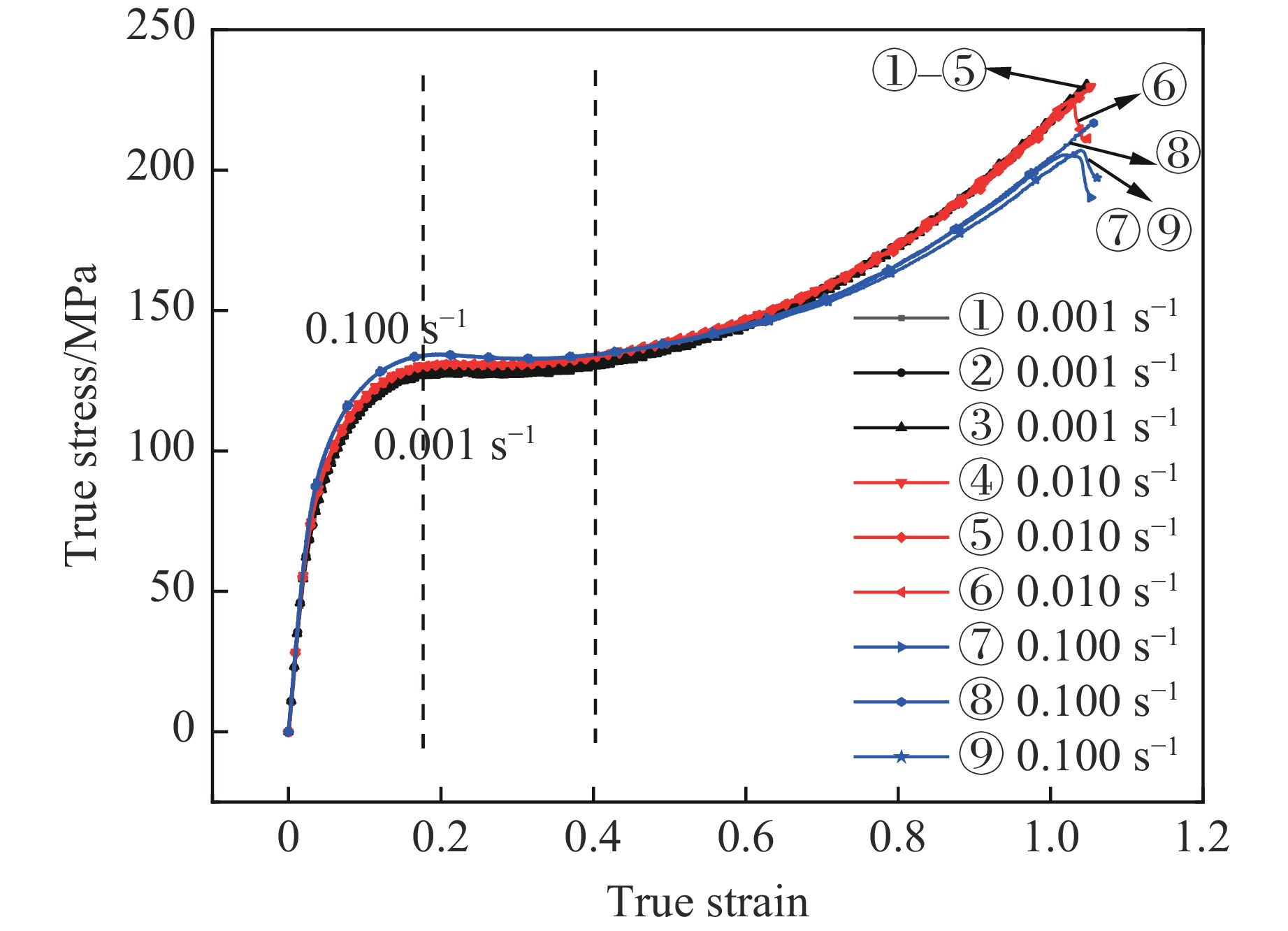
图4给出了试样在准静态压缩下的应力-应变曲线。0~0.17应变范围内,聚酰亚胺的应力随着应变非线性增加,为非线性黏弹性上升阶段,且随着载荷的增加,应力-应变曲线的切线模量逐渐减小,说明黏弹性形变在总形变中的比例逐渐增大;0.17~0.40应变范围内,材料进入屈服流动段,应力出现一定程度的下降,对应高分子黏弹性材料的应变软化现象;应变达到0.40之后,应力随应变的增加而不断增大,表现出应变硬化现象。对比不同应变率下的曲线可以发现,在准静态下,聚酰亚胺的屈服强度随着应变率的增加略微增加,从127.33 MPa增加到134.28 MPa,增幅约为5.46%,即聚酰亚胺具有一定的应变率效应。当试样发生破坏时,曲线出现拐点,该点对应的应力值代表材料的准静态压缩破坏强度。试样6、试样7和试样9在应变为1.0时出现明显拐点,材料发生损伤破坏,抵抗变形能力减弱,对应的准静态压缩强度约为207.00 MPa;试样1~试样5未出现拐点,仍具有较高的抵抗变形能力,加载结束时对应的准静态压缩强度为229.00 MPa。对比这两个准静态压缩强度可以发现,聚酰亚胺的压缩破坏强度随应变率的增加而减小。此外,试样8虽发生表面宏观破坏,但曲线未出现拐点,表明其仍具有一定的抵抗变形能力。准静态应力-应变曲线的重复性较好,在后面的分析中,实验数据将取3次重复实验中相近的2次实验结果的平均值。
2.2 动态压缩实验结果分析
2.2.1
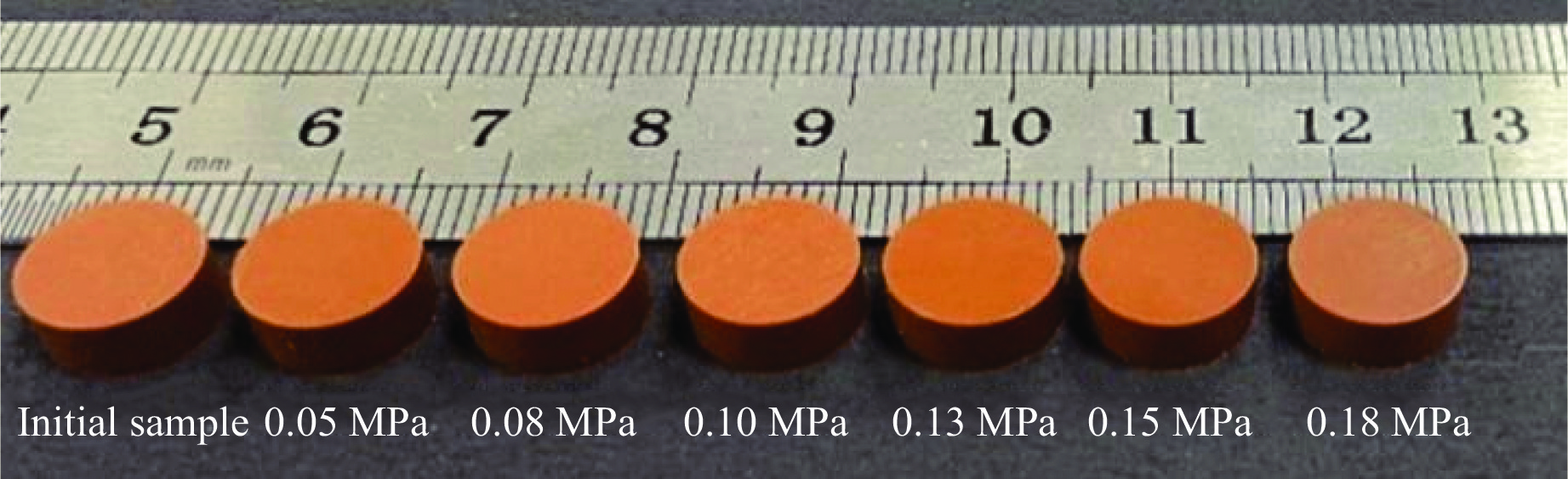
$\varnothing $ 10.00 mm×4.00 mm试样的动态压缩形貌分析采用SHPB装置在6种加载气压下(压力范围0.05~0.18 MPa)对
$\varnothing $ 10.00 mm×4.00 mm试样开展动态压缩实验,回收后试样的宏观形貌如图5所示。当发射气压为0.05、0.08、0.10、0.13、0.15、0.18 MPa时,回收试样的厚度分别为4.00、3.99、3.96、3.90、3.82、3.78 mm,厚度方向产生的最大变形为初始厚度的5.5%,即聚酰亚胺试样尺寸变化不大。2.2.2
$\varnothing $ 10.00 mm×4.00 mm试样的应变率及应力-应变曲线图6给出了发射气压为0.08 MPa时试样的典型应变率-时间曲线。图6显示,存在一个明显的平台区域,表明试样变形过程中应变率近似恒定。将该平台段的应变率平均值(AVG)作为该气压下动态压缩实验的应变率,可得发射气压为0.05、0.08、0.10、0.13、0.15、0.18 MPa时,对应的应变率分别为1494、2322、2862、3452、3842、4427 s−1。
图7给出了
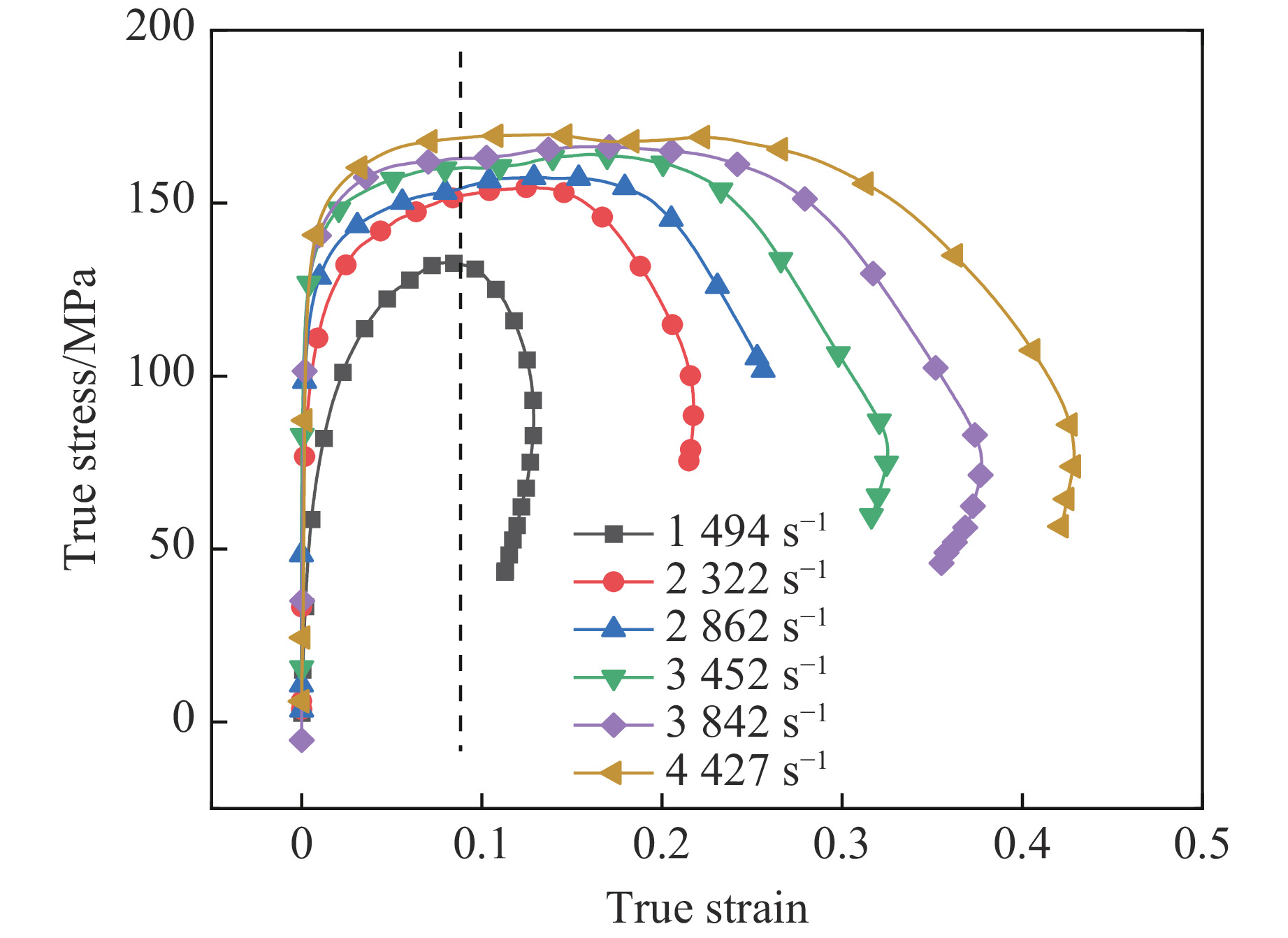
$\varnothing $ 10.00 mm×4.00 mm的试样在1494~4427 s−1应变率范围内的应力-应变曲线。1494 s−1应变率下的应力-应变曲线显示,材料应力随应变呈非线性迅速增加,达到最高点后发生卸载回弹。应变率为2322、2862、3452、3842、4427 s−1的应力-应变曲线显示,材料经过初始非线性黏弹性阶段后,当在真实应变达到0.09时未发生应变软化效应,而是产生屈服平台段,随后卸载。材料并未出现准静态加载中的应变硬化阶段,这是由于试样的几何尺寸过大,聚酰亚胺在初始加载阶段便吸收了第1个压缩波的大部分能量。在应变率从2322 s−1上升至4427 s−1的过程中,聚酰亚胺的屈服强度从153.08 MPa增加到167.56 MPa,增加约9.46%。2.2.3
$\varnothing $ 6.00 mm×2.00 mm试样的动态压缩形貌分析采用SHPB装置在6种加载气压下(压力范围0.05~0.20 MPa)对
$\varnothing $ 6.00 mm×2.00 mm试样开展动态压缩实验,发射气压为0.05、0.08、0.10、0.13、0.10、0.20 MPa,计算得到的应变率分别为3388、5195、6137、7627、8496、10371 s−1,回收后的试样形貌如表2所示。试样厚度从初始的2.00 mm减小至最终的1.00 mm,最大变形为50%,而聚酰亚胺试样仍未出现表面宏观破坏,这是由于动态试样的径厚比较大,在压缩加载下试样只会在加载方向上被压扁,径向中间凸起,呈“鼓状”,如图8所示。表 2 动态压缩后$\varnothing $ 6.00 mm×2.00 mm试样的形貌Table 2. Morphology of the recovered$\varnothing $ 6.00 mm×2.00 mm samples after dynamic compressionNo. Load pressure/MPa Strain rate/s−1 Size/(mm×mm) Specimens after dynamic compression Crack 1 0.05 3388 $\varnothing $6.13×1.91 
No 2 0.08 5195 $\varnothing $6.75×1.61 
No 3 0.10 6137 $\varnothing $7.08×1.48 
No 4 0.13 7627 $\varnothing $7.72×1.29 
No 5 0.17 8496 $\varnothing $8.48×1.10 
No 6 0.20 10371 $\varnothing $8.99×1.00 
No 2.2.4
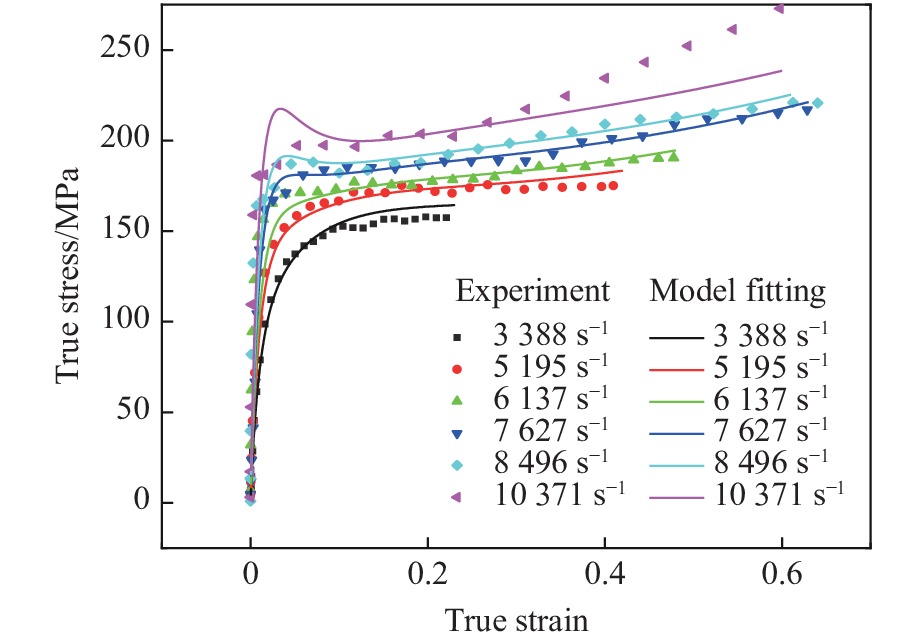
$\varnothing $ 6.00 mm×2.00 mm试样的应力-应变曲线图9给出了
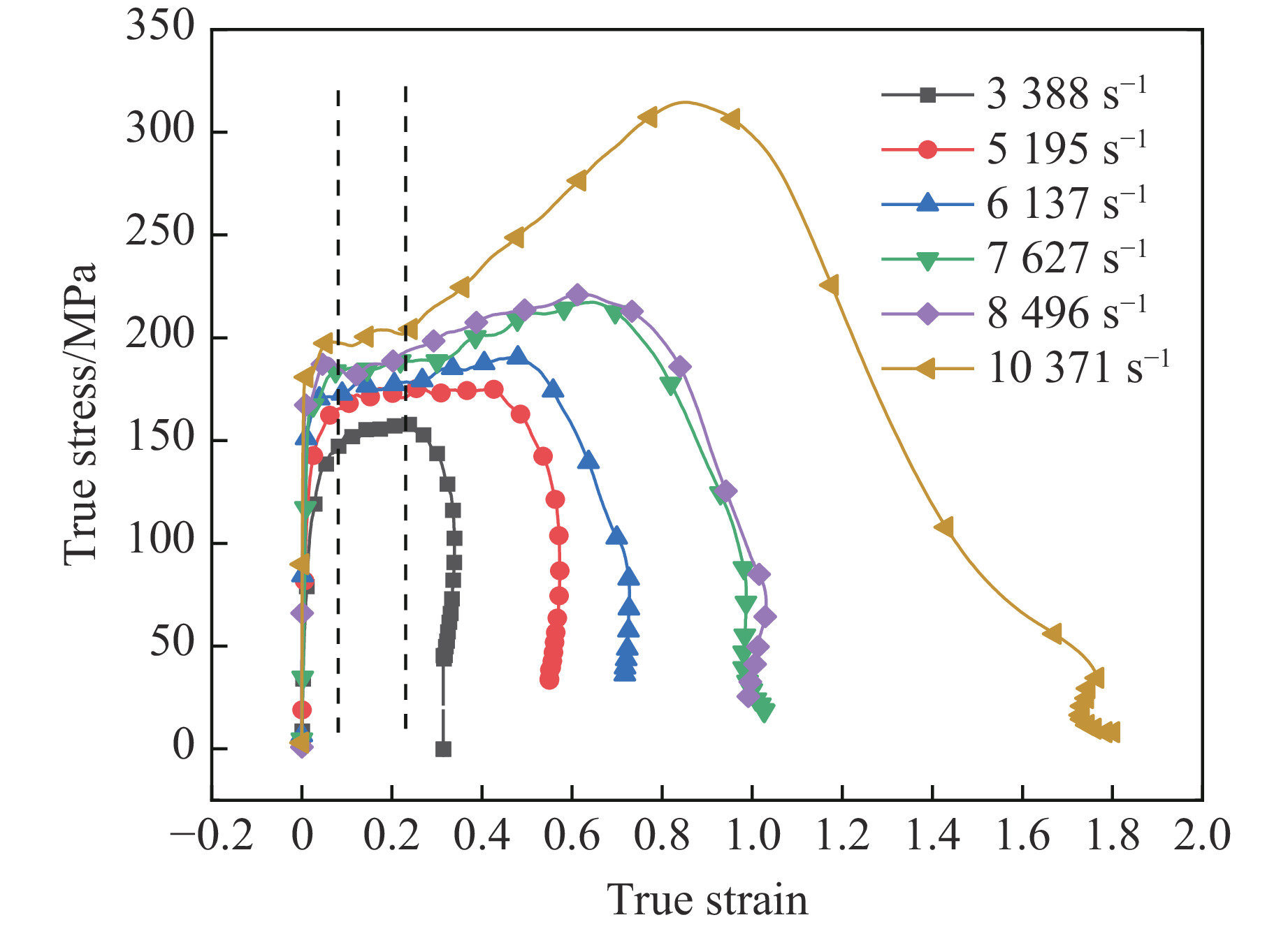
$\varnothing $ 6.00 mm×2.00 mm试样的应力-应变曲线。对比图9和图4可以看出,动态压缩下聚酰亚胺的非线性黏弹性段和屈服段的应变范围小于准静态压缩下,当应变达到0.09左右时,材料发生屈服,当应变达到0.21后,观察到应变硬化效应。此外,$\varnothing $ 6.00 mm×2.00 mm试样的动态应力-应变曲线虽然也出现了与准静态下类似的屈服平台段,但是与图7中$\varnothing $ 10.00 mm×4.00 mm试样的应力-应变曲线更相似。在3388、5195、6137 s−1应变率下,应力-应变曲线中的应变软化现象消失,取而代之的是稳定的塑性流动区,而后进入应力逐渐上升的应变硬化阶段。随着应变率继续升高,7627、8496、10371 s−1应变率下出现了一定的应变软化现象,这是因为虽然高应变率下的应变软化效应会弱化,但是在高应变率的绝热条件下,随着应变率继续升高,分子间相互作用能的变化引起局部温度明显上升,导致聚酰亚胺的热软化增强。因而,高应变率和准静态压缩下聚酰亚胺的压缩变形机制不同。图9显示,应变率变化从3388 s−1增加到10371 s−1的过程中,屈服强度从155.84 MPa增加到197.88 MPa,增加约26.98%。与准静态情况相比,屈服强度及其增幅均明显上升,表明高应变率下聚酰亚胺具有更明显的应变率效应。此外,应变率10371 s−1下的应力-应变曲线显著高于其他曲线,其原因可能是随着加载气压的增大,薄圆柱试样的轴向变形明显,导致试样与杆之间的摩擦效应的影响较大[18]。考虑到10371 s−1已超过聚酰亚胺实际应用中的应变率范围,因而此应变率下的应力-应变曲线不用于本构模型参数的确定。2.3 屈服强度与应变率的关系分析
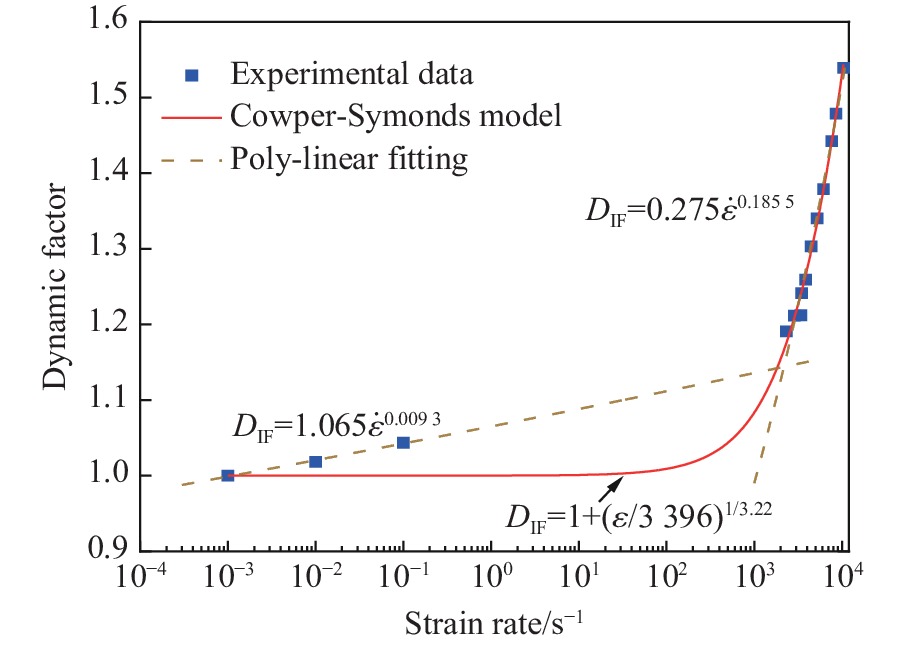
动态增强因子(dynamic increase factor,DIF)为动态强度
$\sigma $ d与静态强度$\sigma $ s之比,用于描述材料在高应变率下的力学性能的增强,解释材料与应变率相关的特性。为分析聚酰亚胺的弹塑性行为,建立宽应变率范围内动态增强因子DIF与应变率$ \dot \varepsilon $ 的关系,如图10所示。实验数据显示出动态增强因子在高、低应变率下的双线性行为:低应变率下,动态增强因子随应变率的增加而缓慢增大;高应变率下,动态增强因子随应变率增长速度快,敏感性较强。聚丙烯、聚苯乙烯等聚合物的宽应变率压缩实验中均有类似现象。这与聚合物材料中不同分子的转变有关,在高温或低应变速率下,只有玻璃态($\alpha $ )转变在聚合物行为中起作用,而在低温或高应变率下,次级($\,\beta $ )转变效应加入,导致较大的变形阻力,影响屈服强度[19]。本研究采用Cowper-Symonds模型[20]和多元线性拟合描述材料的动态增强效应。Cowper-Symonds模型[20]的表达式为
DIF=(1+˙ε/˙εDD)1/q (6) 式中:D和q为模型的两个参数。多元线性拟合由两个直线段组成,形式均为
$ {D_{{\text{IF}}}} = A{\dot \varepsilon ^m} $ ,其中,A和m为模型的参数。表3罗列了两种模型的拟合方程及其判定系数R2。表 3 两种模型的拟合方程与判定系数Table 3. Two models’ fitting equations and their determination coefficientsModel Equations R2 Cowper-Symonds ${ D_{ {\text{IF} } } } = {(1 + \dot \varepsilon /3\,396)^{1/3.22} }$ 0.99067 Poly-linear fitting ${ D_{ {\text{IF} } } } = \left\{ 1.065˙ε0.0093 ˙ε⩽0.100 s−10.275˙ε0.1855 ˙ε⩾3388 s−1 \right.$0.99206
0.98288最小二乘法拟合的Cowper-Symonds模型及多元线性拟合获得的结果也在图10中绘出。图10显示,Cowper-Symonds模型可以很好地描述聚酰亚胺动静态力学行为的梯度剧增行为,但低应变率下动态影响因子的变化不明显,拟合数据与准静态实验结果有所偏差。结合表3的判定系数可知:在准静态实验中,可通过多元线性拟合方程来描述聚酰亚胺的屈服强度;在动态实验中,多元线性拟合方程和Cowper-Symonds模型均可描述聚酰亚胺的屈服强度与应变率的相关性。
3. 本构模型及参数确定
3.1 考虑β转变的本构模型
Wang等[14-15]提出了考虑
$\,\beta $ 转变的黏弹性唯象本构模型σ = wασα + wβσβ (7) σα = Kα[exp(−α1ε) + εα2][1−exp(−α3ε)]exp(α4ε)˙εα5exp(α6/T) (8) σβ = Kβ[exp(−β1ε) + εβ2][1−exp(−β3ε)]exp(β4ε)˙εβ5exp(β6/T) (9) 式中:
$ w_{\alpha} $ 与$ w_{\beta} $ 分别为$\alpha $ 、$\,\beta $ 转变对力学行为的影响权重因子;$ {\sigma _\alpha } $ 和$ {\sigma _\beta } $ 分别为$\alpha $ 、$\,\beta $ 转变对总应力$ \sigma $ 的贡献;模型中的材料参数$ {K_\alpha } $ 、$ {\alpha _1} $ 、$ {\alpha _2} $ 、$ {\alpha _3} $ 、$ {\alpha _4} $ 、$ {\alpha _5} $ 与$\alpha $ 转变有关,$ {K_\beta } $ 、$ {\,\beta _1} $ 、$ {\,\beta _2} $ 、$ {\,\beta _3} $ 、$ {\,\beta _4} $ 、$ {\,\beta _5} $ 与$\,\beta $ 转变有关;$ 1 - \exp ( - {\alpha _3}\varepsilon ) $ 表示与$\alpha $ 转变相关的初始非线性黏弹性;$ \exp ( - {\alpha _1}\varepsilon ) $ 表示与$\alpha $ 转变相关的应变软化,$ {\varepsilon ^{{\alpha _2}}} $ 和$ \exp ({\alpha _4}\varepsilon ) $ 表示与$\alpha $ 转变相关的应变硬化,$ {\dot \varepsilon ^{{\alpha _5}}} $ 表示与$\alpha $ 转变相关的应变率相关项;$ 1 - \exp ( - {\beta _3}\varepsilon ) $ 表示与$\,\beta $ 转变相关的初始非线性黏弹性;$ \exp ( - {\beta _1}\varepsilon ) $ 表示与$\,\beta $ 转变相关的应变软化,$ {\varepsilon ^{{\beta _2}}} $ 和$ \exp ({\beta _4}\varepsilon ) $ 表示与$\,\beta $ 转变相关的应变硬化,$ {\dot \varepsilon ^{{\beta _5}}} $ 表示与$\,\beta $ 转变相关的应变率相关项。$ w_{\alpha} $ 和$ w_{\beta} $ 可以通过$\alpha $ 转变模量$ E_{\alpha} $ 和$\,\beta $ 转变模量$ E_{\beta} $ 来描述wα=Eα/Eα(Eα+Eβ)(Eα+Eβ) (10) wβ=Eβ/Eβ(Eα+Eβ)(Eα+Eβ) (11) 对上述模型进行改型。由于本实验未考虑实验温度对聚酰亚胺动静态力学性能的影响,因此改型后的本构模型不存在温度相关项。同时在唯象本构模型中,直接用
$ {\sigma _\alpha } $ 和$ {\sigma _\beta } $ 分别表示α转变和β转变对总应力$ \sigma $ 的影响。低应变率下,聚酰亚胺的力学行为由$\alpha $ 转变决定,$\,\beta $ 转变处于抑制状态并未激活,因此,低应变率下的本构模型函数为σα = Kα[exp(−α1ε) + εα2][1−exp(−α3ε)]exp(α4ε)˙εα5 (12) 高应变率下,
$\,\beta $ 转变被激活,聚酰亚胺的力学行为产生较大变化,因此,本构模型函数中的应力是α转变和$\,\beta $ 转变共同作用的结果,表示为σ = σα + σβ = Kα[exp(−α1ε) + εα2][1−exp(−α3ε)]exp(α4ε)˙εα5 + Kβ[exp(−β1ε) + εβ2][1−exp(−β3ε)]exp(β4ε)˙εβ5 (13) 动态加载过程中聚酰亚胺未产生失效破坏,应力-应变曲线在达到最高应力之后试样发生卸载,此后的曲线并不能真实反映材料的应力状态,因而,在后面的拟合过程中只选取卸载前的数据。
3.2 本构模型参数估计
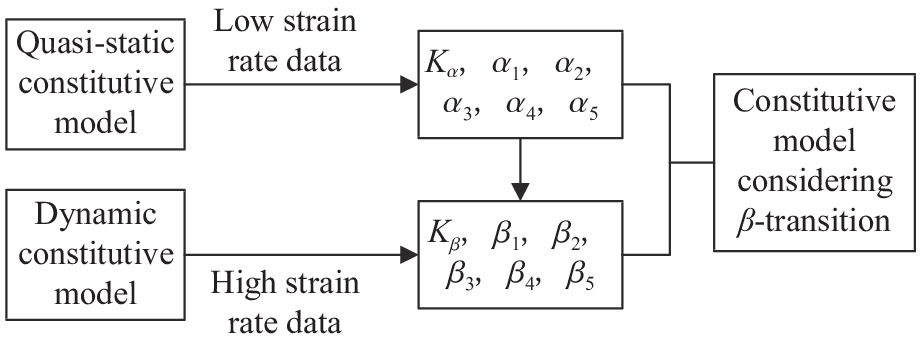
采用贝叶斯方法[21-22]预测本构模型参数:通过前面的MTS实验和SHPB实验依次估计材料在两种不同应变率下的力学行为,具体流程如图11所示,通过低应变率的实验数据拟合α相关的参数,再结合高应变率实验数据拟合
$\,\beta $ 相关的本构模型参数。采用贝叶斯方法估计材料参数时,仅使用4条不同应变率下的应力-应变曲线即可得到低、高应变率下所有应力-应变曲线。(1) 准静态本构关系参数确定
首先,选取低应变率(
$ {\dot \varepsilon _1} $ ,$ {\dot \varepsilon _2} $ )下两条应力-应变曲线,在相同应变处确定真实应力($ \varepsilon $ ,$ {\sigma _1} $ )和($ \varepsilon $ ,$ {\sigma _2} $ ),通过式(14)计算得到$ {\alpha _5} $ α5=ln(σ1σ2)ln(˙ε1˙ε2) (14) 其次,选取低应变率下某条应力-应变曲线,使用Levenberg-Marquardt最小二乘法进行函数拟合,得到
$ {K_\alpha } $ 、$ {\alpha _1} $ 、$ {\alpha _2} $ 、$ {\alpha _3} $ 、$ {\alpha _4} $ 的最优解。(2) 动态本构关系参数确定
首先,将低应变率下与α相关的材料参数作为常数,选取高应变率(
$ {\dot \varepsilon _1} $ ,$ {\dot \varepsilon _2} $ )下的两条应力-应变曲线,在相同应变处得到真实应力($ \varepsilon $ ,$ {\sigma _1} $ )和($ \varepsilon $ ,$ {\sigma _2} $ ),通过以下关系计算得到$ {\beta _5} $ β5=ln(σβ1σβ2)ln(˙ε1˙ε2) = ln(σ1−σα1σ2−σα2)ln(˙ε1˙ε2) (15) 式中:
$ {\sigma _{\beta 1}} = {\sigma _1} - {\sigma _{\alpha 1}} $ ,$ {\sigma _{\beta 2}} = {\sigma _2} - {\sigma _{\alpha 2}} $ ,$ \sigma _{\alpha 1}$ 、$\sigma _{\alpha 2}$ 为通过计算得到的该应变处的应力值。其次,选取高应变率下某条应力-应变曲线,使用Levenberg-Marquardt最小二乘法进行函数拟合,得到
$ {K_\beta } $ 、$ {\,\beta _1} $ 、$ {\,\beta _2} $ 、$ {\,\beta _3} $ 、$ {\,\beta _4} $ 的最优解。表4给出了0.001、0.010、6173、7627 s−1 4条不同应变率下的应力-应变曲线拟合得到的关于
$\alpha $ 、$\,\beta $ 转变相关的材料参数。表 4 α和β转变相关的材料参数Table 4. Material parameters related to the α and β transformations$ {K_\alpha } $ $ {\alpha _1} $ $ {\alpha _2} $ $ {\alpha _3} $ $ {\alpha _4} $ $ {\alpha _5} $ $ {K_\beta } $ ${\,\beta _1}$ ${\,\beta _2}$ ${\,\beta _3}$ ${\,\beta _4}$ ${\,\beta _5}$ 51.069 4.142 −0.306 15.464 1.464 0.011 0.004 20.047 0.837 81.889 −1.519 1.192 4. 本构模型适用性分析
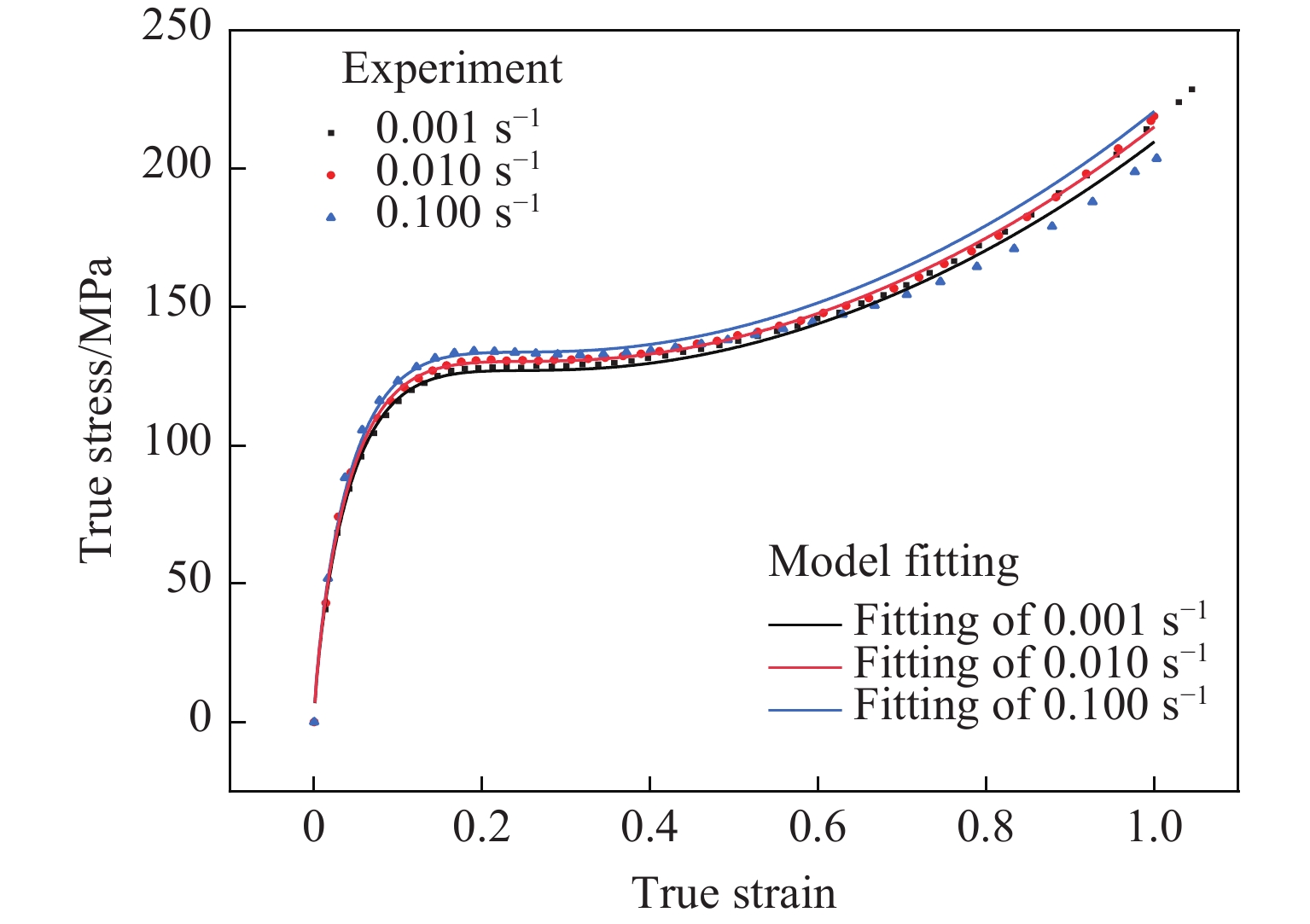
聚酰亚胺在不同应变率下的应力-应变曲线的模型计算结果与实验数据的比较情况如图12、图13所示,可见建立的本构模型与实验数据吻合较好。该模型能够较准确地描述材料的非线性响应,也能较准确地反映材料的应变率相关的力学特性。在低应变率下(0.001~0.100 s−1),可预测到初始黏弹性、屈服、应变软化和应变硬化现象;在3388、5195、6137 s−1应变率下,可准确反映稳定的塑性流动现象和消失的应变软化现象;在7626、8496、10371 s−1应变率下,仍能反映出一定的应变软化现象。
表5列出了应变率在0.001~10371 s−1范围内的预测屈服强度
$\text{ }{\sigma }_{\text{y, pred}}$ 、实验屈服强度$\text{ }{\sigma }_{\text{y, exp}}$ 和预测误差。可以看出,预测误差整体较小,说明该模型能够准确地预测聚酰亚胺在低应变率和高应变率下的屈服强度。表 5 预测屈服强度与实验屈服强度之间的误差Table 5. Error between the predicted yield stresses and the experimental resultsStrain rate/s−1 $\text{ }{\sigma }_{\text{y, exp} }$/MPa $\text{ }{\sigma }_{\text{y, pred} }$/MPa Error/% 0.001 128.55 126.96 −1.23 0.010 130.89 130.29 −0.45 0.100 134.16 133.62 −0.40 3388 155.84 161.92 3.90 5195 172.32 171.81 −0.29 6137 177.28 176.71 −0.32 7627 185.43 181.14 −2.31 8496 190.08 191.45 0.72 10371 197.88 217.53 9.93 5. 结 论
(1) 聚酰亚胺在动静态载荷下具有较好的抵抗变形能力。准静态压缩条件下,随着加载速率的增加,破坏程度增加,宏观破坏后不可恢复变形增加,回弹量减少。动态压缩下,聚酰亚胺在大变形范围内不易发生宏观破坏,延展性高。
(2) 在准静态加载条件下,聚酰亚胺的应力-应变曲线中存在明显的非线性黏弹性段、屈服、软化阶段和应变硬化;在动态加载中,软化阶段消失,取而代之的是稳定的塑性流动段,在应变率达到7627 s−1之后,会在屈服后重新出现软化现象。
(3) 在动态加载条件下,聚酰亚胺具有明显的应变率效应,屈服强度随应变率的增加而明显增加。屈服强度的动态增强因子有明显的双线性特点,这种现象可能与聚合物材料在不同温度、不同应变率下存在玻璃态(
$\alpha $ )转变和次级转变($\,\beta $ )转变有关,多元线性方程和Cowper-Symonds模型均可以描述这种现象。(4) 基于聚酰亚胺在动静态载荷下的不同力学响应,对已有的黏弹性唯象本构模型进行了改型,提出了一个考虑
$\,\beta $ 转变的非线性本构模型。该模型在低应变率下只受$\alpha $ 转变的相关参数影响;而在高应变率下$\,\beta $ 转变被激活,$\,\beta $ 转变的相关参数和$\alpha $ 转变的相关参数共同影响聚酰亚胺的力学行为。通过贝叶斯方法预测的材料模型参数可以较准确地描述聚酰亚胺在宽应变率下的力学行为。 -
表 1 准静态压缩实验后回收试样的形貌
Table 1. Morphology of the recovered samples after quasi-static experiment
No. Strain rate/s−1 Specimens after quasi-static compression Size/(mm×mm×mm) Crack 1 0.001 
6.02×5.83×2.78 No 2 0.001 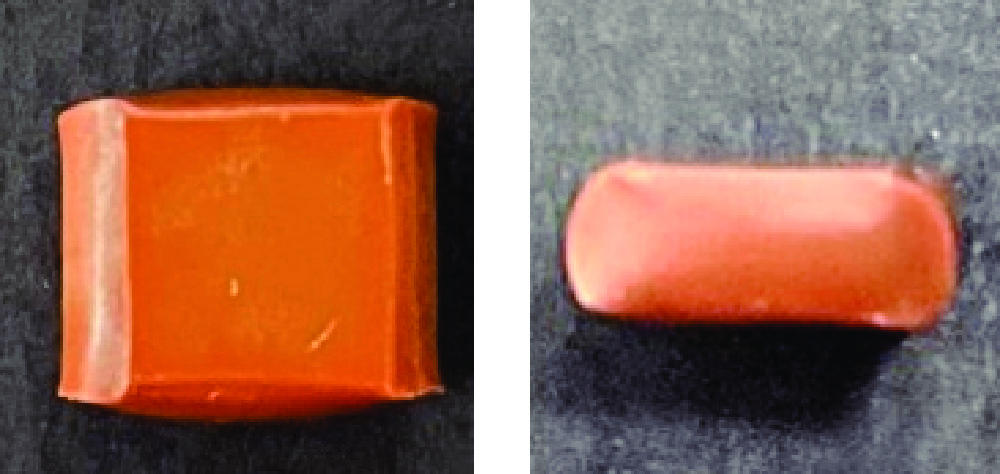
6.15×5.72×2.74 No 3 0.001 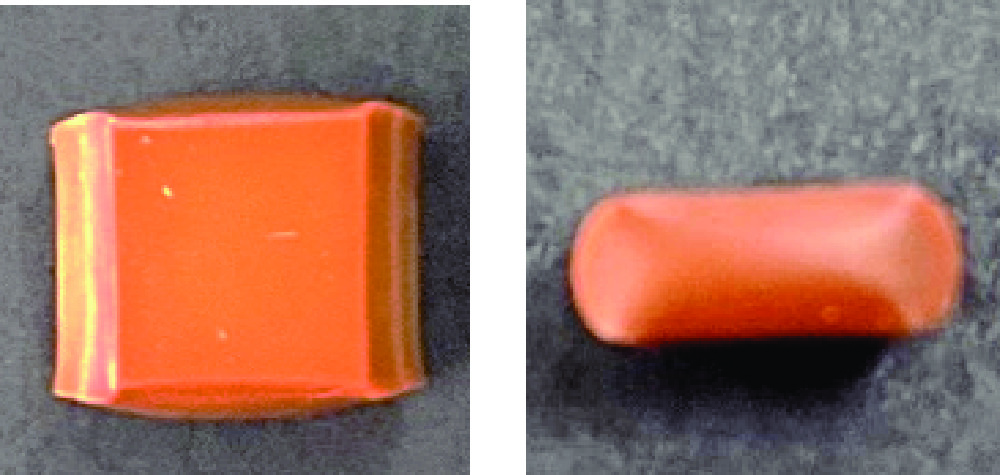
6.18×5.80×2.75 No 4 0.010 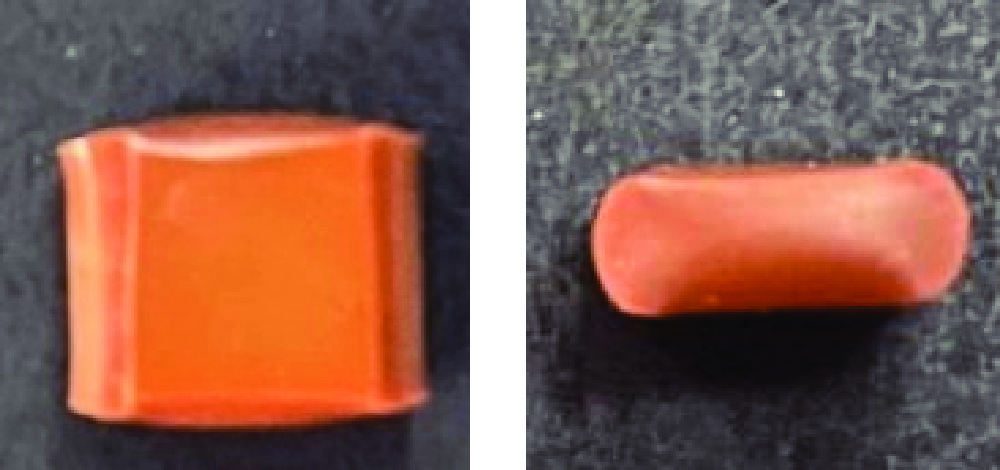
6.13×5.83×2.71 No 5 0.010 
6.25×5.78×2.72 No 6 0.010 
6.46×5.70×2.72 Yes 7 0.100 
6.64×5.86×2.58 Yes 8 0.100 
6.76×5.69×2.59 Yes 9 0.100 
6.54×5.89×2.59 Yes 表 2 动态压缩后
$\varnothing $ 6.00 mm×2.00 mm试样的形貌Table 2. Morphology of the recovered
$\varnothing $ 6.00 mm×2.00 mm samples after dynamic compressionNo. Load pressure/MPa Strain rate/s−1 Size/(mm×mm) Specimens after dynamic compression Crack 1 0.05 3388 $\varnothing $6.13×1.91 
No 2 0.08 5195 $\varnothing $6.75×1.61 
No 3 0.10 6137 $\varnothing $7.08×1.48 
No 4 0.13 7627 $\varnothing $7.72×1.29 
No 5 0.17 8496 $\varnothing $8.48×1.10 
No 6 0.20 10371 $\varnothing $8.99×1.00 
No 表 3 两种模型的拟合方程与判定系数
Table 3. Two models’ fitting equations and their determination coefficients
Model Equations R2 Cowper-Symonds ${ D_{ {\text{IF} } } } = {(1 + \dot \varepsilon /3\,396)^{1/3.22} }$ 0.99067 Poly-linear fitting ${ D_{ {\text{IF} } } } = \left\{ 1.065˙ε0.0093 ˙ε⩽0.100 s−10.275˙ε0.1855 ˙ε⩾3388 s−1 \right.$0.99206
0.98288表 4 α和β转变相关的材料参数
Table 4. Material parameters related to the α and β transformations
$ {K_\alpha } $ $ {\alpha _1} $ $ {\alpha _2} $ $ {\alpha _3} $ $ {\alpha _4} $ $ {\alpha _5} $ $ {K_\beta } $ ${\,\beta _1}$ ${\,\beta _2}$ ${\,\beta _3}$ ${\,\beta _4}$ ${\,\beta _5}$ 51.069 4.142 −0.306 15.464 1.464 0.011 0.004 20.047 0.837 81.889 −1.519 1.192 表 5 预测屈服强度与实验屈服强度之间的误差
Table 5. Error between the predicted yield stresses and the experimental results
Strain rate/s−1 $\text{ }{\sigma }_{\text{y, exp} }$/MPa $\text{ }{\sigma }_{\text{y, pred} }$/MPa Error/% 0.001 128.55 126.96 −1.23 0.010 130.89 130.29 −0.45 0.100 134.16 133.62 −0.40 3388 155.84 161.92 3.90 5195 172.32 171.81 −0.29 6137 177.28 176.71 −0.32 7627 185.43 181.14 −2.31 8496 190.08 191.45 0.72 10371 197.88 217.53 9.93 -
[1] 李敏, 张佐光, 仲伟虹, 等. 聚酰亚胺树脂研究与应用进展 [J]. 复合材料学报, 2000, 17(4): 48–53. doi: 10.3321/j.issn:1000-3851.2000.04.010LI M, ZHANG Z G, ZHONG W H, et al. Study and application development of polyimides [J]. Acta Materiae Compositae Sinica, 2000, 17(4): 48–53. doi: 10.3321/j.issn:1000-3851.2000.04.010 [2] 汪家铭. 聚酰亚胺薄膜技术进展与市场前景 [J]. 合成技术及应用, 2012, 27(3): 24–29. doi: 10.3969/j.issn.1006-334X.2012.03.011WANG J M. Technology advances and market prospects of polyimide film [J]. Synthetic Technology and Application, 2012, 27(3): 24–29. doi: 10.3969/j.issn.1006-334X.2012.03.011 [3] 楚晖娟, 朱宝库, 徐又一. 聚酰亚胺泡沫材料在航空航天飞行器中应用进展 [J]. 宇航材料工艺, 2006, 36(3): 1–3. doi: 10.3969/j.issn.1007-2330.2006.03.001CHU H J, ZHU B K, XU Y Y. Application of polyimide foam materials in aerospace vehicles [J]. Aerospace Materials & Technology, 2006, 36(3): 1–3. doi: 10.3969/j.issn.1007-2330.2006.03.001 [4] 徐立志, 高光发, 赵真, 等. 不同应变率下聚乙烯材料的压缩力学性能 [J]. 爆炸与冲击, 2019, 39(1): 013301.XU L Z, GAO G F, ZHAO Z, et al. Compressive mechanical properties of polyethylene at different strain rates [J]. Explosion and Shock Waves, 2019, 39(1): 013301. [5] WANG J, XU Y J, ZHANG W H. Finite element simulation of PMMA aircraft windshield against bird strike by using a rate and temperature dependent nonlinear viscoelastic constitutive model [J]. Composite Structures, 2014, 108: 21–30. doi: 10.1016/j.compstruct.2013.09.001 [6] 张龙辉, 张晓晴, 姚小虎, 等. 高应变率下航空透明聚氨酯的动态本构模型 [J]. 爆炸与冲击, 2015, 35(1): 51–56. doi: 10.11883/1001-1455(2015)01-0051-06ZHANG L H, ZHANG X Q, YAO X H, et al. Constitutive model of transparent aviation polyurethane at high strain rates [J]. Explosion and Shock Waves, 2015, 35(1): 51–56. doi: 10.11883/1001-1455(2015)01-0051-06 [7] ROLAND C M, TWIGG J N, VU Y, et al. High strain rate mechanical behavior of polyurea [J]. Polymer, 2007, 48(2): 574–578. doi: 10.1016/j.polymer.2006.11.051 [8] 胡文军, 张方举, 田常津, 等. 聚碳酸酯的动态应力应变响应和屈服行为 [J]. 材料研究学报, 2007, 21(4): 439–443. doi: 10.3321/j.issn:1005-3093.2007.04.019HU W J, ZHANG F J, TIAN C J, et al. Dynamic stress-strain response and yield behavior of polycarbonate [J]. Chinese Journal of Materials Research, 2007, 21(4): 439–443. doi: 10.3321/j.issn:1005-3093.2007.04.019 [9] CHOU S C, ROBERTSON K D, RAINEY J H. The effect of strain rate and heat developed during deformation on the stress-strain curve of plastics [J]. Experimental Mechanics, 1973, 13(10): 422–432. doi: 10.1007/BF02324886 [10] WALLEY S M, FIELD J E. Strain rate sensitivity of polymers in compression from low to high rates [J]. DYMAT Journal, 1994, 1(3): 211–227. [11] GOGLIO L, PERONI L, PERONI M, et al. High strain-rate compression and tension behaviour of an epoxy bi-component adhesive [J]. International Journal of Adhesion and Adhesives, 2008, 28(7): 329–339. doi: 10.1016/j.ijadhadh.2007.08.004 [12] 于鹏, 姚小虎, 张晓晴, 等. 聚碳酸酯类非晶聚合物力学性能及其本构关系 [J]. 力学进展, 2016, 46(1): 201603. doi: 10.6052/1000-0992-15-016YU P, YAO X H, ZHANG X Q, et al. Mechanical behaviors and constitutive models of polycarbonate amorphous polymers [J]. Advances in Mechanics, 2016, 46(1): 201603. doi: 10.6052/1000-0992-15-016 [13] 陈春晓, 彭刚, 冯家臣, 等. 聚甲醛动态力学性能及本构行为研究 [J]. 塑料工业, 2018, 46(2): 137–139, 53. doi: 10.3969/j.issn.1005-5770.2018.02.031CHEN C X, PENG G, FENG J C, et al. The research of dynamic mechanical properties and constitutive behavior of POM [J]. China Plastics Industry, 2018, 46(2): 137–139, 53. doi: 10.3969/j.issn.1005-5770.2018.02.031 [14] WANG H T, ZHANG Y, HUANG Z G, et al. Experimental and modeling study of the compressive behavior of PC/ABS at low, moderate and high strain rates [J]. Polymer Testing, 2016, 56: 115–123. doi: 10.1016/j.polymertesting.2016.09.027 [15] WANG H T, ZHOU H M, HUANG Z G, et al. Constitutive modeling of polycarbonate over a wide range of strain rates and temperatures [J]. Mechanics of Time-Dependent Materials, 2017, 21(1): 97–117. doi: 10.1007/s11043-016-9320-1 [16] 王海涛. 聚合物大变形及断裂行为的建模与模拟 [D]. 武汉: 华中科技大学, 2017.WANG H T. Modeling and simulation of the large deformation and fracture behavior of polymers [D]. Wuhan: Huazhong University of Science and Technology, 2017. [17] 宋力, 胡时胜. SHPB数据处理中的二波法与三波法 [J]. 爆炸与冲击, 2005, 25(4): 368–373. doi: 10.3321/j.issn:1001-1455.2005.04.014SONG L, HU S S. Two-wave and three-wave method in SHPB data processing [J]. Explosion and Shock Waves, 2005, 25(4): 368–373. doi: 10.3321/j.issn:1001-1455.2005.04.014 [18] LU F Y, LIN Y L, WANG X Y, et al. A theoretical analysis about the influence of interfacial friction in SHPB tests [J]. International Journal of Impact Engineering, 2015, 79: 95–101. doi: 10.1016/j.ijimpeng.2014.10.008 [19] BAUWENS-CROWET C, BAUWENS J C, HOMÈS G. The temperature dependence of yield of polycarbonate in uniaxial compression and tensile tests [J]. Journal of Materials Science, 1972, 7(2): 176–183. doi: 10.1007/BF02403504 [20] JONES N. Structural impact [M]. Cambridge: Cambridge University Press, 1989. [21] PANCHENKO D. Introduction to probability and statistics [M]. Cambridge: Cambridge University Press, 1980. [22] BAR-SHALOM Y, LI X R, KIRUBARAJAN T. Estimation with applications to tracking and navigation [M]. New York: Wiley, 2001. -
















 下载:
下载:






































































































































































 下载:
下载: