Fracture Characteristics of Ore Components Interface Based on Representative Volume Unit Model
-
摘要: 矿物组分的界面破碎特征一般指黏结界面在外部载荷作用下产生的应力、应变等,其对研究矿物组分解离、提高矿石破碎效率具有重要意义。针对矿石内部组分矿物聚集、有用矿物非均匀分布的特点,开展了岩石内部岩相分析实验和矿物界面原位加载实验,在此基础上,利用非线性、多尺度建模平台DIGIMAT构建符合组分矿物微观结构的代表性体积单元(RVE)模型,并通过DIGIMAT-ABAQUS耦合开展矿石RVE模型原位破碎模拟。结果表明:(1)黑钨矿石中有用矿物以颗粒状分布在矿石内部,主要分布在石英矿物内及其与硅质岩矿物黏结界面处;(2)不同组分黏结界面的力学性质存在差异,且与组成矿物的物理属性、形态特征等相关,石英-硅质岩界面的最小破碎应力(界面发生破坏时的应力)范围为1.1785~1.4826 GPa,石英-钨界面的最小破碎应力范围为1.3355~1.5420 GPa;(3)加载速率为0.010或0.005 kN/s时,矿石破碎峰值应力无明显变化,但其对矿石内部形变的影响较大,且加载速率为0.010 kN/s时,强化阶段应力易突然降低并不断波动;(4)原位载荷产生的破坏主要发生在载荷作用区域边界,且两组界面中石英矿物破碎力学性能参数大于钨矿物和硅质岩矿物,即界面组成矿物中石英矿物优先形成破坏。Abstract: The fracture characteristic of mineral component interface refers to the stress, strain and other processes generated by the bonding interface under the action of external load. It is of great significance for studying the dissociation of component minerals and improving the efficiency of ore crushing. To further study the characteristics of mineral accumulation and non-uniform distribution of useful minerals in the ore, the internal rock facies analysis and the mineral interface in-situ loading experiments were carried out. Based on these two experiments, the non-linear, multi-scale modeling platform DIGIMAT was employed to construct the coincident group. The representative volume element (RVE) model of the mineral microstructure is divided, and the in-situ crushing simulation of the ore RVE model is carried out through the DIGIMAT-ABAQUS coupling. It is suggested by these results that: (1) The useful minerals in the studied wolframite ore are distributed in granular form inside the ore, mainly in the quartz mineral and the bonding interface with the siliceous rock mineral. (2) The mechanical properties of the bonding interface with different components are related to the physical properties and morphological characteristics of the constituent minerals. The minimum fracture stress range of the quartz-siliceous rock interface is 1.1785–1.4820 GPa, and the minimum fracture stress range of the quartz-tungsten interface is 1.3355–1.5420 GPa; (3) Although the peak stress of ore crushing has no obvious effect when the loading rate is 0.010 or 0.005 kN/s, it has a greater impact on the internal deformation of the ore. When the loading rate is 0.010 kN/s, the stress suddenly decreased and constantly fluctuated during the strengthening stage. (4) The damage caused by in-situ loading mainly occurs at the boundary of the loading area. The fracture mechanical characteristics of quartz minerals in the two sets of interfaces are greater than that of tungsten minerals and siliceous rock minerals. Quartz minerals in the interface composition minerals are preferentially formed and destroyed.
-
全浸水带间隙发射作为一种新的水下发射方式[1],通过间隙燃气在膛内贴壁运动,卷吸回流后逐渐汇聚成弹前气幕,排出弹前水柱,将射弹在膛口的发射环境由水介质转化为气体介质。当气体射流流出枪口后,膛口处射流迅速膨胀成球形气体空腔,射弹穿过气体空腔与水介质接触,产生强烈的冲击载荷。水下高速射弹的弹体主要由硬铝合金尾杆和钨合金头部组成,两者镶嵌连接,连接强度有限,入水瞬间弹头会承受巨大冲击,因此射弹的入水冲击载荷成为水下射弹设计中的一个重要问题。
Karman[2]最先开始对入水冲击现象进行研究,采用动量定理并引入附加质量的概念,推导出入水冲击载荷的计算公式。Wagner[3]将Karman的方法理论化,提出了近似平板理论及自相似解法,得出了冲击压力在结构沾湿面的分布情况,使理论分析更加符合实际情况,为后来学者的理论研究奠定了基础。在国内,秦洪德等[4]、王永虎等[5]对入水冲击问题的现状和进展进行了详细的分析。宋保维等[6]基于不可压缩的非定常势流理论,建立了空投水雷入水冲击计算的数学模型。卢炽华等[7]利用不同浸深的附加质量,对刚性细长体斜姿态落水冲击进行建模,得出其入水角很小,会使弹体处在最危险的状态。王永虎等[8-9]先后对刚性尖拱体垂直姿态高速入水和斜入水的冲击理论进行了建模和仿真。魏卓慧等[10]建立了刚性截锥弹体垂直入水冲击载荷的数学模型,并对其进行了数值计算。陈诚等[11]对超空泡航行器倾斜入水冲击载荷进行了试验研究,得出了峰值时刻的阻力系数。朱珠等[12]利用商业软件FLUENT,建立了柱体回转体高速入水冲击的数值模拟模型,得到了速度对入水冲击载荷的影响规律。然而以上研究主要针对由空中入水的冲击载荷分析,对于水下入水冲击问题研究较少。本工作在此前提条件下,计算分析全水下发射高速射弹入水的冲击载荷,这对于水下发射武器研究具有一定的现实意义。
本研究拟建立锥形弹体水平及斜入水的冲击载荷理论模型,模型中考虑弹体重力、弹体浮力、附加质量、弹头锥角及入水攻角的影响,对不同头部结构参数的锥形弹体以不同入水速度入水的冲击载荷进行计算,分析入水速度、弹头锥角和入水攻角对冲击载荷的影响。研究结果对于弹体入水冲击载荷的预测及全水下发射方式发射的射弹头部结构设计具有参考价值。
1. 数学模型
1.1 射弹水平入水
假设射弹为刚体,不考虑射弹入水时空泡的影响,根据动量定理,射弹高速入水冲击时的动量方程为
(1) 式中:M为射弹的质量,m为射弹的附加质量,
对式(1)等号两边进行微分,得到射弹入水冲击时的动力学方程
(2) 式中:h为射弹侵入的距离,A为阻力面积,
射弹沿
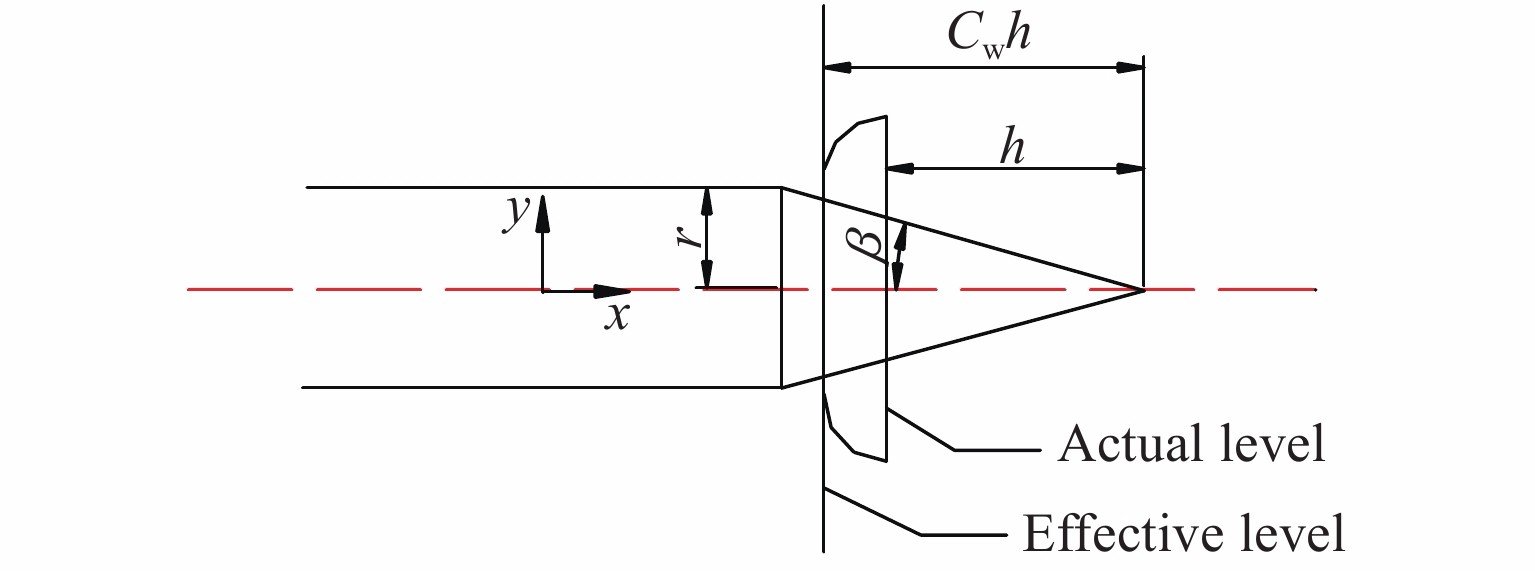
射弹入水冲击过程中,射弹浸没在水中的体积会排挤液面流体产生隆起现象,如图1所示,有效液面决定了自由水平液面的抬高程度,这取决于射弹锥头的外形和入水角等初始状态。沾湿因子定义为有效液面与实际液面的比值。
利用轴长体假设和Tayler关于在不同浸深时附加质量的表达式[13],参考垂直入水相关文献[10, 14],求出锥形射弹入水的附加质量为
(3) (4) (5) 式中:
将式(3)~式(5)代入式(2),得到总方程为
(6) 采用MATLAB软件,利用龙格-库塔方法进行求解,可以得到弹体入水时的冲击载荷。
1.2 带攻角入水
如图2所示,采用全新的发射方式时,燃气排出并在膛口形成球形气体空腔。以地面为坐标系,枪口斜向上(即
其他条件与水平入水时保持不变,攻角为正时,根据动量方程,得到动力学方程
(7) 式中:α为射弹攻角,射弹斜向上发射时为正值,斜向下时为负值。
射弹所受浮力为
(8) 当攻角为正值时,得到的总方程为
(9) 同理,攻角为负值时的总方程为
(10) 对式(9)、式(10)进行求解,可以得到不同攻角下的入水冲击载荷。
2. 计算结果与分析
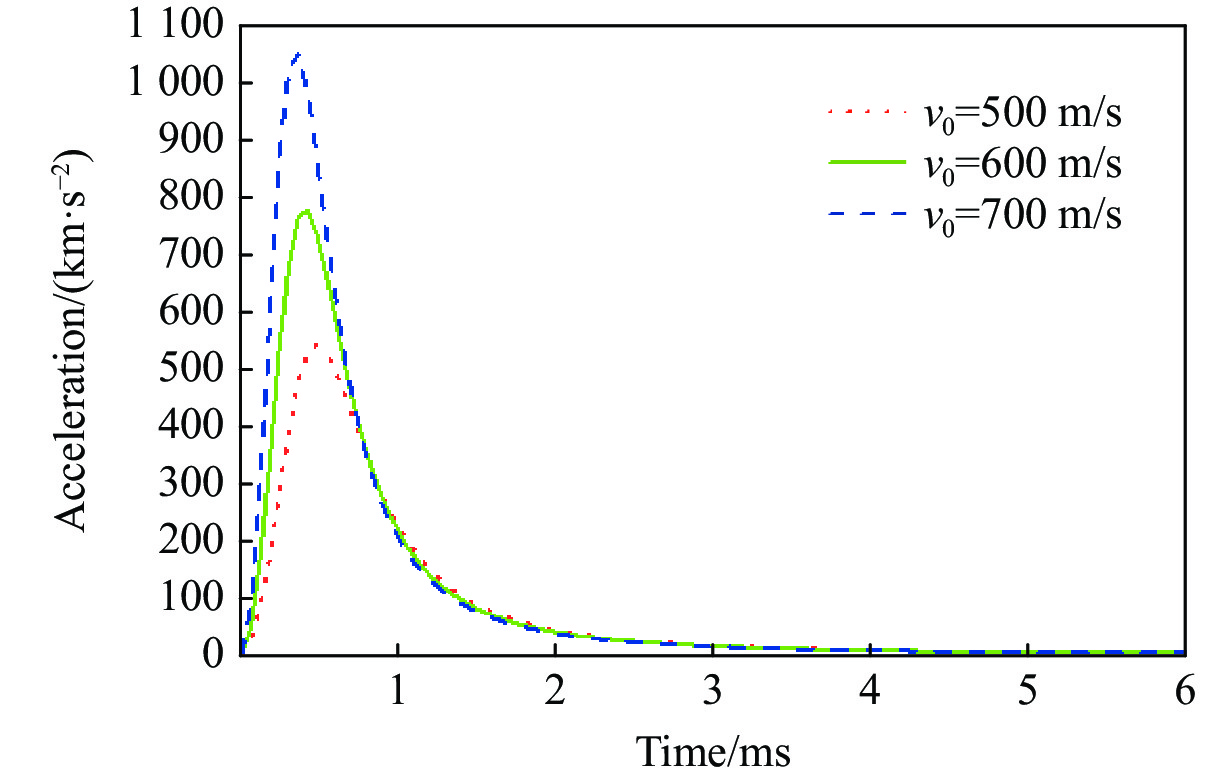
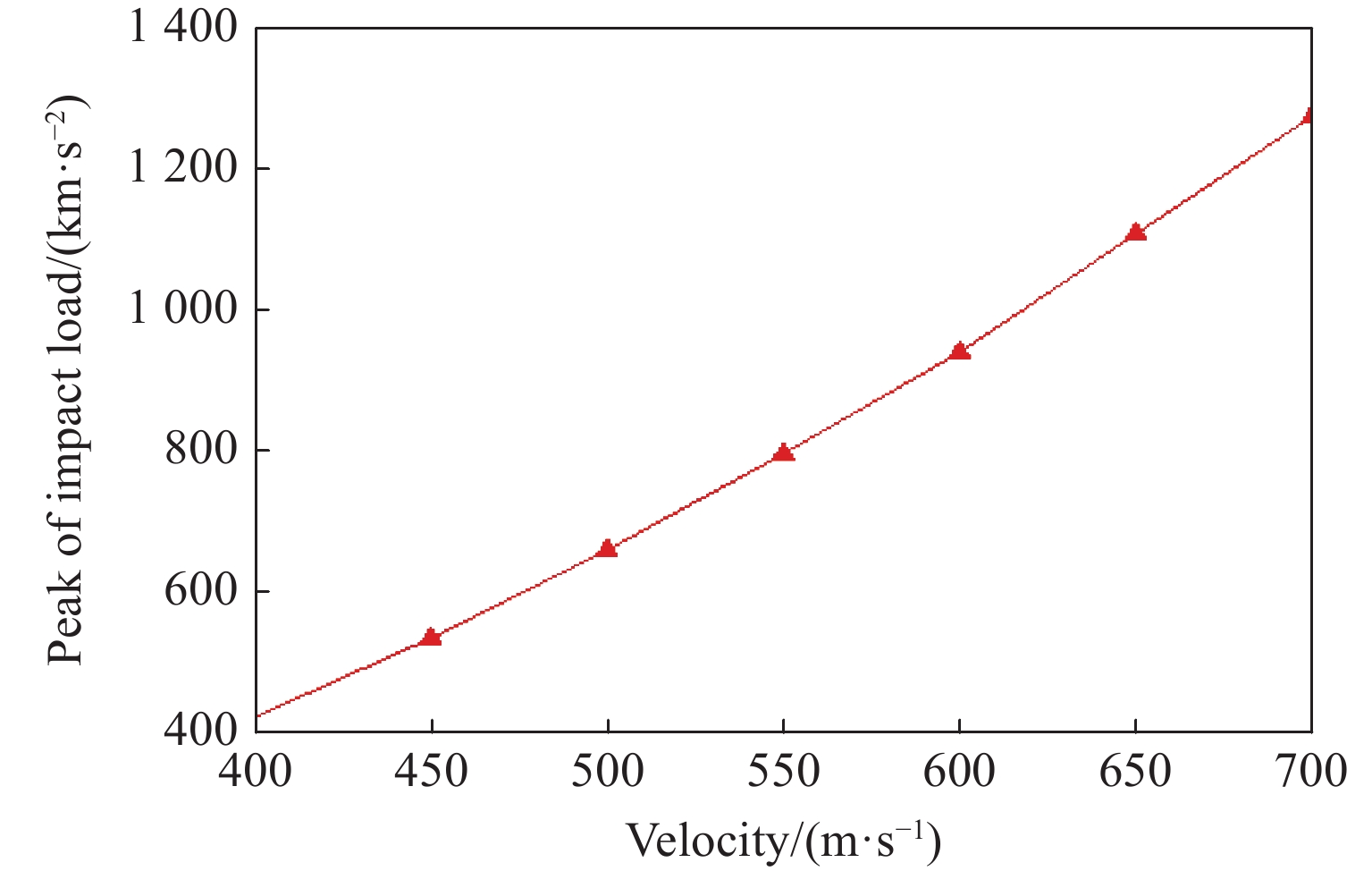
由于水下射弹的质量较轻,入水速度较大,水下超空泡射弹入水平均速度约为600 m/s,因此入水速度对射弹入水冲击载荷的影响很大。本计算中,设射弹质量为0.14 kg,射弹头部半锥角为6°,计算得到不同速度时射弹的入水冲击载荷曲线,如图3所示,其中用射弹加速度反映入水冲击载荷。可以看出:射弹的入水冲击载荷先增大后减小,载荷变化主要发生在射弹入水前1 ms,最后渐渐趋于稳定;入水速度越大,冲击载荷峰值越大,入水后达到峰值的时间越短。此外,计算了不同速度下的入水冲击载荷峰值,如图4所示。入水速度在400~700 m/s范围时,射弹入水冲击载荷峰值一般为103g量级(g为重力加速度)。从冲击载荷峰值与速度的关系可以得出入水冲击载荷的峰值与速度基本呈线性关系。
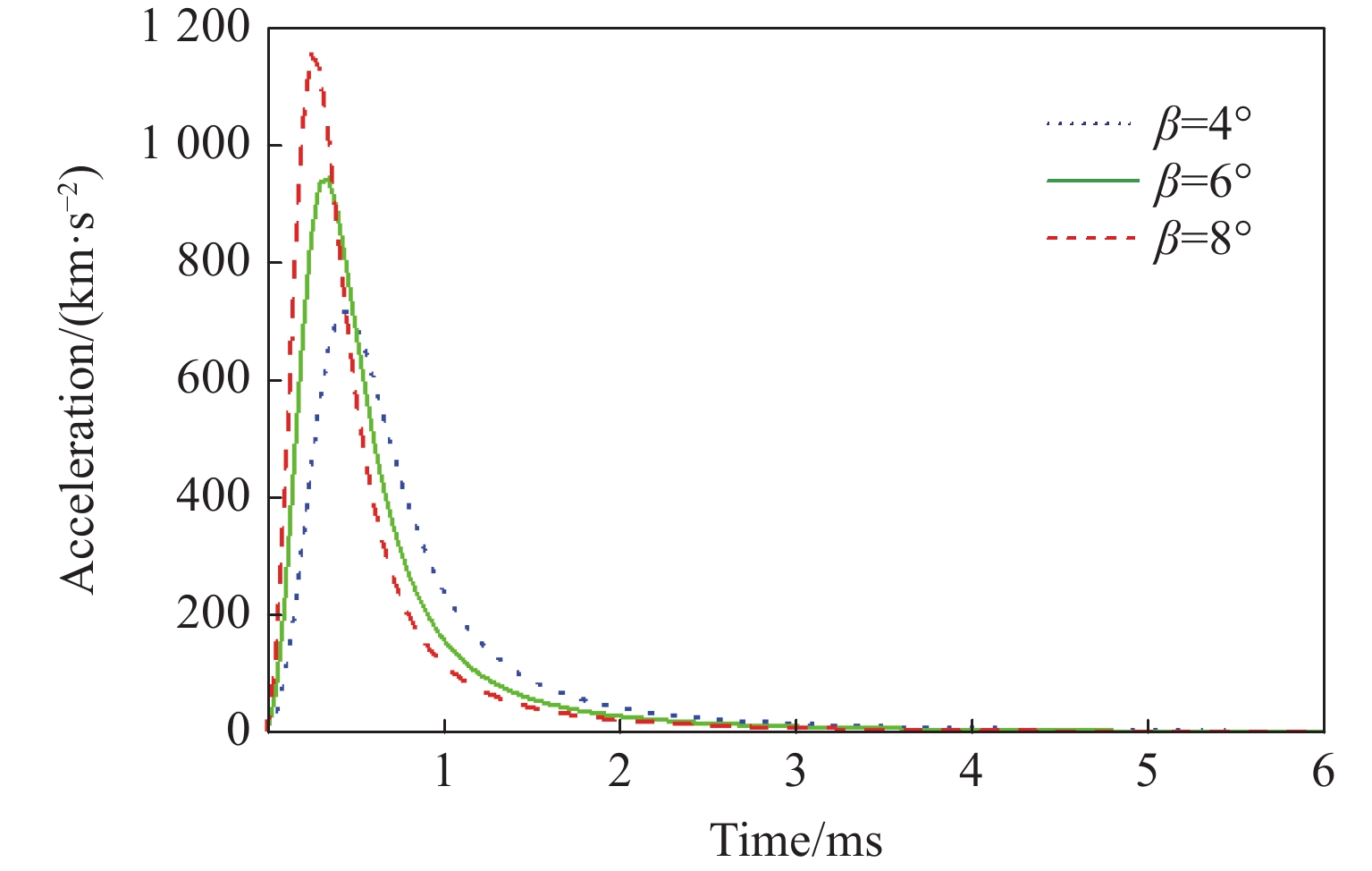
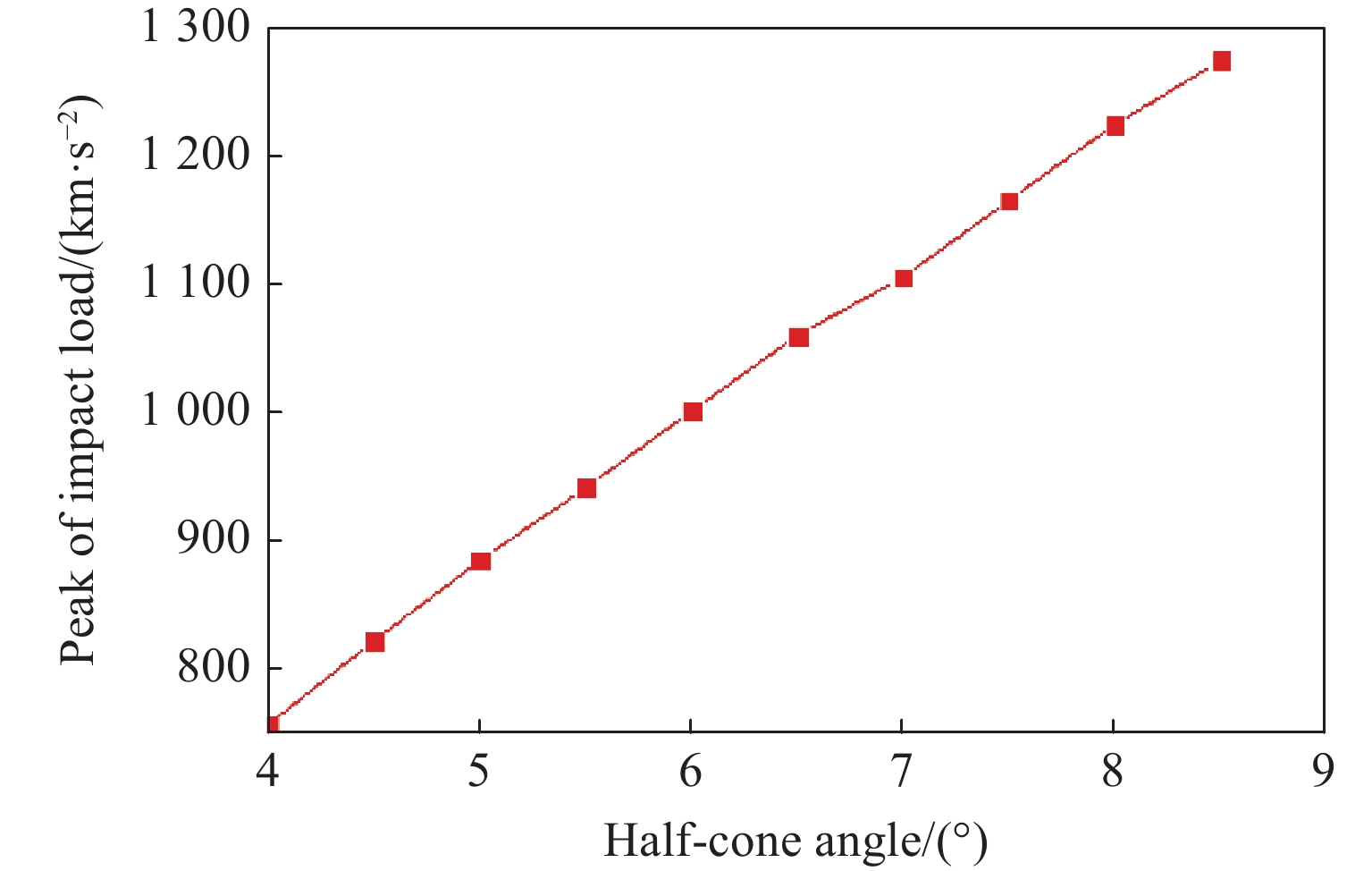
设射弹质量为0.14 kg,通过计算获得了不同锥角的锥形射弹以600 m/s入水时的冲击载荷曲线,如图5所示。可以看出:射弹的入水冲击载荷先增大后减小,最后趋于稳定;半锥角
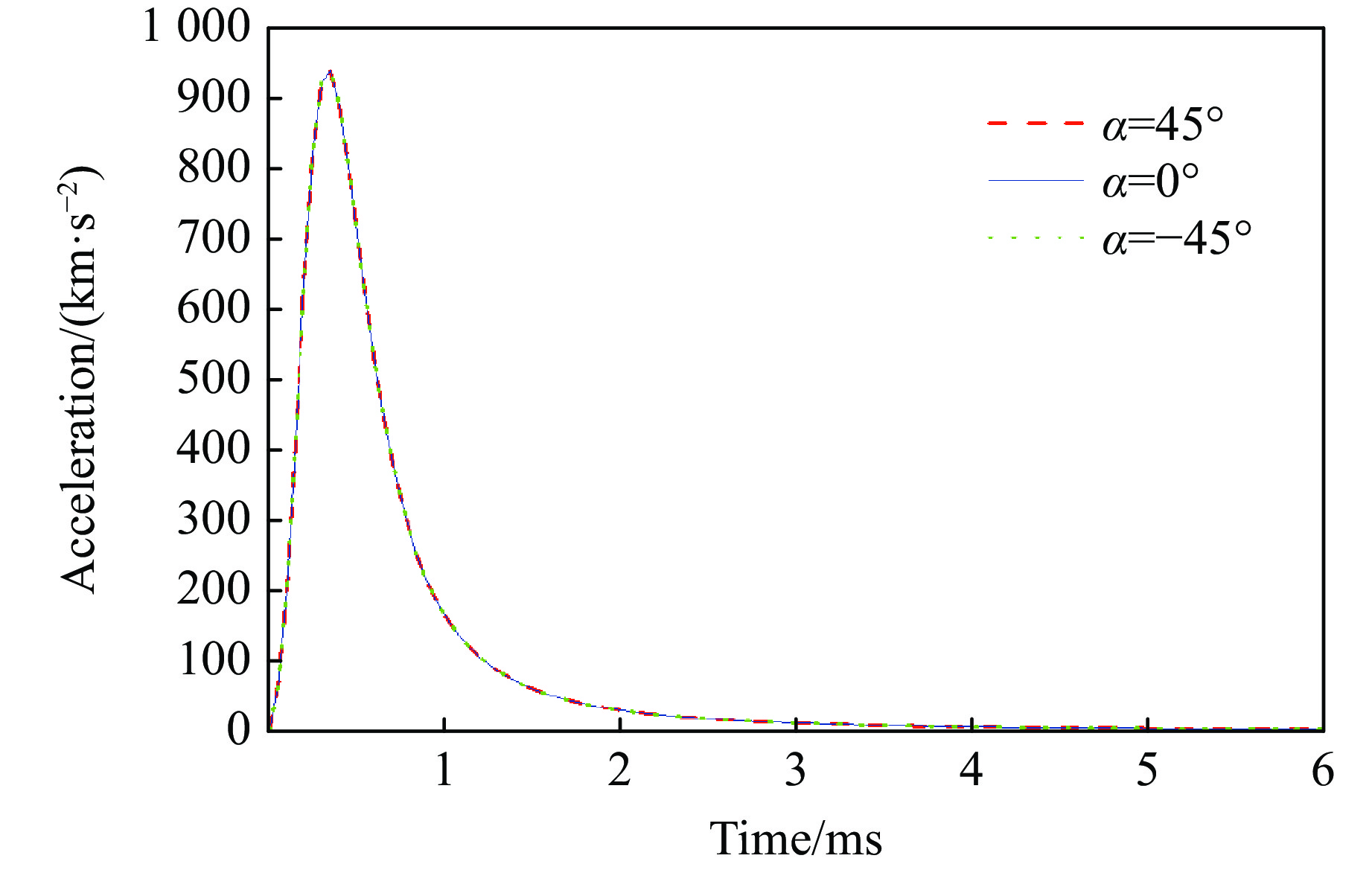
当射弹质量为0.14 kg,入水速度为600 m/s,攻角
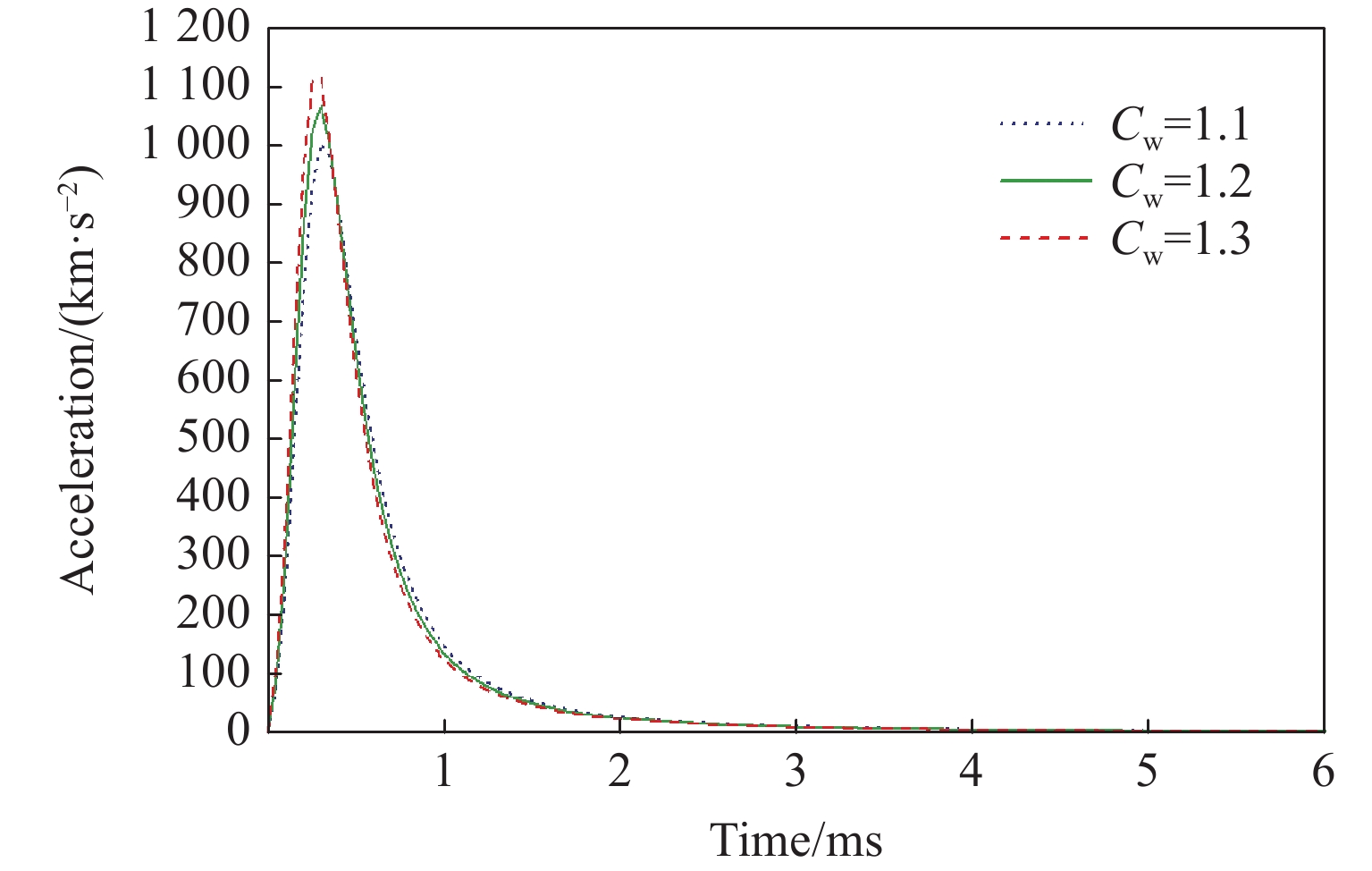
此外,射弹在高速入水状态下,从液面进入水中的实际深度与理想深度存在一定的偏差,为了表达该误差,引入沾湿因子,沾湿因子的取值对冲击载荷的结果也存在影响。沾湿因子不同时射弹的入水冲击载荷如图8所示。从图8可以看出:沾湿因子越大,入水冲击载荷峰值越大;但沾湿因子对入水冲击载荷的影响较小,当沾湿因子变化值为0.1时,入水冲击载荷的变化在10%以内。
3. 结 论
(1)射弹头部锥角相同时,入水冲击载荷峰值与速度呈正线性相关,入水速度越大,冲击载荷达到峰值的时间越短;射弹入水速度相同时,入水冲击载荷的大小与锥角呈正线性相关,锥角越大,冲击载荷达到峰值的时间越短。
(2)射弹锥角和入水速度相同、入水攻角不同时,入水冲击载荷曲线与水平入水曲线基本重合,说明射弹重力和浮力在轴向上对入水冲击载荷的影响相对入水阻力几乎可以忽略不计。当射弹带有攻角入水后,重力和浮力更多的是对射弹产生径向力矩影响。
(3)沾湿因子越大,入水冲击载荷峰值越大,但对入水冲击载荷影响较小,当沾湿因子变化为0.1时,入水冲击载荷的变化在10%以内。沾湿因子作为一个变量,其大小反过来也取决于入水冲击载荷,入水冲击载荷越大,沾湿因子也越大。
(4)理论模型借鉴了高速弹体垂直入水的理论模型,数值模拟计算结果与已报道的高速射弹垂直入水冲击载荷数值计算和仿真结果高度一致,验证了本数学建模和数值计算的准确性。
(5)探讨了全水下发射高速射弹入水瞬间的冲击载荷,高速射弹入水后形成超空泡,冲击载荷迅速减小。本工作对射弹未形成超空泡的情况进行了模型推导和数值模拟计算,对形成超空泡之前的理论研究具有重要意义。
-
表 1 钨颗粒的特征尺寸
Table 1. Characteristic sizes of different tungsten particles
W particle No. Length/mm Width/mm Perimeter/mm Area/mm2 1 1.609 1.509 6.990 1.2295 2 2.966 2.144 10.980 3.6125 3 2.645 2.233 13.930 2.8330 4 1.849 1.186 7.634 2.3356 5 0.922 0.137 2.230 0.0703 6 1.198 0.775 4.720 0.4160 7 1.047 0.622 3.442 0.6056 8 1.040 0.674 3.436 0.6587 表 2 矿物界面的最小破碎应力
Table 2. Minimum fracture stress of component mineral interface
Interface types Minimum fracture stress/GPa 1 2 3 4 5 6 7 8 Quartz-siliceous rock interface 1.4034 1.1785 1.3355 1.2732 1.2845 1.3171 1.4784 1.4826 Quartz-tungsten interface 1.5420 1.4373 1.4458 1.4671 1.3638 1.3355 1.4317 1.4571 表 3 不同黑钨矿石矿物组分的材料属性
Table 3. Mineral material properties of different wolframite components
Mineral Density/(g·cm−3) Poisson’s ratio Elastic modulus/GPa Constitutive model Structural symmetry Quartz 2.65 0.13 4 Elastoplastic Isotropic Siliceous rock 2.72 0.23 5 Elastoplastic Isotropic Tungsten 7.25 0.20 35 Elastoplastic Isotropic 表 4 内含物的形态参数
Table 4. Inclusion morphological parameters
Mineral Shape Volume fraction Aspect ratio Diameter/cm Siliceous rock Cylinder 0.50 2 Tungsten Ellipsoid 0.05 1 0.2 表 5 石英-硅质岩界面结点的应力和应变
Table 5. Quartz-siliceous rock interface junction stress and strain
Area of loading Node No. Stress/GPa Strain/10−4 Area of loading Node No. Stress/GPa Strain/10−4 Boundary 11598 1.39879 1.32 Internal 60752 1.54668 0.12 11605 1.40542 1.28 60753 1.61314 0.14 11610 1.40867 1.30 *61394 1.58947 0.26 11612 1.15526 1.06 *61396 1.21756 0.09 11613 1.69745 1.53 *61398 1.36577 0.09 60746 1.45794 1.48 *61400 1.62167 0.30 60747 1.63980 1.53 External *61390 0.24988 0.42 60748 1.65526 1.61 *61404 0.23054 0.38 60749 1.61249 1.58 11597 0.42094 0.63 60754 1.53791 1.42 11601 0.24354 0.63 *61392 1.33085 1.35 11604 0.32136 0.47 *61402 1.19414 1.29 60745 0.37922 0.62 Internal 11599 1.38796 0.47 60750 0.52646 0.87 11608 1.61647 0.54 60755 0.29399 0.55 11609 1.30032 0.25 60759 0.36765 0.69 60751 1.61276 0.26 61520 0.41291 0.72 表 6 石英-钨界面结点的应力和应变
Table 6. Quartz-tungsten interface junction stress and strain
Area of loading Node No. Stress/GPa Strain/10−4 Area of loading Node No. Stress/GPa Strain/10−4 Boundary 36367 1.54842 0.21 Internal 60830 1.96183 0.54 36369 1.58328 0.24 *61349 1.42606 1.89 36370 1.85187 0.28 *61351 1.61453 1.84 60813 1.72878 1.59 *61353 1.26790 1.46 60814 1.98327 2.18 External 60810 0.31663 0.54 60815 1.51771 1.55 60812 0.37034 0.64 60828 1.91692 1.96 60826 0.41069 0.71 60831 2.00689 2.53 60829 0.50412 0.85 60836 2.00115 2.31 61359 0.58631 1.02 *61347 0.96973 1.13 61361 0.52418 0.85 *61355 1.48255 1.90 *61333 0.57504 0.72 Internal 36366 1.59434 0.09 *61343 0.51355 0.57 60816 1.72296 0.46 *61345 0.67765 0.95 60817 2.14309 0.82 *61357 1.13598 1.54 -
[1] 张成良, 刘磊, 王超. 高等岩石力学及工程应用 [M]. 长沙: 中南大学出版社, 2016.ZHANG C L, LIU L, WANG C. Advanced rock mechanics and engineering application [M]. Changsha: Central South University Press, 2016. [2] GAUDIN A M. Principles of mineral dressing [M]. New York: McGraw-Hill, 1939: 70−89. [3] 王雅蓉, 周乐光. 相界特征对矿物单体解离度的影响 [J]. 东北大学学报(自然科学版), 1996, 17(3): 88–92.WANG Y R, ZHOU L G. Effects of interfacial characteristics on mineral liberation [J]. Journal of Northeastern University (Natural Science), 1996, 17(3): 88–92. [4] 刘建远, 应平. 颗粒床压载粉碎对某硫化铜矿石矿物解离的影响 [J]. 有色金属(选矿部分), 2018(5): 81–87. doi: 10.3969/j.issn.1671-9492.2018.05.016LIU J Y, YING P. Impact of comminution by particle-bed compressive stressing on mineral liberation of a copper sulfide ore [J]. Nonferrous Metals (Mineral Processing Section), 2018(5): 81–87. doi: 10.3969/j.issn.1671-9492.2018.05.016 [5] SUN W J, WANG L B, WANG Y Q. Mechanical properties of rock materials with related to mineralogical characteristics and grain size through experimental investigation: a comprehensive review [J]. Frontiers of Structural and Civil Engineering, 2017, 11(3): 322–328. doi: 10.1007/s11709-017-0387-9 [6] 杨小彬, 周杰, 宋义敏, 等. 循环加载岩石界面滑移位移演化特征试验研究 [J]. 煤炭学报, 2019, 44(10): 3041–3048. doi: 10.13225/j.cnki.jccs.2018.1579YANG X B, ZHOU J, SONG Y M, et al. Evolution characteristics of sliding displacement of rock interface under cyclic loading [J]. Journal of China Coal Society, 2019, 44(10): 3041–3048. doi: 10.13225/j.cnki.jccs.2018.1579 [7] ALNEASAN M, BEHNIA M, BAGHERPOUR R. Analytical investigations of interface crack growth between two dissimilar rock layers under compression and tension [J]. Engineering Geology, 2019, 259: 105188. doi: 10.1016/j.enggeo.2019.105188 [8] 蔡改贫, 赵小涛. 基于细观力学的矿石颗粒破碎特性研究 [J]. 应用力学学报, 2020, 37(4): 1792–1797. doi: 10.11776/cjam.37.04.B097CAI G P, ZHAO X T. Study on the fracture characteristics of ore particles based on micromechanics [J]. Chinese Journal of Applied Mechanics, 2020, 37(4): 1792–1797. doi: 10.11776/cjam.37.04.B097 [9] 王光勇, 余锐, 马东方, 等. 饱水细砂岩动态抗拉与抗压强度试验对比研究 [J]. 高压物理学报, 2020, 34(4): 044101. doi: 10.11858/gywlxb.20190857WANG G Y, YU R, MA D F, et al. Comparative study on dynamic tensile and compressive strength of the saturated fine sandstone [J]. Chinese Journal of High Pressure Physics, 2020, 34(4): 044101. doi: 10.11858/gywlxb.20190857 [10] 纪杰杰, 李洪涛, 吴发名, 等. 冲击荷载作用下岩石破碎分形特征 [J]. 振动与冲击, 2020, 39(13): 176–183, 214. doi: 10.13465/j.cnki.jvs.2020.13.026JI J J, LI H T, WU F M, et al. Fractal characteristics of rock fragmentation under impact load [J]. Journal of Vibration and Shock, 2020, 39(13): 176–183, 214. doi: 10.13465/j.cnki.jvs.2020.13.026 [11] 蔡改贫, 宣律伟, 张雪涛, 等. 多尺度内聚颗粒模型破碎过程研究 [J]. 岩土力学, 2020, 41(6): 1809–1817. doi: 10.16285/j.rsm.2019.1218CAI G P, XUAN L W, ZHANG X T, et al. Investigation into the crushing process in multi-scale cohesive particle model [J]. Rock and Soil Mechanics, 2020, 41(6): 1809–1817. doi: 10.16285/j.rsm.2019.1218 -







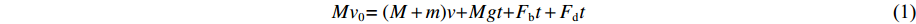
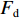
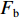
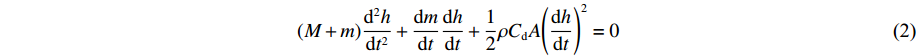
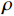

 下载:
下载:
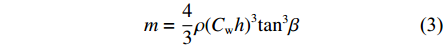
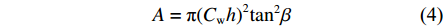
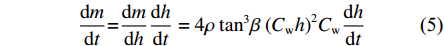
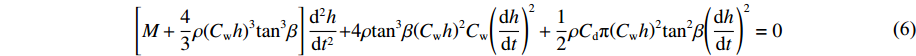

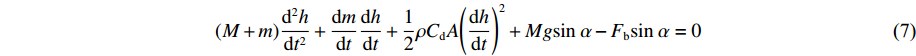
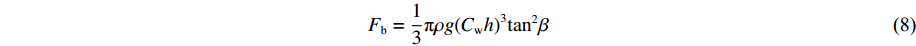
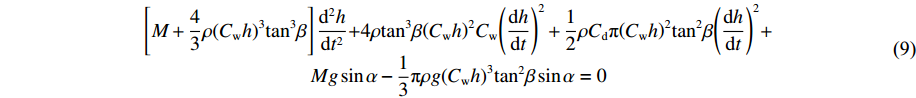
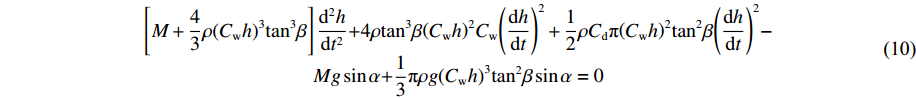







 下载:
下载: