Simulation of Dynamic Crack Propagation in Superconducting Nb3Sn at Extreme Low Temperature
-
摘要: 研究Nb3Sn超导体的损伤断裂行为对于揭示超导临界性能弱化背后的力学机制具有重要的意义。采用分子动力学模拟方法，研究了极低温下不含裂纹和含中心裂纹的Nb3Sn单晶在力学拉伸变形作用下的断裂机制和裂纹扩展行为，同时分析了应变率效应对Nb3Sn单晶断裂机制与裂纹扩展行为的影响。结果表明：不含裂纹的Nb3Sn单晶在结构受力后出现滑移，滑移带上位错塞积导致应力集中，应力集中使原子键断裂从而萌生裂纹致使Nb3Sn单晶断裂；而含中心裂纹的Nb3Sn单晶则由于裂纹尖端应力集中使得原子键断裂形成微裂纹，裂纹扩展致使Nb3Sn单晶断裂。Nb3Sn单晶在不同的应变率下表现出不同的断裂机制，在低应变率下表现为脆性断裂，而在高应变率下表现为韧性断裂。Abstract: The study on damage and fracture in superconducting Nb3Sn is an indispensible part of understanding the origin of strain sensitivity in Nb3Sn. In this paper, by using molecular dynamic simulations, the fracture and crack propagation behavior of single crystal Nb3Sn with ideal lattice and with central crack at extreme low temperature are studied, respectively. The strain rate effects on the crack initiation and growth in both Nb3Sn sample cases are also carefully analyzed. The results show that for stressed Nb3Sn single crystal with ideal lattice, it slips with the dislocations plugging emerging on the slip band, which contributes to stress concentration and atomic bond breaking, resulting in the failure of Nb3Sn. While for Nb3Sn single crystal with central crack, the atomic bonds break due to the stress concentration concurring at the crack tip, microcracks form and propagate to induce the Nb3Sn fracture. The analysis on the damage fracture and failure mechanism of single crystal Nb3Sn at different strain rates reveals that it shows brittle fracture at low strain rate and ductile fracture at high strain rate.
-
Key words:
- Nb3Sn single crystal /
- molecular dynamic simulation /
- crack propagation /
- fracture
-
具有钙钛矿结构的稀土-3d过渡金属氧化物RMO3（R为稀土，M为3d过渡金属）因表现出诸如多铁性[1–3]、高温超导[4]、巨磁阻效应[5]等奇异的物性而一直备受关注。从晶体结构来看，3d过渡金属阳离子M与氧离子形成共角连接的MO6八面体，稀土阳离子R位于MO6八面体的空隙中间。这种结构不仅可以容纳多种不同离子半径的稀土和过渡金属阳离子，造成MO6八面体的扭转和倾斜，而且还允许氧离子空位存在有序或无序排列。从电子结构来看，随着过渡金属阳离子3d价电子和稀土阳离子4f价电子的排布变化，考虑到电子间的强关联库仑相互作用，系统的轨道序和磁基态将发生显著改变。因此，稀土-3d过渡金属钙钛矿中存在结构、电荷、轨道和自旋多个量子自由度之间的相互耦合和竞争，为研究结构与物性的构效关系提供了绝佳平台。
在钙钛矿结构中，用几何结构容忍因子
$ t\equiv d_{\mathrm{R}-\mathrm{O}}\big/\left(\sqrt{2}d_{\mathrm{M}-\mathrm{O}}\right) $ 描述结构的晶格畸变，其中$ d_{\mathrm{R}-\mathrm{O}}$ 和$ d_{\mathrm{M}-\mathrm{O}}$ 分别表示稀土阳离子和过渡金属阳离子与配位氧离子之间的键长。实验发现，稀土-3d过渡金属氧化物RMO3的钙钛矿结构通常在$ 1 - \delta \leqslant t \leqslant 1 $ 时保持稳定[6]。在高压作用下，体系将倾向于对称性更高的结构，从而减小晶格体积、增大物质密度。一方面，对于$ t < 1 - \delta $ 的低对称非钙钛矿结构，其八面体在高压下扭转或畸变减弱，将导致晶格对称性提高，进而转变为钙钛矿结构；另一方面，对于$ {t} > 1 $ 的类钙钛矿共面变形体，由于在压力作用下R―O键比M―O键更易压缩，使得$ t $ 因子在高压下逐渐减小并趋近于1，最终成为钙钛矿结构。因此，高压可极大拓宽钙钛矿结构稳定存在的范围，在合成新型钙钛矿材料方面具有独特优势。然而，过去关于稀土-3d过渡金属钙钛矿RMO3的研究主要集中在过渡金属钒基[7–9]、铬基[10–12]、锰基[3, 13–14]、铁基[15–17]、钴基[18–20]和镍基[21–23]体系，对铜基稀土钙钛矿RCuO3的探索非常缺乏。其中一个主要原因是，含有反常高价态Cu3+的钙钛矿RCuO3需要在超高氧压下合成，使得该体系的样品制备异常困难。虽然LaCuO3可在5.0 GPa高压下合成[24]且已被广泛研究，但是随着稀土离子R半径的进一步减小，RCuO3钙钛矿结构的几何容忍因子t变得更小，因此需要更高压力条件才能合成。Zhou等[25]在1600 ℃、6.5 GPa的高温高压条件下合成了一系列La1–xNdxCuO3（0≤x≤0.6）固溶体，并系统研究了该体系的结构和物性演化规律，其中LaCuO3表现出金属性和增强的泡利顺磁性。Chen等[26]在15.0 GPa高压下合成出正交结构的钙钛矿NdCuO3样品，样品呈现绝缘体行为，但尚未系统研究其物性。以上结果表明，随着Nd3+的掺杂浓度提高，La1–xNdxCuO3（0.7≤x≤1）需要更高的合成压力，同时由于其σ*带逐渐变窄，将成为Cu3+单价钙钛矿体系中研究电子从巡游向局域渡越的关键材料。
为此，本研究将利用自主设计的高压组件，在Walker型二级推进压机上探索在最佳压力和温度条件下合成新型铜基稀土钙钛矿La1–xNdxCuO3（0≤x≤1）样品，并通过精修X射线衍射谱图获得详细的结构相图，为进一步研究压力对其晶格结构和电子结构的调控规律提供实验依据。
1. 实验技术
1.1 前驱体制备
将La2O3、Nd2O3 （Macklin公司，纯度99.9%）在900 ℃条件下预烧12 h，去除水分和附着气体；将预烧过的La2O3、Nd2O3和CuO粉末（Aladdin公司，纯度99.9%）按照La1–xNdxCuO3（0≤x≤1）的化学计量比混合均匀，研磨30 min以上；将混合粉末放入坩埚中，在马弗炉上于1000 ℃烧结20 h，获得La1–xNdxCuO3（0≤x≤0.4）样品的前驱体；将研磨均匀的原料放入球磨机，以55 Hz频率高速球磨12 h，获得La1–xNdxCuO3（0.5≤x≤1）样品的前驱体。高能球磨可以制造纳米级微观结构，使原料具有更小的粒径和更窄的粒度分布，促进其在非常规条件下发生反应[27–28]，从而降低制备高浓度掺杂Nd3+样品的反应压力和温度条件。
1.2 高压高温制备
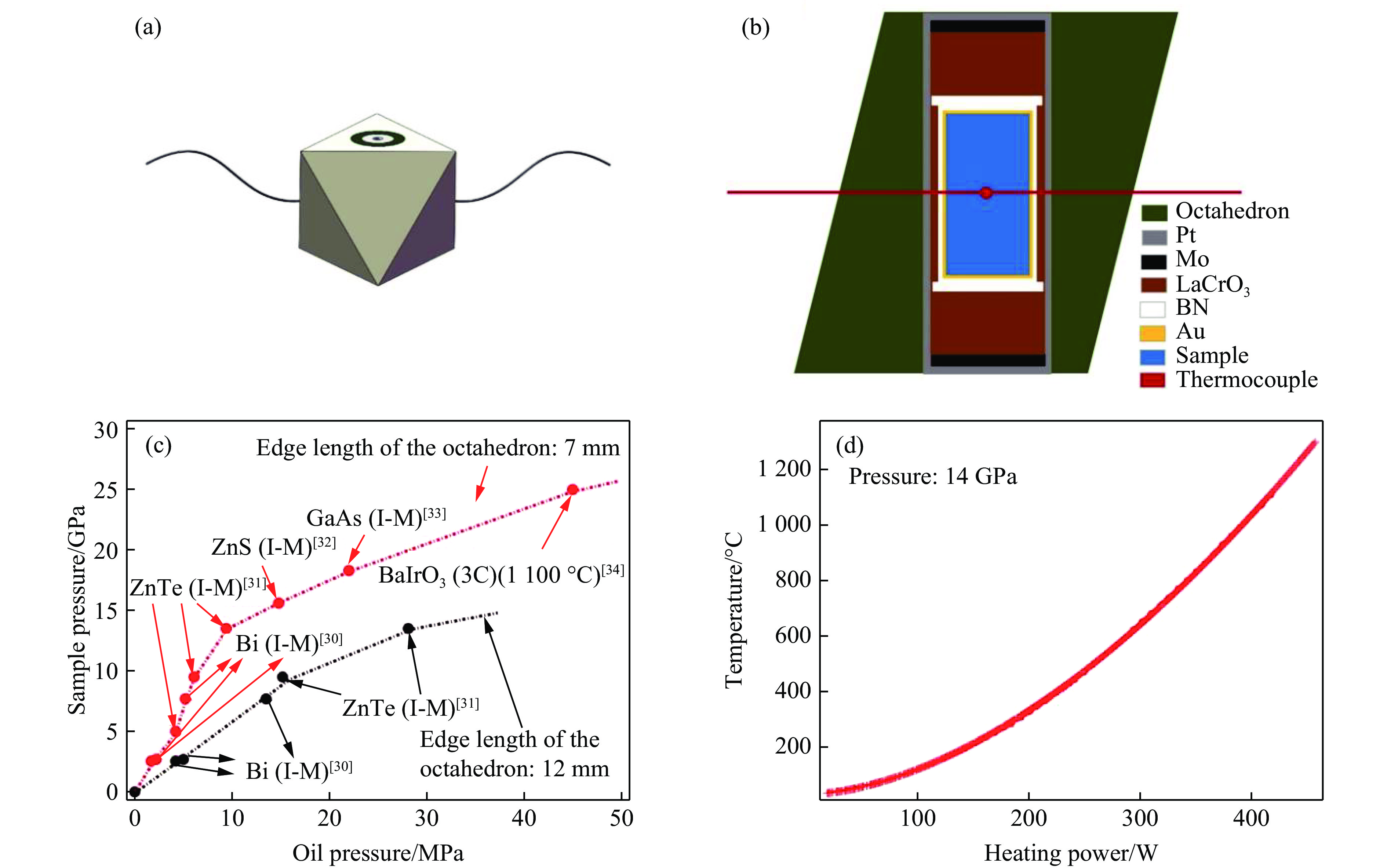
如图1所示，本研究通过在高温下分解KClO4产生氧气并封装在密闭金筒中提供高氧压环境[29]，利用Walker型二级推进压机在高温高压条件下合成高质量的La1–xNdxCuO3（0≤x≤1）样品。
图 1 (a) 高压合成组件侧面图；(b) 高压合成组件剖面图；(c)高压合成组件的校压曲线；(d) 高压合成组件在14 GPa下的标温曲线Figure 1. (a) Side view of the high-pressure assembly; (b) sectional drawing of the high-pressure assembly; (c) pressure calibration of high-pressure assembly; (d) temperature calibration of high-pressure assembly under 14 GPa首先，自主设计加工了Walker型高压组件，通过建立组件尺寸、强度与压力的关系模型，优化其尺寸精度、材料强度和受力结构。通过在压机中对特定的压力标定材料加压，测量其电阻随外部油压的变化曲线[30–34]，获得样品压强与外部油压的对应关系。每套组件由传压介质八面体（主要成分为氧化镁）、铬酸镧、氮化硼、铂筒、金箔、钼片和热电偶组成，其中热电偶放置在样品中心处，用来标定样品中心的反应温度。通过压力和温度校准，该高压组件可实现的最高压强和温度分别达到25 GPa和1300 ℃。
在此基础上，将样品的前驱体粉末冷压成圆柱体置于金箔筒中，并在样品两端装填近似等量的KClO4粉末，KClO4粉末与前驱体粉末的体积比约为1∶2，保证加热过程中释放过量的氧气与样品充分反应减少氧空位。合成La1–xNdxCuO3（0≤x≤1）样品所需的优化压力和温度条件见表1。实验结果表明，随着Nd3+离子掺杂浓度的提高，La1–xNdxCuO3（0.7≤x≤1）需要更高的合成压力。
表 1 La1–xNdxCuO3 （0≤x≤1）样品合成的压力和温度优化条件Table 1. Pressure and temperature conditions of synthesizing La1–xNdxCuO3 (0≤x≤1)Sample Pressure/GPa Temperature/℃ Time/min LaCuO3 6 1000 30 La0.9Nd0.1CuO3 6 1000 30 La0.8Nd0.2CuO3 6 1000 30 La0.7Nd0.3CuO3 10 1000 30 La0.6Nd0.4CuO3 10 1000 30 La0.5Nd0.5CuO3 10 1000 30 La0.4Nd0.6CuO3 10 1000 30 La0.3Nd0.7CuO3 14 1000 30 La0.2Nd0.8CuO3 14 1000 30 La0.1Nd0.9CuO3 14 1000 30 NdCuO3 14 1000 30 1.3 样品表征与精修
将合成的样品研磨30 min，用去离子水清洗3遍以上去除KCl杂质，利用Bruker D8 Advance X射线粉末衍射仪（PXRD，X射线波长λ=1.54056 Å）在衍射角度为20°～100°、步长为0.02°条件下测量PXRD数据，通过Fullprof 软件对La1–xNdxCuO3（0≤x≤1）的PXRD谱图进行Rietveld精修，获取晶体结构信息[35]。
2. 实验结果和讨论
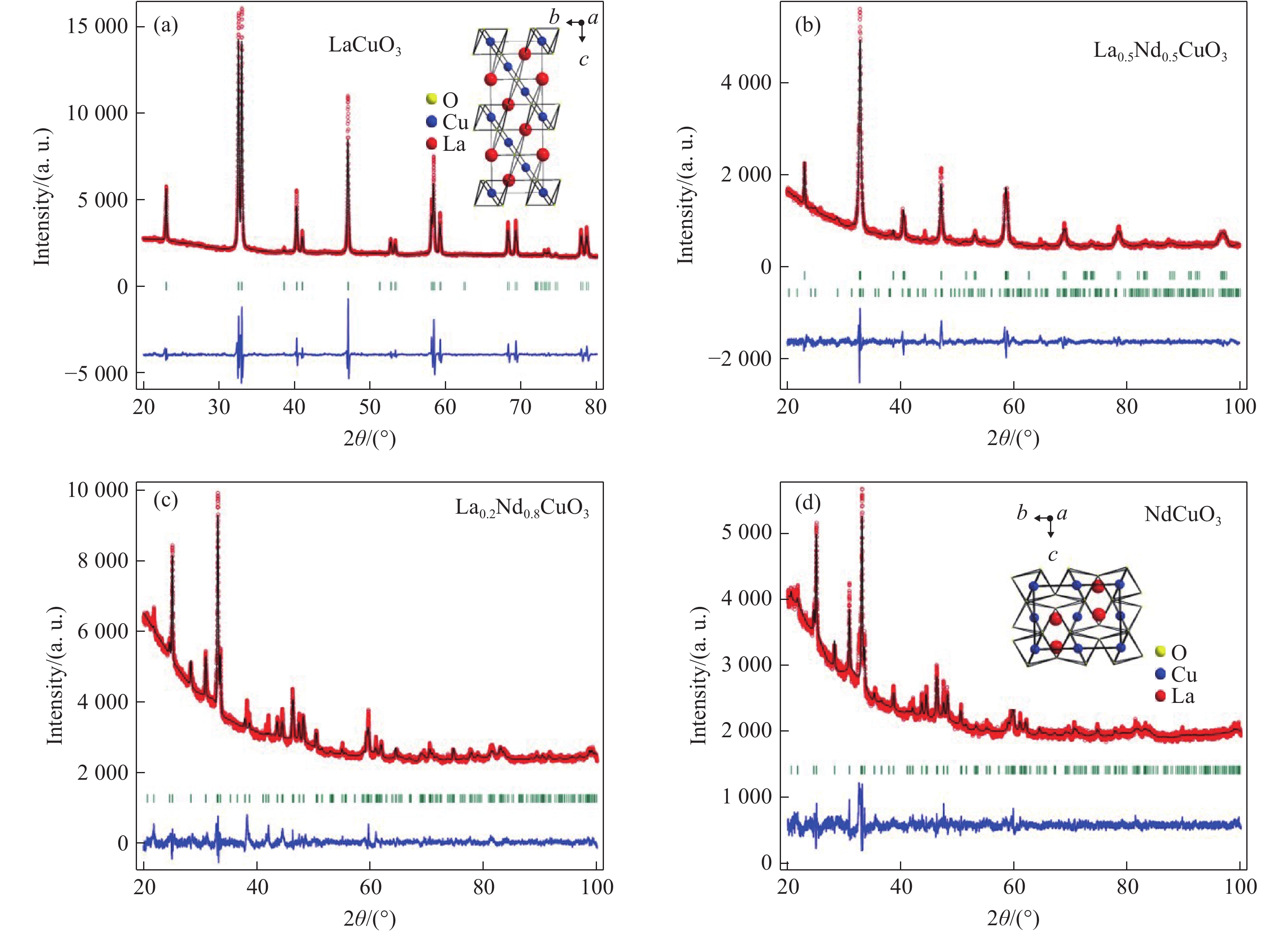
图2显示了LaCuO3、La0.5Nd0.5CuO3、La0.2Nd0.8CuO3和NdCuO3的PXRD数据精修结果，其他更多组分的La1–xNdxCuO3（0≤x≤1）结构精修结果见表2，其中：RP、RWP、Chi2为精修品质因子。
图 2 (a) LaCuO3、(b) La0.5Nd0.5CuO3、(c) La0.2Nd0.8CuO3和(d) NdCuO3 的PXRD Rietveld 精修谱图Figure 2. Rietveld refinement of PXRD patterns of (a) LaCuO3, (b) La0.5Nd0.5CuO3, (c) La0.2Nd0.8CuO3 and (d) NdCuO3表 2 La1–xNdxCuO3（0≤x≤1）的晶格参数Table 2. Structural parameters of La1–xNdxCuO3 (0≤x≤1)Sample Space group Lattice parameters/Å RP/% RWP/% Chi2 LaCuO3 $R\overline 3 c $ a=b=5.4976(6), c=13.2062(9) 3.05 5.59 7.01 La0.9Nd0.1CuO3 $R\overline 3 c $ a=b=5.4976(9), c=13.1917(5) 1.45 1.92 1.10 La0.8Nd0.2CuO3 $R\overline 3 c $ a=b=5.4988(9), c=13.1732(7) 1.25 1.68 0.79 La0.7Nd0.3CuO3 $R\overline 3 c $ a=b=5.4961(1), c=13.1625(7) 1.91 2.73 2.48 La0.6Nd0.4CuO3 $R\overline 3 c $ a=5.4933(9), c=13.1326(6) 2.41 4.14 7.09 La0.5Nd0.5CuO3 Phase 1: $R\overline 3 c $ (36.43%) a=b=5.4627(1), c=13.2988(9) 4.23 5.85 2.42 Phase 2: Pnma (63.57%) a=6.1821(1), b=7.3597(8), c=5.4318(7) 4.23 5.85 2.42 La0.4Nd0.6CuO3 Phase 1: $R\overline 3 c $ (43.49%) a=b=5.4571(7), c=13.2982(1) 1.25 1.68 0.79 Phase 2: Pnma (56.51%) a=6.5015(2), b=7.6552(6), c=5.3354(4) 1.25 1.68 0.79 La0.3Nd0.7CuO3 Phase 1: $R\overline 3 c $ (44.42%) a=b=5.4542(6), c=13.3191(9) 3.76 4.81 1.43 Phase 2: Pnma (55.58%) a=6.3189(6), b=7.2736(7), c=5.3706(8) 3.76 4.81 1.43 La0.2Nd0.8CuO3 Pnma a=6.3197(8), b=7.2408(1), c=5.3561(1) 7.75 9.92 2.20 La0.1Nd0.9CuO3 Pnma a=6.3045(3), b=7.2421(7), c=5.3462(9) 1.54 2.24 1.66 NdCuO3 Pnma a=6.3039(2), b=7.2176(2), c=5.3334(5) 1.72 2.48 1.42 LaCuO3具有空间群为
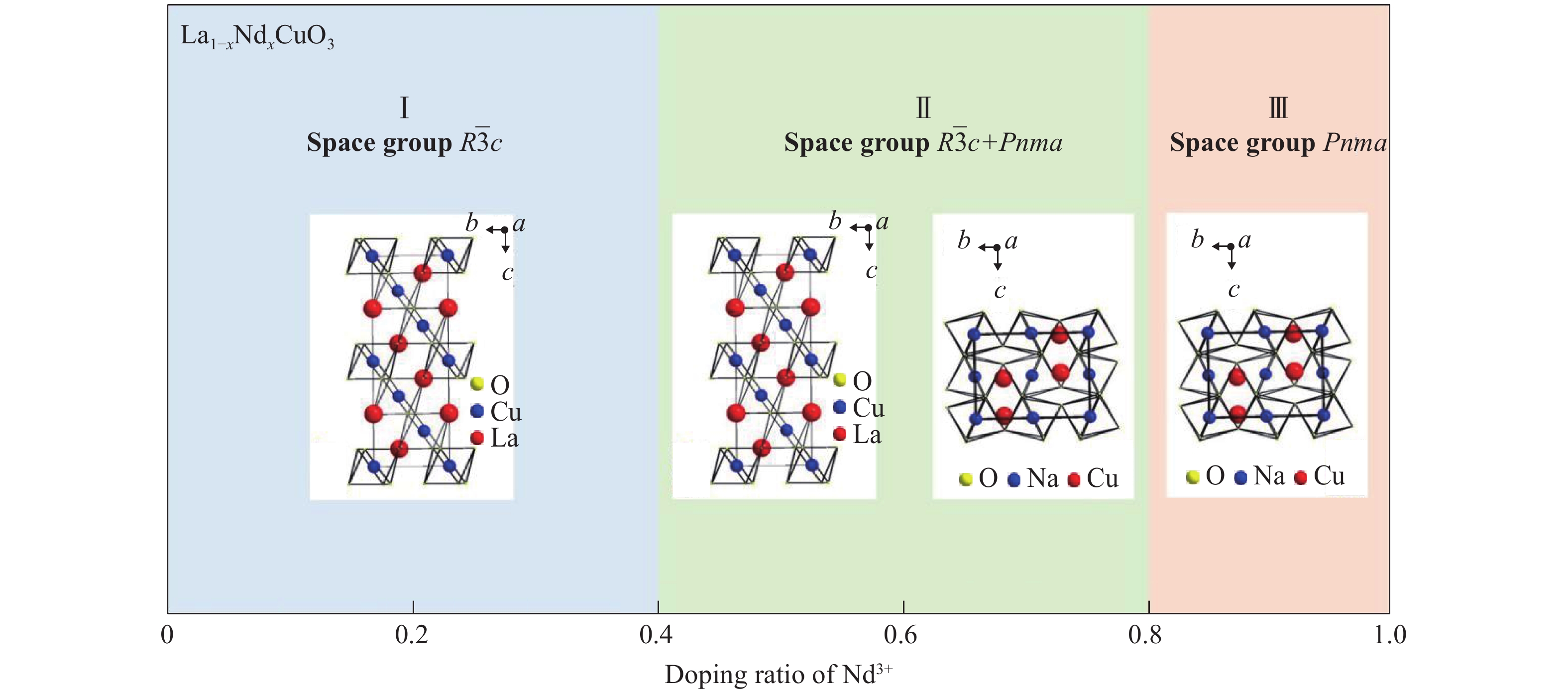
$R\overline 3 c $ 的菱方结构，而NdCuO3具有空间群为Pnma的正交结构，与之前报道[24, 26]一致。随着Nd3+掺杂浓度的增加，当x为0.5、0.6、0.7时，样品中存在$R\overline 3 c $ 菱方结构与Pnma正交结构共存的情况。这一结果与之前Zhou等[25]报道的La1–xNdxCuO3（0≤x≤0.6）保持菱方结构的结论不同，考虑可能与样品合成的压力和温度条件有关。进一步增大固溶体中Nd3+的掺杂浓度，发现样品在x≥0.8时完全转变为Pnma正交结构，并具有较大的晶胞参数和晶格畸变。基于以上结果，绘制了La1–xNdxCuO3（0≤x≤1）体系随Nd3+掺杂浓度变化的结构相图，如图3所示。该体系是Cu3+的单价钙钛矿，包含一个半填充的σ*窄带。LaCuO3在低温下表现出金属行为[36]。随着Nd3+掺杂增加，σ*的带宽将逐渐变窄，因此可在该材料中系统研究巡游σ*电子向局域e电子的渡越行为。与之类似的镍基RNiO3氧化物体系中，随着稀土离子半径的减小，在PrNiO3与NdNiO3之间发生了从金属到反铁磁绝缘体的一级相变，Ni―O键长和Ni―O―Ni键角的变化与巡游σ*电子向局域e电子的渡越密切相关[37]。之前的报道并未在La1–xNdxCuO3（0≤x≤0.6）样品中发现金属-绝缘体相变[25]。由于NdCuO3表现出绝缘体行为[26]，后续可在具有更高Nd3+掺杂浓度的La1–xNdxCuO3 （0.7≤x≤1）样品中探索电子临界行为和磁性转变。
图 3 La1–xNdxCuO3（0≤x≤1）的结构相图Figure 3. Structural phase diagram of La1–xNdxCuO3 (0≤x≤1)3. 结 论
通过自主设计加工的Walker型高压组件，实现了1300 ℃、25 GPa的高温高压合成条件。在此基础上，成功合成了La1–xNdxCuO3（0≤x≤1）系列样品，并对其进行了详细的结构研究。实验结果表明：当x在0～0.4范围内时，样品具有
$R\overline 3 c $ 菱方结构的单相；当x为0.5、0.6、0.7时，样品具有$R\overline 3 c $ 菱方结构与Pnma正交结构共存的混合相；而当x在0.8～1.0范围内时，样品具有Pnma正交结构的单相。该体系为深入研究Cu3+单价钙钛矿的结构和物性演化规律提供了材料基础。 -
图 2 Nb3Sn单晶的分子动力学计算模型
Figure 2. Molecular dynamics simulation model of Nb3Sn single crystal
图 3 300 K下不含裂纹和含中心裂纹的Nb3Sn单晶拉伸应力-应变曲线
Figure 3. Tensile stress-strain curves of Nb3Sn single crystal without crack and with central crack at 300 K
图 4 4.2 K下Nb3Sn单晶的拉伸应力-应变曲线
Figure 4. Tensile stress-strain curves of Nb3Sn single crystal at 4.2 K
图 5 4.2 K下不含裂纹的Nb3Sn单晶的原子结构演化
Figure 5. Atomic structure evolution of Nb3Sn single crystal without crack at 4.2 K
图 6 4.2 K下含中心裂纹的Nb3Sn单晶的原子结构演化
Figure 6. Atomic structure evolution of Nb3Sn single crystal with central crack at 4.2 K
图 7 4.2 K下不含裂纹的Nb3Sn单晶在拉伸方向的原子应力云图
Figure 7. Atomic stress distribution of Nb3Sn single crystal without crack at 4.2 K
图 8 4.2 K下含中心裂纹的Nb3Sn单晶在拉伸方向的原子应力云图
Figure 8. Atomic stress distribution of Nb3Sn single crystal with central crack at 4.2 K
图 9 4.2 K不同应变率下Nb3Sn单晶的拉伸应力-应变曲线
Figure 9. Tensile stress-strain curves of Nb3Sn single crystal under different strain rates at 4.2 K
图 10 应变率为5×109 s−1时不含裂纹的Nb3Sn单晶的原子结构演化
Figure 10. Atomic structure evolution in Nb3Sn single crystal without crack at strain rate of 5×109 s−1
图 11 应变率为1×1010 s−1时不含裂纹的Nb3Sn单晶的原子结构演化
Figure 11. Atomic structure evolution in Nb3Sn single crystal without crack at strain rate of 1×1010 s−1
图 12 应变率为5×109 s−1时含中心裂纹的Nb3Sn单晶的原子结构演化
Figure 12. Atomic structure evolution in Nb3Sn single crystal with central crack at strain rate of 5×109 s−1
图 13 应变率为1×1010 s−1时含中心裂纹的Nb3Sn单晶的原子结构演化
Figure 13. Atomic structure evolution in Nb3Sn single crystal with central crack at strain rate of 1×1010 s−1
图 14 不同应变率下应力峰值处的原子应力云图
Figure 14. Atomic stress distribution at stress peak under different strain rates
表 1 Nb3Sn单晶的弹性常数和晶格常数
Table 1. Elastic constants and lattice constant of Nb3Sn single crystal
Method C11/GPa C12/GPa C44/GPa a/Å This work 284.10 95.84 53.76 5.21 First principle 284.32 107.70 67.07 5.32 
下载: 导出CSV
-
[1] 王秋良. 高磁场超导磁体科学 [M]. 北京: 科学出版社, 2008: 24–37.WANG Q L. High-field superconducting magnets science [M]. Beijing: Science Press, 2008: 24–37. [2] 周又和, 王省哲. ITER超导磁体设计与制备中的若干关键力学问题 [J]. 中国科学(物理学 力学 天文学), 2013, 43(12): 1558–1569. doi: 10.1360/132013-166ZHOU Y H, WANG X Z. Review on some key issues related to design and fabrication of superconducting magnets in ITER [J]. Scientia Sinica (Physica, Mechanica & Astronomica), 2013, 43(12): 1558–1569. doi: 10.1360/132013-166 [3] 梁明, 张平祥, 卢亚锋, 等. 磁体用Nb3Sn超导体研究进展 [J]. 材料导报, 2006, 20(12): 1–4. doi: 10.3321/j.issn:1005-023X.2006.12.001LIANG M, ZHANG P X, LU Y F, et al. Advances in Nb3Sn superconductor for magnet application [J]. Materials Review, 2006, 20(12): 1–4. doi: 10.3321/j.issn:1005-023X.2006.12.001 [4] DEVRED A, BACKBIER I, BESSETTE D, et al. Challenges and status of ITER conductor production [J]. Superconductor Science and Technology, 2014, 27(4): 044001. doi: 10.1088/0953-2048/27/4/044001 [5] SANABRIA C, LEE P J, STARCH W, et al. Evidence that filament fracture occurs in an ITER toroidal field conductor after cyclic Lorentz force loading in SULTAN [J]. Superconductor Science and Technology, 2012, 25(7): 075007. doi: 10.1088/0953-2048/25/7/075007 [6] SHETH M K, LEE P J, MCRAE D M, et al. Study of filament cracking under uniaxial repeated loading for ITER TF strands [J]. IEEE Transactions on Applied Superconductivity, 2012, 22(3): 4802504. doi: 10.1109/TASC.2011.2174554 [7] LU J, HAN K, GODDARD R E, et al. The IC irreversible strain of some ITER high JC Nb3Sn wires [J]. IEEE Transactions on Applied Superconductivity, 2009, 19(3): 2637–2640. doi: 10.1109/TASC.2009.2019597 [8] NIJHUIS A, MIYOSHI Y, JEWELL M C, et al. Systematic study on filament fracture distribution in ITER Nb3Sn strands [J]. IEEE Transactions on Applied Superconductivity, 2009, 19(3): 2628–2632. doi: 10.1109/TASC.2009.2018082 [9] CHEGGOUR N, LEE P J, GOODRICH L F, et al. Influence of the heat-treatment conditions, microchemistry, and microstructure on the irreversible strain limit of a selection of Ti-doped internal-tin Nb3Sn ITER wires [J]. Superconductor Science and Technology, 2014, 27(10): 105004. doi: 10.1088/0953-2048/27/10/105004 [10] DYLLA M T, SCHULTZ S E, JEWELL M C, et al. Fracture strength distribution of individual Nb3Sn filaments [J]. IEEE Transactions on Applied Superconductivity, 2016, 26(8): 6001907. doi: 10.1109/TASC.2016.2602819 [11] ZHAI Y H, BIRD M D. Florida electro-mechanical cable model of Nb3Sn CICCs for high-field magnet design [J]. Superconductor Science and Technology, 2008, 21(11): 115010. doi: 10.1088/0953-2048/21/11/115010 [12] WANG X, GAO Y W. Tensile behavior analysis of the Nb3Sn superconducting strand with damage of the filaments [J]. IEEE Transactions on Applied Superconductivity, 2016, 26(4): 6000304. doi: 10.1109/TASC.2015.2509601 [13] PAPADIMITRIOU I, UTTON C, TSAKIROPOULOS P. Ab initio investigation of the intermetallics in the Nb-Sn binary system [J]. Acta Materialia, 2015, 86: 23–33. doi: 10.1016/j.actamat.2014.12.017 [14] SUNDARESWARI M, RAMASUBRAMANIAN S, RAJAGOPALAN M. Elastic and thermodynamical properties of A15 Nb3X (X = Al, Ga, In, Sn and Sb) compounds-first principles DFT study [J]. Solid State Communications, 2010, 150(41/42): 2057–2060. doi: 10.1016/j.ssc.2010.08.004 [15] ZHANG R, GAO P F, WANG X Z, et al. First-principles study on elastic and superconducting properties of Nb3Sn and Nb3Al under hydrostatic pressure [J]. AIP Advances, 2015, 5(10): 1–9. doi: 10.1063/1.4935099 [16] DE MARZI G, MORICI L, MUZZI L, et al. Strain sensitivity and superconducting properties of Nb3Sn from first principles calculations [J]. Journal of Physics: Condensed Matter, 2013, 25(13): 135702. doi: 10.1088/0953-8984/25/13/135702 [17] REN Z, GAMPERLE L, FETE A, et al. Evolution of T2 resistivity and superconductivity in Nb3Sn under pressure [J]. Physical Review B, 2017, 95(18): 184503. doi: 10.1103/PhysRevB.95.184503 [18] 严六明, 朱素华. 分子动力学模拟的理论与实践 [M]. 北京: 科学出版社, 2013: 1−6.YAN L M, ZHU S H. Theory and practice of molecular dynamics simulation [M]. Beijing: Science Press, 2013: 1−6. [19] CHUDINOV V G, GOGOLIN V P, GOSHCHITSKII B N, et al. Simulation of collision cascades in intermetallic Nb3Sn compounds [J]. Physica Status Solidi (A), 1981, 67(1): 61–67. doi: 10.1002/pssa.2210670103 [20] 陈伟. 第Ⅰ类超导体中的平衡磁结构及其动力学研究 [D]. 南京: 南京大学, 2016: 1−20.CHEN W. Equilibrium intermediate-state patterns and dynamics in a type-Ⅰ superconducting slab [D]. Nanjing: Nanjing University, 2016: 1−20. [21] HALL D L. New insights into the limitations on the efficiency and achievable gradients in Nb3Sn SRF cavities [D]. New York: Cornell University, 2017: 1−60. [22] ZHANG Y Q, JIANG S Y. Molecular dynamics simulation of crack propagation in nanoscale polycrystal nickel based on different strain rates [J]. Metals, 2017, 7(10): 432. doi: 10.3390/met7100432 [23] LI L, CHEN H T, FANG Q H, et al. Effects of temperature and strain rate on plastic deformation mechanisms of nanocrystalline high-entropy alloys [J]. Intermetallics, 2020, 120: 106741. doi: 10.1016/j.intermet.2020.106741 [24] WU W P, YAO Z Z. Influence of a strain rate and temperature on the crack tip stress and microstructure evolution of monocrystalline nickel: a molecular dynamics simulation [J]. Strength of Materials, 2014, 46(2): 164–171. doi: 10.1007/s11223-014-9531-0 [25] ZHANG Y, ASHCRAFT R, MENDELEV M I, et al. Experimental and molecular dynamics simulation study of structure of liquid and amorphous Ni62Nb38 alloy [J]. The Journal of Chemical Physics, 2016, 145(20): 204505. doi: 10.1063/1.4968212 [26] KO W S, KIM D H, KWON Y J, et al. Atomistic simulations of pure tin based on a new modified embedded-atom method interatomic potential [J]. Metals, 2018, 8(11): 900. doi: 10.3390/met8110900 [27] 石震天, 杨绪佳, 王豪阳, 等. 高压下Nb3Sn单晶的超导相转变 [J]. 高压物理学报, 2021, 35(2): 021102. doi: 10.11858/gywlxb.20200615SHI Z T, YANG X J, WANG H Y, et al. Superconducting transition of Nb3Sn single crystal under high-pressure [J]. Chinese Journal of High Pressure Physics, 2021, 35(2): 021102. doi: 10.11858/gywlxb.20200615 [28] DARKINS R, SUSHKO M L, LIU J, et al. Stress in titania nanoparticles: an atomistic study [J]. Physical Chemistry Chemical Physics, 2014, 16(20): 9441–9447. doi: 10.1039/C3CP54357A [29] 钟群鹏, 赵子华. 断口学 [M]. 北京: 高等教育出版社, 2006: 54–77, 176−241.ZHONG Q P, ZHAO Z H. Fractography [M]. Beijing: Higher Education Press, 2006: 54−77, 176−241. [30] 上海交通大学《金属断口分析》编写组. 金属断口分析 [M]. 北京: 国防工业出版社, 1979: 4−26. [31] WEST A W, RAWLINGS R D. The microstructure and mechanical properties of Nb3Sn filamentary superconducting composites [J]. Journal of Materials Science, 1979, 14(5): 1179–1186. doi: 10.1007/BF00561303 [32] TAKEUCHI T, TSUCHIYA K, SAEDA M, et al. Electron backscatter diffraction analysis on Nb3Sn and Nb3Al multifilaments [J]. IEEE Transactions on Applied Superconductivity, 2011, 21(3): 2541–2545. doi: 10.1109/TASC.2010.2083625 [33] GODEKE A. Performance boundaries in Nb3Sn superconductors [D]. Enschede: University of Twente, 2005: 1−30. -
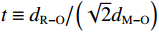
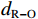
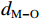
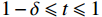
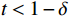
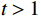
下载:
下载:
点击查看大图
计量
- 文章访问数: 1339
- HTML全文浏览量: 442
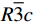
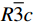
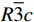
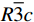
- PDF下载量: 30
