Response Law of Subway Platform and Surrounding Rock under Solid Explosion
-
摘要: 地铁站内发生爆炸将造成巨大的人员伤亡和财产损失。依托上海某地铁站工程,将HJC模型嵌入开源物质点法程序中,研究了固体炸药爆炸作用下地铁站台及围岩的响应规律。结果表明:受爆炸应力波的影响,站台顶板和底板响应压强在短时间内达到峰值后迅速降低,站台结构在爆炸过程中既存在受拉区又存在受压区;在站台边墙处,由于应力波与反射波叠加,会出现超压突变区;爆炸作用使站台结构整体下沉,且起爆点正下方围岩会形成塌陷坑,起爆点正上方围岩和车站结构相对周围向上隆起;结构受损区域主要集中在结构底板,呈椭圆形;站台有柱区域的抗爆能力强于无柱区域。Abstract: An explosion in the subway station will lead to huge losses of personnel and property. In order to study the response law of subway platform and surrounding rock of a subway station project in Shanghai under the action of solid explosive explosion, HJC model is embedded in the open source material point method program. The results show that the response pressure of platform roof and floor will decrease rapidly after reaching the peak in a short time under the influence of explosion wave, and the platform structure forms tension zone and compression zone during explosion. Due to the superposition of stress wave and reflected wave, overpressure sudden change zone will appear at the platform side wall. The whole platform structure sink under explosion. Moreover, the surrounding rock directly below the detonation point forms a collapse pit, and the surrounding rock directly above the detonation point and the station structure rises up. The damaged area is mainly concentrated in the bottom plate of the structure, and the damaged area is oval. The blast resistance of platform with column area is stronger than that without column area.
-
Key words:
- subway platform /
- HJC model /
- explosion /
- structural response /
- material point method
-
地铁站通常具有人流量大[1]、结构封闭等特点,一旦发生爆炸,将会造成巨大的人员伤亡和财产损失,爆炸产生的能量还会影响地铁站结构的安全性,因此研究地铁站台及围岩爆炸响应规律对于地铁站的安全运营具有重要意义。
针对地下空间爆炸问题开展实验研究,成本高且安全风险大,因此国内外学者一般采用有限元方法进行模拟研究。例如:王勇等[2]基于LS-DYNA3D算法,对某地铁区间隧道爆炸响应情况进行数值模拟,验证了隧道结构能抵抗10 kg TNT炸药的爆炸;Choi等[3]对隧道内外不同位置起爆的响应情况进行了数值模拟,为坑道易损性评估提供了一种简单实用的方法;Zhang等[4]研究了典型矿山密封在冲击载荷作用下的力学响应性能;柴永生等[5]模拟了爆炸冲击波在地铁出入口的传播规律,为地铁及其他地下工程出入口部位的技术标准制定和防护装备设施设计提供了参考。有限元方法在模拟由爆炸引起的岩土体大变形问题时,常采用输入应力波的方式模拟爆炸应力,与气体爆炸冲击岩土体的实际情况存在较大差异,此外,岩土体大变形还会造成有限元网格畸变[6]。物质点法是采用欧拉法与拉格朗日法双重描述的无网格粒子法,将连续体离散成一组带有质量的质点,并在背景网格节点上求解动量方程,计算空间导数。物质点法兼具拉格朗日法和欧拉法的双重优势,能够避免网格畸变和对流项处理造成的误差和计算量增大问题[7],对物质交界面无需特殊处理,自动满足无滑移的接触条件[8],能够直接模拟物体之间的冲击现象。这些特点使物质点法在处理爆炸冲击[6, 9]和岩土大变形[10-11]问题上较有限元方法更具优势。
由于地铁站结构多为混凝土结构,需要考虑爆炸作用下混凝土孔隙坍缩现象,因此在数值模拟中应选择适宜的材料模型。Holmquist、Johnson和Cook提出了用于模拟混凝土材料的HJC模型[7],该模型能够描述爆炸作用对地下基础设施的损伤区域大小和损伤强度,为此,本研究将HJC模型嵌入物质点法程序中,以模拟站台结构响应情况。
本研究依托上海某地铁站工程,基于张雄教授公开的开源版物质点法MPM3D-F90程序[7],将HJC模型嵌入程序,模拟地铁站内固体炸药爆炸冲击结构和围岩过程,探究爆炸作用下地铁站台及围岩的响应规律,以期为合理应对地铁爆炸灾害以及灾后人员救援、结构抢修和加固提供一定的指导。
1. 材料本构模型
1.1 材料本构模型基本原理
针对站台结构和大厅底板等混凝土结构,采用HJC本构模型进行模拟。该模型考虑了混凝土材料的受损情况和高压下混凝土孔隙坍缩现象,能够描述爆炸作用下地下基础设施的损伤区域大小及损伤强度[7]。
HJC 模型的极限面被描述为损伤、应变率与静水压力的函数,其破坏失效面可以表示为
σ∗=[A(1−D)+Bp∗N](1+Cln˙ε∗) (1) 式中:
σ∗ 为归一化等效强度,σ∗=σ/fc ,σ 为真实应力,fc 为单轴抗压强度,且满足\mathop \sigma \nolimits^ * \leqslant {S_{\max }} ,{S_{\max }} 为归一化最大等效屈服强度;\mathop p\nolimits^ * 为归一化压力,\mathop p\nolimits^ * = p/{f_{\rm c}} ,p为真实压力;{\dot \varepsilon ^ * } = \dot \varepsilon /{\dot \varepsilon _0} ,{\dot \varepsilon ^ * } 为等效应变率,\dot \varepsilon 为真实应变率,{\dot \varepsilon _0} 为参考应变率;D为损伤因子(0 \leqslant D \leqslant 1 );A为归一化黏聚强度;B为归一化压力硬化系数;N为压力硬化指数;C为应变率系数。损伤因子 D 由等效塑性应变和体积应变累加得到,即损伤演化方程可表示为
D={\displaystyle \sum (\Delta {\varepsilon }_{\rm p}+\Delta {\mu }_{\rm p})}/({\varepsilon }_{\rm p}^{\rm f}+{\mu }_{\rm p}^{\rm f}) (2) 式中:
\Delta {\varepsilon _{\rm p}} 为等效塑性应变增量,\Delta {\mu _{\rm p}} 为塑性体积应变增量,\varepsilon _{\rm p}^{\rm f} 和\mu _{\rm p}^{\rm f} 分别为常压下材料破碎时的塑性应变和塑性体积应变。多数情况下,HJC模型的损伤主要由等效塑性应变引起。针对围岩结构,采用适用于岩石材料的Drucker-Prager(D-P)模型进行模拟,其屈服函数为
{f_{\rm s}} = \alpha \frac{{{I_1}}}{3} + \sqrt {{J_2}} - k (3) 式中:I1为应力张量第一不变量,J2为偏应力张量第二不变量,参数
\alpha 和k可由岩石黏聚力c和内摩擦角\phi 确定。若取屈服面为Mohr-Coulomb准则外接圆,则\alpha 和k可由下式计算a = \frac{{6\sin \phi }}{{\sqrt 3 \left( {3 - \sin \phi } \right)}}, \;\;\;\;\;\; k = \frac{{6c\cos \phi }}{{\sqrt 3 \left( {3 - \sin \phi } \right)}} (4) 1.2 应力更新
更新后的应力
{\sigma '_{ij}} 可以由应力率{\dot \sigma _{ij}} 获得,即{\sigma '_{ij}} = {\sigma _{ij}} + \Delta t \; {\dot \sigma _{ij}} (5) 式中:
\Delta t 为时间增量。在大变形物体的本构关系中,为了确保应力率不受刚体旋转的影响,需要将其转化为在与物体固定连接的动态坐标系中的焦曼应力率\dot \sigma _{ij}^{\rm J} \dot \sigma _{ij}^{\rm J} = \dot \sigma _{ij}^{} - {\sigma _{ik}}{\varOmega _{jk}} - {\varOmega _{ik}}{\sigma _{kj}} (6) 式中:
{\varOmega _{ij}} 为旋率张量分量。将式(6)代入式(5),得到更新后的应力{\sigma '_{ij}} = {\sigma _{ij}} + \Delta t\left( {\dot \sigma _{ij}^{\rm J} + {\sigma _{ik}}{\varOmega _{jk}} + {\varOmega _{ik}}{\sigma _{kj}}} \right) (7) 1.3 接触算法
本研究中多个物体之间的接触算法与文献[6]相同,受篇幅限制,这里不再赘述。
1.4 HJC模型验证
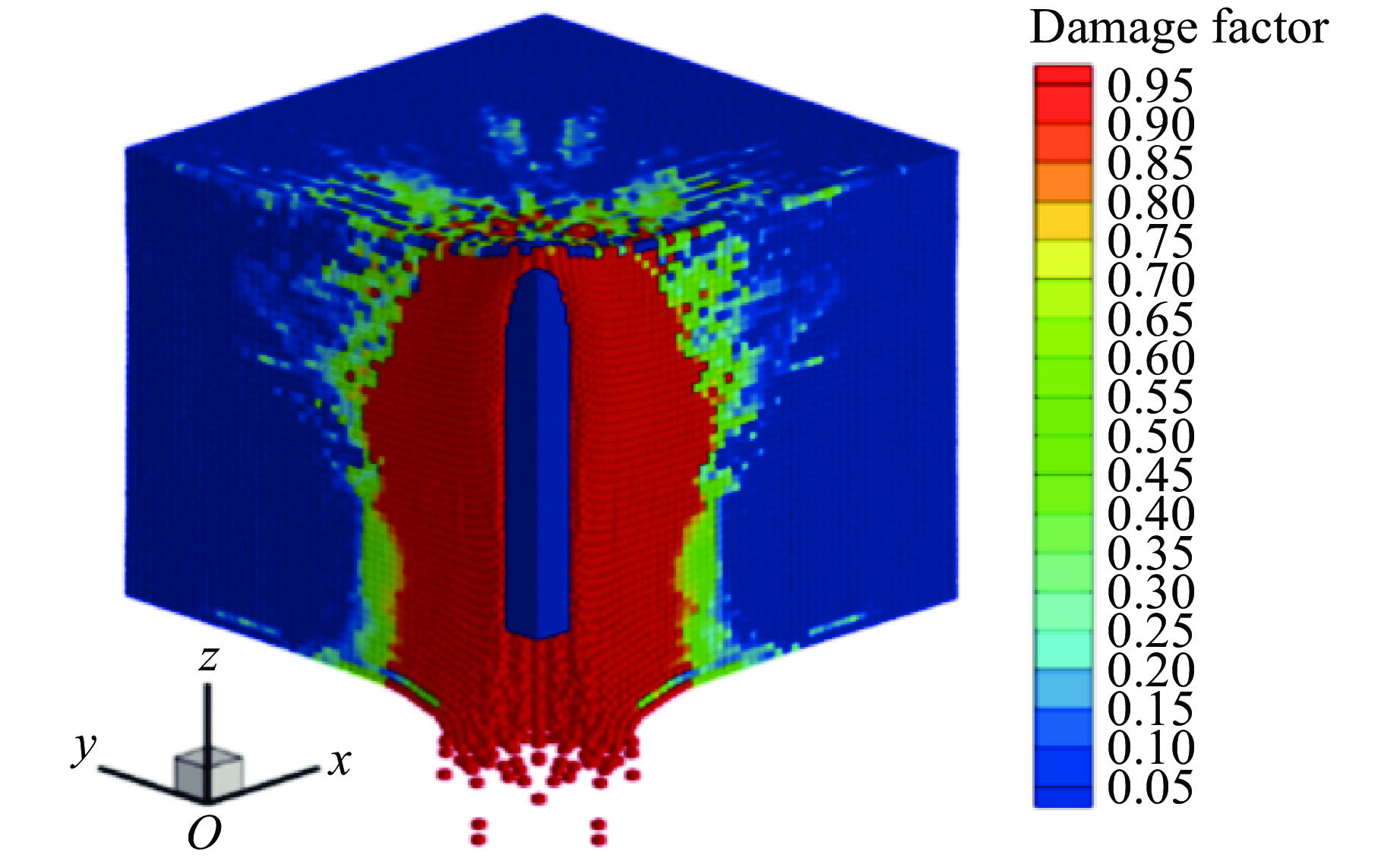
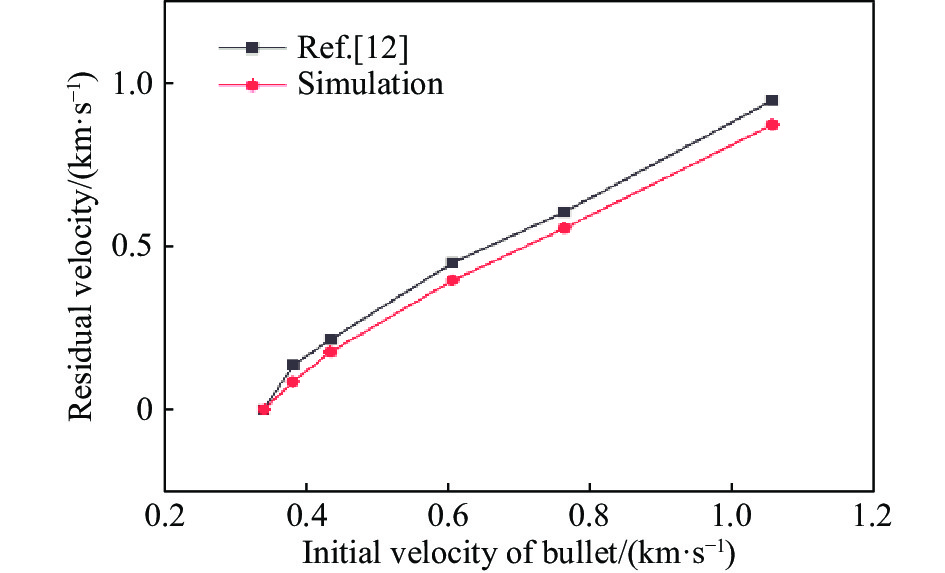
参照Hanchak等[12]的实验结果,模拟子弹穿越无筋混凝土板过程,记录子弹以不同初速度穿越混凝土板后的剩余速度,并将结果与实验数据进行对比。模拟中混凝土材料采用HJC模型,子弹采用理想弹性模型,具体参数与文献[12]一致。弹体初速度为1058 m/s时的侵彻结果如图1所示,从中可以清晰地看到,在子弹的冲击作用下混凝土质点受损因子呈辐射状分布。图2显示了子弹剩余速度的对比结果,可见物质点法模拟得到的数据与实验结果吻合较好,模拟结果正确可靠。
2. 计算模型设置
2.1 物质点法模型建立
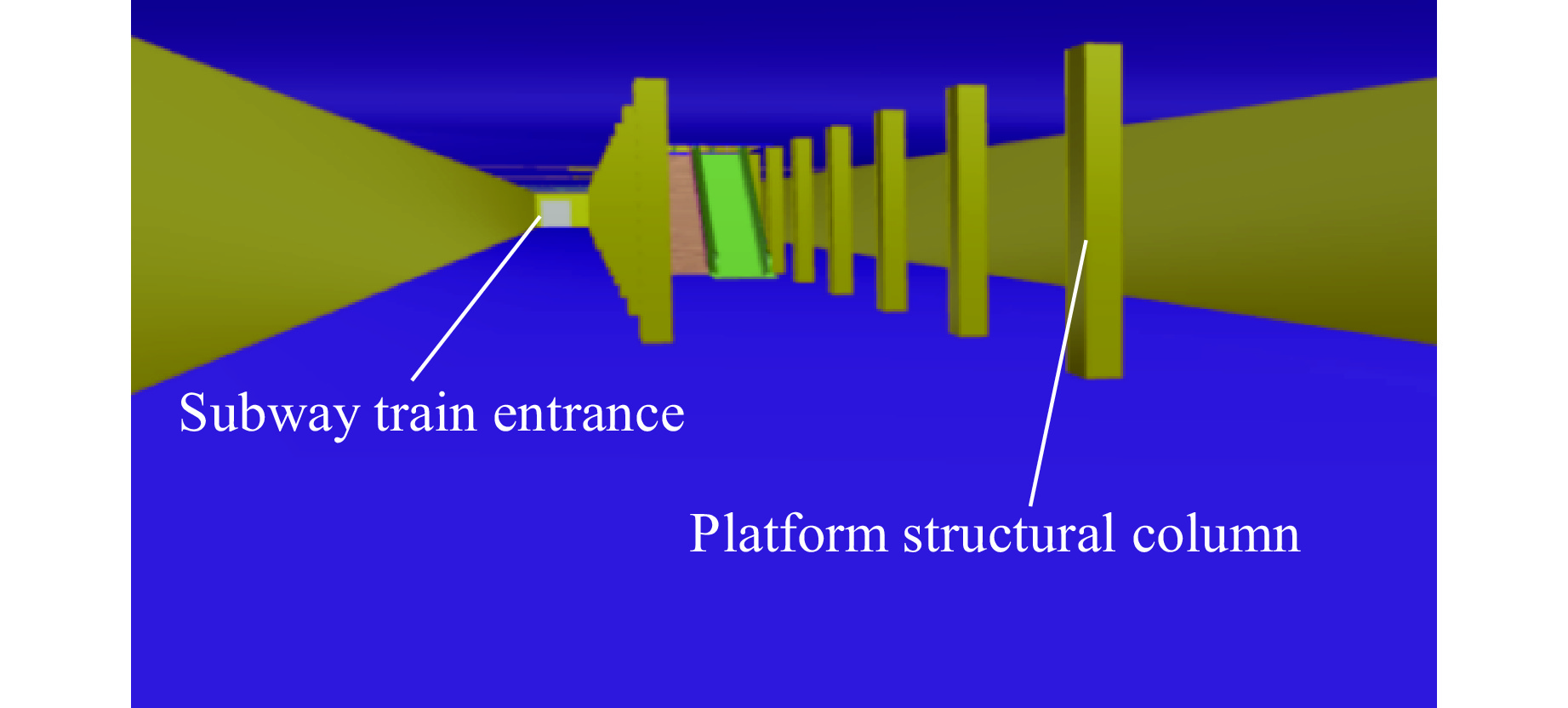
上海某地铁站站台全长271.7 m(其中有柱区域长205.0 m,其余为无柱区域),宽19.1 m,层高6.15 m。站台有柱区域均匀分布52根方柱,站台与上部车站大厅之间的围岩厚度为5 m,方柱贯穿此围岩,将站台与车站大厅相连。站台本体结构材料为钢筋混凝土,厚度为0.9 m,标号为C40,内部结构的BIM模型如图3所示。
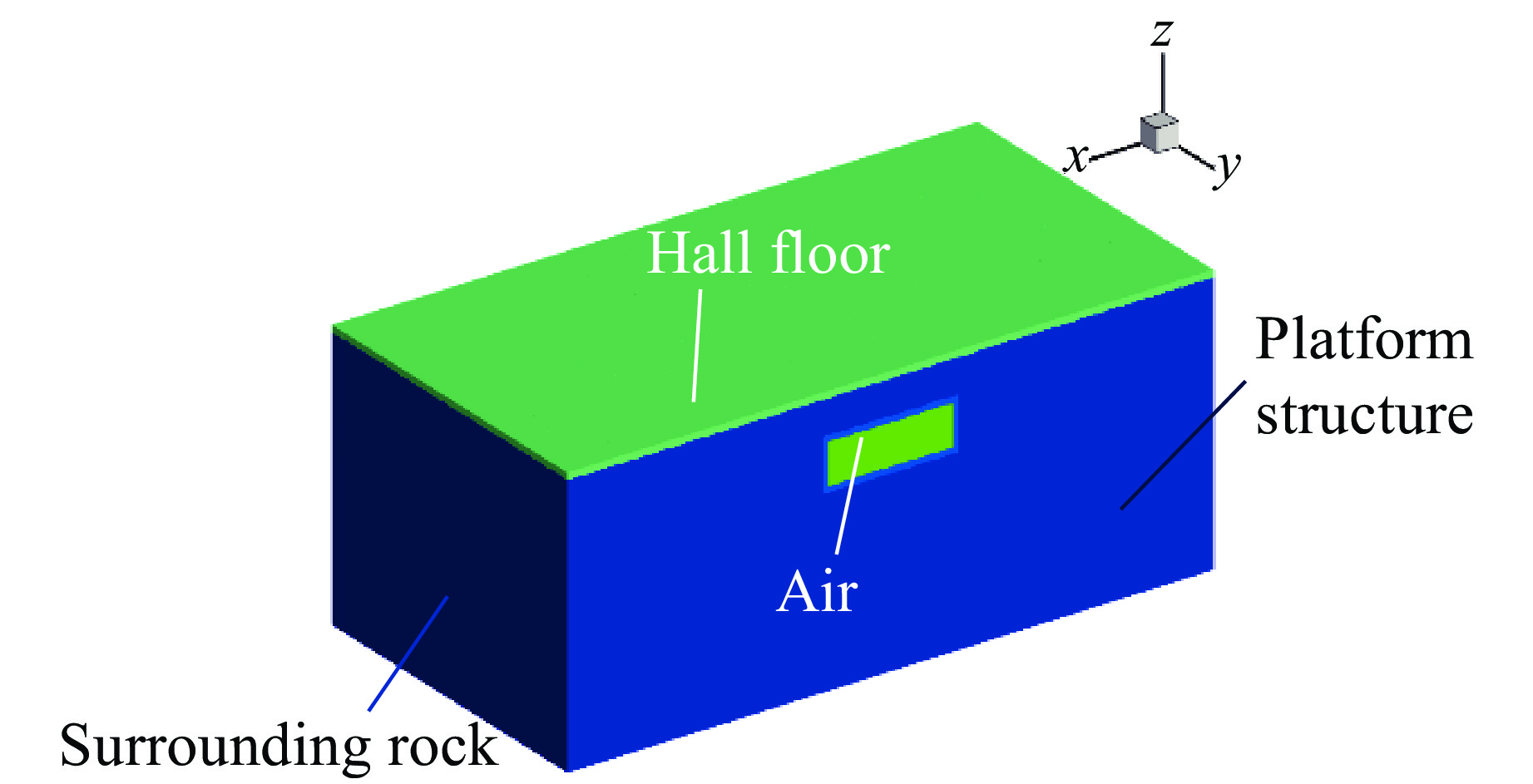
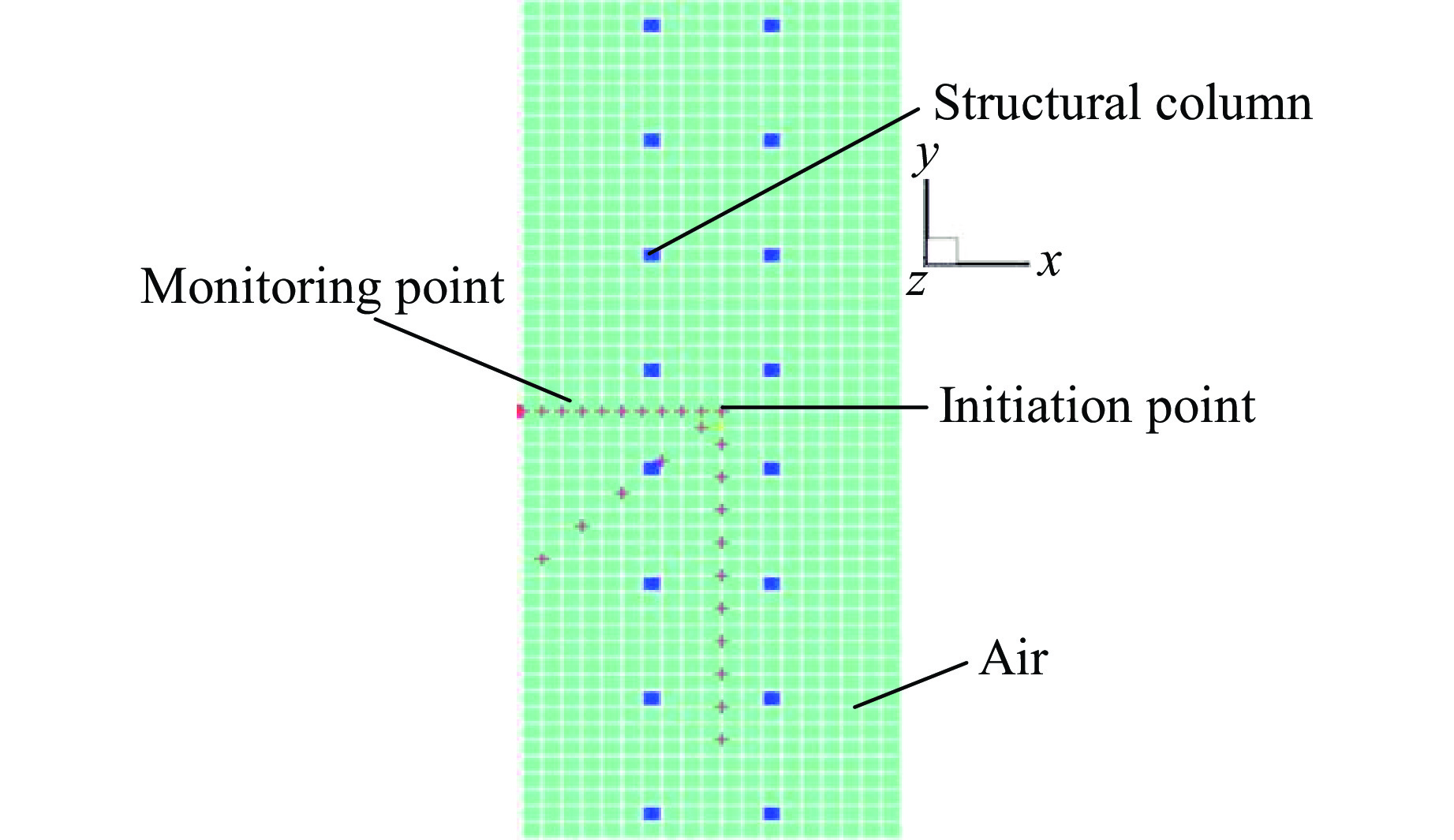
约定模型中与站台列车运行方向平行的方向为纵向,与运行方向垂直的方向为横向。同时,为模拟车站上部的建筑物和人员荷载,设置站台埋深为5 m,并在模型顶部添加90 kPa竖向荷载,模拟站台顶部建筑荷载。由于爆炸作用范围有限,为减小数值模拟的计算量,本研究按照无柱区域与有柱区域分别建立站台模型。无柱区域模型长度为99 m(5.2倍横向尺寸),如图4所示,模型整体尺寸为99 m×61 m×40 m。该地铁站台结构柱的标准间距为横向4.900 m,纵向7.125 m,距边墙6.100 m。结构柱将站台顶板和底板与大厅底板连接,据此建立站台净空内结构柱模型,如图5所示。
2.2 模型参数选取
模型四周采用无反射边界模拟侧向无限围岩,顶部采用自由边界模拟车站大厅地面。站台底部围岩在水平方向上均匀分布,在竖直方向上,上部围岩的强度较低,而底部50 m处围岩为中低压缩性砂土,土质密实,故底部采用对称边界模拟其界面。站台本体结构和上部车站大厅采用混凝土HJC强度准则,除最大静水压力外(站台本体结构为4 MPa,车站大厅底板为2 MPa),其他材料参数均相同,如表1所示,其中:
\,\rho 为密度,E为弹性模量,ν为泊松比,{f'_{\rm c}} 为无侧限抗压强度,\varepsilon _{\min }^{\rm f} 为最小破碎塑性应变,pcrush为孔洞坍塌压力,plock为压实压力,D1、D2、K1、K2、K3为材料参数,vp为纵波波速,vs为横波波速。围岩为淤泥质砂土,采用D-P模型描述,物理力学参数如表2所示,其中:q_{\phi} 和K_{\phi} 为模型参数,q_{\varPsi} 为剪胀角,\sigma_{\rm{t}} 为岩石抗拉强度。内部空气采用无偏应力空气模型模拟,其状态方程采用理想气体状态方程,物理力学参数如表3所示,其中:c为波速,E0为初始内能,\kappa 为比热容比。表 1 混凝土结构的HJC模型参数Table 1. HJC model parameters of concrete structure\,\rho /(kg·m–3) E/GPa \nu { f{'_ {\rm{c} } } }/MPa Smax A B N C \varepsilon {_{\min }^{\rm f} } 2439 32.5 0.2 48 7.0 0.79 1.6 0.61 0.007 0.01 pcrush/GPa plock/GPa D1 D2 K1/GPa K2/GPa K3/GPa vp/(m·s−1) vs/(m·s−1) 0.016 0.8 0.04 1 85 −171 208 3872 2375 表 2 围岩的物理力学参数Table 2. Physical and mechanical parameters of surrounding rock\,\rho /(kg·m–3) E/GPa \nu q{_{\phi}}/(°) K{_{\phi}} q{_{\varPsi} }/(°) \sigma t/kPa vp/(m·s−1) vs/(m·s−1) 1850 0.04 0.35 0.388 11171 0 0.1 185 89 表 3 空气(空模型)的物理力学参数Table 3. Physical and mechanical parameters of air (air model)\,\rho /(kg·m–3) c/(m·s−1) E0/(MJ·m–3) \kappa vp/(m·s−1) vs/(m·s−1) 1.29 340 0 1.4 340 0 2.3 固体爆炸模型建立
JWL状态方程已广泛应用于以TNT炸药为代表的固体炸药爆炸过程模拟[13],且有大量的实验参数可供参考。JWL状态方程为
p = {A_{\rm {JWL}}}\left(1 - \frac{\omega }{{{R_1}V}}\right){{\rm e}^{ - {R_1}V}} + {B_{\rm {JWL}}}\left(1 - \frac{\omega }{{{R_2}V}}\right){{\rm e}^{ - {R_2}V}} + \frac{{\omega e}_0}{V} (8) 式中:e0为单位体积炸药的初始内能,
\omega 、AJWL、BJWL、R1和R2为JWL状态方程参数,取值见表4,其中:\,\rho_0 为初始密度,pCJ为CJ爆轰压力,\gamma 为爆轰产物的绝热指数,DJ为爆速。表 4 固体爆炸物参数Table 4. Calculation parameters of solid explosives\,\rho 0/(kg·m–3) e0/(GJ·m–3) pCJ/GPa \gamma DJ/(m·s–1) 1500 7.0 21 2.727 6930 AJWL/GPa BJWL/GPa R1 R2 \omega 371.2 3.23 4.15 0.95 0.30 本研究参考李志鹏[14]在ALE模拟中所用体积填充法的装药网格数目,选用的基础模型中爆炸物由8个质点构成,每个质点的质量为50 kg,替换相应体积的空气,炸药爆炸的TNT当量为444.4 kg[15]。爆炸物位于站台底板处,以模拟恐怖袭击中常见的固体爆炸装置置于站台层底板的爆炸情况。
3. 爆炸作用下站台及围岩响应规律分析
3.1 爆炸作用下站台及围岩的响应压强
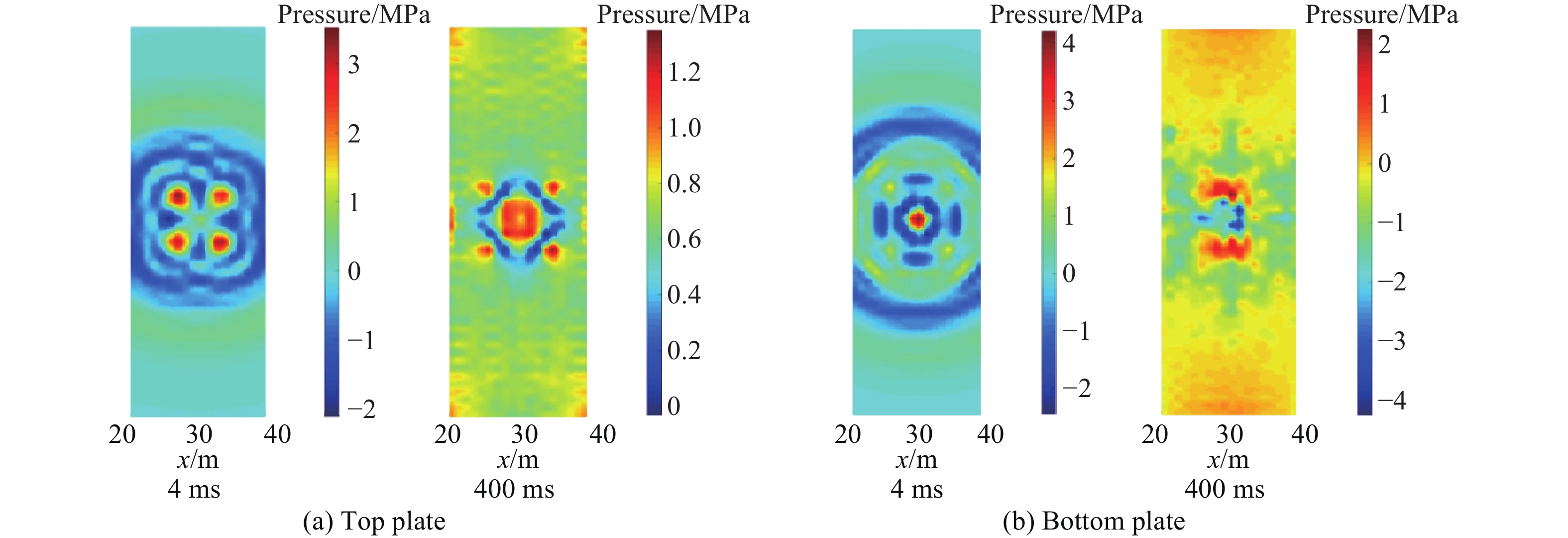
图6为爆炸发生后4和400 ms时站台无柱区域顶板和底板的压强分布云图。随着爆炸后时间的增加,顶板和底板的响应压强峰值均逐渐减小。顶板压强在爆炸后出现应力集中现象,这是由起爆后爆炸物粒子撞击结构导致的,撞击点偏离了起爆点在结构顶板上的投影位置,当爆炸物被继续细化时,这种偏离距离会消失。响应压强以应力波的形式从起爆点向外辐射传播,使得站台结构内部既存在正压强也出现负压强,即站台底板和顶板既存在受压区也存在受拉区。400 ms时顶板中心处出现正压强,这是由爆炸应力波向上传递到大厅底板后反射回来的反射波导致的;而底板中心处正压强与负压强分布不规则是由于在爆炸作用下底部围岩出现塌陷坑,由底部地质界面反射回来的应力波又发生多次反射,使得结构底板压强分布不规律。
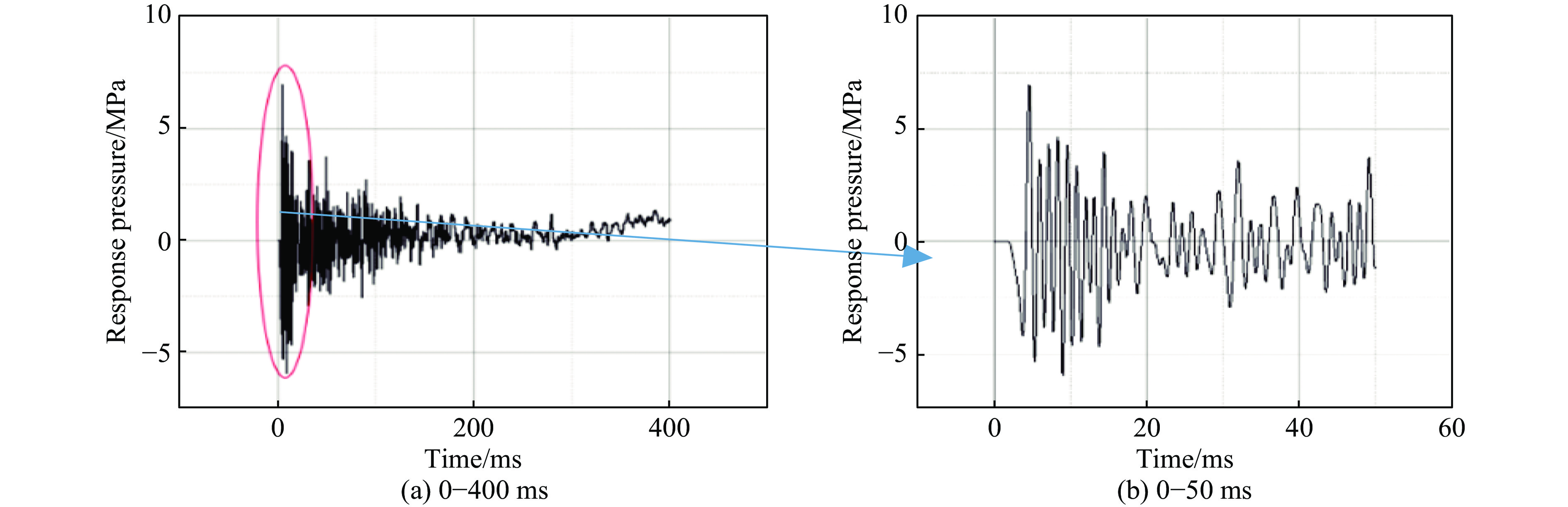
图7为站台结构顶板中心质点压强随时间变化曲线。爆炸应力波在起爆后1.6 ms时到达顶板,4.5 ms时顶板质点压强出现峰值,为6.97 MPa,此后压强峰值和振幅快速减小,这是由于应力波在围岩-站台结构和站台结构-空气两个界面之间不断传播和反射,能量快速损耗,响应压强迅速减小。
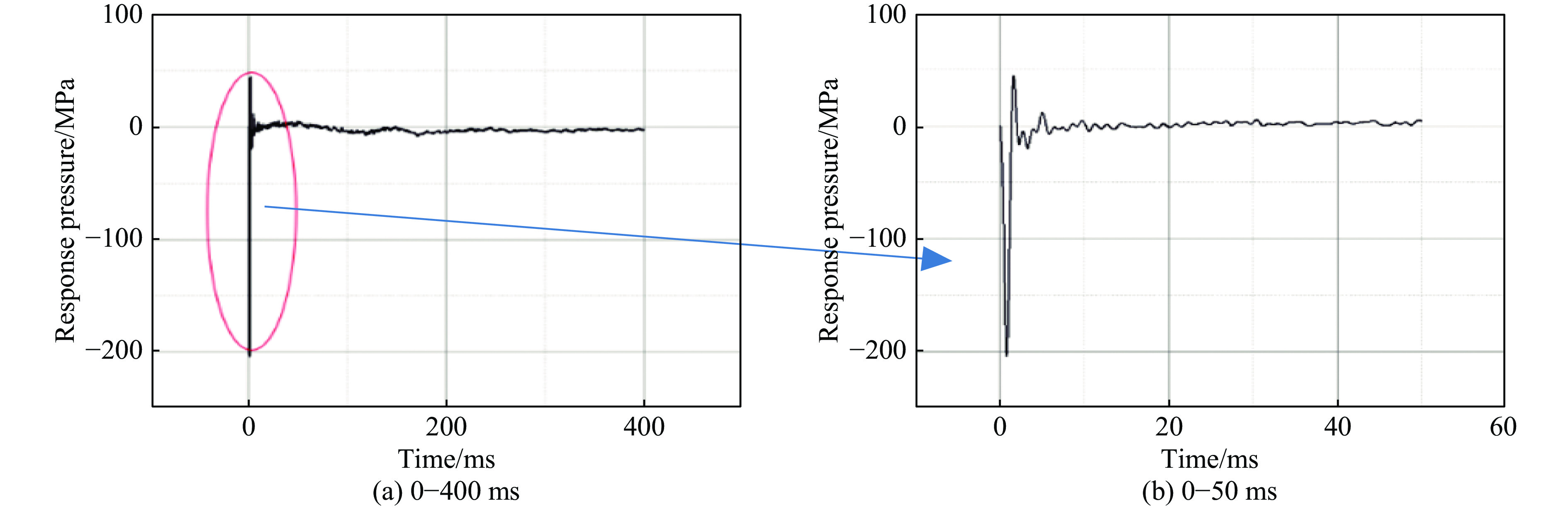
图8为站台结构底板中心质点压强随时间变化曲线。从图8可以看出,爆炸应力波在起爆后0.2 ms时到达顶板, 7.0 ms时达到峰值200 MPa,随后迅速减小并在0 kPa附近振荡,这是由于起爆后底板质点区域下方的围岩形成塌陷坑,使该质点处于悬空状态,不受反射波影响,因此响应压强迅速减小。底板质点先于顶板质点1.1 ms受到爆炸作用,结合站台层高,可以求得爆炸应力波的传播速度为4615 m/s,小于文献[16]中描述的5800~7000 m/s,主要原因是离散的空气质点减缓了传播速度。
3.2 爆炸作用下站台净空内爆炸超压分析
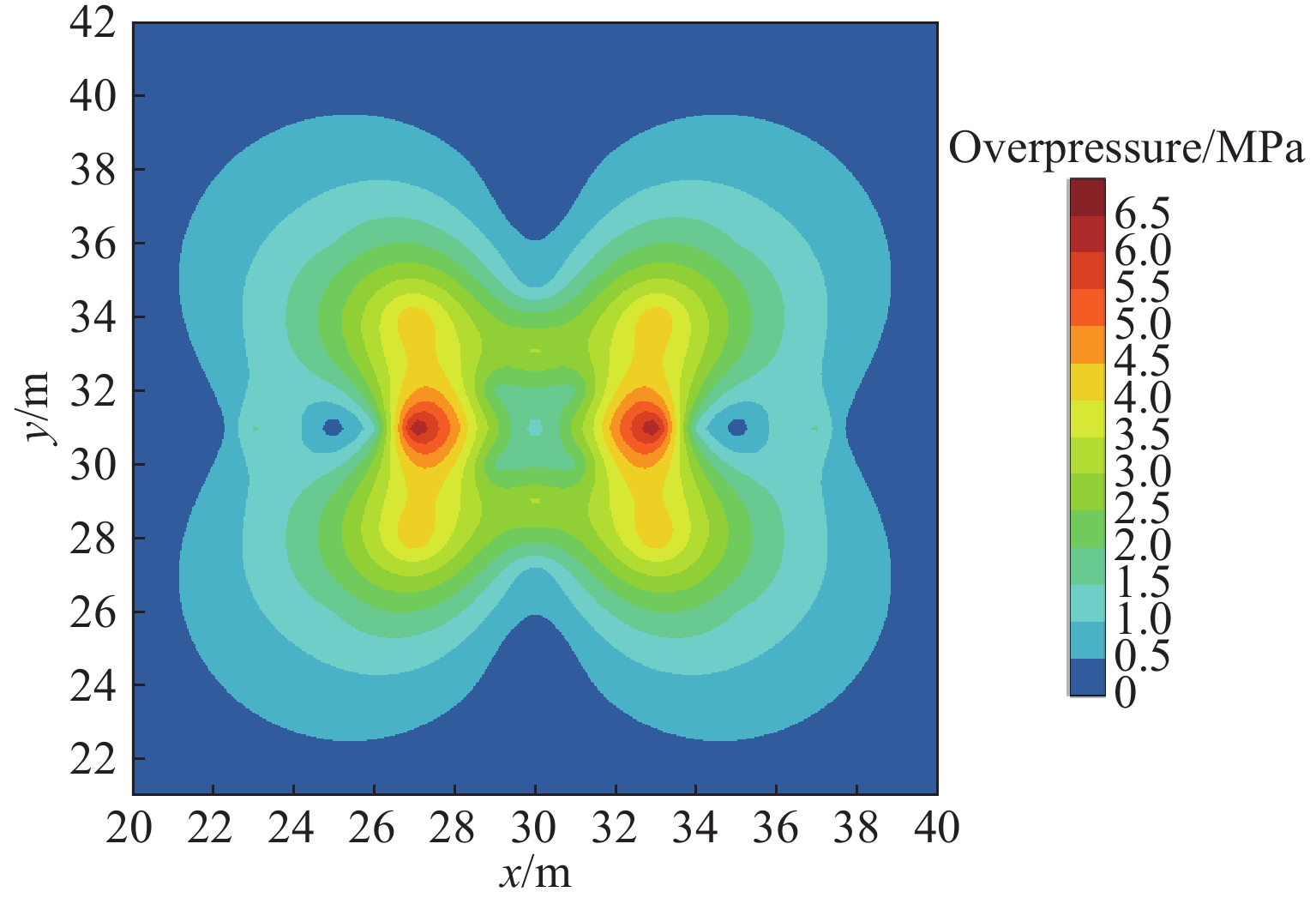
根据距地面3.0 m高的监测数据,采用Kriging插值法,计算得到爆炸过程中起爆点附近的超压峰值分布,如图9所示。可以看出,爆炸产生的超压峰值出现在纵向上偏离站台中轴线2.5 m处,即出现超压峰值偏移,这是由于爆炸物位于站台底部,起爆后冲击波波阵面呈半球形,与3.0 m高平面的交点与起爆点有一定的偏移距离。图9中爆炸超压峰值为6.5 MPa,且在起爆点横向上出现超压峰值突变情况,这是由于初始爆轰应力波传递至隧道边墙后产生反射,与后续爆轰反应形成的应力波叠加,致使横向超压峰值发生突变。在以往研究中,站台净空爆炸超压峰值分布计算结果未出现这样的突变区域,其原因在于他们仅考虑了结构净空内压力分布,将结构-围岩界面简化为无反射边界,忽略了应力波由结构传递至围岩时发生的折射和反射现象[17]。
Henrych[18]、Brode[19]、Sadovskyi[20]等通过理论推导提出了不同适用范围超压峰值ps的经验公式。Wu等[21]基于前人的研究,提出了更加精确的超压峰值公式
{p_{\rm {s}}} = \left\{ \begin{gathered} \frac{{{1.059}}}{{{{\bar R}^{2.56}}}} - {0.051}\quad{\text{ 0}}{\text{.1 }}\leqslant\bar R \leqslant {1.0} \hfill \\ \frac{{{1.008}}}{{{{\bar R}^{2.01}}}}\quad\quad\quad\quad\;{1.0} < \bar R \leqslant {10.0} \hfill \\ \end{gathered} \right. (9) 式中:ps的单位为MPa;
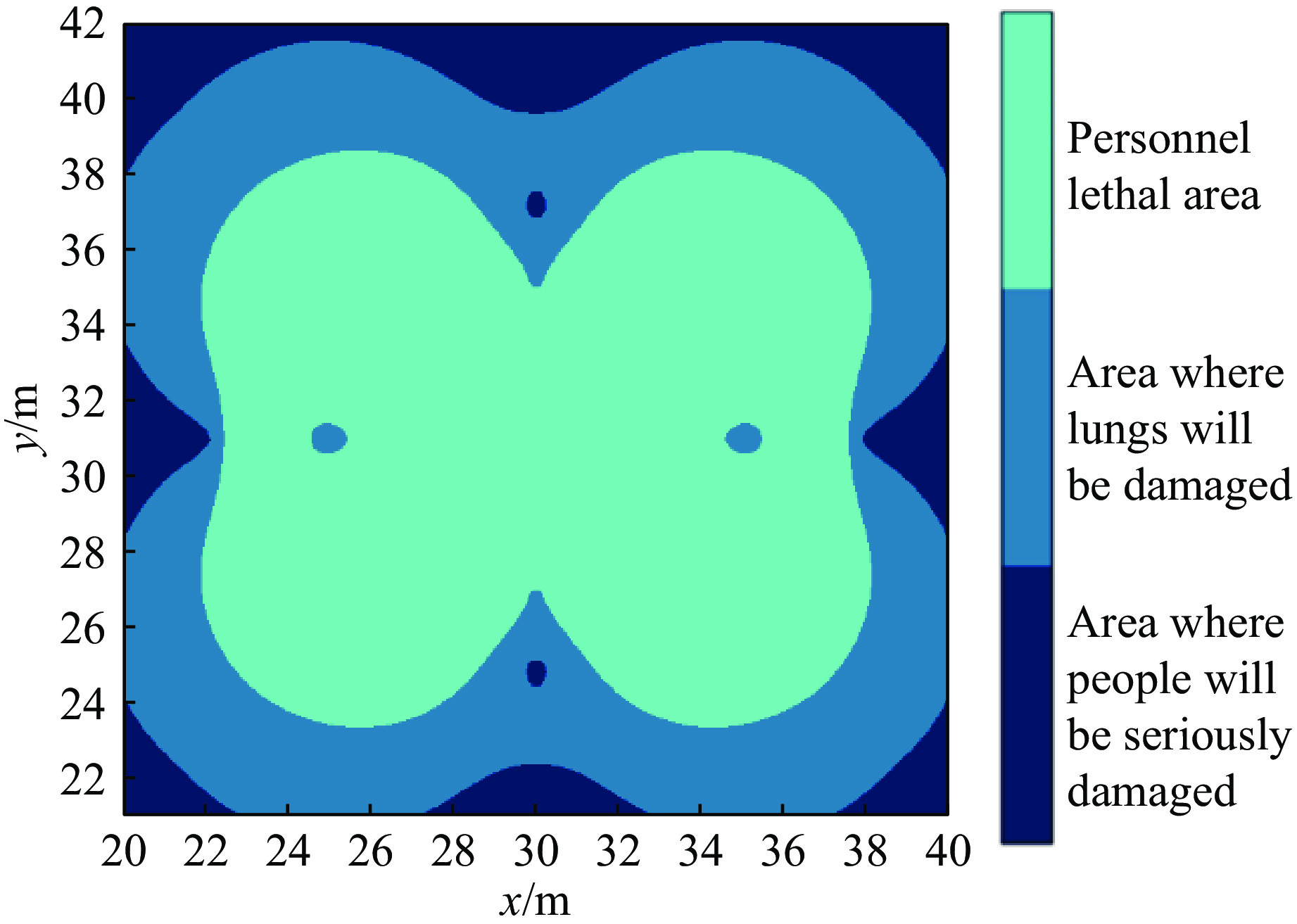
\bar R 为比例距离,\bar R = R/{W^{1/3}} ,其中W为装药量(单位kg),R为距起爆点距离(单位m)。表5列出了本研究模拟得到的5和10 m范围内的超压峰值与式(9)的计算结果,可见二者相近,进一步验证了本研究提出的数值模拟方法的准确性。表 5 超压模拟结果与经验公式计算结果对比Table 5. Comparison of overpressure simulation results and empirical formula calculation resultsDistance/m Overpressure/MPa Error/% Theoretical formula Numerical simulation 5 2.35 2.25 4.44 10 0.47 0.52 −7.69 为评价爆炸对不同范围内人员的损伤情况,基于师光达[22]提出的爆炸对人员伤亡损失评价标准和图9,建立了人员伤亡区域云图,如图10所示。可见,人员致死区域是半径为8 m、面积为200 m2的蝴蝶形区域,实际爆炸造成的伤亡区域面积远大于此范围,这是由于爆炸过程中产生的飞散弹片以及后期救治不及时造成的[23]。
3.3 爆炸作用下站台结构及围岩位移
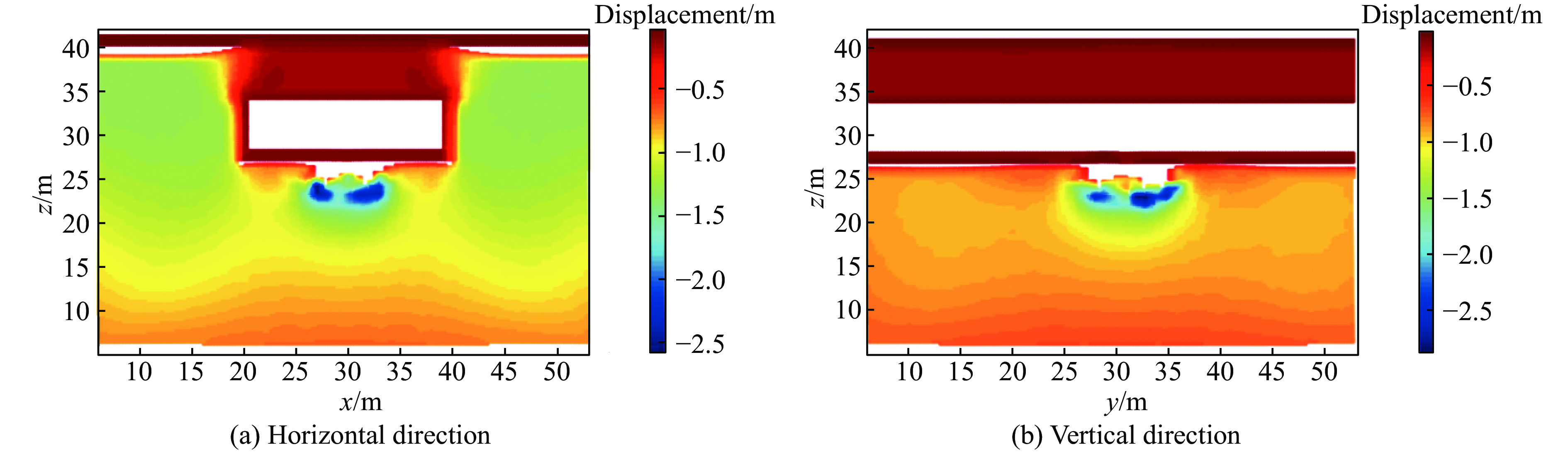
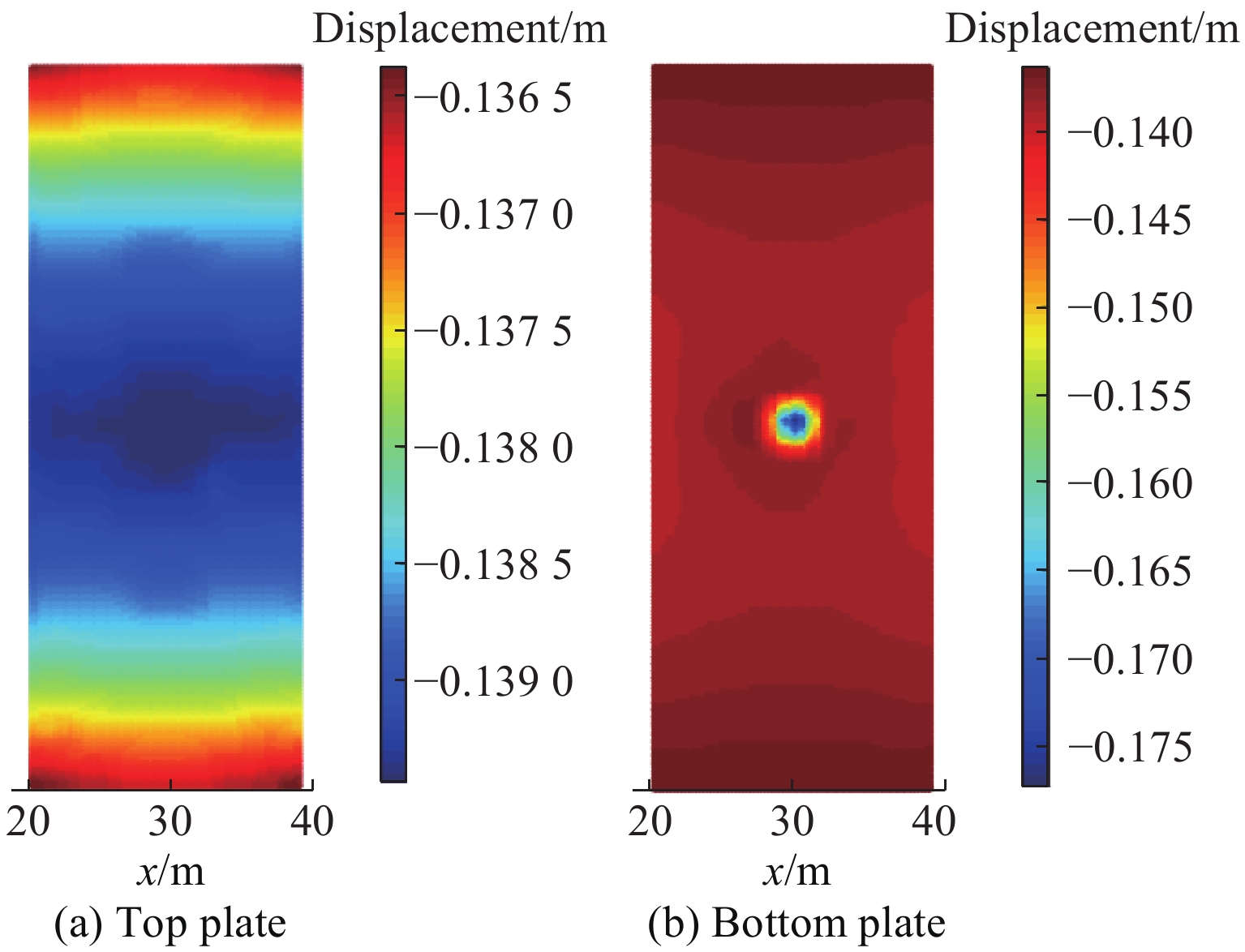
图11为起爆后400 ms时围岩和车站结构位移分布云图。从图11中可以看出,爆炸作用使站台结构发生整体沉降,最大沉降位移为2.57 m,起爆点下方围岩出现深度为1.64 m的凹陷深坑。站台上下覆围岩均与大厅底板或站台底板分离。图12为爆炸发生400 ms时站台结构顶板和底板竖向位移分布云图。可见,横向上顶板位移分布较一致,纵向上则随着与起爆点距离的增加而逐渐减小;底板竖向位移在横向和纵向上均随距起爆点距离的增加而减小,起爆点附近的底板位移急剧增大,并且随着时间的增加,该处质点位移继续增大,该区域发生明显破裂。以下结合爆炸后应力波的传递情况,对站台结构和围岩位移进行分析。
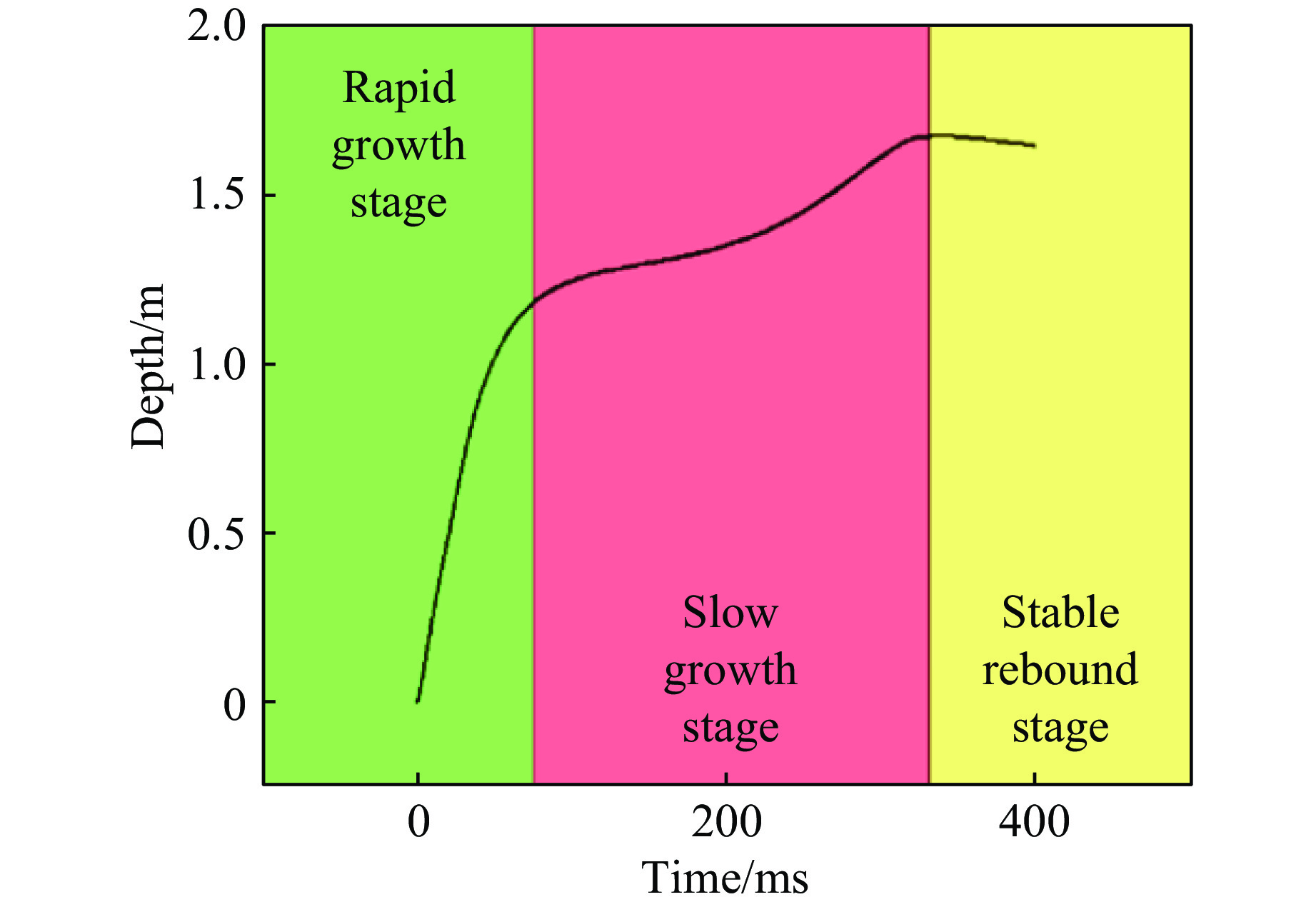
爆炸物起爆后,空气冲击波波阵面扩散到站台本体结构,此时本体结构受力发生振动并向围岩传递应力波。应力波产生的原因有两个:一是飞散的爆炸产物直接撞击本体结构,二是气流冲击波冲击至本体结构。爆炸应力波向下透过站台底板传至围岩,使其产生大量向下的位移,由于围岩之间的黏结力,站台侧向围岩也向下运动,因此站台结构发生整体沉降。与此同时,因站台底板受损,应力波向下传播较为集中,使得起爆点下方围岩产生较大变形,局部凹陷。图13显示了塌陷坑深度随时间的变化规律。0~80 ms内,受初始应力波的作用,塌陷坑迅速下沉;80~320 ms内,反射应力波传递至围岩与站台底板接触面,降低围岩的下降速度,此时塌陷坑深度进入缓慢增长阶段。另外,当站台底板与围岩整体沉降时,由于围岩的弹性模量较小,其沉降位移比站台结构大,因此围岩与站台底板发生分离。
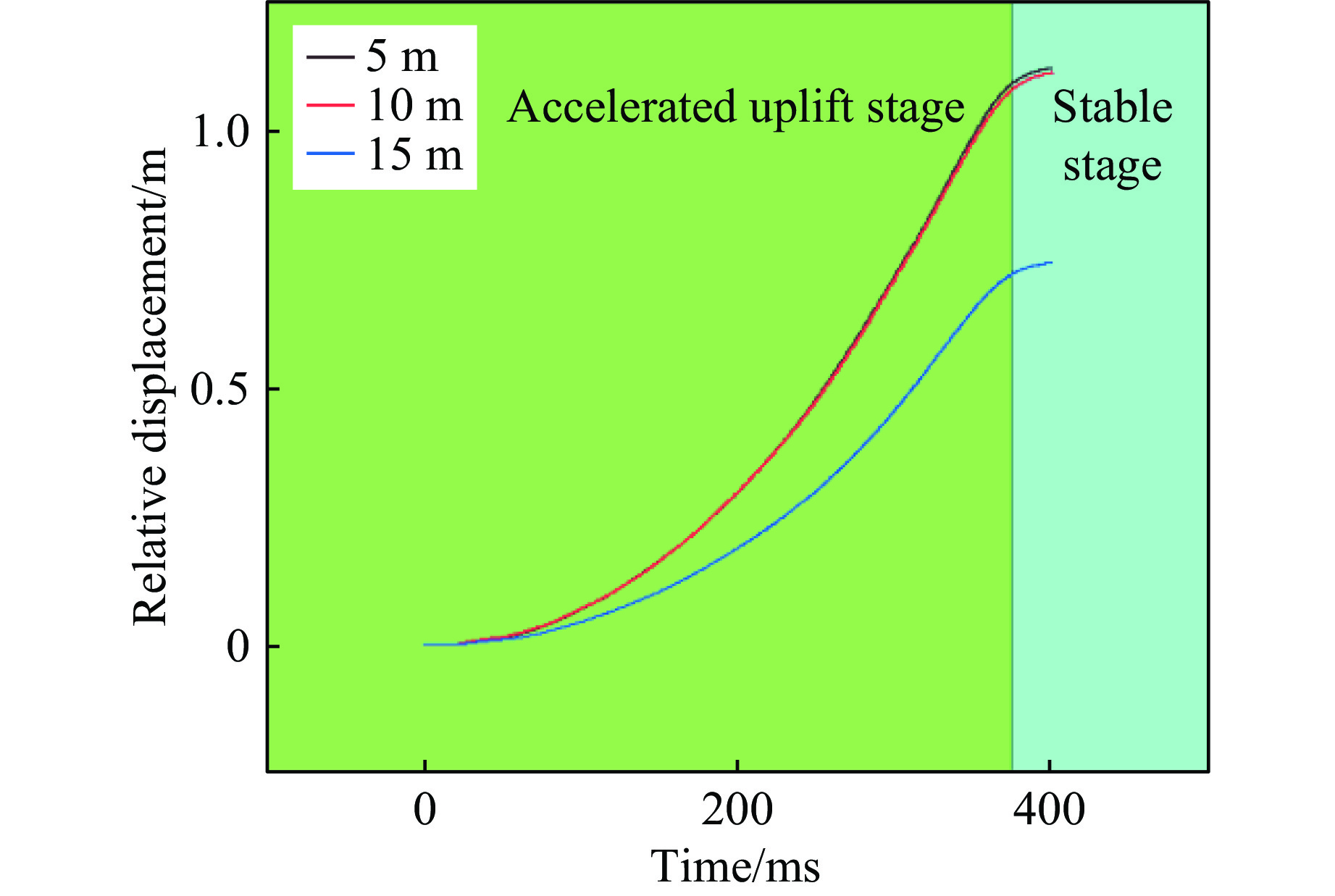
站台上方围岩和大厅底板在向上传播的冲击波作用下相对于周围向上隆起,且大厅底板和围岩发生分离,这是因为冲击波传播至围岩与车站大厅底板接触面时发生反射和折射,反射冲击波将减缓围岩整体向上隆起的速度。取距离起爆点20 m处质点为参考点,分析站台纵向距起爆点5、10、15 m处围岩表面质点的相对位移随时间变化曲线,如图14所示。可以看出,各质点的相对位移随时间的增加呈现相似的平滑增加规律。首先0~350 ms内,在爆炸冲击波及其次生波的作用下,围岩加速隆起;在350~400 ms内,由于界面之间的反射和黏弹性边界的吸收,应力波能量全部耗散,围岩的位移也趋于稳定。
3.4 站台本体结构损伤因子
站台结构的受损部位主要集中于靠近爆炸物的车站本体结构底板,为此,本研究着重分析结构底板受损因子的分布规律。
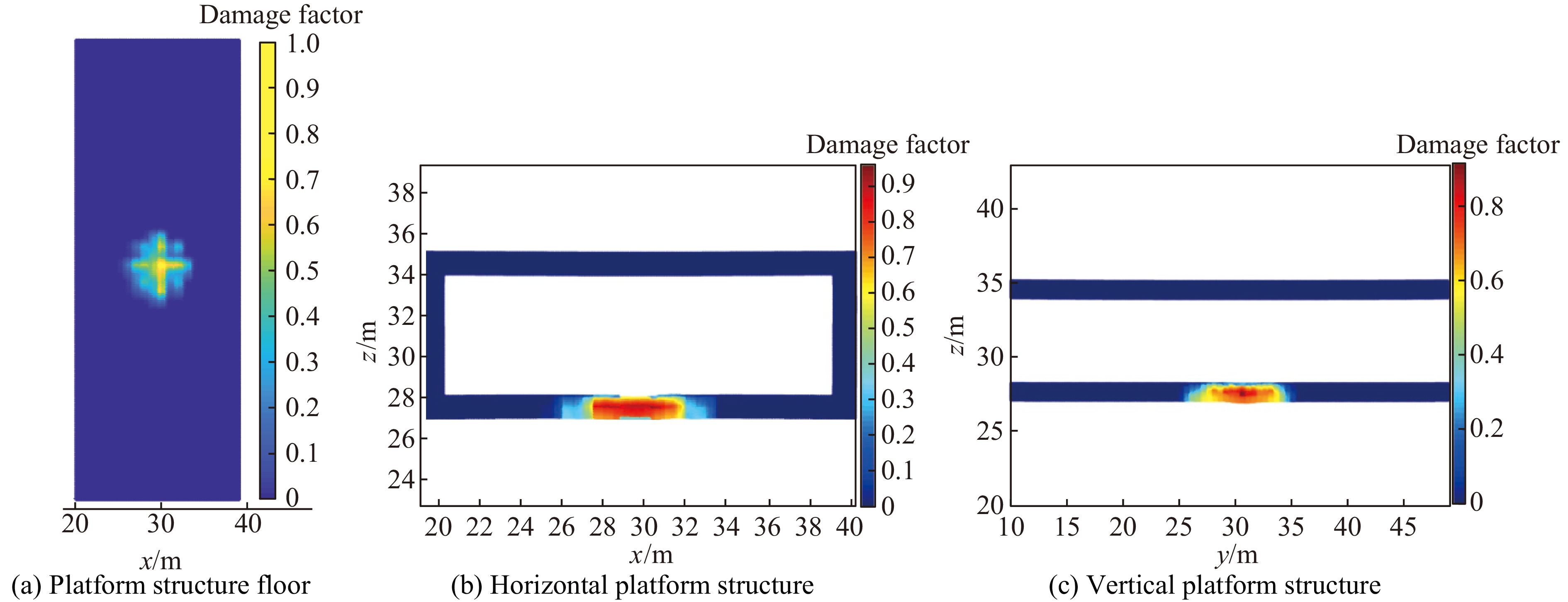
图15为爆炸产生的本体结构受损因子分布云图。可以看出,受损区域呈椭圆形,其中椭圆长轴平行于站台纵向,这是由于站台横向尺寸小于纵向尺寸,竖向墙壁结构增加了横向上站台的稳定性,使其受损情况较纵向上的站台轻。图15中出现了集中损伤区域,这是由于爆炸产物直接冲击本体结构造成的,而均布损伤区域是由空气冲击波冲击产生的。此外,当质点受损因子达到1时会被判定为失效,此时底板有效承重区域分布不规则,致使后续质点受损因子的计算结果出现不均质性。
在站台横纵截面受损因子分布云图中,横纵向受损因子均集中在固体爆炸物正下方的结构底板,且在截面上损伤因子上小下大,底板上表面的受损程度小于底板下表面的受损程度,此外,由于结构底板内应力波在上下表面内反复反射叠加,导致中部区域受损最严重。通过底板截面受损区域的梯形分布,可以看出应力波在结构内的传播趋势。
3.5 站台有柱区域与无柱区域的爆炸响应对比
表6列出了站台有柱区域和无柱区域在固体爆炸物爆炸作用下结构围岩、净空超压等各项指标的对比,其中:pm为净空超压峰值,Rd为净空超压致死区半径,nd为结构受损因子峰值,Cd为结构受损面积,pr、pf1、pf2分别为结构顶板、结构底板和大厅底板的响应压强峰值,dr、df1、df2分别为结构顶板、结构底板、大厅底板的位移峰值,dp为围岩塌陷坑深度。表6中,站台有柱区域的pm、Rd、nd、Cd、pf1、df1、dp均较无柱区域有所下降,但pr、dr、pf2、df2有所增加。这是由于结构柱增加了整个结构体系的刚度,使大厅底板和站台结构顶板分担了炸药对站台结构底板的冲击作用,提升了站台结构的抗爆能力。此外,结构柱的存在增加了站台结构封堵率,削弱了爆炸冲击波的传播,导致超压致死区半径减小。
表 6 站台有柱区域和无柱区域在固体爆炸作用下结构参数的对比Table 6. Comparison of various parameters under the solid explosion in the pillared and non-pillared areas of the platformArea pm/MPa Rd/m nd Cd/m2 pr/MPa pf1/MPa With volumns 6.5 8 1.00 21 5.025 218.0 Without volumns 6.0 5 0.67 13 5.667 110.5 Error/% −7.69 −37.50 −33.00 −38.10 12.78 −49.31 Area pf2/MPa dr/m df1/m df2/MPa dp/m With volumns 0.034 0.124 0.188 0.037 1.64 Without volumns 0.041 0.084 0.103 0.089 0.43 Error/% 20.59 −32.26 −45.21 140.54 −73.78 通过各项数据的对比分析可以看出,站台有柱区域结构整体性优于无柱区域,抗爆能力更强。
4. 结 论
将HJC模型嵌入开源版物质点法程序,研究了爆炸荷载作用下地铁站台及围岩的压强、位移、净空超压和结构受损的响应规律,得到如下主要结论。
(1) 结构在爆炸应力波作用下既存在受拉区又存在受压区;应力波在围岩-站台结构和站台结构-空气两个界面之间不断传播和反射,能量逐渐损耗,压强逐渐减小;空间内超压分布存在峰值偏移,同时在站台边墙处应力波与反射波叠加出现超压突变区;爆炸超压致死区域半径约为8 m。
(2) 结构体系在爆炸作用下会发生整体沉降,起爆点上方围岩和大厅地板相对周围向上隆起,起爆点正下方围岩出现塌陷坑,上下覆围岩短时间内会出现与站台结构体脱离的情况。
(3) 结构受损区域主要集中在结构底板,受损区域呈椭圆形,底板中部受损程度最严重,上表面的受损程度小于下表面的受损程度,站台有柱区域的抗爆能力强于无柱区域。
-
表 1 混凝土结构的HJC模型参数
Table 1. HJC model parameters of concrete structure
\,\rho /(kg·m–3) E/GPa \nu { f{'_ {\rm{c} } } }/MPa Smax A B N C \varepsilon {_{\min }^{\rm f} } 2439 32.5 0.2 48 7.0 0.79 1.6 0.61 0.007 0.01 pcrush/GPa plock/GPa D1 D2 K1/GPa K2/GPa K3/GPa vp/(m·s−1) vs/(m·s−1) 0.016 0.8 0.04 1 85 −171 208 3872 2375 表 2 围岩的物理力学参数
Table 2. Physical and mechanical parameters of surrounding rock
\,\rho /(kg·m–3) E/GPa \nu q{_{\phi}}/(°) K{_{\phi}} q{_{\varPsi} }/(°) \sigma t/kPa vp/(m·s−1) vs/(m·s−1) 1850 0.04 0.35 0.388 11171 0 0.1 185 89 表 3 空气(空模型)的物理力学参数
Table 3. Physical and mechanical parameters of air (air model)
\,\rho /(kg·m–3) c/(m·s−1) E0/(MJ·m–3) \kappa vp/(m·s−1) vs/(m·s−1) 1.29 340 0 1.4 340 0 表 4 固体爆炸物参数
Table 4. Calculation parameters of solid explosives
\,\rho 0/(kg·m–3) e0/(GJ·m–3) pCJ/GPa \gamma DJ/(m·s–1) 1500 7.0 21 2.727 6930 AJWL/GPa BJWL/GPa R1 R2 \omega 371.2 3.23 4.15 0.95 0.30 表 5 超压模拟结果与经验公式计算结果对比
Table 5. Comparison of overpressure simulation results and empirical formula calculation results
Distance/m Overpressure/MPa Error/% Theoretical formula Numerical simulation 5 2.35 2.25 4.44 10 0.47 0.52 −7.69 表 6 站台有柱区域和无柱区域在固体爆炸作用下结构参数的对比
Table 6. Comparison of various parameters under the solid explosion in the pillared and non-pillared areas of the platform
Area pm/MPa Rd/m nd Cd/m2 pr/MPa pf1/MPa With volumns 6.5 8 1.00 21 5.025 218.0 Without volumns 6.0 5 0.67 13 5.667 110.5 Error/% −7.69 −37.50 −33.00 −38.10 12.78 −49.31 Area pf2/MPa dr/m df1/m df2/MPa dp/m With volumns 0.034 0.124 0.188 0.037 1.64 Without volumns 0.041 0.084 0.103 0.089 0.43 Error/% 20.59 −32.26 −45.21 140.54 −73.78 -
[1] 城市轨道交通2017年度统计和分析报告 [J]. 城市轨道交通, 2018(4): 6−25.Statistics and analysis report of urban rail transit in 2017 [J]. China Metros, 2018(4): 6−25. [2] 王勇, 王德荣, 陈灿寿, 等. 某地铁区间隧道内爆炸效应的数值模拟 [J]. 防护工程, 2006, 28(6): 55–58.WANG Y, WANG D R, CHEN C S, et al. Numerical simulation on interior blasting effect in the section of tunnel in some subway [J]. Protective Engineering, 2006, 28(6): 55–58. [3] CHOI S, WANG J, MUNFAKH G, et al. 3D nonlinear blast model analysis for underground structures [C]//GeoCongress 2006. Atlanta, Georgia, USA: ASCE, 2006. [4] ZHANG X X, CHENG J W, SHI C L, et al. Numerical simulation studies on effects of explosion impact load on underground mine seal [J]. Mining, Metallurgy & Exploration, 2020, 37(2): 665–680. doi: 10.1007/s42461-019-00143-2 [5] 柴永生, 王月桂, 章毅. 地铁口部爆炸冲击波传播规律与超压荷载研究 [J]. 防护工程, 2019, 41(1): 42–46.CHAI Y S, WANG Y G, ZHANG Y. Study on propagation of blast wave and the overpressure load subjected to metro entrance explosion [J]. Protective Engineering, 2019, 41(1): 42–46. [6] 王桂林, 欧阳啸天, 翟俊, 等. 浅埋三舱管廊甲烷爆炸的地面响应规律 [J]. 高压物理学报, 2021, 35(1): 015202. doi: 10.11858/gywlxb.20200616WANG G L, OUYANG X T, ZHAI J, et al. Ground response law of methane explosion in shallow buried three-cabin pipe gallery [J]. Chinese Journal of High Pressure Physics, 2021, 35(1): 015202. doi: 10.11858/gywlxb.20200616 [7] 张雄, 廉艳平, 刘岩, 等. 物质点法 [M]. 北京: 清华大学出版社, 2013: 31−32.ZHANG X, LIAN Y P, LIU Y, et al. Material point method [M]. Beijing: Tsinghua University Press, 2013: 31−32. [8] 陈卫东, 杨文淼, 张帆. 基于物质点法的水下爆炸冲击波数值模拟 [J]. 高压物理学报, 2013, 27(6): 813–820. doi: 10.11858/gywlxb.2013.06.004CHEN W D, YANG W M, ZHANG F. Material point method for numerical simulation of underwater explosion blast wave [J]. Chinese Journal of High Pressure Physics, 2013, 27(6): 813–820. doi: 10.11858/gywlxb.2013.06.004 [9] 王宇新, 陈震, 张洪武, 等. 多层抗爆结构冲击响应无网格MPM法分析 [J]. 工程力学, 2007, 24(12): 186–192. doi: 10.3969/j.issn.1000-4750.2007.12.032WANG Y X, CHEN Z, ZHANG H W, et al. Response of multi-layered structure due to impact load using material point method [J]. Engineering Mechanics, 2007, 24(12): 186–192. doi: 10.3969/j.issn.1000-4750.2007.12.032 [10] 张芮瑜, 孙玉进, 宋二祥. 强夯的物质点法模拟及其能量转化规律分析 [J]. 岩土工程学报, 2019, 41(7): 1208–1216. doi: 10.11779/CJGE201907004ZHANG R Y, SUN Y J, SONG E X. Simulation of dynamic compaction using material point method and analysis of its energy conversion law [J]. Chinese Journal of Geotechnical Engineering, 2019, 41(7): 1208–1216. doi: 10.11779/CJGE201907004 [11] 董友扣, 马家杰, 王栋, 等. 深海滑坡灾害的物质点法模拟 [J]. 海洋工程, 2019, 37(5): 141–147. doi: 10.16483/j.issn.1005-9865.2019.05.016DONG Y K, MA J J, WANG D, et al. Investigation of landslide in deep sea using material point method [J]. The Ocean Engineering, 2019, 37(5): 141–147. doi: 10.16483/j.issn.1005-9865.2019.05.016 [12] HANCHAK S J, FORRESTAL M J, YOUNG E R, et al. Perforation of concrete slabs with 48 MPa (7 ksi) and 140 MPa (20 ksi) unconfined compressive strengths [J]. International Journal of Impact Engineering, 1992, 12(1): 1–7. doi: 10.1016/0734-743X(92)90282-X [13] 李科斌, 董新龙, 李晓杰, 等. 水下爆炸实验法在工业炸药JWL状态方程测定中的应用研究 [J]. 兵工学报, 2020, 41(3): 488–494. doi: 10.3969/j.issn.1000-1093.2020.03.009LI K B, DONG X L, LI X J, et al. Research on parameters determination of JWL EOS for commercial explosives based on underwater explosion test [J]. Acta Armamentarii, 2020, 41(3): 488–494. doi: 10.3969/j.issn.1000-1093.2020.03.009 [14] 李志鹏. 瓦斯爆炸作用下隧道衬砌致损机理及修复技术研究 [D]. 北京: 北京科技大学, 2019.LI Z P. Study on damage mechanism and repair technology of tunnel lining subject to gas explosion [D]. Beijing: University of Science and Technology, 2019. [15] 涂国勇, 王海锋, 禄晓飞, 等. 整体爆破弹头爆炸当量定量评价研究 [J]. 现代防御技术, 2020, 48(2): 30–34. doi: 10.3969/j.issn.1009-086x.2020.02.005TU G Y, WANG H F, LU X F, et al. Explosion equivalent quantitative evaluation of global blowup warhead [J]. Modern Defense Technology, 2020, 48(2): 30–34. doi: 10.3969/j.issn.1009-086x.2020.02.005 [16] 张程娇. 炸药爆轰产物参数的特征线差分反演方法研究 [D]. 大连: 大连理工大学, 2016.ZHANG C J. Research of inversion method of detonation products physical parameters based on modified method of characteristics [D]. Dalian: Dalian University of Technology, 2016. [17] 闫秋实, 刘晶波, 伍俊. 典型地铁车站内爆炸致人员伤亡区域的预测研究 [J]. 工程力学, 2012, 29(2): 81–88.YAN Q S, LIU J B, WU J. Estimation of casualty areas in subway station subjected to terrorist bomb [J]. Engineering Mechanics, 2012, 29(2): 81–88. [18] HENRYCH J. The dynamics of explosion and its use [M]. Amsterdam: Elsevier Scientific Publishing Company, 1979: 178−181. [19] BRODE H L. Blast wave from a spherical charge [J]. Physics of Fluids, 1959, 2(2): 217. doi: 10.1063/1.1705911 [20] SADOVSKYI M A. Mechanical action of air shock waves of explosion based on experimental data [M]. Moscow: Izd Akad Nauk SSSR, 1952. [21] WU C Q, HAO H. Modeling of simultaneous ground shock and airblast pressure on nearby structures from surface explosions [J]. International Journal of Impact Engineering, 2005, 31(6): 699–717. doi: 10.1016/j.ijimpeng.2004.03.002 [22] 师光达. 化工园区危险性评价研究 [D]. 沈阳: 沈阳理工大学, 2020.SHI G D. Study on risk assessment of chemical industry park [D]. Shenyang: Shenyang Ligong University, 2020. [23] 王新建. 爆炸中缺口效应及其防护研究 [J]. 中国人民公安大学学报(自然科学版), 2008, 14(3): 88–90.WANG X J. Study on explosion notch effect and its protecton [J]. Journal of Chinese People’s Public Security University (Science and Technology), 2008, 14(3): 88–90. -







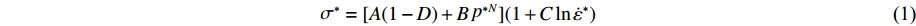
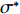
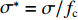
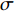
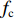
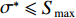

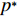
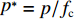
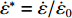
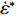
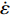
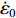
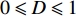
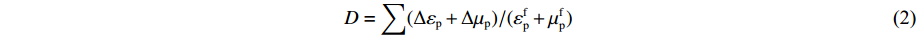
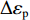
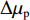
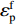

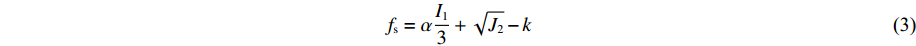
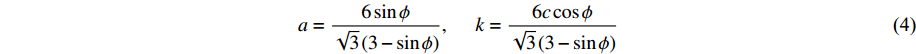
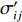
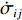
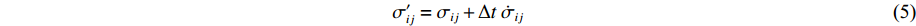
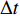
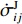
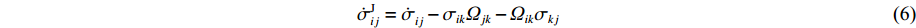
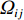
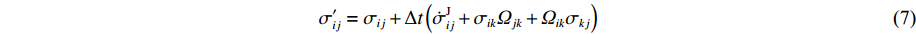

 下载:
下载:




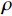
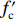
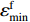
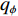
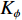
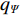

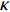
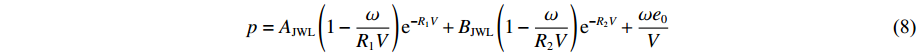
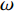

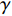




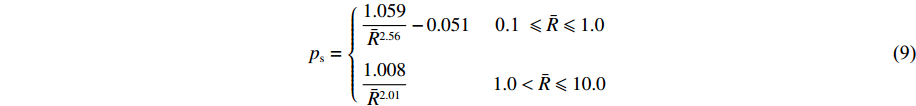

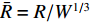







 下载:
下载: