Response Law of Subway Platform and Surrounding Rock under Solid Explosion
-
摘要: 地铁站内发生爆炸将造成巨大的人员伤亡和财产损失。依托上海某地铁站工程,将HJC模型嵌入开源物质点法程序中,研究了固体炸药爆炸作用下地铁站台及围岩的响应规律。结果表明:受爆炸应力波的影响,站台顶板和底板响应压强在短时间内达到峰值后迅速降低,站台结构在爆炸过程中既存在受拉区又存在受压区;在站台边墙处,由于应力波与反射波叠加,会出现超压突变区;爆炸作用使站台结构整体下沉,且起爆点正下方围岩会形成塌陷坑,起爆点正上方围岩和车站结构相对周围向上隆起;结构受损区域主要集中在结构底板,呈椭圆形;站台有柱区域的抗爆能力强于无柱区域。Abstract: An explosion in the subway station will lead to huge losses of personnel and property. In order to study the response law of subway platform and surrounding rock of a subway station project in Shanghai under the action of solid explosive explosion, HJC model is embedded in the open source material point method program. The results show that the response pressure of platform roof and floor will decrease rapidly after reaching the peak in a short time under the influence of explosion wave, and the platform structure forms tension zone and compression zone during explosion. Due to the superposition of stress wave and reflected wave, overpressure sudden change zone will appear at the platform side wall. The whole platform structure sink under explosion. Moreover, the surrounding rock directly below the detonation point forms a collapse pit, and the surrounding rock directly above the detonation point and the station structure rises up. The damaged area is mainly concentrated in the bottom plate of the structure, and the damaged area is oval. The blast resistance of platform with column area is stronger than that without column area.
-
Key words:
- subway platform /
- HJC model /
- explosion /
- structural response /
- material point method
-
Nanoscale inorganic materials with unique electrical, optical and mechanical properties, which are distinct from their bulk counterparts, attract attention in both fundamental scientific research and industrial applications[1-3]. Investigations on the phase transformation and the structural stability of nanomaterials under high pressure are conducted in physics, materials science, geophysics[4-9]. Numerous studies on nanomaterials under high pressure have revealed that the grain size plays an important role in the pressure-induced phase transition behaviors. A number of interesting high-pressure behaviors and new properties in nanomaterials appear when the grain size is smaller than a critical size. Studies on CdSe, CdS, ZnO and ZnS nanocrystals observe the size-dependent phase transformations[10-13]. Lv, et al. [14] reports that the Mn3O4 nanoparticles show a different phase transformation route and a new high-pressure phase at 14.5–23.5 GPa, which has been recognized to be the orthorhombic CaTi2O4-type structure. The crystalline-amorphous transition is discovered in materials such as Y2O3, TiO2, PbTe and BaF2[15-19]. Therefore, high-pressure studies on nanomaterials are significant for the discovery of new structures and properties of materials.
Calcium fluoride (CaF2), a typical face-centered-cubic ionic crystal, has been widely used in many fields[2-3]. Due to its simple crystal structure, CaF2 becomes an ideal material for high-pressure research. High-pressure X-ray diffraction (XRD) and Raman spectroscopy studies of bulk CaF2 have shown that it undergoes two structural transitions and finally reaches highly coordinated structures. The pressure-induced phase transition from the fluorite structure (space group: Fm3m) to the α-PbCl2-type structure (space group: Pnma) has been found in the pressure range of 8–10 GPa[20-21]. Dorfman, et al.[22] have reported that bulk CaF2 transforms from the α-PbCl2-type structure into the hexagonal structure at 79 GPa and 2 000 K. Inspired by the size effect of nano-sized materials, our group has made progresses in different structural phase transitions and in the compressibility for nanosized MF2 (M=Ca, Sr, Ba) particles at high pressures[19, 23-26]. Previous high-pressure studies on the 8 nm CaF2 nanocrystals reveals that the structural phase transition from the fluorite-type structure into the orthorhombic α-PbCl2-type structure starts at 14 GPa, and the transition pressure is higher than that of the bulk CaF2[23]. Recently, we have performed high-pressure studies on the 23 nm CaF2 nanocrystals up to 23.5 GPa using synchrotron XRD measurement. We have found that the transition from the fluorite to the orthorhombic phase occurrs at 9.5 GPa, significantly lower than the transition pressure of the 8 nm CaF2 nanocrystals, but close to that of the bulk materials[24]. Further analysis indicates that the defect effect in the 23 nm CaF2 nanocrystals plays a key role in the structural stability. Nevertheless, the high pressure induced structural phase transition of the CaF2 nanocrystals with other sizes has not been reported. The phase-transition mechanism and the compressibility of the nanoscale CaF2 are still unclear. In order to further investigate the high pressure behaviors of the nanosized CaF2, and to confirm whether there is a size-dependent phase transformation at high pressure, more high pressure experimental data for various sized CaF2 nanocrystals is urgently needed.
Here we investigate the high-pressure behaviors of the CaF2 nanocrystals with an average grain size of 11 nm using in-situ XRD. We analyse the phase stability, the bulk modulus and the compressibility of the synthesized 11 nm-sized CaF2 nanocrystals in details. Through comparing the high pressure experimental data of different sized CaF2 materials, the main factor influencing the structural stability and compression properties of the CaF2 nanomaterials is explored.
1. Materials and Methods
The 11 nm-sized CaF2 nanocrystals were prepared by a typical synthesis procedure, as reported in literatures[27-28]. 2 mmol Ca(NO3)2 and 4 mmol NaF were added into a mixture which consisted of ethanol, oil acid, and sodium hydroxide. After being vigorously stirred, the white suspension was transferred into a 40 mL autoclave. The heat-treatment condition at a temperature of 160℃ was maintained for 24 h. Then, the autoclave was cooled down to room temperature, and the final products were collected after centrifugation and drying treatments.
The crystalline structure, the morphology and the particle size of the CaF2 nanocrystals were examined by XRD (D8 DISCOVER GADDS) with Cu Kα radiation (
$\lambda $ =1.5418 Å) and by high-resolution transmission electron microscopy (HRTEM, H-7500). The sample was loaded into a diamond anvil cell (DAC) with a culet size of 400 μm for high-pressure analysis. The fluorescence shift of the ruby R1 line was utilized to calibrate the pressure, and an methanol-ethanol mixture with a volume ratio of 4∶1 was chosen as the pressure-transmitting medium. High-pressure XRD experiment was performed at B2 High-Pressure Station of Cornell High Energy Synchrotron Source (CHESS) with a wavelength of 0.485946 Å. MAR165 CCD detector was used to collect the XRD data. The 2D XRD images were integrated using FIT2D software. Materials Studio program was performed to refine the crystal structure using the high-pressure synchrotron XRD patterns.2. Results and Discussion
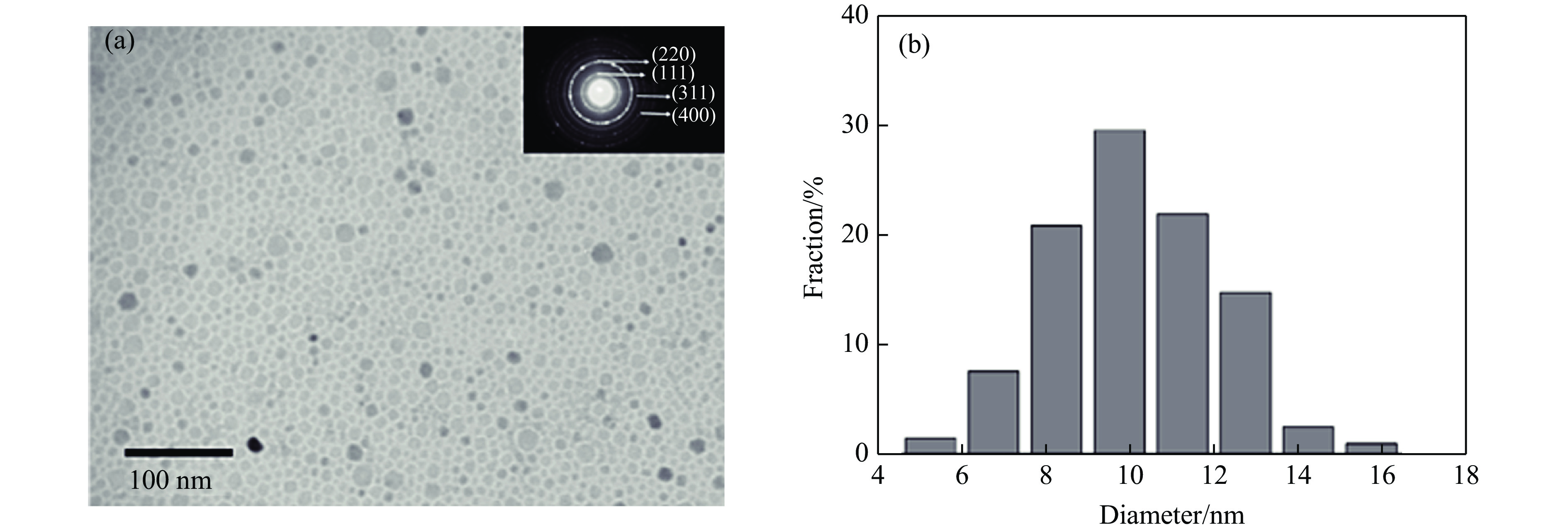
Fig.1(a) exhibits the dimension and the morphology of the synthesized CaF2 nanocrystals, and the corresponding particles’ size distribution histogram is presented in Fig.1(b). Fig.1 reveals that all the nanoparticles are well dispersed and almost sphere with an average diameter of (11 ± 2) nm. The selected-area electron diffraction (SAED) pattern (inset in Fig.1(a)) shows that the major diffraction rings of the fluorite structure, indicating that the synthezied CaF2 nanocrystals have probably the fluorite structure.
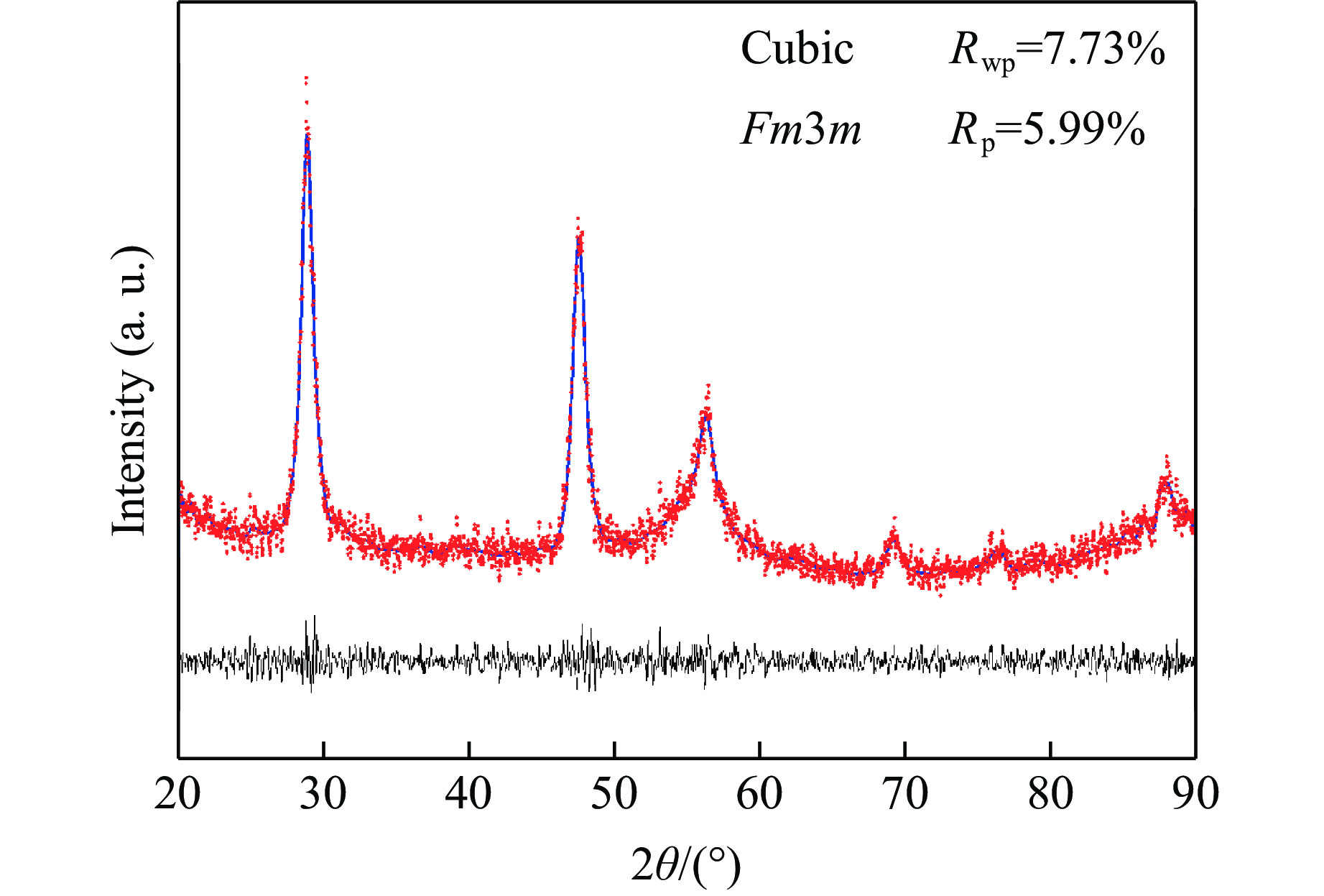
Fig.2 presents the Rietveld refinement of the diffraction pattern of the synthesized CaF2 nano-particles at ambient conditions. The great agreement between simulations and XRD experiments at ambient conditions with the residuals Rwp=7.73% and Rp=5.99% unraveled that the ambient pressure phase adopts a fluorite structure with a space group of Fm3m. The fluorite structure is constructed by the Ca atoms occupying the (0, 0, 0) positions and by the F atoms occupying the (0.25, 0.25, 0.25) positions. The cubic structure has an lattice constant of 5.461(2) Å. It is consistent with the value of a0=5.463 Å (JCPDS Card No. 35-0816).
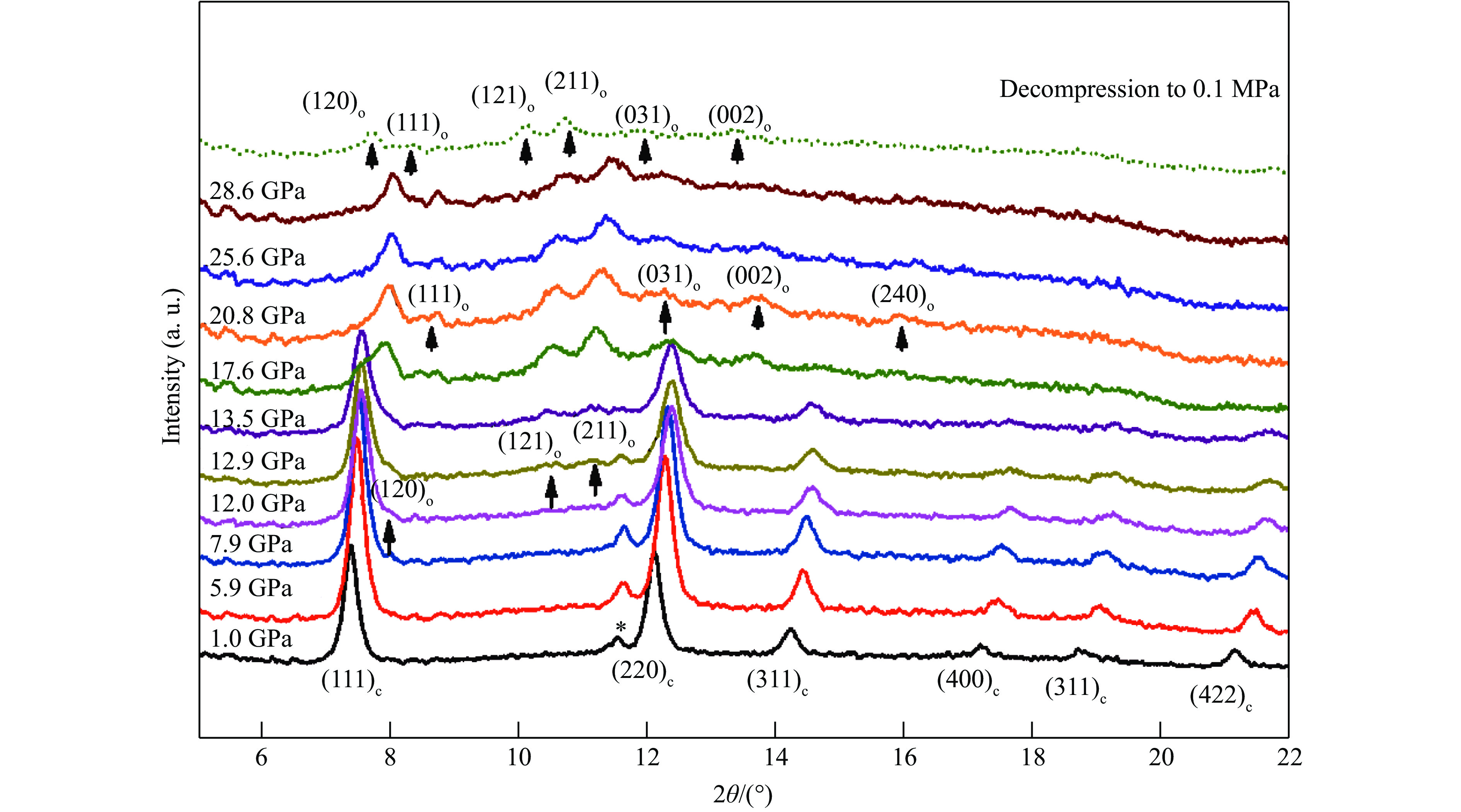
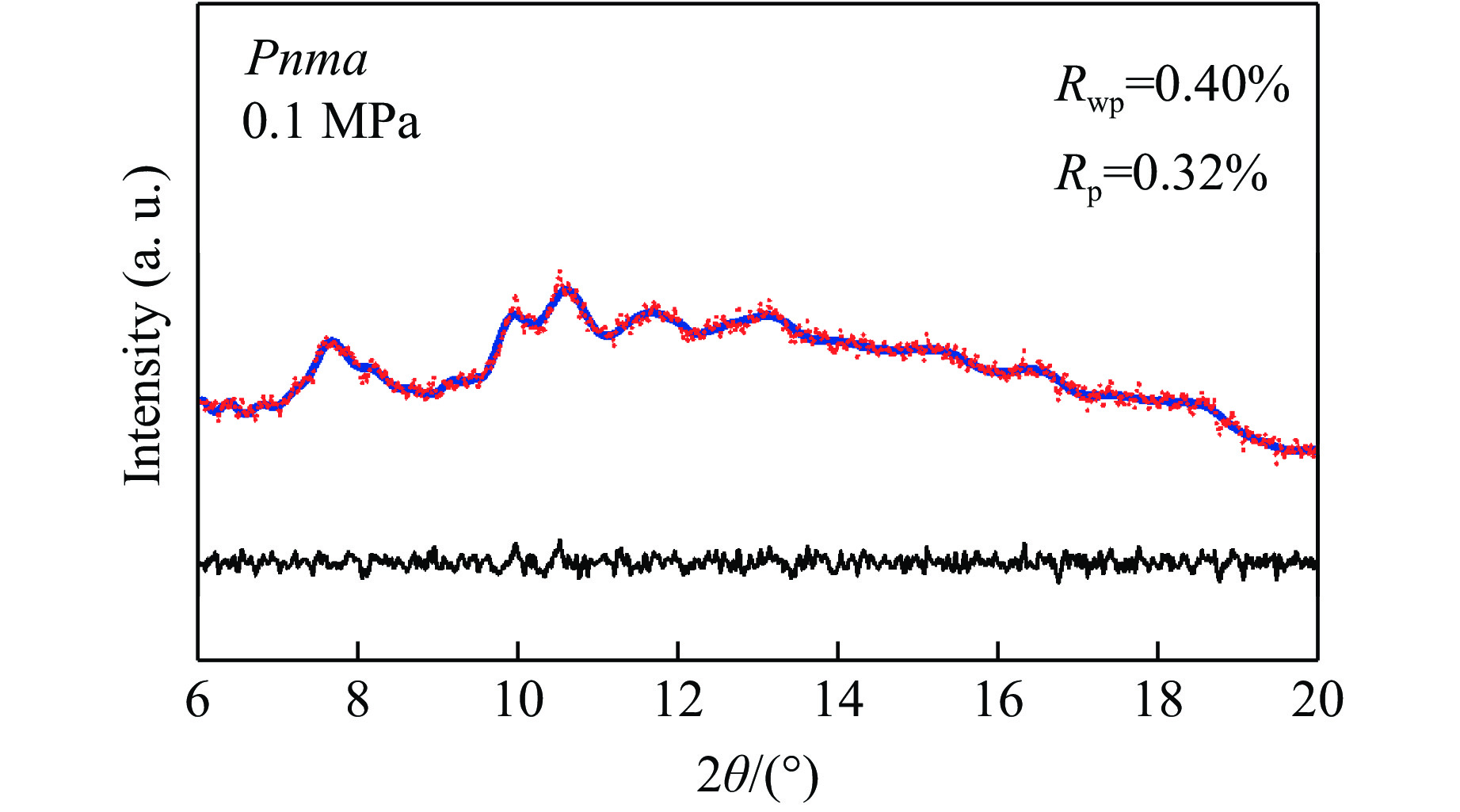
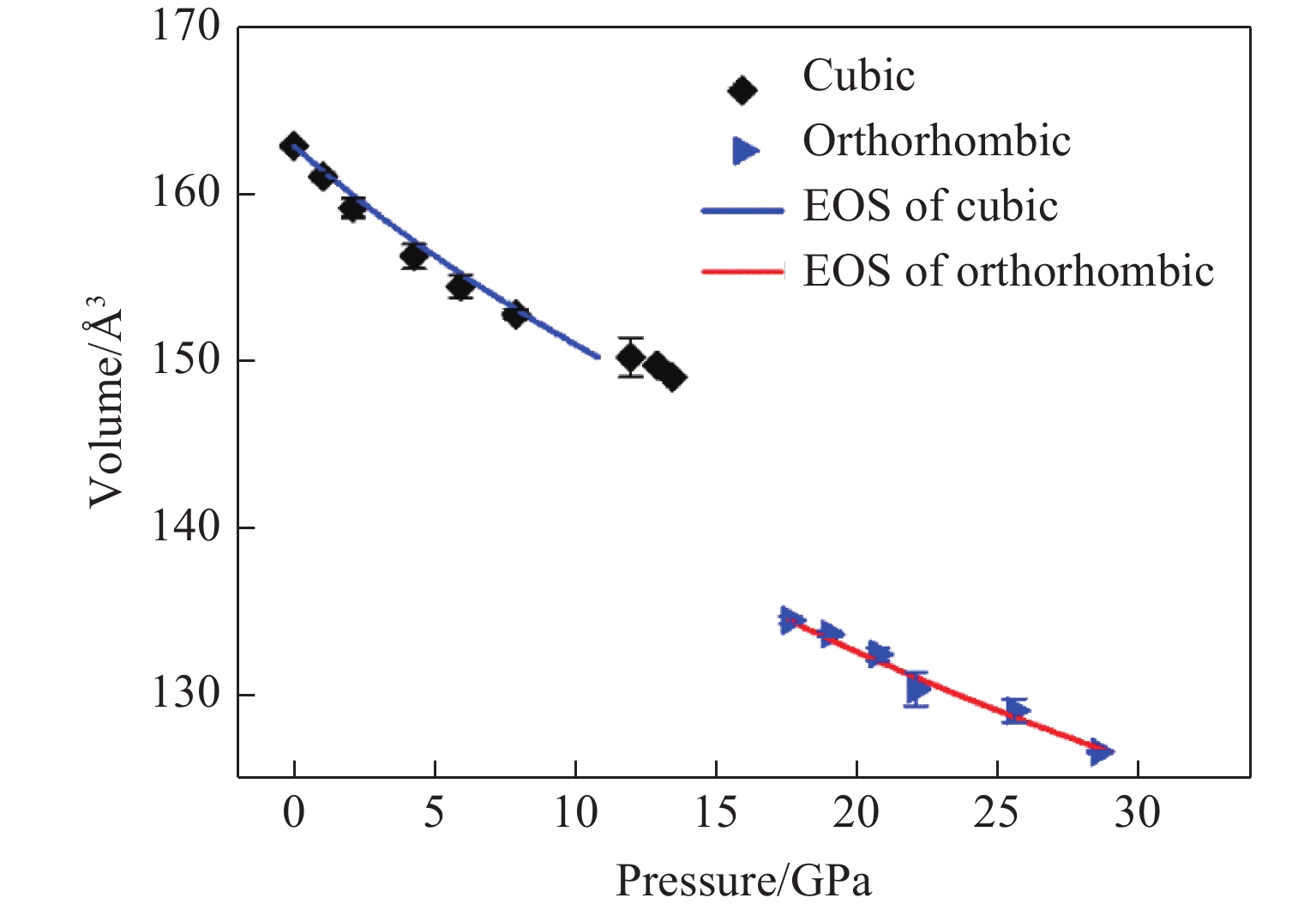
Fig.3 displays the selected high-pressure XRD patterns of the CaF2 nanocrystals under different pressures up to 28.6 GPa. At 1.0 GPa, six diffraction peaks of (111)c, (220)c, (311)c, (400)c, (331)c and (422)c of the CaF2 nanocrystals are observed, together with one peak of the T-301 stainless steel gasket (marked by asterisk). When the pressure reaches 12 GPa, the (111) diffraction peak becomes asymmetric, and a new diffraction peak starts to appear at the right side of the (111) peak, which indicates the occurrence of a phase transition from the fluorite structure to the α-PbCl2-type structure. The transition pressure is much higher than the one reported in the bulk CaF2 materials and slightly lower than that of the 8 nm-sized CaF2 nanocrystals[23]. When the pressure increases to 20.8 GPa, all the diffraction peaks (120)o, (111)o, (121)o, (211)o, (031)o, (002)o and (240)o can be assigned to the arised high pressure phase, illustrating the completion of the phase transition. The α-PbCl2-type structure is stable up to 28.6 GPa (the highest pressure in this study). Then the sample is decompressed to ambient pressure, and it turns out that the pure high-pressure α-PbCl2-type structure is retained, which indicates the phase transformation is irreversible. Fig.4 presents the Rietveld refinement of the diffraction pattern of the CaF2 nanocrystals at ambient conditions after decompression, and it shows a quite good agreement with the α-PbCl2-type structure (with the residual Rwp=0.40 %).
Fig.5 shows the compressibility of the CaF2 nanocrystals. A third-order Birch-Murnaghan (BM) equation of state (EOS) is fitted to the experimental p-V data[29]
p=(3/2)B0[(V/V0)−7/3−(V/V0)−5/3]{1+(3/4)(B′0−4)×[(V/V0)−2/3−1]} (1) where V0 is the zero-pressure volume, V is the volume at pressure p given in GPa (V0 and V were calculated by JADE program), B0 is the isothermal bulk modulus,
${{B}}_0'$ is the first pressure derivative of the bulk modulus. For the CaF2 nanocrystals, the fitting yield B0 = 109(5) GPa,${{B}}_0'$ = 5 for the fluorite structure, and B0 = 89(1) GPa,${{B}}_0' $ = 4 for the α-PbCl2-type structure. The isothermal bulk modulus of the α-PbCl2-type phase is lower than that of the fluorite phase. The lower bulk modulus of the high-pressure phase of the CaF2 nanocrystals at high pressure indicates a higher compressibility. This result is consistent with the previous studies on the bulk CaF2, but it is different from the bulks SrF2 and BaF2 which have lower compressibility under high pressure[22, 30]. The bulk moduli of the 11 nm-sized CaF2 nanocrystals for the fluorite and the α-PbCl2-type structure are both significantly larger than those of the bulk CaF2[21–22] and the 23 nm-sized CaF2 nanocrystals[24], indicating a higher incompressibility for the CaF2 nanocrystals with smaller grain size. In terms of the Hall-Petch effect[31-32], a continuous decrease of grain size could further elevate material hardness, thus, the increase in bulk modulus of 11 nm-sized CaF2 nanocrystals can be easily understood.Table 1 summarizes the phase transition pressure (pT), the EOS parameters (B0 and
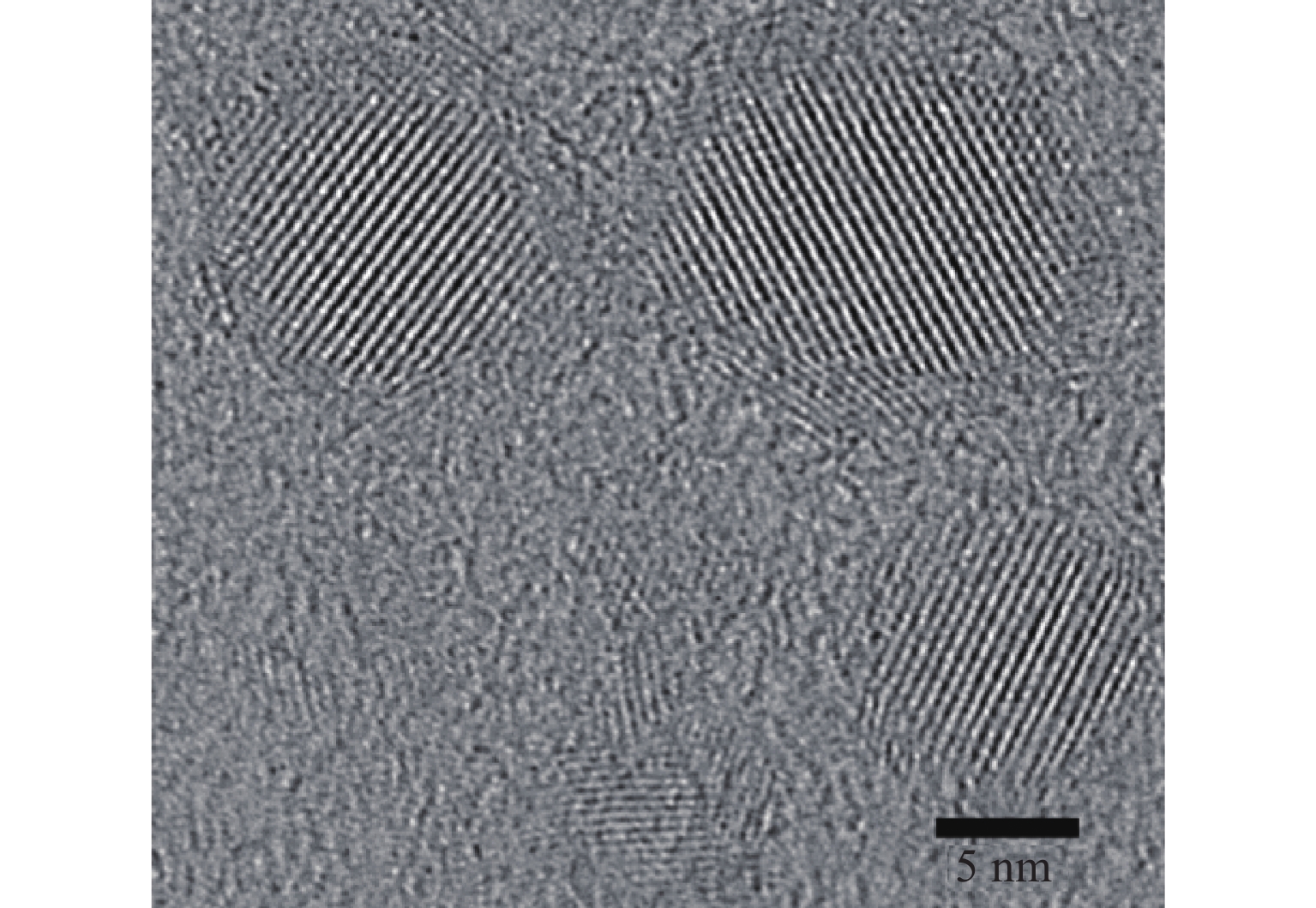
$B_{0}'$ ) of the bulk CaF2 and the CaF2 nanocrystals with different grain sizes, which clearly reveals the differences between the bulk and the nanoscale CaF2. It is found that the phase transition pressure and the bulk modulus of the 11 nm-sized CaF2 nanocrystals are higher than those of the bulk CaF2 and the 23 nm-sized CaF2 nanocrystals. A large number of high pressure investigations indicate that many nanomaterials (e.g., CdSe, ZnS and PbS) exhibit obvious elevations of structural stability compared with their bulk materials, which is attributed to the higher surface energies in nanomaterials[10, 13, 33]. Compared with the bulk CaF2 and the 23 nm-sized CaF2 nanocrystals, a relatively higher surface energy is expected for the 11 nm-sized CaF2 nanocrystals, and thus the elevations both in the transition pressure and in the bulk modulus can be easily understood.Table 1. Transition pressure (${p}{_{\rm T}}$ ), and EOS parameters (B0 and B0 ′) of the fluorite-type and the α-PbCl2-type CaF2Besides the different high-pressure behaviors with the bulk CaF2 and the 23 nm-sized CaF2 nanocrystals, Table 1 shows that the 11 nm-sized CaF2 nanocrystals exhibit a lower transition pressure[23] and a lower bulk modulus compared with the 8 nm-sized CaF2 nanocrystals. To the best of our knowledge, defects and grain size are considered to be the main factors in influencing the high-pressure behaviors of the 11 nm-sized CaF2 nanocrystals and the 8 nm-sized CaF2 nanocrystals. For further analyses, we have carried out HRTEM measurements of many grains of the 11 nm-sized CaF2. The HRTEM image is given in Fig.6, it shows that the 11 nm-sized CaF2 nanocrystals have no visible defects and dislocations, indicating a relatively low defect concentration. Obviously, the decrease of the transition pressure in the 11 nm-sized CaF2 nanocrystals cannot be attributed to the defects (or dislocation) effect. Therefore, the differences in the phase transformations between the 11 nm and the 8 nm-sized CaF2 nanocrystals may be caused by the grain size effect. The 8 nm-sized CaF2 nanocrystals, which have a smaller grain size, possess a higher surface energy, that could result in the elevation of the phase transition pressure.
Our results illustrate that the transition pressure dramatically increases as the size of the grains decreases, and the CaF2 nanocrystals show a noticeable size-dependence of phase transformation at high pressure when the grain size below 11 nm. Therefore, it can be reasonably concluded that the critical size of the CaF2 nanocrystals, marking the oneset of nanoscal effect, is larger than 11 nm. For the 11 nm-sized CaF2 nanocrystals, the high-pressure metastable structure (α-PbCl2-type structure) is retained after the pressure is released, without observing the fluorite structure. This result is in good accordance with the oberservation for the 8 nm-sized CaF2 nanocrystals[23], but it is different with those of the bulk CaF2 and the 23 nm-sized CaF2 nanocrystals whose transformations are completely or partially reversible[21, 24]. The inreversibility of the 11 nm-sized CaF2 nanocrystals might be due to the high surface energy, which lead to the solid-solid phase transition hysteresis after decompression. Discovering novel high-pressure metastable structure is one of the main purposes of the high pressure study of the CaF2 nanocrystals.
3. Conclusions
In summary, the high-pressure behaviors of the CaF2 nanocrystals with an average grain size of 11 nm have been investigated by in-situ XRD. The phase transition from the fluorite structure to the α-PbCl2-type structure occurrs at 12 GPa, which is much higher than the value observed for the bulk CaF2 and slightly lower than that of the 8 nm-sized CaF2 nanocrystals. The bulk moduli of the CaF2 nanocrystals with the fluorite or the α-PbCl2-type structures are all larger than those of the bulk CaF2, indicating a high incompressibility of nanosized CaF2. The pure α-PbCl2-type metastable structure is retained in the 11 nm-sized CaF2 nanocrystals after decompression. Such distinct high-pressure behaviors of the 11 nm-sized CaF2 nanocrystals are considered to be mainly due to the grain size effect. When the size is below the critical size, the high surface energy begins directing the enhancement of the structural stability and the increase of the bulk modulus.
-
表 1 混凝土结构的HJC模型参数
Table 1. HJC model parameters of concrete structure
$\,\rho $/(kg·m–3) E/GPa $\nu $ ${ f{'_ {\rm{c} } } }$/MPa Smax A B N C $\varepsilon {_{\min }^{\rm f} }$ 2439 32.5 0.2 48 7.0 0.79 1.6 0.61 0.007 0.01 pcrush/GPa plock/GPa D1 D2 K1/GPa K2/GPa K3/GPa vp/(m·s−1) vs/(m·s−1) 0.016 0.8 0.04 1 85 −171 208 3872 2375 表 2 围岩的物理力学参数
Table 2. Physical and mechanical parameters of surrounding rock
$\,\rho $/(kg·m–3) E/GPa $\nu $ $q{_{\phi}}$/(°) $K{_{\phi}}$ $q{_{\varPsi} }$/(°) $\sigma $t/kPa vp/(m·s−1) vs/(m·s−1) 1850 0.04 0.35 0.388 11171 0 0.1 185 89 表 3 空气(空模型)的物理力学参数
Table 3. Physical and mechanical parameters of air (air model)
$\,\rho $/(kg·m–3) c/(m·s−1) E0/(MJ·m–3) $\kappa $ vp/(m·s−1) vs/(m·s−1) 1.29 340 0 1.4 340 0 表 4 固体爆炸物参数
Table 4. Calculation parameters of solid explosives
$\,\rho $0/(kg·m–3) e0/(GJ·m–3) pCJ/GPa $\gamma $ DJ/(m·s–1) 1500 7.0 21 2.727 6930 AJWL/GPa BJWL/GPa R1 R2 $\omega $ 371.2 3.23 4.15 0.95 0.30 表 5 超压模拟结果与经验公式计算结果对比
Table 5. Comparison of overpressure simulation results and empirical formula calculation results
Distance/m Overpressure/MPa Error/% Theoretical formula Numerical simulation 5 2.35 2.25 4.44 10 0.47 0.52 −7.69 表 6 站台有柱区域和无柱区域在固体爆炸作用下结构参数的对比
Table 6. Comparison of various parameters under the solid explosion in the pillared and non-pillared areas of the platform
Area pm/MPa Rd/m nd Cd/m2 pr/MPa pf1/MPa With volumns 6.5 8 1.00 21 5.025 218.0 Without volumns 6.0 5 0.67 13 5.667 110.5 Error/% −7.69 −37.50 −33.00 −38.10 12.78 −49.31 Area pf2/MPa dr/m df1/m df2/MPa dp/m With volumns 0.034 0.124 0.188 0.037 1.64 Without volumns 0.041 0.084 0.103 0.089 0.43 Error/% 20.59 −32.26 −45.21 140.54 −73.78 -
[1] 城市轨道交通2017年度统计和分析报告 [J]. 城市轨道交通, 2018(4): 6−25.Statistics and analysis report of urban rail transit in 2017 [J]. China Metros, 2018(4): 6−25. [2] 王勇, 王德荣, 陈灿寿, 等. 某地铁区间隧道内爆炸效应的数值模拟 [J]. 防护工程, 2006, 28(6): 55–58.WANG Y, WANG D R, CHEN C S, et al. Numerical simulation on interior blasting effect in the section of tunnel in some subway [J]. Protective Engineering, 2006, 28(6): 55–58. [3] CHOI S, WANG J, MUNFAKH G, et al. 3D nonlinear blast model analysis for underground structures [C]//GeoCongress 2006. Atlanta, Georgia, USA: ASCE, 2006. [4] ZHANG X X, CHENG J W, SHI C L, et al. Numerical simulation studies on effects of explosion impact load on underground mine seal [J]. Mining, Metallurgy & Exploration, 2020, 37(2): 665–680. doi: 10.1007/s42461-019-00143-2 [5] 柴永生, 王月桂, 章毅. 地铁口部爆炸冲击波传播规律与超压荷载研究 [J]. 防护工程, 2019, 41(1): 42–46.CHAI Y S, WANG Y G, ZHANG Y. Study on propagation of blast wave and the overpressure load subjected to metro entrance explosion [J]. Protective Engineering, 2019, 41(1): 42–46. [6] 王桂林, 欧阳啸天, 翟俊, 等. 浅埋三舱管廊甲烷爆炸的地面响应规律 [J]. 高压物理学报, 2021, 35(1): 015202. doi: 10.11858/gywlxb.20200616WANG G L, OUYANG X T, ZHAI J, et al. Ground response law of methane explosion in shallow buried three-cabin pipe gallery [J]. Chinese Journal of High Pressure Physics, 2021, 35(1): 015202. doi: 10.11858/gywlxb.20200616 [7] 张雄, 廉艳平, 刘岩, 等. 物质点法 [M]. 北京: 清华大学出版社, 2013: 31−32.ZHANG X, LIAN Y P, LIU Y, et al. Material point method [M]. Beijing: Tsinghua University Press, 2013: 31−32. [8] 陈卫东, 杨文淼, 张帆. 基于物质点法的水下爆炸冲击波数值模拟 [J]. 高压物理学报, 2013, 27(6): 813–820. doi: 10.11858/gywlxb.2013.06.004CHEN W D, YANG W M, ZHANG F. Material point method for numerical simulation of underwater explosion blast wave [J]. Chinese Journal of High Pressure Physics, 2013, 27(6): 813–820. doi: 10.11858/gywlxb.2013.06.004 [9] 王宇新, 陈震, 张洪武, 等. 多层抗爆结构冲击响应无网格MPM法分析 [J]. 工程力学, 2007, 24(12): 186–192. doi: 10.3969/j.issn.1000-4750.2007.12.032WANG Y X, CHEN Z, ZHANG H W, et al. Response of multi-layered structure due to impact load using material point method [J]. Engineering Mechanics, 2007, 24(12): 186–192. doi: 10.3969/j.issn.1000-4750.2007.12.032 [10] 张芮瑜, 孙玉进, 宋二祥. 强夯的物质点法模拟及其能量转化规律分析 [J]. 岩土工程学报, 2019, 41(7): 1208–1216. doi: 10.11779/CJGE201907004ZHANG R Y, SUN Y J, SONG E X. Simulation of dynamic compaction using material point method and analysis of its energy conversion law [J]. Chinese Journal of Geotechnical Engineering, 2019, 41(7): 1208–1216. doi: 10.11779/CJGE201907004 [11] 董友扣, 马家杰, 王栋, 等. 深海滑坡灾害的物质点法模拟 [J]. 海洋工程, 2019, 37(5): 141–147. doi: 10.16483/j.issn.1005-9865.2019.05.016DONG Y K, MA J J, WANG D, et al. Investigation of landslide in deep sea using material point method [J]. The Ocean Engineering, 2019, 37(5): 141–147. doi: 10.16483/j.issn.1005-9865.2019.05.016 [12] HANCHAK S J, FORRESTAL M J, YOUNG E R, et al. Perforation of concrete slabs with 48 MPa (7 ksi) and 140 MPa (20 ksi) unconfined compressive strengths [J]. International Journal of Impact Engineering, 1992, 12(1): 1–7. doi: 10.1016/0734-743X(92)90282-X [13] 李科斌, 董新龙, 李晓杰, 等. 水下爆炸实验法在工业炸药JWL状态方程测定中的应用研究 [J]. 兵工学报, 2020, 41(3): 488–494. doi: 10.3969/j.issn.1000-1093.2020.03.009LI K B, DONG X L, LI X J, et al. Research on parameters determination of JWL EOS for commercial explosives based on underwater explosion test [J]. Acta Armamentarii, 2020, 41(3): 488–494. doi: 10.3969/j.issn.1000-1093.2020.03.009 [14] 李志鹏. 瓦斯爆炸作用下隧道衬砌致损机理及修复技术研究 [D]. 北京: 北京科技大学, 2019.LI Z P. Study on damage mechanism and repair technology of tunnel lining subject to gas explosion [D]. Beijing: University of Science and Technology, 2019. [15] 涂国勇, 王海锋, 禄晓飞, 等. 整体爆破弹头爆炸当量定量评价研究 [J]. 现代防御技术, 2020, 48(2): 30–34. doi: 10.3969/j.issn.1009-086x.2020.02.005TU G Y, WANG H F, LU X F, et al. Explosion equivalent quantitative evaluation of global blowup warhead [J]. Modern Defense Technology, 2020, 48(2): 30–34. doi: 10.3969/j.issn.1009-086x.2020.02.005 [16] 张程娇. 炸药爆轰产物参数的特征线差分反演方法研究 [D]. 大连: 大连理工大学, 2016.ZHANG C J. Research of inversion method of detonation products physical parameters based on modified method of characteristics [D]. Dalian: Dalian University of Technology, 2016. [17] 闫秋实, 刘晶波, 伍俊. 典型地铁车站内爆炸致人员伤亡区域的预测研究 [J]. 工程力学, 2012, 29(2): 81–88.YAN Q S, LIU J B, WU J. Estimation of casualty areas in subway station subjected to terrorist bomb [J]. Engineering Mechanics, 2012, 29(2): 81–88. [18] HENRYCH J. The dynamics of explosion and its use [M]. Amsterdam: Elsevier Scientific Publishing Company, 1979: 178−181. [19] BRODE H L. Blast wave from a spherical charge [J]. Physics of Fluids, 1959, 2(2): 217. doi: 10.1063/1.1705911 [20] SADOVSKYI M A. Mechanical action of air shock waves of explosion based on experimental data [M]. Moscow: Izd Akad Nauk SSSR, 1952. [21] WU C Q, HAO H. Modeling of simultaneous ground shock and airblast pressure on nearby structures from surface explosions [J]. International Journal of Impact Engineering, 2005, 31(6): 699–717. doi: 10.1016/j.ijimpeng.2004.03.002 [22] 师光达. 化工园区危险性评价研究 [D]. 沈阳: 沈阳理工大学, 2020.SHI G D. Study on risk assessment of chemical industry park [D]. Shenyang: Shenyang Ligong University, 2020. [23] 王新建. 爆炸中缺口效应及其防护研究 [J]. 中国人民公安大学学报(自然科学版), 2008, 14(3): 88–90.WANG X J. Study on explosion notch effect and its protecton [J]. Journal of Chinese People’s Public Security University (Science and Technology), 2008, 14(3): 88–90. -







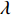

 DownLoad:
DownLoad:




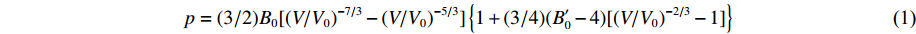
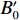
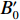
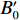
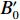
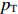
 DownLoad:
DownLoad:


 下载:
下载: