A Review of the Experimental Determination of the Melting Curve of Iron at Ultrahigh Pressures
-
摘要: 铁是典型的d电子过渡金属之一,其在高压下的熔化行为对于揭示地核的成分、热结构和热演化至关重要。在实验室中创造极端高温高压条件以及诊断和测量凝聚介质的熔化行为和熔化温度比较困难,导致长期以来不同实验之间以及实验与理论之间获得的铁的高压熔化线存在较大争议。近年来,随着高压实验技术的发展,对铁高压熔化线的认识逐渐趋于一致。本文介绍了用于研究铁在高压下熔化行为和熔化温度的动、静高压实验技术,总结了高压下诊断铁和过渡金属熔化的方法及其优缺点,并分析了不同实验之间产生差异的原因。基于目前关于铁高压熔化温度的实验和理论研究结果,铁在内外核边界压力(约330 GPa)下的熔化温度可限定为5900~6300 K。系统总结铁在高压下的熔化行为对于进一步认识熔化的物理机制、研究其他过渡金属的高压熔化行为等具有重要的指导和借鉴意义。Abstract: Iron is one of the typical d-orbital transition metals. Its melting behavior and melting curve at high pressure are of great importance for revealing the composition, thermal structure, and thermal evolution of the Earth’s core. Creating the extreme high pressure-temperature conditions and measuring the melting temperatures of the condensed matters in the laboratory are quite challenging, resulting in a long-term controversy on the melting curves of iron at high pressures among various experiments and between experiments and theories. With the development of various experimental techniques, the results between experimental and theoretical studies are generally consistent with each other. This review presents the main static and dynamic compression techniques that have been used to study the high-pressure melting curve of iron in recent years; it also explores the advantages and disadvantages of melting diagnostics for iron and transition metals. The possible reasons for the discrepancy in the melting curves of iron are also discussed. Based on the experimental and theoretical results of the melting curve of iron, the melting temperature of iron can be anchored to 5900–6300 K at the pressure of the inner core boundary (ICB, about 330 GPa). Summarizing the current researches on the melting behaviors of iron under static and dynamic compression has an important implication for studying the melting curves of other transition metals, as well as the melting mechanism.
-
Key words:
- iron /
- high-pressure melting /
- static compression /
- dynamic compression /
- Earth’s core temperature
-
随着“双碳”战略的推进,新能源利用对中国经济的可持续发展至关重要[1]。锂离子电池(lithium-ion battery,LIB)由于具有高能量密度、长寿命和可减少环境污染等优点,成为新能源结构的重要组成部分[2]。然而,随着电池能量密度的提高,安全风险也随之增大。国内外学者对导致电池形变及故障的因素,如机械滥用、电气滥用和热滥用等[3],进行了研究,发现机械滥用是最重要、最直接的诱因[4]。因此,研究锂离子电池在机械荷载下失效的影响因素对电动汽车组的耐撞性设计具有重要意义。
目前,已开展了大量机械滥用下锂离子电池的力学行为和失效机理研究。Attara等[5]发现将锂离子聚合物电池嵌入多功能复合材料结构可以提高其总体压缩强度和刚度;董思捷等[6]通过实验观察和分析锂离子电池在不同挤压载荷下的失效情况,讨论了失效机理以及挤压载荷对其性能的影响;Greve等[7]通过实验和有限元模拟研究了圆柱形锂离子电池在受到外部载荷时发生的塑性变形和断裂,发现有可能引起短路。受限于实验条件,大多数学者主要采用有限元模拟方法研究锂离子电池。在力学模拟方面,Jia等[8]提出了一种基于代表性体积元素方法的锂离子电池热-电-力学建模框架;Liu等[9]研究了18650锂离子电池模块在不同堆叠密度和堆叠方式下的机械完整性问题;Beaumont等[10]提出了一种锂离子电池薄片的宏观有限元模型开发方法,用于预测电池在多种机械载荷下的力学响应;李志杰等[11]采用精细化模型,对动力电池包进行了有限元模拟;Wang等[12]利用详细的力学模型研究了圆柱形锂离子电池在机械载荷下的力学响应和内部短路损伤机制。然而,关于准静态工况下锂离子电池失效的影响因素仍需进一步研究,例如:如何建立锂离子电池的失效模型是锂离子电池失效研究的关键问题之一。
此外,动态冲击工况下锂离子电池的失效行为和机制也引起了国内外学者的广泛关注。在冲击实验方面,Zhou等[13]研究了在动态和准静态机械荷载下锂离子电池的力-电-化学耦合失效机制,并进行了安全评估;Hu等[14]研究了动态冲击下可充电电池组的失效行为;Jia等[15]研究了锂离子电池在动态机械加载下的力-电-化学耦合行为。在有限元模拟方面,冲击工况下锂离子电池的失效研究还较少。Zhang等[16]基于应力波理论,通过实验和数值模拟,研究了电池内部的应力波传播机制以及应力波对电池结构和材料的影响;Xing等[17]通过实验和数值模拟研究了锂离子电池在不同撞击速度下的动态失效机制。Pan等[18]研究了锂离子电池在不同冲击载荷下的响应特征和失效模式。如何对不同冲击工况下锂离子电池失效时的影响因素进行分析,建立各影响因素与锂离子电池动态失效机理之间的关联性,也是电动汽车耐撞性领域亟待解决的关键问题。
本研究将通过实验与有限元模拟相结合的方法研究锂离子电池在挤压/冲击工况下的失效行为,分析在不同工况下锂离子电池失效时的影响因素,给出锂离子电池的力-电-热响应和失效模式,建立不同工况下锂离子电池的失效位移、失效峰值力与各影响因素的关系。
1. 方法和模型
1.1 样品、设备和挤压实验方法
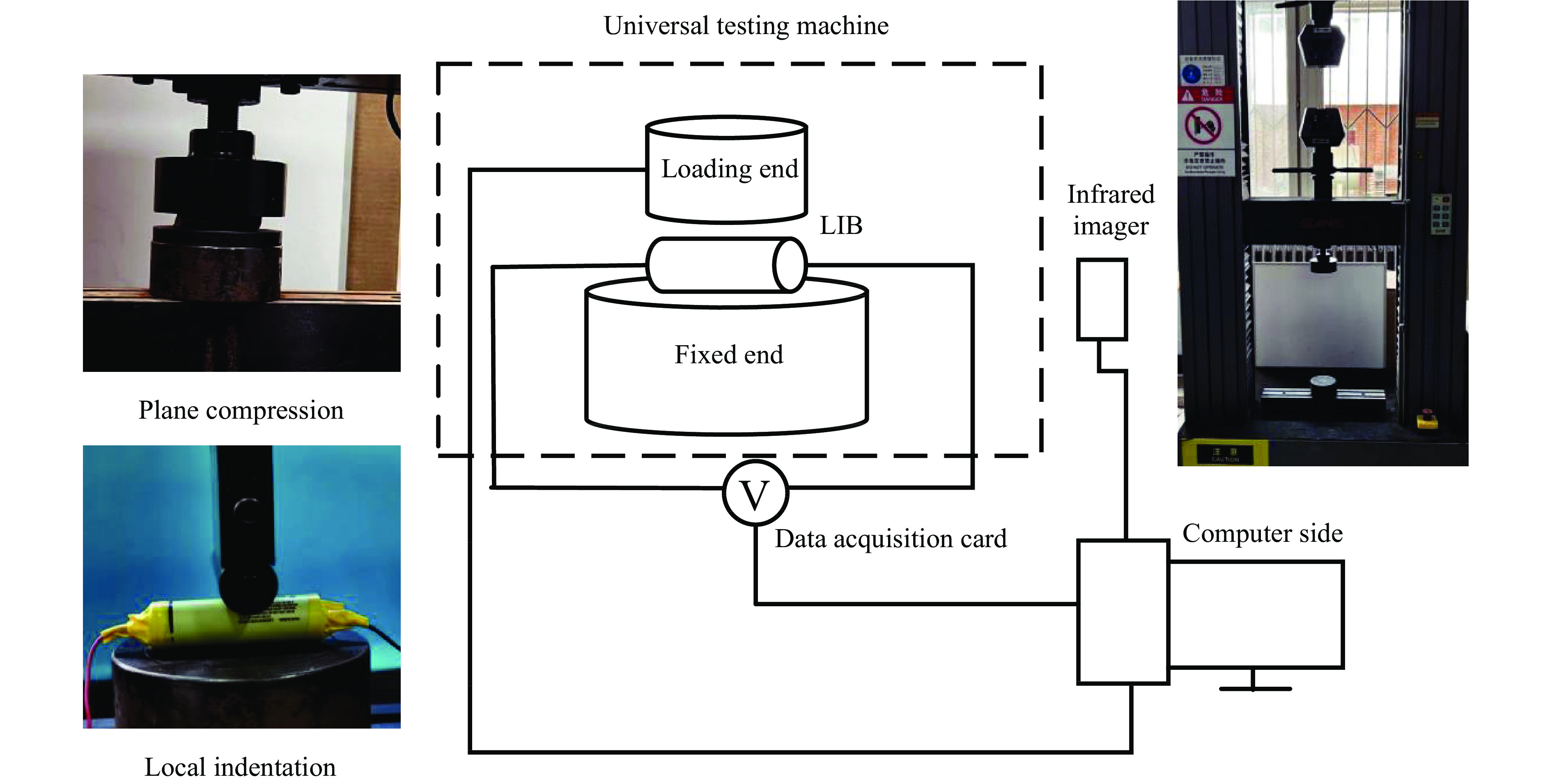
选用NCR18650圆柱形锂离子电池作为实验研究对象。正极活性物质为镍钴锰酸锂化合物,覆盖在铝箔表面;负极由负极活性材料石墨和集流体铜箔组成;隔膜通常由聚乙烯(PE)和聚丙烯(PP)等材料制成;额定容量为3400 mA·h。表1为NCR18650圆柱形锂离子电池的相关参数。
表 1 NCR18650圆柱形锂离子电池参数Table 1. Parameters of the NCR18650 cylindrical lithium-ion batteryRated capacity/
(mA·h)Diameter/mm Length/mm Nominal voltage/V Charge termination
voltage/VDischarge cut-off
voltage/V3400 18 65 3.7 4.2 2.75 采用SANS微机控制式电子万能试验机加载设备进行位移测量,分辨率为0.01 mm,量程100 kN;实验采用加载压板,加载弯曲压头直径10 mm;温度采集装置为Ulid UTi85A红外热成像仪,采集温度的位置为电池侧面的几何中心,经热电偶标定后,发射率设定为0.95。利用数据采集软件对电压进行采集,电压采集线分别与电池两端正负极相连并绝缘,实验过程如图1所示。
为尽可能地降低锂离子电池失效故障造成的热失控风险和因充电不均匀而产生的误差影响,在实验前对电池进行完全放电处理,荷电状态(state-of-charge,SOC)值均为零[6]。为保证实验结果的准确性,每组实验重复3次。具体实验步骤如下:
(1) 在加载前,将不同容量的电池样本放置在万能试验机起支撑作用的工作平台中央,缓慢控制压头下降,在即将达到电池上表面位置处停止;
(2) 将电池采集电压用的导线与万用表连接,并对试验机压头与电池的接触位置进行绝缘处理;
(3) 启动红外热成像仪、万用表,并将其与控制电脑连接,打开对应数据的采集软件;
(4) 启动万能试验机,加载速度均为2 mm/min,局部压痕实验的加载位置位于电池侧面的几何中心线;
(5) 观察实验现象、载荷-位移曲线、电压行为和温度响应,当电池开路电压下降到零时,万能试验机停止加载;
(6) 整理失效的电池样本和实验数据,清理并检查实验系统。
1.2 有限元模型
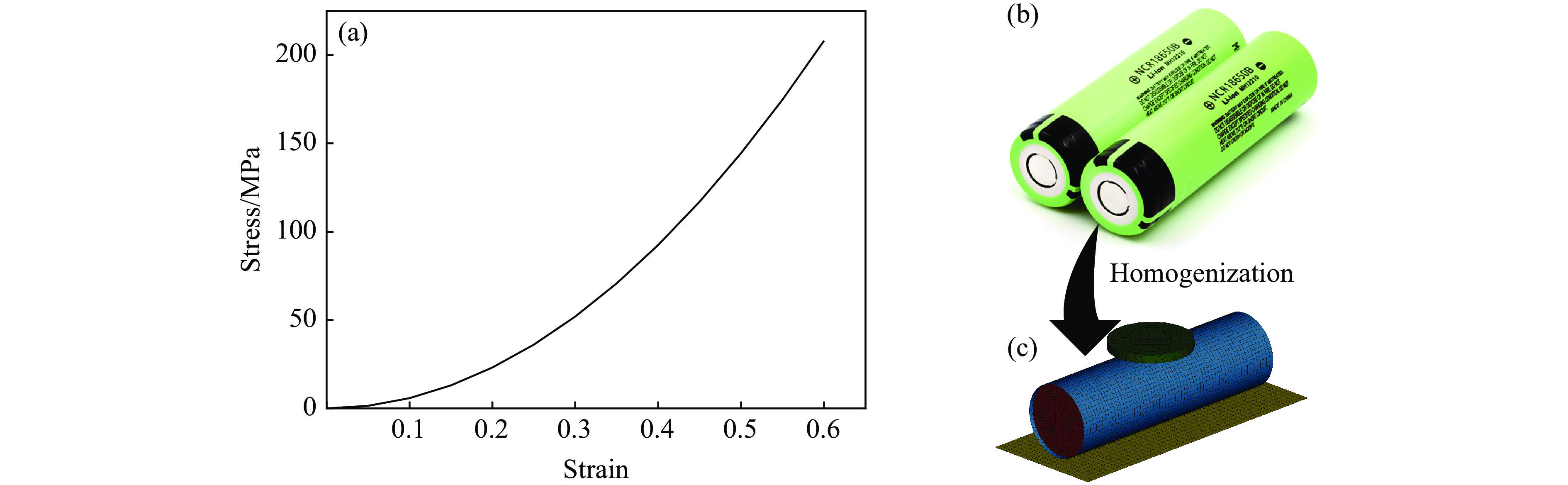
基于NCR18650圆柱形锂离子电池建立有限元模型。电池内芯由集流体、活性物质和隔膜等组成[2–3]。在建立电池内芯模型时,可以将其看成均质各向同性材料,即采用均质化方法建立电池内芯模型[3],均质化模型计算简单,能够满足工程需求。
为了建立圆柱形锂离子电池单体模型,需要获得电池内芯的材料力学特性。通过实验测得挤压后的电池内芯载荷-位移曲线,将荷载-位移曲线转换为名义应力-应变关系[19]。电池内芯所受的名义应力
σ=F/S (1) 式中:F为电池承受的载荷,S为电池的受力面积。
由于电池为圆柱形电池,受力面积随着压缩过程发生变化,且
S=Ld (2) 式中:L为电池内芯的长度,d为受力面积的宽度。在压缩过程中,受力面积的宽度是不断变化的
d=2rarcos(r−x/2r) (3) 式中:r为电池内芯的半径,x为万能试验机挤压盘的位移。
根据式(1)~式(3),电池内芯所受的名义应力
σ=F2Lrarcos(r−x/2r) (4) 电池内芯所受的名义应变
ε=x/2r (5) 根据单轴压缩测试得到的结果,圆柱形电池内芯的应力-应变曲线如图2所示。电池内芯材料与泡沫材料的性质类似,在压缩过程中,逐渐出现了压缩密实阶段。为了建立适用于锂离子电池的本构方程,可以采用参数拟合的方法。根据文献[20],采用均质化本构方程描述锂离子电池的力学行为
σ=Aεn (6) 式中:A为材料参数,n为硬化指数。根据图2所示的电池内芯单轴压缩应力-应变数据,通过幂指函数拟合得到圆柱形锂离子电池内芯的A=276 MPa,n=1.8。最终得到圆柱形锂离子电池的本构方程[20]
σ=276ε1.8 (7) 利用ANSYS/LS-DYNA非线性有限元软件对电池进行准静态挤压模拟,加载速度为0.1 m/s,该速度可视为准静态加载[21]。电池内芯采用SOLID164体单元(8节点六面体单元)描述,为保证计算精度,单元尺寸设为1 mm。为提高计算效率,对外壳和压头采用四边形SHELL163壳单元(4节点四边形壳单元)描述,单元尺寸统一设为2 mm,采用Belytschko-Wong-Chiang算法,支撑平台为刚性板。平面压缩实验表明,电池内芯具有低平台应力泡沫材料属性。电池内芯选择63号可压缩泡沫材料,外壳采用24号弹塑性材料,压头采用20号刚性材料,具体参数见表2。支撑平台底端固定,压头以恒定速度向下运动,限制其他方向位移。接触均选择面-面自动接触算法,摩擦系数为0.3。
表 2 有限元模型材料参数Table 2. Finite element model material parametersComponent Material type Poisson’s ratio Density/(kg·m−3) Elasticity modulus/GPa Indenter MAT_20 0.30 7800 210 Shell MAT_24 0.30 2700 69 Inner core MAT_63 0.01 2000 0.38 1.3 可靠性验证
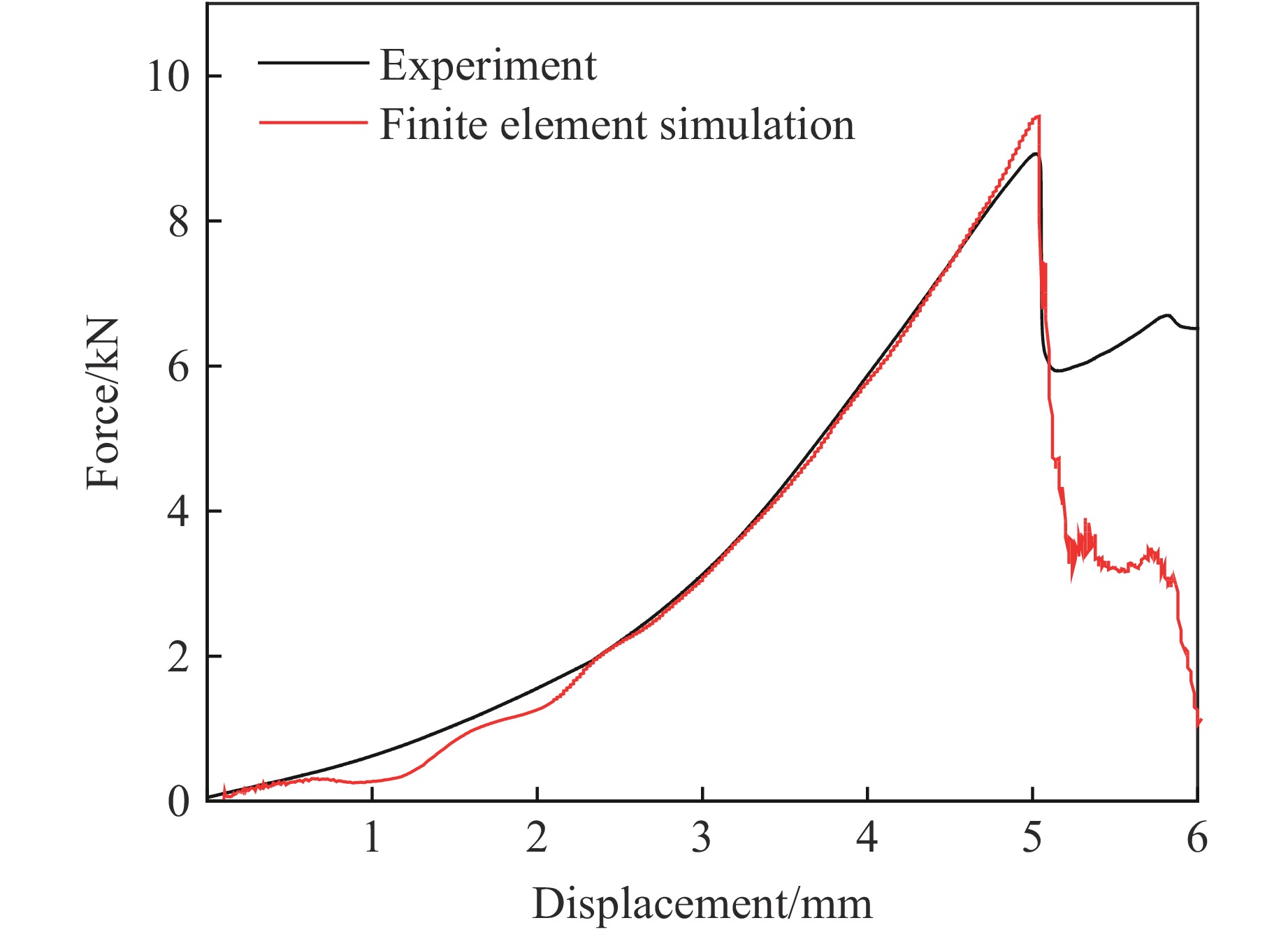
图3显示了圆柱形锂离子电池准静态加载实验和有限元模拟结果。目前没有统一标准判断锂离子电池失效,Xu等[22]用统一强度理论描述圆柱形锂离子电池在压缩过程中的失效。Sahraei等[23]采用拉伸截止应力作为锂离子电池短路的标准。局部压痕实验中,常用力-位移曲线的突变点判断电池失效。本研究采用失效参数Ts(拉伸截止应力)和最大失效主应变εf判断电池失效,并确定所用锂离子电池的Ts=26 MPa,εf=0.14[23]。当模型尺寸、加载速度和边界条件均相同时,由图3可知,局部压痕载荷下有限元模拟与实验结果吻合较好。由于本研究为均匀化模型,而实际上电池内芯为层级结构,计算时难免产生误差,考虑到压缩趋势是一致的,由此证明了内芯材料模型的正确性。
2. 结果和讨论
2.1 实验数据及分析
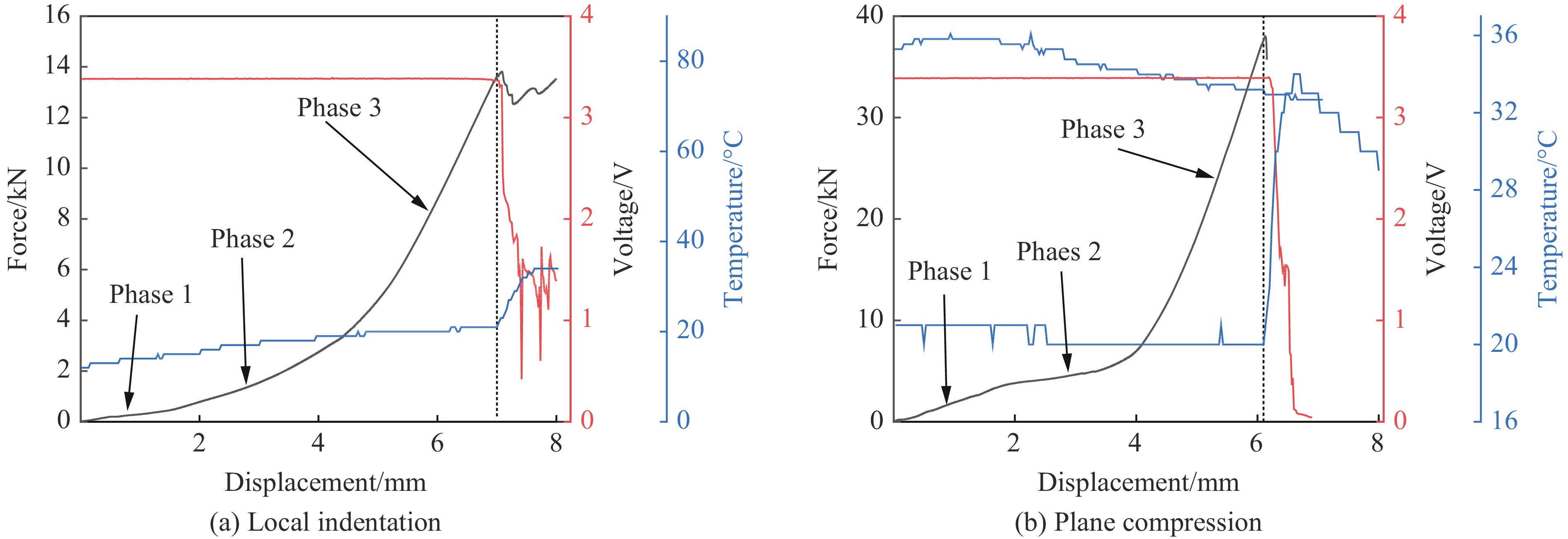
设计了平面压缩和局部压痕2种加载方案,平面挤压实验主要研究锂离子电池受大面积整体压缩工况,而局部压痕实验具体讨论锂离子电池局部区域受到外物挤压的工况。图4分别给出了平面压缩、局部压痕实验中,容量为3400 mA·h的锂离子电池的力-电-热曲线。可以看出,在2种加载工况下,初始阶段负载均随压缩位移的增大而增加,而温度和电压几乎保持不变。在电池失效前的变形过程中特征点为拐点和峰值力,整个变形过程可分为3个阶段:第1阶段,电池壳体承受主要负载;第2阶段,电池内部的空隙逐渐被压实,刚度随之增大,压缩载荷也逐步增大;第3阶段,电池整体受力,内部开始出现损坏并逐渐扩展,累积为整体故障,该阶段也被称为损坏扩展阶段。第3阶段以后,电池发生整体故障,压缩力迅速降低。在平面压缩和局部压痕加载形式下,电压下降、温度上升、承载能力减弱以及电解液流出等现象同时出现,进一步证实了电池已发生故障。实验测得的力-位移曲线可以反映电池在压缩过程中的受力情况和变形行为,特别是在第3阶段,可以观察到力的突然减小,表明电池内部出现了明显损伤。
2.2 准静态挤压工况下的电池失效影响因素分析
2.2.1 压头形状的影响
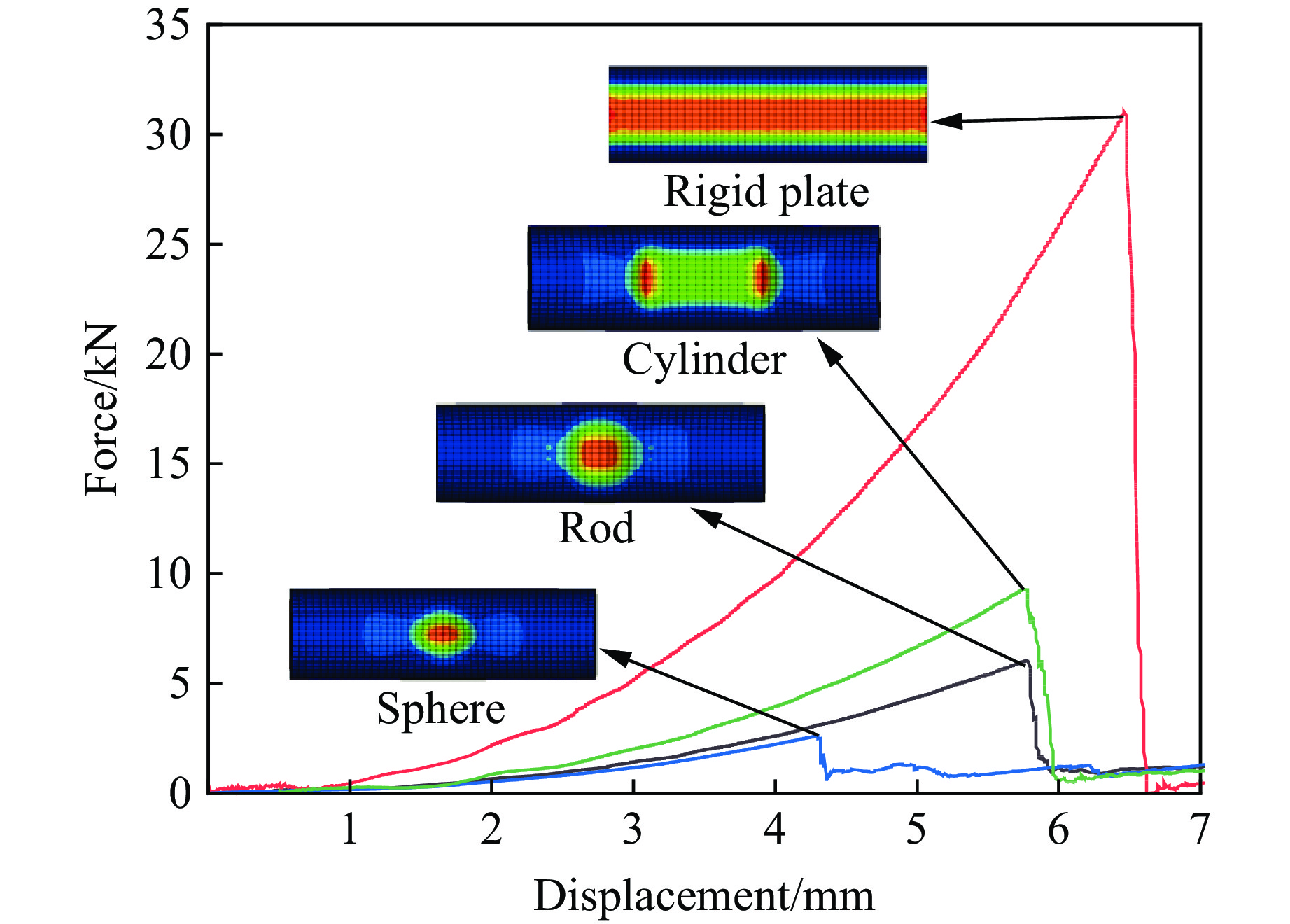
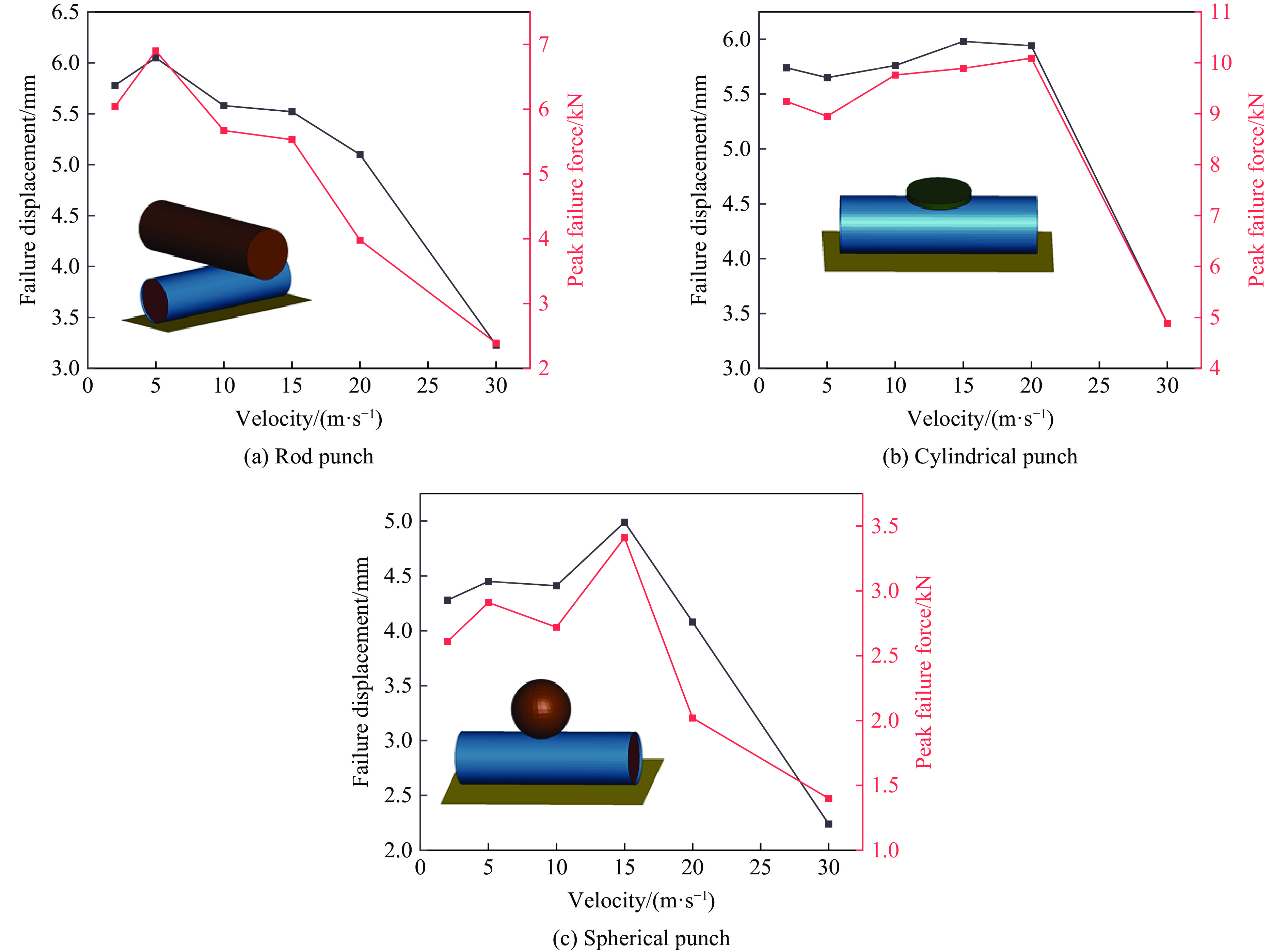
为了研究压头形状对锂离子电池失效行为的影响,通过改变压头形貌进行整体压缩和局部压痕有限元模拟。在计算过程中,加载速度均设置为0.1 m/s。图5给出了不同压头形状下的力-位移曲线及应力云图。当压头为刚性板时,电池被整体压缩,横截面由圆形变成椭圆形,接触挤压头中间位置的应力最大。在杆棒、球形和圆柱压头挤压下,相当于局部压痕作用,电池中间部分局部变形凹陷,两侧翘起,中间部分变形为挤压头的轮廓。接触挤压头的中心位置应力最大。从图5中可以看出,整体压缩比局部压痕作用下电池失效的峰值力更大,失效位移也更大,说明在局部压痕作用下,电池更容易发生破坏、应力集中和失效。
2.2.2 压头速度的影响
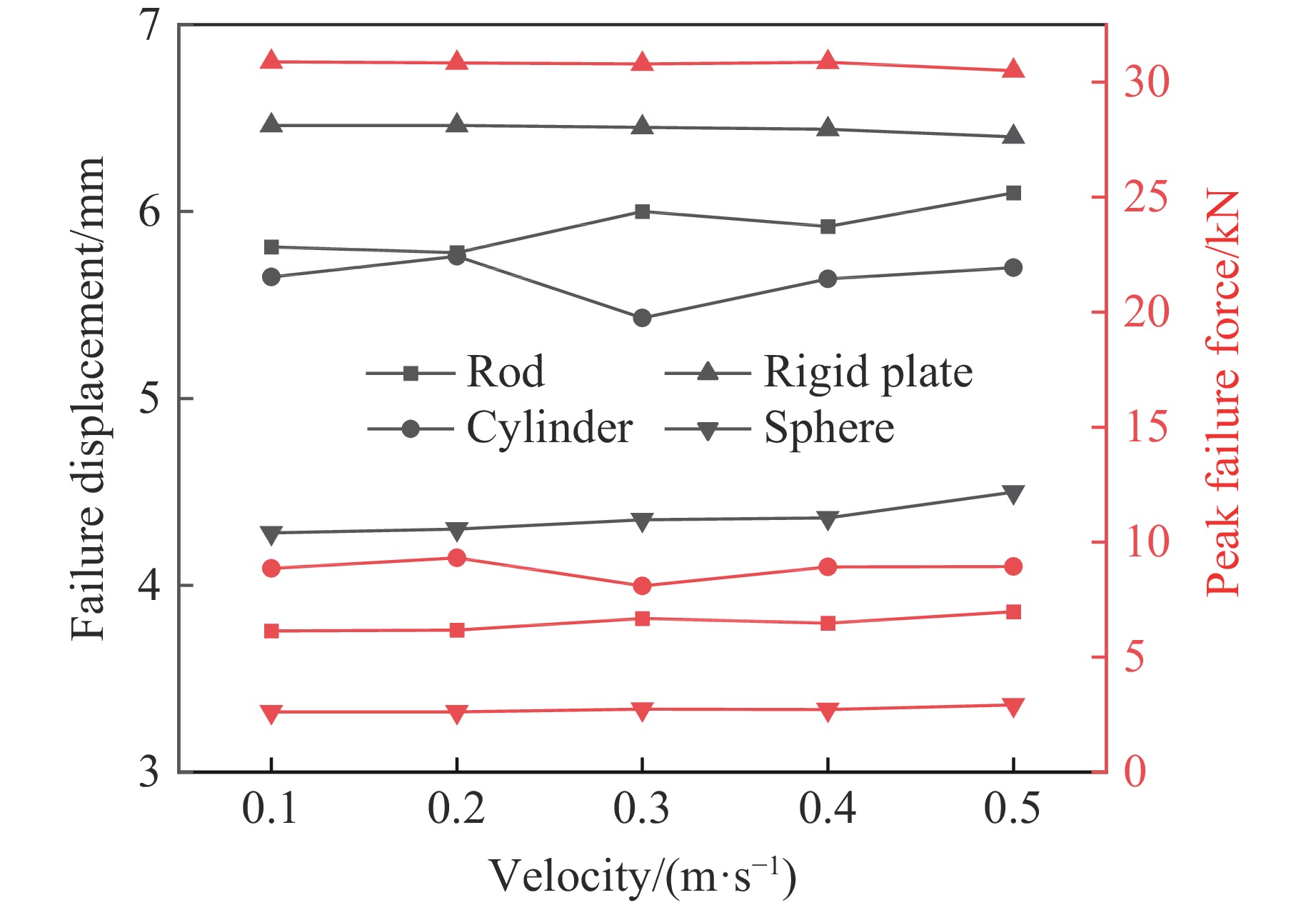
为了研究压头速度对电池失效的影响,在计算过程中,分别对杆棒压头、刚性板、球形压头、圆柱压头施加不同的速度v(0.1、0.2、0.3、0.4和0.5 m/s),观察不同速度下准静态挤压锂离子电池在受到平面压缩和局部压痕时的失效模式。图6给出了不同准静态挤压速度下电池的失效位移和失效峰值力关系。失效位移是指电池在受到冲击或挤压过程中发生失效时的位移。失效峰值力是指电池在受到冲击或挤压过程中,达到失效状态时所承受的最大外力。研究结果表明,尽管压头加载速度存在差异,但相同类型压头的失效位移和失效峰值力基本相同。这说明在准静态挤压工况下,挤压速度并不是电池失效的主要影响因素。
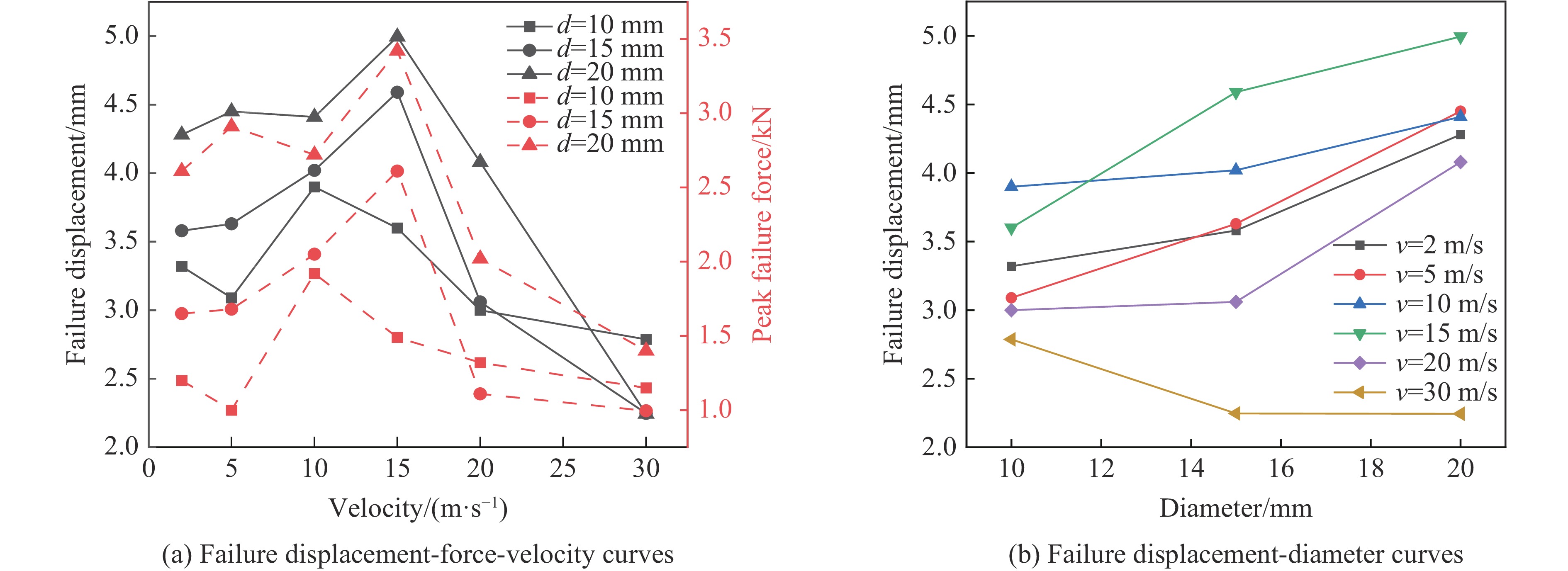
2.2.3 压头直径的影响
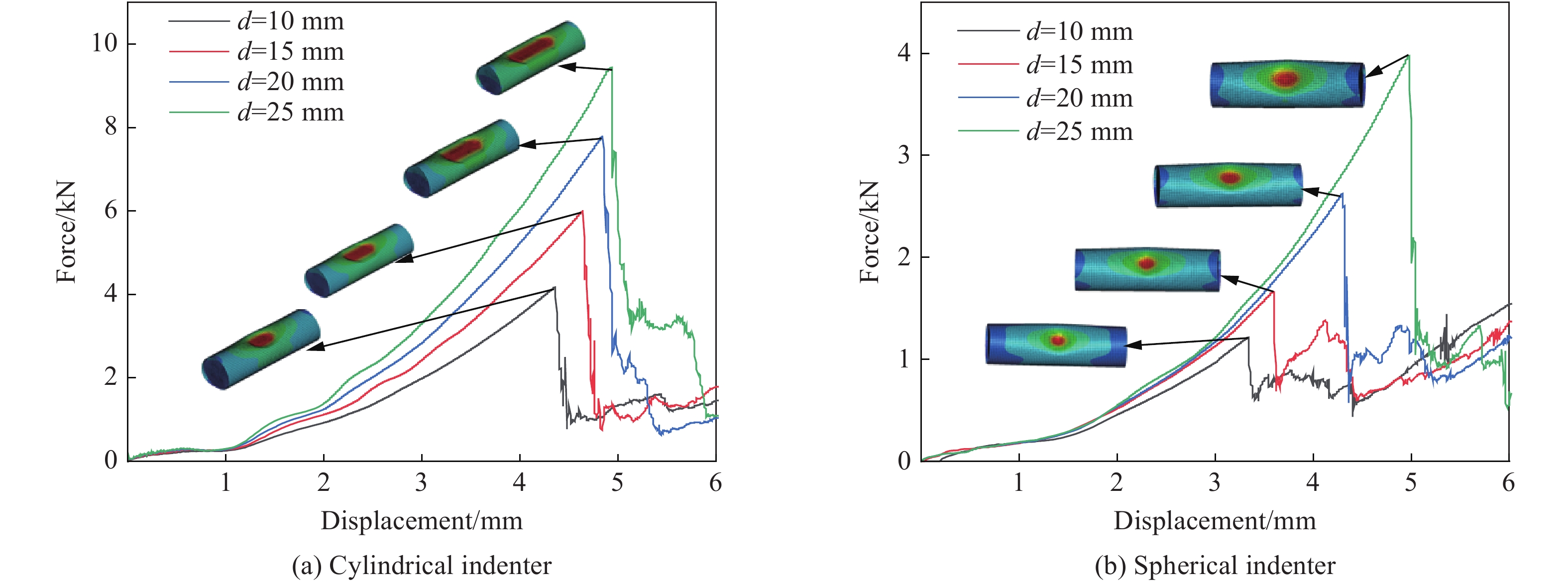
为了研究压头直径对电池失效的影响,对不同直径d(10、15、20和25 mm)的球形和圆柱压头进行数值模拟,加载速度均设置为0.1 m/s。图7分别给出了不同直径的球形和圆柱压头加载下电池的失效力学响应曲线和应力云图。在相同的冲击速度下,随着压头直径的增大,电池发生失效时的位移和峰值力呈增大趋势。究其原因,压头直径越小,电池受力区域越小,更容易诱发电池层间剪切破坏,导致电池局部变形增大,发生破坏所需的最大作用力和最大位移减小。并且,在圆柱和球形压头的压痕中心处,层间剪切破坏现象较为严重。在前一阶段,力-位移曲线呈现上升趋势,说明随着压头的下压,电池所承受的作用力逐渐增大;在后一阶段,曲线出现了明显的峰值后下降趋势,说明电池所承受的作用力达到最大值后开始减小,电池的内部结构已经无法继续承载更多的外部作用力,导致作用力迅速下降,表明电池已经发生失效。
2.3 不同冲击工况下电池失效的影响因素分析
2.3.1 冲击速度的影响
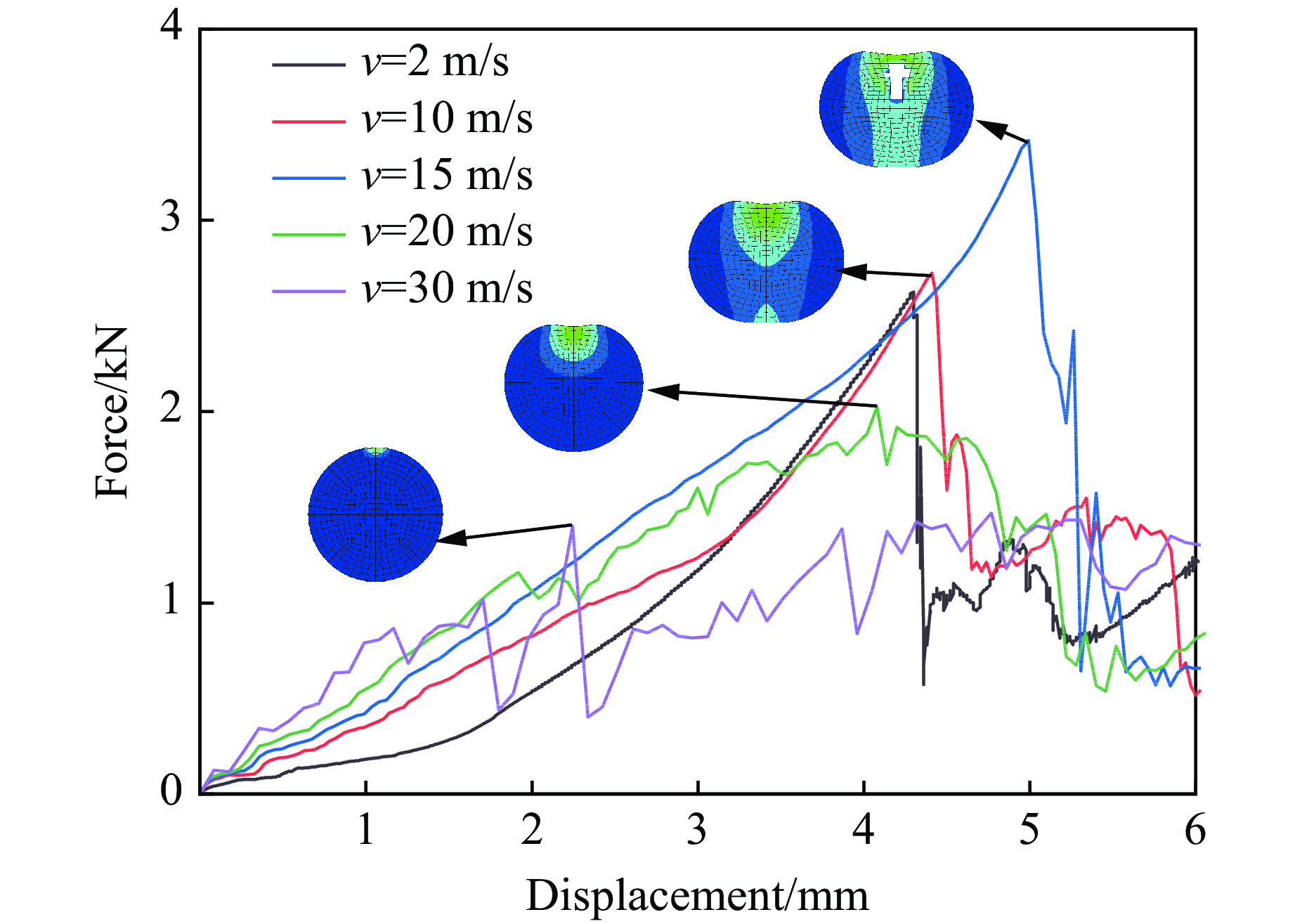
对于球形压头,图8给出了不同冲击速度(v)下电池失效的冲击力-位移曲线(d=20 mm)。选择曲线上的不同位移点分析电池的变形情况。通过前述实验可以看出,电池内部在峰值力点处出现小裂纹,将出现这种现象对应的位移点作为判断电池失效的失效点。研究结果表明,低速冲击时的力-位移曲线具有相似性。在加载过程中,电池受到的冲击力持续增大,短路后迅速减小。图8中的峰值力点表示电池在不同速度下的失效点,并给出了此时的失效情况。不同速度下的冲击力-位移曲线存在差异。在动态冲击下,当发生短路时,冲击力不会减小,甚至可能继续增大,这一现象与低速载荷下短路后峰值力的快速下降[3]不同。当冲击速度提高至30 m/s时,冲击力增大,随后迅速下降,并出现一个平台期。在这个过程中,电池将遭受严重损坏。压缩过程结束时,冲击力再次呈现上升趋势,这些现象就是典型的动态损伤。值得注意的是,高速冲击加载下,电池发生短路失效时的峰值力低于低速冲击下电池短路失效的峰值力,这一现象与文献[3]相同。
2.3.2 冲头形状的影响
图9给出了不同冲头形状下电池的失效位移-失效峰值力曲线和冲头形状。冲击速度相同时,球形冲头冲击电池发生失效时的位移和峰值力最小,杆棒冲头次之,圆柱压头下电池失效位移最大,说明圆柱冲头冲击下的电池最不易发生失效。随着冲击速度的增加,不同冲头下的失效位移基本相同,除圆柱压头外,失效峰值力的差值逐渐减小,说明高速冲击下压头形状不是影响电池失效的主要因素。
2.3.3 冲头直径的影响
图10给出了不同冲击速度下冲头直径对锂离子电池失效位移和失效峰值力的影响。可以看出,当冲击速度小于15 m/s时,随着冲击速度的增加,失效位移和失效峰值力都将增大。对于相同的冲击速度:冲头的直径越大,失效位移和失效峰值力越大;当冲头直径较小时,由于冲击面积较小,承受的冲击力相对集中,导致被冲击物体局部应力过大而发生失效。当冲击速度超过15 m/s时,随着冲击速度的增加,相应的失效位移和失效峰值力均减小,而且不同直径冲头下的失效位移点和失效峰值力点接近,冲击力-位移曲线基本重合。尤其当冲击速度为30 m/s时,随着冲头直径的增加,失效位移几乎不变,说明在高速冲击下冲头直径将不再是影响电池失效的主要因素。
3. 结 论
利用实验与有限元模拟相结合的方法,对不同挤压/冲击工况下圆柱形锂离子电池失效的影响因素进行了研究,得到如下主要结论。
(1) 在平面压缩和局部压痕准静态实验中,初始阶段负载随着电池位移的增加而增大,而温度和电压几乎保持不变。以拐点和峰值力为特征点描述变形过程,在电池失效前分为3个阶段:第1阶段电池壳体承受主要负载;第2阶段电池内部的空隙逐渐被压实;第3阶段电池整体受力,内部开始损坏并逐渐扩展,累积至整体发生故障。
(2) 在准静态挤压工况下,加载速度不变,而改变挤压形式,则局部压痕相较于平面压缩更容易使锂离子电池发生失效。同种压头下,改变加载速度得到的力-位移曲线基本吻合;改变压头直径后,随着压头直径的减小,电池峰值力明显降低,失效位移减小。
(3) 动态冲击工况下,高速冲击过程中电池失效前冲击力会有一个短暂的平台期,发生破坏后冲击力仍然增大。当冲击速度不大于15 m/s时,随着冲头直径的增加,失效位移和失效峰值力均会增加,且差值较大;当冲击速度超过15 m/s时,不同直径冲头的失效位移和失效峰值力差值均逐渐减小,尤其当冲击速度大于30 m/s时,不同直径冲头下的力-位移曲线几乎重合,冲头直径对电池失效的影响较小。
-
图 3 金刚石压腔静高压实验中一些典型的用于研究铁高压熔化的诊断方法与标准:(a) XRD[10],(b) SMS[151], (c) XAS[154],(d) 电阻率[155],(e) 激光功率与温度的关系[10]
Figure 3. Some typical diagnostic methods and criteria used to study the melting behavior of iron at high pressures in heated DAC experiments: (a) XRD[10], (b) SMS[151], (c) XAS[154], (d) resistivity[155], (e) relationship between laser power and temperature[10]
图 4 动高压实验中常用的冲击熔化诊断方法[40, 120, 156]:(a) 冲击压力与Hugoniot温度关系的不连续,(b) 沿着冲击绝热线声速的不连续,(c) 冲击下hcp结构的X射线衍射峰消失[120]
Figure 4. Typical diagnostics for the shock-induced melting in dynamic compression experiments[40, 120, 156]: (a) discontinuity of the relationship between shock pressure and Hugoniot temperature, (b) discontinuity of sound velocity along the Hugoniot, (c) the disappearance of XRD peak for hcp structure under shock loading[120]
图 5 铁高压熔化温度的典型静高压实验研究结果[10, 143-155](绿色、红色、蓝色和洋红色图例分别表示采用直接显微观察样品表面、XRD、SMS和XAS获得的LH-DAC静高压实验结果,橙色图例表示采用电阻率作为诊断方法获得的RH-DAC静高压实验结果)
Figure 5. Typical results of the melting temperatures of iron at high pressures using static compression experiments[10, 143-155] (The green, red, blue, and magenta legends represent the experimental results of LH-DAC experiments obtained by direct microscopic observation of sample surface, XRD, SMS, and XAS as diagnostic methods, respectively; the orange legends represent the experimental results of RH-DAC experiments using resistivity measurements as the diagnostic method.)
图 6 铁高压熔化温度的典型动高压实验研究结果[47, 119-120, 143, 157, 161, 163-164](实心图例表示采用多通道瞬态辐射高温计直接测得的铁的Hugoniot温度和熔化温度,其中绿色[143]、蓝色[157]和洋红色[119]图例代表使用微米厚铁膜或铁箔、4~6个波长通道的高温计测得的Hugoniot温度,红色图例[47]代表使用毫米厚块状铁、16个波长通道的高温计测得的Hugoniot温度;空心图例表示高功率激光加载动高压实验中采用XAS和XRD测得的铁的冲击熔化,通过热力学计算得到的Hugoniot温度)
Figure 6. Typical results of the melting temperatures of iron at high pressures by dynamic compression experiments[47, 119-120, 143, 157, 161, 163-164] (The solid legends show that Hugoniot temperatures and melting temperatures of iron are directly measured by a multichannel transient radiation pyrometer; the legends of green[143], blue[157] and magenta[119] represent the Hugoniot temperatures of iron measured by a pyrometer with 4–6 wavelength channels using iron film (foil) sample; the red legend[47] represent the Hugoniot temperatures of iron measured by a pyrometer with 16 wavelength channels using bulk iron sample; the open legends represent that the Hugoniot temperatures of iron are calculated through thermodynamic calculations from the shock melting measurements by XAS and XRD in the dynamic compression experiments of high power laser loading.)
图 7 铁高压熔化温度的典型动高压和静高压实验结果对比[10, 47, 120, 154-155, 163](动高压实验结果与静高压实验结果具有良好的一致性,尤其是采用XAS[154]和XRD[10]作为诊断技术的LH-DAC静高压实验和改进样品靶结构并采用多通道瞬态辐射高温计[47]的动高压实验结果之间)
Figure 7. A comparison of the typical results on the melting temperatures of iron at high pressures obtained by the static and dynamic compression experiments[10, 47, 120, 154–155, 163] (The results are generally consistent with each other between dynamic and static experiments. In particular, the results of LH-DAC static experiments using XAS[154] and XRD[10] as diagnostic techniques are in good agreement with those of dynamic experiments using improved pyrometry[47].)
图 8 铁高压熔化温度的理论模拟和热力学计算结果[129-142](理论研究主要包括两类:基于分子动力学或第一性原理的理论模拟以及基于热力学状态参数的热力学计算)
Figure 8. Melting temperatures of iron at high pressures using theoretical simulations and thermodynamic calculations[129-142] (Theoretical studies mainly include two categories: simulations based on molecular dynamics or first-principles and thermodynamic calculations based on thermodynamic parameters.)
图 9 铁高压熔化线的典型研究结果[10, 47, 119, 129-135, 138-139, 141, 143, 145-146, 155, 157](20年前铁高压熔化线的实验与理论研究结果之间存在较大的差异,而目前实验与理论研究结果之间已经基本吻合)
Figure 9. Typical results of the melting curves of iron at high pressures[10, 47, 119, 129-135, 138-139, 141, 143, 145-146, 155, 157] (Twenty years ago, there was a big difference in the melting curves of iron between experimental and theoretical studies, while the current studies show an overall agreement between experimental and theoretical results.)
表 1 高压下铁的熔化温度的实验和理论研究总结
Table 1. Summary of experimental and theoretical studies on the melting temperature of iron at high pressures
Technique Method Melting diagnostic Expt. and theo. conditions TM, ICB/K Reference, year p/GPa TM(TH)/K Theory Ab initio DFT Free energies 50–350 3020–6860 6680±600 Ref.[129], 1999 Thermodynamics Database 0–330 1811–5790 5790 Ref.[130], 2000 AIMD Free energies 60–330 2460–7100 7100 Ref.[131], 2000 FP-MD A single potential 100–330 2830–5400 5400±400 Ref.[132], 2000 Ab initio DFT Free energies 50–350 2550–6380a 6210±600a Ref.[133], 2002 Ab initio DFT Free energies 50–350 2890–6510b 6350±600b Ref.[133], 2002 Thermodynamics Free energy 58–462 2840–7240 6050 Ref.[134], 2003 FP Number of atoms 323–332 6270–6440 6370±100 Ref.[135], 2009 Monte Carlo Free energies 330 6900 6900±400 Ref.[136], 2009 Thermodynamics Database 0–353 1810–5080 4900 Ref.[137], 2010 AIMD Free energies 190–1500 4500–12500 6150 Ref.[138], 2013 AIMD Structure 0–365 1700–6740 6350 Ref.[139], 2015 Thermodynamics Database 107–350 3790–6020 5880 Ref.[140], 2017 AIMD Free energies 330 6170 6170±200 Ref.[141], 2018 SMM LC 0–350 1810–5720 5570 Ref.[142], 2021 Static LH-DAC(s) Textural 0–102 1750–4180 7600±500 Ref.[143], 1987 Motion 16–197 2220–3860 4850±200 Ref.[144-145], 1993 Visual bservation 0–144 1811–3530 6130±350 Ref.[146], 1994 XRD 11–80 2100–3090 Ref.[147-148], 2004 XRD 60–105 2750–3510 5800±200 Ref.[149], 2004 XRD 27–130 2580–3180 Ref.[150], 2008 SMS 20–82 2220–3030 Ref.[151], 2013 Fast XRD 57–158 3140–4470 6230±500 Ref.[10], 2013 XANES 75–117 2840–3090 Ref.[152], 2015 SMS 19–60 2120–2800 5700±200 Ref.[153], 2016 XANES 43–133 2660–4700 Ref.[154], 2018 RH-DAC Resistivity 6–290 1900–5360 5500±220 Ref.[155], 2019 Dynamic
(shock wave)TSLGG SVD 40–400 655–10024c 5800±500 Ref.[156], 1986 TSLGG T-p discontinuity 202–301 5500–9370* 7600±500 Ref.[143], 1987 TSLGG T-p discontinuity 203–300 5200–8990* 7800±500 Ref.[157], 1987 TSLGG T-p discontinuity 159–339 4460–8360* 6830±500 Ref.[119], 1993 TSLGG SVD 84–171 4380–5440e* 6000 Ref.[158-159], 2001 PG SVD 14–73 1820–2780f 5300±400 Ref.[160], 2002 TSLGG SVD 225–260 5100–6100d 6350±500 Ref.[40], 2004 HP laser SED 50–150 4000–5000# 7800±1200 Ref.[41], 2005 HP laser EXAFS 90–560 1320–8160# 6400 Ref.[161], 2013 TSLGG SVD 73–127 3240–3680e 5885±500 Ref.[162], 2009 HP laser XANES 260–420 5680–10800# Ref.[163], 2015 HP laser EXAFS 40–500 660–17000# Ref.[164], 2016 HP laser XRD 144–273 3100–5560# 6400 Ref.[120], 2020 TSLGG SVD 120–256 4250–5500* 5950±500 Ref.[47], 2020 Note: Superscript lowercase letters “a” and “b” represent the theoretical results of ab initio molecular dynamics simulation
without and with free-energy correction, respectively; “c” and “d” represent that the Hugoniot temperatures were calculated
based on the measurements of sound velocities of iron and preheated iron, respectively; “e” represents the porous iron was
used in shock compression experiments; “*” represents that the Hugoniot temperatures were measured by a multi-channel
quasi-spectral optical pyrometer; “#” represents that the Hugoniot temperatures were calculated through thermodynamic
calculations based on the results of dynamic compression experiments of high power laser loading. -
[1] 谭华. 实验冲击波物理 [M]. 北京: 国防工业出版社, 2018.TAN H. Experimental shock wave physics [M]. Beijing: National Defense Industry Press, 2018. [2] GORMAN M G, BRIGGS R, MCBRIDE E E, et al. Direct observation of melting in shock-compressed bismuth with femtosecond X-ray diffraction [J]. Physical Review Letters, 2015, 115(9): 095701. doi: 10.1103/PhysRevLett.115.095701 [3] JENSEN B J, CHERNE F J, COOLEY J C, et al. Shock melting of cerium [J]. Physical Review B, 2010, 81(21): 214109. doi: 10.1103/PhysRevB.81.214109 [4] ROSS M, YANG L H, BOEHLER R. Melting of aluminum, molybdenum, and the light actinides [J]. Physical Review B, 2004, 70(18): 184112. doi: 10.1103/PhysRevB.70.184112 [5] ERRANDONEA D, BOEHLER R, ROSS M. Melting of the rare Earth metals and f-electron delocalization [J]. Physical Review Letters, 2000, 85(16): 3444–3447. doi: 10.1103/PhysRevLett.85.3444 [6] PATEL N N, SUNDER M. High pressure melting curve of osmium up to 35 GPa [J]. Journal of Applied Physics, 2019, 125(5): 055902. doi: 10.1063/1.5045823 [7] YAP C Y, CHUA C K, DONG Z L, et al. Review of selective laser melting: materials and applications [J]. Applied Physics Reviews, 2015, 2(4): 041101. doi: 10.1063/1.4935926 [8] BOYER R R. An overview on the use of titanium in the aerospace industry [J]. Materials Science and Engineering: A, 1996, 213(1/2): 103–114. doi: 10.1016/0921-5093(96)10233-1 [9] FISCHER R A. Melting of Fe alloys and the thermal structure of the core [M]//TERASAKI H, FISCHER R A. Deep Earth: Physics and Chemistry of the Lower Mantle and Core. Hoboken: John Wiley, 2016: 3–12. [10] ANZELLINI S, DEWAELE A, MEZOUAR M, et al. Melting of iron at Earth’s inner core boundary based on fast X-ray diffraction [J]. Science, 2013, 340(6131): 464–466. doi: 10.1126/science.1233514 [11] PARISIADES P. A review of the melting curves of transition metals at high pressures using static compression techniques [J]. Crystals, 2021, 11(4): 416. doi: 10.3390/cryst11040416 [12] PARISIADES P, COVA F, GARBARINO G. Melting curve of elemental zirconium [J]. Physical Review B, 2019, 100(5): 054102. doi: 10.1103/PhysRevB.100.054102 [13] JAPEL S, SCHWAGER B, BOEHLER R, et al. Melting of copper and nickel at high pressure: the role of d electrons [J]. Physical Review Letters, 2005, 95(16): 167801. doi: 10.1103/PhysRevLett.95.167801 [14] ROSS M, BOEHLER R, ERRANDONEA D. Melting of transition metals at high pressure and the influence of liquid frustration: the late metals Cu, Ni, and Fe [J]. Physical Review B, 2007, 76(18): 184117. doi: 10.1103/PhysRevB.76.184117 [15] ALFÈ D, VOČADLO L, PRICE G D, et al. Melting curve of materials: theory versus experiments [J]. Journal of Physics: Condensed Matter, 2004, 16(14): S973–S982. doi: 10.1088/0953-8984/16/14/006 [16] DAI C D, HU J B, TAN H. Hugoniot temperatures and melting of tantalum under shock compression determined by optical pyrometry [J]. Journal of Applied Physics, 2009, 106(4): 043519. doi: 10.1063/1.3204941 [17] SANTAMARÍA-PÉREZ D, ROSS M, ERRANDONEA D, et al. X-ray diffraction measurements of Mo melting to 119 GPa and the high pressure phase diagram [J]. The Journal of Chemical Physics, 2009, 130(12): 124509. doi: 10.1063/1.3082030 [18] ERRANDONEA D. Improving the understanding of the melting behaviour of Mo, Ta, and W at extreme pressures [J]. Physica B: Condensed Matter, 2005, 357(3/4): 356–364. doi: 10.1016/j.physb.2004.11.087 [19] PIGOTT J S, VELISAVLJEVIC N, MOSS E K, et al. Experimental melting curve of zirconium metal to 37 GPa [J]. Journal of Physics: Condensed Matter, 2020, 32(35): 355402. doi: 10.1088/1361-648X/ab8cdb [20] ERRANDONEA D. High-pressure melting curves of the transition metals Cu, Ni, Pd, and Pt [J]. Physical Review B, 2013, 87(5): 054108. doi: 10.1103/PhysRevB.87.054108 [21] LAZOR P, SHEN G, SAXENA S K. Laser-heated diamond anvil cell experiments at high pressure: melting curve of nickel up to 700 kbar [J]. Physics and Chemistry of Minerals, 1993, 20(2): 86–90. doi: 10.1007/BF00207200 [22] GREGORYANZ E, DEGTYAREVA O, SOMAYAZULU M, et al. Melting of dense sodium [J]. Physical Review Letters, 2005, 94(18): 185502. doi: 10.1103/PhysRevLett.94.185502 [23] NARYGINA O, MCBRIDE E E, STINTON G W, et al. Melting curve of potassium to 22 GPa [J]. Physical Review B, 2011, 84(5): 054111. doi: 10.1103/PhysRevB.84.054111 [24] GUILLAUME C L, GREGORYANZ E, DEGTYAREVA O, et al. Cold melting and solid structures of dense lithium [J]. Nature Physics, 2011, 7(3): 211–214. doi: 10.1038/nphys1864 [25] KULYAMINA E Y, ZITSERMAN V Y, FOKIN L R. Titanium melting curve: data consistency assessment, problems and achievements [J]. Technical Physics, 2018, 63(3): 369–373. doi: 10.1134/S1063784218030131 [26] BUFFETT B A. Earth’s core and the geodynamo [J]. Science, 2000, 288(5473): 2007–2012. doi: 10.1126/science.288.5473.2007 [27] LABROSSE S. Thermal evolution of the core with a high thermal conductivity [J]. Physics of the Earth and Planetary Interiors, 2015, 247: 36–55. doi: 10.1016/j.pepi.2015.02.002 [28] LAY T, HERNLUND J W, BUFFETT B A. Core-mantle boundary heat flow [J]. Nature Geoscience, 2008, 1(1): 25–32. doi: 10.1038/ngeo.2007.44 [29] ALBOUSSIÈRE T, DEGUEN R, MELZANI M. Melting-induced stratification above the Earth’s inner core due to convective translation [J]. Nature, 2010, 466(7307): 744–747. doi: 10.1038/nature09257 [30] GUBBINS D, SREENIVASAN B, MOUND J, et al. Melting of the Earth’s inner core [J]. Nature, 2011, 473(7347): 361–363. doi: 10.1038/nature10068 [31] BUFFETT B A. The thermal state of Earth’s core [J]. Science, 2003, 299(5613): 1675–1677. doi: 10.1126/science.1081518 [32] NIMMO F. Energetics of the core [J]. Treatise on Geophysics, 2015, 8: 27–55. doi: 10.1016/B978-0-444-53802-4.00139-1 [33] DEWAELE A, LOUBEYRE P, OCCELLI F, et al. Toroidal diamond anvil cell for detailed measurements under extreme static pressures [J]. Nature Communications, 2018, 9(1): 2913. doi: 10.1038/s41467-018-05294-2 [34] DUBROVINSKAIA N, DUBROVINSKY L, SOLOPOVA N A, et al. Terapascal static pressure generation with ultrahigh yield strength nanodiamond [J]. Science Advances, 2016, 2(7): e1600341. doi: 10.1126/sciadv.1600341 [35] DUBROVINSKY L, DUBROVINSKAIA N, PRAKAPENKA V B, et al. Implementation of micro-ball nanodiamond anvils for high-pressure studies above 6 Mbar [J]. Nature Communications, 2012, 3(1): 1163. doi: 10.1038/ncomms2160 [36] SWIFT D C, JOHNSON R P. Quasi-isentropic compression by ablative laser loading: response of materials to dynamic loading on nanosecond time scales [J]. Physical Review E, 2005, 71(6): 066401. doi: 10.1103/PhysRevE.71.066401 [37] KALITA P, BROWN J, SPECHT P, et al. Dynamic X-ray diffraction and nanosecond quantification of kinetics of formation of β-zirconium under shock compression [J]. Physical Review B, 2020, 102(6): 060101. doi: 10.1103/PhysRevB.102.060101 [38] EGGERT J H, HICKS D G, CELLIERS P M, et al. Melting temperature of diamond at ultrahigh pressure [J]. Nature Physics, 2010, 6(1): 40–43. doi: 10.1038/nphys1438 [39] DUFFY T S, SMITH R F. Ultra-high pressure dynamic compression of geological materials [J]. Frontiers in Earth Science, 2019, 7: 23. doi: 10.3389/FEART.2019.00023 [40] NGUYEN J H, HOLMES N C. Melting of iron at the physical conditions of the Earth’s core [J]. Nature, 2004, 427(6972): 339–342. doi: 10.1038/nature02248 [41] HUSER G, KOENIG M, BENUZZI-MOUNAIX A, et al. Temperature and melting of laser-shocked iron releasing into an LiF window [J]. Physics of Plasmas, 2005, 12(6): 060701. doi: 10.1063/1.1896375 [42] GLENZER S H, FLETCHER L B, GALTIER E, et al. Matter under extreme conditions experiments at the Linac Coherent Light Source [J]. Journal of Physics B: Atomic, Molecular and Optical Physics, 2016, 49(9): 092001. doi: 10.1088/0953-4075/49/9/092001 [43] NAGLER B, ARNOLD B, BOUCHARD G, et al. The matter in extreme conditions instrument at the linac coherent light source [J]. Journal of Synchrotron Radiation, 2015, 22(3): 520–525. doi: 10.1107/S1600577515004865 [44] MASON P, BANERJEE S, SMITH J, et al. Development of a 100 J, 10 Hz laser for compression experiments at the High Energy Density instrument at the European XFEL [J]. High Power Laser Science and Engineering, 2018, 6: e65. doi: 10.1017/hpl.2018.56 [45] ZHANG Y J, SEKINE T, LIN J F, et al. Shock compression and melting of an Fe-Ni-Si alloy: implications for the temperature profile of the Earth’s core and the heat flux across the core-mantle boundary [J]. Journal of Geophysical Research: Solid Earth, 2018, 123(2): 1314–1327. doi: 10.1002/2017JB014723 [46] ZHANG Y J, TAN Y, GENG H Y, et al. Melting curve of vanadium up to 256 GPa: consistency between experiments and theory [J]. Physical Review B, 2020, 102(21): 214104. doi: 10.1103/PhysRevB.102.214104 [47] LI J, WU Q, LI J B, et al. Shock melting curve of iron: a consensus on the temperature at the Earth’s inner core boundary [J]. Geophysical Research Letters, 2020, 47(15): e2020GL087758. doi: 10.1029/2020GL087758 [48] MCMAHAN A K. Pressure-induced changes in the electronic structure of solids [J]. Physica B, 1986, 139/140: 31–41. doi: 10.1016/0378-4363(86)90519-X [49] POUROVSKII L V. Electronic correlations in dense iron: from moderate pressure to Earth’s core conditions [J]. Journal of Physics: Condensed Matter, 2019, 31(37): 373001. doi: 10.1088/1361-648X/ab274f [50] LUO S N, AHRENS T J, ÇAĞIN T, et al. Maximum superheating and undercooling: systematics, molecular dynamics simulations, and dynamic experiments [J]. Physical Review B, 2003, 68(13): 134206. doi: 10.1103/PhysRevB.68.134206 [51] MORRIS J R, WANG C Z, HO K M, et al. Melting line of aluminum from simulations of coexisting phases [J]. Physical Review B, 1994, 49(5): 3109–3115. doi: 10.1103/PhysRevB.49.3109 [52] BELONOSHKO A B. Molecular dynamics of MgSiO3 perovskite at high pressures: equation of state, structure, and melting transition [J]. Geochimica et Cosmochimica Acta, 1994, 58(19): 4039–4047. doi: 10.1016/0016-7037(94)90265-8 [53] GILLAN M J, ALFÈ D, BRODHOLT J, et al. First-principles modelling of Earth and planetary materials at high pressures and temperatures [J]. Reports on Progress in Physics, 2006, 69(8): 2365–2441. doi: 10.1088/0034-4885/69/8/R03 [54] GENG H Y, WU Q. Predicted reentrant melting of dense hydrogen at ultra-high pressures [J]. Scientific Reports, 2016, 6(1): 36745. doi: 10.1038/srep36745 [55] GENG H Y, WU Q, MARQUÉS M, et al. Thermodynamic anomalies and three distinct liquid-liquid transitions in warm dense liquid hydrogen [J]. Physical Review B, 2019, 100(13): 134109. doi: 10.1103/PhysRevB.100.134109 [56] CAZORLA C, ALFÈ D, GILLAN M J. Constraints on the phase diagram of molybdenum from first-principles free-energy calculations [J]. Physical Review B, 2012, 85(6): 064113. doi: 10.1103/PhysRevB.85.064113 [57] BELONOSHKO A B, ROSENGREN A. High-pressure melting curve of platinum from ab initio Z method [J]. Physical Review B, 2012, 85(17): 174104. doi: 10.1103/PhysRevB.85.174104 [58] BELONOSHKO A B, DAVIS S, SKORODUMOVA N V, et al. Properties of the fcc Lennard-Jones crystal model at the limit of superheating [J]. Physical Review B, 2007, 76(6): 064121. doi: 10.1103/PhysRevB.76.064121 [59] WANG S C, ZHANG G M, LIU H F, et al. Modified Z method to calculate melting curve by molecular dynamics [J]. The Journal of Chemical Physics, 2013, 138(13): 134101. doi: 10.1063/1.4798225 [60] FROST D J, POE B T, TRØNNES R G, et al. A new large-volume multianvil system [J]. Physics of the Earth and Planetary Interiors, 2004, 143/144: 507–514. doi: 10.1016/J.PEPI.2004.03.003 [61] SHCHEKA S S, WIEDENBECK M, FROST D J, et al. Carbon solubility in mantle minerals [J]. Earth and Planetary Science Letters, 2006, 245(3/4): 730–742. doi: 10.1016/j.jpgl.2006.03.036 [62] NIETO-SANZ D, LOUBEYRE P, CRICHTON W, et al. X-ray study of the synthesis of boron oxides at high pressure: phase diagram and equation of state [J]. Physical Review B, 2004, 70(21): 214108. doi: 10.1103/PhysRevB.70.214108 [63] HORVATH-BORDON E, RIEDEL R, ZERR A, et al. High-pressure chemistry of nitride-based materials [J]. Chemical Society Reviews, 2006, 35(10): 987–1014. doi: 10.1039/b517778m [64] ITO E, YAMAZAKI D, YOSHINO T, et al. Pressure generation and investigation of the post-perovskite transformation in MgGeO3 by squeezing the Kawai-cell equipped with sintered diamond anvils [J]. Earth and Planetary Science Letters, 2010, 293(1/2): 84–89. doi: 10.1016/j.jpgl.2010.02.023 [65] ZHOU X F, MA D J, WANG L F, et al. Large-volume cubic press produces high temperatures above 4000 Kelvin for study of the refractory materials at pressures [J]. Review of Scientific Instruments, 2020, 91(1): 015118. doi: 10.1063/1.5128190 [66] LAZICKI A, FEI Y W, HEMLEY R J. High-pressure differential thermal analysis measurements of the melting curve of lithium [J]. Solid State Communications, 2010, 150(13/14): 625–627. doi: 10.1016/j.ssc.2009.12.029 [67] SILBER R E, SECCO R A, YONG W J. Constant electrical resistivity of Ni along the melting boundary up to 9 GPa [J]. Journal of Geophysical Research: Solid Earth, 2017, 122(7): 5064–5081. doi: 10.1002/2017JB014259 [68] EZENWA I C, SECCO R A. Invariant electrical resistivity of Co along the melting boundary [J]. Earth and Planetary Science Letters, 2017, 474: 120–127. doi: 10.1016/j.jpgl.2017.06.032 [69] BERRADA M, SECCO R A, YONG W J. Decreasing electrical resistivity of gold along the melting boundary up to 5 GPa [J]. High Pressure Research, 2018, 38(4): 367–376. doi: 10.1080/08957959.2018.1493476 [70] BRAND H, DOBSON D P, VOČADLO L, et al. Melting curve of copper measured to 16 GPa using a multi-anvil press [J]. High Pressure Research, 2006, 26(3): 185–191. doi: 10.1080/08957950600873089 [71] MING L C, BASSETT W A. Laser heating in the diamond anvil press up to 2000 ℃ sustained and 3000 ℃ pulsed at pressures up to 260 kilobars [J]. Review of Scientific Instruments, 1974, 45(9): 1115–1118. doi: 10.1063/1.1686822 [72] ANZELLINI S, BOCCATO S. A practical review of the laser-heated diamond anvil cell for university laboratories and synchrotron applications [J]. Crystals, 2020, 10(6): 459. doi: 10.3390/cryst10060459 [73] LIN J F, SHU J F, MAO H K, et al. Amorphous boron gasket in diamond anvil cell research [J]. Review of Scientific Instruments, 2003, 74(11): 4732–4736. doi: 10.1063/1.1621065 [74] FUNAMORI N, SATO T. A cubic boron nitride gasket for diamond-anvil experiments [J]. Review of Scientific Instruments, 2008, 79(5): 053903. doi: 10.1063/1.2917409 [75] HEMLEY R J, MAO H K, SHEN G Y, et al. X-ray imaging of stress and strain of diamond, iron, and tungsten at megabar pressures [J]. Science, 1997, 276(5316): 1242–1245. doi: 10.1126/science.276.5316.1242 [76] MAO H K, SHU J F, SHEN G Y, et al. Elasticity and rheology of iron above 220 GPa and the nature of the Earth’s inner core [J]. Nature, 1998, 396(6713): 741–743. doi: 10.1038/25506 [77] WU T C, BASSETT W A. Deviatoric stress in a diamond anvil cell using synchrotron radiation with two diffraction geometries [J]. Pure and Applied Geophysics, 1993, 141(2): 509–519. doi: 10.1007/BF00998343 [78] KLOTZ S, CHERVIN J C, MUNSCH P, et al. Hydrostatic limits of 11 pressure transmitting media [J]. Journal of Physics D: Applied Physics, 2009, 42(7): 075413. doi: 10.1088/0022-3727/42/7/075413 [79] DEWAELE A, LOUBEYRE P. Pressurizing conditions in helium-pressure-transmitting medium [J]. High Pressure Research, 2007, 27(4): 419–429. doi: 10.1080/08957950701659627 [80] MAO H K, BELL P M, SHANER J W, et al. Specific volume measurements of Cu, Mo, Pd, and Ag and calibration of the ruby R1 fluorescence pressure gauge from 0.06 to 1 Mbar [J]. Journal of Applied Physics, 1978, 49(6): 3276–3283. doi: 10.1063/1.325277 [81] BOSE S. Planck’s law and the light quantum hypothesis [J]. Journal of Astrophysics and Astronomy, 1994, 15(1): 3–7. doi: 10.1007/BF03010400 [82] WEIK M H. Planck’s law [M]//WEIK M H. Computer Science and Communications Dictionary. Boston: Springer, 2000: 1284. [83] BENEDETTI L R, LOUBEYRE P. Temperature gradients, wavelength-dependent emissivity, and accuracy of high and very-high temperatures measured in the laser-heated diamond cell [J]. High Pressure Research, 2004, 24(4): 423–445. doi: 10.1080/08957950412331331718 [84] BENEDETTI L R, ANTONANGELI D, FARBER D L, et al. An integrated method to determine melting temperatures in high-pressure laser-heating experiments [J]. Applied Physics Letters, 2008, 92(14): 141903. doi: 10.1063/1.2906893 [85] DEWAELE A, TORRENT M, LOUBEYRE P, et al. Compression curves of transition metals in the Mbar range: experiments and projector augmented-wave calculations [J]. Physical Review B, 2008, 78(10): 104102. doi: 10.1103/PhysRevB.78.104102 [86] SHEN G Y, WANG Y B, DEWAELE A, et al. Toward an international practical pressure scale: a proposal for an IPPS ruby gauge (IPPS-Ruby2020) [J]. High Pressure Research, 2020, 40(3): 299–314. doi: 10.1080/08957959.2020.1791107 [87] DATCHI F, DEWAELE A, LOUBEYRE P, et al. Optical pressure sensors for high-pressure-high-temperature studies in a diamond anvil cell [J]. High Pressure Research, 2007, 27(4): 447–463. doi: 10.1080/08957950701659593 [88] AKAHAMA Y, KAWAMURA H. Pressure calibration of diamond anvil Raman gauge to 410 GPa [J]. Journal of Physics: Conference Series, 2010, 215: 012195. doi: 10.1088/1742-6596/215/1/012195 [89] DEWAELE A, BELONOSHKO A B, GARBARINO G, et al. High-pressure-high-temperature equation of state of KCl and KBr [J]. Physical Review B, 2012, 85(21): 214105. doi: 10.1103/PhysRevB.85.214105 [90] TANGE Y, NISHIHARA Y, TSUCHIYA T. Unified analyses for P-V-T equation of state of MgO: a solution for pressure-scale problems in high P-T experiments [J]. Journal of Geophysical Research: Solid Earth, 2009, 114(B3): B03208. doi: 10.1029/2008JB005813 [91] MATSUI M, PARKER S C, LESLIE M. The MD simulation of the equation of state of MgO: application as a pressure calibration standard at high temperature and high pressure [J]. American Mineralogist, 2000, 85(2): 312–316. doi: 10.2138/am-2000-2-308 [92] MAO H K, BELL P M. Equations of state of MgO and ε-Fe under static pressure conditions [J]. Journal of Geophysical Research: Solid Earth, 1979, 84(B9): 4533–4536. doi: 10.1029/JB084iB09p04533 [93] DECKER D L. High-pressure equation of state for NaCl, KCl, and CsCl [J]. Journal of Applied Physics, 1971, 42(8): 3239–3244. doi: 10.1063/1.1660714 [94] DECKER D L. Equation of state of NaCl and its use as a pressure gauge in high-pressure research [J]. Journal of Applied Physics, 1965, 36(1): 157–161. doi: 10.1063/1.1713864 [95] YE Y, SHIM S H, PRAKAPENKA V, et al. Equation of state of solid Ne inter-calibrated with the MgO, Au, Pt, NaCl-B2, and ruby pressure scales up to 130 GPa [J]. High Pressure Research, 2018, 38(4): 377–395. doi: 10.1080/08957959.2018.1493477 [96] SHEELENDRA K, VIJAY A. Equation of state, thermoelastic properties and melting behavior of NaCl at high temperatures and high pressures [J]. Journal of Physics and Chemistry of Solids, 2018, 123: 364–370. doi: 10.1016/j.jpcs.2018.08.027 [97] FAN D W, ZHOU W G, WEI S Y, et al. A simple external resistance heating diamond anvil cell and its application for synchrotron radiation X-ray diffraction [J]. Review of Scientific Instruments, 2010, 81(5): 053903. doi: 10.1063/1.3430069 [98] JENEI Z, CYNN H, VISBECK K, et al. High-temperature experiments using a resistively heated high-pressure membrane diamond anvil cell [J]. Review of Scientific Instruments, 2013, 84(9): 095114. doi: 10.1063/1.4821622 [99] WEIR S T, JACKSON D D, FALABELLA S, et al. An electrical microheater technique for high-pressure and high-temperature diamond anvil cell experiments [J]. Review of Scientific Instruments, 2009, 80(1): 013905. doi: 10.1063/1.3069286 [100] PASTERNAK S, AQUILANTI G, PASCARELLI S, et al. A diamond anvil cell with resistive heating for high pressure and high temperature X-ray diffraction and absorption studies [J]. Review of Scientific Instruments, 2008, 79(8): 085103. doi: 10.1063/1.2968199 [101] DU Z X, MIYAGI L, AMULELE G, et al. Efficient graphite ring heater suitable for diamond-anvil cells to 1300 K [J]. Review of Scientific Instruments, 2013, 84(2): 024502. doi: 10.1063/1.4792395 [102] DUBROVINSKY L S, SAXENA S K, LAZOR P. High-pressure and high-temperature in situ X-ray diffraction study of iron and corundum to 68 GPa using an internally heated diamond anvil cell [J]. Physics and Chemistry of Minerals, 1998, 25(6): 434–441. doi: 10.1007/s002690050133 [103] BALZARETTI N M, GONZALEZ E J, PIERMARINI G J, et al. Resistance heating of the gasket in a gem-anvil high pressure cell [J]. Review of Scientific Instruments, 1999, 70(11): 4316–4323. doi: 10.1063/1.1150095 [104] LIERMANN H P, MERKEL S, MIYAGI L, et al. Experimental method for in situ determination of material textures at simultaneous high pressure and high temperature by means of radial diffraction in the diamond anvil cell [J]. Review of Scientific Instruments, 2009, 80(10): 104501. doi: 10.1063/1.3236365 [105] BASSETT W A, SHEN A H, BUCKNUM M, et al. A new diamond anvil cell for hydrothermal studies to 2.5 GPa and from −190 to 1200 ℃ [J]. Review of Scientific Instruments, 1993, 64(8): 2340–2345. doi: 10.1063/1.1143931 [106] ZHA C S, BASSETT W A. Internal resistive heating in diamond anvil cell for in situ X-ray diffraction and Raman scattering [J]. Review of Scientific Instruments, 2003, 74(3): 1255–1262. doi: 10.1063/1.1539895 [107] WEIR S T, LIPP M J, FALABELLA S, et al. High pressure melting curve of tin measured using an internal resistive heating technique to 45 GPa [J]. Journal of Applied Physics, 2012, 111(12): 123529. doi: 10.1063/1.4730968 [108] KOMABAYASHI T, FEI Y W, MENG Y, et al. In-situ X-ray diffraction measurements of the γ-ε transition boundary of iron in an internally-heated diamond anvil cell [J]. Earth and Planetary Science Letters, 2009, 282(1/2/3/4): 252–257. doi: 10.1016/J.EPSL.2009.03.025 [109] KOMABAYASHI T, PESCE G, SINMYO R, et al. Phase relations in the system Fe-Ni-Si to 200 GPa and 3900 K and implications for Earth’s core [J]. Earth and Planetary Science Letters, 2019, 512: 83–88. doi: 10.1016/j.jpgl.2019.01.056 [110] BOEHLER R, ROSS M, SÖDERLIND P, et al. High-pressure melting curves of argon, krypton, and xenon: deviation from corresponding states theory [J]. Physical Review Letters, 2001, 86(25): 5731–5734. doi: 10.1103/PhysRevLett.86.5731 [111] BOEHLER R, ROSS M, BOERCKER D B. High-pressure melting curves of alkali halides [J]. Physical Review B, 1996, 53(2): 556–563. doi: 10.1103/PhysRevB.53.556 [112] BOEHLER R, ROSS M, BOERCKER D B. Melting of LiF and NaCl to 1 Mbar: systematics of ionic solids at extreme conditions [J]. Physical Review Letters, 1997, 78(24): 4589–4592. doi: 10.1103/PhysRevLett.78.4589 [113] SHEN G Y, LAZOR P. Measurement of melting temperatures of some minerals under lower mantle pressures [J]. Journal of Geophysical Research: Solid Earth, 1995, 100(B9): 17699–17713. doi: 10.1029/95JB01864 [114] SANTAMARÍA-PÉREZ D, MUKHERJEE G D, SCHWAGER B, et al. High-pressure melting curve of helium and neon: deviations from corresponding states theory [J]. Physical Review B, 2010, 81(21): 214101. doi: 10.1103/PhysRevB.81.214101 [115] KIMURA T, OHFUJI H, NISHI M, et al. Melting temperatures of MgO under high pressure by micro-texture analysis [J]. Nature Communications, 2017, 8(1): 15735. doi: 10.1038/ncomms15735 [116] ANDRAULT D, MORARD G, GARBARINO G, et al. Melting behavior of SiO2 up to 120 GPa [J]. Physics and Chemistry of Minerals, 2020, 47(2): 10. doi: 10.1007/s00269-019-01077-3 [117] SEILER F, IGRA O. Hypervelocity launchers [M]. Cham: Springer, 2016. [118] CHEN G Q, AHRENS T J. High-pressure melting of iron: new experiments and calculations [J]. Philosophical Transactions of the Royal Society of London Series A: Mathematical, Physical and Engineering Sciences, 1996, 354(1711): 1251–1263. doi: 10.1098/rsta.1996.0047 [119] YOO C S, HOLMES N C, ROSS M, et al. Shock temperatures and melting of iron at Earth core conditions [J]. Physical Review Letters, 1993, 70(25): 3931–3934. doi: 10.1103/PhysRevLett.70.3931 [120] TURNEAURE S J, SHARMA S M, GUPTA Y M. Crystal structure and melting of Fe shock compressed to 273 GPa: in situ X-ray diffraction [J]. Physical Review Letters, 2020, 125(21): 215702. doi: 10.1103/PhysRevLett.125.215702 [121] HERTZBERG A, BRUCKNER A P, BOGDANOFF D W. Ram accelerator: a new chemical method for accelerating projectiles to ultrahigh velocities [J]. AIAA Journal, 1988, 26(2): 195–203. doi: 10.2514/3.9872 [122] CROZIER W D, HUME W. High-velocity, light-gas gun [J]. Journal of Applied Physics, 1957, 28(8): 892–894. doi: 10.1063/1.1722882 [123] BOSLOUGH M B, AHRENS T J. A sensitive time-resolved radiation pyrometer for shock-temperature measurements above 1500 K [J]. Review of Scientific Instruments, 1989, 60(12): 3711–3716. doi: 10.1063/1.1140480 [124] RAV A S, SAXENA A K, JOSHI K D, et al. Time resolved radiation pyrometer for transient temperature measurement [J]. AIP Conference Proceedings, 2011, 1349(1): 451–452. doi: 10.1063/1.3605928 [125] BOSLOUGH M B. Shock-induced chemical reactions in nickel-aluminum powder mixtures: radiation pyrometer measurements [J]. Chemical Physics Letters, 1989, 160(5/6): 618–622. doi: 10.1016/0009-2614(89)80074-0 [126] TAN H, DAI C D, ZHANG L Y, et al. Method to determine the melting temperatures of metals under megabar shock pressures [J]. Applied Physics Letters, 2005, 87(22): 221905. doi: 10.1063/1.2043248 [127] TAN H, AHRENS T J. Shock temperature measurements for metals [J]. High Pressure Research, 1990, 2(3): 159–182. doi: 10.1080/08957959008201036 [128] NELLIS W J, YOO C S. Issues concerning shock temperature measurements of iron and other metals [J]. Journal of Geophysical Research:Solid Earth, 1990, 95(B13): 21749–21752. doi: 10.1029/JB095iB13p21749 [129] ALFÈ D, GILLAN M J, PRICE G D. The melting curve of iron at the pressures of the Earth’s core from ab initio calculations [J]. Nature, 1999, 401(6752): 462–464. doi: 10.1038/46758 [130] ANDERSON O L, ISAAK D G. Calculated melting curves for phases of iron [J]. American Mineralogist, 2000, 85(2): 376–385. doi: 10.2138/am-2000-2-317 [131] BELONOSHKO A B, AHUJA R, JOHANSSON B. Quasi-ab initio molecular dynamic study of Fe melting [J]. Physical Review Letters, 2000, 84(16): 3638–3641. doi: 10.1103/PhysRevLett.84.3638 [132] LAIO A, BERNARD S, CHIAROTTI G L, et al. Physics of iron at Earth’s core conditions [J]. Science, 2000, 287(5455): 1027–1030. doi: 10.1126/science.287.5455.1027 [133] ALFÈ D, PRICE G D, GILLAN M J. Iron under Earth’s core conditions: liquid-state thermodynamics and high-pressure melting curve from ab initio calculations [J]. Physical Review B, 2002, 65(16): 165118. doi: 10.1103/PhysRevB.65.165118 [134] ANDERSON O L, ISAAK D G, NELSON V E. The high-pressure melting temperature of hexagonal close-packed iron determined from thermal physics [J]. Journal of Physics and Chemistry of Solids, 2003, 64(11): 2125–2131. doi: 10.1016/S0022-3697(03)00112-4 [135] ALFÈ D. Temperature of the inner-core boundary of the Earth: melting of iron at high pressure from first-principles coexistence simulations [J]. Physical Review B, 2009, 79(6): 060101(R). doi: 10.1103/PhysRevB.79.060101 [136] SOLA E, ALFÈ D. Melting of iron under Earth’s core conditions from diffusion Monte Carlo free energy calculations [J]. Physical Review Letters, 2009, 103(7): 078501. doi: 10.1103/PhysRevLett.103.078501 [137] KOMABAYASHI T, FEI Y W. Internally consistent thermodynamic database for iron to the Earth’s core conditions [J]. Journal of Geophysical Research: Solid Earth, 2010, 115(B3): B03202. doi: 10.1029/2009JB006442 [138] BOUCHET J, MAZEVET S, MORARD G, et al. Ab initio equation of state of iron up to 1500 GPa [J]. Physical Review B, 2013, 87(9): 094102. doi: 10.1103/PhysRevB.87.094102 [139] ZHANG W J, LIU Z Y, LIU Z L, et al. Melting curves and entropy of melting of iron under Earth’s core conditions [J]. Physics of the Earth and Planetary Interiors, 2015, 244: 69–77. doi: 10.1016/j.pepi.2014.10.011 [140] DOROGOKUPETS P I, DYMSHITS A M, LITASOV K D, et al. Thermodynamics and equations of state of iron to 350 GPa and 6000 K [J]. Scientific Reports, 2017, 7: 41863. doi: 10.1038/srep41863 [141] SUN T, BRODHOLT J P, LI Y G, et al. Melting properties from ab initio free energy calculations: iron at the Earth’s inner-core boundary [J]. Physical Review B, 2018, 98(22): 224301. doi: 10.1103/PhysRevB.98.224301 [142] CUONG T D, PHAN A D. Efficient analytical approach for high-pressure melting properties of iron [J]. Vacuum, 2021, 185: 110001. doi: 10.1016/j.vacuum.2020.110001 [143] WILLIAMS Q, JEANLOZ R, BASS J, et al. The melting curve of iron to 250 gigapascals: a constraint on the temperature at Earth’s center [J]. Science, 1987, 236(4798): 181–182. doi: 10.1126/science.236.4798.181 [144] BOEHLER R, VON BARGEN N, CHOPELAS A. Melting, thermal expansion, and phase transitions of iron at high pressures [J]. Journal of Geophysical Research: Solid Earth, 1990, 95(B13): 21731–21736. doi: 10.1029/JB095iB13p21731 [145] BOEHLER R. Temperatures in the Earth’s core from melting-point measurements of iron at high static pressures [J]. Nature, 1993, 363(6429): 534–536. doi: 10.1038/363534a0 [146] SAXENA S K, SHEN G, LAZOR P. Temperatures in Earth’s core based on melting and phase transformation experiments on iron [J]. Science, 1994, 264(5157): 405–407. doi: 10.1126/science.264.5157.405 [147] SHEN G Y, MAO H K, HEMLEY R J, et al. Melting and crystal structure of iron at high pressures and temperatures [J]. Geophysical Research Letters, 1998, 25(3): 373–376. doi: 10.1029/97GL03776 [148] SHEN G Y, PRAKAPENKA V B, RIVERS M L, et al. Structure of liquid iron at pressures up to 58 GPa [J]. Physical Review Letters, 2004, 92(18): 185701. doi: 10.1103/PhysRevLett.92.185701 [149] MA Y Z, SOMAYAZULU M, SHEN G Y, et al. In situ X-ray diffraction studies of iron to Earth-core conditions [J]. Physics of the Earth and Planetary Interiors, 2004, 143/144: 455–467. doi: 10.1016/j.pepi.2003.06.005 [150] BOEHLER R, SANTAMARÍA-PÉREZ D, ERRANDONEA D, et al. Melting, density, and anisotropy of iron at core conditions: new X-ray measurements to 150 GPa [J]. Journal of Physics: Conference Series, 2008, 121: 022018. doi: 10.1088/1742-6596/121/2/022018 [151] JACKSON J M, STURHAHN W, LERCHE M, et al. Melting of compressed iron by monitoring atomic dynamics [J]. Earth and Planetary Science Letters, 2013, 362: 143–150. doi: 10.1016/j.jpgl.2012.11.048 [152] AQUILANTI G, TRAPANANTI A, KARANDIKAR A, et al. Melting of iron determined by X-ray absorption spectroscopy to 100 GPa [J]. Proceedings of the National Academy of Sciences of the United States of America, 2015, 112(39): 12042–12045. doi: 10.1073/pnas.1502363112 [153] ZHANG D Z, JACKSON J M, ZHAO J Y, et al. Temperature of Earth’s core constrained from melting of Fe and Fe0.9Ni0.1 at high pressures [J]. Earth and Planetary Science Letters, 2016, 447: 72–83. doi: 10.1016/j.jpgl.2016.04.026 [154] MORARD G, BOCCATO S, ROSA A D, et al. Solving controversies on the iron phase diagram under high pressure [J]. Geophysical Research Letters, 2018, 45(20): 11074–11082. doi: 10.1029/2018GL079950 [155] SINMYO R, HIROSE K, OHISHI Y. Melting curve of iron to 290 GPa determined in a resistance-heated diamond-anvil cell [J]. Earth and Planetary Science Letters, 2019, 510: 45–52. doi: 10.1016/j.jpgl.2019.01.006 [156] BROWN J M, MCQUEEN R G. Phase transitions, Grüneisen parameter, and elasticity for shocked iron between 77 GPa and 400 GPa [J]. Journal of Geophysical Research: Solid Earth, 1986, 91(B7): 7485–7494. doi: 10.1029/JB091iB07p07485 [157] BASS J D, SVENDSEN B, AHRENS T J. The temperature of shock compressed iron [M]//MANGHNANI M H, SYONO Y. High-Pressure Research in Mineral Physics: A Volume in Honor of Syuniti Akimoto. Terra Scientific Publishing Company, 1987: 393–402. [158] 李西军. 铁高压熔化线研究 [D]. 北京: 中国工程物理研究院, 2000.LI X J. Study on high pressure iron melting curve [D]. Beijing: China Academy of Engineering Physics, 2000. [159] 李西军, 龚自正, 刘福生, 等. 铁高压熔化线的测量——熔化机理的影响 [J]. 高压物理学报, 2001, 15(3): 221–225. doi: 10.11858/gywlxb.2001.03.009LI X J, GONG Z Z, LIU F S, et al. A problem in measurements of high pressure melting curve of iron: influence of melting mechanism on the melting temperature [J]. Chinese Journal of High Pressure Physics, 2001, 15(3): 221–225. doi: 10.11858/gywlxb.2001.03.009 [160] AHRENS T J, HOLLAND K G, CHEN G Q. Phase diagram of iron, revised-core temperatures [J]. Geophysical Research Letters, 2002, 29(7): 1150. doi: 10.1029/2001GL014350 [161] PING Y, COPPARI F, HICKS D G, et al. Solid iron compressed up to 560 GPa [J]. Physical Review Letters, 2013, 111(6): 065501. doi: 10.1103/PhysRevLett.111.065501 [162] 孙峪怀. 铁的高压物态方程与熔化线研究 [D]. 成都: 西南交通大学, 2009.SUN Y H. Study on the equation of state and melting curve of iron [D]. Chengdu: Southwest Jiaotong University, 2009. [163] HARMAND M, RAVASIO A, MAZEVET S, et al. X-ray absorption spectroscopy of iron at multimegabar pressures in laser shock experiments [J]. Physical Review B, 2015, 92(2): 024108. doi: 10.1103/PhysRevB.92.024108 [164] TORCHIO R, OCCELLI F, MATHON O, et al. Probing local and electronic structure in warm dense matter: single pulse synchrotron X-ray absorption spectroscopy on shocked Fe [J]. Scientific Reports, 2016, 6(1): 26402. doi: 10.1038/srep26402 [165] ERRANDONEA D, SCHWAGER B, DITZ R, et al. Systematics of transition-metal melting [J]. Physical Review B, 2001, 63(13): 132104. doi: 10.1103/PhysRevB.63.132104 [166] JEANLOZ R, KAVNER A. Melting criteria and imaging spectroradiometry in laser-heated diamond-cell experiments [J]. Philosophical Transactions of the Royal Society of London Series A: Mathematical, Physical and Engineering Sciences, 1996, 354(1711): 1279–1305. doi: 10.1098/rsta.1996.0049 [167] DEWAELE A, MEZOUAR M, GUIGNOT N, et al. High melting points of tantalum in a laser-heated diamond anvil cell [J]. Physical Review Letters, 2010, 104(25): 255701. doi: 10.1103/PhysRevLett.104.255701 [168] ERRANDONEA D, MACLEOD S G, BURAKOVSKY L, et al. Melting curve and phase diagram of vanadium under high-pressure and high-temperature conditions [J]. Physical Review B, 2019, 100(9): 094111. doi: 10.1103/PhysRevB.100.094111 [169] STUTZMANN V, DEWAELE A, BOUCHET J, et al. High-pressure melting curve of titanium [J]. Physical Review B, 2015, 92(22): 224110. doi: 10.1103/PhysRevB.92.224110 [170] ANZELLINI S, MONTESEGURO V, BANDIELLO E, et al. In situ characterization of the high pressure-high temperature melting curve of platinum [J]. Scientific Reports, 2019, 9(1): 13034. doi: 10.1038/s41598-019-49676-y [171] WECK G, RECOULES V, QUEYROUX J A, et al. Determination of the melting curve of gold up to 110 GPa [J]. Physical Review B, 2020, 101(1): 014106. doi: 10.1103/PhysRevB.101.014106 [172] LORD O T, WOOD I G, DOBSON D P, et al. The melting curve of Ni to 1 Mbar [J]. Earth and Planetary Science Letters, 2014, 408: 226–236. doi: 10.1016/j.jpgl.2014.09.046 [173] ERRANDONEA D, BURAKOVSKY L, PRESTON D L, et al. Experimental and theoretical confirmation of an orthorhombic phase transition in niobium at high pressure and temperature [J]. Communications Materials, 2020, 1(1): 60. doi: 10.1038/s43246-020-00058-2 [174] DEWAELE A, MEZOUAR M, GUIGNOT N, et al. Melting of lead under high pressure studied using second-scale time-resolved X-ray diffraction [J]. Physical Review B, 2007, 76(14): 144106. doi: 10.1103/PhysRevB.76.144106 [175] ANDRAULT D, MORARD G, BOLFAN-CASANOVA N, et al. Study of partial melting at high-pressure using in situ X-ray diffraction [J]. High Pressure Research, 2006, 26(3): 267–276. doi: 10.1080/08957950600897013 [176] BOCCATO S, TORCHIO R, KANTOR I, et al. The melting curve of nickel up to 100 GPa explored by XAS [J]. Journal of Geophysical Research: Solid Earth, 2017, 122(12): 9921–9930. doi: 10.1002/2017JB014807 [177] LI F F, CUI Q L, HE Z, et al. Brillouin scattering spectroscopy for a laser heated diamond anvil cell [J]. Applied Physics Letters, 2006, 88(20): 203507. doi: 10.1063/1.2205164 [178] ZHANG J S, BASS J D, ZHU G H. Single-crystal Brillouin spectroscopy with CO2 laser heating and variable q [J]. Review of Scientific Instruments, 2015, 86(6): 063905. doi: 10.1063/1.4922634 [179] DI CICCO A, TRAPANANTI A. Study of local icosahedral ordering in liquid and undercooled liquid copper [J]. Journal of Non-Crystalline Solids, 2007, 353(32): 3671–3678. doi: 10.1016/j.jnoncrysol.2007.05.150 [180] PASCARELLI S, MATHON O, MAIRS T, et al. The time-resolved and extreme-conditions XAS (TEXAS) facility at the European Synchrotron Radiation Facility: the energy-dispersive X-ray absorption spectroscopy beamline ID24 [J]. Journal of Synchrotron Radiation, 2016, 23(1): 353–368. doi: 10.1107/S160057751501783X [181] LORD O T, WALTER M J, DASGUPTA R, et al. Melting in the Fe-C system to 70 GPa [J]. Earth and Planetary Science Letters, 2009, 284(1/2): 157–167. doi: 10.1016/J.EPSL.2009.04.017 [182] HRUBIAK R, MENG Y, SHEN G Y. Microstructures define melting of molybdenum at high pressures [J]. Nature Communications, 2017, 8: 14562. doi: 10.1038/ncomms14562 [183] KARANDIKAR A, BOEHLER R. Flash melting of tantalum in a diamond cell to 85 GPa [J]. Physical Review B, 2016, 93(5): 054107. doi: 10.1103/PhysRevB.93.054107 [184] YANG L X, KARANDIKAR A, BOEHLER R. Flash heating in the diamond cell: melting curve of rhenium [J]. Review of Scientific Instruments, 2012, 83(6): 063905. doi: 10.1063/1.4730595 [185] ERRANDONEA D. The melting curve of ten metals up to 12 GPa and 1600 K [J]. Journal of Applied Physics, 2010, 108(3): 033517. doi: 10.1063/1.3468149 [186] BASU A, FIELD M R, MCCULLOCH D G, et al. New measurement of melting and thermal conductivity of iron close to outer core conditions [J]. Geoscience Frontiers, 2020, 11(2): 565–568. doi: 10.1016/j.gsf.2019.06.007 [187] BOEHLER R. The phase diagram of iron to 430 kbar [J]. Geophysical Research Letters, 1986, 13(11): 1153–1156. doi: 10.1029/GL013i011p01153 [188] KUPENKO I, DUBROVINSKY L, DUBROVINSKAIA N, et al. Portable double-sided laser-heating system for Mössbauer spectroscopy and X-ray diffraction experiments at synchrotron facilities with diamond anvil cells [J]. Review of Scientific Instruments, 2012, 83(12): 124501. doi: 10.1063/1.4772458 [189] APRILIS G, STROHM C, KUPENKO I, et al. Portable double-sided pulsed laser heating system for time-resolved geoscience and materials science applications [J]. Review of Scientific Instruments, 2017, 88(8): 084501. doi: 10.1063/1.4998985 [190] ZHANG D Z, JACKSON J M, ZHAO J Y, et al. Fast temperature spectrometer for samples under extreme conditions [J]. Review of Scientific Instruments, 2015, 86(1): 013105. doi: 10.1063/1.4905431 [191] BELONOSHKO A B, AHUJA R, JOHANSSON B. Molecular dynamics study of melting and fcc-bcc transitions in Xe [J]. Physical Review Letters, 2001, 87(16): 165505. doi: 10.1103/PhysRevLett.87.165505 [192] BELONOSHKO A B, AHUJA R, JOHANSSON B. Stability of the body-centred-cubic phase of iron in the Earth’s inner core [J]. Nature, 2003, 424(6952): 1032–1034. doi: 10.1038/nature01954 [193] SALAMAT A, FISCHER R A, BRIGGS R, et al. In situ synchrotron X-ray diffraction in the laser-heated diamond anvil cell: melting phenomena and synthesis of new materials [J]. Coordination Chemistry Reviews, 2014, 277/278: 15–30. doi: 10.1016/j.ccr.2014.01.034 [194] GLAZYRIN K, POUROVSKII L V, DUBROVINSKY L, et al. Importance of correlation effects in hcp iron revealed by a pressure-induced electronic topological transition [J]. Physical Review Letters, 2013, 110(11): 117206. doi: 10.1103/PhysRevLett.110.117206 [195] WANG Y, WANG J J, HERMANN A, et al. Electronically driven 1D cooperative diffusion in a simple cubic crystal [J]. Physical Review X, 2021, 11(1): 011006. doi: 10.1103/PhysRevX.11.011006 [196] HRUBIAK R, MENG Y, SHEN G Y. Experimental evidence of a body centered cubic iron at the Earth’s core condition [J/OL]. arXiv preprint arXiv: 1804.05109. (2018-04-13)[2021-08-02]. https://arxiv.org/abs/1804.05109. [197] LORD O T, WANN E T H, HUNT S A, et al. The NiSi melting curve to 70 GPa [J]. Physics of the Earth and Planetary Interiors, 2014, 233: 13–23. doi: 10.1016/j.pepi.2014.05.005 [198] GEBALLE Z M, JEANLOZ R. Origin of temperature plateaus in laser-heated diamond anvil cell experiments [J]. Journal of Applied Physics, 2012, 111(12): 123518. doi: 10.1063/1.4729905 [199] MENG Y, SHEN G, MAO H K. Double-sided laser heating system at HPCAT for in situ X-ray diffraction at high pressures and high temperatures [J]. Journal of Physics: Condensed Matter, 2006, 18(25): S1097–S1103. doi: 10.1088/0953-8984/18/25/S17 [200] SHEN G Y, MAO H K, HEMLEY R J. Laser-heated diamond anvil cell technique: double-sided heating with multimode Nd: YAG laser [C]//Proceedings of the Advance Materials '96 New Trends in High Pressure Research, 3rd International Symposium Advanced Materials. Ibaraki: National Institute for Research in Inorganic Materials, 1996. [201] BOEHLER R. Laser heating in the diamond cell: techniques and applications [J]. Hyperfine Interactions, 2000, 128(1): 307–321. doi: 10.1023/A:1012648019016 [202] ERRANDONEA D, SOMAYAZULU M, HÄUSERMANN D, et al. Melting of tantalum at high pressure determined by angle dispersive X-ray diffraction in a double-sided laser-heated diamond-anvil cell [J]. Journal of Physics: Condensed Matter, 2003, 15(45): 7635–7649. doi: 10.1088/0953-8984/15/45/003 [203] NGUYEN J H, HOLMES N C. Iron sound velocities in shock wave experiments [J]. AIP Conference Proceedings, 2000, 505(1): 81–84. doi: 10.1063/1.1303426 [204] WASSERMAN E, STIXRUDE L, COHEN R E. Thermal properties of iron at high pressures and temperatures [J]. Physical Review B, 1996, 53(13): 8296–8309. doi: 10.1103/PhysRevB.53.8296 [205] HONG N T, HIEU H K. High-pressure melting curves of and phases of iron [J]. VNU Journal of Science: Mathematics-Physics, 2019, 35(4): 99–105. doi: 10.25073/2588-1124/VNUMAP.4382 [206] BELASHCHENKO D K. Estimation of the thermodynamic characteristics of the Earth’s core using the embedded atom model [J]. Geochemistry International, 2014, 52(6): 456–466. doi: 10.1134/S0016702914060020 [207] ZHUANG J Y, WANG H J, ZHANG Q, et al. Thermodynamic properties of ε-Fe with thermal electronic excitation effects on vibrational spectra [J]. Physical Review B, 2021, 103(14): 144102. doi: 10.1103/PhysRevB.103.144102 [208] HIEU H K, HAI T T, HONG N T, et al. Pressure dependence of melting temperature and shear modulus of hcp-iron [J]. High Pressure Research, 2017, 37(3): 267–277. doi: 10.1080/08957959.2017.1318131 [209] VAN HUNG V, HAI D T, BINH L T T. Melting curve of metals with defect: pressure dependence [J]. Computational Materials Science, 2013, 79: 789–794. doi: 10.1016/j.commatsci.2013.07.042 [210] KRESSE G, JOUBERT D. From ultrasoft pseudopotentials to the projector augmented-wave method [J]. Physical Review B, 1999, 59(3): 1758–1775. doi: 10.1103/PhysRevB.59.1758 [211] BLÖCHL P E. Projector augmented-wave method [J]. Physical Review B, 1994, 50(24): 17953–17979. doi: 10.1103/PhysRevB.50.17953 [212] DAW M S, FOILES S M, BASKES M I. The embedded-atom method: a review of theory and applications [J]. Materials Science Reports, 1993, 9(7/8): 251–310. doi: 10.1016/0920-2307(93)90001-U [213] LINDEMANN F A. The calculation of molecular vibration frequencies [J]. Zeitschrift fur Physik, 1910, 11: 609–612. [214] WOLF G H, JEANLOZ R. Lindemann melting law: anharmonic correction and test of its validity for minerals [J]. Journal of Geophysical Research: Solid Earth, 1984, 89(B9): 7821–7835. doi: 10.1029/JB089iB09p07821 [215] KUSHWAH S S, TOMAR Y S, UPADHYAY A K. On the volume-dependence of the Grüneisen parameter and the Lindemann law of melting [J]. Journal of Physics and Chemistry of Solids, 2013, 74(8): 1143–1145. doi: 10.1016/j.jpcs.2013.03.014 [216] SIMON F, GLATZEL G. Bemerkungen zur Schmelzdruckkurve [J]. Zeitschrift für Anorganische und Allgemeine Chemie, 1929, 178(1): 309–316. doi: 10.1002/zaac.19291780123 [217] KRAUT E A, KENNEDY G C. New melting law at high pressures [J]. Physical Review, 1966, 151(2): 668–675. doi: 10.1103/PhysRev.151.668 [218] 杜宜瑾. 凝聚态物理研究 [M]. 合肥: 安徽大学出版社, 1998.DU Y J. Research on condensed matter physics [M]. Hefei: Anhui University Press, 1998. [219] WANG Z W, LAZOR P, SAXENA S K. A simple model for assessing the high pressure melting of metals: nickel, aluminum and platinum [J]. Physica B: Condensed Matter, 2001, 293(3/4): 408–416. doi: 10.1016/S0921-4526(00)00542-1 [220] LI J, FEI Y. Experimental constraints on core composition [J]. Treatise on Geochemistry, 2014, 3: 527–557. doi: 10.1016/B978-0-08-095975-7.00214-X [221] MCDONOUGH W F. Compositional model for the Earth’s core [J]. Treatise on Geochemistry, 2014, 3: 559–577. doi: 10.1016/B978-0-08-095975-7.00215-1 [222] SAKAMAKI K, TAKAHASHI E, NAKAJIMA Y, et al. Melting phase relation of FeH x up to 20 GPa: implication for the temperature of the Earth’s core [J]. Physics of the Earth and Planetary Interiors, 2009, 174(1/2/3/4): 192–201. doi: 10.1016/j.pepi.2008.05.017 [223] ASANUMA H, OHTANI E, SAKAI T, et al. Static compression of Fe0.83Ni0.09Si0.08 alloy to 374 GPa and Fe0.93Si0.07 alloy to 252 GPa: implications for the Earth’s inner core [J]. Earth and Planetary Science Letters, 2011, 310(1/2): 113–118. doi: 10.1016/j.jpgl.2011.06.034 [224] ASANUMA H, OHTANI E, SAKAI T, et al. Melting of iron-silicon alloy up to the core-mantle boundary pressure: implications to the thermal structure of the Earth’s core [J]. Physics and Chemistry of Minerals, 2010, 37(6): 353–359. doi: 10.1007/s00269-009-0338-7 [225] FISCHER R A, CAMPBELL A J, CARACAS R, et al. Equation of state and phase diagram of Fe-16Si alloy as a candidate component of Earth’s core [J]. Earth and Planetary Science Letters, 2012, 357/358: 268–276. doi: 10.1016/j.jpgl.2012.09.022 [226] FISCHER R A, CAMPBELL A J, REAMAN D M, et al. Phase relations in the Fe-FeSi system at high pressures and temperatures [J]. Earth and Planetary Science Letters, 2013, 373: 54–64. doi: 10.1016/j.jpgl.2013.04.035 [227] FISCHER R A, CAMPBELL A J, CARACAS R, et al. Equations of state in the Fe-FeSi system at high pressures and temperatures [J]. Journal of Geophysical Research: Solid Earth, 2014, 119(4): 2810–2827. doi: 10.1002/2013JB010898 [228] SEAGLE C T, HEINZ D L, CAMPBELL A J, et al. Melting and thermal expansion in the Fe-FeO system at high pressure [J]. Earth and Planetary Science Letters, 2008, 265(3/4): 655–665. doi: 10.1016/j.jpgl.2007.11.004 [229] KOMABAYASHI T. Thermodynamics of melting relations in the system Fe-FeO at high pressure: implications for oxygen in the Earth’s core [J]. Journal of Geophysical Research: Solid Earth, 2014, 119(5): 4164–4177. doi: 10.1002/2014JB010980 [230] OZAWA H, TAKAHASHI F, HIROSE K, et al. Phase transition of FeO and stratification in Earth’s outer core [J]. Science, 2011, 334(6057): 792–794. doi: 10.1126/science.1208265 [231] FEI Y W, BROSH E. Experimental study and thermodynamic calculations of phase relations in the Fe-C system at high pressure [J]. Earth and Planetary Science Letters, 2014, 408: 155–162. doi: 10.1016/j.jpgl.2014.09.044 [232] CAMPBELL A J, SEAGLE C T, HEINZ D L, et al. Partial melting in the iron-sulfur system at high pressure: a synchrotron X-ray diffraction study [J]. Physics of the Earth and Planetary Interiors, 2007, 162(1/2): 119–128. doi: 10.1016/j.pepi.2007.04.001 [233] KAMADA S, OHTANI E, TERASAKI H, et al. Melting relationships in the Fe-Fe3S system up to the outer core conditions [J]. Earth and Planetary Science Letters, 2012, 359/360: 26–33. doi: 10.1016/j.jpgl.2012.09.038 [234] KAMADA S, TERASAKI H, OHTANI E, et al. Phase relationships of the Fe-FeS system in conditions up to the Earth’s outer core [J]. Earth and Planetary Science Letters, 2010, 294(1/2): 94–100. doi: 10.1016/j.jpgl.2010.03.011 [235] MORARD G, ANDRAULT D, GUIGNOT N, et al. In situ determination of Fe-Fe3S phase diagram and liquid structural properties up to 65 GPa [J]. Earth and Planetary Science Letters, 2008, 272(3/4): 620–626. doi: 10.1016/j.jpgl.2008.05.028 [236] CHUDINOVSKIKH L, BOEHLER R. Eutectic melting in the system Fe-S to 44 GPa [J]. Earth and Planetary Science Letters, 2007, 257(1/2): 97–103. doi: 10.1016/j.jpgl.2007.02.024 [237] MORI Y, OZAWA H, HIROSE K, et al. Melting experiments on Fe-Fe3S system to 254 GPa [J]. Earth and Planetary Science Letters, 2017, 464: 135–141. doi: 10.1016/j.jpgl.2017.02.021 [238] TERASAKI H, KAMADA S, SAKAI T, et al. Liquidus and solidus temperatures of a Fe-O-S alloy up to the pressures of the outer core: implication for the thermal structure of the Earth’s core [J]. Earth and Planetary Science Letters, 2011, 304(3/4): 559–564. doi: 10.1016/j.jpgl.2011.02.041 [239] HUANG H J, HU X J, JING F Q, et al. Melting behavior of Fe-O-S at high pressure: a discussion on the melting depression induced by O and S [J]. Journal of Geophysical Research: Solid Earth, 2010, 115(B5): B05207. doi: 10.1029/2009JB006514 [240] ZHANG Y J, SEKINE T, HE H L, et al. Shock compression of Fe-Ni-Si system to 280 GPa: implications for the composition of the Earth’s outer core [J]. Geophysical Research Letters, 2014, 41(13): 4554–4559. doi: 10.1002/2014GL060670 [241] ZHANG L, FEI Y W. Effect of Ni on Fe-FeS phase relations at high pressure and high temperature [J]. Earth and Planetary Science Letters, 2008, 268(1/2): 212–218. doi: 10.1016/j.jpgl.2008.01.028 [242] HIROSE K, LABROSSE S, HERNLUND J. Composition and state of the core [J]. Annual Review of Earth and Planetary Sciences, 2013, 41(1): 657–691. doi: 10.1146/annurev-earth-050212-124007 [243] ZHANG Y J, SEKINE T, HE H L, et al. Experimental constraints on light elements in the Earth’s outer core [J]. Scientific Reports, 2016, 6: 22473. doi: 10.1038/srep22473 [244] DZIEWONSKI A M, ANDERSON D L. Preliminary reference Earth model [J]. Physics of the Earth and Planetary Interiors, 1981, 25(4): 297–356. doi: 10.1016/0031-9201(81)90046-7 [245] ALFÈ D, GILLAN M J, PRICE G D. Composition and temperature of the Earth’s core constrained by combining ab initio calculations and seismic data [J]. Earth and Planetary Science Letters, 2002, 195(1/2): 91–98. doi: 10.1016/S0012-821X(01)00568-4 [246] GUBBINS D, ALFÈ D, MASTERS G, et al. Can the Earth’s dynamo run on heat alone? [J]. Geophysical Journal International, 2003, 155(2): 609–622. doi: 10.1046/j.1365-246X.2003.02064.x [247] VOČADLO L, ALFÈ D, GILLAN M J, et al. The properties of iron under core conditions from first principles calculations [J]. Physics of the Earth and Planetary Interiors, 2003, 140(1/2/3): 101–125. doi: 10.1016/j.pepi.2003.08.001 [248] NGUYEN J H, AKIN M C, CHAU R, et al. Molybdenum sound velocity and shear modulus softening under shock compression [J]. Physical Review B, 2014, 89(17): 174109. doi: 10.1103/PhysRevB.89.174109 [249] WANG J, COPPARI F, SMITH R F, et al. X-ray diffraction of molybdenum under shock compression to 450 GPa [J]. Physical Review B, 2015, 92(17): 174114. doi: 10.1103/PhysRevB.92.174114 [250] LUO S N, AHRENS T J. Shock-induced superheating and melting curves of geophysically important minerals [J]. Physics of the Earth and Planetary Interiors, 2004, 143/144: 369–386. doi: 10.1016/j.pepi.2003.04.001 [251] DAI C D, JIN X G, ZHOU X M, et al. Sound velocity variations and melting of vanadium under shock compression [J]. Journal of Physics D: Applied Physics, 2001, 34(20): 3064–3070. doi: 10.1088/0022-3727/34/20/310 [252] LANDA A, SÖDERLIND P, YANG L H. Ab initio phase stability at high temperatures and pressures in the V-Cr system [J]. Physical Review B, 2014, 89(2): 020101(R). doi: 10.1103/PhysRevB.89.020101 [253] ZHANG T T, WANG S C, SONG H F, et al. Melting curve of vanadium up to 470 GPa simulated by ab initio molecular dynamics [J]. Journal of Applied Physics, 2019, 126(20): 205901. doi: 10.1063/1.5124520 [254] KLUG D D. Melting points agree under pressure [J]. Physics, 2010, 3: 52. doi: 10.1103/Physics.3.52 [255] SAMANTA A, TUCKERMAN M E, YU T Q, et al. Microscopic mechanisms of equilibrium melting of a solid [J]. Science, 2014, 346(6210): 729–732. doi: 10.1126/science.1253810 -







 下载:
下载:

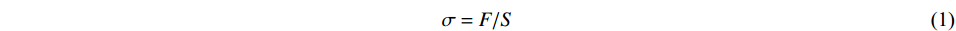
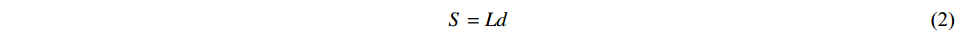
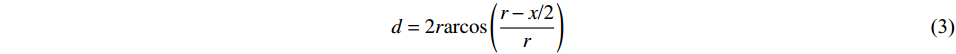
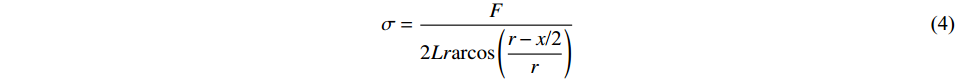
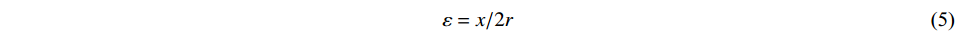


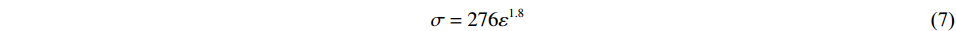









 下载:
下载: