Discrete Element Simulation of Blasting Damage Characteristics of Granite under Different Decoupling Coefficients
-
摘要: 为研究径向装药不耦合系数对花岗岩爆破损伤程度的影响,在考虑爆炸冲击波和爆生气体共同作用的基础上,提出了一种“动”、“静”荷载混合施加的PFC爆破模拟方法,采用该方法分别进行了6种不耦合系数下花岗岩爆破过程的数值模拟。模拟结果显示,随着装药不耦合系数的增大,花岗岩爆破损伤程度先增强后减弱。耦合装药下,爆生裂纹数量为9367;不耦合系数为1.2时,裂纹数量增加至最多,为24975;不耦合系数为2.0时,裂纹数量减少为292。对比耦合装药和不耦合系数为1.4时的花岗岩损伤模式发现,爆生气体的准静态压力对爆生裂纹的扩展具有重要作用。根据不同不耦合系数下的爆生裂纹数量,建立了不耦合系数大于或等于1.2时的岩石爆破损伤程度预测模型,拟合度达0.9808。该预测模型对爆破施工设计等工作具有一定的参考意义。Abstract: In order to study the effect of charge decoupling coefficients on the extent of granite blasting damage, a particle flow code (PFC) blasting simulation method with mixed “dynamic” and “quasi-static” loads is proposed based on the joint action of blast shock wave and detonation gas, and then the numerical simulation of granite blasting process under six decoupling coefficients was carried out. The results show that the extent of granite blasting damage increases and then decreases as the decoupling coefficients increases; the number of blasting induced cracks under coupled charge is 9367, which increases to 24975 when the decoupling coefficient is 1.2, and then decreases to 292 when the decoupling coefficient is 2.0. Comparing to the damage pattern under coupled charge, the extension distance of blasting induced crack is obviously shorter when the decoupling coefficient is 1.4, which indicates that the quasi-static pressure of blast gas plays an important role in crack extension. According to the number of blasting cracks, the prediction model of rock blasting damage under different decoupling coefficients greater than or equal to 1.2 was established, and the fitting degree reaches 0.9808. The prediction model presented in this paper is of certain reference significance for practical blasting design.
-
Key words:
- granite /
- decoupling coefficient /
- explosion damage /
- crack /
- particle flow code
-
不耦合装药结构是爆破开采过程中常用的施工手段。大量实验和工程实际表明[1-3],选取合适的不耦合系数能够提升炸药能量的利用率和岩石的破碎效率。因此,研究装药不耦合系数与岩石爆破损伤特性的关系,对于指导爆破施工设计等工作具有重要的现实意义。
常见的爆破破岩理论[4]认为,炸药在岩体内部的爆炸过程可分为爆炸应力波预裂破岩的动态冲击作用和爆生气体膨胀扩腔的准静态作用。叶志伟等[5]通过轮廓爆破方法,研究了不耦合装药结构下爆破孔壁压力的变化规律,实验结果表明,薄壁钢管内爆生气体的准静态压力峰值随不耦合系数的增大近似呈线性增长。徐颖等[6]利用不耦合装药结构对爆生裂纹数量进行了有效控制,并通过实验获取了具有最佳破岩效应的不耦合系数。费鸿禄等[7]较早地论证了在不耦合装药结构的光面爆破过程中爆炸冲击波压力和爆生气体压力的计算方法。杨仁树等[8]通过实验证明了不耦合偏心装药的有机玻璃爆破损伤的分形效应,建立了损伤变量与分形维数之间的预测模型。Yuan等[9]利用颗粒流程序(particle flow code,PFC),对不同耦合系数下的岩体爆破损伤过程进行了有效模拟,论证了爆炸冲击波和爆生气体对岩体爆破损伤的破坏模式。
本研究根据已有理论,在考虑爆炸冲击波和爆生气体共同作用的基础上,提出一种“动”、“静”荷载混合施加的PFC爆破模拟方法,并以此进行花岗岩爆破数值模拟研究,建立花岗岩爆破损伤程度与装药不耦合系数之间的预测模型,以期为指导爆破施工设计提供理论支持。
1. 理论分析与计算
1.1 炮孔压力计算
耦合装药结构下,球形药包在岩体内部爆炸时,炮孔压力
pm 为[10]pm =ρcv2cκ+12ρrvrρrvr+ρcvc (1) 式中:
ρc 为炸药密度,vc 为炸药爆速,κ 为绝热指数,ρr 为岩石密度,vr 为岩石纵波波速。空气不耦合装药结构下,设炮孔半径为
rp ,装药半径为rt ,则装药不耦合系数ζ=rp/rt 。由于存在空气间隔,因此爆轰压力pt 到达孔壁时为pt=ρrvrρrvr+ρcvcρcv2cκ+1ζ−2κη (2) 式中:
η 为压力增大倍数,一般取8~11,本研究取8。考虑理想情况下岩石与炸药的阻抗匹配时,有
ρrvr/(ρrvr+ρcvc) = 1/2 ;将爆生气体视为等熵膨胀,且取κ =3。此时,耦合装药下,炮孔压力为爆轰波阵面压力;不耦合装药结构下,由于爆炸冲击波在炮孔内的多次反射,压力峰值发生变化,则爆炸冲击压力pd可表示为pd={14ρcv2cζ=118ρcv2cζ−6ηζ>1 (3) 爆生气体压力pg的计算公式[11]为
pg={ρcv2c8(1ζ)6pd⩽pκpκ(ρcv2c1.6×109)1.43(1ζ)2.8pd>pκ (4) 式中:
pκ 为临界压力,pκ = 200MPa 。由式(3)和式(4)可知,装药不耦合系数
ζ 是影响爆炸冲击压力和爆生气体压力的主要因素。1.2 爆生气体扩腔作用起始时间的确定
不耦合装药结构下,爆生气体扩腔作用的起始时间是关系到爆破参量计算结果是否符合实际的重要因素。爆炸冲击波和爆生气体对炮孔壁产生作用的时间间隔Δt可采用如下近似计算方法[9]
Δt=Dsvcp (5) 式中:
Ds 为粉碎区半径;vcp 为爆炸冲击作用下的裂纹扩展速度,可由分离式霍普金森压杆(split Hopkinson pressure bar,SHPB)实验获得。有研究认为,爆生气体对炮孔的准静态作用完全发生在爆炸冲击波的动态作用之后。然而,实际上,随着炸药的不断消耗,高温高压的爆生产物不断累积,爆生气体的扩腔作用随着炸药的爆轰同步进行。为符合实际爆破过程,本研究将爆生气体扩腔作用的起始时间设置为炸药完全消耗时刻,即爆炸冲击压力达到峰值时开始对炮孔壁施加爆生气体的准静态作用。
1.3 PFC爆破“动”、“静”荷载混合施加原理
为进一步研究不同耦合系数下岩体爆破损伤特性,利用PFC对岩体爆破过程进行模拟分析,并提出爆破“动”、“静”荷载混合施加方法来模拟爆炸冲击波的动态作用和爆生气体的准静态作用。
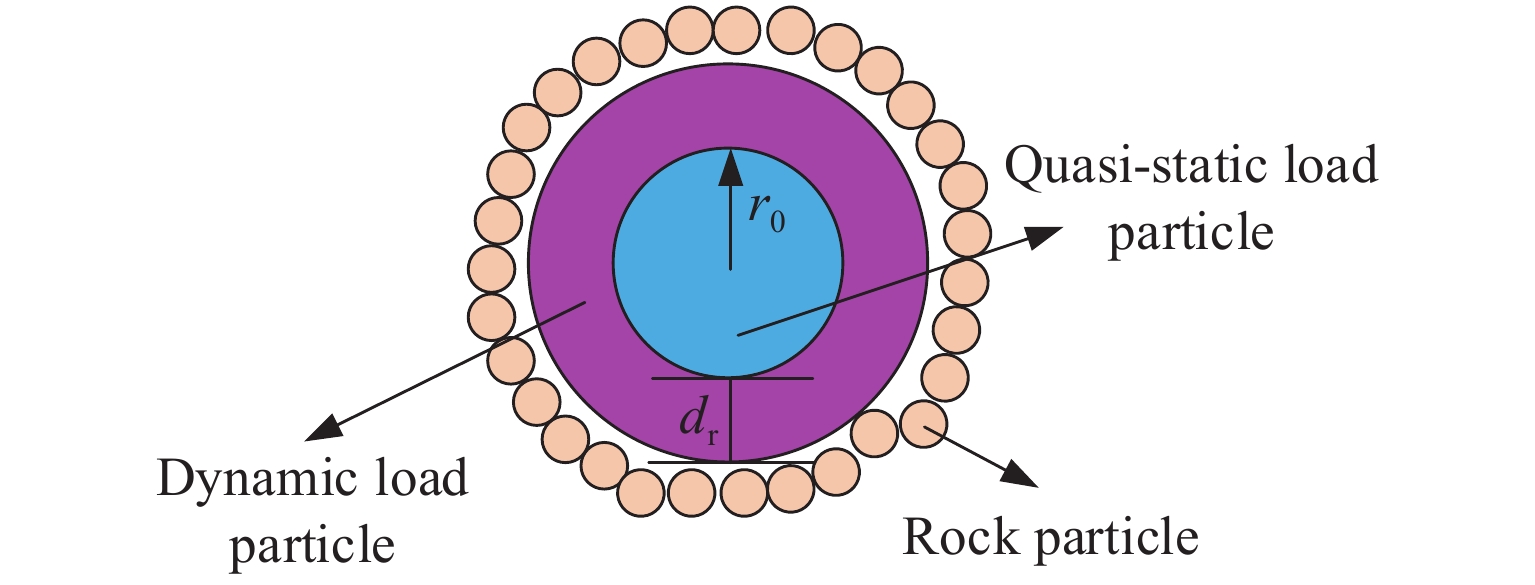
颗粒膨胀加载法[12-13]是采用PFC模拟爆破过程的主要方法之一。如图1所示,在炮孔中心位置设置动态荷载施加颗粒和准静态荷载施加颗粒。假定初始动、静荷载施加颗粒半径均为
r0 ,随着荷载施加,颗粒逐渐膨胀,当爆轰压力为p时,荷载施加颗粒半径增量为dr=2πr0pSn (6) 式中:
dr 为荷载施加颗粒半径增量,Sn 为颗粒间法向接触刚度。根据装药结构的不同,可由式(3)计算出爆炸冲击压力峰值pdmax,则动态荷载颗粒对爆破空腔壁产生的最大径向接触力之和为
Fdmax=Sdnddr=2πr0pdmaxη!r0!(η−r0)! (7) 式中:Fdmax为爆炸冲击的动荷载作用下岩石颗粒受到的最大推力,
Sdn 为动荷载颗粒刚度,ddr 为动荷载颗粒半径增量。同理,根据式(4)可计算出不同不耦合系数下的爆生气体峰值压力pgmax,则静态荷载颗粒对爆破空腔产生的最大径向接触力为
Fgmax=Sgndgr=2πr0pgmaxη!r0!(η−r0)! (8) 式中:Fgmax为爆炸冲击的静荷载作用下岩石颗粒受到的最大推力,
Sgn 为静荷载颗粒刚度,dgr 为静荷载颗粒半径增量。由式(6)可知,荷载施加颗粒的法向接触刚度
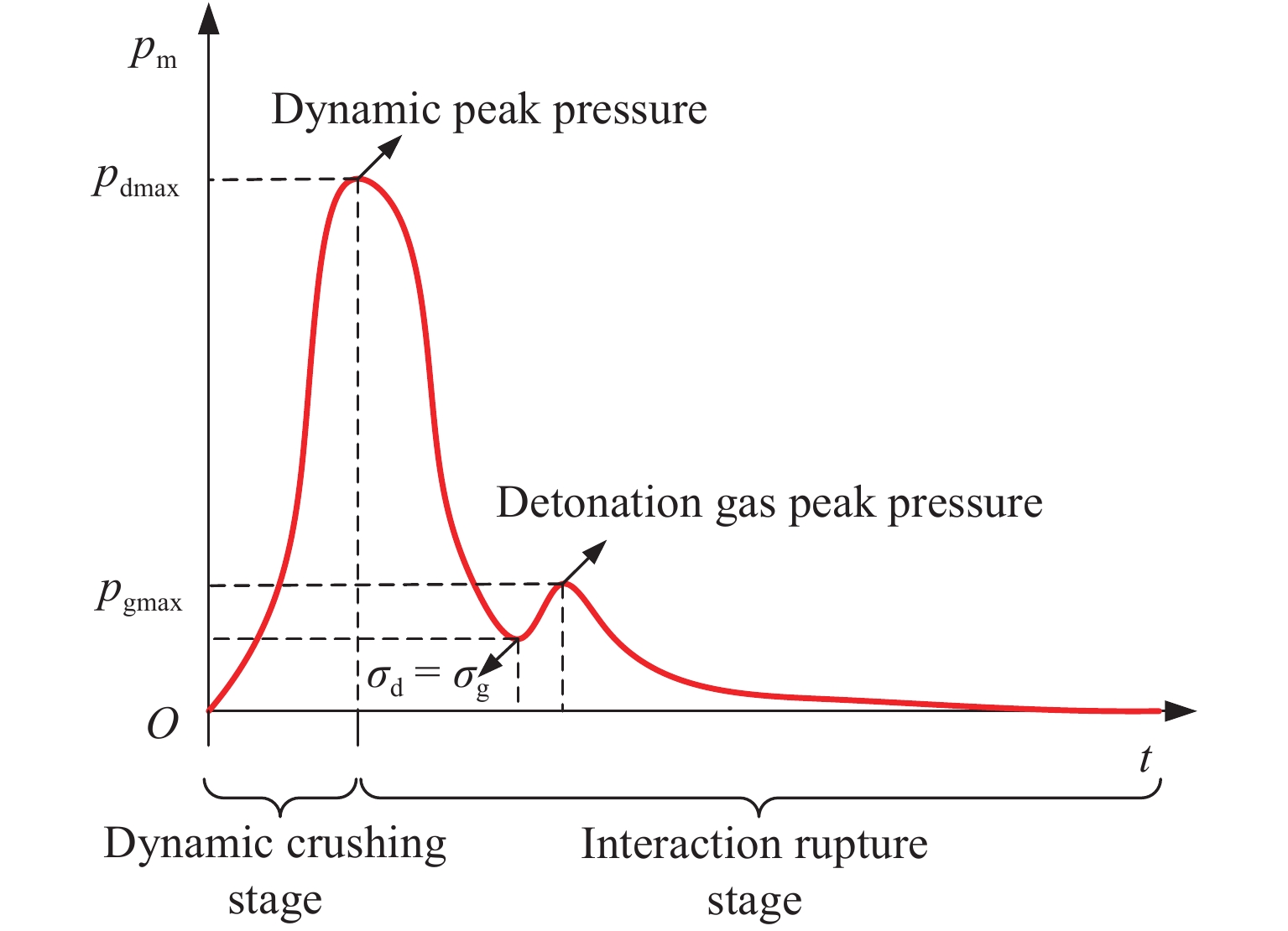
Sn 是影响动静荷载大小的关键参量。由于两种颗粒始终处于重叠状态,如果同时将它们的刚度设定为较大值,则会导致荷载施加颗粒之间的接触力过大,进而影响通过“监测圆”获取的炮孔压力大小。为了解决这一问题,在动态冲击阶段将静载施加颗粒的刚度Sgn 和半径增量dgr 设置为很低的数值(本研究取10−10),从而无法对动态冲击阶段的炮孔荷载产生影响。爆炸冲击压力达到峰值pdmax后,恢复Sgn 和dgr 至正常增量大小,此时动载压力开始降低,静载压力逐渐上升。当岩体内部动载残存应力σ d与静载压力σ g相等时,爆生气体的扩腔运动开始,经峰值pgmax后,爆生气体压力缓慢下降至零。这也与实际炸药爆炸过程中爆炸冲击压力和爆生气体对孔壁的作用过程基本相同。现有研究中多将爆炸应力波简化为三角波[14]或简谐波[15]。由于三角波荷载的变化趋势单一,难以表达爆炸冲击作用后爆生气体扩腔作用对炮孔压力的影响,因此,为了符合实际爆破过程,本研究采用半正弦波作为爆炸应力波形,实现爆破荷载的施加。爆生气体的准静态作用对孔壁荷载的变化较复杂。张玉磊等[16]指出,爆生气体的膨胀做功过程与正弦函数的半周期变化相似。为此,本研究采用半正弦波作为爆生气体作用下炮孔压力的变化曲线,然而其半正弦周期比爆炸应力波的衰减时间更长。图2为本研究采用的爆炸冲击波与爆生气体共同作用下的炮孔压力时程曲线。
2. 数值模拟
2.1 模型建立与细观参数标定
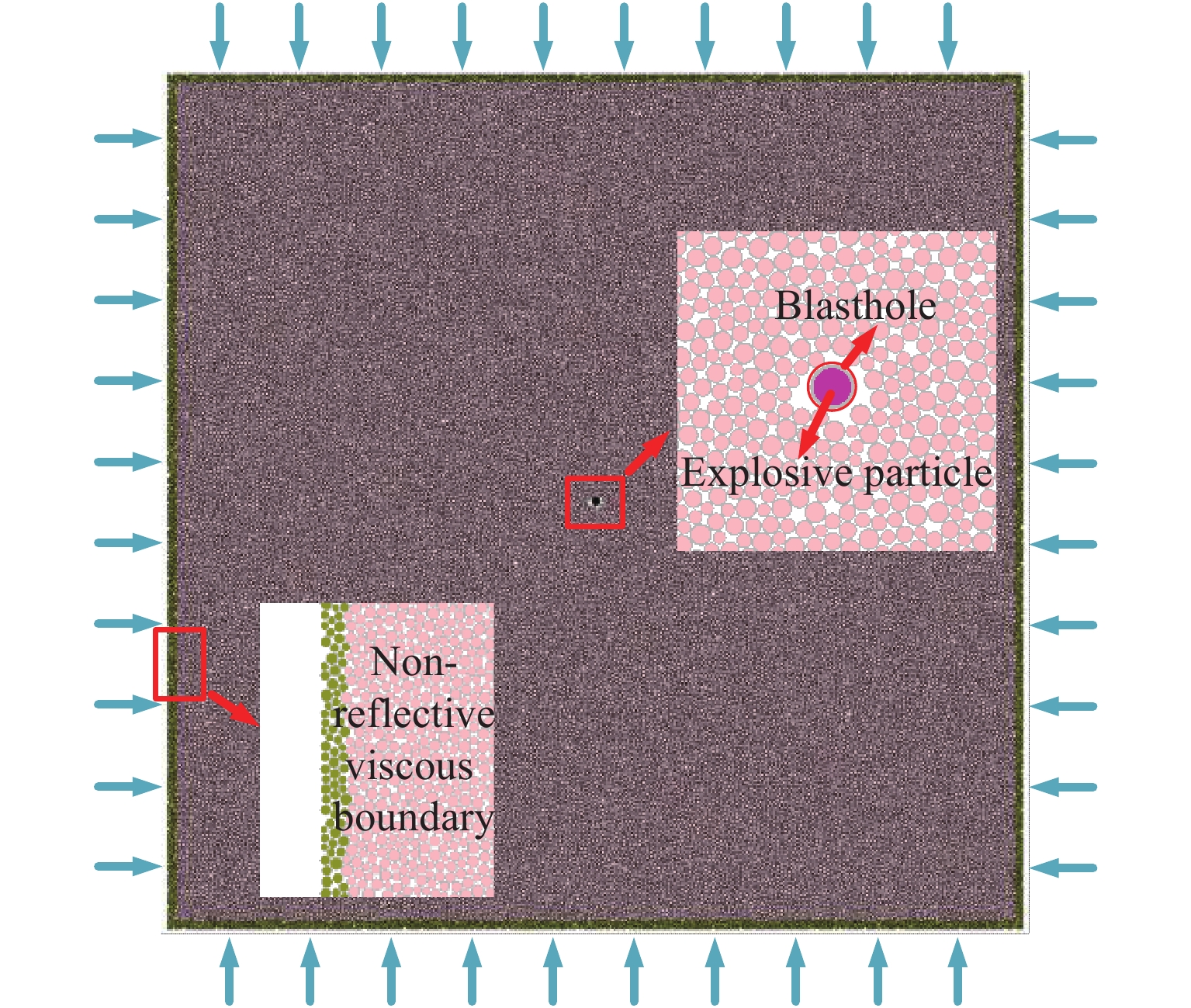
如图3所示,在长20 m、宽20 m的范围内共生成颗粒76445个,颗粒半径在0.0280~0.0465 m之间服从均匀分布,将模型伺服至稳定状态。为模拟无限岩体中的爆破过程,需在岩体周边设置无反射边界[17],以吸收爆炸应力波在边界处的反射拉伸作用。然而,在PFC中无法直接设置无反射边界,为此选取模型边界0.1 m范围内的颗粒作为无反射边界,在PFC中通过自编FISH语言,时刻监测爆破过程中边界颗粒的应力变化,施加与其大小相同、方向相反的外力,使其受力始终处于恒定状态,以此达到设置无反射边界的目的。
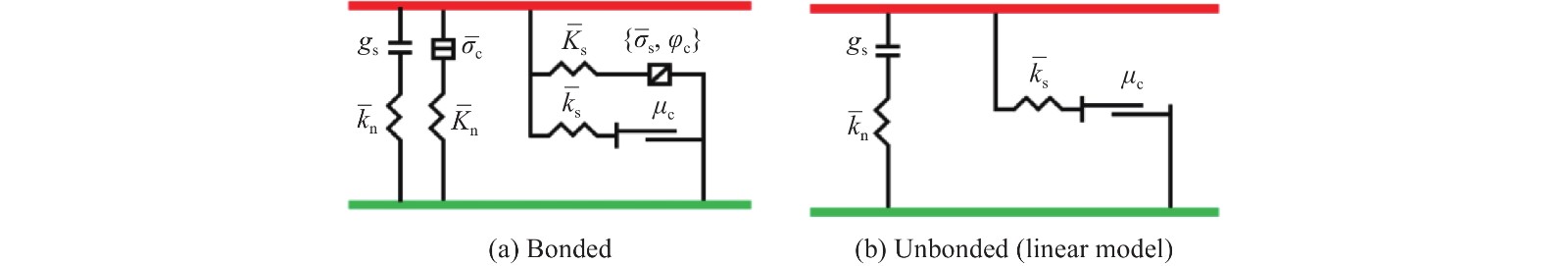
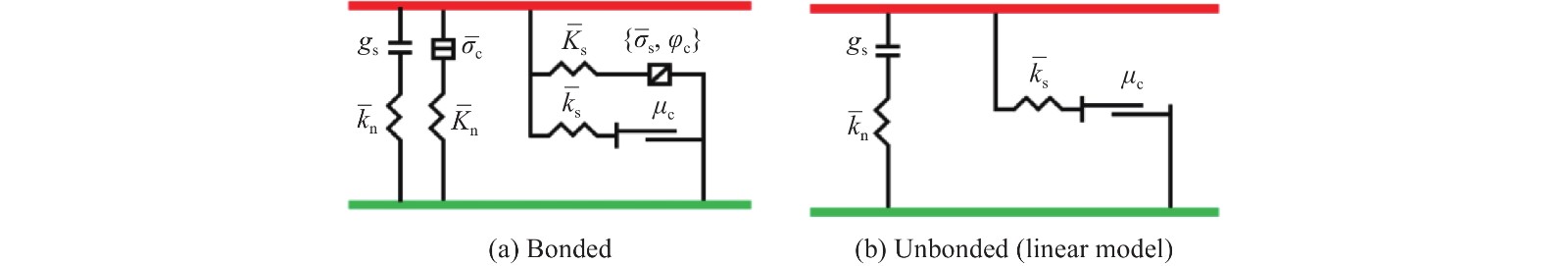
颗粒间的黏结采用PFC中模拟岩土类材料常用的平行黏结模型(parallel bond model,PBM)[18],其最大的优点是既可以承受力又可以承受力矩。PBM由黏结元件和弹簧元件组成,黏结状态(bonded)下,颗粒间的接触具有一定的法向刚度和切向刚度。黏结键强度符合莫尔-库仑准则,当外界作用力超过其黏结强度时,黏结键发生破坏,颗粒间的接触变为无黏结状态(unbonded),此时颗粒间的接触退化为完全弹性接触。PBM黏结元件的基本结构如图4所示。图4中,gs为黏结元件法向累积相对位移,
ˉkn 为线性接触法向刚度,ˉks 为线性接触切向刚度,ˉKn 为平行黏结法向刚度,ˉKs 为平行黏结切向刚度,μc 为摩擦系数,ˉσn 为法向黏结强度,ˉσs 为切向黏结强度,φc 为内摩擦角。细观参数的合理选取是保证PFC数值模拟结果符合实际的重要前提。以弹性模量、单轴抗压强度和泊松比作为标准,通过PFC静力学模拟实验标定数值模型的细观参数,使数值模型与真实岩石材料具有相近的宏观力学性质。在只关注岩石破坏模式和损伤状态的前提下,以该套细观参数进行动力学实验或大变形过程模拟同样具有一定的合理性[9, 12, 18]。
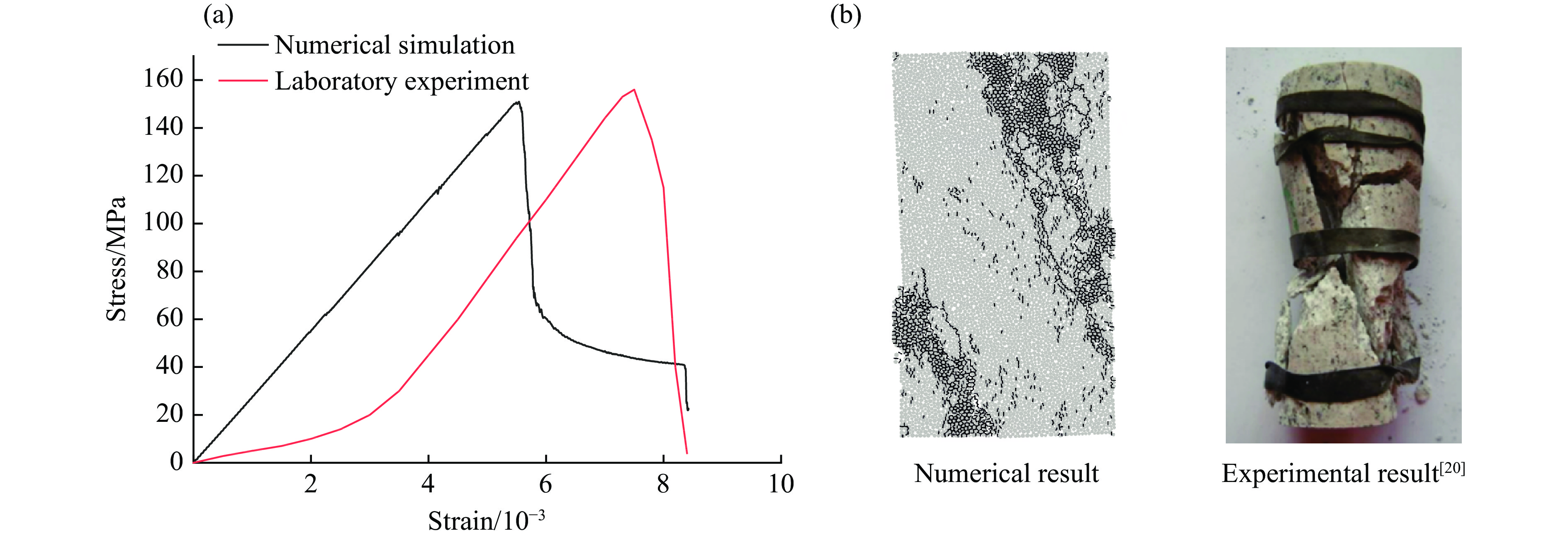
张学朋等[19]的研究表明,当PFC数值模拟的试样颗粒数较少时,模型无法获得稳定的力学性质。为了避免颗粒数目对标定结果产生影响,将待试标定样大小增加至宽4 m、长8 m,共生成6067个颗粒,颗粒体系与上述岩石介质的数值模型相同。岩石材质选取花岗岩,根据室内实验研究结果[20],采用“试错法”[21]在PFC中进行细观参数标定工作,最终确定了花岗岩试样的应力-应变曲线和破坏模式,如图5所示。试样的主要细观参数如表1所示,其中:Rmin为最小颗粒半径,Rmax为最大颗粒半径,Rmax/Rmin为粒径比,
ˉEl 为有效模量,ˉEp 为线性接触模量,ˉkn /ˉks 为线性接触刚度比,ˉKn /ˉKs 为平行黏结刚度比。表2列出了数值模拟和室内实验得到的试样抗拉强度σt 、弹性模量Et和泊松比vt 。表 1 主要细观参数Table 1. Main microscopic parametersLinear contact parameters Rmin/m Rmax/Rmin ˉEl/GPa ˉkn/ˉks μc 0.028 1.66 45 2.5 1.0 Parallel bond parameters ˉσn/MPa ˉσs/MPa ˉEp/GPa ˉKn/ˉKs φc/(°) 72 150 45 2.5 30 表 2 试样的主要力学参数Table 2. Main mechanical parameters of the sampleMethod Et/GPa σt/MPa vt ρ/(kg·m−3) Experiment 21.4 – 63.7 137.7 – 163.8 0.23 – 0.26 2650 – 2740 Numerical simulation 27.2 150.93 0.24 2700 2.2 不同不耦合系数下炮孔压力变化
根据Yuan等[9]的研究,炸药密度取
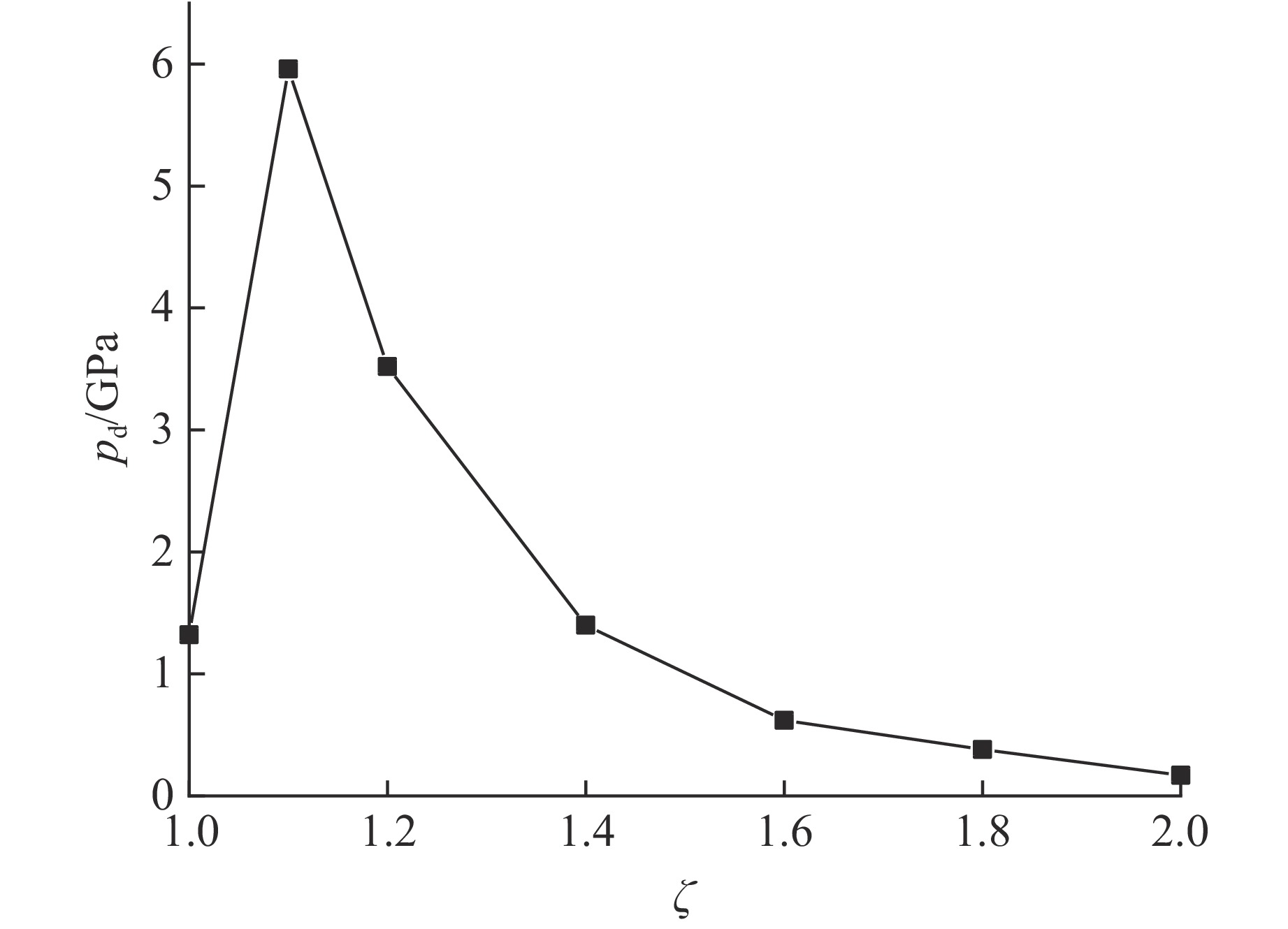
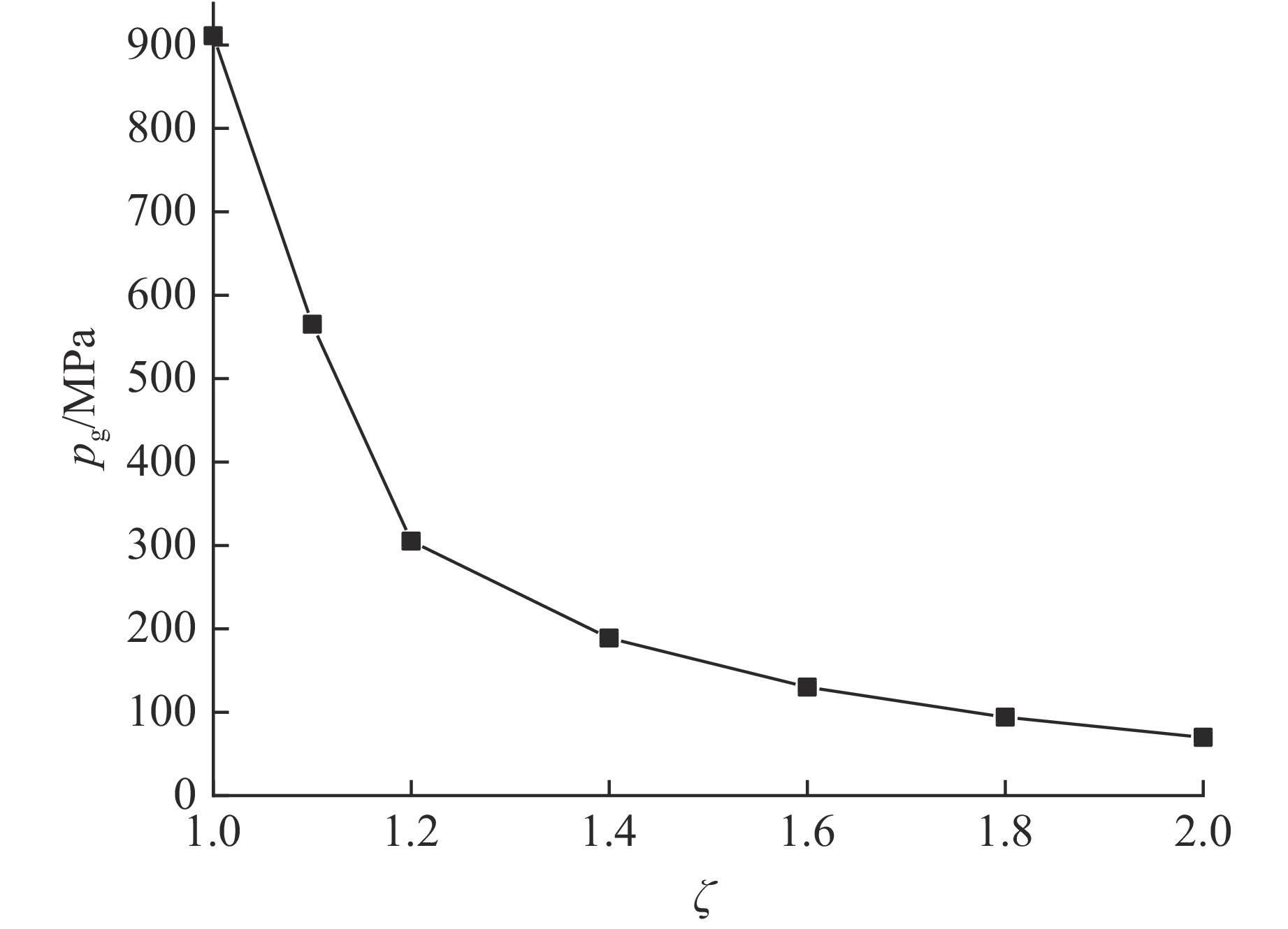
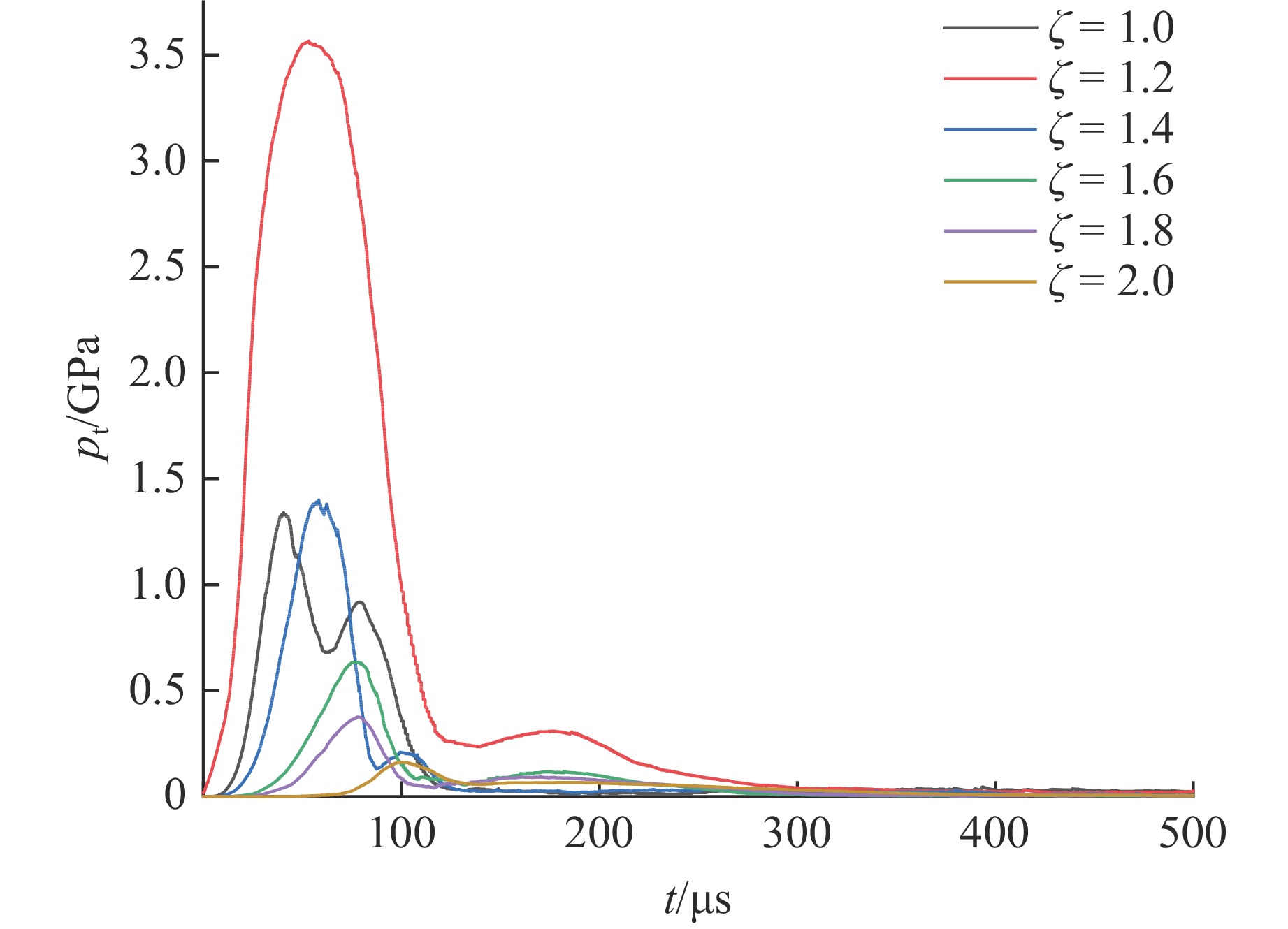
ρc = 1000 kg/m3,爆速vc = 2700 m/s。数值模拟中共取6种不耦合系数,不耦合系数与炮孔半径的对应关系见表3,其中:ζ =1.0对应耦合装药结构,其余为不耦合装药结构。由式(3)、式(4)和式(5),可计算出不同不耦合系数下的爆炸冲击压力和爆生气体压力,分别如图6和图7所示。表 3 不同不耦合系数对应的炮孔半径Table 3. Blasthole radius vs. decoupling coefficientζ rp ζ rp 1.0 0.060 1.6 0.096 1.2 0.072 1.8 0.108 1.4 0.084 2.0 0.120 根据计算结果,利用“动”、“静”荷载混合施加方法,在PFC中进行不同不耦合系数下花岗岩爆破模拟实验,得到的炮孔压力时程曲线见图8。
3. 计算结果分析
3.1 爆生裂纹扩展模式
为研究爆炸冲击波的“动”荷载和爆生气体的“静”荷载对爆生裂纹扩展模式的影响,以
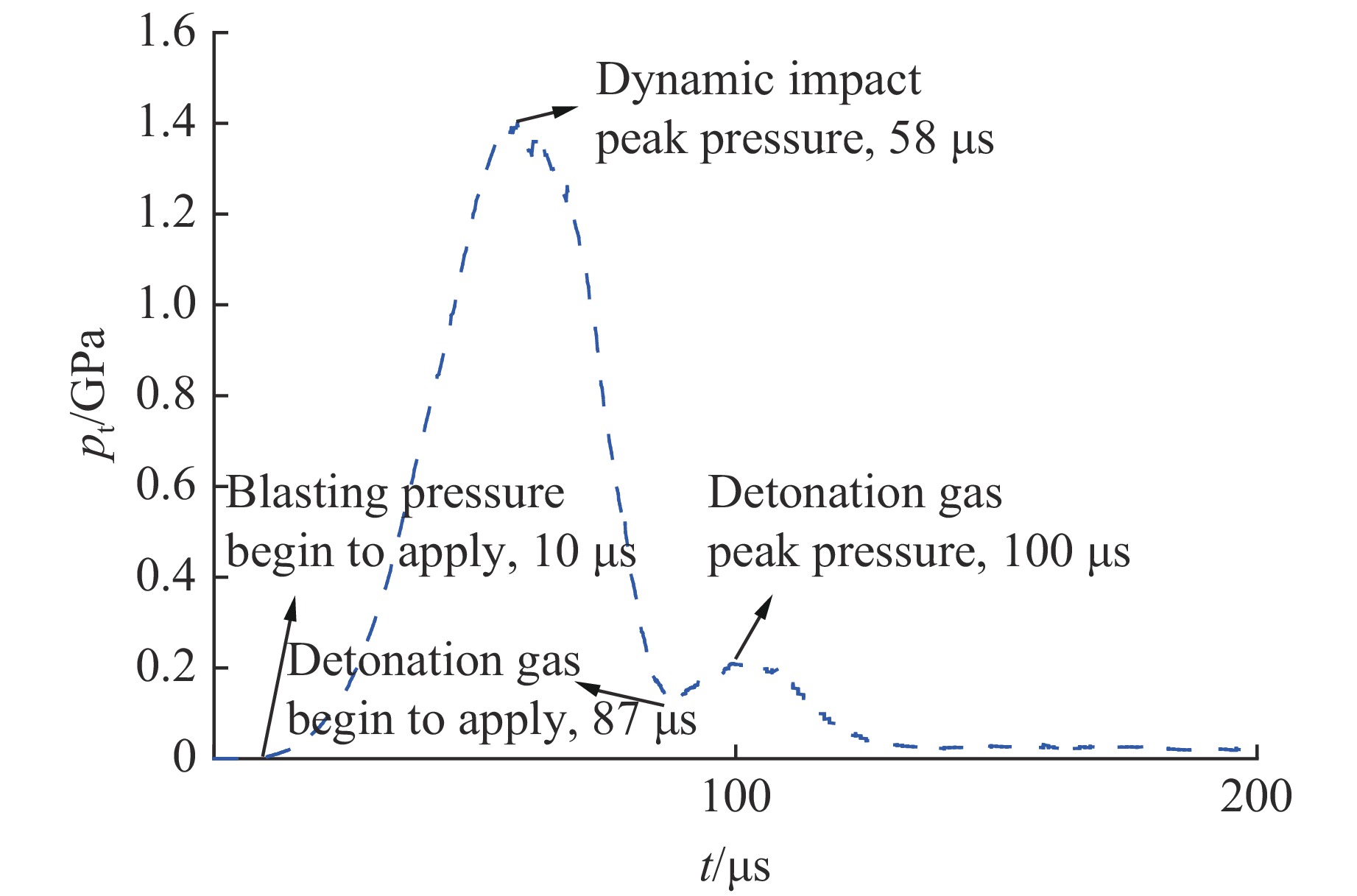
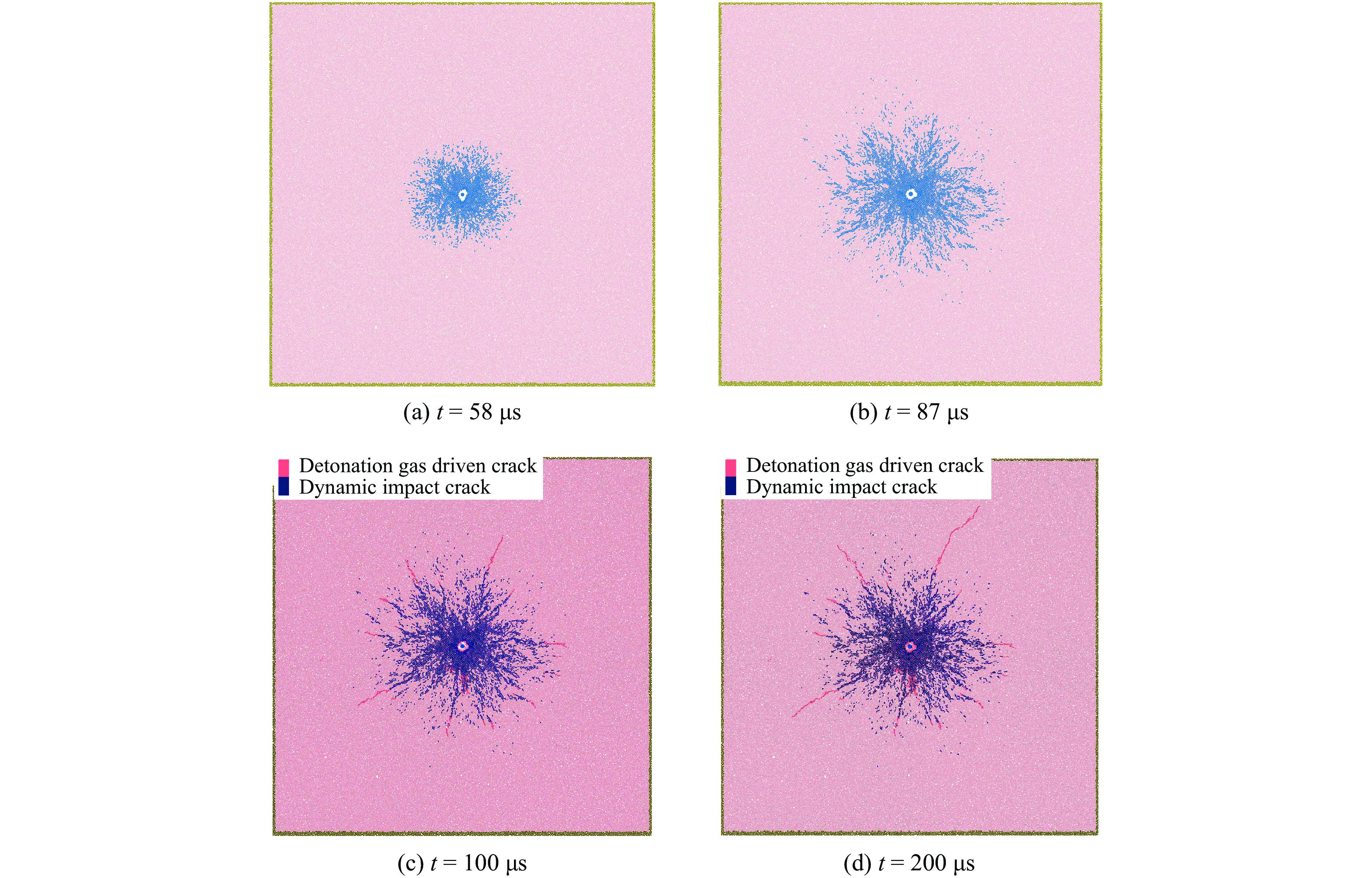
ζ =1.4时的爆生裂纹扩展过程为例进行说明。图9显示了ζ =1.4时的炮孔压力时程曲线和爆生裂纹扩展过程。对于不耦合装药,孔壁压力在10 μs左右开始施加。58 μs左右炮孔压力达到峰值,如图10(a)所示,此时炮孔周围岩石介质的损伤程度高,形成粉碎区[4]。至87 μs,为裂纹扩展阶段,由图10(b)可知,粉碎区外的径向裂纹在各个方向均有发育。由炮孔压力时程曲线可知,87 μs时爆生气体的扩腔作用开始施加,至100 μs时,在爆炸冲击波的动态预裂破岩作用下,爆生气体对径向裂纹产生驱裂作用,如图10(c)所示。爆生气体对裂纹的驱裂作用主要体现在对径向主裂纹尖端的延展作用,而对岩石的粉碎没有过多的作用。图10(d)显示了200 μs时爆生裂纹的最终分布情况。可以看出,100~200 μs区间爆生裂纹的扩展几乎全部源于爆生气体对裂纹尖端的延展作用。
3.2 不同不耦合系数下花岗岩爆破损伤模式及分析
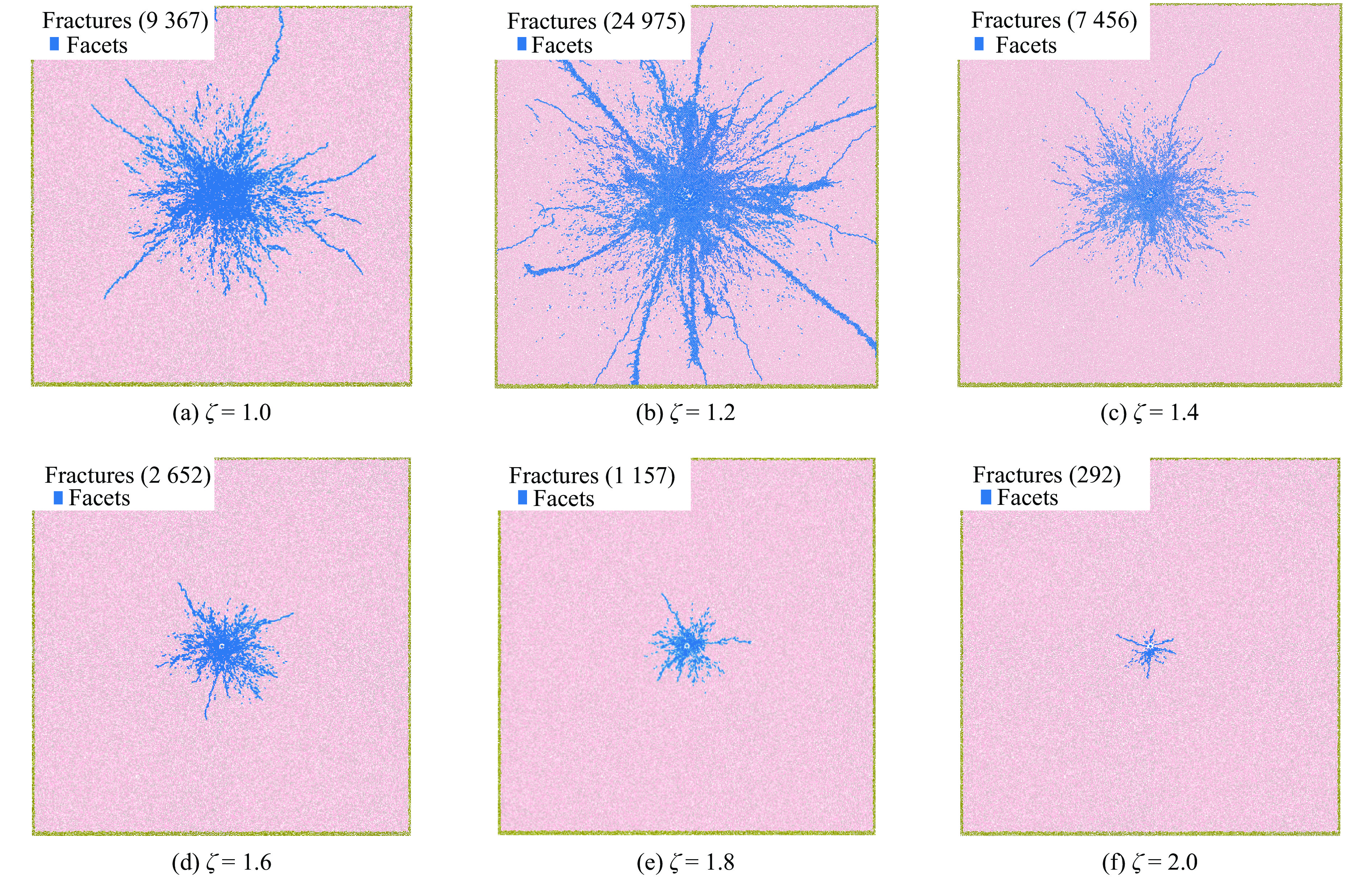
图11为计算至裂纹停止扩展时爆生裂纹的分布情况。
ζ =1.0时,即耦合装药结构下,爆炸冲击压力pt=1.32 GPa,爆生气体压力pg=911 MPa。炮孔周围各向裂纹发育较好,在爆生气体的扩腔作用和对裂纹的驱裂作用下,径向裂纹延展距离较远,个别径向裂纹扩展至边界处。岩石介质各部分弹性应变能的累积差异造成了部分裂纹的不连续传播。ζ =1.2时,pt=3.52 GPa,pg=305 MPa。较强的爆炸冲击应力使炮孔近区岩石得到充分破碎,径向裂纹数量极大地提升,爆生气体对裂纹的驱裂作用非常明显。ζ =1.4时,pt=1.40 GPa,pg=189 MPa,裂纹发育模式与ζ =1.0时相似,但裂纹扩展距离明显较小。ζ =1.6时,pt=0.62 GPa,pg=130 MPa,随着爆炸冲击压力的大幅降低,爆破损伤区域减小,裂纹数量减少,爆生气体的准静态压力降低至岩石的单轴抗压强度以下,对爆生裂纹的扩展作用明显减弱。ζ =1.8时,pt=0.38 GPa,pg=94 MPa,此时只有炮孔近区很小区域的岩石发生破坏,爆生气体压力对爆生裂纹扩展只起到微弱作用。ζ =2.0时,pt=0.17 GPa,pg=70 MPa,爆炸冲击应力比岩石的单轴抗压强度稍大,只能造成炮孔近区花岗岩介质的起裂,而较低的爆生气体压力在没有爆炸冲击应力预裂破碎的情况下对花岗岩介质的爆破损伤过程几乎没有影响。由上述花岗岩爆破损伤模拟结果可知,装药不耦合系数对爆生裂纹的产生、扩展和分布具有非常重要的影响。究其原因,是不耦合装药结构对爆炸冲击压力和爆生气体压力产生较大的影响,从而改变了岩石的爆破破坏模式。随着不耦合系数的增大,爆炸冲击压力先升高后降低;爆生气体压力随着不耦合系数的增大呈快速递减趋势。由爆破破岩理论[22]可知,粉碎区半径为药包半径的3~7倍,爆炸粉碎区域过大,会消耗掉绝大部分炸药能量,导致传入岩体内部用于切割破坏岩石的能量较少,造成炸药能量的不合理分布。因此,盲目地增加炮孔的入射能量并不能提高岩石整体的破岩效率。根据
ζ =1.2时岩体的爆破损伤模式可知,与ζ =1.0时相比,粉碎区域仅略微增大,但爆生裂纹宽度和扩展距离得到显著增加,提升了炸药能量的有效利用率。对比模拟的其他情况,ζ =1.2时爆破破岩效率最佳。因此,选取合理的不耦合系数对于提升炸药能量利用效率和岩石破碎效率具有重要意义。ζ =1.4时的爆炸冲击压力比ζ =1.0时只增加了80 MPa,爆生气体压力却下降了722 MPa。由爆生裂纹的最终分布情况可知,两种装药结构下,炮孔近区爆破破坏模式相似,损伤范围大致相同,说明爆生气体的准静态压力对岩石爆破破碎作用很小。然而,对于裂纹扩展,ζ =1.0时较大的爆生气体压力是爆生裂纹得以进一步扩展的主要原因。由此可知,爆生气体的准静态压力对于爆生裂纹的扩展具有重要作用。随着不耦合系数的进一步增大,ζ 为 1.6、1.8、2.0时,爆炸冲击波和爆生气体的压力大幅降低,爆破损伤程度均比耦合装药时小,说明较大的装药不耦合系数不利于爆破破岩工作的进行。值得注意的是,
ζ =1.2时,多数径向裂纹扩展至模型边界,由于设置的岩石模型区域较小,模拟中无法获取裂纹完全扩展后的分布情况,因此所获取的裂纹数量比完全扩展后的数量少。若进一步增大模型区域,会导致计算时间显著增加。考虑到本研究只关注模型区域内岩石介质的爆破损伤情况,因此研究结果仍然具有一定的合理性。另外,实际岩石具有不均质性,存在原生裂隙、节理等力学弱势面,可能对岩石的爆破损伤模式产生影响,对此本研究均不予考虑。3.3 不同不耦合系数下花岗岩爆破损伤程度的预测模型
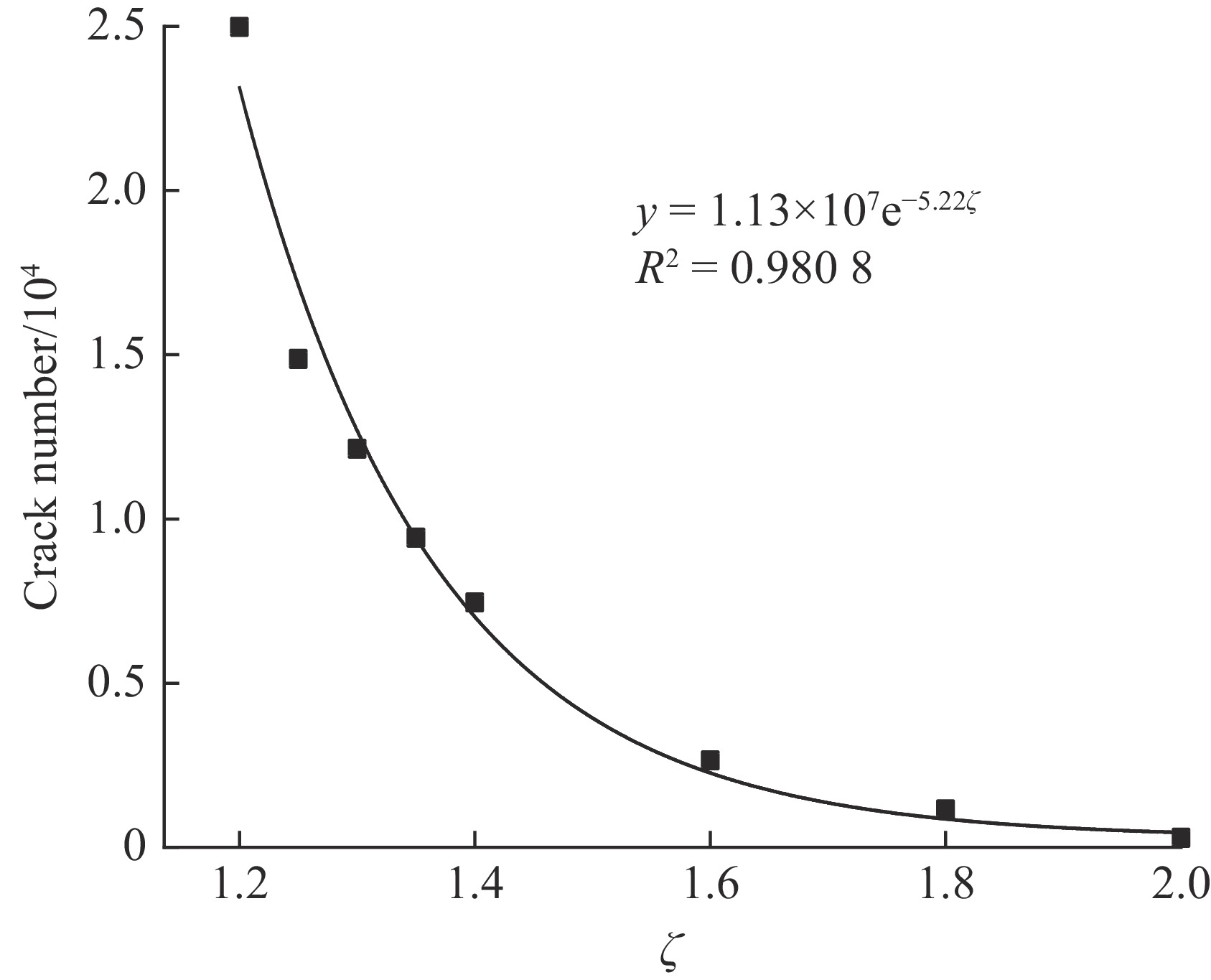
爆生裂纹数是反映岩石介质爆破损伤程度的重要参数。通过PFC中的DFN模块统计出不同不耦合系数下的爆生裂纹数y,如图12所示。
由于
ζ =1.0和ζ =1.2时的数据量较少,这里仅给出ζ ≥1.2时的爆生裂纹数预测模型,如图13所示,拟合公式为y =1.13×107e−5.22ζ (9) 从图13可以看出,e指数曲线对小不耦合系数拟合度更加敏感。为了提高预测模型的精度,通过模拟实验获得了
ζ 为1.25、1.30、1.35时的爆生裂纹数,依次为14916、12135和9427,实验过程不再赘述。由此得到拟合曲线的拟合精度R2为0.9808。该预测模型对于爆破施工设计具有较高的参考价值。4. 结 论
通过理论分析,结合数值模拟方法,研究了径向装药不耦合系数对花岗岩爆破损伤特性的影响,得到以下结论:
(1) 根据实际爆破过程,基于“颗粒膨胀加载法”,提出了一种“动”、“静”荷载混合施加的PFC爆破模拟方法,从实验中炮孔压力的变化情况来看,该方法可以很好地模拟爆炸冲击波和爆生气体共同作用下炮孔压力的变化;
(2) 分析了不同不耦合系数下爆炸冲击作用和爆生气体作用对花岗岩爆破损伤程度的影响,不耦合系数为1.2时花岗岩的爆破破岩最佳,由不耦合系数为1.4时的岩体损伤情况可知,爆生气体对爆生裂纹扩展具有重要作用;
(3) 花岗岩爆生裂纹数随着装药不耦合系数的增大近似呈指数下降,根据不耦合系数大于或等于1.2时的爆生裂纹数量,建立了岩体损伤程度与不耦合系数的预测模型,为
y=1.13×107e−5.22ζ ,拟合度达0.9808,预测模型对爆破施工设计具有较高的参考价值。 -
表 1 主要细观参数
Table 1. Main microscopic parameters
Linear contact parameters Rmin/m Rmax/Rmin ˉEl/GPa ˉkn/ˉks μc 0.028 1.66 45 2.5 1.0 Parallel bond parameters ˉσn/MPa ˉσs/MPa ˉEp/GPa ˉKn/ˉKs φc/(°) 72 150 45 2.5 30 表 2 试样的主要力学参数
Table 2. Main mechanical parameters of the sample
Method Et/GPa σt/MPa vt ρ/(kg·m−3) Experiment 21.4 – 63.7 137.7 – 163.8 0.23 – 0.26 2650 – 2740 Numerical simulation 27.2 150.93 0.24 2700 表 3 不同不耦合系数对应的炮孔半径
Table 3. Blasthole radius vs. decoupling coefficient
ζ rp ζ rp 1.0 0.060 1.6 0.096 1.2 0.072 1.8 0.108 1.4 0.084 2.0 0.120 -
[1] 宗琦, 孟德君. 炮孔不同装药结构对爆破能量影响的理论探讨 [J]. 岩石力学与工程学报, 2003, 22(4): 641–645. doi: 10.3321/j.issn:1000-6915.2003.04.027ZONG Q, MENG D J. Influence of different kinds of hole charging structue on explosion energy transmission [J]. Chinese Journal of Rock Mechanics and Engineering, 2003, 22(4): 641–645. doi: 10.3321/j.issn:1000-6915.2003.04.027 [2] 岳中文, 胡晓冰, 陈志远, 等. 不耦合装药对炸药能量利用率影响的实验研究 [J]. 爆破, 2020, 37(3): 34–39. doi: 10.3963/j.issn.1001-487X.2020.03.006YUE Z W, HU X B, CHEN Z Y, et al. Experimental study of effect of uncoupled charge on energy utilization efficiency of explosives [J]. Blasting, 2020, 37(3): 34–39. doi: 10.3963/j.issn.1001-487X.2020.03.006 [3] WANG Y B. Study of the dynamic fracture effect using slotted cartridge decoupling charge blasting [J]. International Journal of Rock Mechanics and Mining Sciences, 2017, 96: 34–46. doi: 10.1016/j.ijrmms.2017.04.015 [4] 汪旭光. 爆破设计与施工 [M]. 北京: 冶金工业出版社, 2011.WANG X G. Blasting design and construction [M]. Beijing: Metallurgical Industry Press, 2011. [5] 叶志伟, 陈明, 李桐, 等. 小不耦合系数装药爆破孔壁压力峰值计算方法 [J]. 爆炸与冲击, 2021, 41(6): 064901. doi: 10.11883/bzcyj-2020-0185YE Z W, CHEN M, LI T, et al. A calculation method of the peak pressure on borehole wall for low decoupling coefficient charge blasting [J]. Explosion and Shock Waves, 2021, 41(6): 064901. doi: 10.11883/bzcyj-2020-0185 [6] 徐颖, 孟益平, 程玉生. 装药不耦合系数对爆破裂纹控制的试验研究 [J]. 岩石力学与工程学报, 2002, 21(12): 1843–1847. doi: 10.3321/j.issn:1000-6915.2002.12.020XU Y, MENG Y P, CHENG Y S. Study on control of blast crack by decoupling charge index [J]. Chinese Journal of Rock Mechanics and Engineering, 2002, 21(12): 1843–1847. doi: 10.3321/j.issn:1000-6915.2002.12.020 [7] 费鸿禄, 李守巨, 何庆志. 光面爆破装药不偶合系数的计算 [J]. 爆炸与冲击, 1992, 12(3): 270–274.FEI H L, LI S J, HE Q Z. Determintion of decouple coefficient and analysis of decouple action in the smooth blasting [J]. Explosion and Shock Waves, 1992, 12(3): 270–274. [8] 杨仁树, 肖成龙, 李永亮, 等. 不耦合偏心装药结构爆破损伤破坏的分形研究 [J]. 振动与冲击, 2020, 39(12): 129–134. doi: 10.13465/j.cnki.jvs.2020.12.017YANG R S, XIAO C L, LI Y L, et al. A fractal study on blasting damage of an eccentric decouple charge structure [J]. Journal of Vibration and Shock, 2020, 39(12): 129–134. doi: 10.13465/j.cnki.jvs.2020.12.017 [9] YUAN W, WANG W, SU X B, et al. Numerical study of the impact mechanism of decoupling charge on blasting-enhanced permeability in low-permeability sandstones [J]. International Journal of Rock Mechanics and Mining Sciences, 2018, 106: 300–310. doi: 10.1016/j.ijrmms.2018.04.029 [10] 杜俊林, 罗强, 宗琦. 空气不耦合装药爆破孔壁冲击压力分析 [J]. 西安科技大学学报, 2005, 25(3): 306–310. doi: 10.3969/j.issn.1672-9315.2005.03.009DU J L, LUO Q, ZONG Q. Analysis on preliminary shock pressure on borehole of air-de-coupling charging [J]. Journal of Xi’an University of Science and Technology, 2005, 25(3): 306–310. doi: 10.3969/j.issn.1672-9315.2005.03.009 [11] PARK D, JEON B, JEON S. A numerical study on the screening of blast-induced waves for reducing ground vibration [J]. Rock Mechanics and Rock Engineering, 2009, 42(3): 449–473. doi: 10.1007/s00603-008-0016-y [12] 吴再海, 安龙, 齐兆军, 等. 基于LS-DYNA与PFC联合的岩体爆破数值模拟方法分析 [J]. 采矿与安全工程学报, 2021, 38(3): 609–614. doi: 10.13545/j.cnki.jmse.2020.0133WU Z H, AN L, QI Z J, et al. The numerical simulation method of rock mass blasting based on PFC combined with LS-DYNA [J]. Journal of Mining & Safety Engineering, 2021, 38(3): 609–614. doi: 10.13545/j.cnki.jmse.2020.0133 [13] 许彪. 基于PFC的岩石控制爆破技术研究 [D]. 淮南: 安徽理工大学, 2018.XU B. Research on controlled blasting technology of rock based on PFC [D]. Huainan: Anhui University of Science and Technology, 2018. [14] ZHAO J J, ZHANG Y, RANJITH P G. Numerical modelling of blast-induced fractures in coal masses under high in-situ stresses [J]. Engineering Fracture Mechanics, 2019, 225: 106749. doi: 10.1016/j.engfracmech.2019.106749 [15] 孙宁新, 雷明锋, 张运良, 等. 软弱夹层对爆炸应力波传播过程的影响研究 [J]. 振动与冲击, 2020, 39(16): 112–119, 147. doi: 10.13465/j.cnki.jvs.2020.16.016SUN N X, LEI M F, ZHANG Y L, et al. A study on the influence of weak interlayer on the propagation process of explosion stress wave [J]. Journal of Vibration and Shock, 2020, 39(16): 112–119, 147. doi: 10.13465/j.cnki.jvs.2020.16.016 [16] 张玉磊, 苏健军, 李芝绒, 等. TNT内爆炸准静态压力特性 [J]. 爆炸与冲击, 2018, 38(6): 1429–1434. doi: 10.11883/bzycj-2017-0170ZHANG Y L, SU J J, LI Z R, et al. Quasi-static pressure characteristic of TNT’s internal explosion [J]. Explosion and Shock Waves, 2018, 38(6): 1429–1434. doi: 10.11883/bzycj-2017-0170 [17] 石崇, 张强, 王盛年. 颗粒流(PFC5.0)数值模拟技术及应用 [J]. 岩土力学, 2018, 39(Suppl 2): 36.SHI C, ZHANG Q, WANG S N. Numerical simulation technology and application with particle flow code (PFC 5.0) [J]. Rock and Soil Mechanics, 2018, 39(Suppl 2): 36. [18] POTYONDY D O. Simulating stress corrosion with a bonded-particle model for rock [J]. International Journal of Rock Mechanics and Mining Sciences, 2007, 44(5): 677–691. doi: 10.1016/j.ijrmms.2006.10.002 [19] 张学朋, 王刚, 蒋宇静, 等. 基于颗粒离散元模型的花岗岩压缩试验模拟研究 [J]. 岩土力学, 2014, 35(Suppl 1): 99–105.ZHANG X P, WANG G, JIANG Y J, et al. Simulation research on granite compression test based on particle discrete element model [J]. Rock and Soil Mechanics, 2014, 35(Suppl 1): 99–105. [20] QIU J D, LI D Y, LI X B, et al. Numerical investigation on the stress evolution and failure behavior for deep roadway under blasting disturbance [J]. Soil Dynamics and Earthquake Engineering, 2020, 137: 106278. doi: 10.1016/j.soildyn.2020.106278 [21] 孙闯, 敖云鹤, 张家鸣, 等. 花岗岩细观破裂特征及宏观尺度效应的颗粒流研究 [J]. 岩土工程学报, 2020, 42(9): 1687–1695. doi: 10.11779/CJGE202009013SUN C, AO Y H, ZHANG J M, et al. Particle flow of meso-fracture characteristics and macro-scale effect of granites [J]. Chinese Journal of Geotechnical Engineering, 2020, 42(9): 1687–1695. doi: 10.11779/CJGE202009013 [22] 李夕兵. 岩石动力学基础与应用 [M]. 北京: 科学出版社, 2014: 258–287.LI X B. Rock dynamics fundamentals and applications [M]. Beijing: Science Press, 2014: 258–287. -







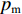
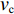
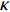
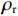
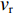
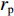
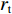
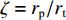
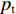
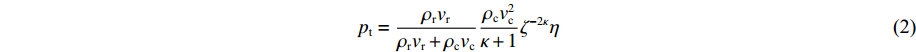
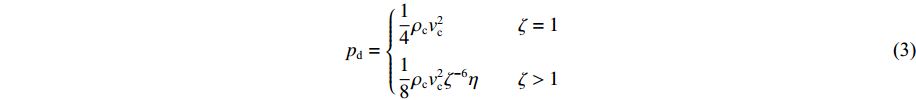
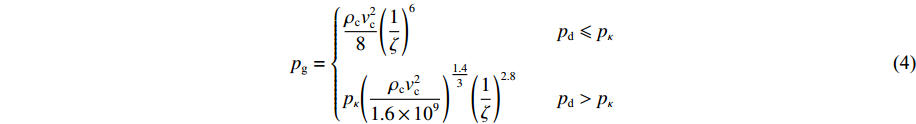
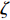
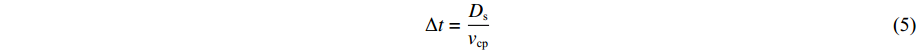
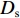
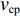


 下载:
下载:
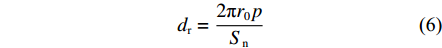
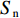
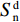
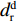
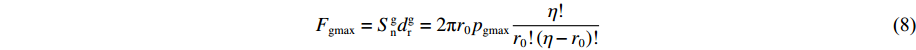
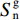
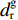
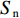
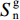
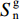
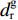
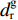


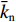
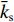

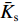
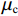
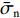
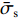
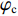

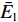

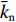
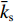
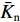
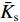
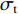
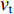

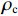





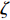
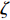







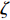
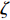
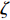
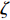
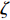
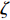

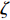
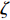
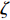
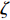
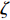
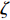
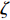
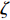

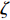

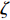

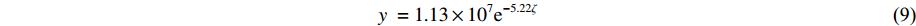
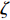
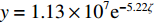

 下载:
下载: