Ground State Study of Quantum Material GaTa4Se8
-
摘要: 量子材料GaTa4Se8(GTS)不仅展现出绝缘体到金属相变、Jeff量子态以及拓扑超导等多种有趣的物理性质,而且还是电阻开关和存储介质材料,一直以来备受关注。当前科学家们对其绝缘基态的结构仍存在争议,基态结构的不确定阻碍了对其各种物理性质的深入理解。GaTa4Se8的绝缘基态长期被认为具有立方对称结构(空间群
Fˉ43m ),其电子能隙是由自旋轨道耦合效应和电子关联共同作用形成的Mott型能隙。最近第一性原理计算表明,立方结构的声子谱存在虚频而不稳定,并预测立方对称存在结构畸变会形成更稳定的三方结构(R3m )或四方结构(Fˉ421m )。为此,本研究通过压力调节该材料的电子能隙,结合Raman光谱、X射线衍射、电阻测量等多种实验表征手段,对比实验得到的数据与第一性原理计算结果,进一步探索GaTa4Se8的基态结构。研究表明三方对称结构(R3m )更符合实验观察结果。Abstract: The quantum material GaTa4Se8 has attracted a substantial amount of attention because it exhibits a variety of interesting physical properties, such as metallization, Jeff quantum state, and topological superconductivity, and moreover, it is a medium for resistive switch and electric storage. However, controversies still exist on its insulating ground state, which hinders from understanding its various physical properties. The insulating ground state of GaTa4Se8 has been considered over a long period of time as a cubic symmetric structure with space groupFˉ43m , and as a Mott-type energy gap driven by the combination of the spin-orbit coupling and the electronic correlation interaction. However, recent first-principles phonon calculations have shown that the cubic structure is mechanically unstable due to the presence of imaginary frequencies, and have predicted to be stabilized into the trigonal structure (R3m ) or the tetragonal structure (Fˉ421m ) through lattice distortion. In order to further investigate the ground state structure of GaTa4Se8, here we combine multiple experimental techniques such as Raman spectroscopy, X-ray diffraction, and resistance measurement to adjust its energy gap by pressure, and compare the experimental results with first-principles calculations. Our results show that the trigonal symmetric structure (R3m ) is more consistent with our experimental observations. -
仿贝壳珍珠层复合材料作为新型复合材料,有着优异的力学性能,不仅具有很高的强度,而且具有很好的韧性,近年来引起了学术界的不断关注[1-6]。很多发达国家非常重视贝壳结构材料和仿生材料研究,如美国等国家设置了专门的经费来研究贝壳生物材料的仿生设计和性能,用于装甲防弹衣和防爆装置。贝壳珍珠层复合材料的优异力学性能与其微观结构密切联系,为此研究人员对珍珠质的微观结构特征(体积分数、片剂长宽比、重叠长度等)进行了深入分析,试图将其与模型的力学性能联系起来[7-12]。Dutta等[13]研究了珍珠层中裂纹的萌生规律,评估了重叠长度对裂纹尖端驱动力的影响。Kotha等[14]的研究显示,低纵横比的文石片可以制造出具有高韧性的复合材料。Barthelat等[15]发现,珍珠层没有实现稳定状态的裂纹扩展,并将其归因于片层拔出增韧机制。其他学者也发现珍珠层内部和外部韧化机制阻止了裂纹的扩展[16-21]。
Barthelat等[15]通过观察发现,在每层贝壳珍珠层中,平板的排列与Voronoi图相似,从一个红色鲍鱼标本的光学图像中可以看到每个贝壳层压板都有矿物片的随机分布,并与其他珍珠层成键。基于这些光学图像,他们生成了一个由两层贝壳的平板结构组成的几何模型,用于有限元分析。自1907年Shamos和Hoey提出分治算法的最初定义和描述之后,Voronoi图便成为众多学科的中心主题之一。Voronoi图所具有的自然描述性和操纵能力,使其获得了广泛应用[22-24]。尽管Voronoi图对科学和工程中的各种应用具有重大的潜在影响,但是在很多领域包括仿生结构领域,Voronoi结构对材料力学性能的影响还未得到透彻的理解,为此本工作将探讨Voronoi结构的随机性对仿贝壳珍珠层结构力学性能的影响。
为了研究仿贝壳珍珠层Voronoi随机模型结构的动态力学响应,首先建立一种铝/乙烯基复合材料结构的三维Voronoi模型,然后对模型在弹丸冲击载荷下的动态力学性能进行有限元模拟分析,最后讨论黏结层厚度和Voronoi模型分块尺寸对模型抗冲击力学性能的影响。
1. Voronoi随机模型生成
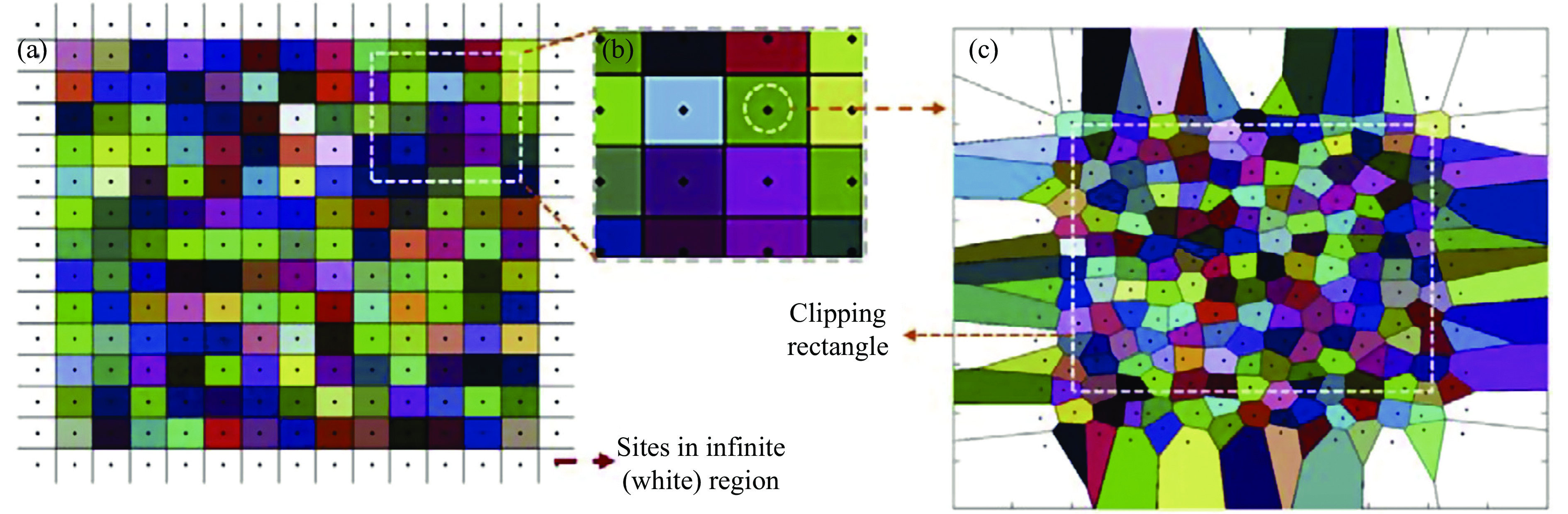
利用文献[25]给出的随机Voronoi技术生成Voronoi随机模型。图1描述了仿贝壳珍珠层随机Voronoi结构的生成技术。图1(a)显示了由网格组成的Voronoi初始构型,每个网格内都包含1个站点;站点可以在圆内随机移动,如图1(b)所示,站点位置
(x,y) 由极坐标控制方程决定 图 1 (a) Voronoi图的初始网格构型;(b)每个站点都在一个圆圈区域内随机移动;(c)新的Voronoi图是从新的站点系统中生成,通过矩形裁剪,形成有限区域的随机Voronoi图[25]Figure 1. (a) Initial grid formation of Voronoi diagram; (b) each site moves randomly within a circle region; (c) a new Voronoi diagram is generated from the new site system, and by a rectangle cut, a finite Voronoi diagram is generated[25]
图 1 (a) Voronoi图的初始网格构型;(b)每个站点都在一个圆圈区域内随机移动;(c)新的Voronoi图是从新的站点系统中生成,通过矩形裁剪,形成有限区域的随机Voronoi图[25]Figure 1. (a) Initial grid formation of Voronoi diagram; (b) each site moves randomly within a circle region; (c) a new Voronoi diagram is generated from the new site system, and by a rectangle cut, a finite Voronoi diagram is generated[25]x=x0+rcosθ,y=y0+rsinθ (1) 式中:
(x0,y0) 为网格内站点的参考位置;r 为(0,R) 之间的随机值,R 为圆的半径;θ 为(0,2π) 之间的随机值。通过站点的随机移动,最终形成新的随机Voronoi图,如图1(c)所示。新生成的Voronoi图由一个无限大的区域组成,采用矩形切割使新生成的Voronoi图限定在有限区域内,丢弃有限区域外的站点和多边形网格,最终形成仿贝壳珍珠层随机Voronoi模型。2. Voronoi模型有限元分析
2.1 几何建模
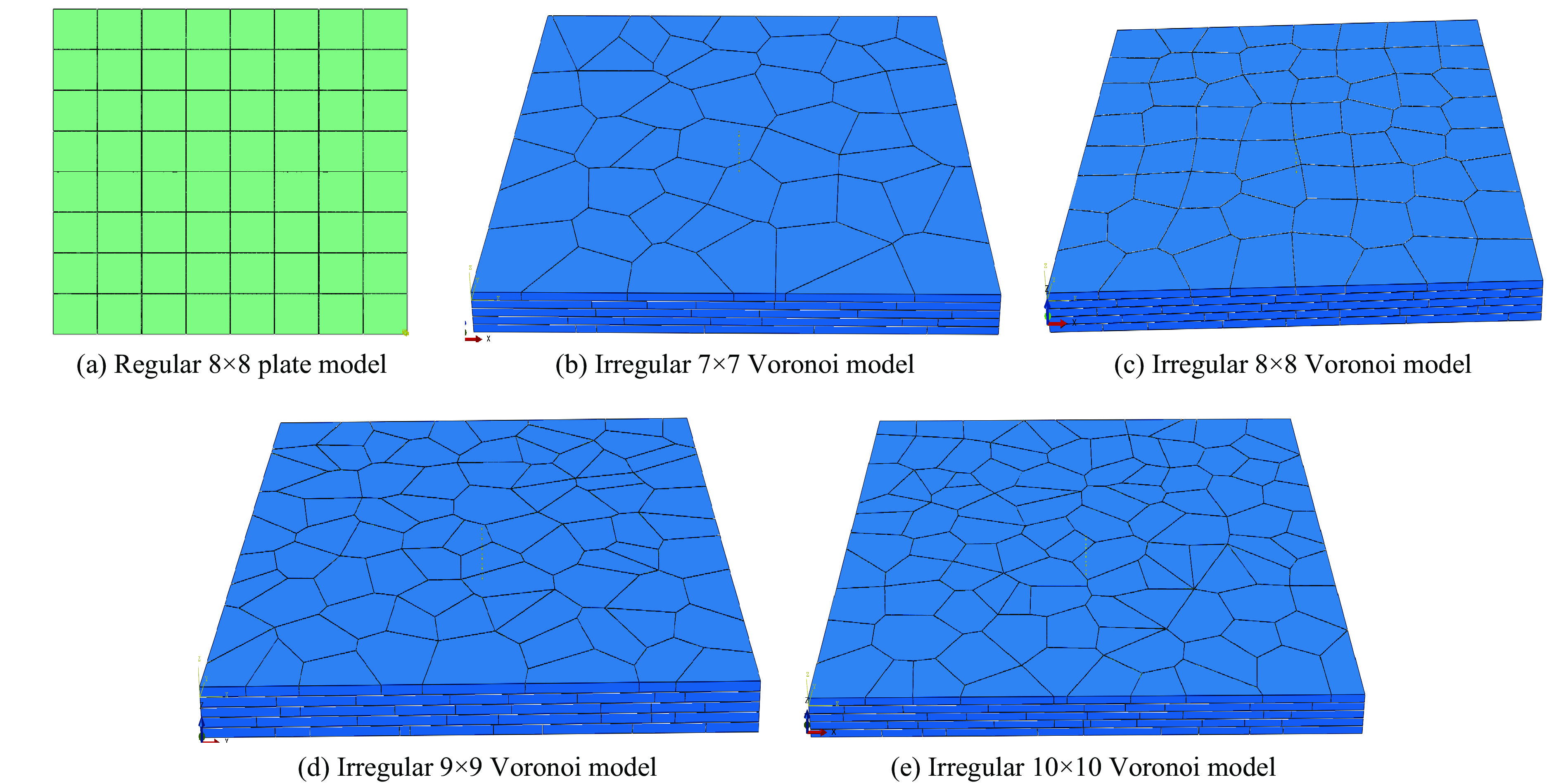
图2给出了规则片板单元模型以及4种不同分块尺寸的不规则Voronoi片板模型,5种模型的总体几何尺寸相同,均为240 mm × 240 mm × 15 mm,模型总层数均为5层。图2(a)为规则片板模型,每个规则片板的几何尺寸为30 mm × 30 mm × 3 mm,每层由8 × 8共64个片板组成,图2(b)~图2(e)分别给出了7 × 7、8 × 8、9 × 9和10 × 10分块的Voronoi不规则模型,每种模型均包括5层不同的随机单层结构,每层厚度为3 mm。
为了模拟仿贝壳珍珠层片层之间受冲击破坏时的脱黏现象,采用了内聚力Cohesive模型。通过合理的参数选择,内聚力Cohesive模型能够部分描述贝壳珍珠层内部层与层之间的变形和失效现象[2]。在片板之间以及板层之间插入Cohesive黏结层,考虑3种黏结层厚度0.1、0.2和0.3 mm,讨论黏结层厚度对模型冲击损伤的影响。
内聚力Cohesive模型的牵引分离定律涉及黏性牵引应力矢量
T={tn,ts,tt} ,其中下标n、s和t分别表示一个法向和两个切向分量。这些变量之间满足双线性二次黏聚律[25](tnt0n)2+(tst0s)2+(ttt0t)2=1 (2) 式中:
t0n 、t0s 、t0t 分别表示变形垂直于界面以及在第一、第二剪切方向上的最大应力。Cohesive黏结层刚度退化速率满足
(GnG0n)2+(GsG0s)2+(GtG0t)2=1 (3) 式中:
Gn 、Gs 、Gt 分别为正向和两个切向的断裂能,G0n 、G0s 、G0t 分别为正向和两个切向引起破坏所需的最大断裂能。2.2 材料参数
仿贝壳珍珠层三维Voronoi结构模型包括两种材料模型,其中片层采用铝AA5083-H116,片层之间Cohesive黏性层使用乙烯树脂材料。表1和表2列出了两种材料参数[19, 25],其中
ρ 为密度,ν 为泊松比,E为弹性模量,Es和Et为两个切向弹性模量。表 1 铝片的材料参数Table 1. Parameters of aluminum plateMaterial ρ/(kg·m−3) ν E/GPa Aluminum 2750 0.3 72 表 2 Cohesive模型的材料参数Table 2. Parameters of Cohesive modelt0n/MPa t0s/MPa t0t/MPa G0n/(kJ·m−2) G0s/(kJ·m−2) G0t/(kJ·m−2) ρ/(kg·m−3) Es/GPa Et/GPa 80 80 80 1 1 1 1 850 4 1.5 在大应变情况下,铝合金的本构关系可采用Johnson-Cook模型描述
σ=(A+Bεn)(1+Cln˙ε˙ε0)[1−(T−TrT−Tm)m] (4) 式中:
σ 为等效应力,A 为材料在常温准静态下的屈服强度,B 为应变强化系数,n 为强化指数,ε 为等效塑性应变,˙ε 为塑性应变率,˙ε0 为初始应变率,C 为应变率敏感系数,m 为温度软化系数,Tm 为材料的熔点,Tr 为参考温度(取常温),T 为当前温度。表3列出了Johnson-Cook模型参数[25]。2.3 加载条件与边界条件
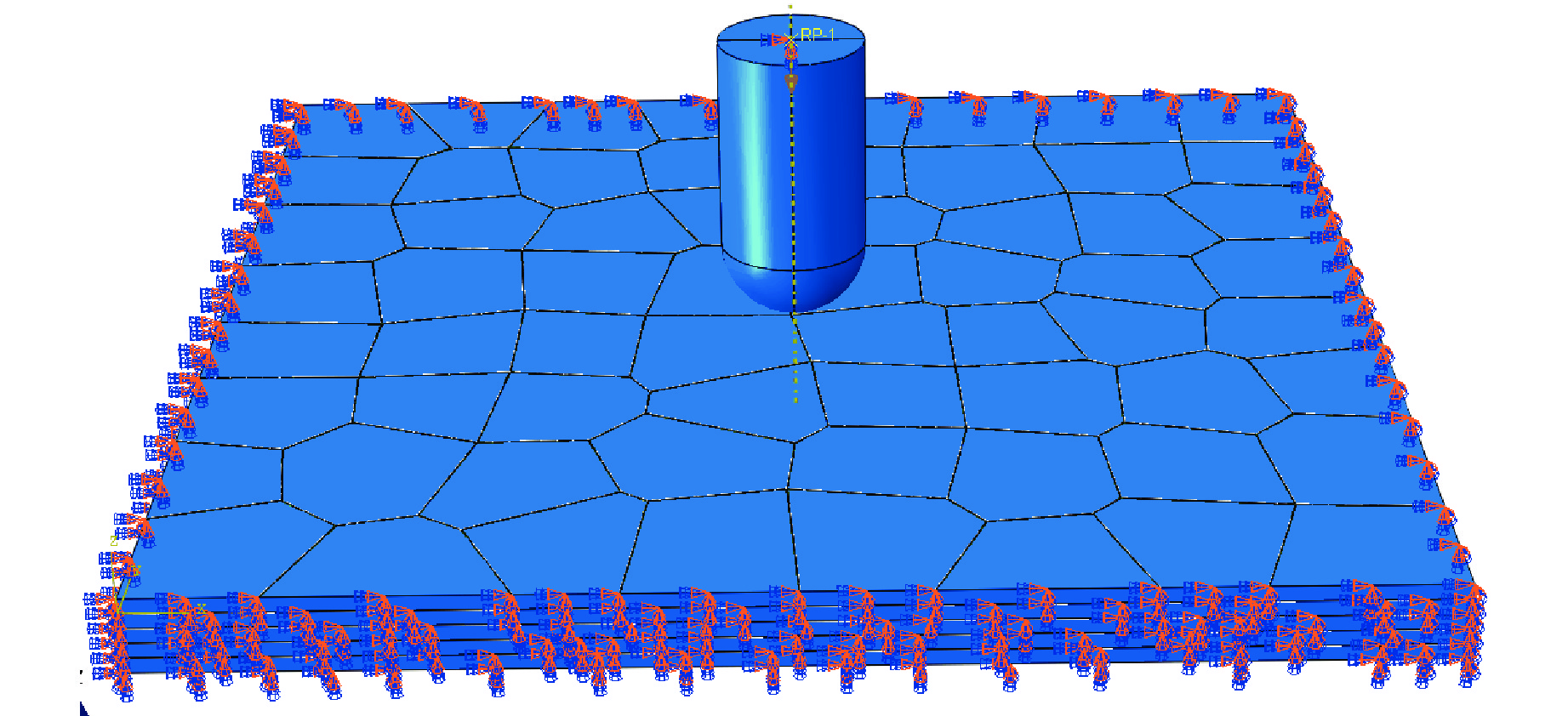
图3显示了模型的边界条件和加载条件。弹丸以18 m/s的初速度冲击Voronoi模型,弹丸速度属于中低速范围。弹丸模型上半部分是一个半径15 mm、长45 mm的圆柱体,下半部分是一个半径为15 mm的半球体,总长为60 mm,刚体属性。冲击载荷下仿贝壳珍珠层三维Voronoi模型的边界条件为4个侧边均完全固定,弹丸与复合结构模型的接触为通用接触,弹丸作用在复合结构模型中心。
黏结层网格类型采用COH3D8,铝片网格类型采用C3D8R,黏结层网格大小为1 mm,铝片网格大小为2 mm。在该网格密度下,模型的网格单元总数达到167230。节点总数为567001时,最大应力值保持稳定,网格的收敛性较好。
3. 模拟结果分析
3.1 有限元分析
在珍珠层结构中,片板滑动机制被认为是激活内在和外在韧化机制的关键因素,可以阻止裂纹扩展。该机制分别引起内聚力和残余塑性应变,从而闭合裂纹。由于弹丸冲击载荷方向垂直于Voronoi板模型,冲击载荷破坏的主要形式是黏性层剥离,因此片板滑动引起的增韧机制在这种特定的冲击加载问题中不占主导地位。在冲击载荷下,损伤和变形耗散的能量比摩擦接触要多得多,Voronoi模型对珍珠层结构负载分配和能量吸收机制的影响是所要考虑的主要因素。本研究首先分析冲击载荷下模型的动态响应,在此基础上考察不规则Voronoi片板等几何因素对动态力学特性和能量分配的影响。
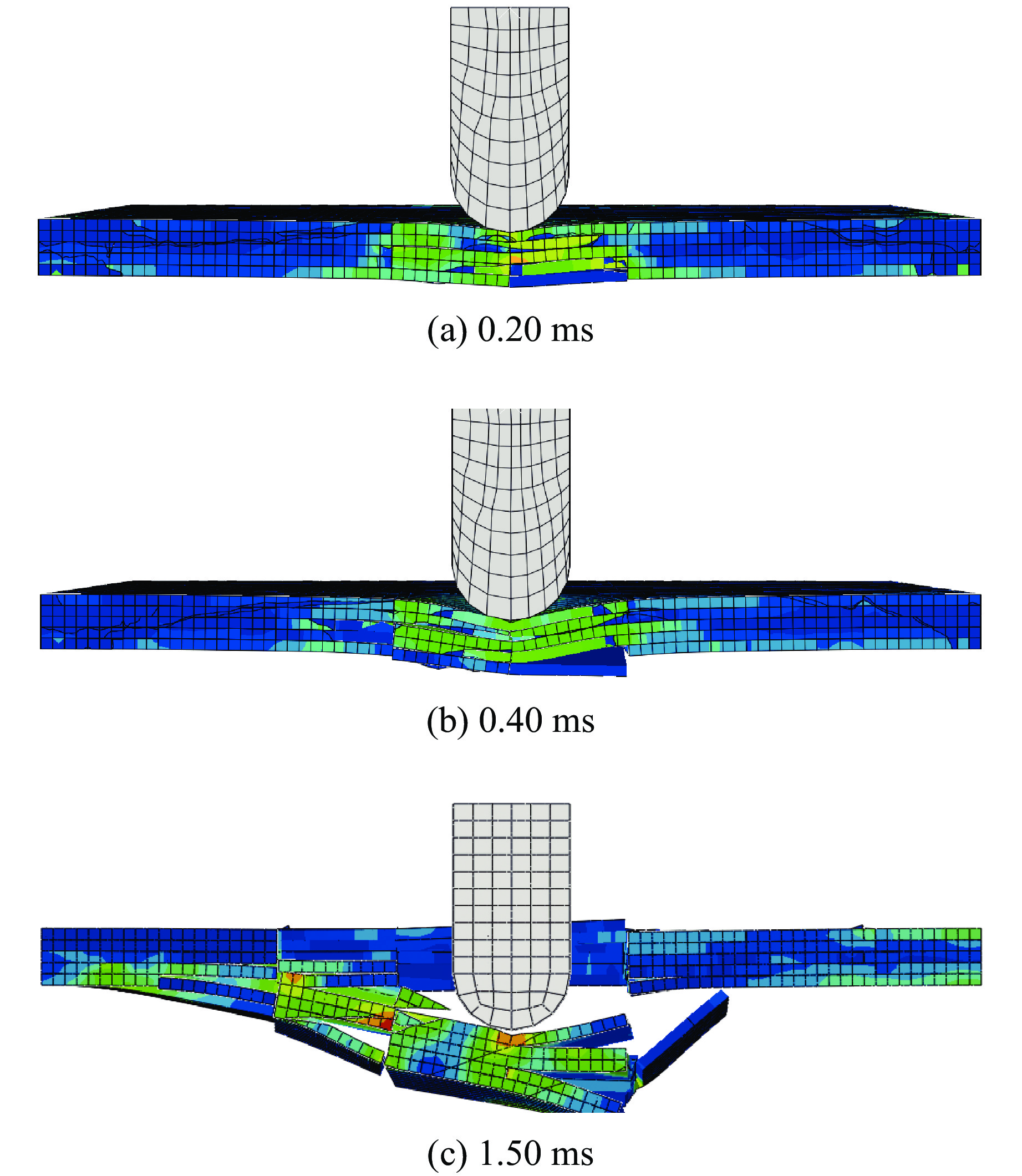
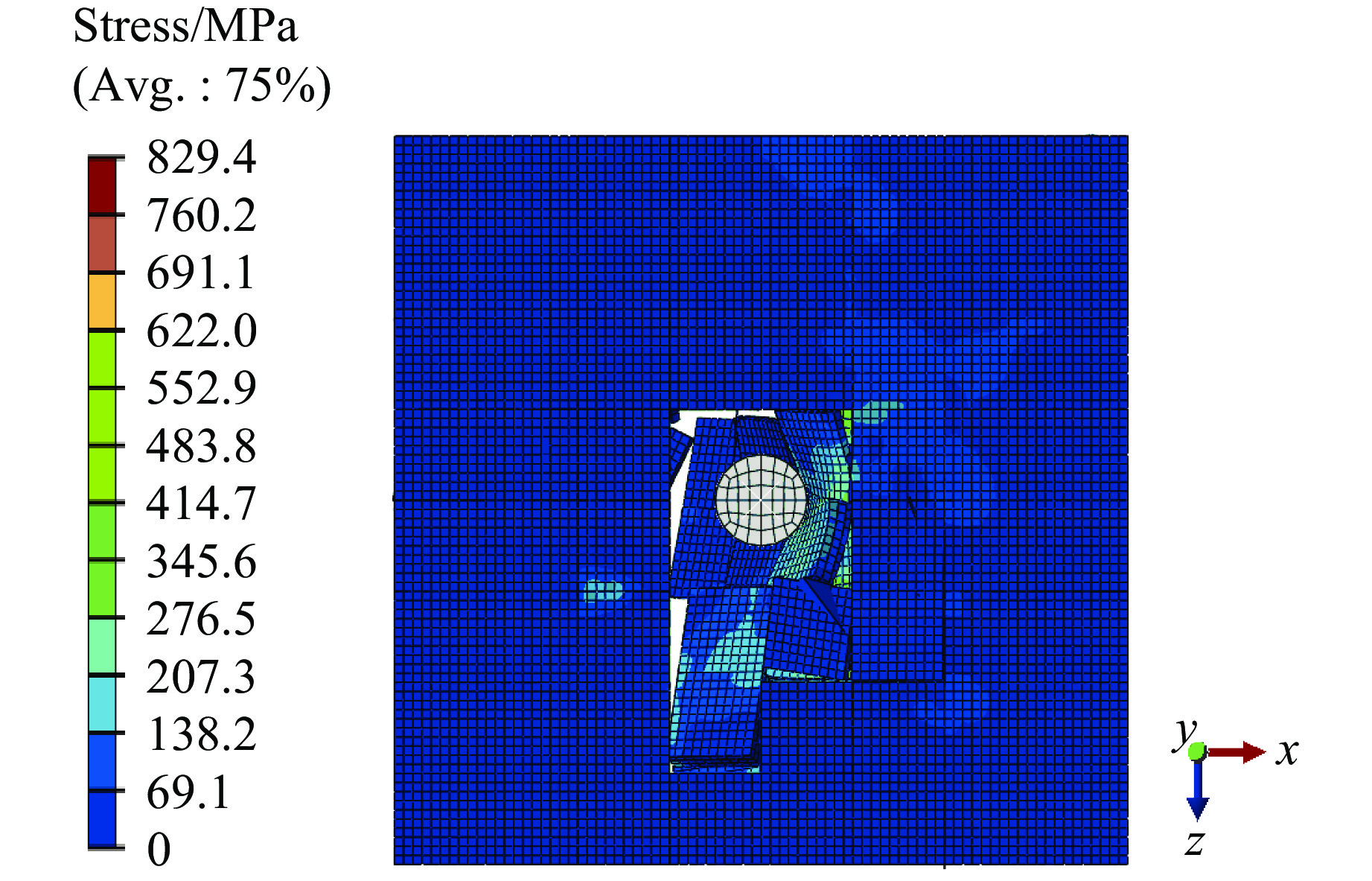
图4和图5分别为不同时刻规则片板模型与Voronoi片板模型受弹丸冲击时的应力云图剖视图。通过对比可以发现:在规则模型中,应力主要集中在弹丸冲击点及附近区域,远离冲击点区域的应力很小;在Voronoi模型中,应力分布区域更大,受力更加均匀。规则模型受冲击后很快就被冲破;而Voronoi模型的冲击模拟结果显示,其最大应力载荷小于规则模型,最终弹丸并未完全贯穿模型。
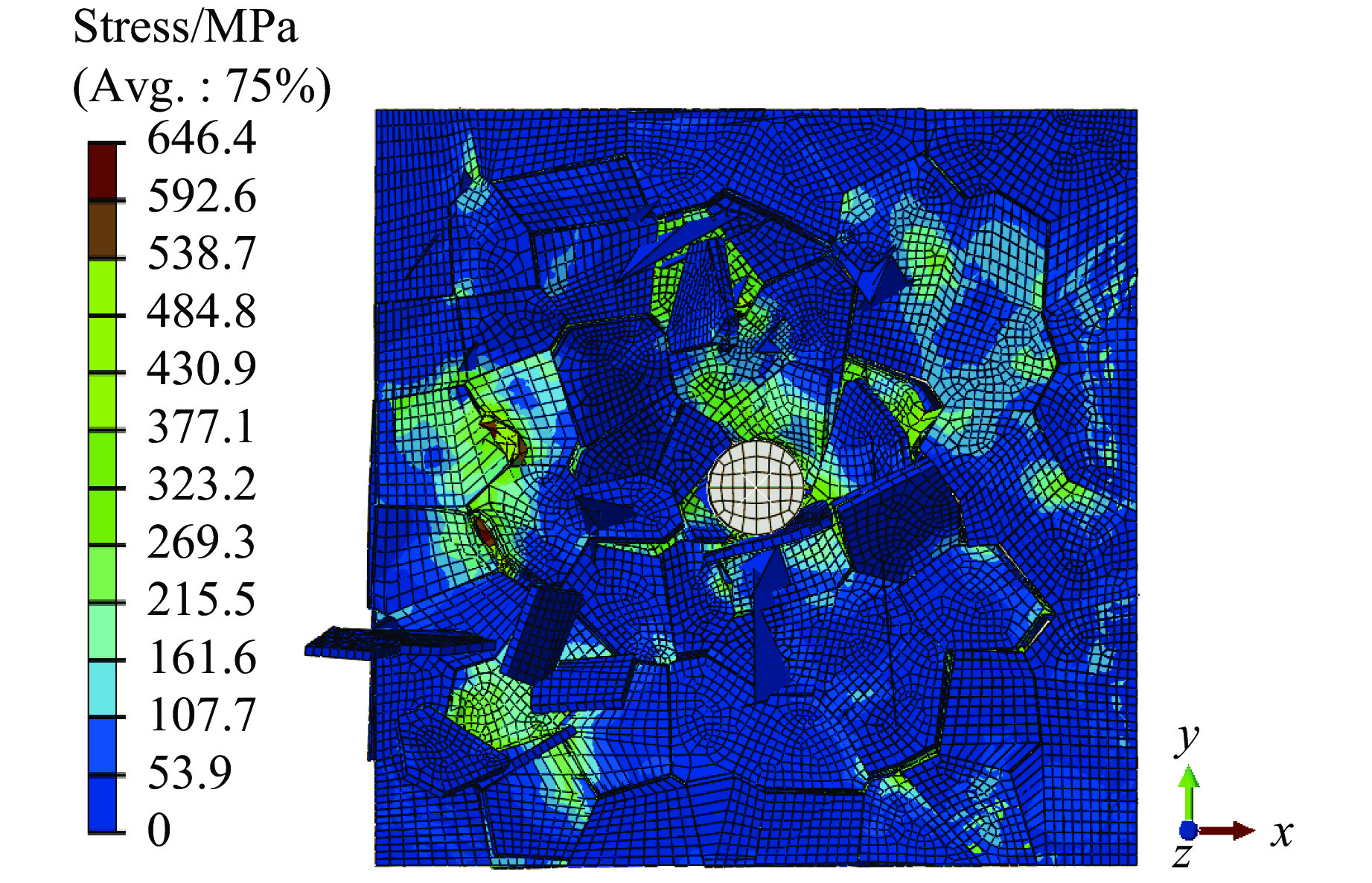
图6和图7分别为规则片板模型和Voronoi片板模型受弹丸冲击3.00 ms时应力云图的俯视图。从受弹丸冲击破坏情况来看:规则模型中脱黏现象主要集中在弹丸冲击点附近区域;而Voronoi模型的冲击影响区域更大,基本遍布整个模型。对于Voronoi模型,冲击区域发生变形时,其余片板受挤压后也发生了脱黏现象,吸收更多的冲击能量,从而有利于冲击能量的扩散与吸收,使模型的更多部分承担冲击负载,即增加承载区域,减小应力集中,更好地发挥能量共享机制。因此Voronoi片板模型抵抗冲击荷载的能力明显优于规则片板模型。
3.2 黏结层厚度和分块尺寸对冲击吸能的影响
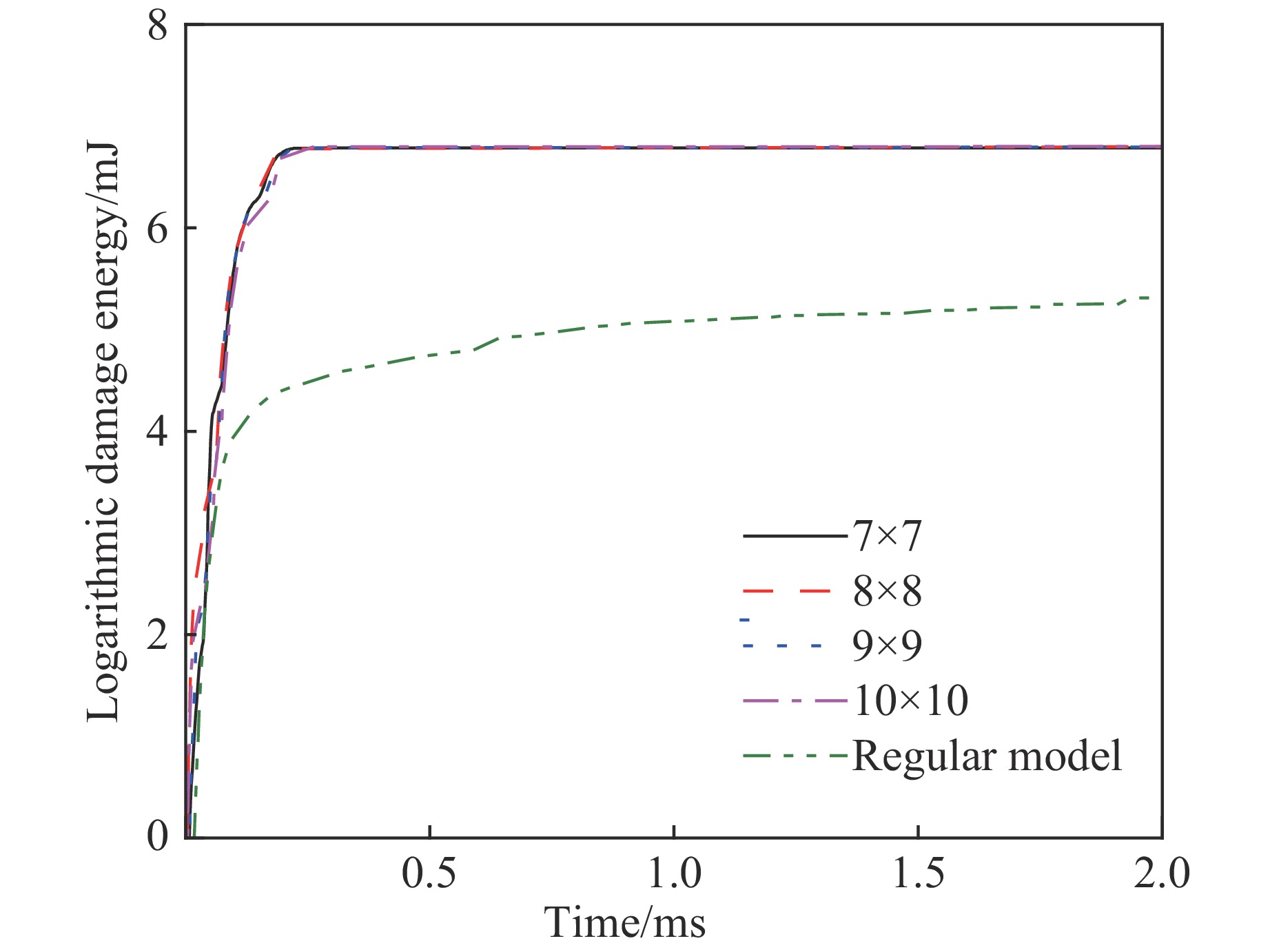
图8和图9分别给出了规则模型和不同分块Voronoi模型的损伤耗散能和塑性耗散能对比。在模型总尺寸相同的情况下,Voronoi模型的损伤耗散能远远高于规则片板模型,而塑性耗散能则小于规则片板模型,说明在冲击载荷作用下Voronoi模型抵抗冲击的能力优于规则片板模型。不同分块Voronoi模型的损伤耗散能和塑性耗散能差别不大,分块尺寸对Voronoi模型抗冲击性能的影响很小。
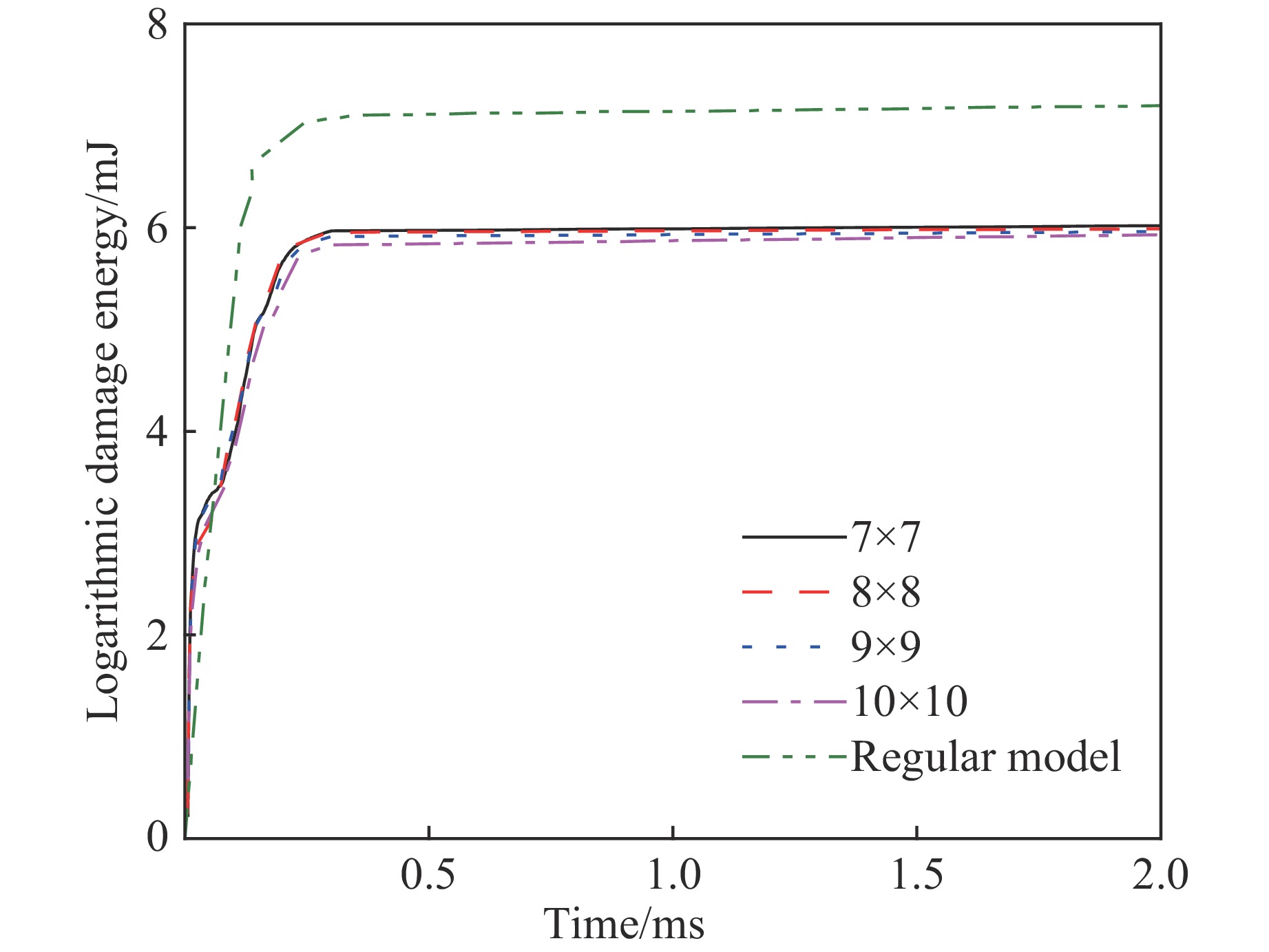
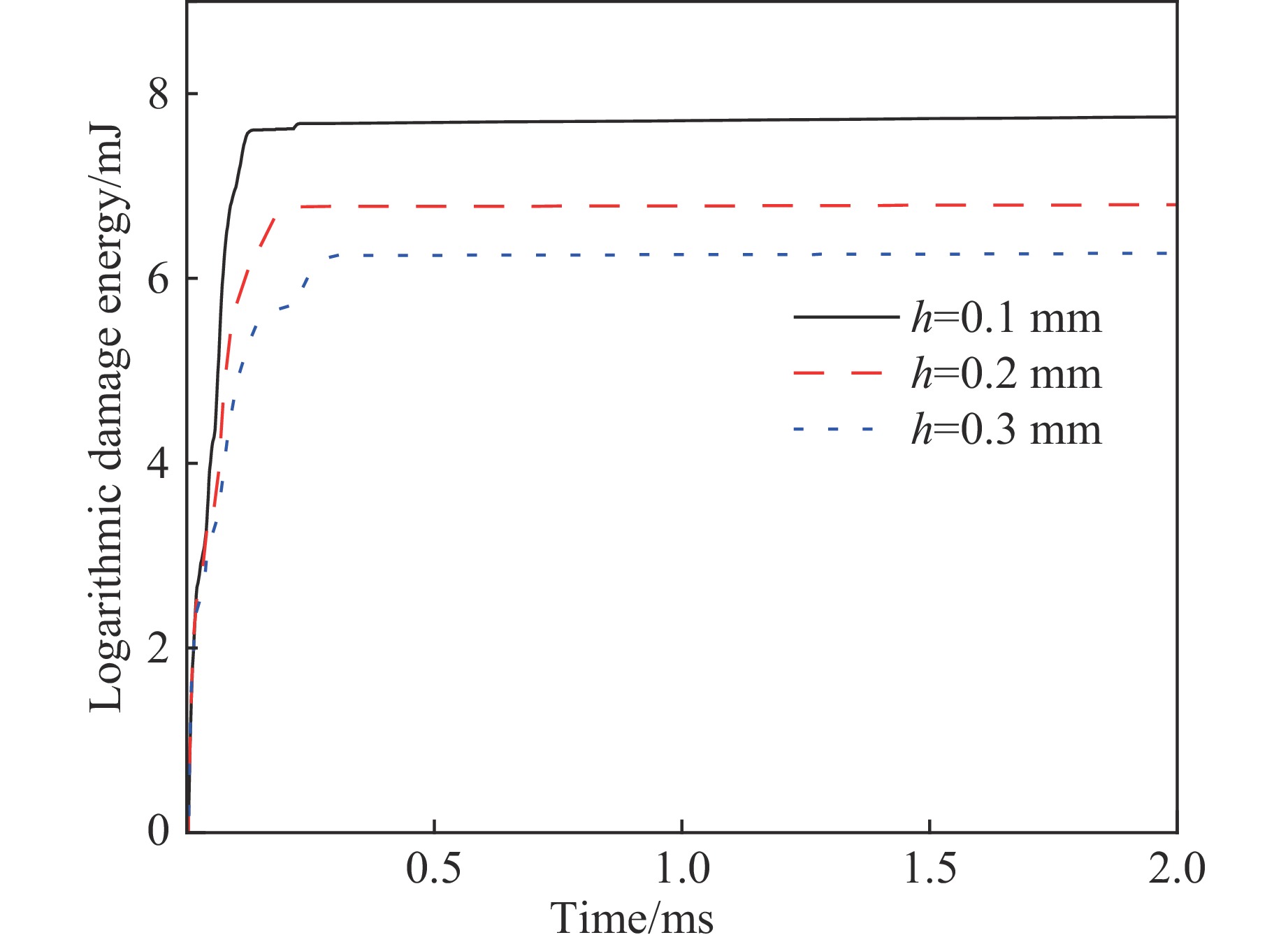
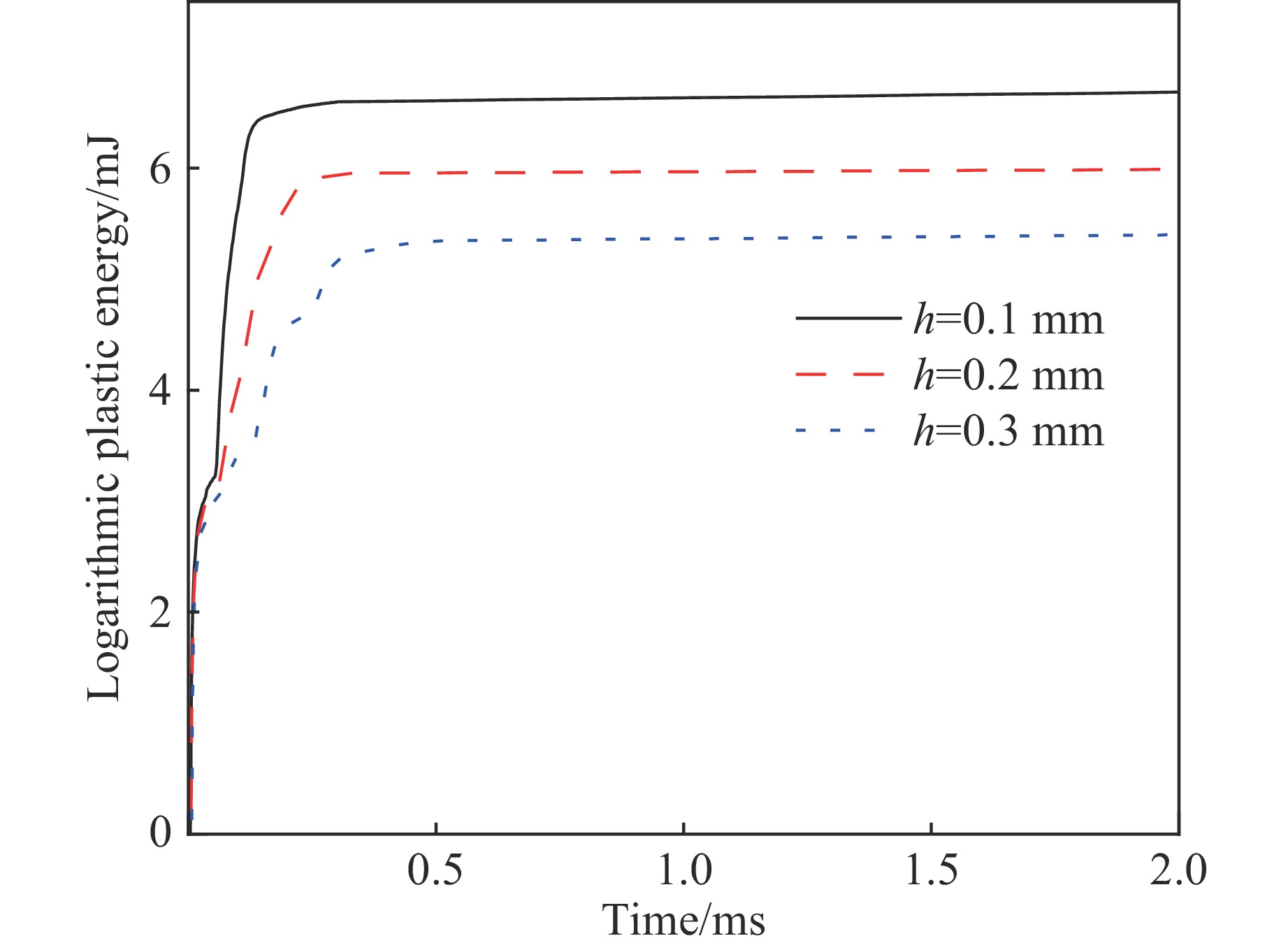
图10和图11分别给出了不同黏结层厚度(h)的Voronoi模型的损伤耗散能和塑性耗散能。可以看出,黏结层对损伤耗散能和塑性耗散能的影响很明显。黏结层越薄,模型整体吸能越大,越薄的黏结层使模型具有更高的抗弯刚度,抗冲击性能越强。由此可见,Voronoi模型的不规则性是仿贝壳珍珠层复合结构模型抗冲击性能的影响因素,对贝壳结构韧性的提升发挥着重要作用。
4. 结 论
通过有限元数值模拟研究了仿贝壳珍珠层Voronoi模型在弹丸冲击载荷下的动态力学响应,得到如下主要结论。
(1)从冲击破坏受损情况来看,不规则Voronoi模型的冲击影响区域比规则模型更大,基本遍布整个模型。对于Voronoi模型,当冲击区域发生变形时,其余片板受挤压后发生脱黏现象,从而吸收更多的冲击能量,有利于冲击能量的扩散与吸收,让模型的更多部分承担冲击负载,即增加承载区域,并且减小应力集中,更好地发挥共享机制。规则模型的脱黏现象主要集中在弹丸冲击点及其附近区域。
(2)在模型总尺寸相同的情况下,Voronoi片板模型的损伤耗散能远远高于规则片板模型,而塑性耗散能则小于规则模型。在冲击载荷作用下,不规则Voronoi片板模型抵抗冲击的能力优于规则片板模型。
(3)分块尺寸对Voronoi模型抗冲击性能的影响很小,而黏结层对损伤耗散能和塑性耗散能的影响很明显,黏结层越薄,模型整体吸能越大,抗冲击性能越强。
鉴于目前制备具有Voronoi结构的金属/高分子材料复合实验模型具有一定困难,因此未对模拟结果进行实验验证。随着3D打印技术的进一步发展,可采用金属/高分子材料混合3D打印技术制备仿贝壳珍珠层Voronoi实验模型,届时即可对铝/乙烯基复合三维Voronoi模型的数值模拟工作进行验证,进而开展更深入的研究。
-
图 1 GaTa4Se8的晶体结构(a)与其立方结构在分别考虑自旋轨道耦合(SOC)效应以及John-Teller效应下的能级分裂示意图(b)(从图1(a)可以看出,Ta4Se4和GaSe4形成交替排列结构。图1(b)中间是立方结构Ta4Se4配位场的能级分裂示意图;图1(b)左边是立方结构的Ta4Se4团簇考虑自旋轨道耦合效应的能级图,其中t2分裂为四重简并的Jeff=3/2轨道以及二重简并的Jeff=1/2轨道,电子占据了更低的Jeff=3/2轨道;图1(b)右边是三方结构的Ta4Se4团簇在John-Teller效应下的能级图,这里t2分裂为二重简并的反键轨道
a∗1 以及四重简并的反键轨道e*,电子占据了更低的a∗1 轨道)Figure 1. (a) Crystal structure of GaTa4Se8 forming a rock-salt type alternating arrangement; (b) schematic diagram of the splitting of the energy level of cubic symmetric structure of GaTa4Se8 (Fig. 1(a) shows the alternating arrangement of clusters Ta4Se4 and GaSe4. Fig.1(b) middle: energy level split diagram of cubic Ta4Se4 ligand field; Fig. 1(b) left: energy level diagram of the cubic structure of the Ta4Se4 cluster considering the spin-orbit coupling effect, t2 splits into the quadruple-degenerated Jeff=3/2 orbital and the dual-degenerated Jeff=1/2 orbital, with the electrons occupying the lower Jeff=3/2 orbital; Fig. 1(b) right: energy level diagram of the trigonal structure of the Ta4Se4 cluster under the John-Teller effect, with t2 splitting into the dual-degenerated anti-bonding orbital
a∗1 and the quadruple-degenerated anti-bonding orbital e*, with the electron occupying the lowera∗1 orbital.)图 2 GaTa4Se8在7 K低温下的高压Raman谱(a)以及Raman特征频率随压力的变化(b)(图2(a)中峰Ⅰ随着压力增大出现软化,预示着其低温下基态结构极不稳定;峰Ⅱ强度的逐渐减弱以及消失表明其结构对称性转变。图2 (b)中峰Ⅰ随着压力增加逐渐软化,而峰Ⅱ随着压力增加出现常规的硬化并在13.1 GPa消失)
Figure 2. (a) High-pressure Raman spectra of GaTa4Se8 at low temperature (7 K); (b) Raman characteristic frequency of GaTa4Se8 varies with the pressures (In Fig.2(a) peak Ⅰ softens when the pressure increases, which predicts its ground state structure mechanically unstable at low temperature; the intensity of peak Ⅱ gradually weakens and disappears, that indicates the change in the structural symmetry. In Fig. 2(b) peak Ⅰ gradually softens with the increasing pressures, while peak Ⅱ shows the regular hardening and the disappearance after 13.1 GPa. )
图 3 300/7 K下压力从 0.8/5.0 GPa到60.7/68.4 GPa范围内GTS的电阻测量结果(当温度从室温降到7 K时,压力增加了5~7 GPa,图中显示了GTS在22.0/29.6 GPa压力下GTS经历的绝缘体到金属的相变)
Figure 3. Resistance results of GTS at the pressures ranging from 0.8/5.0 GPa to 60.7/68.4 GPa under 300/7 K (When the temperature decreases from room temperature to 7 K, the pressure increases 5−7 GPa, these figures demonstrate GTS experiences an insulator to metal transition around 22.0/29.6 GPa.)
图 4 GaTa4Se8的三方结构(R3m)(a)和四方结构(
Fˉ421m )(b)在不考虑电子关联能U的情况下计算得到的高压下的电子态密度结果;(c) 电阻实验拟合的能隙(黑线),三方结构(R3m)理论计算能隙归一化后的宽度(蓝线)以及三方结构(R3m)Fermi面附近的能带宽度(红线)随压力的变化;(d)电阻实验拟合的能隙(黑线)和四方结构(Fˉ421m )理论计算能隙(蓝线)归一化后的宽度随压力的变化Figure 4. (a) Calculated results of the electronic density of states under high pressure for the GaTa4Se8 trigonal structure (R3m) (a) and the tetragonal structure (
Fˉ421m ) (b) without considering the electron correlation energy U; (c) the energy gaps fitted by the resistance experiment (black line), the theoretically calculated energy gaps after normalization (blue line) and the energy bandwidths (red line) near the Fermi surface at different pressures for the trigonal structure (R3m); (d) the comparative results of energy gap fitted by the resistance experiments (black line) and the theoretical calculation results of the normalized energy gap (blue line) of the tetragonal structure (Fˉ421m ) at different pressures图 5 (a) 三方结构(R3m)GaTa4Se8的高压Raman光谱计算结果(随着压力的增加,85 cm−1附近的Raman峰I随着a的减小(压力增加)逐渐软化,(b)计算得到的三方结构R3m GaTa4Se8的能带展宽与计算的Raman峰偏移量的线性关系,(c)计算得到的三方结构R3m GaTa4Se8的能带展宽与实验测得的Raman峰偏移量的线性关系
Figure 5. (a) Calculated high-pressure Raman spectra of trigonal structure (R3m) of GaTa4Se8 (The Raman peak calculated near 85 cm−1 gradually softens with the parameter a decreasing (pressure increasing).); (b) the bandwidth broadening of GaTa4Se8 calculated for the R3m structure shows a linear relationship with the calculated Raman shift; (c) the bandwidth broadening of GaTa4Se8 calculated for the R3m structure exhibits a linear relation with the experimentally measured Raman shift
-
[1] ABD-ELMEGUID M M, NI B, KHOMSKII D I, et al. Transition from Mott insulator to superconductor in GaNb4Se8 and GaTa4Se8 under high pressure [J]. Physical Review Letters, 2004, 93(12): 126403. doi: 10.1103/PhysRevLett.93.126403 [2] POCHA R, JOHRENDT D, NI B F, et al. Crystal structures, electronic properties, and pressure-induced superconductivity of the tetrahedral cluster compounds GaNb4S8, GaNb4Se8, and GaTa4Se8 [J]. Journal of the American Chemical Society, 2005, 127(24): 8732–8740. doi: 10.1021/ja050243x [3] VAJU C, CARIO L, CORRAZE B, et al. Electric-pulse-driven electronic phase separation, insulator-metal transition, and possible superconductivity in a Mott insulator [J]. Advanced Materials, 2008, 20(14): 2760–2765. doi: 10.1002/adma.200702967 [4] GUIOT V, JANOD E, CORRAZE B, et al. Control of the electronic properties and resistive switching in the new series of Mott insulators GaTa4Se8–yTey (0 ≤y≤ 6.5) [J]. Chemistry of Materials, 2011, 23(10): 2611–2618. doi: 10.1021/cm200266n [5] CAMJAYI A, WEHT R, ROZENBERG M J. Localised wannier orbital basis for the Mott insulators GaV4S8 and GaTa4Se8 [J]. Europhysics Letters, 2012, 100(5): 57004. doi: 10.1209/0295-5075/100/57004 [6] GUIOT V, CARIO L, JANOD E, et al. Avalanche breakdown in GaTa4Se8-xTex narrow-gap Mott insulators [J]. Nature Communications, 2013, 4(1): 1722. doi: 10.1038/ncomms2735 [7] TA PHUOC V, VAJU C, CORRAZE B, et al. Optical conductivity measurements of GaTa4Se8 under high pressure: evidence of a bandwidth-controlled insulator-to-metal Mott transition [J]. Physical Review Letters, 2013, 110(3): 037401. doi: 10.1103/PhysRevLett.110.037401 [8] DUBOST V, CREN T, VAJU C, et al. Resistive switching at the nanoscale in the Mott insulator compound GaTa4Se8 [J]. Nano Letters, 2013, 13(8): 3648–3653. doi: 10.1021/nl401510p [9] CAMJAYI A, ACHA C, WEHT R, et al. First-order insulator-to-metal Mott transition in the paramagnetic 3D system GaTa4Se8 [J]. Physical Review Letters, 2014, 113(8): 086404. doi: 10.1103/PhysRevLett.113.086404 [10] JEONG M Y, CHANG S H, KIM B H, et al. Direct experimental observation of the molecular Jeff = 3/2 ground state in the lacunar spinel GaTa4Se8 [J]. Nature Communications, 2017, 8(1): 782. doi: 10.1038/s41467-017-00841-9 [11] PARK M J, SIM G, JEONG M Y, et al. Pressure-induced topological superconductivity in the spin-orbit Mott insulator GaTa4Se8 [J]. NPJ Quantum Materials, 2020, 5(1): 41. doi: 10.1038/s41535-019-0206-8 [12] JEONG M Y, CHANG S H, LEE H J, et al. Jeff = 3/2 metallic phase and unconventional superconductivity in GaTa4Se8 [J]. Physical Review B, 2021, 103(8): L081112. doi: 10.1103/PhysRevB.103.L081112 [13] INOUE I H, ROZENBERG M J. Taming the Mott transition for a novel Mott transistor [J]. Advanced Functional Materials, 2008, 18(16): 2289–2292. doi: 10.1002/adfm.200800558 [14] CARIO L, VAJU C, CORRAZE B, et al. Electric-field-induced resistive switching in a family of Mott insulators: towards a new class of RRAM memories [J]. Advanced Materials, 2010, 22(45): 5193–5197. doi: 10.1002/adma.201002521 [15] STOLIAR P, CARIO L, JANOD E, et al. Universal electric-field-driven resistive transition in narrow-gap Mott insulators [J]. Advanced Materials, 2013, 25(23): 3222–3226. doi: 10.1002/adma.201301113 [16] DUBOST V, CREN T, VAJU C, et al. Electric-field-assisted nanostructuring of a Mott insulator [J]. Advanced Functional Materials, 2009, 19(17): 2800–2804. doi: 10.1002/adfm.200900208 [17] KIM H S, IM J, HAN M J, et al. Spin-orbital entangled molecular Jeff states in lacunar spinel compounds [J]. Nature Communications, 2014, 5(1): 3988. doi: 10.1038/ncomms4988 [18] GEIRHOS K, RESCHKE S, GHARA S, et al. Optical, dielectric, and magnetoelectric properties of ferroelectric and antiferroelectric lacunar spinels [J]. Physica Status Solidi B, 2021: 2100260. [19] POCHA R, JOHRENDT D, PÖTTGEN R. Electronic and structural instabilities in GaV4S8 and GaMo4S8 [J]. Chemistry of Materials, 2000, 12(10): 2882–2887. doi: 10.1021/cm001099b [20] ZHANG S, ZHANG T T, DENG H S, et al. Crystal and electronic structure of GaTa4Se8 from first-principles calculations [J]. Physical Review B, 2020, 102(21): 214114. doi: 10.1103/PhysRevB.102.214114 [21] CHEN X J. Exploring high-temperature superconductivity in hard matter close to structural instability [J]. Matter and Radiation at Extremes, 2020, 5(6): 068102. doi: 10.1063/5.0033143 [22] SHEN G Y, MAO H K. High-pressure studies with X-rays using diamond anvil cells [J]. Reports on Progress in Physics, 2017, 80(1): 016101. doi: 10.1088/1361-6633/80/1/016101 [23] CHEN X H, LOU H B, ZENG Z D, et al. Structural transitions of 4∶1 methanol-ethanol mixture and silicone oil under high pressure [J]. Matter and Radiation at Extremes, 2021, 6(3): 038402. doi: 10.1063/5.0044893 [24] PRESCHER C, PRAKAPENKA V B. DIOPTAS: a program for reduction of two-dimensional X-ray diffraction data and data exploration [J]. High Pressure Research, 2015, 35(3): 223–230. doi: 10.1080/08957959.2015.1059835 [25] ALTOMARE A, CORRIERO N, CUOCCI C, et al. EXPO software for solving crystal structures by powder diffraction data: methods and application [J]. Crystal Research and Technology, 2015, 50(910): 737–742. doi: 10.1002/crat.201500024 [26] DENG H S, ZHANG J B, JEONG M Y, et al. Metallization of quantum material GaTa4Se8 at high pressure [J]. Journal of Physical Chemistry Letters, 2021, 12(23): 5601–5607. doi: 10.1021/acs.jpclett.1c01069 [27] JAYARAMAN A. Diamond anvil cell and high-pressure physical investigations [J]. Reviews of Modern Physics, 1983, 55(1): 65–108. doi: 10.1103/RevModPhys.55.65 [28] HLINKA J, BORODAVKA F, RAFALOVSKYI I, et al. Lattice modes and the Jahn-Teller ferroelectric transition of GaV4S8 [J]. Physcal Review B, 2016, 94(6): 060104. doi: 10.1103/PhysRevB.94.060104 [29] IMADA M, FUJIMORI A, TOKURA Y. Metal-insulator transitions [J]. Reviews of Modern Physics, 1998, 70(4): 1039–1263. doi: 10.1103/RevModPhys.70.1039 [30] WEBER W H, MERLIN R. Raman scattering in materials science [M]. Berlin: Springer Science and Business Media, 2013. -













 下载:
下载:
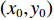
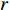
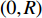
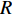
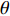
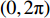

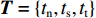
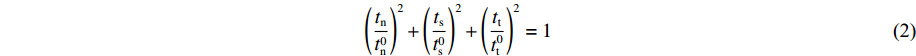
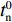
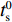
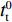
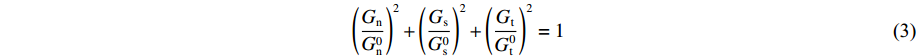
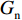
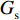
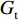
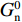
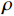

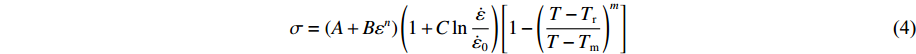
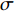
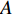
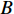

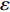
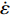
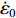

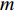
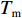
















 下载:
下载: