马氏体相变的相场模型研究进展

于继东; 姚松林; 吴强

doi:10.11858/gywlxb.20210772

马氏体相变的相场模型研究进展

doi: 10.11858/gywlxb.20210772

中国工程物理研究院流体物理研究所，四川绵阳　621999

基金项目: 冲击波物理与爆轰物理重点实验室基金（6142A03191001）；国家自然科学基金（11602249）

详细信息

作者简介:
于继东（1981－），男，博士，副研究员，主要从事高压物理力学研究. E-mail：yujidong@caep.cn

中图分类号: O521.2
计量
- 文章访问数: 4334
- HTML全文浏览量: 1395
- PDF下载量: 78
出版历程
- 收稿日期: 2021-04-13
- 修回日期: 2021-05-21

Advances of Phase Field Modeling of Martensitic Phase Transformation

Institute of Fluid Physics, CAEP, Mianyang 621999, Sichuan, China

摘要

摘要: 马氏体相变是一种无扩散位移型一阶相变，会形成针状、表面浮凸等复杂的特征微结构。马氏体相变的特征微结构会显著影响材料的宏观物理力学特性，相关研究具有重要的科学和工程价值。相场模型具有可处理复杂界面演化问题的独特优势，已成为模拟马氏体相变的有力的理论与计算工具。概述了马氏体相变相场模型的研究进展，并分析了相场模型应用于弱马氏体相变和重构型马氏体相变的特点。
- 马氏体相变 /
- 相场模型 /
- 相变路径 /
- 晶格对称性
Abstract: Martensitic transformation is a diffusionless, displacive and first-order phase transformation, which produces complex microstructures such as needle-like structure and surface tilting. Due to these microstructures having significantly influence on the macroscopic physical and mechanical properties, related studies have important scientific and engineering values. Phase field modeling has become a powerful theoretical and computational tool for simulating martensitic transformation because of its unique advantages to describe the complex interface evolution. In this study, the advances of phase field modeling of martensitic phase transformation was summarized, and the characteristics of the phase field modeling applied to the weak and reconstructive martensitic transformation were analyzed.
- martensitic phase transformation /
- phase field modeling /
- phase transition pathway /
- crystal symmetry

HTML全文

功能梯度材料(Functionally Graded Materials, FGM)的概念是1984年在航空飞机计划中首次提出的^[1]，FGM的特性在于它的组成和结构随着体积的变化而变化，从而导致材料相应性质发生改变。因其材料特性呈幂律分布^[2-3]，FGM被广泛应用于工程领域，如航空航天、机械工程、生物医学等。圆柱壳在联合荷载作用下的屈曲分析备受学术界关注^[4-5]。目前，对FGM板壳的研究较为深入^[6-8]。Beni等^[9]利用改进的偶应力理论，对FGM圆柱壳在不同边界条件下的动力屈曲进行了分析；Kargarnovin等^[10]研究了轴向荷载作用下FGM圆柱壳的动力屈曲；Sofiyev等^[11]研究了横向压力下功能梯度正交各向异性圆柱壳的动力屈曲，推导出基于一阶剪切变形理论的功能梯度正交各向异性圆柱壳的稳定性和相容性方程；Khazaeinejad等^[12]研究了弹性模量在厚度方向上连续变化的FGM圆柱壳在复合外压和轴向压缩载荷作用下的动力屈曲；Khalili等^[13]研究了横向冲击载荷作用下FGM圆柱壳的动力屈曲；Alashti等^[14]对变厚度FGM圆柱壳外压和轴向压缩的动力屈曲问题进行了分析。

基于以上研究，本研究讨论了FGM圆柱壳在轴向荷载作用下的动力屈曲。根据Donnell壳体理论和经典板壳理论，利用Hamilton变分原理得到FGM圆柱壳的动力屈曲控制方程；采用分离变量法求得动力屈曲临界荷载表达式；通过MATLAB软件计算动力屈曲临界荷载，讨论由不同材料(陶瓷和钛、陶瓷和铁、陶瓷和铜)组成的FGM圆柱壳的径厚比(R/h)、梯度指数(k)、环向模态数(m)、轴向模态数(n)等对临界荷载的影响。

1. FGM圆柱壳的屈曲控制方程

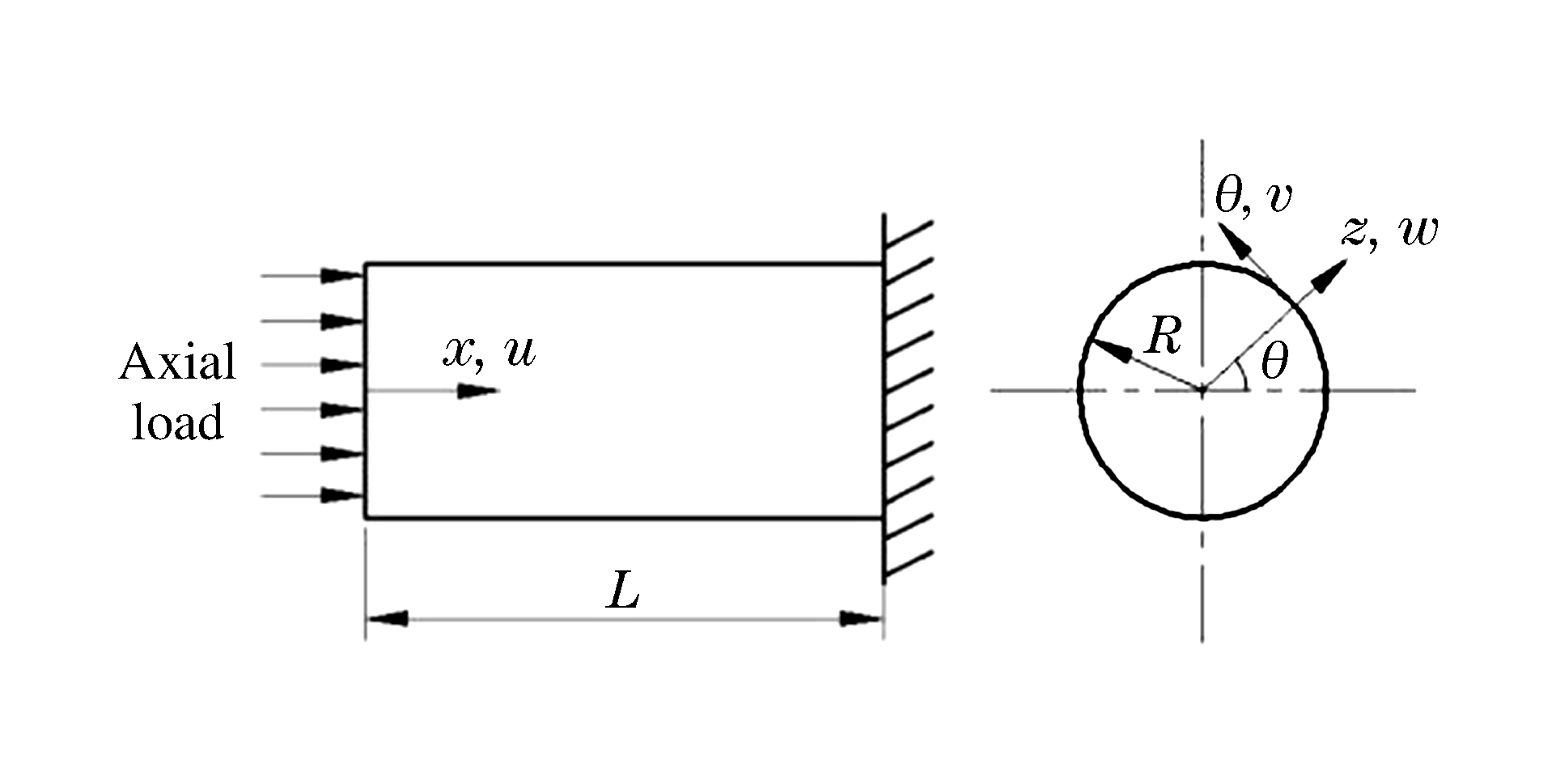
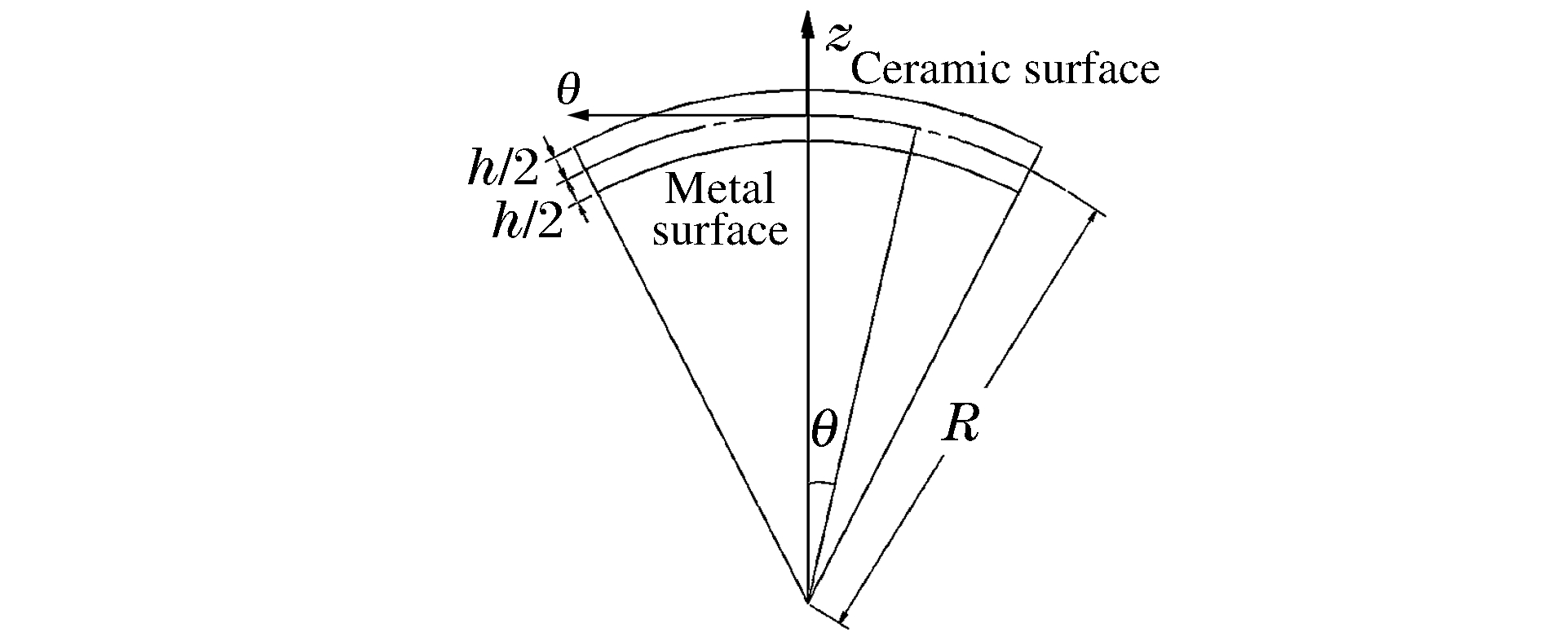
如图 1所示，FGM圆柱壳长度为l，半径为R，总厚度为h，选取柱坐标系(x, θ, z)，其相应位移为(u, v, w)。FGM的材料属性(弹性模量E、密度ρ、泊松比μ等)呈幂律分布^[2-3]，表示为

$P\left( z \right) = \left( {{P_1} - {P_2}} \right){\left( {\frac{{2z + h}}{{2h}}} \right)^k} + {P_2}$

(1)

图 1 圆柱壳坐标系统

Figure 1. Cylindrical shell coordinates

下载: 全尺寸图片幻灯片

式中：P为物性参数，下标“1”和“2”分别代表组分1和组分2；k为梯度指数，k∈(0, ∞)。圆柱壳内任意点的物性参数为

$\left\{ \begin{array}{l} E\left( z \right) = \left( {{E_1} - {E_2}} \right){\left( {\frac{{2z + h}}{{2h}}} \right)^k} + {E_2}\\ \rho \left( z \right) = \left( {{\rho _1} - {\rho _2}} \right){\left( {\frac{{2z + h}}{{2h}}} \right)^k} + {\rho _2}\\ \mu \left( z \right) = \left( {{\mu _1} - {\mu _2}} \right){\left( {\frac{{2z + h}}{{2h}}} \right)^k} + {\mu _2} \end{array} \right.$

(2)

根据Donnell壳体理论，圆柱壳的小挠度几何方程为

$\begin{array}{*{20}{c}} {\left\{ \begin{array}{l} {\varepsilon _x} = \varepsilon _x^0 + z{K_x}\\ {\varepsilon _\theta } = \varepsilon _\theta ^0 + z{K_\theta }\\ {\gamma _{x\theta }} = \gamma _{x\theta }^0 + z{K_{x\theta }} \end{array} \right.,}&{\left\{ \begin{array}{l} u = {u_0} - z\frac{{\partial w}}{{\partial x}}\\ v = {v_0} - z\frac{{\partial w}}{{R\partial \theta }}\\ w = {w_0} \end{array} \right.,}&{\left\{ \begin{array}{l} \varepsilon _x^0 = \frac{{\partial {u_0}}}{{\partial x}}\\ \varepsilon _\theta ^0 = \frac{{\partial {v_0}}}{{R\partial \theta }} - \frac{{{w_0}}}{R}\\ \gamma _{x\theta }^0 = \frac{{\partial {u_0}}}{{R\partial \theta }} - \frac{{\partial {v_0}}}{{\partial x}} \end{array} \right.,}&{\left\{ \begin{array}{l} {K_x} = - \frac{{{\partial ^2}{w_0}}}{{\partial {x^2}}}\\ {K_\theta }{\rm{ = }} - \frac{{{\partial ^2}{w_0}}}{{{R^2}\partial {\theta ^2}}}\\ {K_{x\theta }}{\rm{ = }} - \frac{{2{\partial ^2}{w_0}}}{{R\partial x\partial \theta }} \end{array} \right.} \end{array}$

(3)

式中：ε为正应变，γ为切应变，上、下标“0”表示壳体中面，K为壳体曲率。

根据经典板壳理论，FGM圆柱壳的内力N与内力矩M可表示为

$\left( {\begin{array}{*{20}{c}} {{N_x}}\\ {{N_\theta }}\\ {{N_{x\theta }}}\\ {{M_x}}\\ {{M_\theta }}\\ {{M_{x\theta }}} \end{array}} \right) = \left( {\begin{array}{*{20}{c}} {{A_{11}}}&{{A_{12}}}&{{A_{16}}}&{{B_{11}}}&{{B_{12}}}&{{B_{16}}}\\ {{A_{21}}}&{{A_{22}}}&{{A_{26}}}&{{B_{21}}}&{{B_{22}}}&{{B_{26}}}\\ {{A_{16}}}&{{A_{26}}}&{{A_{66}}}&{{B_{16}}}&{{B_{26}}}&{{B_{66}}}\\ {{B_{11}}}&{{B_{12}}}&{{B_{16}}}&{{D_{11}}}&{{D_{12}}}&{{D_{16}}}\\ {{B_{21}}}&{{B_{22}}}&{{B_{26}}}&{{D_{21}}}&{{D_{22}}}&{{D_{26}}}\\ {{B_{16}}}&{{B_{26}}}&{{B_{66}}}&{{D_{16}}}&{{D_{26}}}&{{D_{66}}} \end{array}} \right)\left( {\begin{array}{*{20}{c}} {\varepsilon _x^0}\\ {\varepsilon _\theta ^0}\\ {\gamma _{x\theta }^0}\\ {{K_x}}\\ {{K_\theta }}\\ {{K_{x\theta }}} \end{array}} \right)$

(4)

式中：A_ij、B_ij、D_ij(i, j=1, 2, 6)分别为FGM圆柱壳的拉伸刚度、耦合刚度和弯曲刚度系数矩阵分量。 ${A_{11}} = {A_{22}} = \int_{ - h/2}^{h/2} {\frac{{E\left( z \right)}}{{1 - {\mu ^2}\left( z \right)}}} {\rm{d}}z$ , ${A_{12}} = {A_{21}} = \int_{ - h/2}^{h/2} {\frac{{\mu \left( z \right)E\left( z \right)}}{{1 - {\mu ^2}\left( z \right)}}} {\rm{d}}z$ , ${A_{66}} = \int_{ - h/2}^{h/2} {\frac{{E\left( z \right)}}{{2\left[ {1 + \mu \left( z \right)} \right]}}} {\rm{d}}z$ , ${B_{11}} = {B_{22}} = \int_{ - h/2}^{h/2} {\frac{{E\left( z \right)}}{{1 - {\mu ^2}\left( z \right)}}z\;}{\rm{d}}z$ , ${B_{12}} = {B_{21}} = \int_{ - h/2}^{h/2} {\frac{{\mu \left( z \right)E\left( z \right)}}{{1 - {\mu ^2}\left( z \right)}}z\;} {\rm{d}}z$ , ${B_{66}} = \int_{ - h/2}^{h/2} {\frac{{E\left( z \right)}}{{2\left[ {1 + \mu \left( z \right)} \right]}}z\;} {\rm{d}}z$ , ${D_{11}} = {D_{22}} = \int_{ - h/2}^{h/2} {\frac{{E\left( z \right)}}{{1 - {\mu ^2}\left( z \right)}}{z^2}\;} {\rm{d}}z$ , ${D_{12}} = {D_{21}} = \int_{ - h/2}^{h/2} {\frac{{\mu \left( z \right)E\left( z \right)}}{{1 - {\mu ^2}\left( z \right)}}{z^2}\;} {\rm{d}}z$ , ${D_{66}} = \int_{ - h/2}^{h/2} {\frac{{E\left( z \right)}}{{2\left[ {1 + \mu \left( z \right)} \right]}}{z^2}\;} {\rm{d}}z$ 。FGM圆柱壳的力学性能为各向同性^[15]，那么：A₁₆=A₂₆=B₁₆=B₂₆=D₁₆=D₂₆=0。

对于圆柱壳，系统的应变能(不考虑剪力)为

$U = \frac{1}{2}\int_0^{2{\rm{ \mathsf{ π} }}} {\int_0^l {\left( {{N_x}\varepsilon _x^0 + {N_\theta }\varepsilon _\theta ^0 + {N_{x\theta }}\gamma _{x\theta }^0 + {M_x}{K_x} + {M_\theta }{K_\theta } + {M_{x\theta }}{K_{x\theta }}} \right)} R{\rm{d}}x{\rm{d}}\theta }$

(5)

动能为

$T = \frac{1}{2}\int_{ - h/2}^{h/2} {\int_0^{2{\rm{ \mathsf{ π} }}} {\int_0^l {\rho \left( z \right)\left[ {{{\left( {\frac{{\partial u}}{{\partial t}}} \right)}^2} + {{\left( {\frac{{\partial v}}{{\partial t}}} \right)}^2} + {{\left( {\frac{{\partial w}}{{\partial t}}} \right)}^2}} \right]R{\rm{d}}x{\rm{d}}\theta {\rm{d}}z} } }$

(6)

外力功为

$W = \frac{1}{2}\int_0^{2{\rm{ \mathsf{ π} }}} {\int_0^l {N\left( t \right){{\left( {\frac{{\partial {w_0}}}{{\partial x}}} \right)}^2}} R{\rm{d}}x{\rm{d}}\theta }$

(7)

Hamilton变分原理为

${\rm{ \mathsf{ δ} }}\int_{{t_2}}^{{t_1}} {\left( {T - U + W} \right){\rm{d}}t} = 0$

(8)

将(3)式~(7)式代入(8)式中，由Donnell壳体理论可知，圆柱壳内力沿环向均匀分布，忽略中面位移^[16]，由u₀、v₀、w₀的变分系数为零，整理得到FGM圆柱壳的动力屈曲控制方程为

$\begin{array}{l} 4{I_0}\frac{{{\partial ^2}{w_0}}}{{\partial t}} - {I_2}\left( {\frac{{{\partial ^4}{w_0}}}{{{R^2}\partial {\theta ^2}\partial {t^2}}} + \frac{{{\partial ^4}{w_0}}}{{\partial {x^2}\partial {t^2}}}} \right) = - 4{A_{22}}\frac{{{w_0}}}{{{R^2}}} - 4\frac{{{B_{12}}}}{{{R^2}}}\frac{{{\partial ^2}{w_0}}}{{\partial {x^2}}} - 4\frac{{{B_{22}}}}{R}\frac{{{\partial ^2}{w_0}}}{{{R^2}\partial {\theta ^2}}} - {D_{11}}\frac{{{\partial ^4}{w_0}}}{{\partial {x^4}}} - \\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;2\left( {{D_{12}} + 2{D_{66}}} \right)\frac{{{\partial ^4}{w_0}}}{{{R^2}\partial {x^2}\partial {\theta ^2}}} - {D_{22}}\frac{{{\partial ^4}{w_0}}}{{{R^4}\partial {\theta ^4}}} + N\left( t \right)\frac{{{\partial ^2}{w_0}}}{{\partial {x^2}}} \end{array}$

(9)

2. 控制方程的求解

设径向位移表示为^[17]

$w = Y\left( x \right)T\left( t \right){{\rm{e}}^{{\rm{i}}m\theta }}$

(10)

将(10)式代入(9)式中，分离变量得

$\left\{ \begin{array}{l} {Y^{\left( 4 \right)}} = {\alpha ^2}Y'' + {\beta ^2}Y = 0\\ \ddot T - \lambda T = 0 \end{array} \right.$

(11)

其中

${\alpha ^2} = \frac{1}{{{D_{11}}}}\left[ {4\frac{{{B_{12}}}}{{{R^2}}} + \frac{2}{{{R^2}}}\left( {{D_{12}} + 2{D_{66}}} \right){m^2} - N\left( t \right) - {I_2}\lambda } \right]$

(12)

${\beta ^2} = \frac{1}{{{D_{11}}}}\left[ {4\frac{{{A_{22}}}}{{{R^2}}} + 4\frac{{{B_{22}}}}{{{R^3}}} + \frac{{{D_{22}}}}{{{R^4}}}{m^4} + 4{I_0}\lambda - \frac{{{I_2}}}{{{R^2}}}{m^2}\lambda } \right]$

(13)

当α⁴＞4β²＞0且λ＞0时，圆柱壳屈曲^[18-20]，其动力屈曲解为

$Y\left( x \right) = {C_1}\sin \left( {{k_1}x} \right) + {C_2}\cos \left( {{k_1}x} \right) + {C_3}\sin \left( {{k_2}x} \right) + {C_4}\cos \left( {{k_2}x} \right)$

(14)

式中：C₁~C₄为系数， ${k_1} = \sqrt {\frac{{{\alpha ^2} - \sqrt {{\alpha ^4} - 4{\beta ^2}} }}{2}} , {k_2} = \sqrt {\frac{{{\alpha ^2} + \sqrt {{\alpha ^4} - 4{\beta ^2}} }}{2}}$ 。

(14)式满足下列两种边界条件：

(1) 对于一端夹支另一端固支的圆柱壳，其边界条件为

$\left\{ \begin{array}{l} Y\left( 0 \right) = Y'\left( 0 \right) = 0\\ Y\left( l \right) = Y'\left( l \right) = 0 \end{array} \right.$

(15)

(2) 对于一端简支另一端固支的圆柱壳，其边界条件为

$\left\{ \begin{array}{l} Y\left( 0 \right) = Y''\left( 0 \right) = 0\\ Y\left( l \right) = Y'\left( l \right) = 0 \end{array} \right.$

(16)

将(14)式代入(15)式中，整理得到如下齐次线性方程组

$\left( {\begin{array}{*{20}{c}} 0&1&0&1\\ {{k_1}}&0&{{k_2}}&0\\ {\sin \left( {{k_1}l} \right)}&{\cos \left( {{k_1}l} \right)}&{\sin \left( {{k_2}l} \right)}&{\cos \left( {{k_2}l} \right)}\\ {{k_1}\cos \left( {{k_1}l} \right)}&{ - {k_1}\sin \left( {{k_1}l} \right)}&{{k_2}\cos \left( {{k_2}l} \right)}&{ - {k_2}\sin \left( {{k_2}l} \right)} \end{array}} \right)\left( {\begin{array}{*{20}{c}} {{C_1}}\\ {{C_2}}\\ {{C_3}}\\ {{C_4}} \end{array}} \right) = 0$

(17)

若要(17)式有非平凡解，其系数行列式必为零，于是

$2{k_1}{k_2} - 2{k_1}{k_2}\cos \left( {{k_1}l} \right)\cos \left( {{k_2}l} \right) - \left( {k_1^2 + k_2^2} \right)\sin \left( {{k_1}l} \right)\sin \left( {{k_2}l} \right) = 0$

(18)

由k₁和k₂可得

$\left\{ \begin{array}{l} k_1^2 + k_2^2 = \left( {n_1^2 + n_2^2} \right){{\rm{ \mathsf{ π} }}^2}/{l^2} = {\alpha ^2}\\ k_1^2k_2^2 = n_1^2n_2^2{{\rm{ \mathsf{ π} }}^4}/{l^4} = {\beta ^2} \end{array} \right.$

(19)

将(19)式代入(12)式和(13)式中，得一端夹支另一端固支时FGM圆柱壳动力屈曲临界荷载N_cr，即

${N_{{\rm{cr}}}} = \frac{{{D_{11}}{{\rm{ \mathsf{ π} }}^2}\left( {n_1^2 + n_2^2} \right)}}{{{l^2}}} - \frac{{{B_{12}}}}{{{R^2}}} + \frac{{2\left( {{D_{12}} + 2{D_{66}}} \right){m^2}}}{{{R^2}}} + \frac{{{h^2}\left( {{D_{11}}n_1^2n_2^2{{\rm{ \mathsf{ π} }}^4}{R^4} - {A_{22}}{l^4}{R^2} + 4{B_{22}}{m^2}{R^2}{l^4} - {D_{22}}{l^4}{m^4}} \right)}}{{{l^4}{R^2}\left( {{h^2}{m^2} - 12{R^2}} \right)}}$

(20)

式中：n₁=n=1, 2, 3, …；m=1, 2, 3, …；n₂=n+2。

同理可得一端简支另一端固支时FGM圆柱壳动力屈曲临界荷载

(21)

此时，n₁=n=1, 2, 3, …；m=1, 2, 3, …；n₂=n+1。

将FGM退化成金属材料，得到金属材料圆柱壳动力屈曲临界荷载

${N_{{\rm{cr}}}} = \frac{{{D_{11}}{{\rm{ \mathsf{ π} }}^2}\left( {n_1^2 + n_2^2} \right)}}{{{l^2}}} + \frac{{2{D_{12}}{m^2}}}{{{R^2}}} + \frac{{{h^2}\left( {{D_{11}}n_1^2n_2^2{{\rm{ \mathsf{ π} }}^4}{R^4} - {A_{22}}{l^4}{R^2} - {D_{22}}{l^4}{m^4}} \right)}}{{{l^4}{R^2}\left( {{h^2}{m^2} - 12{R^2}} \right)}}$

(22)

(22)式与文献[16]中的表达式相同。

根据(10)式，取一端夹支另一端固支时圆柱壳动力屈曲解的表达式^[16]

$w = T\left( t \right)\left[ {\sin \left( {\frac{{{n_1}{\rm{ \mathsf{ π} }}x}}{l}} \right) - \frac{{{n_1}}}{{{n_2}}}\sin \left( {\frac{{{n_2}{\rm{ \mathsf{ π} }}x}}{l}} \right)} \right]\sin \left( {m\theta } \right)$

(23)

将(23)式代入控制方程(9)式中，计算并化简整理得到临界荷载表达式

${N_{{\rm{cr}}}} = \frac{{\left( {n_1^2 + n_2^2} \right){D_{11}}{{\rm{ \mathsf{ π} }}^2}}}{{{l^2}}} + \frac{{2{m^2}\left( {{D_{12}} + 2{D_{66}}} \right)}}{{{R^2}}}$

(24)

(24)式与不考虑转动惯量时用分离变量得到的结果相同，此时n₁=n=1, 2, 3, …; m=1, 2, 3, …; n₂=n+2。同理可得当边界条件为一端简支另一端固支时的临界荷载表达式，与(24)式相同，此时n₁=n=1, 2, 3, …; m=1, 2, 3, …; n₂=n+1。

3. 算例分析

采用MATLAB软件编程，对FGM圆柱壳动力屈曲临界荷载进行计算。讨论由不同材料(陶瓷-钛、陶瓷-铁、陶瓷-铜)组成的FGM圆柱壳(见图 2)的径厚比(R/h)、梯度指数(k)、环向模态数(m)、轴向模态数(n)对临界荷载N_cr的影响。基本材料参数如表 1所示。

图 2 材料沿壁厚分布

Figure 2. Distribution of material along wall thickness

下载: 全尺寸图片幻灯片

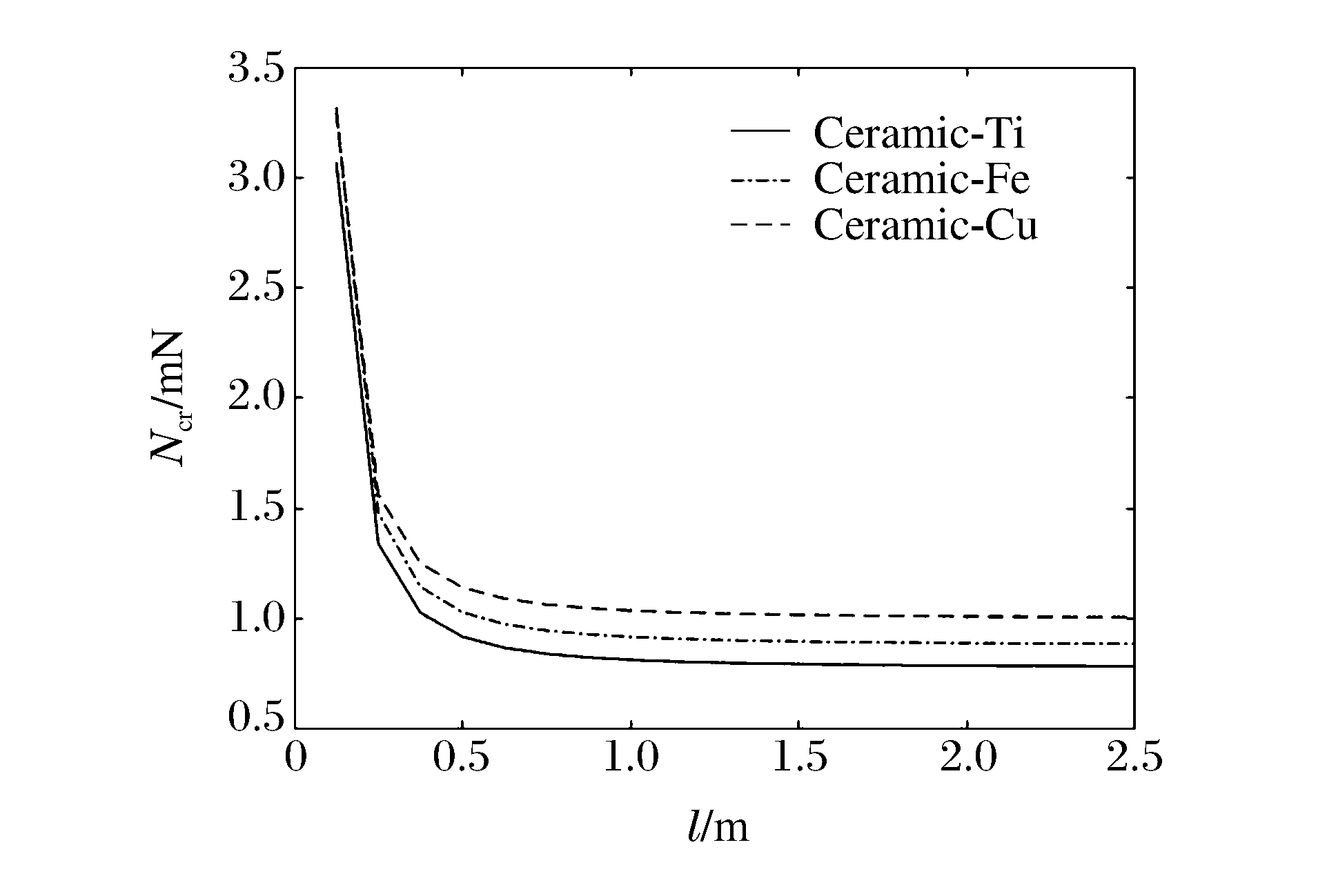
表 1 材料参数

Table 1. Material parameters

Material	E/GPa	ρ/(g·cm^-3)	μ
Ceramic	385	3.96	0.230
Ti	109	4.54	0.410
Fe	155	7.86	0.291
Cu	119	8.96	0.326

下载: 导出CSV

| 显示表格

图 3表示n=1、m=2、k=1、R/h=20时，不同材料组成下N_cr与临界长度l(本研究中临界长度即为圆柱壳长度)的关系曲线。从图 3可以看出：N_cr随l的增加而减小；当l＜0.5 m时，N_cr随l的增加而迅速减小；当l＞0.5 m时，N_cr随l的增大缓慢减小，且逐渐趋于常数；同一l下，陶瓷-铜的N_cr最大，陶瓷-铁次之，陶瓷-钛的N_cr最小。以下均以陶瓷-钛为例进行讨论。

图 3 不同材料组成下临界荷载与临界长度的关系

Figure 3. Critical load vs.critical length under different material composition conditions

下载: 全尺寸图片幻灯片

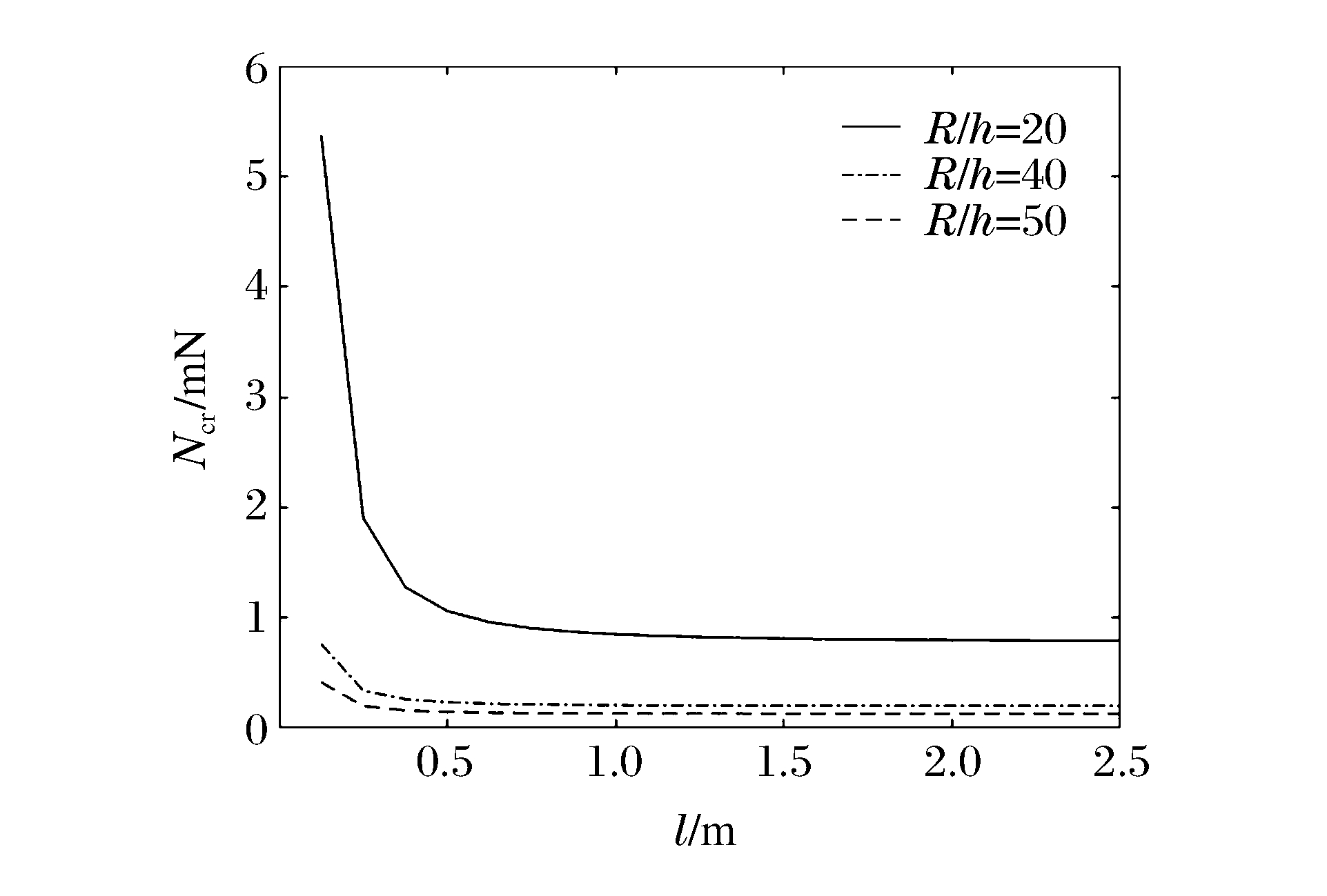
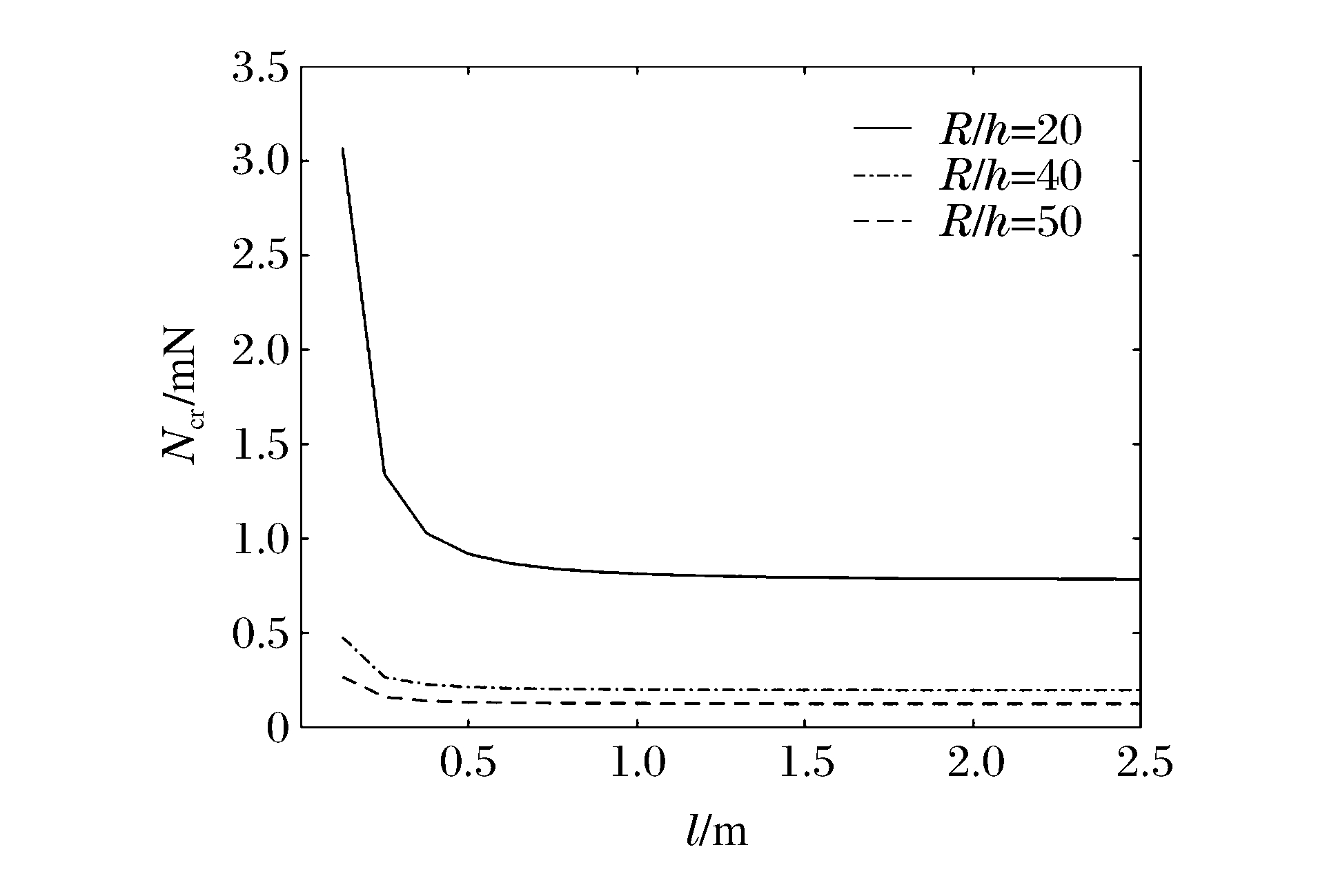
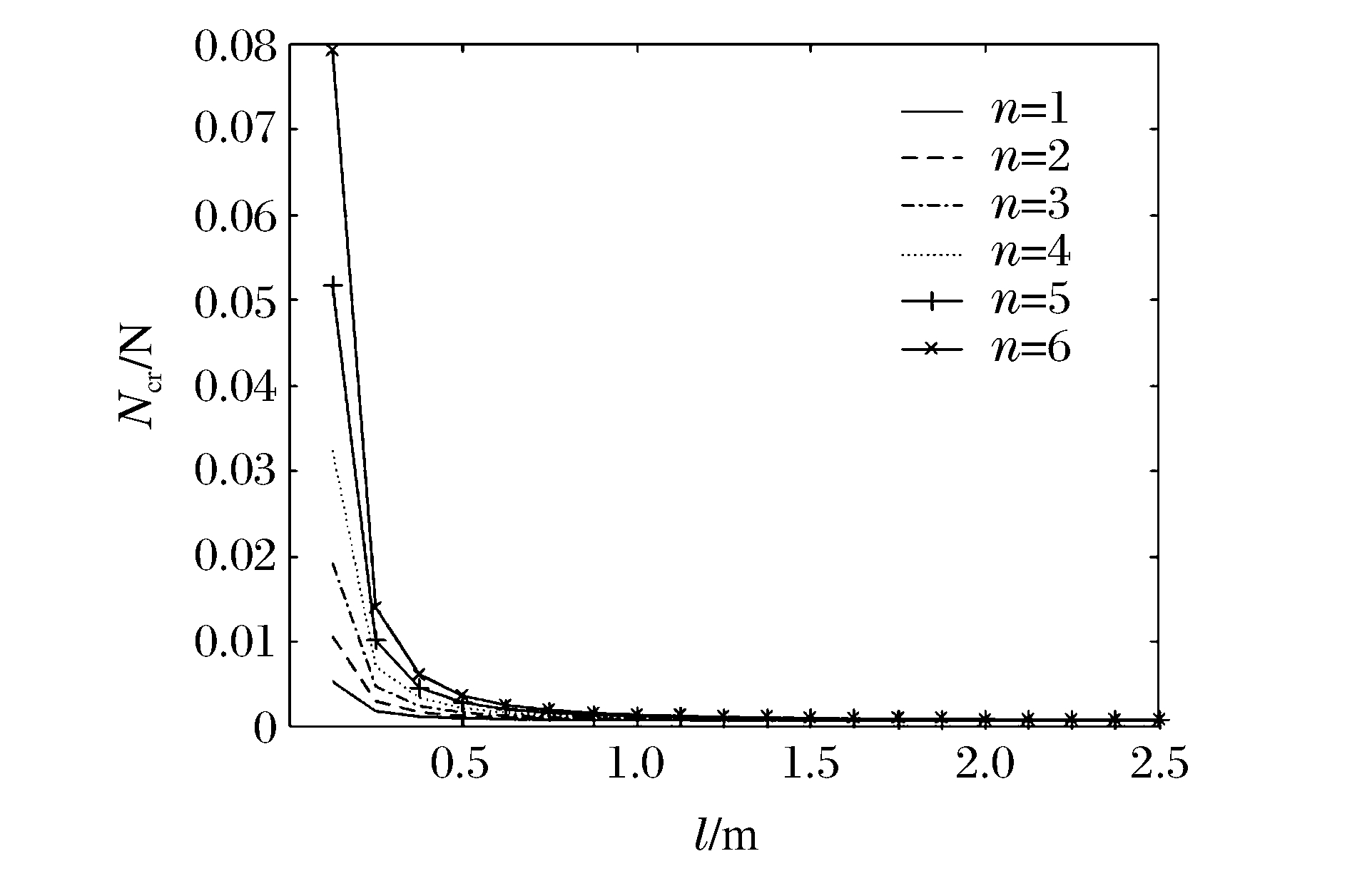
图 4和图 5分别表示冲击端为夹支和简支时n=1、m=2、k=1时不同R/h下N_cr与l的关系曲线。可以看出：当l增加时，N_cr减小，且逐渐趋于常数；在同一l下圆柱壳的N_cr随着R/h的增大而减小；当R/h和l一定时，冲击端为夹支时的N_cr明显比冲击端为简支时的N_cr大，说明约束条件对N_cr有较大影响。

图 4 冲击端为夹支时不同径厚比下临界荷载与临界长度的关系

Figure 4. Critical load vs.critical length under clamped edge and different diameter-thickness ratios conditions

下载: 全尺寸图片幻灯片

图 5 冲击端为简支时不同径厚比下临界荷载与临界长度的关系

Figure 5. Critical load vs.critical length under simple support and different diameter-thickness ratios conditions

下载: 全尺寸图片幻灯片

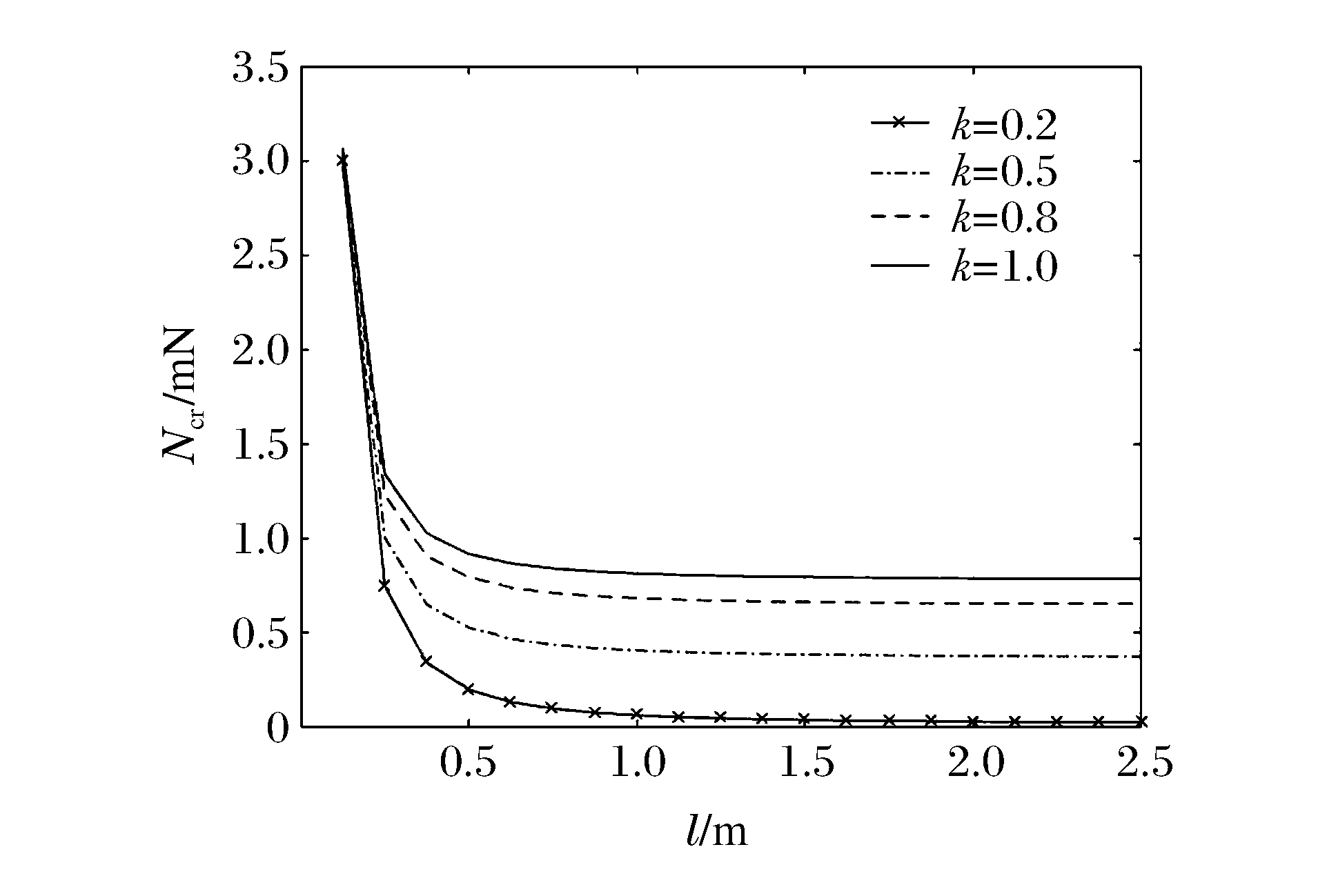
图 6和图 7分别表示冲击端为夹支和简支条件下n=1、m=2、R/h=20时不同k下N_cr与l的关系曲线。可见：FGM圆柱壳的N_cr随着l的增加而减小；在同一l下，FGM圆柱壳的N_cr随着k的增加而增加；当l＜0.5 m时，N_cr随l的增加迅速减小，当l＞0.5 m时，N_cr随l的增加缓慢减小并逐渐趋于常数；当k=1且l一定时，冲击端为夹支时的N_cr明显比冲击端为简支时的N_cr大，再次说明约束条件对N_cr的影响较大。

图 6 冲击端为夹支时不同梯度指数下临界荷载与临界长度的关系

Figure 6. Critical load vs.critical length under clamped edge and different gradient indexes conditions

下载: 全尺寸图片幻灯片

图 7 冲击端为简支时不同梯度指数下临界荷载与临界长度的关系

Figure 7. Critical load vs.critical length under simple support and different gradient indexes conditions

下载: 全尺寸图片幻灯片

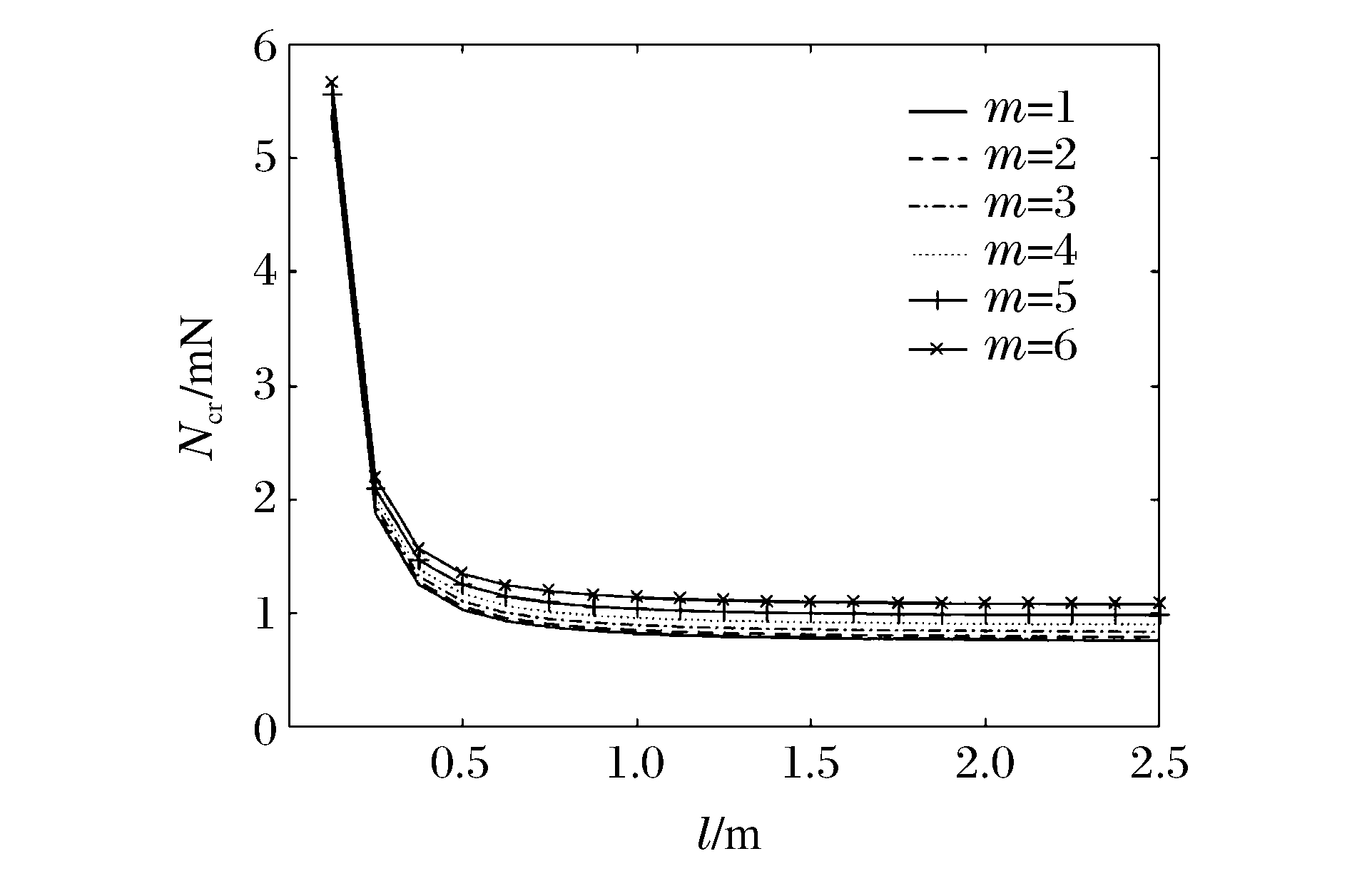
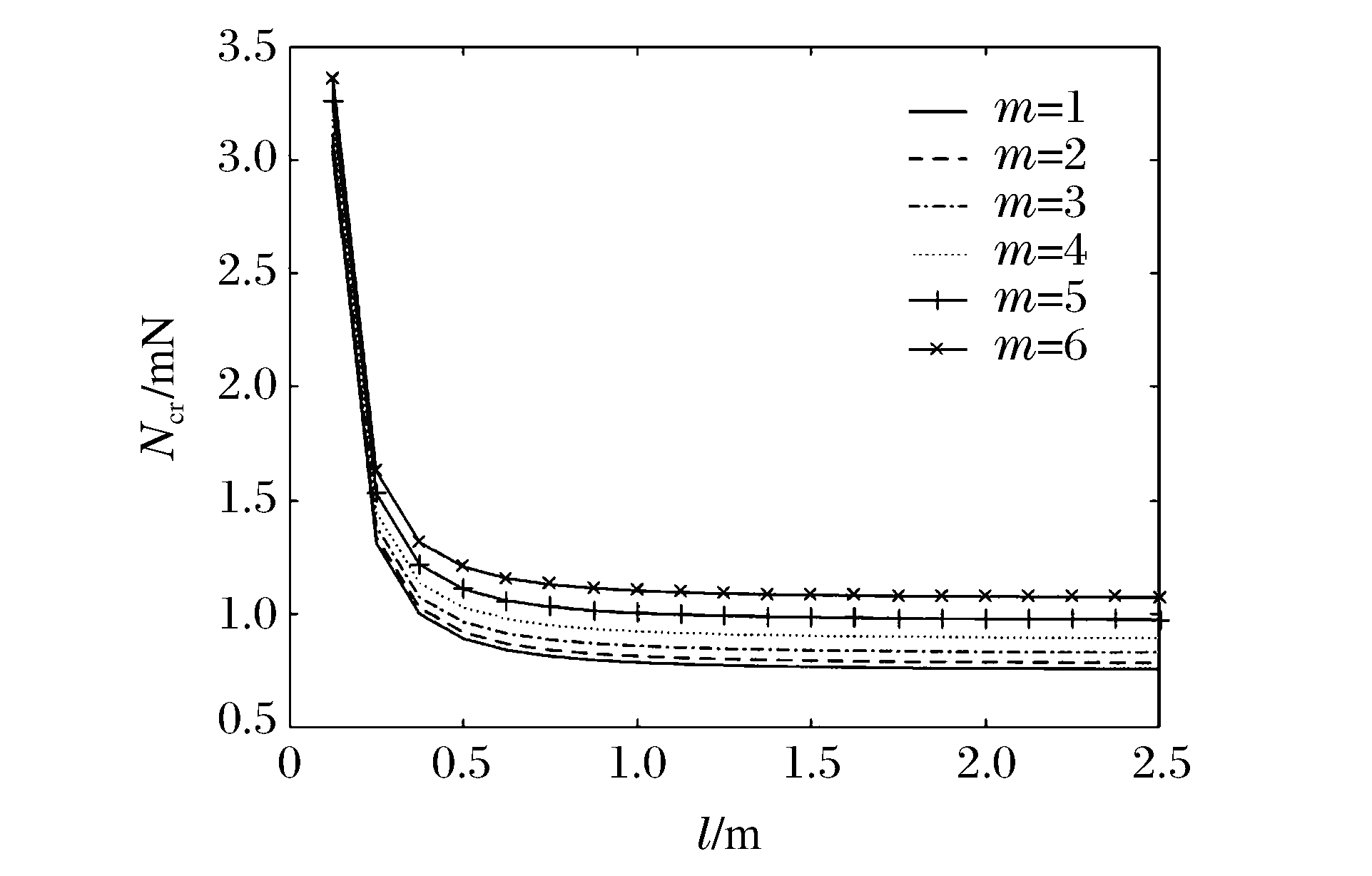
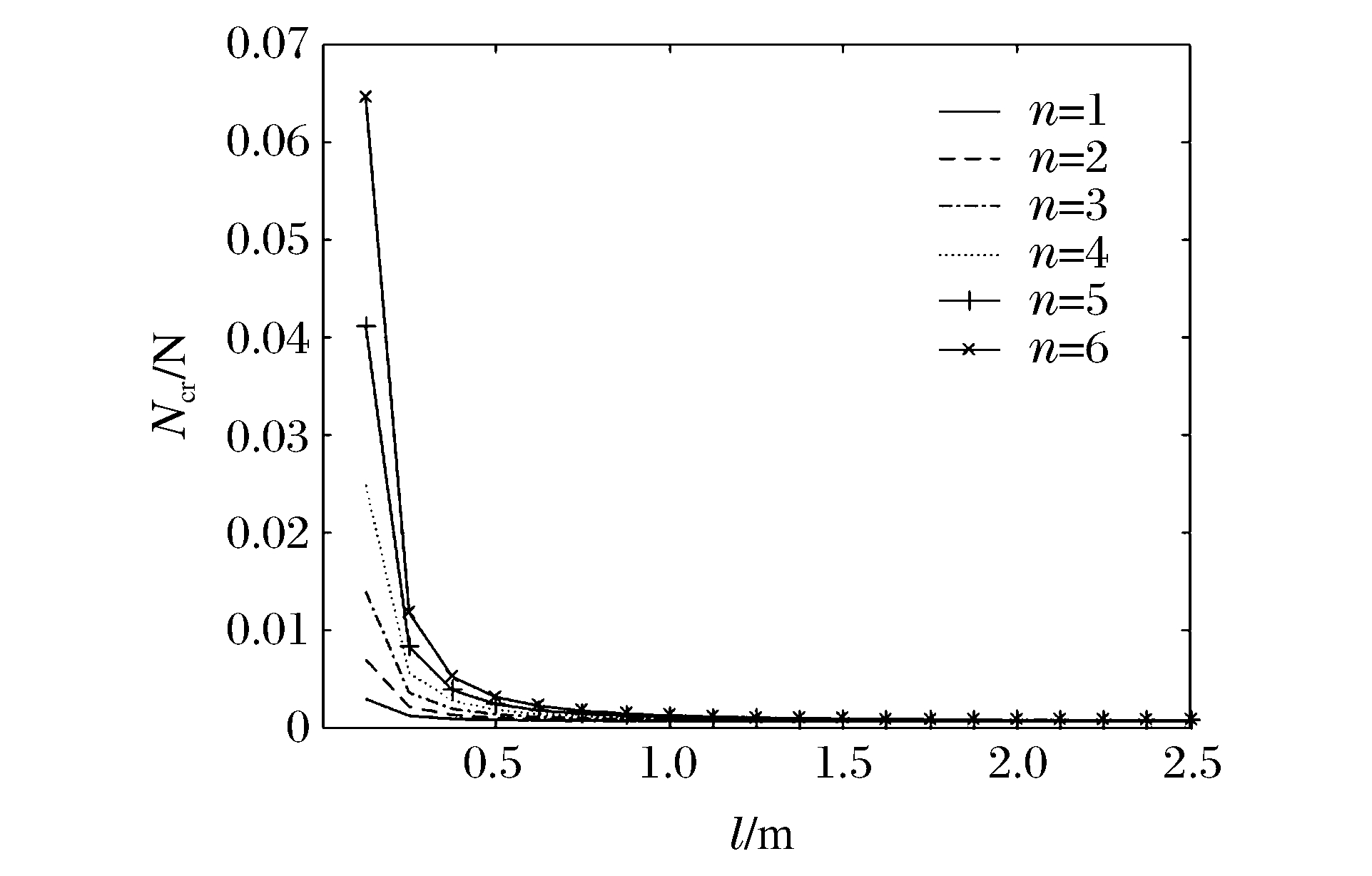
图 8和图 9分别表示冲击端为夹支和简支，n=1、k=1、R/h=20时不同m下N_cr与l的关系曲线。可以看出：当l在一定范围内时，N_cr随l的增加而迅速减小，超出这一范围后N_cr随l的增加而缓慢减小且逐渐趋于常数；同一l下，随着m的增大，FGM圆柱壳的N_cr增大，表明N_cr越大，高阶模态越易被激发。当m=6且l一定时，冲击端为夹支时的N_cr明显比冲击端为简支时的N_cr大，表明约束条件对N_cr有较大影响。

图 8 冲击端为夹支时不同环向模态数下临界荷载与临界长度的关系

Figure 8. Critical load vs.critical length under clamped edge and different circumferential modal number conditions

下载: 全尺寸图片幻灯片

图 9 冲击端为简支时不同环向模态数下临界荷载与临界长度的关系

Figure 9. Critical load vs.critical length under simple support and different circumferential modal number conditions

下载: 全尺寸图片幻灯片

图 10和图 11分别表示冲击端为夹支和简支，m=1、k=1、R/h=20时不同n下N_cr与l的关系曲线。图 10和图 11显示：N_cr随着l的增加而减小；不同n条件下，N_cr随l的增加逐渐趋于同一值；当l＜1 m时，在同一l下N_cr随n的增加而增加，说明N_cr越大，高阶模态越容易被激发。

图 10 冲击端为夹支时不同轴向模态数下临界荷载与临界长度的关系

Figure 10. Critical load vs.critical length under clamped edge and different axial modal number conditions

下载: 全尺寸图片幻灯片

图 11 冲击端为简支时不同轴向模态数下临界荷载与临界长度的关系

Figure 11. Critical load vs.critical length under simple support and different axial modal number conditions

下载: 全尺寸图片幻灯片

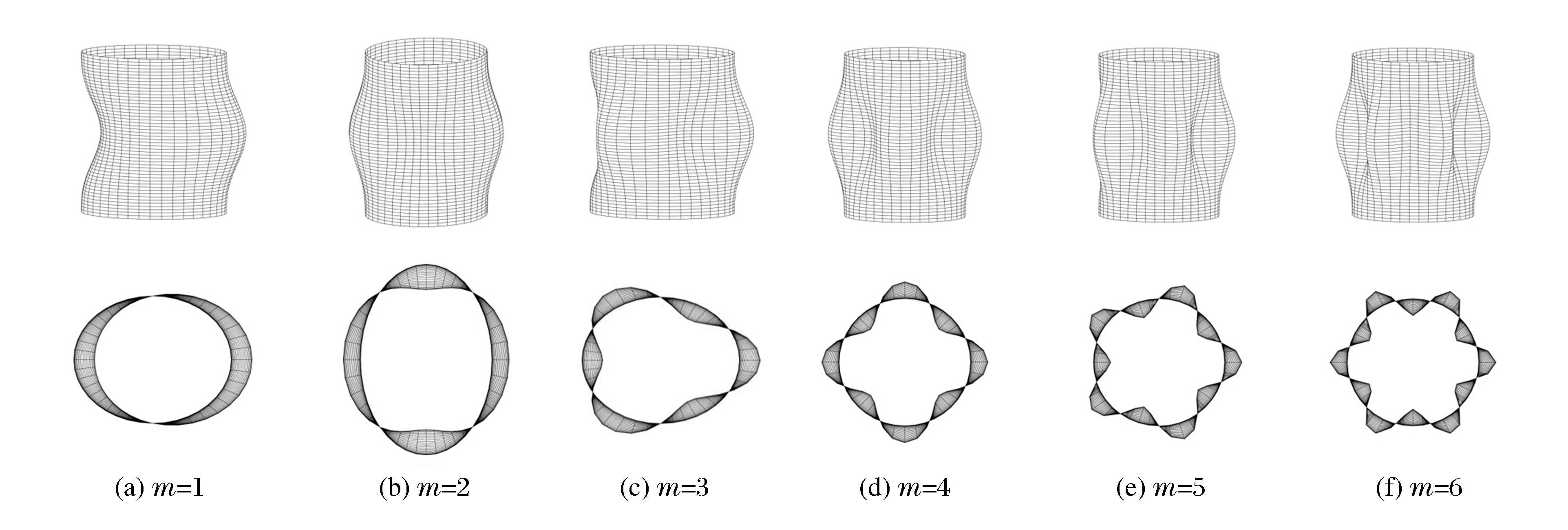
图 12为不同环向模态数m下FGM圆柱壳的动力屈曲模态。可以看出：随着m的增大，圆柱壳的模态变得越来越复杂，俯视图由单一形变为多瓣形；当m=6时，俯视图为12瓣形。

图 12 不同环向屈曲模态图(n=1;m=1, 2, 3, 4, 5, 6)

Figure 12. Different circumferential buckling modes (n=1;m=1, 2, 3, 4, 5, 6)

下载: 全尺寸图片幻灯片

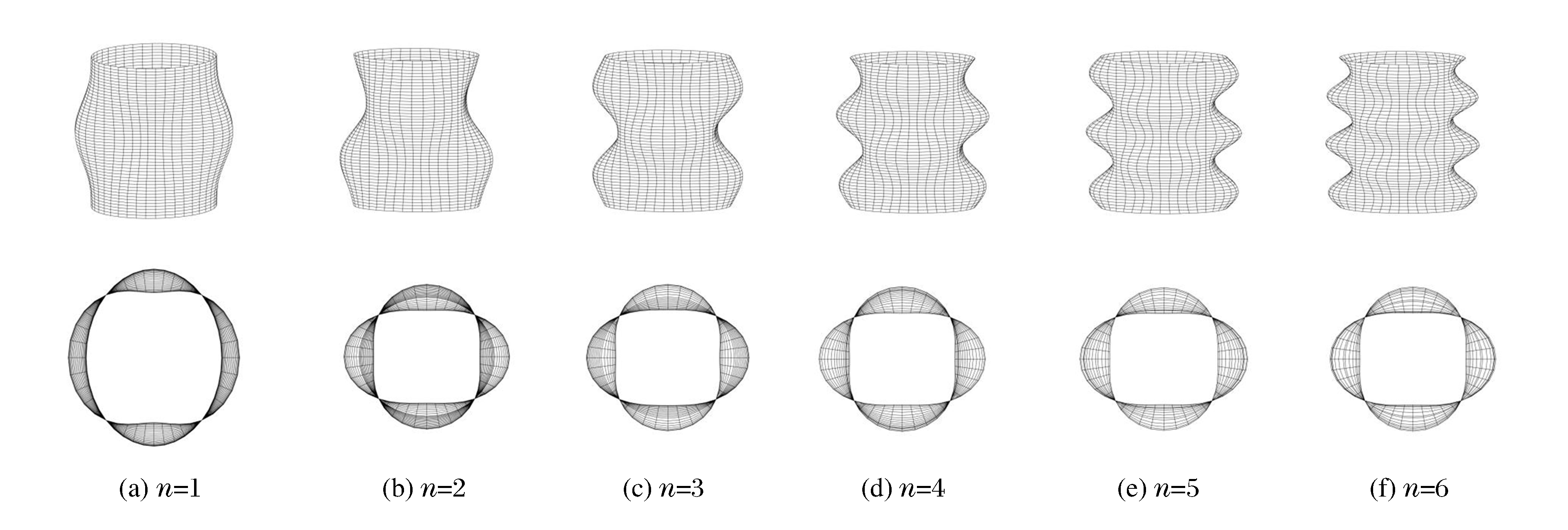
图 13为不同轴向模态数n下FGM圆柱壳的动力屈曲模态图。由图 13可知：随着n的增加，模态图变得越来越复杂。由FGM圆柱壳的俯视图可知，各阶模态数下动力屈曲模态图为轴对称。

图 13 不同轴向屈曲模态图(m=2;n=1, 2, 3, 4, 5, 6)

Figure 13. Different axial buckling modes (m=2;n=1, 2, 3, 4, 5, 6)

下载: 全尺寸图片幻灯片

4. 结论

(1) 根据Donnell壳体理论和经典板壳理论，由Hamilton变分原理得到轴向荷载作用下FGM圆柱壳的动力屈曲控制方程。

(2) 由圆柱壳周向连续性设出径向位移的周向形式，并用分离变量法得到不同约束条件下FGM圆柱壳动力屈曲临界荷载的表达式和屈曲解式。

(3) 利用MATLAB对临界荷载进行计算，得到：在轴向模态数(n)、环向模态数(m)、梯度指数(k)、径厚比(R/h)一定的情况下，同种材料组成的圆柱壳的临界荷载随着临界长度的增加而减小；在n、m、k、l一定的情况下，临界荷载随着径厚比的增大而减小；在n、m、R/h、l一定的情况下，临界荷载随着梯度指数k的增加而增加；不同约束条件下，冲击端为夹支的临界荷载大于冲击端为简支的临界荷载，表明约束条件对临界荷载有较大影响；圆柱壳的临界荷载随模态数的增加而增大，表明临界荷载越大，越容易激发高阶模态；圆柱壳的动力屈曲模态随模态数的增加变得更为复杂。

图 1 TiNi合金降温-升温过程中的原位SEM图像：(a) 290 K，(b)降温至222 K，(c)再升温至265 K，(d)再升温至290 K^[54]

Figure 1. Series of in situ SEM images of TiNi alloy at (a) 290 K and (b) 222 K upon cooling, and (c) 265 K and (d) 290 K upon heating^[54]

下载: 全尺寸图片幻灯片

图 2 冲击加载下金属铁回收样品的TEM图像^[55]

Figure 2. TEM image showing the microstructure of shock-compressed iron^[55]

下载: 全尺寸图片幻灯片

图 3 铁的 $\alpha$ → $\varepsilon$ 正相变过程（红线）及 $\varepsilon$ → $\alpha$ ′逆相变过程（蓝线）中对称性相关的变体示意图^[70]

Figure 3. Schematic illustration of the multiple symmetry-related variants for the forward $\alpha$ → $\varepsilon$ (red) and the reverse $\varepsilon$ → $\alpha$ ′ (blue) phase transitions in iron^[70]

下载: 全尺寸图片幻灯片

图 4 塑性变形对双晶结构在压剪作用下高压相成核生长的影响^[50]

Figure 4. Effect of dislocation band on nucleation and evolution of the high pressure phase of bicrystal under compression and shear^[50]

下载: 全尺寸图片幻灯片

参考文献(100)

[1]	CHEN L Q. Phase-field models for microstructure evolution [J]. Annual Review of Materials Research, 2002, 32: 113–140. doi: 10.1146/annurev.matsci.32.112001.132041
[2]	LOGINOVA I S, SINGER H M. The phase field technique for modeling multiphase materials [J]. Reports on Progress in Physics, 2008, 71(10): 106501. doi: 10.1088/0034-4885/71/10/106501
[3]	EMMERICH H. Advances of and by phase-field modelling in condensed-matter physics [J]. Advances in Physics, 2008, 57(1): 1–87. doi: 10.1080/00018730701822522
[4]	STEINBACH I. Phase-field models in materials science [J]. Modelling and Simulation in Materials Science and Engineering, 2009, 17(7): 073001. doi: 10.1088/0965-0393/17/7/073001
[5]	WANG Y Z, LI J. Phase field modeling of defects and deformation [J]. Acta Materialia, 2010, 58(4): 1212–1235. doi: 10.1016/j.actamat.2009.10.041
[6]	BOETTINGER W J, WARREN J A, BECKERMANN C, et al. Phase-field simulation of solidification [J]. Annual Review of Materials Research, 2002, 32: 163–194. doi: 10.1146/annurev.matsci.32.101901.155803
[7]	WANG Y, KHACHATURYAN A G. Three-dimensional field model and computer modeling of martensitic transformations [J]. Acta Materialia, 1997, 45(2): 759–773. doi: 10.1016/S1359-6454(96)00180-2
[8]	WANG Y Z, KHACHATURYAN A G. Multi-scale phase field approach to martensitic transformations [J]. Materials Science and Engineering: A, 2006, 438/439/440: 55–63. doi: 10.1016/j.msea.2006.04.123
[9]	MAMIVAND M, ZAEEM M A, KADIRI H E. A review on phase field modeling of martensitic phase transformation [J]. Computational Materials Science, 2013, 77: 304–311. doi: 10.1016/j.commatsci.2013.04.059
[10]	DENG Y, GAMMER C, CISTON J, et al. Hierarchically-structured large superelastic deformation in ferroelastic-ferroelectrics [J]. Acta Materialia, 2019, 181: 501–509. doi: 10.1016/j.actamat.2019.10.018
[11]	CLAYTON J D, KNAP J. A phase field model of deformation twinning: nonlinear theory and numerical simulations [J]. Physica D: Nonlinear Phenomena, 2011, 240(9/10): 841–858. doi: 10.1016/j.physd.2010.12.012
[12]	LIU G S, MO H X, WANG J, et al. Coupled crystal plasticity finite element-phase field model with kinetics-controlled twinning mechanism for hexagonal metals [J]. Acta Materialia, 2021, 202: 399–416. doi: 10.1016/j.actamat.2020.11.002
[13]	WANG L Y, LIU Z L, ZHUANG Z. Developing micro-scale crystal plasticity model based on phase field theory for modeling dislocations in heteroepitaxial structures [J]. International Journal of Plasticity, 2016, 81: 267–283. doi: 10.1016/j.ijplas.2016.01.010
[14]	ALBRECHT C, HUNTER A, KUMAR A, et al. A phase field model for dislocations in hexagonal close packed crystals [J]. Journal of the Mechanics and Physics of Solids, 2020, 137: 103823. doi: 10.1016/j.jmps.2019.103823
[15]	BOURDIN B, FRANCFORT G A, MARIGO J J. The variational approach to fracture [M]. Berlin: Springer Verlag, 2008.
[16]	MIEHE C, HOFACKER M, WELSCHINGER F. A phase field model for rate-independent crack propagation: robust algorithmic implementation based on operator splits [J]. Computer Methods in Applied Mechanics and Engineering, 2010, 199(45/46/47/48): 2765–2778. doi: 10.1016/j.cma.2010.04.011
[17]	REZAEI S, MIANROODI J R, BREPOLS T, et al. Direction-dependent fracture in solids: atomistically calibrated phase-field and cohesive zone model [J]. Journal of the Mechanics and Physics of Solids, 2021, 147: 104253. doi: 10.1016/j.jmps.2020.104253
[18]	WANG T, YE X, LIU Z L, et al. Modeling the dynamic and quasi-static compression-shear failure of brittle materials by explicit phase field method [J]. Computational Mechanics, 2019, 64(6): 1537–1556. doi: 10.1007/s00466-019-01733-z
[19]	WANG T, YE X, LIU Z L, et al. A phase-field model of thermo-elastic coupled brittle fracture with explicit time integration [J]. Computational Mechanics, 2020, 65(5): 1305–1321. doi: 10.1007/s00466-020-01820-6
[20]	BALL J M, JAMES R D. Fine phase mixtures as minimizers of energy [J]. Archive for Rational Mechanics and Analysis, 1987, 100(1): 13–52. doi: 10.1007/BF00281246
[21]	BALL J M, JAMES R D. Proposed experimental tests of a theory of fine microstructure and the two-well problem [J]. Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences, 1992, 338(1650): 389–450. doi: 10.1098/rsta.1992.0013
[22]	KOHN R V. The relaxation of a double-well energy [J]. Continuum Mechanics and Thermodynamics, 1991, 3(3): 193–236. doi: 10.1007/BF01135336
[23]	BHATTACHARYA K. Microstructure of martensite [M]. Oxford: Oxford University Press, 2004.
[24]	唐志平. 冲击相变[M]. 北京: 科学出版社, 2008: 87.
[25]	WEN Y H, WANG Y, BENDERSKY L A, et al. Microstructural evolution during the α₂→α₂ + O transformation in Ti-Al-Nb alloys: phase-field simulation and experimental validation [J]. Acta Materialia, 2000, 48(16): 4125–4135. doi: 10.1016/S1359-6454(00)00186-5
[26]	ARTEMEV A, JIN Y, KHACHATURYAN A G. Three-dimensional phase field model of proper martensitic transformation [J]. Acta Materialia, 2001, 49(7): 1165–1177. doi: 10.1016/S1359-6454(01)00021-0
[27]	RAO W F, KHACHATURYAN A G. Phase field theory of proper displacive phase transformations: structural anisotropy and directional ﬂexibility, a vector model, and the transformation kinetics [J]. Acta Materialia, 2011, 59(11): 4494–4503. doi: 10.1016/j.actamat.2011.03.072
[28]	JAVANBAKHT M, ADAEI M. Investigating the effect of elastic anisotropy on martensitic phase transformations at the nanoscale [J]. Computational Materials Science, 2019, 167: 168–182. doi: 10.1016/j.commatsci.2019.05.047
[29]	XIE X, KANG G Z, KAN Q H, et al. Phase-field theory based finite element simulation on thermo-mechanical cyclic deformation of polycrystalline super-elastic NiTi shape memory alloy [J]. Computational Materials Science, 2020, 184: 109899. doi: 10.1016/j.commatsci.2020.109899
[30]	WANG D, LIANG Q L, ZHAO S S, et al. Phase field simulation of martensitic transformation in pre-strained nanocomposite shape memory alloys [J]. Acta Materialia, 2019, 164: 99–109. doi: 10.1016/j.actamat.2018.10.030
[31]	LEVITAS V I, LEVIN V A, ZINGERMAN K M, et al. Displacive phase transitions at large strains: phase-field theory and simulations [J]. Physical Review Letters, 2009, 103(2): 025702. doi: 10.1103/PhysRevLett.103.025702
[32]	VAN DER WAALS J D. The thermodynamic theory capillarity under the hypothesis of a continuous variation of density [J]. Verhandelingen van de Koninklijke Academie voor Wetenschappen, 1893, 1: 1–56.
[33]	GIBBS J W. A method of geometrical representation of the thermodynamic properties of substances by means of surfaces [J]. Transactions of the Connecticut Academy, 1873, 2: 382–404.
[34]	CAHN J W, HILLIARD J E. Free energy of a nonuniform system. I. interfacial free energy [J]. The Journal of Chemical Physics, 1957, 28(2): 258. doi: 10.1063/1.1744102
[35]	ALLEN S M, CAHN J W. A microscopic theory for antiphase boundary motion and its application to antiphase domain coarsening [J]. Acta Metallurgica, 1979, 27(6): 1085–1095. doi: 10.1016/0001-6160(79)90196-2
[36]	KOBAYASHI H, ODE M, KIM S G, et al. Phase-field model for solidification of ternary alloys coupled with thermodynamic database [J]. Scripta Materialia, 2003, 48(6): 689–694. doi: 10.1016/S1359-6462(02)00557-2
[37]	SEOL D J, HU S Y, LI Y L, et al. Computer simulation of spinodal decomposition in constrained films [J]. Acta Materialia, 2003, 51(17): 5173–5185. doi: 10.1016/S1359-6454(03)00378-1
[38]	WANG Y, JIN Y M, CUITIÑO A M, et al. Nanoscale phase field microelasticity theory of dislocations: model and 3D simulations [J]. Acta Materialia, 2001, 49(10): 1847–1857. doi: 10.1016/S1359-6454(01)00075-1
[39]	CAHN J W, HAN S C, MCFADDEN G B. Anisotropy of interfaces in an ordered hcp binary alloy [J]. Journal of Statistical Physics, 1999, 95(5/6): 1337–1360. doi: 10.1023/A:1004583324097
[40]	OGATA S, LI J, YIP S. Ideal pure shear strength of aluminum and copper [J]. Science, 2002, 298(5594): 807–811. doi: 10.1126/science.1076652
[41]	OGATA S, LI J, YIP S. Energy landscape of deformation twinning in bcc and fcc metals [J]. Physical Review B, 2005, 71(22): 224102. doi: 10.1103/PhysRevB.71.224102
[42]	SHEN C, LI J, WANG Y Z. Finding critical nucleus in solid-state transformations [J]. Metallurgical and Materials Transactions A, 2008, 39(5): 976–983. doi: 10.1007/s11661-007-9302-7
[43]	KARMA A, RAPPEL W J. Phase-field method for computationally efficient modeling of solidification with arbitrary interface kinetics [J]. Physical Review E, 1996, 53(4): R3017. doi: 10.1103/PhysRevE.53.R3017
[44]	KARMA A, RAPPEL W J. Quantitative phase-field modeling of dendritic growth in two and three dimensions [J]. Physical Review E, 1998, 57(4): 4323–4349. doi: 10.1103/PhysRevE.57.4323
[45]	FINEL A, BOUAR Y L, DABAS B, et al. Sharp phase field method [J]. Physical Review Letters, 2018, 121(2): 025501. doi: 10.1103/PhysRevLett.121.025501
[46]	PROVATAS N, GOLDENFELD N, DANTZIG J. Efficient computation of dendritic microstructures using adaptive mesh refinement [J]. Physical Review Letters, 1998, 80(15): 3308–3311. doi: 10.1103/PhysRevLett.80.3308
[47]	JEONG D, KIM J. Fast and accurate adaptive finite difference method for dendritic growth [J]. Computer Physics Communications, 2019, 236: 95–103. doi: 10.1016/j.cpc.2018.10.020
[48]	LEVITAS V I, IDESMAN A V, PRESTON D L. Microscale simulation of martensitic microstructure evolution [J]. Physical Review Letters, 2004, 93(10): 105701. doi: 10.1103/PhysRevLett.93.105701
[49]	STEINBACH I, APEL M. Multi phase field model for solid state transformation with elastic strain [J]. Physica D: Nonlinear Phenomena, 2006, 217(2): 153–160. doi: 10.1016/j.physd.2006.04.001
[50]	LEVITAS V I, ESFAHANI S E, GHAMARIAN I. Scale-free modeling of coupled evolution of discrete dislocation bands and multivariant martensitic microstructure [J]. Physical Review Letters, 2018, 121(20): 205701. doi: 10.1103/PhysRevLett.121.205701
[51]	ABBOUD H, KOSSEIFI C A, CHEHAB J P. A stabilized bi-grid method for Allen-Cahn equation in finite elements [J]. Computational and Applied Mathematics, 2019, 38(2): 35. doi: 10.1007/S40314-019-0781-0
[52]	徐祖耀. 材料相变[M]. 北京: 高等教育出版社, 2013: 352.
[53]	BHATTACHARYA K, CONTI S, ZANZOTTO G, et al. Crystal symmetry and the reversibility of martensitic transformations [J]. Nature, 2004, 428(6978): 55–59. doi: 10.1038/nature02378
[54]	SOEJIMA Y, MOTOMURA S, MITSUHARA M, et al. In situ scanning electron microscopy study of the thermoelastic martensitic transformation in Ti-Ni shape memory alloy [J]. Acta Materialia, 2016, 103: 352–360. doi: 10.1016/j.actamat.2015.10.017
[55]	WANG S J, SUI M L, CHEN Y T, et al. Microstructural fingerprints of phase transitions in shock-loaded iron [J]. Scientific Reports, 2013, 3: 1086. doi: 10.1038/srep01086
[56]	FALK F. One-dimensional model of shape memory alloys [J]. Archives of Mechanics, 1983, 35(1): 63–84.
[57]	FALK F, KONOPKA P. Three-dimensional Landau theory describing the martensitic phase transformation of shape-memory alloys [J]. Journal of Physics: Condensed Matter, 1990, 2(1): 61–77. doi: 10.1088/0953-8984/2/1/005
[58]	JACOBS A E, CURNOE S H, DESAI R C. Simulations of cubic-tetragonal ferroelastics [J]. Physical Review B, 2003, 68(22): 224104. doi: 10.1103/PhysRevB.68.224104
[59]	CAHN J W, KALONJI G. Symmetry in solid state transformation morphology [M]. Warrendale, USA, 1981.
[60]	VEDANTAM S, ABEYARATNE R. A Helmholtz free-energy function for a Cu-Al-Ni shape memory alloy [J]. International Journal of Non-Linear Mechanics, 2005, 40(2/3): 177–193. doi: 10.1016/j.ijnonlinmec.2004.05.005
[61]	SHCHYGLO O, SALMAN U, FINEL A. Martensitic phase transformations in Ni-Ti-based shape memory alloys: the Landau theory [J]. Acta Materialia, 2012, 60(19): 6784–6792. doi: 10.1016/j.actamat.2012.08.056
[62]	LEVITAS V I, PRESTON D L. Three-dimensional Landau theory for multivariant stress-induced martensitic phase transformations. I. austenite ↔ martensite [J]. Physical Review B, 2002, 66(13): 134206. doi: 10.1103/PhysRevB.66.134206
[63]	LEVITAS V I, PRESTON D L. Three-dimensional Landau theory for multivariant stress-induced martensitic phase transformations. II. multivariant phase transformations and stress space analysis [J]. Physical Review B, 2002, 66(13): 134207. doi: 10.1103/PhysRevB.66.134207
[64]	SHE H, LIU Y L, WANG B. Phase field simulation of heterogeneous cubic→tetragonal martensite nucleation [J]. International Journal of Solids and Structures, 2013, 50(7/8): 1187–1191. doi: 10.1016/j.ijsolstr.2012.12.020
[65]	GOMEZ H, BURES M, MOURE A. A review on computational modelling of phase-transition problems [J]. Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences, 2019, 377(2143): 20180203. doi: 10.1098/RSTA.2018.0203
[66]	WEN Y H, WANG Y, CHEN L Q. Effect of elastic interaction on the formation of a complex multi-domain microstructural pattern during a coherent hexagonal to orthorhombic transformation [J]. Acta Materialia, 1999, 47(17): 4375–4386. doi: 10.1016/S1359-6454(99)00247-5
[67]	WEN Y H, WANG Y, CHEN L Q. Phase-field simulation of domain structure evolution during a coherent hexagonal-to-orthorhombic transformation [J]. Philosophical Magazine A, 2000, 80(9): 1967–1982. doi: 10.1080/01418610008212146
[68]	DEVILLE S, GUÉNIN G, CHEVALIER J. Martensitic transformation in zirconia: Part I. nanometer scale prediction and measurement of transformation induced relief [J]. Acta Materialia, 2004, 52(19): 5697–5707. doi: 10.1016/j.actamat.2004.08.034
[69]	BAIN E C. The nature of martensite [J]. Trans. AIME, 1924, 70: 25–46.
[70]	VATTRÉ A, DENOUAL C. Polymorphism of iron at high pressure: a 3D phase-field model for displacive transitions with finite elastoplastic deformations [J]. Journal of the Mechanics and Physics of Solids, 2016, 92: 1–27. doi: 10.1016/J.JMPS.2016.01.016
[71]	DENOUAL C, CAUCCI A M, SOULARD L, et al. Phase-field reaction-pathway kinetics of martensitic transformations in a model Fe₃Ni alloy [J]. Physical Review Letters, 2010, 105(3): 035703. doi: 10.1103/PhysRevLett.105.035703
[72]	DENOUAL C, VATTRÉ A. A phase field approach with a reaction pathways-based potential to model reconstructive martensitic transformations with a large number of variants [J]. Journal of the Mechanics and Physics of Solids, 2016, 90: 91–107. doi: 10.1016/j.jmps.2016.02.022
[73]	VATTRÉ A, DENOUAL C. Continuum nonlinear dynamics of unstable shock waves induced by structural phase transformations in iron [J]. Journal of the Mechanics and Physics of Solids, 2019, 131: 387–403. doi: 10.1016/j.jmps.2019.07.012
[74]	HOMAYONIFAR M, MOSLER J. Efficient modeling of microstructure evolution in magnesium by energy minimization [J]. International Journal of Plasticity, 2012, 28(1): 1–20. doi: 10.1016/j.ijplas.2011.05.011
[75]	CLAYTON J D. Nonlinear Eulerian thermoelasticity for anisotropic crystals [J]. Journal of the Mechanics and Physics of Solids, 2013, 61(10): 1983–2014. doi: 10.1016/j.jmps.2013.05.009
[76]	OLSON G B, ROITBURD A L. Martensitic nucleation [M]//OLSON G B, OWEN W S. Martensite. Materials Park, Ohio: ASM International, 1992: 149.
[77]	HENNIG R G, TRINKLE D R, BOUCHET J, et al. Impurities block the α to ω martensitic transformation in titanium [J]. Nature Materials, 2005, 4(2): 129–133. doi: 10.1038/nmat1292
[78]	CASPERSEN K J, CARTER E A. Finding transition states for crystalline solid-solid phase transformations [J]. Proceedings of the National Academy of Sciences of the United States of America, 2005, 102(19): 6738–6743. doi: 10.1073/pnas.0408127102
[79]	DUPÉ B, AMADON B, PELLEGRINI Y P, et al. Mechanism for the α→ε phase transition in iron [J]. Physical Review B, 2013, 87(2): 024103. doi: 10.1103/PhysRevB.87.024103
[80]	BAKHTIARI S, LIU J Z, LIU Y N, et al. Monoclinic angle, shear response, and minimum energy pathways of NiTiCu martensite phases from ab initio calculations [J]. Acta Materialia, 2019, 178: 59–67. doi: 10.1016/j.actamat.2019.07.050
[81]	GAO Y P, SHI R P, NIE J F, et al. Group theory description of transformation pathway degeneracy in structural phase transformations [J]. Acta Materialia, 2016, 109: 353–363. doi: 10.1016/j.actamat.2016.01.027
[82]	GAO Y P, WANG Y Z. Hidden pathway during fcc to bcc/bct transformations: crystallographic origin of slip martensite in steels [J]. Physical Review Materials, 2018, 2(9): 093611. doi: 10.1103/PhysRevMaterials.2.093611
[83]	ZHANG T L, WANG D, WANG Y Z. Novel transformation pathway and heterogeneous precipitate microstructure in Ti-alloys [J]. Acta Materialia, 2020, 196: 409–417. doi: 10.1016/j.actamat.2020.06.048
[84]	GAO Y P. Symmetry and pathway analyses of the twinning modes in Ni-Ti shape memory alloys [J]. Materialia, 2019, 6: 100320. doi: 10.1016/j.mtla.2019.100320
[85]	GAO Y P, ZHENG Y F, FRASER H, et al. Intrinsic coupling between twinning plasticity and transformation plasticity in metastable β Ti-alloys: a symmetry and pathway analysis [J]. Acta Materialia, 2020, 196: 488–504. doi: 10.1016/j.actamat.2020.07.020
[86]	OLSON G B. Computational design of hierarchically structured materials [J]. Science, 1997, 277(5330): 1237–1242. doi: 10.1126/science.277.5330.1237
[87]	CISSÉ C, ZAEEM M A. A phase-field model for non-isothermal phase transformation and plasticity in polycrystalline yttria-stabilized tetragonal zirconia [J]. Acta Materialia, 2020, 191: 111–123. doi: 10.1016/j.actamat.2020.03.025
[88]	WEI S L, KIM J W, CANN J L, et al. Plastic strain-induced sequential martensitic transformation [J]. Scripta Materialia, 2020, 185: 36–41. doi: 10.1016/j.scriptamat.2020.03.060
[89]	LEVITAS V I. High pressure phase transformations revisited [J]. Journal of Physics: Condensed Matter, 2018, 30(16): 163001. doi: 10.1088/1361-648X/aab4b0
[90]	XU Y, MING P B, CHEN J. A phase field framework for dynamic adiabatic shear banding [J]. Journal of the Mechanics and Physics of Solids, 2020, 135: 103810. doi: 10.1016/j.jmps.2019.103810
[91]	JAVANBAKHT M, LEVITAS V I. Phase field simulations of plastic strain-induced phase transformations under high pressure and large shear [J]. Physical Review B, 2016, 94(21): 214104. doi: 10.1103/PhysRevB.94.214104
[92]	GUO X H, SHI S Q, MA X Q. Elastoplastic phase field model for microstructure evolution [J]. Applied Physics Letters, 2005, 87(22): 221910. doi: 10.1063/1.2138358
[93]	PARANJAPE H M, MANCHIRAJU S, ANDERSON P M. A phase field-finite element approach to model the interaction between phase transformations and plasticity in shape memory alloys [J]. International Journal of Plasticity, 2016, 80: 1–18. doi: 10.1016/j.ijplas.2015.12.007
[94]	KUNDIN J, RAABE D, EMMERICH H. A phase-field model for incoherent martensitic transformations including plastic accommodation processes in the austenite [J]. Journal of the Mechanics and Physics of Solids, 2011, 59(10): 2082–2102. doi: 10.1016/j.jmps.2011.07.001
[95]	ESFAHANI S E, GHAMARIAN I, LEVITAS V I. Strain-induced multivariant martensitic transformations: a scale-independent simulation of interaction between localized shear bands and microstructure [J]. Acta Materialia, 2020, 196: 430–443. doi: 10.1016/j.actamat.2020.06.059
[96]	SCHMITT R, KUHN C, MÜLLER R, et al. Crystal plasticity and martensitic transformations: a phase field approach [J]. Technische Mechanik, 2014, 34(1): 23–38. doi: 10.24352/UB.OVGU-2017-051
[97]	ROTERS F, EISENLOHR P, HANTCHERLI L, et al. Overview of constitutive laws, kinematics, homogenization and multiscale methods in crystal plasticity finite-element modeling: theory, experiments, applications [J]. Acta Materialia, 2010, 58(4): 1152–1211. doi: 10.1016/j.actamat.2009.10.058
[98]	RICE J R. Inelastic constitutive relations for solids: an internal-variable theory and its application to metal plasticity [J]. Journal of the Mechanics and Physics of Solids, 1971, 19(6): 433–455. doi: 10.1016/0022-5096(71)90010-X
[99]	MA A, ROTERS F. A constitutive model for fcc single crystals based on dislocation densities and its application to uniaxial compression of aluminium single crystals [J]. Acta Materialia, 2004, 52(12): 3603–3612. doi: 10.1016/j.actamat.2004.04.012
[100]	YAO S L, YU J D, CUI Y N, et al. Revisiting the power law characteristics of the plastic shock front under shock loading [J]. Physical Review Letters, 2021, 126(8): 085503. doi: 10.1103/PhysRevLett.126.085503