-
Abstract: Dynamic fracture behavior of rocks under blasting loading are a major concern in civil engineering, mining, oil and gas industries. This study presented herein is on the borehole blasting-induced fractures in rocks. The paper consists of two parts: the first part gives a brief description of a constitutive model for rocks subjected to dynamic loading, which is mainly based on a recently developed model for concrete; the second part deals with numerical simulations of borehole blasting-induced fractures in rocks. The values of various parameters in the constitutive model for granite are first estimated and then employed in the numerical simulations. It is demonstrated that the numerical results in terms of peak pressures and crack patterns predicted from the present model are in good agreement with the experimental observations made both in cylindrical granite sample reported in the literature and in square granite specimens conducted in our own laboratory. Moreover, the analysis shows that the experimentally observed crack patterns are mainly caused by tensile stress, while the smaller cracks around borehole are created largely by compression/shear stress.
-
Key words:
- granite rocks /
- constitutive model /
- borehole blasting /
- fracture
-
It is very important for mining and civil construction to predict the morphology distribution of cracks induced by blasting. Hence, many researchers have paid their attention to dynamic fracture behavior of rocks due to drilling and blasting operations[1-3]. A number of experiments and numerical simulations have been conducted to investigate the blasting-induced fractures in the near borehole zone as well as in the far field[4-5]. In order to gain high fidelity in simulating the complex responses of rocks subjected to blasting loading, a realistic constitutive model is required. In the last 20 years, various macro-scale material models have been proposed, from relatively simple ones to more sophisticated, and their capabilities in describing actual nonlinear behavior of material under different loading conditions have been evaluated[6].
During blasting operation, chemical reactions of explosive in borehole occur rapidly, and instantaneously a shock/stress wave applies to borehole wall. Initially, a crushed zone around the borehole is developed by the shock/stress wave. Then, a radial shock/stress wave propagates away from borehole, and its magnitude decreases. Once the radial shock/stress drops below the local dynamic compressive strength, no shear damage occurs. At the same time, a tensile tangential stress with enough strength can be developed behind the radial compressive stress wave, which results in an extension of the existing flaws or a creation of new radial cracks. If there is a nearby free boundary, the incident compressive stress wave changes to a tensile stress wave upon reflection, and reflects back into the rock. In this case, if the dynamic tensile strength of rock is exceeded, spall cracks appear close to the free boundary.
The purpose of this paper is to conduct a numerical study on borehole blasting-induced fractures in rocks. First, a dynamic constitutive model for rocks based on the previous work of concrete[7] is briefly described, and the values of various parameters in the model for granite are estimated. The model is then employed to simulate the borehole blasting-induced fractures in granitic rocks. Comparisons between the numerical results and the experimental observations are made, and a discussion is given.
1. Dynamic Constitutive Model for Rocks
A number of models for concrete-like materials, such as TCK model[8], HJC model[9], RHT model[10], K&C model[11], have been developed. The sophisticated numerical models are increasingly used as they are capable of describing the material behavior under high strain rate loading. However, these models have been found to have some serious flaws, and cannot predict the experimentally observed crack patterns or exhibit improper behavior under certain loading conditions[7, 12-15].
In the following, a dynamic constitutive model for rocks is briefly described according to equation of state (EOS) and strength model, based on the previous work on concrete[7].
1.1 EOS
A typical form of EOS is the so-called p-
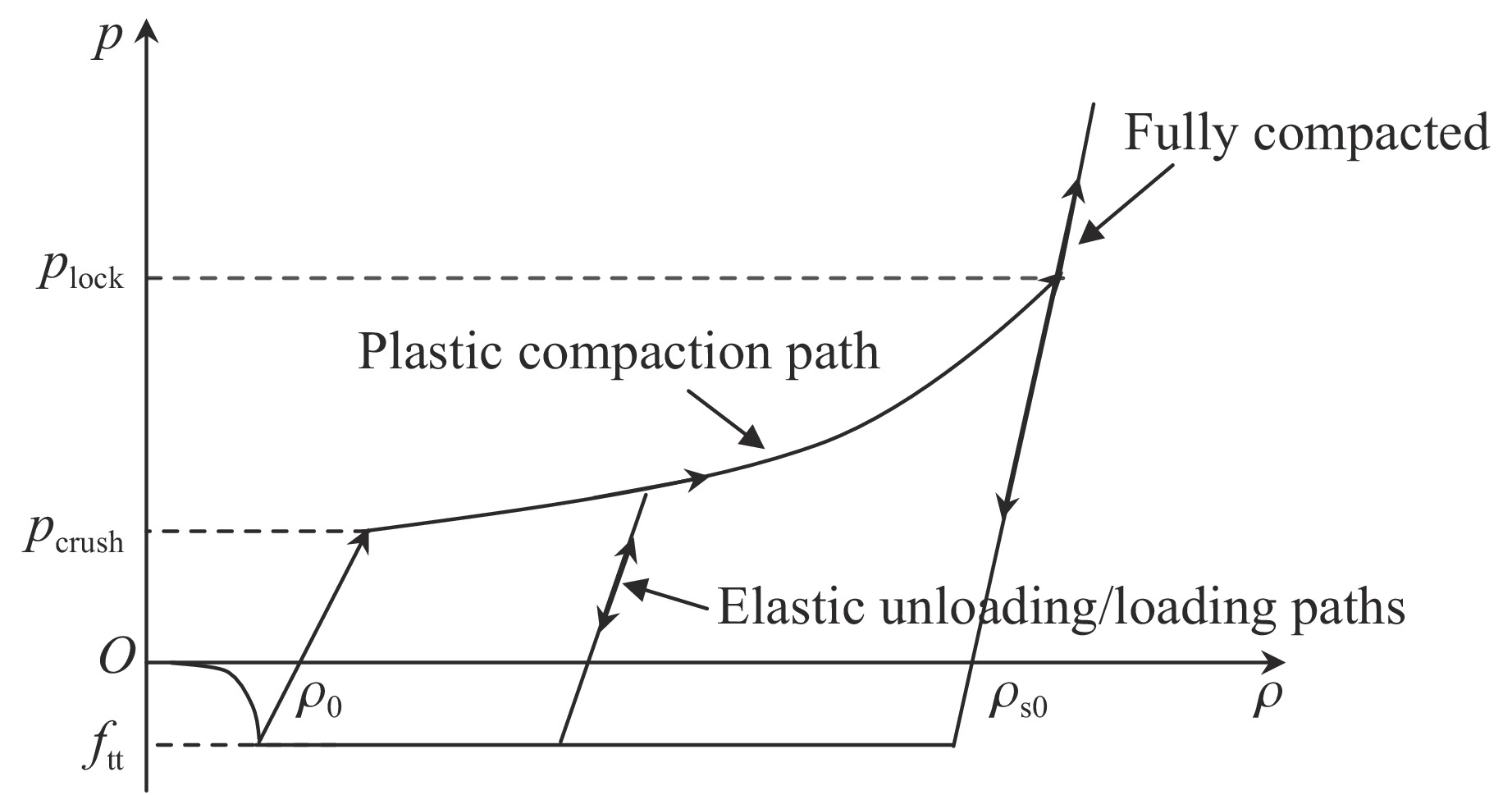
α relation, which is proved to be capable of representing brittle material’s response behavior at high pressures, and it allows for a reasonably detailed description of the compaction behavior at low pressure ranges as well, as shown schematically in Fig.1.pcrush corresponds to the pore collapse pressure beyond which plastic compaction occurs, andplock is the pressure when porosityα reaches 1,ftt is the tensile strength,ρ0 is the initial density,ρs0 refers to the density of the initial solid. Figure 1. Schematic diagram of EOS[7]
Figure 1. Schematic diagram of EOS[7]The EOS for compression (p≥0) is given by
p=K1ˉμ+K2ˉμ2+K3ˉμ3 (1) where p denotes pressure, K1, K2, K3 are constants, and
ˉμ is defined byˉμ=ραρ0α0−1=αα0(1+μ)−1 (2) where
ρ is the current density,μ=ρ/ρ0−1 specifies the volumetric strain,α0 =ρ s0/ρ 0 andα=ρs/ρ represent the initial porosity and the current porosity, respectively.ρs refers to the density of fully compacted solid. Physically,α is a function of the hydro-static pressure p, and is expressed asα=1+(α0−1)(plock−pplock−pcrush)n (3) where n is the compaction exponent.
When material withstands hydro-static tension, the EOS for tension (p<0) is given by
p=K1ˉμ (4) α=α0(1+p/K1)/(1+μ) (5) 1.2 Strength Model
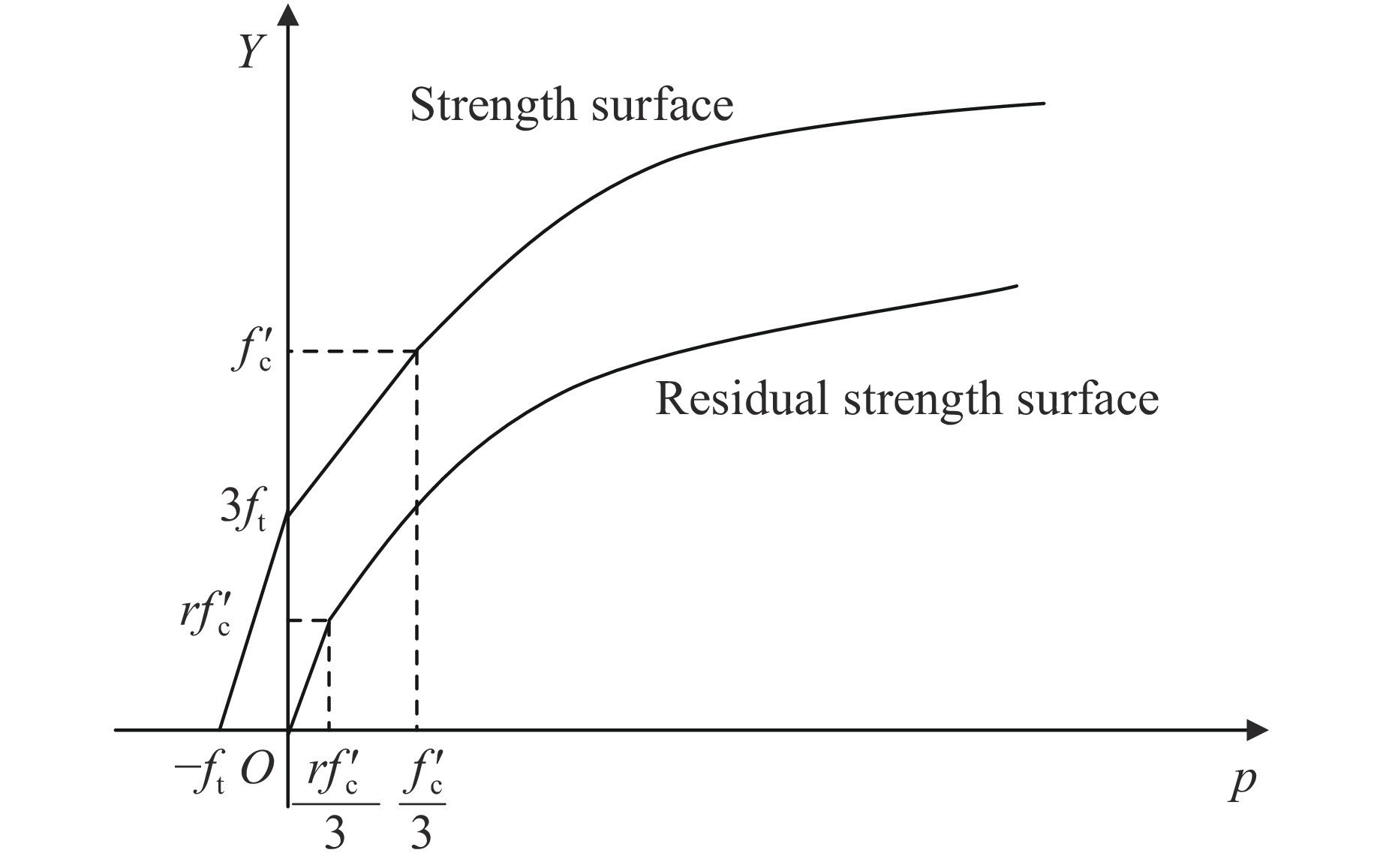
The strength model takes into account various effects, such as pressure hardening, damage softening, third stress invariant (Lode angle) and strain rate. The strength surface Y, shown schematically in Fig.2, can be written as[7]
Y={3(p+ftt)R(θ,e) p<0[3ftt + 3p(fcc−3ftt)/fcc]R(θ,e) 0⩽p⩽fcc/3{fcc+Bfc′[p/fc′−fcc/(3fc′)]N}R(θ,e) p>fcc/3 (6) where p is the hydro-static pressure, parameters B and N are constants, R(θ,e) is a function of the Lode angle θ and the tensile-to-compressive meridian ratio e,
fc′ is the static uni-axial compressive strength, the compressive strengthfcc and the tensile strengthftt are defined byfcc=fc′Dm_tηc (7) ftt=ftDtηt (8) where
ft is the static uni-axial tensile strength.Dm_t is the compression dynamic increase factor due to strain rate effect only, and can be expressed as[7, 16]Dm_t=(Dt−1)ft/fc′+1 (9) where
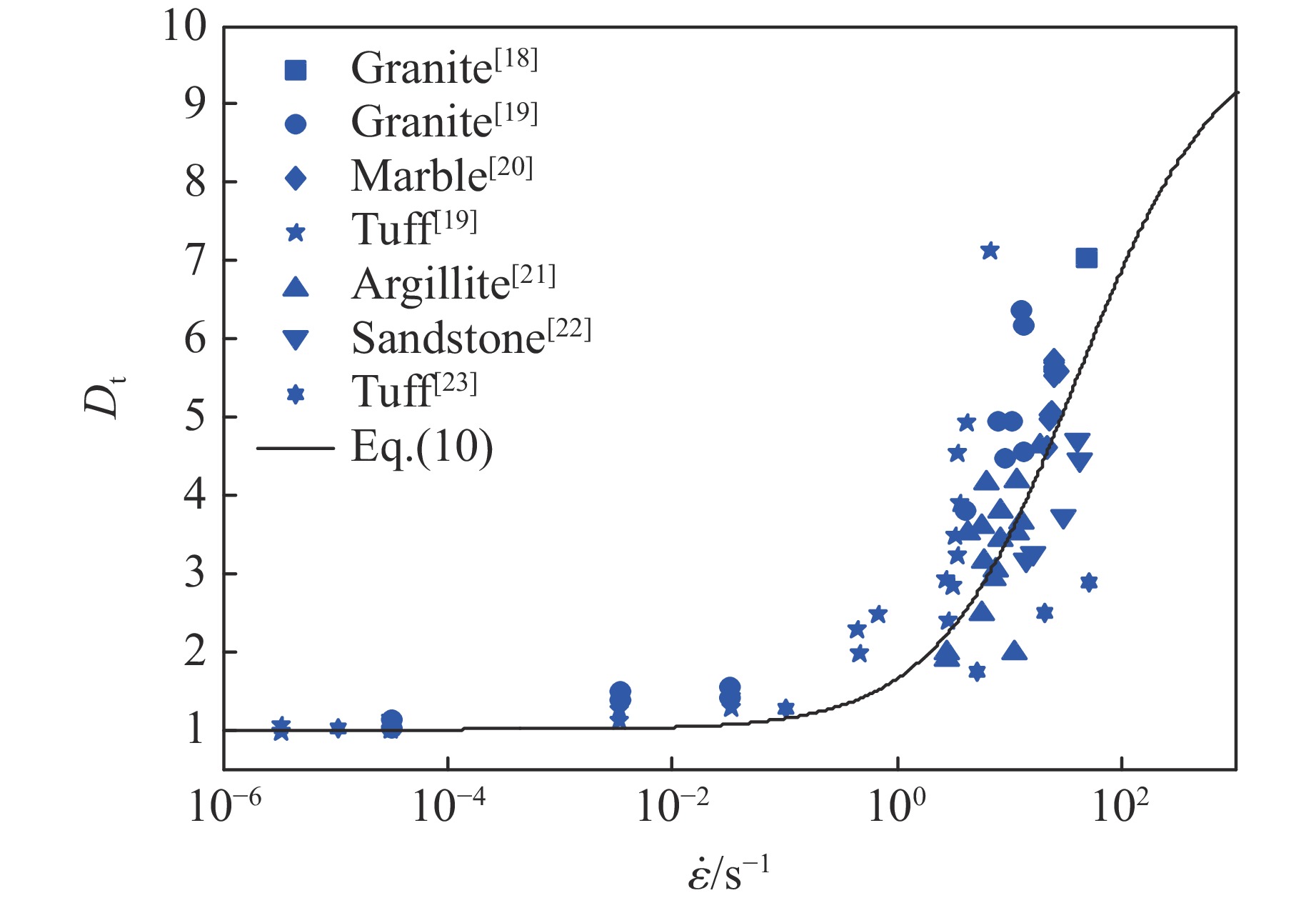
Dt is the tension dynamic increase factor determined byDt={tanh[(lg˙ε˙ε0−Wx)S][FmWy−1]+1}Wy (10) where
Fm ,Wx ,Wy andS are experimental constants,˙ε is the strain rate, and˙ε0 is the reference strain rate, usually taken˙ε0=1.0 s−1 .ηc is the damage function for compression, which can be expressed asηc={l+(1−l)η(λ)λ⩽λmr+(1−r)η(λ)λ>λm (11) where l and r are constants[7],
λm is the value of shear damage (λ ) when strength reaches its maximum value under compression.η(λ) is defined asη(λ)=aλ(λ−1)exp(−bλ) (12) in which a and b can be determined by setting
η(λ) = 1 and∂η∂λ=0 whenλ =λ m.ηt is the damage function for tension which can be written asηt=[1+(c1εtεfrac)3]exp(−c2εtεfrac)−εtεfrac(1+c31)exp(−c2) (13) where c1 and c2 are constants[7],
εt denotes the tensile strain andεfrac is the fracture strain.The residual strength (
rfc′ ) surface for rocks, shown schematically in Fig.2, can be obtained from Eq.(6) by settingftt=0 andfcc=rfc′ , vizY={3pR(θ,e) 0<p⩽rfc′/3{rfc′+Bfc′[p/fc′−rfc′/(3fc′)]N}R(θ,e) p>rfc′/3 (14) 2. Numerical Simulations
Granite is selected for investigating the dynamic fractures which result from borehole blast loading.
2.1 Evaluation of Various Parameters in the Model
Table 1 lists the values of the various parameters used in the dynamic constitutive model for granite. As to how to determine the values of the various parameters in the model, more details are presented in [7, 15-17].
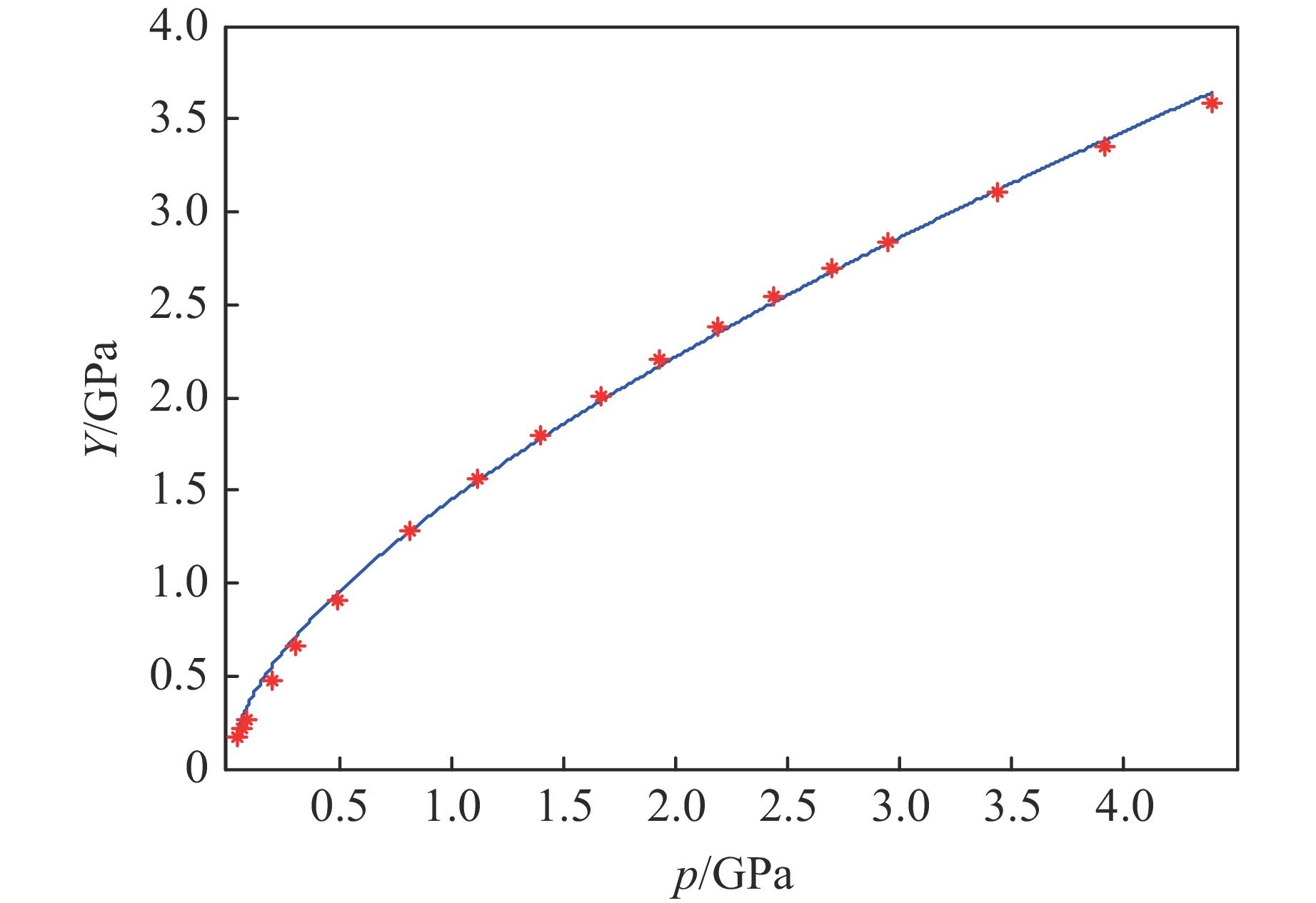
p-α relation ρ0/(kg∙m−3) pcrush/MPa plock/GPa n K1/GPa K2/TPa K3/TPa 2660 50.5 3 3 25.7 −3 150 Strength surface Strain rate effect fc′/MPa ft/MPa B N G/GPa Fm Wx 161.5 7.3 2.59 0.66 21.9 10 1.6 Strain rate effect Shear damage Wy S ˙ε0/s−1 λs λm l r 5.5 0.8 1.0 4.6 0.3 0.45 0.3 Lode effect Tensile damage e1 e2 e3 c1 c2 εfrac 0.65 0.01 5 3 6.93 0.007 Fig.3 shows the comparison of the strength surface between Eq.(6) (with B=2.59, N=0.66) and the triaxial test data for granite[17]. It can be seen from Fig.3 that a good agreement is obtained. Similarly, Fig.4 shows the tensile strengths/dynamic increase factor obtained by Eq.(10) and the test results of various rocks at different strain rates[18-23]. It is clear from Fig.4 that a good agreement is achieved.
 Figure 3. Comparison of the strength surface between Eq.(6) (with B=2.59, N=0.66) and the triaxial test data for granite[17]
Figure 3. Comparison of the strength surface between Eq.(6) (with B=2.59, N=0.66) and the triaxial test data for granite[17]2.2 Numerical Results
In the following, numerical simulations are carried out for the response of the granite targets subjected to borehole blasting loading. The dynamic fracture behavior of two kinds of granite samples are studied, namely, cylindrical sample as reported in the literature and square sample as examined in our own laboratory.
2.2.1 Cylindrical Rock Sample
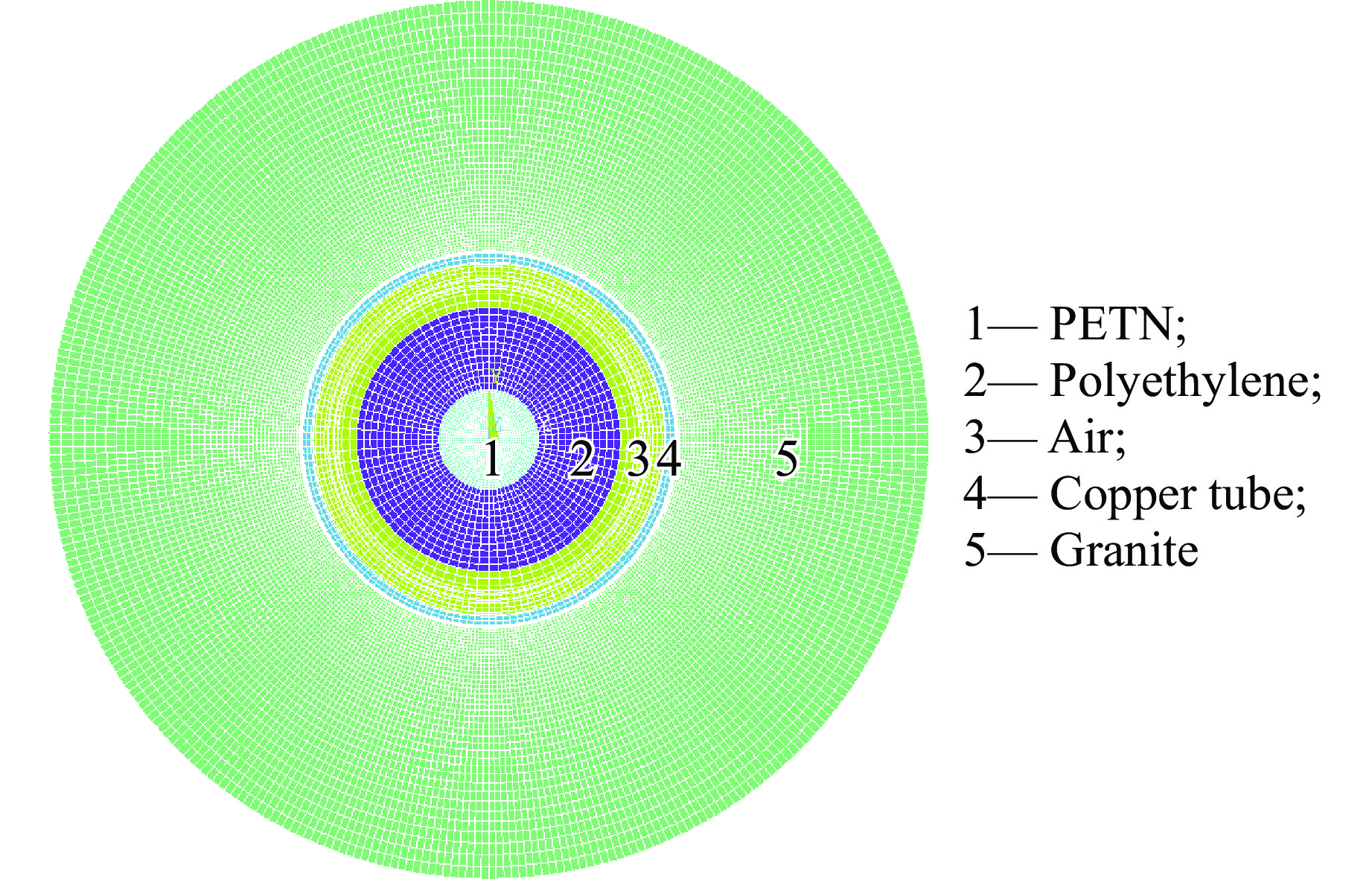
In consideration of the sizes of the cylindrical granite samples prepared for laboratory-scale blasting experiments by Dehghan Banadaki and Mohanty[17] (with a diameter of 144 mm, a height of 150 mm and a borehole diameter of 6.45 mm), a circular plane strain model with an outer diameter of 144 mm is made in our simulation, as shown in Fig.5, being a scaled close-up view of the borehole region. Multi-material Euler solver is used for modeling PETN explosive, polyethylene and air. Lagrangian descriptions are used for modeling the copper tube and granite.
The material model and the properties of PETN explosive, polyethylene, air and copper tube used in the simulation are given in Ref.[17]. The values of various parameters in the constitutive model for granite are listed in Table 1.
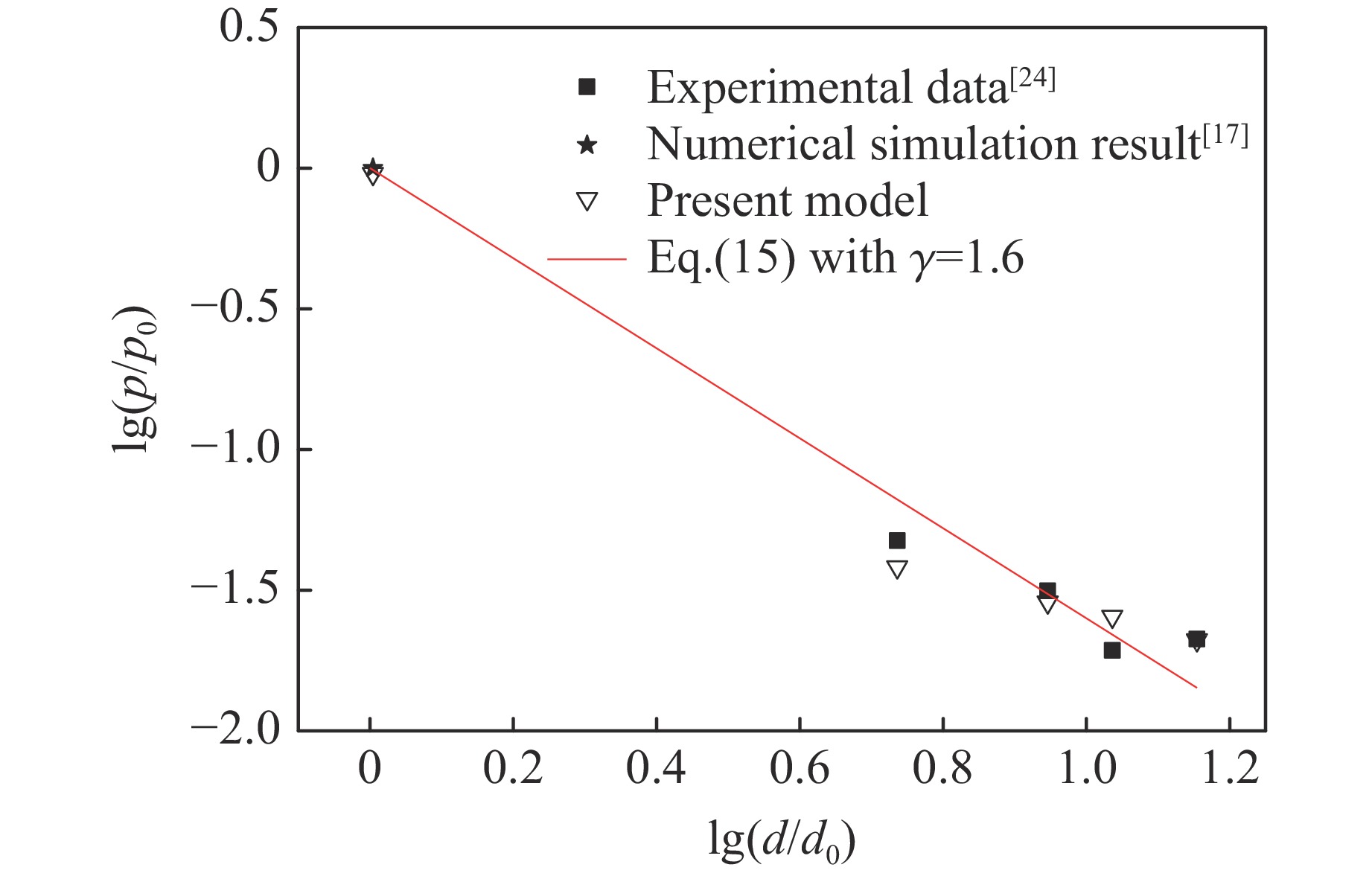
Fig.6 shows the comparison of the peak pressures between our simulation results of the present model, the numerical results[17], and the experimental results by Dehghan Banadaki and Mohanty[24]. It can be seen from Fig.6 that a good agreement is obtained.
In order to characterize the damping behavior of stress in granite, the peak pressure p in granite is expressed in an exponential form as
pp0=(dd0)−γ (15) where p0 is the peak pressure on the borehole wall, d0 is the initial radius of the borehole, d is the distance from the center point of the borehole,
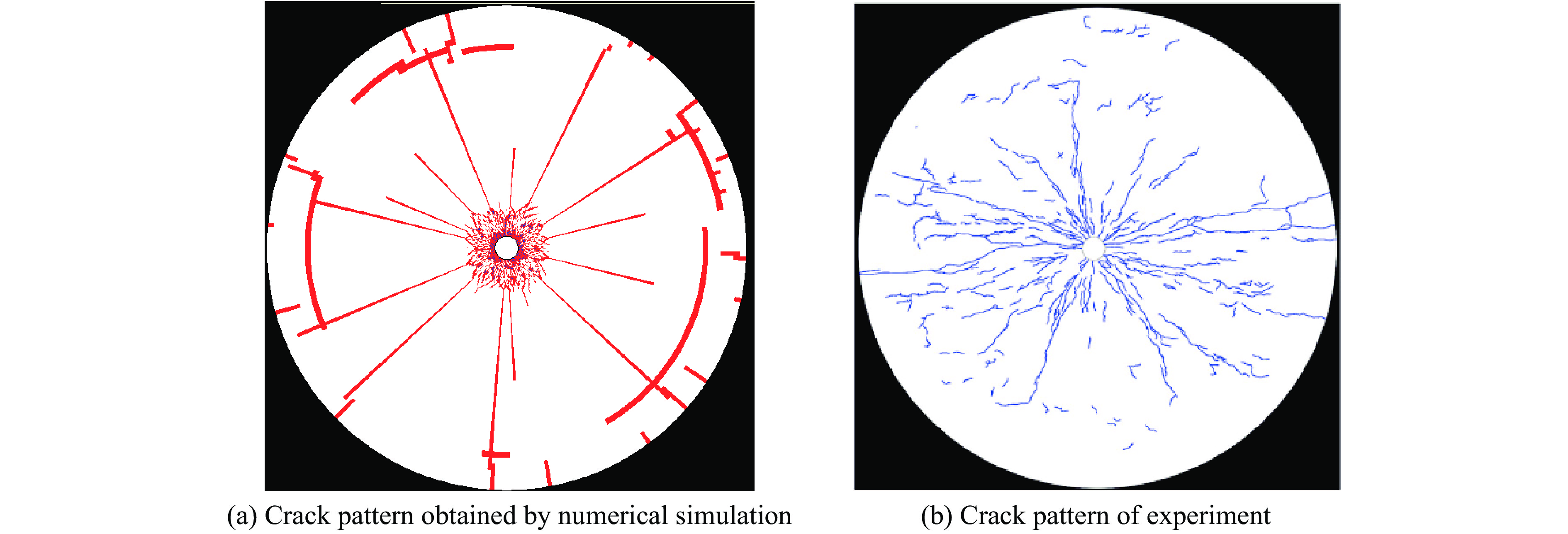
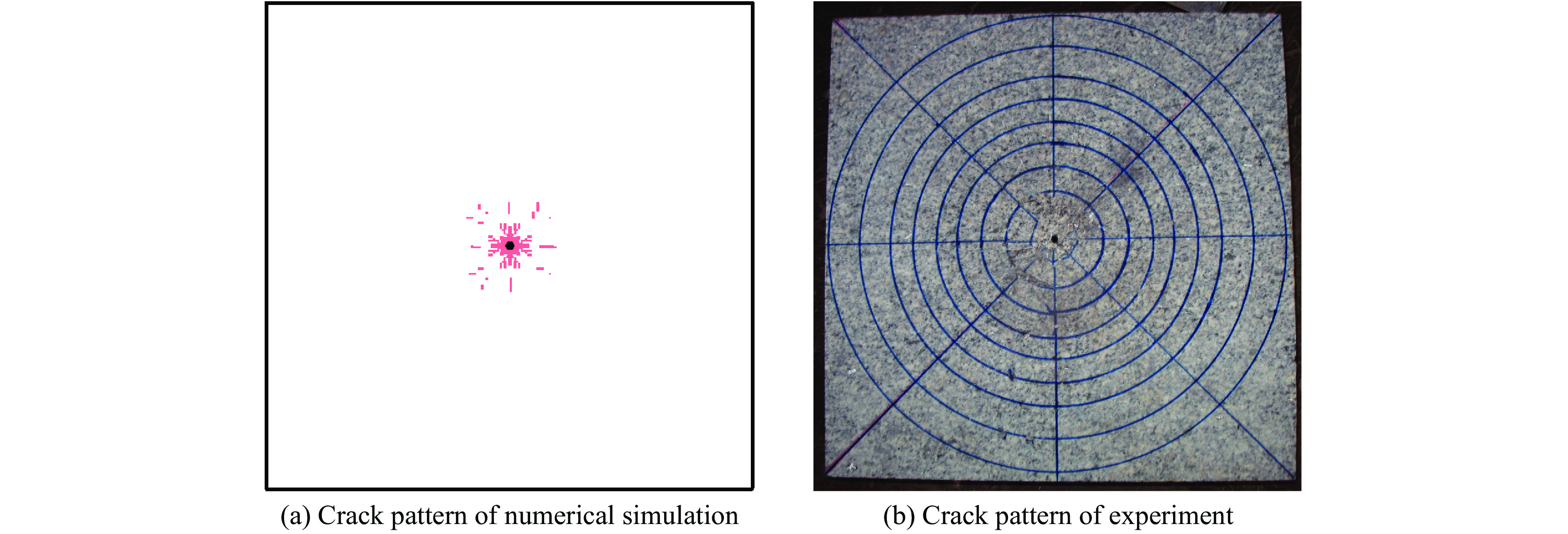
γ is an index. It is evident from Fig.6 that Eq.(15) withγ =1.6 correlates well with the experimental results.Fig.7 shows the comparison between the crack patterns predicted numerically based on the present model and the one observed experimentally in the cylindrical granite sample[17]. It is clear that a relatively good agreement on the crack pattern is obtained. It is also clear that the stress waves produce three distinct crack regions in the cylindrical granite sample: densely populated smaller cracks around the borehole, a few large radial cracks propagating towards the outer boundary, and circumferential cracks close to the sample boundary which are due to the reflected tension stress.
 Figure 7. Comparison of the crack patterns between the numerical prediction and the experiment of the cylindrical granite sample[17]
Figure 7. Comparison of the crack patterns between the numerical prediction and the experiment of the cylindrical granite sample[17]In order to make an assessment of the contributions of both the compression/shear stress and the tensile stress to the crack patterns, Fig.8 shows the numerically predicted crack pattern which results from the tension stress only. Fom Fig.8 and Fig.7(a), it is apparent that there are virtually few changes in crack patterns, both having the large radial and circumferential cracks caused by the same tensile stress, however, the smaller cracks around the borehole in Fig.8 are much less than those in Fig.7(a). In another word, crack patterns are mainly caused by tensile stress, and smaller cracks around borehole are created largely by compression/shear stress.
2.2.2 Square Granite Sample
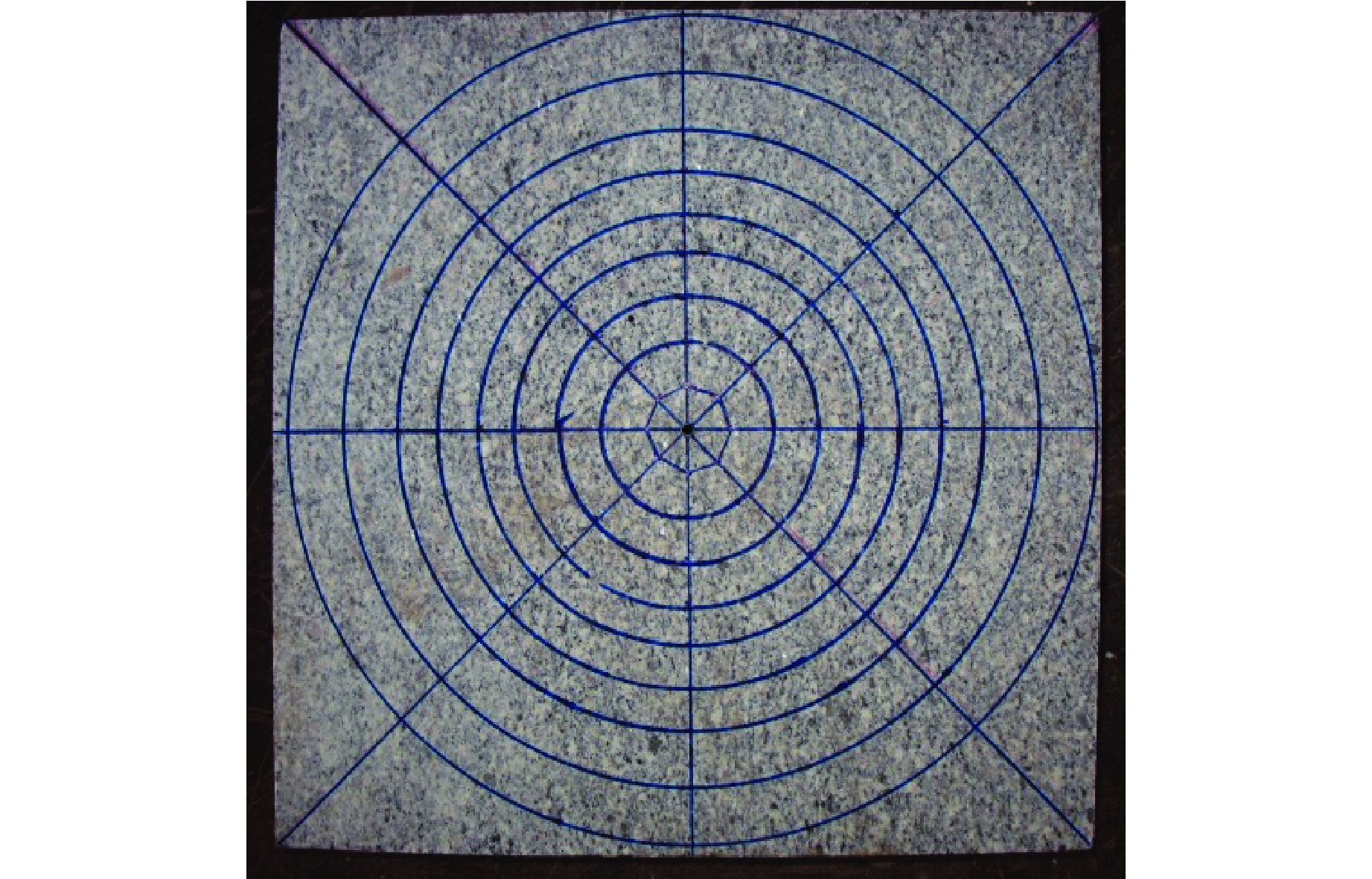
The laboratory-scale single-hole blasting tests are also carried out in order to validate further the accuracy and the reliability of the present model. Two square granite samples with a side length of 400 mm and a height of 100 mm are employed in the experiments. The borehole diameter is 4 mm and a series of concentric rings is drawn on the top surface of the samples (see Fig.9), so that the damage regions induced by detonation can be assessed visually.
A cylindrical RDX explosive enclosed by an aluminum sheath (see Fig.10) is tightly installed in the borehole of the No.1 sample, while an unwrapped RDX explosive is inserted into the borehole of the No.2 sample. The density of the RDX is 1700 kg/m3, and the material model and properties of the RDX explosive and the aluminum sheath used in the experiments are given in ref.[25]. The values of the various parameters in the constitutive model for granite are listed in Table 1.
Fig.11 and Fig.12 show the comparisons of the crack patterns between the numerical predictions from the present model and the ones observed experimentally in the square granite samples. It can be seen from Fig.11 and Fig.12 that good agreements are obtained. It should be mentioned here that No.1 sample receives less damage due to less RDX explosive used in the test, and that No.2 sample is broken up into four major pieces due to more RDX explosive employed in the experiment. Severe damages and small cracks are induced in the vicinity of the boreholes of both samples, as can be seen clearly from Fig.11(b) and Fig.12(b).
3. Conclusions
A numerical study on the borehole blasting-induced fractures in rocks is conducted in this paper, using a dynamic constitutive model developed previously for concrete. Two kinds of granite rocks are simulated numerically, one in the cylindrical form and the other in the square form. The numerical results are compared with the corresponding experiments. Main conclusions can be drawn as follows.
(1) The crack patterns predicted numerically from the present model are found to be in good agreement with the experimental observations, both in cylindrical and square granite samples subjected to borehole blasting loading.
(2) The peak pressures predicted numerically based on the present model are found to be in good agreement with the test data.
(3) Crack pattern observed experimentally in the rock sample is mainly caused by the tensile stress, while the smaller cracks in the vicinity of the borehole are created largely by compression/shear stress.
(4) The consistency between the numerical results and the experimental observations demonstrates the accuracy and reliability of the present model. Thus the model can be used in the numerical simulations of the response and the failure of rocks under blasting loading.
-
Figure 1. Schematic diagram of EOS[7]
Figure 3. Comparison of the strength surface between Eq.(6) (with B=2.59, N=0.66) and the triaxial test data for granite[17]
Figure 7. Comparison of the crack patterns between the numerical prediction and the experiment of the cylindrical granite sample[17]
p-α relation ρ0/(kg∙m−3) pcrush/MPa plock/GPa n K1/GPa K2/TPa K3/TPa 2660 50.5 3 3 25.7 −3 150 Strength surface Strain rate effect fc′/MPa ft/MPa B N G/GPa Fm Wx 161.5 7.3 2.59 0.66 21.9 10 1.6 Strain rate effect Shear damage Wy S ˙ε0/s−1 λs λm l r 5.5 0.8 1.0 4.6 0.3 0.45 0.3 Lode effect Tensile damage e1 e2 e3 c1 c2 εfrac 0.65 0.01 5 3 6.93 0.007 -
[1] GRADY D E, KIPP M E. Continuum modelling of explosive fracture in oil shale [J]. International Journal of Rock Mechanics and Mining Sciences & Geomechanics Abstracts, 1980, 17(3): 147–157. [2] ROSSMANITH H P, DAEHNKE A, KNASMILLNER R E, et al. Fracture mechanics applications to drilling and blasting [J]. Fatigue & Fracture of Engineering Materials & Structures, 1997, 20(11): 1617–1636. [3] ZHANG Y Q, HAO H, LU Y. Anisotropic dynamic damage and fragmentation of rock materials under explosive loading [J]. International Journal of Engineering Science, 2003, 41(9): 917–929. doi: 10.1016/S0020-7225(02)00378-6 [4] KUTTER H K, FAIRHURST C. On the fracture process in blasting [J]. International Journal of Rock Mechanics and Mining Sciences & Geomechanics Abstracts, 1971, 8(3): 181–202. [5] ZHU Z M, MOHANTY B, XIE H P. Numerical investigation of blasting-induced crack initiation and propagation in rocks [J]. International Journal of Rock Mechanics and Mining Sciences, 2007, 44(3): 412–424. doi: 10.1016/j.ijrmms.2006.09.002 [6] TU Z G, LU Y. Evaluation of typical concrete material models used in hydrocodes for high dynamic response simulations [J]. International Journal of Impact Engineering, 2009, 36(1): 132–146. doi: 10.1016/j.ijimpeng.2007.12.010 [7] XU H, WEN H M. A computational constitutive model for concrete subjected to dynamic loadings [J]. International Journal of Impact Engineering, 2016, 91: 116–125. doi: 10.1016/j.ijimpeng.2016.01.003 [8] TAYLOR L M, CHEN E P, KUSZMAUL J S. Microcrack-induced damage accumulation in brittle rock under dynamic loading [J]. Computer Methods in Applied Mechanics and Engineering, 1986, 55(3): 301–320. doi: 10.1016/0045-7825(86)90057-5 [9] HOLMQUIST T J, JOHNSON G R. A computational constitutive model for concrete subjected to large strains, high strain rates, and high pressures [C]//14th International Symposium on Ballistics. Quebec, 1993: 591−600. [10] RIEDEL W, THOMA K, HIERMAIER S, et al. Penetration of reinforced concrete by BETA-B-500 numerical analysis using a new macroscopic concrete model for hydrocodes [C]//Proceedings of the 9th International Symposium on the Effects of Munitions with Structures. Berlin: ISIEMS, 1999: 315−322. [11] MALVAR L J, CRAWFORD J E, WESEVICH J W, et al. A plasticity concrete material model for DYNA3D [J]. International Journal of Impact Engineering, 1997, 19(9/10): 847–873. [12] TU Z G, LU Y. Modifications of RHT material model for improved numerical simulation of dynamic response of concrete [J]. International Journal of Impact Engineering, 2010, 37(10): 1072–1082. doi: 10.1016/j.ijimpeng.2010.04.004 [13] KONG X Z, FANG Q, WU H, et al. Numerical predictions of cratering and scabbing in concrete slabs subjected to projectile impact using a modified version of HJC material model [J]. International Journal of Impact Engineering, 2016, 95: 61–71. doi: 10.1016/j.ijimpeng.2016.04.014 [14] KONG X Z, FANG Q, LI Q M, et al. Modified K&C model for cratering and scabbing of concrete slabs under projectile impact [J]. International Journal of Impact Engineering, 2017, 108: 217–228. doi: 10.1016/j.ijimpeng.2017.02.016 [15] XU L Y, XU H, WEN H M. On the penetration and perforation of concrete targets struck transversely by ogival-nosed projectiles—a numerical study [J]. International Journal of Impact Engineering, 2019, 125: 39–55. doi: 10.1016/j.ijimpeng.2018.11.001 [16] XU H, WEN H M. Semi-empirical equations for the dynamic strength enhancement of concrete-like materials [J]. International Journal of Impact Engineering, 2013, 60: 76–81. doi: 10.1016/j.ijimpeng.2013.04.005 [17] DEHGHAN BANADAKI M M D, MOHANTY B. Numerical simulation of stress wave induced fractures in rock [J]. International Journal of Impact Engineering, 2012, 40/41: 16–25. doi: 10.1016/j.ijimpeng.2011.08.010 [18] KHAN A S, IRANI F K. An experimental study of stress wave transmission at a metallic-rock interface and dynamic tensile failure of sandstone, limestone, and granite [J]. Mechanics of Materials, 1987, 6(4): 285–292. doi: 10.1016/0167-6636(87)90027-5 [19] CHO S H, OGATA Y, KANEKO K. Strain-rate dependency of the dynamic tensile strength of rock [J]. International Journal of Rock Mechanics and Mining Sciences, 2003, 40(5): 763–777. doi: 10.1016/S1365-1609(03)00072-8 [20] WANG Q Z, LI W, XIE H P. Dynamic split tensile test of flattened Brazilian disc of rock with SHPB setup [J]. Mechanics of Materials, 2009, 41(3): 252–260. doi: 10.1016/j.mechmat.2008.10.004 [21] CAI M, KAISER P K, SUORINENI F, et al. A study on the dynamic behavior of the Meuse/Haute-Marne argillite [J]. Physics and Chemistry of the Earth, Parts A/B/C, 2007, 32(8/9/10/11/12/13/14): 907−916. [22] KUBOTA S, OGATA Y, WADA Y, et al. Estimation of dynamic tensile strength of sandstone [J]. International Journal of Rock Mechanics and Mining Sciences, 2008, 45(3): 397–406. doi: 10.1016/j.ijrmms.2007.07.003 [23] ASPRONE D, CADONI E, PROTA A, et al. Dynamic behavior of a Mediterranean natural stone under tensile loading [J]. International Journal of Rock Mechanics and Mining Sciences, 2009, 46(3): 514–520. doi: 10.1016/j.ijrmms.2008.09.010 [24] DEHGHAN BANADAKI M M, MOHANTY B. Blast induced pressure in some granitic rocks [C]//Proceedings of the 5th Asian Rock Mechanics Symposium (ARMS-ISRM). Tehran: Curran Associates, 2008: 933−939. [25] GUO X J. A study of fracture mechanisms in brittle materials under borehole blasting [D]. Hefei: University of Science and Technology of China, 2013. -


推荐:氢氘物态方程研究进展(特约综述)
第五届高压科学卓越青年学者评选通知
2024年度《高压物理学报》优秀审稿人评选结果
2024年上海光源同步辐射大压机实验技术培训班通知
《高压物理学报》将于2025年1月由双月刊变更为月刊
动载下材料物性机器学习与高通量研究专刊征稿启事
《高压物理学报》门户网站和投审稿系统7月28日~8月6日期间暂停运行
《高压物理学报》第二届青年编委会招募启事
《高压物理学报》2023年度优秀审稿人和优秀论文评选结果
第十四届全国爆炸力学学术会议 第二轮通知
第九届“从原子到地球”——高压科学研讨会第三轮通知
第九届“从原子到地球”——高压科学研讨会第二轮通知
第四届全国超高速碰撞学术会议通知(第二轮)
第二十一届中国高压科学学术会议第一轮通知
通知
推荐:高压下Fe92.5O2.2S5.3的熔化温度(特约稿件)
推荐:透明陶瓷的超高压制备研究进展(特约综述)
推荐:氢的高压奇异结构与金属化(特约综述)
推荐:第二主族氢化物的高压研究(特约综述)
推荐:氢氘物态方程研究进展(特约综述)
第五届高压科学卓越青年学者评选通知







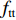
 DownLoad:
DownLoad:
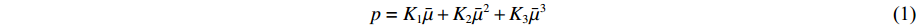
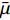


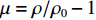
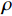
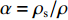
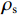
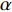
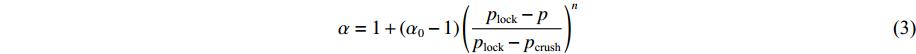
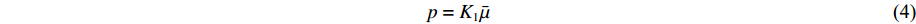
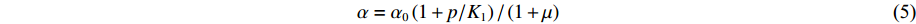

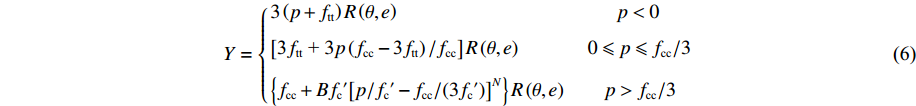
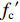
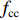


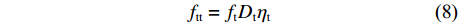
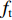
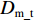
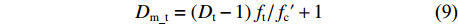
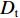
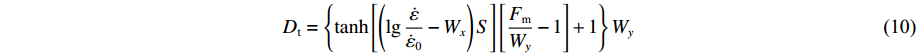
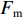
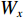
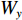

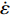
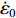
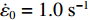
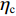
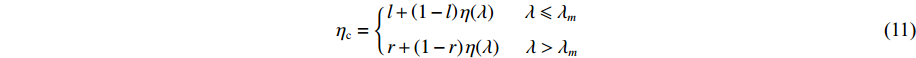
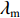
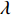
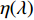
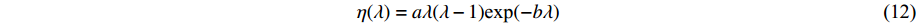
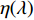
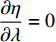
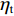
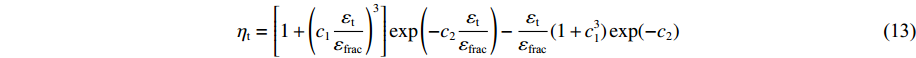
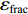
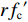
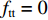
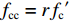
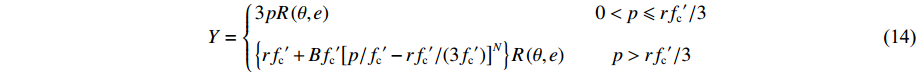
 DownLoad:
DownLoad:



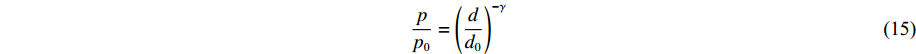







 下载:
下载: