Dynamic Response of Aluminum Foam Filled Pipes under Lateral Explosive Load
-
摘要: 采用数值模拟与理论分析相结合的方法,研究横向爆炸载荷下泡沫铝填充管的动态响应。利用有限元软件ABAQUS/EXPLICIT对横向爆炸载荷下泡沫铝填充管的塑性变形进行了数值模拟研究,分析了泡沫铝的相对密度、外管的直径与壁厚等因素对结构动态响应的影响。基于理想刚塑性地基梁模型,结合模态分析法,建立了预测横向爆炸载荷下泡沫铝填充管跨中挠度的理论分析模型,并进行了无量纲分析,给出了跨中无量纲挠度随无量纲冲量的变化规律。泡沫铝填充管跨中挠度的理论预测与数值模拟结果的误差在20%以内,表明所建立的理论分析模型合理可行。泡沫铝相对密度对横向爆炸载荷下填充管的跨中挠度有较大的影响,随着泡沫铝相对密度的增大,填充管跨中挠度减小。随着外管直径与壁厚的增大,跨中挠度减小。理论分析中,假设的两种模态函数对填充管跨中挠度的影响较小。Abstract: A combination of numerical simulation and theoretical analysis is used to study the dynamic response of foamed aluminum filled pipes under lateral explosion loads. The finite element software ABAQUS/EXPLICIT was used to carry out a numerical simulation study on the plastic deformation of the aluminum foam-filled tube under lateral explosive load, and the influence of the relative density of the foam aluminum, the diameter and wall thickness of the outer tube and other factors on the dynamic response of the structure was analyzed. Based on the ideal rigid-plastic foundation beam model, combined with the modal analysis method, a theoretical analysis model for predicting the mid-span deflection of the foamed aluminum filled pipe under lateral explosive load is established, and a dimensionless analysis is carried out. The change of the dimensionless deflection of the mid-span with the elementary impulse is obtained. The error between the theoretical prediction and the numerical simulation result of the foamed aluminum filled pipe mid-span deflection is within 20%, indicating that the established theoretical analysis model is reasonable and feasible. The relative density of foamed aluminum has a great influence on the mid-span deflection of the filled pipe under lateral explosive load. As the relative density of foamed aluminum increases, the mid-span deflection of the filled pipe decreases. As the diameter and wall thickness of the outer tube increase, the mid-span deflection decreases. The two modal functions assumed in the theoretical analysis have little effect on the mid-span deflection of the filled pipe.
-
Key words:
- beam-on-founding /
- transverse explosion load /
- aluminum foam filled pipe /
- modal solution
-
泡沫铝填充管因具有构造和加工技术简单、生产成本低且效率高等优点,被广泛应用在各种类型工程结构中。在实际工程中,填充管会承受各种类型的冲击载荷,甚至爆炸载荷,因此冲击载荷下填充管的安全性受到了越来越多的关注,填充管的动态响应引起了国内外学者的研究兴趣。
基于空管的特点及其在工程中的广泛使用,学者们开始了对空管的研究。根据大量爆炸实验,Henrych[1]建立了经验公式,对TNT炸药在空气中爆炸形成的冲击波压力峰值进行理论分析。Wierzbicki等[2]基于地基梁模型,考虑圆柱壳在冲击和爆炸载荷作用下产生轴向拉伸与圆周方向弯曲的特点,提出了研究壳的动态响应问题的分析方法。于博丽等[3]基于地基梁模型,采用模态分析法,建立了横向爆炸载荷下圆柱壳跨中挠度的理论分析模型,实验研究与数值模拟验证了该模型的合理性,结果表明圆柱壳的长径比、厚度对圆柱壳的变形模式有较大影响。Li等[4]通过实验研究了横向爆炸载荷下圆柱壳的变形模式,包括局部塑性变形、菱形形状的大的塑性变形与大的非弹性变形。基于圆的变形模式,使用刚塑性分析法,建立了圆柱壳跨中挠度的理论分析模型,解析解小于最终跨中挠度。Brochard等[5]基于理想刚塑性地基梁模型,提出了一种可以预测水下爆炸产生的冲击波致使圆柱壳破坏的分析方法。Yuen等[6]通过对外部集中爆炸载荷作用下的圆柱壳进行实验和数值模拟,验证了地基梁模型分析其动态响应的合理性。Karagiozova等[7]将爆炸冲击近似为脉冲载荷,采用刚塑性分析法,研究了冲击载荷作用下空心梁的变形与能量吸收特点。Walters等[8]使用刚塑性分析法提出了可预测任意形状的壳结构动态塑性响应的理论方法,证明了爆炸载荷作用下夹支圆柱壳的塑性响应与均布压力脉冲载荷作用下圆柱壳的塑性响应基本一致。国内外学者对爆炸载荷下圆柱壳的动态响应进行了分析,发现圆柱壳的抗冲击性较差,该结构在实际工程中较难满足安全性要求。
与空管相比,填充管因显著提高了诸多力学性能而吸引了学者的广泛关注。Wang等[9]基于三相法建立了横向冲击下预测填充管冲击力与位移响应的理论分析模型,实验与数值模拟验证了该理论分析模型的合理性。Hall等[10]通过实验对泡沫铝填充管进行准静态与动态加载,研究了填充管的横向与轴向压溃,结果表明,与空管相比,填充管的能量吸收能力显著增加。Wang等[11]实验研究了爆炸载荷下混凝土填充管的抗爆防护性能,分析了炸药用量、钢管壁厚及横截面形状对结构动态响应的影响。Zhang等[12]结合数值模拟与实验研究了静态与动态载荷下混凝土填充管的弯曲行为,结果表明该结构具有良好的抗弯曲性能。Yousuf等[13]采用数值模拟与实验研究了横向冲击载荷下空心管与混凝土填充管的力学性能,发现与空管相比,填充管具有更高的强度和延展性,能量耗散能力有了显著提高。邓旭辉等[14]采用数值模拟研究了近爆载荷下钢管混凝土柱的损伤机理、能量吸收特性与影响参数。
综上所述,已有的研究主要集中于填充管的数值模拟与实验研究,结果表明,与空管相比,填充管具有更优异的能量吸收能力和抗爆性能。但是填充管在横向爆炸载荷下动态响应的理论分析尚未见报道。为探明横向爆炸载荷下泡沫铝填充管的动力特性与变形机理,采用数值模拟与理论分析相结合的方法研究其动态响应。利用ABAQUS/EXPLICIT建立泡沫铝填充管的有限元模型,分析填充管的几何参数与泡沫铝的相对密度对结构塑性变形的影响。基于理想塑性地基梁模型,结合模态分析法,建立预测爆炸载荷下泡沫铝填充管跨中挠度的理论分析模型,并进行无量纲化分析。对比理论分析与有限元模拟结果,进一步探究填充管的几何参数、爆炸载荷参数、泡沫铝相对密度与模态函数等因素对其跨中挠度的影响。
1. 数值模拟
1.1 爆炸载荷
根据Henrych[1]的研究可知,炸药爆炸产生的反射爆炸超压
Δpφr 可由炸药量W 与炸药距结构的距离H得到Δpφ=6.1938¯H−0.3262¯H2+2.1324¯H3(0.3⩽¯H⩽1.0) (1) ¯H=H3√W (2) Δpφr=8Δp2φ+14ΔpφΔpφ+7.2(Δpφ⩽40kg/cm2) (3) 式中:
Δpφ 为爆炸形成的超压,¯H 为比例距离,W=35 g[3],H=150 mm[3]。由反射爆炸超压可得爆炸载荷峰值
p0 为p0=Δpφr×10−1(MPa) (4) 假设爆炸载荷的近似方程[15]为
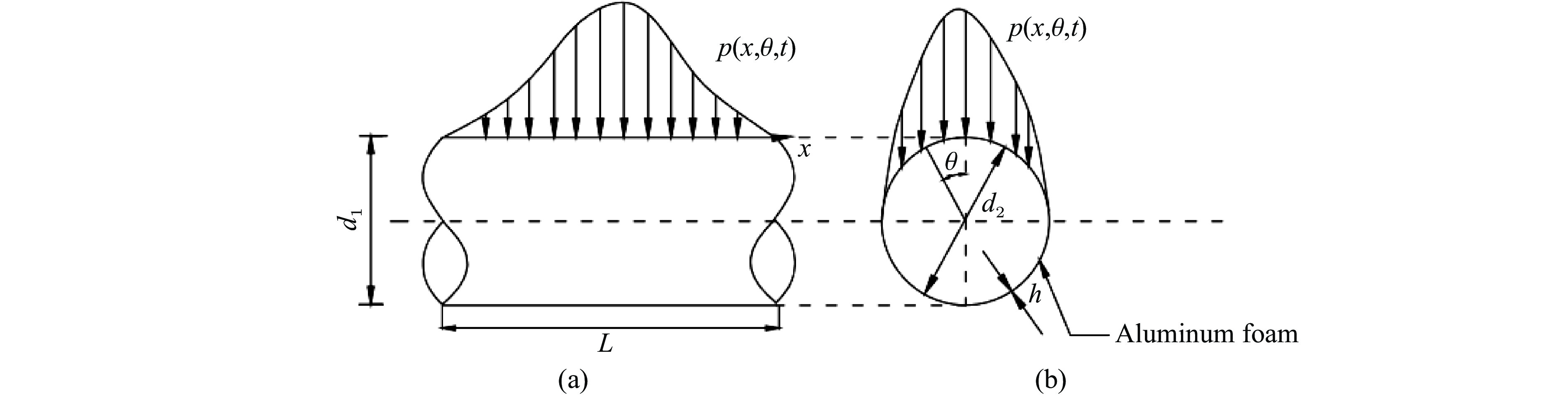
p(x,θ,t)=p0e−tτf(x)g(θ) (5) 式中:
τ 为衰减常数,且τ=50μs [16];f(x)、g(θ) 分别为轴向与周向的初始压力分布函数,并且f(x)= cos2(πxL) [2],g(θ)=sinθ [7],L =280 mm,为填充管的长度。由此得到横向爆炸载荷
p(x,θ,t)=p0e−tτcos2(πxL)sinθ (6) 式中:
0⩽x⩽L/2 ,0⩽θ⩽π/2 。填充管承受轴向应力作用如图1(a)所示,实心泡沫铝填充管承受径向应力作用如图1(b)所示,爆炸载荷作用的长度为泡沫铝与薄壁圆管的长度L,d2为填充实心泡沫铝的直径。1.2 材料参数
泡沫铝填充管是由不锈钢管与相对密度为8%、12%与20%的泡沫铝组成。不锈钢管的密度为7830 kg/m3,弹性模量为193 GPa,泊松比
μ 为0.25,屈服应力为205 MPa。当应变为0.4时,极限抗拉强度σUTS=520MPa [3]。填充管的壁厚分别为0.7、0.8 mm,外径分别为76、89 mm。泡沫铝与不锈钢管的长度均为280 mm,泡沫铝参数[17]如表1所示,其中:E为弹性模量,σs为圆管的屈服应力。1.3 数值模型
采用有限元软件
ABAQUS/EXPLICIT 对3种相对密度泡沫铝填充的不同壁厚有限长不锈钢管在横向爆炸载荷作用下的动态响应进行数值模拟分析。根据式(4)得到的爆炸载荷峰值,在填充管的上半部作用如式(6)所示的爆炸载荷,分析其动态响应。不锈钢管采用S4R单元,材料模型采用Cowper-Symonds本构方程,其中不锈钢管应变率敏感率D=100 s−1,参数q=10[18]。考虑到泡沫铝对应变率效应不敏感,为此材料模型采用双线性弹塑性模型,选用C3D8R单元。该爆炸载荷作用在结构上产生的效果与实际爆炸载荷作用下两端固定的结构产生的效果相同,因此边界条件为填充管的两端固定,圆管的内表面与泡沫铝的外表面绑定,且为通用接触。1.4 网格敏感性验证
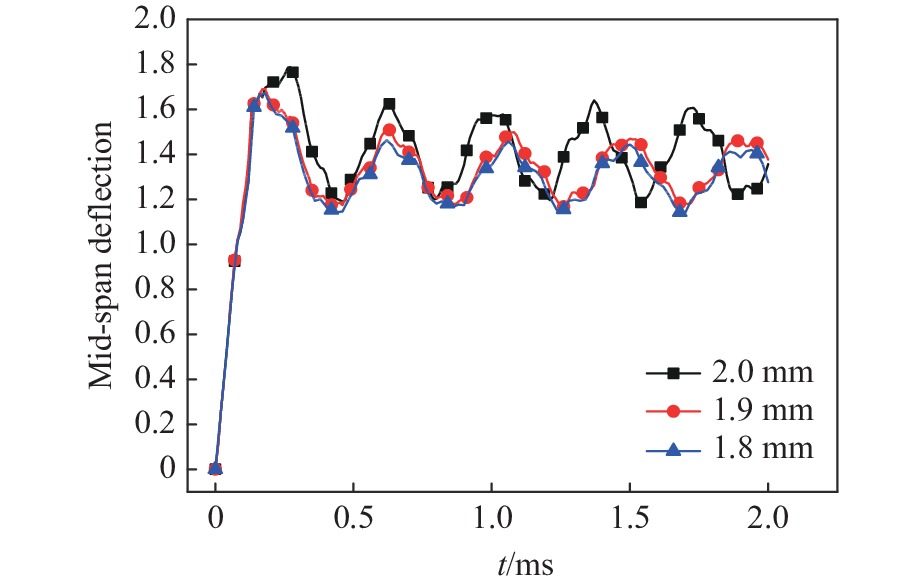
网格尺寸大小对数值模拟结果具有重要的影响。以外径为89 mm、壁厚为0.7 mm、填充相对密度为12%的填充管为研究对象,网格尺寸分别取1.8、1.9与2.0 mm进行数值模拟分析,研究网格尺寸对填充管跨中挠度的影响,结果如图2所示。由图2可知,网格尺寸小于1.9 mm时计算结果收敛。为了节省计算时间,本数值模拟采用1.9 mm的网格尺寸。
1.5 数值模拟结果与讨论
根据本研究的药量与距离,得到爆炸载荷峰值为23.6 MPa。在该爆炸载荷作用下,泡沫铝填充管跨中挠度随着时间的增加而增加,当达到最大值后,跨中挠度的数值模拟结果随着时间的增加而小范围波动,将这一阶段的挠度取平均值作为数值模拟结果。表2给出了2种外管直径、2种外管壁厚、3种泡沫铝相对密度共计12个填充管的数值模拟结果,其中W为跨中挠度,下标theor和sim分别表示理论解和数值模拟结果。由表2可知:外管的外径d1与壁厚h一定时,填充管跨中挠度随着泡沫铝相对密度的增大而减小;外管的壁厚与泡沫铝相对密度一定时,填充管跨中挠度随着外径的增大而减小;当外管的外径与泡沫铝相对密度一定时,填充管跨中挠度随着壁厚的增大而减小。
表 2 实心泡沫铝填充管跨中挠度的模态解与数值模拟结果对比Table 2. Comparison of mid-span deflection of foamed aluminum filled tubes of modal solutions and numerical simulation resultsd1/mm h/mm Relative density/% Wtheor/mm Wsim/mm Wsim−WtheorWtheor/% 76 0.7 8 3.0 3.1 3 12 1.6 1.7 6 20 0.7 0.8 14 0.8 8 2.8 2.9 4 12 1.4 1.5 7 20 0.6 0.7 17 89 0.7 8 2.5 2.7 8 12 1.3 1.4 8 20 0.5 0.6 20 0.8 8 2.3 2.3 0 12 1.2 1.2 0 20 0.5 0.5 0 2. 理论分析
2.1 初始速度
在爆炸载荷作用下,刚塑性结构通常会先发生瞬态响应,后出现模态响应。在瞬态响应阶段,由于结构的变形随时间不断发生显著的变化,由此产生的速度场和加速度场的大小和分布都在不断地变化,因此精确分析结构的瞬态响应具有很大的难度。在模态响应阶段,可以认为结构的变形不发生变化,速度场的分布形式不发生变化,若不考虑几何大变形,加速度场也保持不变,因此刚塑性结构做匀减速运动。考虑到结构的塑性变形与破坏发生在瞬态冲击阶段,模态响应阶段处于衰减阶段,基于模态响应阶段的特征,提出模态分析法,在实现简化分析的同时,得到的模态解趋同于瞬态冲击的完全解。采用理想刚塑性地基梁模型,得到填充管跨中挠度的模态解,研究横向爆炸载荷下有限长泡沫铝填充管的动态响应问题[18]。
基于填充管结构与施加载荷左右对称的特点,以填充管的一半作为研究对象,假设填充管变形的模态函数为
ϕ∗1=1−2xL ,则初始速度场为v01(x,θ)=v0f∗(x)g∗(θ)=v0(1−2xL)sinθ (7) 式中:
0⩽x⩽L/2 ;0⩽θ⩽π/2 ;v0 为填充管跨中的初始速度;f∗(x)、g∗(θ) 分别为轴向与周向初始速度场函数,且f∗(x)=1−2xL ,g∗(θ)=sinθ 。在爆炸载荷作用下,填充管承受的横向动量为
Ω1=4∫L20∫π20mA⋅d12⋅v0(1−2xL)sinθdxdθ+2∫L20mLf⋅v0(1−2xL)dx (8) 式中:
mLf=π8ρfd22 ,为实心泡沫铝上半部分单位长度的质量,ρf 为泡沫铝的密度;mA=ρh 为不锈钢圆管上半部分单位面积的质量,ρ 为不锈钢圆管的密度,h为不锈钢圆管的壁厚。发生爆炸后,填充管受到的脉冲
IT=∫L0∫π0∫∞0p(x,θ,t)⋅d12dxdθdt (9) 根据
IT=Ω1 ,填充管跨中的初始速度可以表示为v01=p0d1τρhd1+π8ρfd22 (10) 2.2 跨中挠度的模态解
根据模态解初始速度的定义,可得
w01=∫vρv0f∗ϕ∗idv∫vρϕ∗iϕ∗idv=2∫L20mLv0(1−2xL)2dx+2∫L20mLfv0(1−2xL)2dx2∫L20mL(1−2xL)2dx+2∫L20mLf(1−2xL)2dx (11) 式中:
mL=12πd1hρ ,为圆管上半部分单位长度的质量。基于模态分析法,认为加速度大小不变,可以表示为
a=∫VQ∗jk∗jdV∫Vρϕ∗iϕ∗idV (12) 式中:
Q∗j 为广义应力,弯矩Q∗1=M ,轴力Q∗2=N ,地基反力Q∗3=q ;k∗j 与广义应变率˙qj 有关,且˙qj=˙w∗(t)k∗j(x) ,其中速度˙w∗(t) 是时间t的线性函数。若广义应力为弯矩,则转动角速度
θ 等于广义应变率,且θ=2˙w∗(t)/L ,结合˙qj=˙w∗(t)k∗j(x) ,˙q3= ˙w∗(t)(1−2xL) ,得k∗1(x)=2L (13) k∗3(x)=1−2xL (14) 广义应变率与轴向应变率满足如下关系[3]
˙q1˙q2=N2p2NMp (15) 式中:
Mp=43σs(d22+h)3+43(σsf−σs)(d22)3 ,为泡沫铝填充管的塑性极限弯矩,σs 为圆管的屈服应力,σsf 为泡沫铝的屈服应力;Np 为轴力。整理式(13)和式(15),
k∗2(x) 可以表示为k∗2(x)=4NMpLN2p (16) 对于横向爆炸载荷作用下有限长泡沫铝填充管的动态响应,采用如图3所示的地基梁模型进行分析。
假设地基梁模型满足如下式所示的屈服准则
|MMp|+|NNp|2=1 (17) 基于理想塑性地基上刚塑性梁的屈服条件[8],假设
M=0 ,N=Np ,q=16M0/d1 ,其中,M0=14σsh2+ 14σsfd22 ,为实心泡沫铝填充管的单位长度的塑性极限弯矩,则加速度可以表示为a∗=3(16Mp/L+8M0L/d1)(mL+mLf)L (18) 结构的总响应时间为
t∗f=w0∗a∗ ,结合Wf∗=12w0∗t∗f ,可得填充管跨中的最大挠度Wf1=p02τ2L2d31(mL+mLf)48(ρhd1+π8ρfd22)2(2Mpd1+M0L2) (19) 式(19)表明,横向爆炸载荷下填充管跨中的最大挠度与填充管的几何参数、材料参数与加载参数有关,以下研究中跨中最大挠度均称为跨中挠度。
3. 结果与讨论
3.1 跨中挠度的模态解与数值模拟结果的对比
对泡沫铝相对密度分别为8%、12%和20%,外管壁厚分别为0.7、0.8 mm,外管直径分别为76、89 mm,长度为280 mm的填充管进行理论分析。利用第2节推导的式(19)计算泡沫铝填充管在横向爆炸载荷下的跨中挠度,计算结果如表2所示。由表2可知,泡沫铝填充管的外径与壁厚一定时,随着泡沫铝相对密度的增大,填充管的跨中挠度减小。根据文献[3],爆炸载荷下薄壁圆管的跨中挠度的模态解与数值模拟解的相对偏差最大,达到41%,而在表2中,跨中挠度的模态解与数值模拟结果的最大相对偏差为20%,可以认为两者之间具有较好的一致性。对于外径为89 mm、壁厚为0.8 mm、填充3种相对密度的泡沫铝填充管而言,跨中挠度的模态解比数值模拟结果略小,当保留小数点后一位时,相对偏差为零。
3.2 跨中挠度的无量纲化
基于无量纲理论,分析填充管的长径比(1/λ)、径厚比(1/η)对填充管跨中无量纲挠度(
¯W )的影响。将冲量无量纲化In=8IT(4πρd1Lh+πρfd22L)√σs/ρ (20) 令
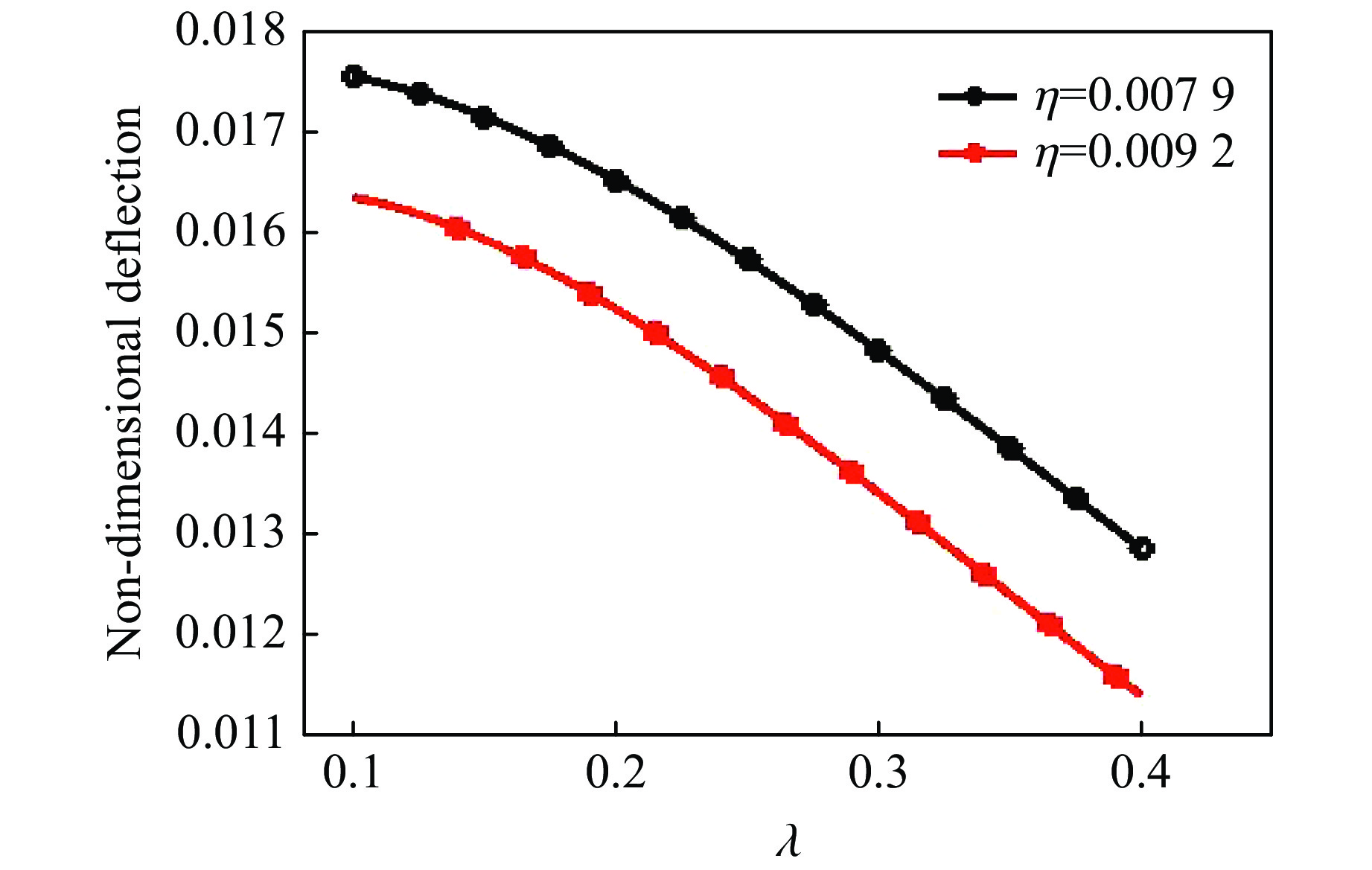
λ=d1L ,η=hd1 ,α=ρfρ ,β=σsfσs ,则跨中无量纲挠度可表示为¯W=Wf1d1=π3[4λη+α(λ−2η)2]38[8λη+πα(λ−2η)2]2[4λ4+4η2+3(β−1)(λ−2η)3λ+3β(λ−2η2)]I2n (21) 当In=0.18、α=0.022、β=0.042(填充相对密度为12%的泡沫铝)时,研究填充管的长径比、径厚比对填充管跨中挠度的影响。长径比对填充管跨中挠度的影响如图4所示,分析可知跨中无量纲挠度随着无量纲量
λ 的增大而减小。填充管的壁厚比对跨中挠度的影响如图5所示。由图5可知,跨中无量纲挠度随着径厚比的减小而减小。3.3 理论分析与数值模拟结果的无量纲化对比
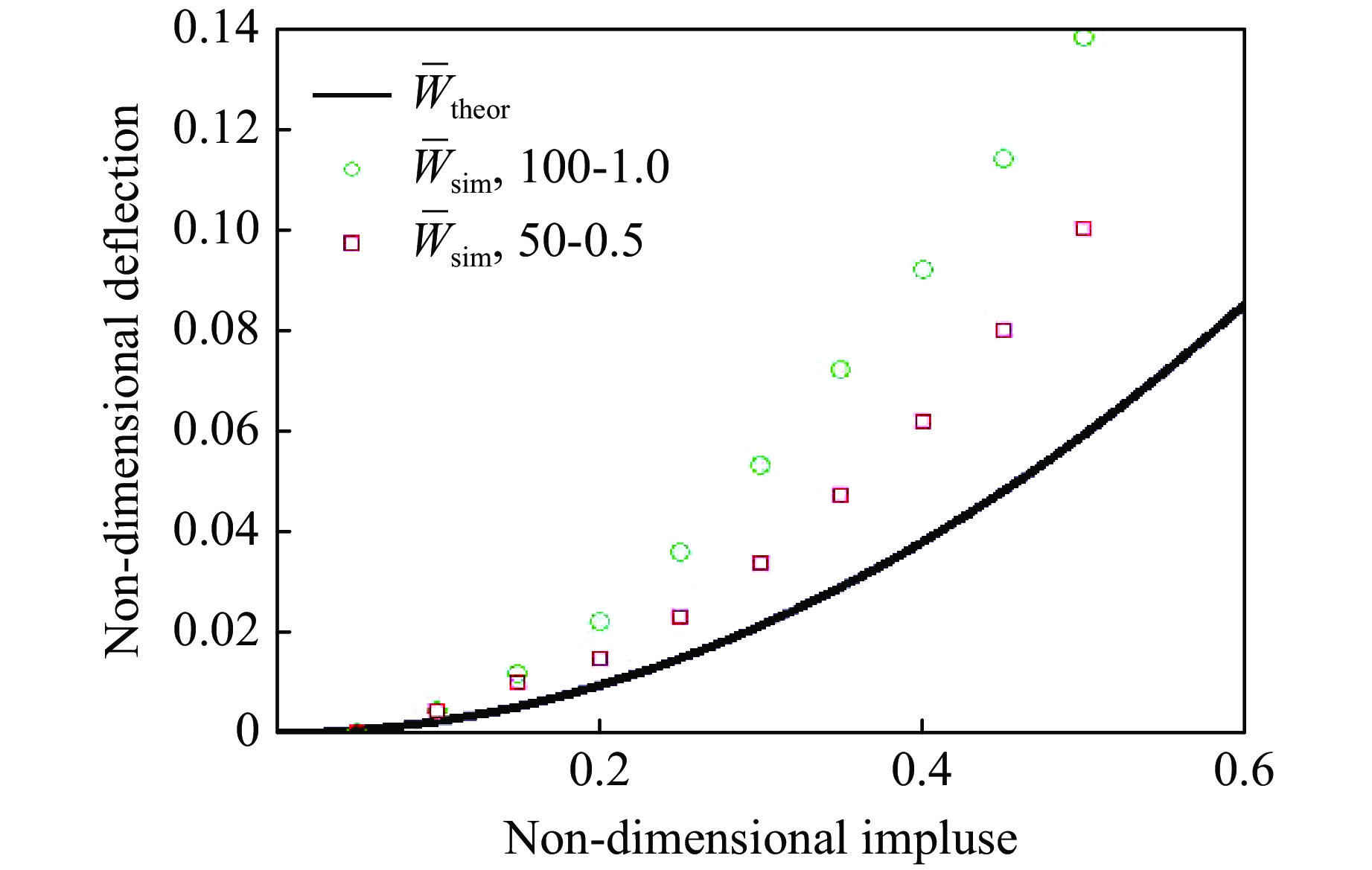
选择外管尺寸为d1=100 mm、L=300 mm、h=1.0 mm和d1=50 mm、L=150 mm、h=0.5 mm两种填充管,填充泡沫铝的相对密度为12%,对应的无量纲参数λ=1/3,η=0.01,进行理论分析和数值模拟。利用式(21)对上述两种填充管进行计算,得到跨中无量纲挠度,如图6所示。图6中,
¯Wtheor 为理论无量纲挠度,¯Wsim 为数值模拟无量纲挠度,100-1.0表示外径为100 mm、壁厚为1.0 mm的实心泡沫铝填充管,50-0.5表示外径为50 mm、壁厚为0.5 mm的实心泡沫铝填充管。将式(6)、式(9)代入式(20),得到爆炸载荷峰值,其表达式为
p0=(4πρd1Lh+πρfd22L)√σs/ρ4d1LτIn (22) 对上述两种尺寸的填充管施加如式(22)所示的爆炸载荷峰值,并对其进行数值模拟,模拟结果如图6所示。
由图6可知,填充管的跨中无量纲挠度随着无量纲冲量的增大而增大。当无量纲冲量较小时,跨中挠度的解析解与数值模拟结果吻合较好;当无量纲冲量较大时,跨中无量纲挠度的模态解与数值模拟结果的相对偏差较大。原因是爆炸产生的无量纲冲量越大,结构产生的塑性变形越大,跨中挠度越大,而膜力做功所占的比例随着跨中挠度的增大而增大;在跨中挠度模态解的分析过程中,膜力做功与塑性极限弯矩有关,导致跨中挠度的模态解小于数值模拟结果。
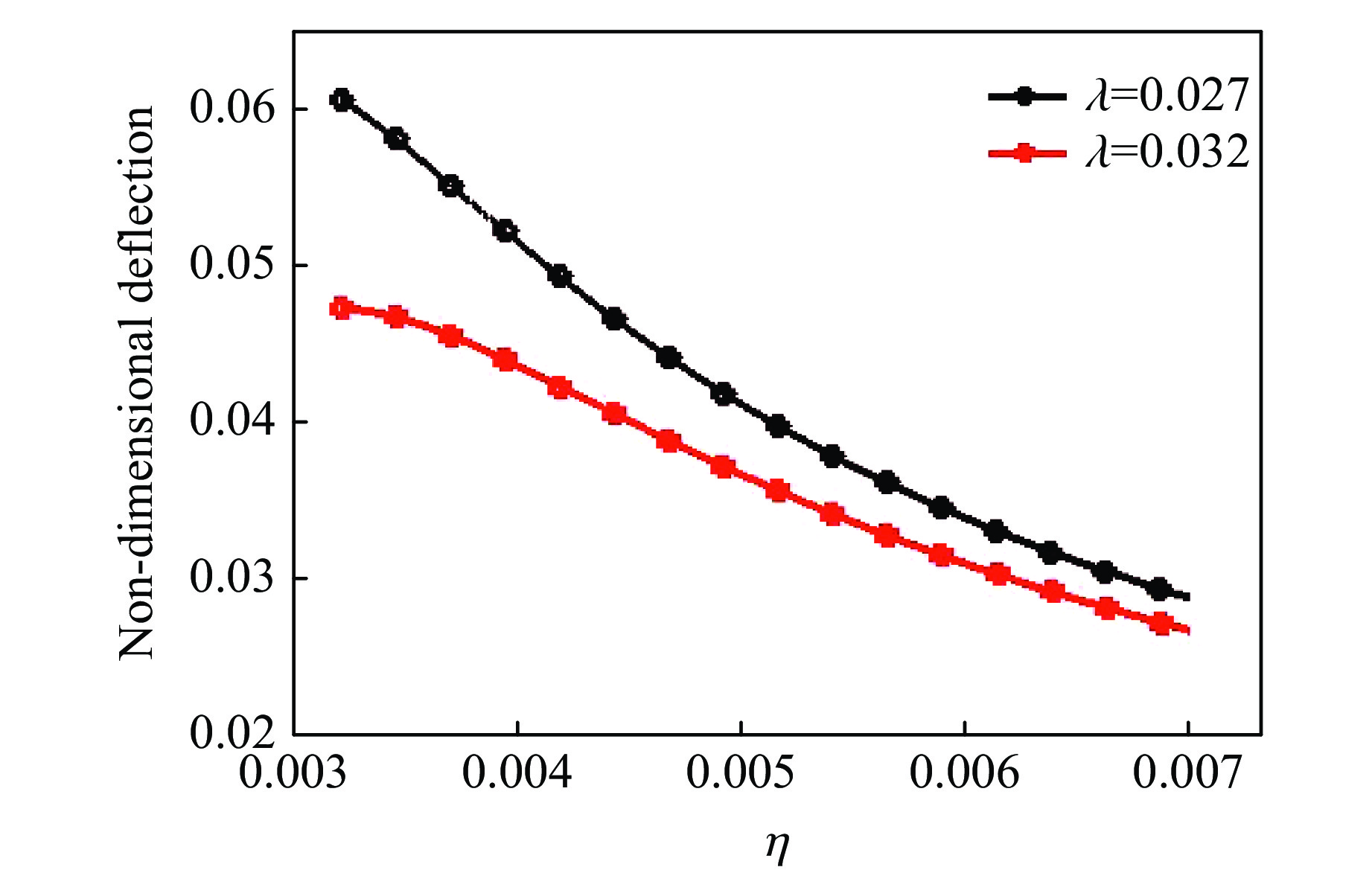
3.4 泡沫铝相对密度对跨中挠度的影响
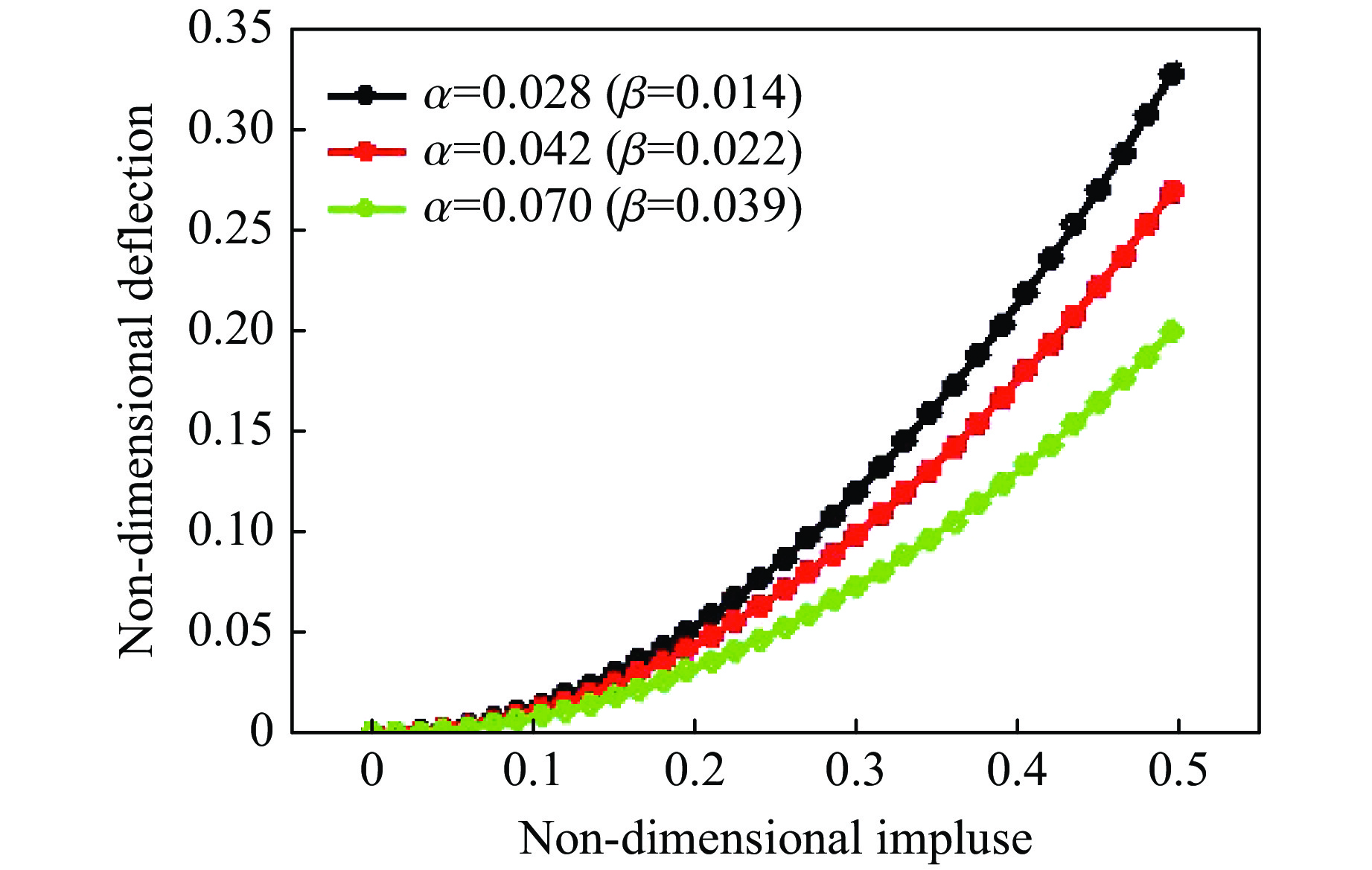
以外管直径76 mm、壁厚0.7 mm、长度280 mm(对应的无量纲参数为
λ=0.27 ,η=0.0092 )的填充管为研究对象,利用式(21)分析无量纲密度 (无量纲屈服应力 )对填充管跨中无量纲挠度的影响。图7给出了3种无量纲密度α=0.028 (β =0.014)、α=0.042 (β =0.022)与α=0.070 (β =0.039)填充管的跨中无量纲挠度随无量纲冲量的变化曲线。由图7可知,在横向爆炸载荷作用下,泡沫铝的相对密度对填充管跨中挠度具有显著的影响。当无量纲冲量一定时,
α=0.028 对应的无量纲挠度最大,α=0.070 对应的无量纲挠度最小,即填充管跨中挠度随着泡沫铝相对密度的增大而减小。3.5 爆炸载荷峰值
p0 与衰减常数τ 对跨中挠度的影响以外管直径76 mm、壁厚0.7 mm、相对密度为12%的泡沫铝填充管为研究对象,在给定的3种无量纲挠度(
¯W=0.02, 0.10, 0.50 )下研究爆炸载荷峰值p0与衰减常数τ 对跨中挠度的影响。基于¯W=Wf1/d1 ,结合式(19),可以得到爆炸载荷峰值与无量纲挠度的关系p0=[48(ρhd1+π8ρfd22)2(2Mpd1+M0L2)¯W]τLd1(mL+mLf)1212 (23) 从式(23)可以看出,爆炸载荷峰值p0与衰减常数
τ 成反比,即对于给定的无量纲挠度,爆炸载荷峰值随着衰减常数的增大而减小。图8为无量纲挠度分别为0.02、0.10、0.50时的3条p0-τ 曲线。由图8可知:当衰减常数τ 一定时,填充管跨中挠度随着爆炸载荷峰值p0的增加而增加;当爆炸载荷峰值p0一定时,跨中挠度随着衰减常数τ 的增加而增加。3.6 模态函数对跨中挠度的影响
采用模态分析法求解填充管跨中挠度的过程中,速度场与模态函数相关,因此模态函数与跨中的初始速度、模态解初始速度及加速度密切相关。假设模态函数为余弦函数,与第2节中的结果进行对比,分析模态函数对结果的影响。
假设填充管的模态函数为
ϕ∗2(x)=cos(πxL) ,则速度场为v02(x,θ)=v0cos2(πxL)sinθ (24) 作用在填充管上的横向动量
Ω2=4∫L20∫π20mA⋅d12⋅v0cos2(πxL)sinθdxdθ+2∫L20mLf1⋅v0cos2(πxL)dx (25) 根据
IT=Ω2 ,得到填充管跨中的等效初始速度为v02=p0d1τρhd1+π8ρfd22 (26) 该模态函数对应的模态解的初始速度
w02=2∫L20mLv0cos2(πxL)cos(πxL)dx+2∫L20mLf1v0cos2(πxL)cos(πxL)dx2∫L20mLcos2(πxL)dx+2∫L20mLf1cos2(πxL)dx (27) 对应的加速度为
a∗=32Mp/L+64M0L/(πd1)(mL+mLf)L (28) 采用该模态函数得到的跨中挠度的表达式为
Wf2=p20τ2L2d31(mL+mLf)9π(ρhd1+π8ρfd22)2(Mpπd1+2M0L2) (29) 将填充管的几何参数与材料参数代入式(29)可得到填充管的跨中挠度。表3给出了两种模态函数下不同泡沫填充管的跨中挠度与误差分析。由表3可知,两种模态函数假设下泡沫铝填充管的跨中挠度均随泡沫铝相对密度的增大而减小,随外管壁厚和直径的增大而减小。由余弦形式的模态函数得到的跨中挠度小于由线性分布的模态函数得到的结果。假设两种模态函数得到的跨中挠度的相对偏差在14%以内,则模态函数对最终跨中挠度的影响较小。
表 3 两种模态函数跨中挠度的对比Table 3. Comparison of mid-span deflection corresponding to two modal functionsd1/mm h/mm Relative density/% W/mm Relative error/% ϕ∗2(x)=1−2x/L ϕ∗2(x)=cos(πx/L) 76 0.7 8 3.0 2.8 −7 12 1.6 1.4 −13 20 0.7 0.6 −14 0.8 8 2.8 2.6 −7 12 1.4 1.4 0 20 0.6 0.6 0 89 0.7 8 2.5 2.4 −4 12 1.3 1.2 −8 20 0.5 0.5 0 0.8 8 2.3 2.2 −4 12 1.2 1.1 −8 20 0.5 0.5 0 4. 结 论
(1) 利用有限元软件ABAQUS/EXPLICIT对横向爆炸载荷下泡沫铝填充管的塑性变形进行了数值模拟分析,研究了泡沫铝的相对密度、外管直径与壁厚等因素对结构动态响应的影响。结果表明,泡沫铝相对密度对横向爆炸载荷下填充管的跨中挠度有较大的影响,随着泡沫铝相对密度的增大,填充管跨中挠度减小;随着外管直径与壁厚的增大,跨中挠度减小。
(2) 采用理想塑性地基梁模型和模态分析法,根据横向爆炸载荷下泡沫铝填充管塑性变形的特点,假定其模态函数,建立预测泡沫铝填充管跨中挠度的理论分析模型,并与数值模拟结果进行了对比,误差在20%以内,表明所建立的理论分析模型合理可行。
(3) 基于无量纲理论,推导了横向爆炸载荷下填充管跨中挠度的无量纲计算公式,分析了爆炸冲量对结构塑性变形的影响规律,并与对应的有限元模拟结果进行了对比。结果表明,无量纲冲量较小时,理论预测与数值模拟结果较为吻合,无量纲冲量较大时,二者之间的相对偏差逐渐增大。
-
Relative density/% Density/(kg·m−3) μ σs/MPa E/GPa 8 220.8 0.33 2.81 0.9 12 331.2 0.33 4.52 1.9 20 552.0 0.33 7.99 5.7 表 2 实心泡沫铝填充管跨中挠度的模态解与数值模拟结果对比
Table 2. Comparison of mid-span deflection of foamed aluminum filled tubes of modal solutions and numerical simulation results
d1/mm h/mm Relative density/% Wtheor/mm Wsim/mm Wsim−WtheorWtheor/% 76 0.7 8 3.0 3.1 3 12 1.6 1.7 6 20 0.7 0.8 14 0.8 8 2.8 2.9 4 12 1.4 1.5 7 20 0.6 0.7 17 89 0.7 8 2.5 2.7 8 12 1.3 1.4 8 20 0.5 0.6 20 0.8 8 2.3 2.3 0 12 1.2 1.2 0 20 0.5 0.5 0 表 3 两种模态函数跨中挠度的对比
Table 3. Comparison of mid-span deflection corresponding to two modal functions
d1/mm h/mm Relative density/% W/mm Relative error/% ϕ∗2(x)=1−2x/L ϕ∗2(x)=cos(πx/L) 76 0.7 8 3.0 2.8 −7 12 1.6 1.4 −13 20 0.7 0.6 −14 0.8 8 2.8 2.6 −7 12 1.4 1.4 0 20 0.6 0.6 0 89 0.7 8 2.5 2.4 −4 12 1.3 1.2 −8 20 0.5 0.5 0 0.8 8 2.3 2.2 −4 12 1.2 1.1 −8 20 0.5 0.5 0 -
[1] HENRYCH J. The dynamics of explosion and its use [M]. New York: Elsevier Scientific Publishing Company, 1979. [2] WIERZBICKI T, HOO F, MICHELLE S. Damage assessment of cylinders due to impact and explosive loading [J]. International Journal of Impact Engineering, 1993, 13(2): 215–241. doi: 10.1016/0734-743X(93)90094-N [3] 于博丽, 冯根柱, 李世强, 等. 横向爆炸载荷下薄壁圆管的动态响应 [J]. 爆炸与冲击, 2019, 39(10): 103101. doi: 10.11883/bzycj-2018-0295YU B L, FENG G Z, LI S Q, et al. Dynamic response of thin-wall circular tubes under transverse blast loading [J]. Explosion and Shock Waves, 2019, 39(10): 103101. doi: 10.11883/bzycj-2018-0295 [4] LI S Q, YU B L, KARAGIOZOVA D, et al. Experimental, numerical, and theoretical studies of the response of short cylindrical stainless steel tubes under lateral air blast loading [J]. International Journal of Impact Engineering, 2019, 124: 48–60. doi: 10.1016/j.ijimpeng.2018.10.004 [5] BROCHARD K, LE SOURNE H, BARRAS G. Extension of the string-on-foundation method to study the shock wave response of an immersed cylinder [J]. International Journal of Impact Engineering, 2018, 117: 138–152. doi: 10.1016/j.ijimpeng.2018.03.007 [6] YUEN S C K, NURICK G N, BRINCKMANN H B, et al. Response of cylindrical shells to lateral blast load [J]. International Journal of Protective Structures, 2013, 4(3): 209–230. doi: 10.1260/2041-4196.4.3.209 [7] KARAGIOZOVA D, YU T X, LU G, et al. Response of a circular metallic hollow beam to an impulsive loading [J]. Thin-Walled Structures, 2014, 80: 80–90. doi: 10.1016/j.tws.2014.02.021 [8] WALTERS R M, JONES N. An approximate theoretical study of the dynamic plastic behavior of shells [J]. International Journal of Non-Linear Mechanics, 1972, 7(3): 255–273. doi: 10.1016/0020-7462(72)90049-2 [9] WANG Y, QIAN X D, LIEW J Y R, et al. Impact of cement composite filled steel tubes: an experimental, numerical and theoretical treatise [J]. Thin-Walled Structures, 2015, 87: 76–88. doi: 10.1016/j.tws.2014.11.007 [10] HALL I W, GUDEN M, CLAAR T D. Transverse and longitudinal crushing of aluminum-foam filled tubes [J]. Scripta Materialia, 2002, 46(7): 513–518. doi: 10.1016/S1359-6462(02)00024-6 [11] WANG H W, WU C Q, ZHANG F R, et al. Experimental study of large-sized concrete filled steel tube columns under blast load [J]. Construction and Building Materials, 2017, 134: 131–141. doi: 10.1016/j.conbuildmat.2016.12.096 [12] ZHANG F R, WU C Q, WANG H W, et al. Numerical simulation of concrete filled steel tube columns against blast loads [J]. Thin-Walled Structures, 2015, 92: 82–92. doi: 10.1016/j.tws.2015.02.020 [13] YOUSUF M, UY B, TAO Z, et al. Transverse impact resistance of hollow and concrete filled stainless steel columns [J]. Journal of Constructional Steel Research, 2013, 82: 177–189. doi: 10.1016/j.jcsr.2013.01.005 [14] 邓旭辉, 王达锋. 近爆作用下中空夹层超高性能钢管混凝土柱的抗爆性能 [J]. 高压物理学报, 2020, 34(6): 065201.DENG X H, WANG D F. Anti-blast performance of ultra-high performance concrete-filled double steel tubes under close-in blast loading [J]. Chinese Journal of High Pressure Physics, 2020, 34(6): 065201. [15] COLE R H, WELLER R. Underwater explosions [J]. Physics Today, 1948, 1(6): 35. doi: 10.1063/1.3066176 [16] KARAGIOZOVA D, NURICK G N, LANGDON G S. Behaviour of sandwich panels subject to intense air blasts–Part 2: numerical simulation [J]. Composite Structures, 2009, 91(4): 442–450. doi: 10.1016/j.compstruct.2009.04.010 [17] 刘志芳, 王军, 秦庆华. 横向冲击载荷下泡沫铝夹芯双圆管的吸能研究 [J]. 兵工学报, 2017, 38(11): 2259–2267. doi: 10.3969/j.issn.1000-1093.2017.11.024LIU Z F, WANG J, QIN Q H. Research on energy absorption of aluminum foam-filled double circular tubes under lateral impact loadings [J]. Acta Armamentarii, 2017, 38(11): 2259–2267. doi: 10.3969/j.issn.1000-1093.2017.11.024 [18] 余同希, 华云龙. 结构塑性动力学引论[M]. 合肥: 中国科学技术大学出版社, 1994: 88–89.YU T X, HUA Y L. Introduction to structural plasticity dynamics [M]. Hefei: University of Science and Technology of China Press, 1994: 88–89. -







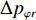
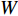
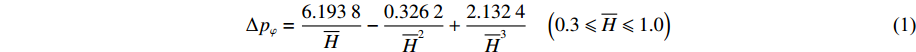



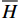
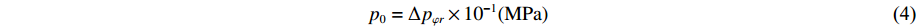
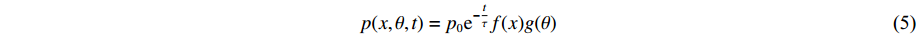
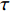
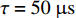
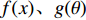
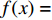

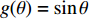


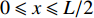
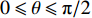

 下载:
下载:
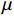
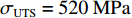

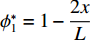
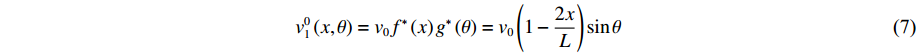
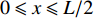


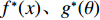
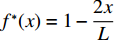
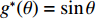
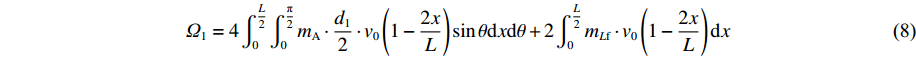
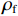
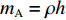
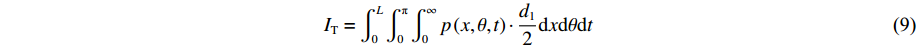
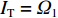
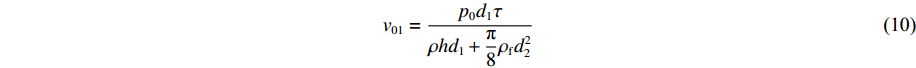
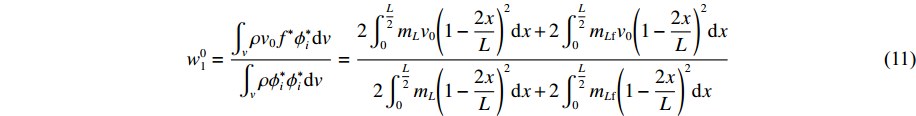
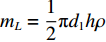
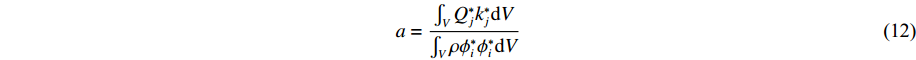
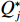

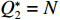
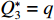
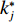
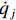

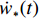
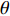
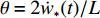
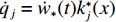

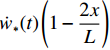
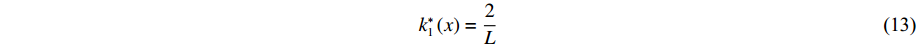
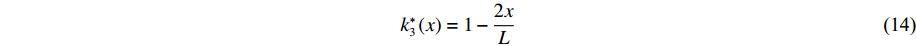
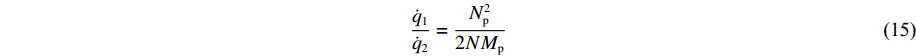
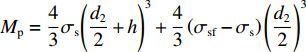

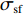
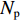

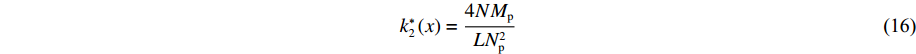

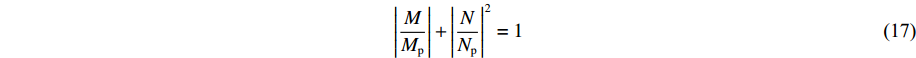

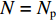
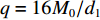

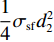
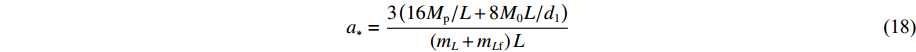
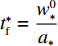
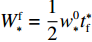
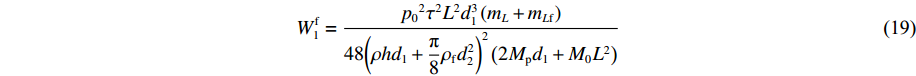

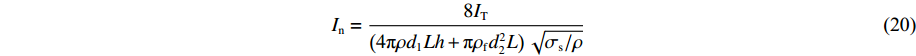
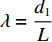
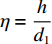
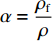
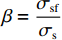
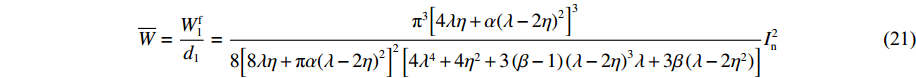



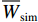

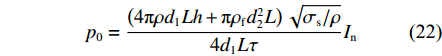
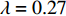
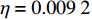
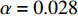
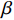

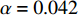
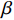


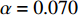
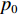
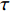
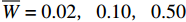
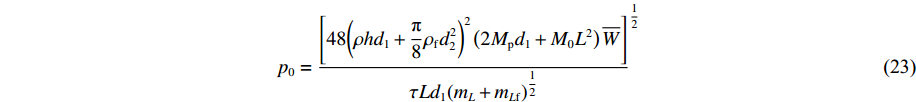
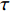
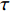
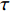
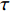

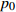

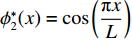
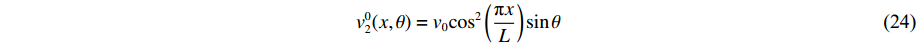
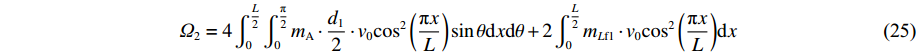
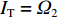
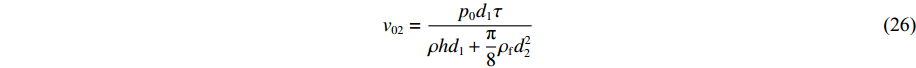
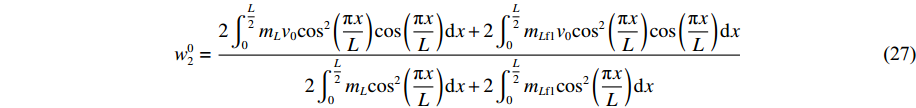
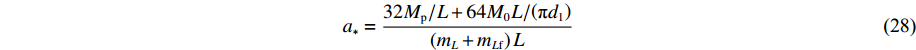
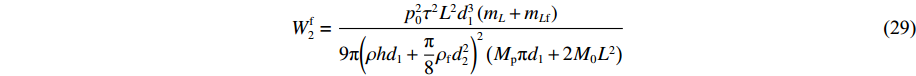

 下载:
下载: