Advances of Experimental and Theoretical Models of Magnesium Twin Deformation
-
摘要: 孪晶变形作为密排六方（HCP）镁金属的重要变形机制，对镁金属的塑性硬化、破坏和织构演变等具有重要影响。影响孪晶变形的因素较多，有取向织构、晶粒尺寸、应变率、温度、晶界和应力状态等。首先重点介绍了前3种因素对镁金属孪晶变形的影响，孪晶的启动不再单一地考虑与取向相关的Schmid定律，需结合与临近晶粒间的应变兼容，晶粒尺寸对孪晶的影响同样可以采用Hall-Petch关系描述，只是关系式的斜率比滑移更大，提高应变率对孪晶成核和成长都有一定的促进作用；然后分析了现有常见的孪晶理论模型，最后展望了孪晶变形在实验和理论模型方面的发展方向。Abstract: Twin deformation is an important deformation mechanism of hexagonal close-packed (HCP) magnesium, and has a significant influence on the plastic hardening, failure and texture evolution of materials. There are many factors affecting twin deformation: orientation texture, grain size, strain rate, temperature, grain boundary and stress state, etc. Firstly, this paper focuses on the influence of the first three factors on the twin deformation. The activation of twins should consider the combination of the strain accommodation between adjacent grains and the orientation dependent Schmid’s law, instead of the later one solely. The effect of grain size on twinning can be described by Hall-Petch relation. However, the slope of Hall-Petch relation dominated by twin is larger than that of slip. And the twin nucleation and growth could be promoted by increasing the strain rate. Then, the common twin theory models are analyzed. Finally, the developments of twin deformation in experiment and theoretical models are prospected.
-
Key words:
- twinning /
- magnesium /
- deformation mechanism /
- theoretical model
-
1. 引言
液体燃料抛撒成雾后能和空气形成爆炸性混合物。通常该爆炸性混合物有两种截然不同的化学反应模式:一种是速度缓慢的层流燃烧模式, 火焰-未反应燃料的相对速度约为1 m/s; 另一种是伴随着高温高压的高速(约2 km/s)化学反应模式, 称之为爆轰。与气相爆轰[1-5]相比, 气-液两相或气-液-固三相爆轰更复杂, 伴随液-固燃料的抛撒、破碎等物理过程, 其反应特征时间和反应区尺度远大于气相爆轰。相比于凝聚相炸药, 多相爆轰的容积能量密度要低很多, 但其质量能量密度却远超过一般凝聚相炸药。
近年来, 关于多相爆轰的研究持续升温。一个主要原因是出于对燃料-空气炸药(Fuel-Air Explosive, FAE)的关注, 通过采用不同种类和配比的燃料, 寻找高能燃料以提高FAE的威力。另一方面, 是出于对工业生产中安全问题的考虑。煤矿中的煤尘-瓦斯-空气爆炸, 食品制药行业中的可燃粉尘-空气爆炸, 石油化工企业中的燃料-空气爆炸等多相爆轰现象都能造成重大的灾难性事故。
国内已进行了很多关于多相爆轰的研究。刘光烈[6]设计了一台多相爆轰试验管, 并利用它测量了环氧丙烷-铝粉-空气混合物的爆轰压力和正压作用时间, 测量结果与外场试验结果基本一致。刘庆明等人[7]对多相FAE的分散、爆轰过程进行了光学测量, 并测量了FAE爆炸压力场, 分析了气-液-固多相爆轰的特征和压力波形的特点, 研究了冲击波峰值超压及比冲量随传播距离变化的规律。汤明钧[8]归纳总结了多相爆轰的特点, 并对影响多相爆轰的因素进行了分析。贵大勇等人[9]研究了典型液态燃料和固-液混合燃料配方对FAE爆轰性能的影响规律, 并对各典型液态燃料的FAE爆轰威力进行了排序。徐晓峰等人[10]以立式激波管为主要实验手段, 对4种燃料(C5H8.68、环氧丙烷、正己烷和癸烷)与空气混合物的云雾爆轰性能进行了较为系统的研究。姚干兵等人[11]采用烟迹技术, 在立式激波管中测定了环氧丙烷、90号汽油、硝酸异丙酯、庚烷、癸烷、戊二烯等几种燃料气-液两相云雾爆轰的胞格尺寸, 认为液滴的碎解、汽化过程以及燃烧区前导是控制气-液两相云雾爆轰的主要因素。陈默等人[12]在长为32.4 m、内径为0.199 m的大型长直水平管道中, 对环氧丙烷-铝粉-空气三相流云雾的爆燃转爆轰(Deflagration to Detonation, DDT)过程进行了实验研究, 实现了多相体系爆燃向爆轰转变的过程, 并测得其胞格尺寸。
本研究以立式激波管为载体, 以RDX-混合燃料-空气三相体系为对象, 借助压力测试系统、烟熏技术, 进行爆轰波压力、速度和胞格尺寸的测量, 目的是获得气-液-固三相爆轰的相关参数, 以期了解多相爆轰波的结构及其传播规律。
2. 实验装置及测试系统
2.1 实验设备
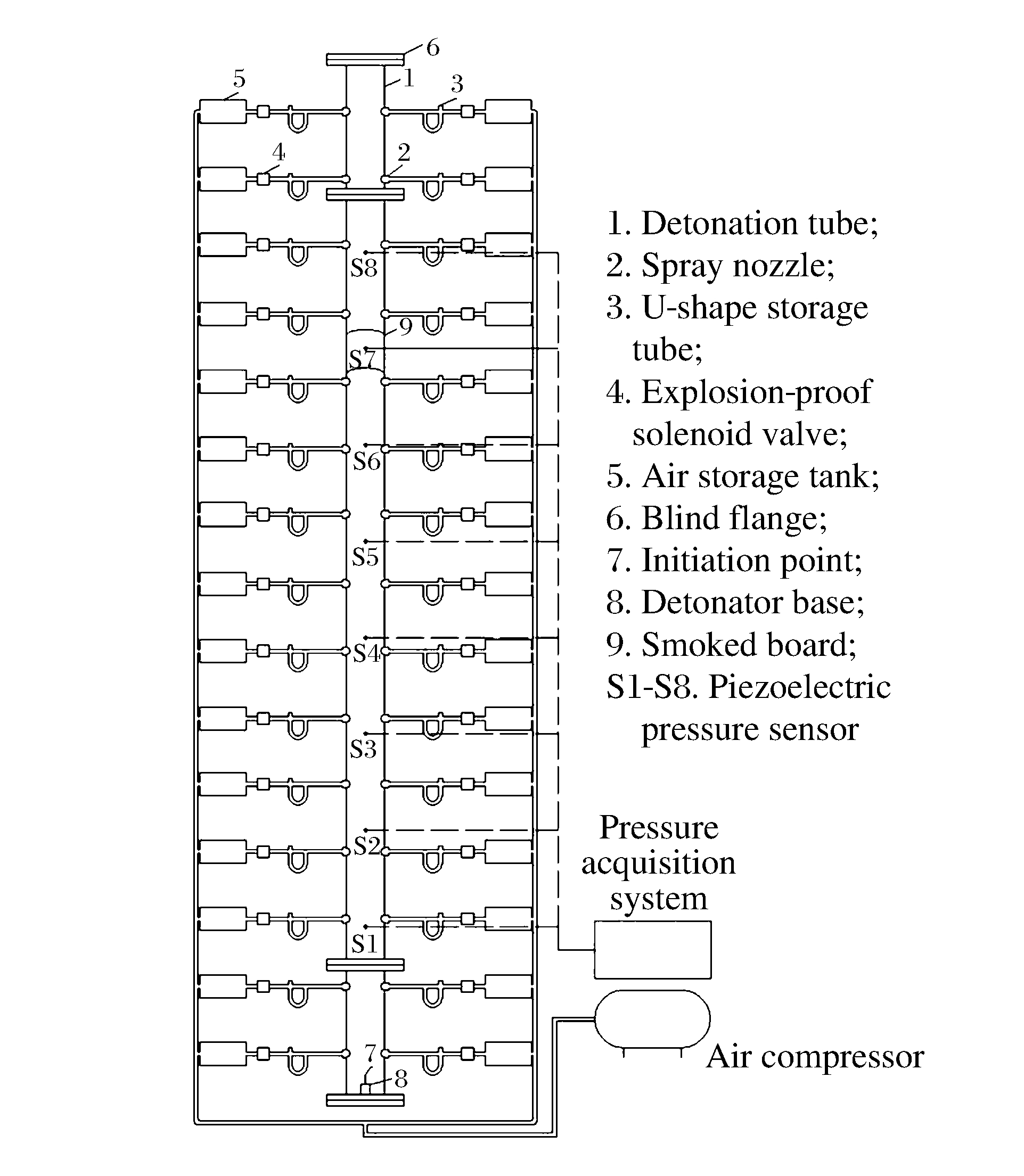
整套立式激波管设备由激波管管体、喷粉喷雾系统和点火系统组成。其结构如图 1所示。激波管内径为200 mm, 高5.4 m, 管体分为3个部分:主体部分, 长4 m; 底端为起爆端, 长0.7 m; 顶端为观察窗, 长0.7 m。不使用连着观察窗的4个喷头, 以防止实验中观察窗遭到破坏。故仅利用4.7 m的管体, 有效容积为147.6 L。喷粉喷雾系统由空气压缩机、储气罐、U型管储液储粉装置、喷头等组成。点火系统由延时点火器、起爆线、雷管基座等组成。
实验开始前, 先将烟熏板置于激波管内指定位置(见图 1), 安装好起爆装置; 然后向U型管内注入燃料和粉尘, 开启空气压缩机, 向储气罐内注入一定量的压缩空气; 最后开启压力采集系统, 设置点火延时器。准备就绪后, 打开电磁阀使压缩空气冲出, 夹带着液体和粉尘的空气由喷头抛撒进入激波管, 至云团达到最佳状态点火, 记录压力曲线并取下烟熏板保存。点火延迟时间是指从开启电磁阀到点火的这段时间。根据多次重复实验, 液体-空气云团在电磁阀开启1 s后发展到最大, 故将点火延迟时间取为1 s。充入储气罐中的气压值经过多次尝试, 取0.4 MPa时, 能在500 ms内将U型管内的液体、粉尘喷撒干净[10]。为确保将U型管内粉尘吹干净, 设定电磁阀开启持续时间为1 s。电磁阀关闭后即刻开始点火。即吹粉时间为1 s, 点火延迟时间也为1 s。
本研究采用了两种液体燃料:一种是90#溶剂油, 另一种是90#溶剂油和硝酸异丙酯(IPN)按质量比4:1制成的混合物。液体燃料和RDX粉尘交叉注入26个U型管中, 即:若液体燃料注入某一个U型管, 则RDX粉尘注入其对面和临近的U型管中。除特别说明外, 起爆物均为一发雷管加3 g塑性炸药, 起爆能量为23.52 kJ。
2.2 测试系统
测试系统由压力测试系统、胞格记录装置等组成。其中, 压力测试系统由传感器、电荷放大器、数据采集卡、微机等组成。采用PCB压电式石英传感器, 编号为S1~S8, 分布如图 1所示。每两个传感器间距为0.5 m, 传感器S2距离起爆端1.4 m。
胞格记录装置主要由烟熏板、固定支架组成。其中, 烟熏板为半圆形铝板, 使用前将板清洗干净并晾干, 表面涂一层很薄的机油, 用煤油灯烟熏黑其内表面, 出现一层薄而均匀的烟灰, 用0.4 MPa压缩空气吹之, 烟灰不脱落者为合格。烟熏板用支架固定在法兰上, 板中心距离上法兰1.5 m。
3. 实验结果与讨论
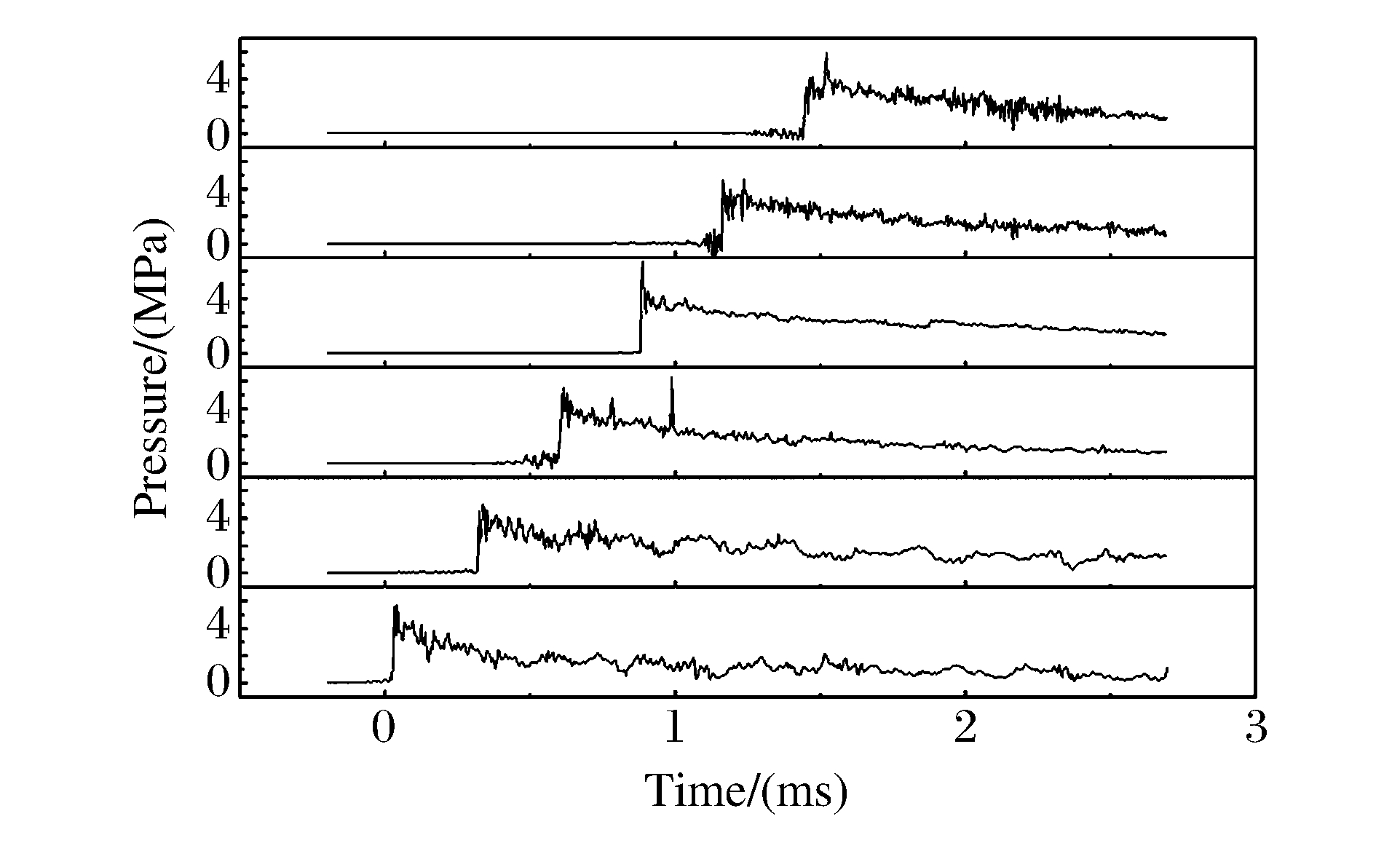
表 1给出了各传感器的具体位置和参数。经过多次测试发现, 距离起爆点较近处压力大, 较远处压力稍小。故将量程大的传感器安置在激波管底端附近, 量程小的传感器安置在管体顶端附近。图 2为典型的爆轰波压力-时间曲线, 传感器同时记录了各测试点处的爆压和爆轰波到达该点的时刻, 以两个传感器之间的距离除以爆轰波到达的时间差, 即可推算出爆轰波在这两个传感器间的平均速度。
表 1 传感器位置和量程Table 1. Position and range of pressure sensorsNo. Distance from the
detonation point/
(m)Maximum
pressure/
(MPa)S2 1.4 15.330 S3 1.9 15.270 S4 2.4 6.784 S5 2.9 6.757 S6 3.4 6.220 S7 3.9 6.000 实验所用RDX为白品造粒, 经BT-9300S粒度分析仪测得其平均粒径(D50)为85 μm。90#溶剂油和IPN的理化性质如表 2所示, 二者按质量比4:1制成的混合物密度为0.736 g/cm3。如前所述, 液体燃料和RDX粉尘是交叉注入26个U型管的, 即13个装液体, 13个装粉尘。经多次重复实验得到, 当单个U型管中加入4 mL、即总共加入52 mL燃料时, 刚好发生爆轰, 故纯液体燃料的点火浓度下限为260 g/m3。在此基础上, 改变RDX粉尘的加入量, 每次向单个U型管中分别加入1、2、3、4、5 g, 对应粉尘浓度依次为88、176、264、352、440 g/m3。在激波管顶部加观察窗, 记录云雾形成、爆轰过程, 得到如图 3所示的时序照片。
表 2 90#溶剂油和IPN的理化性质Table 2. Physicochemical properties of 90# solvent oil and IPNLiquid
fuelDensity/
(g/cm3)Composition Boiling
point/
(℃)Flash
point/
(℃)Ignition
temperature/
(℃)Upper
explosive
limit/(%)Lower
explosive
limit/(%)90# solvents oil 0.65 C5H12, C6H14 35-60 13 228 5.9 1.1 IPN 1.04 C3H7NO3 98-102 11 - 100 2 
图 3 三相云雾爆轰时序照片Figure 3. A series of experimental photos of three-phase cloud detonation3.1 爆轰压力
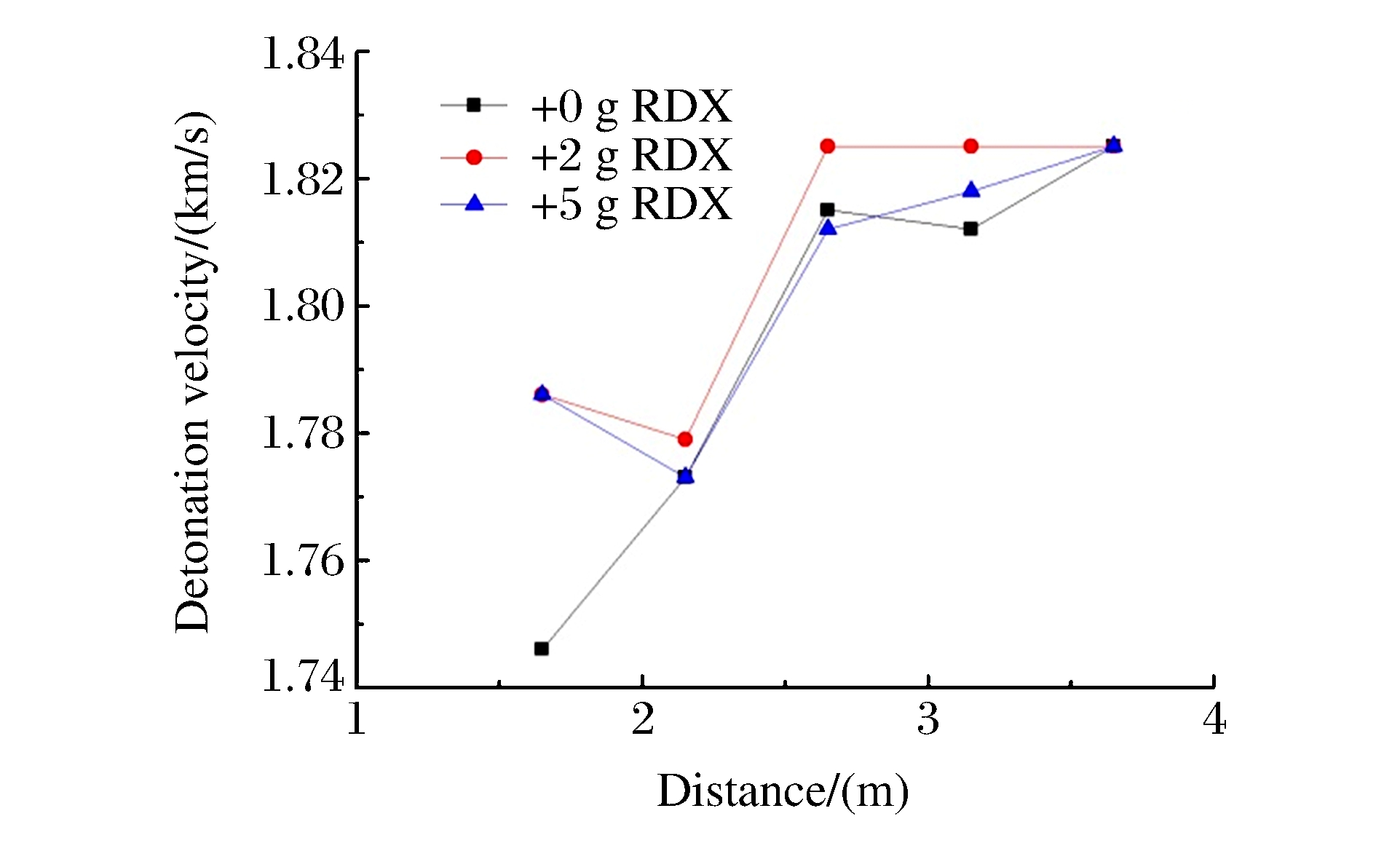
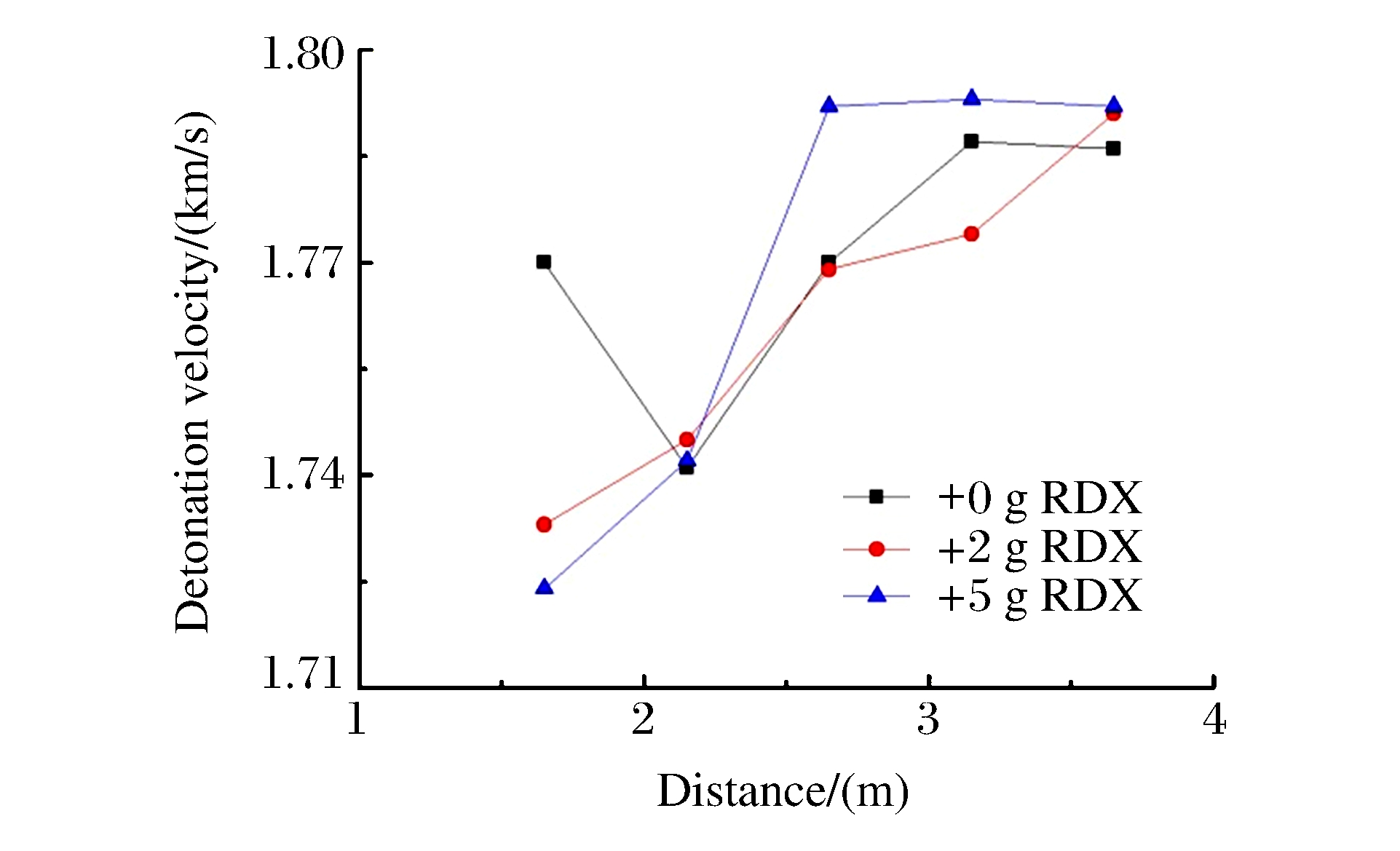
不同配比的RDX-90#溶剂油及RDX-混合物的爆轰压力(简称爆压)随距起爆点距离的变化趋势如图 4、图 5所示, 图中:+n g RDX(n=0, 2, 5)意为每次向单个U型管中加入n g RDX, 下同。
图 4 不同RDX添加量的RDX-90#溶剂油体系的爆压随距离变化趋势Figure 4. Detonation pressure with distance for RDX-90# solvent oil with various RDX additive amounts
图 5 不同RDX添加量的RDX-混合溶剂体系的爆压随距离变化趋势Figure 5. Detonation pressure with distance for RDX-mixed fuel with various RDX additive amounts多相爆轰为不稳定爆轰, 爆轰波的压力、速度并非一成不变。由图 5、图 6可知, 同一组实验中, 随着爆轰波的传播, 爆轰压力的波动较大, 整体呈下降趋势。分析其原因, 可能是因为液滴、粉尘喷出之后, 较大颗粒的液滴、粉尘部分沉降, 出现激波管底部粉尘浓度较大、顶部粉尘浓度较小的情况, 所以压力呈现递减趋势。但不论是纯溶剂油还是混合溶剂体系, 其气云爆轰的最低压力均在5 MPa左右, 比一般碳氢液体燃料-空气的气云爆轰压力高出很多。这主要是因为90#溶剂油的挥发性较强, 且含碳量较高, 能量密度较大, 与空气形成爆炸性混合物后, 反应较为充分。
图 6 不同RDX添加量的RDX-90#溶剂油体系的爆速随距离变化趋势Figure 6. Detonation velocity with distance for RDX-90# solvent oil with various RDX additive amounts由图 4、图 5得知, 随着RDX粉尘浓度的增加, RDX-90#溶剂油和RDX-混合溶剂三相体系的平均爆压均呈整体上升趋势, 相比而言, 前者爆压的上升趋势不如后者明显。
3.2 爆轰速度
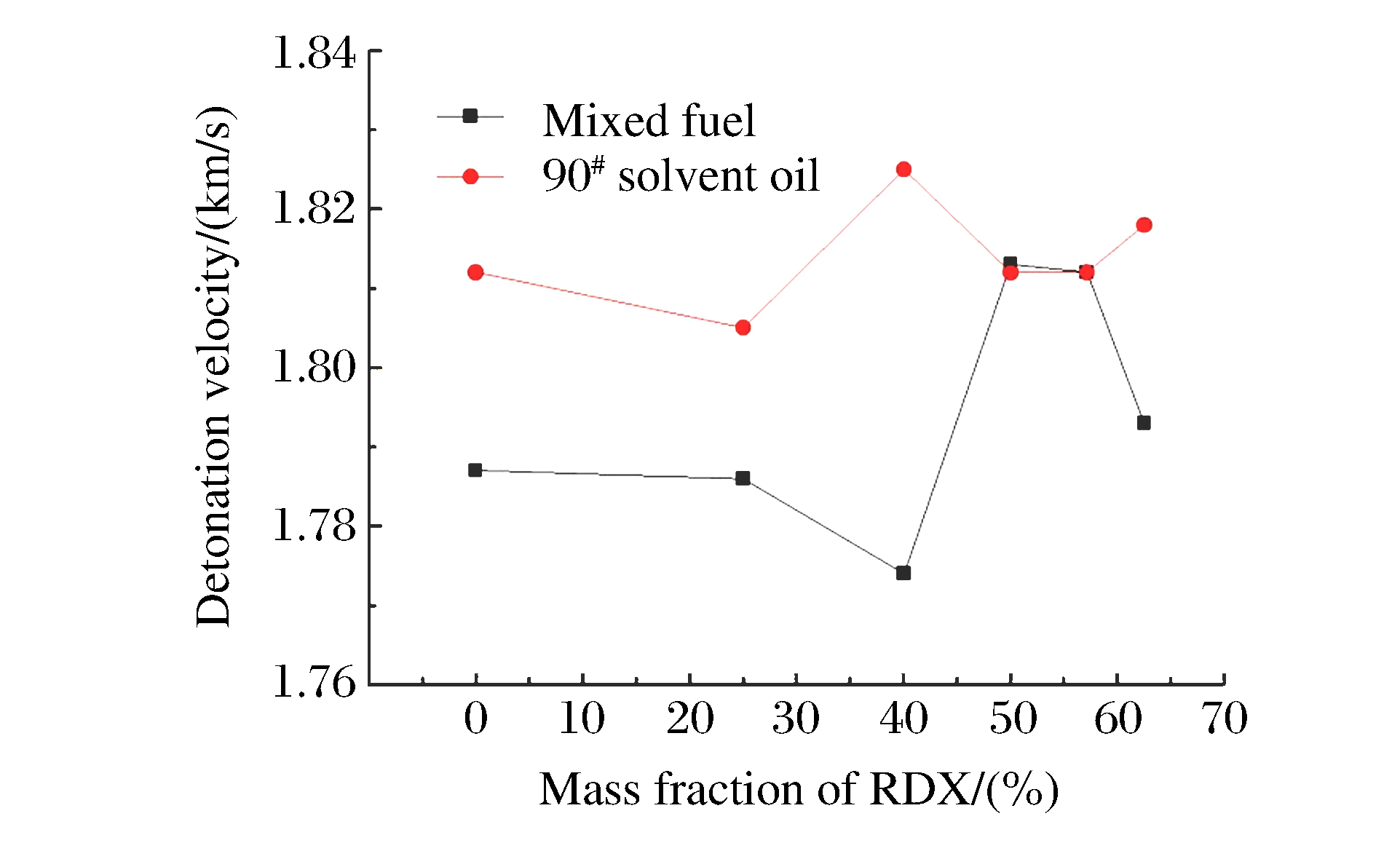
不同配比的RDX-90#溶剂油、RDX-混合溶剂的爆轰速度(简称爆速)随距起爆点距离的变化趋势如图 6、图 7所示。
图 7 不同RDX添加量的RDX-混合溶剂体系的爆速随距离变化趋势Figure 7. Detonation velocity with distance for RDX-mixed fuel with various RDX additive amounts距离起爆点较近(1.5~2.5 m)处, 爆速起伏上升; 距离大于2.5 m以后, 爆速趋于稳定, 并达到最大值。从图 6、图 7中可以得知, 随着RDX粉尘浓度的增加, RDX-90#溶剂油和RDX-混合溶剂的爆速均呈现整体上升的趋势。
图 8为RDX-燃料-空气三相体系在距离起爆点3.15 m处的平均爆速随RDX质量分数的变化趋势。无论何种配比, RDX-90#溶剂油体系的平均爆速均不小于RDX-混合溶剂。这一点也不难理解, 由表 2可知, 90#溶剂油的密度较小, 沸点较低, 饱和蒸汽压较高, 容易挥发汽化, 在激波阵面到达以前, 体系中就有一定的燃料蒸汽存在, 故而其破碎雾化的时间较短, 爆轰压力和爆轰速度也较大。
图 8 距离起爆点3.15 m处, 爆速随RDX含量的变化趋势Figure 8. Detonation velocity with the mass fraction of RDX at the distance of 3.15 m3.3 RDX参与了爆轰反应的验证
由于90#溶剂油和IPN混合物原本的爆速、爆压就比较高, 故对RDX是否参与了爆轰有所怀疑, 设计如下实验进行验证。其它条件(如:加入液体和粉尘的量、电磁阀开启持续时间、起爆能量等)不变, 将液体燃料换成环氧丙烷(Propylene Oxide, PO), 且注入U型管后不进行静置, 不待其发生汽化, 直接进行抛撒起爆, 进行3次重复实验。传感器S3~S7的参数和位置见表 1。
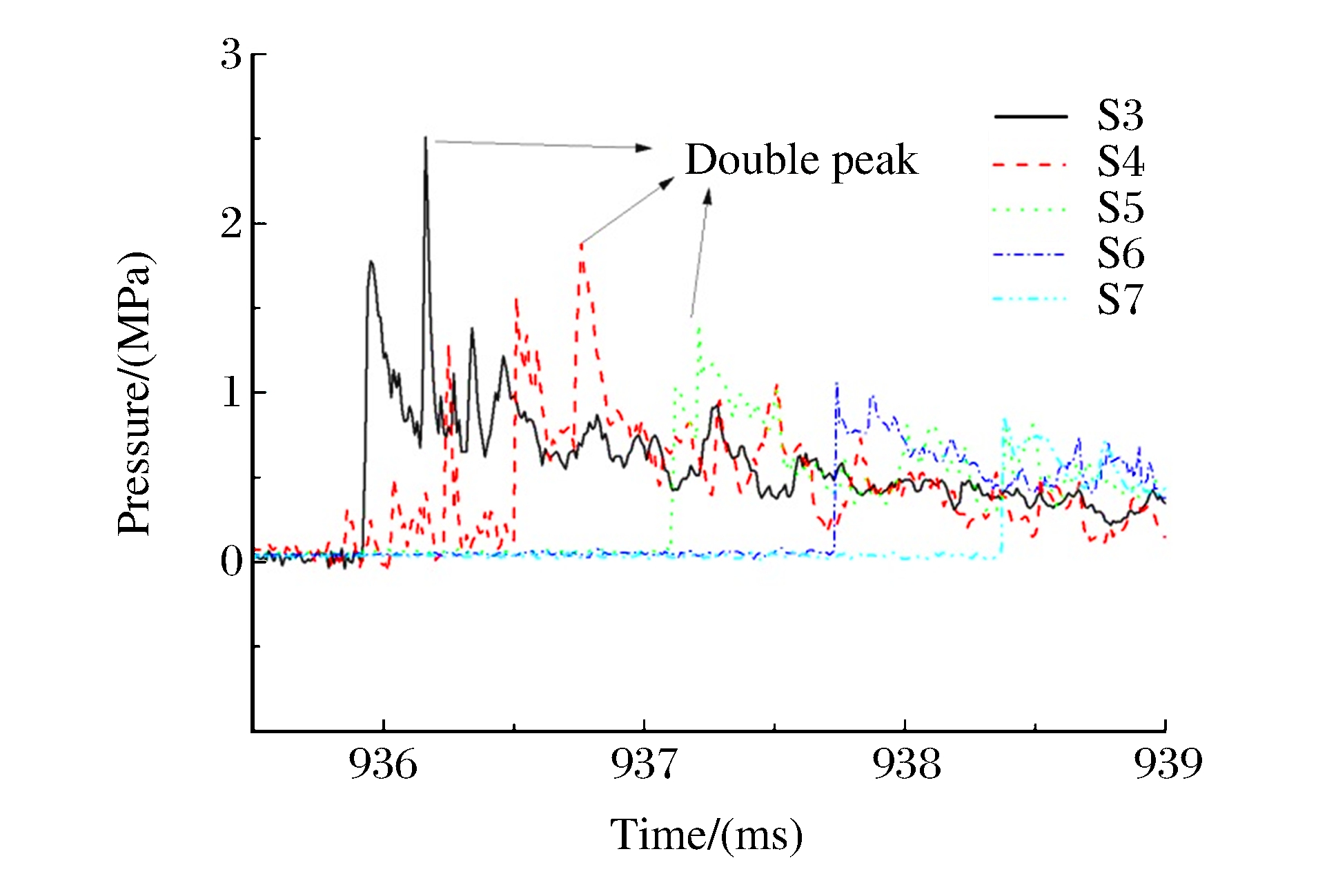
单纯注入PO时, 压力-时间曲线如图 9所示。由图 9可以看出, 波形出现双峰结构, 说明云团内发生了二次反应, 且二次反应比第一次猛烈。第一和第二个峰之间正是液滴发生破碎雾化的过程, 特征时间Δt=0.2 ms。从爆压和爆速(1 km/s以下)的数据来看, PO-空气体系并没有发生爆轰。
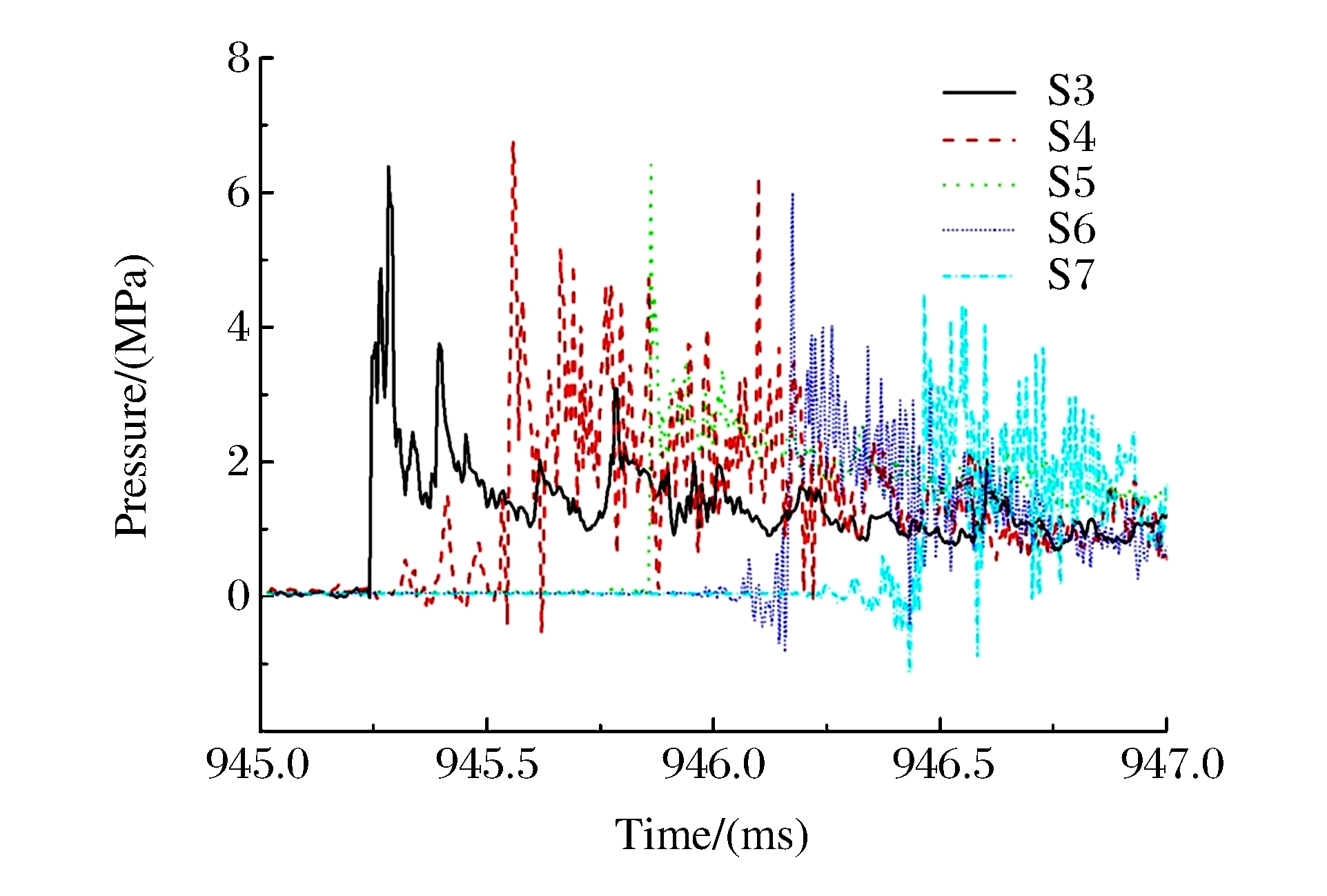
加入RDX后, 压力-时间曲线如图 10所示。由图 10可以看出, 爆压达到5~6 MPa, 爆速达到1.5 km/s以上。加入RDX粉尘之后, PO由爆燃达到了爆轰, 压力和速度都有很大突跃, 可见RDX确实参与了爆轰, 而不仅仅是燃烧。
3.4 多相爆轰胞格的测量与爆压分析
3.4.1 胞格尺寸和长宽比
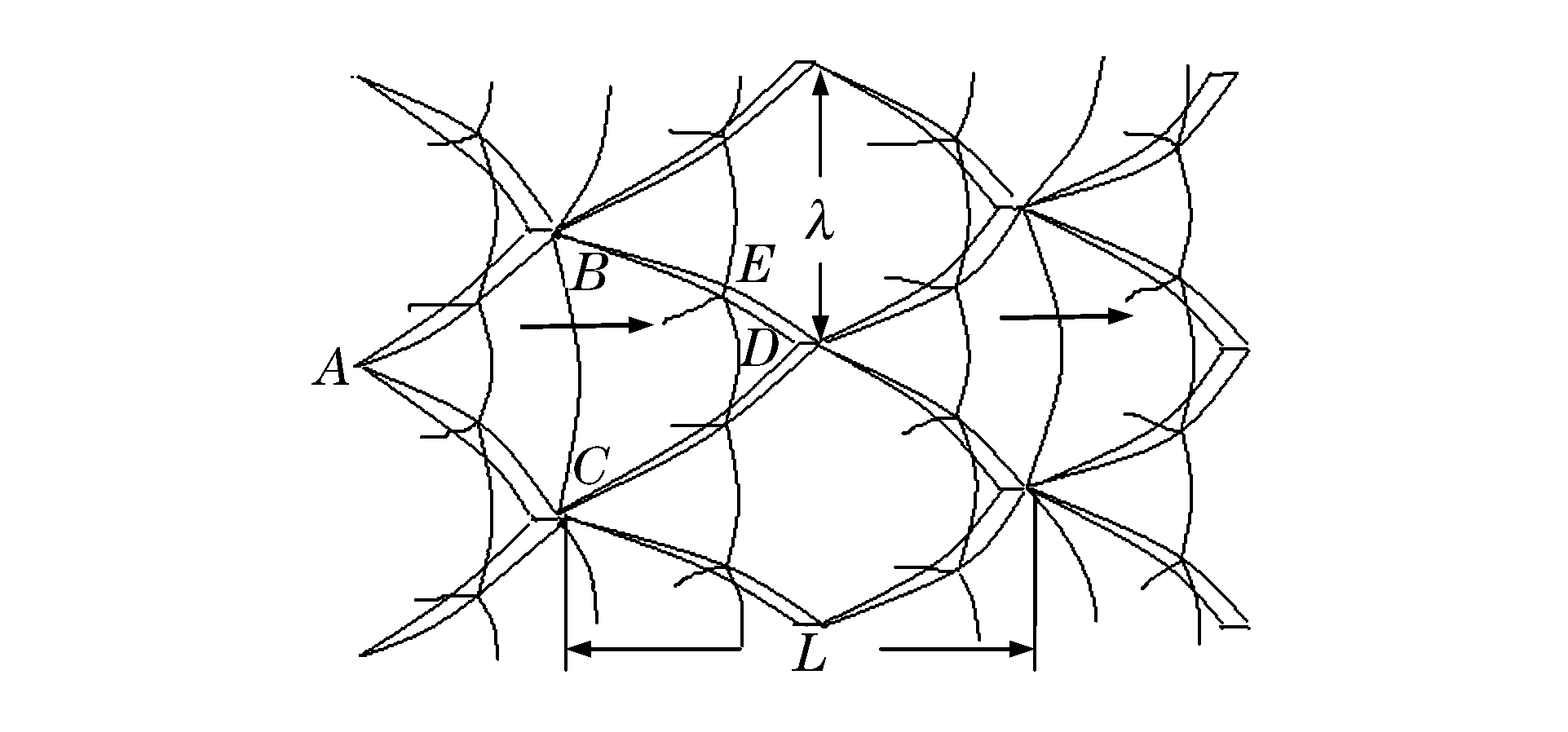
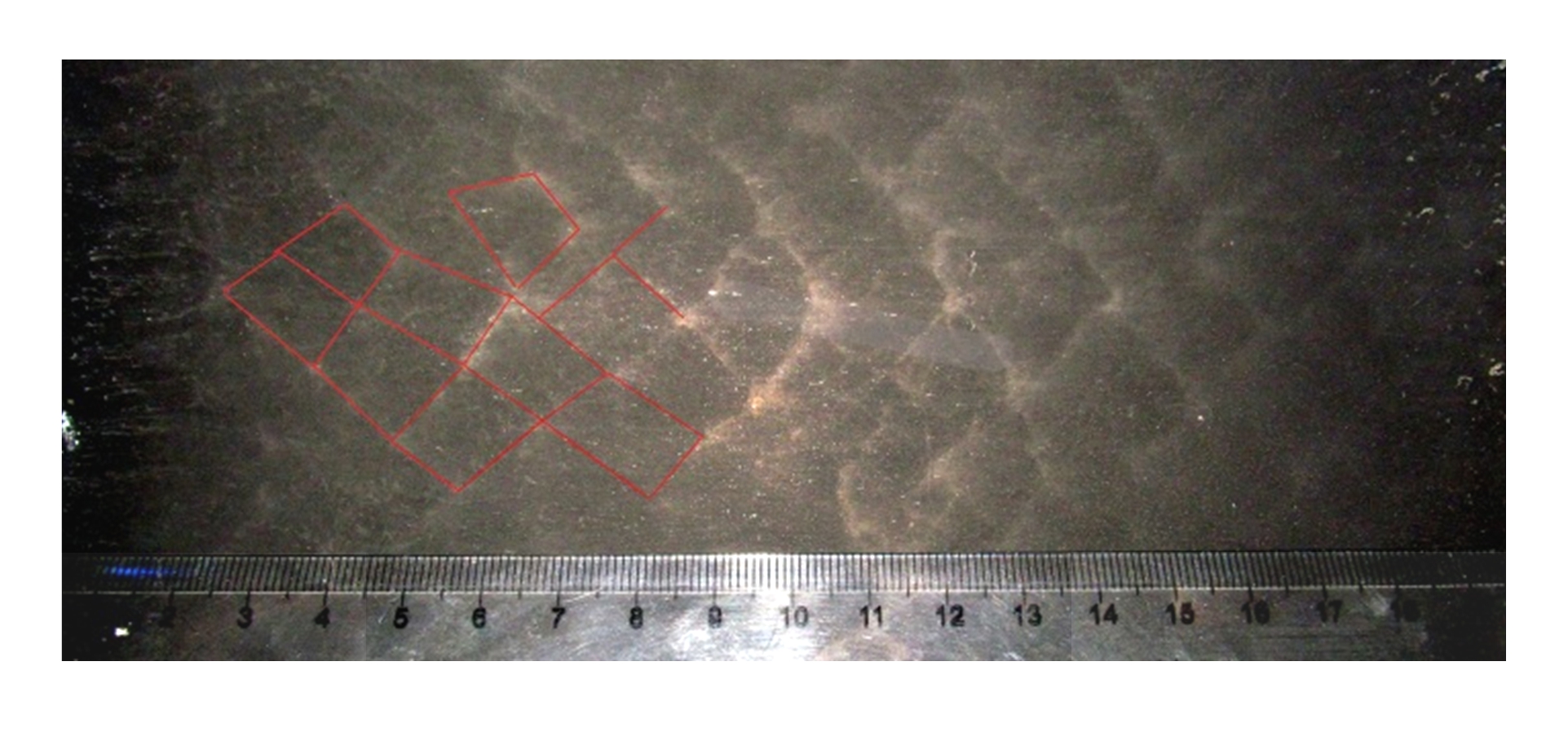
所谓爆轰胞格就是爆轰波在管道内传播过程中, 横波、入射波、马赫杆三波交合点的运动轨迹。爆轰的胞格尺寸是判断可燃混合气体的最基本特征参数, 爆轰参数诸如临界管径、爆轰极限以及直接气爆能量等, 都可以通过胞格尺寸进行预测[13]。如图 11所示, 爆轰波从左至右传播, 其前导冲击波是由马赫杆和入射冲击波交替组合的波阵面。自1959年Denisov和Troshin发现爆轰波具有胞格结构以来, 实验中都使用烟迹技术观测胞格结构。本研究所用的烟迹载体为半圆形铝制板, 利用支架将其固定在激波管的内壁上, 以最大限度减少对爆轰波传播过程的干扰。
图 12是用上述铝制板得到的RDX-燃料-空气三相爆轰的典型胞格, 其中L为胞格长度, λ为胞格宽度。爆轰波从左至右传播, 图 12中用红色线标记了几个典型的胞格。与气相爆轰的胞格(见图 11)相比, 三相爆轰胞格的长宽比(L/λ)要小得多, 有些胞格长、宽相等甚至长比宽还要小。
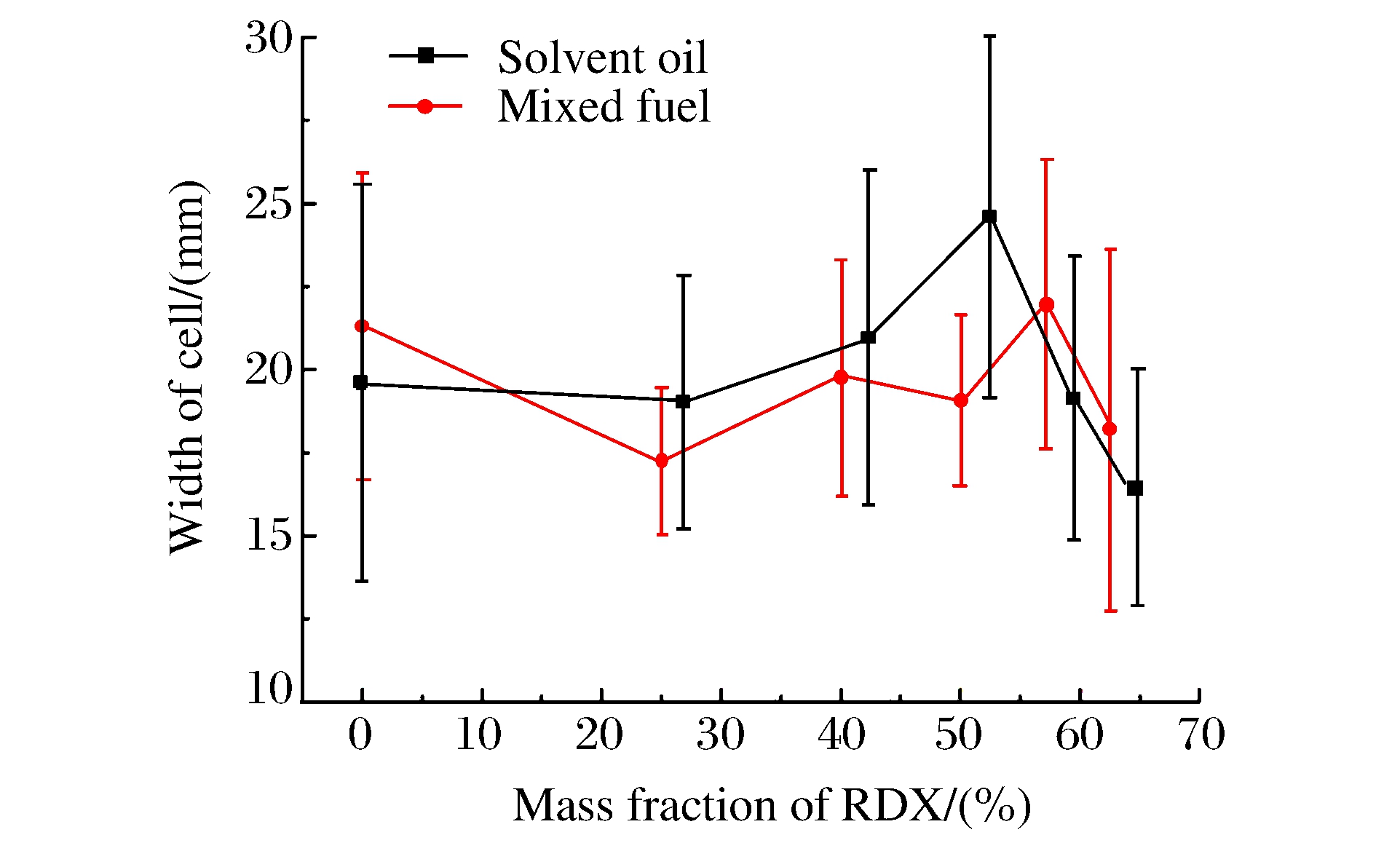
多相爆轰为非均相爆轰, 胞格尺寸、形状不统一, 波动性较大(见图 12)。经过重复实验, 取胞格尺寸(此处特指胞格的宽度)平均值, 并利用数据处理软件计算其误差线, 如图 13所示。
图 13 RDX-90#溶剂油-空气和RDX-混合溶剂-空气体系爆轰胞格尺寸随RDX质量分数的变化趋势Figure 13. Detonation cell width with mass fraction of RDX for RDX-90# solvent oil-air and RDX-mixed fuel-air system图 13分别为RDX-90#溶剂油-空气体系及RDX-混合溶剂-空气体系的爆轰胞格尺寸随RDX质量分数的变化趋势。两种体系相比, 单纯90#溶剂油体系的平均胞格尺寸比加入IPN的混合溶剂体系要大, 也即前者的临界起爆能量较高。这一点很好解释, 因为IPN中带有-O-NO2基团, 相当于“敏化剂”, 降低了体系的直接起爆能量。另外, 两种体系的爆轰胞格尺寸都在RDX质量分数为55%左右时达到最大, 也即当RDX粉尘质量与液体燃料质量之比为55:45时, 体系最不敏感, 需要较大的能量才能起爆。在爆轰过程中, 液滴、粉尘的破碎相变过程需要吸收较多能量。RDX粉尘的比表面积较大, 增加的RDX粉尘自身蕴含的能量不足以抵消加热它们所需的能量, 所以随着粉尘量的增加, 起爆所需要的能量也增加。但是, RDX的能量密度远大于液体燃料, 当RDX质量分数达到一定程度(约55%)时, 体系增加的能量开始大于需要多消耗的能量, 故此后, 起爆所需的能量就迅速减少。如图 13所示, 当RDX质量分数大于55%时, 体系的爆轰胞格尺寸迅速减小。
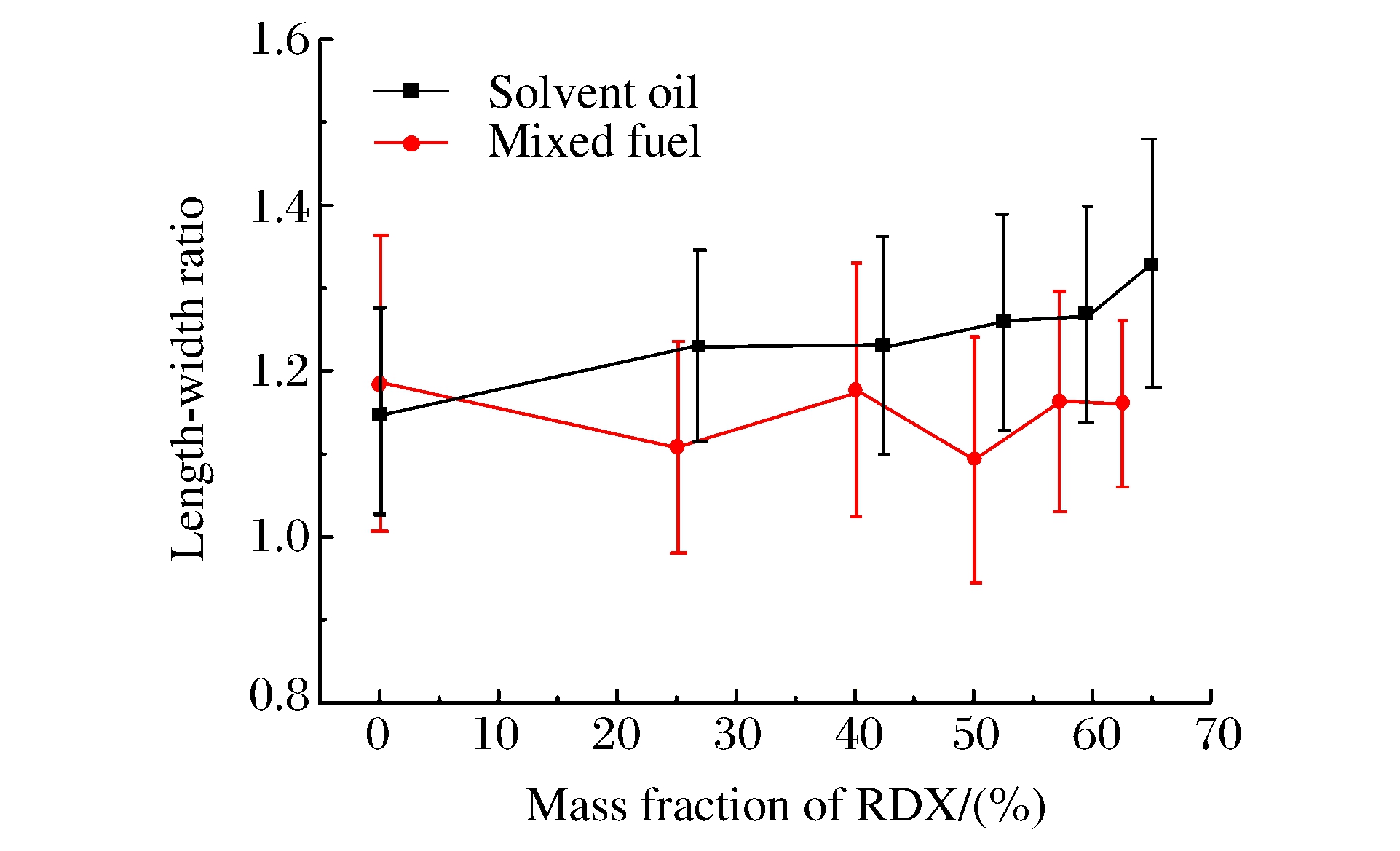
在测量胞格宽度的同时, 也测定了胞格的长度, 并计算其长宽比, 用数据处理软件计算得到误差线, 如图 14所示。Strehlow等人发现, 低爆轰压力下, 气相爆轰波的胞格长宽比近似满足L/λ≈1.61。不过正如图 12和图 14所示, 多相爆轰波的胞格长宽比较小, 仅为1.2左右。胞格长宽比反映的是爆轰波和横波平均传播速度的比值, 其中:爆轰波的平均传播速度为定值, 由总放热量决定; 而横波的平均传播速度与波后声速相关[16]。如图 8所示, 90#溶剂油体系的爆轰波平均传播速度比混合溶剂体系大; 又由表 3知, 二者介质密度接近, 故其波后声速也接近, 因此前者的胞格长宽比大于后者。
图 14 RDX-90#溶剂油-空气和RDX-混合溶剂-空气体系爆轰胞格长宽比随RDX质量分数的变化趋势Figure 14. L/λ of Detonation cell with mass fraction of RDX for RDX-90# solvent oil-air and RDX-mixed fuel-air system在二次引爆型FAE的云雾起爆阶段, 若发生蹿火将大大降低FAE的作用威力。如果在液固云爆药剂中采用前述配方(RDX的质量分数为55%), 则FAE云团的直接气爆能量增加, 能够降低发生蹿火的风险。不过其效果还需要进一步的实验验证。
3.4.2 胞格尺寸与临界起爆能
根据Lee提出的表面积能量模型[13-15], 即球形爆轰波表面所含的能量等于平面爆轰在临界管径内的能量, 此时所需满足的临界条件为
4πR∗2=πd2c4 (1) 式中:R*为爆轰内核半径, dc为临界管径。
此外, 由强爆轰波理论可知, 爆轰波能量为
Ec=4πIγp0Ma2R3Sh (2) 式中:Ec为临界起爆能量, γ为气体绝热指数, I为定常数(当γ=1.4时, I=0.423), p0为压强, Ma为球形爆轰波的马赫数, RSh是球形爆轰波的半径。
依据Zeldovich准则, 当爆炸波衰减到Ma=MaCJ(MaCJ为CJ爆轰波的马赫数)时, 有RSh=R*。对于未稀释的混合气体, 临界管径与爆轰胞格尺寸之间存在如下经验公式
dc=13λ (13) 因此, 联合(1)式~(3)式, 可得气相爆轰参数中, 临界起爆能量与胞格尺寸之间的关系为
Ec=4πIγp0Ma2CJ(13λ4)3=2197πρ0v2CJIλ316 (4) 式中:ρ0为混合物密度; vCJ为CJ爆速, 近似取实测爆速。根据(4)式, 即可由气相爆轰胞格尺寸预测其直接起爆的临界起爆能量。
多相爆轰中, 无论液体还是固体粉尘, 都要先经历物理破碎、相变过程, 之后的阶段就和气相爆轰一样, 所以推测多相爆轰也能采用气相爆轰的方法预测其临界起爆能量。为了验证(4)式是否能应用于多相爆轰中, 测试了RDX-90#溶剂油-空气和RDX-混合溶剂-空气体系的临界起爆能量, 起爆物分别为一发雷管加1.5 g塑性炸药和一发雷管。测试结果与理论值相比较, 列于表 3。
表 3 临界起爆能实验值与理论值的比较Table 3. Comparison between experimental and theoretical value of critical initiation energyConstituents ρ0/
(kg/m3)vCJ/
(m/s)λ/
(mm)Theoretical
Ec/(kJ)Experimental
Ec/(kJ)RDX-90# solvent oil-air 1.633 2 1 794 24.6 14.279 14.735 RDX-mixed fuel-air 1.659 5 1 808 19.1 6.893 5.945 由此可见, 多相爆轰参数——临界起爆能和胞格尺寸之间确实存在类似(4)式的关系, 但还需要进一步的实验和理论验证。
4. 结论
(1) 立式激波管可以对液、固两相燃料进行同时抛撒, 实现气-液-固三相爆轰, 并能对爆压、爆速、最小起爆能、胞格尺寸等爆轰参数进行测量。
(2) 90#溶剂油-空气体系的平均爆压达到5~6 MPa, 可以将其作为燃料-空气炸药的主燃料。
(3) 添加RDX有助于提高90#溶剂油-IPN混合燃料体系的爆速和爆压。
(4) 加入IPN后, 混合溶剂的直接起爆能量降低, 较易实现爆轰, 故IPN可以作为一种敏化剂加入FAE燃料之中。此外, 加入IPN的混合溶剂爆轰胞格也较小, 可见临界起爆能量和爆轰胞格之间存在与气相爆轰类似的关系, 但还需要进一步的实验和理论验证。
-
图 2 (a)应变为0.08时AM30样品2个压缩孪晶的EBSD图（黑色圆圈表示应变兼容）；(b)临近轧制方向取向的晶粒内形成的{
10¯11 }压缩孪晶（红色）的EBSD图；(c) 2(b)图中基体的(0002)极图（黑色六边形）、6个孪晶取向（黑色正方形）和选择的临近晶粒孪晶取向（黑色圈内）以及它们的Schmid因子[9]Figure 2. (a) EBSD map of AM30 sample pulled to 0.08 strain showing two contraction twins (The black circles indicate strain compatibility.); (b) EBSD map of a {
10¯11 } contraction twin (in red) formed within a near rolling direction orientation; (c) (0002) pole figure for the Fig.2 (b) matrix (black hexagon), the six twin variants (black squares), the selected variant (circled in black), and their respective Schmid factors[9]
图 5 (a)基于传统孪晶理论的孪晶相互作用（由于孪晶位错不能穿透孪晶界，当两者相互靠近时将阻碍孪晶的增长）；(b)非位错形式的孪晶增长（T1）（孪晶能够通过改变孪晶面产生分支而包围另一个孪晶）；(c) 非位错形式的孪晶增长（T2）（孪晶通过侧向成长而绕过另一个孪晶）[45]
Figure 5. (a) Twin-twin interaction based on classical twinning theory (The growth of the twin variants will be impeded as the variants approach close to each other because the twinning dislocations are unable to penetrate the twin boundaries.); (b) non-dislocation mediated twin growth (T1) (A twin variant can branch out by changing the habit plane and surround the other variant.); (c) non-dislocation mediated twin growth (T2) (A twin variant can spread laterally and grow around the other variant.)[45]
图 6 冲击波传播和材料微结构：(a)冲击示意图和3个观测点；(b)冲击波传播的时间-位置图和3个观测点在不同时刻（t）的微结构[1]
Figure 6. The propagation of shock wave and the microstructure of material: (a) The schematic diagram of shock and three observation point; (b) the time-distance diagram of the shock experiment and corresponding schematic diagrams of the microstructure at 3 location at different times (t)[1]
图 9 晶粒内的孪晶和退孪晶过程示意图（绿色实线为孪晶界，蓝色和红色点线代表晶格取向；
sαM 和nαM 分别表示基体中孪晶面和法线，sαT 和nαT 表示孪晶区域中孪晶面和法线方向）[91]Figure 9. Schematic representation of twinning and de-twinning in a grain (Solid green lines represent twinning boundaries; Lattices orientations are represented by dotted blue lines and dotted red lines;
sαM andnαM are the twinning plane and normal direction in matrix;sαT andnαT are the twinning plane and normal direction in twin domain.)[91]
图 10 (a)孪晶不全位错通过孪晶面滑动和垂直于孪晶面增长到临近点X的传播示意图（
lglide 和lgrowth 为孪晶成核点到增长点X的水平和垂直距离，vprop 和vgrowth 为孪晶沿孪晶面和孪晶面法线的增长速度）；(b)包含孪晶的多晶微结构（晶粒内不同颜色代表不同取向）[95]Figure 10. (a) Sketch map of a twin partial dislocation propagation to a neighboring point X by respectively gliding on the twin plane and growing normal to it (
lglide andlgrowth are the horizontal and vertical distances from twin nucleation point to growth point X.vprop andvgrowth are the growth velocity of twins along the twin plane and its normal.); (b) polycrystalline microstructure with twins (Different colors in grains represent different orientations.)[95] -
[1] YU X, LI T, LI L, et al. Influence of initial texture on the shock property and spall behavior of magnesium alloy AZ31B [J]. Materials Science and Engineering: A, 2017, 700: 259–268. doi: 10.1016/j.msea.2017.06.015 [2] ZHANG F, HAO M, WANG F C, et al. Role of {10–12} twinning and detwinning in the shock-hardening behavior of rolled Mg-3Al-1Zn alloy [J]. Scripta Materialia, 2012, 67(12): 951–954. doi: 10.1016/j.scriptamat.2012.08.027 [3] PROUST G, TOMÉ C N, JAIN A, et al. Modeling the effect of twinning and detwinning during strain-path changes of magnesium alloy AZ31 [J]. International Journal of Plasticity, 2009, 25(5): 861–880. doi: 10.1016/j.ijplas.2008.05.005 [4] WANG H, WU P D, TOMÉ C N, et al. A constitutive model of twinning and detwinning for hexagonal close packed polycrystals [J]. Materials Science and Engineering: A, 2012, 555: 93–98. doi: 10.1016/j.msea.2012.06.038 [5] CHRISTIAN J W, MAHAJAN S. Deformation twinning [J]. Progress in Materials Science, 1995, 39(1/2): 1–157. doi: 10.1016/0079-6425(94)00007-7 [6] PARK S H, HONG S G, LEE J H, et al. Multiple twinning modes in rolled Mg-3Al-1Zn alloy and their selection mechanism [J]. Materials Science and Engineering: A, 2012, 532: 401–406. doi: 10.1016/j.msea.2011.11.003 [7] XU S, TYSON W R, EAGLESON R, et al. Dependence of flow strength and deformation mechanisms in common wrought and die cast magnesium alloys on orientation, strain rate and temperature [J]. Journal of Magnesium and Alloys, 2013, 1(4): 275–282. doi: 10.1016/j.jma.2013.11.003 [8] BEYERLEIN I J, CAPOLUNGO L, MARSHALL P E, et al. Statistical analyses of deformation twinning in magnesium [J]. Philosophical Magazine, 2010, 90(16): 2161–2190. doi: 10.1080/14786431003630835 [9] JONAS J J, MU S J, AL-SAMMAN T, et al. The role of strain accommodation during the variant selection of primary twins in magnesium [J]. Acta Materialia, 2011, 59(5): 2046–2056. doi: 10.1016/j.actamat.2010.12.005 [10] MU S J, JONAS J J, GOTTSTEIN G. Variant selection of primary, secondary and tertiary twins in a deformed Mg alloy [J]. Acta Materialia, 2012, 60(5): 2043–2053. doi: 10.1016/j.actamat.2012.01.014 [11] BARNETT M R, KESHAVARZ Z, BEER A G, et al. Non-Schmid behaviour during secondary twinning in a polycrystalline magnesium alloy [J]. Acta Materialia, 2008, 56(1): 5–15. doi: 10.1016/j.actamat.2007.08.034 [12] LOU C, ZHANG X Y, REN Y. Non-Schmid-based {10-12} twinning behavior in polycrystalline magnesium alloy [J]. Materials Characterization, 2015, 107: 249–254. doi: 10.1016/j.matchar.2015.07.022 [13] SONG B, XIN R L, LIANG Y C, et al. Twinning characteristic and variant selection in compression of a pre-side-rolled Mg alloy sheet [J]. Materials Science and Engineering: A, 2014, 614: 106–115. doi: 10.1016/j.msea.2014.07.026 [14] LUO J R, GODFREY A, LIU W, et al. Twinning behavior of a strongly basal textured AZ31 Mg alloy during warm rolling [J]. Acta Materialia, 2012, 60(5): 1986–1998. doi: 10.1016/j.actamat.2011.12.017 [15] BARNETT M R, KESHAVARZ Z, NAVE M D. Microstructural features of rolled Mg-3Al-1Zn [J]. Metallurgical and Materials Transactions A, 2005, 36(7): 1697–1704. doi: 10.1007/s11661-005-0033-3 [16] CHEN S F, SONG H W, ZHANG S H, et al. An effective Schmid factor in consideration of combined normal and shear stresses for slip/twin variant selection of Mg-3Al-1Zn alloy [J]. Scripta Materialia, 2019, 167: 51–55. doi: 10.1016/j.scriptamat.2019.03.026 [17] GUO C F, XIAO Y, XIN R L. Evaluation of twinning behavior in rolling of Mg alloys with three kinds of textures by a generalized schmid factor [J]. Metals and Materials International, 2020, 26(9): 1366–1372. doi: 10.1007/s12540-019-00378-0 [18] XIN R L, LIU Z, SUN Y J, et al. Understanding common grain boundary twins in Mg alloys by a composite Schmid factor [J]. International Journal of Plasticity, 2019, 123: 208–223. doi: 10.1016/j.ijplas.2019.07.018 [19] GUO C F, XIN R L, DING C H, et al. Understanding of variant selection and twin patterns in compressed Mg alloy sheets via combined analysis of Schmid factor and strain compatibility factor [J]. Materials Science and Engineering: A, 2014, 609: 92–101. doi: 10.1016/j.msea.2014.04.103 [20] LIU G D, XIN R L, SHU X G, et al. The mechanism of twinning activation and variant selection in magnesium alloys dominated by slip deformation [J]. Journal of Alloys and Compounds, 2016, 687: 352–359. doi: 10.1016/j.jallcom.2016.06.136 [21] SHI Z Z, ZHANG Y D, WAGNER F, et al. On the selection of extension twin variants with low Schmid factors in a deformed Mg alloy [J]. Acta Materialia, 2015, 83: 17–28. doi: 10.1016/j.actamat.2014.10.004 [22] HE W J, CHEN X, CHEN H, et al. Grain size effect on the thermally activated twin boundary migration in a zirconium alloy [J]. Materials Science and Engineering: A, 2018, 724: 576–585. doi: 10.1016/j.msea.2018.03.118 [23] KIM Y J, LEE J U, KIM S H, et al. Grain size effect on twinning and annealing behaviors of rolled magnesium alloy with bimodal structure [J]. Materials Science and Engineering: A, 2019, 754: 38–45. doi: 10.1016/j.msea.2019.03.041 [24] KIM Y J, LEE J U, KIM S H, et al. Variation in crystallographic orientation and twinning activation with size of individual grains in rolled magnesium alloy [J]. Metals and Materials International, 2019, 25(6): 1541–1547. doi: 10.1007/s12540-019-00321-3 [25] DOBROŇ P, CHMELÍK F, YI S B, et al. Grain size effects on deformation twinning in an extruded magnesium alloy tested in compression [J]. Scripta Materialia, 2011, 65(5): 424–427. doi: 10.1016/j.scriptamat.2011.05.027 [26] GHADERI A, BARNETT M R. Sensitivity of deformation twinning to grain size in titanium and magnesium [J]. Acta Materialia, 2011, 59(20): 7824–7839. doi: 10.1016/j.actamat.2011.09.018 [27] KUMAR M A, WROŃSKI M, MCCABE R J, et al. Role of microstructure on twin nucleation and growth in HCP titanium: a statistical study [J]. Acta Materialia, 2018, 148: 123–132. doi: 10.1016/j.actamat.2018.01.041 [28] ASGARI H, ODESHI A G, SZPUNAR J A, et al. Grain size dependence of dynamic mechanical behavior of AZ31B magnesium alloy sheet under compressive shock loading [J]. Materials Characterization, 2015, 106: 359–367. doi: 10.1016/j.matchar.2015.06.030 [29] MEYERS M A, VÖHRINGER O, LUBARDA V A. The onset of twinning in metals: a constitutive description [J]. Acta Materialia, 2001, 49(19): 4025–4039. doi: 10.1016/S1359-6454(01)00300-7 [30] CERRETA E, YABLINSKY C A, GRAY Ⅲ T G, et al. The influence of grain size and texture on the mechanical response of high purity hafnium [J]. Materials Science and Engineering: A, 2007, 456(1/2): 243–251. doi: 10.1016/j.msea.2006.11.111 [31] MARCINKOWSKI M J, LIPSITT H A. The plastic deformation of chromium at low temperatures [J]. Acta Metallurgica, 1962, 10(2): 95–111. doi: 10.1016/0001-6160(62)90055-X [32] ARMSTRONG R W, WORTHINGTON P J. A constitutive relation for deformation twinning in body centered cubic metals [M]//ROHDE R W, BUTCHER B M, HOLLAND J R, et al. Metallurgical Effects at High Strain Rates. Boston, MA: Springer, 1973: 401-414. [33] HONG S G, PARK S H, LEE C S. Role of {10–12} twinning characteristics in the deformation behavior of a polycrystalline magnesium alloy [J]. Acta Materialia, 2010, 58(18): 5873–5885. doi: 10.1016/j.actamat.2010.07.002 [34] BOHLEN J, DOBROŇ P, SWIOSTEK J, et al. On the influence of the grain size and solute content on the AE response of magnesium alloys tested in tension and compression [J]. Materials Science and Engineering: A, 2007, 462(1/2): 302–306. doi: 10.1016/j.msea.2006.02.470 [35] YU H H, XIN Y C, WANG M Y, et al. Hall-petch relationship in Mg alloys: a review [J]. Journal of Materials Science & Technology, 2018, 34(2): 248–256. doi: 10.1016/j.jmst.2017.07.022 [36] YU H H, LI C Z, XIN Y C, et al. The mechanism for the high dependence of the Hall-Petch slope for twinning/slip on texture in Mg alloys [J]. Acta Materialia, 2017, 128: 313–326. doi: 10.1016/j.actamat.2017.02.044 [37] SOMEKAWA H, MUKAI T. Hall-Petch relation for deformation twinning in solid solution magnesium alloys [J]. Materials Science and Engineering: A, 2013, 561: 378–385. doi: 10.1016/j.msea.2012.10.040 [38] JIN Z Z, ZHA M, YU Z Y, et al. Exploring the Hall-Petch relation and strengthening mechanism of bimodal-grained Mg-Al-Zn alloys [J]. Journal of Alloys and Compounds, 2020, 833: 155004. doi: 10.1016/j.jallcom.2020.155004 [39] ZHOU P, XIAO D W, JIANG C L, et al. Twin interactions in pure Ti under high strain rate compression [J]. Metallurgical and Materials Transactions A, 2017, 48(1): 126–138. doi: 10.1007/s11661-016-3832-9 [40] WANG B S, XIN R L, HUANG G J, et al. Strain rate and texture effects on microstructural characteristics of Mg-3Al-1Zn alloy during compression [J]. Scripta Materialia, 2012, 66(5): 239–242. doi: 10.1016/j.scriptamat.2011.10.046 [41] DUDAMELL N V, ULACIA I, GÁLVEZ F, et al. Twinning and grain subdivision during dynamic deformation of a Mg AZ31 sheet alloy at room temperature [J]. Acta Materialia, 2011, 59(18): 6949–6962. doi: 10.1016/j.actamat.2011.07.047 [42] MAKSOUD I A, AHMED H, RÖDEL J. Investigation of the effect of strain rate and temperature on the deformability and microstructure evolution of AZ31 magnesium alloy [J]. Materials Science and Engineering: A, 2009, 504(1/2): 40–48. doi: 10.1016/j.msea.2008.10.033 [43] LI L, MURÁNSKY O, FLORES-JOHNSON E A, et al. Effects of strain rate on the microstructure evolution and mechanical response of magnesium alloy AZ31 [J]. Materials Science and Engineering: A, 2017, 684: 37–46. doi: 10.1016/j.msea.2016.12.015 [44] AHMAD I R, SHU D W. Compressive and constitutive analysis of AZ31B magnesium alloy over a wide range of strain rates [J]. Materials Science and Engineering: A, 2014, 592: 40–49. doi: 10.1016/j.msea.2013.10.056 [45] MAO B, LIAO Y L, LI B. Abnormal twin-twin interaction in an Mg-3Al-1Zn magnesium alloy processed by laser shock peening [J]. Scripta Materialia, 2019, 165: 89–93. doi: 10.1016/j.scriptamat.2019.02.028 [46] BERGE F, KRÜGER L, OUAZIZ H, et al. Influence of temperature and strain rate on flow stress behavior of twin-roll cast, rolled and heat-treated AZ31 magnesium alloys [J]. Transactions of Nonferrous Metals Society of China, 2015, 25(1): 1–13. doi: 10.1016/S1003-6326(15)63572-5 [47] WANG M, LU L, LI C, et al. Deformation and spallation of a magnesium alloy under high strain rate loading [J]. Materials Science and Engineering: A, 2016, 661: 126–131. doi: 10.1016/j.msea.2016.03.009 [48] DIXIT N, XIE K Y, HEMKER K J, et al. Microstructural evolution of pure magnesium under high strain rate loading [J]. Acta Materialia, 2015, 87: 56–67. doi: 10.1016/j.actamat.2014.12.030 [49] YU J C, SONG B, XIA D B, et al. Dynamic tensile properties and microstructural evolution of extruded EW75 magnesium alloy at high strain rates [J]. Journal of Magnesium and Alloys, 2020, 8(3): 849–859. doi: 10.1016/j.jma.2020.02.013 [50] KURUKURI S, WORSWICK M J, TARI D G, et al. Rate sensitivity and tension-compression asymmetry in AZ31B magnesium alloy sheet [J]. Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences, 2014, 372: 20130216. doi: 10.1098/rsta.2013.0216 [51] ULACIA I, DUDAMELL N V, GÁLVEZ F, et al. Mechanical behavior and microstructural evolution of a Mg AZ31 sheet at dynamic strain rates [J]. Acta Materialia, 2010, 58(8): 2988–2998. doi: 10.1016/j.actamat.2010.01.029 [52] HAZELL P J, APPLEBY-THOMAS G J, WIELEWSKI E, et al. The influence of microstructure on the shock and spall behaviour of the magnesium alloy, Elektron 675 [J]. Acta Materialia, 2012, 60(17): 6042–6050. doi: 10.1016/j.actamat.2012.07.041 [53] DIXIT N, FARBANIEC L, RAMESH K T. Twinning in single crystal Mg under microsecond impact along the 〈a〉axis [J]. Materials Science and Engineering: A, 2017, 693(1): 22–25. doi: 10.1016/j.msea.2017.03.074 [54] WINEY J M, RENGANATHAN P, GUPTA Y M. Shock wave compression and release of hexagonal-close-packed metal single crystals: inelastic deformation of c-axis magnesium [J]. Journal of Applied Physics, 2015, 117(10): 105903. doi: 10.1063/1.4914525 [55] KANEL G I, GARKUSHIN G V, SAVINYKH A S, et al. Shock response of magnesium single crystals at normal and elevated temperatures [J]. Journal of Applied Physics, 2014, 116(14): 143504. doi: 10.1063/1.4897555 [56] RESSÉGUIER D T, HEMERY S, LESCOUTE E, et al. Spall fracture and twinning in laser shock-loaded single-crystal magnesium [J]. Journal of Applied Physics, 2017, 121(16): 165104. doi: 10.1063/1.4982352 [57] MILLETT J C F, STIRK S M, BOURNE N K, et al. On the behaviour of the magnesium alloy, AZ61 to one-dimensional shock loading [J]. Acta Materialia, 2010, 58(17): 5675–5682. doi: 10.1016/j.actamat.2010.06.042 [58] CEPEDA-JIMÉNEZ C M, MOLINA-ALDAREGUIA J M, PÉREZ-PRADO M T. Origin of the twinning to slip transition with grain size refinement, with decreasing strain rate and with increasing temperature in magnesium [J]. Acta Materialia, 2015, 88: 232–244. doi: 10.1016/j.actamat.2015.01.032 [59] MISHRA B, MUKHOPADHYAY A, SIVA KUMAR K, et al. Effect of test temperature on flow behavior and strain hardening of magnesium under high strain rate deformation conditions [J]. Materials Science and Engineering: A, 2020, 770: 138546. doi: 10.1016/j.msea.2019.138546 [60] KALIDINDI S R. Incorporation of deformation twinning in crystal plasticity models [J]. Journal of the Mechanics and Physics of Solids, 1998, 46(2): 267–271, 273–290. doi: 10.1016/S0022-5096(97)00051-3 [61] IZADBAKHSH A, INAL K, MISHRA R K, et al. New crystal plasticity constitutive model for large strain deformation in single crystals of magnesium [J]. Computational Materials Science, 2011, 50(7): 2185–2202. doi: 10.1016/j.commatsci.2011.02.030 [62] FAN H D, AUBRY S, ARSENLIS A, et al. The role of twinning deformation on the hardening response of polycrystalline magnesium from discrete dislocation dynamics simulations [J]. Acta Materialia, 2015, 92: 126–139. doi: 10.1016/j.actamat.2015.03.039 [63] NAMAKIAN R, VOYIADJIS G Z, KWAŚNIAK P. On the slip and twinning mechanisms on first order pyramidal plane of magnesium: molecular dynamics simulations and first principal studies [J]. Materials & Design, 2020, 191: 108648. doi: 10.1016/j.matdes.2020.108648 [64] HEO T W, WANG Y, BHATTACHARYA S, et al. A phase-field model for deformation twinning [J]. Philosophical Magazine Letters, 2011, 91(2): 110–121. doi: 10.1080/09500839.2010.537284 [65] TAYLOR G I. Plastic strain in metals [J]. Journal of the Institute of Metals, 1938, 62: 307–324. [66] HILL R. Generalized constitutive relations for incremental deformation of metal crystals by multislip [J]. Journal of the Mechanics and Physics of Solids, 1966, 14(2): 95–105. doi: 10.1016/0022-5096(66)90040-8 [67] ASARO R J, RICE J R. Strain localization in ductile single crystals [J]. Journal of the Mechanics and Physics of Solids, 1977, 25(5): 309–338. doi: 10.1016/0022-5096(77)90001-1 [68] SACHS G. Plasticity problems in metals [J]. Zeitschrift Verein Deutcher Ingenieur, 1928, 72: 734–736. [69] LEBENSOHN R A, TOMÉ C N. A self-consistent anisotropic approach for the simulation of plastic-deformation and texture development of polycrystals: application to zirconium alloys [J]. Acta Metallurgica et Materialia, 1993, 41(9): 2611–2624. doi: 10.1016/0956-7151(93)90130-K [70] LEBENSOHN R A, TOMÉ C N. A self-consistent viscoplastic model: prediction of rolling textures of anisotropic polycrystals [J]. Materials Science and Engineering: A, 1994, 175(1/2): 71–82. doi: 10.1016/0921-5093(94)91047-2 [71] ROTERS F, EISENLOHR P, HANTCHERLI L, et al. Overview of constitutive laws, kinematics, homogenization and multiscale methods in crystal plasticity finite-element modeling: theory, experiments, applications [J]. Acta Materialia, 2010, 58(4): 1152–1211. doi: 10.1016/j.actamat.2009.10.058 [72] 郑松林. 晶体塑性有限元在材料动态响应研究中的应用进展 [J]. 高压物理学报, 2019, 33(3): 030108. doi: 10.11858/gywlxb.20190725ZHENG S L. Advances in the study of dynamic response of crystalline materials by crystal plasticity finite element modeling [J]. Chinese Journal of High Pressure Physics, 2019, 33(3): 030108. doi: 10.11858/gywlxb.20190725 [73] 刘静楠, 叶常青, 刘桂森, 等. 高温、高压、高应变速率动态过程晶体塑性有限元理论模型及其应用 [J]. 高压物理学报, 2020, 34(3): 030102. doi: 10.11858/gywlxb.20190874LIU J N, YE C Q, LIU G S, et al. Crystal plasticity finite element theoretical models and applications for high temperature, high pressure and high strain-rate dynamic process [J]. Chinese Journal of High Pressure Physics, 2020, 34(3): 030102. doi: 10.11858/gywlxb.20190874 [74] VANHOUTTE P. Simulation of the rolling and shear texture of brass by the Taylor theory adapted for mechanical twinning [J]. Acta Metallurgica, 1978, 26(4): 591–604. doi: 10.1016/0001-6160(78)90111-6 [75] TOMÉ C N, LEBENSOHN R A, KOCKS U F. A model for texture development dominated by deformation twinning: application to zirconium alloys [J]. Acta Metallurgica et Materialia, 1991, 39(11): 2667–2680. doi: 10.1016/0956-7151(91)90083-D [76] SALEM A A, KALIDINDI S R, SEMIATIN S L. Strain hardening due to deformation twinning in α-titanium: constitutive relations and crystal-plasticity modeling [J]. Acta Materialia, 2005, 53(12): 3495–3502. doi: 10.1016/j.actamat.2005.04.014 [77] ABDOLVAND H, DAYMOND M R, MAREAU C. Incorporation of twinning into a crystal plasticity finite element model: evolution of lattice strains and texture in Zircaloy-2 [J]. International Journal of Plasticity, 2011, 27(11): 1721–1738. doi: 10.1016/j.ijplas.2011.04.005 [78] TADANO Y, YOSHIHARA Y, HAGIHARA S. A crystal plasticity modeling considering volume fraction of deformation twinning [J]. International Journal of Plasticity, 2016, 84: 88–101. doi: 10.1016/j.ijplas.2016.05.002 [79] LIU Q, ROY A, SILBERSCHMIDT V V. Temperature-dependent crystal-plasticity model for magnesium: a bottom-up approach [J]. Mechanics of Materials, 2017, 113: 44–56. doi: 10.1016/j.mechmat.2017.07.008 [80] ZHANG J, JOSHI S P. Phenomenological crystal plasticity modeling and detailed micromechanical investigations of pure magnesium [J]. Journal of the Mechanics and Physics of Solids, 2012, 60(5): 945–972. doi: 10.1016/j.jmps.2012.01.005 [81] ARDELJAN M, MCCABE R J, BEYERLEIN I J, et al. Explicit incorporation of deformation twins into crystal plasticity finite element models [J]. Computer Methods in Applied Mechanics and Engineering, 2015, 295: 396–413. doi: 10.1016/j.cma.2015.07.003 [82] SUN C Y, GUO N, FU M W, et al. Modeling of slip, twinning and transformation induced plastic deformation for TWIP steel based on crystal plasticity [J]. International Journal of Plasticity, 2016, 76: 186–212. doi: 10.1016/j.ijplas.2015.08.003 [83] 潘昊, 王升涛, 吴子辉, 等. 孪晶对Be材料冲击加-卸载动力学影响的数值模拟研究 [J]. 物理学报, 2018, 67(16): 164601. doi: 10.7498/aps.67.20180451PAN H, WANG S T, WU Z H, et al. Effect of twining on dynamic behaviors of beryllium materials under impact loading and unloading [J]. Acta Physica Sinica, 2018, 67(16): 164601. doi: 10.7498/aps.67.20180451 [84] 郑华雷, 杨合, 李宏伟. 纯钛压缩变形下的晶体塑性有限元分析 [J]. 塑性工程学报, 2013, 20(1): 95–99. doi: 10.3969/j.issn.1007-2012.2013.01.020ZHENG H L, YANG H, LI H W. Crystal plasticity finite element modeling for uniaxial compression of commercially pure titanium [J]. Journal of Plasticity Engineering, 2013, 20(1): 95–99. doi: 10.3969/j.issn.1007-2012.2013.01.020 [85] STAROSELSKY A, ANAND L. Inelastic deformation of polycrystalline face centered cubic materials by slip and twinning [J]. Journal of the Mechanics and Physics of Solids, 1998, 46(4): 671–673, 675–696. doi: 10.1016/S0022-5096(97)00071-9 [86] STAROSELSKY A, ANAND L. A constitutive model for hcp materials deforming by slip and twinning: application to magnesium alloy AZ31B [J]. International Journal of Plasticity, 2003, 19(10): 1843–1864. doi: 10.1016/S0749-6419(03)00039-1 [87] STEINMETZ D R, JÄPEL T, WIETBROCK B, et al. Revealing the strain-hardening behavior of twinning-induced plasticity steels: theory, simulations, experiments [J]. Acta Materialia, 2013, 61(2): 494–510. doi: 10.1016/j.actamat.2012.09.064 [88] WONG S L, MADIVALA M, PRAHL U, et al. A crystal plasticity model for twinning- and transformation-induced plasticity [J]. Acta Materialia, 2016, 118: 140–151. doi: 10.1016/j.actamat.2016.07.032 [89] PROUST G, TOMÉ C N, KASCHNER G C. Modeling texture, twinning and hardening evolution during deformation of hexagonal materials [J]. Acta Materialia, 2007, 55(6): 2137–2148. doi: 10.1016/j.actamat.2006.11.017 [90] BEYERLEIN I J, TOMÉ C N. A dislocation-based constitutive law for pure Zr including temperature effects [J]. International Journal of Plasticity, 2008, 24(5): 867–895. doi: 10.1016/j.ijplas.2007.07.017 [91] WANG H, WU P D, WANG J, et al. A crystal plasticity model for hexagonal close packed (HCP) crystals including twinning and de-twinning mechanisms [J]. International Journal of Plasticity, 2013, 49: 36–52. doi: 10.1016/j.ijplas.2013.02.016 [92] WU P D, GUO X Q, QIAO H, et al. A constitutive model of twin nucleation, propagation and growth in magnesium crystals [J]. Materials Science and Engineering: A, 2015, 625: 140–145. doi: 10.1016/j.msea.2014.11.096 [93] XIE Q J, ZHU Z W, KANG G Z, et al. Crystal plasticity-based impact dynamic constitutive model of magnesium alloy [J]. International Journal of Mechanical Sciences, 2016, 119: 107–113. [94] CHENG J H, GHOSH S. A crystal plasticity FE model for deformation with twin nucleation in magnesium alloys [J]. International Journal of Plasticity, 2015, 67(1): 148–170. doi: 10.1016/j.ijmecsci.2016.10.012 [95] CHENG J H, GHOSH S. Crystal plasticity finite element modeling of discrete twin evolution in polycrystalline magnesium [J]. Journal of the Mechanics and Physics of Solids, 2017, 99: 512–538. doi: 10.1016/j.jmps.2016.12.008 [96] WINEY J M, GUPTA Y M. Shock wave compression of hexagonal-close-packed metal single crystals: time-dependent, anisotropic elastic-plastic response of beryllium [J]. Journal of Applied Physics, 2014, 116(3): 033505. doi: 10.1063/1.4889886 [97] RENGANATHAN P, WINEY J M, GUPTA Y M. Shock compression and release of a-axis magnesium single crystals: anisotropy and time dependent inelastic response [J]. Journal of Applied Physics, 2017, 121(3): 035901. doi: 10.1063/1.4974365 [98] FENG B, BRONKHORST C A, ADDESSIO F L, et al. Coupled elasticity, plastic slip, and twinning in single crystal titanium loaded by split-Hopkinson pressure bar [J]. Journal of the Mechanics and Physics of Solids, 2018, 119: 274–297. doi: 10.1016/j.jmps.2018.06.018 [99] FENG B, BRONKHORST C A, ADDESSIO F L, et al. Coupled nonlinear elasticity, plastic slip, twinning, and phase transformation in single crystal titanium for plate impact loading [J]. Journal of the Mechanics and Physics of Solids, 2019, 127: 358–385. doi: 10.1016/j.jmps.2019.03.019 [100] KONDO R, TADANO Y, SHIZAWA K. A phase-field model of twinning and detwinning coupled with dislocation-based crystal plasticity for HCP metals [J]. Computational Materials Science, 2014, 95: 672–683. doi: 10.1016/j.commatsci.2014.08.034 [101] LIU C, SHANTHRAJ P, DIEHL M, et al. An integrated crystal plasticity-phase field model for spatially resolved twin nucleation, propagation, and growth in hexagonal materials [J]. International Journal of Plasticity, 2018, 106: 203–227. [102] LIU G S, MO H X, WANG J, et al. Coupled crystal plasticity finite element-phase field model with kinetics-controlled twinning mechanism for hexagonal metals [J]. Acta Materialia, 2021, 202: 399–416. doi: 10.1016/j.actamat.2020.11.002 -
下载:
下载:
点击查看大图
计量
- 文章访问数: 3244
- HTML全文浏览量: 1330
- PDF下载量: 99
