Density Functional Theory of New Double “A” Layer MAX Phase V2Ga2C under High Pressure
-
摘要: 基于密度泛函理论的第一性原理,研究了压强对双“A”层MAX相V2Ga2C晶体结构、弹性和电子性质的影响,并利用玻恩稳定准则预测了V2Ga2C力学稳定状态下的压强范围。计算结果表明:在0~70 GPa下,V2Ga2C的晶体结构处于力学稳定状态;随着压强的增大,V2Ga2C的晶格常数和体积均有不同程度的缩小,a轴随压强的增大收缩得最快,晶胞体积收缩了24%左右;随着压强的增加,V2Ga2C材料的维氏硬度从0 GPa压强下的18.23 GPa减小为70 GPa压强下的2.30 GPa,在20.15 GPa时从脆性材料转变为韧性材料;V2Ga2C的态密度和能带结构等电子性质随压强的变化较小,即压强对V2Ga2C的电子性质影响不大。Abstract: The structural, elastic and electronic properties of double “A” layer MAX phase V2Ga2C under high pressure were studied by the first-principles calculations of density functional theory, and the stable state of V2Ga2C was predicted by using the Born stability criteria. The results show that V2Ga2C crystal structure at the state of mechanical stability within the pressure range of 0–70 GPa. With the increase of pressure, the lattice parameters and volumes of V2Ga2C decreased. V2Ga2C is more compressible in the a-axis direction than c-axis direction, and the volume shrinks by about 24%. With increasing pressure, Vickers hardness of V2Ga2C material decreased from 18.23 GPa (0 GPa) to 2.30 GPa (70 GPa), and from brittle material transform into ductile material at 20.15 GPa. With the change of pressure, the electronic properties have changed slightly such as density of states and band structures, which have almost no effect on the electronic properties of V2Ga2C.
-
Key words:
- MAX phase /
- V2Ga2C /
- high pressure /
- mechanical stability /
- elastic property /
- electronic property
-
Mn+1AXn相材料是三元层状化合物(简称MAX相材料),其中:M代表Ti、V、Zr等过渡金属元素;A代表A组元素;X代表C或者N;n=1, 2, 3, ···[1]。MAX相这一概念最早由Barsoum[2]提出,这类材料普遍具有陶瓷材料和金属材料的双重特性,可在高压、高温、强腐蚀等极端条件下稳定存在[3-4],并表现出较好的稳定性和抗氧化性,具有极其重要的研究价值和广阔的发展前景[5],因此探究高压等极端状态下的晶体性质变化具有重要意义。
近年来,有关三元层状Mn+1AXn相材料的研究很多,主要集中在211相、312相和413相[6]。随着研究的深入,Mn+1A2Xn双“A”层221相、322相等结构被陆续得到,第一种双“A”层MAX相化合物Mo2Ga2C由Hu等[7]于2015年成功制备,2016年Thore等[8]通过第一性原理计算预测出V2Ga2C的存在,V2Ga2C、Ti3Au2C2等双“A”型MAX相的理论预测和实验制备极大地丰富了MAX族化合物[9]。V2Ga2C 是典型的由理论预测得到的新型双“A”层MAX相材料, Thore等[10]根据声子谱没有虚频判定V2Ga2C具有稳定结构,研究发现这类双“A”层的MAX相材料普遍具有较强的金属性,如更好的机械延展性、易于加工等[11]。目前,常压下V2Ga2C的研究日趋丰富,受限于实验条件的复杂性,高压下V2Ga2C的结构、电子、弹性等性能研究较为困难,为此基于密度泛函理论的第一性原理计算能够很好地解决这一问题。
本研究通过第一性原理对V2Ga2C六方结构的能带结构、态密度等电子结构和弹性性能等力学性质进行计算,根据玻恩稳定准则等相关理论,预测高压状态下V2Ga2C结构的力学稳定性,并对高压下V2Ga2C的晶体结构、电子结构和弹性性质等进行分析,为新型双“A”型Mn+1A2Xn相的相关研究提供理论参考。
1. 计算方法与参数
采用第一性原理计算方法,运用基于密度泛函理论的Materials Studio软件中的CASTEP量子力学程序[12-13],选用倒易点阵空间表征的Cepeley-Alder超软赝势[14]。利用总能量的平面波赝势替代离子势,并通过广义梯度近似(Generalized gradient approximation, GGA)中的PBE(Perdew, Burke and Ernzerhof)[15-16]方法对电子间的相互作用和相关势进行校正。为确保总能量和原子间的作用力最小化,采用Broyden-Fletcher-Goldfarb-Shanno(BFGS)算法,布里渊区K点网格数为17 × 17 × 3,平面波截断能选取550 eV。进行原胞的几何优化(Geometry)时,能量收敛标准为5×10−6 eV/atom,最大作用力为0.01 eV/Å,应力偏差小于0.02 GPa,自洽场收敛精度为5×10−7 eV/atom。
2. 结果与讨论
2.1 晶体结构
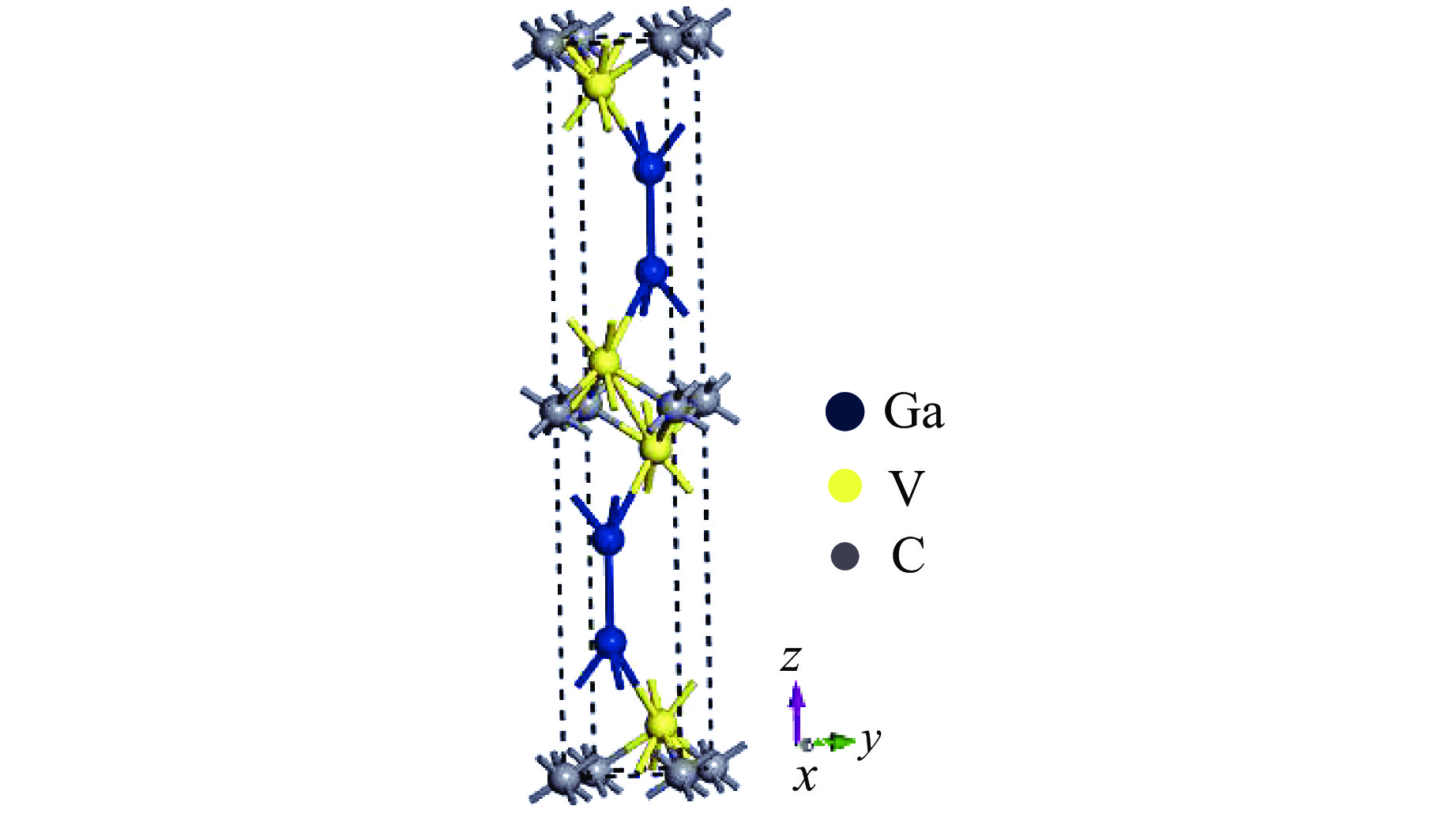
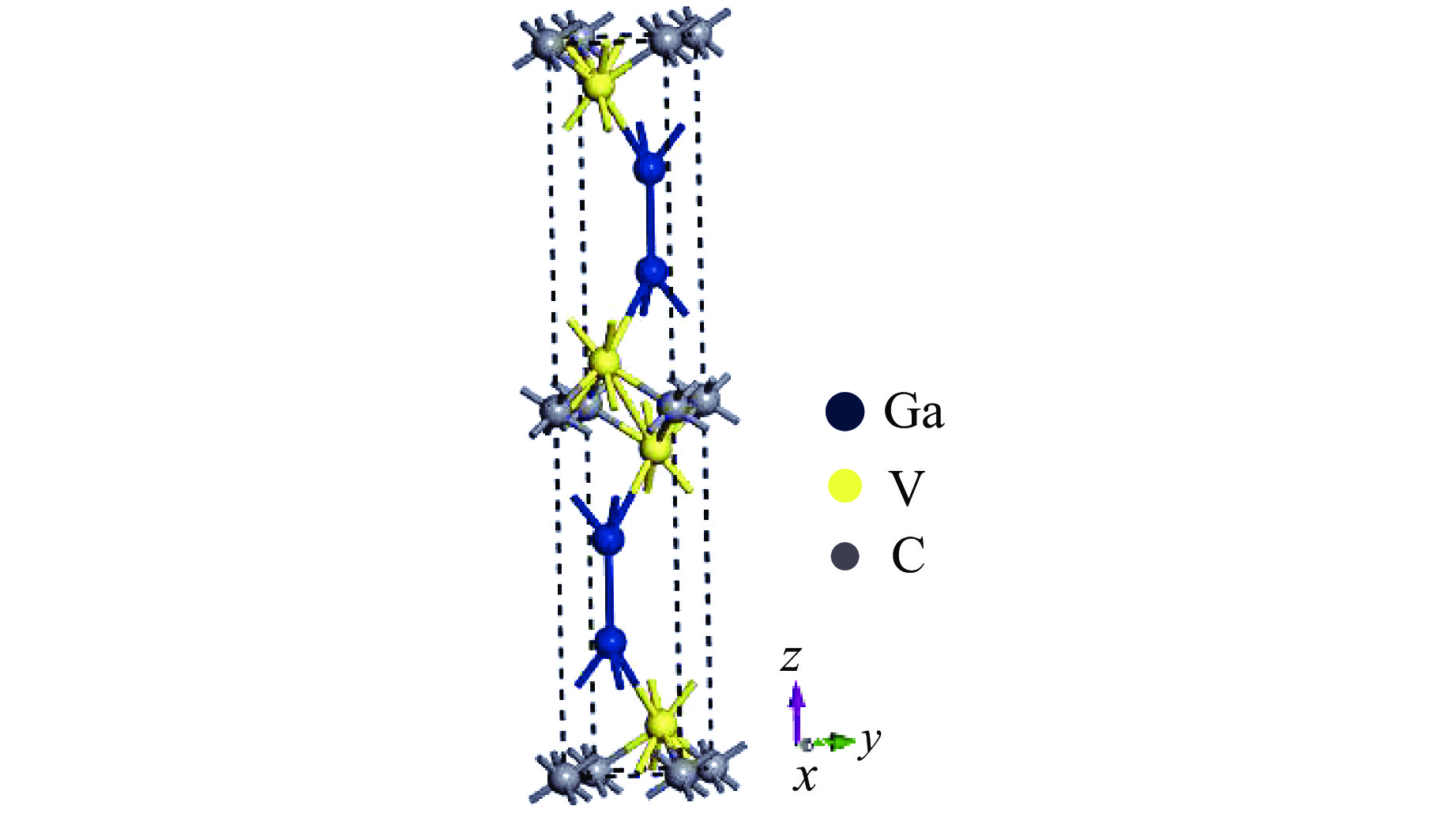
V2Ga2C为六方晶系,空间群是P63/mmc,每个晶胞有10个原子,晶体结构见图1。V2Ga2C晶体与常见的V2GaC的结构和性质类似,晶胞键角
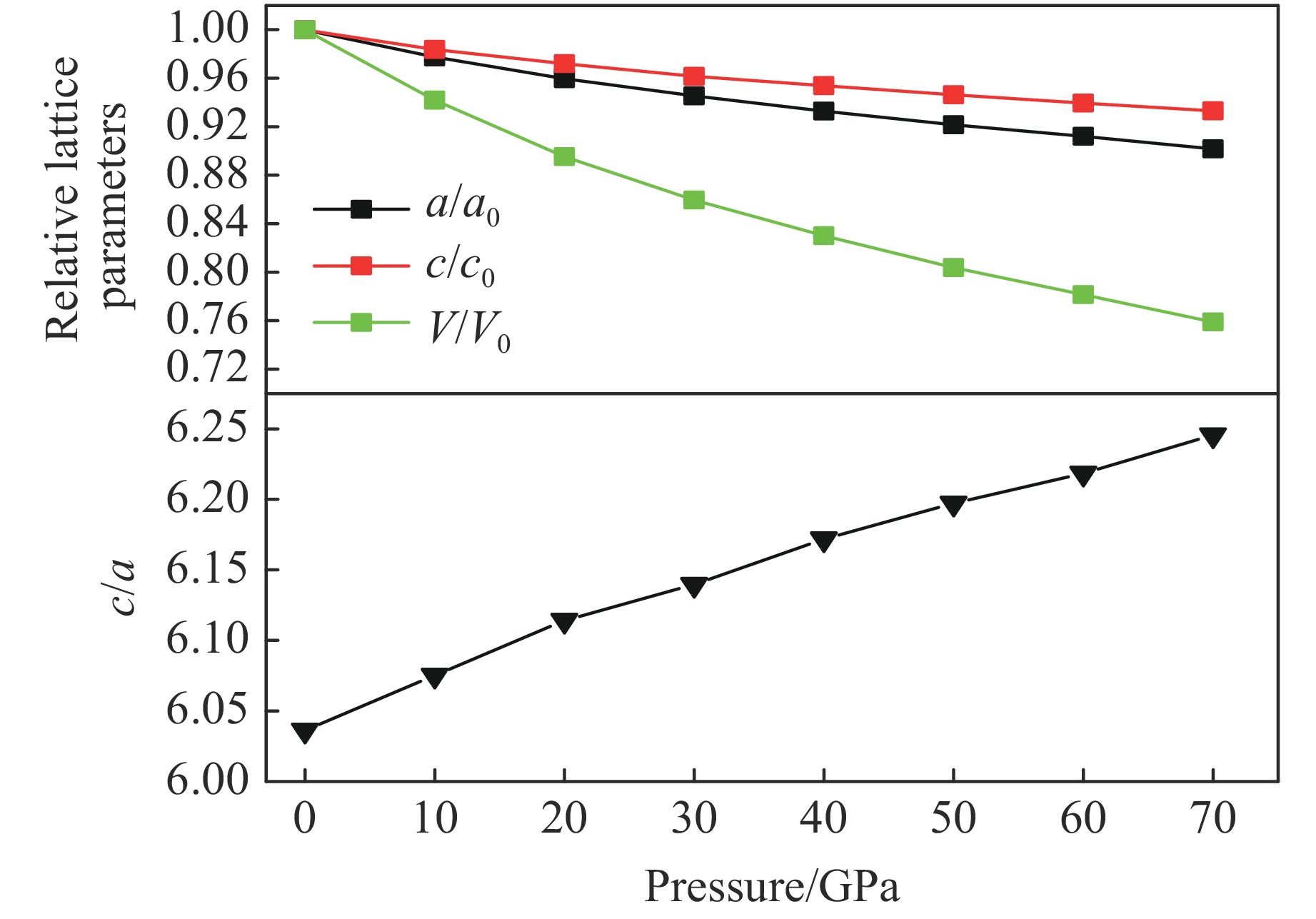
α =β =90°,γ =120°,不同的是V2Ga2C有双Ga层结构及不同的晶胞键长a(a=b)、c,V2Ga2C晶胞中各原子坐标为V(1/3, 2/3, 0.0645)、Ga(1/3, 2/3, 0.6814)、C(0, 0, 0)。经优化计算,得到V2Ga2C的晶胞参数为a=b=2.950 Å,c=17.807 Å,与Thore等[8]计算得到的数据(a=b=3.064 Å,c=18.153 Å)基本一致,即本研究构建的模型是准确可行的。为了研究高压对V2Ga2C晶胞结构的影响,在0~70 GPa压强范围内以10 GPa为间隔进行结构优化,得到V2Ga2C晶胞的相对晶格参数变化情况,见图2。从图2可以看出,随着压强增大,晶格常数a、c和体积V均有不同程度的减小,同时在压强范围内V2Ga2C晶胞表现出较好的可压缩性,其中相对键长比a/a0和c/c0从1逐渐减小到0.9019和0.9331,相对晶格参数c/a从0 GPa的6.0362上升到70 GPa的6.2455,c轴较a轴随压强增大收缩得较慢,且键长的减小导致了晶胞体积V的缩小,上述晶胞参数的变化均体现了V2Ga2C的各向异性。此外,根据计算得到的V2Ga2C晶胞在不同压力下的晶格参数及相对晶格常数a/a0、c/c0、c/a和相对晶胞体积V/V0的变化趋势平缓,可判定在0~70 GPa压力范围内V2Ga2C很难发生相变,即本研究利用图1的V2Ga2C结构探究压力对其电子性质、弹性性质的影响是合理准确的。
2.2 高压下的力学稳定性
力学稳定性是晶体材料稳定存在的重要因素。为研究压强对V2Ga2C晶胞力学稳定性的影响,从0 GPa开始,以每10 GPa为一个间隔进行结构优化,通过不同压强下的弹性常数预测V2Ga2C晶胞的力学稳定性。通过各个压强状态下V2Ga2C晶胞的结构优化,得到0~80 GPa不同压强状态下的晶体结构,各压强状态下的弹性常数见表1。
表 1 不同压强下V2Ga2C的弹性常数Table 1. Pressure dependences of elastic constants for V2Ga2CPressure/GPa C11/GPa C33/GPa C44/GPa C12/GPa C13/GPa 0 275.07 309.04 88.92 65.69 48.31 10 325.89 437.85 103.70 79.38 101.23 20 392.18 492.57 112.70 113.70 122.66 30 475.27 582.90 99.80 176.94 174.81 40 462.17 636.80 103.00 156.69 180.10 50 524.87 696.25 51.41 228.45 221.66 60 558.67 743.34 29.53 220.05 219.34 70 640.36 854.90 4.53 280.99 275.36 80 618.66 892.67 −84.84 305.13 305.24 弹性常数是晶体对作用力反应最直观的数据体现。根据V2Ga2C的晶胞结构,V2Ga2C晶体的弹性常数具有对称性,即C11= C22,C13=C31=C32=C23,C12=C21,C44=C55。由表1数据可知,弹性常数C11、C33随压强的增大逐渐增大,C44先增大后逐渐变小直至减小到负数,C12、C13和C66也有不同程度的增大。V2Ga2C晶胞为六方晶系,因此可以通过玻恩稳定准则[17]、正交系统的力学稳定性公式[18]以及弹性常数的变化规律,预测V2Ga2C六方三元层状化合物的力学稳定性。
玻恩稳定准则可写为
C12>0,C33>0,C44>0,C11−C12>0,(C11+C12)C33−2C213>0 (1) 验证V2Ga2C晶胞正交系统力学稳定性的公式为
Cij>0(i=j,0⩽i⩽6),C11+C22−2C12>0,C11+C33−2C13>0,C22+C33−2C23>0,C11+C22+C33+2C12+2C13+2C23>0 (2) 将表1的弹性常数代入式(1)、式(2),可知V2Ga2C晶胞在0~70 GPa符合式(1),在80 GPa时不符合式(1)。因此六方V2Ga2C晶胞的的弹性常数在0~70 GPa压强范围内处于力学稳定状态,80 GPa下V2Ga2C晶胞结构不稳定。
2.3 弹性性质
为了研究压强对V2Ga2C晶体弹性性质的影响,在不同的压强下对晶胞结构进行优化,在此基础上计算不同压强状态下的弹性常数(见表1)。弹性常数C11、C22和C33分别表示晶胞受压沿a、b和c轴的线性压缩阻力,C11、C22较小而C33最大,说明V2Ga2C在a、b轴上容易压缩,在c轴上难压缩;弹性常数C44、C55和C66与材料抗剪切变形能力有关,C44还与硬度有关,随压强增大而减小的C44表明V2Ga2C材料抵抗形变的能力一般。
根据Voigt-Reuss-Hill近似理论[19],V2Ga2C的体积弹性模量B的最大值BV、最小值BR和平均值BH,以及剪切弹性模量G的最大值GV、最小值GR和平均值GH可以通过式(3)~式(8)得到
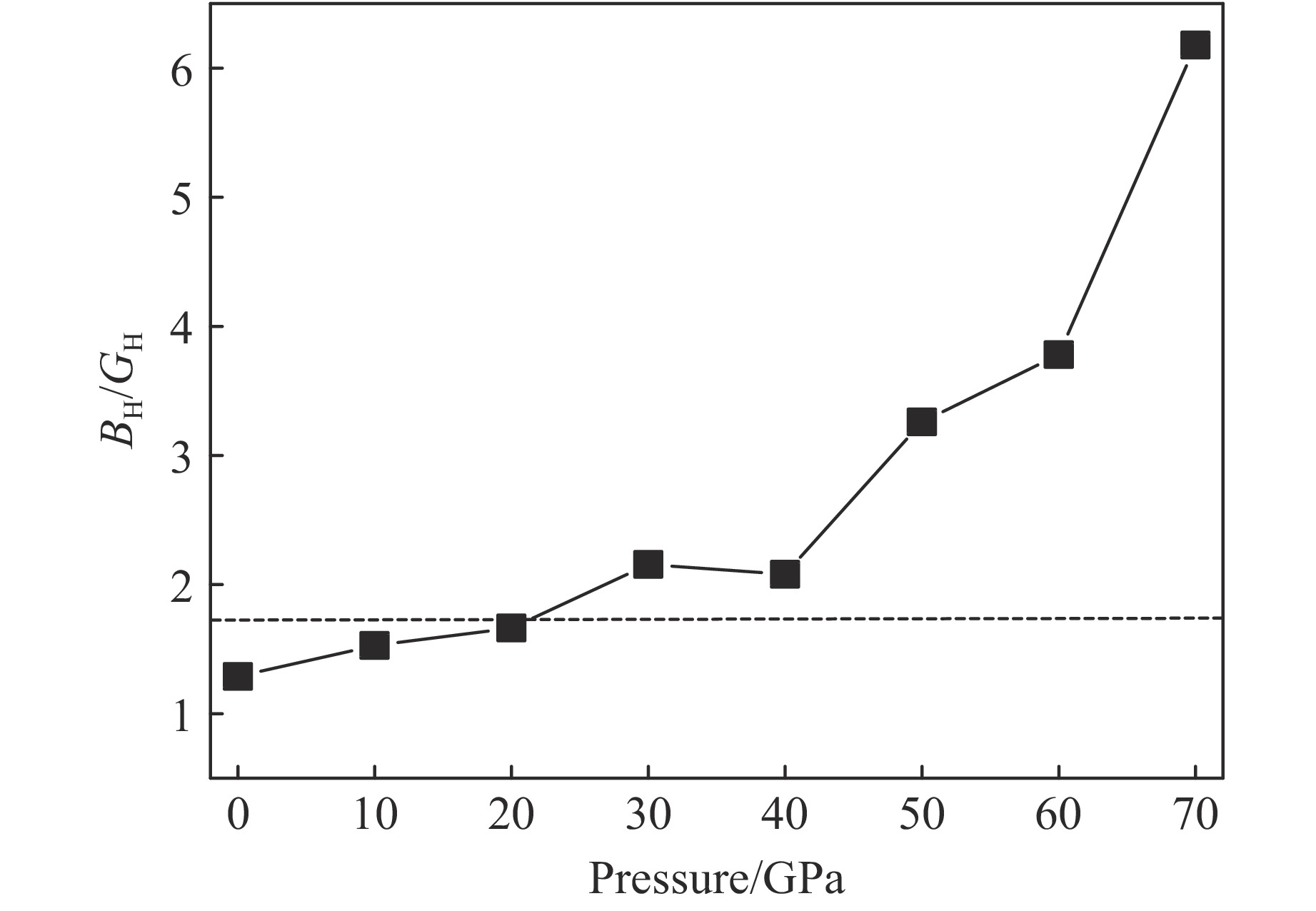
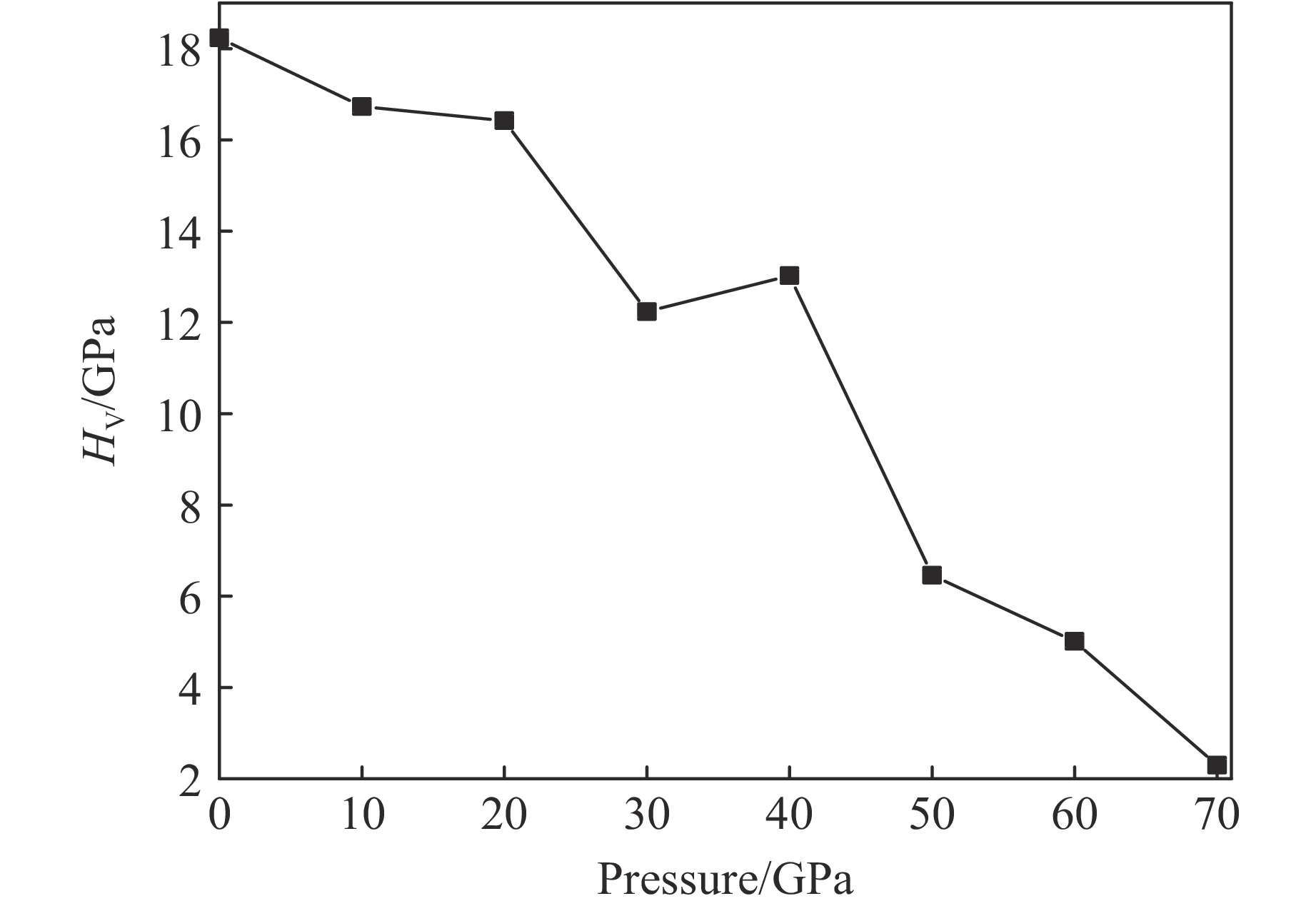
BV=2(C11+C12)+C33+4C139 (3) BR=(C11+C12)C33−2C213C11+C12+2C33−4C13 (4) BH=BV+BR2 (5) GV=C11+C12+2C33−4C13+12C55+12C663 (6) GR=52[(C11+C12)C33−2C213]C55C663BVC55C66+[(C11+C12)C33−2C213](C55+C66) (7) GH=GV+GR2 (8) 根据Pugh准则[17]可以鉴别晶体的韧脆性,BH/GH < 1.74为脆性材料,相反为韧性材料。根据表1弹性常数和式(3)~式(8),可以计算体积模量BH和剪切模量GH,得到压强与BH/GH的关系曲线,见图3。从图3可以明显看出,压强小于20.15 GPa时,V2Ga2C表现为脆性材料,压强为20.15~70.00 GPa时表现为韧性材料。此外,通过式(9)可以预测维氏硬度(HV)的变化(其中K = GH/BH,HV的单位为GPa),维氏硬度随压强的变化见图4。从图4中曲线的变化趋势可以看出,维氏硬度随着压强的增大逐渐变小,原因是V2Ga2C晶胞的键长和a、b轴随压强的增大急剧压缩,故维氏硬度随之减小。
HV=0.92K1.137G0.708H (9) 然而遗憾的是,目前公开发表的有关V2Ga2C在高压状态下的力学性能研究报道较少,难以与本计算得到的理论预测进行对比分析。
2.4 电子性质
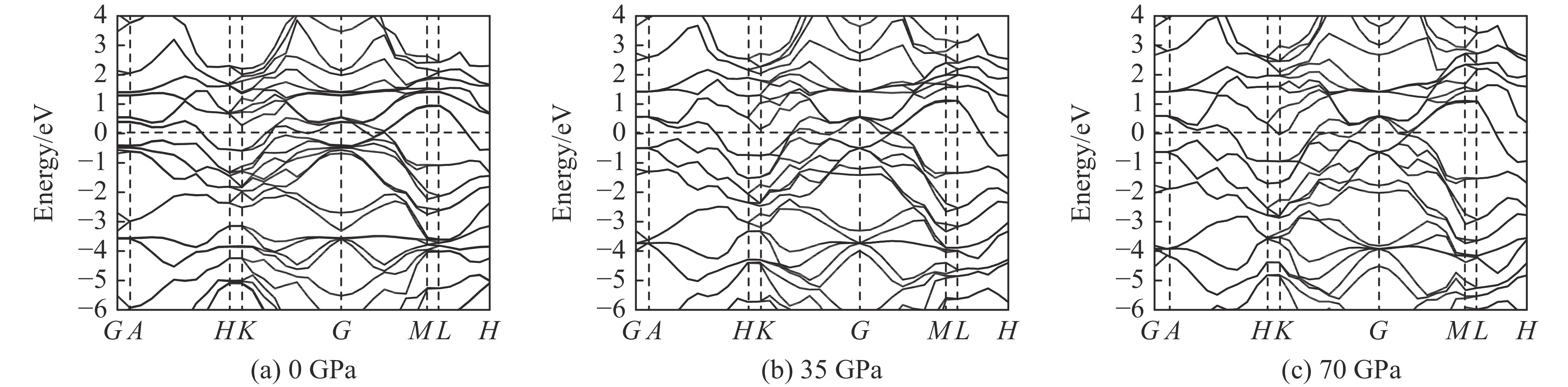
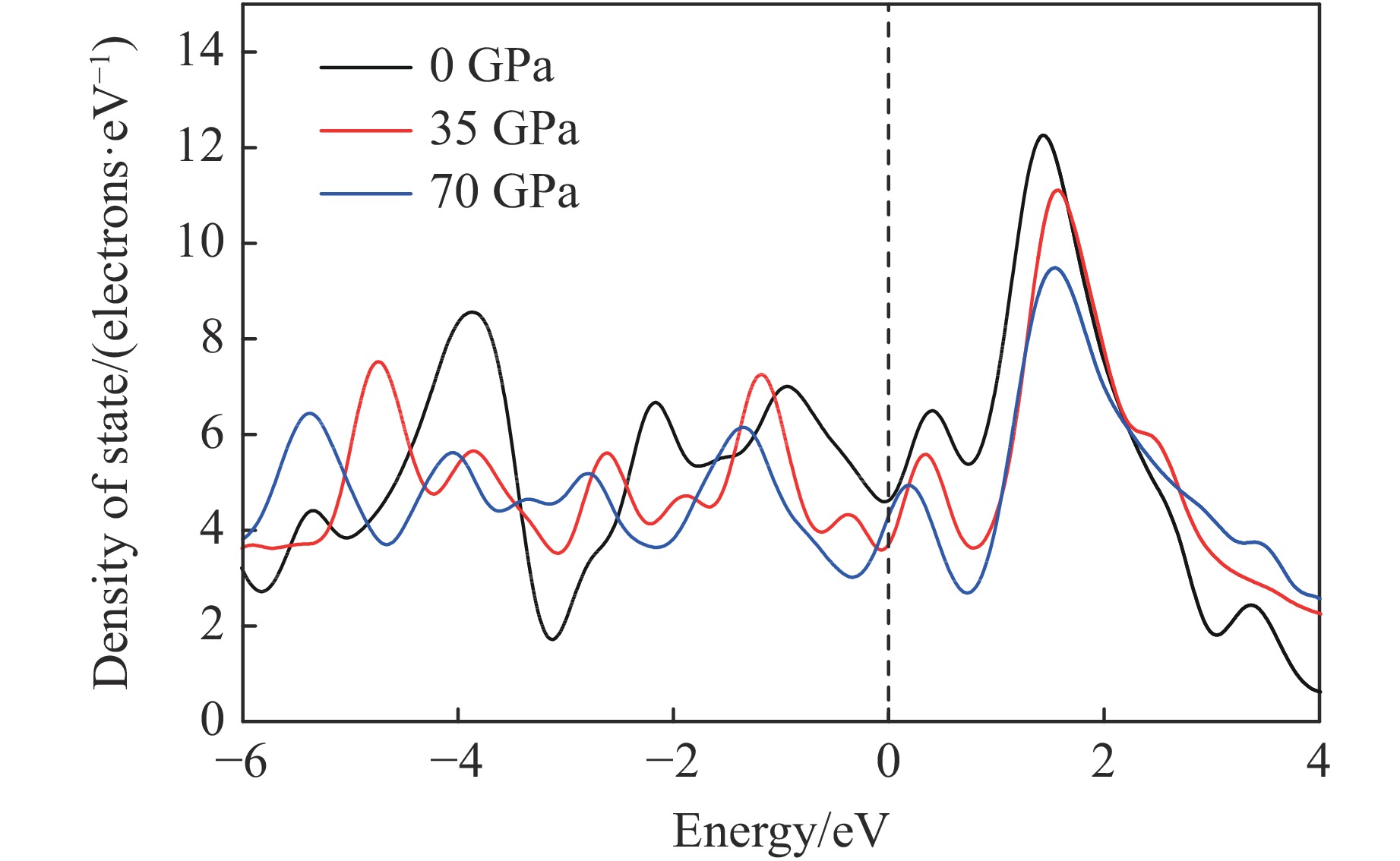
为探究压强与V2Ga2C电子性质的关系,在0~70 GPa的压强范围内通过GGA-PBE密度泛函理论计算,得到V2Ga2C的能带结构图、电子总态密度图,其中0 eV处的虚线表示费米能。
下面以0、35和70 GPa的能带结构为例进行分析,如图5所示。从图5可以明显看出,0 GPa下V2Ga2C无带隙,35 GPa下仍无带隙,直到接近力学稳定临界状态的70 GPa下仍未产生带隙,总体上能带曲线仅有很小幅度的变化。由此可知,在力学稳定范围内,V2Ga2C均无带隙,且压强的增加对能带结构的影响很小,即V2Ga2C材料为导体材料且压强对其影响较小或几乎没有影响。
电子态密度也是V2Ga2C电子性质的重要组成部分,选取0、35和70 GPa状态下的电子态密度分析压强与电子态密度的关系,见图6。由图6可知,随着压强增大,V2Ga2C的总态密度在费米能级附近变动较小,对电子性质影响较小。
3. 结 论
基于密度泛函理论的第一性原理,研究了压强对V2Ga2C晶体的力学稳定性及压强对V2Ga2C结构、弹性和电子性质的影响。根据玻恩稳定准则预测了V2Ga2C结构稳定存在的压强区间为0~70 GPa,并通过正交系统的力学稳定公式验证结果可靠。同时,研究了0~70 GPa压强下V2Ga2C的晶体结构、弹性性质与电子结构,压强使V2Ga2C压缩,体积、相对晶胞参数a/a0、c/c0等均有不同程度的减小,都体现了V2Ga2C具有各向异性;随着压强增大,通过弹性常数可知V2Ga2C在a、b轴上较c轴易压缩,且在20.15 GPa时从韧性转变为脆性,其硬度也随之变小;从V2Ga2C在各个压强状态下的态密度和能带结构可知,在力学稳定的条件下压强对V2Ga2C材料的电子性质影响不大。然而目前有关V2Ga2C材料的研究较少,希望本研究结果可以为双“A”型MAX相材料的实验制备和理论研究提供参考。
-
表 1 不同压强下V2Ga2C的弹性常数
Table 1. Pressure dependences of elastic constants for V2Ga2C
Pressure/GPa C11/GPa C33/GPa C44/GPa C12/GPa C13/GPa 0 275.07 309.04 88.92 65.69 48.31 10 325.89 437.85 103.70 79.38 101.23 20 392.18 492.57 112.70 113.70 122.66 30 475.27 582.90 99.80 176.94 174.81 40 462.17 636.80 103.00 156.69 180.10 50 524.87 696.25 51.41 228.45 221.66 60 558.67 743.34 29.53 220.05 219.34 70 640.36 854.90 4.53 280.99 275.36 80 618.66 892.67 −84.84 305.13 305.24 -
[1] WAI-YIM C, YUXIANG M, ARYAL S, et al. Intrinsic mechanical properties of 20 MAX-phase compounds [J]. Journal of the American Ceramic Society, 2013, 96(7): 2292–2297. doi: 10.1111/jace.12376 [2] BARSOUM M W. The M N+1AXN phases: a new class of solids [J]. Progress in Solid State Chemistry, 2000, 28(1/2/3/4): 201–281. doi: 10.1016/S0079-6786(00)00006-6 [3] BARSOUM M W, BRODKIN D, EL-RAGHY T. Layered machinable ceramics for high temperature applications [J]. Scripta Materialia, 1997, 36(5): 535–541. doi: 10.1016/S1359-6462(96)00418-6 [4] SONG G M, LI S B, ZHAO C X, et al. Ultra-high temperature ablation behavior of Ti2AlC ceramics under an oxyacetylene flame [J]. Journal of the European Ceramic Society, 2011, 31(5): 855–862. doi: 10.1016/j.jeurceramsoc.2010.11.035 [5] JIAO Z Y, MA S H, WANG T X. High-pressure phase stability, mechanical properties and bonding characteristics of Ti4GeC3 compound [J]. Solid State Sciences, 2015, 39: 97–104. doi: 10.1016/j.solidstatesciences.2014.12.003 [6] HOPFELD M, GRIESELER R, VOGEL A, et al. Tribological behavior of selected M n+1AXn phase thin films on silicon substrates [J]. Surface and Coatings Technology, 2014, 257: 286–294. [7] HU C F, LAI C C, TAO Q, et al. Mo2Ga2C: a new ternary nanolaminated carbide [J]. Chemical Communications, 2015, 51(30): 6560–6563. doi: 10.1039/C5CC00980D [8] THORE A, DAHLQVIST M, ALLING B, et al. Phase stability of the nanolaminates V2Ga2C and (Mo1- xVx)2Ga2C from first-principles calculations [J]. Physical of Materials Science, 2016, 51(18): 12682–12688. [9] FASHANDI H, LAI C C, DAHLPVIST M, et al. Ti2Au2C and Ti3Au2C2 formed by soild state reaction of gold with Ti2AlC and Ti3AlC2 [J]. Chemical Communications, 2017, 53(69): 9554–9557. doi: 10.1039/C7CC04701K [10] THORE A, DAHLQVIST M, ALLING B, et al. Temperature dependent phase stability of nanolaminated ternaries from first-principles calculations [J]. Computational Materials Science, 2014, 91(2): 251–257. [11] 金森, 周爱国, 胡前库, 等. 三元碳化物Mo2Ga2C及其二维衍生物的研究进展 [J]. 硅酸盐通报, 2020, 39(3): 866–872.JIN S, ZHOU A G, HU Q K, et al. Progress in ternary carbide Mo2Ga2C and its two-dimensional derivatives [J]. Bulletin of the Chinese Ceramic Society, 2020, 39(3): 866–872. [12] CLARK S J, SEGALL M D, PICKARD C J, et al. First principles methods using CASTEP [J]. Zeitschrift für Kristallographie-Crystalline Materials, 2005, 220(5): 567–570. [13] SEGALL M D, LINDAN P J D, PROBERT M J, et al. First-principles simulation: ideas, illustrations and the CASTEP code [J]. Journal of Physics Condensed Matter, 2002, 14(11): 2717–2744. doi: 10.1088/0953-8984/14/11/301 [14] CHEN Z Y, ALBRIGHT P C, SENGERS J V. Crossover from singular critical to regular classical thermodynanic behavior of fluids [J]. Physical Review A, 1990, 41(6): 3161–3177. doi: 10.1103/PhysRevA.41.3161 [15] PERDEW J P, BURKE K, ERNZERHOF M. Generalized gradient approximation made simple [J]. Physical Review Letters, 1996, 77(18): 3865. doi: 10.1103/PhysRevLett.77.3865 [16] KRESSE G, JOUBERT D. From ultrasoft pseudopotentials to the projector augmented-wave method [J]. Physical Review B, 1999, 59(3): 1758–1775. doi: 10.1103/PhysRevB.59.1758 [17] PUGH S F. XCII. Relations between the elastic moduli and the plastic properties of polycrystalline pure metals [J]. Philosophical Magazine, 1954, 45(367): 823–843. [18] HUANG X, NAUMOV I I, RABE K M. Phonon anomalies and elastic constants of cubic NiAl from first principles [J]. Physical Review B, 2004, 70(6): 064301. [19] HILL R. The elastic behaviour of a crystalline aggregate [J]. Proceedings of the Physical Society, 1952, 65(5): 349–354. doi: 10.1088/0370-1298/65/5/307 -







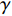

 下载:
下载:

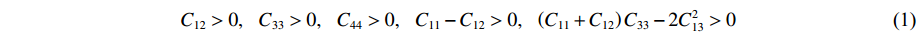
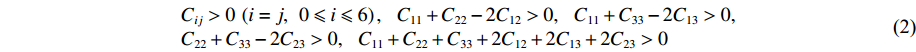

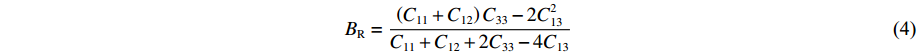
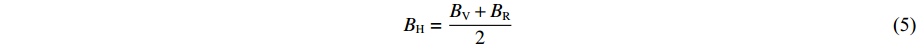

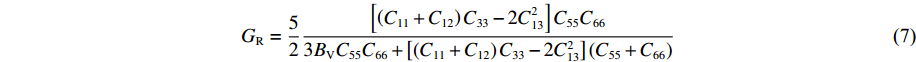






 下载:
下载: