Space Conversion Model of Peak Overpressure in Near-Earth Air Blast Shockwave with Cylindrical Charge
-
摘要: 为研究近地空爆冲击波峰值超压空间数值关系,基于镜像法、角等分和超压归一化思想,确定了冲击波空间传播界线,建立了混合流场中超压的理论计算方法。首先,利用三波点轨迹与爆高水平线交点、虚拟爆源、真实爆心三者连线构成的几何约束以及马赫反射终点条件,确定了冲击波流场分布界限。其次,等分测点角度,并基于超压归一化值分段线性假设构建归一化值方程。然后将归一化值方程扩展为圆柱装药长径比、爆高、当量、测点角度和比例距离的函数。最后,基于控制变量法,利用符合经验公式和实爆结果的圆柱装药近地空爆AUTODYN-2D数值模型的计算结果代入上述函数求解。结果表明:以长径比、比例爆高、比例距离和测点角度为输入参数的峰值超压空间转换模型可描述圆柱装药近地空爆峰值超压的空间数值关系,转换效果良好。Abstract: The shockwave overpressure is one of the main damage elements of the high energy warhead, and many researchers have paid great attention on it. The spatial propagation boundary of shockwave is determined based on the method of image, division angle and overpressure normalization, and the theoretical calculation method of overpressure in mixed flow field is also established. Firstly, the boundary of shockwave flow field distribution is determined by using the terminal condition of Mach reflection and the geometric constraints formed by connecting three points, including the intersection of triple point trajectory and the horizontal line of height of burst (HOB), the imaginary burst point and real blast center. Secondly, the angle of measuring point (AMP) is equalized and the normalized value equation is constructed based on the piecewise linear assumption of the normalized value of overpressure. Then, the normalized value equation is extended to the functions of the length diameter ratio (k) of cylindrical charge, HOB, equivalent, AMP and scaled distance. Finally, based on the control variable method, the above function is solved by using the calculated results of AUTODYN-2D numerical model of near-earth air blast with cylindrical charge in accordance with the empirical equations and the real explosion results. The results show that the spatial conversion model of peak overpressure with k, scaled HOB, scaled distance and AMP as input parameters can describe the spatial numerical relation of peak overpressure of cylindrical charge in near-earth air blast, and the conversion effect is well.
-
高能战斗部爆炸毁伤威力大,产生的高能量冲击波超压是其杀伤目标的主要毁伤元之一,受到相关研究人员的重视。学者们已提出一系列爆炸冲击波峰值超压的经验公式,如叶晓华公式[1]、Mills公式[2]、Henrych公式[3]、Sadovskyi公式[4]、Brode公式[5]及国防工程设计规范[6]等。上述公式描述了自由场峰值超压的一维分布规律。在近地空爆(本研究中均指比例爆高不小于0.35且不大于3的空中自由场爆炸)中,存在入射波、反射波、马赫波及三波叠加现象,使波阵面峰值超压的空间分布不均匀,无法直接应用上述公式。
根据峰值超压数值大小关系,最大者为三波叠加即三波点超压,其次为马赫波超压。三波点轨迹随冲击波传播的时间演化而逐渐升高。通常有3种确定三波点轨迹、超压及马赫超压的方法。第1种方法是试验法,又可分为两类:一类是通过设置足够多的超压传感器测点,根据测点上的超压变化关系,利用多次试验得到三波点近似位置,同时得到马赫超压[7];另一类是利用高速纹影技术得到爆炸波传播过程图像,从中解读出三波点位置,并推算三波点迹线[8]。该方法的试验成本较大,不易推广。第2种方法是基于Whitham的几何激波动力学理论[9],利用边界条件,计算得到近似解[10-11]。第2种方法及其改进方法已有计算机程序,可在较短的时间内获取三波点迹线及马赫波超压[10]。第3种方法是镜像法[12-14],即将近地爆炸视为真实空间中爆炸与相对于地面对称的虚拟爆炸的叠加,可求得冲击波反射流场参数。与前两种方法相比,第3种方法不仅试验成本较低,而且方便建模分析,尤其适合中小当量装药和爆高较大的工况[15]。
应用上述方法得到马赫反射超压,结合自由场超压经验公式,可在一定程度上描述爆炸冲击波空间分布规律。然而在近地空爆中,目前少有直接研究马赫反射超压与入射波超压空间分布关系的工作。揭示超压空间分布关系,对冲击波超压毁伤元空间威力评估具有重要意义。
为了建立与空间位置相关的近地自由场冲击波超压转换模型,本研究采用镜像法确定与爆高平齐时的三波点位置,将其作为建模起始边界,将马赫反射终点作为建模结束边界。将测点与爆心连接起来,该连线与过爆心的水平面的锐角夹角定义为测点角度,简称测角。在起始和结束边界之间的区域,应用测角等分和超压归一化值分段线性假设得到超压空间归一化关系,从而建立超压空间转换模型,并将模型推广至不同爆高、当量、长径比的圆柱装药近地空爆中。利用符合经验公式和实爆结果的AUTODYN-2D数值模型获取试验数据,确定模型形式并求解系数;通过将模型转换结果与数值结果和实爆数据对比,验证该模型的可靠性。
1. 理论分析
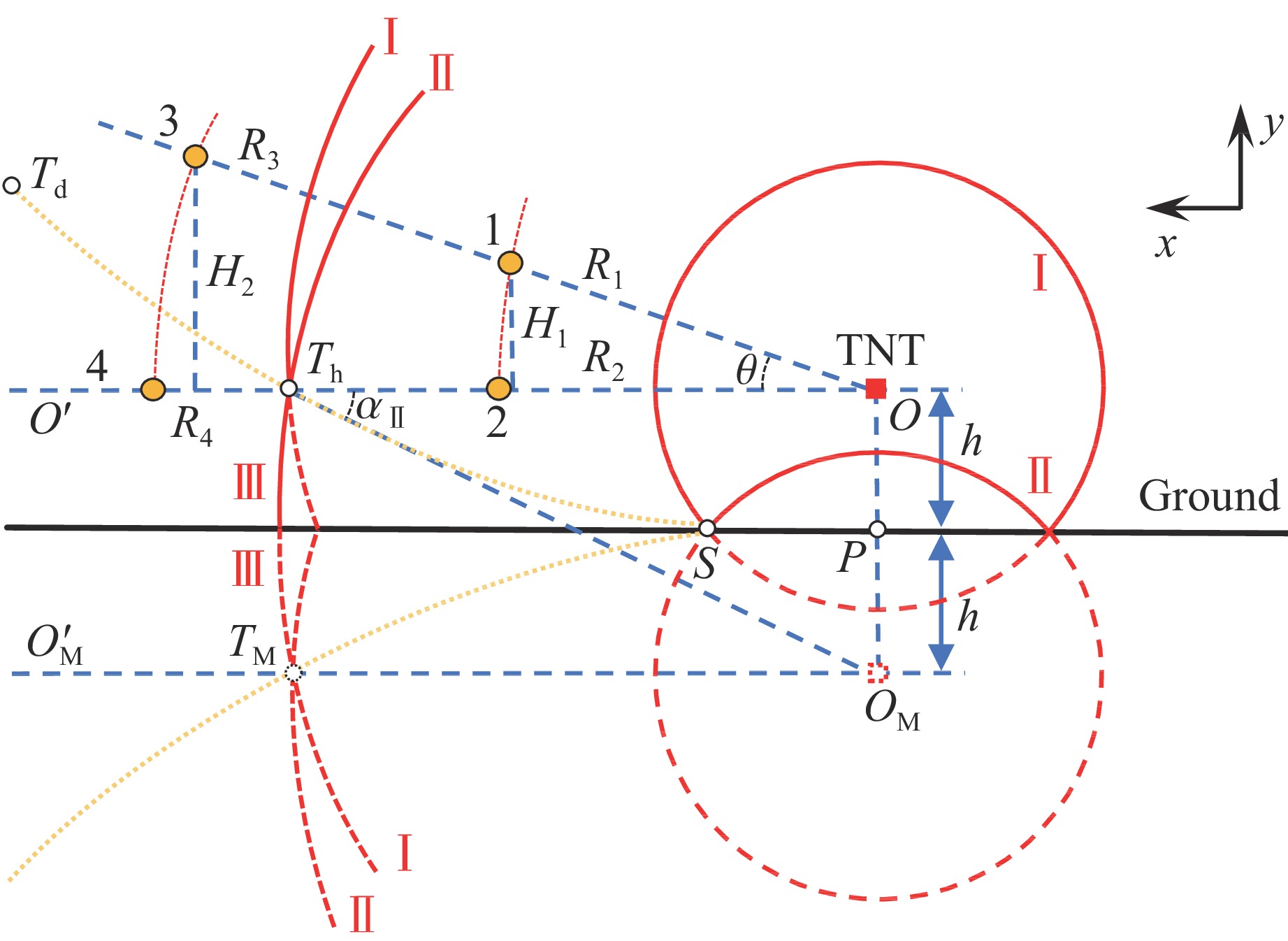
近地空中爆炸产生的冲击波在到达地面前符合自由场传播规律,到达地面后依次经历正反射、正规反射,入射角超过一定角度后发生马赫反射。以长径比为1的圆柱装药TNT为研究对象,采用镜像法分析近地自由场爆炸时空中各点超压关系。如图1所示,爆高为h,爆心为O,爆心在刚性地面投影点为P,虚拟爆源OM与O相对于地面镜像对称。刚好发生马赫反射时,入射波Ⅰ与反射波Ⅱ在地面的交点为S。Th为入射波Ⅰ、反射波Ⅱ和马赫波Ⅲ的交点,位于过爆心的水平线OO′上;TM为虚拟三波点,位于虚拟水平线
OMO′M 上。弧线STd为真实三波点轨迹,STM为虚拟三波点轨迹。直线ThOM与OO′的锐角夹角记为αⅡ,该角与反射波Ⅱ的反射角互为余角。水平线OO′上有测点2,与爆心O的直线距离为R2;测点1与测点2在同一波阵面上,与爆心O的直线距离为R1,两测点高度差为H1,夹角为
θ ;测点3与测点1在过爆心O的同一直线上,与爆心O的直线距离为R3;过水平线OO′上有测点4,与测点3在同一波阵面上,高度差为H2,与爆心O的直线距离为R4。显然,测点4在三波点以下,其超压大于同一波阵面上的测点3;而测点1、测点2和测点3均在三波点轨迹以上,前两者超压接近。测点2处峰值超压可用Sadovskyi公式[4]计算Δp\!Ⅰ=76ˉr+255ˉr2+650ˉr31⩽ˉr⩽15 (1) 式中:
ˉr 为比例距离,是爆心距R与炸药当量m的三次根之比,m·kg−1/3。用R\!Ⅰ 表示OTh长度,R\!Ⅱ 表示OMTh长度,则根据图1的限定条件,点Th处反射角余角α\!Ⅱ 满足cosα\!Ⅱ=R\!Ⅰ/R\!Ⅱ=D\!Ⅰ/D\!Ⅱ=M\!Ⅰ/M\!Ⅱ (2) 式中:DI和DII分别为入射和反射波阵面传播速度,MI和MII为对应的马赫数。马赫数M与超压Δp满足
Δp=p0(1+μ2)(M2−1) (3) 故可建立
α\!Ⅱ 与入射波阵面超压(Δp\!Ⅰ )及反射波阵面超压(Δp\!Ⅱ )的关系cosα\!Ⅱ=√Δp\!Ⅰ+(1+μ2)p0Δp\!Ⅱ+(1+μ2)p0 (4) 式中:p0为标准大气压,p0 = 101.325 kPa;
μ2=(1−γ)/(1+γ) ,理想空气绝热指数γ 取1.4,μ 2为1/6。又由于理想空气正反射中反射波超压Δp\!Ⅱ 和入射波超压Δp\!Ⅰ 有如下关系式Δp\!ⅡΔp\!Ⅰ=2+61+7p0/Δp\!Ⅰ (5) 因此式(4)可表示为
cosα\!Ⅱ=√Δp\!Ⅰ+118.21252Δp\!Ⅰ+6Δp\!Ⅰ1+709.275/Δp\!Ⅰ+118.2125 (6) 将一系列比例距离代入经验公式(如Sadovskyi公式)中可得到一系列超压值,利用
α\!Ⅱ 的约束方程α\!Ⅱ=arctan(R\!Ⅰ/2h) (7) 通过迭代法可求得Th处爆心距
R\!Ⅰ 。按照图1规定坐标轴方向,以P为坐标原点时Th点坐标为(R\!Ⅰ , h)。随着时间推移,Th点之后三波点的纵坐标大于Th处的纵坐标。当马赫反射超压与两倍当量自由场爆炸冲击波峰值超压相等时达到马赫反射终点[16],将马赫反射终点记为Td,如图2所示。
理想空气中马赫波地面超压公式[16]为
{ΔpM=A2[(6Δp\!Ⅰ+118.2125)−118.2125]A=1sinα\!Ⅰ+f[(1−R∗\!ⅠR)cos2α\!Ⅰcos(α\!Ⅰ−α∗\!Ⅰ)sinα\!Ⅰ+R∗\!ⅠRtan(α\!Ⅰ−α∗\!Ⅰ)cosα\!Ⅰ]f=0.13exp(−0.01ˉh)+27/(ˉh+59) (8) 式中:ΔpM为马赫波阵面超压,kPa;
Δp\!Ⅰ 为入射波阵面超压,kPa;α\!Ⅰ 为Td处入射角;R为Td处爆心距;α∗\!\!Ⅰ 为马赫起始处入射角;R∗\!\!Ⅰ 为α∗\!\!Ⅰ 对应的爆心距;ˉh 为比例爆高,即爆高h与炸药当量m的三次方根之比,m·kg−1/3。α∗\!\!Ⅰ (参照式(4))和R∗\!\!Ⅰ 可按式(9)求解{α∗\!Ⅰ=arcsin√Δp\!Ⅰ+118.2125ΔpM+118.2125R∗\!Ⅰ=h/cosα∗\!Ⅰ (9) 同时还有如下约束关系
{cosα\!Ⅰ=hRΔp\!Ⅰ=A1ˉr+A2ˉr2+A3ˉr3ΔpM=3√2A1ˉr+3√4A2ˉr2+2A3ˉr3 (10) 式中:A1、A2和A3为无量纲系数,基于试验数据确定(或直接应用经验公式)。
联立式(8)、式(9)、式(10)可求得Td处R值。为简便起见,用Td在水平线OO′上的垂足
T′d 表示马赫反射终点界限,并将T′d 坐标约定为(R, h)。根据爆炸冲击波对称传播特性,任意垂直于地面且过爆心的截面上分布的冲击波参数均相同。以该截面的一半为研究对象,从而利用Th与
T′d 处平行于y轴的直线分隔待研究对象为3个区域,如图2所示。峰值超压空间分布模型按上述3个区域进行构建。其一为OTh段,边界条件为R0⩽x1⩽R\!Ⅰ, y1⩾h ,其中R0为冲击波脱离爆炸产物的位置,对于球形装药可取为装药半径的12倍。此时三波点在水平线OO′以下,OO′线及以上空间不受马赫反射影响,所测结果的第一个峰值超压为入射波超压,直接对测试结果进行三次多项式拟合即可得到超压预测公式。其二为ThT′d 段,边界条件为R\!Ⅰ⩽x2⩽R, y2⩾h ,此时,在OO′线以上空间既存在马赫反射(如2、4)又存在自由传播(如1、3),由三波点轨迹区分,但三波点轨迹较难得到。其三为Td点外,边界条件为x3⩾R,y3⩾h ,此时超压空间分布数值趋于一致,可采用三次多项式拟合方法处理测试结果,获取超压预测模型。针对区域二超压分布的复杂性,以O为中心,对90°平面进行每15°等分。建立7种测角下峰值超压与比例距离的关系式,然后以90°超压为参考,其他方向峰值超压与参考值求比值,测角
θ 相同时的比值称为峰值超压归一化值,记为yθ。建立该值与测角θ 的线性关系式yθ=aθ+b (11) 根据归一化值的定义,在已知某测角处超压值和式(11)的基础上,可得到任意测角α处相同比例距离的超压,即
Δpα=Δpθyθyα (12) 对式(12)进行扩展,不考虑地面材质、不平度、环境等因素,区域1和区域2中不同空间位置的峰值超压与圆柱装药长径比k、爆高h、当量m及由测角
θ 和比例距离ˉr 表示的空间位置有关。长径比增加引起入射波形状改变,即等压线曲率变化,应对空间不同测角处峰值超压进行修正。以ˉk 表示与长径比有关的修正因子,以比例爆高ˉh 表示爆高和当量的影响,以Δpθ 表示已知测角θ处峰值超压,将待求测角α处峰值超压Δpα 表示为上述影响因素的函数,即Δpα=f(ˉk,ˉh,ˉr,θ,yθ,Δpθ) (13) 2. 数据来源
AUTODYN在爆炸问题的求解上有广泛应用[17-20],利用该软件建立典型环境圆柱装药空中爆炸数值模型并获取数据,利用经验公式和实爆试验数据验证数值模型,利用数值结果完成式(12)的构建,利用数值结果和实爆数据评估所构建的超压转换模型性能。
2.1 AUTODYN数值模型
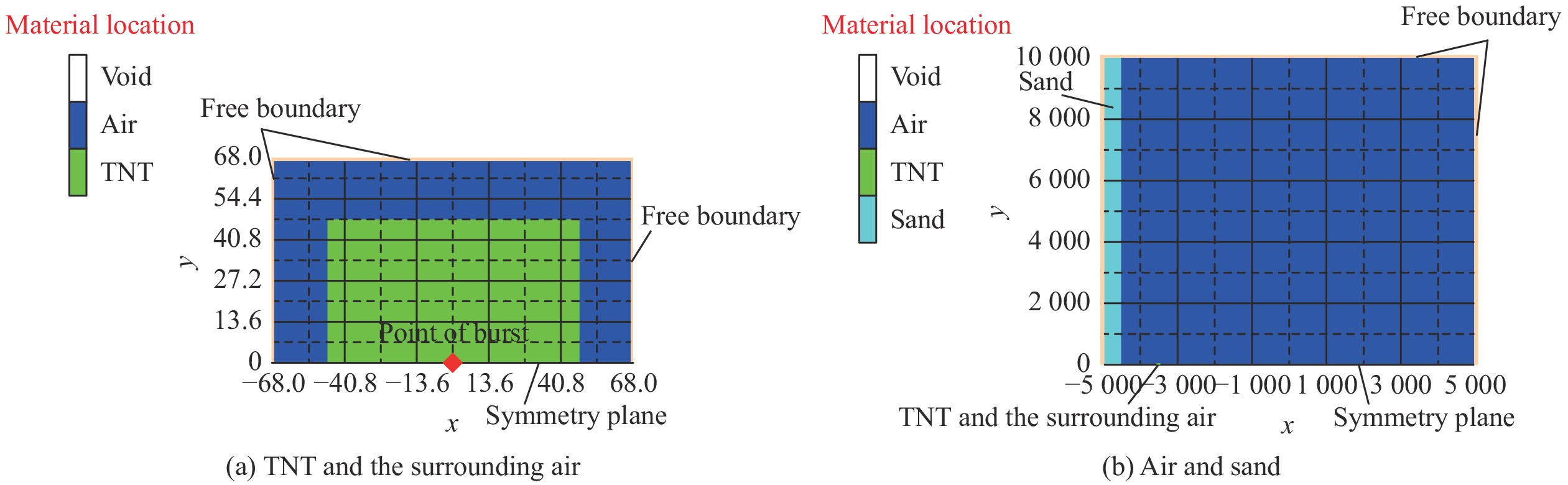
利用冲击波传播的对称性特点,在AUTODYN-2D中构建二维1/2平面模型。研究对象为不同当量、长径比、中心起爆的圆柱装药TNT近地自由场爆炸(比例爆高不小于0.35)。x轴沿TNT模型圆柱轴线方向,如图3(a)所示;地面模型沿y轴放置,并位于空气模型左侧,如图3(b)所示。
空气模型网格粗细与爆炸冲击波求解的精确性正相关,与求解速度负相关。当网格尺寸减小为10和5 mm时,两者的超压时程曲线基本一致[21]。故网格不大于10 mm时可较准确计算超压。为确保冲击波快速精确计算,建立两种尺度和网格粗细的空气模型。第1个空气模型小,仅包裹TNT,网格尺寸为1 mm;第2个空气模型较大,包裹第1个空气模型,网格尺寸为10 mm。空气和地面模型均采用二维多物质欧拉方法求解。利用欧拉和拉格朗日方法实现各模型间的网格自动连接。对称面不设置边界条件,自由边界采用压力流出(Flow-out)类型。计算时间根据TNT当量及所需传播的距离确定,设置为20~150 ms不等。
TNT采用材料库中的“TNT”,密度为1.630 g/cm3;空气模型采用材料库中的“AIR”,内能设置为2.068 × 105 J,密度为1.225 kg/m3;地面模型采用材料库中的“SAND”;其余参数均按照AUTODYN默认设置。采用JWL状态方程描述TNT的爆轰过程。JWL状态方程为
p=A(1−ωR1V)e−R1V+B(1−ωR2V)e−R2V+ωEV (14) 式中:p为爆轰压力,V为相对体积,E为单位体积内能,A、B、R1、R2和
ω 为材料常数。JWL方程参数列于表1[22]。2.2 数值方法验证
以爆心为起点,测点呈辐射状分布,将90°范围的二维仿真平面进行分隔。从0°开始,每15°设置一系列角度相同但比例距离逐渐增大的测点。
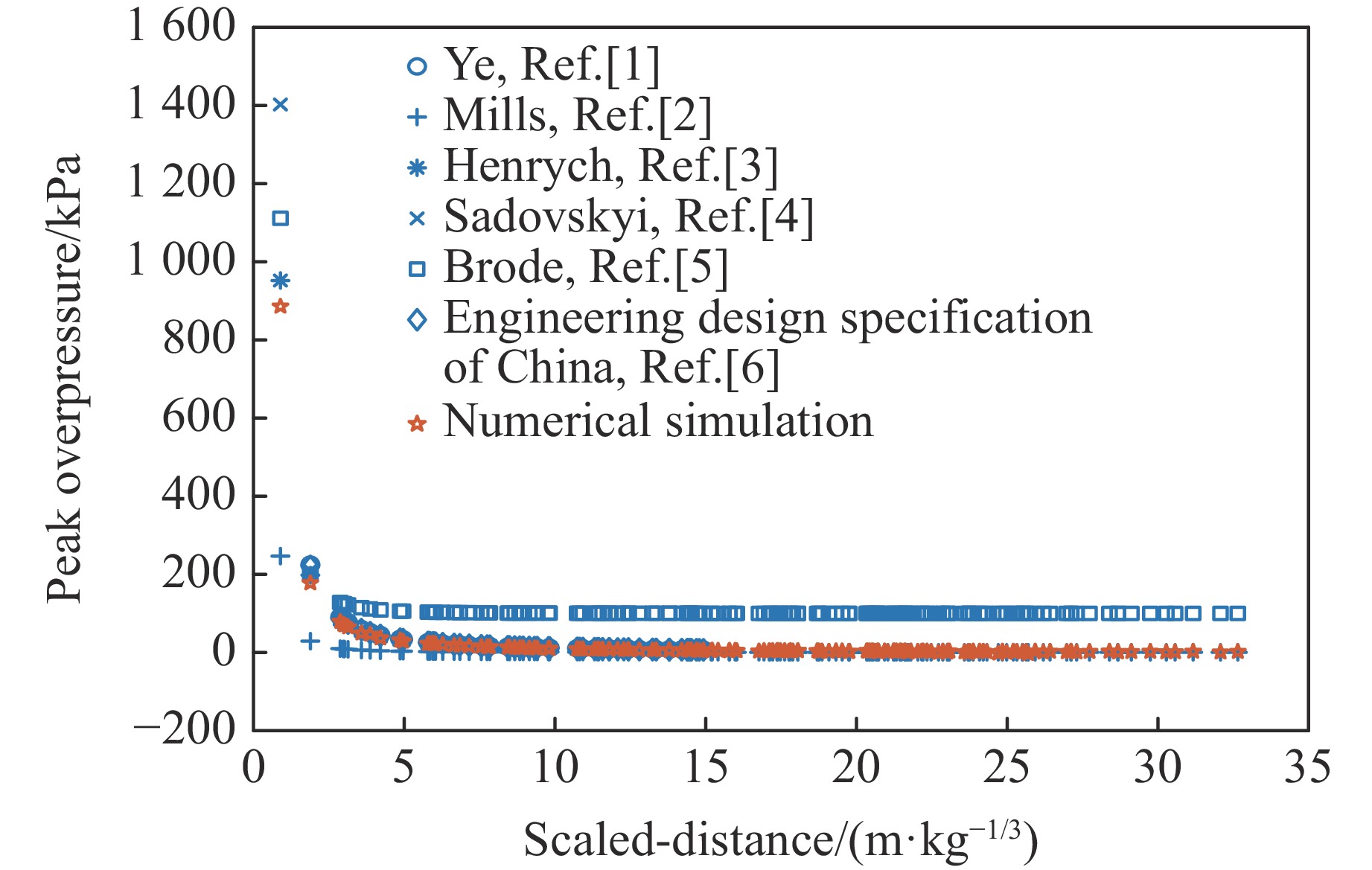
经验公式与TNT实爆试验的入射波峰值超压相符[2],可用经验公式评估数值模型的正确性。将1 kg TNT爆高4 m的数值仿真结果同经验公式求解结果对比,如图4所示,图中横坐标为比例距离,纵坐标为峰值超压;数值结果用五角星表示,经验公式计算结果用其他形状表示。其中,数值仿真结果与Henrych公式的一致性好,平均相对偏差仅为8.9%;与叶晓华公式、Sadovskyi公式及国防工程设计规范的一致性均较好,其中相对于Sadovskyi公式的偏差最小,平均相对偏差为9.8%;相对于国防工程设计规范和叶晓华公式的平均相对偏差分别为15.0%和16.8%。
2020年10月中下旬,团队在中部某靶场进行了10 kg长径比为1.0的圆柱装药TNT空中实爆(爆高1.5 m)试验,地面为平整沙土地,现场微风,天气晴朗,如图5所示。中间悬吊TNT,下方为平整钢板,自由场传感器(PCB137A)与爆高等高并指向爆心。实爆、数值仿真结果及仿真误差如表2所示。表中实测超压值为有效重复试验测试结果的均值,其中工况1和工况2为3次重复试验测试结果的均值,工况3和工况4为两次重复试验结果均值。传感器标定后线性度不大于满量程的1%;以线缆将超压信号传输至存储测试仪中,采样率为1 MHz。相同比例距离时仿真结果偏小;仿真较实爆值的最大偏差约为 −15%;其余测点偏差在−10%以内。
表 2 实爆及数值模拟结果对比Table 2. Real blast and numerical simulation resultsCase No. Scaled distance/(m·kg−1/3) Peak overpressure/kPa Error/% Real blast Numerical simulation 1 3.2 111.8 102.017 −8.75 2 4.2 69.8 64.177 −8.06 3 5.1 47.6 44.663 −6.17 4 6.0 39.3 33.311 −15.24 综上,数值模型与经验公式和实爆结果均较吻合,表明构建的数值模型及相关设置合理,可代替实爆试验作进一步研究。
3. 模型参数求解
3.1 峰值超压与比例爆高及测点位置的关系
为求式(13),首先固定
ˉk 不变,研究峰值超压与ˉh 和(ˉr,θ) 的关系。利用100 kg 长径比为1的圆柱装药TNT在爆高3 m下的试验数据,建立7种测角的峰值超压与比例距离的关系式。7个方程系数项如表3所示。
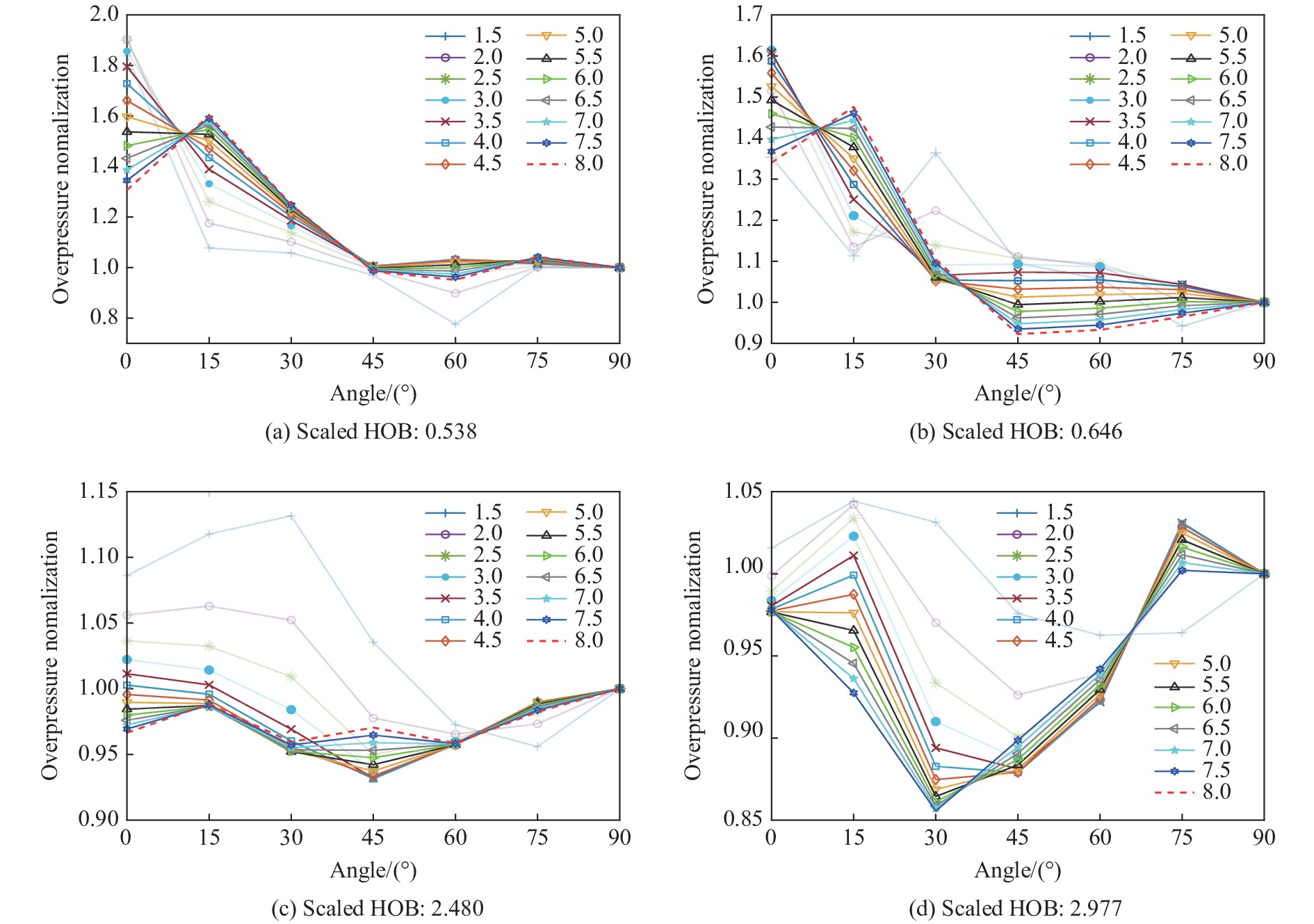
表 3 系数汇总表Table 3. Summary of coefficientsNo. θ/(°) A1 A2 A3 1 0 70.08 764.30 −69.75 2 15 175.40 −38.19 677.70 3 30 137.30 −161.10 1177.00 4 45 67.84 317.10 370.50 5 60 68.28 330.50 315.00 6 75 75.65 315.50 216.10 7 90 99.50 123.10 503.60 以90°测点结果为参考,其他方向峰值超压与参考值求比值。依此方法分别对比例爆高为0.538、0.646、2.480和2.977的4种工况进行计算,所得结果见图6。横坐标表示测角,纵坐标为相同比例距离处峰值超压与90°测点超压之比,即峰值超压归一化值。图6中各曲线对应的比例距离为1.5~8.0。
假设在比例爆高不变的条件下,归一化值与测角呈线性关系;在测角不变时,归一化值与比例爆高呈线性关系;此外,由于45°前后归一化值与比例爆高的关系明显不同,以45°为界分为两段。
固定比例爆高,计算45°内的平均归一化值,将该值对应的测角记为22.5°,然后计算45°处平均归一化值,由此确定45°内归一化值与测角的关系。通过不同比例爆高与22.5°和45°处平均归一化值的线性拟合关系,将比例爆高引入。最终建立的归一化值方程为
yθ_ˉh=(aˉhˉh+bˉh)θ−4522.5−a′ˉhˉh+b′ˉh (15) 式中:
yθ_ˉh 为比例爆高ˉh 时测角θ 的归一化值;θ 的范围为0°~45°;a′ˉh 和b′ˉh 为线性系数,来自于45°处平均归一化值与比例爆高的线性模型;aˉh 和bˉh 由两组线性系数对应求差得到,作为被减数的一组线性系数为a′ˉh 、b′ˉh ,作为减数的线性系数来自于22.5°处平均归一化值与比例爆高的线性模型。基于线性最小二乘法将图6所示典型工况的比例爆高、测角和对应归一化值数据代入式(15),得到对应系数。将45°~90°内平均归一化值对应测角设定为67.5°,同理得到对应方程和系数。完整的归一化值方程为
yθ_¯h={(0.10399¯h−0.323)θ−4522.5−0.04031¯h+1.0350°⩽θ⩽45°(0.02261¯h−0.019)θ−4522.5−0.04031¯h+1.03545°<θ⩽90° (16) 由式(12)可知,在已知长径比为1的圆柱装药比例爆高
ˉh 时测角α 的某比例距离处峰值超压Δpα_ˉh ,求相同比例距离的测角β 处峰值超压Δpβ_ˉh ,超压转换公式为Δpβ_ˉh=yβ_ˉh⋅Δpα_ˉhyα_ˉh (17) 3.2 峰值超压与长径比及测点位置的关系
固定
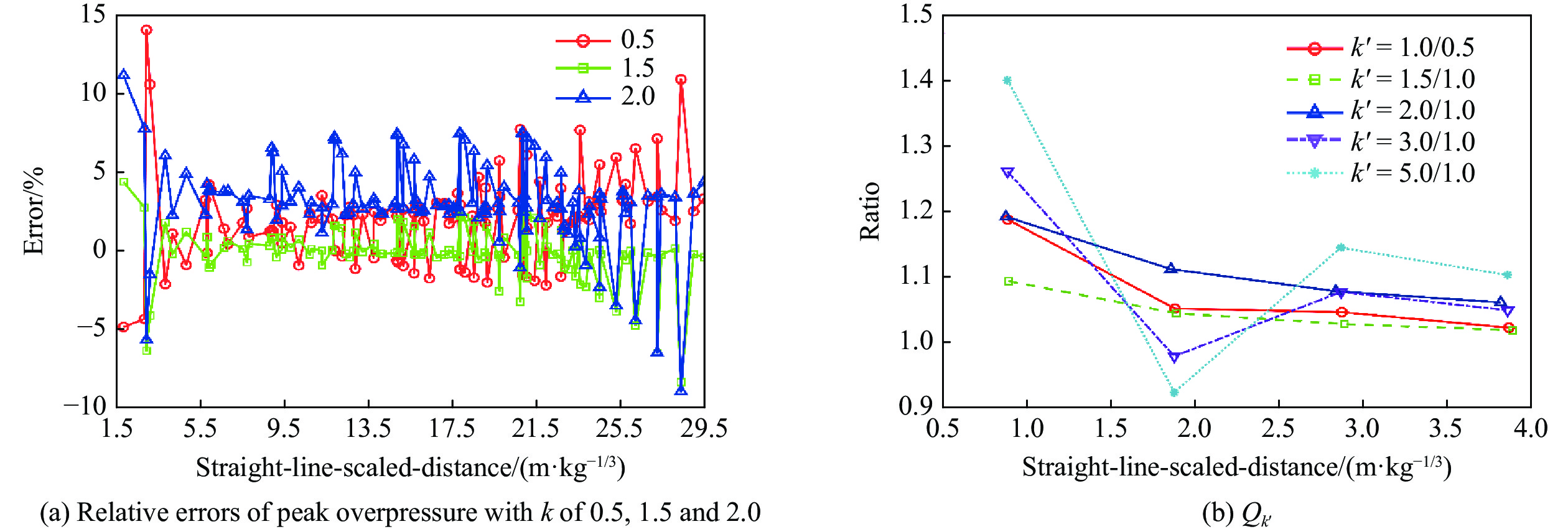
ˉh ,研究峰值超压与ˉk 和(ˉr,θ) 的关系。建立1 kg当量圆柱装药TNT爆高0.5 m,长径比分别为0.5、1.0、1.5和2.0时的数值模型。以长径比1.0时各测点的峰值超压为基准,计算其他长径比时测点峰值超压相对基准的偏差,以该偏差为纵坐标,以比例距离为横坐标,得到图7(a)所示结果。近场最大正偏差约15%,最小负偏差约−7%;中远场最大正偏差约7%,最小负偏差约−3%;远场最大正偏差约10%,最小负偏差在−10%以内。
显然,近场峰值超压受长径比影响最明显;中远场受影响稍小;远场由于其峰值超压接近零,即使差值不大,经比值运算后也会表现出一定误差。本研究主要修正45°内比例距离小于4时的峰值超压转换误差。比例距离小于4时,长径比为1.5、2.0、3.0和5.0与长径比为1.0时对应测角和比例距离处测点的峰值超压求比值,结果记为Qk′,下标k′表示参与求比值的两个长径比,如长径比为5.0与长径比为1.0时对应0°处测点峰值超压之比记为Q5.0/1.0。为便于比较,取长径比0.5与1.0的峰值超压比值之倒数为Qk′,Qk′与比例距离的关系如图7(b)所示。图中展现出的变化趋势较一致,长径比相差小的Qk′较长径比相差大者随比例距离的变化更小。进一步,用线性模型构建Qk′与比例距离的关系。
基于多项式拟合法建立0°处Q1.0/0.5与比例距离的多项式关系
Q1.0/0.5=0.05533¯r2−0.33140¯r+1.451θ=0° (18) 因长径比增加引起轴向峰值超压减小,水平峰值超压增大。故针对某个长径比工况,以Q1.0/0.5为基准,引入长径比修正方程,得到对应长径比k下以长径比1.0为基准的0°测点处的Qk/1;进一步,通过长径比k下任意测角
θ 与0°测点Qk/1的关系,得到长径比k工况下目标测角处的新值,记为Q′k/1 。利用Q′k/1 对已知峰值超压进行转换,即可得到待求长径比、测角和比例距离处的峰值超压。为实现上述目标,计算各长径比时,任意测角
θ 与0°测点的Qk′之比(记为Qθk′ ),显然θ 为0°时Qθk′ 为1。首先固定长径比,构建Qθk′ 与测角θ 的线性模型;然后将模型中自变量θ 前的系数构建为k的线性模型,由此建立起Qθk′ 与θ 、ˉr 和ˉk 的模型。以1 kg圆柱装药TNT爆高0.5 m,长径比分别为0.5、1.0、1.5、2.0、3.0和5.0的工况数据求解模型系数,完整模型表示为Qθk′=(−0.05384k−0.02)θ90+10°⩽θ⩽90°,0.5⩽k⩽5.0 (19) 爆高不变,长径比为1.0时0°测点峰值超压记为
Δp0° ,则k=0.5以及1.5≤k≤5.0时其他长径比和测角的Δpθk 表示为Δpθk={Δp0°Q1/0.5Qθk′0°⩽θ⩽45°and1⩽¯r⩽4Δp0°45°<θ⩽90°or¯r>4 (20) 3.3 转换模型验证
综合3.1节和3.2节,同时考虑爆高和长径比的峰值超压转换关系式可表示为
Δp={yβ_ˉhΔpα_ˉhyα_ˉhk=1.0y0°_ˉhQk′Qβk′Δpα_ˉhyα_ˉhk=0.5,1.5⩽k⩽5.0 (21) 在已知某长径比的圆柱装药空爆时测角
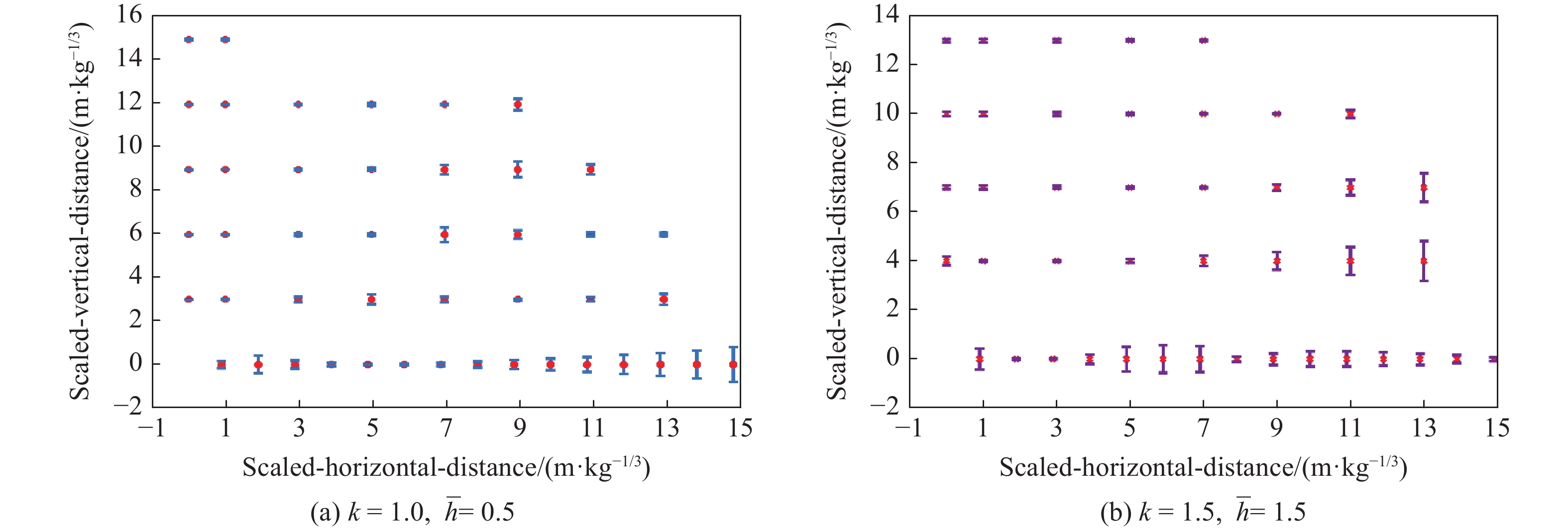
α 某些比例距离处的峰值超压,求约束范围内某工况下各测角处的峰值超压。首先利用数值拟合得到三次多项式形式的超压预测公式,然后利用式(21)实现由已知峰值超压转换为所约束范围内任意长径比和爆高工况下β 测角处的峰值超压。利用仿真数据和实爆结果验证上述超压空间转换模型的可靠性。基于仿真数据,在已知长径比1.0、比例爆高0.5的圆柱装药在90°处峰值超压,求该工况其他测角峰值超压,并求长径比1.5、比例爆高1.5的圆柱装药各测角处峰值超压,将所得结果同原值比较,见图8。
对同一工况其他空间位置峰值超压的转换精度较不同工况时稍高,前者平均相对误差为8.0%,后者为9.6%。两者在水平方向误差大于15%的点数多于其他方向,这是由于水平方向受马赫反射的影响大,超压同其他方向的差异明显。
基于在中部某靶场的同一批次实爆试验结果,求0°处相同比例距离的峰值超压,并与试验数据对比,如表4所示。可见,误差均在20%以内,转换效果良好。
表 4 模型计算结果对比Table 4. Comparison of model calculation resultsm/kg k HOB/m Scaled distance/
(kg·m−1/3)Peak overpressure/kPa Error/% Test value (90°) Test value (0°) Predicted value 20 1.0 1.5 2.58 143.8 221.1 191.3 13.5 3.32 90.3 110.6 92.7 16.2 需要注意的是,转换模型构建方法采用了诸多假设,对于近地爆炸冲击波流场的非线性效应明显的区域,模型转换结果与真实值会存在一定差异。
4. 结 论
基于镜像法,利用与爆高平齐的三波点处特殊几何关系及马赫反射终点条件,确定了冲击波流场分布界限;基于角等分和超压归一化值分段线性假设,建立了超压空间转换模型,并推广至圆柱装药不同长径比、当量和爆高的工况。
通过将数值模型与经验公式和实爆结果对比,验证了所用数值模型的可靠性,同时也验证了超压空间转换模型的合理性和准确性,可为冲击波超压毁伤元空间威力评估提供参考。
-
A/GPa B/GPa R1 R2 ω E/(GJ·m−3) 371.2 3.231 4.15 0.95 0.3 7 表 2 实爆及数值模拟结果对比
Table 2. Real blast and numerical simulation results
Case No. Scaled distance/(m·kg−1/3) Peak overpressure/kPa Error/% Real blast Numerical simulation 1 3.2 111.8 102.017 −8.75 2 4.2 69.8 64.177 −8.06 3 5.1 47.6 44.663 −6.17 4 6.0 39.3 33.311 −15.24 表 3 系数汇总表
Table 3. Summary of coefficients
No. θ/(°) A1 A2 A3 1 0 70.08 764.30 −69.75 2 15 175.40 −38.19 677.70 3 30 137.30 −161.10 1177.00 4 45 67.84 317.10 370.50 5 60 68.28 330.50 315.00 6 75 75.65 315.50 216.10 7 90 99.50 123.10 503.60 表 4 模型计算结果对比
Table 4. Comparison of model calculation results
m/kg k HOB/m Scaled distance/
(kg·m−1/3)Peak overpressure/kPa Error/% Test value (90°) Test value (0°) Predicted value 20 1.0 1.5 2.58 143.8 221.1 191.3 13.5 3.32 90.3 110.6 92.7 16.2 -
[1] 叶晓华. 军事爆破工程[M]. 北京: 解放军出版社, 1995: 216–219.YE X H. Military blasting engineering [M]. Beijing: PLA Press, 1995: 216–219. [2] TOLBA A F F. Response of FRP-retrofitted reinforced concrete panels to blast loading [D]. Ottawa, Canada: Charleton University, 2002. [3] HENRYCH J. 爆炸动力学及其应用 [M]. 熊建国, 译. 北京: 科学出版社, 1987: 127.HENRYCH J. The dynamics of explosion and its use [M]. Translated by XIONG J G. Beijing: Science Press, 1987: 127. [4] SADOVSKYI M A. Mechanical action of air shock waves of explosion, based on experimental data [M]. Moscow: Izd Akad Nauk SSSR, 1952: 1–2. [5] BRODE H L. Numerical solutions of spherical blast wave [J]. Journal of Applied Physics, 1955, 26(6): 766. doi: 10.1063/1.1722085 [6] 李翼祺, 马素贞. 爆炸力学 [M]. 北京: 科学出版社, 1992.LI Y Q, MA S Z. Mechanics of explosion [M]. Beijing: Science Press, 1992. [7] 郭炜, 俞统昌, 金朋刚. 三波点的测量与实验技术研究 [J]. 火炸药学报, 2007, 30(4): 55–57, 61. doi: 10.3969/j.issn.1007-7812.2007.04.014GUO W, YU T C, JIN P G. Test of triple point and study on its test technology [J]. Chinese Journal of Explosives & Propellants, 2007, 30(4): 55–57, 61. doi: 10.3969/j.issn.1007-7812.2007.04.014 [8] 徐彬, 张寒虹, 陈志坚, 等. 球面激波在固壁的马赫反射 [J]. 爆炸与冲击, 1988, 8(1): 25–28.XU B, ZHANG H H, CHEN Z J, et al. Mach reflection of spherical shock wave on rigid wall [J]. Explosion and Shock Waves, 1988, 8(1): 25–28. [9] 赵升, 恽寿榕, 陈权,等. 马赫反射效应在炸药爆轰合成金刚石中的应用 [J]. 高压物理学报, 1997, 11(2): 110–116. doi: 10.11858/gywlxb.1997.02.006ZHAO S, YUN S R, CHEN Q, et al. Using Mach reflection effect in synthesis of ultrafine diamond by detonation wave method [J]. Chinese Journal of High Pressure Physics, 1997, 11(2): 110–116. doi: 10.11858/gywlxb.1997.02.006 [10] 徐彬, 陈志坚, 郭长铭. 球面激波在固壁上马赫反射的数值计算及实验研究(I) [J]. 爆炸与冲击, 1987, 7(3): 223–229.XU B, CHEN Z J, GUO C M. Numerical computation and experiments of Mach reflection of spherical shock wave on rigid wall (I) [J]. Explosion and Shock Waves, 1987, 7(3): 223–229. [11] 彭荣强. 几何激波动力学在激波绕射反射和聚焦中的应用 [J]. 四川工业学院学报, 1996, 15(1): 50–54.PENG R Q. Application of geometrical shock dynamics in shock diffraction reflection and focus [J]. Journal of Sichuan Institute of Technology, 1996, 15(1): 50–54. [12] WU Z, GUO J, YAO X, et al. Analysis of explosion in enclosure based on improved method of images [J]. Shock Waves, 2016, 27(2): 1–9. [13] KONG B, LEE K, LEE S, et al. Indoor propagation and assessment of blast waves from weapons using the alternative image theory [J]. Shock Waves, 2016, 26(2): 75–85. doi: 10.1007/s00193-015-0581-4 [14] KONG X S, WU W G, LI J, et al. Experimental investigation on characteristics of blast load in partially confined cabin structure [J]. Journal of Shanghai Jiaotong University, 2013, 18(5): 583–589. doi: 10.1007/s12204-013-1431-0 [15] EHRHARDT L, BOUTILLIER J, MAGNAN P, et al. Evaluation of overpressure prediction models for air blast above the triple point [J]. Journal of Hazardous Materials, 2016, 311(5): 176–185. [16] 易仰贤. 空爆冲击波马赫反射近似计算 [J]. 爆炸与冲击, 1983, 3(2): 44–49.YI Y X. Approximate calculation of Mach reflection of explosive shock waves in air [J]. Explosion and Shock Waves, 1983, 3(2): 44–49. [17] 陈材, 石全, 尤志锋, 等. 圆柱形弹药空气中爆炸相似性规律 [J]. 爆炸与冲击, 2019, 39(9): 092202. doi: 10.11883/bzycj-2018-0255CHEN C, SHI Q, YOU Z F, et al. Similarity law of cylindrical ammunition explosions in air [J]. Explosion and Shock Waves, 2019, 39(9): 092202. doi: 10.11883/bzycj-2018-0255 [18] 耿振刚, 李秀地, 苗朝阳, 等. 温压炸药爆炸冲击波在坑道内的传播规律研究 [J]. 振动与冲击, 2017, 36(5): 23–29.GENG Z G, LI X D, MIAO C Y, et al. Propagation of blast wave of thermobaric explosive inside a tunnel [J]. Journal of Vibration and Shock, 2017, 36(5): 23–29. [19] 徐森, 刘大斌, 彭金华, 等. 药柱冲击波在有机玻璃中的衰减特性研究 [J]. 高压物理学报, 2010, 24(6): 431–437. doi: 10.11858/gywlxb.2010.06.005XU S, LIU D B, PENG J H, et al. Study on the shock wave attenuation of the booster charge in the PMMA gap [J]. Chinese Journal of High Pressure Physics, 2010, 24(6): 431–437. doi: 10.11858/gywlxb.2010.06.005 [20] 张学伦, 张团, 王昭明. 基于AUTODYN爆炸场三波点的数值模拟 [J]. 兵器装备工程学报, 2015, 36(3): 17–19. doi: 10.11809/scbgxb2015.03.005ZHANG X L, ZHANG T, WANG Z M. Numerical simulation on triple point explosion field with AUTODYN [J]. Journal of Ordnance Equipment Engineering, 2015, 36(3): 17–19. doi: 10.11809/scbgxb2015.03.005 [21] 廖真, 唐德高, 李治中, 等. 近地面空中爆炸马赫反射数值模拟研究 [J]. 振动与冲击, 2020, 39(5): 164–169.LIAO Z, TANG D G, LI Z Z, et al. Numerical simulation for Mach reflection in air explosion near ground [J]. Journal of Vibration and Shock, 2020, 39(5): 164–169. [22] 辛春亮, 徐更光, 刘科种, 等. 考虑后燃烧效应的TNT空气中爆炸的数值模拟 [J]. 含能材料, 2008, 16(2): 160–163. doi: 10.3969/j.issn.1006-9941.2008.02.011XIN C L, XU G G, LIU K Z, et al. Numerical simulation of TNT explosion with post-detonation burning effect in air [J]. Chinese Journal of Energetic Materials, 2008, 16(2): 160–163. doi: 10.3969/j.issn.1006-9941.2008.02.011 -







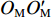

 下载:
下载:
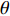
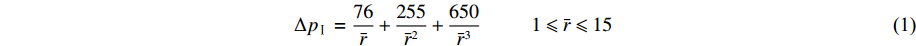
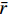
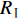
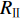
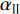
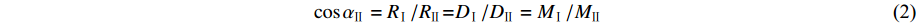
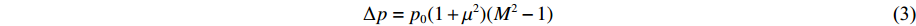
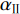
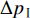
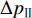
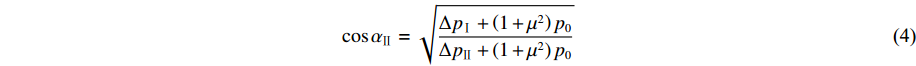
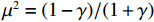
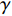
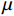
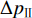
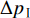
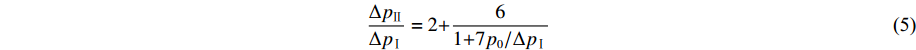






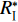
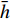
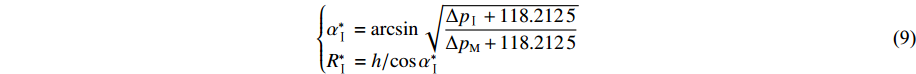
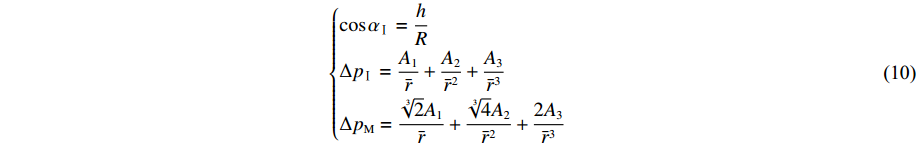

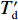
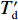
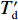
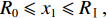
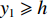
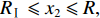

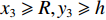
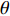
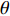
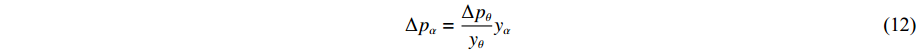
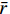
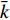
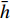
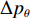
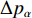
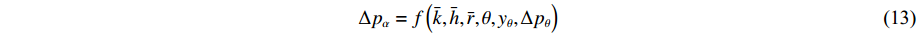



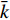
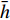
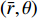

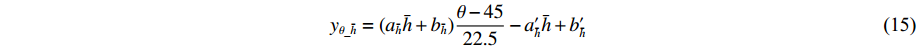
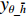
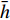
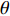

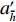
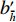
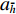
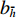
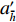
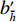
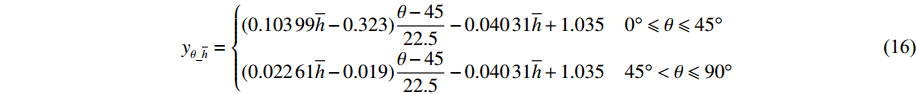
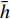

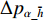
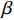
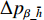
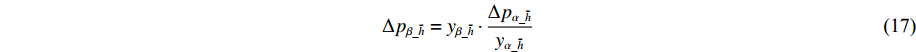
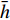
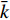
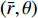

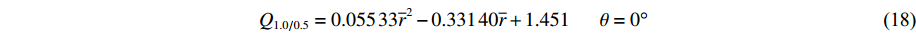
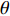
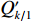
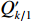
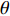
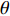
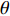
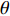
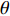
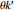
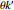

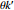
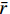

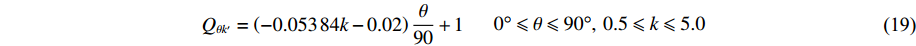
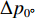
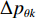
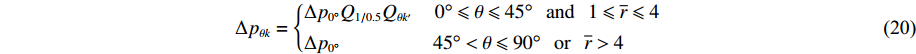
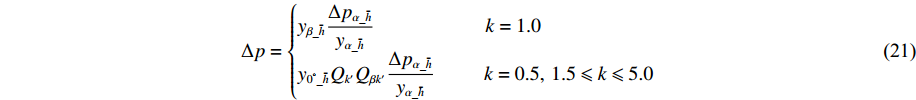
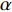
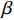


 下载:
下载: