冲击载荷下金属材料的微结构-加载特性-层裂响应关系概述

蔡洋; 李超; 卢磊

doi:10.11858/gywlxb.20200648

冲击载荷下金属材料的微结构-加载特性-层裂响应关系概述

doi: 10.11858/gywlxb.20200648

蔡洋^1,,
李超^2,*, ,,
卢磊²

1.
顶峰多尺度科学研究所，四川成都　610027
2.
西南交通大学材料科学与工程学院，四川成都　610031

基金项目: 科学挑战计划（TZ2018001）

详细信息

作者简介:
蔡　洋（1990－），男，博士，副研究员，主要从事材料动态力学响应研究. E-mail：caiy@pims.ac.cn

通讯作者:
李　超（1990－），男，博士，助理教授，主要从事金属材料变形损伤研究. E-mail：cli@swjtu.edu.cn

中图分类号: O347.1
计量
- 文章访问数: 4576
- HTML全文浏览量: 891
- PDF下载量: 121
出版历程
- 收稿日期: 2020-12-01
- 修回日期: 2021-02-01

Effects of Microstructure and Loading Characteristics on Spallation of Metallic Materials under Shock Loading

CAI Yang^1
,,
LI Chao^{2,*
, ,},
LU Lei²

1.
The Peac Institute of Multiscale Sciences, Chengdu 610027, Sichuan, China
2.
School of Materials Science and Engineering, Southwest Jiaotong University, Chengdu 610031, Sichuan, China

摘要

摘要: 鉴于冲击载荷下金属材料的变形损伤具有微结构和加载特征的依赖性，对国内外有关层裂现象的研究进展进行了简要梳理与总结。概述了晶粒尺寸、织构、晶界类型、相界和元素偏析带等结构对金属材料变形损伤的影响，重点阐述了脉冲宽度、应变率和峰值应力等加载特征对金属材料层裂强度的耦合作用，为了解冲击载荷下金属材料微结构、加载特征与变形损伤行为之间的关系提供参考。
- 损伤 /
- 层裂强度 /
- 微结构 /
- 加载特征
Abstract: Deformation and damage of metallic materials under shock loading depend on microstructure and loading characteristics. The effects of microstructures such as grain size, texture, grain boundary, phase boundary and element segregation bands on deformation and damage of metallic materials are reviewed, as well as the coupled effects of pulse duration, peak stress and strain rate on spall strength. This review provides a reference for establishing the relationship among microstructure, loading characteristics and deformation and damage behavior of metallic materials under shock loading.
- damage /
- spall strength /
- microstructure /
- loading characteristics

HTML全文

目前，国内武装贩毒、劫持人质、抢劫银行等严重武装犯罪以及恐怖活动均不断出现。为应对这些危险的武装犯罪事件，需要一些停止作用大、侵彻力低的武器装备，以求能形成较高的制止犯罪能力。而我国目前主要的警用武器装备均存在枪弹的穿透力太大，导致停止作用不足的缺点。国外警方在遇到这种紧急情况时都会使用特制的低侵彻手枪弹头^[1]。Kneubuehl等^[2]在试验中发现，将美国M193步枪弹的撞击速度提升到750 m/s以上时，弹体在软介质中会破碎，从而达到低侵彻的效果。美国温彻斯特公司生产的“黑爪”弹，其弹体为纯黑色，射入目标后被甲会均匀地向后翻开成6瓣，就像6个带有倒钩的爪子，弹头直径最终可膨胀至口径的两倍左右。近几年国内学者也注意到了低侵彻枪弹的研究意义。李晓杰等^[3]对国产5.8 mm口径手枪弹头进行改进，提出了新式的软尖弹和空尖弹，并与标准弹对比，使用ANSYS/LS-DYNA进行弹丸入水的数值仿真，得到3种弹型的速度、位移曲线，以及子弹入水产生的瞬时空腔，结果表明，空尖弹具有良好的低侵彻性能。金永喜等^[4]提出了子弹在软目标介质内的翻滚模型，证明弹丸翻滚时产生的离心力能使子弹在撞击目标过程中头部发生破碎，从而使其侵彻深度得到明显的降低，并通过与制式枪弹的实验对比进行了论证。尽管近几年我国开始对低侵彻枪弹进行相关研究，但都仅局限在仿真或者理论的基础上，相关的实验验证少之又少，因此，本工作通过实验与仿真相结合，对低侵彻枪弹做进一步的研究。

根据创伤弹道学^[5]，子弹在撞击目标介质过程中，弹头的翻转、变形、破碎都能使弹头的能量更快地向介质传递。本工作研究一种空心开花型低侵彻弹。该弹击中目标后弹体头部会向外开裂，利用子弹头部在侵彻过程中产生的大变形，实现子弹的高能量传递性，使开花弹在低速侵彻下初速低、子弹头部变形小、侵彻阻力低，而在高速侵彻下初速高、子弹头部变形大、侵彻阻力高，最终达到开花弹在低速和高速侵彻条件下，侵彻深度始终控制在某一范围之内的目标。因此，该空心开花型低侵彻弹无论近距离还是远距离击中目标，都不会将其射穿，具备良好的低侵彻性能。在应对紧急情况时，只会制止犯罪人员而不会穿透目标误伤群众。

1. 弹丸入水实验

1.1 实验设计

鉴于生物组织的复杂性，采用水介质模拟生物组织。因为大多数生物组织含水80%左右，其密度与水相近，而且水具有均匀、透明、便于直接观察的特点。以5.8 mm口径弹道枪作为发射平台进行弹体侵彻水介质实验，实验现场布置如图 1所示，通过装药量控制弹体着靶速度，用锡箔靶和双通道测试仪测量速度。

图 1 实验现场

Figure 1. Experiment set-up

下载: 全尺寸图片幻灯片

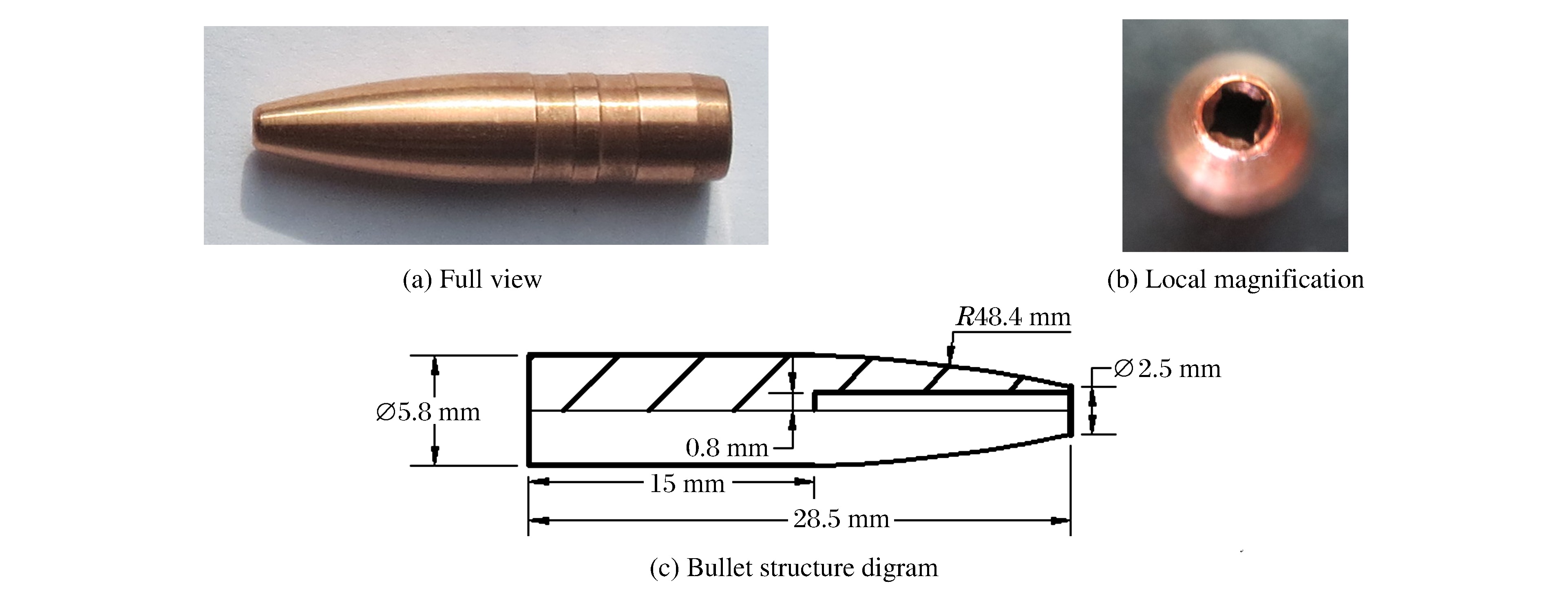
实验中空心开花型低侵彻弹的直径为5.8 mm，长径比为5，质量为5.6 g，弹丸头部开有矩形槽，边长为2 mm，凹槽边界上沿轴向设有预开槽，如图 2所示。

图 2 实验用开花弹

Figure 2. Shrapnel of experiment

下载: 全尺寸图片幻灯片

1.2 实验结果及分析

通过改变子弹的装药量，得到开花弹在撞击速度为408、501、610、702、814 m/s下弹丸头部的变形情况，实验结果如表 1所示。从表 1中可以看出，开花弹入射水箱后，弹体头部发生不同程度的变形。这是由于开花弹在侵彻靶体过程中会受到较大的侵彻阻力，产生沿弹体轴向的剪切力，弹丸头部发生变形，由于弹头开有矩形凹槽，在矩形槽的轴向边界处会产生相应的拉应力，拉应力使凹槽不断向外膨胀，达到弹体材料的抗拉强度后，弹头沿矩形槽边界断裂，开裂成4瓣，断裂的部分在水的阻力作用下向后翻转，最后呈“花瓣状”。

表 1 实验结果和仿真结果对比

Table 1. Comparison of experiment and simulation results

Experiment		Simulation
Velocity/(m·s^-1)	Results	Velocity/(m·s^-1)	Results
408		400
501		500
610		600
702		700
814		800

下载: 导出CSV

| 显示表格

对比表 1可知，弹丸头部的变形程度与子弹撞击速度有关。在408 m/s较低速度下入水时，弹丸头部部分开裂，矩形槽沿径向略微膨胀。子弹以501~610 m/s速度入水时，弹丸头部开裂成4瓣并伴有外翻现象，但头部凹槽未开裂完全，仍有清晰的孔洞，此时弹丸头部最大横截面处直径为弹体直径的2倍。子弹以702~814 m/s速度入水时，弹头沿矩形槽轴向边界完全开裂成“花瓣状”，且凹槽完全消失，此时弹丸头部最大横截面处直径达到弹体直径的2.4倍。因此，开花弹在低速入射时，初速低，侵彻阻力较小，弹头变形小；在高速入射时，初速高，侵彻阻力大，弹头变形大。

2. 数值模拟的有限元建模及算法

弹头入水的模型如图 3所示。弹头在空气中，空气和水的边界采用无反射边界，空气和水的接触面采用共节点方式。

图 3 弹头入水的有限元模型

Figure 3. Finite element model of projectilepenetrating into water

下载: 全尺寸图片幻灯片

弹头由紫铜构成，保证了弹体材料的一致性。在算法的选择上：铜选用Lagrange算法，空气和水选用欧拉算法，弹头和水之间采用耦合算法^[6]。在材料模型的选择上：空气和水采用LS-DYNA提供的MAT_NULL流体模型；紫铜选用Johnson-Cook材料模型，这种本构方程考虑了高速下的应变率效应和温度效应，适用于高速下的流固耦合。在状态方程的选择上：紫铜、空气和水都采用Grüneisen状态方程^[5-7]。

3. 仿真结果比较

3.1 不同速度入水的头部变形比较

使用LS-DYNA软件可以较理想地模拟开花弹的入水过程，图 4为开花弹撞击水介质时弹头的变形过程。从图 4中可以看出，开花弹撞击水介质后，弹头在侵彻阻力作用下发生变形，并沿着矩形槽发生开裂，最后以花瓣状弹头继续运动。

图 4 弹头入水的变形过程

Figure 4. Deformation process of projectilepenetrating into water

下载: 全尺寸图片幻灯片

通过模拟开花弹的入水过程，得到开花弹以不同速度撞击水介质后稳定侵彻阶段的弹体变形图，并与实验结果进行对比，如表 1所示。可以看出，仿真结果与实验中弹体变形情况基本吻合。开花弹在较低速撞击下，弹头轻微变形，矩形槽口向外扩张，但未达到开裂的程度，这是因为此时开花弹受到的侵彻阻力过小，弹头矩形槽轴向边界产生的拉应力未达到弹体材料的抗拉强度，无法使弹头开裂。开花弹以600 m/s速度撞击水靶时，弹头受到的拉应力达到一定强度，使头部沿槽口开裂，但从表 1中可以看出，此时弹丸头部未完全开裂，这是由于子弹的撞击速度仍然不足，达不到子弹需要的侵彻阻力。进一步提高开花弹的侵彻速度，在700 m/s速度撞击下，侵彻阻力较大，弹头完全开裂成4瓣，矩形槽消失。

表 2是开花弹在不同入射速度下弹丸头部最大直径的实验数据和仿真数据的对比，其中：D_max为弹丸头部最大直径，D_max是其平均值。可以看出，实验结果和仿真结果基本吻合。开花弹速度低于500 m/s时，弹丸头部变形较小；速度为500 m/s时，弹丸头部最大直径为弹体直径的1.2倍；子弹速度为600 m/s时，弹头变形较大，弹丸头部最大直径为弹体直径的2倍；子弹速度达到700 m/s时，开花弹弹头开裂完全，变形达到极限，弹丸头部最大直径为弹体直径的2.4倍。弹头变形程度与实验结果一致，说明数值仿真可以较理想地模拟开花弹的入水过程。

表 2 不同速度入水的弹丸头部变形比较

Table 2. Comparison of deformation of projectiles entering water at different velocities

Experiment			Simulation
Velocity/(m·s^-1)	D_max/mm	D_max/mm	Velocity/(m·s^-1)	D_max/mm
408	5.10，5.08	5.090	400	5.18
501	6.71，6.79	6.750	500	6.82
610	11.30，11.23	11.265	600	11.48
702	13.82，13.80	13.810	700	13.83
814	13.81，13.81	13.810	800	13.82

下载: 导出CSV

| 显示表格

3.2 不同速度入水的速度衰减比较

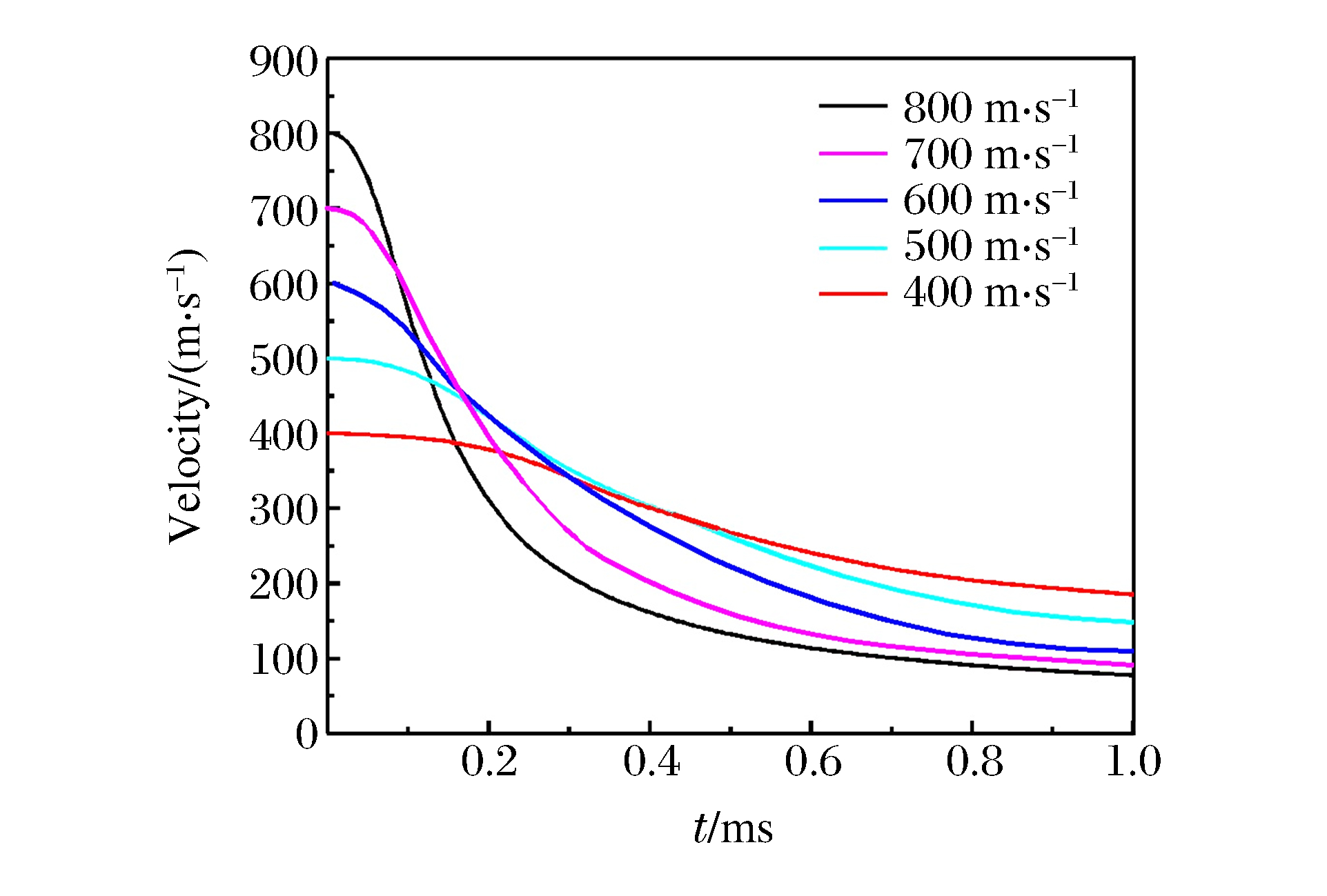
图 5为开花弹在不同速度下撞击水介质的速度衰减曲线，开花弹从空气中射入水后，速度开始降低。从图 5中可以看出，子弹的入射速度越高，速度衰减越明显。在400和500 m/s速度下，子弹速度衰减得比较缓慢，说明低速条件下开花弹头部未发生开裂，变形较小，子弹受到的侵彻阻力低，1 ms后子弹速度分别为182.13和143.58 m/s；600 m/s速度下，子弹速度衰减的速率有所提高，此时开花弹头部已开裂，但仍未变形完全，1 ms后子弹速度为106.36 m/s；700和800 m/s侵彻速度下，开花弹速度衰减十分明显，说明弹体头部完全开裂成“花瓣状”，与水的接触面增大，侵彻阻力高，1 ms后子弹速度分别降低到87.74和75.78 m/s。可以看出，开花弹的入射速度越高，1 ms后子弹的速度反而越低。这是因为开花弹低速入射时，弹头开裂程度小，侵彻过程中弹体最大横截面积小，与水的接触面小，受到的侵彻阻力低，因此速度衰减得较缓慢；随着入射速度的提高，弹头变形增大，与水的接触面增大，开花弹受到的侵彻阻力较大，导致速度衰减得更快。说明开花弹弹头开裂变形对提高子弹的侵彻阻力、降低子弹的速度起很大作用。

图 5 速度衰减曲线

Figure 5. Velocity attenuation curve

下载: 全尺寸图片幻灯片

3.3 不同速度入水的位移比较

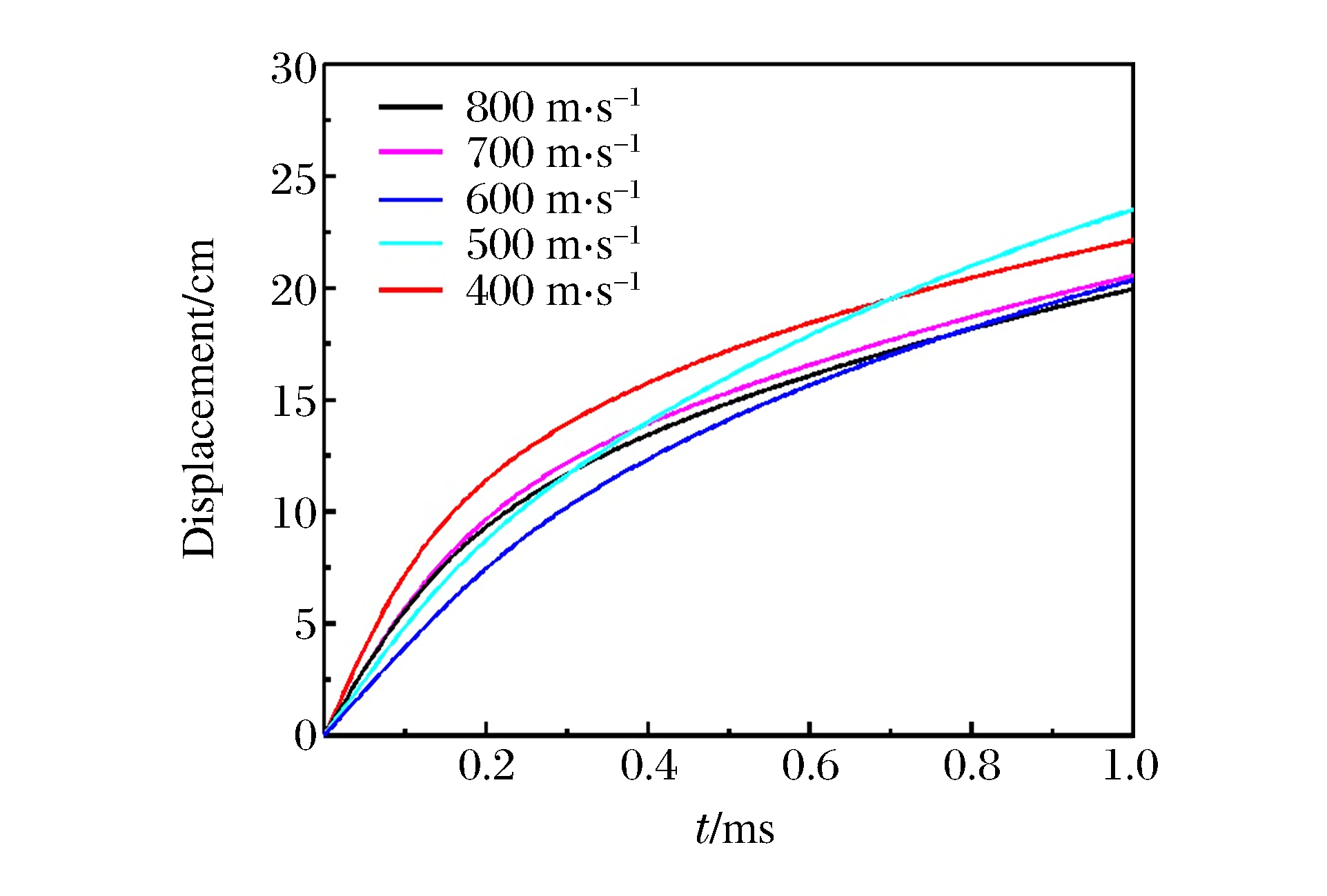
图 6为开花弹在不同速度下撞击水介质的位移曲线。从图 6中可以看出：开花弹以400 m/s入射时，1 ms内在水中的位移是22.13 cm；以500 m/s入射时位移有所增加，达到23.55 cm。这是因为子弹的初速增大，动能变大，而开花弹以500 m/s速度撞击水介质时弹体头部变形很小，矩形槽口发生略微扩张，达不到增大与水的接触面，进而增大侵彻阻力的目的，所以在水中的侵彻深度有所增加。开花弹以600、700和800 m/s速度撞击水介质时，1 ms内在水中的位移分别为20.36、20.55和19.95 cm，高速撞击下子弹在水中的位移明显低于低速撞击下的位移，这是因为开花弹在较高速度下撞击水介质时，弹头发生开裂变形，呈花瓣状，弹体的最大横截面积提高1~2倍，增大了子弹撞击过程中与水的接触面积，极大地提高了子弹在水中受到的侵彻阻力，降低子弹的运动速度，使子弹在高速入射条件下的位移比低速入射时更低。不同速度入射下1 ms后子弹的位移都在20~24 cm范围内，由此可以说明开花弹具有良好的低侵彻特性。

图 6 位移衰减曲线

Figure 6. Displacement attenuation curve

下载: 全尺寸图片幻灯片

4. 结论

对开花型低侵彻弹进行了不同速度侵彻水介质的实验研究，结果表明：开花弹以500 m/s以下低速撞击时，弹丸头部变形较小，弹形保持良好，稳定侵彻阶段弹头最大直径为弹体直径的1.0~1.2倍；以501~610 m/s中速撞击时，弹头发生开裂，但未变形完全，弹头最大直径达到弹体直径的2倍，受到的侵彻阻力较大；以702~814 m/s高速撞击时，弹头完全开裂，呈花瓣状，弹头最大直径达到弹体直径的2.4倍，受到的侵彻阻力最大。利用LS-DYNA软件模拟开花弹在不同速度下的入水过程，仿真结果与实验结果基本一致，说明数值仿真可以较理想地模拟开花弹的入水过程；得到了子弹的速度衰减曲线和位移曲线，表明开花弹弹头开裂变形对提高子弹的侵彻阻力、降低子弹速度起很大作用，证明开花弹具有良好的低侵彻特性。

图 1 轻气炮加载装置示意图^[8]

Figure 1. Schematic setup for gas-gun experiment^[8]

下载: 全尺寸图片幻灯片

图 2 层裂原理示意图^[8]

Figure 2. Schematic illustration of spallation^[8]

下载: 全尺寸图片幻灯片

图 3 层裂强度及空隙密度与晶粒尺寸的关系^[27]

Figure 3. Contour map demonstrating the grain size dependence of spall strength as a function of void density^[27]

下载: 全尺寸图片幻灯片

图 4 金属钽层裂损伤的微结构表征^[14]

Figure 4. Microstructure characterization of spallation damage for tantalum^[14]

下载: 全尺寸图片幻灯片

图 5 不锈钢的自由面速度历史曲线^[43]

Figure 5. Representative free surface velocity histories for stainless steel^[43]

下载: 全尺寸图片幻灯片

图 6 镁合金层裂损伤的X射线计算机断层图像^[41]

Figure 6. X-ray computed tomography images of spallation damage for the magnesium alloy^[41]

下载: 全尺寸图片幻灯片

图 7 304不锈钢中的元素偏析带以及层裂损伤特征^[40]

Figure 7. Element segregation bands and damage features of 304 stainless steel^[40]

下载: 全尺寸图片幻灯片

图 8 304不锈钢层裂强度与冲击压力的关系^[40]

Figure 8. Spall strength of 304 stainless steel as a function of peak stress^[40]

下载: 全尺寸图片幻灯片

图 9 冲击加载下样品不同截面处的应力历史^[66]

Figure 9. Stress histories in different cross-sections of a sample under shock loading^[66]

下载: 全尺寸图片幻灯片

图 10 低碳钢的自由面速度历史^[28]

Figure 10. Free surface velocity histories of mild carbon steel^[28]

下载: 全尺寸图片幻灯片

图 11 金属材料的层裂强度与应变率的关系^[28]

Figure 11. Spall strength of metallic materials as a function of strain rate^[28]

下载: 全尺寸图片幻灯片

图 12 低碳钢层裂强度与峰值应力的关系^[28]

Figure 12. Spall strength of mild carbon steel as a function of peak compression stress^[28]

下载: 全尺寸图片幻灯片

图 13 拉应力历史对层裂强度影响的示意图^[28]

Figure 13. Schematic illustration of the effect of tensile stress history on spall strength^[28]

下载: 全尺寸图片幻灯片

图 14 低碳钢层裂损伤的微结构表征及层裂强度的减少量 $\Delta\sigma\rm_{tw}$ 与孪晶密度的关系^[28]

Figure 14. Microstructure characterization of spallation damage for mild carbon steel, and reduction in spall strength $\Delta\sigma\rm_{tw}$ as a function of deformation twin density^[28]

下载: 全尺寸图片幻灯片

参考文献(99)

[1]	WHELCHEL R L, JR SANDERS T H, THADHANI N N. Spall and dynamic yield behavior of an annealed aluminum-magnesium alloy [J]. Scripta Materialia, 2014, 92: 59–62. doi: 10.1016/j.scriptamat.2014.08.014
[2]	ANTOUN T, CURRAN D R, SEAMAN L, et al. Spall fracture [M]. New York: Springer, 2003.
[3]	TRIVEDI P, ASAY J, GUPTA Y, et al. Influence of grain size on the tensile response of aluminum under plate-impact loading [J]. Journal of Applied Physics, 2007, 102(8): 083513. doi: 10.1063/1.2798497
[4]	LUO S N, AN Q, GERMANN T C, et al. Shock-induced spall in solid and liquid Cu at extreme strain rates [J]. Journal of Applied Physics, 2009, 106(1): 013502. doi: 10.1063/1.3158062
[5]	CHEN X, ASAY J, DWIVEDI S, et al. Spall behavior of aluminum with varying microstructures [J]. Journal of Applied Physics, 2006, 99(2): 023528. doi: 10.1063/1.2165409
[6]	ARMAN B, LUO S N, GERMANN T C, et al. Dynamic response of Cu₄₆Zr₅₄ metallic glass to high-strain-rate shock loading: plasticity, spall, and atomic-level structures [J]. Physical Review B, 2010, 81(14): 144201. doi: 10.1103/PhysRevB.81.144201
[7]	JOHNSON J N, GRAY Ⅲ G T, BOURNE N K. Effect of pulse duration and strain rate on incipient spall fracture in copper [J]. Journal of Applied Physics, 1999, 86(9): 4892–4901. doi: 10.1063/1.371527
[8]	BUTCHER B, BARKER L, MUNSON D, et al. Influence of stress history on time-dependent spall in metals [J]. AIAA Journal, 1964, 2(6): 977–990. doi: 10.2514/3.2484
[9]	桂毓林, 刘仓理, 王彦平, 等. AF1410钢的层裂断裂特性研究 [J]. 高压物理学报, 2006, 20(1): 34–38. doi: 10.11858/gywlxb.2006.01.008 GUI Y L, LIU C L, WANG Y P, et al. Spall fracture properties of AF1410 steel [J]. Chinese Journal of High Pressure Physics, 2006, 20(1): 34–38. doi: 10.11858/gywlxb.2006.01.008
[10]	DAVISON L, STEVENS A, KIPP M. Theory of spall damage accumulation in ductile metals [J]. Journal of the Mechanics and Physics of Solids, 1977, 25(1): 11–28. doi: 10.1016/0022-5096(77)90017-5
[11]	GRADY D. The spall strength of condensed matter [J]. Journal of the Mechanics and Physics of Solids, 1988, 36(3): 353–384. doi: 10.1016/0022-5096(88)90015-4
[12]	HANSEN N. Hall-Petch relation and boundary strengthening [J]. Scripta Materialia, 2004, 51(8): 801–806. doi: 10.1016/j.scriptamat.2004.06.002
[13]	JR CALLISTER W D. Fundamentals of materials science and engineering [M]. London: Wiley London, 2000.
[14]	CHENG M, LI C, TANG M, et al. Intragranular void formation in shock-spalled tantalum: mechanisms and governing factors [J]. Acta Materialia, 2018, 148: 38–48. doi: 10.1016/j.actamat.2018.01.029
[15]	REMINGTON T, HAHN E, ZHAO S, et al. Spall strength dependence on grain size and strain rate in tantalum [J]. Acta Materialia, 2018, 158: 313–329. doi: 10.1016/j.actamat.2018.07.048
[16]	CHEN T, JIANG Z, PENG H, et al. Effect of grain size on the spall fracture behaviour of pure copper under plate-impact loading [J]. Strain, 2015, 51(3): 190–197. doi: 10.1111/str.12132
[17]	MINICH R W, CAZAMIAS J U, KUMAR M, et al. Effect of microstructural length scales on spall behavior of copper [J]. Metallurgical and Materials Transactions A, 2004, 35(9): 2663–2673. doi: 10.1007/s11661-004-0212-7
[18]	ESCOBEDO J, CERRETA E, DENNIS-KOLLER D. Effect of crystalline structure on intergranular failure during shock loading [J]. JOM, 2014, 66(1): 156–164. doi: 10.1007/s11837-013-0798-6
[19]	KANEL G I. Unusual behaviour of usual materials in shock waves [C]//Proceedings of the Journal of Physics Conference Series, 2014, 500: 012001.
[20]	GARKUSHIN G, IGNATOVA O, KANEL G, et al. Submicrosecond strength of ultrafine-grained materials [J]. Mechanics of Solids, 2010, 45(4): 624–632. doi: 10.3103/S0025654410040114
[21]	RAZORENOV S, GARKUSHIN G, IGNATOVA O. Resistance to dynamic deformation and fracture of tantalum with different grain and defect structures [J]. Physics of the Solid State, 2012, 54(4): 790–797. doi: 10.1134/S1063783412040233
[22]	BUCHAR J, ELICES M, CORTEZ R. The influence of grain size on the spall fracture of copper [J]. Journal de Physique Ⅳ, 1991, 1(C3): 623–630.
[23]	SCHWARTZ A J, CAZAMIAS J U, FISKE P S, et al. Grain size and pressure effects on spall strength in copper [C]//AIP Conference Proceedings. New York: American Institute of Physics, 2002.
[24]	MACKENCHERY K, VALISETTY R R, NAMBURU R R, et al. Dislocation evolution and peak spall strengths in single crystal and nanocrystalline Cu [J]. Journal of Applied Physics, 2016, 119(4): 044301. doi: 10.1063/1.4939867
[25]	DONGARE A M, RAJENDRAN A M, LAMATTINA B, et al. Atomic scale studies of spall behavior in nanocrystalline Cu [J]. Journal of Applied Physics, 2010, 108(11): 113518. doi: 10.1063/1.3517827
[26]	YUAN F, WU X. Shock response of nanotwinned copper from large-scale molecular dynamics simulations [J]. Physical Review B, 2012, 86(13): 134108. doi: 10.1103/PhysRevB.86.134108
[27]	WILKERSON J, RAMESH K. Unraveling the anomalous grain size dependence of cavitation [J]. Physical Review Letters, 2016, 117(21): 215503. doi: 10.1103/PhysRevLett.117.215503
[28]	LI C, YANG K, TANG X, et al. Spall strength of a mild carbon steel: effects of tensile stress history and shock-induced microstructure [J]. Materials Science and Engineering: A, 2019, 754: 461–469. doi: 10.1016/j.msea.2019.03.019
[29]	CAI Y, WU H A, LUO S N. A loading-dependent model of critical resolved shear stress [J]. International Journal of Plasticity, 2018, 109: 1–17. doi: 10.1016/j.ijplas.2018.03.011
[30]	TURLEY W, FENSIN S J, HIXSON R, et al. Spall response of single-crystal copper [J]. Journal of Applied Physics, 2018, 123(5): 055102. doi: 10.1063/1.5012267
[31]	PEREZ-BERGQUIST A, CERRETA E K, TRUJILLO C P, et al. Orientation dependence of void formation and substructure deformation in a spalled copper bicrystal [J]. Scripta Materialia, 2011, 65(12): 1069–1072. doi: 10.1016/j.scriptamat.2011.09.015
[32]	OWEN G, CHAPMAN D, WHITEMAN G, et al. Spall behaviour of single crystal aluminium at three principal orientations [J]. Journal of Applied Physics, 2017, 122(15): 155102. doi: 10.1063/1.4999559
[33]	LIN E, SHI H, NIU L. Effects of orientation and vacancy defects on the shock Hugoniot behavior and spallation of single-crystal copper [J]. Modelling and Simulation in Materials Science and Engineering, 2014, 22(3): 035012. doi: 10.1088/0965-0393/22/3/035012
[34]	AN Q, RAVELO R, GERMANN T C, et al. Shock compression and spallation of single crystal tantalum [C]//AIP Conference Proceedings. New York: American Institute of Physics, 2012.
[35]	HAHN E N, FENSIN S J, GERMANN T C, et al. Orientation dependent spall strength of tantalum single crystals [J]. Acta Materialia, 2018, 159: 241–248. doi: 10.1016/j.actamat.2018.07.073
[36]	KANEL G, RAZORENOV S, UTKIN A, et al. Spall strength of molybdenum single crystals [J]. Journal of Applied Physics, 1993, 74(12): 7162–7165. doi: 10.1063/1.355032
[37]	VIGNJEVIC R, BOURNE N, MILLETT J, et al. Effects of orientation on the strength of the aluminum alloy 7010-T6 during shock loading: experiment and simulation [J]. Journal of Applied Physics, 2002, 92(8): 4342–4348. doi: 10.1063/1.1505996
[38]	GRAY G, BOURNE N, VECCHIO K, et al. Influence of anisotropy (crystallographic and microstructural) on spallation in Zr, Ta, HY-100 steel, and 1080 eutectoid steel [J]. International Journal of Fracture, 2010, 163(1/2): 243–258. doi: 10.1007/s10704-009-9440-6
[39]	TAN J, LU L, LI H, et al. Anisotropic deformation and damage of dual-phase Ti-6Al-4V under high strain rate loading [J]. Materials Science and Engineering: A, 2019, 742: 532–539. doi: 10.1016/j.msea.2018.10.088
[40]	MA L, LIU J, LI C, et al. Effects of alloying element segregation bands on impact response of a 304 stainless steel [J]. Materials Characterization, 2019, 153: 294–303. doi: 10.1016/j.matchar.2019.05.015
[41]	DAI Z H, LU L, CHAI H W, et al. Mechanical properties and fracture behavior of Mg-3Al-1Zn alloy under high strain rate loading [J]. Materials Science and Engineering: A, 2020, 789: 139690.
[42]	YU X, LI T, LI L, et al. Influence of initial texture on the shock property and spall behavior of magnesium alloy AZ31B [J]. Materials Science and Engineering: A, 2017, 700: 259–268. doi: 10.1016/j.msea.2017.06.015
[43]	LI C, HUANG J, TANG X, et al. Effects of structural anisotropy on deformation and damage of a duplex stainless steel under high strain rate loading [J]. Materials Science and Engineering: A, 2017, 705: 265–272. doi: 10.1016/j.msea.2017.08.091
[44]	GALITSKIY S, IVANOV D S, DONGARE A M. Dynamic evolution of microstructure during laser shock loading and spall failure of single crystal Al at the atomic scales [J]. Journal of Applied Physics, 2018, 124(20): 205901. doi: 10.1063/1.5051618
[45]	KOLLER D D, HIXSON R S, GRAY Ⅲ G T, et al. Influence of shock-wave profile shape on dynamically induced damage in high-purity copper [J]. Journal of Applied Physics, 2005, 98(10): 103518. doi: 10.1063/1.2128493
[46]	YU Y, LI C, MA H H, et al. Deformation and spallation of explosive welded steels under gas gun shock loading [J]. Chinese Physics Letters, 2018, 35(1): 104–108.
[47]	LI C, LI B, HUANG J Y, et al. Spall damage of a mild carbon steel: effects of peak stress, strain rate and pulse duration [J]. Materials Science and Engineering: A, 2016, 660: 139–147. doi: 10.1016/j.msea.2016.02.080
[48]	MEYERS M A. Dynamic behavior of materials [M]. John Wiley & Sons, 1994.
[49]	ESCOBEDO J P, CERRETA E K, DENNIS-KOLLER D, et al. Influence of boundary structure and near neighbor crystallographic orientation on the dynamic damage evolution during shock loading [J]. Philosophical Magazine, 2013, 93(7): 833–846. doi: 10.1080/14786435.2012.734638
[50]	WAYNE L, KRISHNAN K, DIGIACOMO S, et al. Statistics of weak grain boundaries for spall damage in polycrystalline copper [J]. Scripta Materialia, 2010, 63(11): 1065–1068. doi: 10.1016/j.scriptamat.2010.08.003
[51]	BROWN A D, WAYNE L, PHAM Q, et al. Microstructural effects on damage nucleation in shock-loaded polycrystalline copper [J]. Metallurgical and Materials Transactions A, 2015, 46(10): 4539–4547. doi: 10.1007/s11661-014-2482-z
[52]	FENSIN S, VALONE S, CERRETA E, et al. Influence of grain boundary properties on spall strength: grain boundary energy and excess volume [J]. Journal of Applied Physics, 2012, 112(8): 083529. doi: 10.1063/1.4761816
[53]	FENSIN S, VALONE S, CERRETA E, et al. Effect of grain boundary structure on plastic deformation during shock compression using molecular dynamics [J]. Modelling and Simulation in Materials Science and Engineering, 2012, 21(1): 015011.
[54]	ZHANG W, KENNEDY G B, MULY K, et al. Effect of aging state on shock induced spall behavior of ultrahigh strength Al-Zn-Mg-Cu alloy [J]. International Journal of Impact Engineering, 2020, 146: 103725. doi: 10.1016/j.ijimpeng.2020.103725
[55]	ZHOU T T, HE A M, WANG P, et al. Spall damage in single crystal Al with helium bubbles under decaying shock loading via molecular dynamics study [J]. Computational Materials Science, 2019, 162: 255–267. doi: 10.1016/j.commatsci.2019.02.019
[56]	RAZORENOV S, KANEL G, HERRMANN B, et al. Influence of nano-size inclusions on spall fracture of copper single crystals [C]//AIP Conference Proceedings. New York: American Institute of Physics, 2007.
[57]	FENSIN S J, WALKER E K, CERRETA E K, et al. Dynamic failure in two-phase materials [J]. Journal of Applied Physics, 2015, 118(23): 235305. doi: 10.1063/1.4938109
[58]	TANG X, LI C, LI H, et al. Cup-cone structure in spallation of bulk metallic glasses [J]. Acta Materialia, 2019, 178: 219–227. doi: 10.1016/j.actamat.2019.08.006
[59]	TANG X, JIAN W, HUANG J, et al. Spall damage of a Ta particle-reinforced metallic glass matrix composite under high strain rate loading [J]. Materials Science and Engineering: A, 2018, 711: 284–292. doi: 10.1016/j.msea.2017.11.032
[60]	CHEN J, TSCHOPP M A, DONGARE A M. Shock wave propagation and spall failure of nanocrystalline Cu/Ta alloys: effect of Ta in solid-solution [J]. Journal of Applied Physics, 2017, 122(22): 225901. doi: 10.1063/1.5001761
[61]	CHEN J, MATHAUDHU S N, THADHANI N, et al. Unraveling the role of interfaces on the spall failure of Cu/Ta multilayered systems [J]. Scientific Reports, 2020, 10(1): 1–15. doi: 10.1038/s41598-019-56847-4
[62]	HAN W, CERRETA E, MARA N, et al. Deformation and failure of shocked bulk Cu-Nb nanolaminates [J]. Acta Materialia, 2014, 63: 150–161. doi: 10.1016/j.actamat.2013.10.019
[63]	YANG Y, JIANG Z, WANG C, et al. Effects of the phase interface on initial spallation damage nucleation and evolution in dual phase titanium alloy [J]. Materials Science and Engineering: A, 2018, 731: 385–393. doi: 10.1016/j.msea.2018.06.066
[64]	YANG Y, WANG H, WANG C, et al. Effects of the phase interface on spallation damage nucleation and evolution in dual-phase steel [J]. Steel Research International, 2020, 91(6): 1900583. doi: 10.1002/srin.201900583
[65]	RIVERA-DIAZ-DEL-CASTILLO P, VAN DER ZWAAG S, SIETSMA J. A model for ferrite/pearlite band formation and prevention in steels [J]. Metallurgical and Materials Transactions A, 2004, 35(2): 425–433. doi: 10.1007/s11661-004-0353-8
[66]	KANEL G. Spall fracture: methodological aspects, mechanisms and governing factors [J]. International Journal of Fracture, 2010, 163(1): 173–91.
[67]	MEYERS M. Shock waves and high-strain-rate phenomena in metals: concepts and applications [M]. New York: Springer Science & Business Media, 2012.
[68]	LUO S N, GERMANN T C, AN Q, et al. Shock-induced spall in copper: the effects of anisotropy, temperature, defects and loading pulse [C]//AIP Conference Proceedings. New York: American Institute of Physics, 2009.
[69]	GRAY Ⅲ G T, BOURNE N K, HENRIE B L. On the influence of loading profile upon the tensile failure of stainless steel [J]. Journal of Applied Physics, 2007, 101(9): 093507. doi: 10.1063/1.2720099
[70]	CAI Y, WU H A, LUO S N. Spall strength of liquid copper and accuracy of the acoustic method [J]. Journal of Applied Physics, 2017, 121(10): 105901. doi: 10.1063/1.4978251
[71]	MOSHE E, ELIEZER S, DEKEL E, et al. An increase of the spall strength in aluminum, copper, and Metglas at strain rates larger than 10⁷ s⁻¹ [J]. Journal of Applied Physics, 1998, 83(8): 4004–4011. doi: 10.1063/1.367222
[72]	JONES D R, FENSIN S J, MARTINEZ D T, et al. Effect of peak stress and tensile strain-rate on spall in tantalum [J]. Journal of Applied Physics, 2018, 124(8): 085901. doi: 10.1063/1.5045045
[73]	TULER F R, BUTCHER B M. A criterion for the time dependence of dynamic fracture [J]. International Journal of Fracture Mechanics, 1968, 4(4): 431–437.
[74]	HAHN E N, GERMANN T C, RAVELO R, et al. On the ultimate tensile strength of tantalum [J]. Acta Materialia, 2017, 126: 313–328. doi: 10.1016/j.actamat.2016.12.033
[75]	WHITEMAN G, KEIGHTLEY P, MILLETT J. The behaviour of 2169 steel under uniaxial stress and uniaxial strain loading [J]. Journal of Dynamic Behavior of Materials, 2016, 2(3): 337–346. doi: 10.1007/s40870-016-0069-z
[76]	PAVLENKO A V, MALUGINA S, KAZAKOV D N, et al. Plastic deformation and spall fracture of structural 12Cr18Ni10Ti steel [C]//AIP Conference Proceedings. New York: American Institute of Physics, 2012.
[77]	STEVENS A, TULER F. Effect of shock precompression on the dynamic fracture strength of 1020 steel and 6061-T6 aluminum [J]. Journal of Applied Physics, 1971, 42(13): 5665–5670. doi: 10.1063/1.1659997
[78]	WANG G. Influence of shock pre-compression stress and tensile strain rate on the spall behaviour of mild steel [J]. Strain, 2011, 47(5): 398–404. doi: 10.1111/j.1475-1305.2009.00700.x
[79]	NGUYEN T, LUSCHER D, WILKERSON J. A physics-based model and simple scaling law to predict the pressure dependence of single crystal spall strength [J]. Journal of the Mechanics and Physics of Solids, 2020, 137: 103875. doi: 10.1016/j.jmps.2020.103875
[80]	WANG Y, HE H, WANG L. Critical damage evolution model for spall failure of ductile metals [J]. Mechanics of Materials, 2013, 56: 131–141. doi: 10.1016/j.mechmat.2012.10.004
[81]	ZENG Z H, LI X Z, LI C, et al. Deformation twinning in a mild steel: loading dependence and strengthening [J]. Materials Science and Engineering: A, 2019, 751: 332–339. doi: 10.1016/j.msea.2019.02.079
[82]	LI C, ZENG Z H, LI Y, et al. Shock-induced twinning and texture in a mild carbon steel [J]. Materials Science and Engineering: A, 2020, 773: 138832.
[83]	CURRAN D, SEAMAN L, SHOCKEY D. Dynamic failure of solids [J]. Physics Reports, 1987, 147(5/6): 253–388. doi: 10.1016/0370-1573(87)90049-4
[84]	SEAMAN L, CURRAN D R, SHOCKEY D A. Computational models for ductile and brittle fracture [J]. Journal of Applied Physics, 1976, 47(11): 4814–4826. doi: 10.1063/1.322523
[85]	TONG W, RAVICHANDRAN G. Inertial effects on void growth in porous viscoplastic materials [J]. ASME Journal of Applied Mechanics, 1995, 62(3): 633–639. doi: 10.1115/1.2895993
[86]	WU X, RAMESH K, WRIGHT T. The dynamic growth of a single void in a viscoplastic material under transient hydrostatic loading [J]. Journal of the Mechanics and Physics of Solids, 2003, 51(1): 1–26. doi: 10.1016/S0022-5096(02)00079-0
[87]	WILKERSON J, RAMESH K. A dynamic void growth model governed by dislocation kinetics [J]. Journal of the Mechanics and Physics of Solids, 2014, 70: 262–280. doi: 10.1016/j.jmps.2014.05.018
[88]	THOMASON P. Ductile spallation fracture and the mechanics of void growth and coalescence under shock-loading conditions [J]. Acta Materialia, 1999, 47(13): 3633–3646. doi: 10.1016/S1359-6454(99)00223-2
[89]	CHEN D, TAN H, YU Y, et al. A void coalescence-based spall model [J]. International Journal of Impact Engineering, 2006, 32(11): 1752–1767. doi: 10.1016/j.ijimpeng.2005.04.009
[90]	白以龙, 柯孚久, 夏蒙棼. 固体中微裂纹系统统计演化的基本描述 [J]. 力学学报, 1991, 23(3): 290–298. BAI Y L, KE F J, XIA M F. Formulation of statistical evolution of microcracks in solids [J]. Chinese Journal of Theoretical and Applied Mechani, 1991, 23(3): 290–298.
[91]	GURSON A. Plastic flow and fracture behavior of ductile materials incorporating void nucleation, growth and coalescence [D]. Providence, RI: Brown University, 1975.
[92]	TVERGAARD V. Material failure by void growth to coalescence [J]. Advances in Applied Mechanics, 1990, 27: 83–151.
[93]	BENZERGA A, LEBLOND J. Ductile fracture by void growth to coalescence [J]. Advances in Applied Mechanics, 2010, 44: 169–305.
[94]	TANG X C, YAO X H, WILKERSON J W. A micromechanics-based framework to predict transitions between dimple and cup-cone fracture modes in shocked metallic glasses [J]. International Journal of Plasticity, 2021, 137: 102884. doi: 10.1016/j.ijplas.2020.102884
[95]	JIANG M Q, DAI L H. On the origin of shear banding instability in metallic glasses [J]. Journal of the Mechanics and Physics of Solids, 2009, 57(8): 1267–1292. doi: 10.1016/j.jmps.2009.04.008
[96]	CORTES R. The growth of microvoids under intense dynamic loading [J]. International Journal of Solids and Structures, 1992, 29(11): 1339–1350. doi: 10.1016/0020-7683(92)90082-5
[97]	SEAMAN L, CURRAN D R. Inertia and temperature effects in void growth [C]//AIP Conference Proceedings. New York: American Institute of Physics, 2002, 620: 607–610.
[98]	封加波. 金属动态延性破坏的损伤度函数模型[D]. 北京: 北京理工大学, 1992.
[99]	王永刚. 延性金属动态拉伸断裂及其临界损伤度研究[D]. 绵阳: 中国工程物理研究院, 2006.

施引文献

资源附件(0)

访问统计

点击查看大图

图(14)

计量

文章访问数: 4576
HTML全文浏览量: 891
PDF下载量: 121

1. 弹丸入水实验
1.1 实验设计
1.2 实验结果及分析
2. 数值模拟的有限元建模及算法
3. 仿真结果比较
3.1 不同速度入水的头部变形比较
3.2 不同速度入水的速度衰减比较
3.3 不同速度入水的位移比较
4. 结论

冲击载荷下金属材料的微结构-加载特性-层裂响应关系概述

doi: 10.11858/gywlxb.20200648

作者简介: 蔡 洋（1990－），男，博士，副研究员，主要从事材料动态力学响应研究. E-mail：caiy@pims.ac.cn

通讯作者: 李 超（1990－），男，博士，助理教授，主要从事金属材料变形损伤研究. E-mail：cli@swjtu.edu.cn

计量

出版历程